Research on Multi-System Coupling Vibration of a Hot Tandem Mill
Abstract
1. Introduction
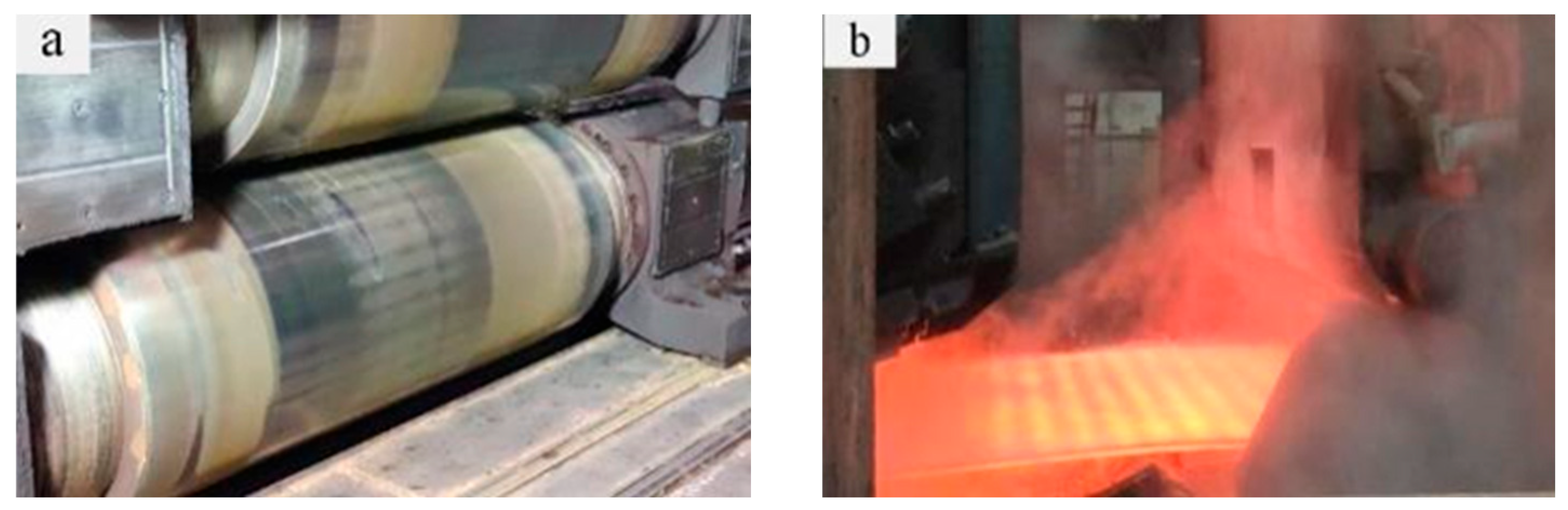
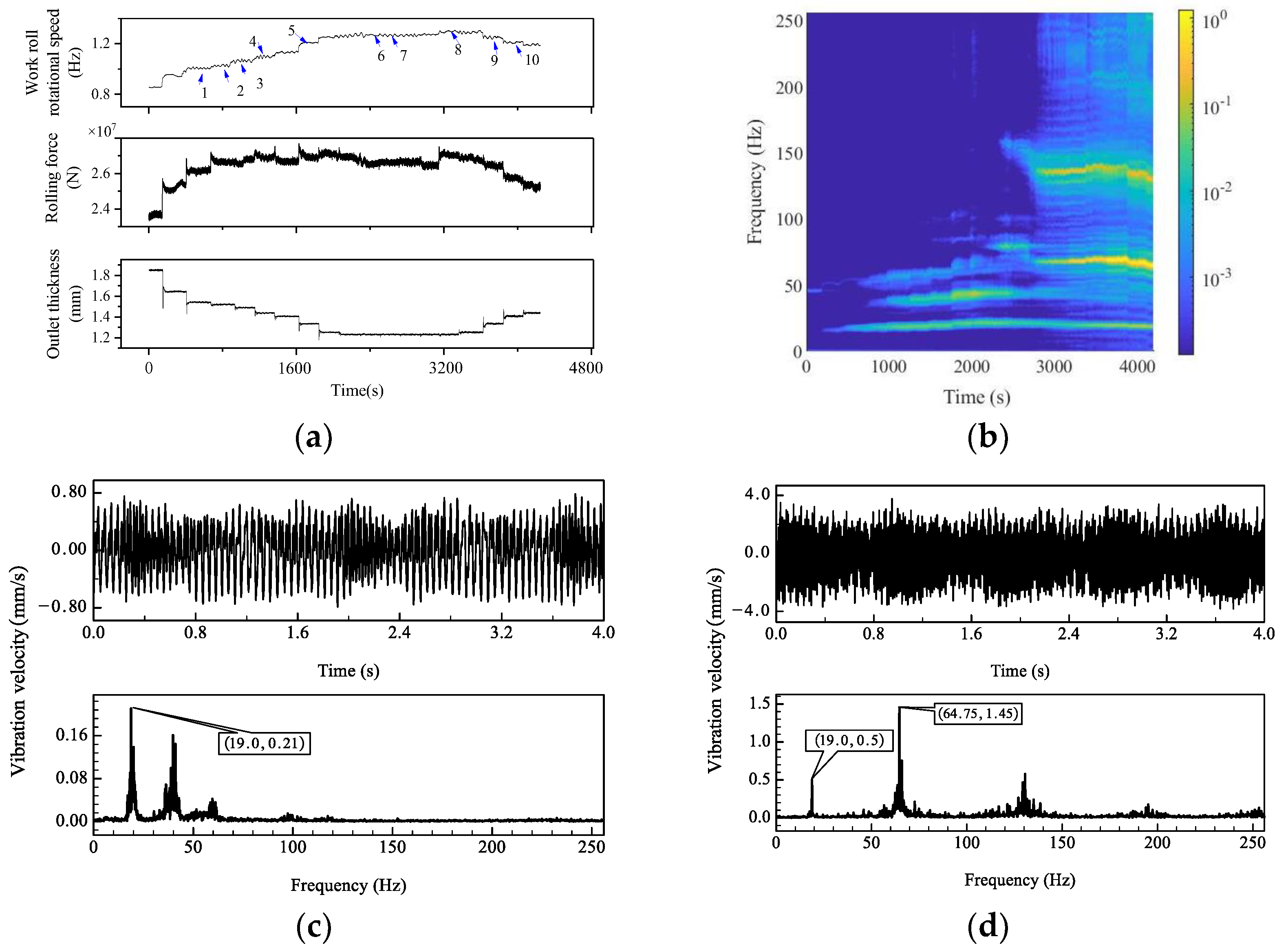
2. Experiments
3. Model Establishment and Verification
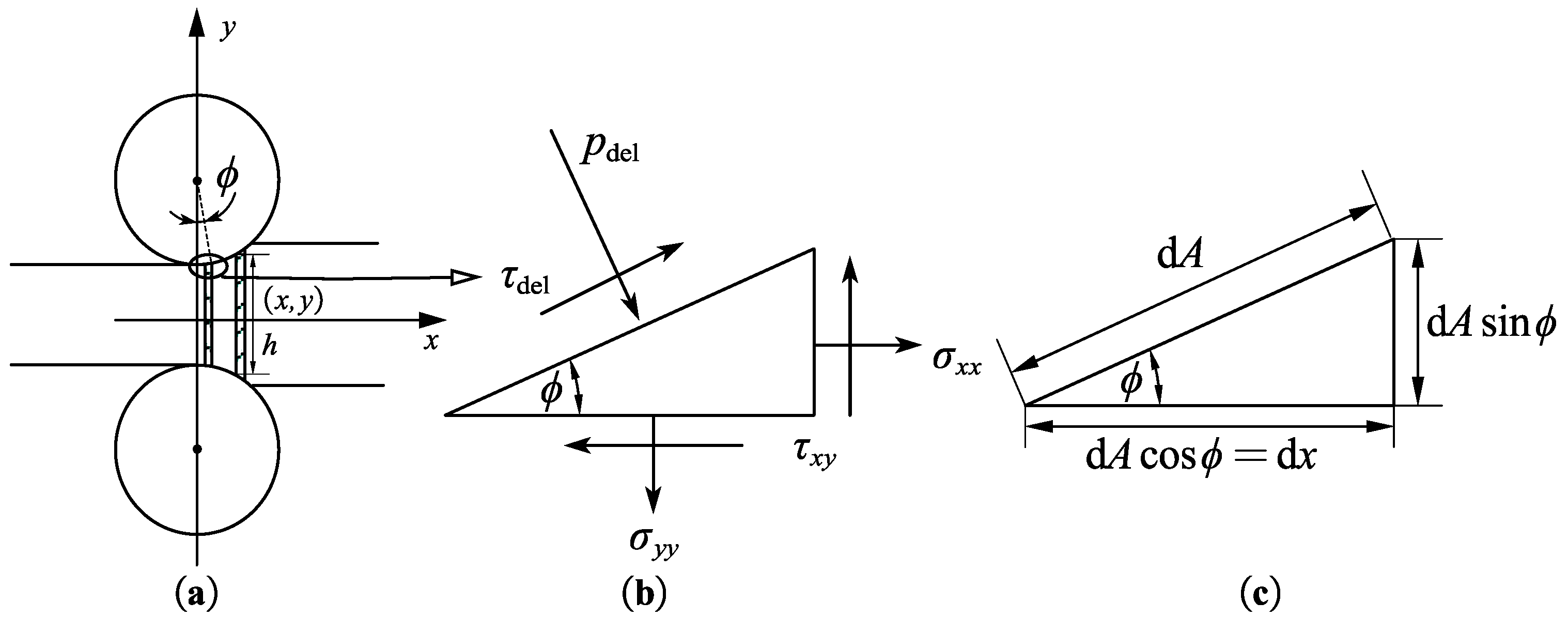
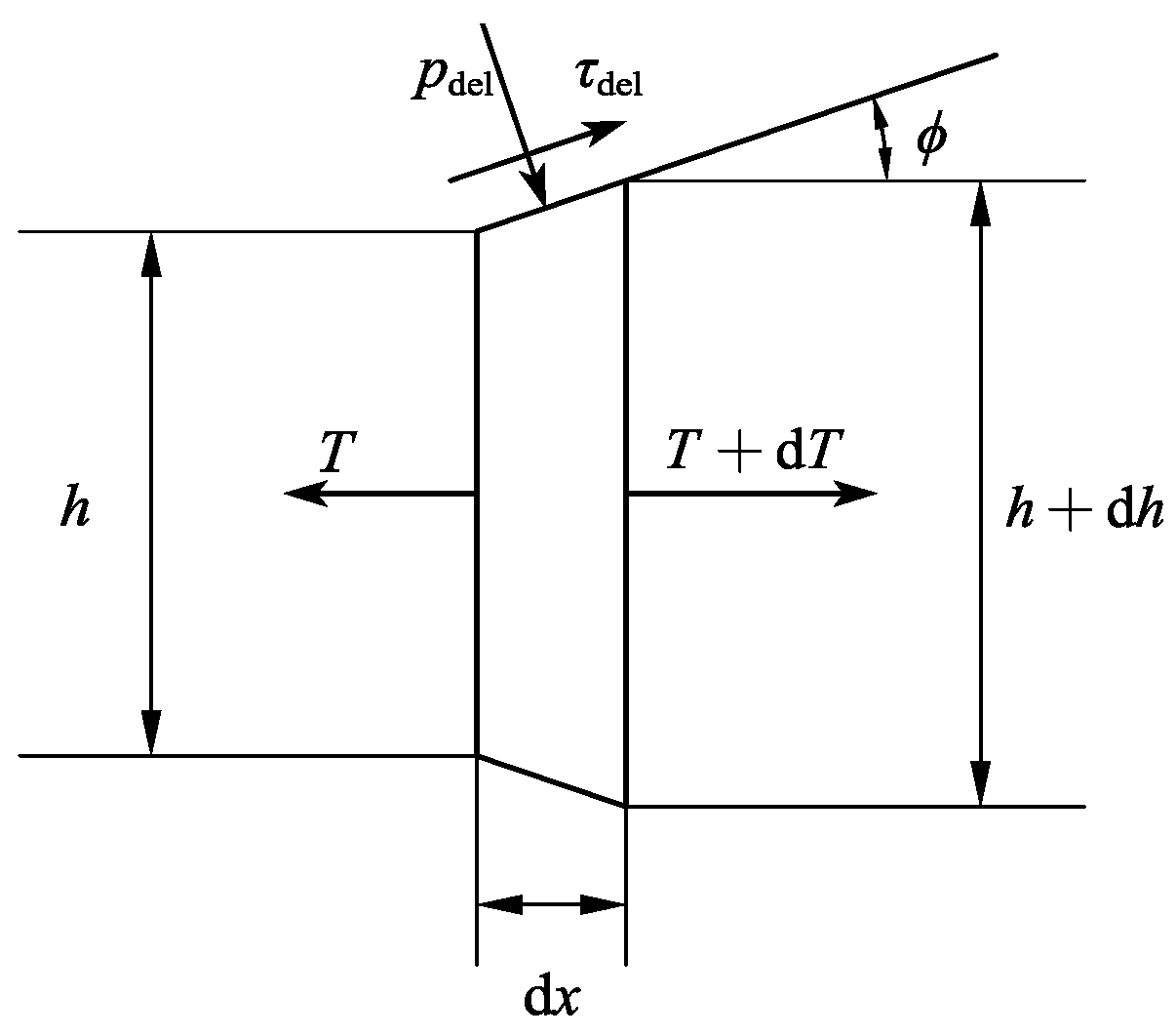
3.1. Rolling Process Model
3.2. Multi-System Coupling Dynamics Model
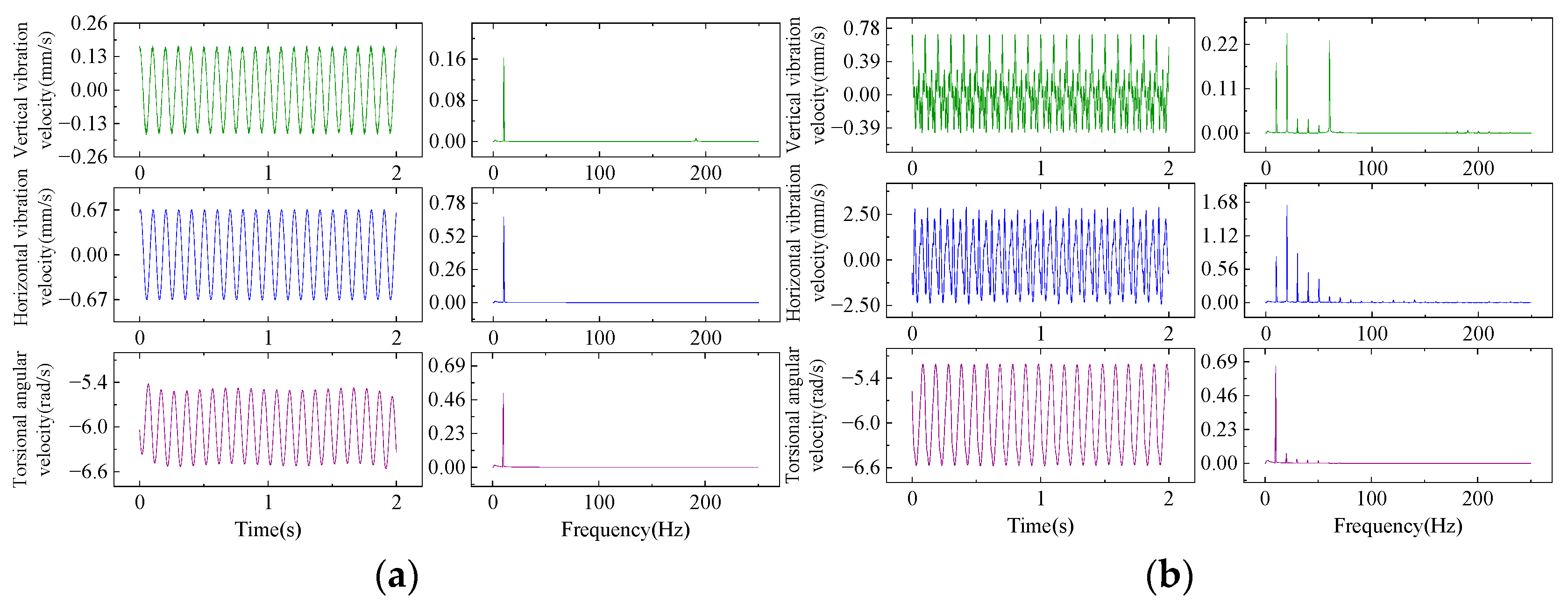
3.3. Model Verification
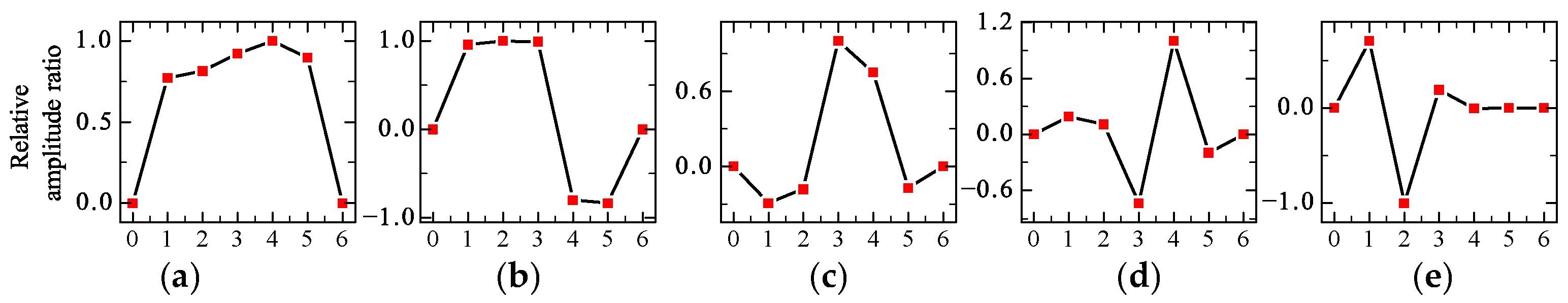
3.4. System Modal Analysis
3.5. Study on the Vibration Influence Law
3.5.1. Vibration Laws of the Rolling Mill with Different Thickness Fluctuation Forms
3.5.2. The Influence of αoff on Vibration
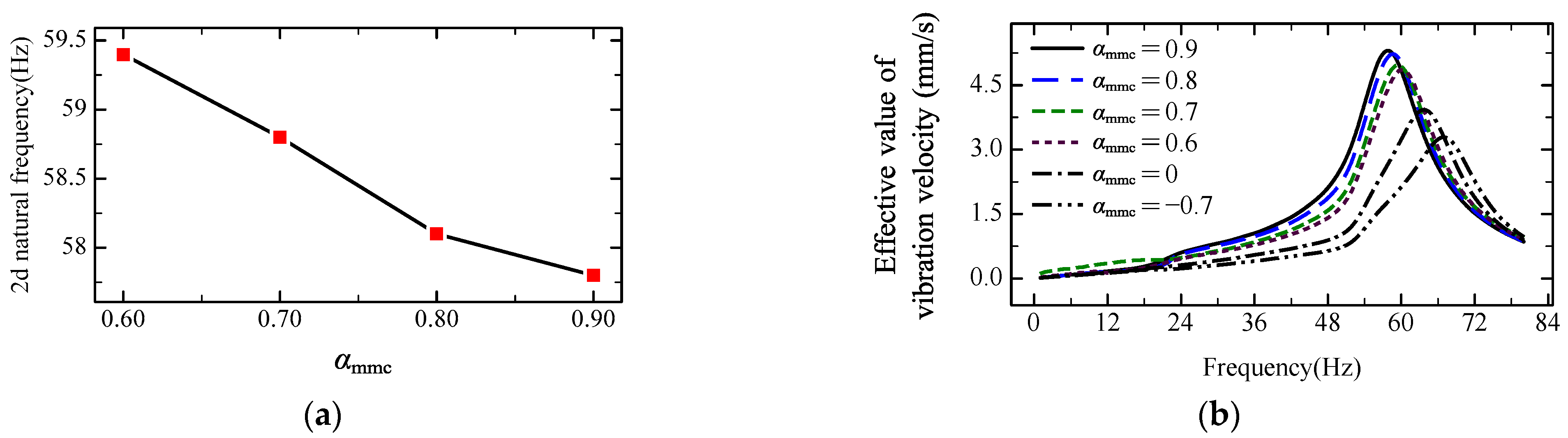
3.5.3. The Influence of Control Parameters αmmc on Vibration
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| F2 | The second finishing mill |
| Ap | Effective area of hydraulic cylinder piston |
| B | Strip width |
| ci | Equivalent damping |
| Cd | Flow coefficient |
| Ctp | Leakage coefficient |
| fi | Natural frequency of order i |
| fx | Horizontal component of rolling force |
| fy | Vertical component of rolling force |
| Fh | Hydraulic cylinder force |
| h | Thickness of the strip at x distance from the exit of the deformation zone |
| J2dev | The second invariant of stress eccentricity |
| k | The yield limit of the strip |
| ki | Equivalent stiffness |
| mi | Equivalent mass |
| M | Rolling torque |
| pdel | The normal stress of the strip surface |
| ps | Charge oil pressure |
| pL | Loading pressure |
| pt | Back pressure |
| R | Work roll radius |
| ΔSmmc | Roll gap compensation value |
| T | The horizontal force of the unit body |
| W | Area gradient |
| xv | Servo valve spool displacement |
| αoff | Roll offset angle |
| αmmc | Dynamic modulus compensation coefficient |
| βe | Hydraulic oil elastic modulus |
| ρ | Hydraulic oil density |
| σxx | The stress components in x direction |
| σinxx | The stress components in x direction of the strip interior |
| σs | Tensile yield strength |
| τs | Shear yield strength |
| τdel | The tangential stress of the strip surface |
References
- Yarita, I.; Furukawa, K.; Seino, Y.; Takimoto, T.; Nakazato, Y.; Nakagawa, K. An analysis of chattering in cold rolling for ultrathin gauge steel strip. Trans. Iron Steel Inst. Jpn. 1978, 18, 1–10. [Google Scholar] [CrossRef]
- Tlusty, J.; Chandra, G.; Critchley, S.; Paton, D. Chatter in Cold Rolling. CIRP Ann. 1982, 31, 195–199. [Google Scholar] [CrossRef]
- Yun, I.S.; Ehmann, K.F.; Wilson, W.R.D. Chatter in the Strip Rolling Process, Part 2: Dynamic Rolling Experiments. J. Manuf. Sci. Eng.-Trans. ASME 1998, 120, 337–342. [Google Scholar] [CrossRef]
- Yun, I.S.; Ehmann, K.F.; Wilson, W.R.D. Chatter in the Strip Rolling Process, Part 3: Chatter Model. J. Manuf. Sci. Eng.-Trans. ASME 1998, 120, 343–348. [Google Scholar] [CrossRef]
- Yun, I.S.; Wilson, W.R.D.; Ehmann, K.F. Chatter in the Strip Rolling Process, Part 1: Dynamic Model of Rolling. J. Manuf. Sci. Eng.-Trans. ASME 1998, 120, 330–336. [Google Scholar] [CrossRef]
- Gao, Z.Y.; Liu, Y.; Zhang, Q.D.; Liao, M.L.; Tian, B. Chatter model with structure-process-control coupled and stability analyses in the cold rolling system. Mech. Syst. Signal Proc. 2020, 140, 106692. [Google Scholar] [CrossRef]
- Heidari, A.; Forouzan, M.R. Optimization of cold rolling process parameters in order to increasing rolling speed limited by chatter vibrations. J. Adv. Res. 2013, 4, 27–34. [Google Scholar] [CrossRef] [PubMed]
- Zheng, Y.J.; Xie, Z.H.; Li, Y.; Shen, G.X.; Liu, H.M. Spatial vibration of rolling mills. J. Mater. Process. Technol. 2013, 213, 581–588. [Google Scholar] [CrossRef]
- Wang, L.D.; Yan, X.Q.; Jia, X.D.; Wang, X.L. Dynamic Amplitude-Frequency Characteristics of Vertical–Torsional Coupling System with Harmonic Response in Hot Tandem Mill. Electronics 2022, 11, 3031. [Google Scholar] [CrossRef]
- Jia, X.D.; Wang, S.; Yan, X.Q.; Wang, L.D.; Wang, H.P. Research on Dynamic Response of Cold Rolling Mill with Dynamic Stiffness Compensation. Electronics 2023, 12, 599. [Google Scholar] [CrossRef]
- Cui, J.X.; Peng, Y.; Wang, J. Instability of roll nonlinear system with structural clearance in rolling process. J. Iron Steel Res. Int. 2023, 30, 112–125. [Google Scholar] [CrossRef]
- Peng, R.R.; Zhang, X.Z.; Shi, P. Coupled Vibration Behavior of Hot Rolling Mill Rolls under Multinonlinear Effects. Shock Vib. 2020, 2020, 6104028. [Google Scholar] [CrossRef]
- Liu, Y.J.; Wang, S.; Qi, J.B.; Yan, X.Q. Vibrations of tandem cold rolling mill: Coupled excitation of rolling force and variable stiffness of fault-free back-up roll bearing. J. Iron Steel Res. Int. 2023, 30, 1792–1802. [Google Scholar] [CrossRef]
- Zeng, L.Q.; Zang, Y.; Gao, Z.Y. Hopf Bifurcation Control for Rolling Mill Multiple-Mode-Coupling Vibration Under Nonlinear Friction. J. Vib. Acoust. 2017, 139, 061015. [Google Scholar] [CrossRef]
- Kapil, S.; Eberhard, P.; Dwivedy, S.K. Nonlinear Dynamic Analysis of a Parametrically Excited Cold Rolling Mill. J. Manuf. Sci. Eng. 2014, 136, 041012. [Google Scholar] [CrossRef]
- Hou, D.X.; Peng, R.R.; Liu, H.R. Analysis of Vertical-Horizontal Coupling Vibration Characteristics of Rolling Mill Rolls Based on Strip Dynamic Deformation Process. Shock Vib. 2014, 2014, 543793. [Google Scholar] [CrossRef]





| Parameter | Value | Unit | Parameter | Value | Unit | Parameter | Value | Unit |
|---|---|---|---|---|---|---|---|---|
| k1 | 2 × 1010 | N/m | m1 | 6 × 104 | kg | c1 | 1 × 107 | N·s/m |
| k2 | 3 × 1010 | N/m | m2 | 3 × 104 | kg | c2 | 1 × 107 | N·s/m |
| k3 | 3 × 1010 | N/m | m3 | 3 × 104 | kg | c3 | 2 × 106 | N·s/m |
| k4 | 2 × 1010 | N/m | m4 | 15 × 104 | kg | c4 | 2 × 106 | N·s/m |
| k5 | 3.1 × 108 | N/m | J1 | 178 | kg·m2 | c5 | 106 | N·s/m |
| k6 | 3.1 × 108 | N/m | J2 | 178 | kg·m2 | c6 | 106 | N·s/m |
| k7 | 8 × 105 | N·m/rad | J3 | 15,000 | kg·m2 | c7 | 3000 | N·s/m |
| k8 | 8 × 105 | N·m/rad | J4 | 15,000 | kg·m2 | c8 | 3000 | N·s/m |
| Process Parameter | Value |
|---|---|
| Deformation resistance | 200 MPa |
| Inlet thickness | 22 mm |
| Outlet thickness | 13.48 mm |
| Breadth | 1500 mm |
| Design stiffness of vertical system | 5.8 × 109–6.1 × 109 N/m |
| Experimental measured rolling force | 2.6 × 107 N |
| Calculation stiffness of vertical system | 6.0 × 109 N/m |
| Model calculation of rolling force | 2.48 × 107 N |
| Vertical System | Horizontal System | Torsional System | |||||||
|---|---|---|---|---|---|---|---|---|---|
| Natural frequency | f1 | f2 | f3 | f4 | f5 | f6 | f7 | f8 | f9 |
| Value/Hz | 52.83 | 59.98 | 174.52 | 184.42 | 399.03 | 19.93 | 19.93 | 10.73 | 10.73 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, Y.; Wang, S.; Wang, X.; Yan, X. Research on Multi-System Coupling Vibration of a Hot Tandem Mill. Machines 2024, 12, 302. https://doi.org/10.3390/machines12050302
Liu Y, Wang S, Wang X, Yan X. Research on Multi-System Coupling Vibration of a Hot Tandem Mill. Machines. 2024; 12(5):302. https://doi.org/10.3390/machines12050302
Chicago/Turabian StyleLiu, Yujie, Shen Wang, Xuewei Wang, and Xiaoqiang Yan. 2024. "Research on Multi-System Coupling Vibration of a Hot Tandem Mill" Machines 12, no. 5: 302. https://doi.org/10.3390/machines12050302
APA StyleLiu, Y., Wang, S., Wang, X., & Yan, X. (2024). Research on Multi-System Coupling Vibration of a Hot Tandem Mill. Machines, 12(5), 302. https://doi.org/10.3390/machines12050302





