Spring-Damped Underactuated Swashplateless Rotor on a Bicopter Unmanned Aerial Vehicle
Abstract
1. Introduction
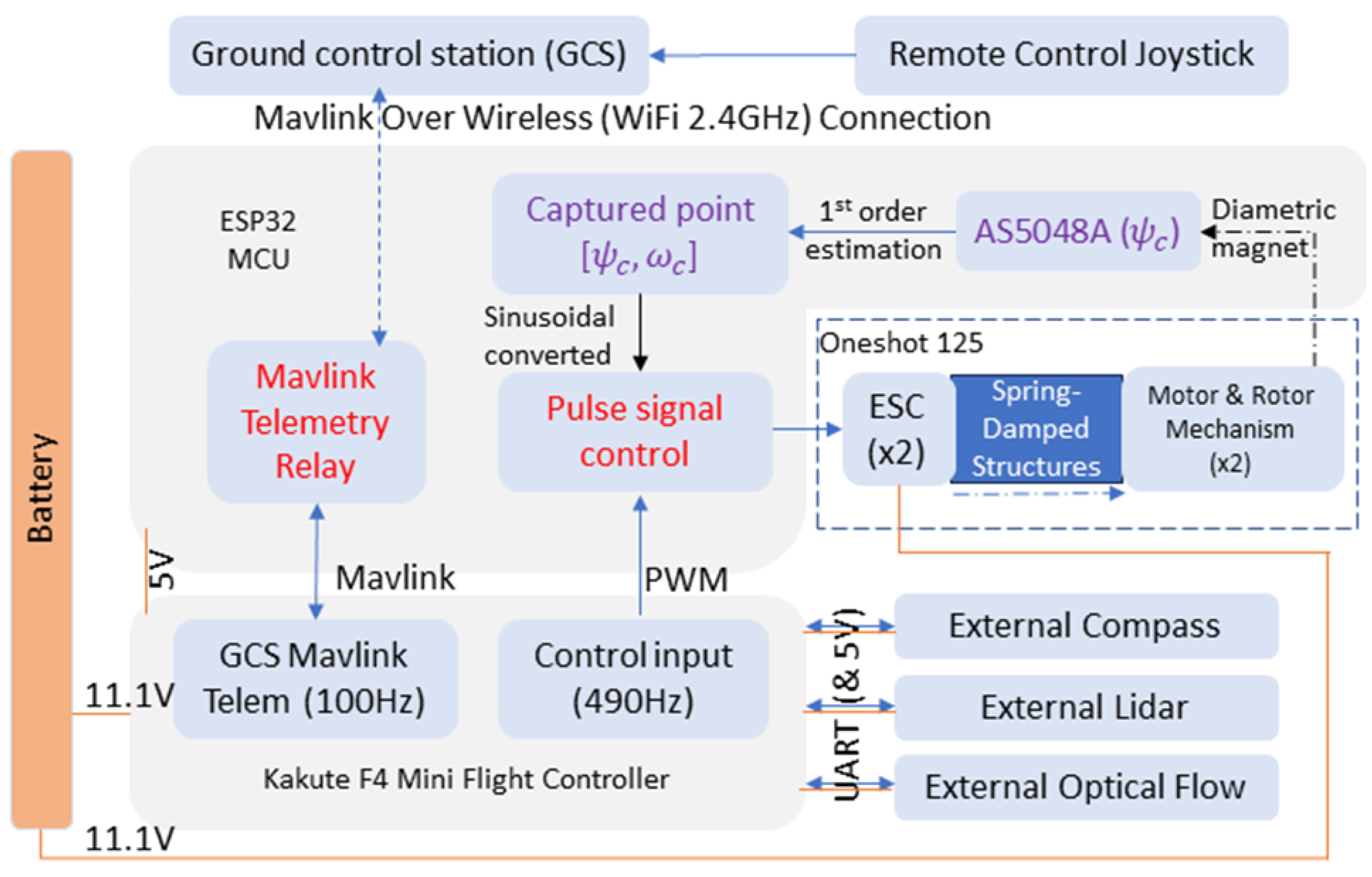
2. Proposed Design
- Generating sinusoidal pulse control signal to ESC using Oneshot125 protocol at 4 kHz (task 1, on MCU core 1).
- Retrieve the 14-bit rotary encoder data with SPI communication with a stable clock following task 1 (task 1, on MCU core 1, SPI protocol).
- Passing the telemetry signal with the rest of the clock time using the MAVLink protocol with a refresh rate of 100 Hz, parallel to other tasks (task 0, on MCU core 0).
- Retrieving PWM signal from flight controller varying from 50 Hz to 490 Hz, parallel to other tasks (digital-pin-change interruption).
3. Materials and Methods
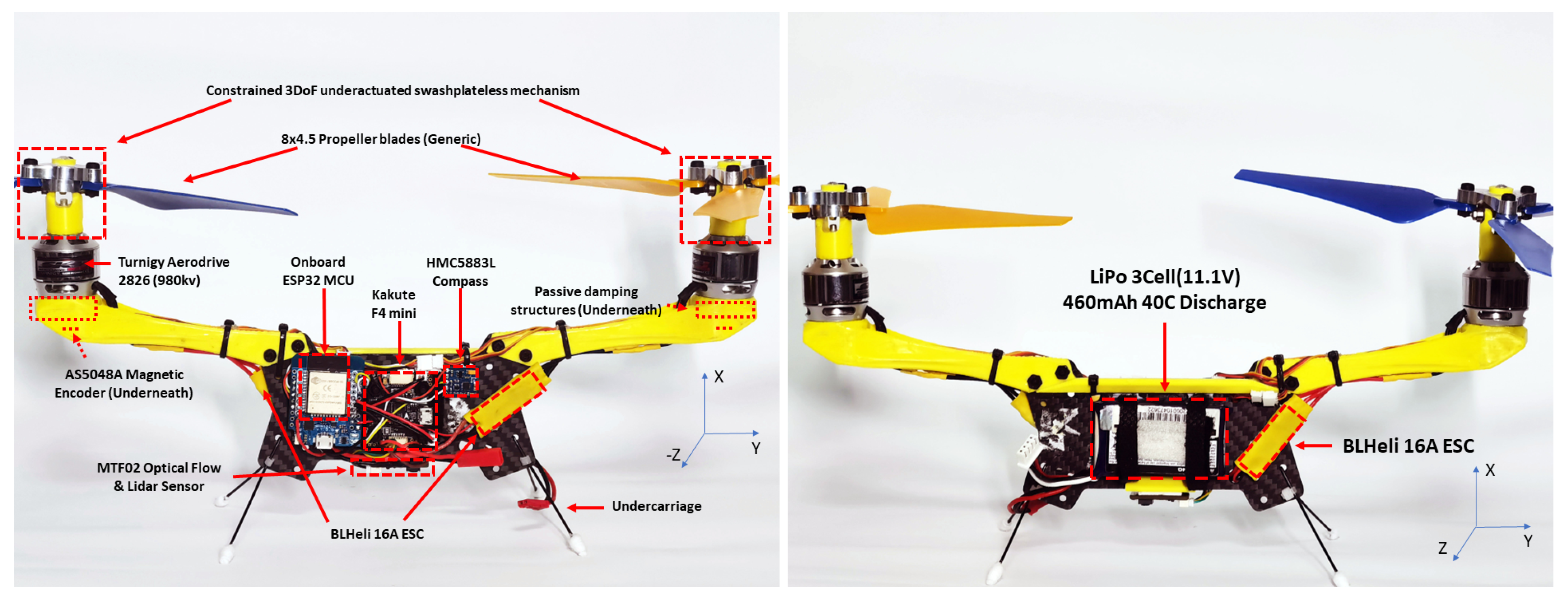
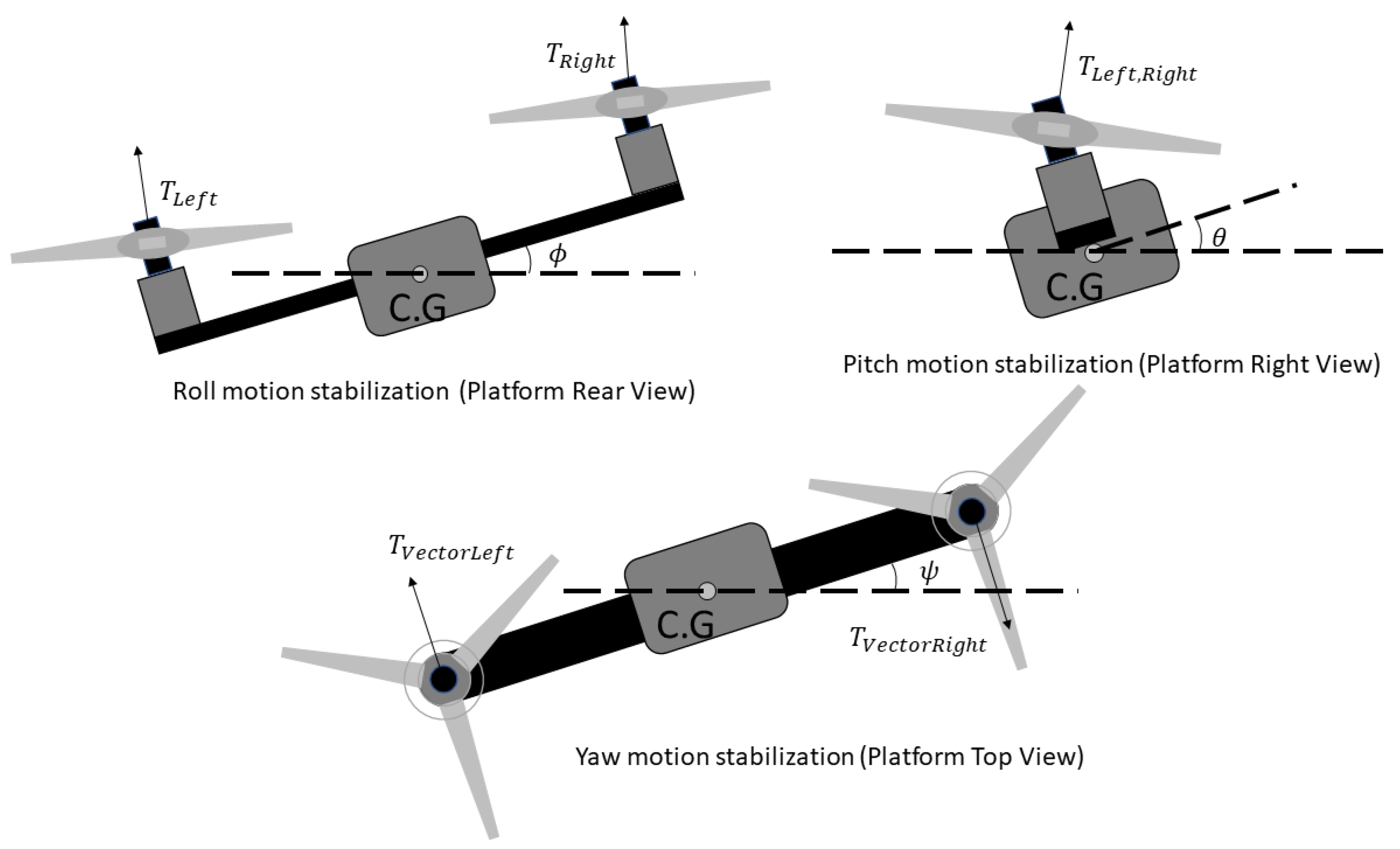
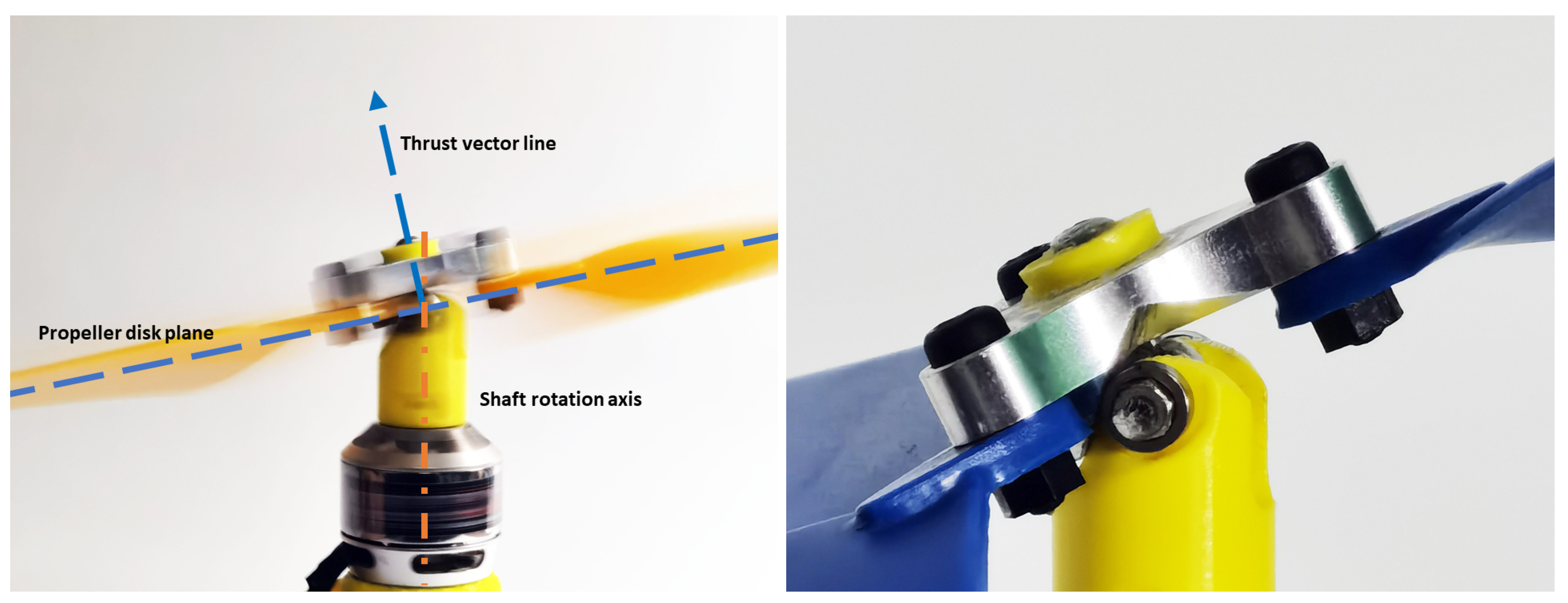
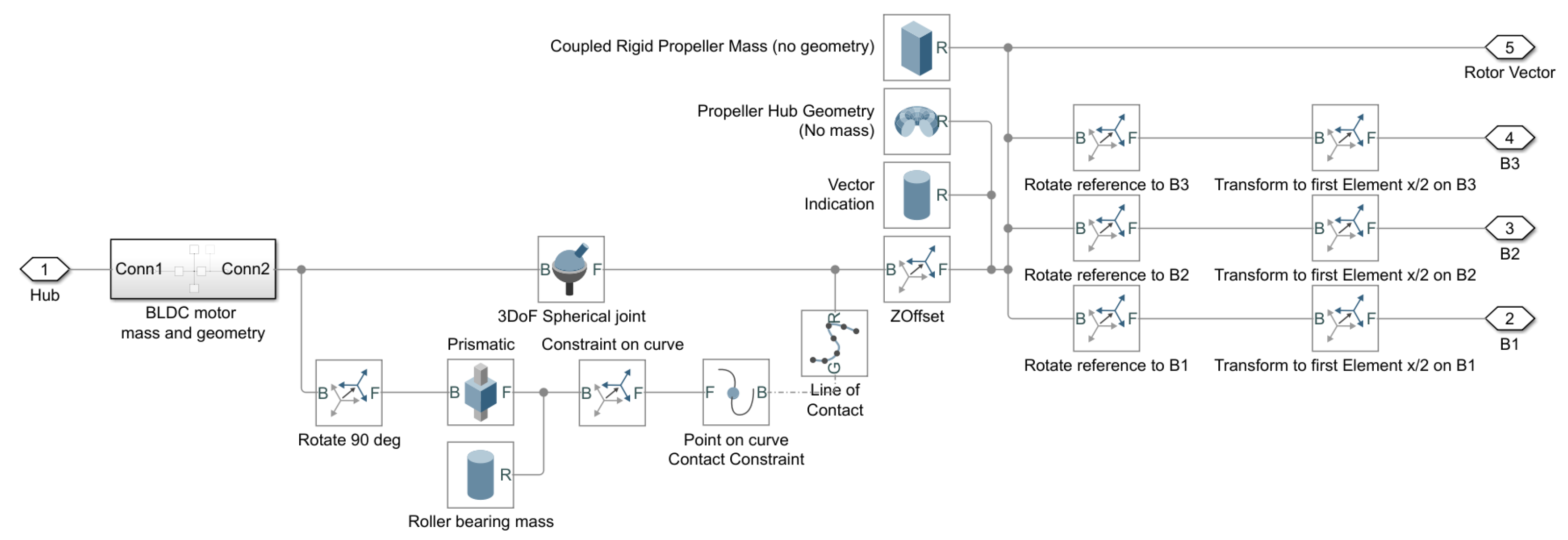
3.1. Constrained 3DoF Underactuated Swashplateless Propeller Mechanism
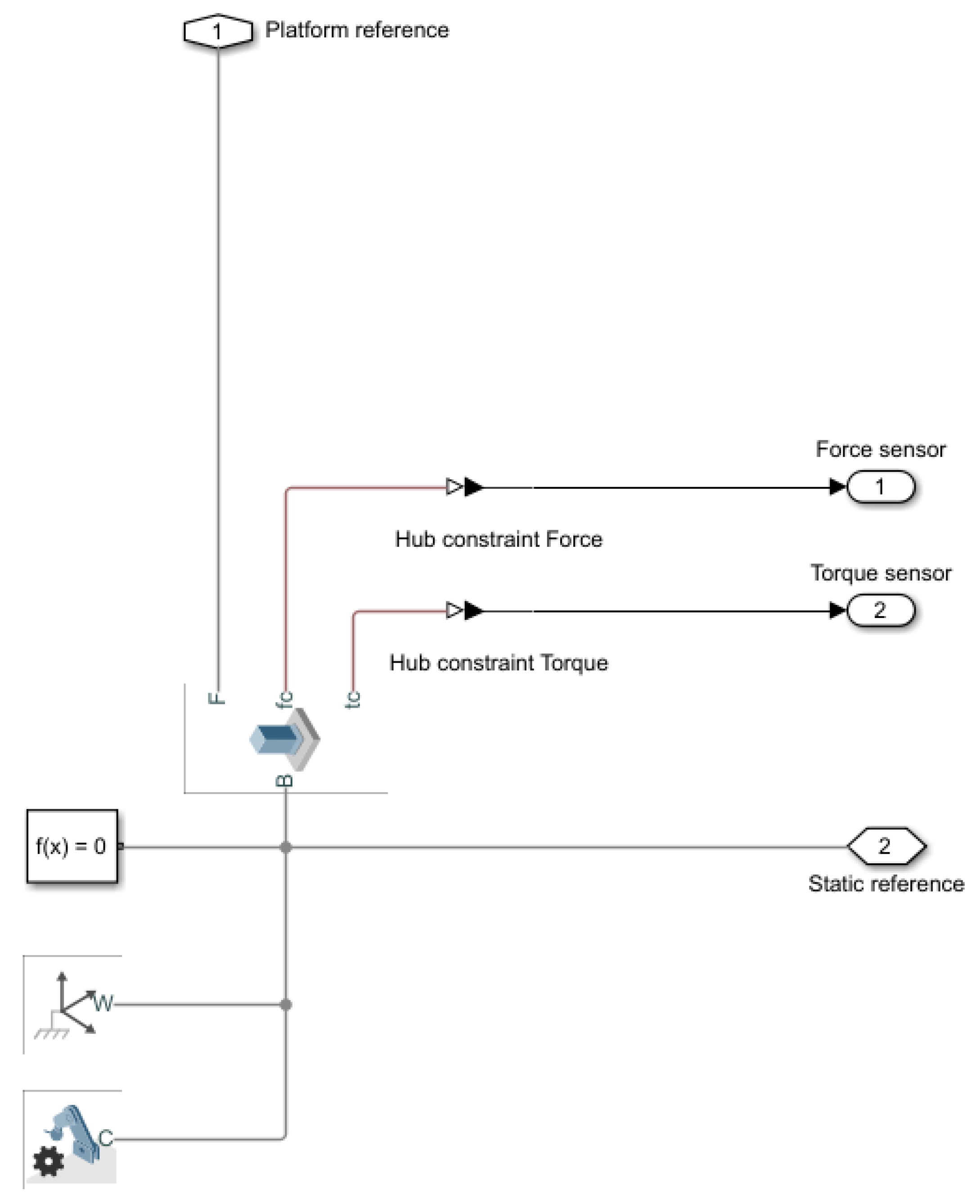
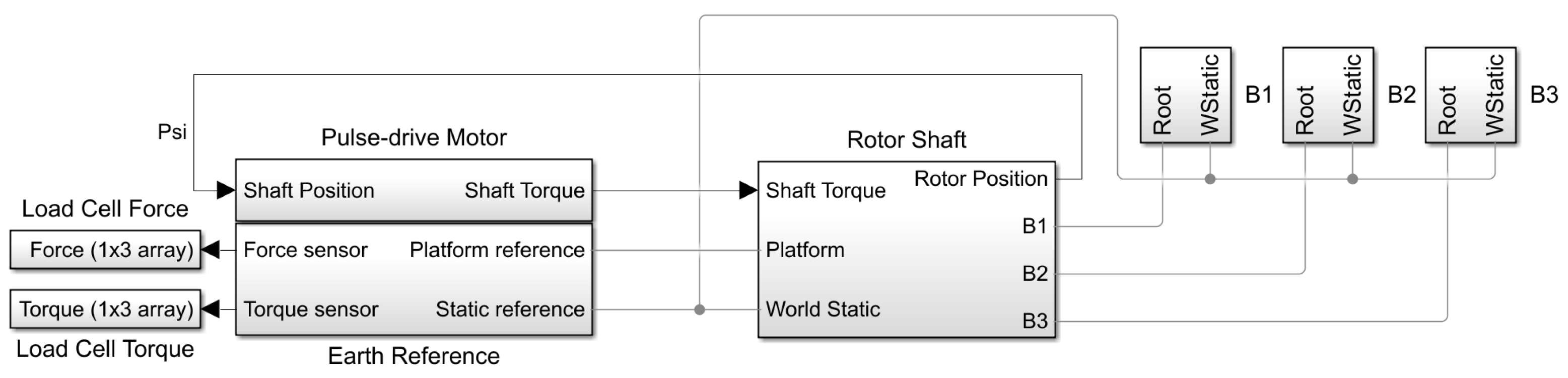
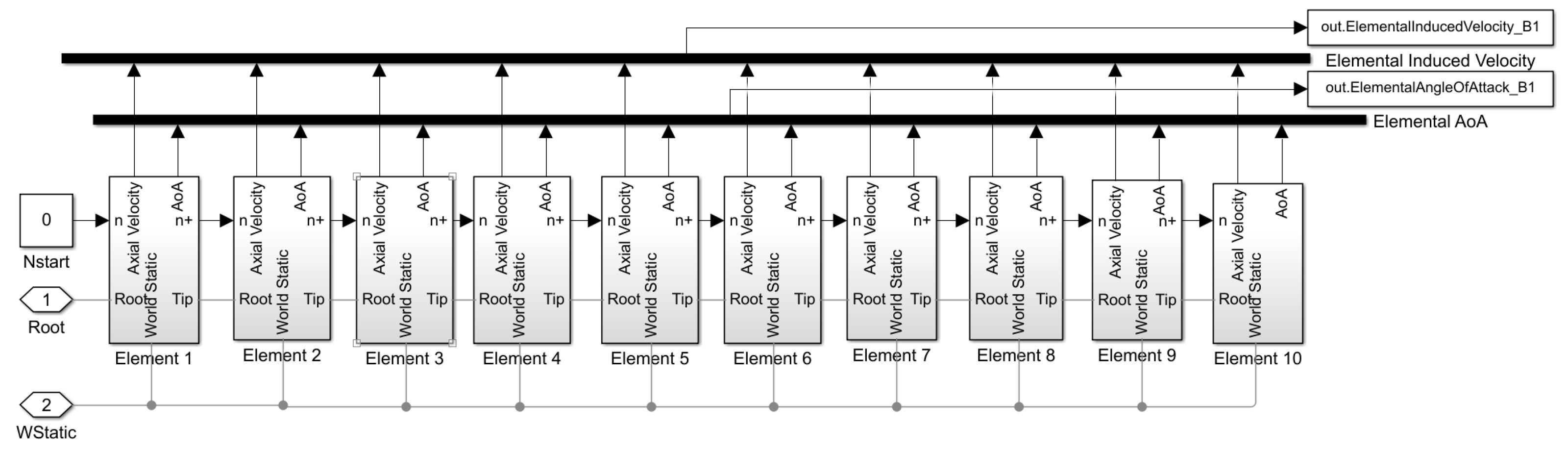
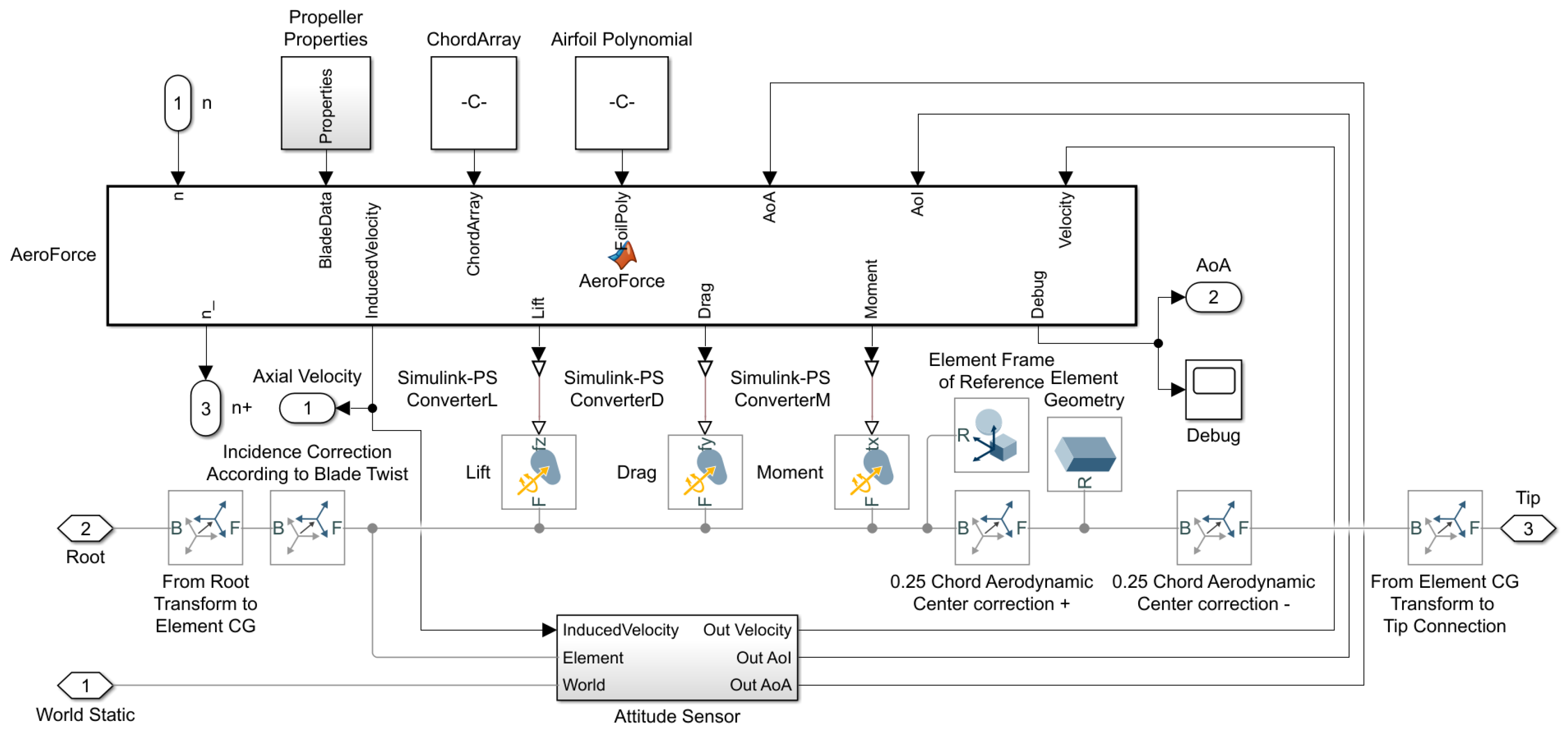
3.2. Simulink Simscape Modelling
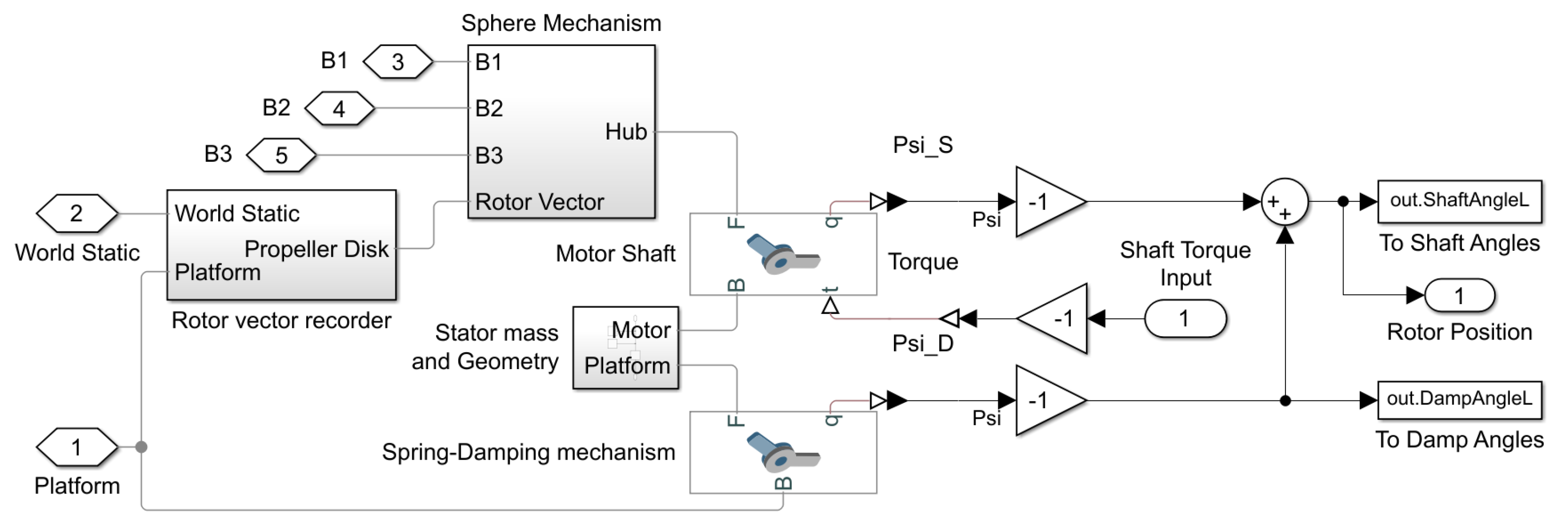
3.2.1. Model of Spring-Damping Structures in Simulation
3.2.2. Model Swashplateless Mechanism in Simulation
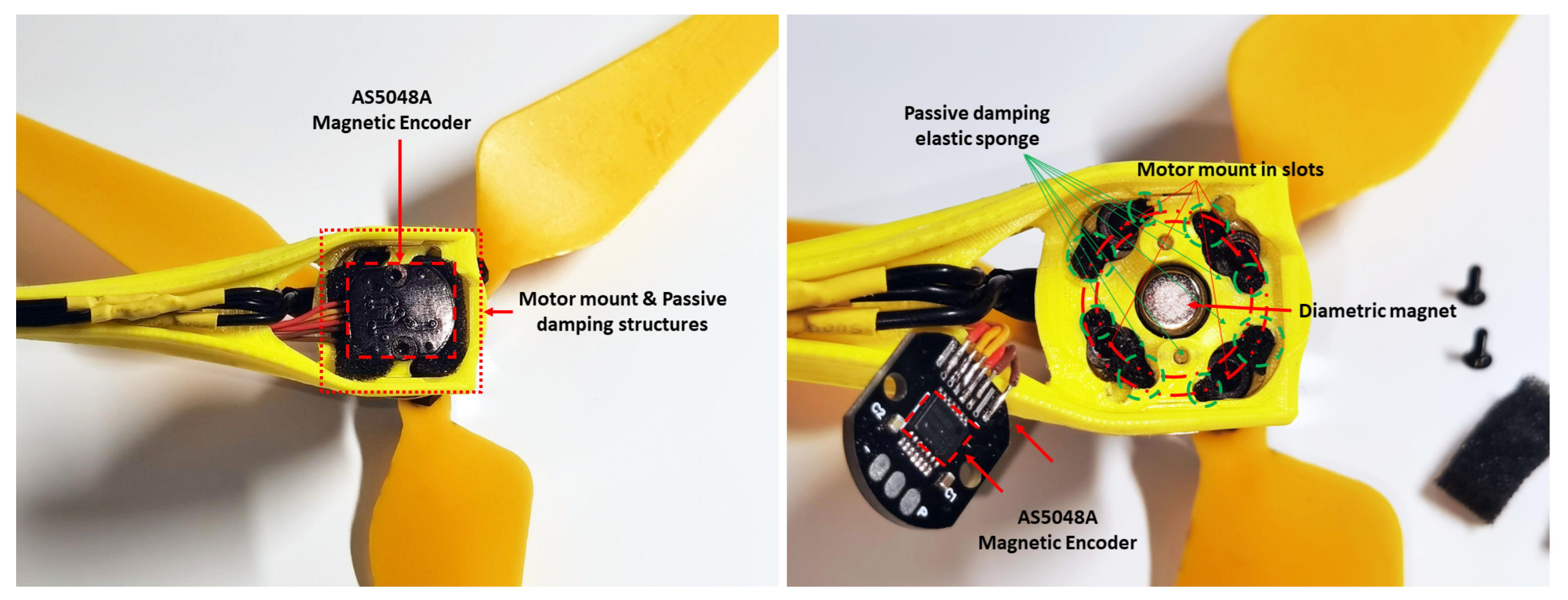
3.3. Components Design and Integration
3.4. Simulation and Experimental Setup
4. Results
4.1. Rotor Mechanism Damping Coefficient Estimation
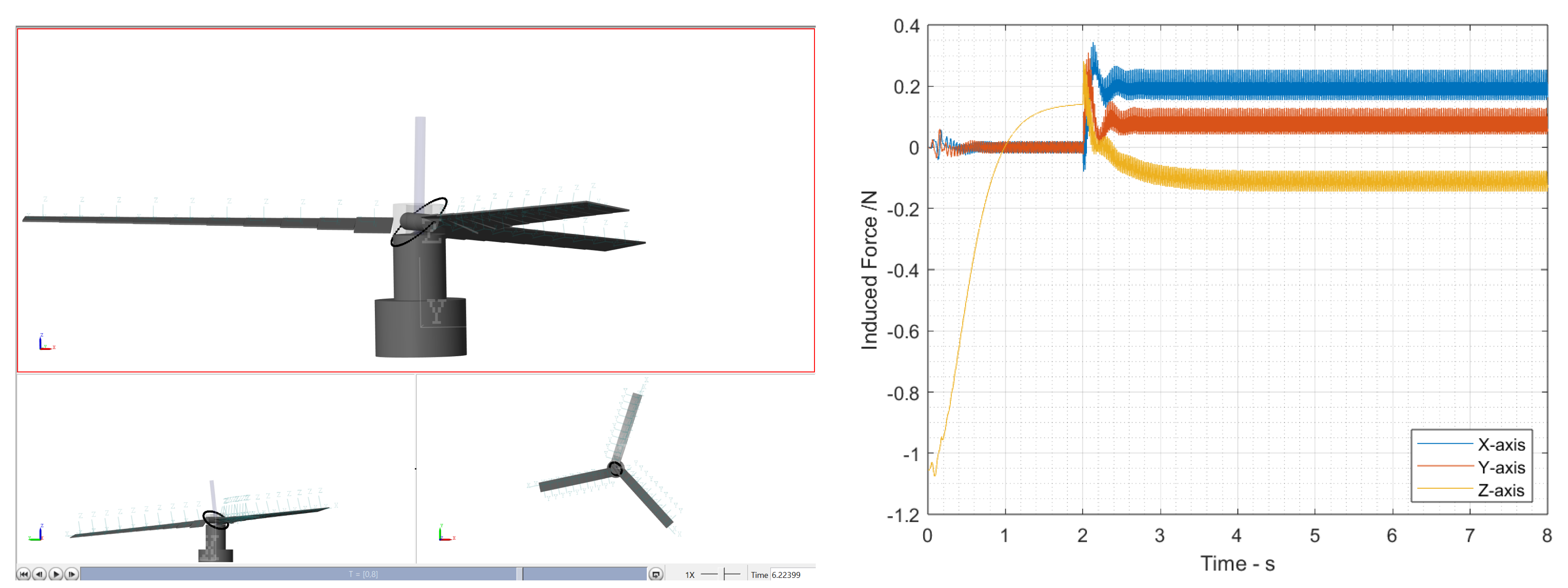
4.2. Simulation Results
Stator Damping Coefficient Estimation
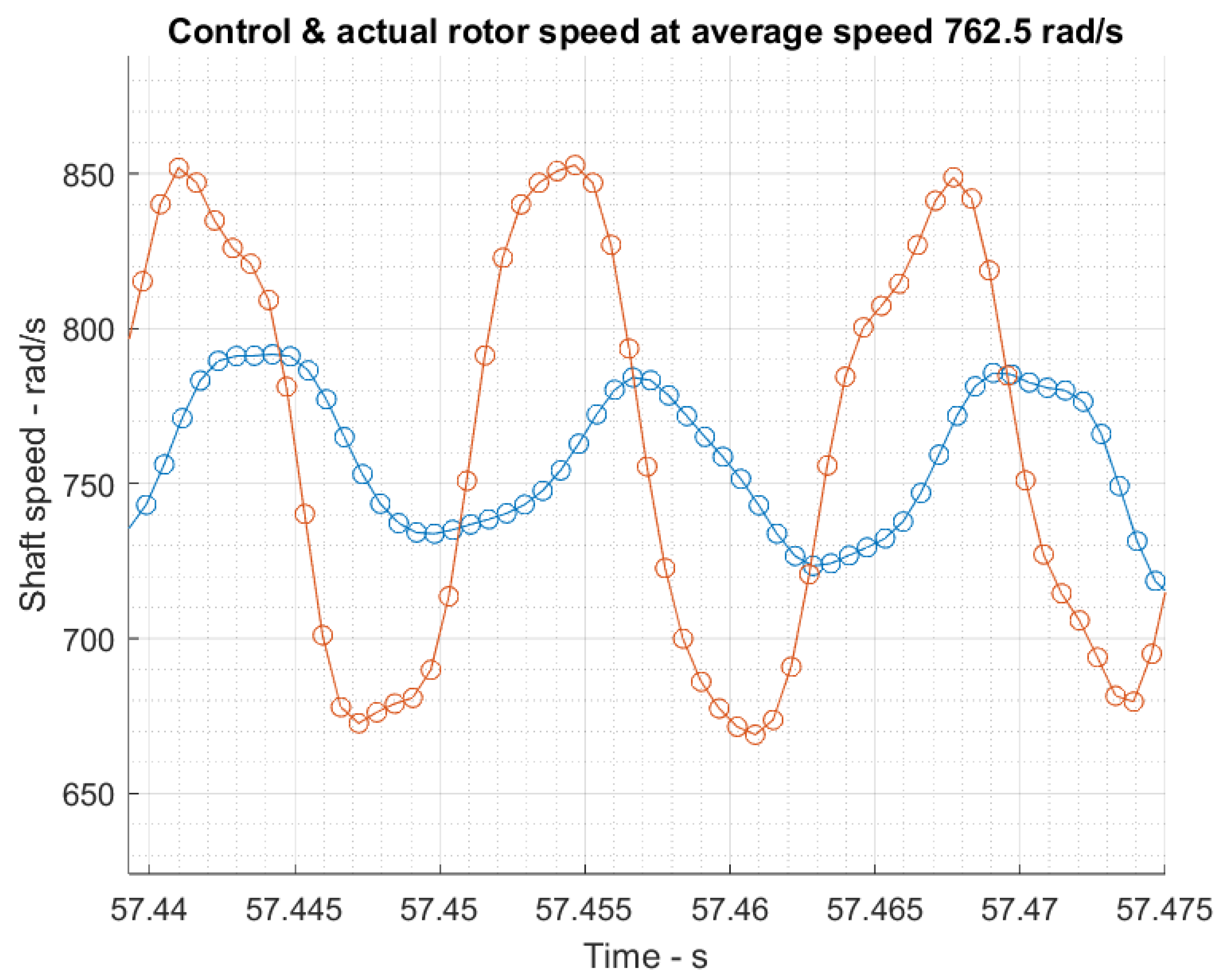
4.3. Platform Test
5. Discussion and Conclusions
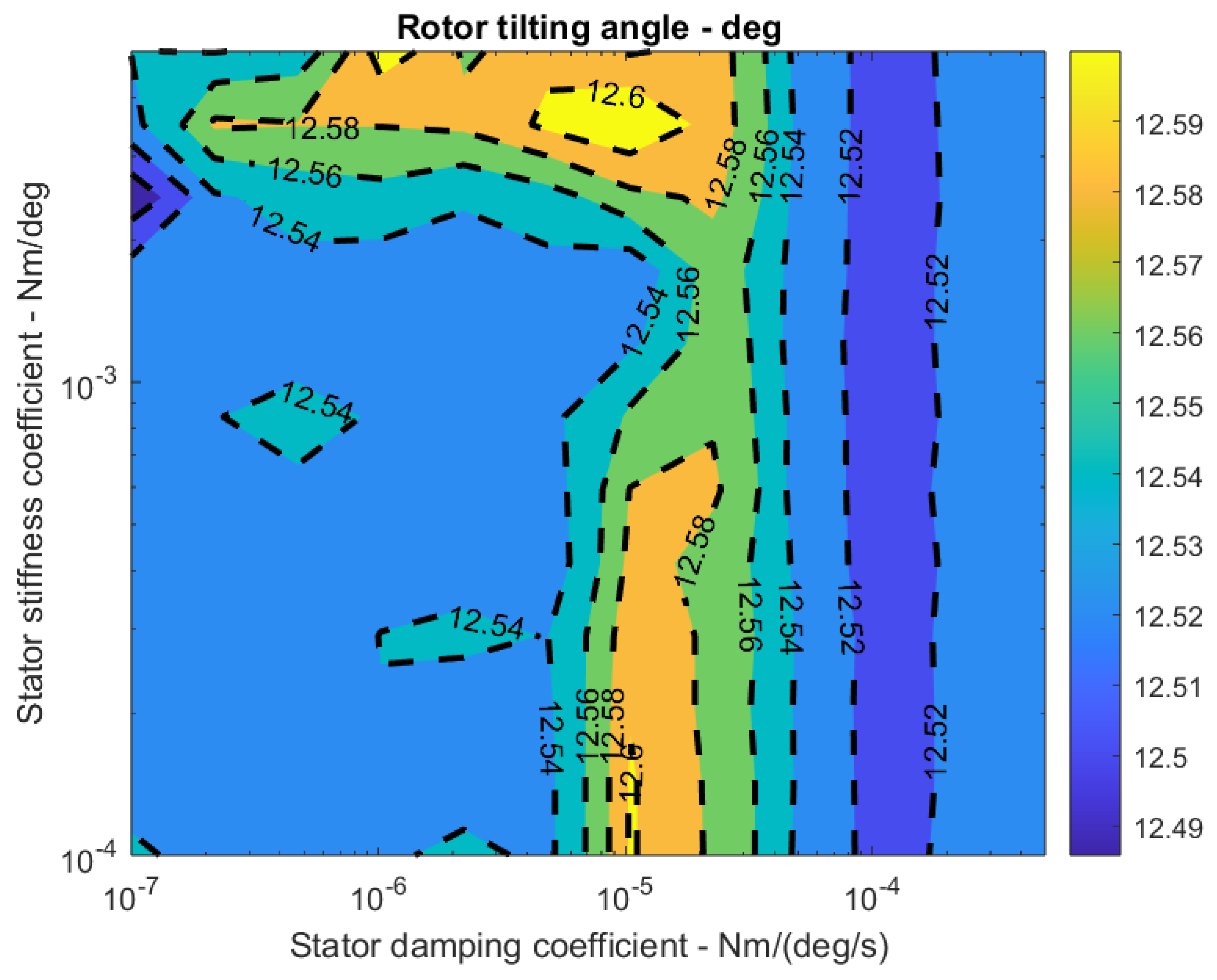
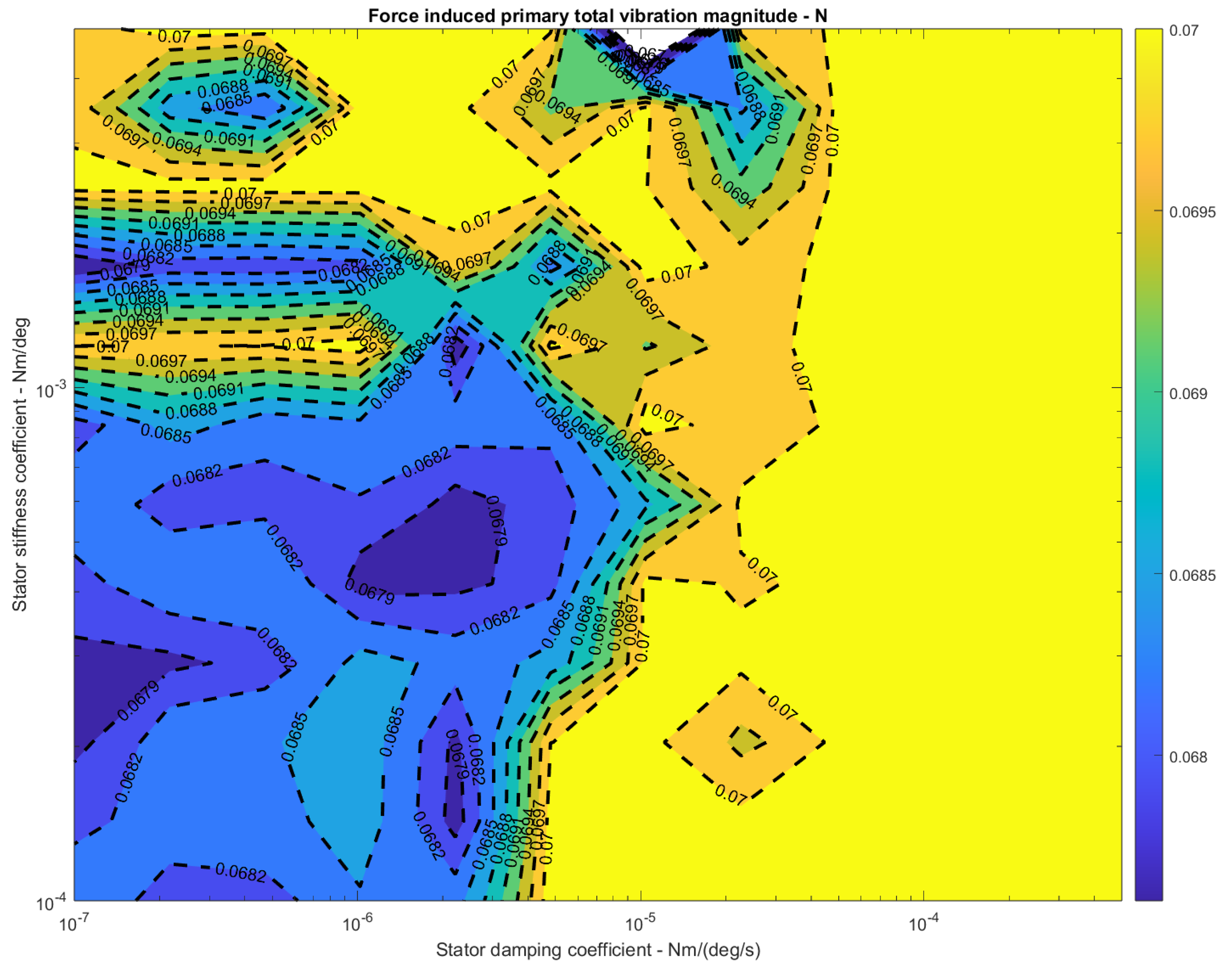
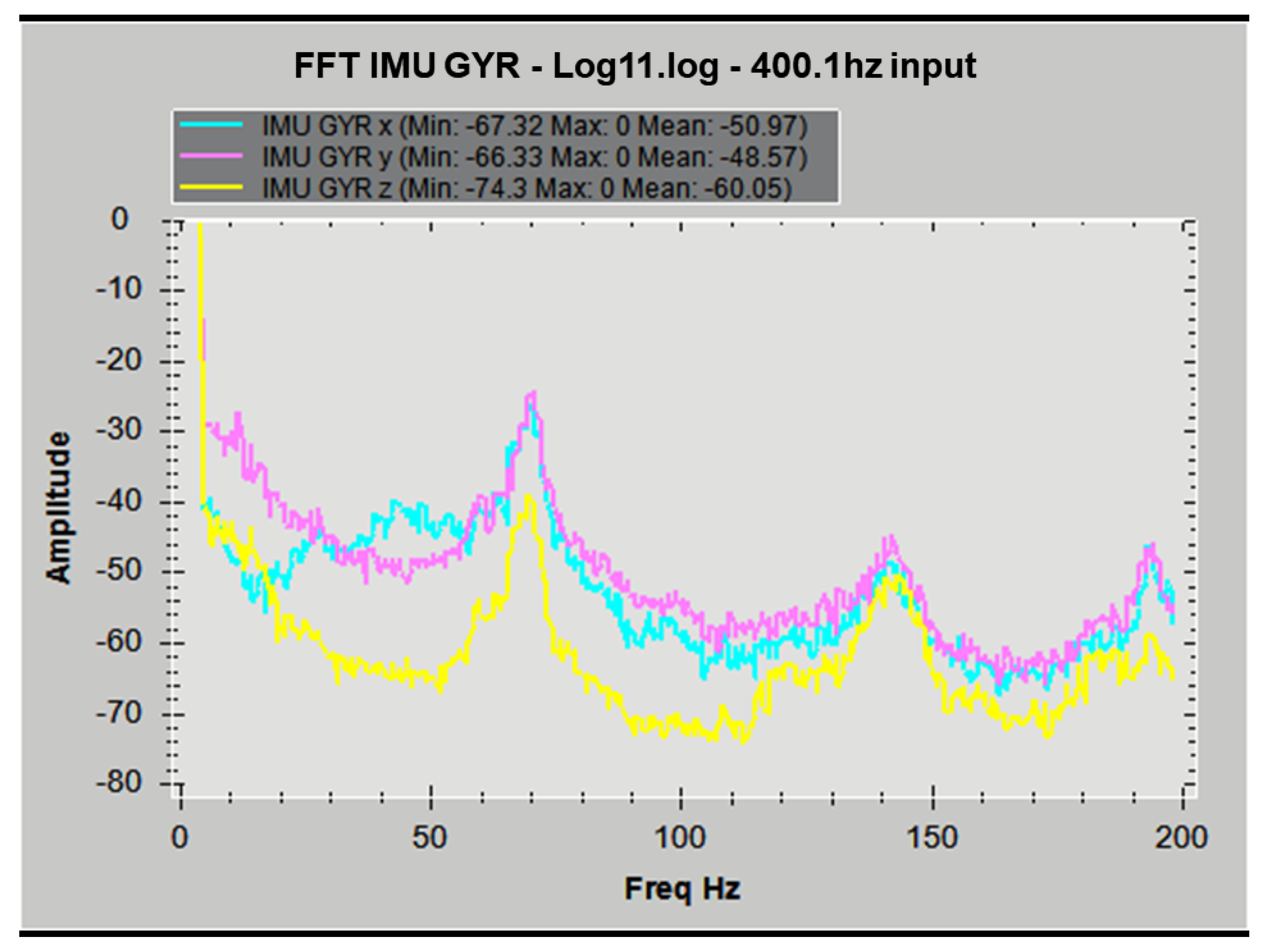
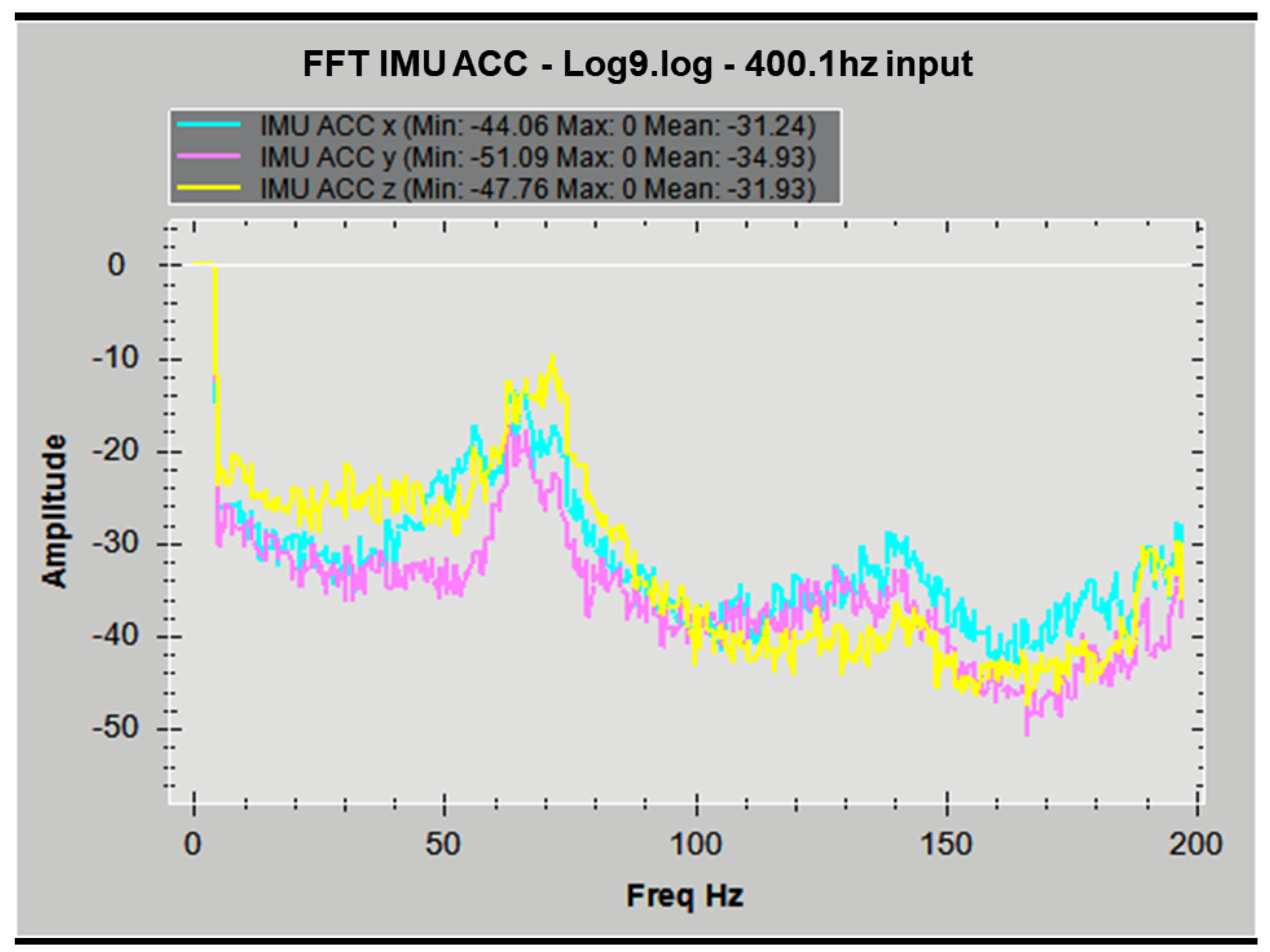
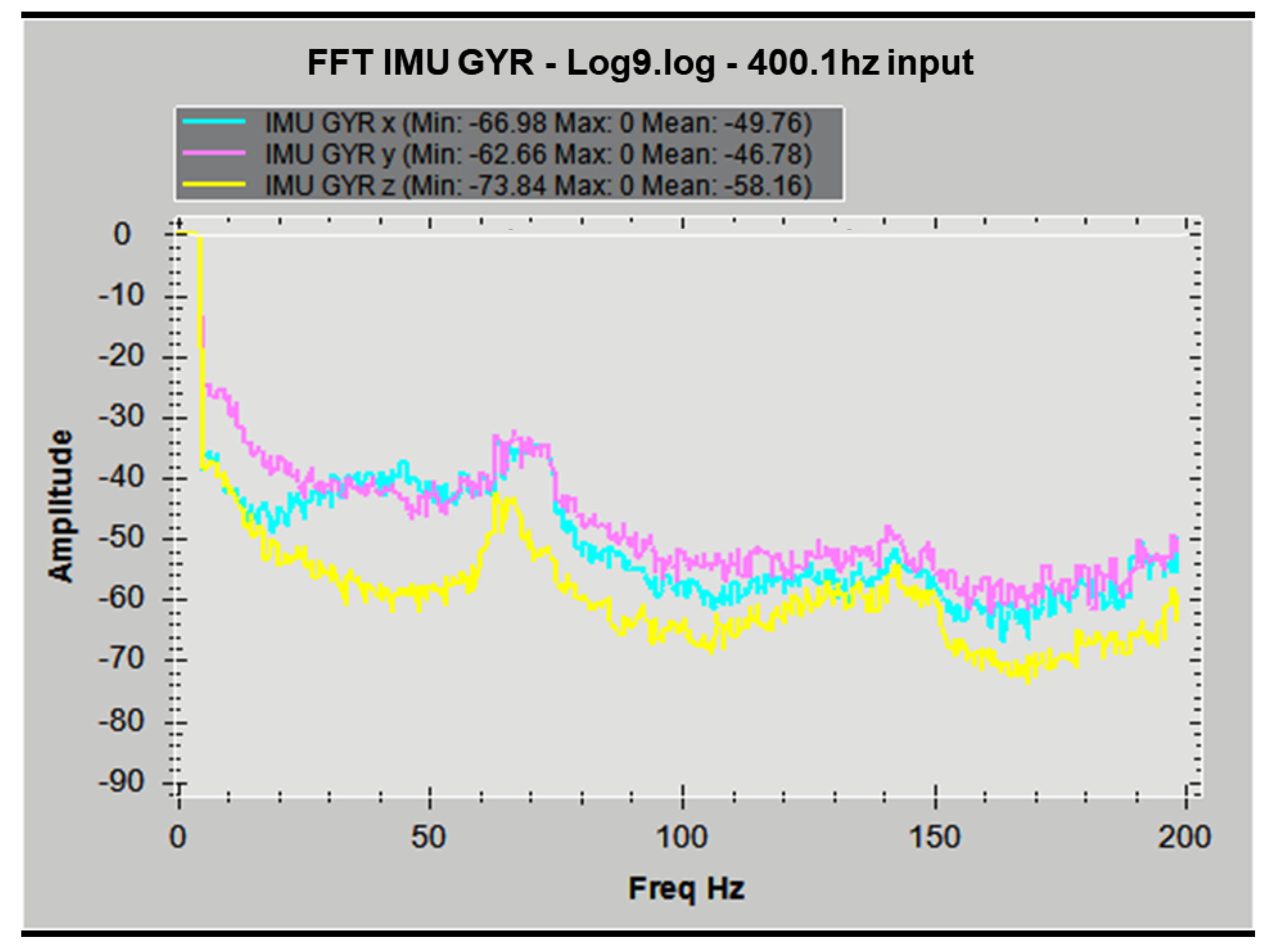
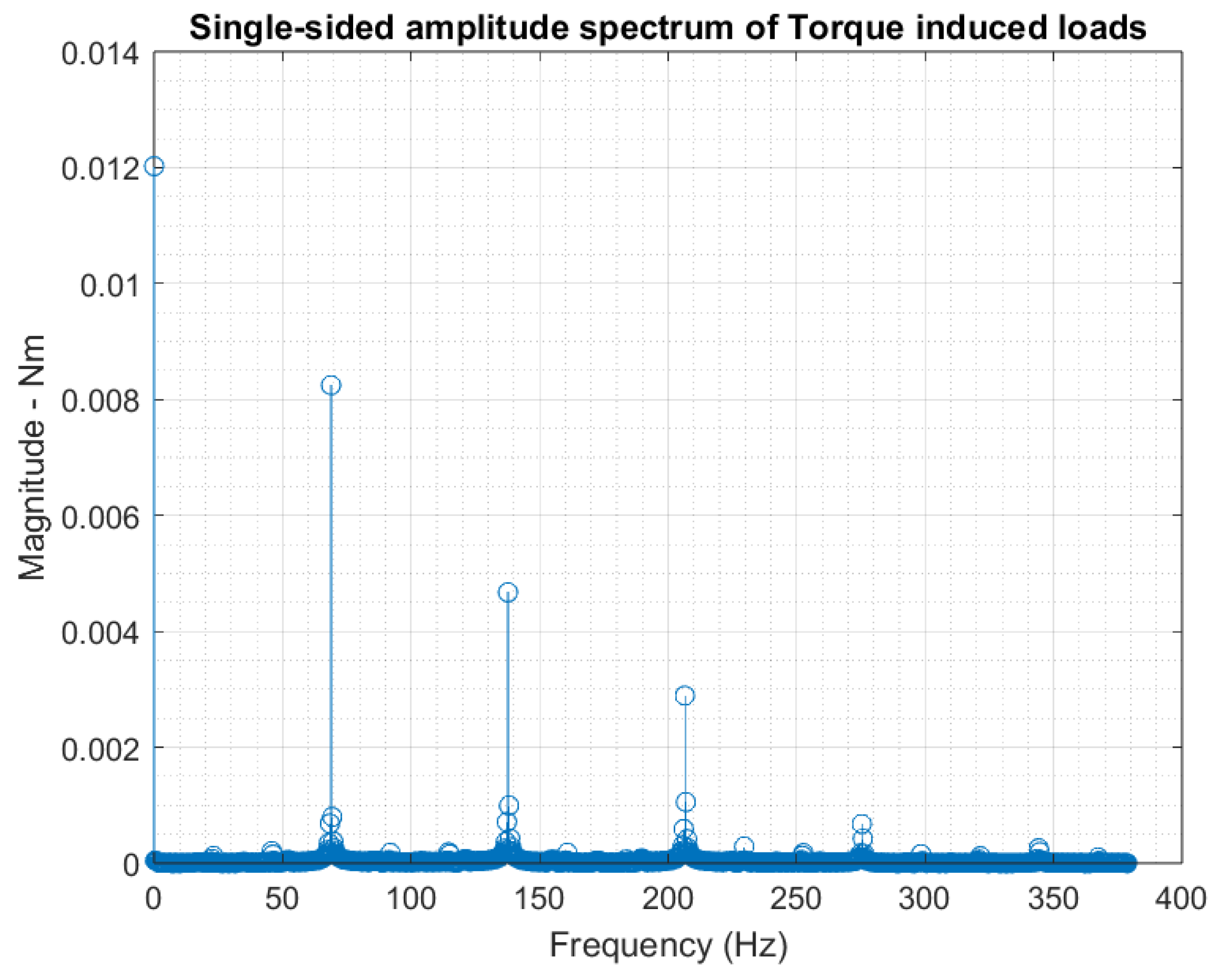
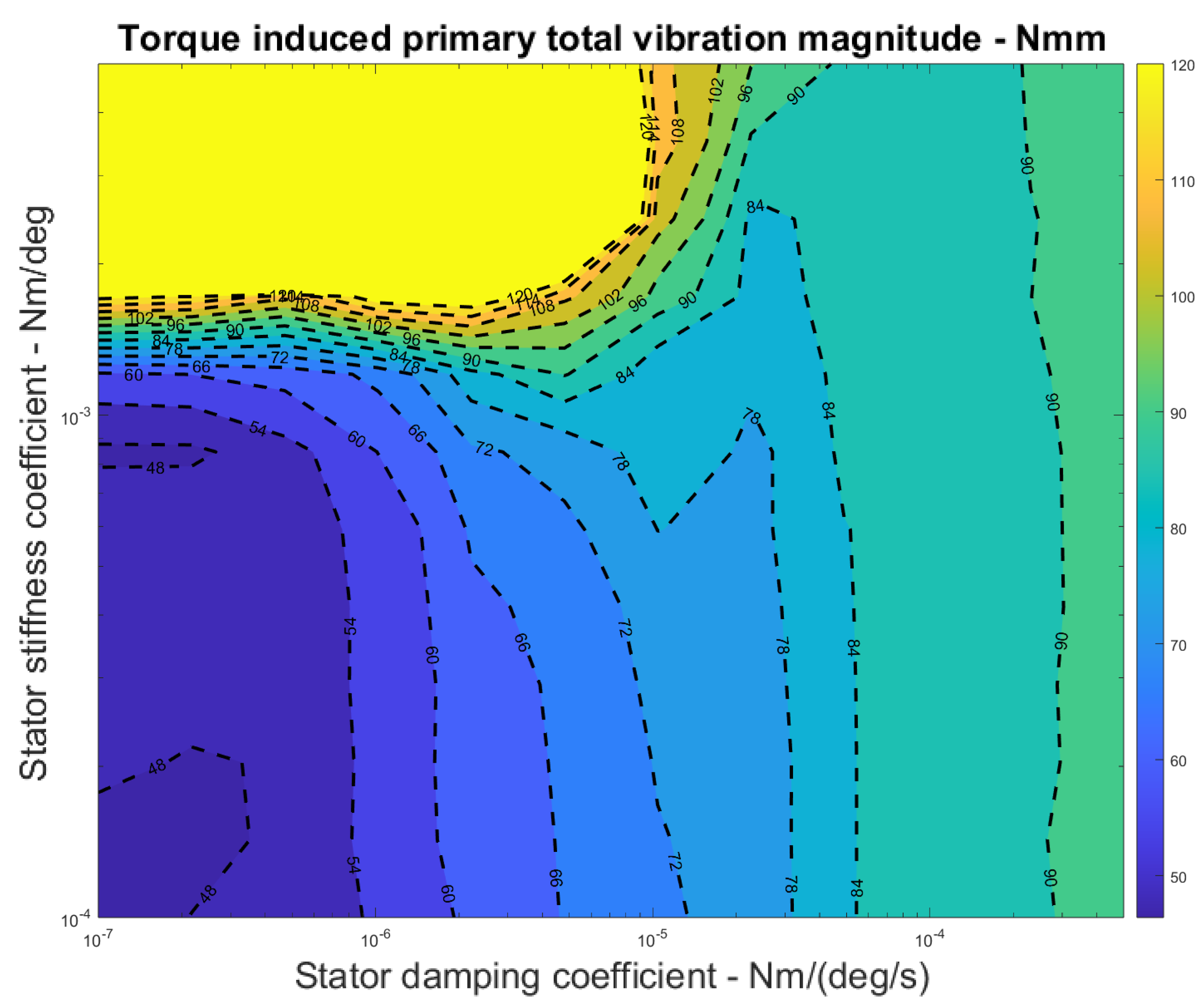
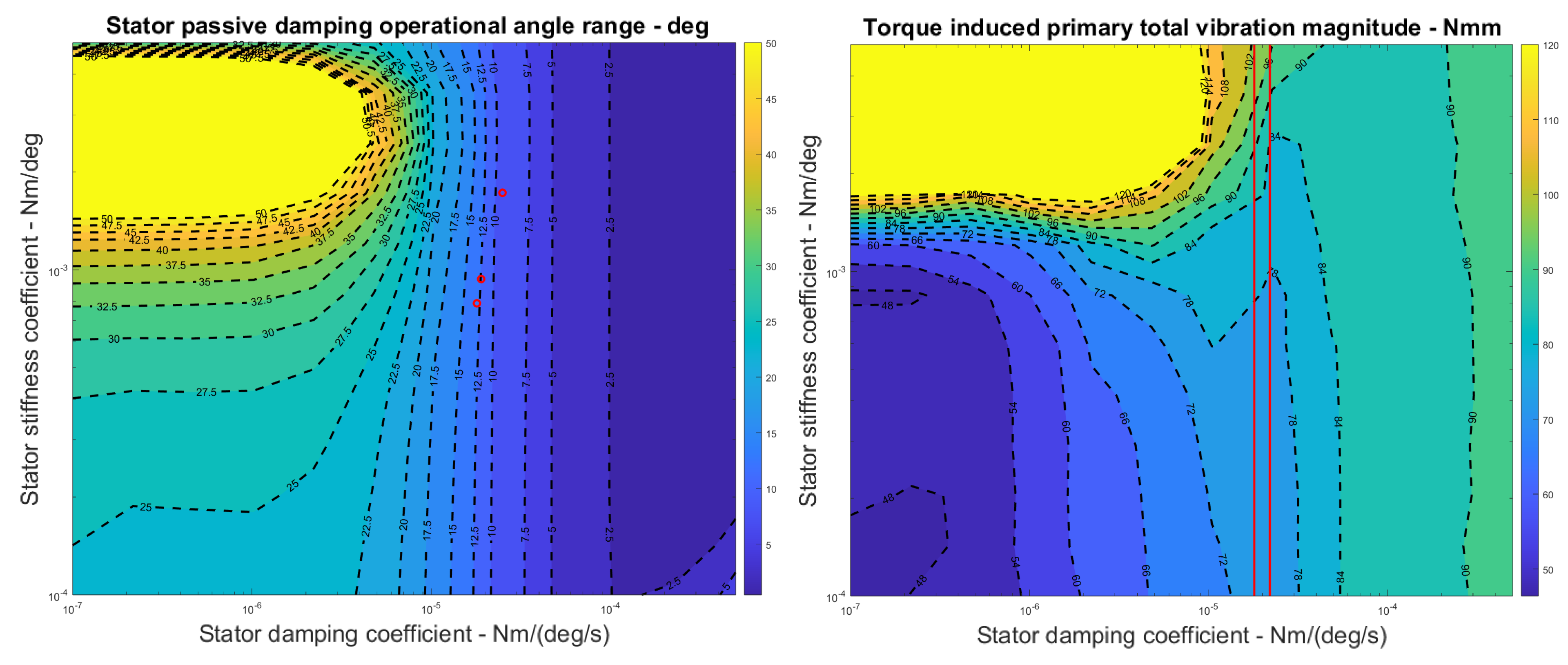
- The simulation in this study provides the results of the parameter study. The simulation shows that the most effective spring-damping structure that best fulfils the control-inherent vibration reduction purpose should have smooth damping and an appropriate spring coefficient magnitude to avoid resonance and make the structural design affordable, appropriate, and acceptable. The design region (range of coefficients) is characterised through simulations with all the parameters validated by experiments. This resultant design region is challenging to fully explore with only experiments or simplified linearized models, as simulations can provide numeric estimation based on finite element methods. Comparing the idealized single-sided spectrum output of the simulation in Figure 14 with Appendix A Figure A4, Figure A5, Figure A6 and Figure A7, it can be shown that simulation results successfully predicted the multiple frequency spectrum peaks instead of only the main peak.
- The simulation in this study shows that even with ideally continuous and clean input torque as simulated, without any internal or external background vibrations, the minimum control-coherent vibration magnitude is still inherent and inevitable, as shown in Figure 14. Because the shaft torque modulation is the source of both system control and the control-inherent vibration at the same time, any study focusing on vibration issues in torque-modulation-type underactuated swashplateless rotors and propellers should separately understand the impact of the control-inherent vibration and motor-propeller natural vibration.
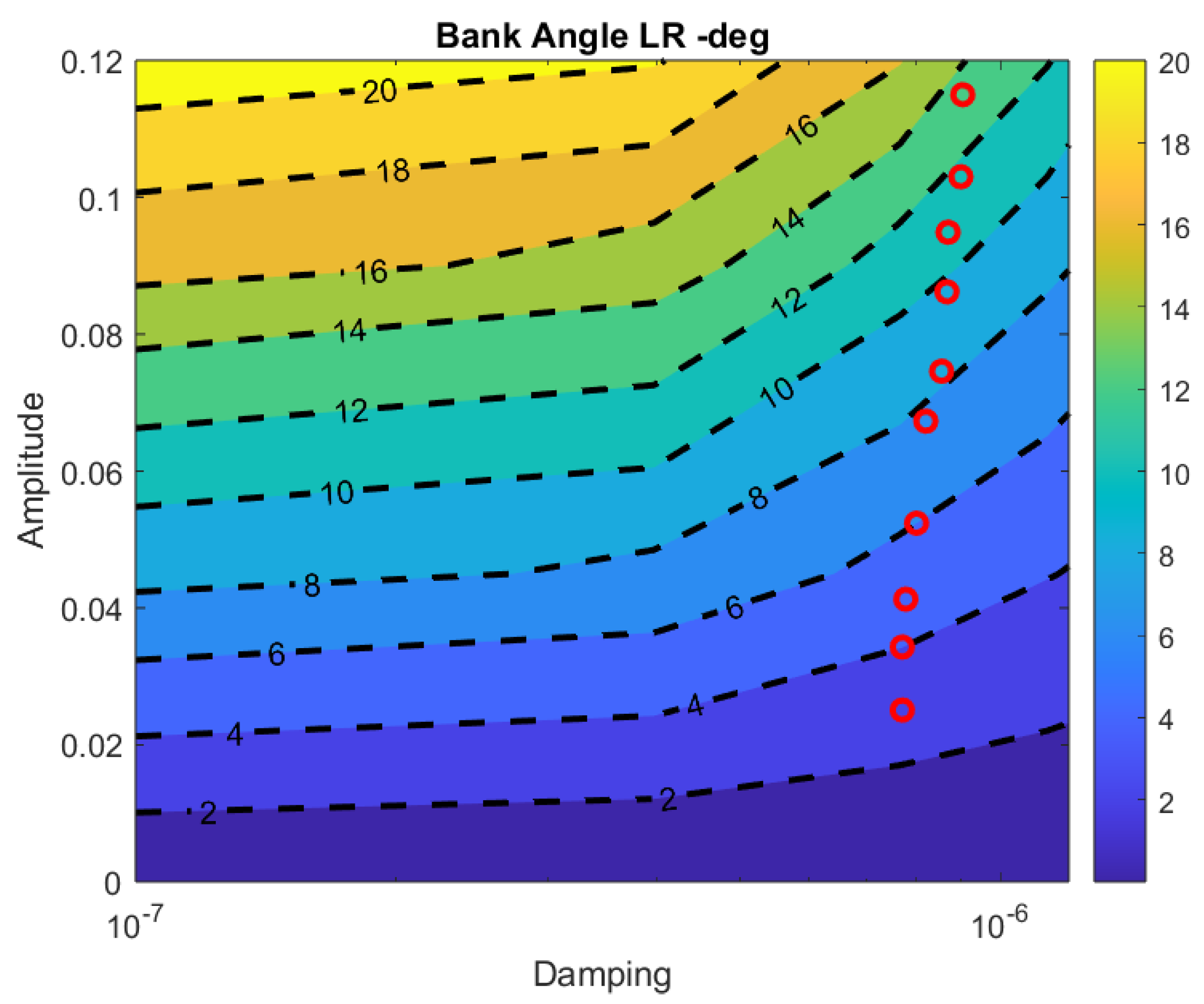
- One of the main contributions of the experiment in this study is in determining the friction-damping coefficient in the simulations. It is worth noting that the purpose of back-calculating (estimating) the friction-damping coefficient is to find the value that fits the physical model best at a design point. On one hand, this value might vary over time, requiring monitoring and trimming over different tests or physical model variations. On the other hand, this value is only of a limited reference significance instead of being an accurate, scientifically measured friction-damping coefficient. The complexity of friction-damping physics is a barrier in value estimation. Luckily, from the parameter study results, a conclusion can be made that under most design conditions, the optimal friction-damping coefficient is always “as low as possible”.
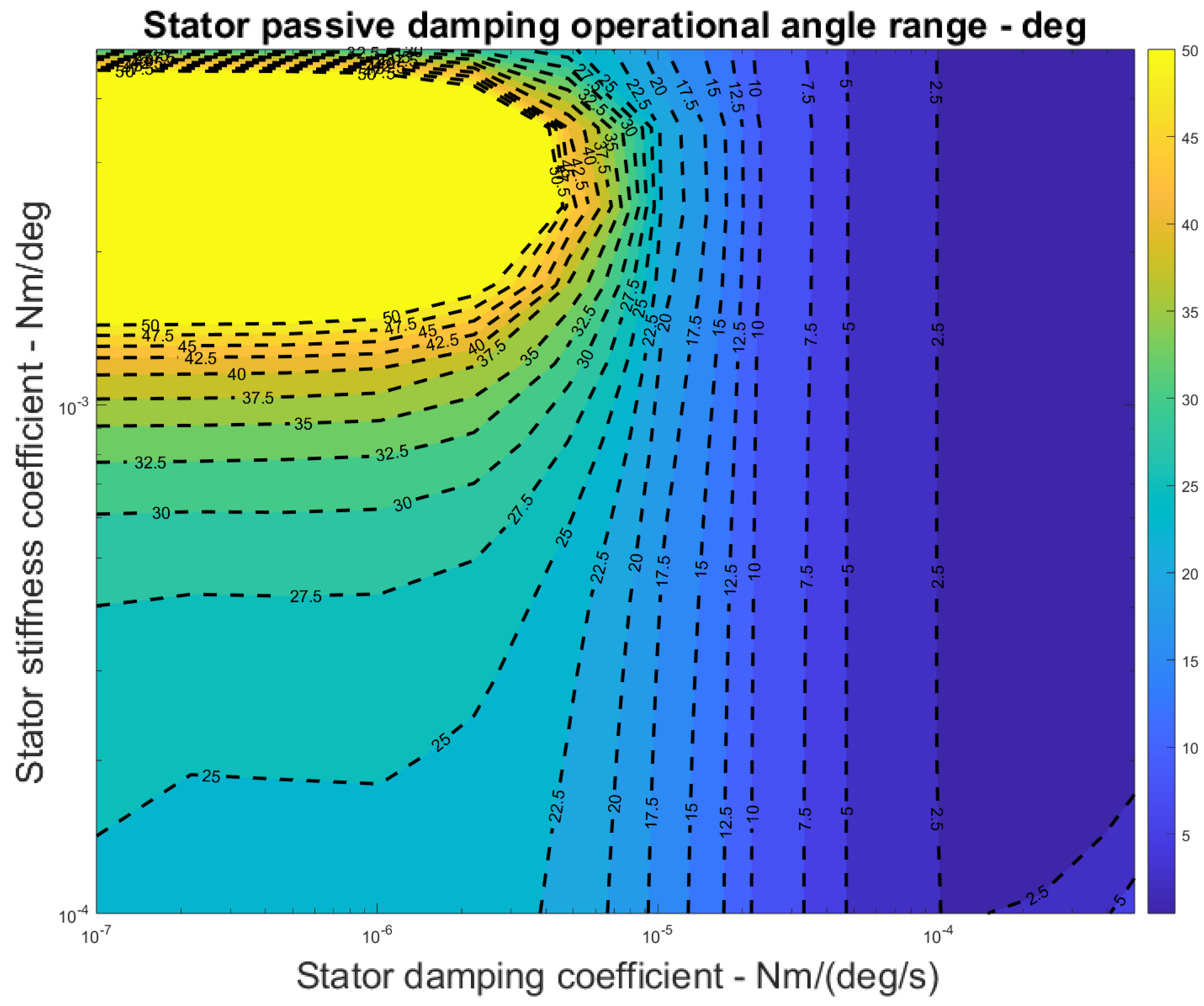
- Most importantly, a different case arises when changing the spring coefficient to avoid resonance is not feasible. In this case, the friction-damping coefficient must be high enough according to Figure 17 and Figure 18. Otherwise, the rotor may be subject to severe damage or even be at risk of destruction. In the same way, for an aircraft with a prescribed mission profile (maximum and minimum rotor frequency, etc.), the simulation must be thoroughly used to determine whether an operational status puts vehicle components under dangerous conditions.
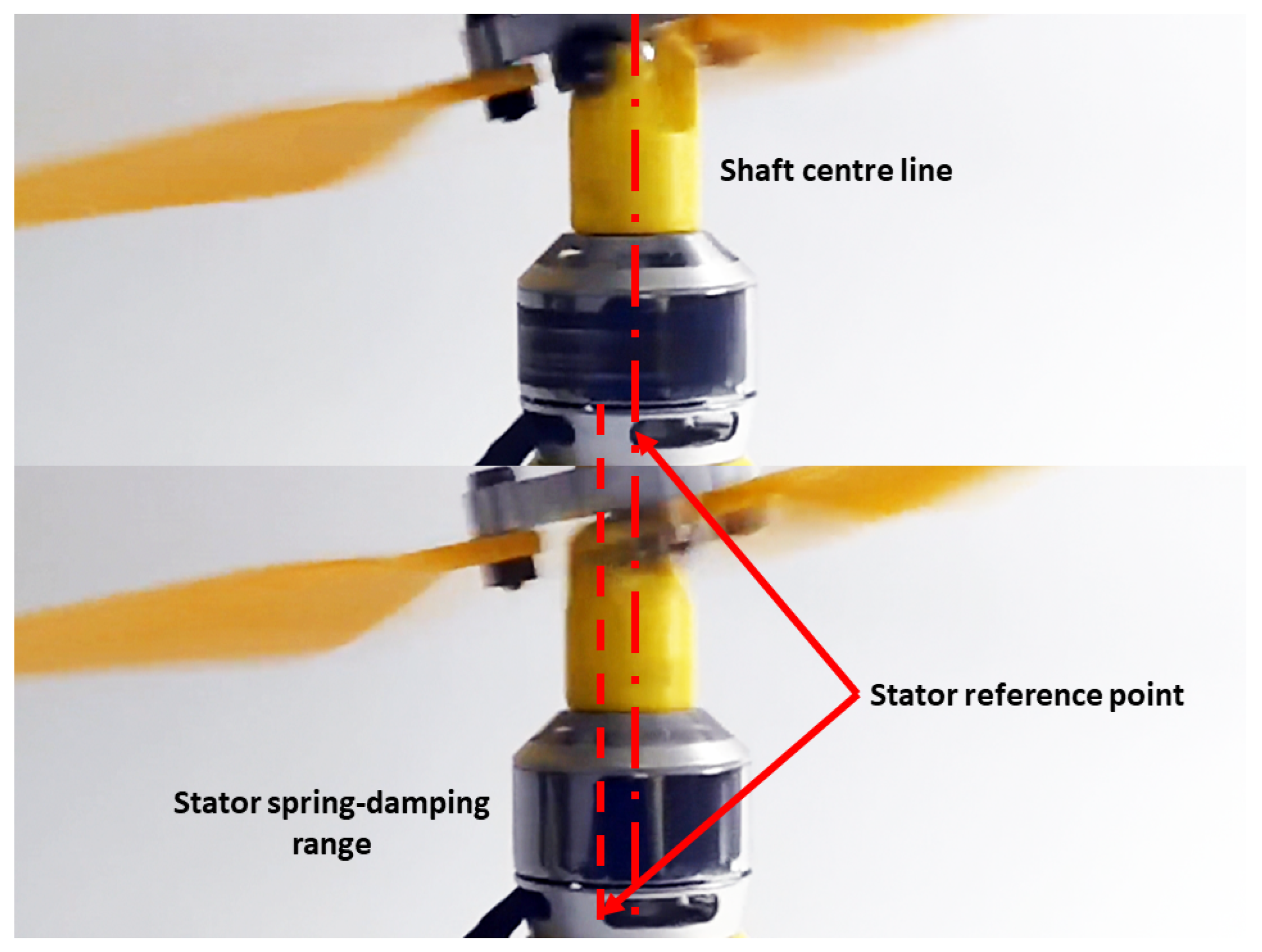
- The experiment in this study validated the hypothesis that adding a spring-damping structure in its torque modulation axis (central shaft axis) can absorb the stator’s excessive cyclic lead–lag motion before transferring the load into the platform’s main structure.
- The design parameter study shows that the control-induced vibration of the prototype can be reduced at hovering frequency. The prototype in this study achieved 10 decibels of peak power reduction comparing two identical free-body hover flight missions maintained at around 1 m high altitude. Thereby, although physical angular constraints still limit the current spring-damping structure design, the main structure-vibrating motion is already reduced to an acceptable level, taking the guide manual of the current Ardupilot firmware as a reference. This also indicated that further optimisation can be carried out if conditions permit.
- Given that the minimal proportion of spring-damping structures in this study is 3–5 g over 240.8 g in total weight, the weight proportion of spring-damping structures can be considered insignificant. However, if a larger passive stator damping angle is considered, the structural weight of this component might drastically increase depending on whether it is cleverly designed; thereby, the actual platform performance might be subject to changes.
6. Future Developments
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| UAV(s) | Unmanned aerial vehicle(s) |
| MAV(s) | Micro aerial vehicle(s) |
| eVToL | Electric vertical take-off and landing |
| GCS | Ground control station |
| MCU | Microcontroller unit |
| ESC | Electronic speed control |
| MAVLink | Micro Air Vehicle Link |
| PWM | Pulse-width modulation |
| SPI | Serial peripheral interface |
| BEM | Blade element method |
| BEMT | Blade element momentum theory |
| CW/CCW | Clock-wise/counter-clock-wise |
| FFT | Fast Fourier transform |
Appendix A

References
- Paulos, J.; Yim, M. An underactuated propeller for attitude control in Micro Air Vehicles. In Proceedings of the 2013 IEEE/RSJ International Conference on Intelligent Robots and Systems, Tokyo, Japan, 3–7 November 2013. [Google Scholar] [CrossRef]
- Paulos, J.; Yim, M. Flight performance of a swashplateless micro air vehicle. In Proceedings of the 2015 IEEE International Conference on Robotics and Automation (ICRA), Seattle, WA, USA, 26–30 May 2015. [Google Scholar] [CrossRef]
- Chen, N.; Kong, F.; Xu, W.; Cai, Y.; Li, H.; He, D.; Qin, Y.; Zhang, F. A self-rotating, single-actuated UAV with extended sensor field of view for Autonomous Navigation. Sci. Robot. 2023, 8, eade4538. [Google Scholar] [CrossRef] [PubMed]
- Paulos, J.J.; Yim, M. Scalability of Cyclic Control without Blade Pitch Actuators. In Proceedings of the 2018 AIAA Atmospheric Flight Mechanics Conference, Kissimmee, FL, USA, 7 January 2018. [Google Scholar] [CrossRef]
- Jin, Y.; Yong, L.; Weidong, Y. Dynamics Research on Actively Controlled Swashplateless Rotor. Proc. Inst. Mech. Eng. Part G J. Aerosp. Eng. 2019, 233, 4492–4508. [Google Scholar] [CrossRef]
- Bennett, G.S. Gyroscopic Effects in a Vibratory System. J. Appl. Phys. 1947, 12, 1110–1112. [Google Scholar] [CrossRef]
- Sandino, L.A.; Bejar, M.; Ollero, A. A Survey on Methods for Elaborated Modeling of the Mechanics of a Small-Size Helicopter. Analysis and Comparison. J. Intell. Robot. Syst. 2013, 72, 219–238. [Google Scholar] [CrossRef]
- Scanlan, R.H.; Truman, J.C. The Gyroscopic Effect of a Rigid Rotating Propeller on Engine and Wing Vibration Modes. J. Aeronaut. Sci. 1950, 17, 653–659. [Google Scholar] [CrossRef]
- Jin, H.; Yang, D.; Liu, Z.; Zang, X.; Li, G.; Zhu, Y. A Gyroscope-Based Inverted Pendulum with Application to Posture Stabilization of Bicycle Vehicle. In Proceedings of the 2015 IEEE International Conference on Robotics and Biomimetics (ROBIO), Zhuhai, China, 6–9 December 2015. [Google Scholar] [CrossRef]
- Paulos, J.; Yim, M. Cyclic blade pitch control without a swashplate for small helicopters. J. Guid. Control Dyn. 2018, 41, 689–700. [Google Scholar] [CrossRef]
- Misra, A.; Jayach ran, S.; Kenche, S.; Katoch, A.; Suresh, A.; Gundabattini, E.; Selvaraj, S.K.; Legesse, A.A. A Review on Vertical Take-off and Landing (VTOL) Tilt-Rotor and Tilt Wing Unmanned Aerial Vehicles (Uavs). J. Eng. 2022, 2022, 1803638. [Google Scholar] [CrossRef]
- Nugroho, H.A.; Cahyadi, A.I.; Ardiyanto, I. Attitude Control and low cost design of UAV bicopter. arXiv 2023, arXiv:2309.08209. [Google Scholar] [CrossRef]
- Yu, Z.; Zhang, J.; Wang, X. Study of Modeling and Optimal Take-off Scheme for a Novel Tilt-Rotor UAV. Sensors 2022, 22, 9736. [Google Scholar] [CrossRef] [PubMed]
- Poyi, G.T.; Wiggins, B.; Wu, M.H.; Bousbaine, A. Validation of a quad-rotor helicopter MATLAB/Simulink and Solidworks models. In Proceedings of the IET Conference on Control and Automation 2013: Uniting Problems and Solutions, Birmingham, UK, 4–5 June 2013. [Google Scholar] [CrossRef]
- Akour, S.N.; Mohammed, A.; Talha, A.; Kamel, A.K. Experimental and Theoretical Investigation of Micro Wind Turbine for Low Wind Speed Regions. Renew. Energy 2018, 116, 215–223. [Google Scholar] [CrossRef]
- Mahmuddin, F. Rotor Blade Performance Analysis with Blade Element Momentum Theory. Energy Procedia 2017, 105, 1123–1129. [Google Scholar] [CrossRef]
- Bell, S. Analysis of a Rotor Blade System Using Blade Element Momentum Theory. MATLAB Central File Exchange. 2020. Available online: https://www.mathworks.com/matlabcentral/fileexchange/21994-analysis-of-a-rotor-blade-system-using-blade-element-momentum-theory (accessed on 5 January 2020).
- El khchine, Y.; Sriti, M. Tip Loss Factor Effects on Aerodynamic Performances of Horizontal Axis Wind Turbine. Energy Procedia 2017, 118, 136–140. [Google Scholar] [CrossRef]
- Ardupilot.org Measuring Vibration—Copter Documentation. Available online: https://ardupilot.org/copter/docs/common-measuring-vibration.html (accessed on 25 March 2024).
- Ardupilot.org Measuring Vibration with IMU Batch Sampler—Copter Documentation. Available online: https://ardupilot.org/copter/docs/common-imu-batchsampling.html (accessed on 25 March 2024).
- Gjelstrup, S.L. Guide to FFT Analysis (Fast Fourier Transform). Data Acquisition|Test and Measurement Solutions. 10 May 2023. Available online: https://dewesoft.com/blog/guide-to-fft-analysis (accessed on 25 March 2024).
- Thomas, K.; Rohnen, A. Chapter 5: Vibration Transducer. In Practice of Vibration Measurement: Measurement Technology and Vibration Analysis with MATLAB, 2nd ed.; Springer Fachmedien Wiesbaden GmbH: Wiesbaden, Germany, 2023; pp. 77–80. [Google Scholar]
- Ahmed, H.E.; Sahandabadi, S.; Bhawya; Ahamed, M.J. Application of MEMS Accelerometers in Dynamic Vibration Monitoring of a Vehicle. Micromachines 2023, 14, 923. [Google Scholar] [CrossRef] [PubMed]


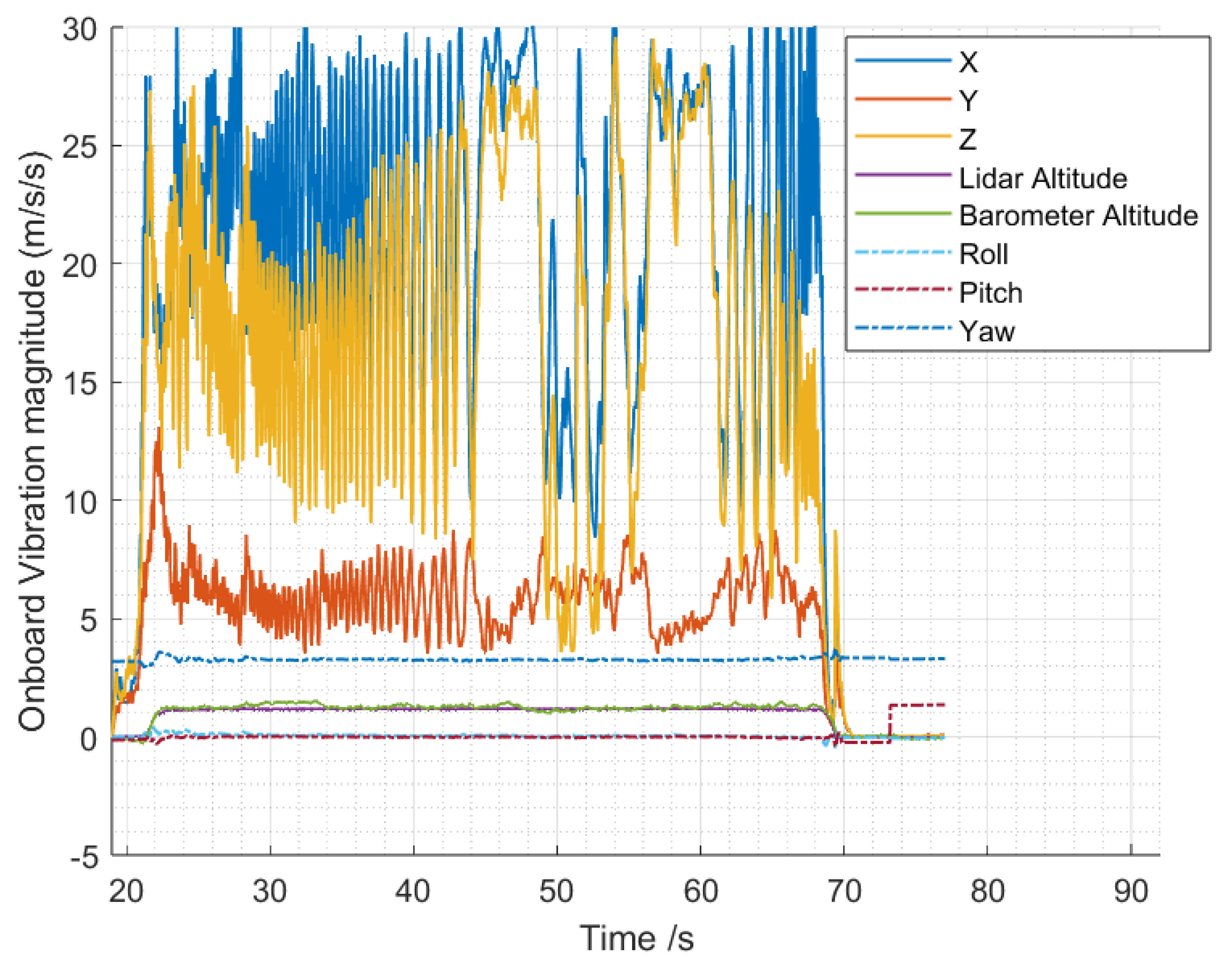
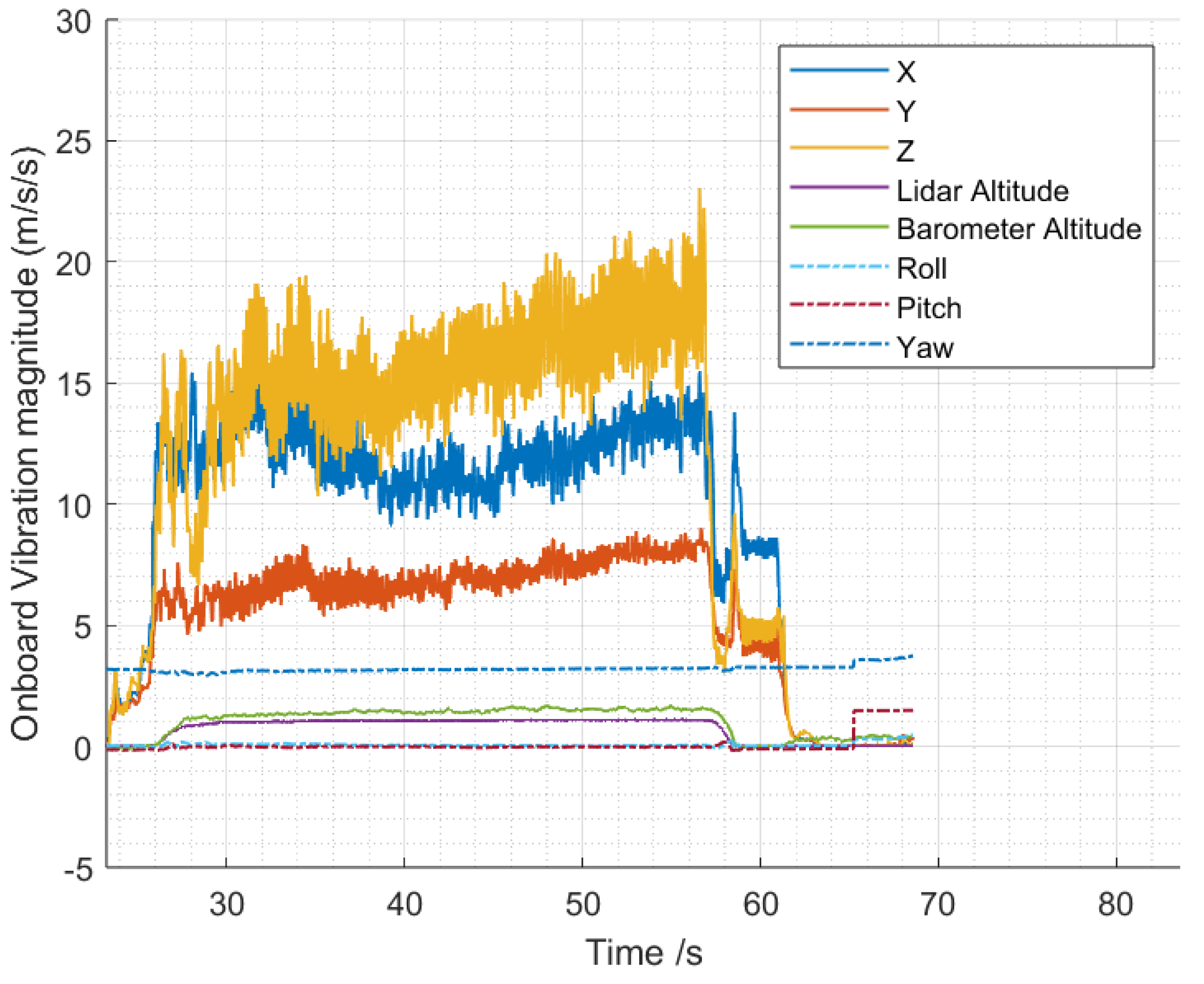
| Variables | Set 1 | Set 2 | Set 3 | Set 4 | Unit |
|---|---|---|---|---|---|
| Stiffness | Tightened | ||||
| Damping | Tightened | ||||
| Mean IMU acceleration magnitudes |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Guan, H.; Wong, K.C. Spring-Damped Underactuated Swashplateless Rotor on a Bicopter Unmanned Aerial Vehicle. Machines 2024, 12, 296. https://doi.org/10.3390/machines12050296
Guan H, Wong KC. Spring-Damped Underactuated Swashplateless Rotor on a Bicopter Unmanned Aerial Vehicle. Machines. 2024; 12(5):296. https://doi.org/10.3390/machines12050296
Chicago/Turabian StyleGuan, Haofei, and K. C. Wong. 2024. "Spring-Damped Underactuated Swashplateless Rotor on a Bicopter Unmanned Aerial Vehicle" Machines 12, no. 5: 296. https://doi.org/10.3390/machines12050296
APA StyleGuan, H., & Wong, K. C. (2024). Spring-Damped Underactuated Swashplateless Rotor on a Bicopter Unmanned Aerial Vehicle. Machines, 12(5), 296. https://doi.org/10.3390/machines12050296






