Scissor Arm for Cambered Snow: Mechanical Theory
Abstract
1. Introduction
2. Materials and Methods
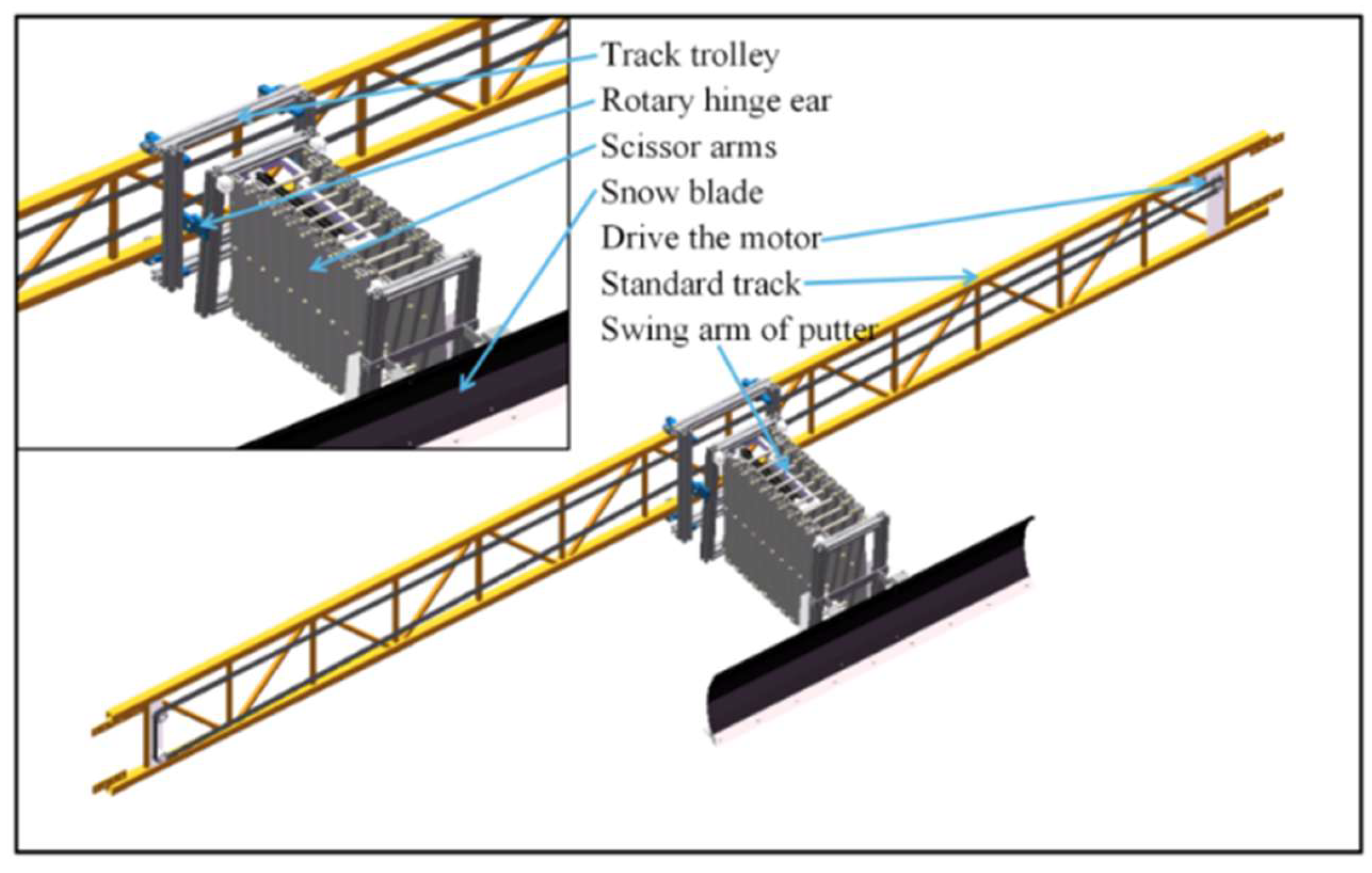
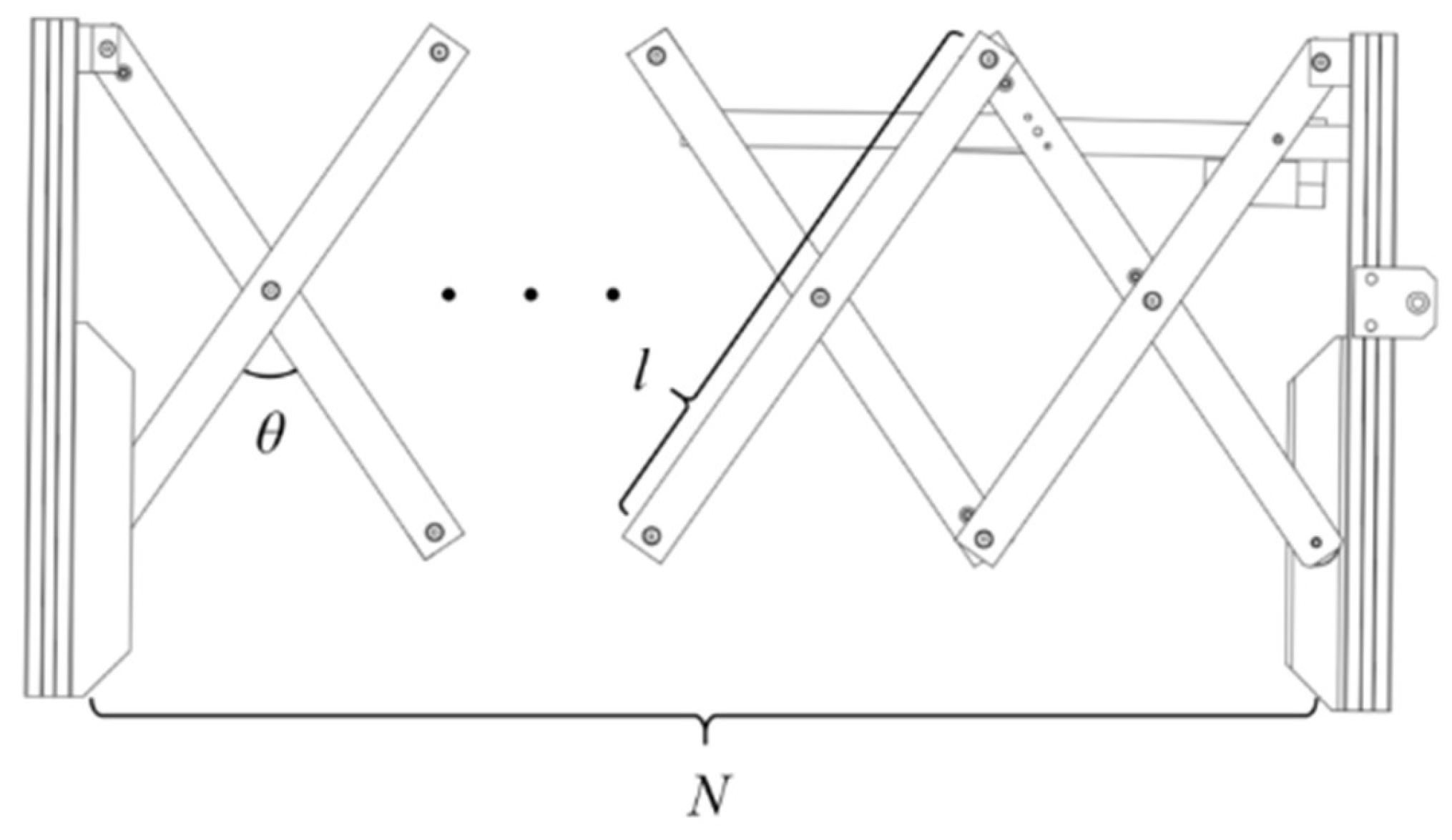
2.1. Model and Working Principle
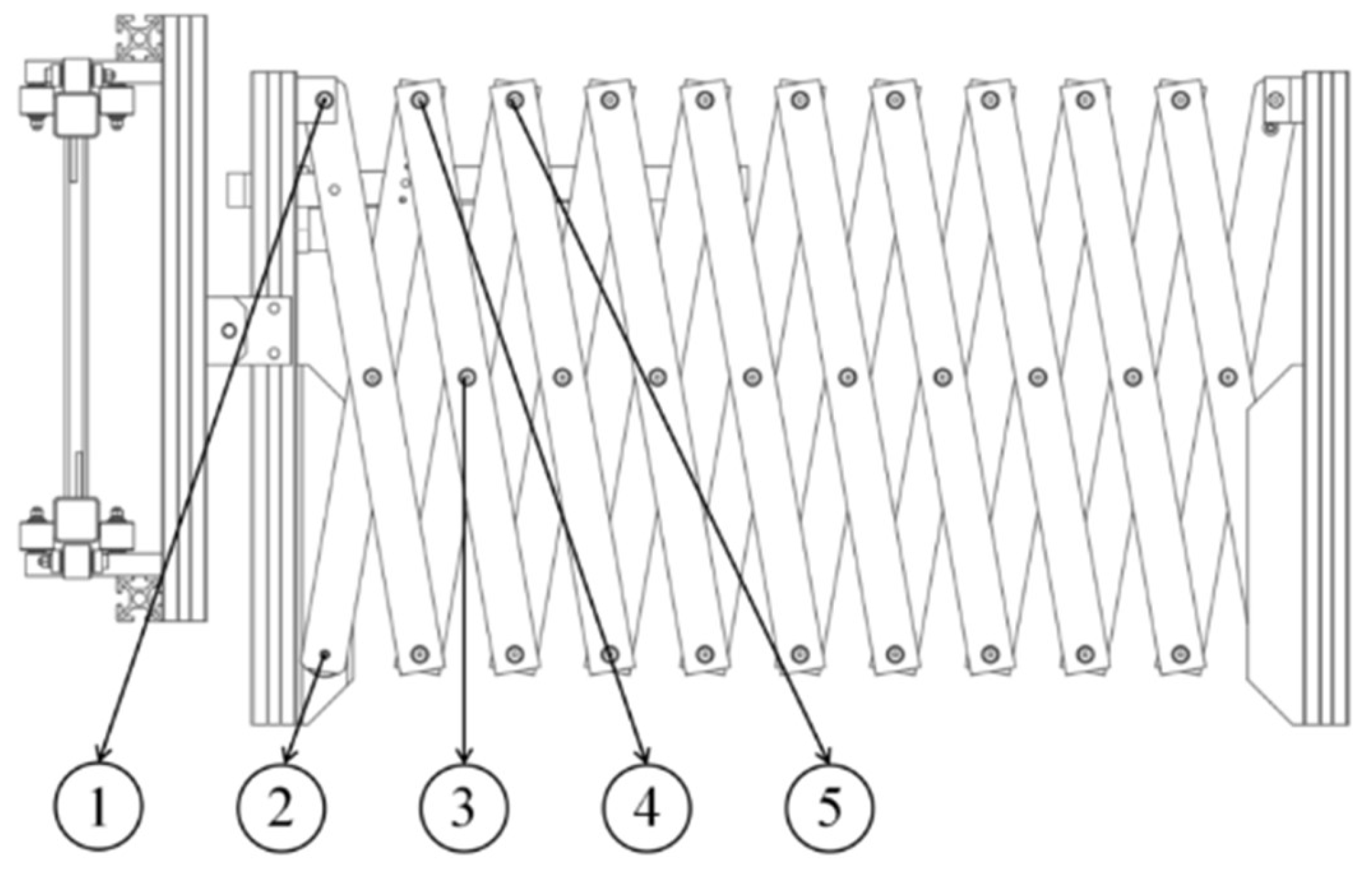
2.2. Transmission Mechanism
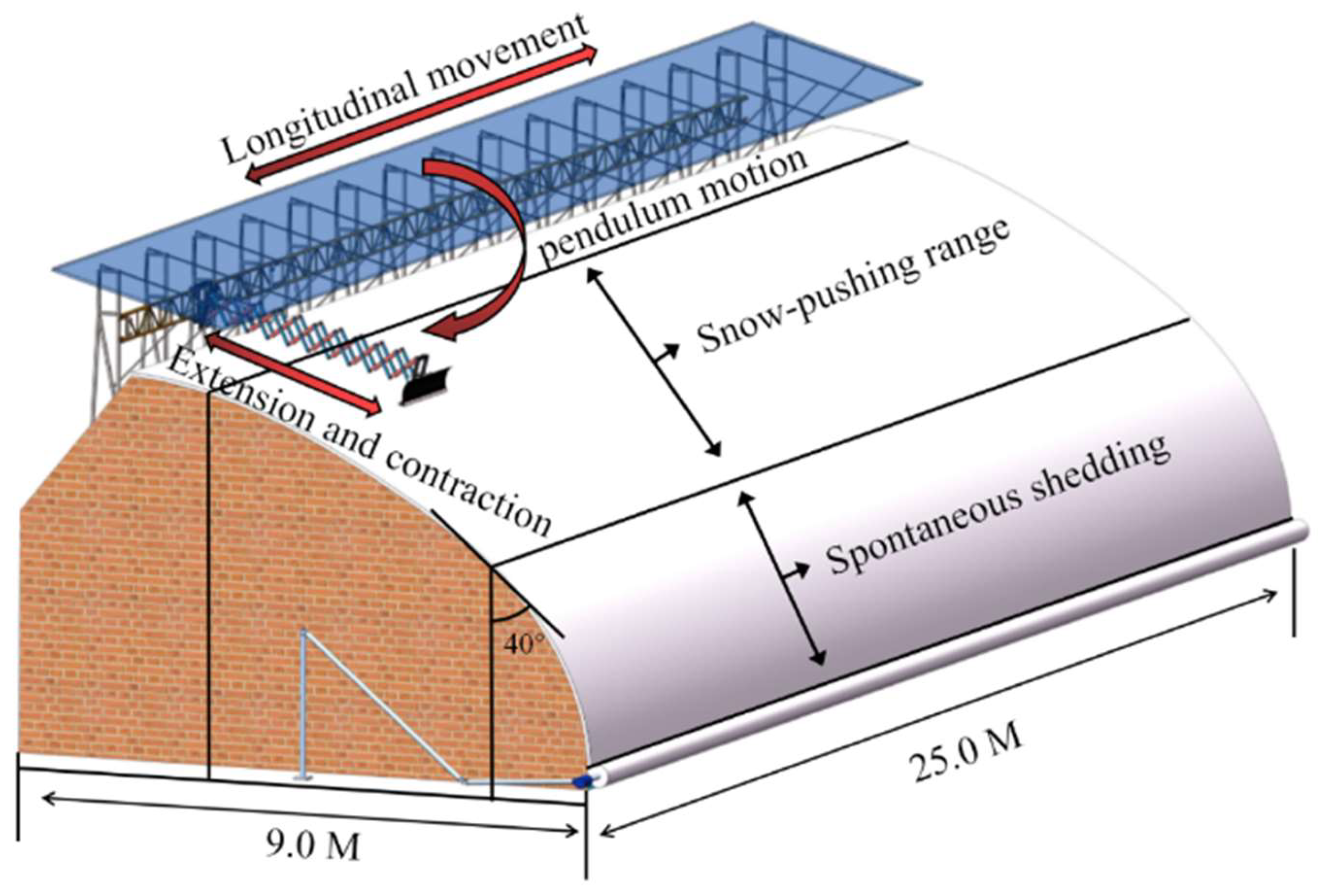
2.3. Range of Snow Accumulation in Solar Greenhouses
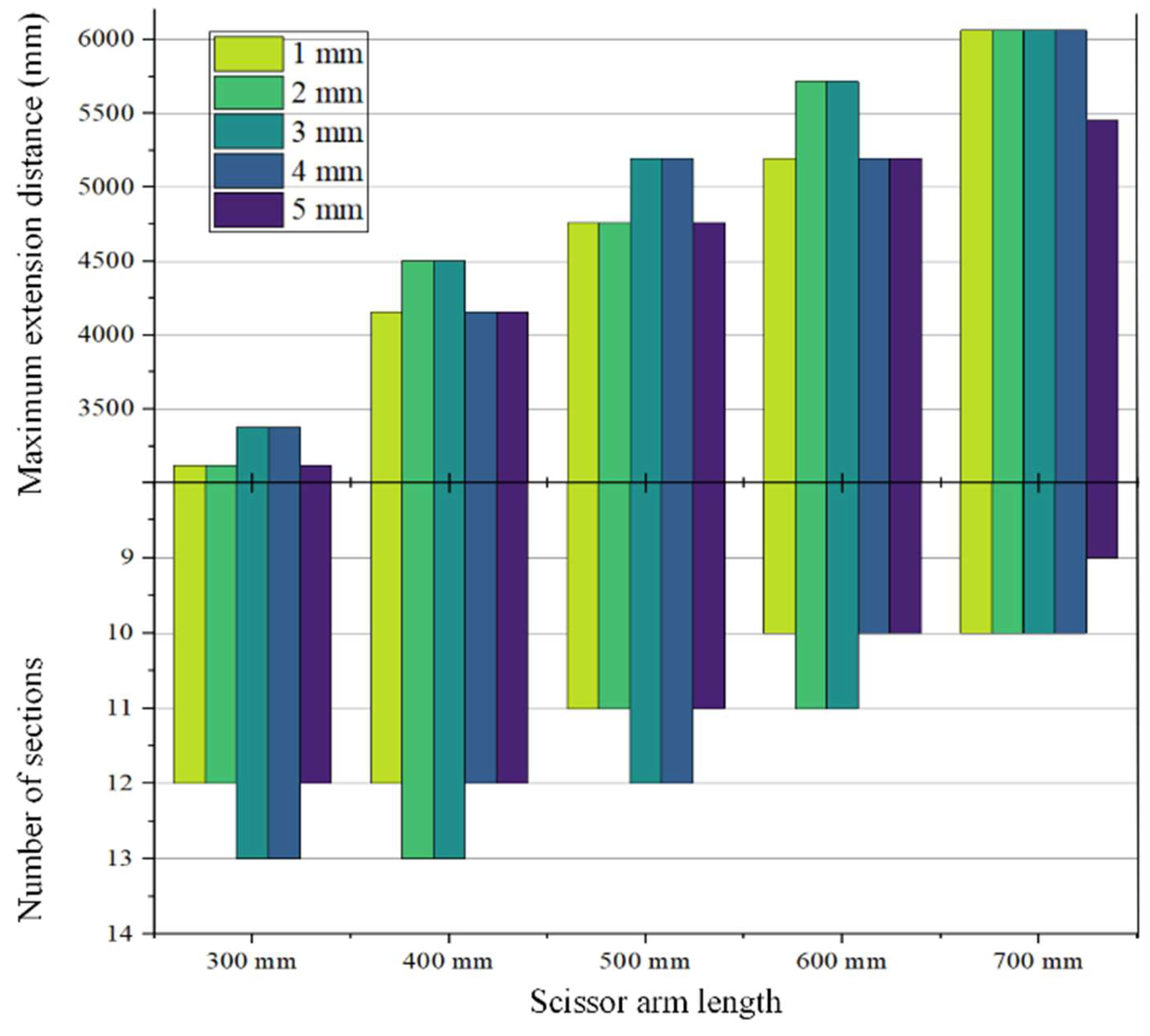
2.4. Relation between Section Number and Span
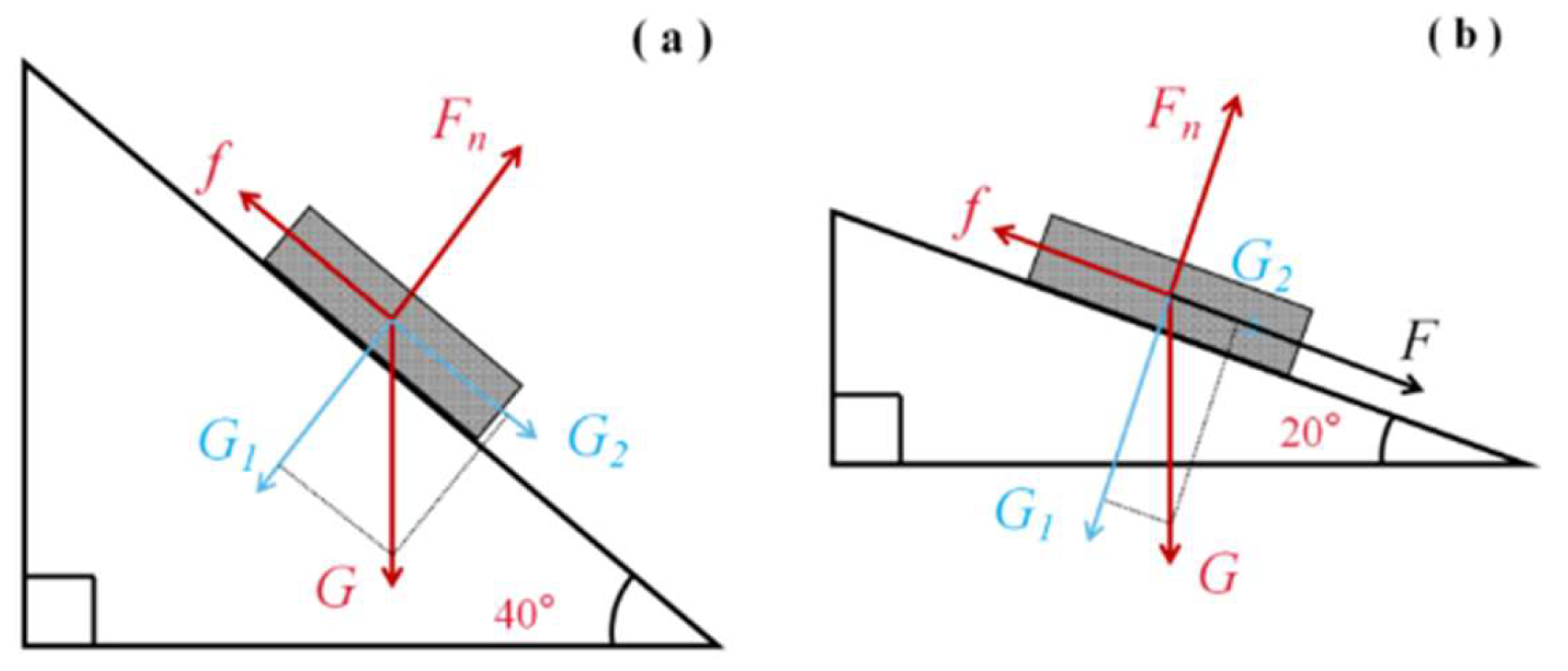
2.5. Greenhouse South Roof Snow Force Analysis
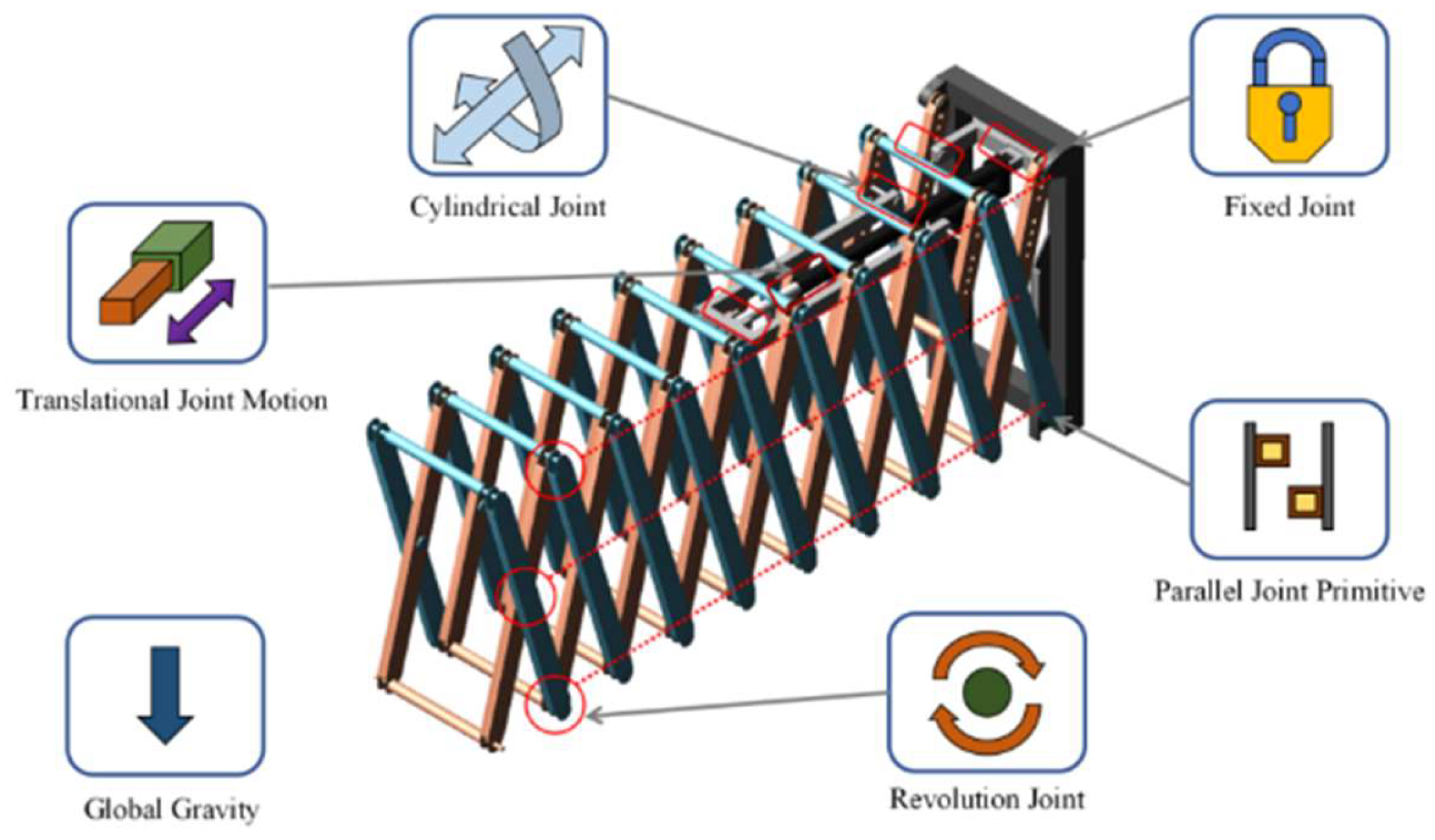
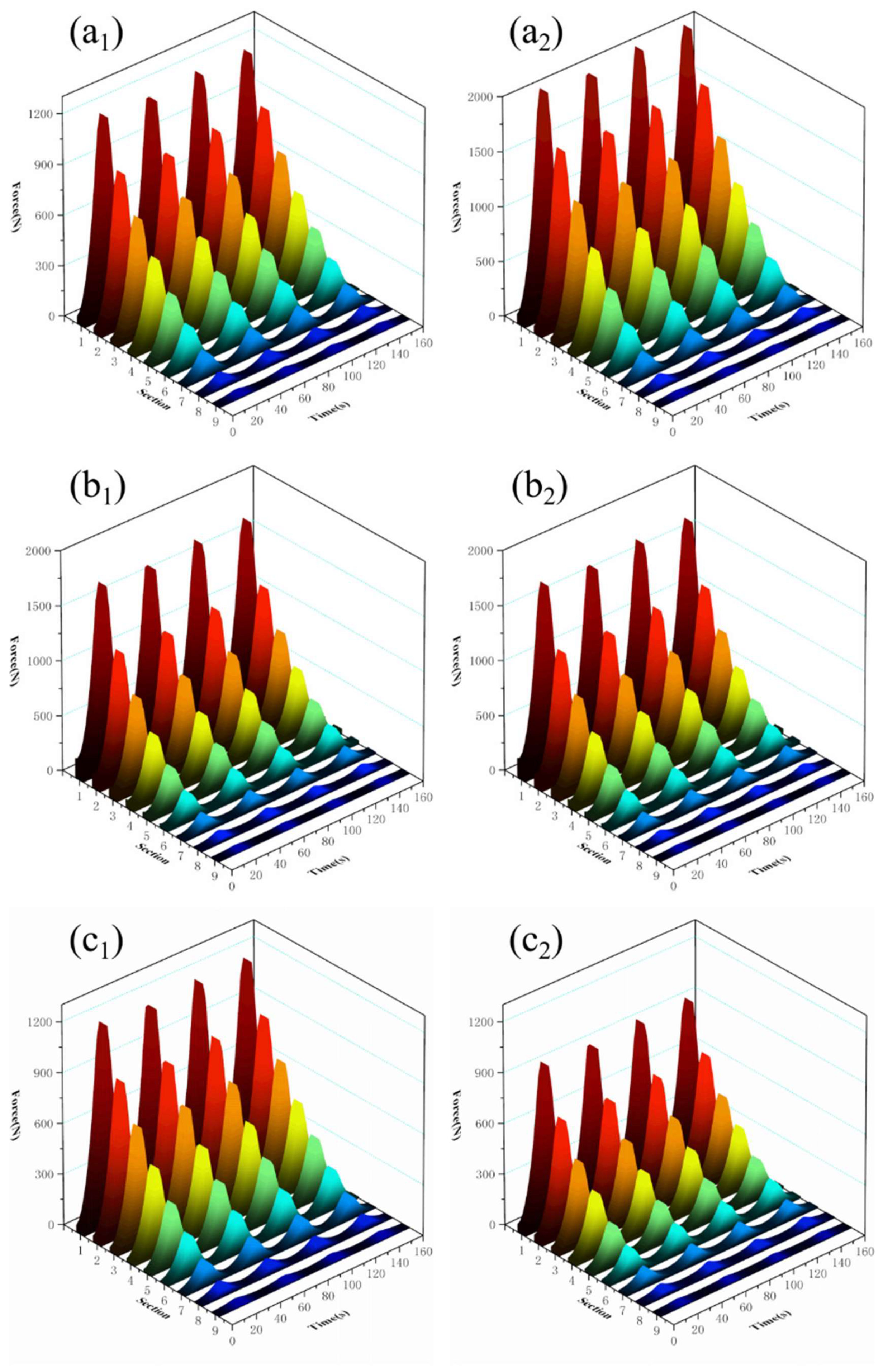
2.6. Dynamics Simulation
3. Results and Discussion
3.1. Relationship between Span, Snow Accumulation, and Stress
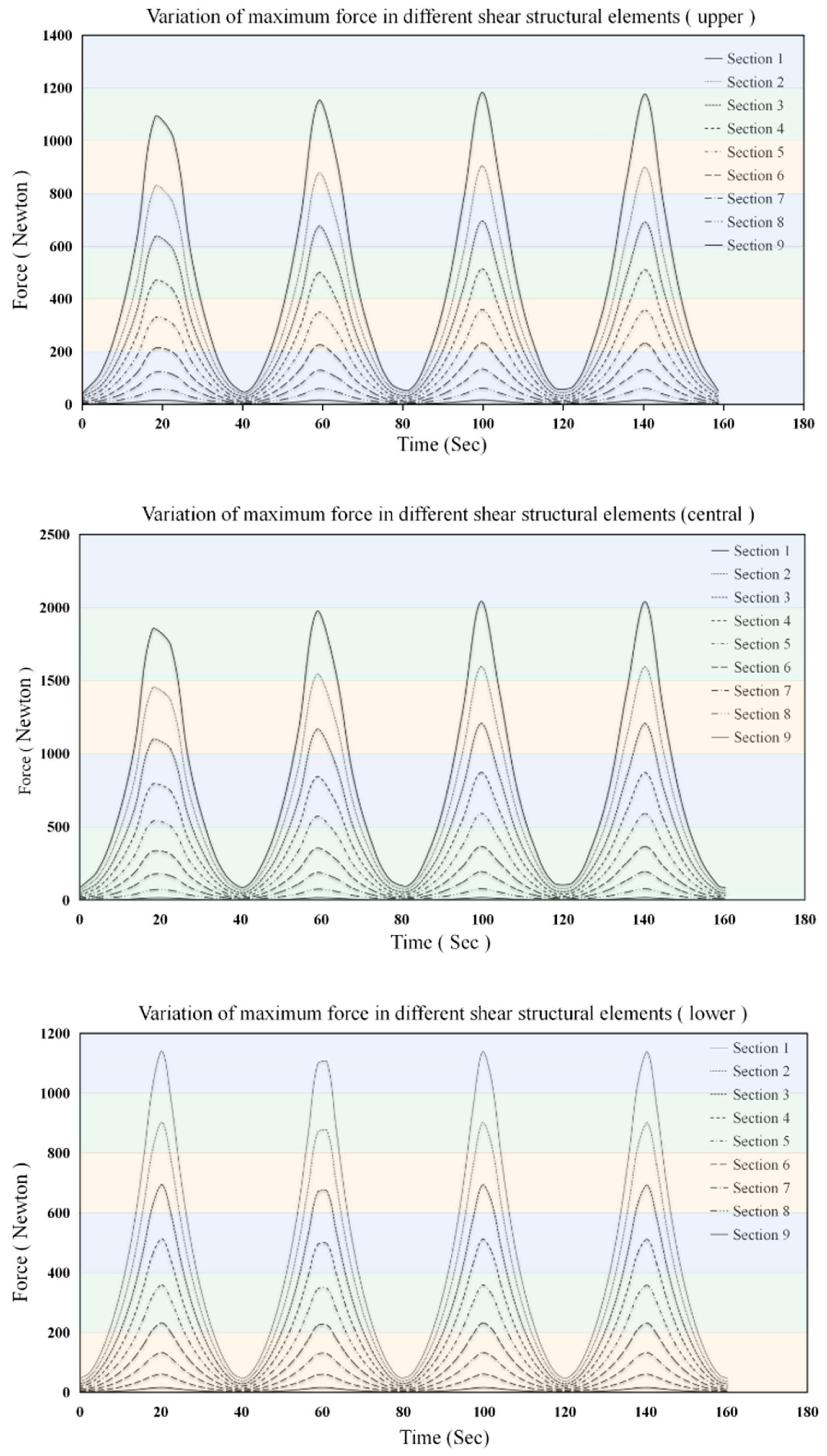
3.2. Dynamics Analysis
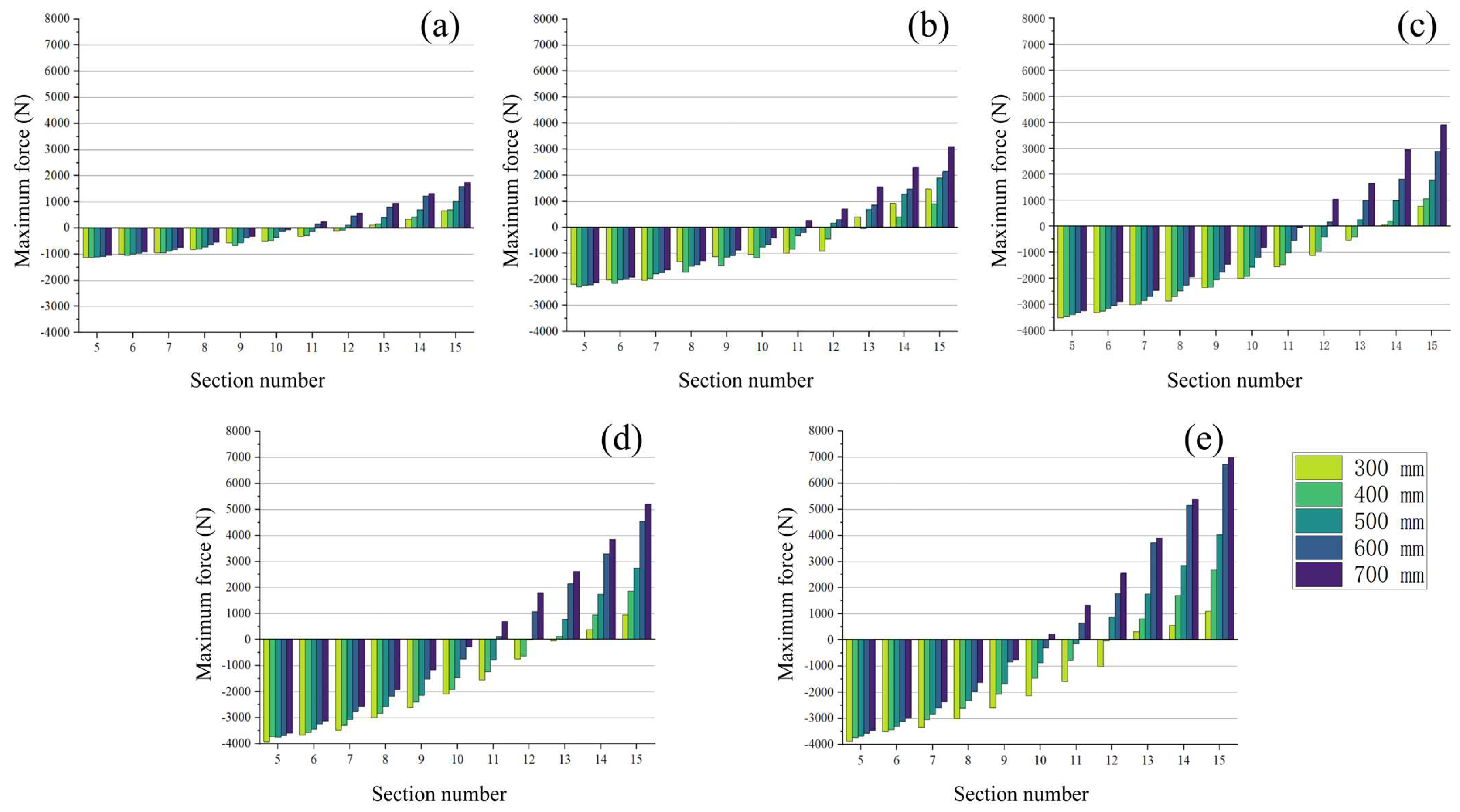
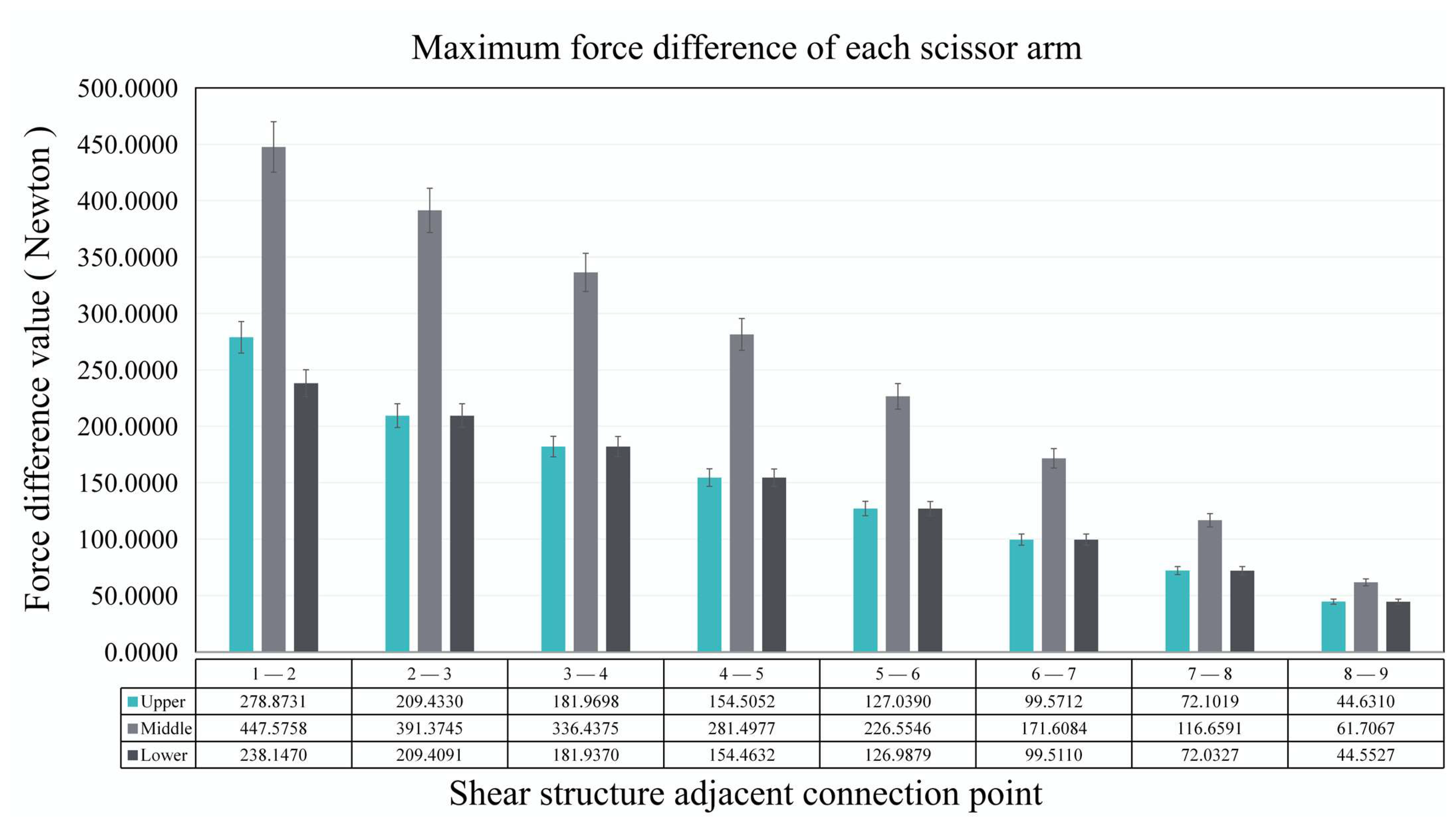
3.3. Scissor Arm Thickness Optimisation
4. Conclusions
- (1)
- The designed SRD can be applied to a large curved area, such as a solar greenhouse; it satisfies the snow removal requirements of a greenhouse, reduces labour intensity, and realises the automatic snow removal function of a greenhouse using telescopic scissor arms, rotating hinge lugs, and transitional rail cars.
- (2)
- Simulation results of scissor arm multi-body dynamics show that increasing the arm length can significantly increase the applied range of the scissor arm but reduce the number of applied knots. Increasing the wall thickness can increase the applied length of the scissor arm. When the thickness increases to a level greater than the strength of the pin roll, the maximum applied length is reduced due to gravity.
- (3)
- The short scissor arms with long knots are used in round-arched solar greenhouses, and the applicable length of scissor arms for greenhouses with a span of 8.0–12.0 m is 300–400 mm. Traditional solar greenhouses utilise long scissor arms with more sections, and the applicable length of scissor arms for greenhouses with a span of 6.5–10.0 m is 500–700 mm.
- (4)
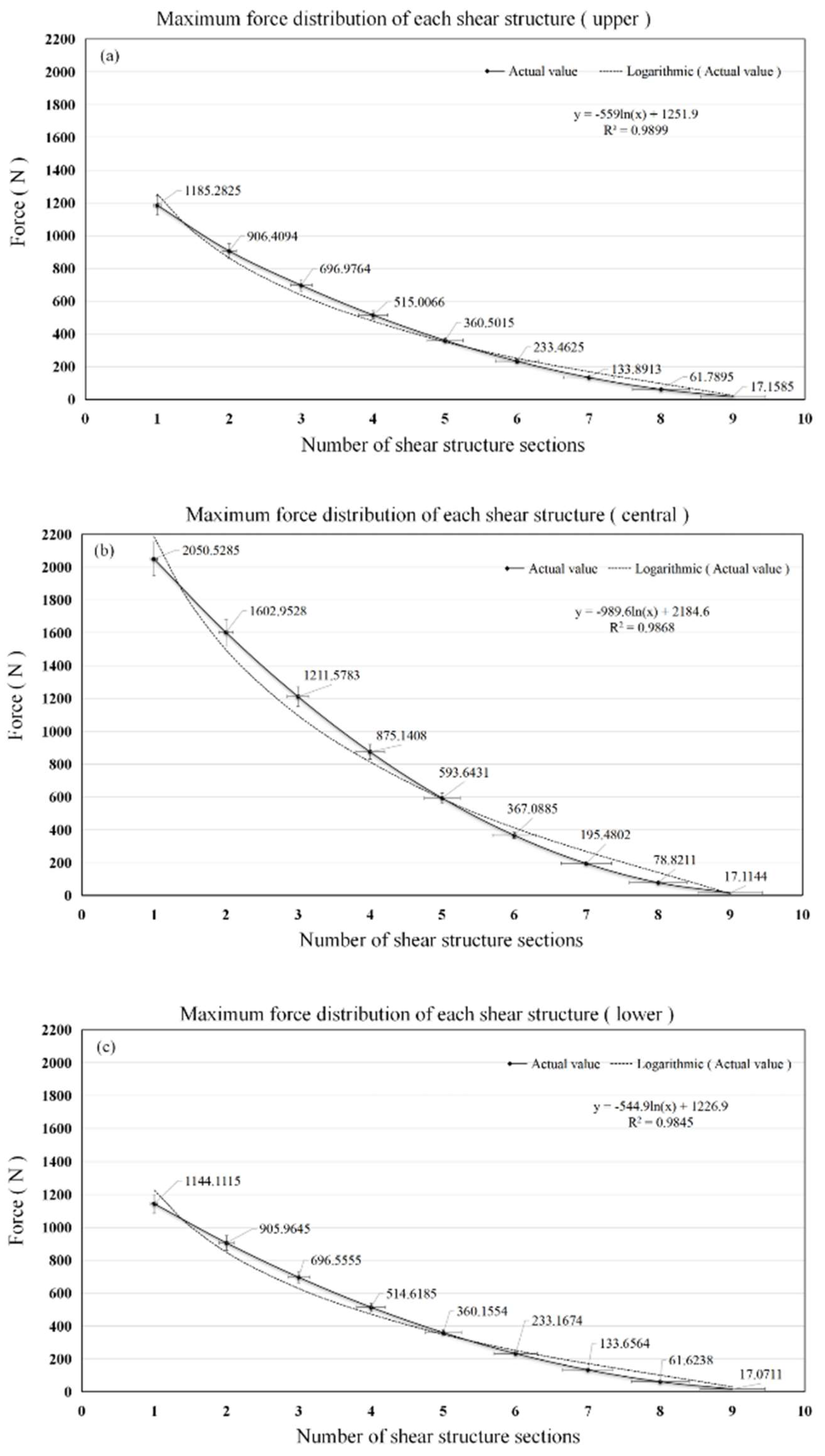
- The wall thickness of the scissor arm is optimised based on the shear force in each section of the scissor arm. Before optimisation, the maximum shear force at the upper, middle, and lower connection points of the scissor arm are 1173.53, 2027.67, and 1131.25 N. After optimisation, the shear force decreases to 953.46, 1673.81, and 937.25 N, with a reduction of 18.75%, 17.45%, and 17.15%, respectively.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
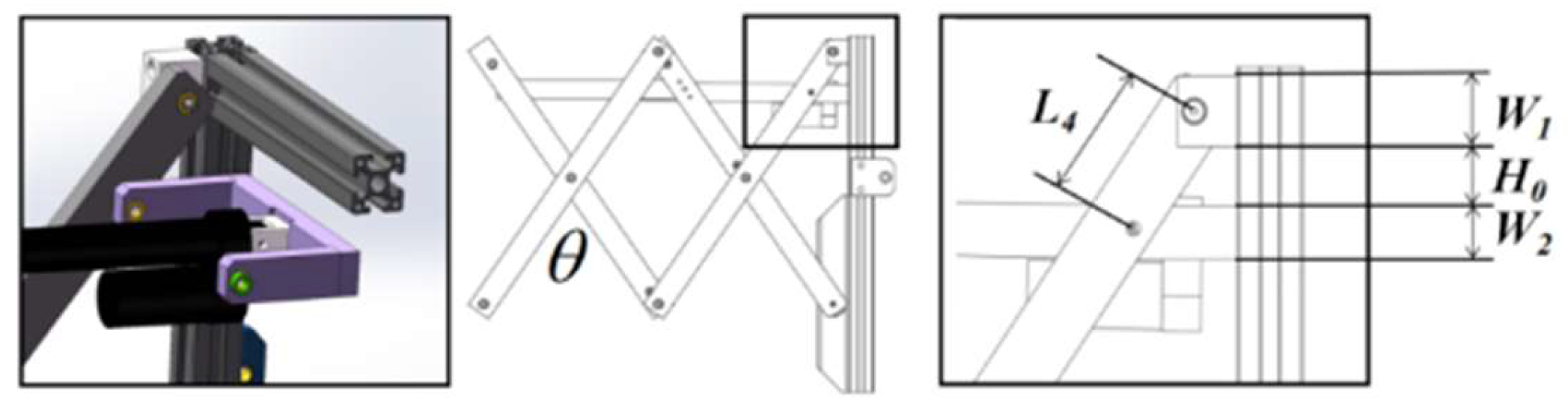
Nomenclature
| Symbols | |||
| L4 | Distance between the connection points (cm) | θ | Stretch angle (°) |
| W1 | Width of the articulated assembly (cm) | σ | Yield strength (N·mm−2) |
| W2 | Width of the pusher lower swing arm (cm) | μ | Coefficient of friction |
| H0 | Distance between the articulated body from the lower arm (cm) | Abs1 | Scissor arm shaft force area (mm2) |
| L | Snow removal range (m) | Abs2 | Shaft cross-sectional area (mm2) |
| l | Scissor arm length (mm) | ρ | Density (kg·m3) |
| α | Extension angle (°) | β | Tilt angle (°) |
| N | Number of sections | d | Shaft diameter (mm) |
| a | Width of snow removal (m) | h | Thickness of scissor arm (mm) |
| b | Scissor arm width (mm) | g | Gravitational acceleration (m/s2) |
| c | Length (mm) | m | Quantity (kg) |
| l2 | Hinge length (mm) | ||
References
- Gao, J. Analysis and assessment of the risk of snow and freezing disaster in China. Int. J. Disaster Risk Reduct. 2016, 19, 334–340. [Google Scholar] [CrossRef]
- Yang, Z.-Q.; Zhang, T.-H.; Huang, H.-J.; Zhu, K.; Zhang, B. Meteorological Disaster Risk Evaluation of Solar Greenhouse in North China. Chin. J. Agrometeorol. 2013, 34, 342–349. [Google Scholar] [CrossRef]
- Han, X.J.; Shen, Y.; Sun, X.; Li, S.; Yu, H.; Wang, M.; Xu, C.; Li, G. The snow disaster zoning and the snowfall impact pre-assessment in Liaoning Province. J. Glaciol. Geocryol. 2016, 38, 21–27. [Google Scholar]
- Wang, C.; Jiang, Y.; Bai, Y.; Wang, T. Numerical study on static properties and failure mechanisms of landing assembled Chinese solar greenhouses. Comput. Electron. Agric. 2021, 188, 106347. [Google Scholar] [CrossRef]
- Yang, S.; Liu, X.; Jiang, X. Countermeasures against extreme wind and snow disasters to solar greenhouses based on temporary reinforcement. J. Jiangsu Univ. Nat. Sci. Ed. 2022, 43, 45–53. [Google Scholar] [CrossRef]
- Xia, T.; Li, Y.; Wu, X.; Fan, Z.; Shi, W.; Liu, X.; Li, T. Performance of a new active solar heat storage–release system for Chinese assembled solar greenhouses used in high latitudes and cold regions. Energy Rep. 2022, 8, 784–797. [Google Scholar] [CrossRef]
- Ren, J.; Wang, J.; Guo, S.; Li, X.; Zheng, K.; Zhao, Z. Finite element analysis of the static properties and stability of a large-span plastic greenhouse. Comput. Electron. Agric. 2019, 165, 104957. [Google Scholar] [CrossRef]
- Liu, X.; Li, Z.; Zhang, L.; Liu, Y.; Li, Y.; Li, T. Effect of single tube sections on the structural safety of Chinese solar greenhouse skeletons. Sci. Rep. 2021, 11, 19307. [Google Scholar] [CrossRef] [PubMed]
- Niu, H.; Zheng, S.; Zhao, Z.; Zhang, J. Design of a new type of snow remover for the color steel tile roof. Hebei J. Ind. Sci. Technol. 2022, 39, 218–223. [Google Scholar] [CrossRef]
- Shen, J.; Gao, B.; Zhang, Y.; Kang, J.; He, L.; Liu, X. Mechanical Structure Design of Multifunctional Snow Remover. Appl. Technol. 2021, 330, 99–103. [Google Scholar] [CrossRef]
- Zhou, X.; Zhang, T.; Liu, Z.; Gu, M. A study of snow drifting on monoslope roofs during snowfall: Wind tunnel test and numerical simulation. Cold Reg. Sci. Technol. 2023, 206, 103731. [Google Scholar] [CrossRef]
- Yan, C.; Niu, C.; Ma, S.; Tan, H.; Xu, L. CFD models as a tool to analyze the deformation behavior of grape leaves under an air-assisted sprayer. Comput. Electron. Agric. 2022, 198, 107112. [Google Scholar] [CrossRef]
- Yan, C.; Qu, M.; Chen, Y.; Feng, M. Snow removal method for self-heating of photovoltaic panels and its feasibility study. Sol. Energy 2020, 206, 374–380. [Google Scholar] [CrossRef]
- Huo, Y.; Gang, S.; Guan, C. FCIHMRT: Feature Cross-Layer Interaction Hybrid Method Based on Res2Net and Transformer for Remote Sensing Scene Classification. Electronics 2023, 12, 4362. [Google Scholar] [CrossRef]
- Lee, S.-I.; Jeong, Y.-J.; Lee, J.-H.; Chung, G.; Choi, W. Development of a heavy snowfall alarm model using a Markov chain for disaster prevention to greenhouses. Biosyst. Eng. 2020, 200, 353–365. [Google Scholar] [CrossRef]
- Chen, Y.; Xu, Z.; Wang, Q.; Mou, Q.; Fang, Y.; Shu, P. Research on Design of New Canopy Snow Removal Machine. J. Qingdao Univ. Eng. Technol. Ed. 2012, 27, 92–96. [Google Scholar] [CrossRef]
- Hao, Z.; Liu, W. Research on the overall design of new greenhouse snow blower. J. Northeast Agric. Univ. 2012, 43, 156–160. [Google Scholar]
- Yu, B.; Yang, J.; Du, R.; Zhong, Y. A Versatile Pneumatic Actuator Based on Scissor Mechanisms: Design, Modeling, and Experiments. IEEE Robot. Autom. Lett. 2021, 6, 1288–1295. [Google Scholar] [CrossRef]
- Sun, Y.; Pancheri, F.; Lueth, T.C. Kinematic Modeling of Scissor-Mechanism-Based Curvilinear Actuator. In Proceedings of the IECON 2022—48th Annual Conference of the IEEE Industrial Electronics Society, Brussels, Belgium, 17–20 October 2022; pp. 1–6. [Google Scholar] [CrossRef]
- Li, G.; Huang, H.; Guo, H.; Li, B. Design, analysis and control of a novel deployable grasping manipulator. Mech. Mach. Theory 2019, 138, 182–204. [Google Scholar] [CrossRef]
- Dinevari, N.F.; Shahbazi, Y.; Maden, F. Geometric and analytical design of angulated scissor structures. Mech. Mach. Theory 2021, 164, 104402. [Google Scholar] [CrossRef]
- Li, Z.-Y.; Zhao, D.-J.; Zhao, J.-S. Structure synthesis and workspace analysis of a telescopic spraying robot. Mech. Mach. Theory 2018, 133, 295–310. [Google Scholar] [CrossRef]
- McHale, C.; Telford, R.; Weaver, P.M. Morphing lattice boom for space applications. Compos. Part B Eng. 2020, 202, 108441. [Google Scholar] [CrossRef]
- Virgin, L.N.; Plaut, R.H. Large deflections of folded cantilever: Experiments and elastica analysis. Int. J. Non-Linear Mech. 2021, 129, 103641. [Google Scholar] [CrossRef]
- Lu, W. Simulation Analysis of Feeding Mechanical System Based on SOLIDWORKS and ADAMS. Chem. Ind. Eng. 2020, 11, 136–139. [Google Scholar] [CrossRef]
- Guo, Y.; Gao, H.; Fu, Y.; Duan, Z. Design and simulation of scissor lift platform based on ADAMS and AMESim. Mach. Des. Manuf. Eng. 2019, 48, 29–34. [Google Scholar] [CrossRef]
- Wang, R.; Zheng, Z.; Lu, X.; Gao, L.; Jiang, D.; Zhang, Z. Design, simulation and test of roller comb type Chrysanthemum (Dendranthema morifolium Ramat) picking machine. Comput. Electron. Agric. 2021, 187, 106295. [Google Scholar] [CrossRef]
- Llopis-Albert, C.; Rubio, F.; Zeng, S. Multiobjective optimization framework for designing a vehicle suspension system. A comparison of optimization algorithms. Adv. Eng. Softw. 2023, 176, 103375. [Google Scholar] [CrossRef]
- Zhang, S.; Chen, Y.; Wang, P.; Han, X.; Ma, S.; Wu, M.; Li, G.; Liu, Q. The maximum snow depth, snow pressure, and critical indicators of sunlight greenhouse collapsing in Northeast China. Chin. J. Ecol. 2016, 35, 1601–1607. [Google Scholar] [CrossRef]
- Kaur, S.; Satyawali, P.K. Estimation of snow density from SnowMicroPen measurements. Cold Reg. Sci. Technol. 2017, 134, 1–10. [Google Scholar] [CrossRef]


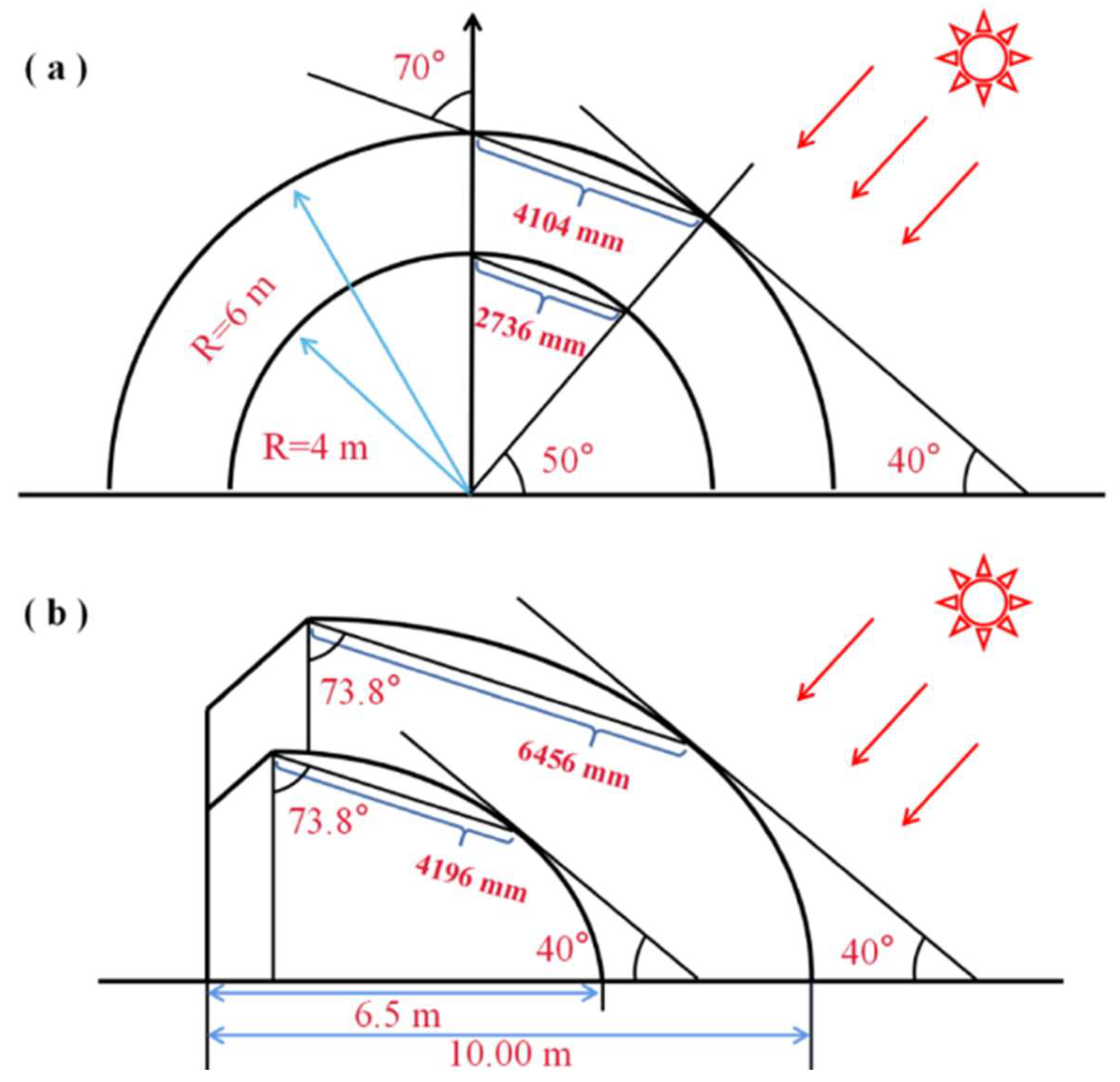



| Span (m) | Snow Removal Range (mm) | Scissor Arm Length (mm) | |||||
|---|---|---|---|---|---|---|---|
| 300 | 400 | 500 | 600 | 700 | |||
| RVSG | 8.0 | 2736 | 11 | 8 | 6 | 5 | 5 |
| 9.0 | 3078 | 12 | 9 | 7 | 6 | 5 | |
| 10.0 | 3420 | 13 | 10 | 8 | 7 | 6 | |
| 11.0 | 3762 | 14 | 11 | 9 | 7 | 6 | |
| 12.0 | 4104 | 16 | 12 | 9 | 8 | 7 | |
| CSG | 6.5 | 4196 | 16 | 12 | 10 | 8 | 7 |
| 7.0 | 4519 | 17 | 13 | 10 | 9 | 7 | |
| 8.0 | 5161 | 20 | 15 | 12 | 10 | 9 | |
| 9.0 | 5810 | 22 | 17 | 13 | 11 | 10 | |
| 10.0 | 6456 | 25 | 19 | 15 | 12 | 11 | |
| Scissor Arm Length (mm) | Thickness (mm) | |||||
|---|---|---|---|---|---|---|
| 1.0 | 2.0 | 3.0 | 4.0 | 5.0 | ||
| 300 | Number | 12 | 12 | 13 | 13 | 12 |
| Range | 3118 | 3118 | 3377 | 3377 | 3118 | |
| Force | 1157 | 1608 | 3257 | 4188 | 3228 | |
| 400 | Number | 12 | 13 | 13 | 12 | 12 |
| Range | 4157 | 4503 | 4503 | 4157 | 4157 | |
| Force | 1186 | 2476 | 3374 | 3606 | 4210 | |
| 500 | Number | 11 | 11 | 12 | 12 | 11 |
| Range | 4763 | 4763 | 5196 | 5196 | 4763 | |
| Force | 1146 | 2196 | 3367 | 4224 | 4100 | |
| 600 | Number | 10 | 11 | 11 | 10 | 10 |
| Range | 5196 | 5716 | 5716 | 5196 | 5196 | |
| Force | 1146 | 2312 | 3235 | 3501 | 3945 | |
| 700 | Number | 10 | 10 | 10 | 10 | 9 |
| Range | 6062 | 6062 | 6062 | 6062 | 5456 | |
| Force | 1204 | 2114 | 2970 | 3959 | 3474 | |
| Span (m) | Scissor Arm Parameters | |||
|---|---|---|---|---|
| Number of Sections | Scissor Arm Length (mm) | Thickness (mm) | ||
| RVSG | 8.0 | 12 | 300 | 2 |
| 9.0 | 13 | 300 | 3 | |
| 10.0 | 12 | 400 | 2 | |
| 11.0 | 12 | 400 | 3 | |
| 12.0 | 13 | 400 | 3 | |
| CSG | 6.5 | 11 | 500 | 2 |
| 7.0 | 12 | 500 | 3 | |
| 8.0 | 11 | 600 | 3 | |
| 9.0 | 10 | 700 | 2 | |
| 10.0 | ||||
| Section | Maximum Shear Force (N) | Total Resistance (N) | Thickness (mm) | Optimised Wall Thickness (mm) |
|---|---|---|---|---|
| 1 | 2050.5285 | 2530.5285 | 2 | 4.027 |
| 2 | 1602.9528 | 2082.95275 | 2 | 3.314 |
| 3 | 1211.5783 | 1691.57825 | 2 | 2.692 |
| 4 | 875.1408 | 1355.140775 | 2 | 2.156 |
| 5 | 593.6431 | 1073.643125 | 2 | 1.708 |
| 6 | 367.0885 | 847.088525 | 2 | 1.348 |
| 7 | 195.4802 | 675.48015 | 2 | 1.075 |
| 8 | 78.8211 | 558.8210625 | 2 | 0.889 |
| 9 | 17.1144 | 497.1143975 | 2 | 0.791 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gang, S.; Gong, Z.; Li, Y.; Liu, Y.; Liu, X.; Li, T. Scissor Arm for Cambered Snow: Mechanical Theory. Machines 2024, 12, 263. https://doi.org/10.3390/machines12040263
Gang S, Gong Z, Li Y, Liu Y, Liu X, Li T. Scissor Arm for Cambered Snow: Mechanical Theory. Machines. 2024; 12(4):263. https://doi.org/10.3390/machines12040263
Chicago/Turabian StyleGang, Shuang, Zhanran Gong, Yiming Li, Yu Liu, Xingan Liu, and Tianlai Li. 2024. "Scissor Arm for Cambered Snow: Mechanical Theory" Machines 12, no. 4: 263. https://doi.org/10.3390/machines12040263
APA StyleGang, S., Gong, Z., Li, Y., Liu, Y., Liu, X., & Li, T. (2024). Scissor Arm for Cambered Snow: Mechanical Theory. Machines, 12(4), 263. https://doi.org/10.3390/machines12040263






