Research on the Aerodynamic Performance and Collaborative Optimization Design of the Full-Scale Compact Inlet Chamber of a Nuclear-Powered Steam Turbine
Abstract
1. Introduction
- (1)
- (2)
- (3)
2. Numerical Method
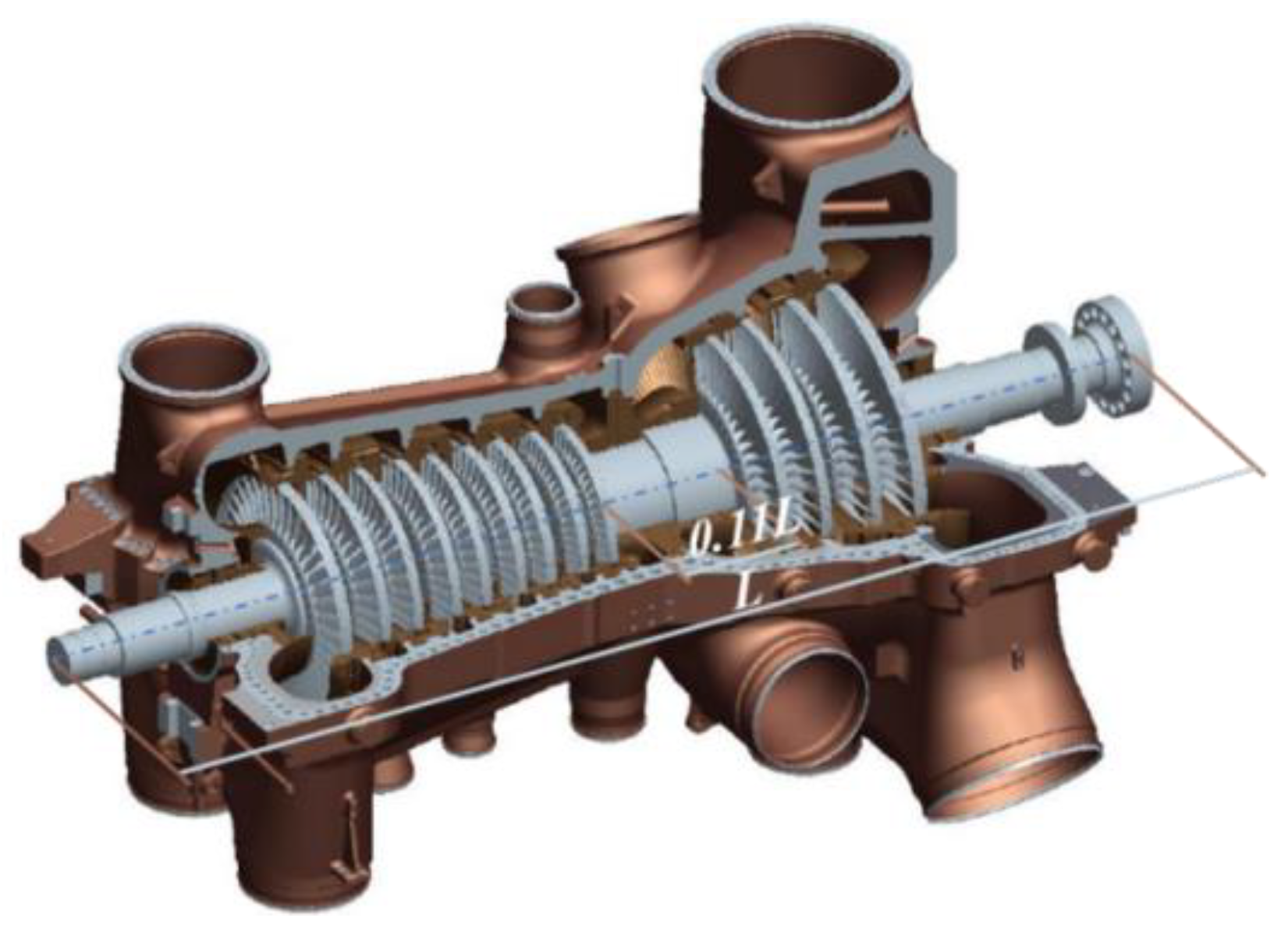
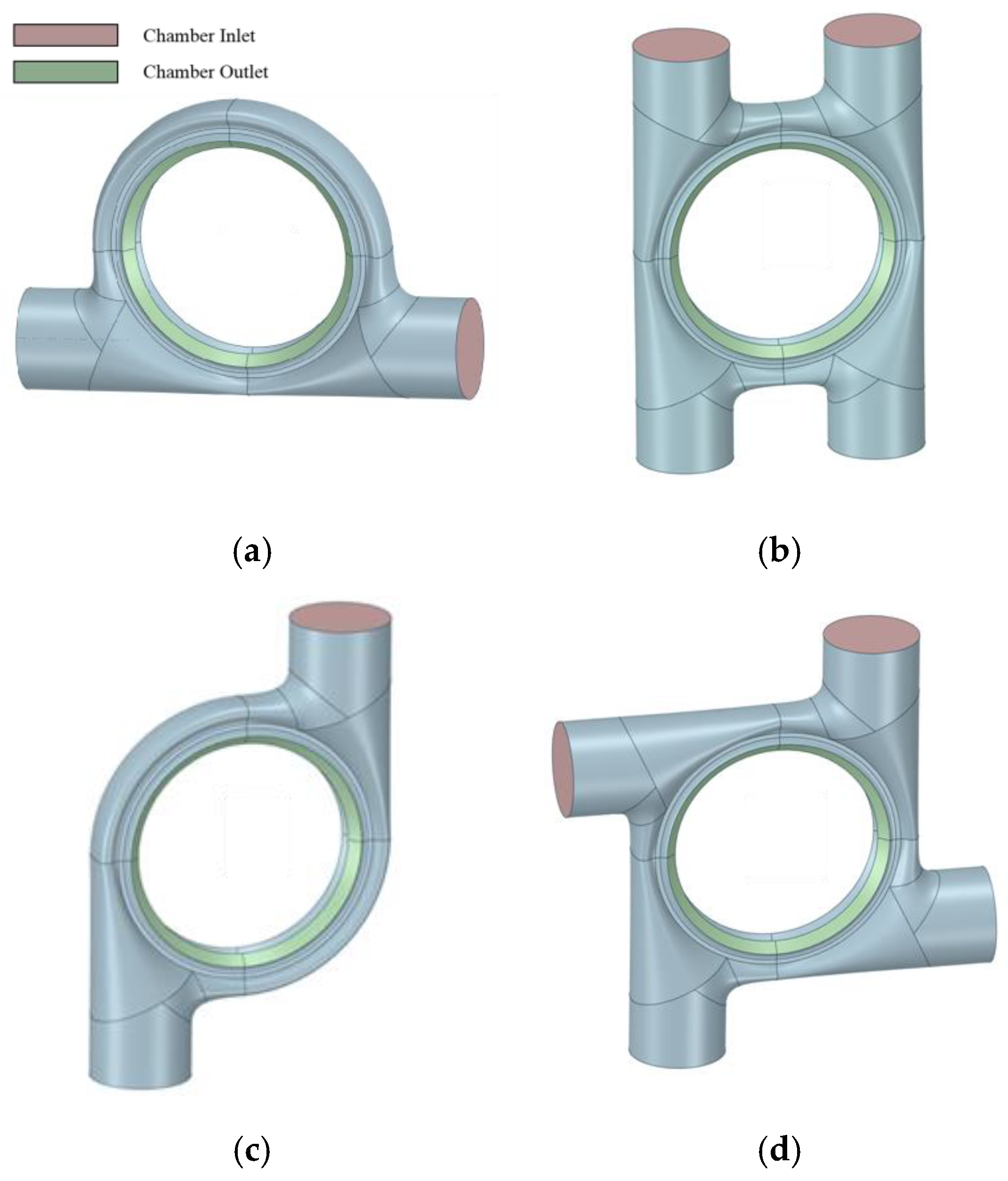
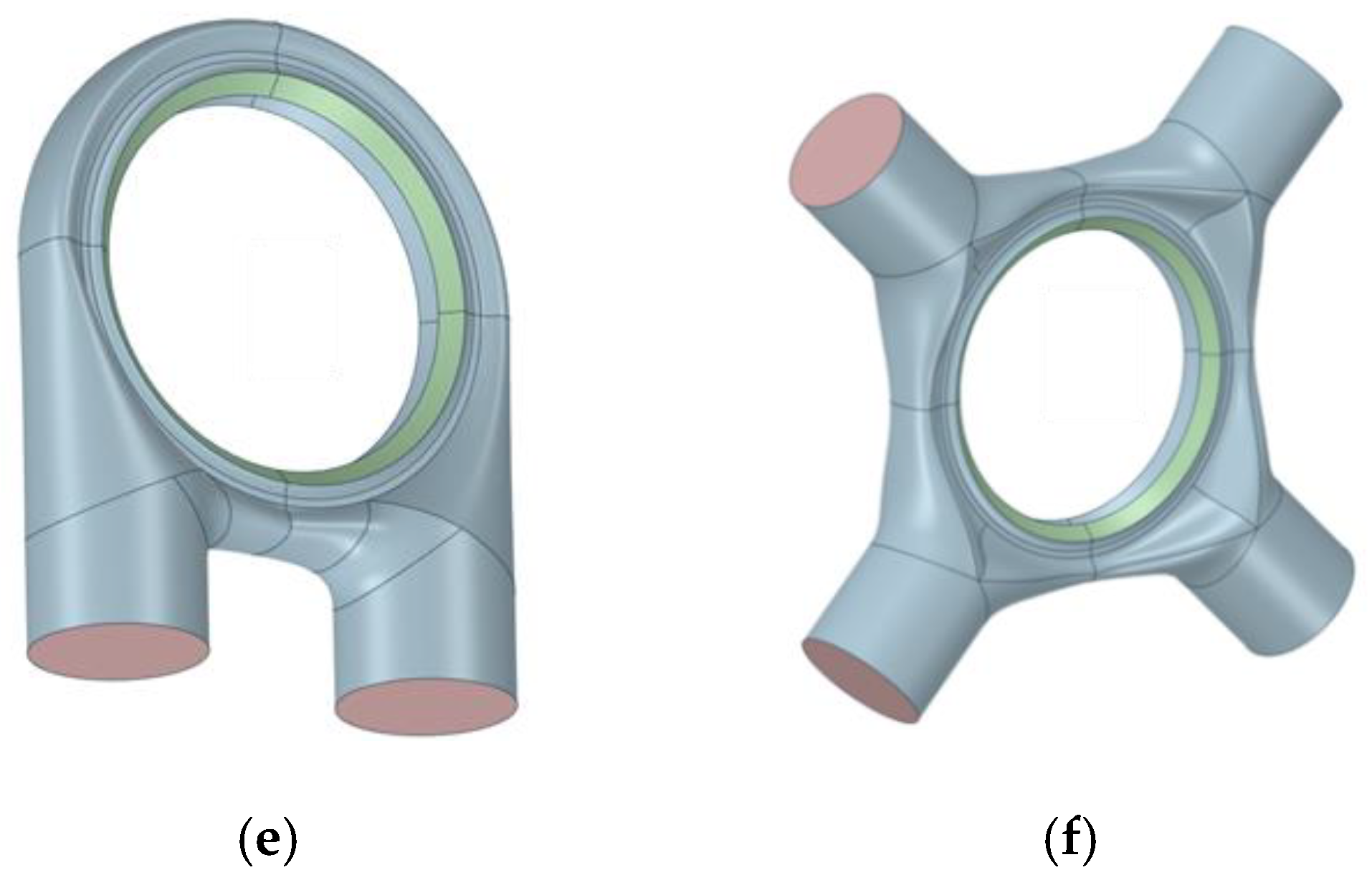
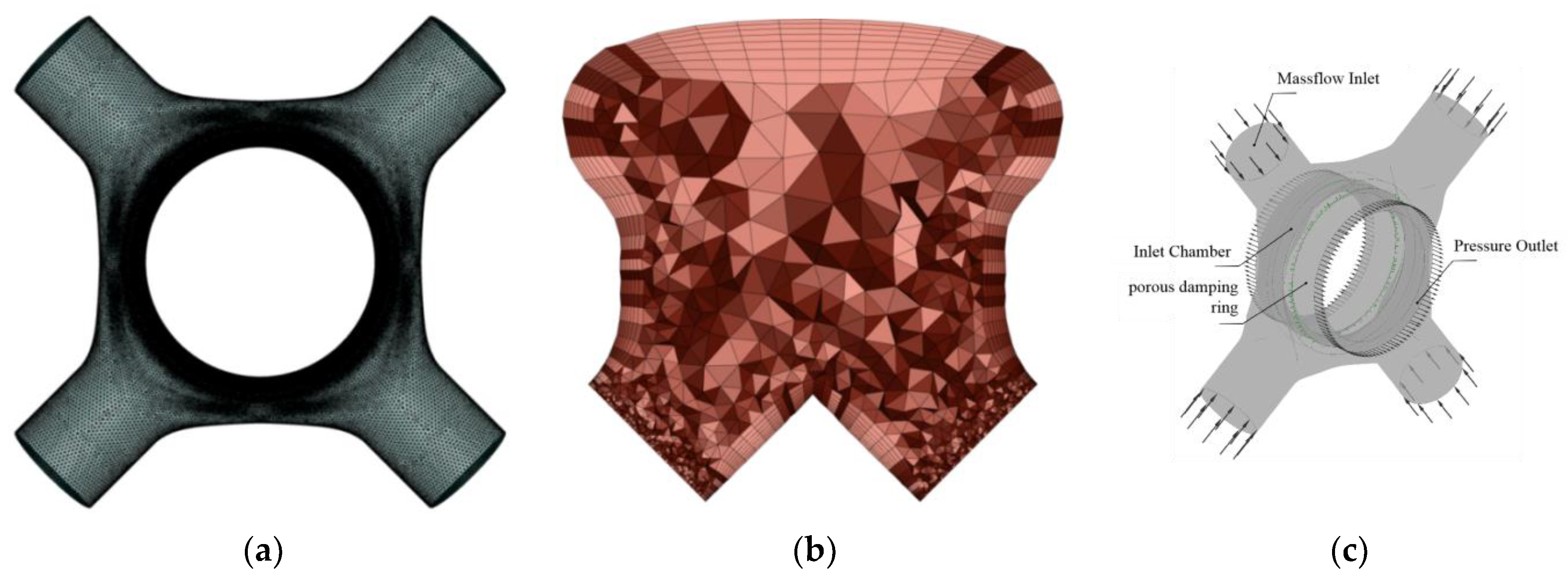
2.1. Geometric Model
2.2. Performance Parameters
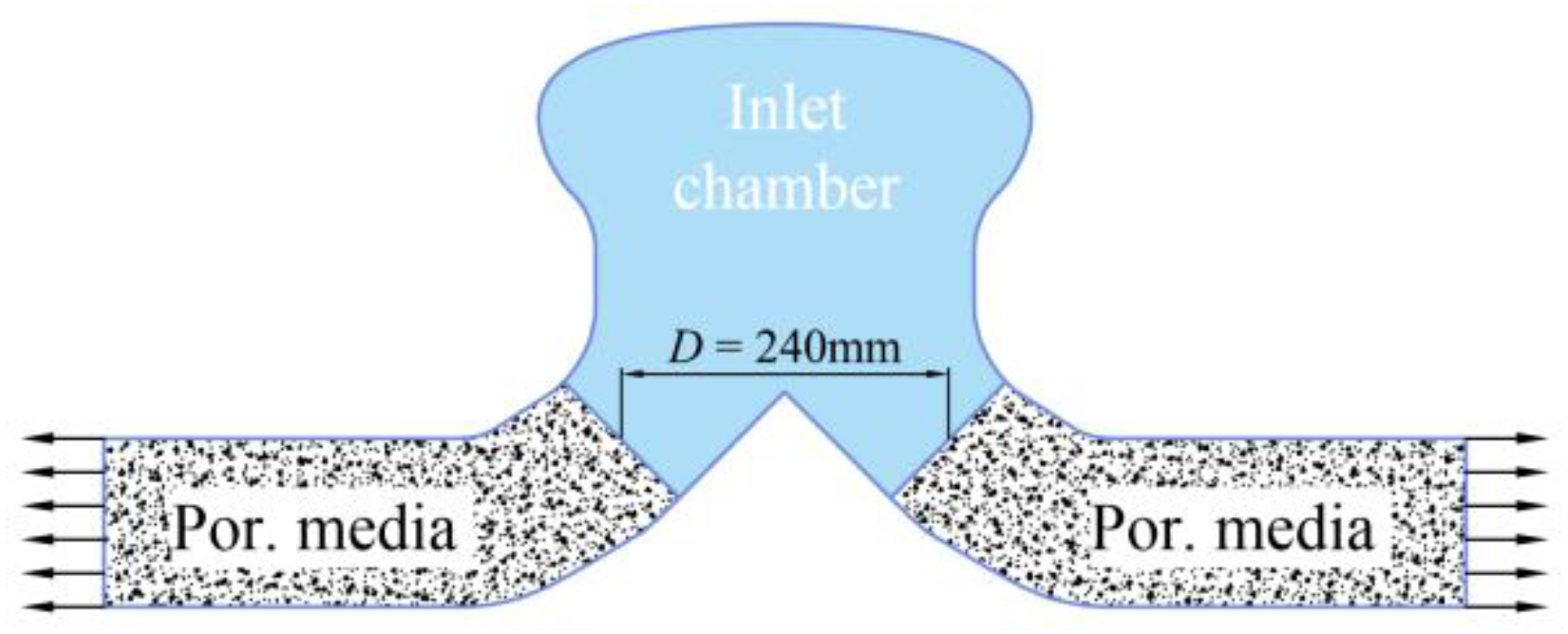
2.3. Numerical Model and Boundary Conditions
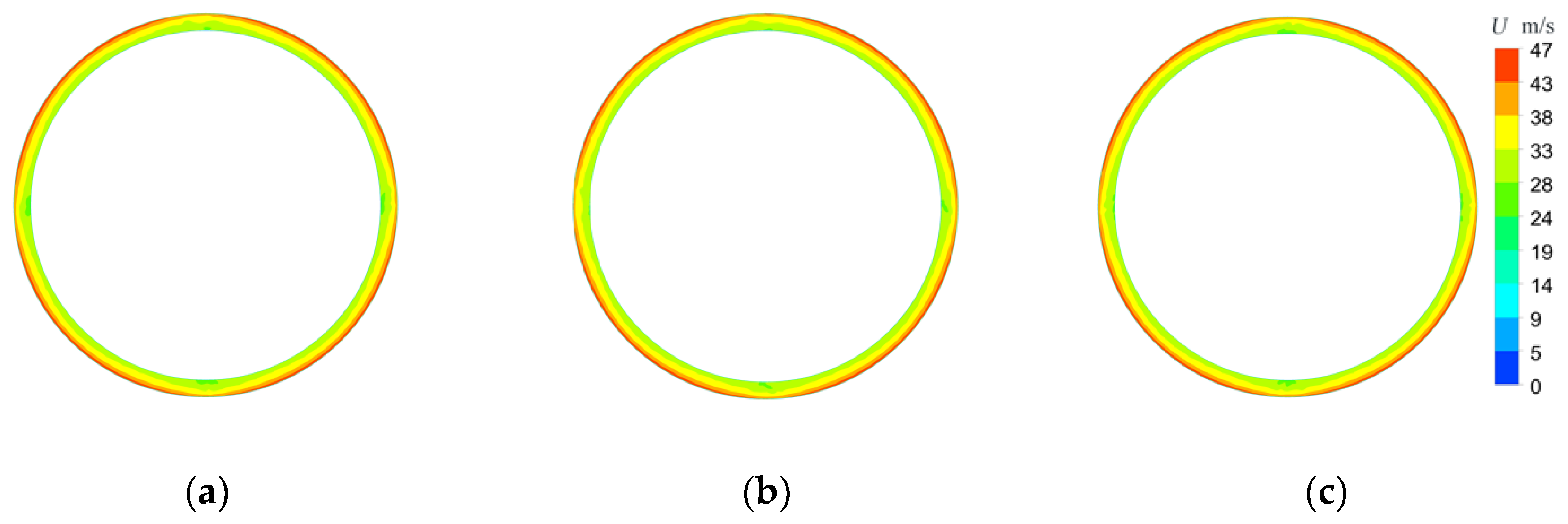
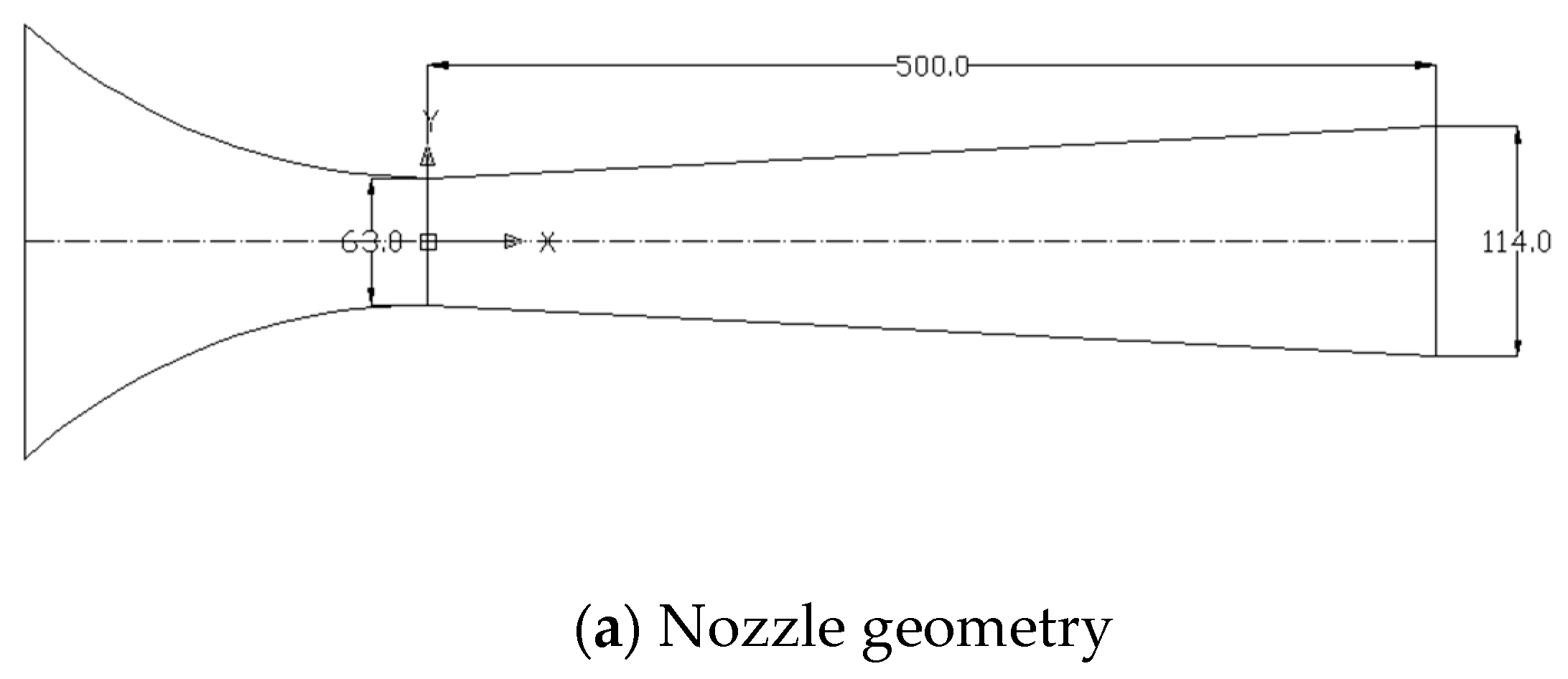
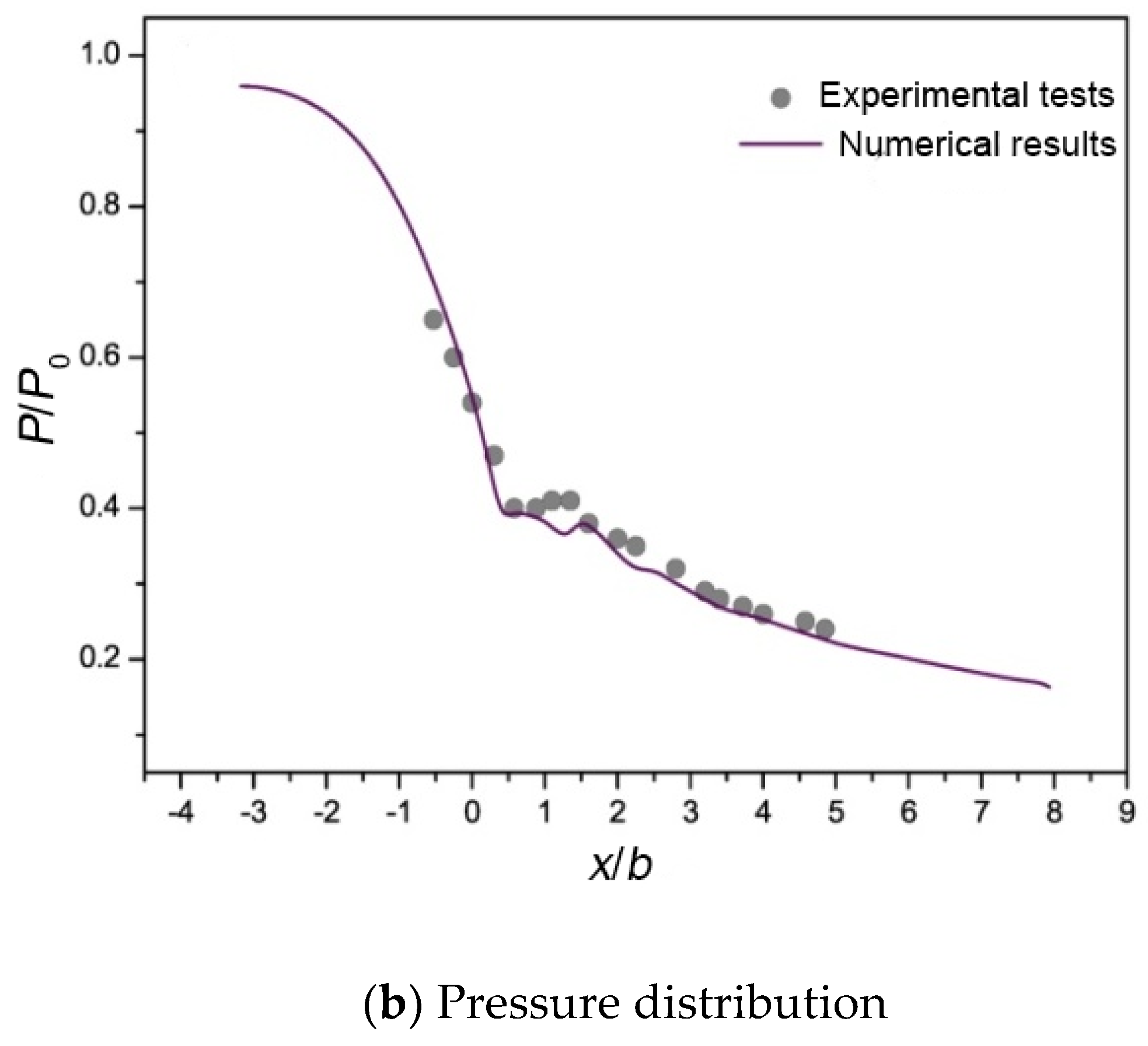
2.4. Grid Irrelevance Verification and Validation
3. Results and Discussion
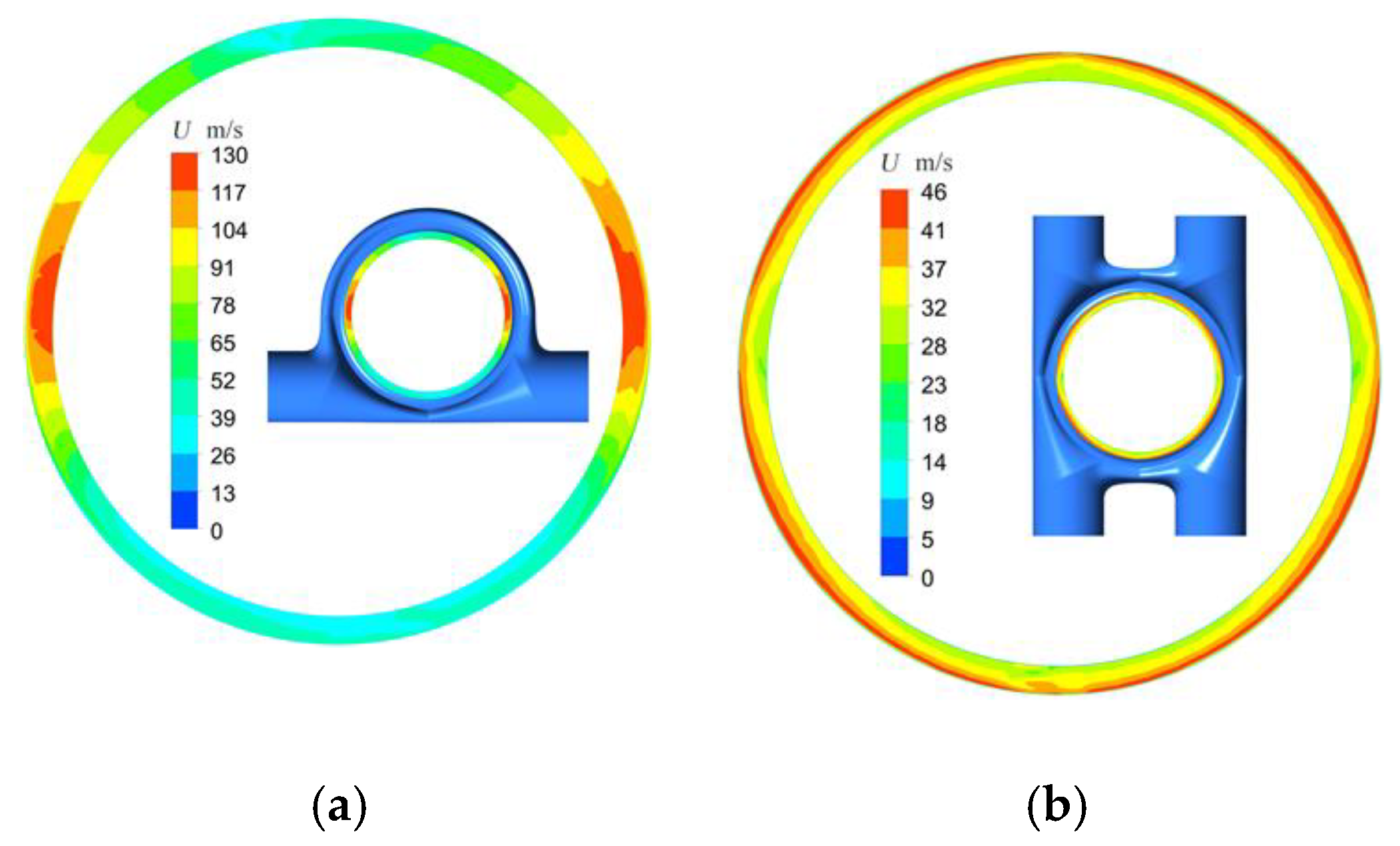
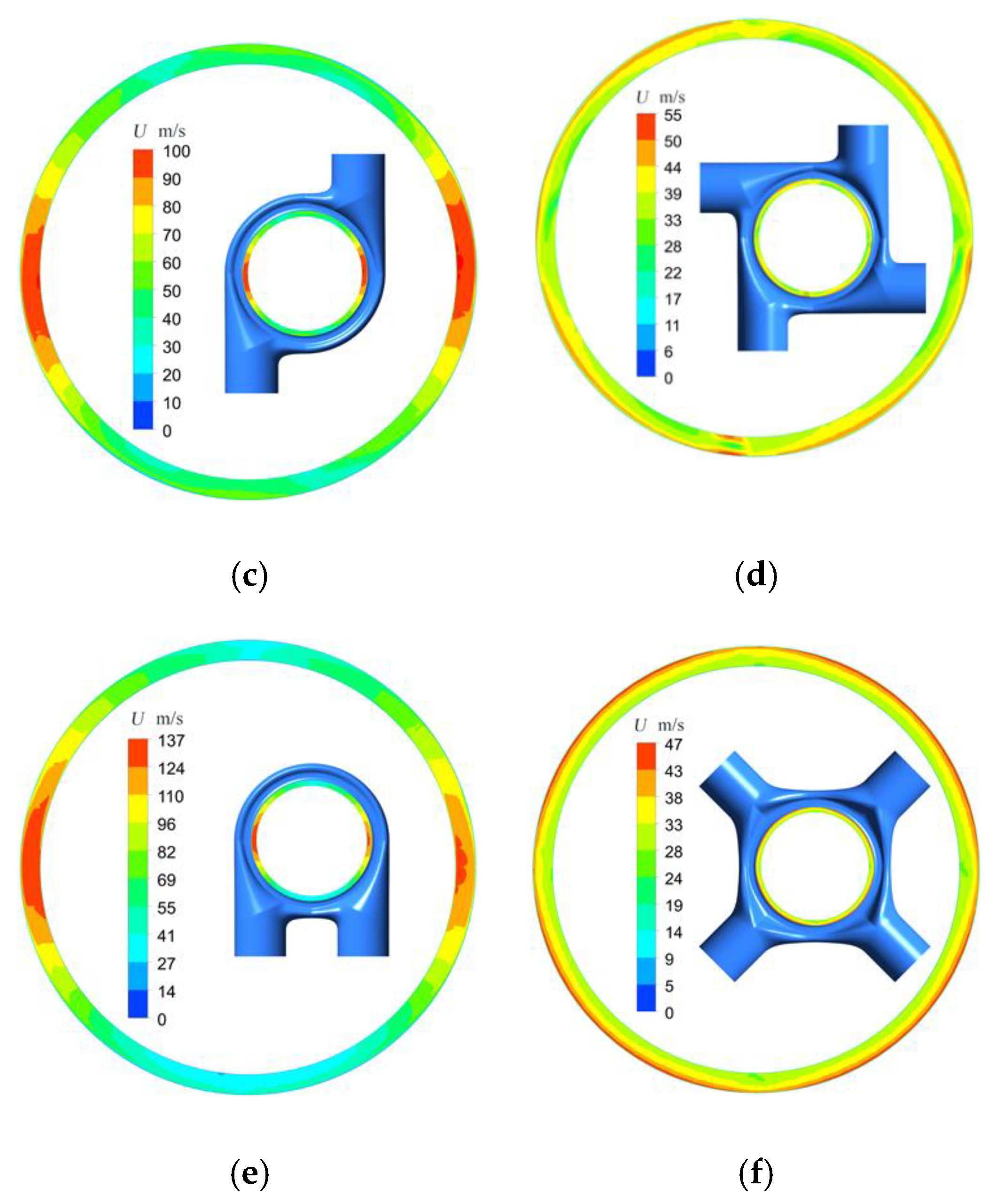
3.1. Effects of Quantity and Arrangement of Inlets
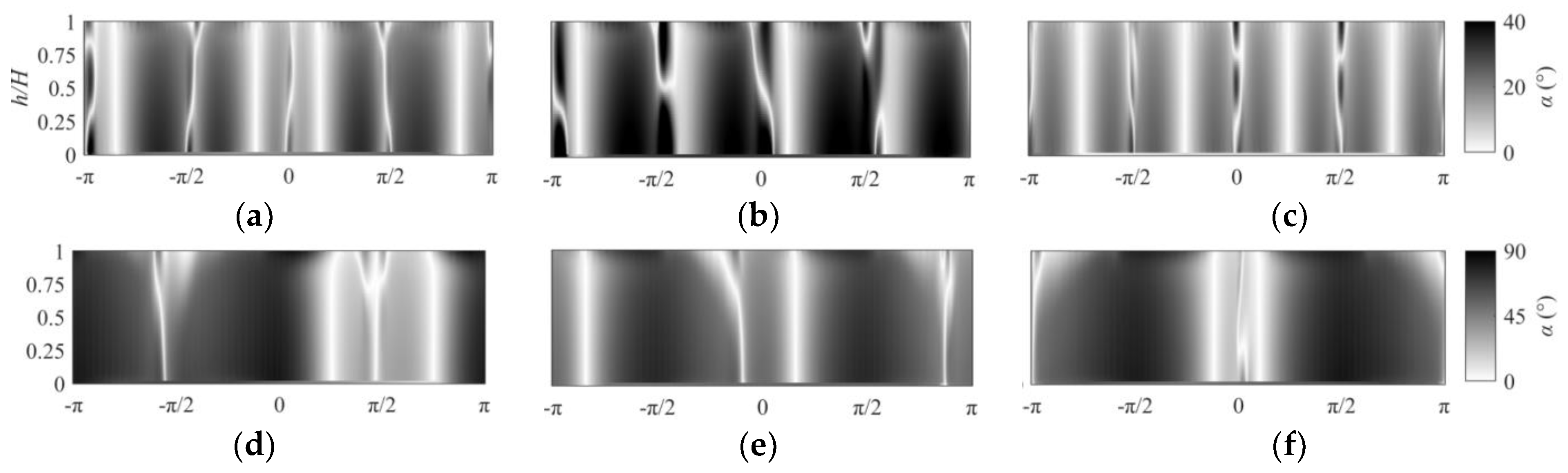
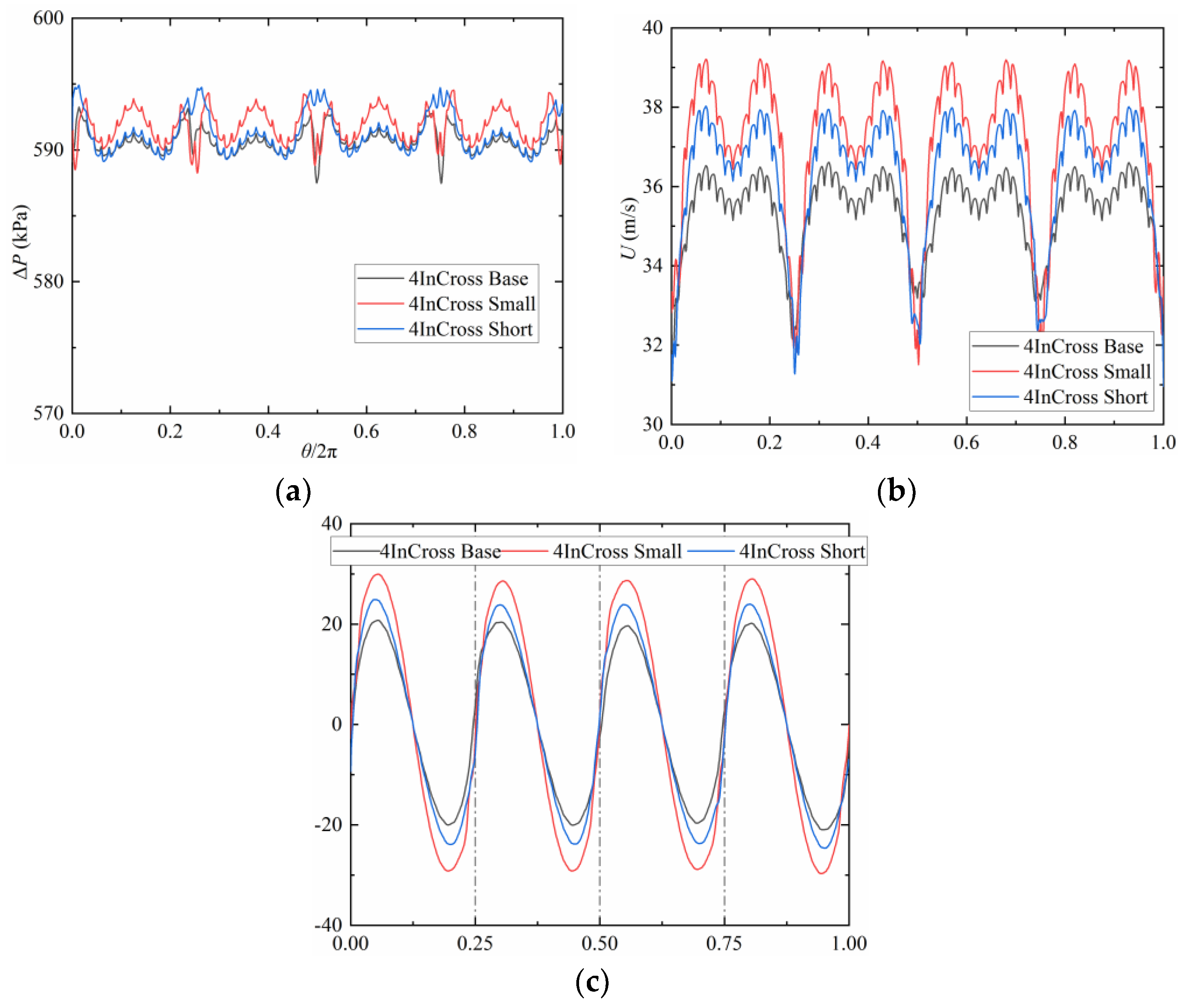
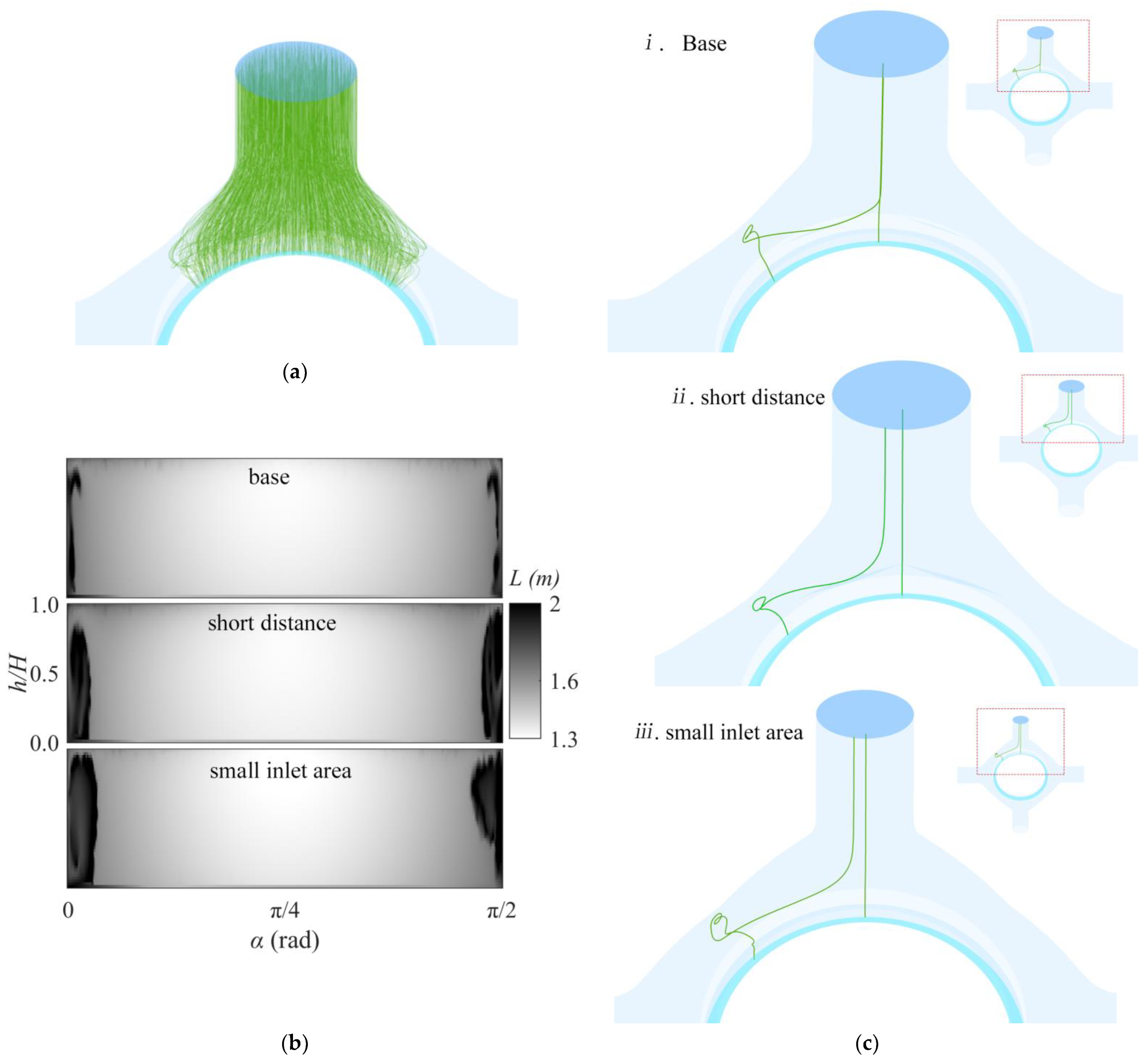
3.2. Effect of Compact Design on Aerodynamic Performance
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Gan, Y.M.; Huang, C.; Wang, W.L. Flow behavior of laval nozzle sets in steam turbine governing stage at low loads. Therm. Sci. Eng. Prog. 2023, 46, 102209. [Google Scholar] [CrossRef]
- Slama, V.; Simurda, D.; Lenhard, R. Pressure Losses Downstream of a Compact Valve in the Inlet Chamber of an Intermediate-Pressure Steam Turbine. Energies 2022, 15, 8753. [Google Scholar] [CrossRef]
- Sláma, V.; Šimurda, D.; Mrózek, L.; Tajč, L.; Hála, J.; Radnic, T. Pressure losses and oscillations in a compact valve of a steam turbine. Power Syst. Eng. 2021, 345, 00027. [Google Scholar] [CrossRef]
- Damavandi, M.D.; Mousavi, S.M.; Hamed Safikhani, H. Pareto optimal design of swirl cooling chambers with tangential injection using CFD, GMDH-type of ANN and NSGA-II algorithm. Int. J. Therm. Sci. 2017, 122, 102–114. [Google Scholar] [CrossRef]
- Simonassi, L.; Zenz, M.; Bruckner, P.; Heitmeir, F.; Marn, A. Aeroelastic and Aerodynamic Investigation of a Low Pressure Turbine Under the Influence of a Circumferential Inlet Distortion. In Proceedings of the ASME Turbo Expo: Power for Land, Sea, and Air, Phoenix, AZ, USA, 17–21 June2019. [Google Scholar]
- Zheng, Y.; Jin, X.B.; Yang, H. Effects of Asymmetric Vane Pitch on Reducing Low-Engine-Order Forced Response of a Turbine Stage. Aerospace 2019, 694, 694. [Google Scholar]
- Gao, L.; Dai, Y.; Wang, Z.; Xu, Y.; Ma, Q. Rotordynamic Stability Under Partial Admission Conditions in a Large Power Steam Turbine. In Proceedings of the ASME Turbo Expo 2009: Power for Land, Sea and Air, Orlando, FL, USA, 8–12 June 2009. [Google Scholar]
- Peng, W.; Ren, X.D.; Li, X.S. Influence of Position of Intake Struts on Unsteady Load and Vibration of First-Stage Rotor. Machines 2022, 10, 1096. [Google Scholar] [CrossRef]
- Trebuňa, P.; Pástor, M.; Trebuňa, F.; Šimčák, F. The analysis of failure causes of the rotor shaft of steam turbines. Metalurgija 2017, 56, 233–236. [Google Scholar]
- Li, S.S.; Qu, T.Z. Causes and troubleshooting of high bearing bush temperature of a nuclear power steam turbine. J. Phys. Conf. Ser. 2022, 2280, 012035. [Google Scholar] [CrossRef]
- Wakeley, G.R.; Potts, I. Origins of Loss Within a Multistage Turbine Environment Under Conditions of Partial Admission. In Proceedings of the ASME International Gas Turbine and Aeroengine Congress and Exhibition, Orlando, FL, USA, 2–5 June 1997. [Google Scholar]
- Sitaram, N.; Prasad, B.V.S.S.S.; Yadav Pillala, V.K.; Purushothama, B. Wake Characteristics of a Steam Turbine Rotor Tip Linear Cascade Bladein Subsonic Flow. Indian J. Eng. Mater. Sci. 2022, 29, 137–143. [Google Scholar]
- Hushmandi, N.B.; Hu, J.; Fridh, J.; Fransson, T.H. Numerical study of unsteady flow phenomena in a partial admission axial steam turbine. In Proceedings of the Turbo Expo: Power for Land, Sea, and Air, Berlin, Germany, 9–13 June 2008. [Google Scholar]
- Pan, Y.; Yuan, Q.; Niu, G.; Gu, J.; Zhu, G. Effect of nozzle box arrangement on the aerodynamic performance of a single stage partial admission turbine. Appl. Therm. Eng. 2019, 159, 113911. [Google Scholar] [CrossRef]
- Sakai, N.; Harada, T.; Imai, Y. Numerical study of partial admission stages in steam turbine (Efficiency improvement by optimizing admission arc position). Fluids Therm. Eng. 2006, 49, 212–217. [Google Scholar] [CrossRef]
- Guo, Z.; Bai, C.; Han, Y.; Zheng, Q. Numerical Study on the Influence of Exhaust Structure on the Performance of a High Expansion Ratio Turbine. J. Phys. Conf. Ser. 2021, 1881, 042090. [Google Scholar] [CrossRef]
- Ahmed, M.; Nagib, E.; Mohey, E. Computational modeling of non-equilibrium condensing steam flows in low-pressure steam turbines. Result Eng. 2020, 5, 100065. [Google Scholar]
- Hhshmandi, N.; Bridh, J.; Eransson, T.H. Unsteady Forces of Rotor Blades in Full and Partial Admission Turbines. J. Turbomach. 2011, 133, 2058–2098. [Google Scholar]
- Domnick, C.B.; Benra, F.-K.; Dohmen, H.J.; Musch, C. Numerical Investigation on the Time-Variant Flow Field and Dynamic Forces Acting in Steam Turbine Inlet Valves. J. Eng. Gas Turbines Power 2015, 137, 1–11. [Google Scholar] [CrossRef]
- Fridh, J.; Laumert, B.T. Forced Response in Axial Turbines Under the Influence of Partial Admission. In Proceedings of the ASME Turbo Expo: Turbine Technical Conference and Exposition, Copenhagen, Denmark, 11–15 June 2012. [Google Scholar]
- Clemens, B.D.; Dieter, B. Flow-Induced Steam Valve Vibrations―A Literature Review of Excitation Mechanisms, Preventive Measures, and Design Improvements. J. Eng. Gas Turbines Power 2019, 141, 051009. [Google Scholar]
- Gao, K.; Wang, C.; Xie, Y.; Zhang, D. Effects of Inlet Chamber Structure of the Control Stage on the Unsteady Aerodynamic Force. In Proceedings of the ASME Turbo Expo: Power for Land, Sea, and Air, Oslo, Norway, 11–15 June 2018. [Google Scholar]
- Hecker, S.; Rohe, A.; Stoff, H. Steam turbine inlet geometry from a structural and fluid dynamics point of view. In Proceedings of the ASME Turbo Expo: Power for Land, Sea, and Air, Copenhagen, Denmark, 11–15 June 2012. [Google Scholar]
- Yada, K.; Uchiumi, M.; Funazakik, I. Thomas/Alford Force on a Partial-Admission Turbine for the Rocket Engine Turbopump. J. Fluids Eng. 2018, 141, 011105. [Google Scholar] [CrossRef]
- Panda, J.P.; Kumar, B.; Patil, A.K.; Kumar, M.; Kumar, R. Machine learning assisted modeling of thermohydraulic correlations for heat exchangers with twisted tape inserts. Acta Mech. Sin. 2023, 39, 322036. [Google Scholar] [CrossRef]
- Panda, J.P.; Kumar, B.; Kumar, M.; Patil, A.K. Influence of twisted tape length on the thermal performance of a heat exchanger tube. Numer. Heat Transf. Part A Appl. 2023, 83, 650–663. [Google Scholar] [CrossRef]
- Miroslav, P.; Petrov, J.F.; Goransson, A. High-Speed Steam Turbine Systems for Small-Scale Power Generation Applications. In Proceedings of the 20th International Conference on Nuclear Engineering and the ASME 2012 Power Conference 2012, Anaheim, CA, USA, 30 July–3 August 2012; pp. 651–657. [Google Scholar]
- Koprowski, A.; Rzadkowski, R. Computational fluid dynamics analysis of 1 MW steam turbine inlet geometries. Arch. Thermodyn. 2021, 42, 35–55. [Google Scholar]
- Kalkkuhl, T.J.; Engelmann, D.; Harbecke, U.; Mailach, R. Numerical analysis of partial admission flow in an industrial steam turbine. In Proceedings of the ASME Turbo Expo: Power for Land, Sea, and Air, Copenhagen, Denmark, 11–15 June 2012. [Google Scholar]
- Moore, M.J.; Walters, P.T.; Crane, R.I.; Davidson, B.J. Predicting the fog-drop size in wet-steam turbines. In Proceedings of the IMechE Conference on Heat and Fluid Flow in Steam and Gas Turbine Plant, Wet Steam 4, Coventry, UK, 3–5 April 1973; pp. 101–109. [Google Scholar]





| Local Grid Size (mm) | Surface Grid Size (mm) | Maximum Body Unit Size (mm) | Boundary Layer Grid Size (mm) | Number of Units/10,000 | (Umax − Umin)/U | |||
|---|---|---|---|---|---|---|---|---|
| Interface 1 and 2 | Diversion Wall Surface | Minimum | Maximum | Layer Number | First Layer Width-to-Height Ratio | |||
| 3 | 10 | 3 | 30 | 40 | 15 | 10 | 514 | 0.130 |
| 3 | 10 | 3 | 30 | 40 | 12 | 10 | 553 | 0.145 |
| 3 | 10 | 3 | 30 | 40 | 8 | 10 | 564 | 0.131 |
| 3 | 10 | 3 | 30 | 40 | 15 | 20 | 609 | 0.119 |
| 2 | 7 | 2 | 20 | 30 | 12 | 10 | 1135 | 0.134 |
| 1.5 | 5 | 1.5 | 20 | 23 | 12 | 10 | 2227 | 0.138 |
| Inlet Chamber Configuration | Dual Parallel | Dual Hedging | Dual Ring | Quadruple Ring | Quadruple Parallel and Hedging | Quadruple Cross | |
|---|---|---|---|---|---|---|---|
| Aerodynamic performance | Mean velocity (m/s) | 71.8 | 66.0 | 58.8 | 38.1 | 35.8 | 35.4 |
| Range of velocity (m/s) | 77.1 | 67.2 | 52.8 | 7.5 | 6.3 | 4.8 | |
| Relative range of velocity (%) | 107.3 | 101.9 | 89.9 | 19.7 | 17.7 | 13.4 | |
| Mean pressure difference (kPa) | 614 | 608 | 605 | 593 | 591 | 591 | |
| Range of pressure difference (kPa) | 286 | 255 | 138 | 34 | 11 | 6 | |
| Relative range of pressure difference (%) | 46.7 | 42.0 | 22.8 | 5.7 | 1.8 | 1.0 | |
| Bending moment (kN·m) | 6.20 | 9.74 | 0.34 | 0.55 | 0.22 | 0.24 | |
| Mean deflection angle (°) | 4.8 | −2.1 | −28.9 | −1.3 | −19.9 | 0.0 | |
| Range of deflection angle (°) | 155.4 | 153.4 | 118.0 | 55.6 | 49.5 | 41.7 | |
| Cases | 4InCross_Base | 4InCross_Small | 4InCross_Short | |||
|---|---|---|---|---|---|---|
| compactness indices | area ratio | 1.48 | 0.74 | −50% | 1.48 | |
| outlet center distance (mm) | 240 | 240 | 120.0 | −50% | ||
| aerodynamic performance indices | Mean velocity (m/s) | 35.4 | 37.1 | 36.3 | ||
| range of velocity (m/s) | 4.76 | 7.71 | 7.07 | |||
| Relative range of velocity (%) | 13.4 | 20.8 | 55% | 19.5 | 45% | |
| Mean pressure difference (kPa) | 591 | 592 | 591.0 | |||
| Range of pressure difference (kPa) | 5.78 | 6.30 | 5.80 | |||
| Relative range of pressure difference (%) | 0.979 | 1.066 | 9.80% | 0.984 | 0.50% | |
| Mean deflection angle (°) | 0.03 | −0.19 | −0.16 | |||
| Range of deflection angle (°) | 41.7 | 59.6 | 21% | 49.5 | 18.60% | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, L.; Jiang, W.; Xie, L.; Chen, G. Research on the Aerodynamic Performance and Collaborative Optimization Design of the Full-Scale Compact Inlet Chamber of a Nuclear-Powered Steam Turbine. Machines 2024, 12, 262. https://doi.org/10.3390/machines12040262
Zhang L, Jiang W, Xie L, Chen G. Research on the Aerodynamic Performance and Collaborative Optimization Design of the Full-Scale Compact Inlet Chamber of a Nuclear-Powered Steam Turbine. Machines. 2024; 12(4):262. https://doi.org/10.3390/machines12040262
Chicago/Turabian StyleZhang, Lei, Wei Jiang, Luotao Xie, and Guobing Chen. 2024. "Research on the Aerodynamic Performance and Collaborative Optimization Design of the Full-Scale Compact Inlet Chamber of a Nuclear-Powered Steam Turbine" Machines 12, no. 4: 262. https://doi.org/10.3390/machines12040262
APA StyleZhang, L., Jiang, W., Xie, L., & Chen, G. (2024). Research on the Aerodynamic Performance and Collaborative Optimization Design of the Full-Scale Compact Inlet Chamber of a Nuclear-Powered Steam Turbine. Machines, 12(4), 262. https://doi.org/10.3390/machines12040262






