Investigation of Force-Controlled Polishing of Complex Curved PMMA Parts on a Machining Center
Abstract
1. Introduction
2. Structure of Force-Controlled Polishing Device and Development of Polishing System
2.1. Structure of Force-Controlled Polishing Device
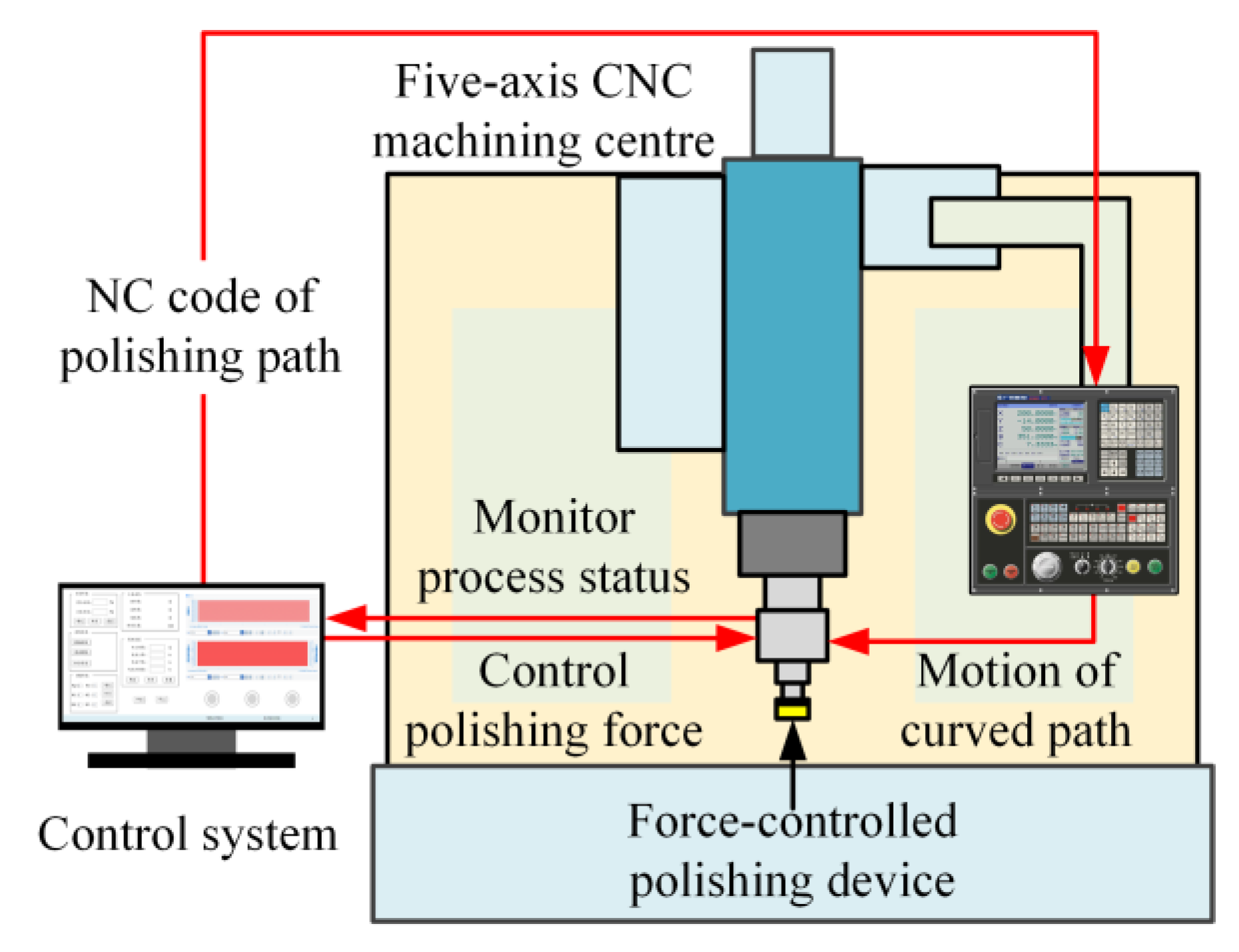
2.2. Development of Polishing System on Machining Center
3. Modeling of Polishing Force and Implementation of Fuzzy PI Controller
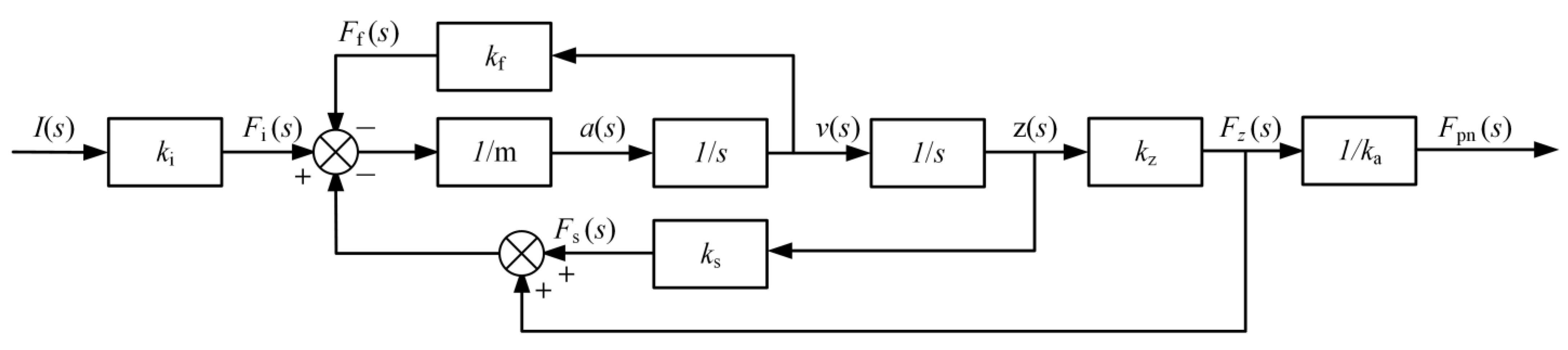
3.1. Polishing Force Analysis and Modeling of Device
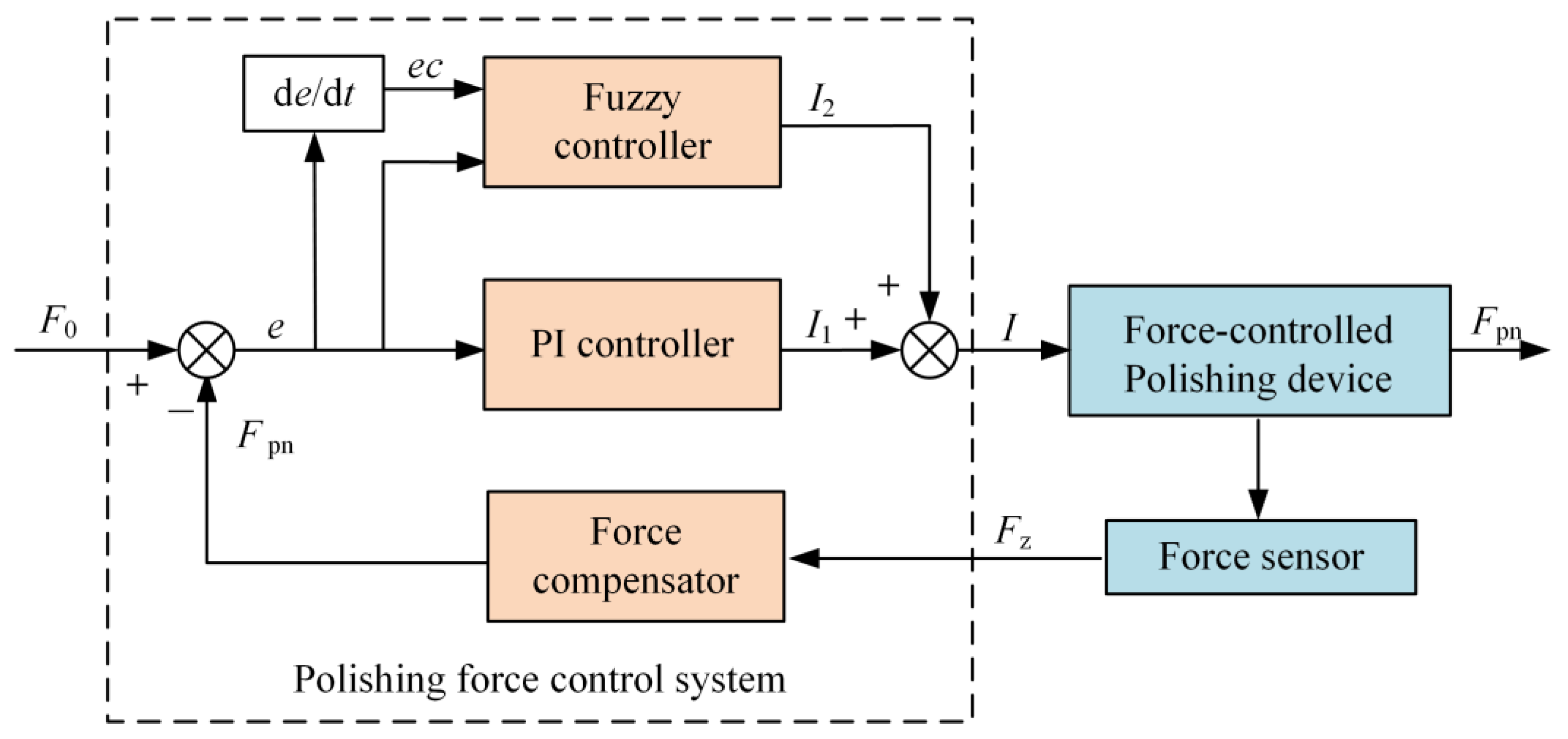
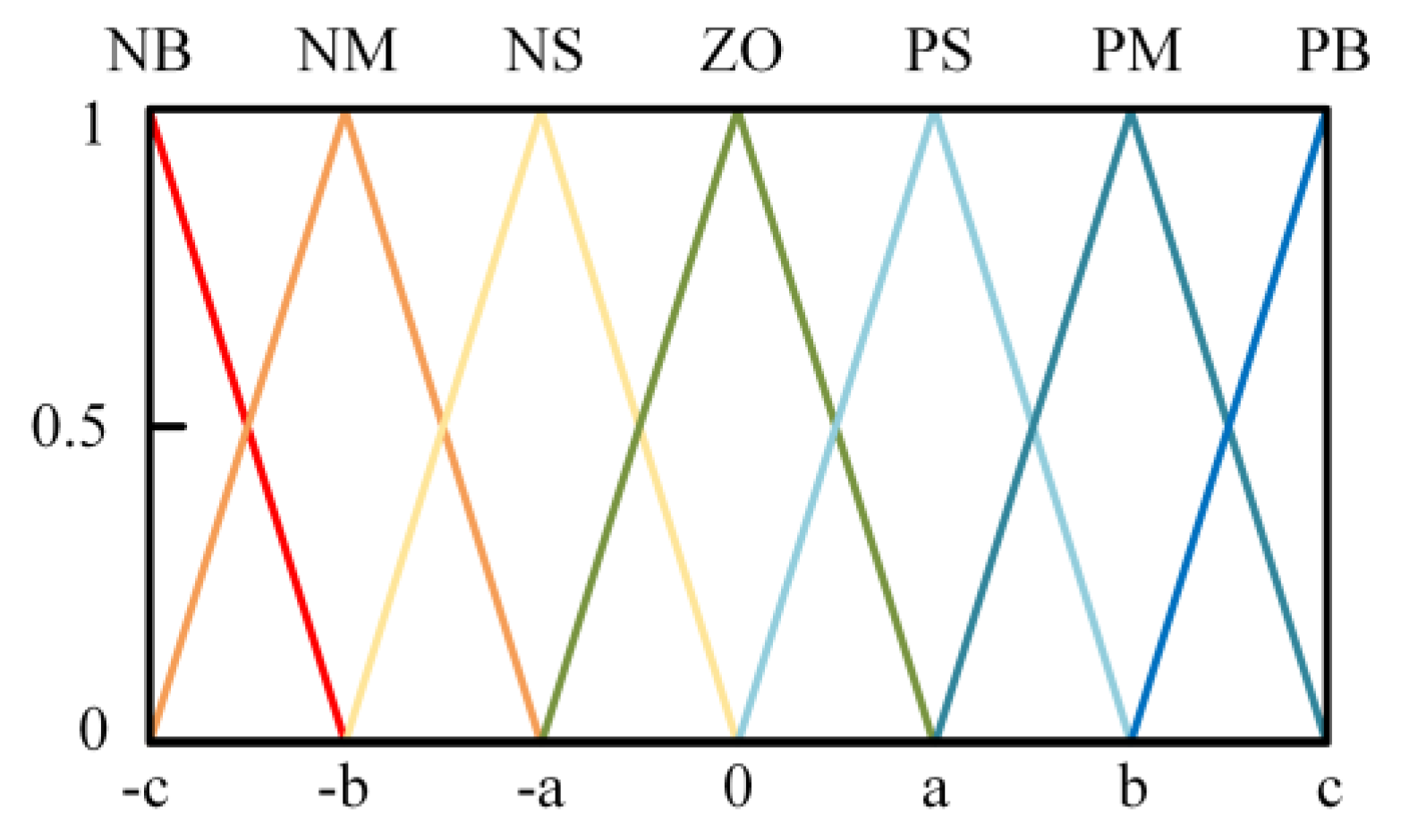
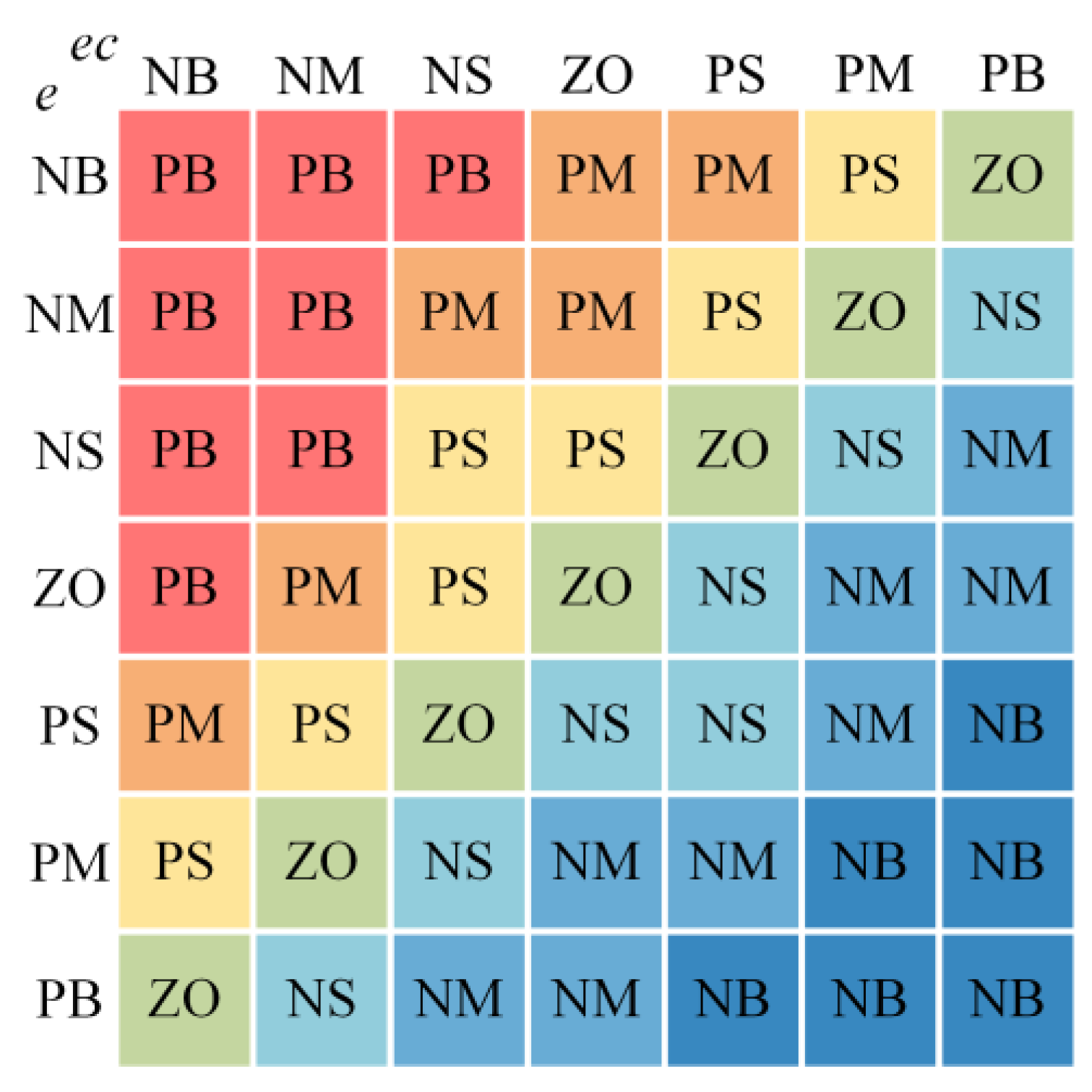
3.2. Force Control with Fuzzy PI Controller
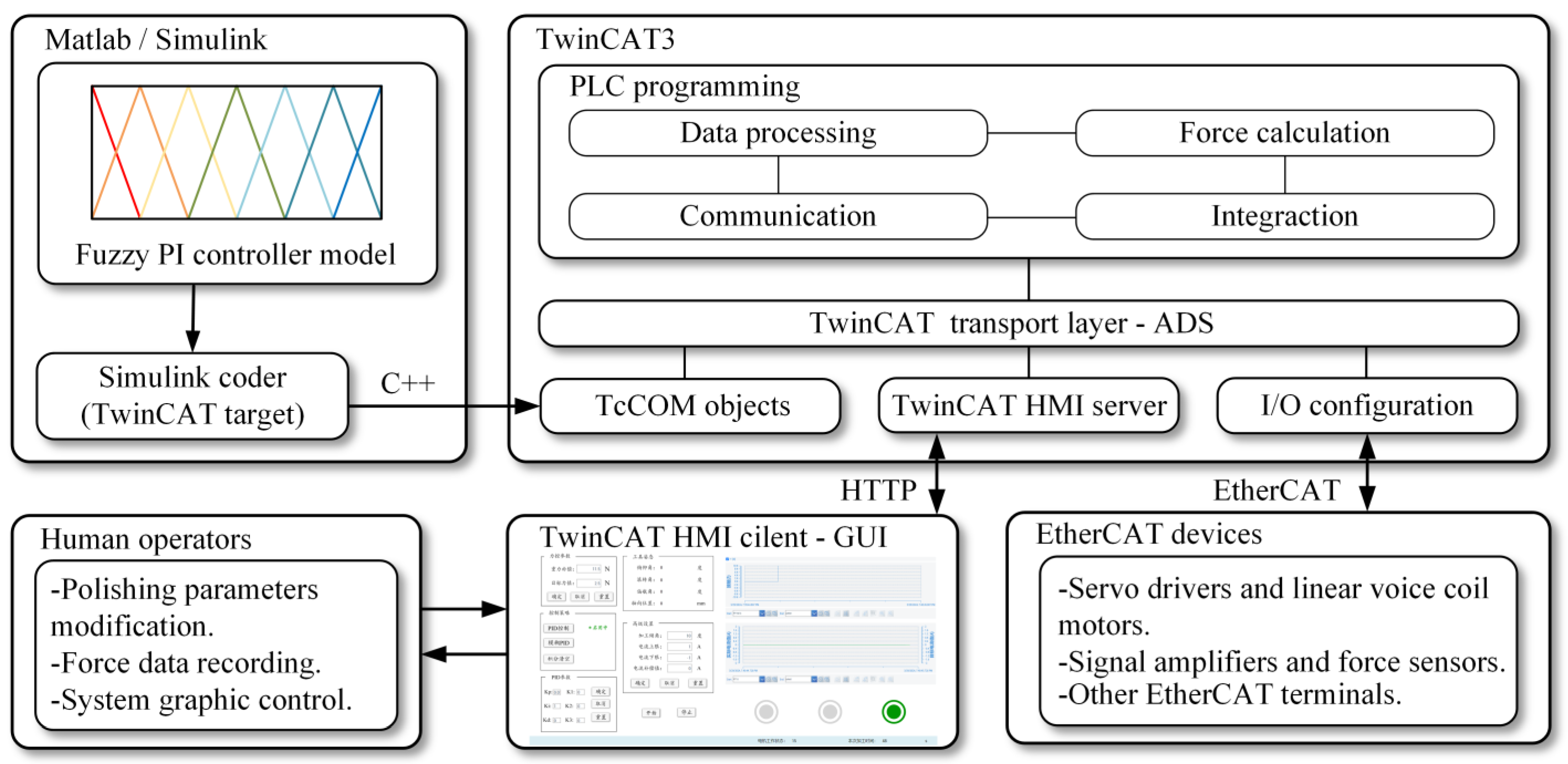
3.3. Implementation of Control Method
4. Experiment and Verification
4.1. Experiment Settings
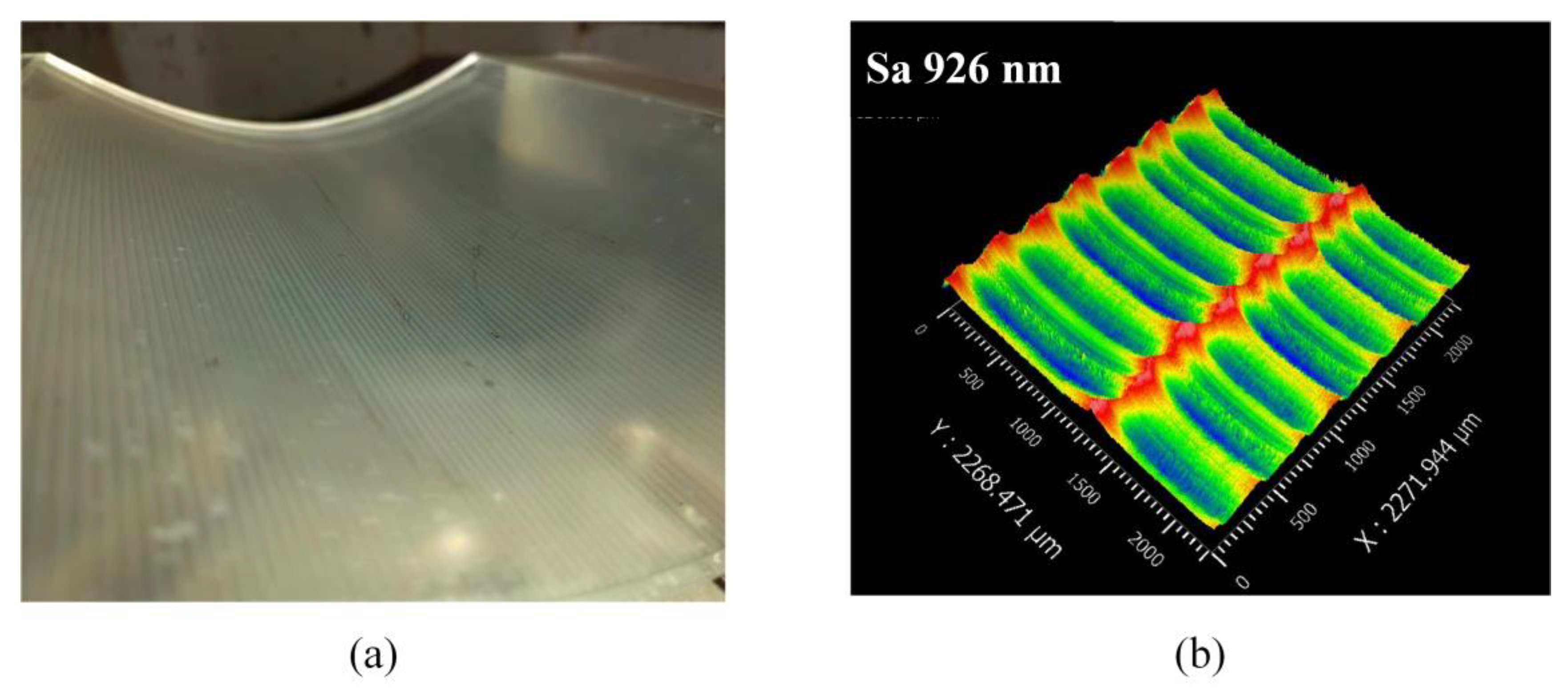
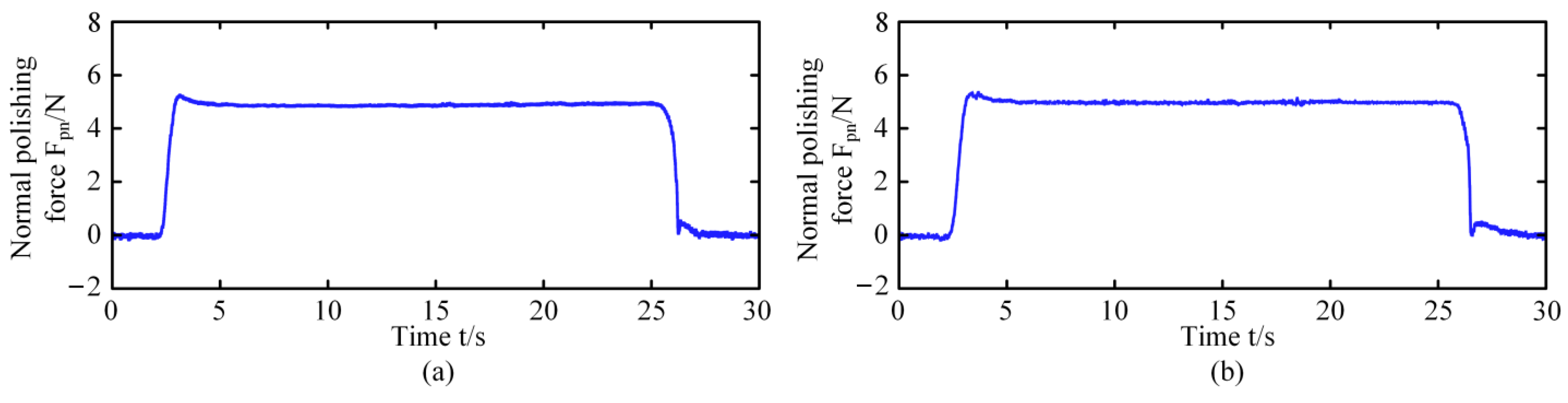
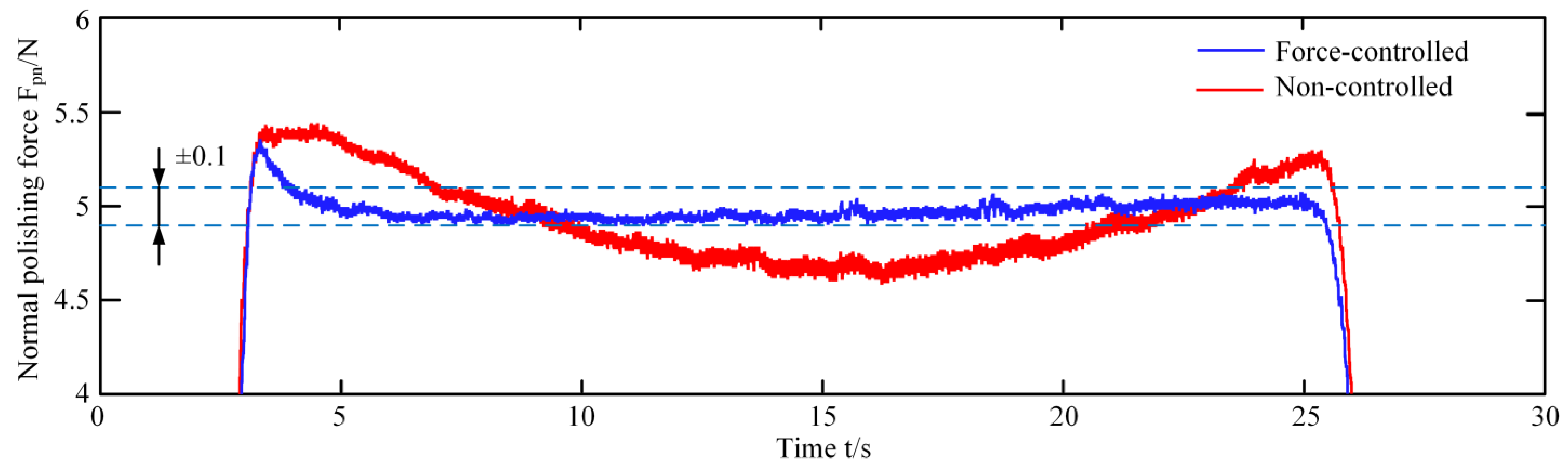
4.2. Results and Verification
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Wang, X.K.; Wei, S.C.; Xu, B.S.; Chen, Y.; Yan, X.; Xia, H.H. Transparent Organic Materials of Aircraft Cockpit Canopies: Research Status and Development Trends. Mater. Res. Innov. 2015, 19, S10-199–S10-206. [Google Scholar] [CrossRef]
- Guo, Q.; Wang, W.; Jiang, Y.; Sun, Y. 3D Surface Topography Prediction in the Five-Axis Milling of Plexiglas and Metal Using Cutters with Non-Uniform Helix and Pitch Angles Combining Runout. J. Mater. Process. Technol. 2023, 314, 117885. [Google Scholar] [CrossRef]
- Yan, S.; Sun, Y. Enhancing Tool Dynamics and Stability in Internal Turning with an Adjustable Clamping Device under Variable Cutting Conditions. Mech. Syst. Signal Process. 2024, 208, 111007. [Google Scholar] [CrossRef]
- Guo, Q.; Yang, Z.; Xu, J.; Jiang, Y.; Wang, W.; Liu, Z.; Zhao, W.; Sun, Y. Progress, Challenges and Trends on Vision Sensing Technologies in Automatic/Intelligent Robotic Welding: State-of-the-Art Review. Robot. Comput.-Integr. Manuf. 2024, 89, 102767. [Google Scholar] [CrossRef]
- Li, B.; Li, G.; Lin, W.; Xu, P. Design and Constant Force Control of a Parallel Polishing Machine. In Proceedings of the 2014 4th IEEE International Conference on Information Science and Technology, Shenzhen, China, 26–28 April 2014; IEEE: Piscataway, NJ, USA, 2014; pp. 324–328. [Google Scholar]
- Fan, C.; Xu, K.; Zhang, L.; Yuan, Q.; Wang, Q.; Wang, K.; Sun, L. Kinematic Planning and In-Situ Measurement of Seven-Axis Five-Linkage Grinding and Polishing Machine Tool for Complex Curved Surface. Mach. Sci. Technol. 2022, 26, 203–228. [Google Scholar] [CrossRef]
- Wang, G.M.; Zhan, J.M. Modeling of Machining Force Error in Aspheric Surface Polishing by Hybrid Movement/Force Control Policy. AMM 2011, 101–102, 795–799. [Google Scholar] [CrossRef]
- Ji, S.M.; Zhang, L.; Yuan, Q.L.; Jin, M.S.; Yuan, J.L. A Novel Ballonet Polishing Tool and Its Robot Control System for Polishing the Curved Surface of Mould. IJCAT 2007, 29, 212. [Google Scholar] [CrossRef]
- Wahjudi, A.; Shiou, F.J. Simulation Study of Sliding Control for Constant Polishing Force Using an Innovative Sphere-Like Polishing Tool on a Machining Center. AMR 2010, 126–128, 505–510. [Google Scholar] [CrossRef]
- Yong, J.; Peng, X.; Hu, H.; Zhao, T. Research on Key Technologies in the Conformal Polishing of Complex Surface Optical Components. In Proceedings of the AOPC 2019: Space Optics, Telescopes, and Instrumentation, Beijing, China, 7–9 July 2019; Xue, S., Zhang, X., Zhang, Z., Nardell, C.A., Eds.; SPIE: Beijing, China, 2019; p. 74. [Google Scholar]
- Zhang, L.; Ding, C.; Fan, C.; Wang, Q.; Wang, K. Process Planning of the Automatic Polishing of the Curved Surface Using a Five-Axis Machine Tool. Int. J. Adv. Manuf. Technol. 2022, 120, 7205–7218. [Google Scholar] [CrossRef]
- Sun, Y.; Feng, D.; Guo, D. An Adaptive Uniform Toolpath Generation Method for the Automatic Polishing of Complex Surfaces with Adjustable Density. Int. J. Adv. Manuf. Technol. 2015, 80, 1673–1683. [Google Scholar] [CrossRef]
- Xu, C.-Y.; Li, J.-R.; Liang, Y.-J.; Wang, Q.-H.; Zhou, X.-F. Trochoidal Toolpath for the Pad-Polishing of Freeform Surfaces with Global Control of Material Removal Distribution. J. Manuf. Syst. 2019, 51, 1–16. [Google Scholar] [CrossRef]
- Zeng, S.; Blunt, L. An Experimental Study on the Correlation of Polishing Force and Material Removal for Bonnet Polishing of Cobalt Chrome Alloy. Int. J. Adv. Manuf. Technol. 2014, 73, 185–193. [Google Scholar] [CrossRef]
- Chen, H.; Chen, C.-Y.; Li, J.; Fang, Z.; Li, H.; Li, G. Research on Polishing Parameters Optimization for Free Curved Surface. In Proceedings of the 2018 13th IEEE Conference on Industrial Electronics and Applications (ICIEA), Wuhan, China, 31 May–2 June 2018; IEEE: Piscataway, NJ, USA, 2018; pp. 2339–2344. [Google Scholar]
- Deng, Y.; Wang, G.; Yue, X.; Zhou, K. A Review of Robot Grinding and Polishing Force Control Mode. In Proceedings of the 2022 IEEE International Conference on Mechatronics and Automation (ICMA), Guilin, China, 7–10 August 2022; IEEE: Piscataway, NJ, USA, 2022; pp. 1413–1418. [Google Scholar]
- Dai, J.; Chen, C.-Y.; Zhu, R.; Yang, G.; Wang, C.; Bai, S. Suppress Vibration on Robotic Polishing with Impedance Matching. Actuators 2021, 10, 59. [Google Scholar] [CrossRef]
- Shiou, F.-J.; Banh, Q.-N. Development of an Innovative Small Ball-Burnishing Tool Embedded with a Load Cell. Int. J. Adv. Manuf. Technol. 2016, 87, 31–41. [Google Scholar] [CrossRef]
- Kakinuma, Y.; Igarashi, K.; Katsura, S.; Aoyama, T. Development of 5-Axis Polishing Machine Capable of Simultaneous Trajectory, Posture, and Force Control. CIRP Ann. 2013, 62, 379–382. [Google Scholar] [CrossRef]
- Oba, Y.; Yamada, Y.; Kakinuma, Y. Simultaneous Control of Tool Posture and Polishing Force on Unknown Curved Surface for Serial-Parallel Mechanism Polishing Machine. In Proceedings of the IECON 2015 41st Annual Conference of the IEEE Industrial Electronics Society, Yokohama, Japan, 9–12 November 2015; IEEE: Piscataway, NJ, USA, 2015; pp. 005351–005356. [Google Scholar]
- Asaga, R.; Yamato, S.; Kakinuma, Y. Analysis of Tool Posture Control Method on Curved Surface Using Polishing Machine with 5-Axis Serial-Parallel Mechanism. In Proceedings of the IECON 2017 3rd Annual Conference of the IEEE Industrial Electronics Society, Beijing, China, 29 October–1 November 2017; IEEE: Piscataway, NJ, USA, 2017; pp. 2979–2984. [Google Scholar]
- Sencer, B.; Kakinuma, Y.; Yamada, Y. Linear Interpolation of Machining Tool-Paths with Robust Vibration Avoidance and Contouring Error Control. Precis. Eng. 2020, 66, 269–281. [Google Scholar] [CrossRef]
- Tian, F.; Lv, C.; Li, Z.; Liu, G. Modeling and Control of Robotic Automatic Polishing for Curved Surfaces. CIRP J. Manuf. Sci. Technol. 2016, 14, 55–64. [Google Scholar] [CrossRef]
- Tian, F.; Li, Z.; Lv, C.; Liu, G. Polishing Pressure Investigations of Robot Automatic Polishing on Curved Surfaces. Int. J. Adv. Manuf. Technol. 2016, 87, 639–646. [Google Scholar] [CrossRef]
- Wang, D.; Li, J.; Guan, Y.; Chen, H.; Wang, B.; Zhang, T.; Liu, X.; Hong, J.; Zhang, H. A High-Bandwidth End-Effector With Active Force Control for Robotic Polishing. IEEE Access 2020, 8, 169122–169135. [Google Scholar] [CrossRef]
- El Khalick Mohammad, A.; Hong, J.; Wang, D. Design of a Force-Controlled End-Effector with Low-Inertia Effect for Robotic Polishing Using Macro-Mini Robot Approach. Robot. Comput.-Integr. Manuf. 2018, 49, 54–65. [Google Scholar] [CrossRef]
- Ma, Z.; Poo, A.-N.; Ang, M.H.; Hong, G.-S.; See, H.-H. Design and Control of an End-Effector for Industrial Finishing Applications. Robot. Comput.-Integr. Manuf. 2018, 53, 240–253. [Google Scholar] [CrossRef]
- Chen, F.; Zhao, H.; Li, D.; Chen, L.; Tan, C.; Ding, H. Contact Force Control and Vibration Suppression in Robotic Polishing with a Smart End Effector. Robot. Comput.-Integr. Manuf. 2019, 57, 391–403. [Google Scholar] [CrossRef]
- Yu, Y.; Wang, R.; Wang, Y.; Sun, Y. Contact Force Controlled Robotic Polishing for Complex PMMA Parts with an Active End-Effector. J. Adv. Manuf. Sci. Technol. 2021, 1, 2021012-0. [Google Scholar] [CrossRef]
- Zhang, X.; Sun, Y. Development of Pneumatic Force-Controlled Actuator for Automatic Robot Polishing Complex Curved PMMA Parts. Machines 2023, 11, 446. [Google Scholar] [CrossRef]
- Grochała, D.; Dudzińska, S.; Bachtiak-Radka, E.; Gubała, R. Micro-Hydraulic Burnishing Tools for Integrated Machining on CNC Milling Center. AIP Conf. Proc. 2018, 2029, 020018. [Google Scholar] [CrossRef]
- Bachtiak-Radka, E.; Dudzińska, S.; Grochała, D.; Berczyński, S.; Olszak, W. The Influence of CNC Milling and Ball Burnishing on Shaping Complex 3D Surfaces. Surf. Topogr. Metrol. Prop. 2017, 5, 015001. [Google Scholar] [CrossRef]
- Mitropoulos, T.; Avrampos, P.; Vosniakos, G.C. Development of a Polishing Jig for Machining Centres. SSP 2017, 261, 167–172. [Google Scholar] [CrossRef]
- Ahn, J.; Park, S.; Sim, J.; Park, J. Dual-Channel EtherCAT Control System for 33-DOF Humanoid Robot TOCABI. IEEE Access 2023, 11, 44278–44286. [Google Scholar] [CrossRef]
- Chuang, W.-L.; Yeh, M.-H.; Yeh, Y.-L. Develop Real-Time Robot Control Architecture Using Robot Operating System and EtherCAT. Actuators 2021, 10, 141. [Google Scholar] [CrossRef]
- Wang, S.; Yang, X.; Geer, J.V.D. Development of EtherCAT Real-Time Control System for Robot Based on Simulink Real-Time. JCM 2021, 21, 49–57. [Google Scholar] [CrossRef]
- Li, J.; Li, L.; Zhou, B.; Zhao, J.; Zhu, G.; Wang, Z. A Robotic Multi-directional Polishing Trajectory Generation Method Based on Preston-PSO Algorithm. China Mech. Eng. 2023, 34, 1729–1740. [Google Scholar]
- Eghbal Ahmadi, M.H.; Royaee, S.J.; Tayyebi, S.; Bozorgmehry Boozarjomehry, R. A New Insight into Implementing Mamdani Fuzzy Inference System for Dynamic Process Modeling: Application on Flash Separator Fuzzy Dynamic Modeling. Eng. Appl. Artif. Intell. 2020, 90, 103485. [Google Scholar] [CrossRef]
- Dang, Q.V.; Allouche, B.; Dequidt, A.; Vermeiren, L.; Dubreucq, V. Real-Time Control of a Force Feedback Haptic Interface via EtherCAT Fieldbus. In Proceedings of the 2015 IEEE International Conference on Industrial Technology (ICIT), Seville, Spain, 17–19 March 2015; IEEE: Piscataway, NJ, USA; pp. 441–446. [Google Scholar]







| Parameters | Values |
|---|---|
| Total mass | 4.0 kg |
| Movable mass | 1.6 kg |
| Total length | 290 mm |
| Motion stroke | 10 mm |
| Polishing tool size | 76 mm |
| Sampling frequency | 1 kHz |
| Resolution of force sensor | 0.01 N |
| Force control range | 0–30 N |
| Parameters | Parameters | ||||
|---|---|---|---|---|---|
| Kp | m2/N | Preston coefficient | ka | coefficient of the force in the Z direction and normal polishing force | |
| P | N/m2 | pressure at the polishing point | z | m | axial displacement |
| V | m/s | instantaneous polishing velocity at the polishing point | Fi | N | axial thrust generated by the voice coil motor |
| dz/dt | m/s | material removal in unit time | Ff | N | friction damping force |
| Fp | N | polishing force | I | A | current passed through the coil |
| Fpn | N | normal polishing force | ki | N/A | voice coil motor force coefficient |
| Fpt | N | tangential polishing force | kf | N·s/m | frictional damping force coefficient |
| θ | degrees | complementary angle of friction angle | ks | N/m | elastic coefficient of the springs |
| Fx | N | force in the X direction | kz | N/m | elastic coefficient of the foam pan |
| Fz | N | force in the Z direction | G | N | gravity of the moving part of the device |
| α | degrees | contact angle | m | kg | mass of the moving part of the device |
| Process | Abrasive Size (μm) | Cycle | Feed Rate (mm/min) | Spindle Speed (r/min) |
|---|---|---|---|---|
| 1 | 60 | 1 | 600 | 1000 |
| 2 | 30 | |||
| 3 | 15 | |||
| 4 | 9 | |||
| 5 | 2.7 | 2 | ||
| 6 | 1.25 | 2 | ||
| 7 | Polishing liquid | 3 | 500 |
| Parameters | Values |
|---|---|
| Path interval | 10 mm |
| Contact angle α | 10° |
| Sensor sample time | 1 ms |
| Controller cycle time | 1 ms |
| Controller coefficient kp, ki, kfuzzy | 1, 0.05, 0.015 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Meng, X.; Wang, Y.; Yin, X.; Fu, H.; Sun, S.; Sun, Y. Investigation of Force-Controlled Polishing of Complex Curved PMMA Parts on a Machining Center. Machines 2024, 12, 259. https://doi.org/10.3390/machines12040259
Meng X, Wang Y, Yin X, Fu H, Sun S, Sun Y. Investigation of Force-Controlled Polishing of Complex Curved PMMA Parts on a Machining Center. Machines. 2024; 12(4):259. https://doi.org/10.3390/machines12040259
Chicago/Turabian StyleMeng, Xiangran, Yingpeng Wang, Xiaolong Yin, Haoyu Fu, Shuoxue Sun, and Yuwen Sun. 2024. "Investigation of Force-Controlled Polishing of Complex Curved PMMA Parts on a Machining Center" Machines 12, no. 4: 259. https://doi.org/10.3390/machines12040259
APA StyleMeng, X., Wang, Y., Yin, X., Fu, H., Sun, S., & Sun, Y. (2024). Investigation of Force-Controlled Polishing of Complex Curved PMMA Parts on a Machining Center. Machines, 12(4), 259. https://doi.org/10.3390/machines12040259






