Vibration Characteristics of Asymmetric Flexible Cantilever Beams Connected to a Central Rigid Body
Abstract
1. Introduction
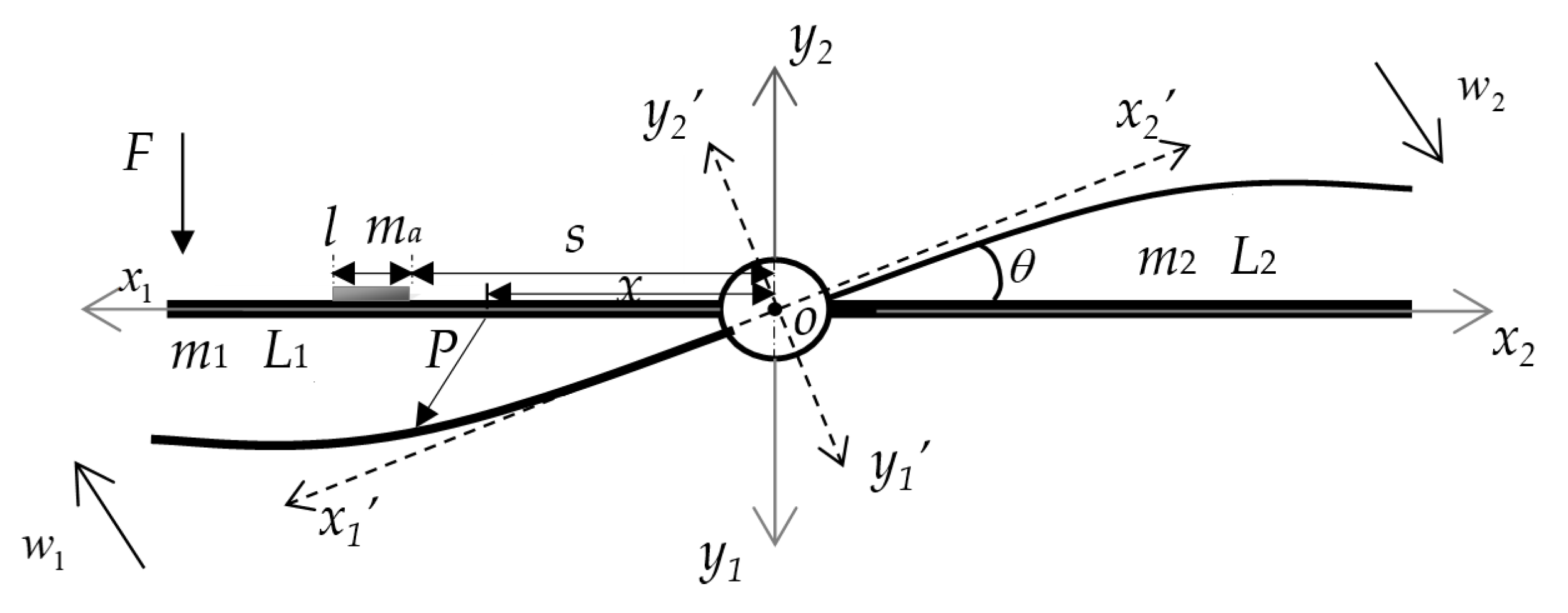
2. Establishment and Solution of Structural Equation of Motion
2.1. Establishment of Equation of Motion
2.2. Theoretical Solutions
2.2.1. Forced Vibration
2.2.2. Free Vibration
3. Validations
3.1. Validation by the FEM
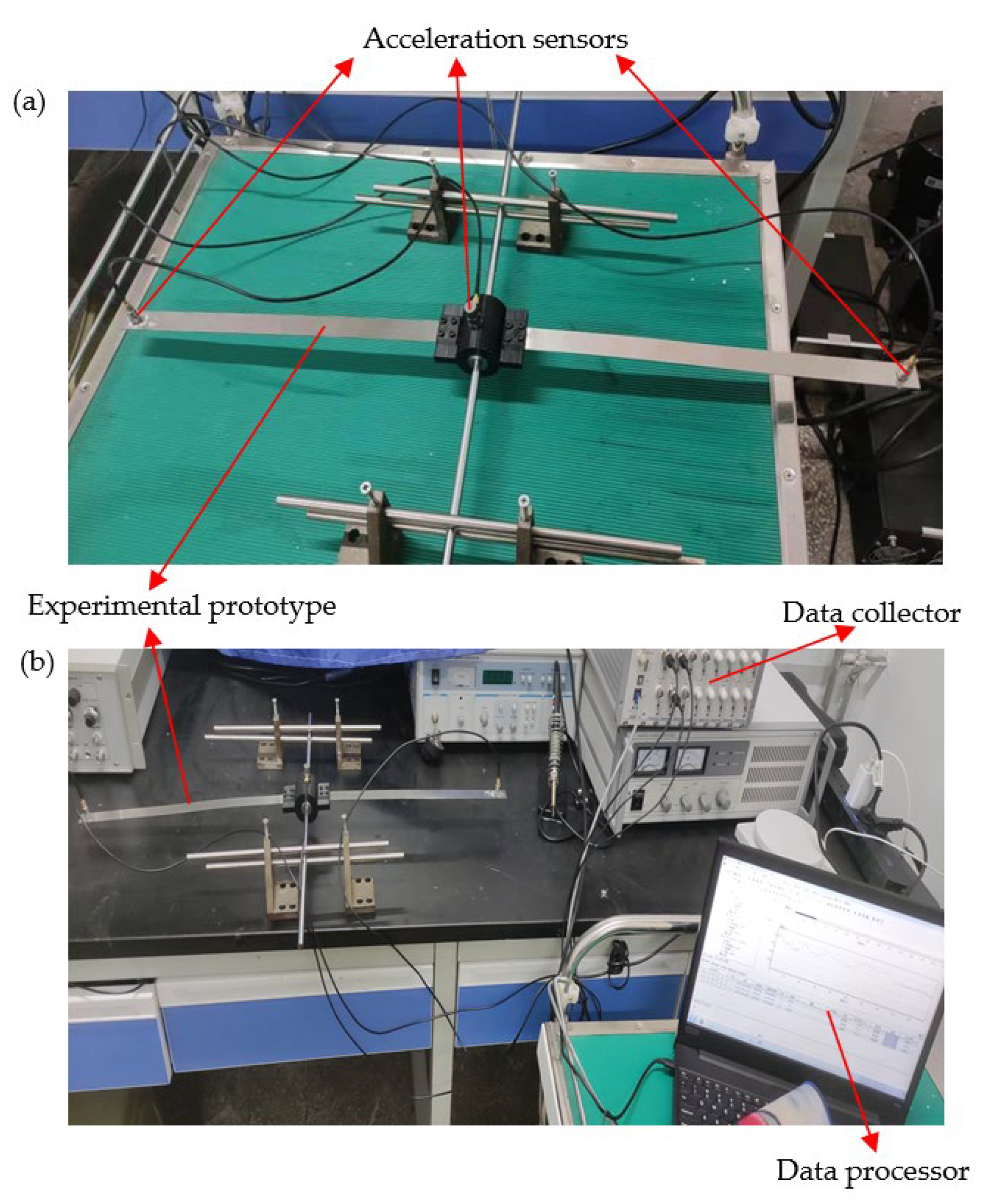
3.2. Validation via the Vibration Experiment
4. Numerical Results and Discussions
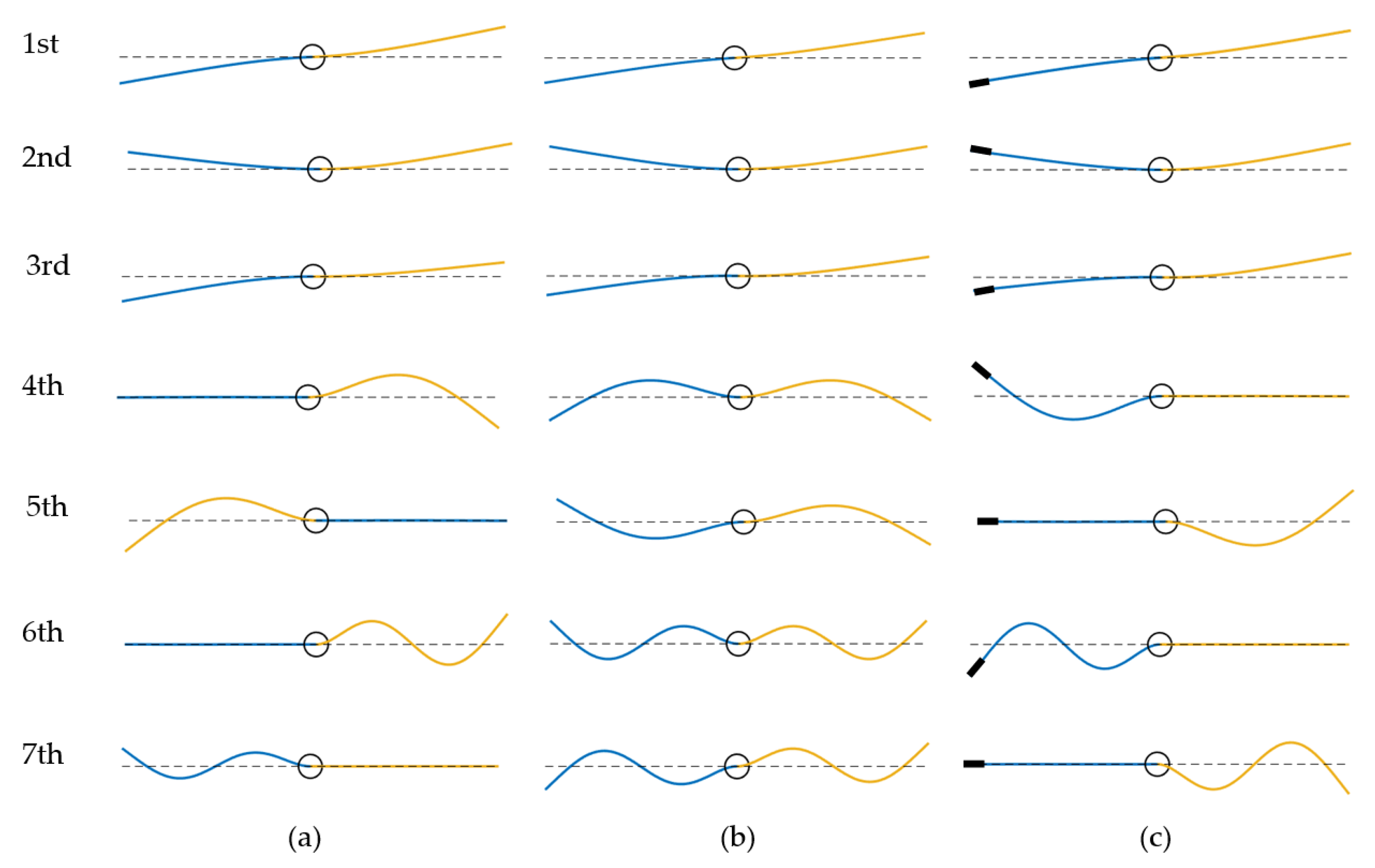
4.1. Natural Frequencies and Mode Shapes
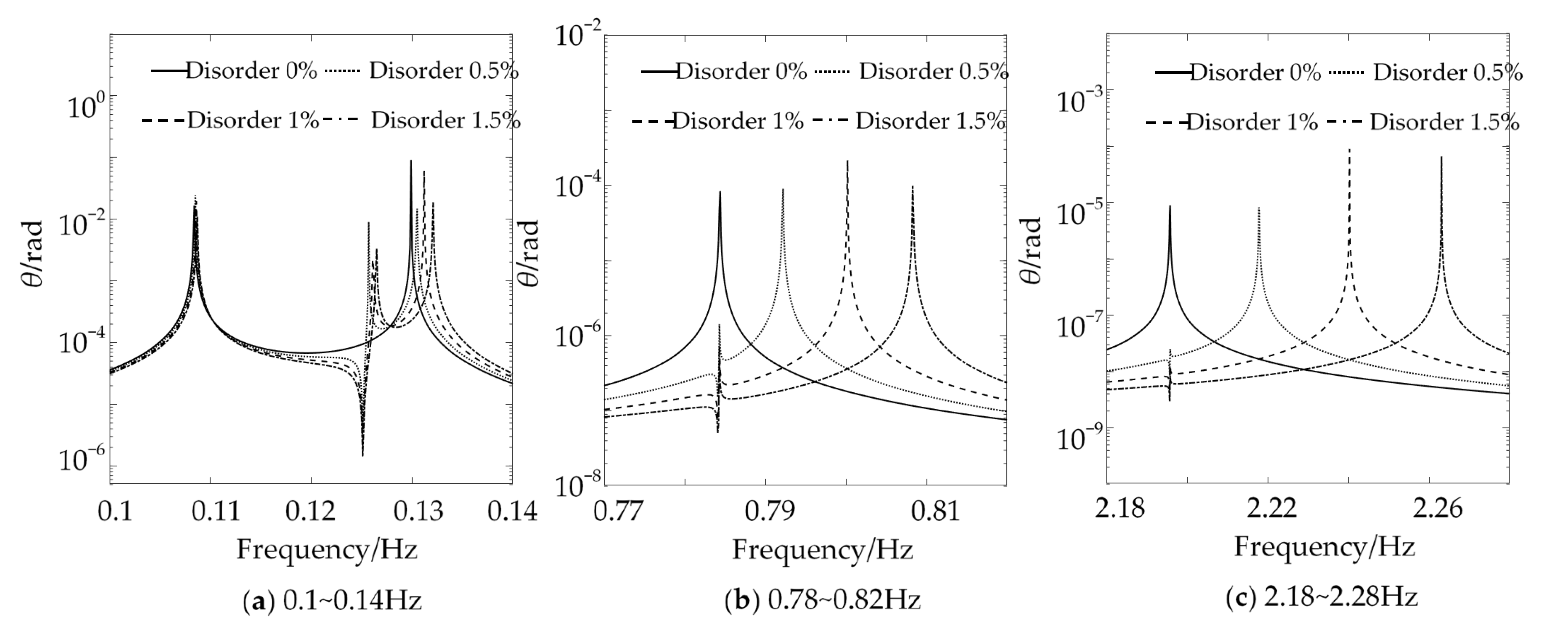
4.1.1. Influence of Mass Disorder
4.1.2. Influence of Other Parameters
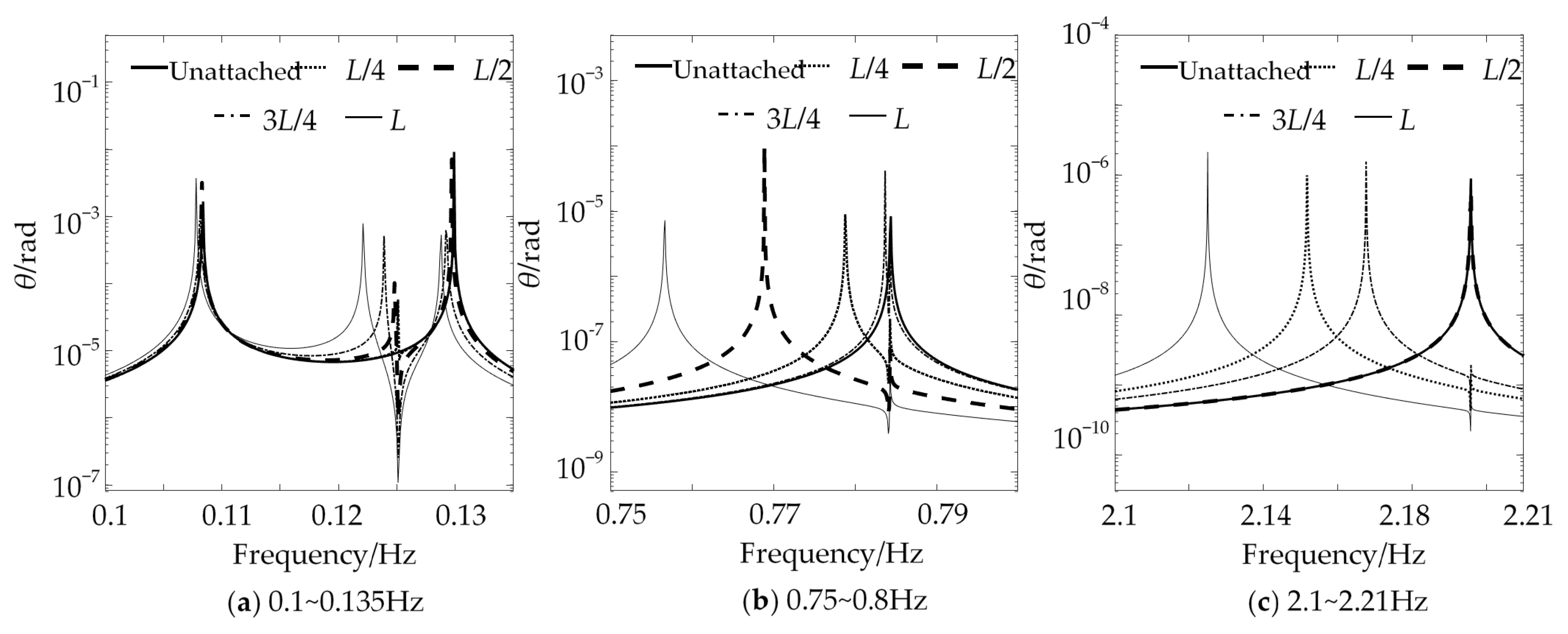
4.2. Forced Vibration
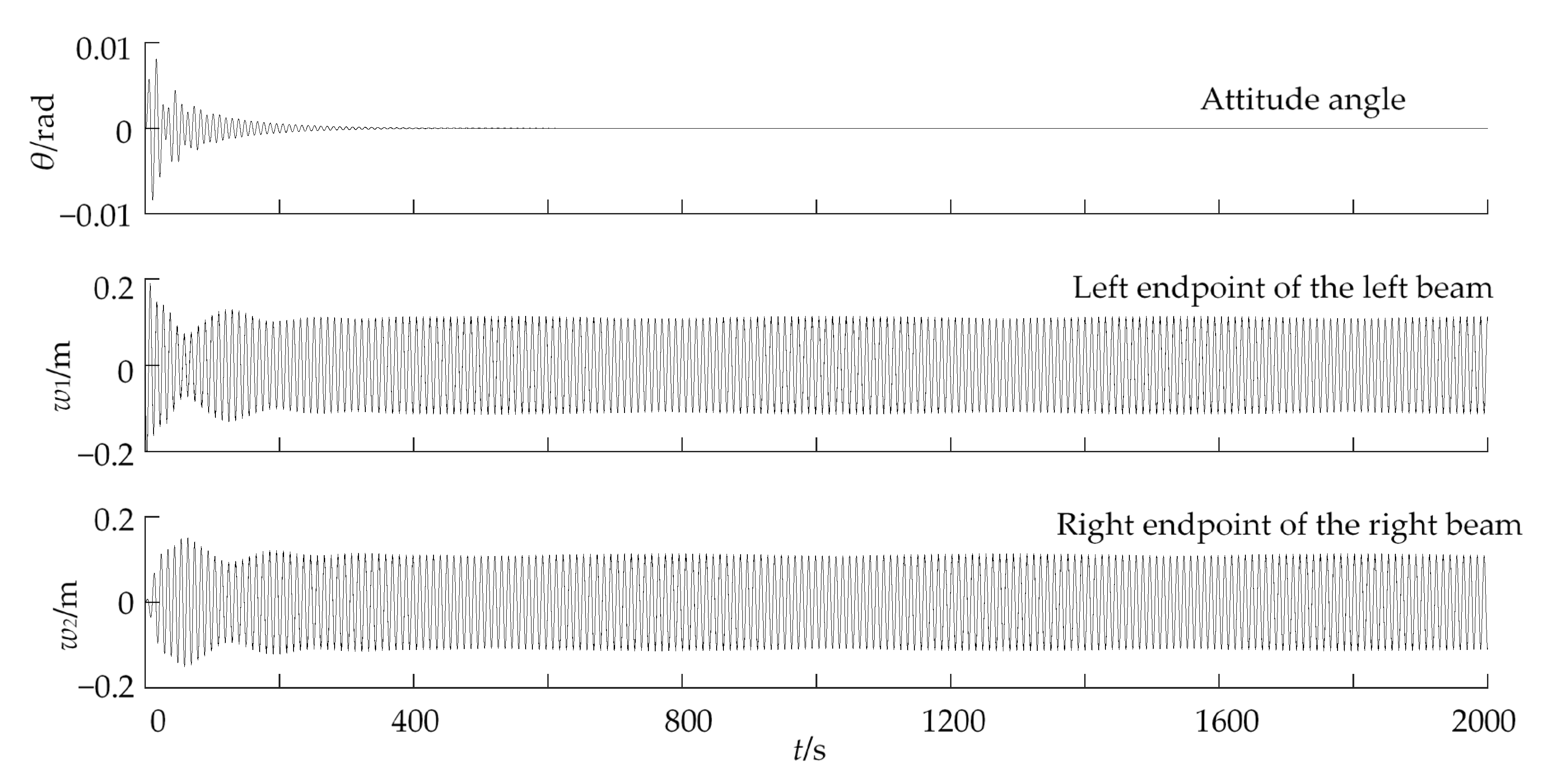
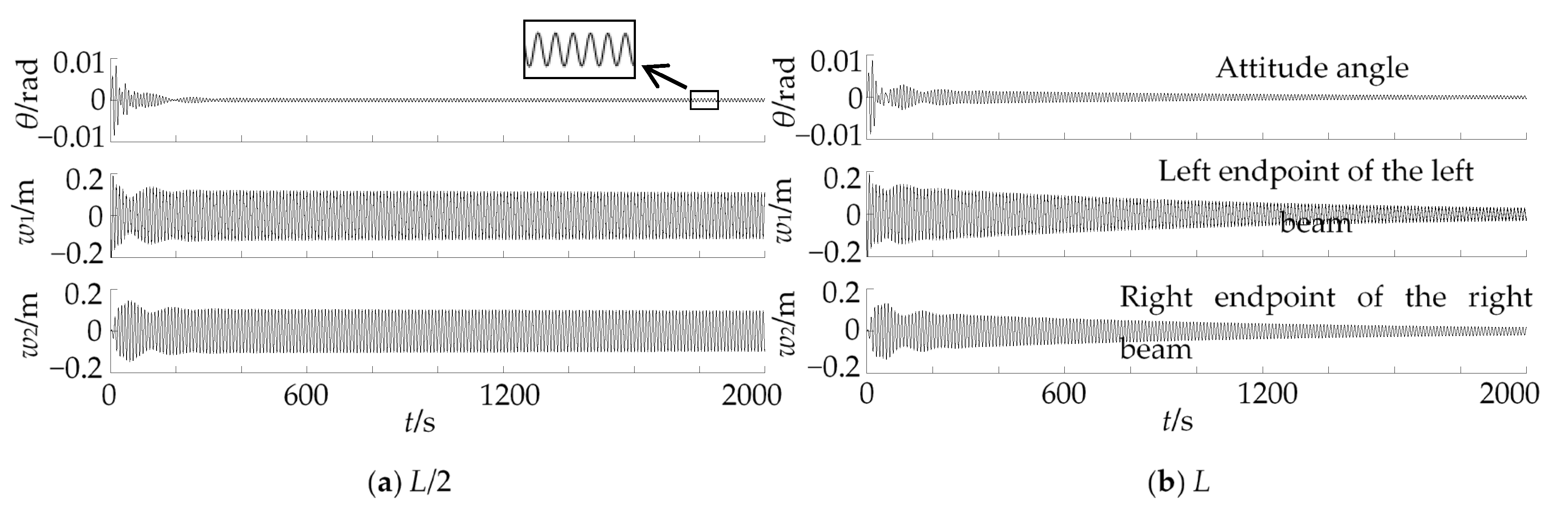
4.3. Free Vibration
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Qiu, Z.; Zhang, X.; Wu, H.; Zhang, H. Optimal placement and active vibration control for piezoelectric smart flexible cantilever plate. J. Sound Vib. 2007, 301, 521–543. [Google Scholar] [CrossRef]
- Shen, Z.; Tian, Q.; Liu, X.; Hu, G. Thermally induced vibrations of flexible beams using absolute nodal coordinate formulation. Aerosp. Sci. Technol. 2013, 29, 386–393. [Google Scholar] [CrossRef]
- Fu, Y. Neural network–based active control of a rigid-flexible spacecraft with bounded input. J. Vib. Control 2023. [Google Scholar] [CrossRef]
- Ghorbani, H.; Vatankhah, R.; Farid, M. General planar motion modeling and control of a smart rigid-flexible satellite considering large deflections. Nonlinear Dyn. 2022, 108, 911–939. [Google Scholar] [CrossRef]
- Gao, X.; Jin, D.; Chen, T. Nonlinear analysis and experimental investigation of a rigid-flexible antenna system. Meccanica 2018, 53, 33–48. [Google Scholar] [CrossRef]
- Azimi, M.; Joubaneh, E.F. Dynamic modeling and vibration control of a coupled rigid-flexible high-order structural system: A comparative study. Aerosp. Sci. Technol. 2020, 102, 105875. [Google Scholar] [CrossRef]
- Yoo, H.H.; Shin, S.H. Vibration analysis of rotating cantilever beams. J. Sound Vib. 1998, 212, 807–828. [Google Scholar] [CrossRef]
- Yang, J.B.; Jiang, L.J.; Chen, D.C.H. Dynamic modelling and control of a rotating Euler–Bernoulli beam. J. Sound Vib. 2004, 274, 863–875. [Google Scholar] [CrossRef]
- Tian, J.; Su, J.; Zhou, K.; Hua, H. A modified variational method for nonlinear vibration analysis of rotating beams including Coriolis effects. J. Sound Vib. 2018, 426, 258–277. [Google Scholar] [CrossRef]
- Cao, Y.; Cao, D.; Liu, L.; Huang, W. Dynamical modeling and attitude analysis for the spacecraft with lateral solar arrays. Appl. Math. Model. 2018, 64, 489–509. [Google Scholar] [CrossRef]
- Xing, W.; Wang, Y. Vibration characteristics analysis of rigid-flexible spacecraft with double direction hinged solar arrays. Acta Astronaut. 2022, 193, 454–468. [Google Scholar] [CrossRef]
- Yu, G.L.; Wang, Y.S.; Lan, J. Vibration localization in disordered periodically stiffened double-leaf panels. Arch. Appl. Mech. 2010, 80, 687–697. [Google Scholar] [CrossRef]
- Yousefzadeh, B.; Phani, A.S. Supratransmission in a disordered nonlinear periodic structure. J. Sound Vib. 2016, 380, 242–266. [Google Scholar] [CrossRef]
- Hao, S.; Wu, Z.; Li, F.; Zhang, C. Enhancement of the band-gap characteristics in disordered elastic metamaterial multi-span beams: Theory and experiment. Mech. Res. Commun. 2021, 113, 103692. [Google Scholar] [CrossRef]
- Ding, L.; Zhu, H.P.; Wu, Q.Y. Seismic response and vibration transmission characteristics of laminated rubber bearings with single disorder. J. Eng. Mech. 2019, 145, 04019093. [Google Scholar] [CrossRef]
- Thomes, R.L.; Mosquera-Sánchez, J.A.; De Marqui, C. Bandgap widening by optimized disorder in one-dimensional locally resonant piezoelectric metamaterials. J. Sound Vib. 2021, 512, 116369. [Google Scholar] [CrossRef]
- Fabro, A.; Meng, H.; Chronopoulos, D. Correlated disorder in rainbow metamaterials for vibration attenuation. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2021, 235, 2610–2621. [Google Scholar] [CrossRef]
- Liu, Y.; Han, C.; Liu, D. Broadband vibration suppression of graded/disorder piezoelectric metamaterials. Mech. Adv. Mater. Struct. 2023, 30, 710–723. [Google Scholar] [CrossRef]
- Laxalde, D.; Pierre, C. Modelling and analysis of multi-stage systems of mistuned bladed disks. Comput. Struct. 2011, 89, 316–324. [Google Scholar] [CrossRef]
- Zhou, S.; Li, F.; Zhang, C. Vibration characteristics analysis of disordered two-span beams with numerical and experimental methods. J. Vib. Control 2018, 24, 3641–3657. [Google Scholar] [CrossRef]
- Machado, M.R.; Moura, B.B.; Dey, S.; Mukhopadhyay, T. Bandgap manipulation of single and multi-frequency smart metastructures with random impedance disorder. Smart Mater. Struct. 2022, 31, 105020. [Google Scholar] [CrossRef]
- Sun, H.; Yuan, H. Mistuning parameter identification and vibration localization analysis of the integration rotor. Proc. Inst. Mech. Eng. Part G J. Aerosp. Eng. 2022, 236, 238–253. [Google Scholar] [CrossRef]
- Zhao, T.; Yang, Z.; Xu, Y.; Tian, W. Mode localization in metastructure with T-type resonators for broadband vibration suppression. Eng. Struct. 2022, 268, 114775. [Google Scholar] [CrossRef]
- She, H.; Li, C.; Zhang, G.; Tang, Q. Statistical investigation on the coupling mode characteristics of a blade-disk-shaft unit. Mech. Based Des. Struct. Mach. 2023, 51, 4237–4254. [Google Scholar] [CrossRef]
- Jana, K.; Haldar, S. Effect of grading pattern on free vibration analysis of FGM plates carrying concentrated and distributed mass. Mech. Based Des. Struct. Mach. 2023. [Google Scholar] [CrossRef]
- Deng, S.T.; Pang, J.; Zhang, Z.; Yang, L.; Li, X.Q.; Dai, H.L. Investigation on characteristics of energy transfer of flexural vibration in a Y-shaped bifurcated beam imposed lumped mass. Appl. Math. Model. 2021, 92, 486–504. [Google Scholar] [CrossRef]
- Chen, C.; Zhou, J.W.; Li, F.; Gong, D. Nonlinear vortex-induced vibration of wind turbine towers: Theory and experimental validation. Mech. Syst. Signal Process. 2023, 204, 110772. [Google Scholar] [CrossRef]
- Jia, T.; Li, C.; Pan, S.; Wang, Y. Investigation of vibration natural characteristics and response for rotating beam with tenon jointed structure under thermal environment. J. Sound Vib. 2023, 560, 117800. [Google Scholar] [CrossRef]
- Nesarhosseini, S.; Ansari, R.; Oskouie, M.F.; Rouhi, H. Thermally induced vibration analysis of Timoshenko beams based on the micropolar thermoelasticity. Acta Mech. 2023, 234, 1957–1971. [Google Scholar] [CrossRef]
- Wu, Z.; Wu, Z.; Lu, F.; Zhang, C.; Liu, Z.; Zhu, Y. Free vibration analysis and multi-objective optimization of lattice sandwich beams. Mech. Adv. Mater. Struct. 2023. [Google Scholar] [CrossRef]


| Modal Order | 1st | 2nd | 3rd | 4th | 5th | 6th | 7th |
|---|---|---|---|---|---|---|---|
| Present method (Hz) | 0.1078 | 0.1221 | 0.1288 | 0.7567 | 0.7843 | 2.1250 | 2.1957 |
| COMSOL (Hz) | 0.1078 | 0.1221 | 0.1288 | 0.7566 | 0.7843 | 2.1225 | 2.1957 |
| Modal Order | 1st | 2nd | 3rd | 4th | 5th | 6th |
|---|---|---|---|---|---|---|
| Present method (Hz) | 4.985 | 23.54 | 36.31 | 73.62 | 108.3 | 138.4 |
| Experiment (Hz) | 4.883 | 20.02 | 37.11 | 67.11 | 113.8 | 141.6 |
| Disorder Degree | 1st | 2nd | 3rd | 4th | 5th | 6th | 7th |
|---|---|---|---|---|---|---|---|
| 0% | 0.1084 | 0.1251 | 0.1299 | 0.7842 | 0.7843 | 2.1957 | 2.1958 |
| 0.5% | 0.1085 | 0.1257 | 0.1305 | 0.7843 | 0.7922 | 2.1957 | 2.2178 |
| 1% | 0.1086 | 0.1261 | 0.1312 | 0.7843 | 0.8002 | 2.1957 | 2.2403 |
| 1.5% | 0.1087 | 0.1265 | 0.1321 | 0.7843 | 0.8083 | 2.1957 | 2.2631 |
| Different Locations | 1st | 2nd | 3rd | 4th | 5th | 6th | 7th |
|---|---|---|---|---|---|---|---|
| Unattached | 0.1084 | 0.1251 | 0.1299 | 0.7842 | 0.7843 | 2.1957 | 2.1958 |
| L/4 | 0.1084 | 0.1251 | 0.1299 | 0.7788 | 0.7843 | 2.1517 | 2.1957 |
| L/2 | 0.1083 | 0.1248 | 0.1297 | 0.7689 | 0.7843 | 2.1957 | 2.1957 |
| 3L/4 | 0.1081 | 0.1239 | 0.1292 | 0.7837 | 0.7843 | 2.1676 | 2.1957 |
| L | 0.1078 | 0.1221 | 0.1288 | 0.7567 | 0.7843 | 2.1250 | 2.1957 |
| Deviation of IB (%) | 1st | 2nd | 3rd | 4th | 5th | 6th | 7th |
|---|---|---|---|---|---|---|---|
| −20 | 0.1162 | 0.1251 | 0.1358 | 0.7842 | 0.7844 | 2.1957 | 2.1958 |
| −10 | 0.1125 | 0.1251 | 0.1320 | 0.7842 | 0.7844 | 2.1957 | 2.1958 |
| 0 | 0.1084 | 0.1251 | 0.1299 | 0.7842 | 0.7843 | 2.1957 | 2.1958 |
| 10 | 0.1042 | 0.1251 | 0.1287 | 0.7842 | 0.7843 | 2.1957 | 2.1958 |
| 20 | 0.1003 | 0.1251 | 0.1279 | 0.7842 | 0.7843 | 2.1957 | 2.1958 |
| Deviation of kθ (%) | 1st | 2nd | 3rd | 4th | 5th | 6th | 7th |
|---|---|---|---|---|---|---|---|
| −20 | 0.0982 | 0.1251 | 0.1282 | 0.7842 | 0.7843 | 2.1957 | 2.1958 |
| −10 | 0.1036 | 0.1251 | 0.1289 | 0.7842 | 0.7843 | 2.1957 | 2.1958 |
| 0 | 0.1084 | 0.1251 | 0.1299 | 0.7842 | 0.7843 | 2.1957 | 2.1958 |
| 10 | 0.1125 | 0.1251 | 0.1313 | 0.7842 | 0.7844 | 2.1957 | 2.1958 |
| 20 | 0.1157 | 0.1251 | 0.1333 | 0.7842 | 0.7844 | 2.1957 | 2.1958 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gong, D.; Wei, X.; Liu, H.; Li, F. Vibration Characteristics of Asymmetric Flexible Cantilever Beams Connected to a Central Rigid Body. Machines 2024, 12, 193. https://doi.org/10.3390/machines12030193
Gong D, Wei X, Liu H, Li F. Vibration Characteristics of Asymmetric Flexible Cantilever Beams Connected to a Central Rigid Body. Machines. 2024; 12(3):193. https://doi.org/10.3390/machines12030193
Chicago/Turabian StyleGong, Dehuang, Xueqian Wei, Hongli Liu, and Fengming Li. 2024. "Vibration Characteristics of Asymmetric Flexible Cantilever Beams Connected to a Central Rigid Body" Machines 12, no. 3: 193. https://doi.org/10.3390/machines12030193
APA StyleGong, D., Wei, X., Liu, H., & Li, F. (2024). Vibration Characteristics of Asymmetric Flexible Cantilever Beams Connected to a Central Rigid Body. Machines, 12(3), 193. https://doi.org/10.3390/machines12030193







