Modeling for Hysteresis Contact Behavior of Bolted Joint Interfaces with Memory Effect Penalty Constitution
Abstract
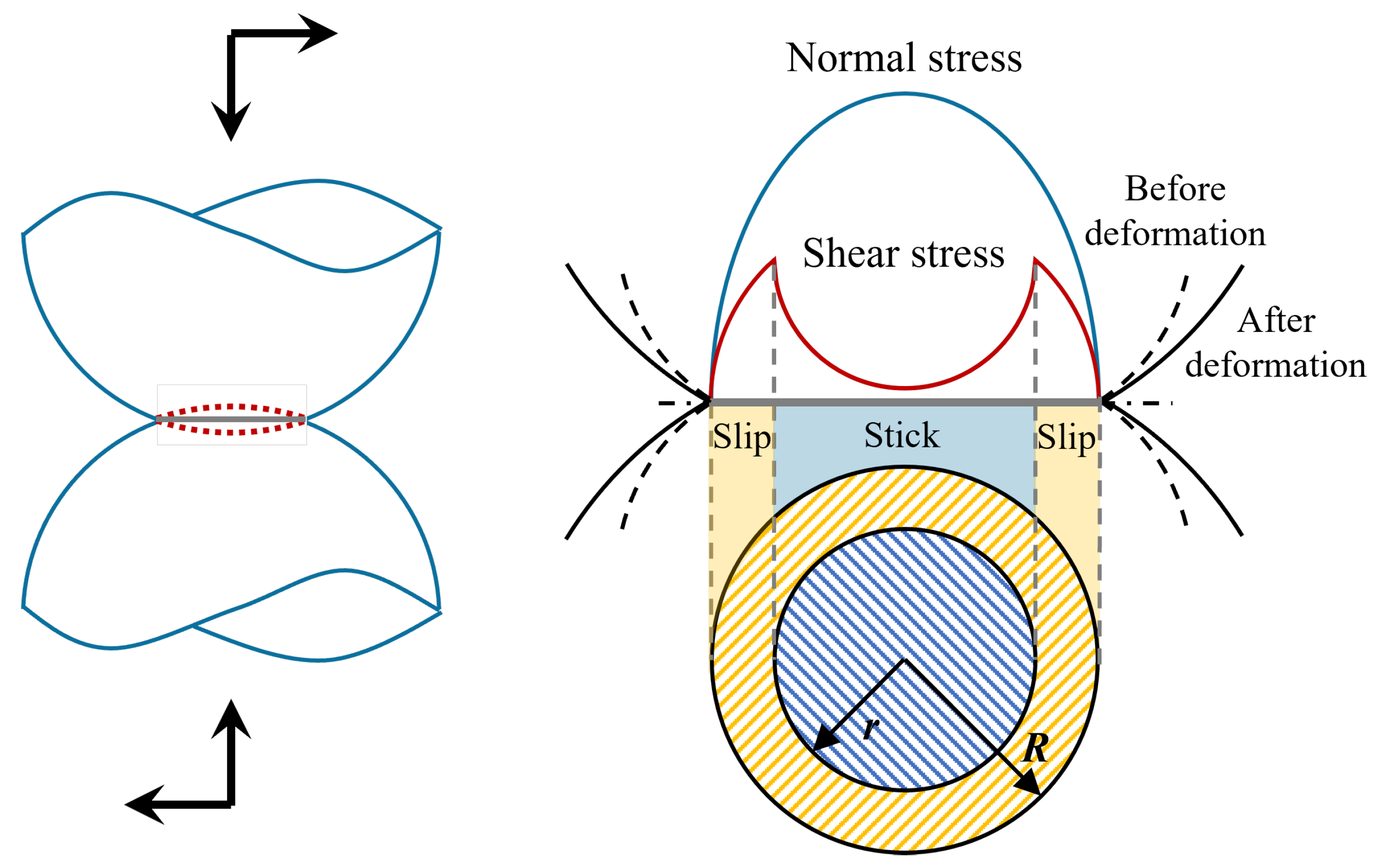
1. Introduction
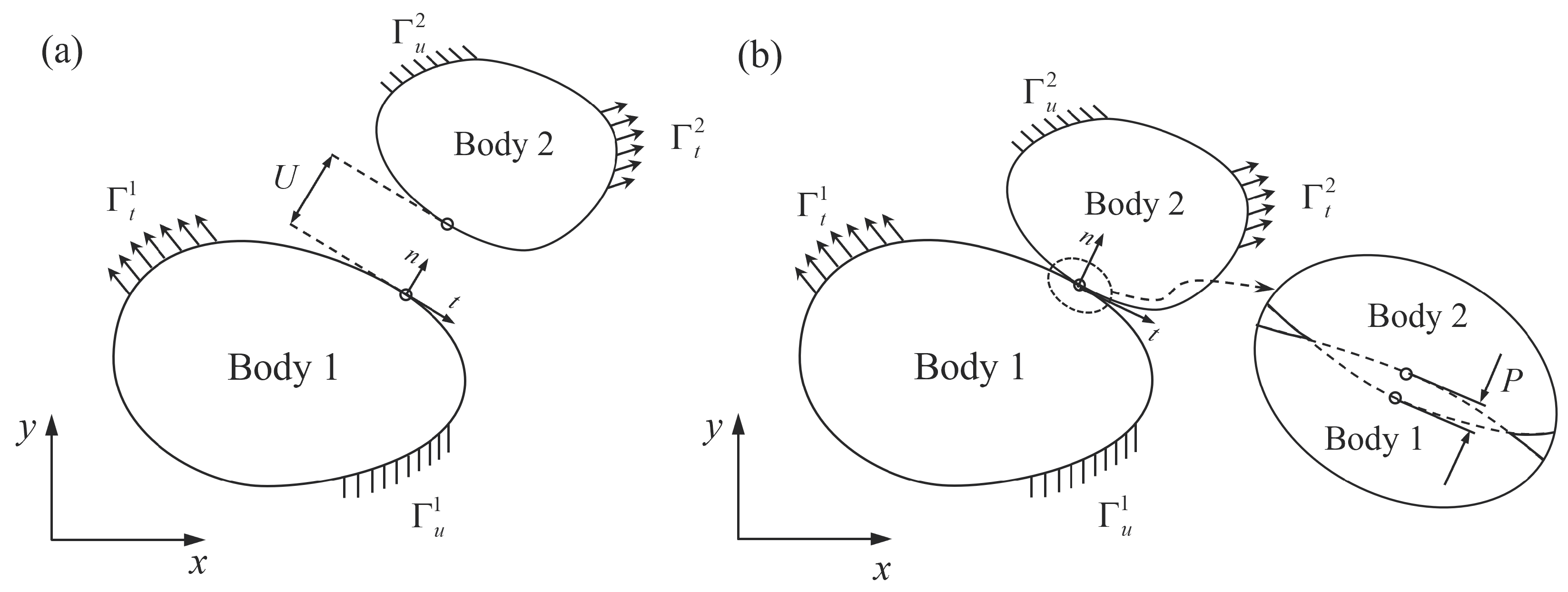
2. Contact Model for Joint Interfaces
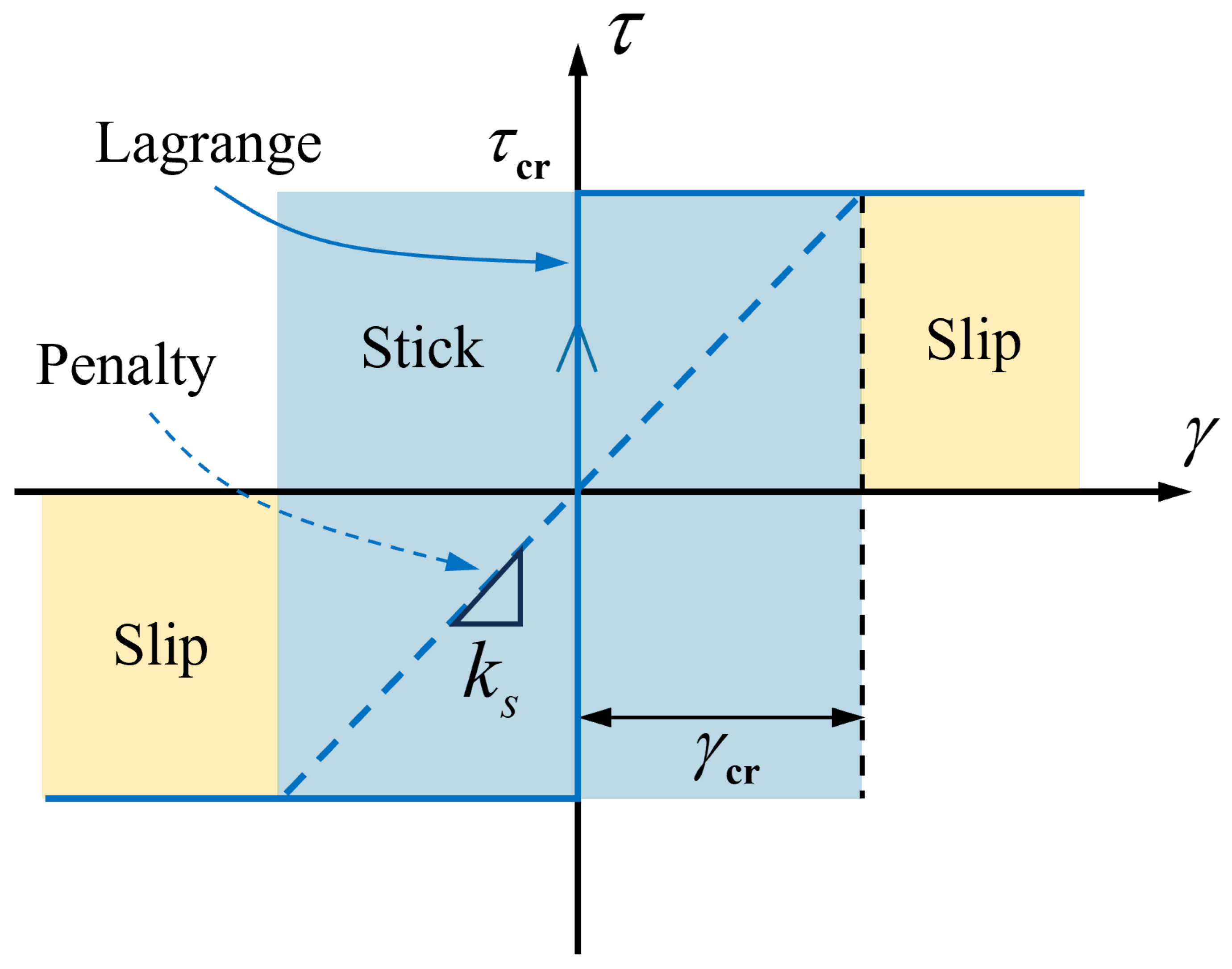
2.1. Standard Penalty Contact Method
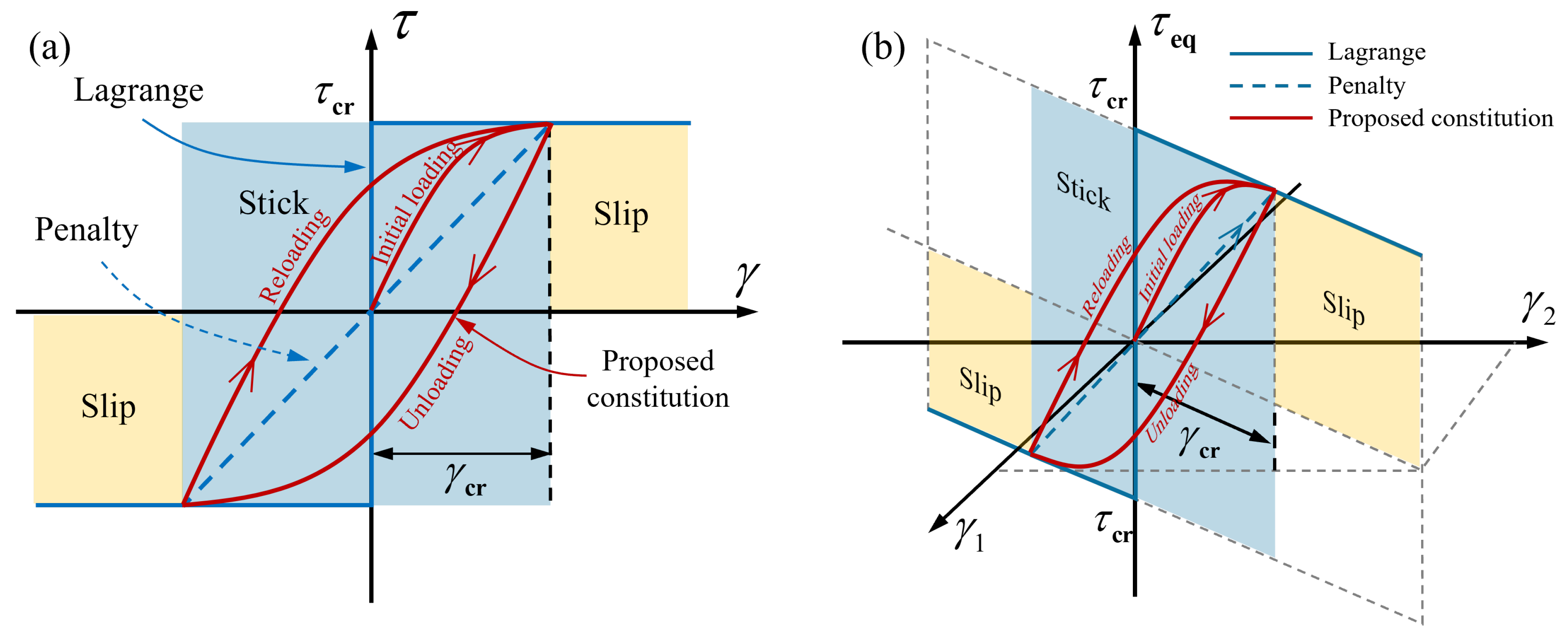
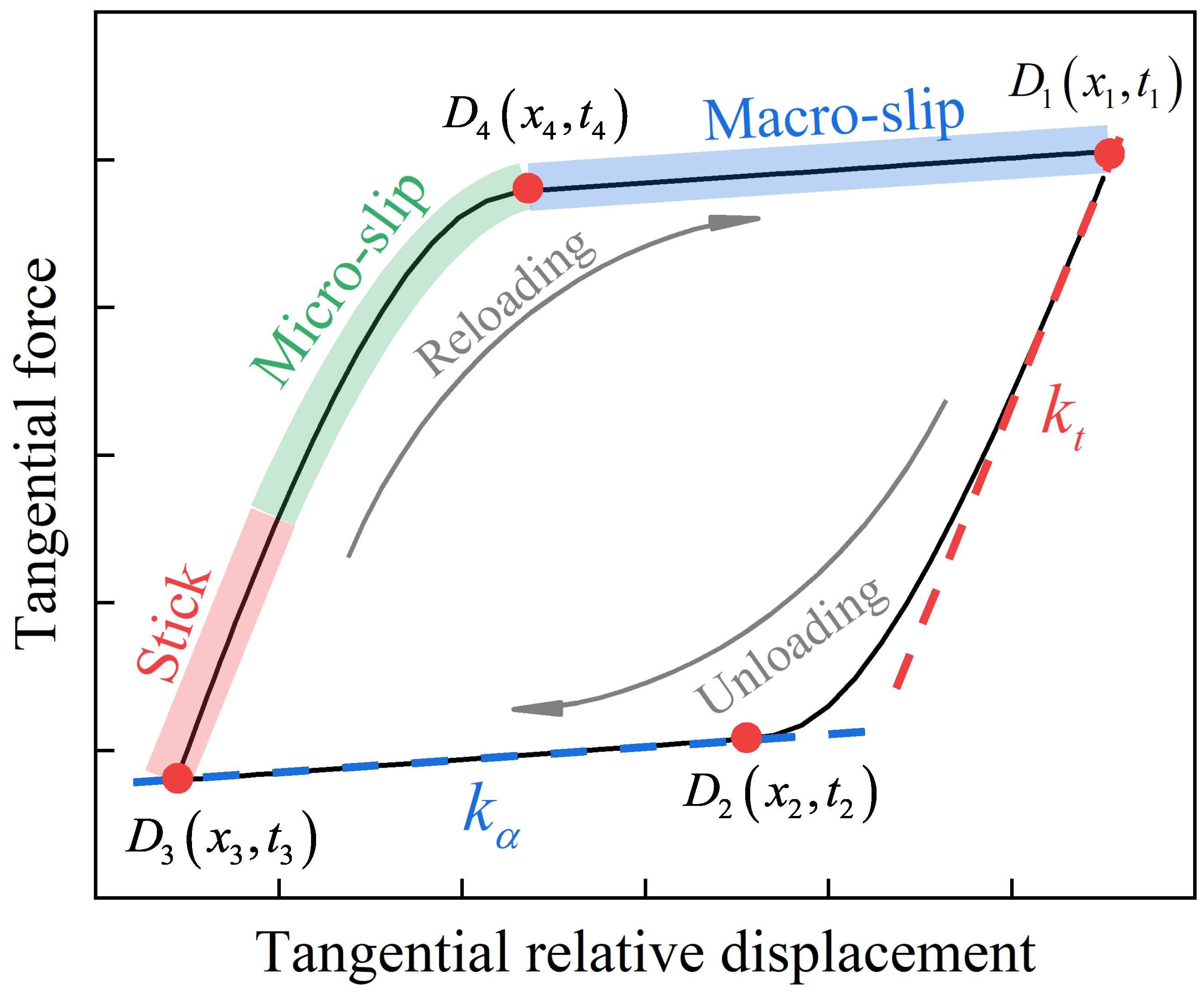
2.2. Memory Effect Penalty Constitution
- Judgment is performed to determine the contact state and the loading phase.
- Based on the loading phase, the equivalent tangential stress is calculated.
- A stick–slip contact state is determined based on the equivalent tangential stress.
- The contact deformation and pressure increments are calculated.
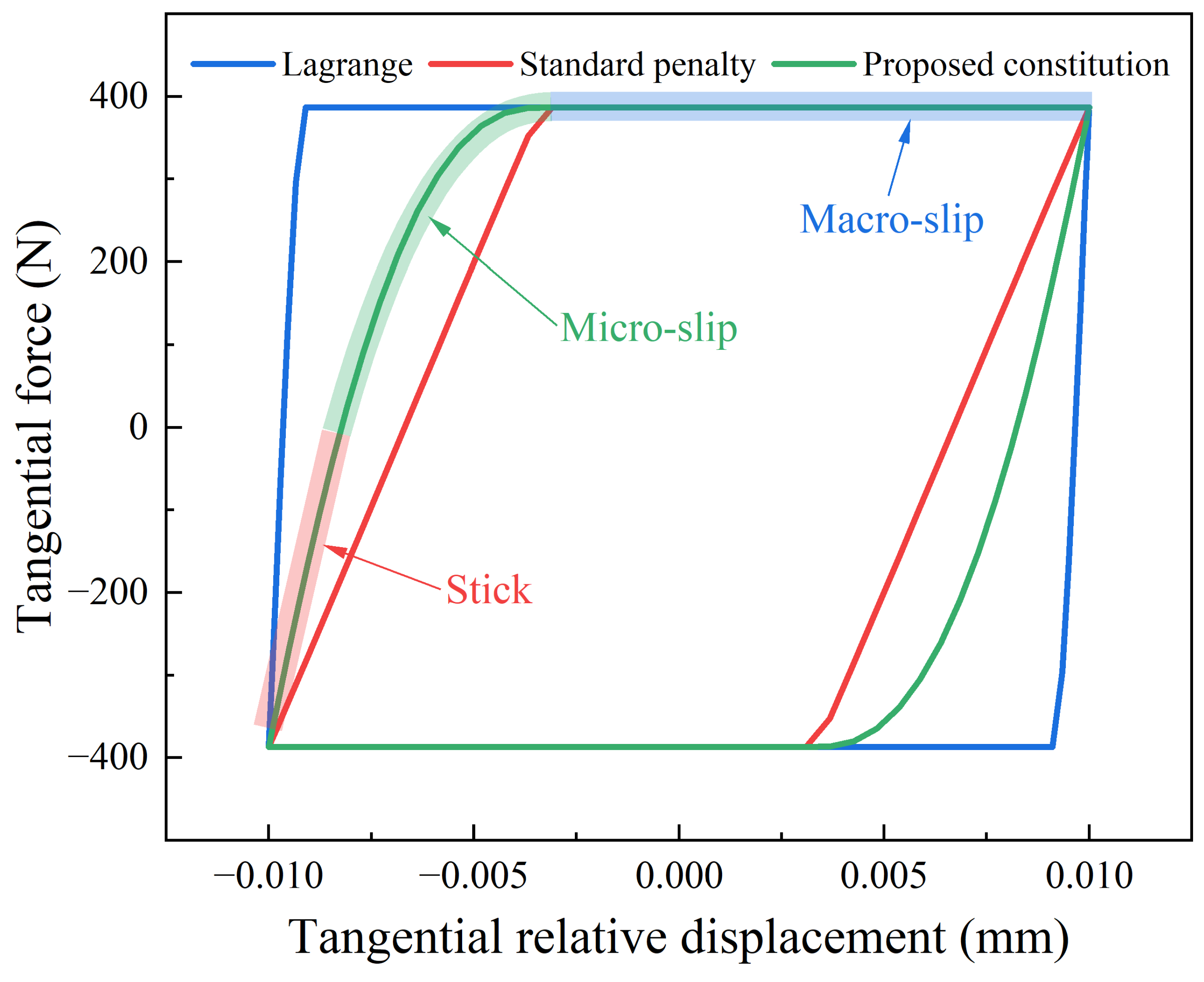
2.3. Comparison of Different Contact Methods
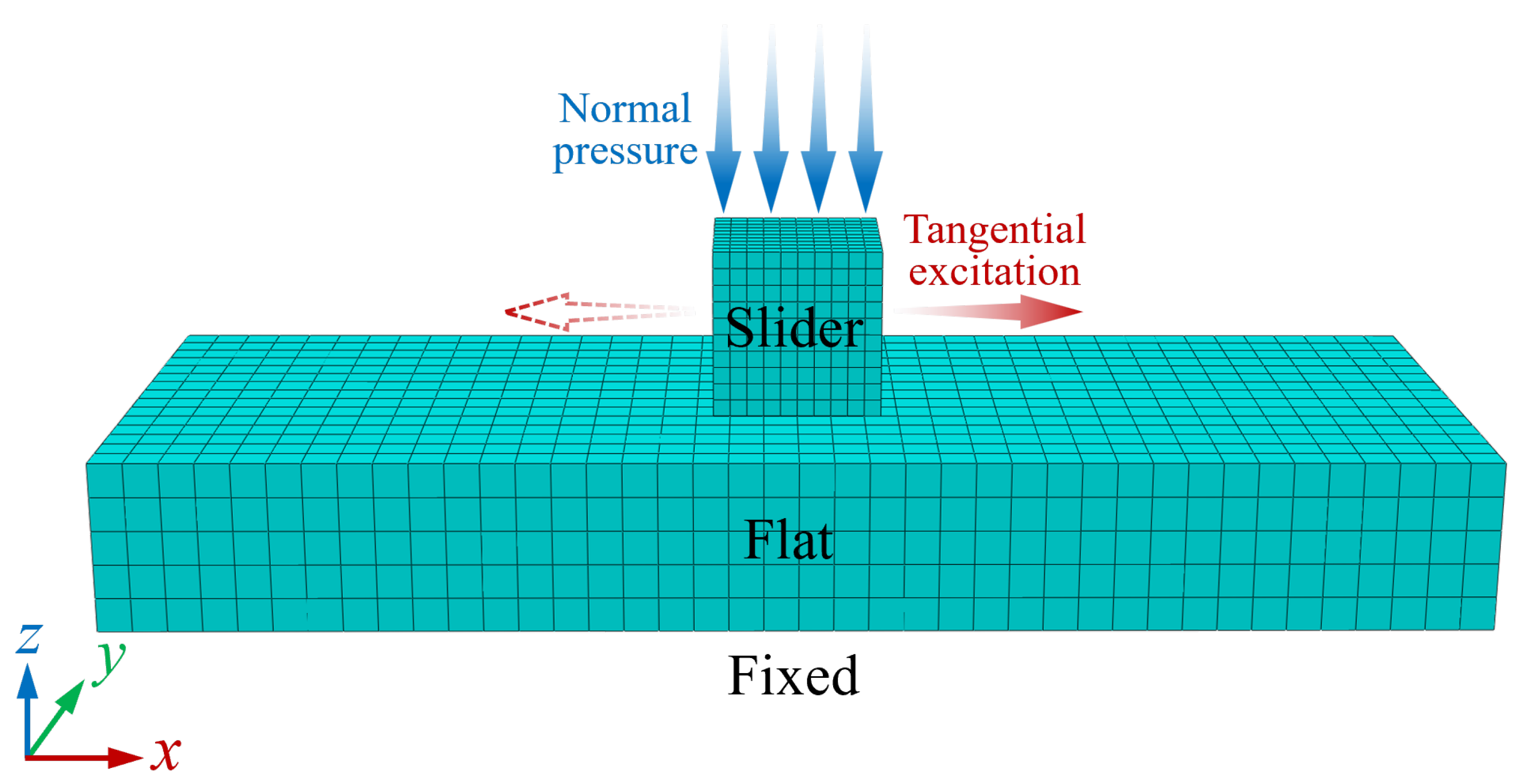
3. Refined FEA for Bolted Joint
3.1. Simulation Setup
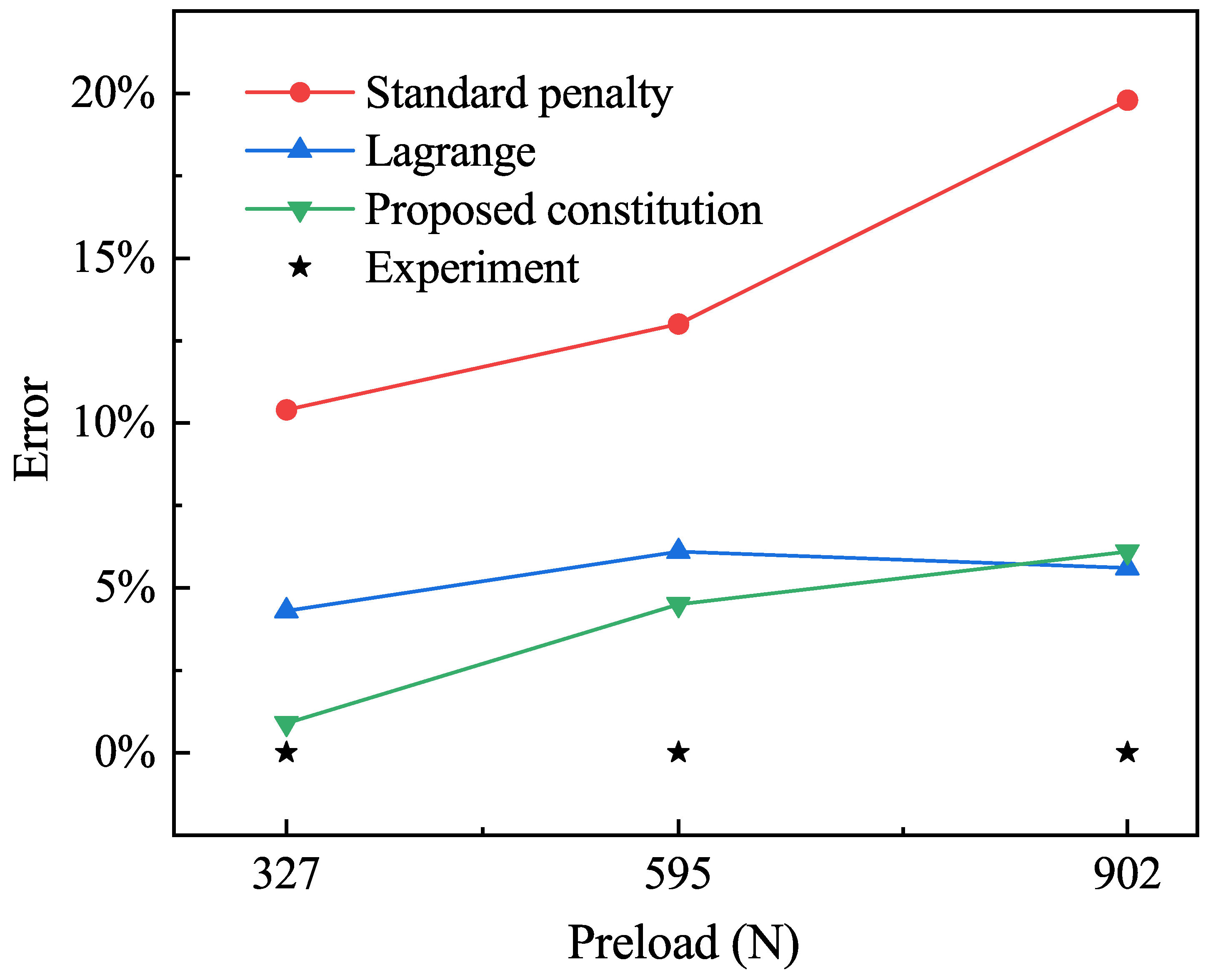
3.2. Model Validation
3.3. Parameter Investigation
4. Modeling for Bolted Joint Interfaces
4.1. Reduced-Order Models (ROMs)
4.2. Results and Discussion
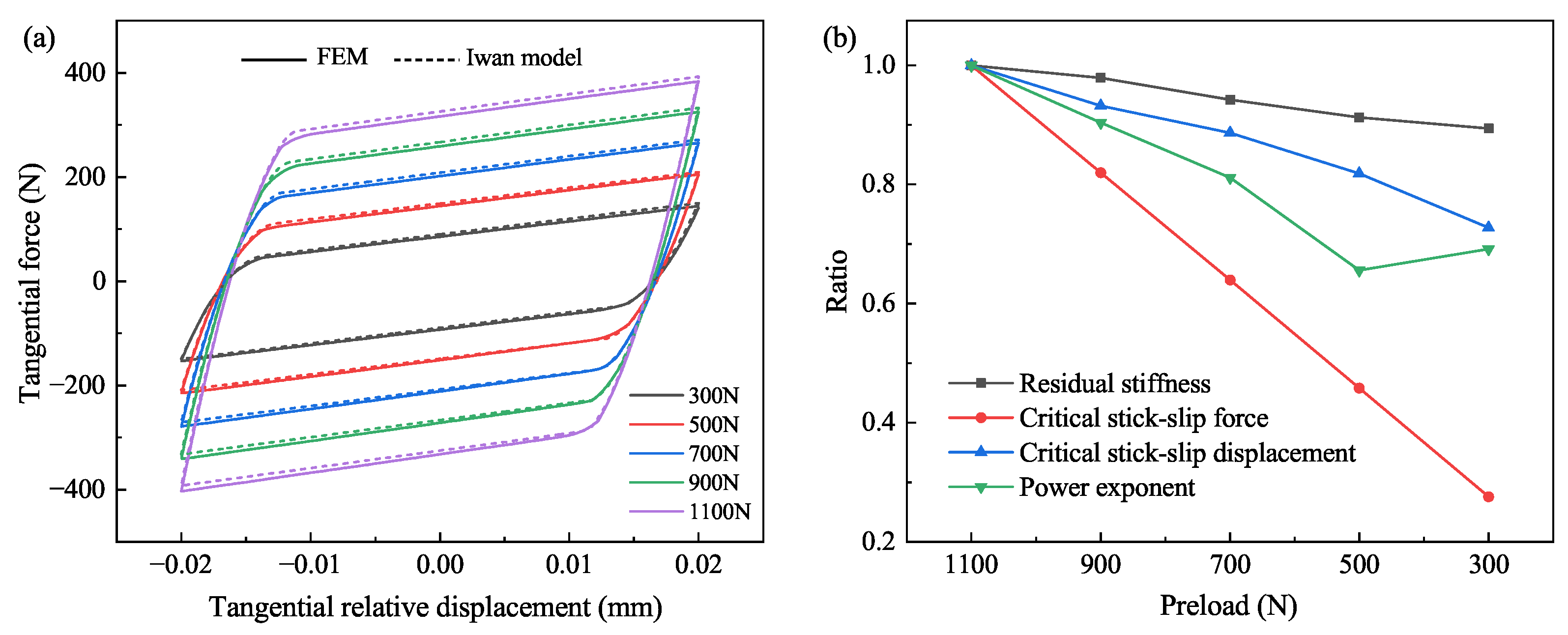
4.2.1. Effect of Bolt Preload
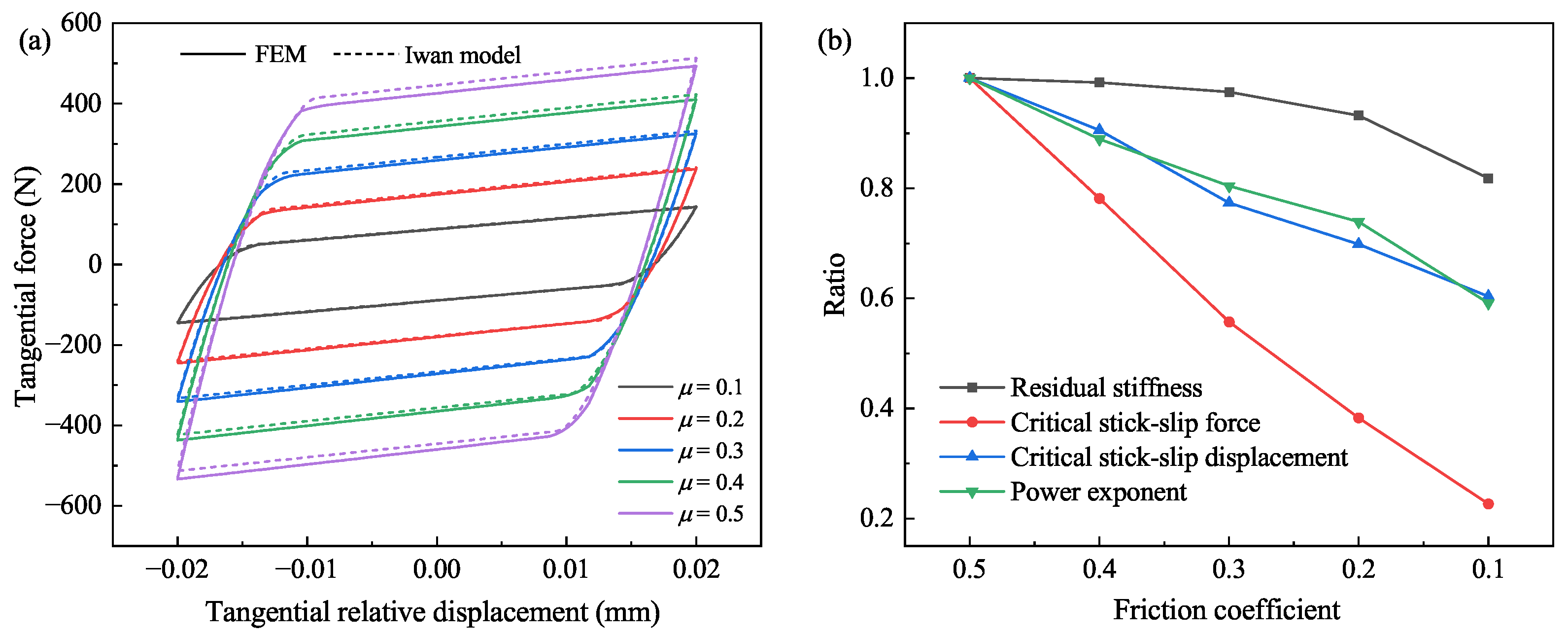
4.2.2. Effect of Friction Coefficients
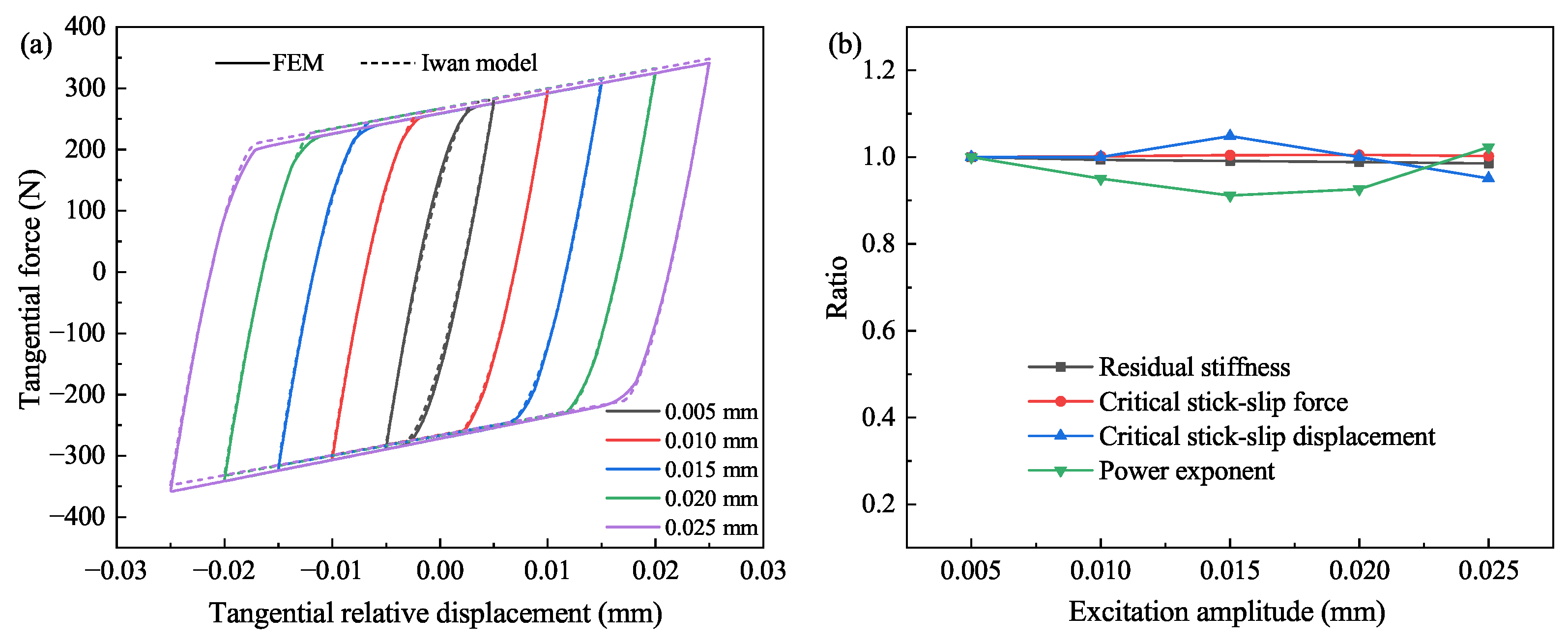
4.2.3. Effect of External Excitation Amplitudes
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Ibrahim, R.A.; Pettit, C.L. Uncertainties and dynamic problems of bolted joints and other fasteners. J. Sound Vib. 2005, 279, 857–936. [Google Scholar] [CrossRef]
- Gaul, L.; Nitsche, R. The role of friction in mechanical joints. Appl. Mech. Rev. 2001, 54, 93–106. [Google Scholar] [CrossRef]
- Li, D.; Botto, D.; Li, R.; Xu, C.; Zhang, W. Experimental and theoretical studies on friction contact of bolted joint interfaces. Int. J. Mech. Sci. 2022, 236, 107773. [Google Scholar] [CrossRef]
- Ranjan, P.; Pandey, A.K. Experimental characterization and parameter identification of bolted joints under vibratory loading. Tribol. Int. 2023, 186, 108636. [Google Scholar] [CrossRef]
- Eriten, M.; Polycarpou, A.A.; Bergman, L.A. Effects of surface roughness and lubrication on the early stages of fretting of mechanical lap joints. Wear 2011, 271, 2928–2939. [Google Scholar] [CrossRef]
- Estakhraji, S.I.Z.; Allen, M.S. Extension of the harmonic balance method for dynamic systems with Iwan joints. Mech. Syst. Signal Process. 2022, 166, 108434. [Google Scholar] [CrossRef]
- Bagheri Sabbagh, A.; Petkovski, M.; Pilakoutas, K.; Mirghaderi, R. Cyclic behaviour of bolted cold-formed steel moment connections: FE modelling including slip. J. Constr. Steel Res. 2013, 80, 100–108. [Google Scholar] [CrossRef]
- Li, Z.; Zhang, Y.; Li, C.; Xu, M.; Dai, W.; Liu, Z. Modeling and nonlinear dynamic analysis of bolt joints considering fractal surfaces. Nonlinear Dyn. 2022, 108, 1071–1099. [Google Scholar] [CrossRef]
- Wang, D.; Fan, X. Nonlinear dynamic modeling for joint interfaces by combining equivalent linear mechanics with multi-objective optimization. Acta Mech. Solida Sin. 2020, 33, 564–578. [Google Scholar] [CrossRef]
- Süß, D.; Willner, K. Investigation of a jointed friction oscillator using the multiharmonic balance method. Mech. Syst. Signal Process. 2015, 52–53, 73–87. [Google Scholar] [CrossRef]
- Mayer, M.H.; Gaul, L. Segment-to-segment contact elements for modelling joint interfaces in finite element analysis. Mech. Syst. Signal Process. 2007, 21, 724–734. [Google Scholar] [CrossRef]
- Bograd, S.; Reuss, P.; Schmidt, A.; Gaul, L.; Mayer, M. Modeling the dynamics of mechanical joints. Mech. Syst. Signal Process. 2011, 25, 2801–2826. [Google Scholar] [CrossRef]
- Yu, P.; Li, L.; Chen, G.; Yang, M. Dynamic modelling and vibration characteristics analysis for the bolted joint with spigot in the rotor system. Appl. Math. Model. 2021, 94, 306–331. [Google Scholar] [CrossRef]
- Lin, M.; Cheng, C.; Zhang, G.; Zhao, B.; Peng, Z.; Meng, G. Identification of Bouc-Wen hysteretic systems based on a joint optimization approach. Mech. Syst. Signal Process. 2022, 180, 109404. [Google Scholar] [CrossRef]
- Nassar, S.A.; Abboud, A. An improved stiffness model for bolted joints. J. Mech. Des. 2009, 131, 121001. [Google Scholar] [CrossRef]
- Sethuraman, R.; Sasi Kumar, T. Finite element based member stiffness evaluation of axisymmetric bolted joints. J. Mech. Des. 2008, 131, 011012. [Google Scholar] [CrossRef]
- Fu, W.P.; Huang, Y.M.; Zhang, X.L.; Guo, Q. Experimental investigation of dynamic normal characteristics of machined joint surfaces. J. Vib. Acoust. 2000, 122, 393–398. [Google Scholar] [CrossRef]
- Zhou, C.; Ren, Z.; Lin, Y.; Huang, Z.; Shi, L.; Yang, Y.; Mo, J. Hysteresis dynamic model of metal rubber based on higher-order nonlinear friction (HNF). Mech. Syst. Signal Process. 2023, 189, 110117. [Google Scholar] [CrossRef]
- Mathis, A.T.; Balaji, N.N.; Kuether, R.J.; Brink, A.R.; Brake, M.R.W.; Quinn, D.D. A review of damping models for structures with mechanical joints1. Appl. Mech. Rev. 2020, 72, 040802. [Google Scholar] [CrossRef]
- Iranzad, M.; Ahmadian, H. Identification of nonlinear bolted lap joint models. Comput. Struct. 2012, 96–97, 1–8. [Google Scholar] [CrossRef]
- Wang, D.; Zhang, Z. High-efficiency nonlinear dynamic analysis for joint interfaces with Newton–Raphson iteration process. Nonlinear Dyn. 2020, 100, 543–559. [Google Scholar] [CrossRef]
- Iwan, W.D. On a class of models for the yielding behavior of continuous and composite systems. J. Appl. Mech. 1967, 34, 612–617. [Google Scholar] [CrossRef]
- Brake, M.R.W. A reduced Iwan model that includes pinning for bolted joint mechanics. Nonlinear Dyn. 2017, 87, 1335–1349. [Google Scholar] [CrossRef]
- Bouc, R. Forced vibrations of mechanical systems with hysteresis. In Proceedings of the Fourth Conference on Nonlinear Oscillations, Prague, Czech Republic, 5–9 September 1967. [Google Scholar]
- Canudas de Wit, C.; Olsson, H.; Astrom, K.; Lischinsky, P. A new model for control of systems with friction. IEEE Trans. Autom. Control 1995, 40, 419–425. [Google Scholar] [CrossRef]
- Gaul, L.; Lenz, J. Nonlinear dynamics of structures assembled by bolted joints. Acta Mech. 1997, 125, 169–181. [Google Scholar] [CrossRef]
- Wang, D.; Zhang, Z. A four-parameter model for nonlinear stiffness of a bolted joint with non-Gaussian surfaces. Acta Mech. 2020, 231, 1963–1976. [Google Scholar] [CrossRef]
- Li, Y.; Hao, Z. A six-parameter Iwan model and its application. Mech. Syst. Signal Process. 2016, 68–69, 354–365. [Google Scholar] [CrossRef]
- Lacayo, R.; Pesaresi, L.; Groß, J.; Fochler, D.; Armand, J.; Salles, L.; Schwingshackl, C.; Allen, M.; Brake, M. Nonlinear modeling of structures with bolted joints: A comparison of two approaches based on a time-domain and frequency-domain solver. Mech. Syst. Signal Process. 2019, 114, 413–438. [Google Scholar] [CrossRef]
- Abad, J.; Medel, F.J.; Franco, J.M. Determination of Valanis model parameters in a bolted lap joint: Experimental and numerical analyses of frictional dissipation. Int. J. Mech. Sci. 2014, 89, 289–298. [Google Scholar] [CrossRef]
- Ranjan, P.; Pandey, A.K. Effect of misaligned plates and varying interfacial area on bolted structures. Int. J. Mech. Sci. 2022, 233, 107640. [Google Scholar] [CrossRef]
- Wang, D.; Xu, C.; Fan, X.; Wan, Q. Reduced-order modeling approach for frictional stick-slip behaviors of joint interface. Mech. Syst. Signal Process. 2018, 103, 131–138. [Google Scholar] [CrossRef]
- Zhao, B.; Wu, F.; Sun, K.; Mu, X.; Zhang, Y.; Sun, Q. Study on tangential stiffness nonlinear softening of bolted joint in friction-sliding process. Tribol. Int. 2021, 156, 106856. [Google Scholar] [CrossRef]
- Li, D.; Xu, C.; Botto, D.; Zhang, Z.; Gola, M. A fretting test apparatus for measuring friction hysteresis of bolted joints. Tribol. Int. 2020, 151, 106431. [Google Scholar] [CrossRef]
- Li, Q.; Jing, X. A second-order output spectrum approach for fault detection of bolt loosening in a satellite-like structure with a sensor chain. Nonlinear Dyn. 2017, 89, 587–606. [Google Scholar] [CrossRef]
- Liu, J.; Ouyang, H.; Feng, Z.; Cai, Z.; Liu, X.; Zhu, M. Study on self-loosening of bolted joints excited by dynamic axial load. Tribol. Int. 2017, 115, 432–451. [Google Scholar] [CrossRef]
- Gong, H.; Ding, X.; Liu, J.; Feng, H. Review of research on loosening of threaded fasteners. Friction 2022, 10, 335–359. [Google Scholar] [CrossRef]
- Li, Z.; Chen, Y.; Sun, W.; Jiang, P.; Pan, J.; Guan, Z. Study on self-loosening mechanism of bolted joint under rotational vibration. Tribol. Int. 2021, 161, 107074. [Google Scholar] [CrossRef]
- Gong, H.; Liu, J.; Ding, X. Thorough understanding on the mechanism of vibration-induced loosening of threaded fasteners based on modified Iwan model. J. Sound Vib. 2020, 473, 115238. [Google Scholar] [CrossRef]
- Botto, D.; Lavella, M. A numerical method to solve the normal and tangential contact problem of elastic bodies. Wear 2015, 330–331, 629–635. [Google Scholar] [CrossRef]
- Donida, G.; Bernetti, R.; Bruschi, R. The ‘penalty function’ method for problems of contact. Comput. Struct. 1990, 36, 777–782. [Google Scholar] [CrossRef]
- Maury, B. Numerical analysis of a finite element/volume penalty method. SIAM J. Numer. Anal. 2009, 47, 1126–1148. [Google Scholar] [CrossRef]
- Underhill, W.R.C.; Dokainish, M.A.; Oravas, G.Æ. A method for contact problems using virtual elements. Comput. Methods Appl. Mech. Eng. 1997, 143, 229–247. [Google Scholar] [CrossRef]
- Wang, D.; Xu, C.; Wan, Q. Modeling tangential contact of rough surfaces with elastic- and plastic-deformed asperities. J. Tribol. 2017, 139, 051401. [Google Scholar] [CrossRef]
- Fukuoka, T.; Nomura, M. Proposition of helical thread modeling with accurate geometry and finite element analysis. J. Press. Vessel Technol. 2008, 130, 011204. [Google Scholar] [CrossRef]
- Segalman, D.J.; Gregory, D.L.; Starr, M.J.; Resor, B.R.; Jew, M.D.; Lauffer, J.P.; Ames, N.M. Handbook on Dynamics of Jointed Structures; Sandia National Laboratories: Albuquerque, NM, USA, 2009. [Google Scholar] [CrossRef]



| Preload/N | 300 | Friction coefficient () | 0.1 | External excitation amplitude (A)/mm | 0.005 |
| 500 | 0.2 | 0.010 | |||
| 700 | 0.3 | 0.015 | |||
| 900 | 0.4 | 0.020 | |||
| 1100 | 0.5 | 0.025 | |||
| Preload (N) | Residual Stiffness (N/mm) | Critical Stick–Slip Force (N) | Critical Stick–Slip Displacement (mm) | Power Exponent |
|---|---|---|---|---|
| 300 | 2996.4 | 89.70 | 0.0032 | −0.644 |
| 500 | 3058.9 | 149.02 | 0.0036 | −0.611 |
| 700 | 3158.3 | 208.09 | 0.0039 | −0.755 |
| 900 | 3280.7 | 266.78 | 0.0041 | −0.842 |
| 1100 | 3351.4 | 325.38 | 0.0044 | −0.931 |
| Friction Coefficients | Residual Stiffness (N/mm) | Critical Stick–Slip Force (N) | Critical Stick–Slip Displacement (mm) | Power Exponent |
|---|---|---|---|---|
| 0.1 | 2753.1 | 89.03 | 0.0032 | −0.551 |
| 0.2 | 3137.3 | 150.98 | 0.0037 | −0.688 |
| 0.3 | 3280.7 | 219.05 | 0.0041 | −0.748 |
| 0.4 | 3338.4 | 307.33 | 0.0048 | −0.828 |
| 0.5 | 3365.2 | 393.15 | 0.0053 | −0.931 |
| Excitation Amplitude (mm) | Residual Stiffness (N/mm) | Critical Stick–Slip Force (N) | Critical Stick–Lip Displacement (mm) | Power Exponent |
|---|---|---|---|---|
| 0.025 | 3270.9 | 266.11 | 0.0039 | −0.930 |
| 0.020 | 3280.7 | 266.78 | 0.0041 | −0.842 |
| 0.015 | 3290.1 | 266.66 | 0.0043 | −0.828 |
| 0.010 | 3297.2 | 265.82 | 0.0041 | −0.864 |
| 0.005 | 3318.6 | 265.35 | 0.0041 | −0.909 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yuan, D.; Wang, D.; Wan, Q. Modeling for Hysteresis Contact Behavior of Bolted Joint Interfaces with Memory Effect Penalty Constitution. Machines 2024, 12, 190. https://doi.org/10.3390/machines12030190
Yuan D, Wang D, Wan Q. Modeling for Hysteresis Contact Behavior of Bolted Joint Interfaces with Memory Effect Penalty Constitution. Machines. 2024; 12(3):190. https://doi.org/10.3390/machines12030190
Chicago/Turabian StyleYuan, Di, Dong Wang, and Qiang Wan. 2024. "Modeling for Hysteresis Contact Behavior of Bolted Joint Interfaces with Memory Effect Penalty Constitution" Machines 12, no. 3: 190. https://doi.org/10.3390/machines12030190
APA StyleYuan, D., Wang, D., & Wan, Q. (2024). Modeling for Hysteresis Contact Behavior of Bolted Joint Interfaces with Memory Effect Penalty Constitution. Machines, 12(3), 190. https://doi.org/10.3390/machines12030190






