Prediction of Fretting Wear Lifetime of a Coated System
Abstract
:1. Introduction
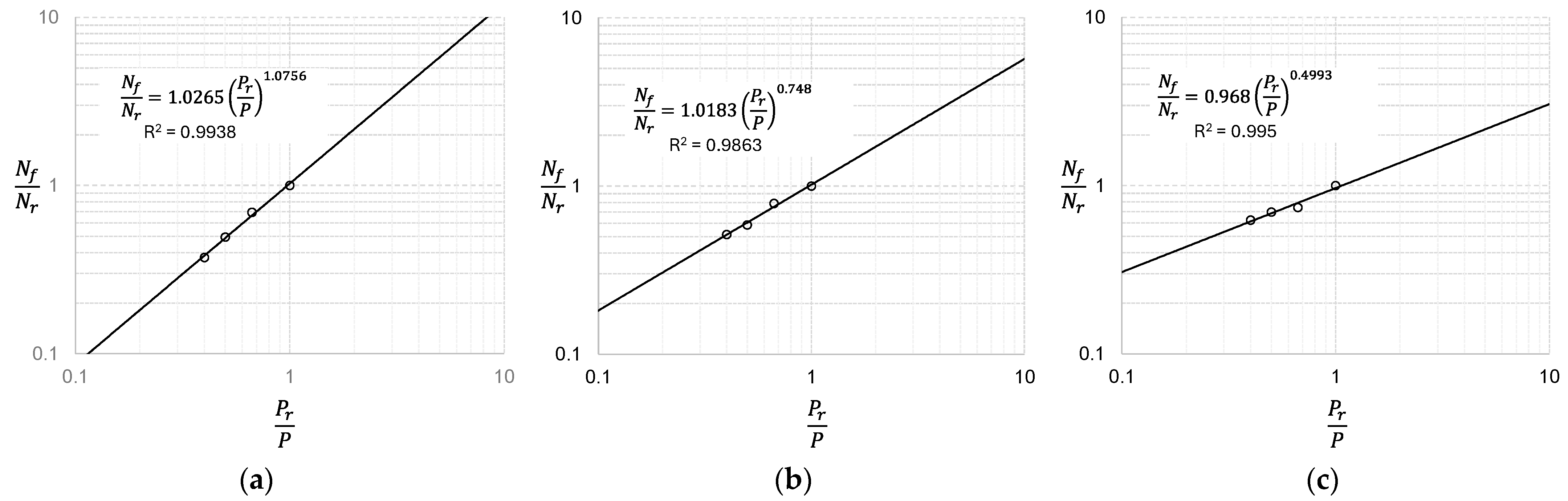
2. Fretting Wear Lifetime Model
3. Results
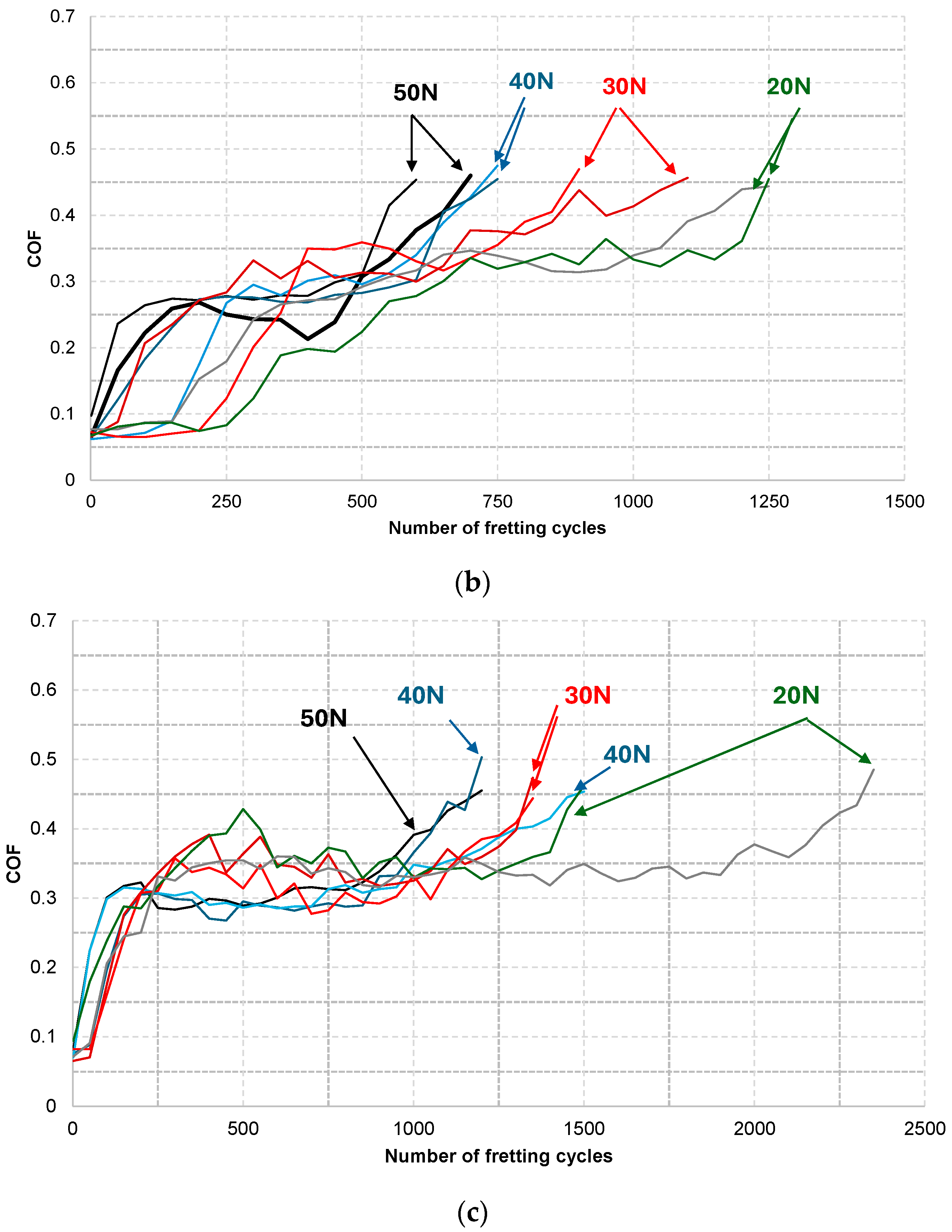
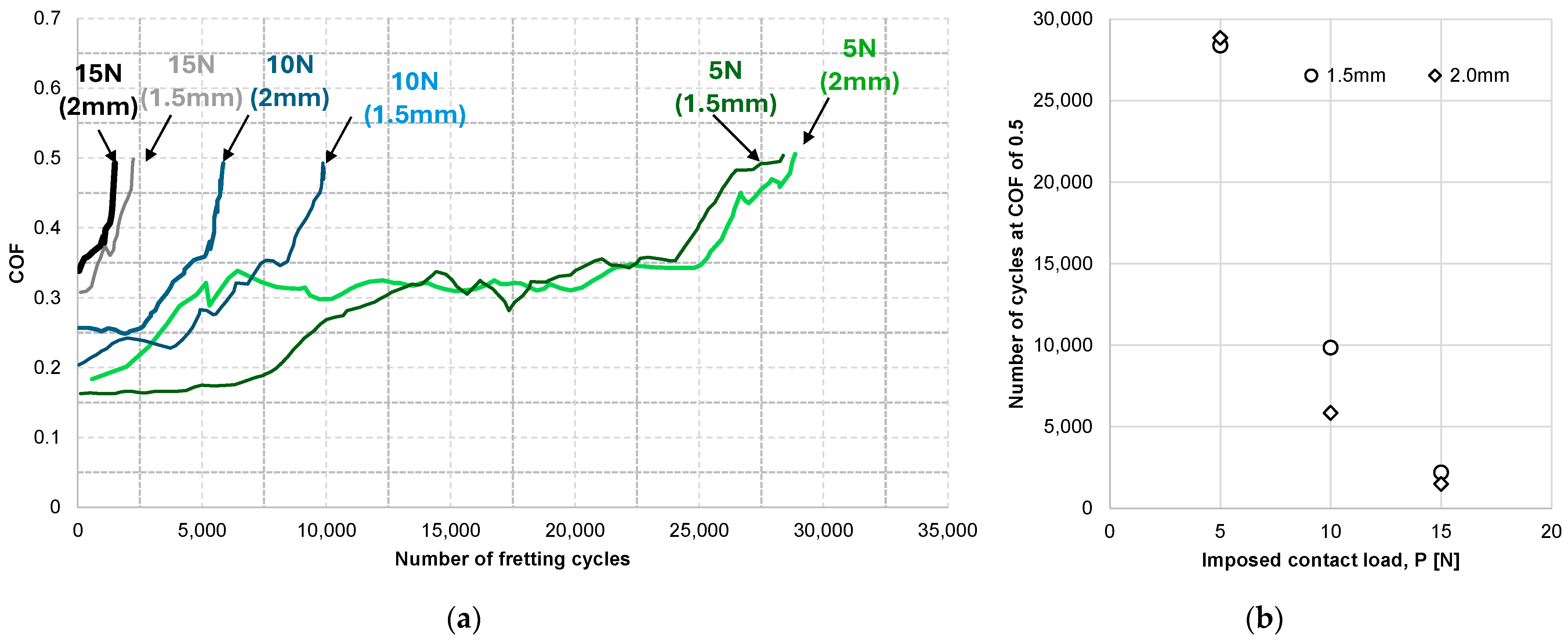
3.1. Effect of Imposed Contact Load
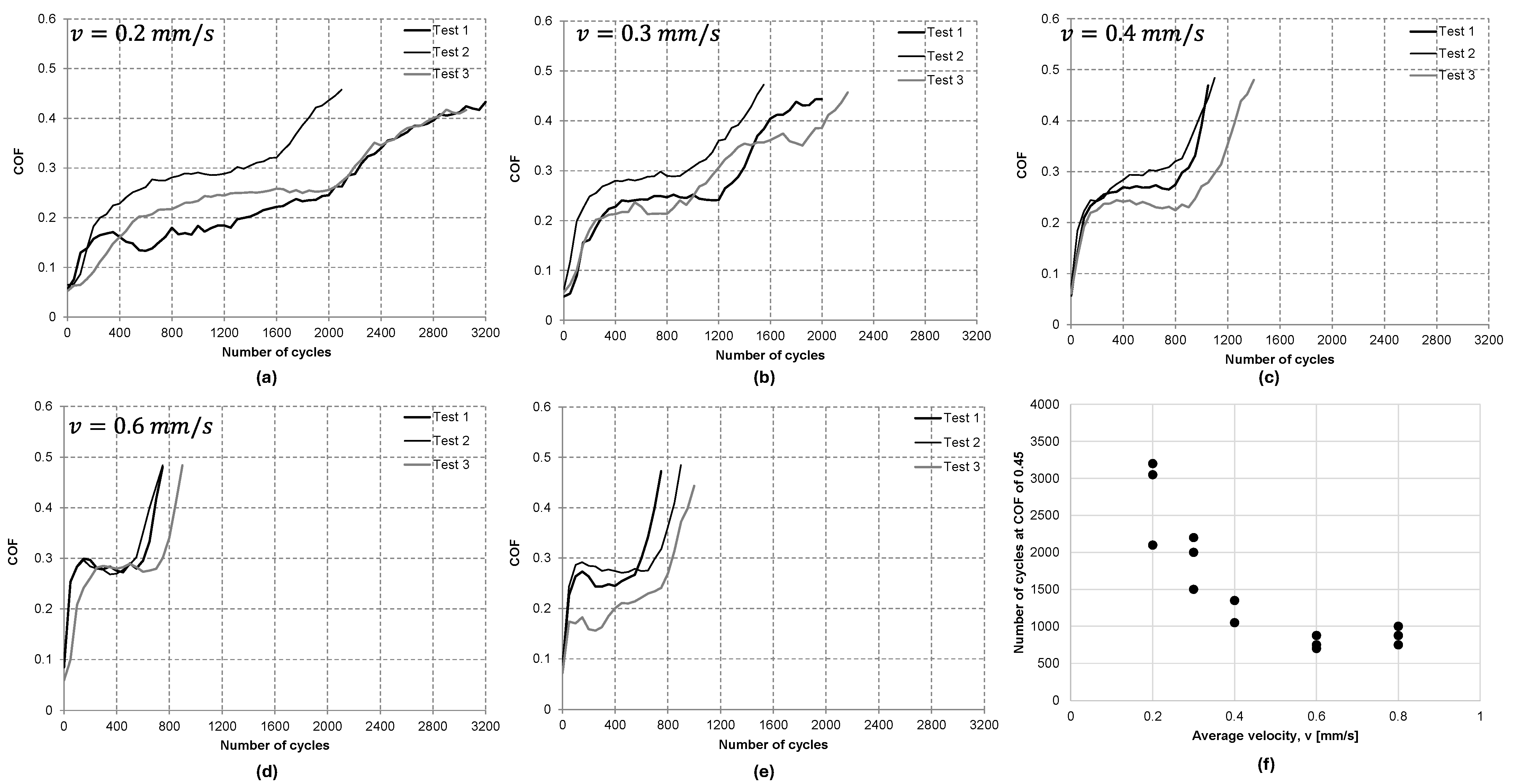
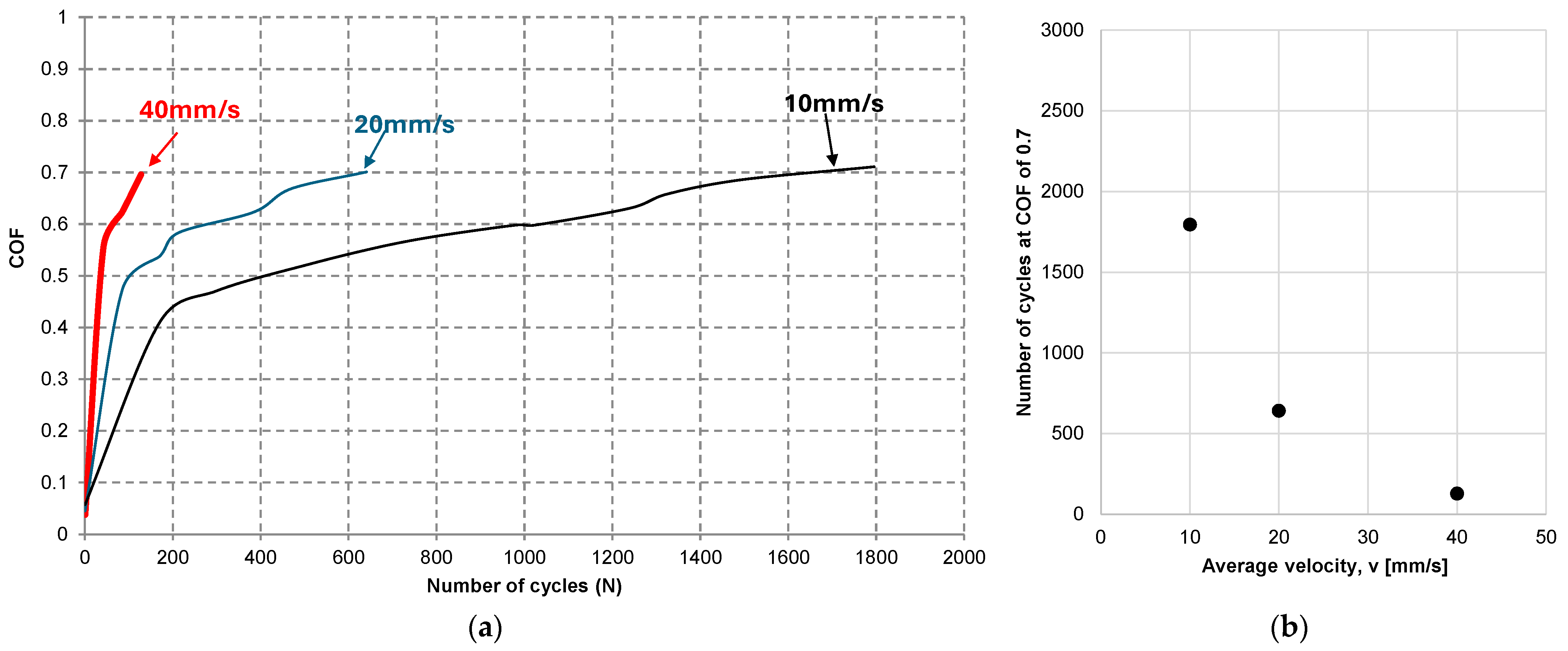
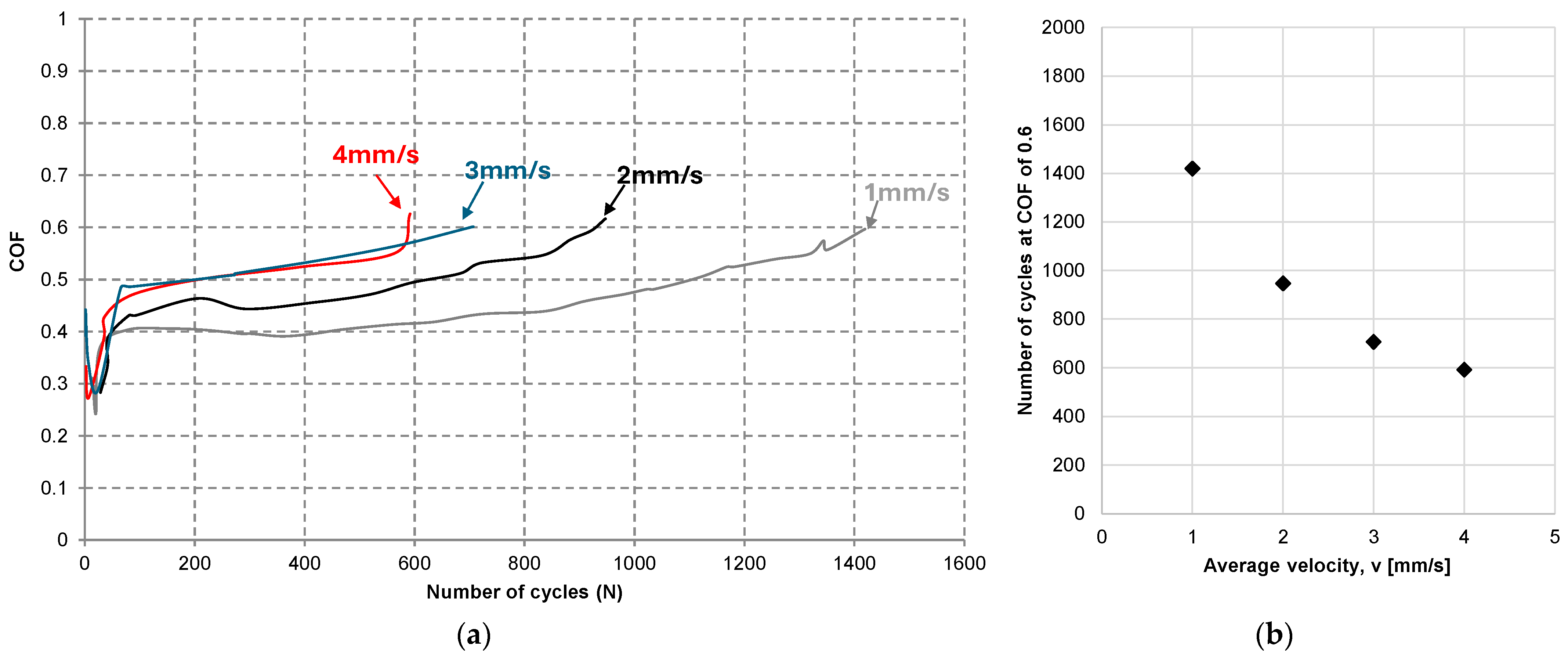
3.2. Effect of Imposed Average Velocity During Gross Slip
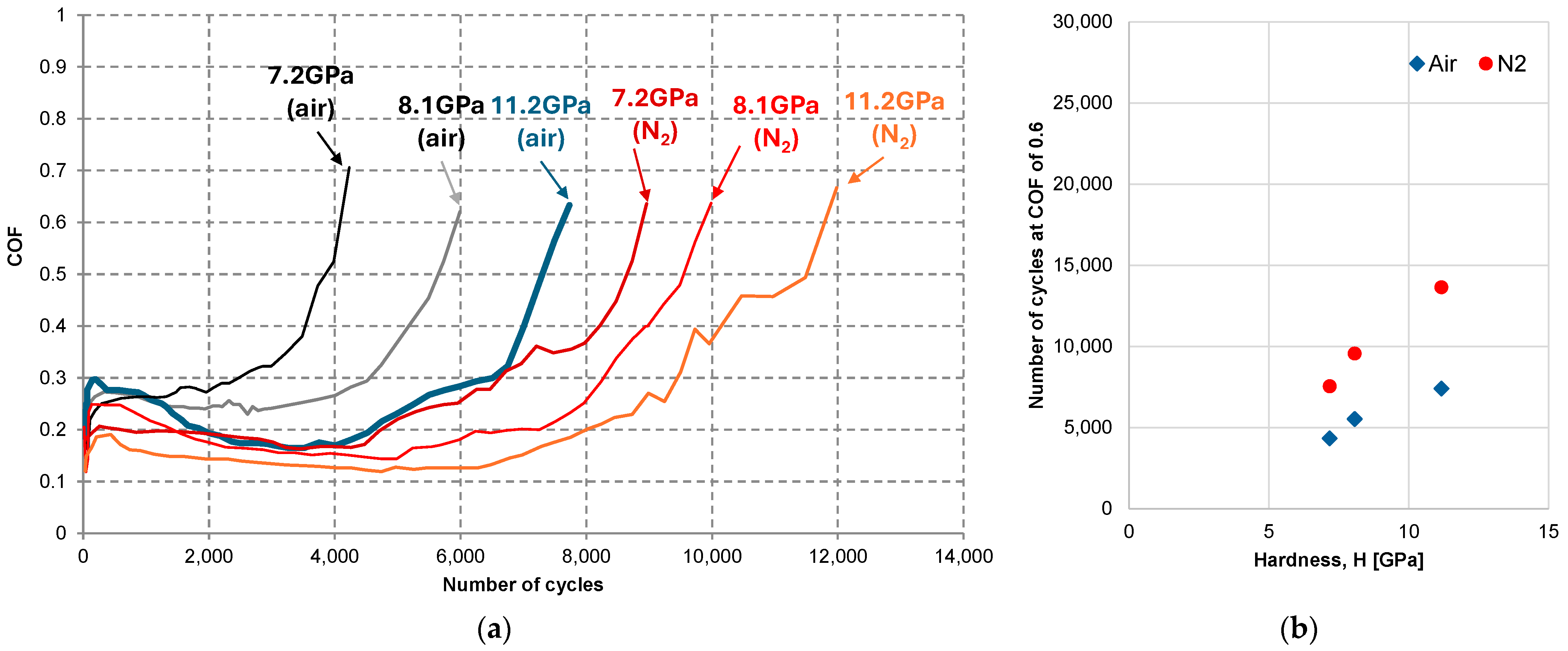
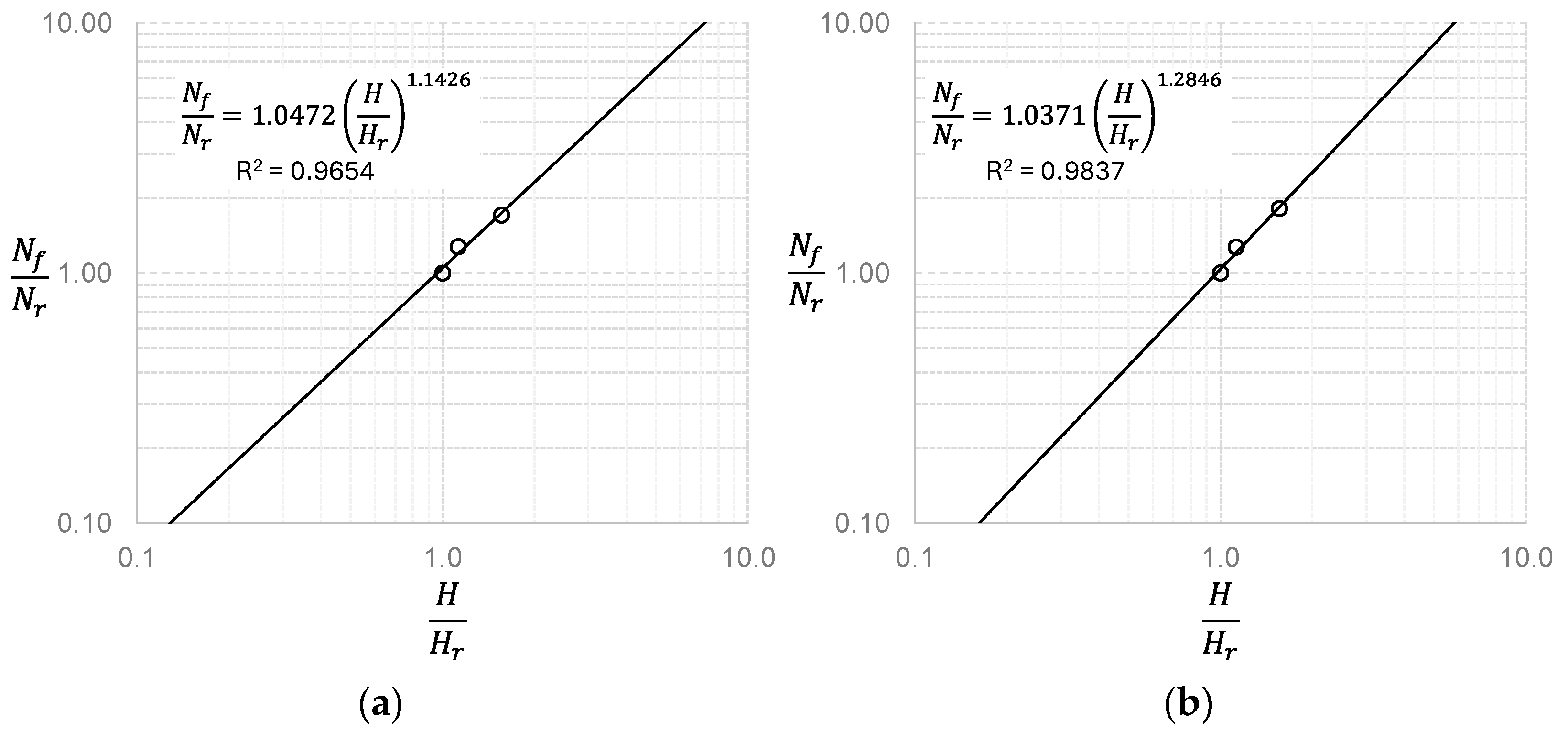
3.3. Effects of Coating Hardness and of Initial Surface Roughness
4. Discussion
5. Conclusions
- The fretting wear lifetimes of coatings were found to decrease with increased contact load. It was noted that the relationship between the imposed contact load and fretting wear lifetimes can be described by the inverse power law. The load-life exponent was observed to vary according to the type of coating. In addition, the type of counterpart for the electro-deposited coating affected the exponent.
- The fretting wear lifetimes of coatings were found to decrease with increased average velocity during gross slip. It was identified that it was possible to describe the relationship between an imposed average velocity and fretting wear lifetimes of a coating with an inverse power law. The velocity-life exponents were found to vary according to the type of coating.
- A fretting wear lifetime model for an electro-deposited coating was given with consideration of two factors: the contact load and average velocity. A direct comparison between the experimental and predicted lifetime values was employed, and a maximum error of 27 percent was found. Considering the variance among the experimental data for the coating (34 percent), one might accept the maximum error of the fretting wear lifetime model.
- Meanwhile, the relationship between the initial surface roughness of a counterpart and the fretting wear lifetime was identified; the fretting wear lifetime of a coating decreased with increased initial surface roughness. The influence of coating hardness on the fretting wear lifetime was identified. It was observed that the relationship between coating hardness and the fretting wear lifetime was described with a power law.
Funding
Data Availability Statement
Conflicts of Interest
References
- Waterhouse, R.B. Fretting wear. Wear 1984, 100, 107–118. [Google Scholar] [CrossRef]
- Vingsbo, O.; Soderberg, S. On Fretting Maps. Wear 1988, 126, 131–147. [Google Scholar] [CrossRef]
- Hills, D.A.; Nowell, D. Mechanics of Fretting Fatigue, 1st ed.; Springer: Berlin/Heidelberg, Germany, 1994. [Google Scholar]
- Geringer, J.; Macdonald, D. Friction/fretting-corrosion mechanisms: Current trends and outlooks for implants. Mater. Lett. 2014, 134, 152–157. [Google Scholar] [CrossRef]
- Kim, H.K.; Lee, Y.H.; Heo, S.P. Mechanical and experimental investigation on nuclear fuel fretting. Tribol. Int. 2016, 39, 1305–1319. [Google Scholar] [CrossRef]
- Antler, M. Survey of contact fretting in electrical connectors. IEEE Trans. Compon. Packag. Technol. 1985, 8, 87–104. [Google Scholar] [CrossRef]
- van Dijk, P.; Rudolphi, A.K.; Klake, D. Investigations on Electrical Contacts Subjected to Fretting Motion. In Proceedings of the 21st International Conference on Electrical Contacts (ICEC), Zurich, Switzerland, 9–12 September 2002. [Google Scholar]
- Koiprasert, H.; Dumrongrattana, S.; Niranatlumpong, P. Thermally sprayed coatings for protection of fretting wear in land-based gas-turbine engine. Wear 2004, 257, 1–7. [Google Scholar] [CrossRef]
- Kim, K.; Korsunsky, A.M. Effects of imposed displacement and initial coating thickness on fretting behaviour of a thermally sprayed coating. Wear 2011, 271, 1080–1085. [Google Scholar] [CrossRef]
- Langlade, C.; Vannes, B.; Taillandier, M.; Pierantoni, M. Fretting behavior of low-friction coatings: Contribution to industrial selection. Tribol. Int. 2001, 34, 49–56. [Google Scholar] [CrossRef]
- Kim, K. Statistical determination of a fretting-induced failure of an electro-deposited coating. Coatings 2017, 7, 48. [Google Scholar] [CrossRef]
- Bill, R.C. Review of factors that influence fretting wear. A.S.L.E. Trans. 1982, 21, 239. [Google Scholar]
- Jun, T.S.; Kim, K. Influence of load on the friction coefficient of fretted electro-deposited coatings against steel and zirconia balls. Int. J. Surf. Sci. Eng. 2016, 10, 514–526. [Google Scholar] [CrossRef]
- Zhang, Z.; Wood, R.J.K.; Lu, P.; Guo, Y.; Wang, D. Effect of pv values on dry fretting and wear characteristics of aromatic thermosetting co-polyester (ATSP)-MoS2 coatings. Tribol. Int. 2023, 188, 108889. [Google Scholar] [CrossRef]
- Hur, J.W.; Baek, S.Y.; Kim, K. Effect of displacement on kinetic frictional behaviour between electro-deposited coating and AISI 52100 steel under fretting conditions. Proc. IME J. J. Eng. Tribol. 2016, 230, 1172–1179. [Google Scholar] [CrossRef]
- Li, Y.; Ying, S.; Zhao, Y.; Li, Z.; Cao, J.; Wu, X.; Du, P.; Wu, T.; Yu, C.; Xie, G. Study on fretting friction properties based on GCr15 alloy and liquid epoxy resin protective coatings on GCr15 alloy. Colloids Surf. A Physicochem. Eng. Asp. 2024, 696, 134364. [Google Scholar] [CrossRef]
- Shi, Z.; Xu, L.; Deng, C.; Liu, M.; Liao, H.; Darut, G.; Panche, M. Effects of frequency on the fretting wear behavior of aluminum bronze coatings. Surf. Coat. Tech. 2023, 457, 129306. [Google Scholar] [CrossRef]
- Baek, D.L.; Khonsari, M.M. Fretting behavior of a rubber coating: Effect of temperature and surface roughness variations. Wear 2008, 265, 620–625. [Google Scholar] [CrossRef]
- Shi, X.; Liskiewicz, T.W.; Beake, B.D.; Sun, Z.; Chen, J. Fretting wear behavior of graphite-like carbon films with bias-graded deposition. App. Surf. Sci. 2019, 494, 929–940. [Google Scholar] [CrossRef]
- Lundberg, G.; Palmgren, A. Dynamic Capacity of Roller Bearings. Acta Polytech. Mech. Eng. Series 1952, 2, 96–127. [Google Scholar]
- Bhushan, B. Introduction to Tribology, 1st ed.; John Wiley & Sons, Inc.: New York, NY, USA, 2002. [Google Scholar]
- Zaretsky, E.V.; Poplawski, J.V.; Miller, C.R. Rolling Bearing Life Prediction—Past, Present, and Future; NASA/TM-2000-210529; National Aeronautics and Space Administration: Washington, DC, USA, 2000.
- ISO 98:1990(E); Rolling Bearing-Dynamic Load Ratios and Rating Life. International Organization for Standardization: Geneva, Switzerland, 1990.
- Endo, K.; Goto, H. Effects of environment on fretting fatigue. Wear 1978, 48, 347–367. [Google Scholar] [CrossRef]
- Rybiak, R.; Fouvry, S.; Liskiewicz, T.; Wendler, B. Fretting wear of a TiN PVD coating under variable relative humidity conditions-development of a ‘composite’ wear law. Surf. Coat. Tech. 2008, 202, 1753–1763. [Google Scholar] [CrossRef]
- Rybiak, R.; Fouvry, S.; Bonnet, B. Fretting wear of stainless steels under variable temperature conditions: Introduction of a ‘composite’ wear law. Wear 2010, 268, 413–423. [Google Scholar] [CrossRef]
- Willert, E. Influence of Profile Geometry on Frictional Energy Dissipation in a Dry, Compliant Steel-on-Steel Fretting Contact: Macroscopic Modeling and Experiment. Machines 2023, 11, 484. [Google Scholar] [CrossRef]






| Test No | Contact Load, N | Average Velocity, mm/s | Measured Lifetime () | Predicted Lifetime (Nf) | Error (%) |
|---|---|---|---|---|---|
| 1 | 20 | 0.8 | 2177 | 2177 | 0% |
| 2 | 30 | 0.8 | 1507 | 1408 | 7% |
| 3 | 40 | 0.8 | 1073 | 1033 | 4% |
| 4 | 50 | 0.8 | 812 | 813 | 0% |
| 5 | 50 | 0.2 | 2783 | 2958 | 6% |
| 6 | 50 | 0.3 | 1900 | 2027 | 6% |
| 7 | 50 | 0.4 | 1150 | 1550 | 26% |
| 8 | 50 | 0.6 | 775 | 1062 | 27% |
| 9 | 50 | 0.8 | 875 | 813 | 8% |
| Test No | Hardness, GPa | Condition | Measured Lifetime () | Predicted Lifetime (Nf) | Error (%) |
|---|---|---|---|---|---|
| 1 | 7.17 | Air | 4329 | 4329 | 0% |
| 2 | 8.07 | Air | 5523 | 4952 | 12% |
| 3 | 11.17 | Air | 7393 | 7178 | 3% |
| 4 | 7.17 | H2 | 7546 | 7546 | 0% |
| 5 | 8.07 | H2 | 9568 | 8779 | 9% |
| 6 | 11.17 | H2 | 13,640 | 13,327 | 2% |
| Test No | Arithmetic Average Surface Roughness (Ra), µm | Measured Lifetime ( ) | Predicted Lifetime (Nf) | Error (%) |
|---|---|---|---|---|
| 1 | 0.02 | 2715 | 2715 | 0% |
| 2 | 0.05 | 1278 | 1157 | 10% |
| 3 | 0.1 | 513 | 607 | 16% |
| 4 | 0.2 | 348 | 319 | 9% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kim, K. Prediction of Fretting Wear Lifetime of a Coated System. Machines 2024, 12, 910. https://doi.org/10.3390/machines12120910
Kim K. Prediction of Fretting Wear Lifetime of a Coated System. Machines. 2024; 12(12):910. https://doi.org/10.3390/machines12120910
Chicago/Turabian StyleKim, Kyungmok. 2024. "Prediction of Fretting Wear Lifetime of a Coated System" Machines 12, no. 12: 910. https://doi.org/10.3390/machines12120910
APA StyleKim, K. (2024). Prediction of Fretting Wear Lifetime of a Coated System. Machines, 12(12), 910. https://doi.org/10.3390/machines12120910







