Drifted Uncertainty Evaluation of a Compact Machine Tool Spindle Error Measurement System
Abstract
1. Introduction
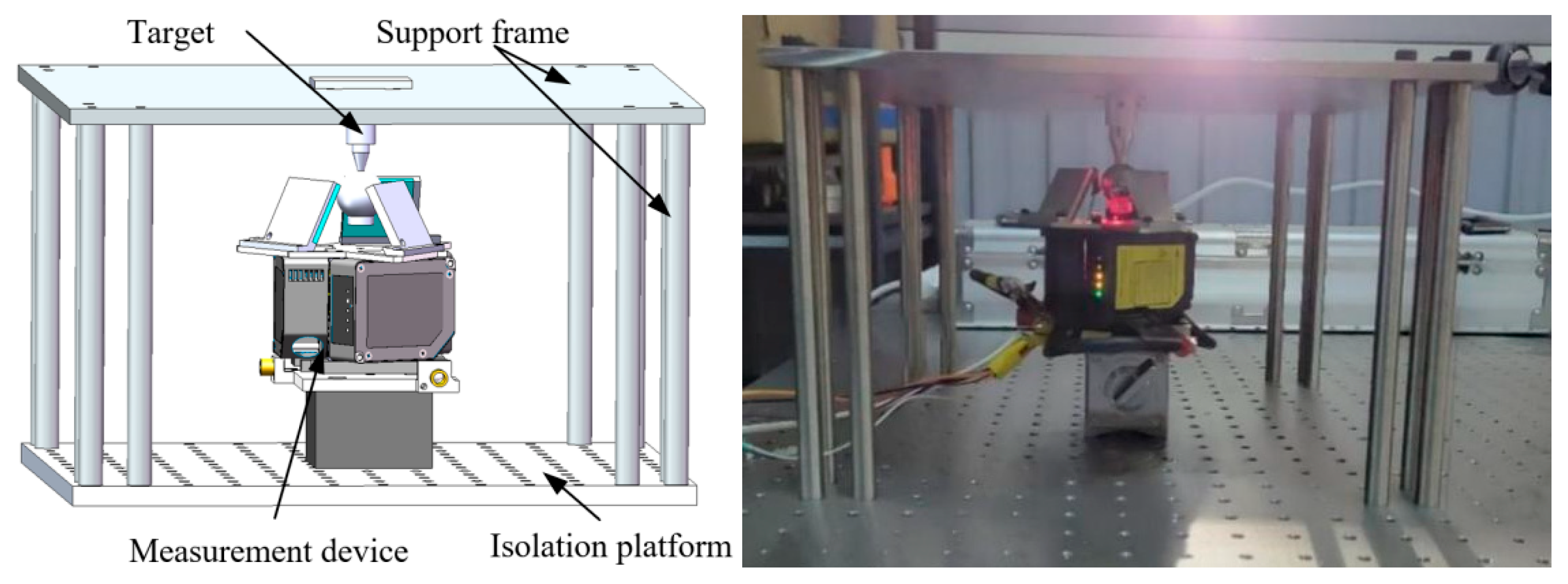
2. Measurement Principle
2.1. Definition of Spindle Error Motion and Design of the Measurement System
2.2. Displacement Measurement Principle
2.3. Tilt Measurement Principle
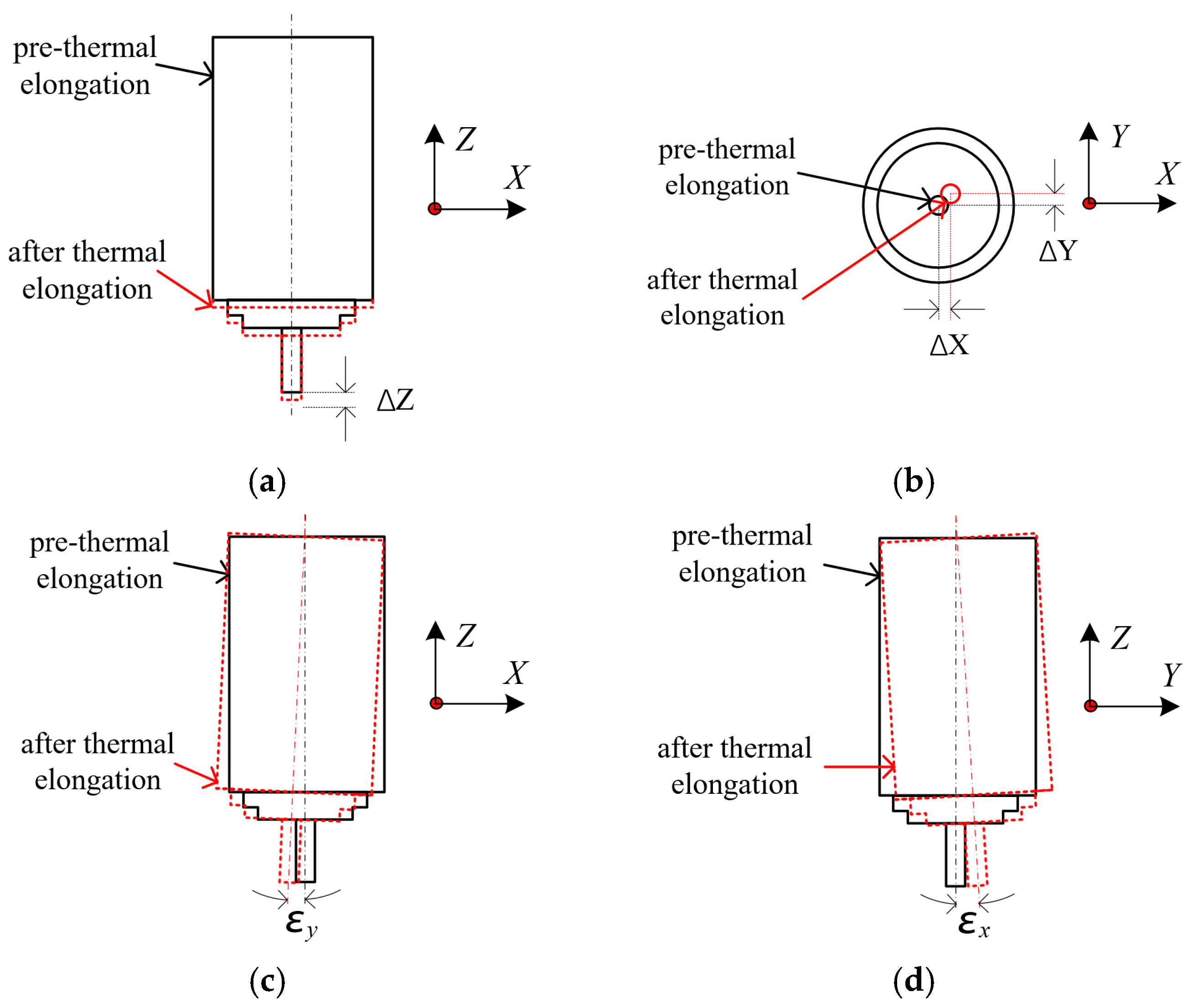
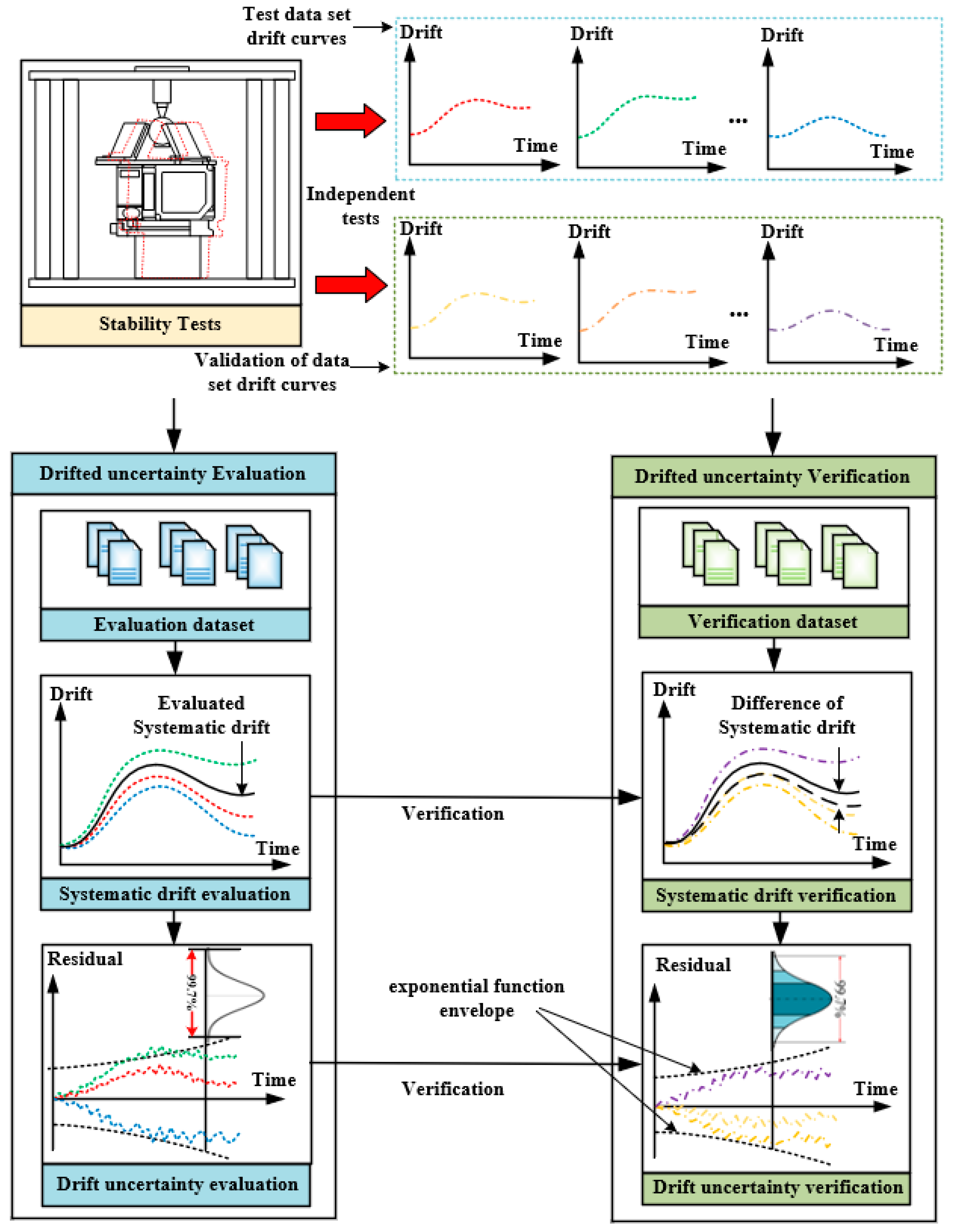
3. Definition and Evaluation of Drift Uncertainty
3.1. Definition of Drift Uncertainty
3.2. Evaluation Method of Drift Uncertainty
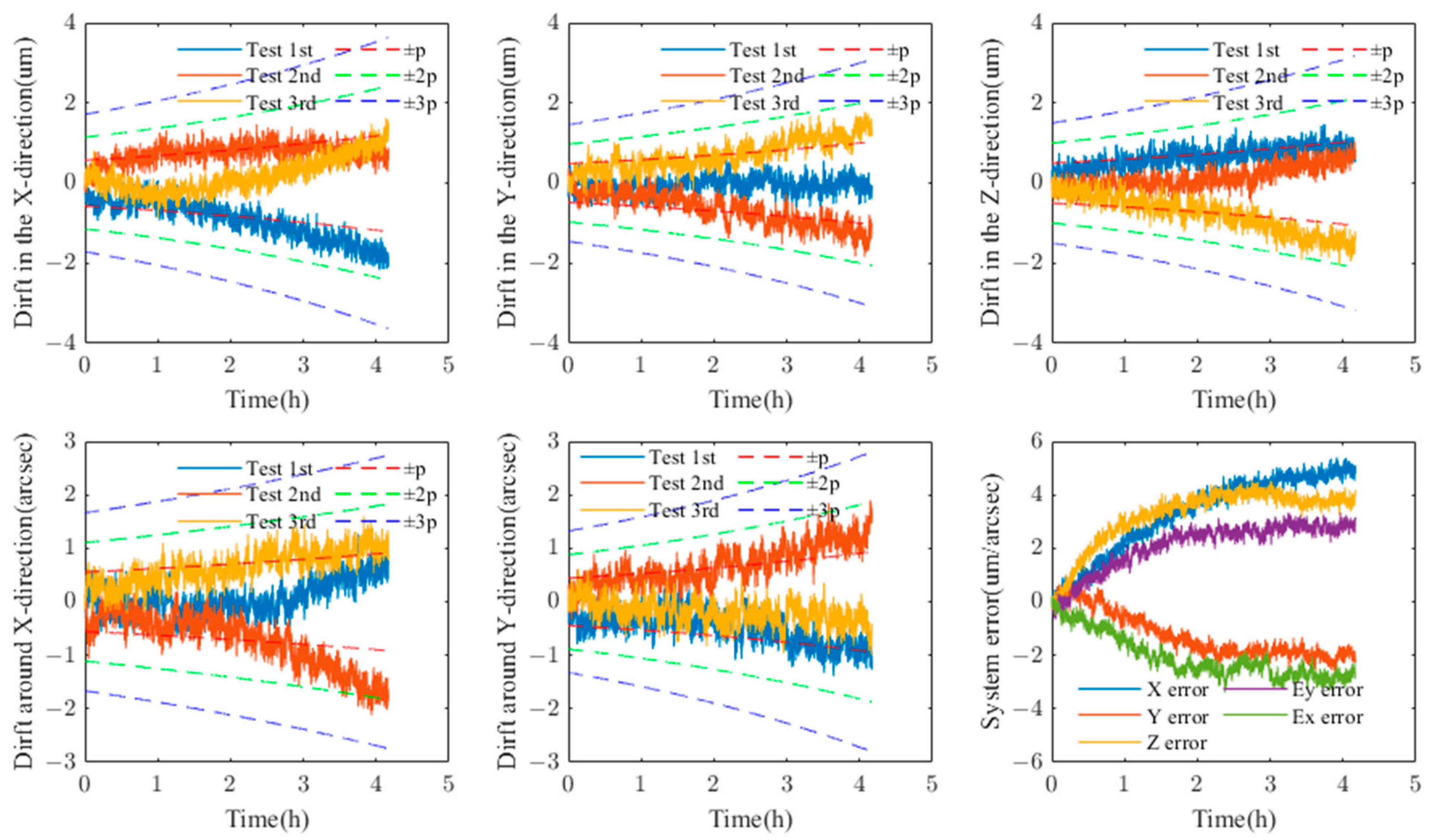
4. Verification of Drift Uncertainty Evaluation
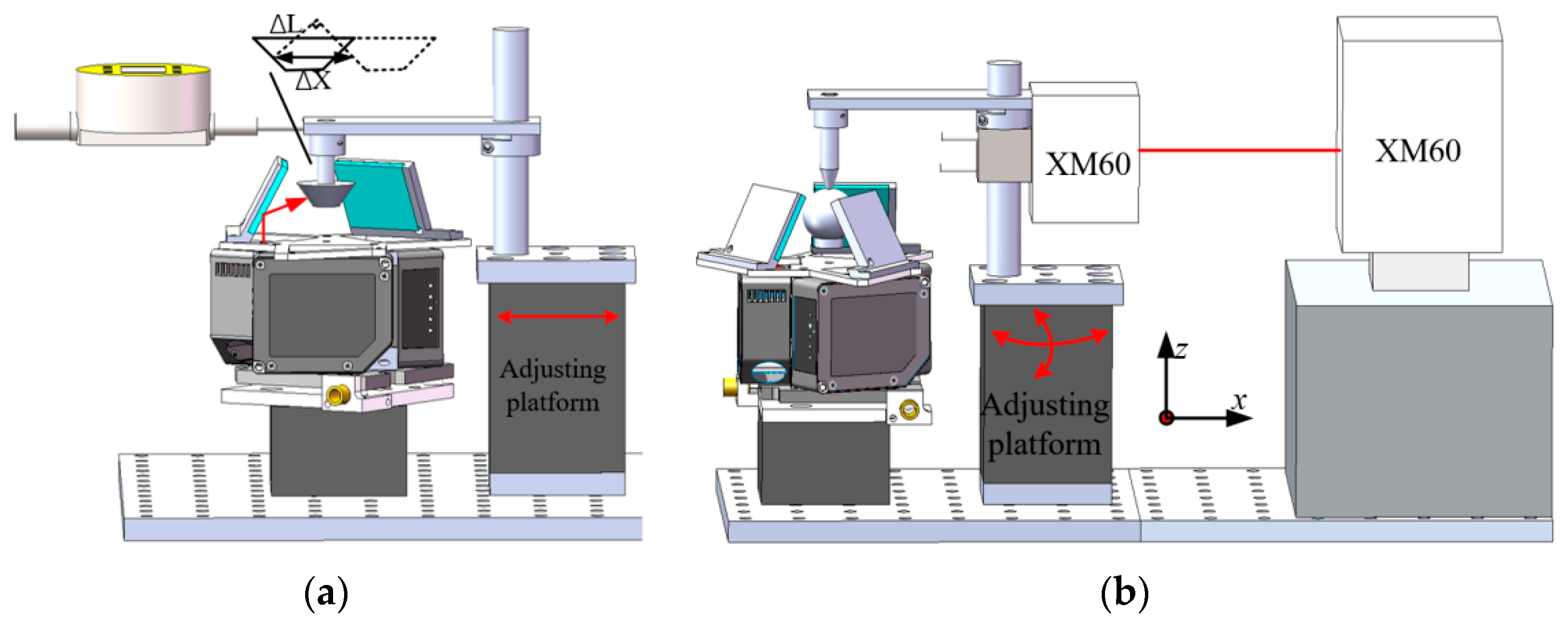
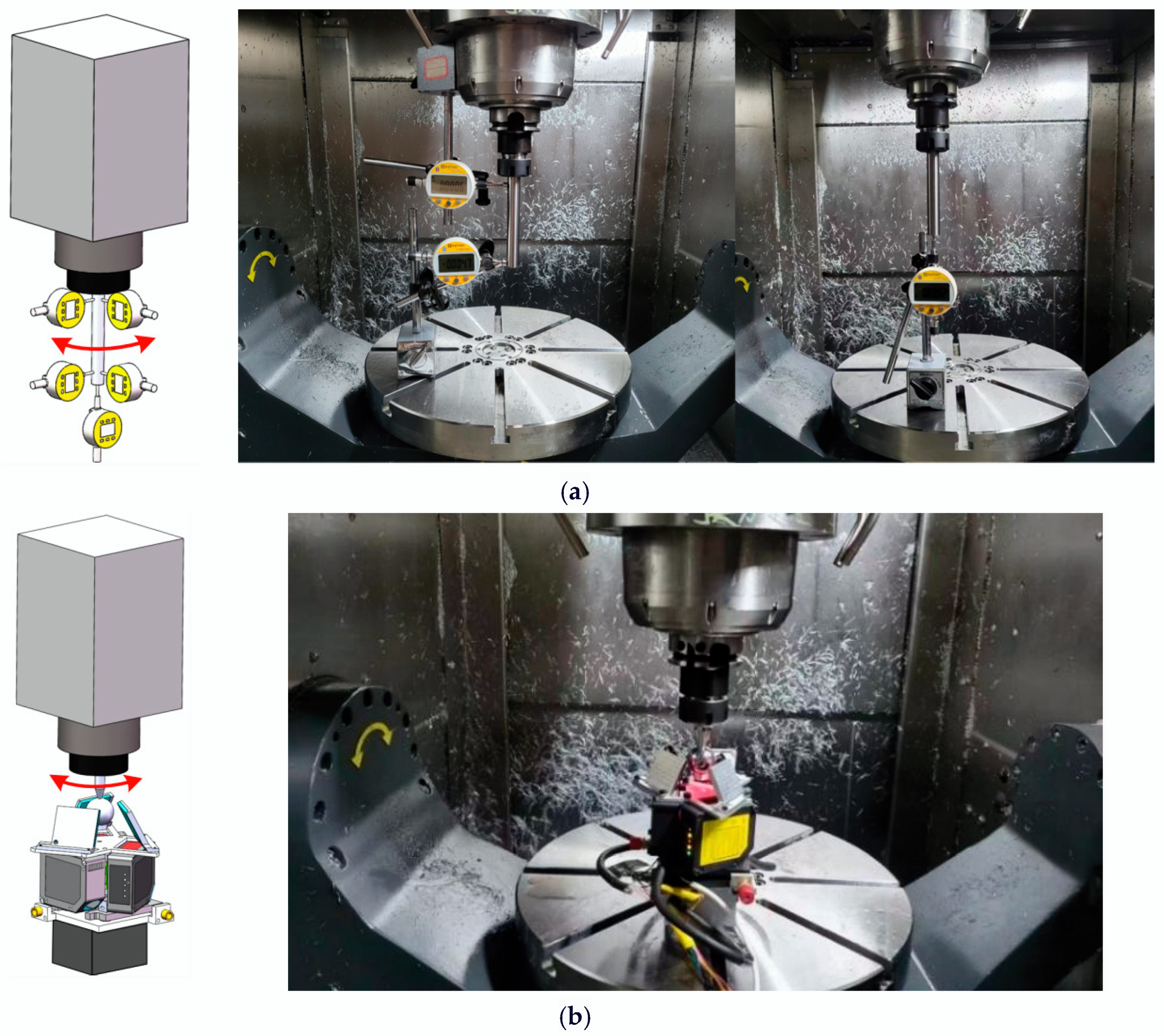
4.1. Experiment Design
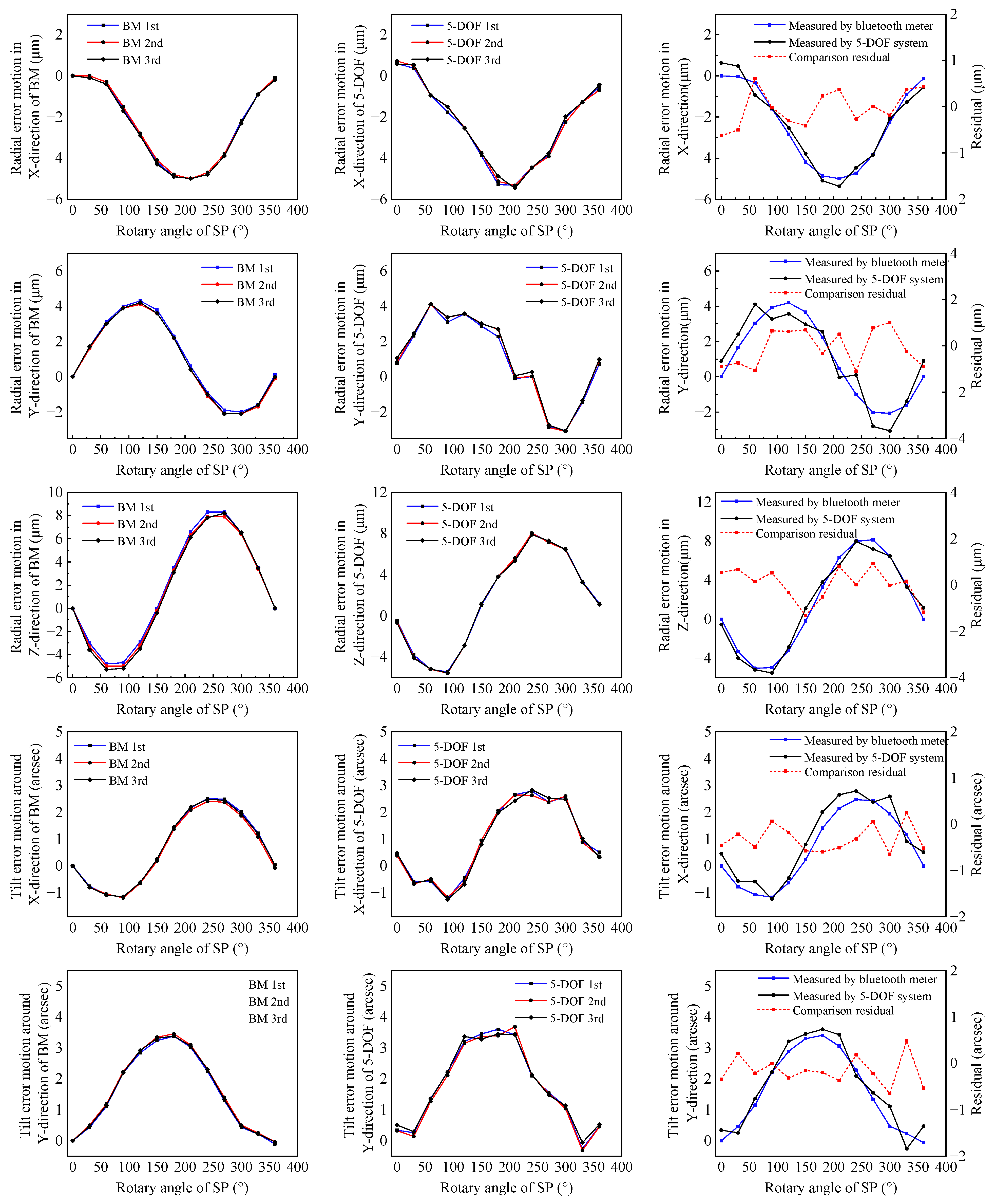
4.2. Evaluation and Verification Results
5. Performance Test
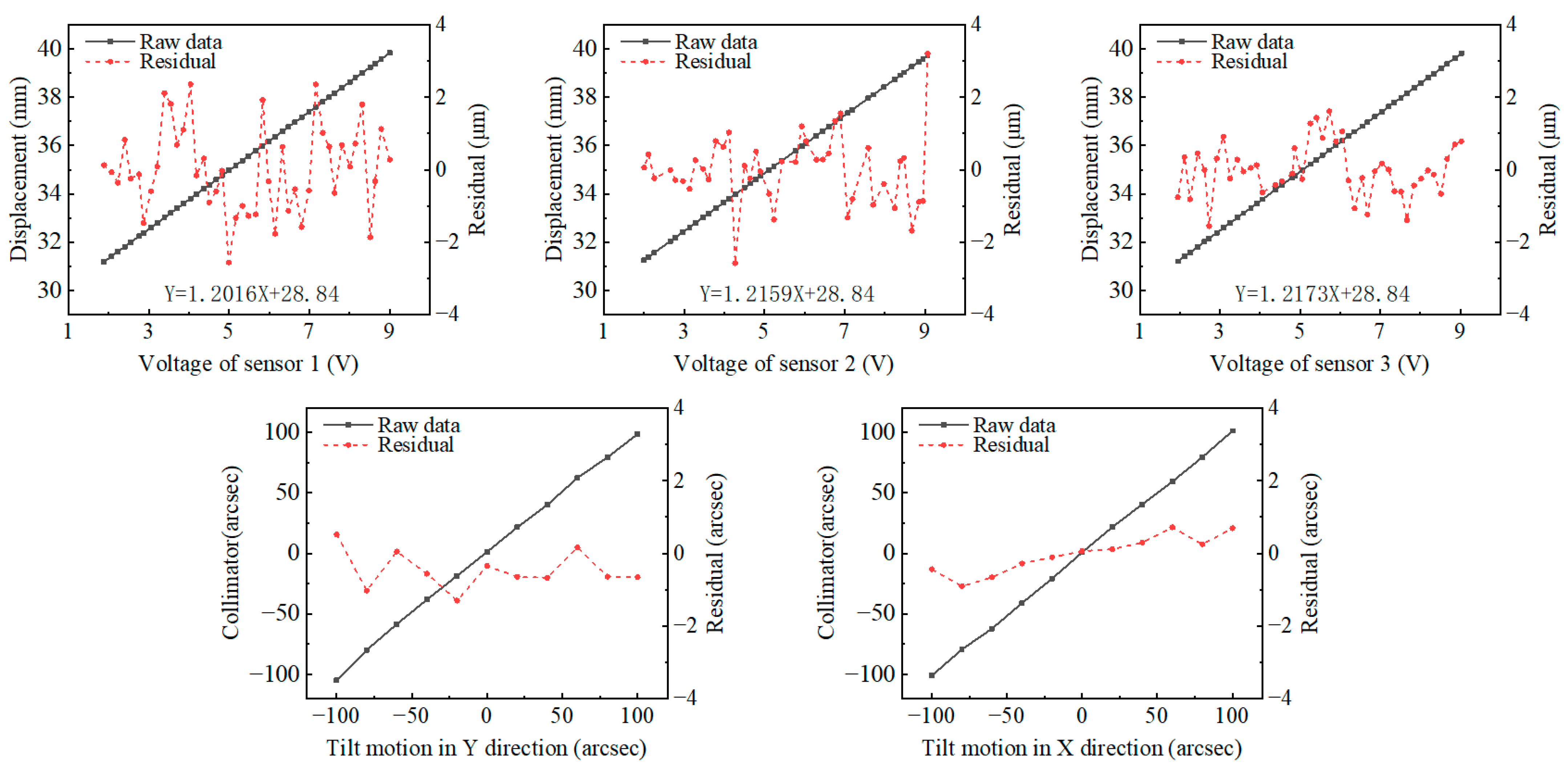
5.1. Calibration
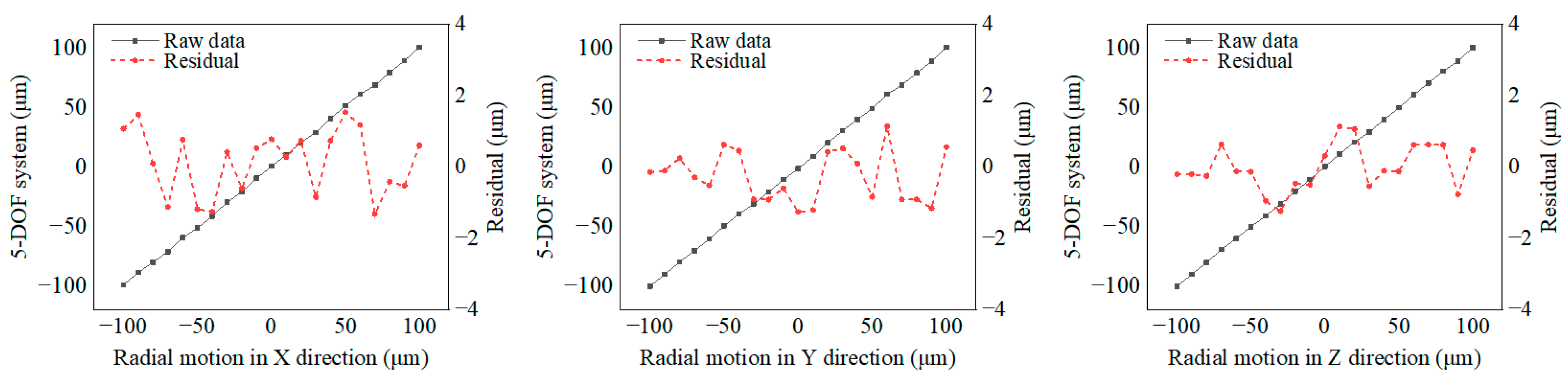
5.2. Performance Test of Ball Center Position Calculation
5.3. Performance of Spindle Geometric Error Measurement Repeatability and Accuracy
5.4. Spindle Thermal Error Measurement
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Liu, L.; Lou, Z.-F.; Huang, Y.-B.; Fan, K.-C.; Zhang, J.-Y. A five degrees-of-freedom errors measurement system for rotary axis with reference laser for reference axis alignment. Rev. Sci. Instrum. 2020, 91, 075101. [Google Scholar] [CrossRef]
- Zhao, Y.; Zhang, B.; Feng, Q. Measurement system and model for simultaneously measuring 6DOF geometric errors. Opt. Express 2017, 25, 20993–21007. [Google Scholar] [CrossRef]
- Jia, P.; Li, P.; Zheng, F.; Feng, Q.; Zhang, B. Simultaneous measurement of 5DOF spindle error motions in CNC machine tools. Appl. Opt. 2022, 61, 5704–5713. [Google Scholar] [CrossRef]
- Hong, Y.; Sato, R.; Shimizu, Y.; Matsukuma, H.; Gao, W. A New Optical Configuration for the Surface Encoder with an Expanded Z-Directional Measuring Range. Sensors 2022, 22, 3010. [Google Scholar] [CrossRef] [PubMed]
- Bitar-Nehme, E.; Mayer, J. Modelling and compensation of dominant thermally induced geometric errors using rotary axes’ power consumption. CIRP Ann.-Manuf. Technol. 2018, 67, 547–550. [Google Scholar] [CrossRef]
- Murakami, H.; Katsuki, A.; Sajima, T. Simple and simultaneous measurement of five-degrees-of-freedom error motions of high-speed microspindle: Error analysis. Precis. Eng. 2014, 38, 249–256. [Google Scholar] [CrossRef]
- Madden, M.; Aketagawa, M.; Kumagai, T.; Maeda, Y.; Okuyama, E. Concurrent measurement method of spindle radial, axial and angular motions using concentric circle grating and phase modulation interferometers. Meas. Sci. Technol. 2014, 25, 094005. [Google Scholar] [CrossRef]
- Zhang, H.; Anders, D.; Löser, M.; Ihlenfeldt, S.; Czarske, J.; Kuschmierz, R. Non-contact, bi-directional tool tip vibration measurement in CNC milling machines with a single optical sensor. Mech. Syst. Signal Process. 2020, 139, 106647. [Google Scholar] [CrossRef]
- Liu, C.; Xiang, S.; Lu, C.; Wu, C.; Du, Z.; Yang, J. Dynamic and static error identification and separation method for three-axis CNC machine tools based on feature workpiece cutting. Int. J. Adv. Manuf. Technol. 2020, 107 (Suppl. S12–S13), 2227–2238. [Google Scholar] [CrossRef]
- Ibaraki, S.; Yanai, E. Identification of rotary axis location errors under spindle rotation by using a laser barrier tool measurement system—Experimental comparison with R-test. Trans. Inst. Syst. Control. Inf. Eng. 2021, 34, 81–88. [Google Scholar]
- Ni, H.; Zhao, W.; Qiu, L. Measurement method of spindle motion error based on composite laser target. Int. J. Mach. Tools Manuf. 2022, 174, 103860. [Google Scholar] [CrossRef]
- Bartel, T.; Stoudt, S.; Possolo, A. Force calibration using errors-in-variables regression and Monte Carlo uncertainty evaluation. Metrologia 2016, 53, 965–980. [Google Scholar] [CrossRef]
- Motra, H.; Hildebrand, J.; Wuttke, F. The Monte Carlo Method for evaluating measurement uncertainty: Application for determining the properties of materials. Probabilistic Eng. Mech. 2016, 45, 220–228. [Google Scholar] [CrossRef]
- Ramsey, H.M. Challenges for the estimation of uncertainty of measurements made in situ. Accredit. Qual. Assur. 2021, 26, 183–192. [Google Scholar] [CrossRef]
- Deng, Y. Uncertainty measure in evidence theory. Sci. China Inf. Sci. 2020, 63, 210201. [Google Scholar] [CrossRef]
- Silva, M.A.; Amado, C.; Ribeiro, Á.; Loureiro, D. Uncertainty evaluation in time-dependent measurements. Measurement 2022, 196, 111196. [Google Scholar] [CrossRef]
- Šafarič, J.; Dolšak, B.; Ačko, B. Analysis of thermal contribution to the measurement uncertainty in step gauge calibration. Precis. Eng. 2020, 66, 52–61. [Google Scholar] [CrossRef]
- Wang, J.; Wang, Z.; de Dear, R.; Luo, M.; Ghahramani, A.; Lin, B. The uncertainty of subjective thermal comfort measurement. Energy Build. 2018, 181, 38–49. [Google Scholar] [CrossRef]
- Jennings, J.; Kim, J.M.; Lee, J.; Taylor, D. Measurement error, fixed effects, and false positives in accounting research. Rev. Account. Stud. 2024, 29, 959–995. [Google Scholar] [CrossRef]
- Zhang, Z.; Feng, S.; Ding, Y.; Mei, X.; Tao, Z. Thermal error modeling of spindle and dynamic machining accuracy reliability analysis of CNC machine tools based on IA and LHSMC. Eksploat. Niezawodn. 2022, 24, 100–113. [Google Scholar] [CrossRef]
- JJF 1059.1-2012; Evaluation and Expression of Uncertainty in Measurement. General Administration of Quality Supervision, Inspection and Quarantine: Beijing, China, 2012.
- ISO 230-7; Test Code for Machine Tools-Part 7: Geometric Accuracy of Axes of Rotation. ISO: Geneva, Switzerland, 2011.



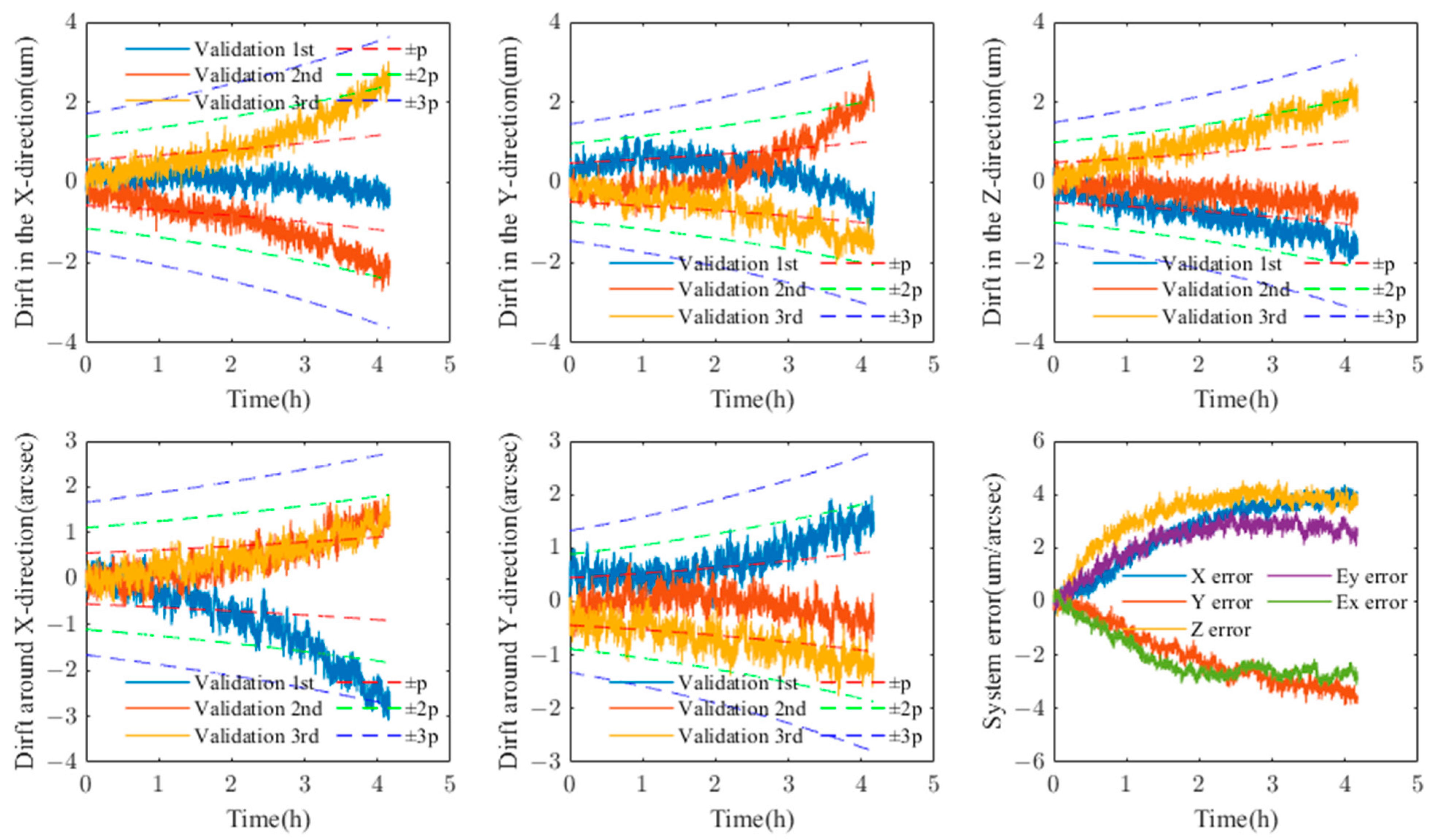


| Short-Time Uncertainty P0 | Time Drift Coefficient S | Drift Standard Deviation Envelope Function | Maximum System Drift | |
|---|---|---|---|---|
| δx | 0.5724 μm | 0.003 | 5.4101 μm | |
| δy | 0.4858 μm | 0.003 | 2.6133 μm | |
| δz | 0.4999 μm | 0.003 | 5.5629 μm | |
| εy | 0.4412 arcsec | 0.003 | 3.3391 arcsecs | |
| εx | 0.5546 arcsec | 0.002 | 3.3880 arcsecs |
| Deviation of Us 68.26 | Deviation of Us 95.44 | Deviation of Us 99.74 | System Drift Difference | ||||
|---|---|---|---|---|---|---|---|
| Traditional Uncertainty | Drift Uncertainty | Traditional Uncertainty | Drift Uncertainty | Traditional Uncertainty | Drift Uncertainty | ||
| δx | 14.59% | 5.01% | 20.94% | 2.52% | 11.51% | 0.26% | 1.845 μm |
| δy | 15.16% | 1.72% | 13.77% | 3.28% | 6.3% | 0.26% | 2.2682 μm |
| δz | 25.02% | 9.75% | 24.31% | 4.6% | 11.17% | 0.26% | 0.9062 μm |
| εy | 24.07% | 9.95% | 18.31% | 4.16% | 4.58% | 0.26% | 1.0514 arcsecs |
| εx | 15.15% | 2.89% | 15.68% | 3.18% | 7.21% | 0.56% | 0.96 arcsec |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Huang, Y.; Zhang, X.; You, K.; Chen, J.; Zhou, H.; Xiang, H. Drifted Uncertainty Evaluation of a Compact Machine Tool Spindle Error Measurement System. Machines 2024, 12, 695. https://doi.org/10.3390/machines12100695
Huang Y, Zhang X, You K, Chen J, Zhou H, Xiang H. Drifted Uncertainty Evaluation of a Compact Machine Tool Spindle Error Measurement System. Machines. 2024; 12(10):695. https://doi.org/10.3390/machines12100695
Chicago/Turabian StyleHuang, Yubin, Xiong Zhang, Kaisi You, Jihong Chen, Hao Zhou, and Hua Xiang. 2024. "Drifted Uncertainty Evaluation of a Compact Machine Tool Spindle Error Measurement System" Machines 12, no. 10: 695. https://doi.org/10.3390/machines12100695
APA StyleHuang, Y., Zhang, X., You, K., Chen, J., Zhou, H., & Xiang, H. (2024). Drifted Uncertainty Evaluation of a Compact Machine Tool Spindle Error Measurement System. Machines, 12(10), 695. https://doi.org/10.3390/machines12100695






