Static and Dynamic Stress of the Combined Rotor with Curvic Couplings Considering the Rough Three-Dimensional Interface at Extreme Operating Conditions
Abstract
1. Introduction
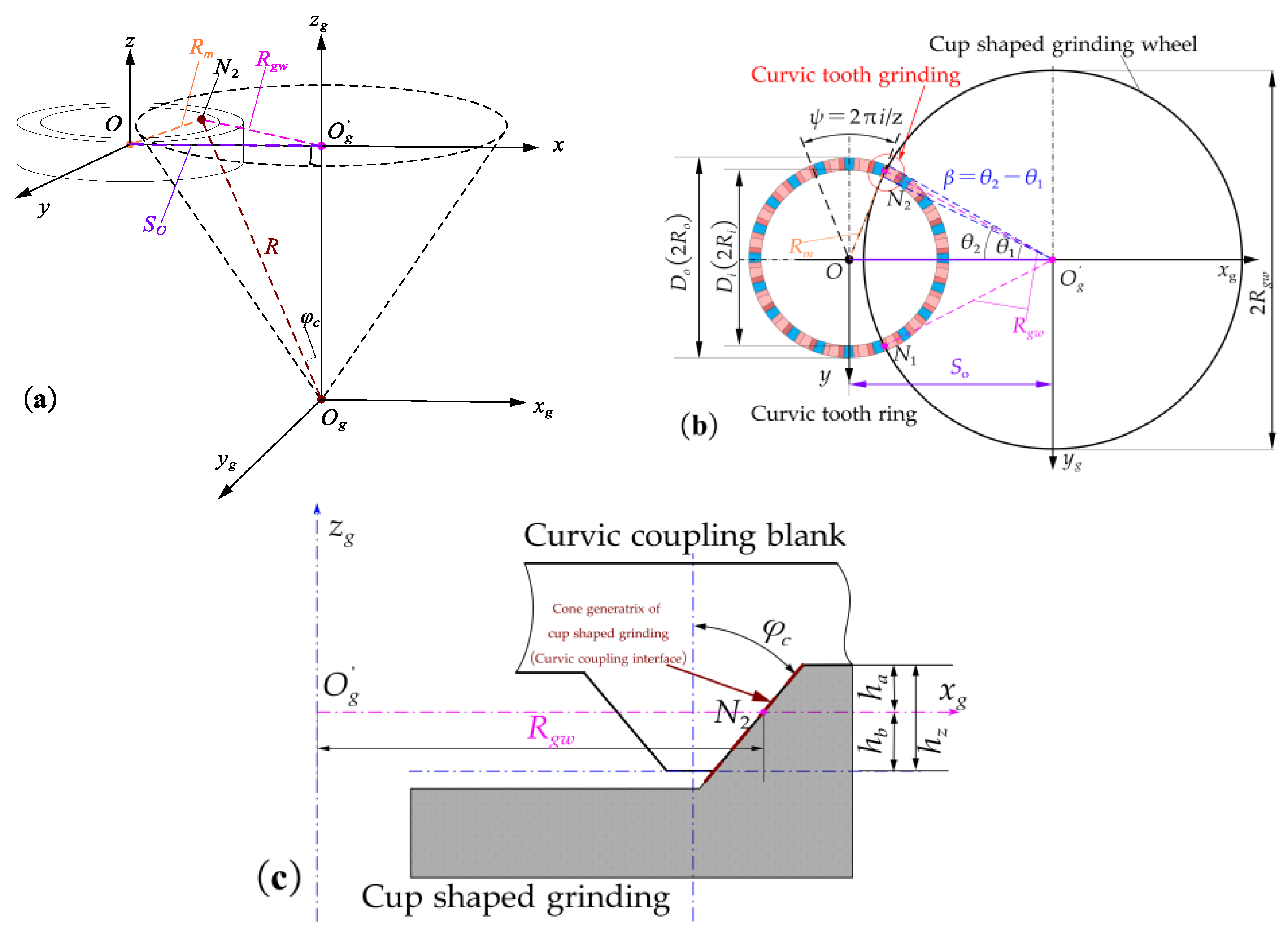
2. Rotor Modeling with Curvic Couplings Considering Rough Three-Dimensional Interfaces
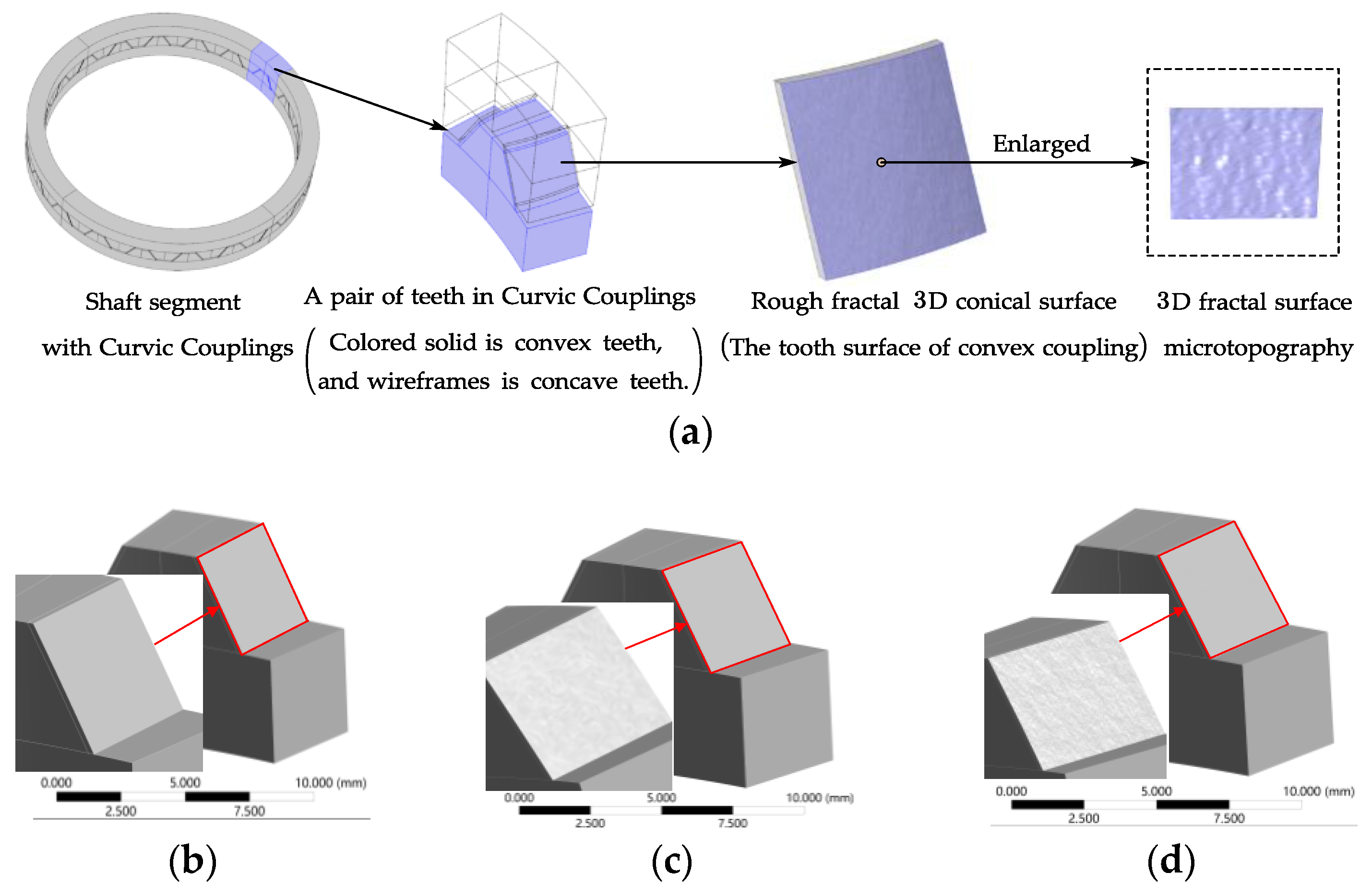
2.1. Rough Three-Dimensional Fractal Surface Modeling of Curvic Couplings Interfaces
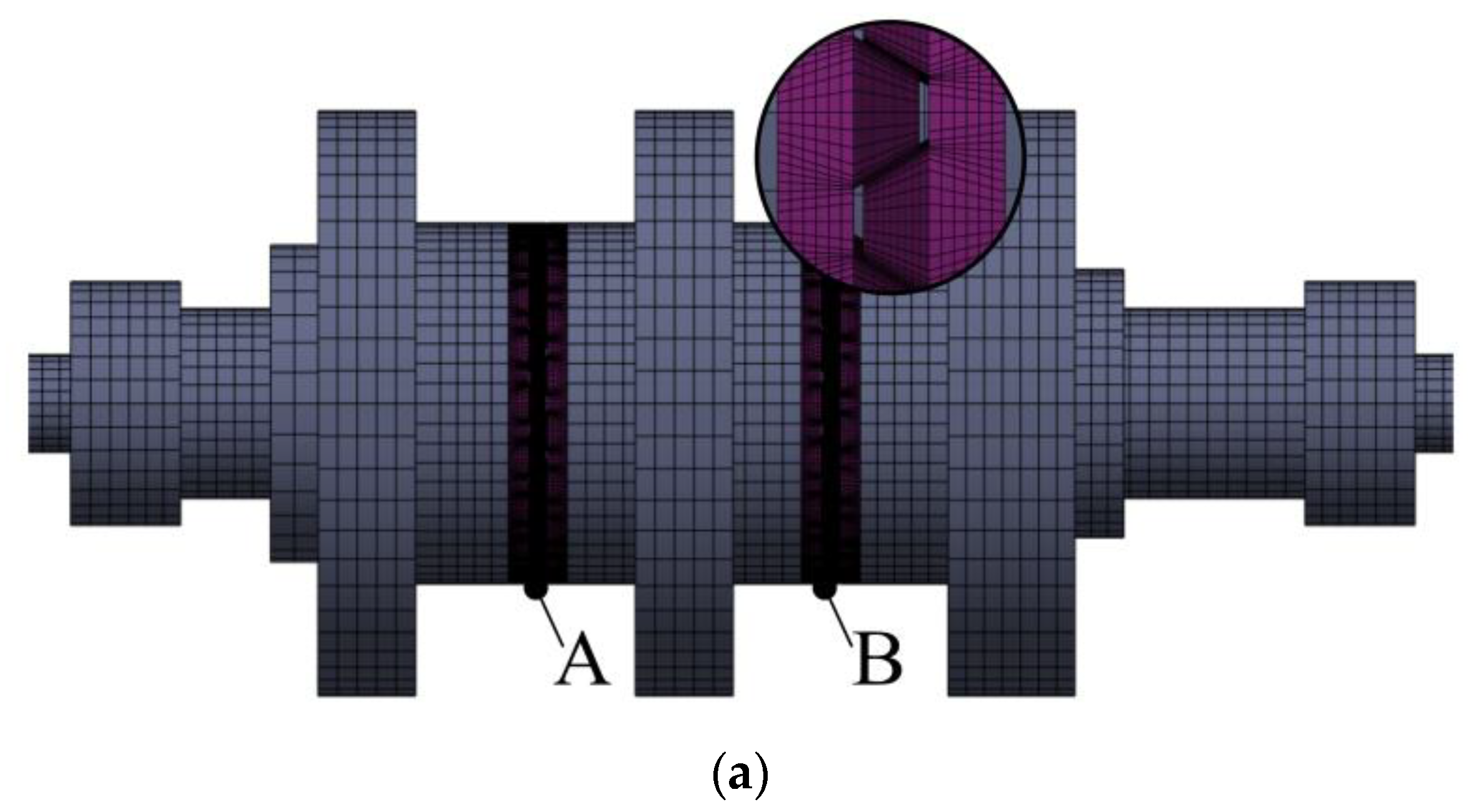
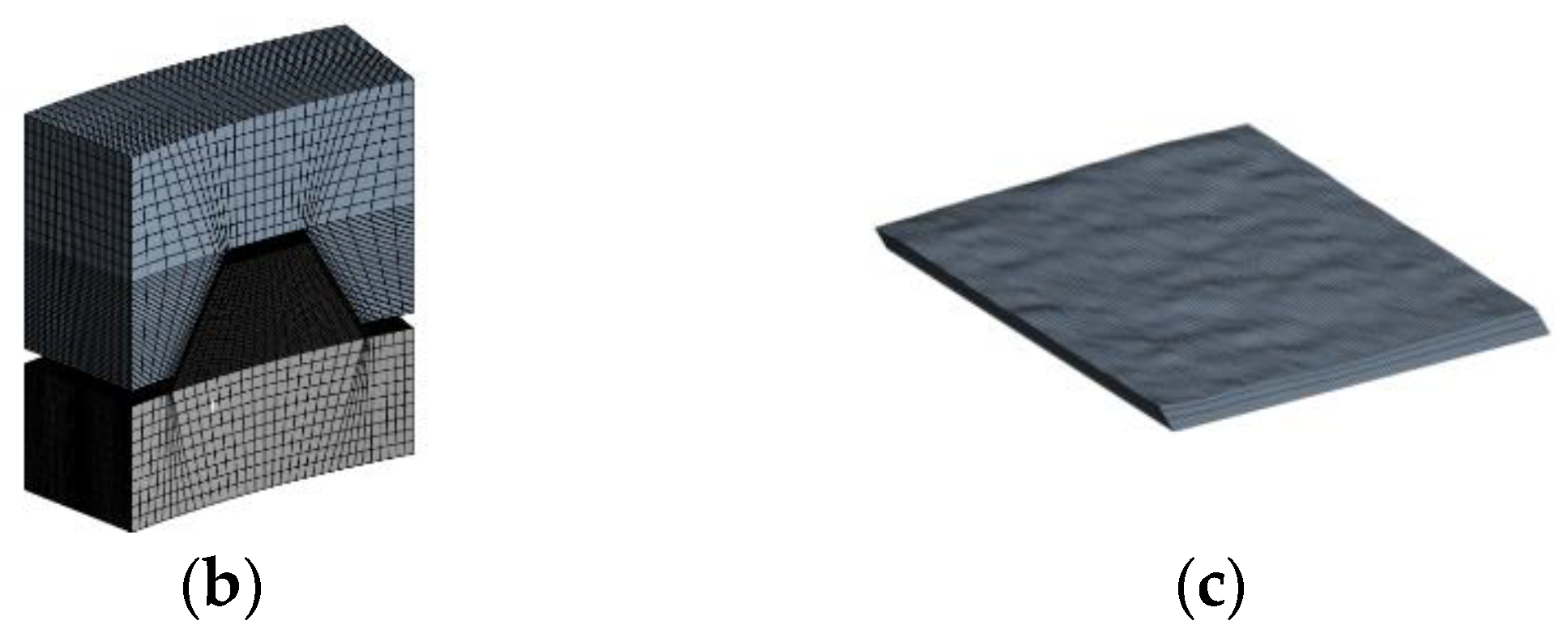
2.2. Combined Rotor Model with Curvic Couplings Considering Rough Interfaces
3. Static Analysis of Curvic Couplings Considering Rough Interfaces
3.1. Boundary Conditions for Static Analysis
3.2. Static Analysis Results
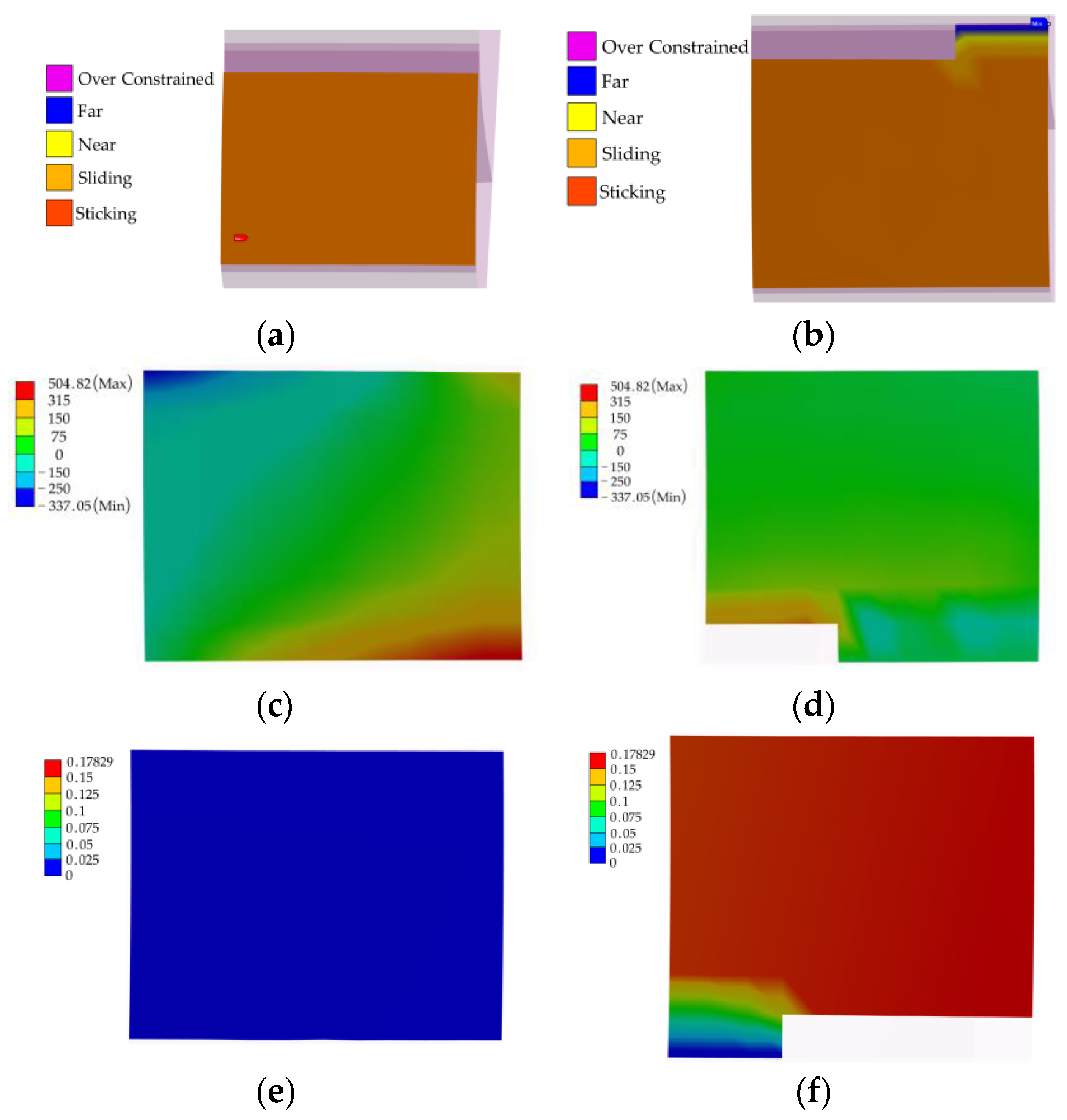
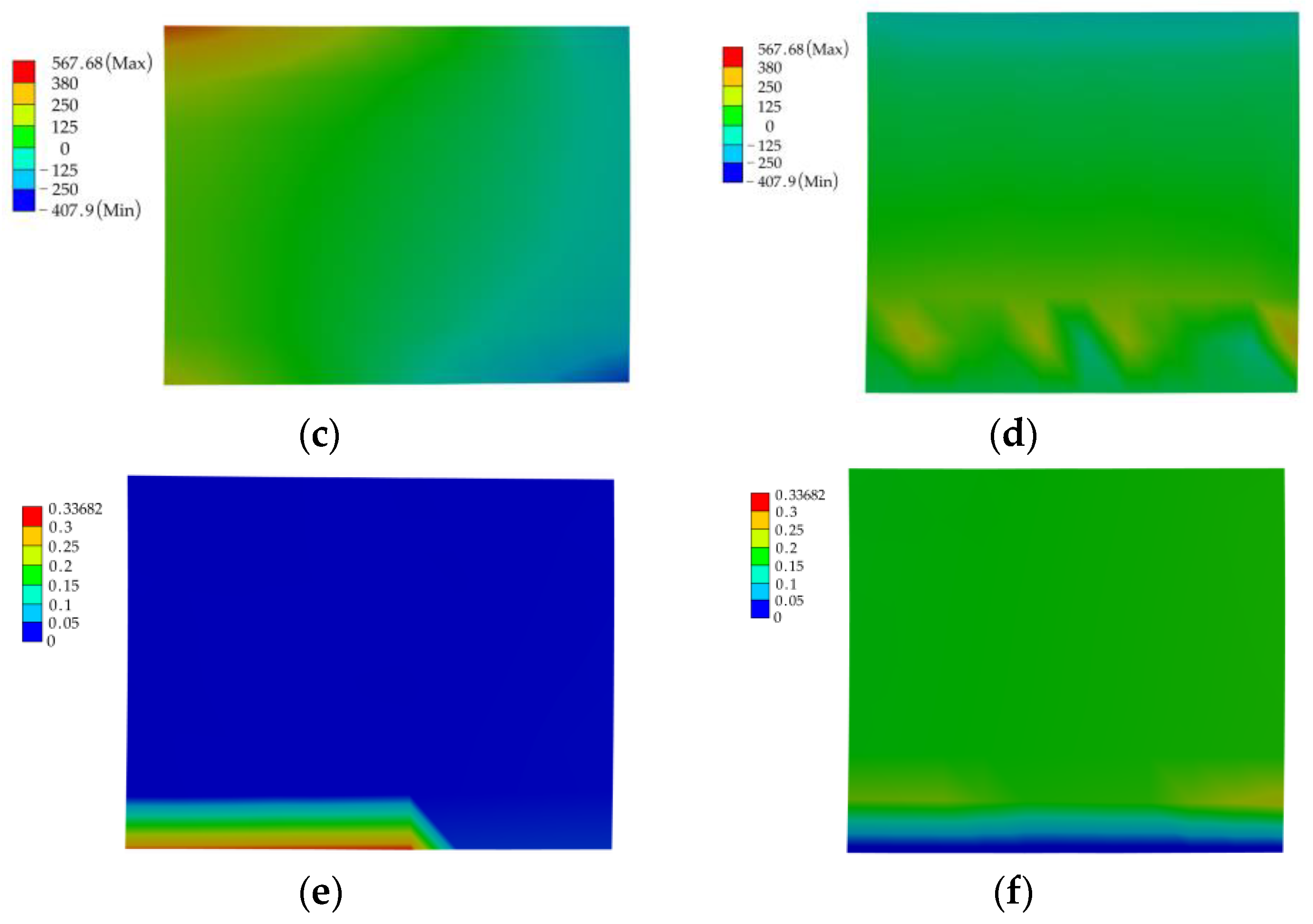
3.2.1. Contact Characteristics of the Curvic Couplings Interface with Different Roughness
3.2.2. Deformation of Curvic Couplings with Different Roughness
3.2.3. Von Mises Stress of Curvic Couplings with Different Roughness
4. Dynamic Analysis of Curvic Couplings Considering Rough Interfaces
- (1)
- The dynamic stress conditions after balance deterioration under extreme conditions of 80 g mm and 160 g mm at the resonance speed (5500 rpm);
- (2)
- The change in dynamic stress as the residual imbalance increases from 5 g mm to 30 g mm under the maximum operating speed limit (4400 rpm).
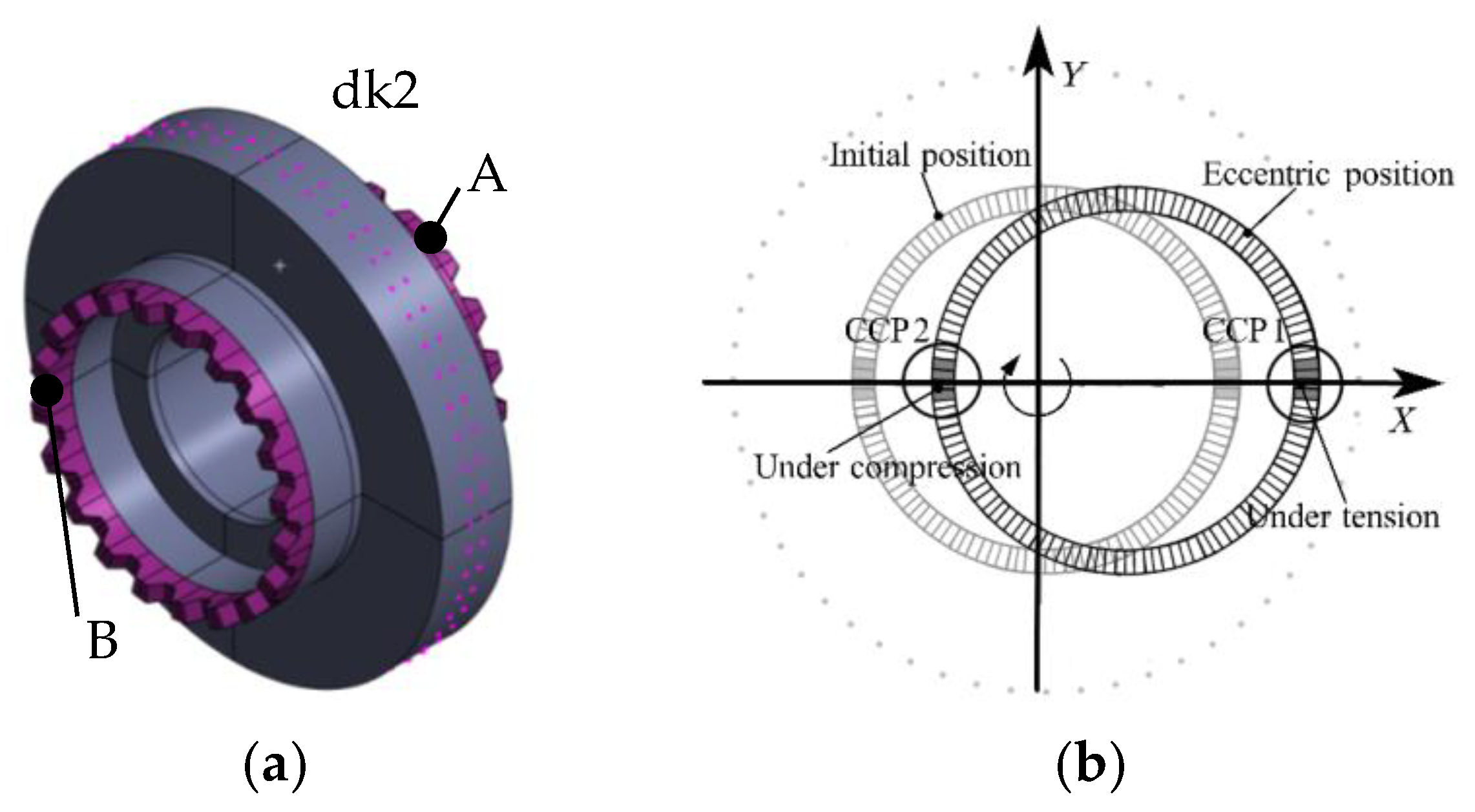
4.1. Boundary Conditions for Dynamic Analysis
4.2. Dynamic Analysis Results
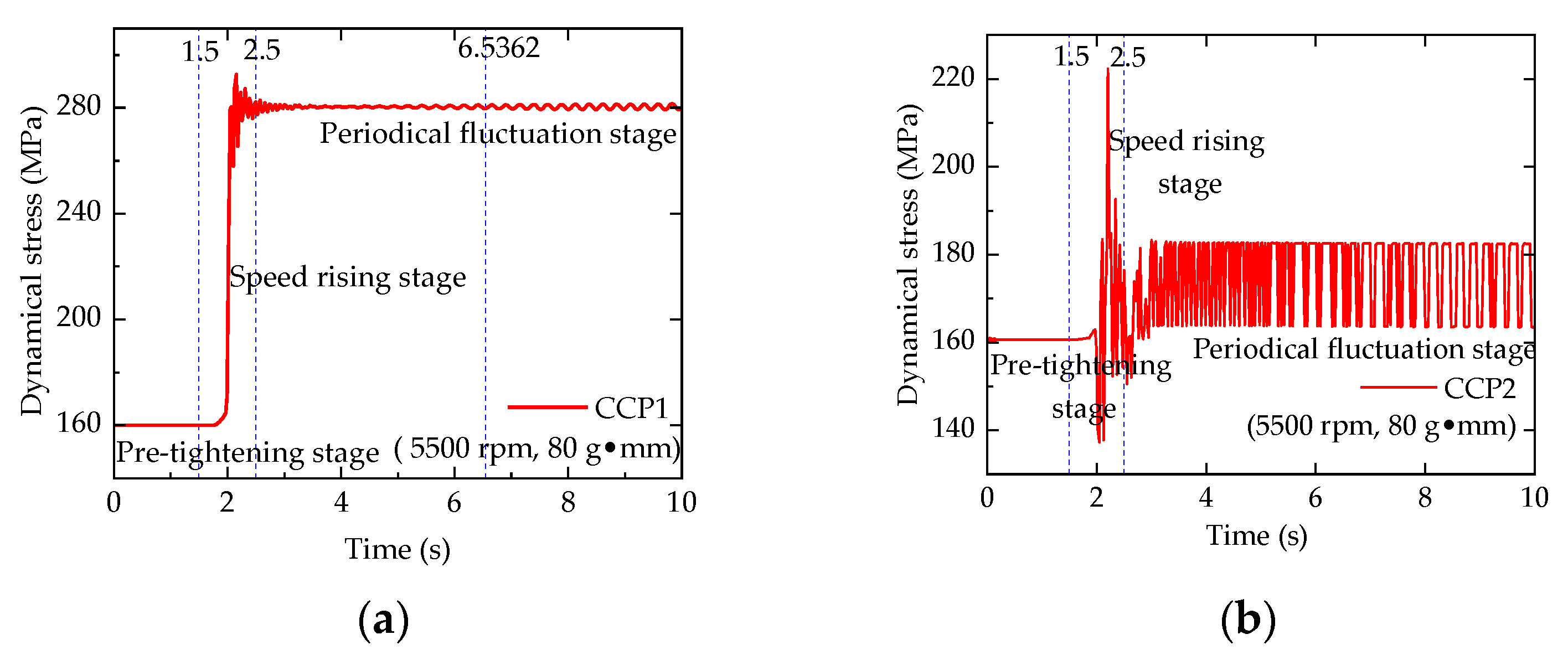
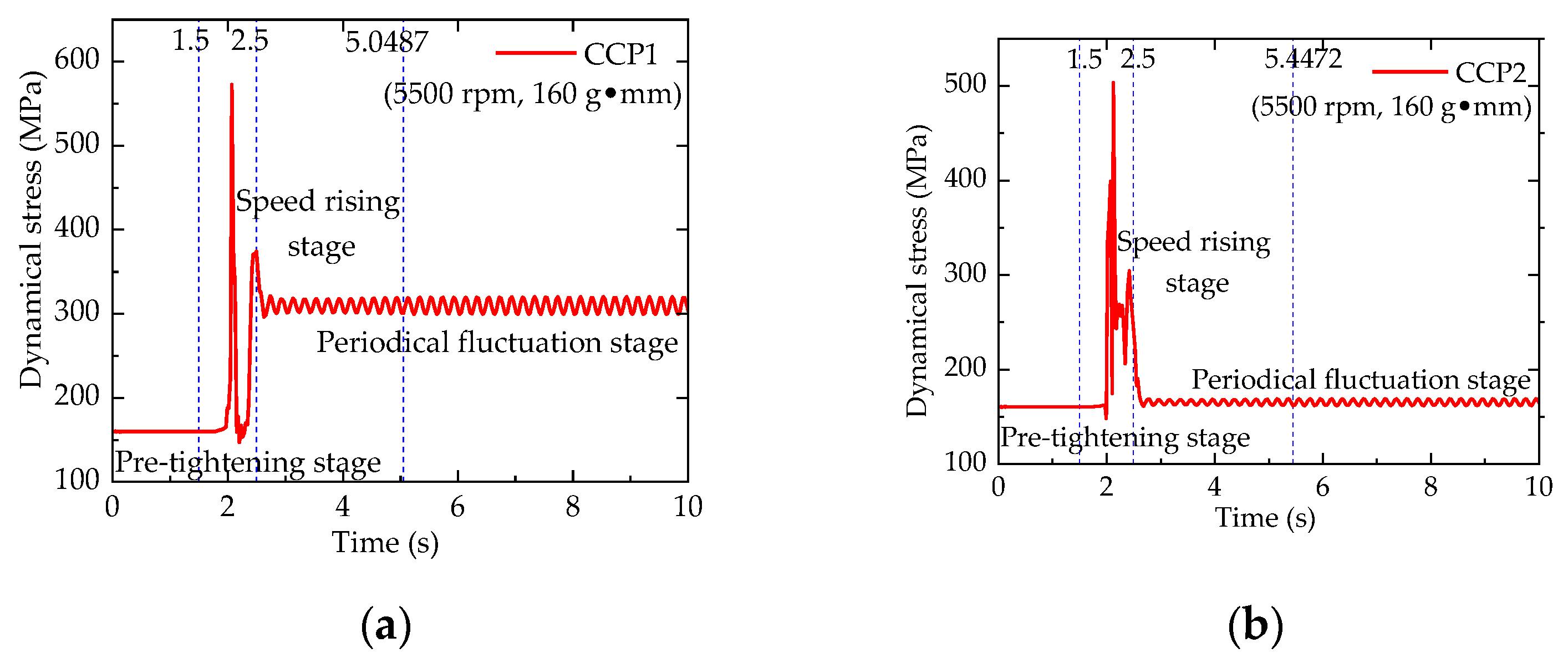
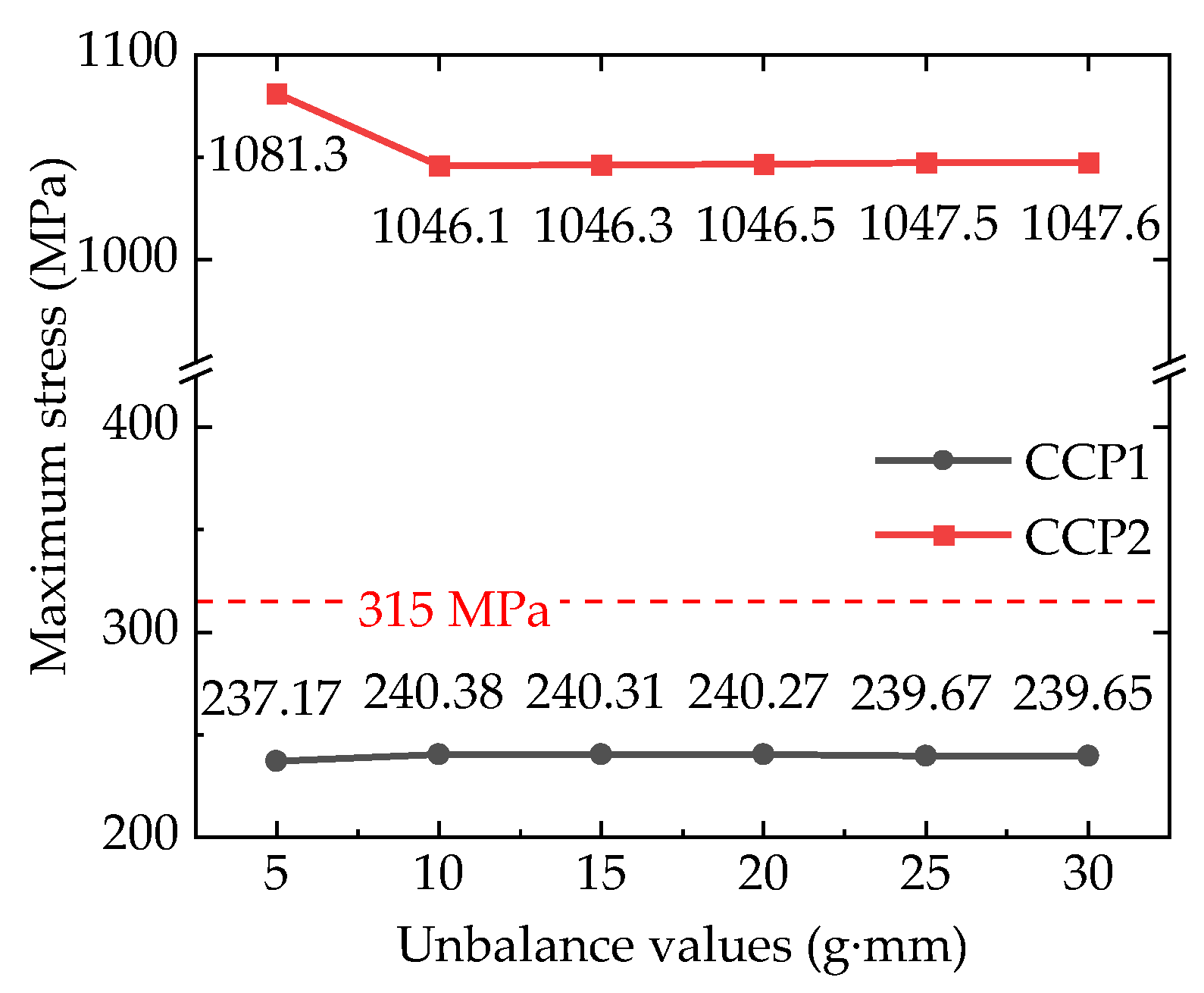
4.2.1. Resonance Speed 5500 rpm with Different Imbalance Values
4.2.2. Maximum Operating Speed 4400 rpm with Different Residual Imbalance Values
5. Experimental Verification
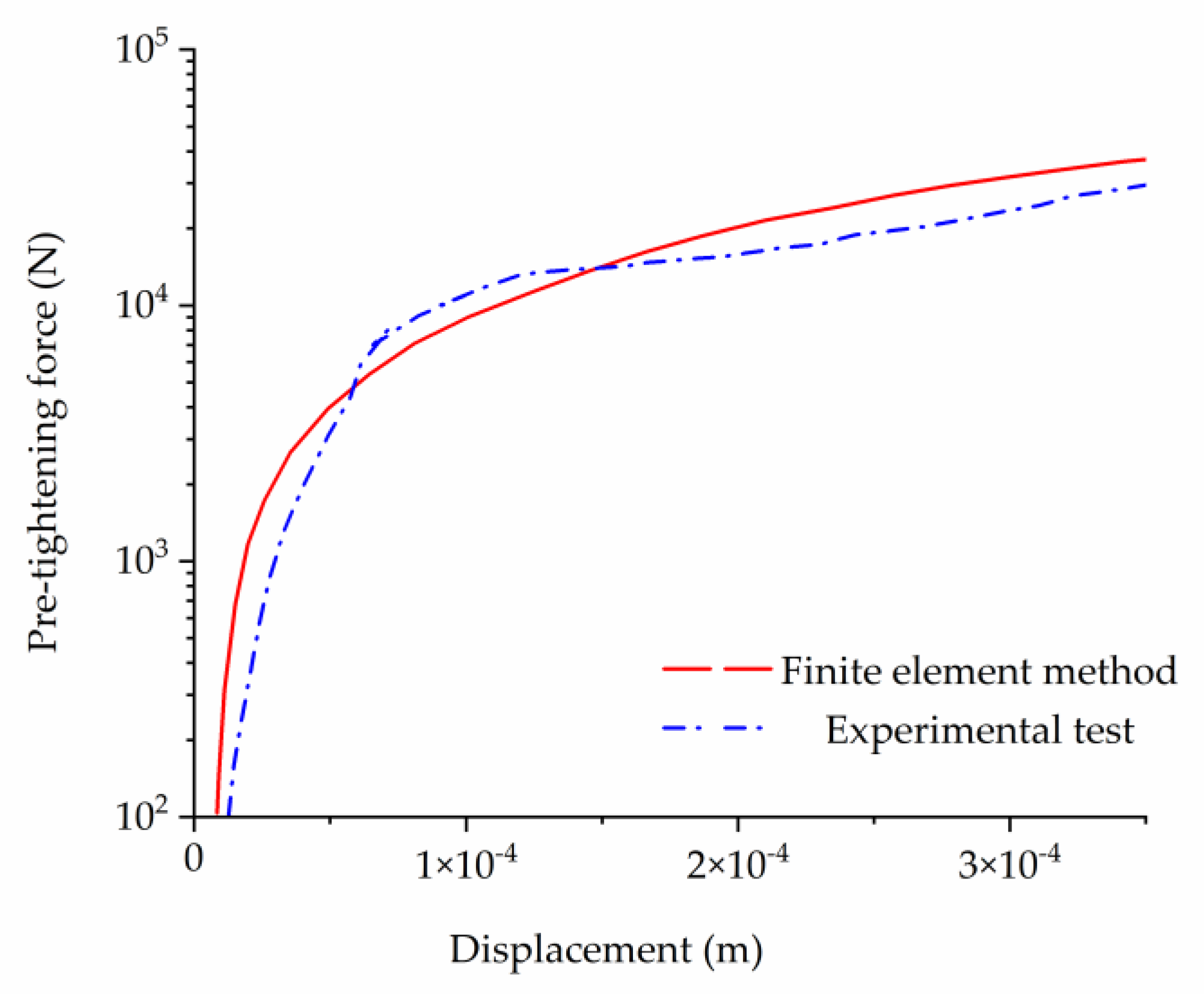
5.1. Compression Experiment
5.2. Modal Experiment
6. Conclusions
- (1)
- This paper proposes a modeling method for combined rotors with curvic couplings that consider rough interfaces. Combined with the finite element method, it can be used for mechanical analysis of the rotors with curvic couplings, providing a theoretical approach for studying the mechanical characteristics of the rotors’ curvic couplings in turboshaft engines in engineering applications;
- (2)
- By analyzing the static characteristics of the rough interface of curvic couplings, this study explores the effects of different roughness levels on the deformation and stress distribution patterns of the contact surface under static loads. The roughness of the interface significantly influences the deformation of the contact surface, but its impact on the overall deformation of the rotor is relatively minor. The static stress distribution across the interface is largely influenced by the geometric features of the teeth;
- (3)
- By analyzing the dynamic characteristics of the contact surface of the rotor with curvic couplings, this study explores the variation patterns of rotor dynamic stress at resonance speed and maximum operating speed. The dynamic stress results of curvic couplings interface exhibit periodic variation characteristics. Under the same rotational speed but different imbalance quantities, the variation period and amplitude of the dynamic stress fluctuation region of the curvic couplings interface both change over time. The dynamic stress amplitude typically appears during the acceleration region of the rotational speed and tends to stabilize at a certain moment. At the maximum operating speed, under the condition of allowable residual imbalance in the rotor system, the imbalance has a change rate of less than 5% on the dynamic stress of the contact surface of curvic couplings. The maximum stress on the contact surface of CCP2 is 243.27% of the yield strength, exceeding the material’s yield limit, which can cause damage to the strength of the curvic couplings interface.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Pisani, S.R.; Rencis, J.J. Investigating curvic coupling behavior by utilizing two- and three-dimensional boundary and finite element methods. Eng. Anal. Bound. Elem. 2000, 24, 271–275. [Google Scholar] [CrossRef]
- Richardson, I.J.; Hyde, T.H.; Becker, A.A.; Taylor, J.W. A validation of the three-dimensional finite element contact method for use with Curvic couplings. Proc. Inst. Mech. Eng. Part G J. Aerosp. Eng. 2002, 216, 63–75. [Google Scholar] [CrossRef]
- Jiang, X.J.; Zhang, Y.Y.; Yuan, S.X. Analysis of the contact stresses in curvic couplings of gas turbine in a blade-off event. Strength Mater. 2012, 44, 539–550. [Google Scholar] [CrossRef]
- Yuan, S.X.; Zhang, Y.Y.; Zhang, Y.C.; Jiang, X.J. Stress distribution and contact status analysis of a bolted rotor with curvic couplings. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2010, 224, 1815–1829. [Google Scholar] [CrossRef]
- Efstathiou, C.; Tsormpatzoglou, I.; Tapoglou, N. Parametric Modeling of Curvic Couplings and Analysis of the Effect of Coupling Geometry on Contact Stresses in High-Speed Rotation Applications. Machines 2023, 11, 822. [Google Scholar] [CrossRef]
- Liu, H.; Hong, J.; Ruan, S.L.; Li, Z.; Cheng, G.D. A Model accounting for Stiffness Weakening of Curvic Couplings under Various Loading Conditions. Math. Probl. Eng. 2020, 2020, 1–17. [Google Scholar] [CrossRef]
- Liu, H.; Hong, J.; Zhang, D.Y. Bending and vibration of a discontinuous beam with a curvic coupling under different axial forces. Front. Mech. Eng. 2020, 15, 417–429. [Google Scholar] [CrossRef]
- Zhang, D.Y.; Yang, C.; He, T.A.; Liu, J.X.; Hong, J. Modelling and stress analysis for double row curvic couplings. Proc. Inst. Mech. Eng. Part C-J. Eng. Mech. Eng. Sci. 2021, 235, 4231–4243. [Google Scholar] [CrossRef]
- Heng, X.; Wang, A.L. Stiffness Loss of Curvic Coupling Under Bending Vibration and Its Effect on Dynamic Characteristics of Rotor Systems. IEEE Access 2024, 12, 102774–102783. [Google Scholar] [CrossRef]
- Wang, C.Y.; Li, Z.H.; Wang, H.Z.; Ji, X.L.; Yang, L.H. Nonlinear modeling and vibration response analysis of rod fastening rotor-bearing system with curvic coupling. Tribol. Int. 2024, 199, 109984. [Google Scholar] [CrossRef]
- Zhao, R.C.; Jiao, Y.H.; Qu, X.Q.; Zhang, S.; Wu, X.L. Modeling method of gas turbine rotor contact surface based on virtual material layer. J. Harbin Inst. Technol. 2021, 53, 117–123. [Google Scholar]
- Rimpel, A.M.; Leopard, M. Simple contact stiffness model validation for tie bolt rotor design with butt joints and pilot fits. J. Eng. Gas Turbines Power 2020, 142, 011014. [Google Scholar] [CrossRef]
- Yu, P.C.; Zhao, Z.M.; Hou, L.; Chen, G. Analysis on analytical modelling and damping characteristics of bolted joint structure with spigot. J. Aerosp. Power 2021, 36, 2490–2502. [Google Scholar]
- Liu, D.; Zhou, L.; Zhang, D.Y.; Wang, H. Modeling and dynamic analysis for rotors with curvic-coupling looseness. J. Vib. Control. 2023, 29, 1996–2009. [Google Scholar] [CrossRef]
- Croccolo, D.; De Agostinis, M.; Fini, S.; Olmi, G.; Robusto, F.; Vincenzi, N. On Hirth Ring Couplings: Design Principles Including the Effect of Friction. Actuators 2018, 7, 79. [Google Scholar] [CrossRef]
- Gao, J.; Yuan, Q.; Li, P.; Feng, Z.P.; Zhang, H.T.; Lv, Z.Q. Effects of Bending Moments and Pre-tightening Forces on the Flexural Stiffness of Contact Interfaces in Rod-Fastened Rotors. J. Eng. Gas. Turbines Power 2012, 134, 102503. [Google Scholar] [CrossRef]
- Jin, M. The nonlinear dynamic characteristics of the aero-turboshaft engine rotor blade casing rubbing system with the curvic couplings considering the elastoplastic stage. Eng. Anal. Bound. Elem. 2024, 161, 78–102. [Google Scholar] [CrossRef]
- Kim, B.J.; Oh, J.; Palazzolo, A. An improved preloaded Curvic coupling model for rotordynamic analyses. J. Sound Vib. 2023, 544, 117391. [Google Scholar] [CrossRef]
- Kim, B.J.; Oh, J.; Palazzolo, A. Beam based rotordynamics modelling for preloaded Hirth, Curvic and butt couplings. J. Sound Vib. 2023, 565, 117921. [Google Scholar] [CrossRef]
- Yuan, S.X. The Structure and Strength of Curvic Couplings; China Petrochemical Press: Beijing, China, 2022; pp. 19–24. [Google Scholar]
- Sun, H.B.; Liu, M.; Zhang, Y.Z.; Wei, C.L. Optimizing circumferential assembly angle of rotor parts connected by curvic couplings based on acquired tooth surface error data. Chin. J. Aeronaut 2024, 37, 404–441. [Google Scholar] [CrossRef]
- Komvopoulos, K.; Ye, N. Three-dimensional contact analysis of elastic-plastic layered media with fractal surface topographies. J. Trib. 2001, 123, 632–640. [Google Scholar] [CrossRef]
- Liou, J.L.; Tsai, C.M.; Lin, J.F. A microcontact model developed for sphere- and cylinder-based fractal bodies in contact with a rigid flat surface. Wear 2010, 268, 431–442. [Google Scholar] [CrossRef]
- Yuan, Y.; Zhang, J.; Cheng, Y. An elastoplastic fractal model for cylinder in contact with a smooth plane. Sci. Sin. Tech. 2017, 47, 502–513. [Google Scholar] [CrossRef]
- Zhang, B.; Zhou, L. Feature Analysis of Fractal Surface Roughness Based on Three-dimensional W-M Function. J. Phys. Conf. Ser. 2021, 1906, 012020. [Google Scholar] [CrossRef]
- Wu, D.G.; Gang, T.Q.; Fan, X.G.; Wu, J.L. Surface Roughness Measuring Based on the Theory of Fractal Geometry. Appl. Mech. Mater. 2014, 551, 434–438. [Google Scholar] [CrossRef]
- Reddy, J.N. Introduction to the Finite Element Method, 4th ed.; McGraw Hill Education: New York, NY, USA, 2019; pp. 759–764. [Google Scholar]
- Song, M.B.; Yan, P.; Wang, X.; Xiang, Y.Z.; Yang, Y.; Xie, R.H. Exploration of Maximum Unbalance of a Turboshaft Engine According to Airworthiness requirements. J. Aerosp. Power 2023, 38, 1467–1473. [Google Scholar]

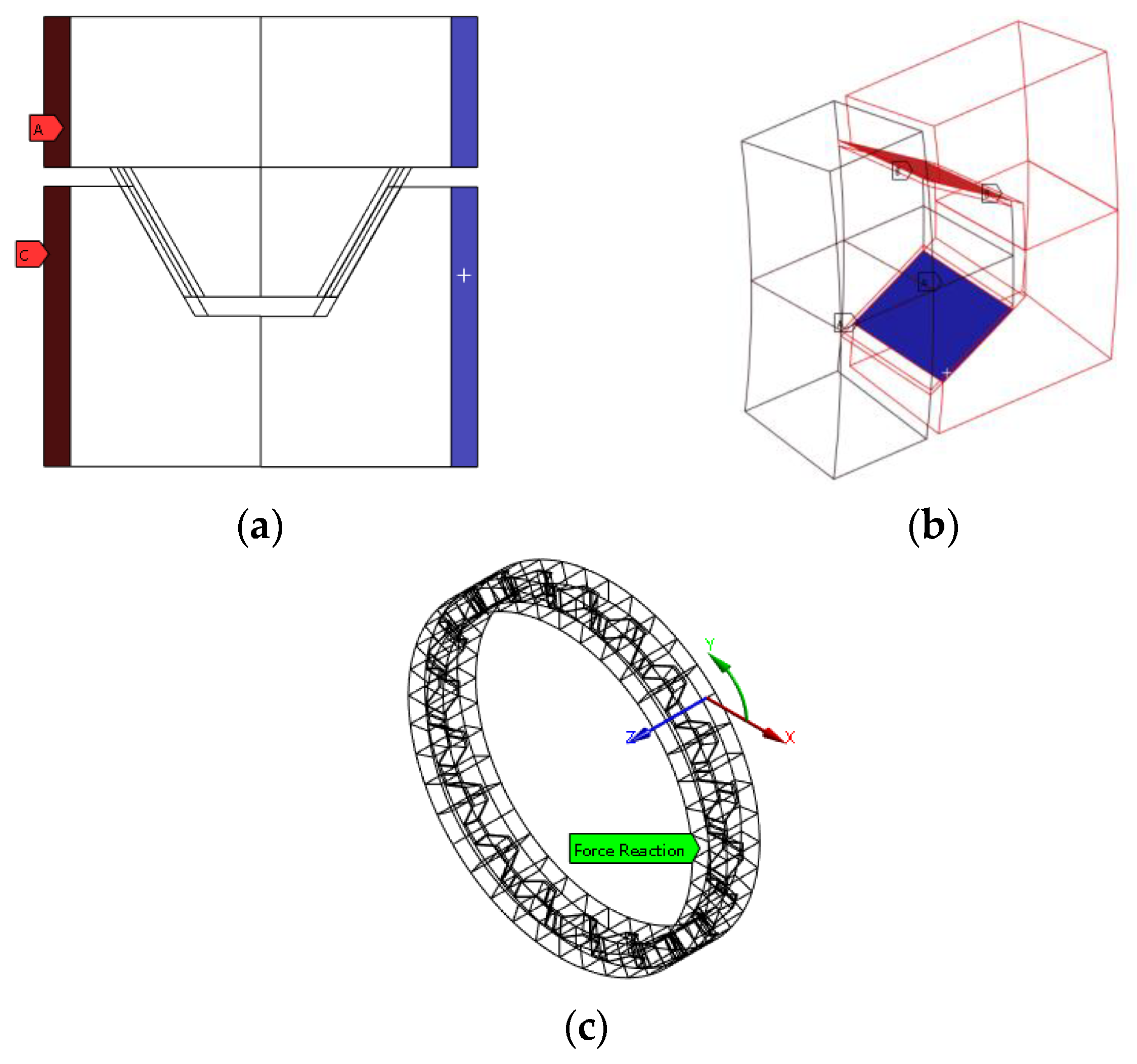
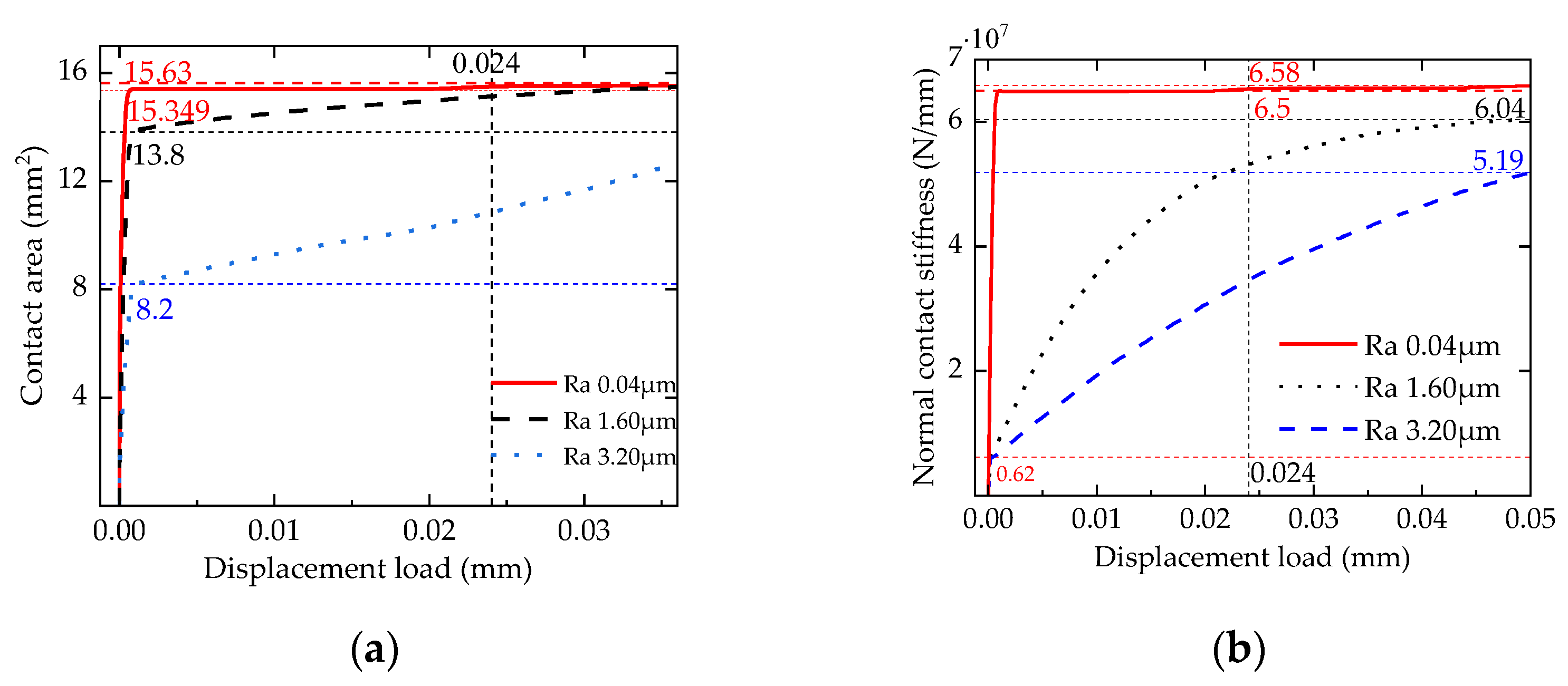

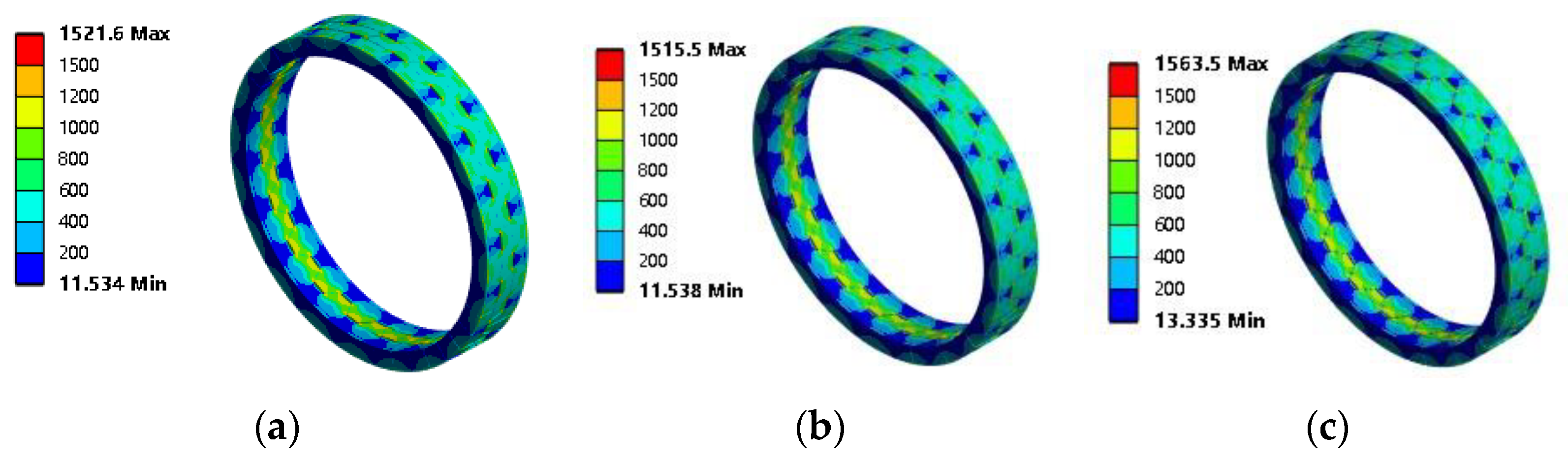
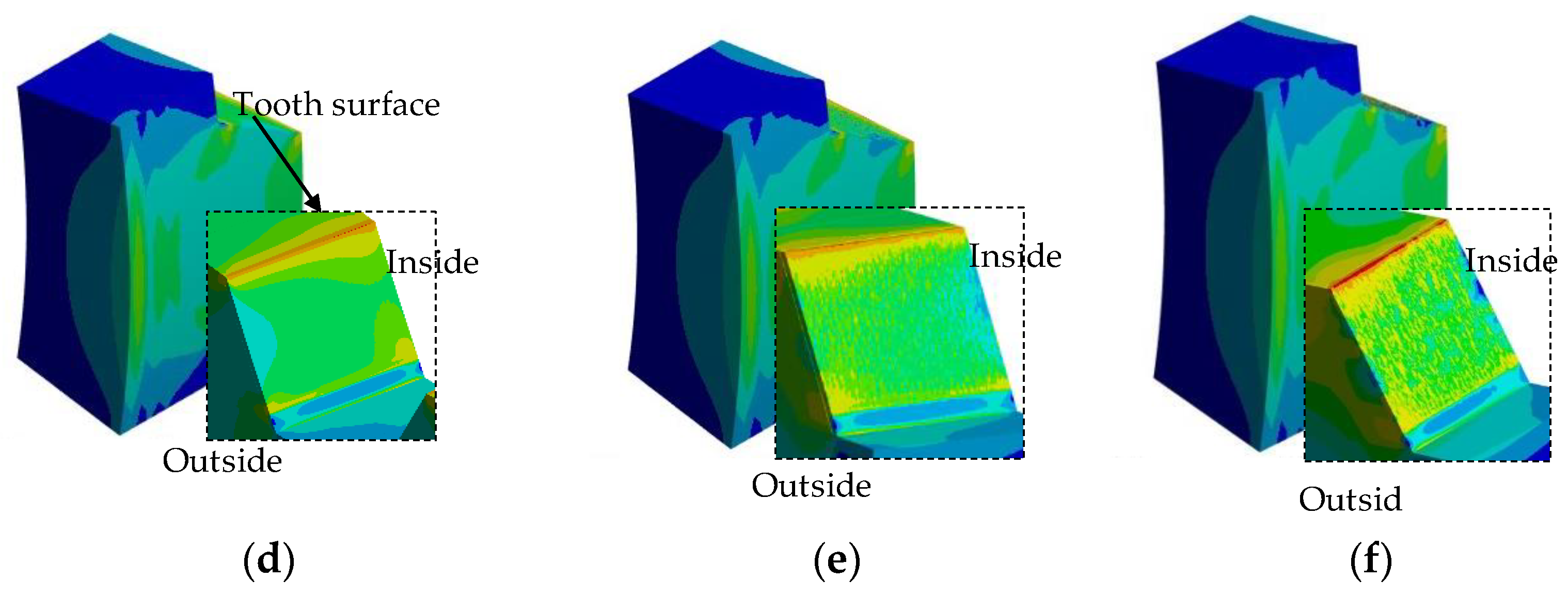





| Surface Roughness Ra/μm | Fractal Dimension D | Characteristic Length Scale G/10−6 mm |
|---|---|---|
| 0.04 | 2.5818 | 0.01012 |
| 1.6 | 2.5334 | 3.1233 |
| 3.2 | 2.5114 | 12.6441 |
| Parameter | Outer Diameter Do | Inner Diameter Di | Number of Teeth Z | Pitch Number Nx | Pressure Angle α | Height of Tooth Head ha | Height of Tooth Root hb |
|---|---|---|---|---|---|---|---|
| Values | 74 mm | 65 mm | 20 | 19 | 30° | 1.48 mm | 1.98 mm |
| Modal Number | FEM (Hz) | Experiment (Hz) | Relative Error (%) |
|---|---|---|---|
| 1 | 6.876 | 7.031 | 2.20 |
| 2 | 68.913 | 66.719 | 3.29 |
| 3 | 198.92 | 199.245 | 0.16 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yin, Y.; Heng, X.; Zhang, H.; Wang, A. Static and Dynamic Stress of the Combined Rotor with Curvic Couplings Considering the Rough Three-Dimensional Interface at Extreme Operating Conditions. Machines 2024, 12, 696. https://doi.org/10.3390/machines12100696
Yin Y, Heng X, Zhang H, Wang A. Static and Dynamic Stress of the Combined Rotor with Curvic Couplings Considering the Rough Three-Dimensional Interface at Extreme Operating Conditions. Machines. 2024; 12(10):696. https://doi.org/10.3390/machines12100696
Chicago/Turabian StyleYin, Yijun, Xing Heng, Haibiao Zhang, and Ailun Wang. 2024. "Static and Dynamic Stress of the Combined Rotor with Curvic Couplings Considering the Rough Three-Dimensional Interface at Extreme Operating Conditions" Machines 12, no. 10: 696. https://doi.org/10.3390/machines12100696
APA StyleYin, Y., Heng, X., Zhang, H., & Wang, A. (2024). Static and Dynamic Stress of the Combined Rotor with Curvic Couplings Considering the Rough Three-Dimensional Interface at Extreme Operating Conditions. Machines, 12(10), 696. https://doi.org/10.3390/machines12100696





