1. Introduction
Oral hygiene is one of the most discussed topics in dentistry. Poor oral hygiene can lead to several oral diseases. In [
1], the World Health Organization highlights that the global situation regarding oral health is alarming and requires urgent action. Over the past 30 years, oral disease cases have increased by about 1 billion. The main reason for this increase is the lack of oral health care. One of the key factors in reducing cases of oral diseases is prevention. Good oral hygiene greatly lowers the chance of developing oral diseases. Therefore, the topic of cleaning teeth is a very important issue. The tools available for oral hygiene can be classified into toothbrushes (and toothpaste), dental floss, and periodic cleanings at professional dentists.
For many years, one of the most discussed dental topics has been the correct use of the toothbrush to clean the teeth. Proper use of the toothbrush allows you to perform a complete cleaning without damaging the tooth. In particular, three main factors can be identified that impact the effectiveness of brushing: the type of toothpaste used; toothbrush handling; and the shape and materials with which the toothbrush is characterized.
In the literature, several experimental or clinical trials have been carried out on the analysis of toothbrush wear. A study [
2] reported on the erosion generated from toothpaste slurries of different abrasive levels and toothbrushes of different filament diameters. A systematic review [
3] reports how the effects of bristle stiffness and bristle end shape influence tooth wear. The impact of toothbrushes with tapered and cross-angled soft bristle designs on dental plaque and gingival inflammation through a randomized and controlled clinical trial is discussed in [
4]. An analysis of the wear of different toothbrushes that have bristles of different stiffness was experimentally verified in [
5]. Analysis shows that as stiffness increases, wear increases despite the overall force applied by the toothbrush being kept constant.
Other experimental comparisons have been made on thin-bristled toothbrushes (ultrasoft toothbrushes) [
6]. In this study, it is demonstrated that different types of ultra-soft toothbrushes have a different effect on the progression of erosive tooth wear as a function of the substrate (dentine or enamel).
As computing resources increase, numerical simulation becomes increasingly important for component design. For this reason, numerical simulation has become a very powerful tool for understanding the influence of design parameters (bristle shape and materials). In recent years, there have been a few attempts to synthesize the toothbrushing phenomenon through mathematical or numerical models. In particular, in 1990, Rawls et al. [
7] developed a mathematical model to predict the stiffness of toothbrushes. Despite this, several numerical or mathematical models have been developed to study the phenomenon of brushing machine tools.
For example, several studies on wire brushes can be found in the literature. Stango, adopting the classical beam theory in [
8], deduced equations for calculating the bending of the bristles acting on a flat surface. Heinrich extended Stango’s formulation for bristles acting on curved surfaces [
9]. Subsequently, they validated the numerical results obtained with an experimental campaign [
10,
11]. Shia, belonging to the same research group, introduced the influence of friction in 1994 [
12], while four years later he developed the first 2D discrete model based on the Lagrange equations to calculate more precisely the contact forces exchanged between the bristles and the surface [
13].
In the same way, many studies on rotary cup brushes are present in the literature. Abded developed the firm finite element model (FEM) to analyze the brush characteristics of rotatory brushes [
14], while Venegas-Useche [
15] determined through a FEM the coefficients of friction between the bristles of a cutting brush of street sweepers and a concrete road surface, and in [
16] the effectiveness of gutter brushes in removing street sweep waste is verified through experiments.
In recent years, several numerical approaches based on multibody dynamics methodology have been introduced to simulate the tridimensional effects of flexible components. Uhlmann in [
17] proposes a three-dimensional dynamic contact analysis of abrasive filaments with a multibody system to analyze abrasive brushes. Ma [
18] developed a dynamic model to analyze satellite detumbling using a brush-type contactor based on flexible multibody dynamics. Liu [
19] adopted a flexible approach to create a dynamic model of a brush sampling mechanism. Ma [
20] introduced a flexible brush-type model to identify wear in soil removal machines and increase the machine’s lifetime. Recently, this approach has been introduced even in the simulation of toothbrushes. In [
21], a comparison of several multibody approaches to modeling toothbrush bristles elasto-kinematics is shown.
According to the authors’ knowledge, numerical models have not yet been developed for the dynamic simulation of toothbrushes capable of accurately identifying the three-dimensional displacement of the bristles and consequently evaluating the force exchanged with the teeth. The advantage of developing a numerical model lies not only in the possibility of being able to predict the overall behavior of the toothbrush but, above all, in the possibility of being able to evaluate the contribution of each bristle. In this way, it is possible to have a more detailed result that will surely give additional information for shape optimization processes.
This paper proposes a flexible multibody model to efficiently simulate the 3D compliance of a toothbrush. Compared to the classical finite element approach, the flexible multibody technique is lighter from a computational point of view and ensures the same degree of accuracy [
22,
23]. There are several approaches to introducing flexibility in multibody methods [
24,
25,
26]. This paper considers each bristle as a flexible beam; for this reason, they have been modeled according to discrete flexible theory. This method considers a flexible body as a series of rigid bodies connected by lumped stiffness entities. Contact between the teeth and the target surface, such as the contact between the tips of the bristles, is established using the penalty contact method (
Section 2.2). The model validation is performed by adopting an ad hoc experimental bench test. It is fulfilled using a Universal Robot and a flat, transparent surface. Validation is provided by comparing the reaction forces of the toothbrush with the reaction forces acquired by the load cells mounted on the end-effector of the Robot. Several trajectories of the toothbrush have been measured to point out the tridimensional motion. The results demonstrate the accuracy of estimating normal and tangential forces.
The paper is organized as follows: the
Section 2 reports a description of the methodology adopted to create the discrete flexible model; the
Section 3 regards the description of the case study; the experimental setup is described in
Section 4 and in
Section 5, the obtained results and the relative discussion are reported. At last, conclusions are presented.
2. Numerical Model
The model developed and used to calculate the dynamic behavior of the toothbrush is based on two important features: the dynamics of flexible structures undergoing large deflection and contact mechanics formulation to evaluate the interaction between the bristles and the surface to be cleaned and the interaction effect among the bristles themselves. The objective of the dynamic model is to examine the bristles’ motion and forces on the bristles to determine their brushing performance. As a result, the approach concentrates on modeling the bristles and simplifies the toothbrush by simply taking into consideration its head and ignoring its flexible handle. Even if the flexibility of the handle plays an important role in the entire elasto-kinematics of the toothbrush, the aim of this analysis is to isolate the specific contribution of bristle flexibility from other contributions that may be either due to the handle or, for example, to the compliance of the hand of the user performing the brushing. It is important to highlight that a tilt of the toothbrush head is reflected in a different decomposition of the tangential and normal forces, but it does not influence the reliability of the model.
2.1. Discrete Flexible Model
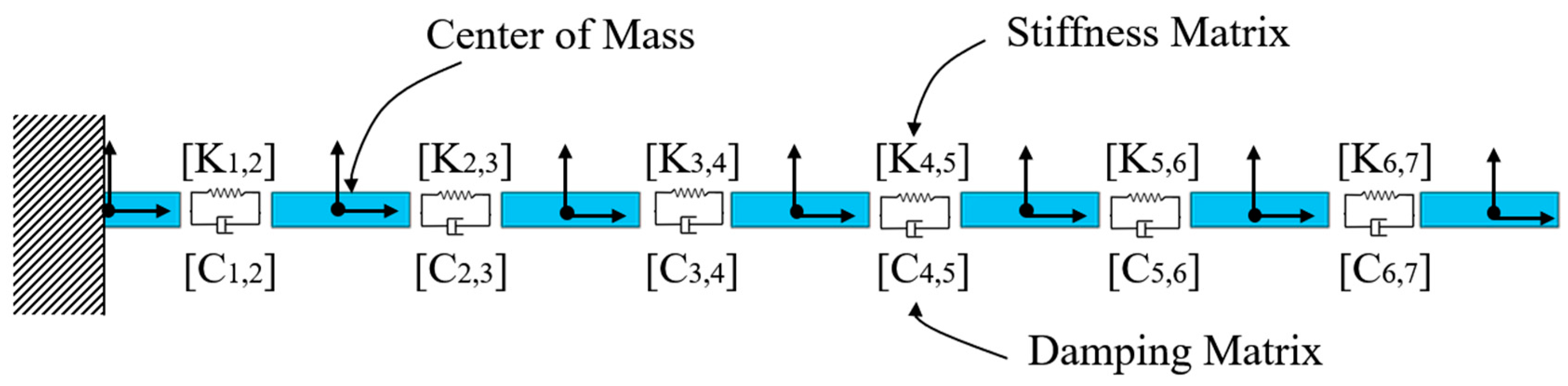
The approach adopted for the simulation of bristle compliance is the discrete flexible multibody model (DFM). Considering this approach, each bristle is discretized into several rigid bodies connected in series through stiffness and damping matrices (
Figure 1). Both inertial properties, stiffness, and damping matrix coefficients depend on the beam’s dimensions and material. Adjacent bodies interact due to stiffness matrices, which produce reaction forces and moment fields depending on the relative displacement between nodes’ coordinate reference systems. Each stiffness matrix can then be represented according to the Timoshenko beam theory [
27] as follows:
where
s is the shear area ratio, is the Young’s Modulus, is the shear modulus, is the section area, is the distance between adjacent nodes, and is the moment of inertia of the cross-section concerning the j-axis.
Moreover, each damping matrix can be defined as follows:
As a result, the forces and moments between the adjacent nodes can be expressed as:
where
and
are the vector of relative displacements (translational and rotational) and the relative velocity between the nodes’ generalized coordinates, respectively.
2.2. Contact Mechanics
The contact mechanics can be split into two phases: the detection phase and the restitution phase. In the detection phase, the bodies that come into contact are identified [
28], and the contact point(s) coordinates are assessed. This phase is subdivided into two separate sub-phases: a first sub-phase called pre-search and a second sub-phase called detailed search. In the first sub-phase, the overlapping of bounding boxes built around the geometric bodies in the simulation is verified. When two bounding boxes overlap, the second sub-phase is activated, reducing the integration time step and thus refining the accuracy of the contact point calculation. In this way, once the actual bodies come into contact, the restitution phase will be activated. The contact force computed in the restitution phase and applied at the contact point(s) is based on a penalty contact model. In this approach, the contact forces and the deformations are simulated with a set of spring-damper elements that represent the surface compliance of the contact bodies [
29]. The original approach, developed from the Hertz theory [
30,
31], was recently revised and modified to be more suitable for integration into full multibody models [
32,
33]. The enhanced contact formulation [
28,
34] allows calculating the normal force through the relationship:
where:
, are the penetration and the penetration rate;
m1, m2, and m3 are the exponents of stiffness, damping, and the indentation factor, respectively;
k and c are the stiffness and damping coefficients, respectively.
Stick-Slip Friction Model
A stick-slip algorithm in a tangential contact force model [
35] is adopted to compute the friction force. The friction force is obtained as follows:
where
and
represent the friction force during the stiction and sliding phases. These terms can be evaluated as
where
: is the stiction deformation that represents the maximum value of the body deformation before the sliding phase [
36].
: is a parameter depending on the sliding (tangential) velocity
: the sliding velocity
: the dynamic/sliding friction coefficient
: the static/stiction friction coefficient
and
represent the maximum value of the stiction deformation and the threshold velocity, respectively. Defining the terms
and
as the static friction force and the dynamic friction force expressed as:
and
, it is possible to introduce
Table 1, which represents the state of the variables as a function of the assumed conditions.
The step function mentioned in
Table 1 smooths the transition from stiction to slip conditions and is expressed as:
2.3. Dynamic Equation
Considering the Equations (5)–(7), the equations of motion of the generic
-th rigid body node can be written as follows:
where
is the mass matrix, i.e., ;
is the vector of the generalized acceleration ;
and are the stiffness matrices of the elastic compliance between node and nodes and , respectively;
and are the damping matrices between node and nodes and , respectively;
and are the relative displacement between nodes and nodes and generalized coordinates, respectively.
and are the contact forces and their moments with respect to the rigid body node location.
As can be seen, the Equation (11) contains first the inertial term related to all the masses in the model; The second, third, fourth, and fifth terms represent the elastic and damping forces generated by the elastic and damping matrices that connect the DFM bodies; and finally, the external forces (normal and tangential) that depend on the contact among the spheres and between the spheres and the outer surface.
3. Case Study
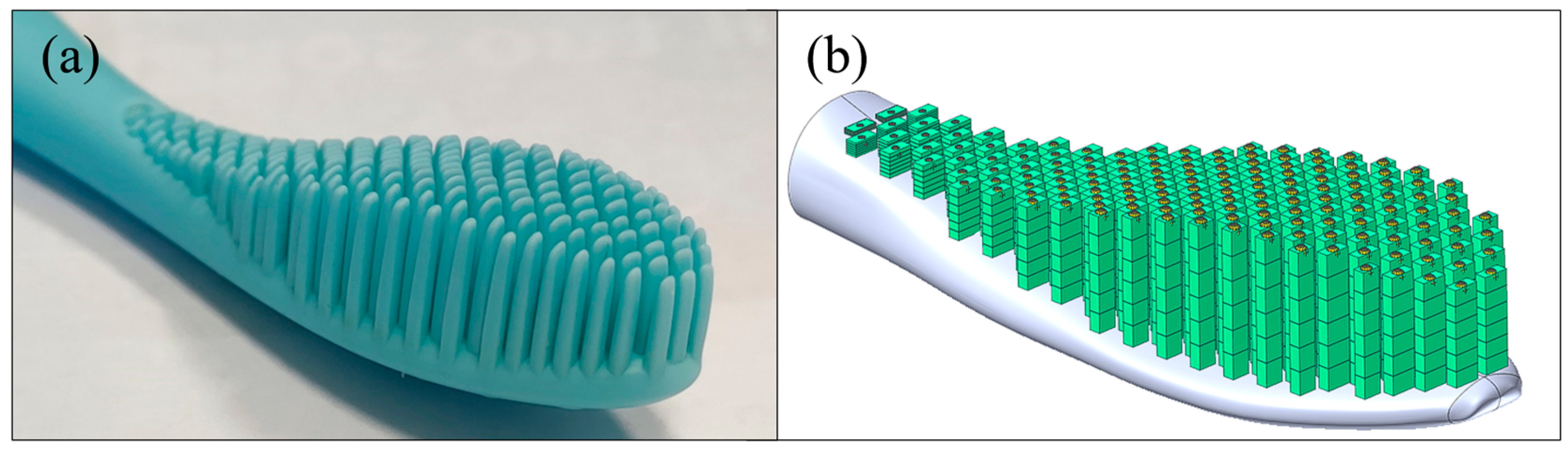
With the aim of verifying the methodology, a case study in which the toothbrush is constrained to slip on a flat surface is performed. The main scope of these boundary conditions is to check the numerical model’s behavior in a systematic and reproducible scenario. The brush is initially positioned in contact with the flat surface. A motion of 3 mm along the bristles axis is applied so that the inflection of the bristles occurs. Subsequently, a circular motion is imposed on the toothbrush. A measure of the normal and tangential forces is provided to compare the numerical model with the experimental. The tridimensional model is created by adopting reverse engineering techniques. In particular, the process was performed using a Microscribe GX2 by Revware, a 3D coordinate measuring machine (CMM) for implementing a point-data measurement of the brush’s base. Data points were taken at high density to reflect the complex shape of the object. The data points were then imported into a CAD environment to build curves and surfaces, thus recreating the main body. On the other hand, the use of a caliber allowed for the reverse engineering of the bristle locations and dimensions. The defined CAD geometry of the toothbrush is reported in
Figure 2b and compared with the real silicon sample in
Figure 2a.
In this phase, a detailed model of the number and shape of the bristles is provided. The next step has been the building of the multibody model. Each bristle is modeled through the DFM approach, considering six rigid bodies with rectangular sections equally distributed along the bristle length. This choice is made because bristles have different lengths. Although the real bristles of the toothbrush are tapered towards the contact end, the section of the modeled bristles is selected constantly along their axis. Moreover, the outer bristles have different section dimensions with respect to the inner ones. Namely, the internal bristle section is 1.8 mm × 0.8 mm, while the external bristle section is 1.3 mm × 0.8 mm. The lumped stiffness matrix is computed using the Equation (1) E = 7 GPa.
Each bristle is provided with a dummy spherical body on the tip with a radius as 0.44 mm to establish smooth contact and reduce the computational burden. A bounding box having is considered for the pre-search contact phase. Thanks to the dummies bodies, it has been possible to establish a contact relationship among the dummies adjacent spherical bodies to reproduce the interaction among the bristles. For this kind of contact, friction is neglected. Moreover, to reproduce the contact between the bristles and the flat surface, the base surface is approximated with a multi-triangular mesh, while the action surface, the analytical relation of the sphere, is considered. In this contact group, a stick-slip contact is considered.
It is crucial to highlight that the auto-interaction among the bristles represents an important contribution to a generalization of the problem because when the direction of motion changes, the interaction between the bristles leads to a reduction of normal forces as they collide with each other, exerting less pressure on the flat surface. The parameters adopted for the contact spheres and the flat surface are reported in
Table 2, while the contact parameters of interaction among the spheres are reported in
Table 3. On the one hand, the contact parameters for the evaluation of the normal force (i.e.,
,
,
mi) are set to ensure a penetration lower than 5 µm in the non-indentation region, therefore ensuring reliable contact detection and evaluation while guaranteeing the lowest possible computational burden. On the other hand, considering the tangential force characterization, the friction coefficients were assumed as suggested in the literature for dry silicon rubber and acrylic contact [
37,
38,
39].
4. Experimental Setup
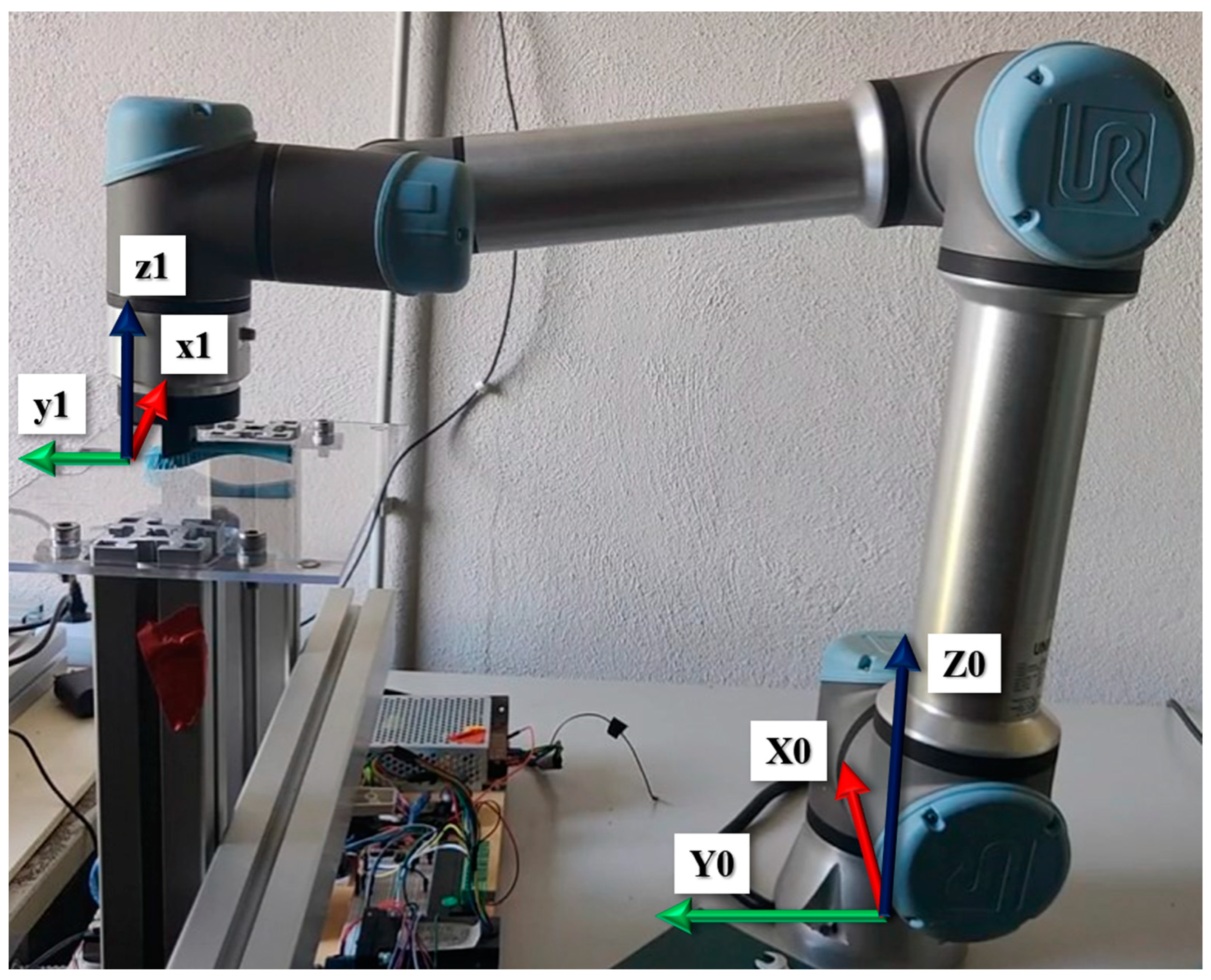
The experimental setup is based on the use of the Cobot UR5 [
40] to move the toothbrush as can be seen in
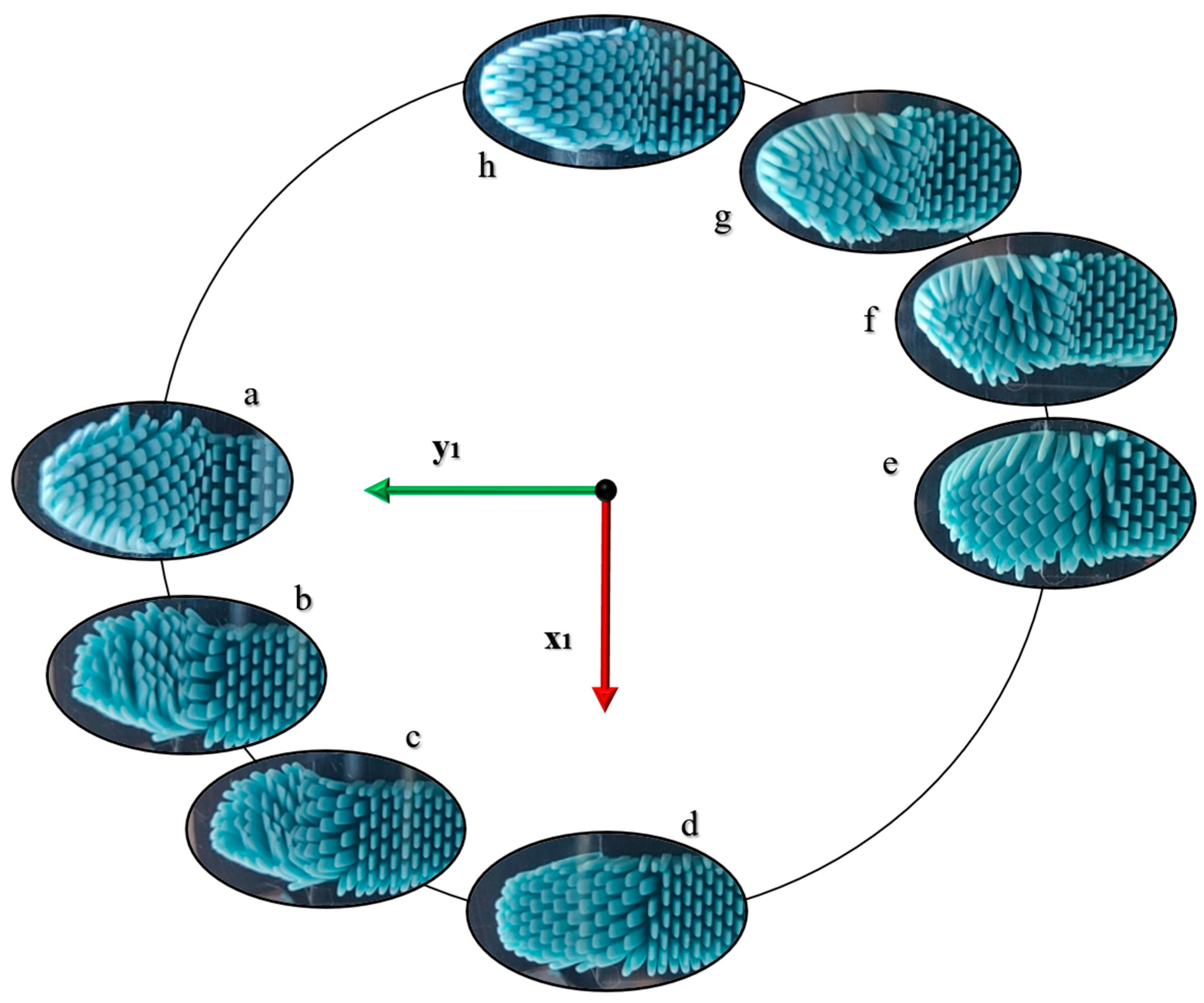
Figure 3. The wrist of the cobot (collaborative robot) is equipped with load cells to assess the forces acting on the Tool Center Position (TCP). The toothbrush is clamped to the robot arm just above the bristles through a 3D-printed flange bolted to the cobot’s wrist. This arrangement allows for the neglect of handling compliance according to the numerical model. The brushing takes place on a flat plexiglass surface, firmly mounted to an aluminum support structure. Furthermore, a Logitech C920 HD Pro webcam is mounted on the aluminum structure, and it was used to monitor the deformation state of the brush bristles during the circular path.
Experimental results are determined with the UR5 algorithm explained below. Considering the system of references adopted in
Figure 4,the circle path is obtained with the
MoveL command, which moves the TCP center of the coordinate system linearly between two control points [
40]. As shown in
Figure 5, two
MoveL commands are imposed in sequence: the first one from
CP1 to
CP2, and the second one from
CP2 to
CP3. In the first command, a fillet radius is set equal to
r, so the TCP trajectory follows the continuous line. As a result, the TCP transits around the
CP2, allowing the arm to not stop at that point. If the fillet radius is not set, the TCP trajectory follows the pointed line.
Four control points, one for each quarter circumference, have been chosen to create a circular motion with the built-in MoveL command, 4. Between these points, the command is imposed with the fillet radius equal to the circle radius. On the other hand, in the first phase of the experiment, the toothbrush’s approach motion to the surface is obtained using the MoveJ command towards the starting point of the circular path. The MoveJ command performs movements calculated in the joint space of the robot arm. This type of motion results in a curved tool path to minimize the time it takes to travel the trajectory.
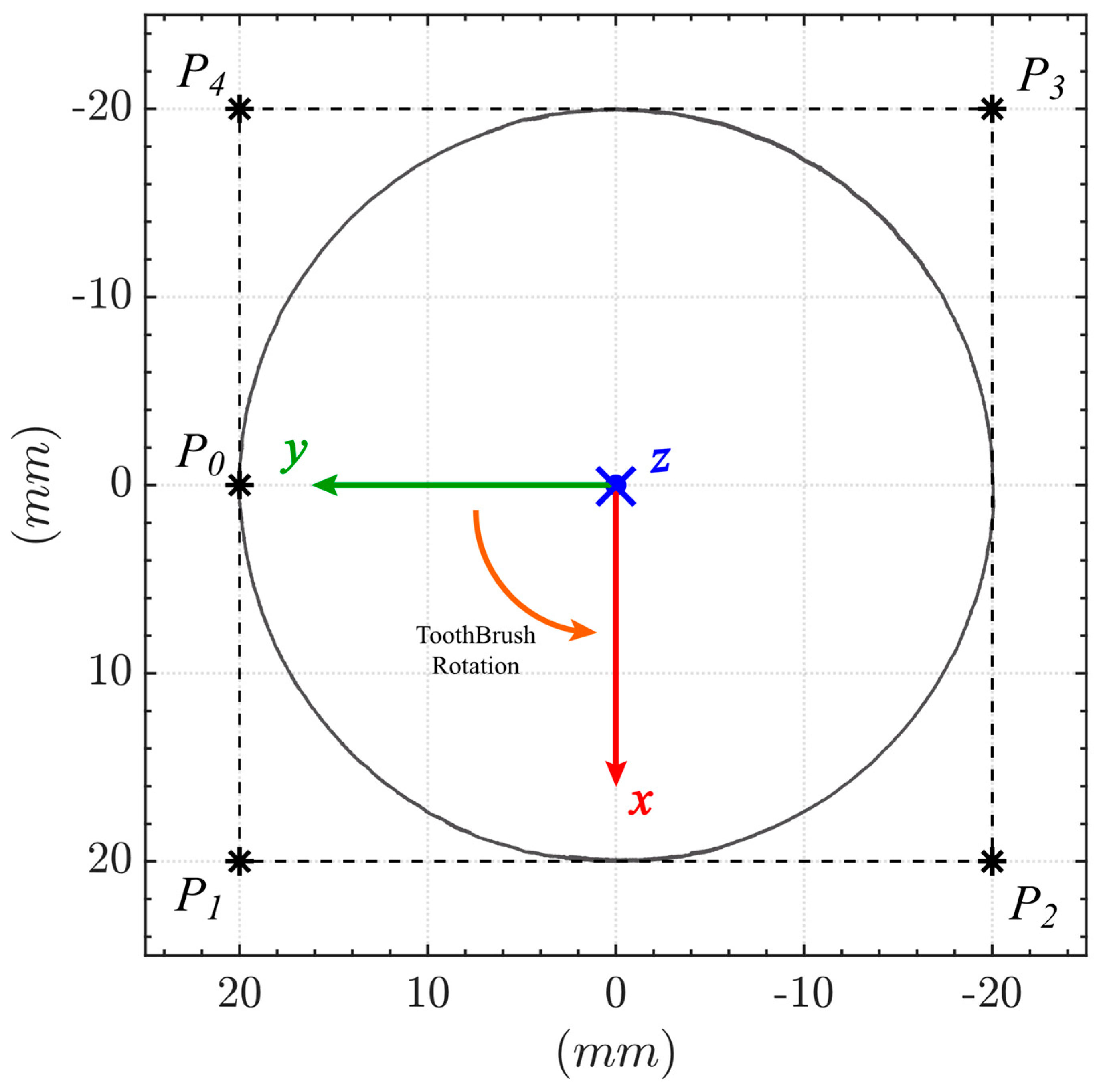
The resulting prescribed motion path on the brushing plane is shown in
Figure 6.
P0 is the starting point of the circle path, while the control points are
P1,
P2,
P3, and
P4 in order. Algorithm 1describes in detail the series of commands imposed on the Robot in order to make its tip describe the circular path. Furthermore, it must be highlighted that the Robot accomplishes four complete rotations and that the first movement imposed (from
P0 to
P1) is not exactly circular and therefore is not taken into account in the postprocess section.
Figure 6.
UR5 circle path and control points.
Figure 6.
UR5 circle path and control points.
| Algorithm 1: Description of the algorithm scripted to impose the robot tip’s circular trajectory. CIRCULAR PATH UR. |
| Input: starting point (P0), blend radius (r), tool acceleration (a), tool speed (v) |
| Control points definition: P1, P2, P3, and P4. | Each point has its 3D coordinate position and the euler angles value. |
while (running = True):
$ 1 “Robot Program”
$ 2 “MoveL”
$ 3 “P0” “breakAfter”
movel(P0, a, v)
$ 4 “Loop”
while (running = True):
$ 5 “MoveL”
$ 6 “P1” “breakAfter”
movel(P1, a, v, r)
$ 7 “P2” “breakAfter”
movel(P2, a, v, r)
$ 8 “P3” “breakAfter”
movel(P3, a, v, r)
$ 9 “P4” “breakAfter”
movel(P4, a, v, r)
end
end |
Functions
MoveL
Syntax: movel(pos, a, v, r)
Parameters:
pos: target position
a: tool acceleration [m/s2]
v: tool speed [m/s]
r: blend radius [m] |
6. Conclusions
This paper proposes a flexible multibody model to efficiently simulate the 3D compliance of a toothbrush. The model is based on the discrete flexible model method adopted in the multibody dynamic approach. The method considers each bristle divided into several rigid bodies connected by stiffness and damping matrices. The contact between the bristles and the target surface is established using the penalty contact method, and the slip-stiction model of friction is considered. Moreover, the contact among the bristles is established using a penalty contact method without a friction component.
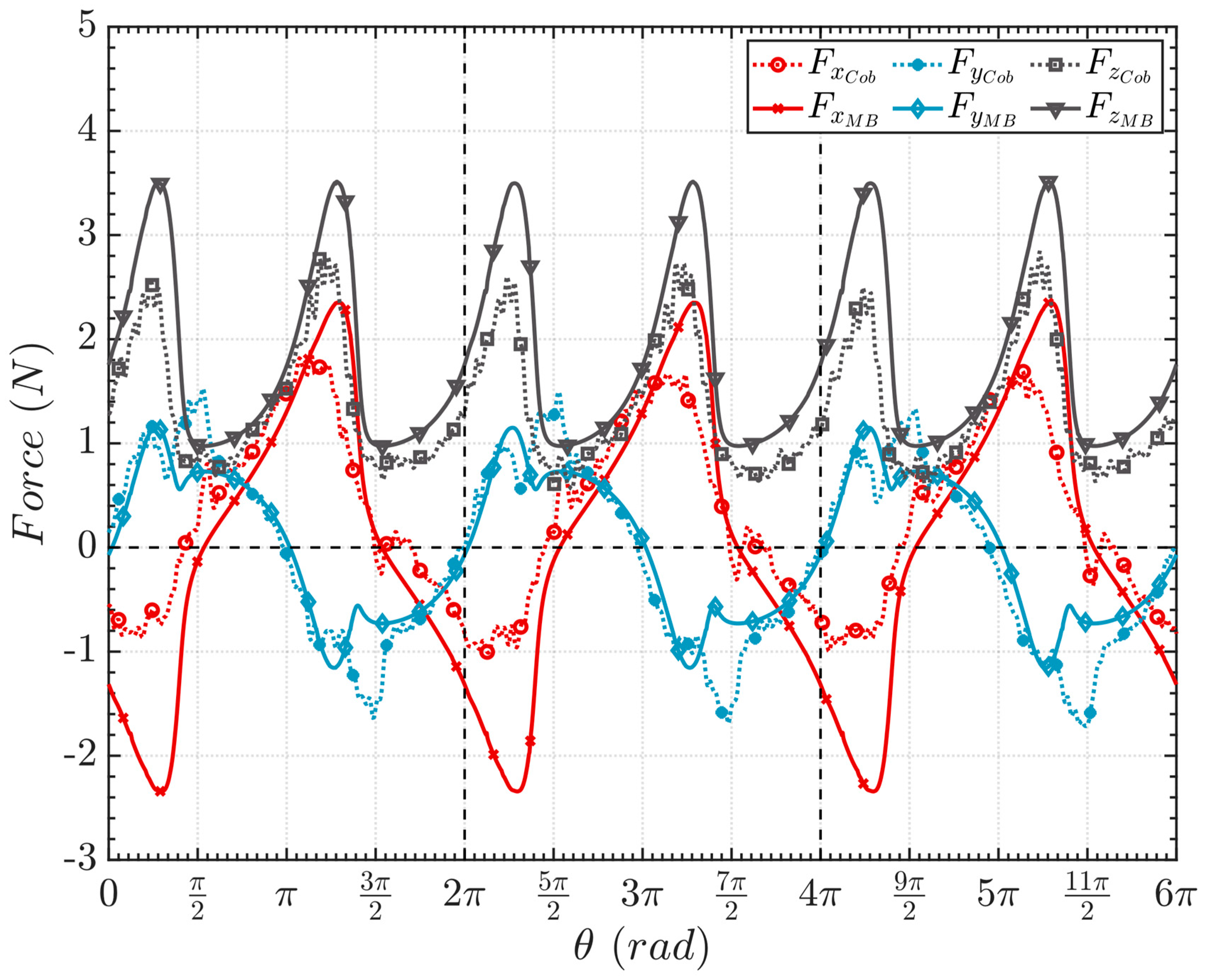
An experimental test bench adopting UR’s collaborative robot and a flat transparent surface are set up. The validation of the MB model is provided by comparing the reaction forces of the toothbrush with the reaction forces acquired by the load cells mounted on the end-effector of the Robot. In addition, the tilting behavior of the experimental toothbrush is detected and considered in the mathematical model. The results demonstrate the model’s accuracy in estimating both normal and tangential forces in several operating conditions.
Moreover, the discrete flexible multibody approach has been proven to be feasible in predicting the displacement of the bristles while the base body of the toothbrush is subjected to a prescribed motion. The positions of the deformed bristles are accurately calculated when the imposed movement is continuous and rectilinear and when the toothbrush is subjected to a rapid change in direction. Therefore, the model could be used properly to evaluate the ability of different toothbrush classes to perform cleaning operations.
Nevertheless, a more in-depth analysis is still necessary to examine the DFM tridimensional bristles’ behavior on a wider range of surfaces, such as bi-curvature or generally specified surfaces, and to assess the model’s performance. Future studies should investigate how sensitive the model is to contact shape and property, for instance, by incorporating additional spheres per bristle or accounting for different contact types. Additionally, considering various materials and geometries, the methodology can be extended to a wider range of toothbrushes. Moreover, the elasticity of the entire handle deserves further investigation.
Finally, the proposed model could offer hints towards holistic modeling and the improvement of toothbrush design to increase their efficiency in performing a complete cleaning without damaging the teeth. An indispensable property to ensure good oral hygiene, thus lowering the chance of developing oral diseases.