Reliability Sensitivity Analysis of Main Shaft Bearings of Wind Turbines Subject to Subsurface Stress
Abstract
1. Introduction
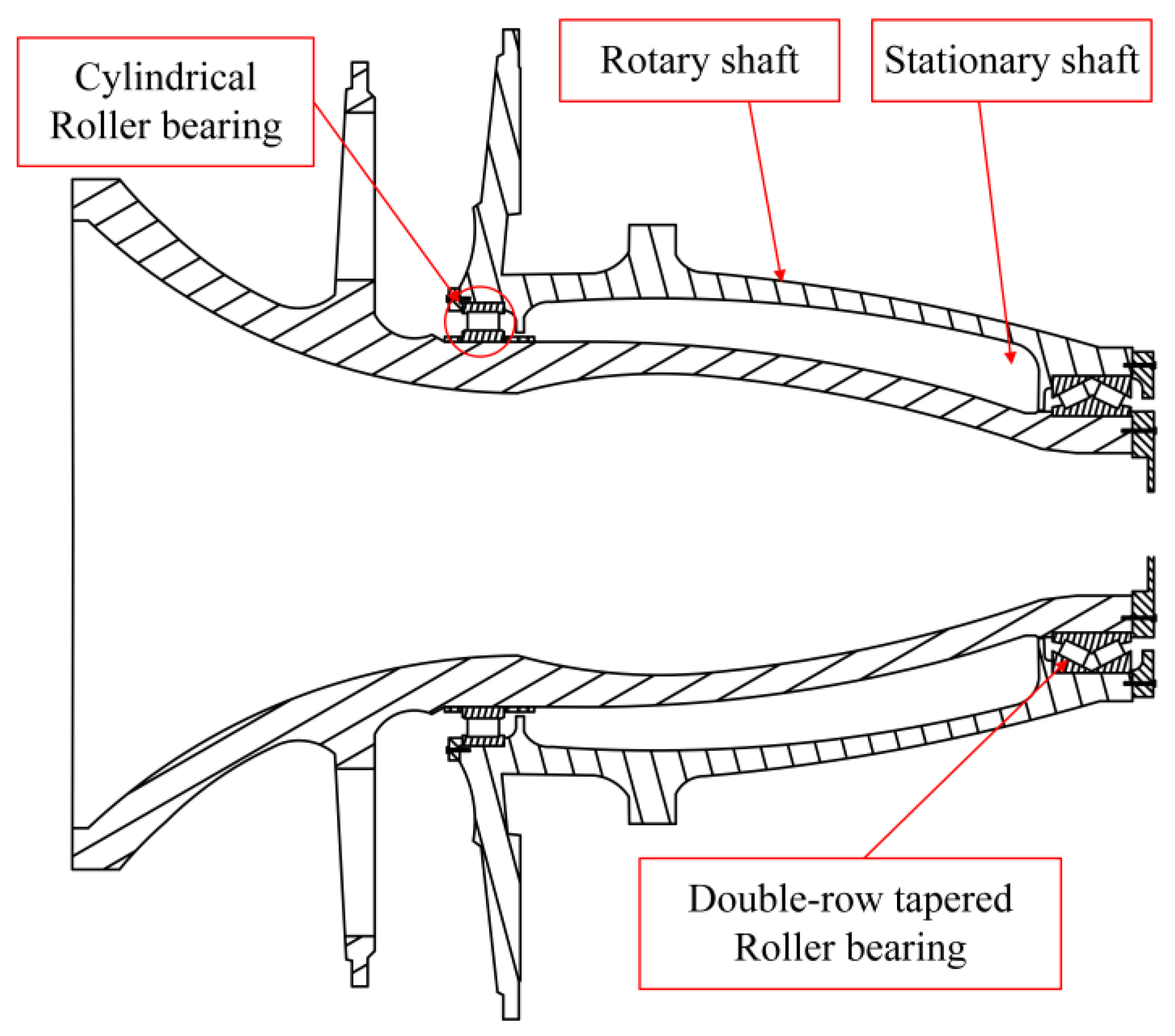
2. Subsurface Stress Analysis of Wind Turbine Main Shaft Bearing
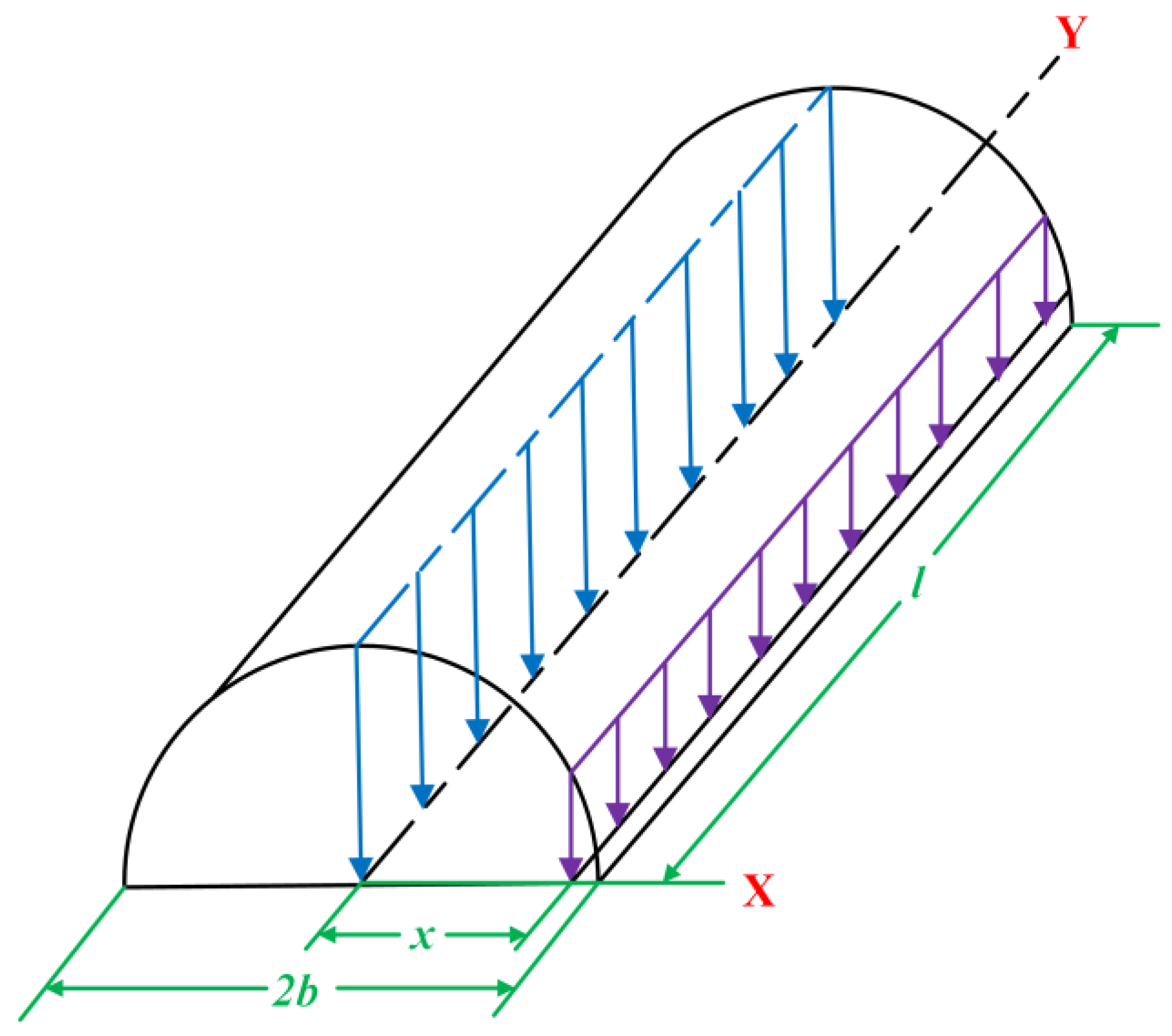
2.1. Stress Analysis of Bearing Subsurface Based on Hertz Contact Theory
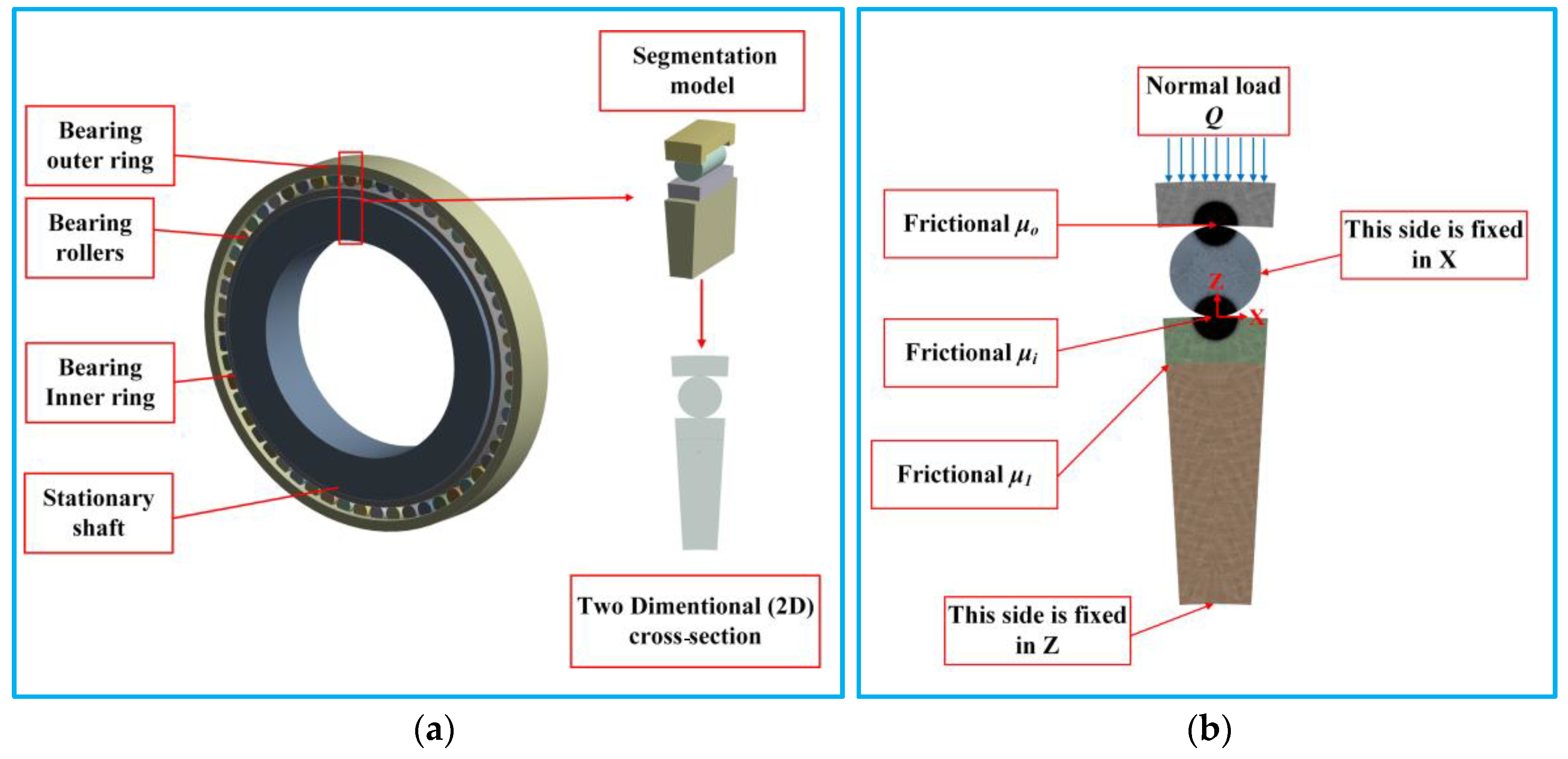
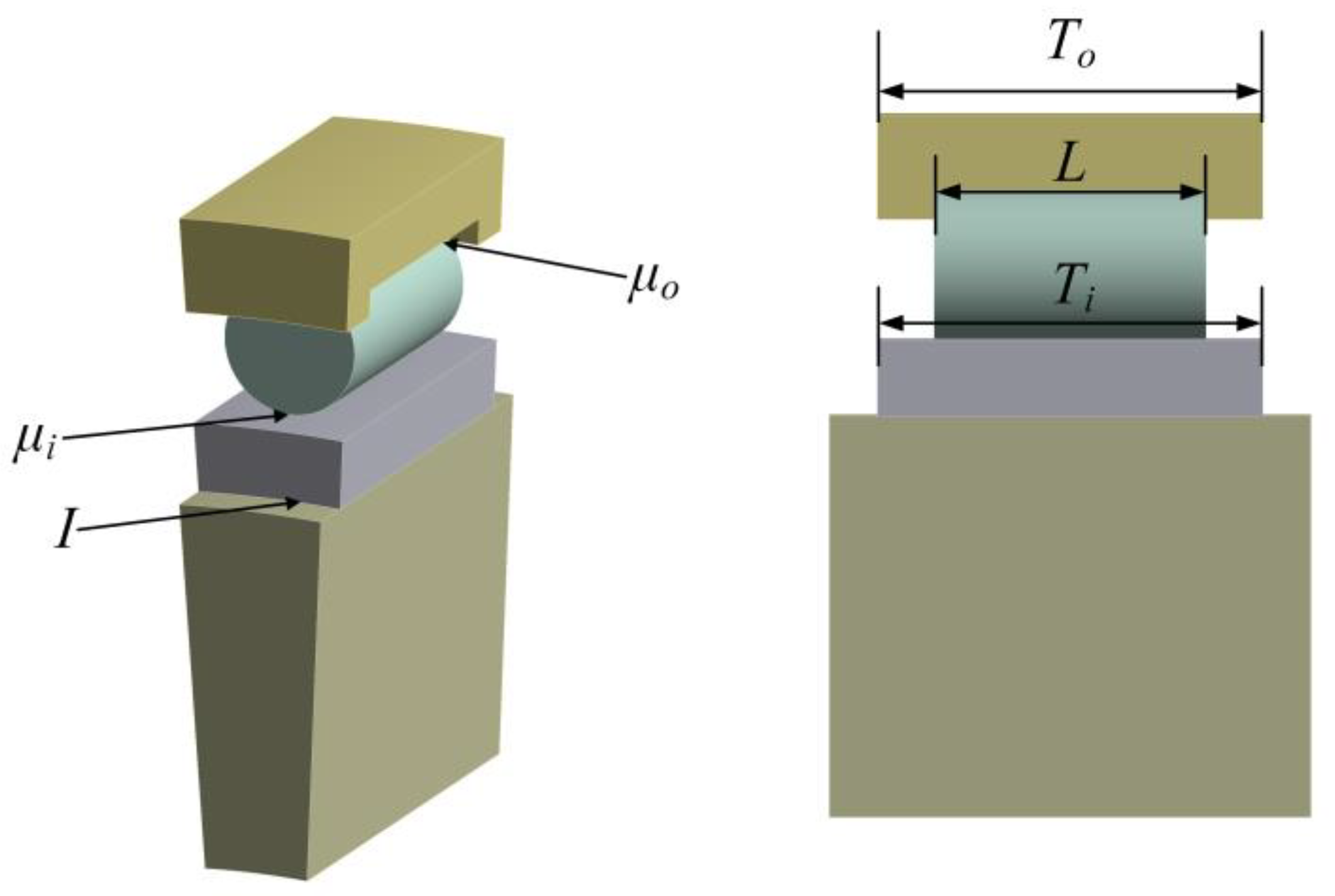
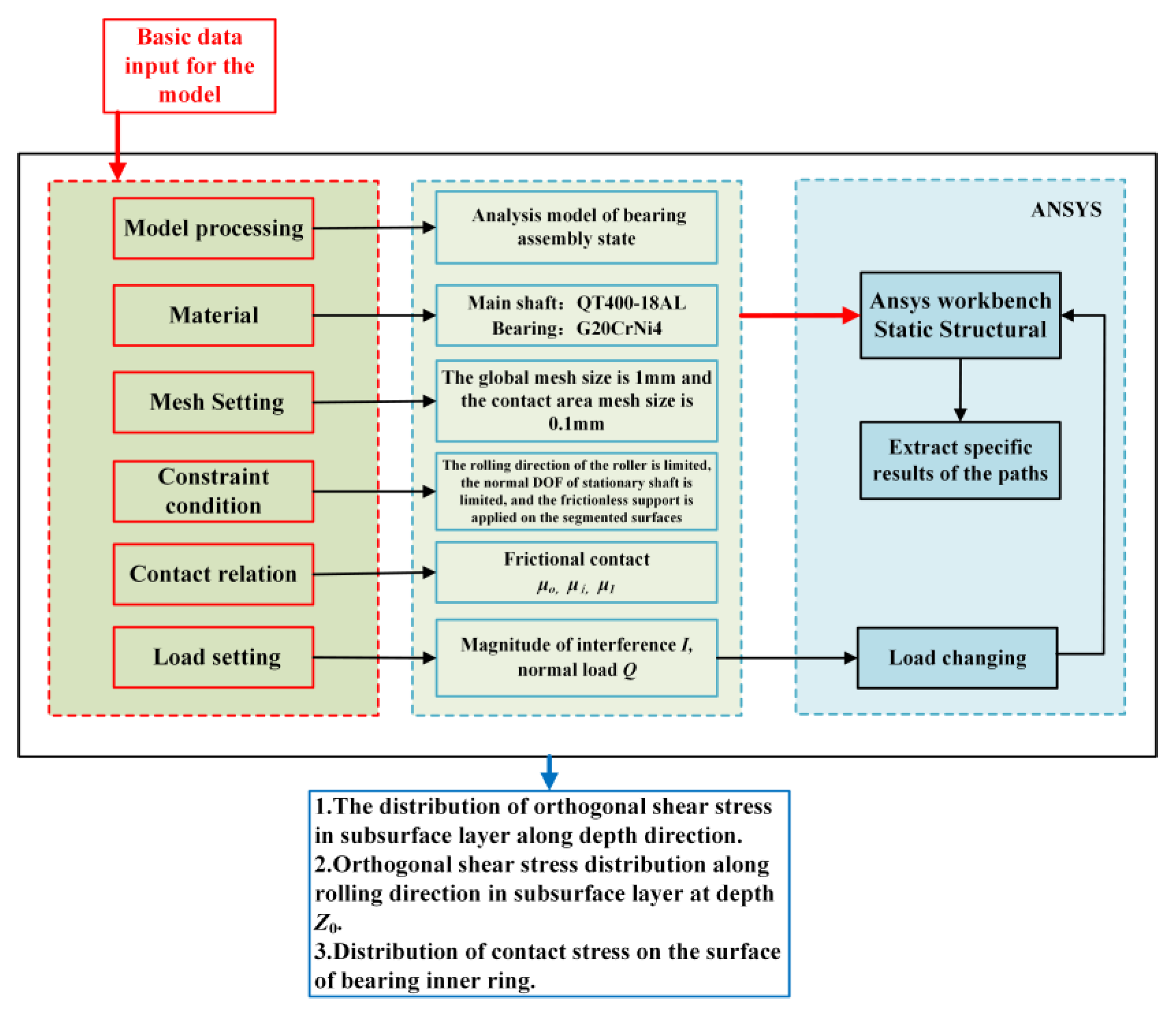
2.2. Stress Analysis of Bearing Subsurface Based on Finite Element Technology
3. Reliability Sensitivity Analysis of Bearing Subsurface Stress
3.1. Determination of Random Variables
3.2. Determination of Limit State Function
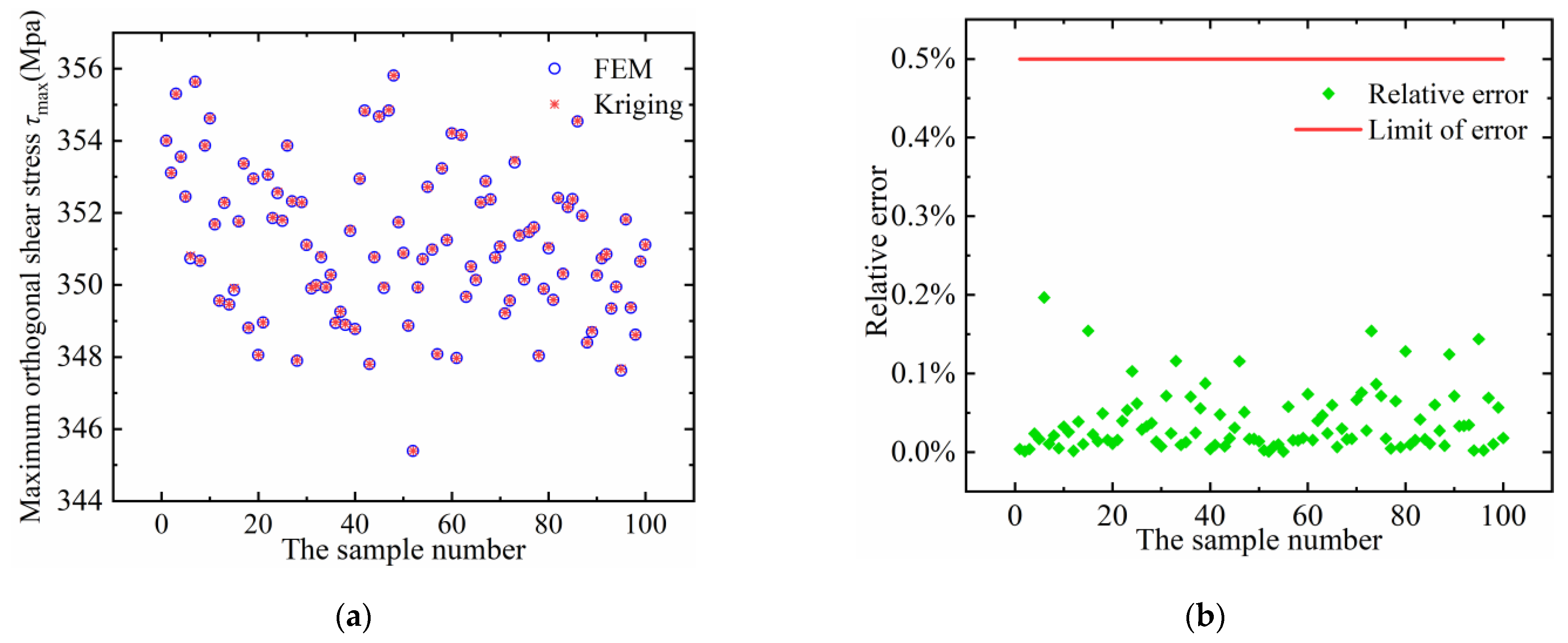
3.3. Kriging Model
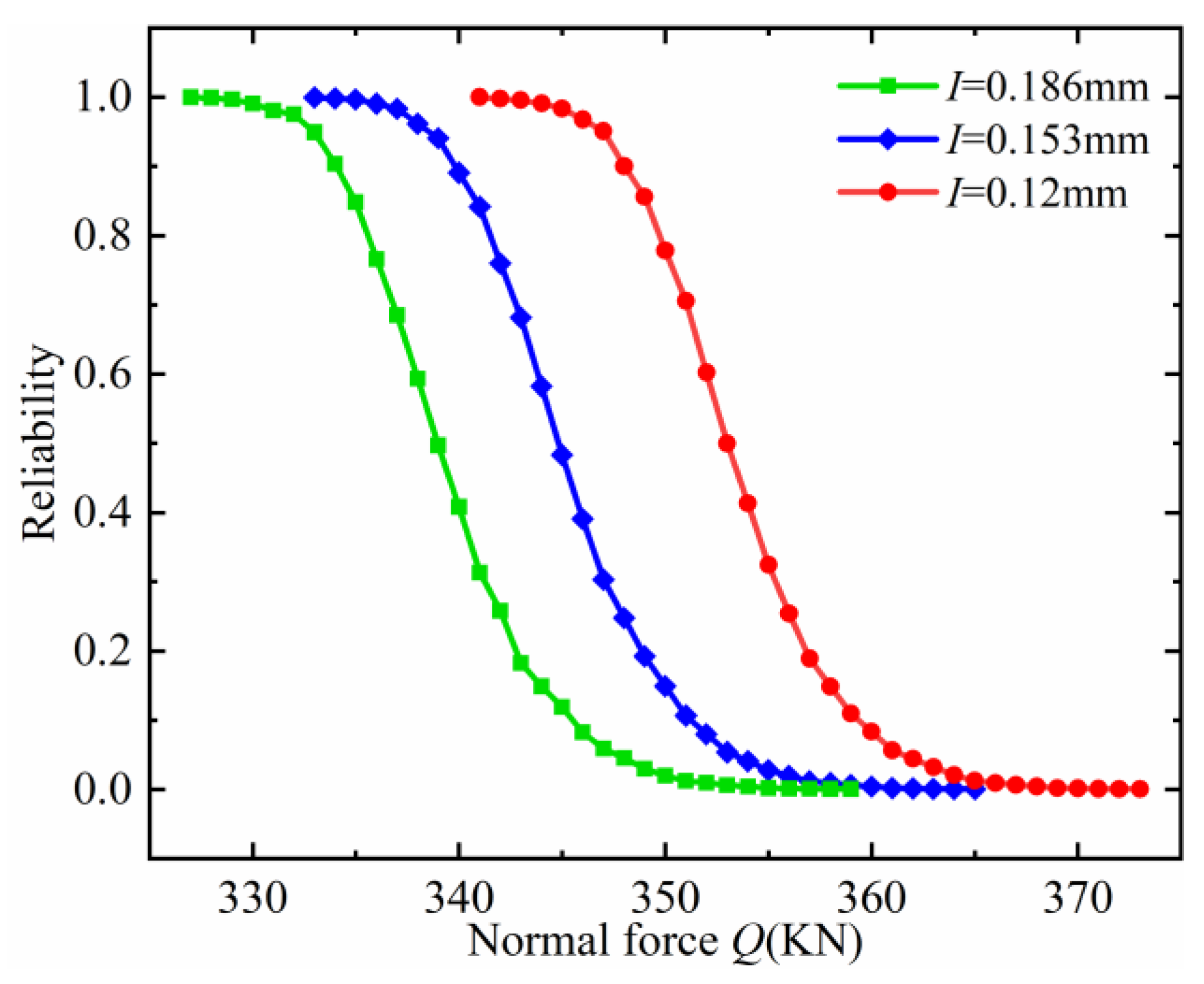
3.4. Reliability Sensitivity Analysis
4. Numerical Example
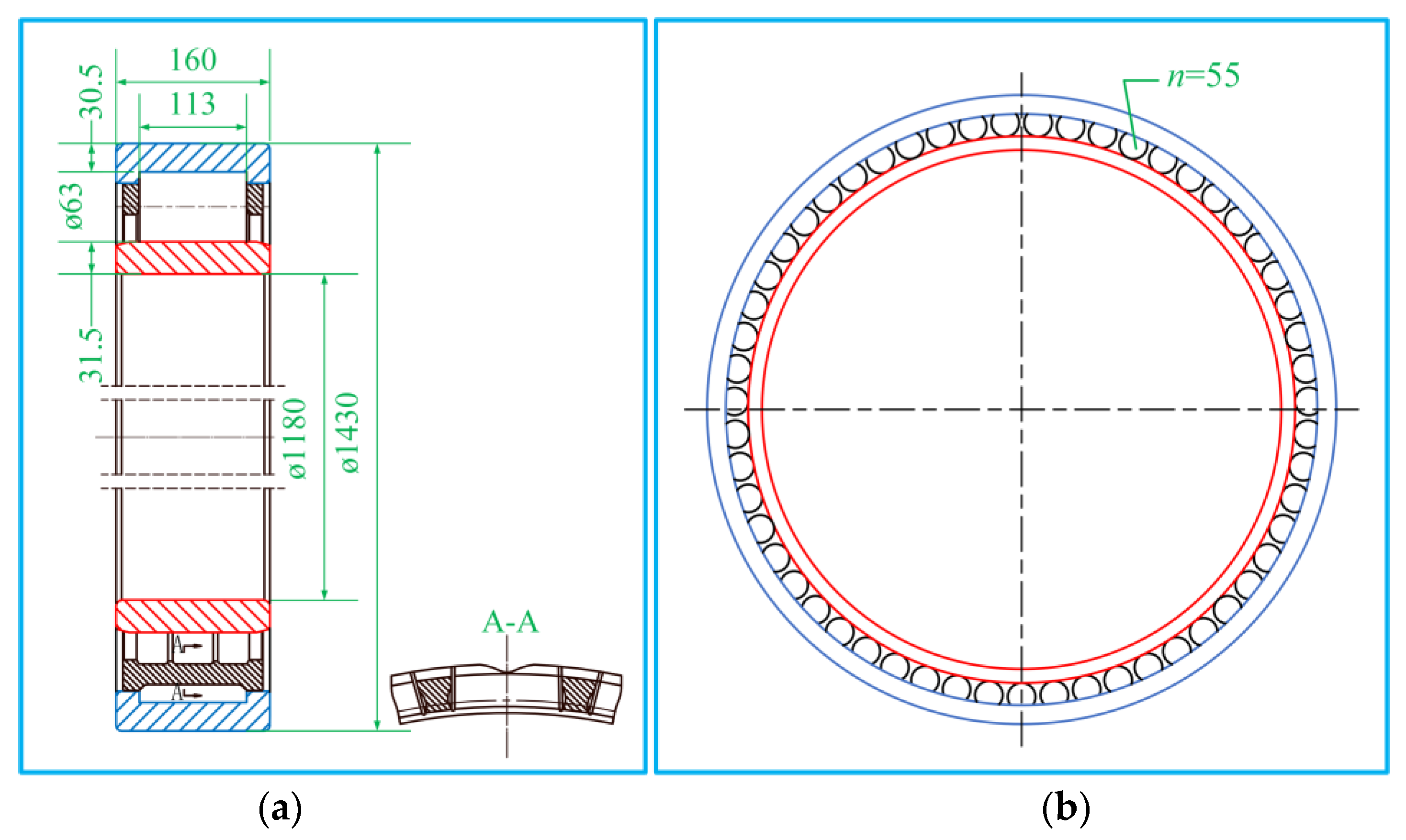
4.1. Material and Method
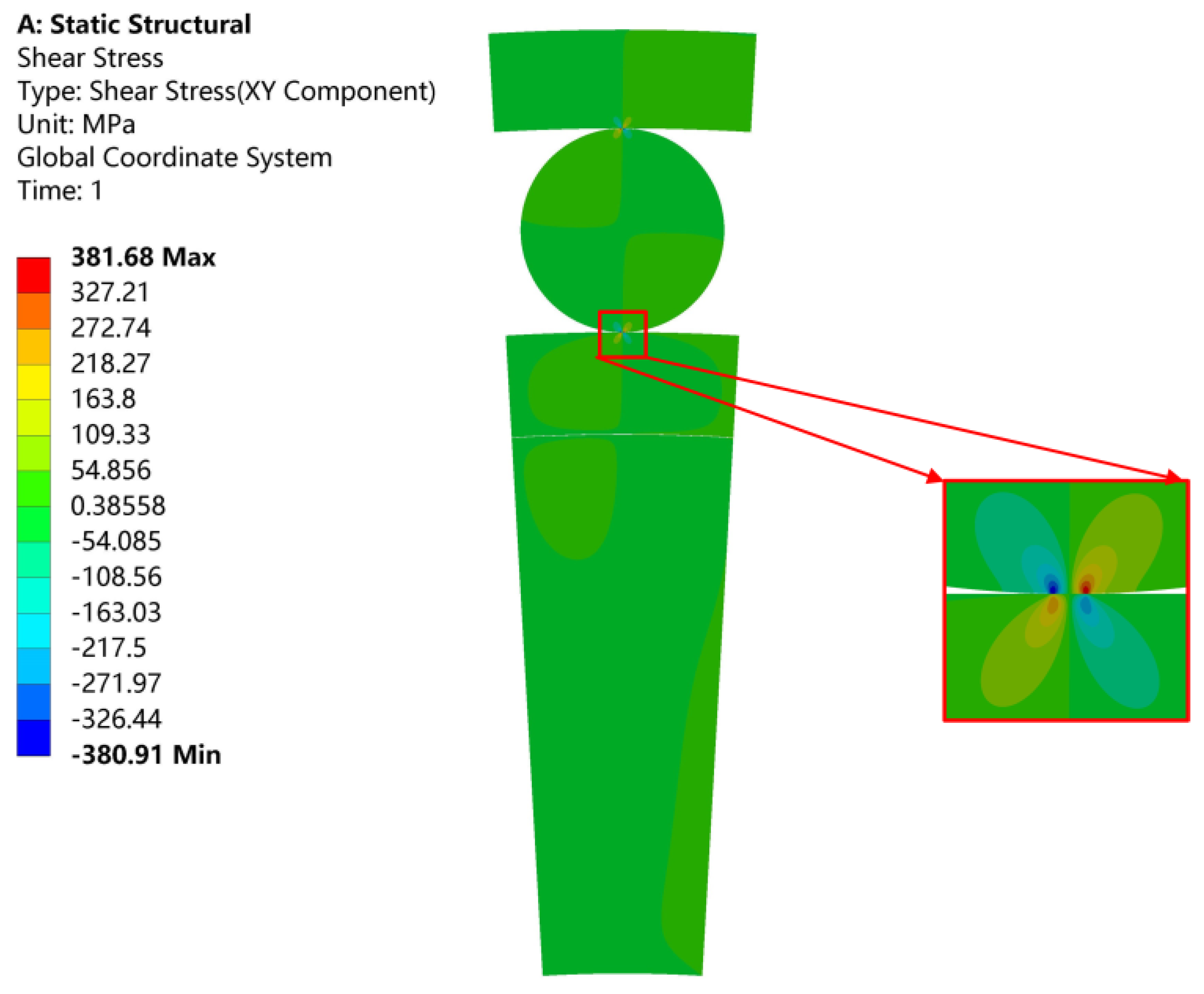
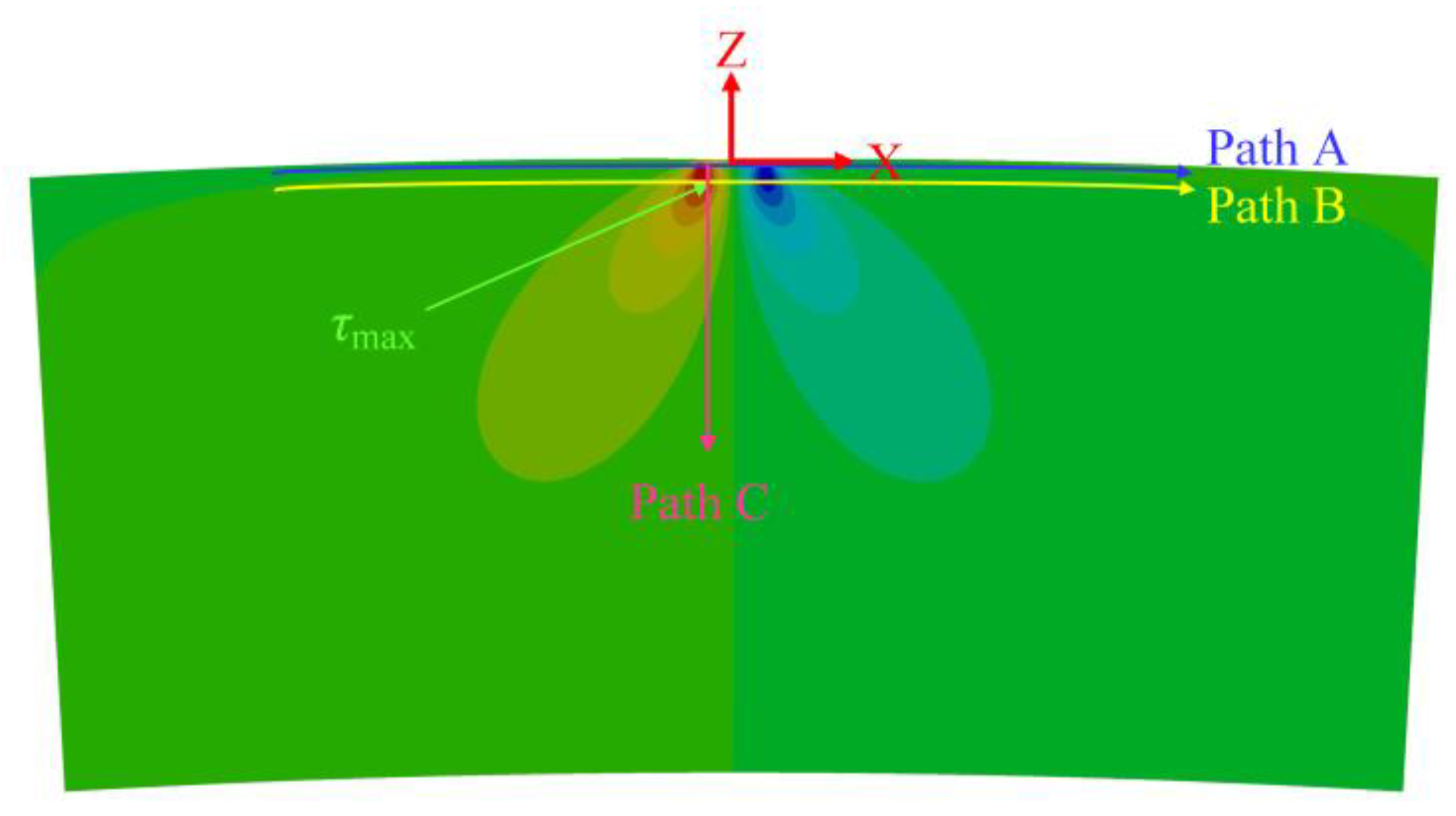
4.2. Subsurface Stress Analysis
4.3. Reliability Sensitivity Investigation
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Mostafaeipour, A. Productivity and development issues of global wind turbine industry. Renew. Sustain. Energy Rev. 2010, 14, 1048–1058. [Google Scholar] [CrossRef]
- Liu, Y.; Tao, T.; Zhao, X.; Zhang, C.; Ma, Y. Support vector regression-based fatigue damage assessment method for wind turbine nacelle chassis. Structures 2021, 33, 759–768. [Google Scholar] [CrossRef]
- de Azevedo, H.D.M.; Araújo, A.M.; Bouchonneau, N. A review of wind turbine bearing condition monitoring: State of the art and challenges. Renew. Sustain. Energy Rev. 2016, 56, 368–379. [Google Scholar] [CrossRef]
- Tavner, P.J.; Xiang, J.; Spinato, F. Reliability analysis for wind turbines. Wind Energy 2007, 10, 1–18. [Google Scholar] [CrossRef]
- Spinato, F.; Tavner, P.J.; van Bussel, G.J.W.; Koutoulakos, E. Reliability of wind turbine subassemblies. IET Renew. Power Gener. 2009, 3, 387–401. [Google Scholar] [CrossRef]
- Ma, C.; Duan, B. Design and application of wind farm fault diagnosis system. In Proceedings of the 2011 Asia-Pacific Power and Energy Engineering Conference, Washington, DC, USA, 25–28 March 2011; IEEE: Manhattan, NY, USA, 2011; pp. 1–4. [Google Scholar]
- Kusiak, A.; Li, W. The prediction and diagnosis of wind turbine faults. Renew Energy 2011, 36, 16–23. [Google Scholar] [CrossRef]
- Sheng, S.; O’Connor, R. Chapter 15—Reliability of Wind Turbines. In Wind Energy Engineering; Letcher, T.M., Ed.; Academic Press: Cambridge, MA, USA, 2017; pp. 299–327. [Google Scholar]
- Otsuka, A.; Sugawara, H.; Shomura, M. A test method for mode ii fatigue crack growth relating to a model for rolling contact fatigue. Fatigue Fract. Eng. Mater. Struct. 1996, 19, 1265–1275. [Google Scholar] [CrossRef]
- Miyashita, Y.; Yoshimura, Y.; Xu, J.Q.; Horikoshi, M.; Mutoh, Y. Subsurface Crack Propagation in Rolling Contact Fatigue of Sintered Alloy. JSME Int. J. Ser. A Solid Mech. Mater. Eng. 2003, 46, 341–347. [Google Scholar] [CrossRef]
- Mason, M.A. A Practical Tool for the Determination of Surface Stresses in Railroad Bearings with Different Contact Geometries and Load Conditions Using Finite Element Analysis; VCU University Archives: Richmond, VA, USA, 2014. [Google Scholar]
- Chudzik, A.; Warda, B. Fatigue life prediction of a radial cylindrical roller bearing subjected to a combined load using FEM. Eksploat. I Niezawodn.-Maint. Reliab. 2020, 22, 212–220. [Google Scholar] [CrossRef]
- Deng, S.; Qin, X.; Huang, S. A study on the effect of subsurface crack propagation on rolling contact fatigue in a bearing ring. J. Mech. Sci. Technol. 2015, 29, 1029–1038. [Google Scholar] [CrossRef]
- Wang, C.; Yu, W.; Ren, C.Z. An accurate method for calculating the contact subsurface stress field of hybrid ceramic ball bearing. Solid State Phenom. 2011, 175, 215–218. [Google Scholar] [CrossRef]
- Ioannides, E.; Harris, T. A New Fatigue Life Model for Rolling Bearings. J. Tribol. ASME 1985, 107, 367–377. [Google Scholar] [CrossRef]
- Xu, J.; Benson, S.; Wetenhall, B. Comparative analysis of fatigue life of a wind turbine yaw bearing with different support foundations. Ocean Eng. 2021, 235, 109293. [Google Scholar] [CrossRef]
- M’Ewen, E.X.L.I. Stresses in elastic cylinders in contact along a generatrix (including the effect of tangential friction). Lond. Edinb. Dublin Philos. Mag. J. Sci. 2010, 40, 454–459. [Google Scholar] [CrossRef]
- Gonzalez-Perez, I.; Iserte, J.L.; Fuentes, A. Implementation of Hertz theory and validation of a finite element model for stress analysis of gear drives with localized bearing contact. Mech. Mach. Theory 2011, 46, 765–783. [Google Scholar] [CrossRef]
- Harris, T.A.; Kotzalas, M.N. Advanced Concepts of Bearing Technology; CRC Press: Boca Raton, FL, USA, 2006. [Google Scholar]
- Palmgren, A. Ball and Roller Bearing Engineering: Philadelphia; SKF Industries Inc.: Gothenburg, Sweden, 1959. [Google Scholar]
- Liu, J.; Wang, L. Dynamic modelling of combination imperfects of a cylindrical roller bearing. Eng. Fail. Anal. 2022, 135, 106102. [Google Scholar] [CrossRef]
- Lundberg, G.; Palmgren, A. Dynamic Capacity of Rolling Bearings. J. Appl. Mech. Jun 1949, 16, 165–172. [Google Scholar] [CrossRef]
- Jones, A.B. Analysis of Stresses and Deflections Vol.1. New Depart. Eng. Data 1946, 1–180. [Google Scholar]
- Williams, J.A.; Dwyer-Joyce, R.S. Contact between solid surfaces. In Modern Tribology Handbook; Springer: New York, NY, USA, 2001; pp. 121–162. [Google Scholar]
- Hills, D.A. Mechanics of fretting fatigue. Wear 1994, 175, 107–113. [Google Scholar] [CrossRef]
- Li, N.; Zhang, D.; Liu, H.; Li, T. Optimal design and strength reliability analysis of pressure shell with grid sandwich structure. Ocean Eng. 2021, 223, 108657. [Google Scholar] [CrossRef]
- International Standard ISO 281; Rolling Bearings—Dynamic Load Ratings and Rating Life, 2nd ed. ISO: Geneva, Switzerland, 2007.
- Khuri, A.I.; Mukhopadhyay, S. Response surface methodology. WIREs Comput. Stat. 2010, 2, 128–149. [Google Scholar] [CrossRef]
- Jeong, S.; Murayama, M.; Yamamoto, K. Efficient Optimization Design Method Using Kriging Model. J. Aircr. 2005, 42, 413–420. [Google Scholar] [CrossRef]
- Kaymaz, I. Application of kriging method to structural reliability problems. Struct. Saf. 2005, 27, 133–151. [Google Scholar] [CrossRef]
- Zhang, L.; Lu, Z.; Wang, P. Efficient structural reliability analysis method based on advanced Kriging model. Appl. Math. Model. 2015, 39, 781–793. [Google Scholar] [CrossRef]
- Kleijnen, J.P.C. Kriging metamodeling in simulation: A review. Eur. J. Oper. Res. 2009, 192, 707–716. [Google Scholar] [CrossRef]
- Liu, Z.; Lu, Z.; Ling, C.; Feng, K.; Hu, Y. An improved AK-MCS for reliability analysis by an efficient and simple reduction strategy of candidate sample pool. Structures 2022, 35, 373–387. [Google Scholar] [CrossRef]
- Huang, X.; Li, Y.; Zhang, Y.; Zhang, X. A new direct second-order reliability analysis method. Appl. Math. Model. 2018, 55, 68–80. [Google Scholar] [CrossRef]
- Al-Tameemi, H.A.; Long, H. Finite element simulation of subsurface initiated damage from non-metallic inclusions in wind turbine gearbox bearings. Int. J. Fatigue 2020, 131, 105347. [Google Scholar] [CrossRef]
- Wu, Y.T.; Mohanty, S. Variable screening and ranking using sampling-based sensitivity measures. Reliab. Eng. Syst. Saf. 2006, 91, 634–647. [Google Scholar] [CrossRef]
- Xinning, Z.; Yingdong, Q.; Hongwang, Y.; Rongde, L. Low temperature impact toughness and fracture mechanism of cast QT400-18L ductile iron with different Ni additions. China Foundry 2013, 10, 310–314. [Google Scholar]








| Parameter | Materials | |
|---|---|---|
| QT400-18AL [37] | G20CrNi4 | |
| Density ρ (kg/m3) | 7100 | 7800 |
| Elastic modulus E (Mpa) | 1.69 × 105 | 2.07 × 105 |
| Poisson’s ratio ν | 0.275 | 0.3 |
| Tensile strength R (Mpa) | 360 | 1176 |
| Yield strength σs (Mpa) | 220 | 835 |
| Variable | CPU Time | Average Relative Error (a) | Average Relative Error (b) |
|---|---|---|---|
| Analytical | 0.023 s | - | - |
| FEM (0.1 mm) | 13,113.62 s | 0.9984% | 1.9876% |
| FEM (0.2 mm) | 6519.312 s | 1.5306% | 1.9876% |
| FEM (0.5 mm) | 2852.984 s | 3.8714% | 8.2805% |
| FEM (1.0 mm) | 1414.453 s | 4.1536% | 8.5563% |
| Variable | Distribution | Mean | Standard Deviation |
|---|---|---|---|
| To (mm) | normal | 160 | 0.8 |
| L (mm) | normal | 113 | 0.565 |
| Ti (mm) | normal | 160 | 0.8 |
| Em (Pa) | normal | 2.07 × 1011 | 1.015 × 109 |
| ρ (kg/m3) | normal | 7800 | 39 |
| I (mm) | normal | 0.153 | 0.00765 |
| μo | normal | 0.1 | 0.005 |
| μi | normal | 0.1 | 0.005 |
| Variable | CPU Time | Average Relative Error |
|---|---|---|
| FEM | 13,113,620.16 s | - |
| Kriging | 16.823 s | 3.80 × 10−5 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhao, W.; Jiang, Z.; Zhang, P.; Huang, X. Reliability Sensitivity Analysis of Main Shaft Bearings of Wind Turbines Subject to Subsurface Stress. Machines 2023, 11, 681. https://doi.org/10.3390/machines11070681
Zhao W, Jiang Z, Zhang P, Huang X. Reliability Sensitivity Analysis of Main Shaft Bearings of Wind Turbines Subject to Subsurface Stress. Machines. 2023; 11(7):681. https://doi.org/10.3390/machines11070681
Chicago/Turabian StyleZhao, Wei, Zhiyuan Jiang, Peng Zhang, and Xianzhen Huang. 2023. "Reliability Sensitivity Analysis of Main Shaft Bearings of Wind Turbines Subject to Subsurface Stress" Machines 11, no. 7: 681. https://doi.org/10.3390/machines11070681
APA StyleZhao, W., Jiang, Z., Zhang, P., & Huang, X. (2023). Reliability Sensitivity Analysis of Main Shaft Bearings of Wind Turbines Subject to Subsurface Stress. Machines, 11(7), 681. https://doi.org/10.3390/machines11070681





