Computational Fluid Dynamics Simulation and Optimization of Hydropneumatic Spring Damper Valves for Heavy Vehicle Applications

Abstract

To satisfy the design requirements for a hydropneumatic spring damper valve, the inlet–outlet pressure drop (ΔP) and the axial force on the spool (F_Z) of a valve were investigated using fluid–solid coupling simulations and multi-objective optimization, along with the effects of the diameters of three internal holes (D_A, D_B, and D_C) in the valve on the ΔP and the F_Z. First, a meshed computational fluid dynamics model of a damper valve was established based on its geometric structure. Next, the effects of the flow rate (Q) and the diameter of the damping hole in the internal structure on the ΔP and the F_Z of the damper valve were investigated. The results showed that the ΔP and the F_Z varied nonlinearly with Q. For a given Q, the ΔP decreased as D_A, D_B, and D_C increased. For a given Q, the F_Z was not related to D_A and D_C, but it decreased as D_B increased. Finally, the structure of the damper valve was optimized by defining the ΔP and the F_Z as the response variables and D_A, D_B, and D_C as the explanatory variables. The results showed that the best configuration of the hole diameters was D_A = 8.8 mm, D_B = 5.55 mm, and D_C = 6 mm. In this configuration, ΔP = 0.704 MPa and F_Z = 110.005 N. The ΔP of the optimized valve was closer to the middle value of the target range than that of the initial valve design. The difference between the simulated and target values of the F_Z decreased from 0.28% to 0.0045%, satisfying application requirements.

1. Introduction

Hydropneumatic spring suspensions are increasingly being used in off-road vehicles, farm trucks, and racing cars. A suspension system is critical to ensuring the normal operation, maneuverability, and mobility of these vehicles. The performance of the hydropneumatic springs directly affects the ride quality of a vehicle. To ensure the performance of a hydropneumatic spring, the damping characteristics of the damper valve must be considered during its design. Xie et al. [1] highlighted the fact that simple-structure, damping-adjustable damper valves have always been a research topic of interest. A damper valve is a core component of a hydropneumatic spring suspension system. Thus, the improvement of the design of a hydropneumatic spring suspension system necessitates a close examination of this core component.

Giliomee and Els [2] analyzed the rigidity and damping characteristics and the random input responses of a hydropneumatic suspension with a double-gas-chamber spring unit for heavy off-road vehicles and the effects of the damping and rigidity on the overall performance of the hydropneumatic suspension, thereby improving the ride quality and maneuverability of heavy off-road vehicles. Regarding the kinetic characteristics of a semi-active hydropneumatic suspension, Saxon et al. [3] investigated the Bradley Fighting Vehicle of the US Army, established a test system for the hydropneumatic suspension and associated piping, computer controller, and dynamic track tensioner, and proposed a method for the continuous adjustment of the damping of caterpillar vehicles. Theron and Els [4] established a damping model for a semi-active hydropneumatic spring suspension with a two-stage damping system by employing linear fluid mechanics theory, calculating the pressure in the accumulators through the integral calculations of the flow rates to the hydraulic cylinder, and using the damping force of the hydropneumatic suspension unit as the output of the model. The predictions yielded using the model were consistent with the experimental data. Zhuang [5] modeled the nonlinear vertical and lateral dynamics of an active hydropneumatic suspension and analyzed the stability of the model with road surface excitations of different frequencies and magnitudes. The accumulator in the model and the mathematical model of the damper valve were linearized, and the control accuracy of the active hydropneumatic suspension was improved by employing robust control theory and a hierarchical control method. Li et al. [6] established a nonlinear mathematical model of a hydropneumatic suspension based on simulations and tests of hydropneumatic springs. The ride quality of the vehicle was improved with linearization and by employing proportional–integral–derivative control. Zhao et al. [7] established a model of the nonlinear rigidity and damping of a semi-active hydropneumatic suspension and linearized the model using a feedback linearization method. Based on an analysis of the effects of the major parameters on the damping of a damper valve, they proposed a sliding mode control system for semi-active hydropneumatic suspensions. In addition, cavitation is also the focus of many scholars in the research and design of vehicle shock absorbers. For example, Skrickij et al. [8] studied the cavitation effect of single-tube shock absorbers by establishing mathematical models, and proposing a simplified method for evaluating the cavitation process of automotive single-tube shock absorbers. Karpenko et al. [9] explored the adaptive performance of passive control systems based on frequency analysis. However, cavitation and amplitude–frequency characteristics are not studied in this paper. Significant research on the characteristics of damper valves has been conducted using various methods, enriching the theoretical understanding of damper valves. However, most of the existing studies focus on the modeling of the flow in damper valves, and few studies have been conducted on the inlet–outlet pressure drop (ΔP) and axial force on the spool (F_Z) that drives the opening of damper valves.

Computational fluid dynamics (CFD) is a useful tool for the in-depth analysis of parameters that are difficult to obtain experimentally, such as flow forces and damping. Numerous studies [10,11,12,13,14] have shown that the flow coefficients of various valves obtained using CFD agree well with experimental data. Morita et al. [15] investigated the unsteady flow in a valve under a partial opening condition and reproduced the unsteady flow phenomenon using CFD. Lisowski et al. [16] proposed a method for calculating the forces associated with the flow in a solenoid-operated directional control valve (pressure and viscous forces). The method was first used to define the surfaces affected by the forces associated with the flow through each path and then to obtain the values of the flow forces using a CFD method. In 2015, Lisowski et al. [17] investigated the pressure compensation problem in a multi-section proportional directional control valve. They obtained the values of the flow resistance through the valve and the axial component of the flow force acting on the valve spool through numerous CFD simulations, established a mathematical model of the flow forces, and examined the possibility of pressure compensation in a three-section control valve based on simulations in the Matlab/Simulink system. Wu et al. [13] described specific CFD simulation methods for fitting the flow–pressure curve of a pressure control valve used in an automotive fuel supply system. Their analysis improved understanding of the coupling mechanism in pressure control valves. Lai et al. [18] investigated the transient characteristics of a dual-disc check valve during the opening period and numerically simulated the transient characteristics and internal flow field of a check valve using an unsteady CFD method. Recent studies [19,20,21,22] have analyzed the dynamic characteristics of various valves using CFD, and the reliability of these CFD results has been widely recognized. In addition, CFD can analyze parameters that are difficult to measure experimentally. However, different relaxation factors are selected for different CFD models, and CFD calculations must be verified against experimental results.

In this study, we analyzed a damper valve that was developed using CFD and a fluid–solid coupling method based on the previous studies reviewed above. This paper first describes the workflow of the hydropneumatic spring damper valve. A CFD model of the initial design of the damper valve was established for finite element verification and analysis, and the verified simulation parameters were used in subsequent multi-parameter model-based simulations and analyses. Finally, to satisfy the engineering application requirements of the damper valve, multi-objective optimization of the damper valve was performed by defining the diameters of three internal holes as the objective of optimization and ΔP and F_Z as the response variables.

2. Establishment, Verification, and Analysis of the Damper Valve Model

2.1. Computer-Aided Design (CAD) Model of the Damper Valve

Figure 1a shows the structure of the hydropneumatic spring damper valve. The valve body was fabricated from #45 steel. Kunlun 10 hydraulic oil was used as the working fluid. The spring had an initial back pressure of 110 N and a toughness of 10 N/mm. The flow of the hydraulic oil is indicated by line L1 in the Figure. To improve the quality of the mesh for subsequent calculations, structural components whose effects were negligible [16,17], such as the chamfers, gasket, and spring, were simplified. Figure 1b shows the simplified CAD model. In the paper, D_X is the diameter of hole X. D_A, D_B, and D_C have lengths of 10 mm, 6 mm, and 6 mm, respectively. The other structural dimensions are given in

Table 1. Structural dimensions of the damper valve.

Parameter	Value (mm)	Parameter	Value (mm)
D1	35	l3	13
D2	33.5	l4	6.5
Din	12	l5	36
Dout	25	l6	16
l1	56	l7	5
l2	14.5	l8	11

.

The workflow of the damper valve is as follows. The hydraulic oil flowed in the direction indicated by line L1. When the flow rate reached a certain value, the axial force acting on the spool was larger than the back pressure. Because the initial back pressure of the spring was inadequate to sustain the spool at its initial position, the spool moved toward the outlet. This process is referred to as the valve opening process. When the valve opened, the direction of the flow of the hydraulic oil changed. This study was focused on the effects of the flow rate (Q) and D_X (D_A, D_B, and D_C) on ΔP and F_Z of the damper valve during the closure stage of the damper valve and the multi-objective optimization of the diameters of the critical holes at the critical flow rate of 70 L/min (representing the transition between the opening and closing of the valve). Therefore, the operation of the valve during the opening stage is not covered here.

2.2. Meshing of Damper Valve Model

Fluid–solid coupling simulations of the damper valve were performed using the Ansys Workbench platform. ΔP in the flow domain was calculated using the Ansys fluent software. The calculation results were imported into the static structural module of the Ansys Workbench platform to calculate F_Z.

A mesh independence study of the mesh model of the flow domain established based on the CAD model was performed before it was used for numerical simulations. The study was performed at Q = 22 L/min (the minimum flow rate used in the experiment) and 500 steady iterative step lengths. The residual error was less than 10⁻⁴. Figure 2 shows the results. The calculation results stabilized after the number of cells reached 260,000.

The wall function, which is usually empirically derived, describes the relationship between a near-wall cell and a wall. In this study, the dimensionless distance between the wall and the first layer of the mesh y⁺ was configured according to the wall function and the height of the first layer of the mesh. The value of y⁺ was verified to verify the quality of the mesh. Based on the structural dimensions of the damper valve given above, most of the values of y⁺ fell in the range of 10–60, which indicated that the standard wall function was acceptable. Based on the structural dimensions of the CAD model and the results of the mesh independence study, and considering the computational complexity and accuracy, the same mesh was used for subsequent simulation calculations. Tetrahedral cells were used that measured 1 mm across. Five boundary layers were defined, and the growth rate was set to 1.2. Figure 1c shows the mesh for the flow domain The solid domain was also meshed using 1 mm tetrahedral cells, as shown in Figure 1d.

2.3. Validation of the Simulation Model

2.3.1. Experimental and Simulation Setups

To verify the reliability of the numerical model, the ΔP values of the initial design of the damper valve at five different steady flow rates (Q = 22 L/min, 41.7 L/min, 51 L/min, 60 L/min, and 70 L/min) were obtained experimentally using a finite element model. Figure 3 shows the experimental setup. Pressure sensors were mounted at the inlet and outlet to measure the inlet and outlet pressures. An electromagnetic flow meter was mounted at the inlet piping to measure the flow rate through the damper valve.

In the CFD simulation, a second-order turbulence model was used to fit the Navier–Stokes equation [23]. The ambient temperature was set to 40 °C. The temperature inside the damper valve did not vary significantly and could be assumed to be constant [13,18,24]. Thus, the energy equation and cavitation were not considered. The governing equations involved are shown in Appendix A. Based on parameter testing, the turbulence was simulated using the k-ε model and the standard wall function. The boundary conditions were the velocity inlet and the pressure outlet. The inlet velocity was calculated from the flow velocity and inlet area using the SIMPLEC algorithm.

2.3.2. Verification and Analysis of Simulation Results

Figure 4a shows a comparison of the simulation and experimental results. ΔP increased quasi-quadratically as the flow rate increased. The simulation and experimental results exhibited similar trends. The maximum, minimum, and mean errors were 8.10%, 0.28%, and 4.10%, respectively. The error might have been due to the simplification of the valve structural components such as the chamfers and spring. Figure 4b shows the nephogram of the pressure distribution in the plane of symmetry of the flow field at Q = 22 L/min. The simulated stress distribution was consistent with the theoretical stress distribution. The simulation error was acceptable, confirming the reliability of the CFD model. Therefore, the same parameters were used in subsequent simulations.

3. Parameter Configuration and Multi-Objective Optimization of Damper Valve

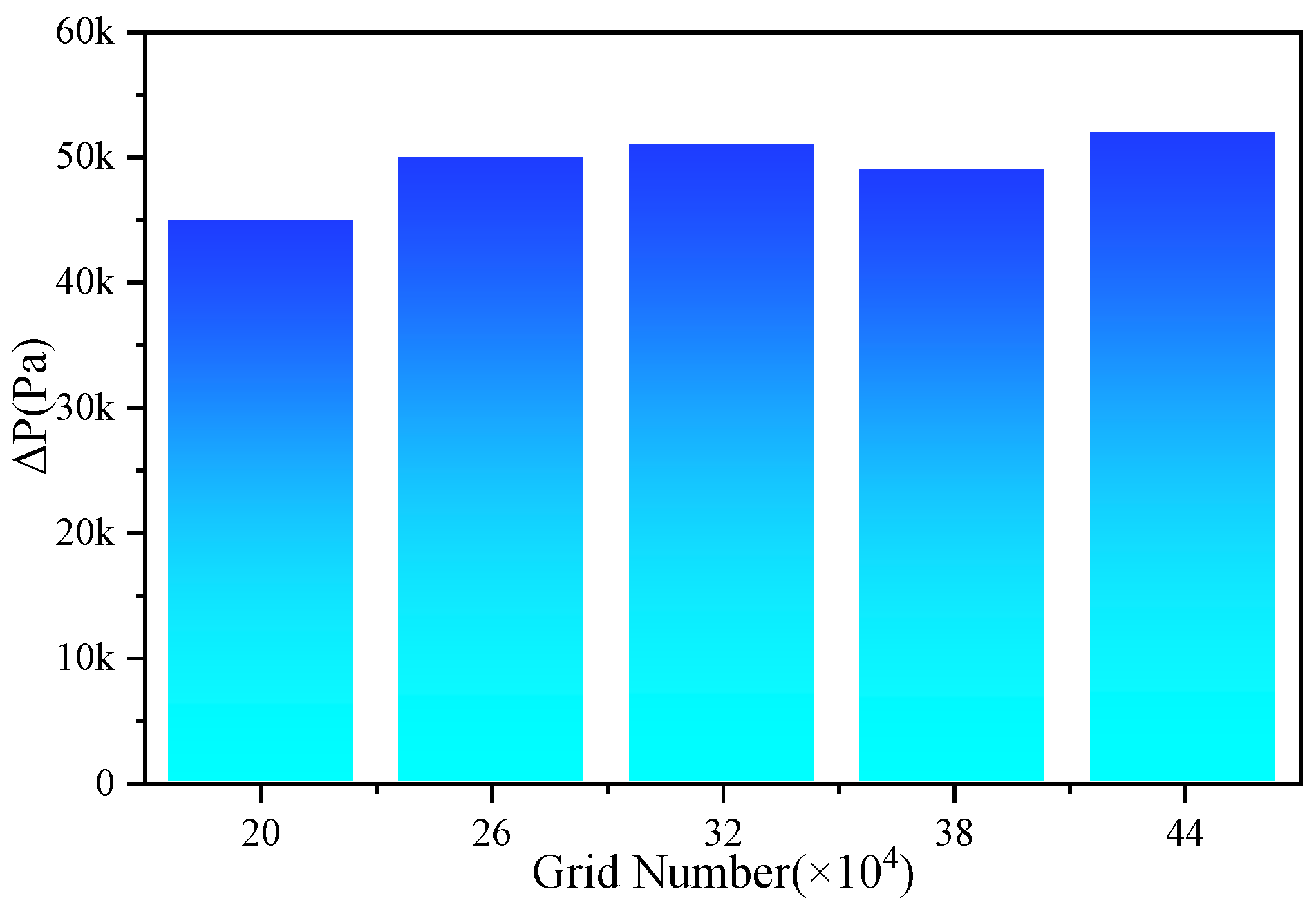
3.1. Parameterized Model Analysis of the Damper Valve

To investigate the effects of D_A, D_B, and D_C on the inlet–outlet pressure drop, the effect of the axial force on the spool of the damper valve, and the effects of the diameters of the internal holes on the opening force of the damper valve, a multi-parameter model of the damper valve was established using different settings for the diameters of the internal holes. First, D_A was varied (10 mm, 9 mm, 8 mm, 11 mm, and 12 mm) with both D_B and D_C held constant at 6 mm. Next, D_B was varied (6 mm, 5 mm, 4 mm, 7 mm, and 8 mm), with D_A and D_C held constant at 10 mm and 6 mm, respectively. Finally, D_C was varied (6 mm, 5 mm, and 7 mm), with D_A and D_B held constant at 10 mm and 6 mm, respectively.

Two indicators of most concern were selected to be analyzed in the subsequent design and optimization of the hydropneumatic spring, which were ΔP and F_Z in the flow domain of the damper valve. This was because ΔP was a critical factor that determined the correct opening of the damper valve and ΔP directly affected the stress in the damper valve during the opening process. F_Z was a critical factor that affected the service life of the damper valve. ΔP and F_Z at the 11 different configurations of D_A, D_B, and D_C as well as five different flow rates were calculated.

3.2. Analysis of the Inlet–Outlet Pressure Drop of the Damper Valve

Overall, ΔP increased quasi-quadratically as Q increased, as shown in Figure 5. Figure 5a–c shows the variations of ΔP with Q at different configurations of D_A, D_B, and D_C. Figure 5d–f shows three-dimensional (3D) representations of the variation trends. In the following, the configuration of D_A (x mm), D_B (y mm), and D_C (z mm) is designated as AxByCz. Figure 5a shows the variations of ΔP with Q and AxByCz with D_A varied and D_B and D_C held constant. ΔP increased as D_A or Q increased. Figure 5d shows a 3D representation of the above variation trend. ΔP increased more significantly with Q at a smaller value of D_A. ΔP also increased more significantly with Q as D_B or D_A increased, as shown in Figure 5b,c,e,f. However, D_B had a greater effect on ΔP, and D_C had an insignificant effect on ΔP. Following this, the variation of ΔP as D_A, D_B, or D_C was varied and the other two diameters were held constant was designated as ΔP^x_ij. For example, ΔP^A₈₉ designated the variation of ΔP as D_A increased from 8 mm to 9 mm and D_B and D_C were held constant. As shown in Figure 5a–c, ΔP^x_ij increased quasi-quadratically as Q increased. In particular, D_B had the greatest effect on ΔP, while D_C had the smallest effect on ΔP. Additionally, ΔP increased insignificantly with D_A at a smaller Q but increased more significantly at a larger Q, as shown in Figure 5e. This indicated that the effect of D_A on ΔP increased as Q increased. The effect of D_B on ΔP also increased as Q increased, as shown in Figure 5e. ΔP was insignificantly affected by D_C and was affected mainly by Q, as shown in Figure 5f.

The above results for the variations of ΔP were consistent with those published by Jian et al. [25], who stated that ΔP increased quasi-quadratically as Q increased. In particular, D_B had a major effect on ΔP. This was because of the special location and direction of the hole.

3.3. Analysis of the Spool Axial Force of the Damper Valve

Figure 6 shows the variations of F_Z with D_X and Q. F_Z exhibited similar but not identical variations to those of ΔP. Figure 6a–c shows the variations of F_Z with Q as D_A, D_B, and D_C were varied. Figure 6d–f shows the 3D representations of the variations. Overall, F_Z increased as Q increased, as shown in Figure 6a. However, in contrast to the variations in ΔP (which varied the most significantly with Q at the hole diameter configuration of A₈B₆C₆), F_Z increased the most significantly with Q at the hole diameter configuration of A₁₂B₆C₆. This was because F_Z was larger when D_A = 12 mm = D_in compared with when D_A < D_in. F_Z varied in a manner similar to ΔP as Q increased and D_B was varied, as shown in Figure 6b. In addition, at a given Q, F_Z increased as D_B decreased. D_C had an insignificant effect on F_Z, and the F_Z-Q curves at different values of D_C differed insignificantly, with the maximum difference being 1525.6 Pa, as shown in Figure 6c. F_Z was insignificantly affected by D_A and was mainly affected by Q, as shown in Figure 6d. However, D_A had a critical effect on F_Z at a higher Q, but only at a higher Q. F_Z was significantly affected by both D_B and Q, and F_Z reached the minimum when D_B reached the maximum and Q reached the minimum, as shown in Figure 6e. F_Z increased nonlinearly as D_B decreased and Q increased. F_Z reached the maximum when D_B reached the minimum and Q reached the maximum. This indicated the critical effect of D_B on F_Z because of the location of hole B. F_Z was insignificantly affected by D_C and was mainly affected by Q, as shown in Figure 6f. Similarly, this was because of the location of hole C, which was located at the outlet and whose effect on the spool axial force was negligible.

The above results showed that D_B also had the most significant effect on F_Z and the effect of D_B on F_Z was similar to its effect on ΔP. Both ΔP and F_Z increased with Q at various values of D_A. However, the effect of D_A on F_Z was significantly different from its effect on ΔP.

3.4. Multi-Objective Optimization of the Damper Valve

Multi-objective optimization of the damper valve was performed under the condition that it did not open and remained in its initial state when Q ≤ 70 L/min. The initial back pressure of the spring was set to 110 N, and its toughness was set to 10 N/mm. The target value of the ΔP optimization was set to 0.75 MPa, the middle value of the range of ΔP for automobile applications of the damper valve. Therefore, the objective was to optimize ΔP and the maximum spool axial force F_Z at Q = 70 L/min. Prior to the optimization of the damper valve, a mathematical model for describing the relationship between the explanatory and response variables was established. The functional relationship between the explanatory and response variables was identified based on massive experimental data. Experimental data were obtained using an orthogonal experimental design because the accuracy of this type of response function is dependent on the quality of the experimental data.

A three-factor (D_A, D_B, and D_C) five-level orthogonal experimental design was adopted. The L₂₅(5⁶) (with “25” indicating the number of tests, “5” indicating the number of levels, and 6 indicating the number of factors) orthogonal table was used. Since only three factors were tested, the last three columns of the table were not used (because there were no parametric values).

Table 2. Orthogonal experimental design and test results.

Test	Factors			ΔP (MPa)	FZ (N)
	1 (DA)	2 (DB)	3 (DC)
1	1 (10 mm)	1 (6 mm)	1 (6 mm)	0.51	116.33
2	1	2 (5 mm)	3 (5 mm)	0.90	190.36
3	1	3 (4 mm)	5 (7 mm)	0.88	179.02
4	1	4 (7 mm)	2 (5.5 mm)	0.32	65.18
5	1	5 (8 mm)	4 (6.5 mm)	0.21	43.78
6	2 (9 mm)	1	5	0.51	86.62
7	2	2	2	0.94	143.14
8	2	3	4	1.93	298.26
9	2	4	1	0.37	54.06
10	2	5	3	0.32	48.48
11	3 (8 mm)	1	4	0.63	84.30
12	3	2	1	1.04	184.26
13	3	3	3	2.15	254.00
14	3	4	5	0.45	57.08
15	3	5	2	0.40	43.28
16	4 (11 mm)	1	3	0.49	137.40
17	4	2	5	0.87	251.10
18	4	3	2	1.90	516.80
19	4	4	4	0.27	85.76
20	4	5	1	0.24	61.52
21	5 (12 mm)	1	2	0.45	163.38
22	5	2	4	0.80	290.02
23	5	3	1	1.97	767.24
24	5	4	3	0.34	103.00
25	5	5	5	0.17	69.32

shows the orthogonal experimental design and the test results.

Tests were performed according to the orthogonal experimental design, with test #1 performed using the initial valve designs. Simulations were performed using the corresponding configurations of D_A, D_B, and D_C (designated as x₁, x₂, and x₃, respectively) at Q = 70 L/min. The simulation results for ΔP and F_Z are shown in Table 2.

Two functions were established for describing the responses of ΔP and F_Z to D_A, D_B, and D_C using response surface methodology. To investigate the overall effects of the explanatory variables on the response variables, the two response variables were weighted to combine the two functions into one function (mathematical model for multi-objective optimization). Based on ΔP and F_Z at different levels of the explanatory variables (x₁, x₂, and x₃) obtained from the orthogonal experiment, two functions (Equations (1) and (2)) for describing the responses of ΔP and F_Z to the explanatory variables were established.

$$\Delta P_{\max }=a_0+a_1{x}_1+a_2{x}_2+a_3{x}_3+a_{11}{x}_1^2+a_{12}{x}_1{x}_2+a_{13}{x}_1{x}_3+a_{22}{x}_2^2+a_{23}{x}_2{x}_3+a_{33}{x}_3^2$$

(1)

$$F_Z{}_{\max }=b_0+b_1{x}_1+b_2{x}_2+b_3{x}_3+b_{11}{x}_1^2+b_{12}{x}_1{x}_2+b_{13}{x}_1{x}_3+b_{22}{x}_2^2+b_{23}{x}_2{x}_3+b_{33}{x}_3^2$$

(2)

The response surface functions obtained based on the experimental data shown in Table 2 are as follows:

$$\begin{array}{l}\Delta P_{\max }=0.47-0.087x_1-0.71x_2-0.11x_3+0.15x_1^2\\ \begin{array}{cc}\begin{array}{cc}& \end{array}& \end{array}-0.045x_1{x}_2-0.062x_1{x}_3+0.54x_2^2+0.18x_2{x}_3-0.1x_3^2\end{array}$$

(3)

$$\begin{array}{l}{F}_Z{}_{\max }=108.51+78.48x_1-167.67x_2-12.39x_3+53.22x_1^2\\ \begin{array}{ccc}& & \end{array}-111.97x_1{x}_2-23.02x_1{x}_3+124.69x_2^2+56.99x_2{x}_3-51.43x_3^2\end{array}$$

(4)

The closeness of fit of the response functions was verified based on the multiple correlation coefficient R and correction coefficient ${\overline{R}}^2$.

Table 3. Multiple correlation coefficient R and correction coefficient ${\overline{R}}^2$.

	R	${\overline{\mathit{R}}}^2$
ΔPmax	0.9672	0.8968
FZmax	0.9549	0.8589

shows the results. Both R and ${\overline{R}}^2$ of the two response functions were greater than 0.85, confirming that the closeness of fit of the response functions for ΔP_max and F_Zmax was acceptable for subsequent optimization.

Because ΔP_max and F_Zmax were two major performance indicators of the damper valves, the following objective function was established to investigate the overall effect of the explanatory variables on the two major performance indicators:

$$W=w_1{\left(\frac{\Delta P_{\max }-\Delta P_m}{\Delta P_m}\right)}^2+w_2{\left(\frac{F_Z{}_{\max }-F_Z{}_m}{F_Z{}_m}\right)}^2$$

(5)

where w₁ and w₂ are the weight factors and w₁ + w₂ = 1, based on the multiple correlation coefficients for ΔP_max = 0.9672 and F_Zmax = 0.9549 obtained above. The analytic hierarchy process is used to calculate w₁ and w₂.

Table 4. The comparison value of index m and n.

Indicator m and n	Equally Important	Relative Importance	Importance
emn	1	3/2	2

is the corresponding table of the comparative values of indicator m and indicator n, and e_mn is the comparative value of the two. According to Table 4, the e_mn of comparison values between indicators is determined, and the judgment matrix is constructed, as shown in

Table 5. Theoretical judgment matrix.

Comparison Value	Indicator 1	Indicator 2
Indicator 1	1	e12
Indicator 2	e21 (1/e12)	1

. ΔP is a key factor in determining whether the damping valve can be opened. ΔP directly affects the stress on the damping valve when it is opened, and its importance is slightly higher than F_Z. Therefore, the judgment matrix of this study is shown in

Table 6. Judgment matrix.

Comparison Value	Indicator 1	Indicator 2
Indicator 1	1	2/3
Indicator 2	3/2	1

. According to Table 6, w₁ = 0.4 and w₂ = 0.6; ΔP_m and F_Zm are the means of ΔP and F_Z, respectively, with ΔP_m = 0.7624 and F_Zm = 85.8736. ΔP_max and F_Zmax are as defined in Equations (3) and (4), respectively. With the values of the x₁, x₂, and x₃ limited, the mathematical optimization model can be expressed as:

$$\begin{array}{c}\min W=0.6{\left(\frac{\Delta P_{\max }-\Delta P_m}{\Delta P_m}\right)}^2+0.4{\left(\frac{F_Z{}_{\max }-F_Z{}_m}{F_Z{}_m}\right)}^2\\ s.t.\{\begin{array}{c}{x}_1\in \left[8,12\right]\\ x_2\in \left[4,8\right]\\ x_1\in \left[5,7\right]\end{array}\end{array}$$

(6)

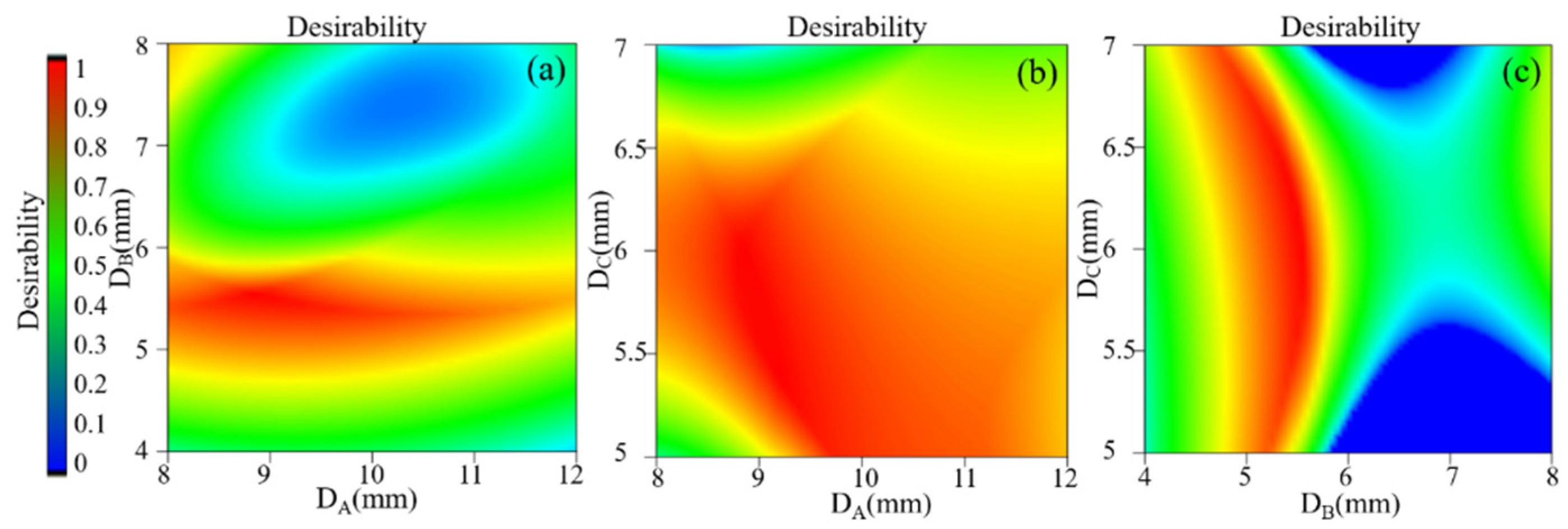
Figure 7 shows the nephogram of the relationship between the desirability of the damper valve and the explanatory variables: the effect of the interaction between any pair of explanatory variables on the performance of the damper. Figure 7a shows the nephogram of the effect of the interaction between D_A and D_B on the desirability. The desirability of the damper valve was high when D_B fell in the range of 5–6 mm and D_A ≤ 10 mm. Figure 7b shows the nephogram of the effect of the interaction between D_A and D_C on the desirability. The desirability was high when D_C was approximately 6 mm and D_A was approximately 9 mm. Figure 7c shows the nephogram of the effect of the interaction between D_B and D_C on the desirability. The desirability was high when D_C was approximately 6 mm and D_A was approximately 5.5 mm. Figure 7 reveals the approximate ranges of the optimal solutions of the explanatory variables and the variations of the response variables with the explanatory variables in a straightforward, quantitative manner.

Table 7. Optimized hole diameters.

	DA (mm)	DB (mm)	DC (mm)
Initial parameters	10	6	6
Value of optimization	8.83	5.55	6.01
Reasonable value	8.8	5.55	6

shows the optimum solutions obtained based on the response functions for ΔP_max and F_Zmax (Equations (3) and (4)) and the optimization model (Equations (5) and (6)) with rounding based on the drill bit sizes used in actual manufacturing.

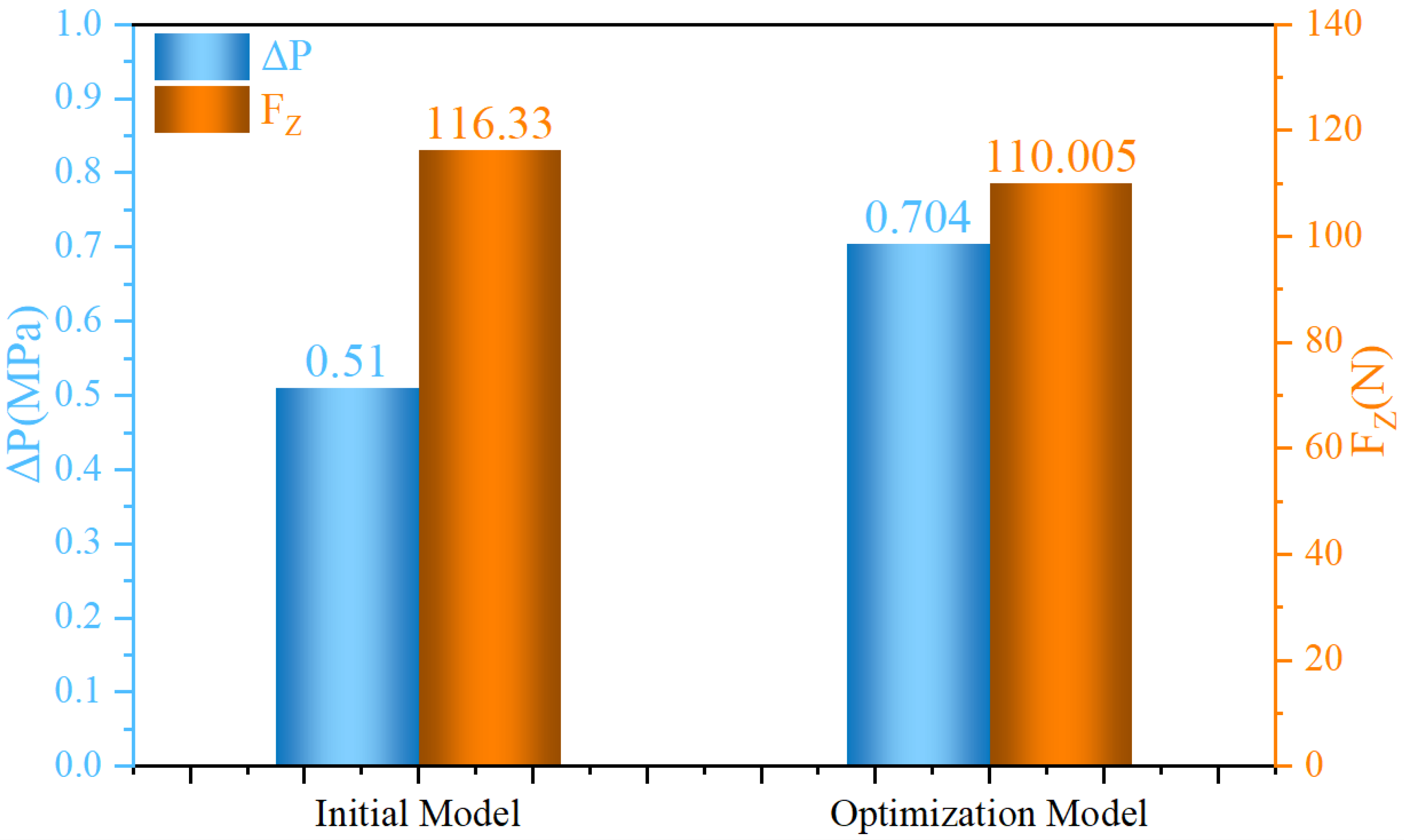
The holes in the damper valves were optimized based on the optimization results. Fluid–solid coupling simulations were performed using the optimized hole sizes. The simulated ΔP and F_Z were 0.704 MPa and 110.005 N, respectively. Figure 8 shows a comparison of the simulated ΔP and F_Z of the initial and optimized valve designs at Q = 70 L/min. Figure 8 reveals the difference in the performance of the initial and optimized valve designs in a straightforward manner. The simulated ΔP values of the initial and optimized designs of the damper valve were 0.51 and 0.704, respectively, while the target range of ΔP was 0.5–1 MPa. Despite the ΔP of the initial damper valve meeting the design requirements, its value was close to the lower limit of the target range and was less desirable than the value expected by the designers. The ΔP of the optimized damper valve was close to the middle value of the target range. A comparison showed that the ΔP of the optimized valve was better than that of the initial design of the valve. Using ΔP = 0.75 MPa as a reference, the error in ΔP of the initial valve design was 32%, while that of the optimized valve decreased to 6.13%. The simulated F_Z values of the initial and optimized valve designs were 116.33 N and 110.005 N, respectively, while the target range was ≤110 N. The F_Z of the optimized valve was improved compared with that of the initial valve design. The error in F_Z for the initial design was 0.28%, while that of the optimized valve decreased to a negligible level of 0.0045%. It was noteworthy that D_A, D_B, and D_C depended on the drill bit sizes used in actual manufacturing and, thus, could not be designed to the exact dimensions yielded using the multi-objective optimization. This was a major reason for the difference between the simulated and required target values.

Figure 9 shows the nephogram of the flow field in the optimized damper valve. A comparison of Figure 9a and Figure 4b showed that the numerically simulated ΔP and F_Z were closer to the target ΔP and F_Z desired for engineering applications. Additionally, the stress distribution in the flow field changed significantly. The reason for this was that the D_A and D_B of the optimized valve were smaller than those of the initial design. Additionally, as revealed by the simulation results provided in Section 3.2, ΔP increased as D_A and D_B decreased. It was also noteworthy that significant turbulence occurred at the outlet and in the central chamber of the valve. This was because of the geometric structure of the valve. Figure 9c is highly consistent with Figure 9a,b. The flow velocity near the outlet was low, which indicated that the damper valve had a strong damping effect.

This section describes how an orthogonal simulation experiment was performed using the simulation model to meet the design objective. Next, multi-objective optimization was performed by establishing response surfaces. Finally, a configuration of the hole diameters of the damper valve that met the requirements for actual automobile applications was determined. The optimization results were verified using simulation calculations. Figure 9 displays the results. The optimization results showed that the simulated results of the optimization model were good, the simulated results were largely consistent with the target values, and the numerically simulated flow field was acceptable. This provides an effective reference for the future development of hydropneumatic springs. In addition, we plan to investigate the effects of the parameters not explored in this study (such as the sectional length, length-to-diameter ratio, and number of holes) on the performance of the damper valves.

4. Conclusions

The inlet–outlet pressure drop and spool axial force of a hydropneumatic spring damper valve for heavy vehicle applications were investigated using a fluid–solid coupling method. The objective was to optimize these two parameters to meet design expectations. This work helps lay a foundation for the further design and optimization of damper valves and provides a reference for future redevelopment. Our results are summarized as follows:

(1) With the diameters of the damping holes held constant, the inlet–outlet pressure drop of the damper valve increased quasi-quadratically and nonlinearly with the inlet flow rate. For a given inlet flow rate, the inlet–outlet pressure drop of the damper valve increased nonlinearly with the diameters of the holes. In particular, the diameter of hole B had the greatest effect on the inlet–outlet pressure drop, while D_C had the smallest effect on the inlet–outlet pressure drop. The different effects were due to the different locations of the holes.

(2) When the diameters of the damping holes were held constant, the spool axial force increased quasi-quadratically and nonlinearly with the flow rate. For a given inlet flow rate, the spool axial force increased nonlinearly as D_A increased, decreased nonlinearly as D_B increased, and fluctuated as D_C increased. In particular, D_B had the greatest effect on the spool axial force, while D_C had the smallest effect on the spool axial force. Similarly, the different effects were due to the different locations of the holes.

(3) Multi-objective optimization of the valve was performed against the actual engineering requirements using response surface methodology. The results showed that the design expectations were satisfied when D_A, D_B, and D_C were set to 8.8 mm, 5.55 mm, and 6 mm, respectively. Using an inlet–outlet pressure drop of 0.75 MPa as a reference, the error in ΔP for the initial valve design was 32%, while that of the optimized valve decreased to 6.13%. The error in F_Z of the initial valve design was 0.28%, while that of the optimized valve decreased to 0.0045%.

This study had limitations. A small range of Q values was investigated. Overall, this study was focused on low flow-rate, closure operating conditions. The high flow-rate, opening operating conditions, and circulation flow of the damper valve were not investigated. We plan to investigate these topics in future work, especially the flow field in the damper valve in high flow-rate operating conditions and its failure under high flow-velocity operating conditions.