Influence of Cutting Parameters and Tool Geometry on Topography of Hard Turned Surfaces
Abstract
1. Introduction
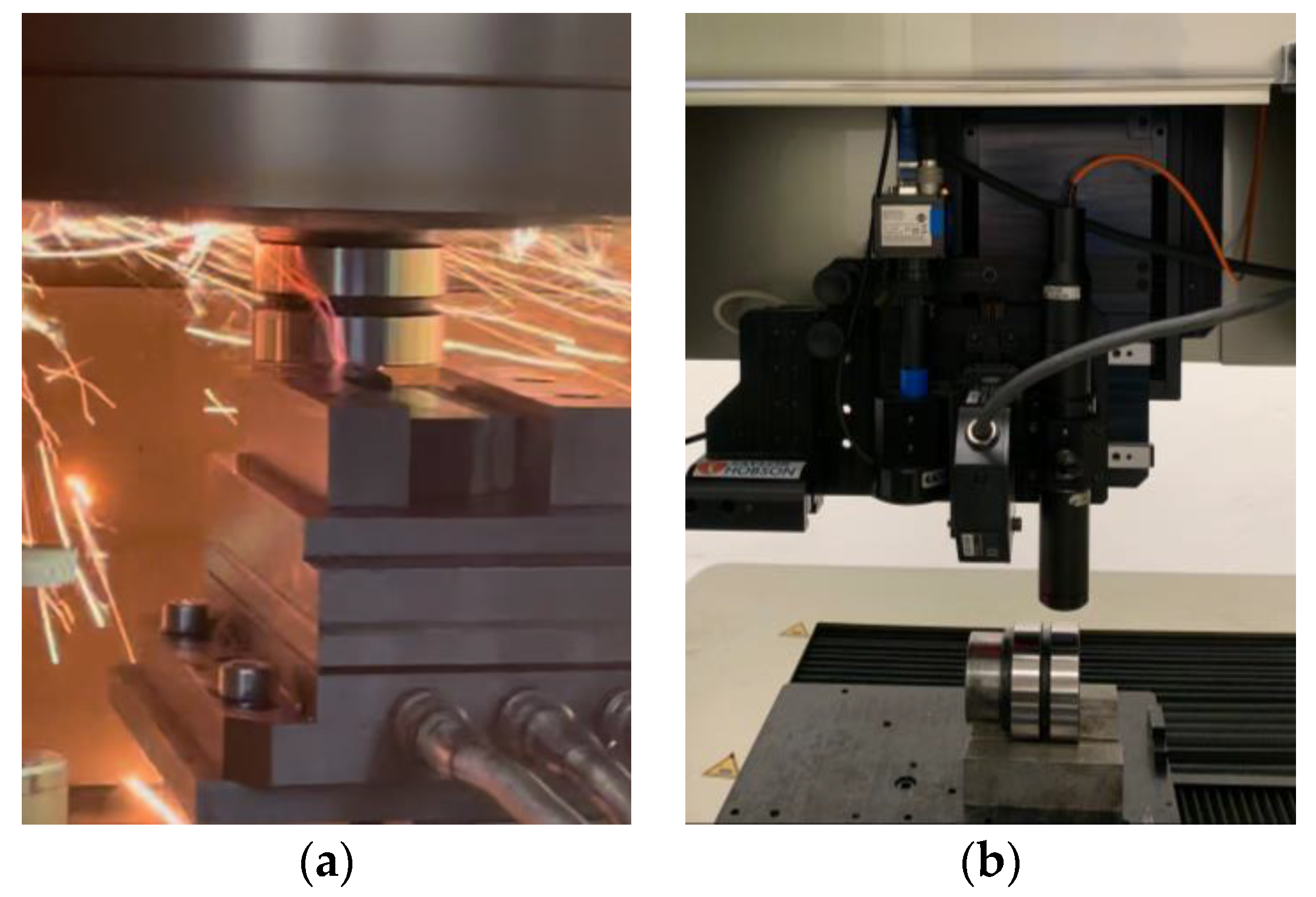
2. Material and Methods
3. Results and Analysis
3.1. Normality Test and Analysis of Variance
- Spk and Svk were not influenced by the depth-of-cut when the standard insert was used. Ssk, Sku, Sa, and Sk were not influenced by the depth-of-cut when the wiper insert was used.
- Ssk, Sz, Sa, Spk, and Svk were not influenced by the cutting speed when the standard insert was used. Ssk, Sku, Sa, Sk, and Svk were not influenced by the cutting speed when the wiper insert was used.
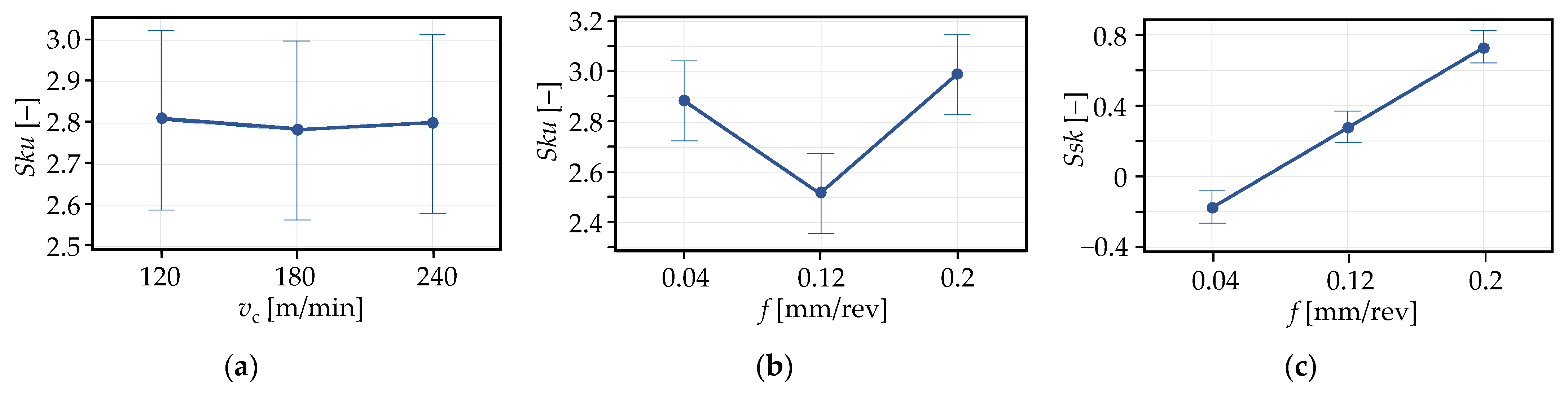
- Sku, Spk, and Svk were influenced by the feed rate when the standard insert was used. Ssk and Sku were influenced by the feed rate when the wiper insert was used.
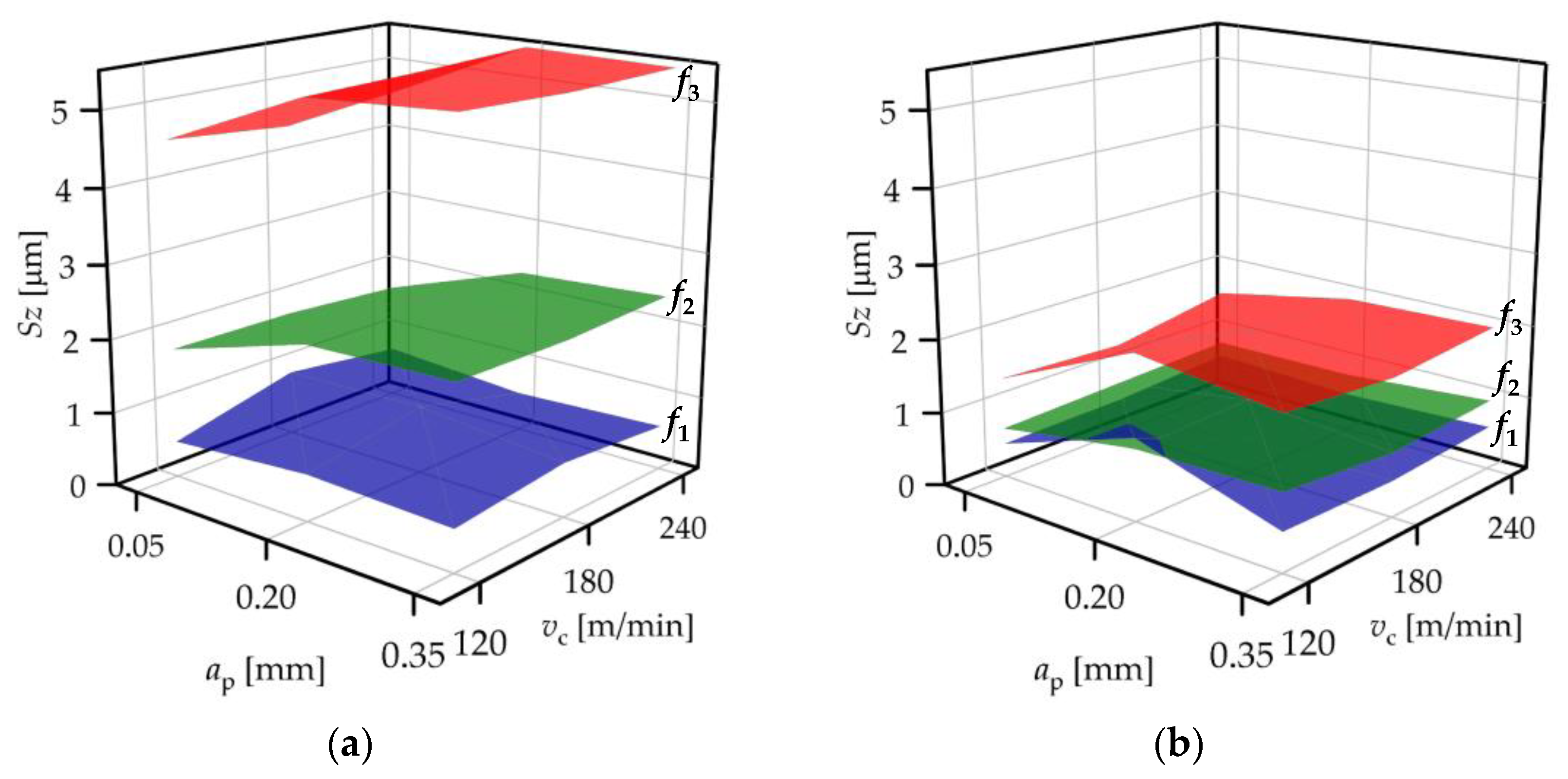
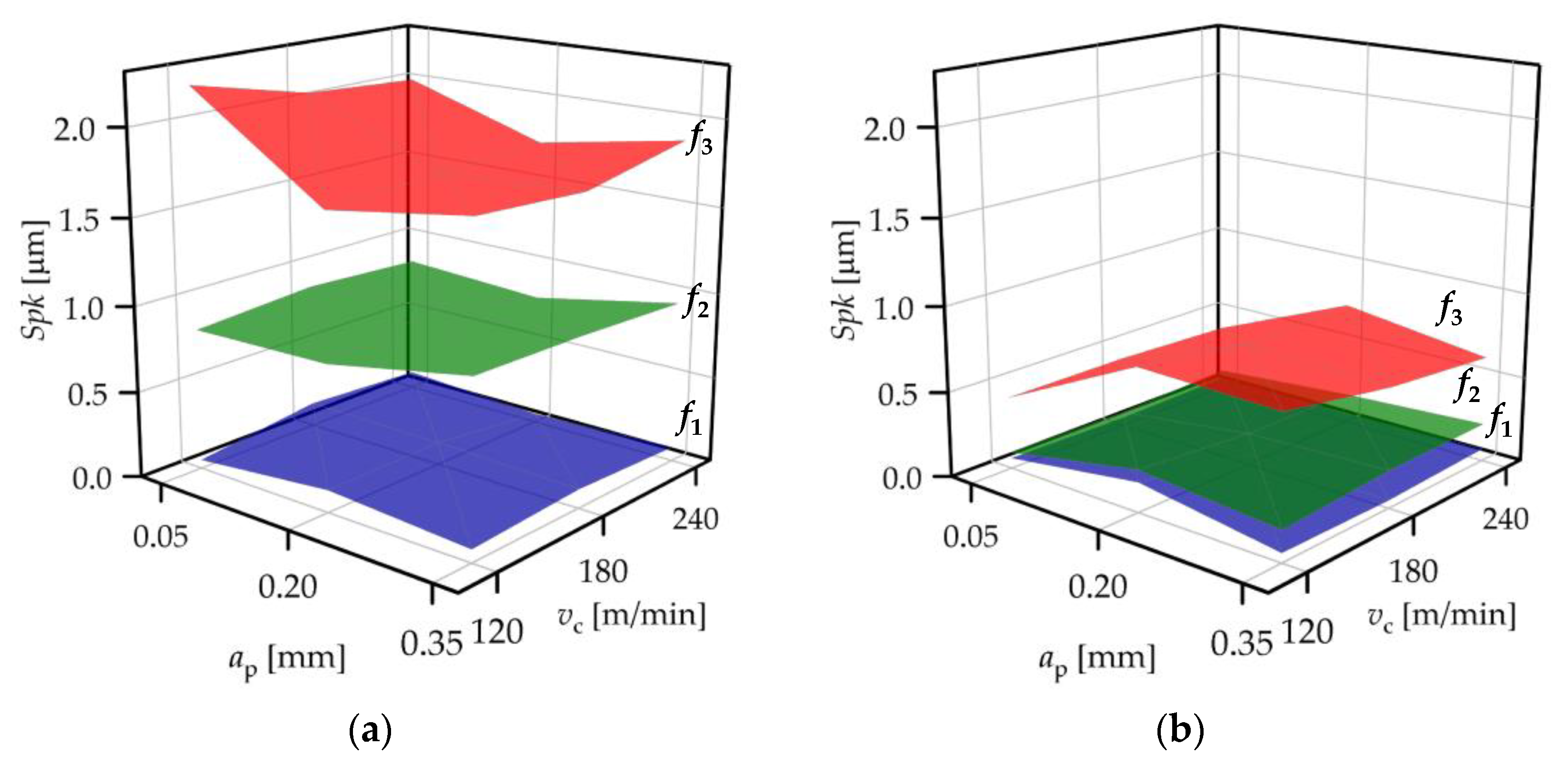
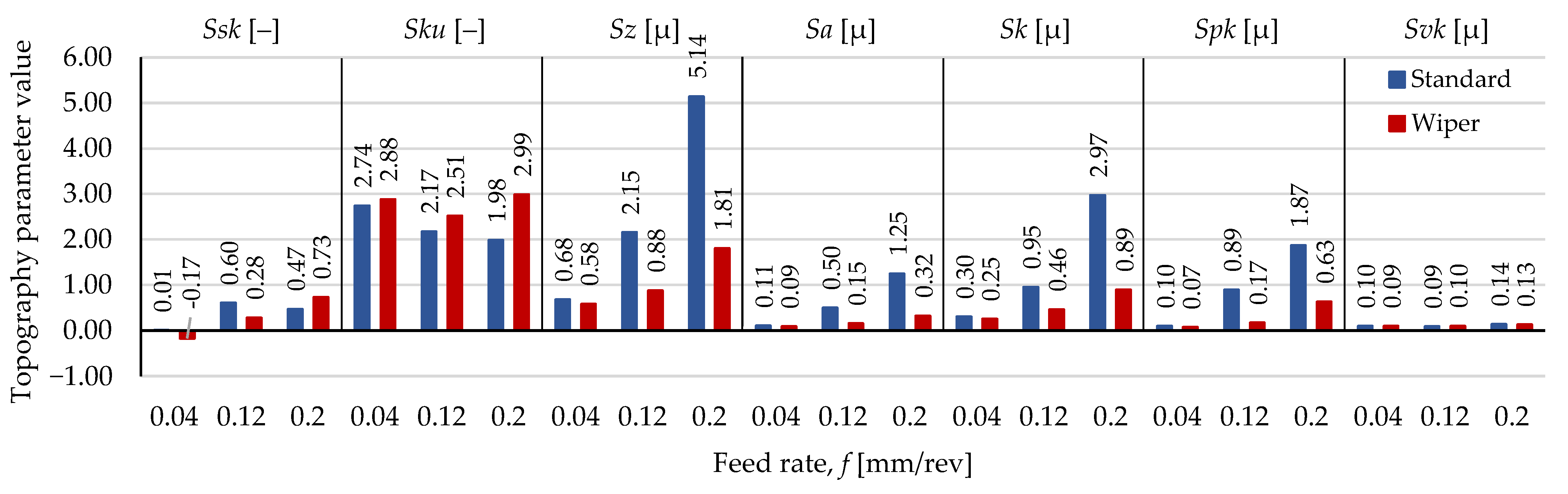
3.2. Analysis of the Topography Parameters
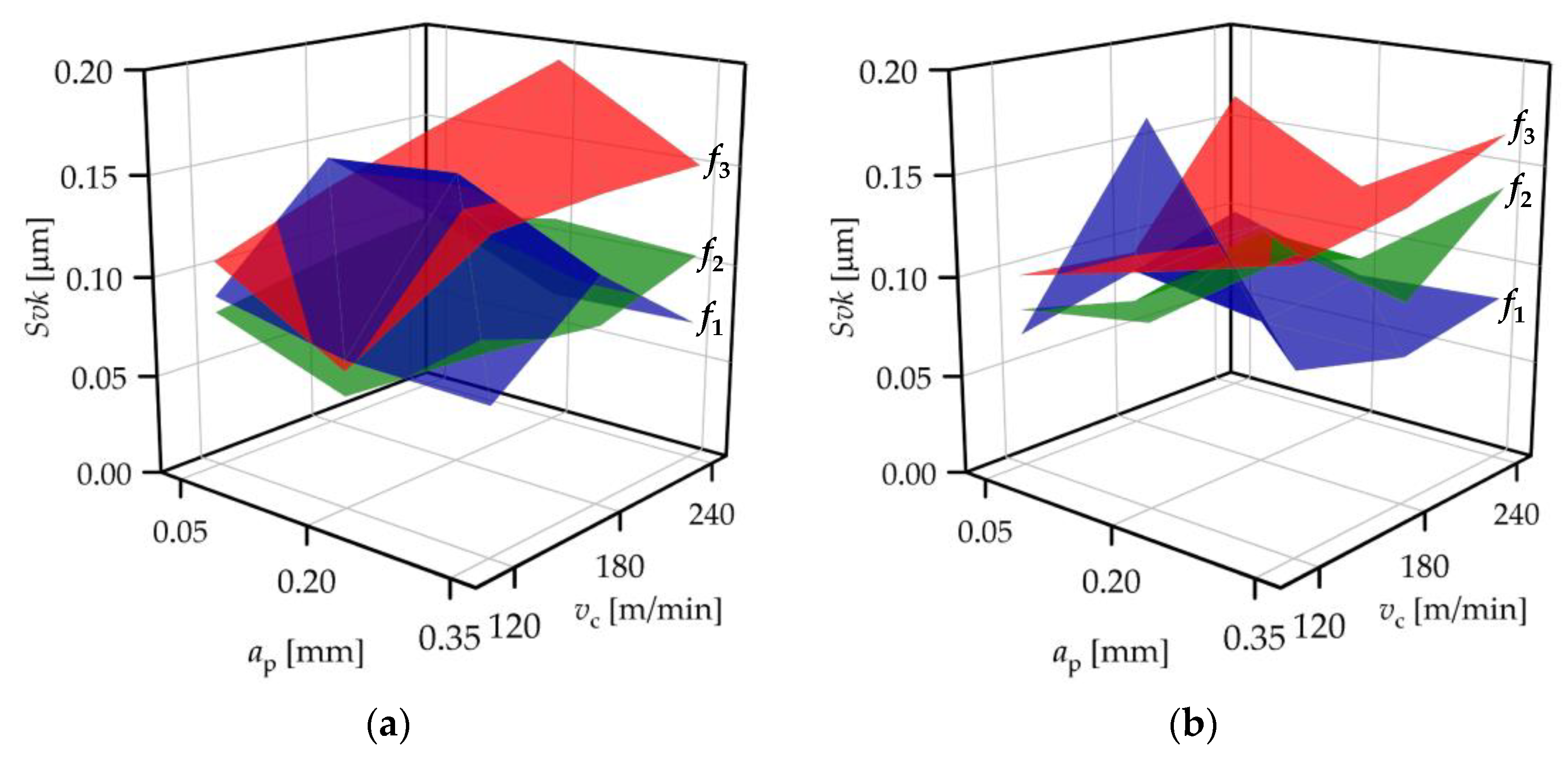
3.3. Response Functions for the Topography Parameters
4. Discussion of the Results
5. Conclusions
- A total of 126 data groups were analyzed for normality by the Shapiro–Wilk test. In 90 groups, normality could not be rejected, and in 36, the normality was rejected.
- Where the ANOVA test was carried out (normality fulfilled), the following statements can be made: the Sku, Spk, and Svk parameters were influenced by the feed rate when the standard insert was used, and Ssk and Sku were influenced by the feed rate when the wiper insert was used.
- When the standard insert was used, the strongest influencing cutting parameter was f in the case of the topography parameters Ssk, Sku, and Spk; f2 in the case of Sa, Sz, and Sk; and the vcf interaction in the case of Svk. When the wiper insert was used, the strongest influencing cutting parameter was f in the case of the topography parameter Ssk; f2 in the case of Sa, Sz, Sk, and Spk; and the apf interaction in the case of Sku and Svk.
- Based on the correlation analysis, it can be stated that the Sa and Sz parameters could be useful in assessing surface characteristics related to the functional or tribological properties connected to the Ssk, Sku, Sk, and Spk parameters.
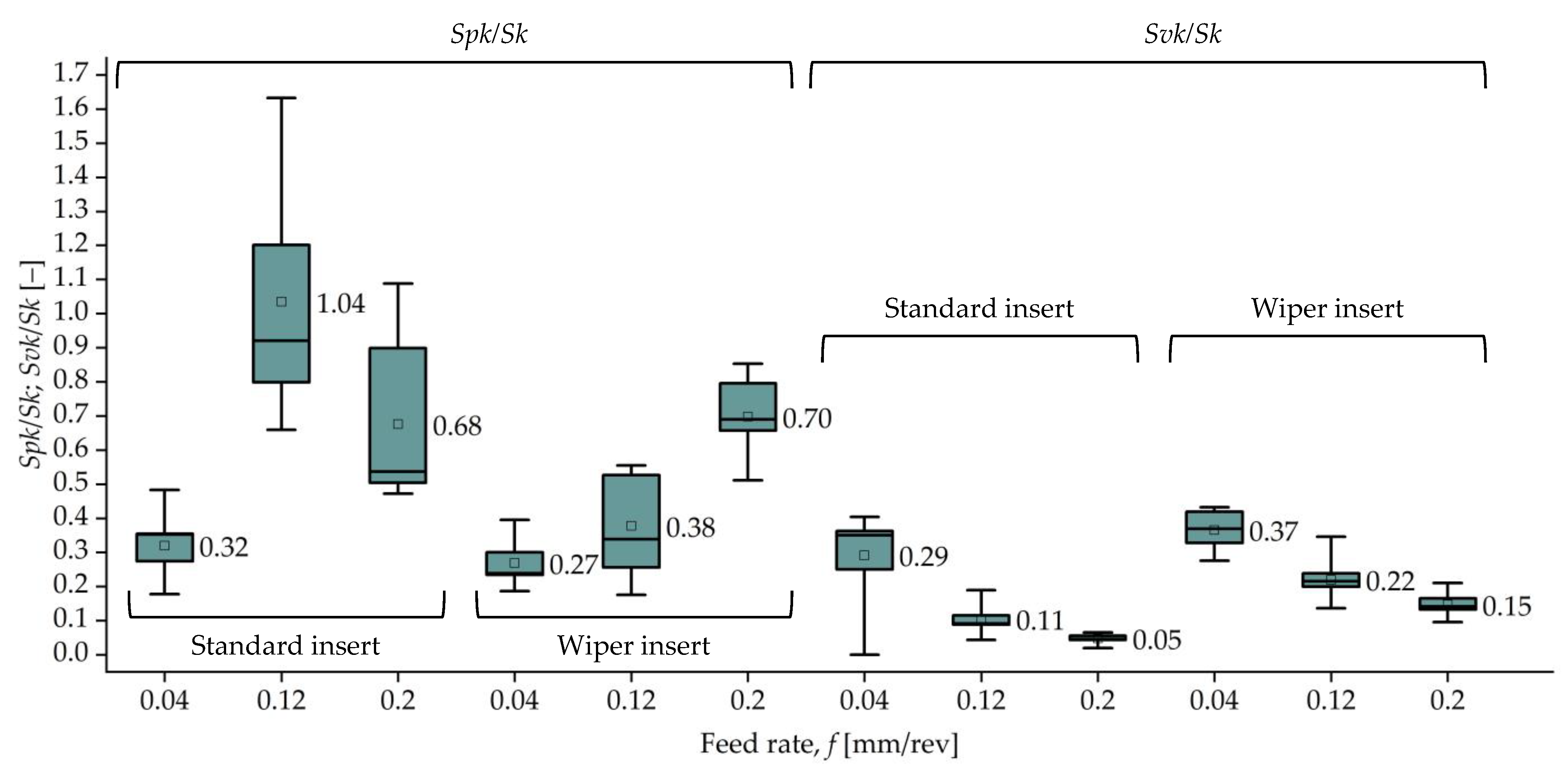
- The Ssk parameter has a strong or extremely strong relationship with the Spk/Sk and the Svk/Sk rates, which indicates the possibility for substituting Ssk for the rate parameters.
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A
| Insert | Cutting Data | Ssk | Sku | Sz | Sa | Sk | Spk | Svk | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| W | p | W | p | W | p | W | p | W | p | W | p | W | p | |||
| Standard | ap (mm) | 0.05 | 0.872 | 0.130 | 0.915 | 0.355 | 0.810 | 0.026 | 0.801 | 0.021 | 0.771 | 0.010 | 0.852 | 0.078 | 0.887 | 0.186 |
| 0.2 | 0.747 | 0.005 | 0.766 | 0.008 | 0.826 | 0.040 | 0.823 | 0.037 | 0.796 | 0.018 | 0.859 | 0.093 | 0.883 | 0.168 | ||
| 0.35 | 0.812 | 0.028 | 0.778 | 0.011 | 0.818 | 0.033 | 0.818 | 0.033 | 0.776 | 0.011 | 0.852 | 0.079 | 0.876 | 0.142 | ||
| vc (m/min) | 120 | 0.913 | 0.341 | 0.873 | 0.132 | 0.860 | 0.096 | 0.861 | 0.098 | 0.845 | 0.065 | 0.907 | 0.293 | 0.882 | 0.165 | |
| 180 | 0.901 | 0.258 | 0.800 | 0.021 | 0.852 | 0.078 | 0.854 | 0.083 | 0.814 | 0.029 | 0.884 | 0.173 | 0.899 | 0.249 | ||
| 240 | 0.937 | 0.549 | 0.893 | 0.215 | 0.853 | 0.081 | 0.849 | 0.073 | 0.826 | 0.041 | 0.872 | 0.131 | 0.918 | 0.377 | ||
| f (mm/rev) | 0.04 | 0.890 | 0.199 | 0.944 | 0.627 | 0.923 | 0.417 | 0.929 | 0.473 | 0.918 | 0.378 | 0.920 | 0.395 | 0.836 | 0.052 | |
| 0.12 | 0.884 | 0.175 | 0.857 | 0.088 | 0.738 | 0.004 | 0.781 | 0.012 | 0.870 | 0.123 | 0.924 | 0.429 | 0.939 | 0.574 | ||
| 0.2 | 0.802 | 0.021 | 0.866 | 0.112 | 0.715 | 0.002 | 0.721 | 0.002 | 0.741 | 0.004 | 0.911 | 0.321 | 0.932 | 0.503 | ||
| Wiper | ap (mm) | 0.05 | 0.919 | 0.388 | 0.872 | 0.129 | 0.865 | 0.108 | 0.894 | 0.218 | 0.921 | 0.404 | 0.720 | 0.002 | 0.768 | 0.009 |
| 0.2 | 0.913 | 0.339 | 0.910 | 0.314 | 0.889 | 0.193 | 0.874 | 0.134 | 0.902 | 0.265 | 0.793 | 0.017 | 0.881 | 0.162 | ||
| 0.35 | 0.924 | 0.426 | 0.896 | 0.231 | 0.829 | 0.044 | 0.836 | 0.053 | 0.837 | 0.054 | 0.826 | 0.040 | 0.905 | 0.282 | ||
| vc (m/min) | 120 | 0.948 | 0.667 | 0.938 | 0.561 | 0.908 | 0.304 | 0.902 | 0.263 | 0.928 | 0.460 | 0.798 | 0.020 | 0.920 | 0.396 | |
| 180 | 0.941 | 0.588 | 0.984 | 0.981 | 0.867 | 0.113 | 0.897 | 0.236 | 0.921 | 0.400 | 0.805 | 0.024 | 0.926 | 0.446 | ||
| 240 | 0.953 | 0.727 | 0.953 | 0.720 | 0.830 | 0.045 | 0.851 | 0.077 | 0.869 | 0.120 | 0.828 | 0.042 | 0.890 | 0.200 | ||
| f (mm/rev) | 0.04 | 0.928 | 0.458 | 0.914 | 0.345 | 0.599 | 0.000 | 0.696 | 0.001 | 0.798 | 0.020 | 0.563 | 0.000 | 0.629 | 0.000 | |
| 0.12 | 0.902 | 0.261 | 0.894 | 0.220 | 0.924 | 0.430 | 0.928 | 0.465 | 0.921 | 0.400 | 0.910 | 0.313 | 0.904 | 0.274 | ||
| 0.2 | 0.978 | 0.954 | 0.969 | 0.884 | 0.892 | 0.211 | 0.963 | 0.827 | 0.982 | 0.972 | 0.864 | 0.105 | 0.949 | 0.682 | ||
| β0 | β1 | β2 | β3 | β11 | β22 | β33 | β12 | β13 | β23 | ||
|---|---|---|---|---|---|---|---|---|---|---|---|
| Standard insert | Ssk | −0.7754 | −0.3392 | 0.0011 | 18.1603 | 3.8672 | 0.000000 | −57.0061 | −0.0036 | −7.0882 | −0.0009 |
| Sku | 2.6876 | −4.1695 | 0.0106 | −12.7357 | 8.6985 | −0.000027 | 29.4687 | −0.0034 | 2.5132 | 0.0022 | |
| Sa | −0.0433 | 1.2206 | 0.0004 | −0.3570 | −3.5973 | −0.000001 | 28.0512 | 0.0005 | 4.9007 | −0.0012 | |
| Sz | −0.3005 | 3.8343 | 0.0077 | −4.2442 | −11.2526 | −0.000020 | 117.8880 | −0.0008 | 20.9708 | −0.0019 | |
| Sk | 0.2555 | 6.0296 | 0.0000 | −13.8065 | −17.5195 | 0.000001 | 106.9340 | −0.0012 | 25.5389 | −0.0014 | |
| Spk | −0.3094 | −1.5050 | 0.0015 | 8.9316 | 4.2114 | −0.000005 | 13.9748 | 0.0017 | −5.4125 | −0.0008 | |
| Svk | 0.0008 | −0.1270 | 0.0018 | −1.4901 | 0.2790 | −0.000005 | 4.2648 | −0.0005 | 1.0125 | 0.0028 | |
| Wiper insert | Ssk | −0.3629 | 1.4551 | −0.0008 | 4.9441 | −5.1165 | −0.000002 | −0.0556 | 0.0046 | 2.1590 | 0.0016 |
| Sku | 4.2310 | −2.4694 | −0.0035 | −20.7776 | 1.4988 | 0.000006 | 65.8160 | −0.0001 | 17.7944 | 0.0115 | |
| Sa | 0.1477 | 0.9556 | −0.0013 | −0.6355 | −2.0748 | 0.000004 | 8.2057 | −0.0009 | 1.4354 | −0.0009 | |
| Sz | 1.3660 | 4.1173 | −0.0097 | −7.3540 | −9.4770 | 0.000026 | 49.8099 | −0.0041 | 10.7340 | 0.0050 | |
| Sk | 0.3117 | 2.1808 | −0.0027 | −0.3833 | −4.3785 | 0.000009 | 18.0677 | −0.0028 | 3.0132 | −0.0030 | |
| Spk | 0.2374 | 1.6341 | −0.0019 | −3.8877 | −4.3193 | 0.000006 | 28.2292 | −0.0010 | 6.0757 | −0.0033 | |
| Svk | 0.2625 | 0.2208 | −0.0018 | −0.9264 | −0.2793 | 0.000004 | 1.8021 | −0.0006 | 0.6701 | 0.0033 | |
References
- Mallick, R.; Kumar, R.; Panda, A.; Sahoo, A.K. Current status of hard turning in manufacturing: Aspects of cooling strategy and sustainability. Lubricants 2023, 11, 108. [Google Scholar] [CrossRef]
- Mamalis, A.G.; Kundrak, J.; Horvath, M. On a novel tool life relation for precision cutting tools. J. Manuf. Sci. Eng. 2005, 127, 328–332. [Google Scholar] [CrossRef]
- Kundrak, J.; Palmai, Z. Application of general tool-life function under changing cutting conditions. Acta Polytech. Hung. 2014, 11, 61–76. [Google Scholar] [CrossRef]
- Kundrak, J.; Mamalis, A.G.; Szabo, G.; Palmai, Z.; Gyani, K. Numerical examination of residual stresses developing during hard turning at different rake angles. Int. J. Adv. Manuf. Technol. 2017, 89, 1989–1999. [Google Scholar] [CrossRef]
- Vaxevanidis, N.M.; Kechagias, J.D.; Fountas, N.A.; Manolakos, D.E. Evaluation of Machinability in Turning of Engineering Alloys by Applying Artificial Neural Networks. Open Constr. Build. Technol. J. 2014, 8, 389–399. [Google Scholar] [CrossRef]
- Fountas, N.; Koutsomichalis, A.; Kechagias, J.D.; Vaxevanidis, N.M. Multi-response optimization of CuZn39Pb3 brass alloy turning by implementing Grey Wolf algorithm. Fract. Struct. Integr. 2019, 50, 584–594. [Google Scholar] [CrossRef]
- Jouini, N.; Revel, P.; Thoquenne, G. Influence of surface integrity on fatigue life of bearing rings finished by precision hard turning and grinding. J. Manuf. Process. 2020, 57, 444–451. [Google Scholar] [CrossRef]
- Elbah, M.; Laouici, H.; Benlahmidi, S.; Nouioua, M.; Yallese, M. Comparative assessment of machining environments (dry, wet and MQL) in hard turning of AISI 4140 steel with CC6050 tools. Int. J. Adv. Manuf. Technol. 2019, 105, 2581–2597. [Google Scholar] [CrossRef]
- Li, S.; Chen, T.; Qiu, C.; Wang, D.; Liu, X. Experimental investigation of high-speed hard turning by PCBN tooling with strengthened edge. Int. J. Adv. Manuf. Technol. 2017, 92, 3785–3793. [Google Scholar] [CrossRef]
- Arnold, D.C.M.; de Oliveira, V.C.; Kazmierczak, C.S.; Tonietto, L.; Menegotto, C.W.; Gonzaga, L.; da Costa, C.A.; Veronez, M.R. A critical analysis of red ceramic blocks roughness estimation by 2D and 3D methods. Remote Sens. 2021, 13, 789. [Google Scholar] [CrossRef]
- Zhao, T.; Zhou, J.M.; Bushlya, V.; Stahl, J.E. Effect of cutting edge radius on surface roughness and tool wear in hard turning of AISI 52100 steel. Int. J. Adv. Manuf. Technol. 2017, 91, 3611–3618. [Google Scholar] [CrossRef]
- Gao, H.; Ma, B.; Singh, R.P.; Yang, H. Areal surface roughness of AZ31B magnesium alloy processed by dry face turning: An experimental framework combined with regression analysis. Materials 2020, 13, 2303. [Google Scholar] [CrossRef]
- Grzesik, W.; Zak, K.; Kiszka, P. Comparison of Surface textures generated in hard turning and grinding operations. Procedia CIRP 2014, 13, 84–89. [Google Scholar] [CrossRef]
- Lu, X.; Hu, X.; Jia, Z.; Liu, M.; Gao, S.; Qu, C.; Liang, S.Y. Model for the prediction of 3D surface topography and surface roughness in micro-milling Inconel 718. Int. J. Adv. Manuf. Technol. 2017, 94, 2043–2056. [Google Scholar] [CrossRef]
- Charles, A.; Elkaseer, A.; Thijs, L.; Hagenmeyer, V.; Scholz, S. Effect of process parameters on the generated surface rough-ness of down-facing surfaces in selective laser melting. Appl. Sci. 2019, 9, 1256. [Google Scholar] [CrossRef]
- Sanaei, N.; Fatemi, A. Analysis of the effect of surface roughness on fatigue performance of powder bed fusion additive manufactured metals. Theor. Appl. Fract. Mech. 2020, 108, 102638. [Google Scholar] [CrossRef]
- Kumar, R.; Seetharamu, S.; Kamaraj, M. Quantitative evaluation of 3D surface roughness parameters during cavitation exposure of 16Cr–5Ni hydro turbine steel. Wear 2014, 320, 16–24. [Google Scholar] [CrossRef]
- Mesicek, J.; Ma, Q.P.; Hajnys, J.; Zelinka, J.; Pagac, M.; Petru, J.; Mizera, O. Abrasive surface finishing on SLM 316L parts fabricated with recycled powder. Appl. Sci. 2021, 11, 2869. [Google Scholar] [CrossRef]
- Kundrak, J.; Nagy, A.; Markopoulos, A.P.; Karkalos, N.E. Investigation of surface roughness on face milled parts with round insert in planes parallel to the feed at various cutting speeds. Cut. Tools Technol. Syst. 2019, 91, 87–96. [Google Scholar] [CrossRef]
- Wrobel, N.; Rejek, M.; Krolczyk, J.; Franka, M.; Gupta, M.K.; Sliwinski, M.; Krolczyk, G. Parametric assessment of surface topography and its influence on joint tightness of non-separable joints for thin wall applications. Appl. Sci. 2022, 12, 8917. [Google Scholar] [CrossRef]
- Yong, Q.; Chang, J.; Liu, Q.; Jiang, F.; Wei, D.; Li, H. Matt Polyurethane coating: Correlation of surface roughness on measurement length and gloss. Polymers 2020, 12, 326. [Google Scholar] [CrossRef]
- Shi, R.; Wang, B.; Yan, Z.; Wang, Z.; Dong, L. Effect of surface topography parameters on friction and wear of random rough surface. Materials 2019, 12, 2762. [Google Scholar] [CrossRef]
- Zhu, L.; Guan, Y.; Wang, Y.; Xie, Z.; Lin, J.; Zhai, J. Influence of process parameters of ultrasonic shot peening on surface roughness and hydrophilicity of pure titanium. Surf. Coat. Technol. 2017, 317, 38–53. [Google Scholar] [CrossRef]
- Liang, G.; Schmauder, S.; Lyu, M.; Schneider, Y.; Zhang, C.; Han, Y. An Investigation of the influence of initial roughness on the friction and wear behavior of ground surfaces. Materials 2018, 11, 237. [Google Scholar] [CrossRef]
- Sedlacek, M.; Gregorcic, P.; Podgornik, B. Use of the roughness parameters Ssk and Sku to control friction—A method for designing surface texturing. Tribol. Trans. 2017, 60, 260–266. [Google Scholar] [CrossRef]
- Naylor, A.; Talwalkar, S.C.; Trail, I.A.; Joyce, T.J. Evaluating the surface topography of pyrolytic carbon finger prostheses through measurement of various roughness parameters. J. Funct. Biomater. 2016, 7, 9. [Google Scholar] [CrossRef]
- Kulisz, M.; Zagórski, I.; Matuszak, J.; Klonica, M. Properties of the surface layer after trochoidal milling and brushing: Experimental study and artificial neural network simulation. Appl. Sci. 2020, 10, 75. [Google Scholar] [CrossRef]
- Kovacs, Z.; Viharos, Z.J.; Kodacsy, J. The effects of machining strategies of magnetic assisted roller burnishing on the resulted surface structure. Mater. Sci. Eng. 2018, 448, 012002. [Google Scholar] [CrossRef]
- Ba, E.C.T.; Dumont, M.R.; Martins, P.S.; Drumond, R.M.; Martins da Cruz, M.P.; Vieira, V.F. Investigation of the effects of skewness Rsk and kurtosis Rku on tribological behavior in a pin-on-disc test of surfaces machined by conventional milling and turning processes. Mater. Res. 2021, 24, e20200435. [Google Scholar] [CrossRef]
- Nagy, A. Investigation of the effect of areal roughness measurement length on face milled surface topographies. Cut. Tools Technol. Syst. 2021, 94, 60–69. [Google Scholar] [CrossRef]
- Kovalev, A.; Yazhao, Z.; Hui, C.; Meng, Y. A Concept of the effective surface profile to predict the roughness parameters of worn surface. Front. Mech. Eng. 2019, 5, 31. [Google Scholar] [CrossRef]
- Krawczyk, B.; Szablewski, P.; Mendak, M.; Gapinski, B.; Smak, K.; Legutko, S.; Wieczorowski, M.; Miko, E. Surface topography description of threads made with turning on Inconel 718 shafts. Materials 2023, 16, 80. [Google Scholar] [CrossRef]
- Peng, P.W.; Yang, J.C.; Lee, W.F.; Fang, C.Y.; Chang, C.M.; Chen, I.J.; Hsu, C.; Yang, T.S. Effects of heat treatment of selective laser melting printed Ti-6Al-4V specimens on surface texture parameters and cell attachment. Appl. Sci. 2021, 11, 2234. [Google Scholar] [CrossRef]
- Kulisz, M.; Zagórski, I.; Józwik, J.; Korpysa, J. Research, modelling and prediction of the influence of technological parameters on the selected 3D roughness parameters, as well as temperature, shape and geometry of chips in milling AZ91D alloy. Materials 2022, 15, 4277. [Google Scholar] [CrossRef]
- Karmiris-Obratanski, P.; Zagorski, K.; Cieslika, J.; Papazoglou, E.L.; Markopoulos, A.P. Surface topography of Ti 6A l4V ELI after high power EDM. Procedia Manuf. 2020, 47, 788–794. [Google Scholar] [CrossRef]
- Pawlus, P.; Reizer, R.; Wieczorowski, M. Parametric characterization of machined textured surfaces. Materials 2023, 16, 163. [Google Scholar] [CrossRef]
- Pawlus, P.; Reizer, R.; Wieczorowski, M.; Krolczyk, G. Material ratio curve as information on the state of surface topography—A review. Precis. Eng. 2020, 65, 240–258. [Google Scholar] [CrossRef]
- Johansson, S.; Nilsson, P.H.; Ohlsson, R.; Anderberg, C.; Rosen, B.G. New cylinder liner surfaces for low oil consumption. Tribol. Int. 2008, 41, 854–859. [Google Scholar] [CrossRef]
- Javidikiaa, M.; Sadeghifar, M.; Songmene, V.; Jahazi, M. Analysis and optimization of surface roughness in turning of AA6061-T6 under various environments and parameters. Procedia CIRP 2021, 101, 17–20. [Google Scholar] [CrossRef]
- Kluz, R.; Bucior, M.; Dzierwa, A.; Antosz, K.; Bochnowski, W.; Ochal, K. Effect of diamond burnishing on the properties of FSW joints of EN AW-2024 aluminum alloys. Appl. Sci. 2023, 13, 1305. [Google Scholar] [CrossRef]
- Longhai Special Steel. Available online: https://www.steelss.com/Carbon-steel/16mncr5.html (accessed on 10 January 2023).
- Balanou, M.; Karmiris-Obratanski, P.; Leszczynska-Madej, B.; Papazoglou, E.L.; Markopoulos, A.P. Investigation of surface modification of 60CrMoV18-5 steel by EDM with Cu-ZrO2 powder metallurgy green compact electrode. Machines 2021, 9, 268. [Google Scholar] [CrossRef]
- Abbas, A.T.; El Rayes, M.M.; Al-Abduljabbar, A.A.; Ragab, A.E.; Benyahia, F.; Elkaseer, A. Effects of tool edge geometry and cutting conditions on the performance indicators in dry turning AISI 1045 steel. Machines 2023, 11, 397. [Google Scholar] [CrossRef]





| Tensile Strength | Yield Strength | Elongation | Thermal Conductivity | Specific Heat | Melting Temperature |
|---|---|---|---|---|---|
| 1158 MPa | 1034 MPa | 15% | 16 N/mK | 500 J/kgK | 1370–1400 °C |
| C | Si | Mn | Cr | S | P |
|---|---|---|---|---|---|
| 0.14–0.19 | <0.40 | 1.00–1.30 | 0.80–1.10 | <0.035 | <0.025 |
| Cutting Speed, vc (m/min) | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| 120 | 180 | 240 | |||||||||
| Feed Rate, f (m/rev) | |||||||||||
| Insert | 0.04 | 0.12 | 0.20 | 0.04 | 0.12 | 0.20 | 0.04 | 0.12 | 0.20 | ||
| Depth-of-cut, ap (mm) | 0.05 | Standard | S1 | S2 | S3 | S4 | S5 | S6 | S7 | S8 | S9 |
| 0.20 | S10 | S11 | S12 | S13 | S14 | S15 | S16 | S17 | S18 | ||
| 0.35 | S19 | S20 | S21 | S22 | S23 | S24 | S25 | S26 | S27 | ||
| 0.05 | Wiper | S28 | S29 | S30 | S31 | S32 | S33 | S34 | S35 | S36 | |
| 0.20 | S37 | S38 | S39 | S40 | S41 | S42 | S43 | S44 | S45 | ||
| 0.35 | S46 | S47 | S48 | S49 | S50 | S51 | S52 | S53 | S54 | ||
| Cutting Data | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| ap (mm) | vc (m/min) | f (mm/rev) | ||||||||
| 0.05 | 0.2 | 0.35 | 120 | 180 | 240 | 0.04 | 0.12 | 0.2 | ||
| Standard insert | Ssk | NR | R | R | NR | NR | NR | NR | NR | R |
| Sku | NR | R | R | NR | R | NR | NR | NR | NR | |
| Sz | R | R | R | NR | NR | NR | NR | R | R | |
| Sa | R | R | R | NR | NR | NR | NR | R | R | |
| Sk | R | R | R | NR | R | R | NR | NR | R | |
| Spk | NR | NR | NR | NR | NR | NR | NR | NR | NR | |
| Svk | NR | NR | NR | NR | NR | NR | NR | NR | NR | |
| Wiper insert | Ssk | NR | NR | NR | NR | NR | NR | NR | NR | NR |
| Sku | NR | NR | NR | NR | NR | NR | NR | NR | NR | |
| Sz | NR | NR | R | NR | NR | R | R | NR | NR | |
| Sa | NR | NR | NR | NR | NR | NR | R | NR | NR | |
| Sk | NR | NR | NR | NR | NR | NR | R | NR | NR | |
| Spk | R | R | R | R | R | R | R | NR | NR | |
| Svk | R | NR | NR | NR | NR | NR | R | NR | NR | |
| Insert | Cutting Parameter | Topography Parameter | |||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Ssk | Sku | Sz | Sa | Sk | Spk | Svk | |||||||||
| F | p | F | p | F | p | F | p | F | p | F | p | F | p | ||
| Standard | ap | 0.43 | 0.658 | 2.17 | 0.137 | 0.20 | 0.820 | 0.25 | 0.784 | 0.76 | 0.477 | 0.05 | 0.948 | 0.10 | 0.903 |
| vc | 0.02 | 0.976 | 0.20 | 0.823 | 0.00 | 0.996 | 0.00 | 0.997 | 0.00 | 1.000 | 0.00 | 0.997 | 2.67 | 0.090 | |
| f | 36.49 | 0.000 | 26.04 | 0.000 | 404.5 | 0.000 | 371.5 | 0.000 | 101.3 | 0.000 | 547.1 | 0.000 | 6.87 | 0.004 | |
| Wiper | ap | 0.55 | 0.584 | 0.17 | 0.846 | 0.87 | 0.434 | 0.84 | 0.442 | 0.52 | 0.601 | 0.96 | 0.398 | 1.67 | 0.208 |
| vc | 0.03 | 0.972 | 0.01 | 0.985 | 0.11 | 0.900 | 0.11 | 0.896 | 0.08 | 0.921 | 0.08 | 0.924 | 0.6 | 0.556 | |
| f | 107.2 | 0.000 | 10.31 | 0.001 | 68.37 | 0.000 | 68.62 | 0.000 | 75.6 | 0.000 | 70.3 | 0.000 | 3.73 | 0.039 | |
| Insert | Topography Parameter | ||||||
|---|---|---|---|---|---|---|---|
| Ssk | Sku | Sa | Sz | Sk | Spk | Svk | |
| Standard | 83.1% | 85.9% | 99.6% | 99.6% | 98.3% | 98.7% | 69.3% |
| Wiper | 95.3% | 71.9% | 94.1% | 95.2% | 92.3% | 97.1% | 54.7% |
| Insert | Topography Parameter | Cutting Parameters | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| ap | vc | f | ap2 | vc2 | f2 | apvc | apf | vcf | ||
| Standard | Ssk | 1.5% | 0.2% | 41.3% | 0.8% | 0.2% | 27.1% | 1.2% | 5.7% | 33.1% |
| Sku | 9.7% | 0.2% | 63.3% | 6.5% | 0.1% | 54.5% | 7.4% | 47.1% | 44.6% | |
| Sa | 1.4% | 0.0% | 93.8% | 1.0% | 0.0% | 96.9% | 1.0% | 50.1% | 69.5% | |
| Sz | 1.2% | 0.0% | 93.6% | 0.9% | 0.0% | 97.1% | 1.0% | 49.8% | 70.6% | |
| Sk | 3.6% | 0.0% | 82.2% | 2.3% | 0.0% | 88.6% | 2.7% | 56.8% | 61.7% | |
| Spk | 0.1% | 0.0% | 97.5% | 0.0% | 0.0% | 96.2% | 0.1% | 32.1% | 72.7% | |
| Svk | 0.1% | 11.9% | 21.8% | 0.2% | 10.2% | 28.2% | 1.9% | 15.8% | 38.3% | |
| Wiper | Ssk | 2.4% | 0.2% | 89.9% | 1.5% | 0.2% | 86.7% | 1.9% | 50.2% | 65.1% |
| Sku | 1.1% | 0.0% | 2.1% | 1.3% | 0.0% | 7.1% | 0.8% | 11.6% | 2.2% | |
| Sa | 2.3% | 0.5% | 79.7% | 1.1% | 0.4% | 84.8% | 0.9% | 48.4% | 54.6% | |
| Sz | 3.6% | 0.3% | 78.0% | 2.2% | 0.2% | 84.2% | 1.9% | 53.5% | 56.5% | |
| Sk | 1.6% | 0.4% | 82.7% | 0.8% | 0.3% | 86.3% | 0.5% | 45.9% | 57.0% | |
| Spk | 4.4% | 0.5% | 75.0% | 2.8% | 0.5% | 83.1% | 2.3% | 55.8% | 50.7% | |
| Svk | 11.4% | 0.1% | 20.9% | 9.7% | 0.2% | 23.1% | 8.1% | 33.3% | 23.4% | |
| Topography Parameter | ||||||
|---|---|---|---|---|---|---|
| Insert | Sk | Spk | Svk | Spk/Sk | Svk/Sk | |
| Standard | Ssk | 0.34 | 0.62 | 0.05 | 0.89 | −0.73 |
| Sku | −0.74 | −0.76 | −0.13 | −0.19 | 0.94 | |
| Wiper | Ssk | 0.88 | 0.89 | 0.49 | 0.92 | −0.82 |
| Sku | 0.21 | 0.42 | 0.49 | 0.53 | 0.28 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Molnar, V. Influence of Cutting Parameters and Tool Geometry on Topography of Hard Turned Surfaces. Machines 2023, 11, 665. https://doi.org/10.3390/machines11060665
Molnar V. Influence of Cutting Parameters and Tool Geometry on Topography of Hard Turned Surfaces. Machines. 2023; 11(6):665. https://doi.org/10.3390/machines11060665
Chicago/Turabian StyleMolnar, Viktor. 2023. "Influence of Cutting Parameters and Tool Geometry on Topography of Hard Turned Surfaces" Machines 11, no. 6: 665. https://doi.org/10.3390/machines11060665
APA StyleMolnar, V. (2023). Influence of Cutting Parameters and Tool Geometry on Topography of Hard Turned Surfaces. Machines, 11(6), 665. https://doi.org/10.3390/machines11060665






