Lifetime Extension Method for Three-Phase Voltage Source Converters Using Discontinuous PWM Scheme with Hybrid Offset Voltage
Abstract
1. Introduction
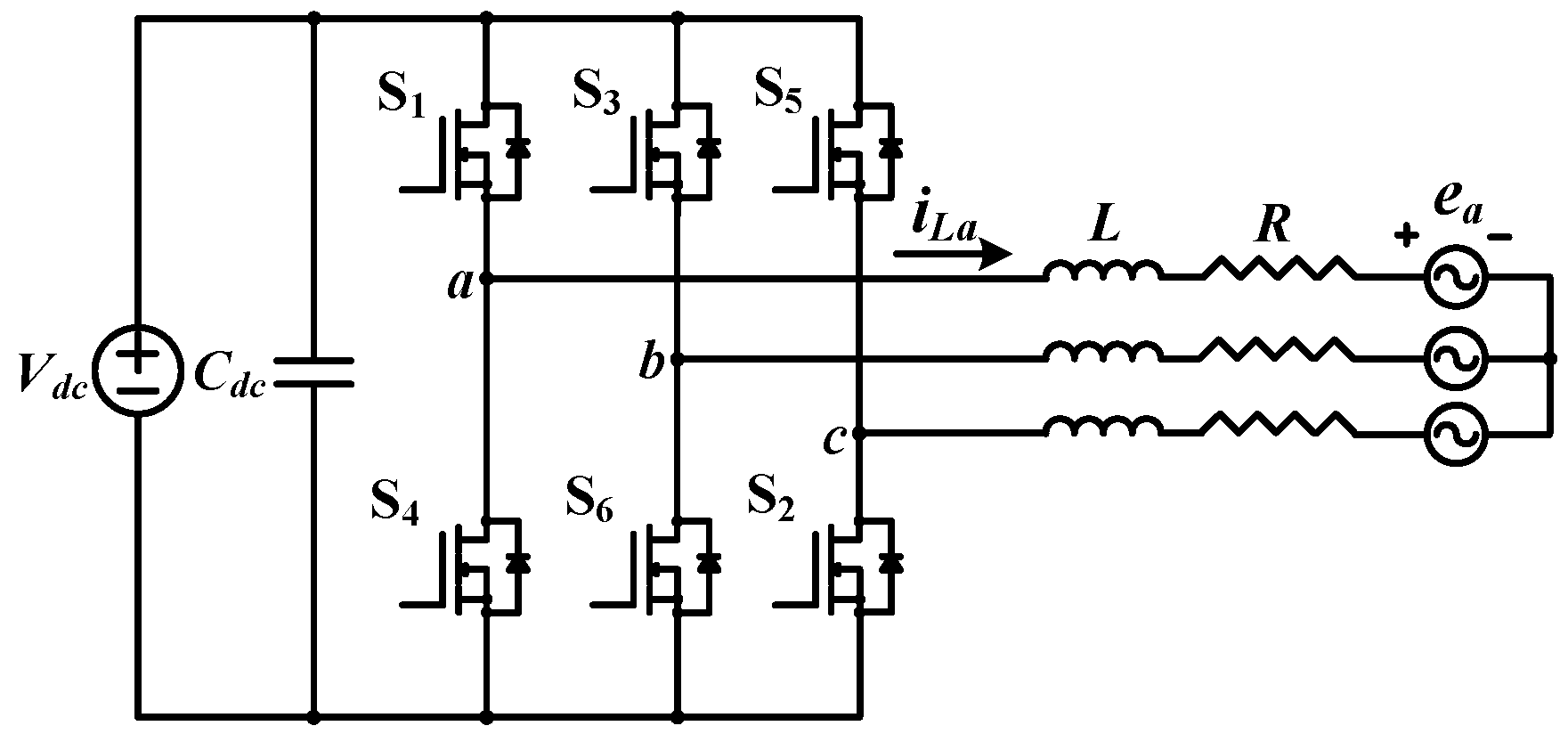
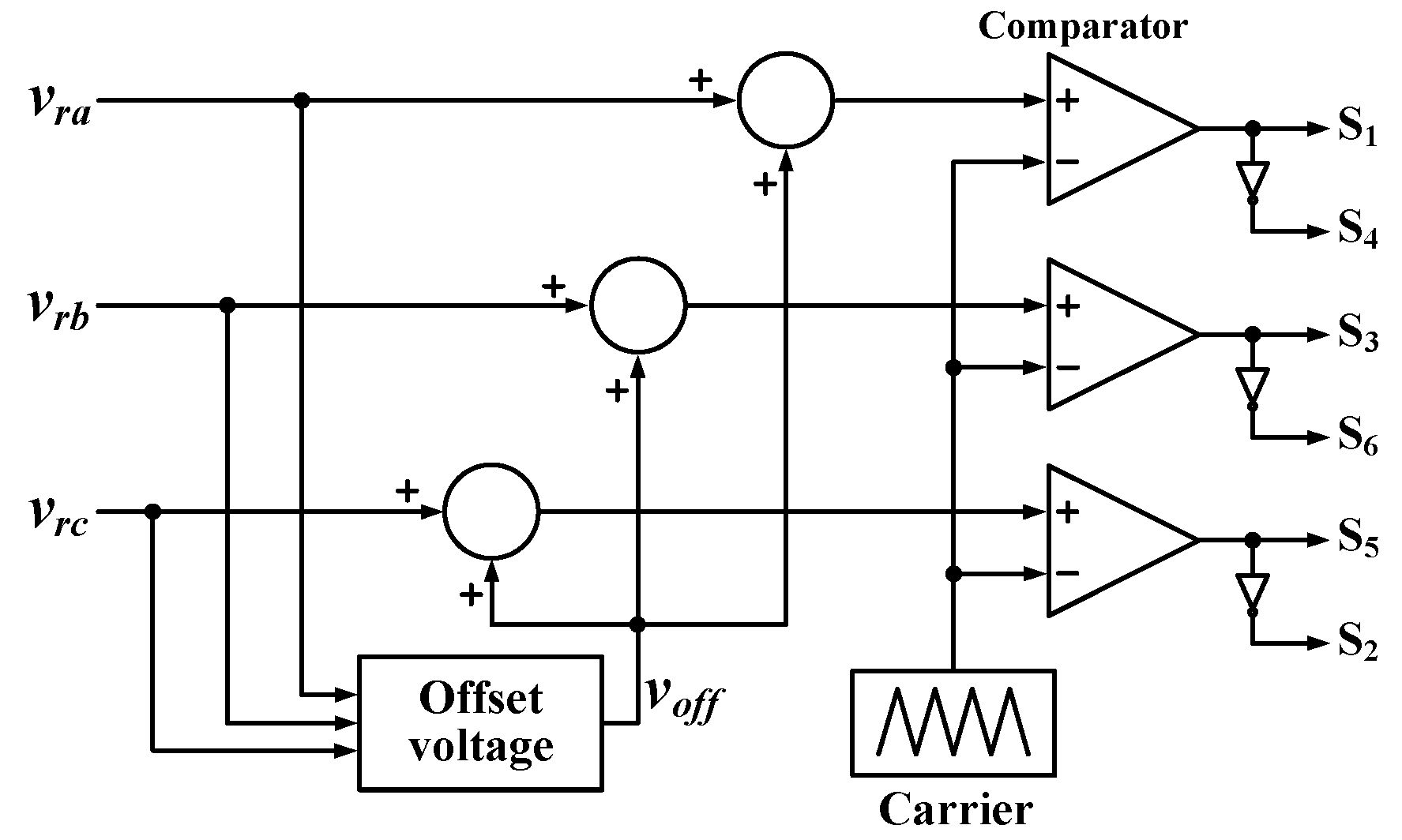
2. Carrier-Based PWM
2.1. Space Vector PWM
2.2. Generalized Discontinuous PWM
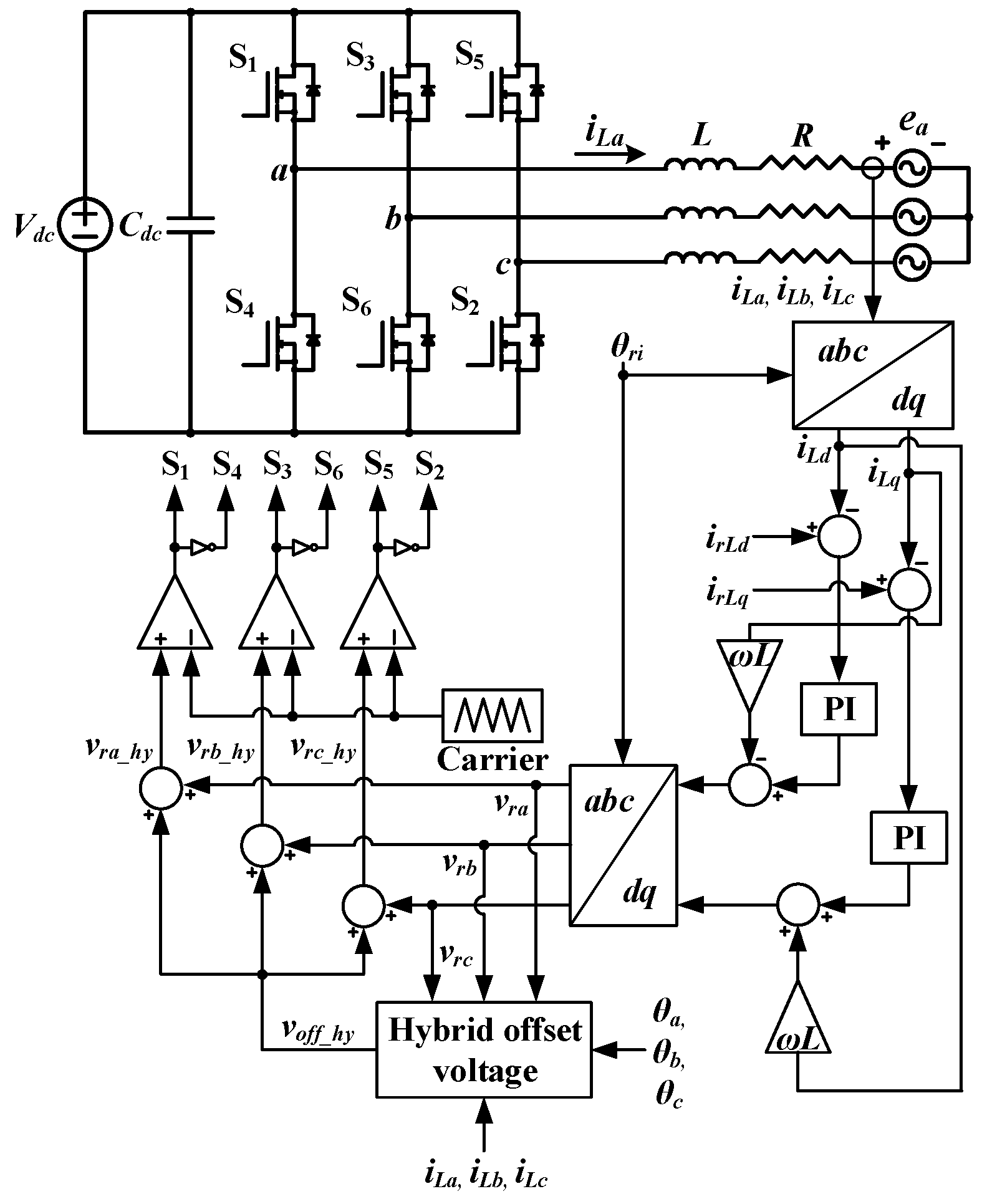
3. Proposed Inverter Lifetime Extension Method Using Hybrid Offset Voltage
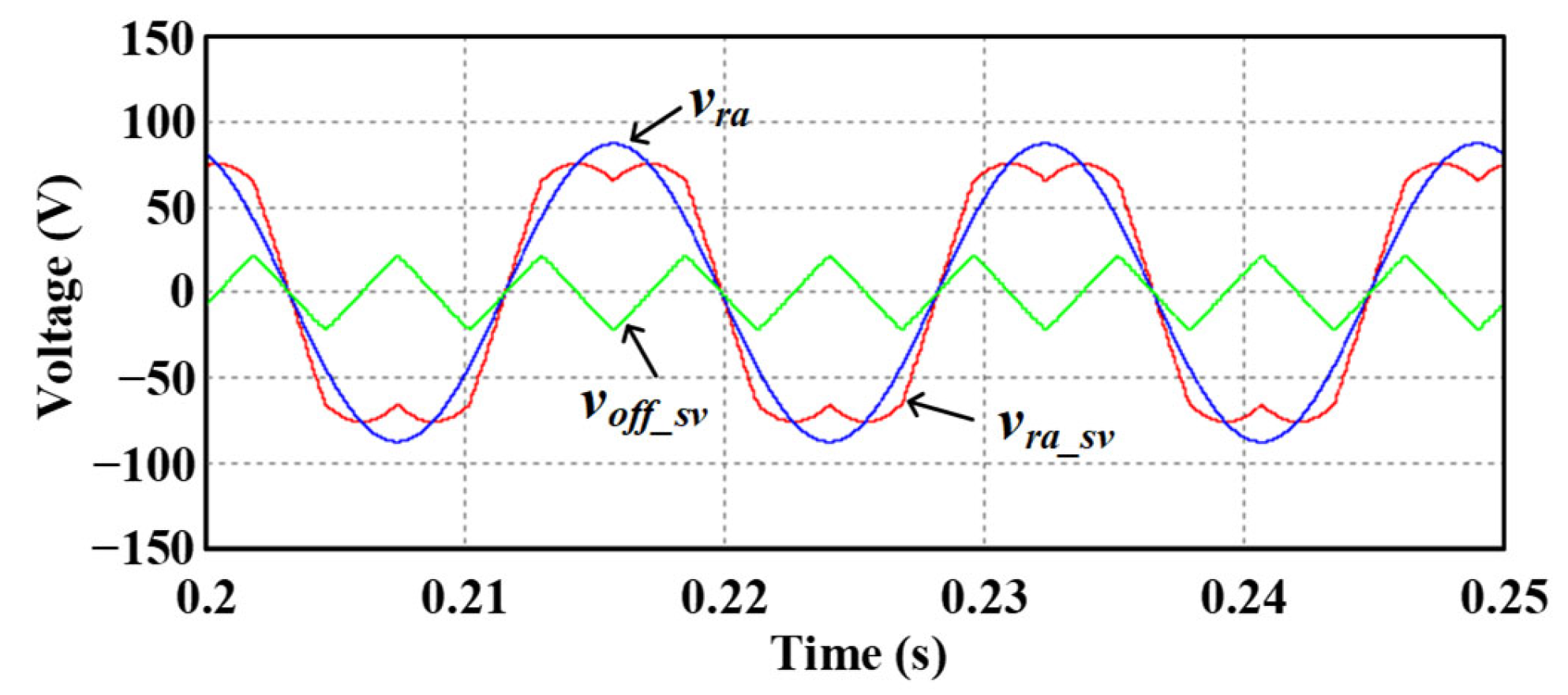
3.1. Hybrid Offset Voltage
3.2. Determination of Clamping Region
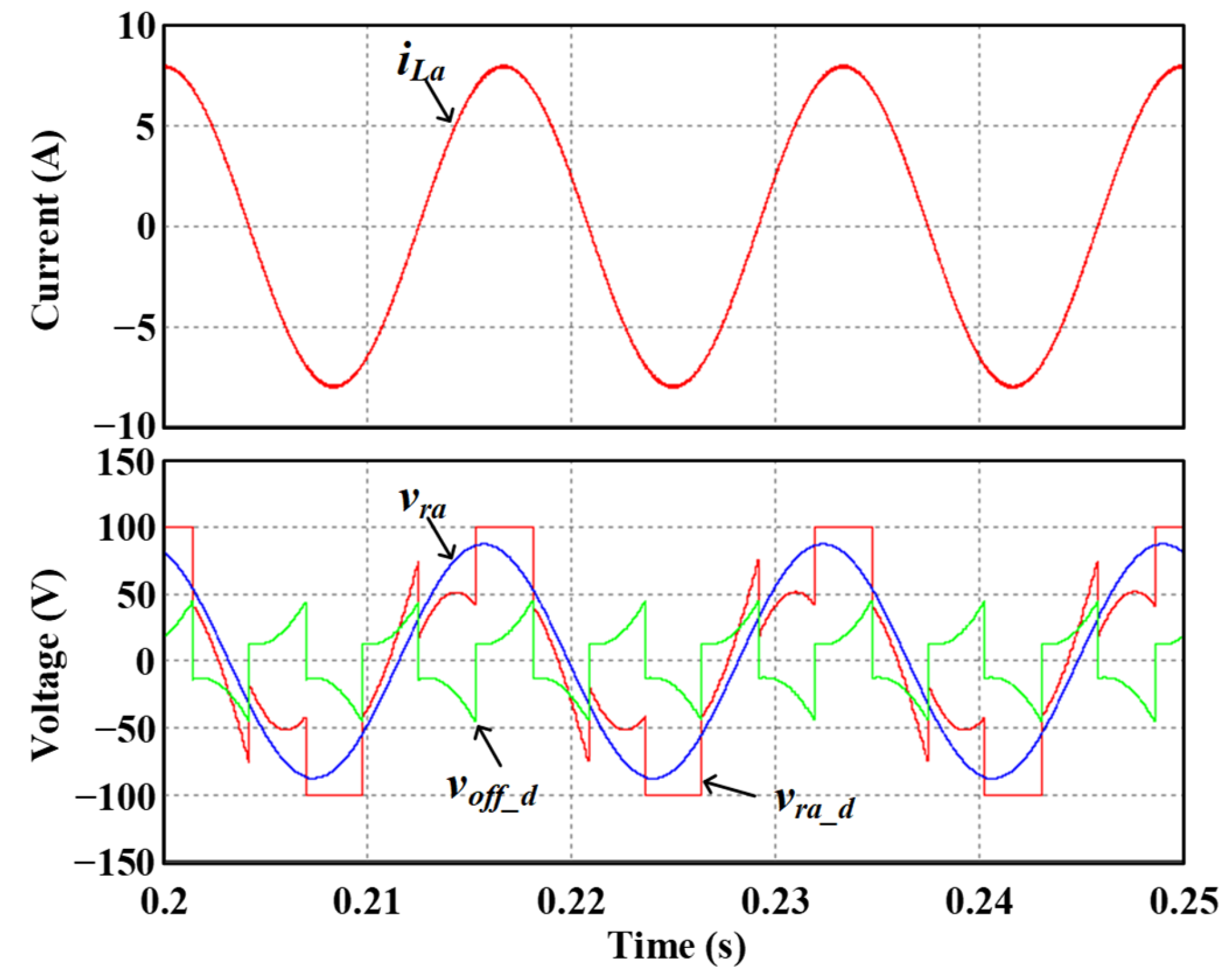
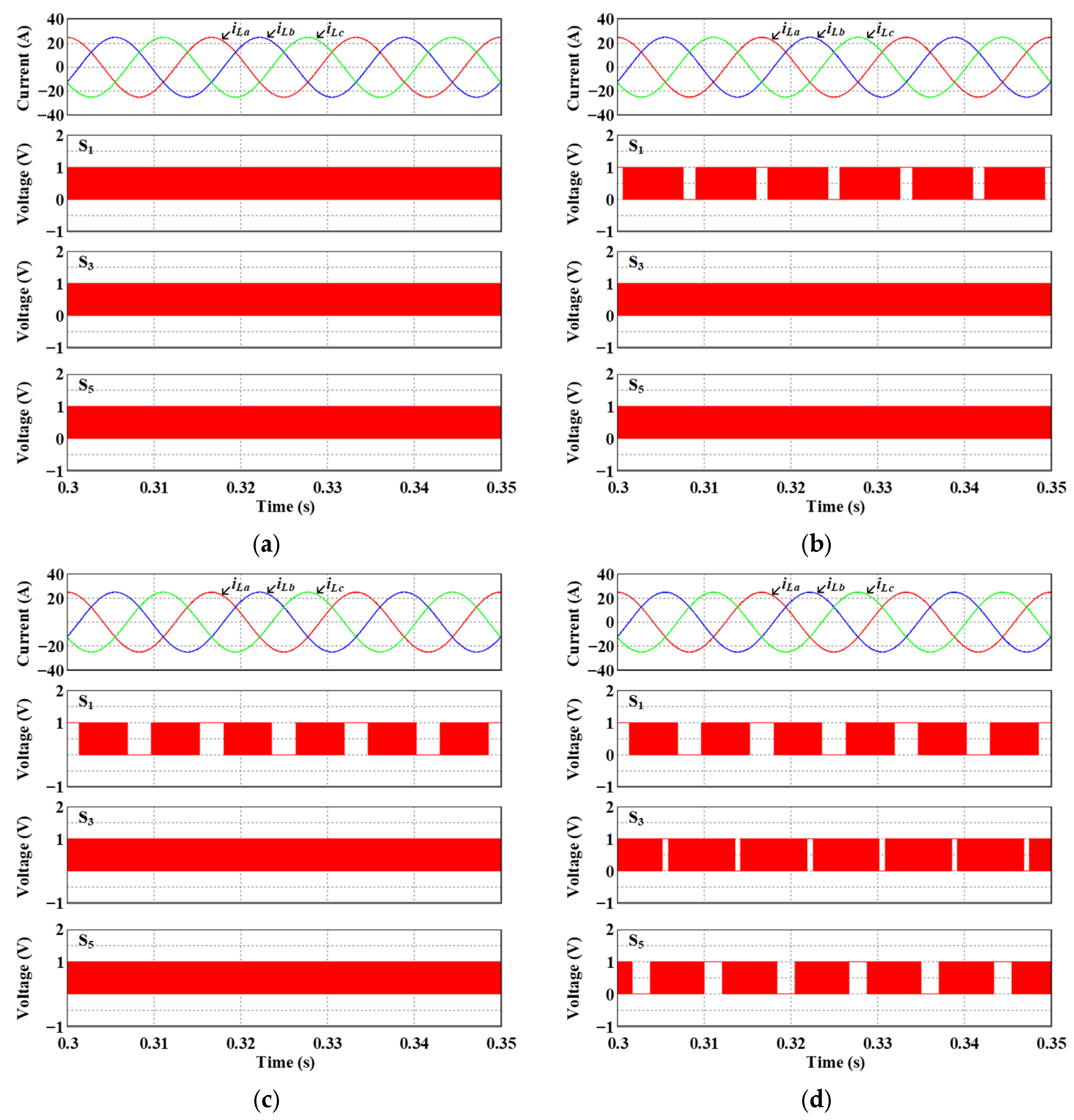
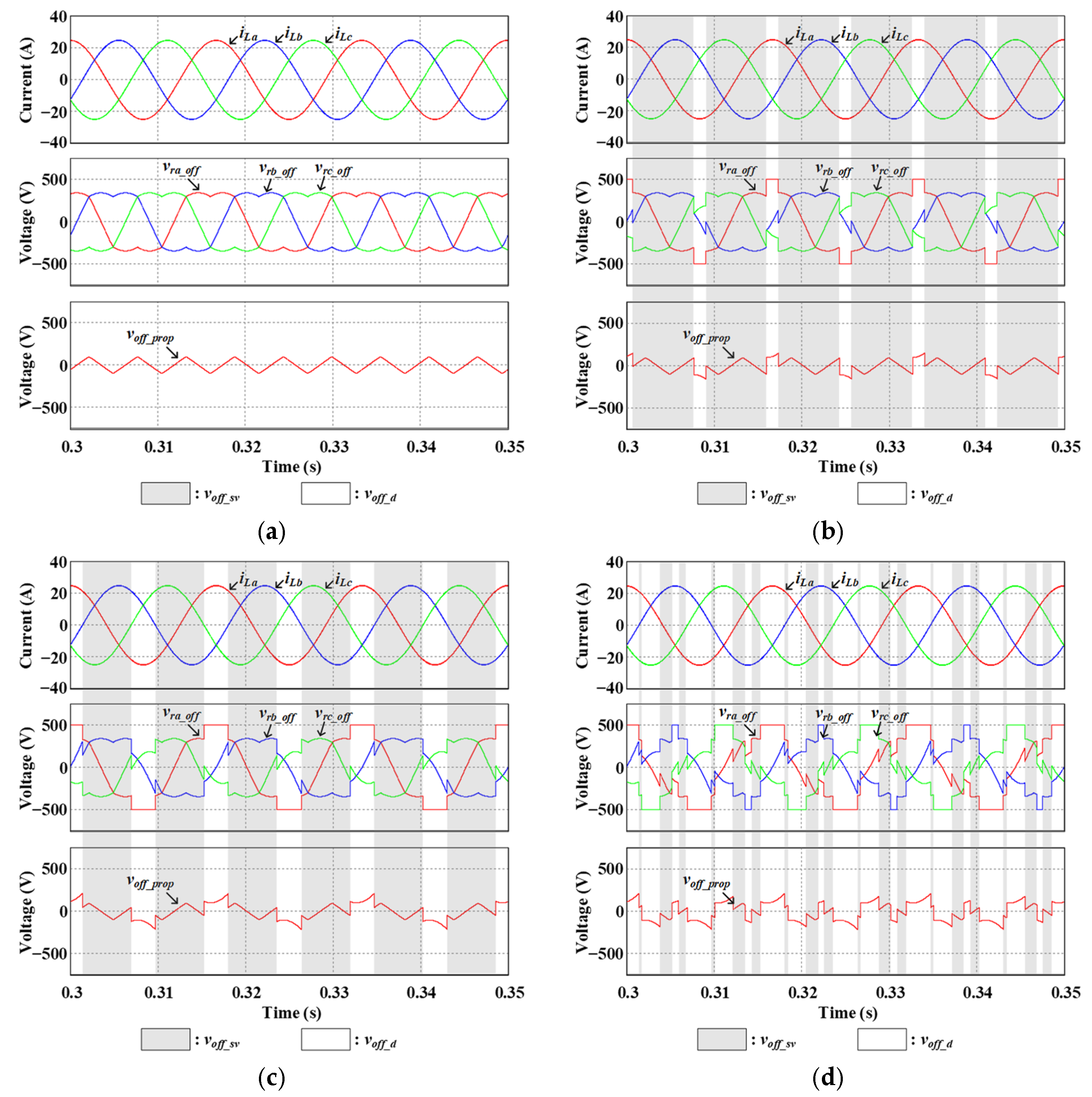
4. Simulation Results
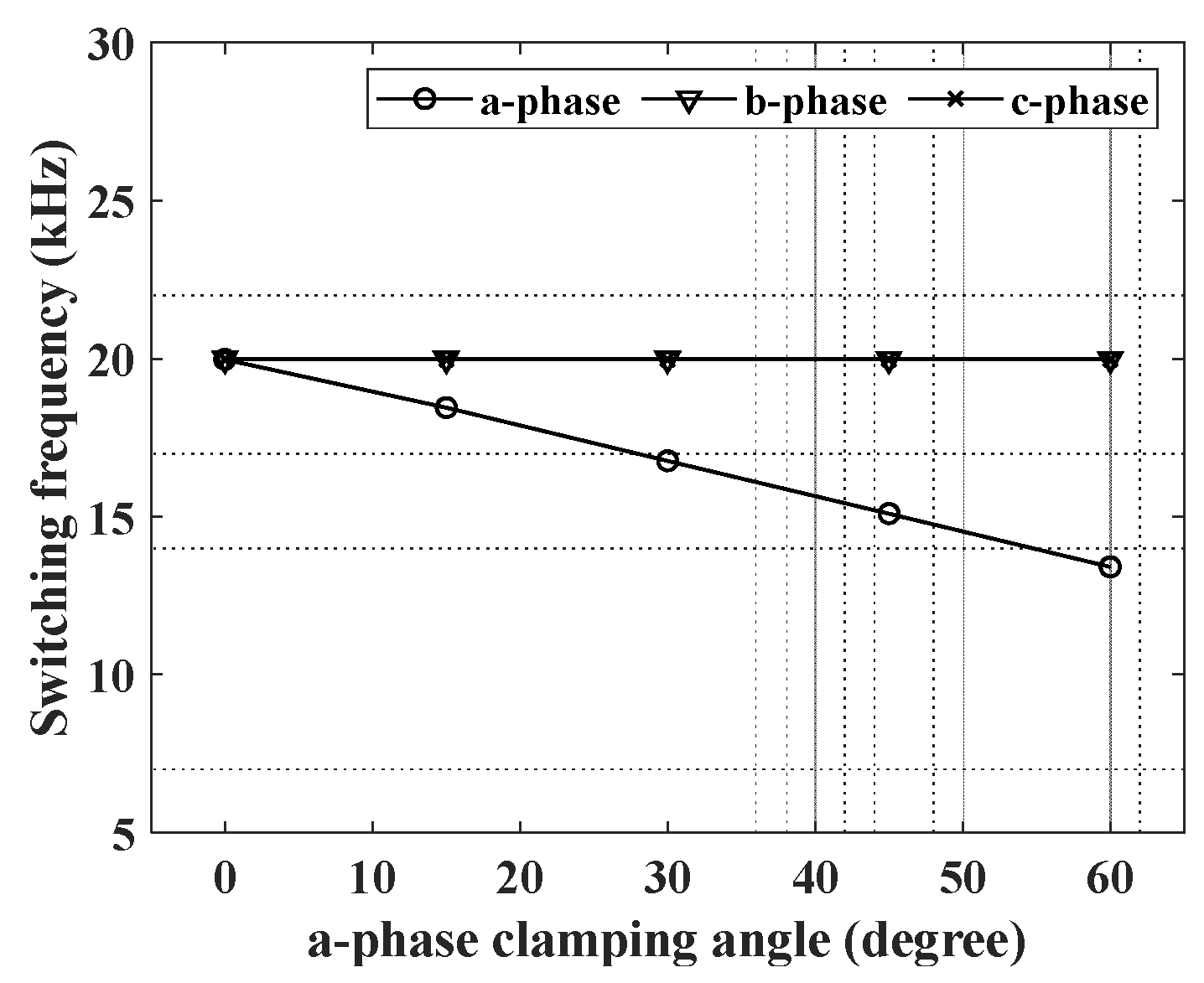
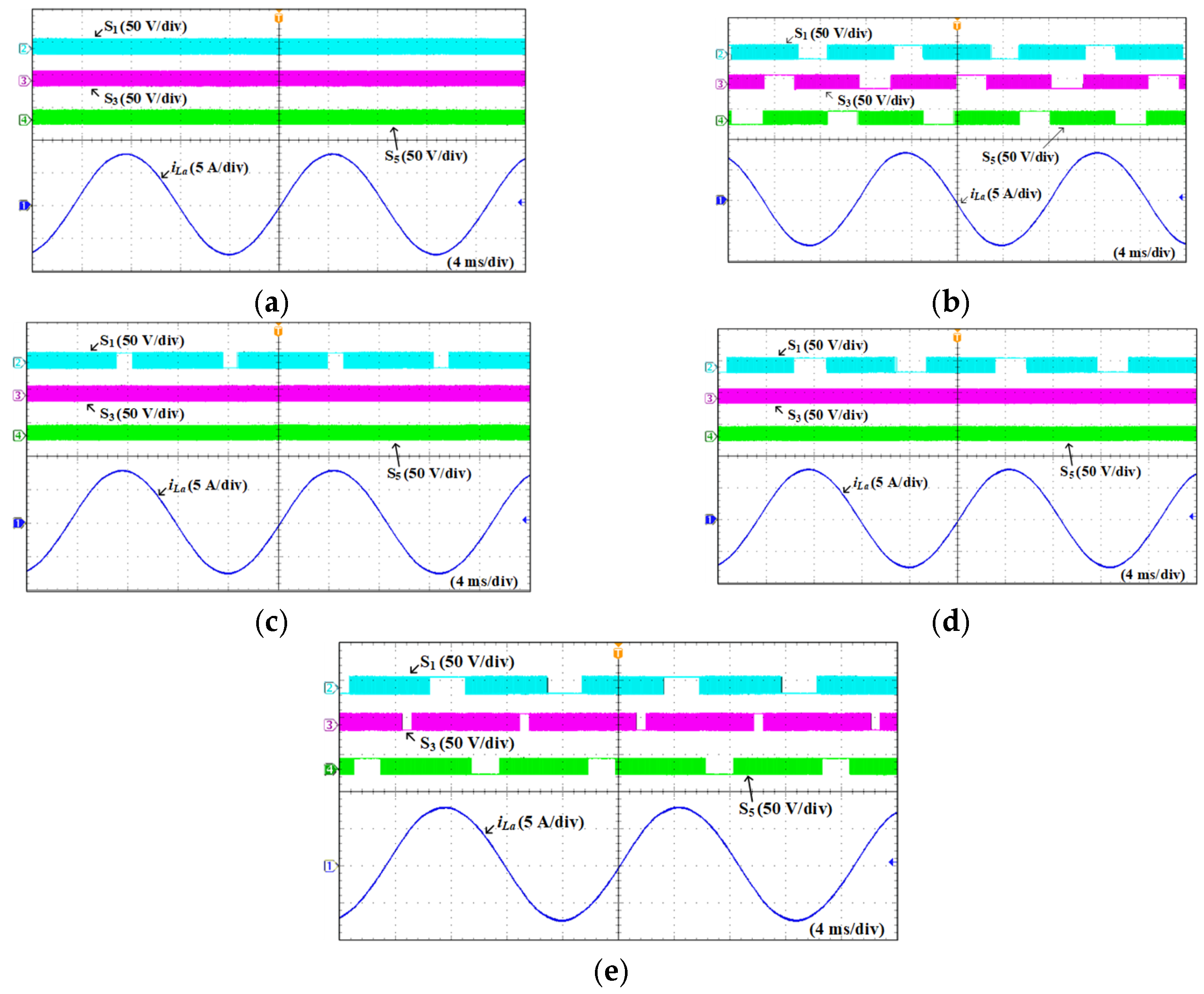
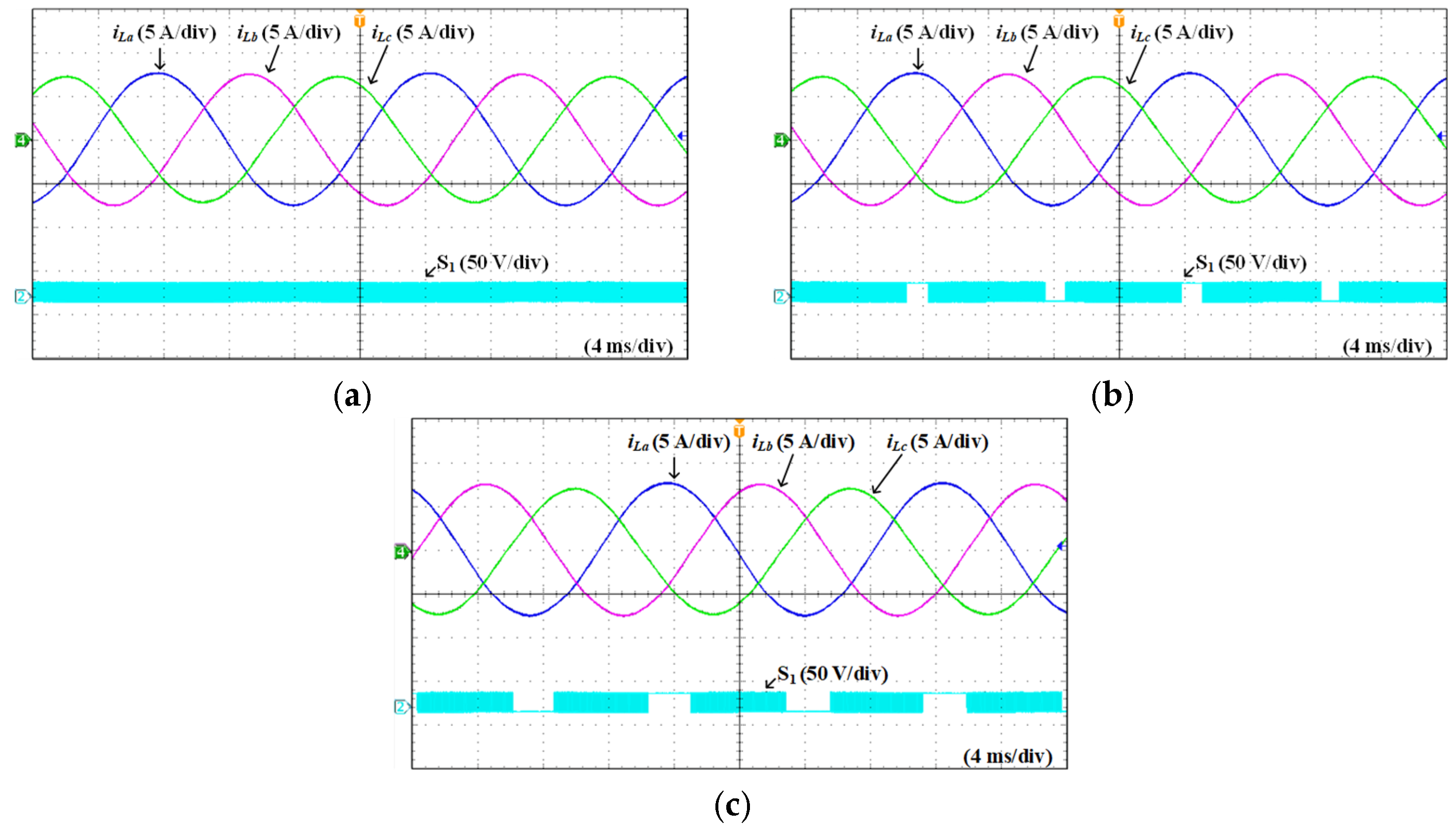
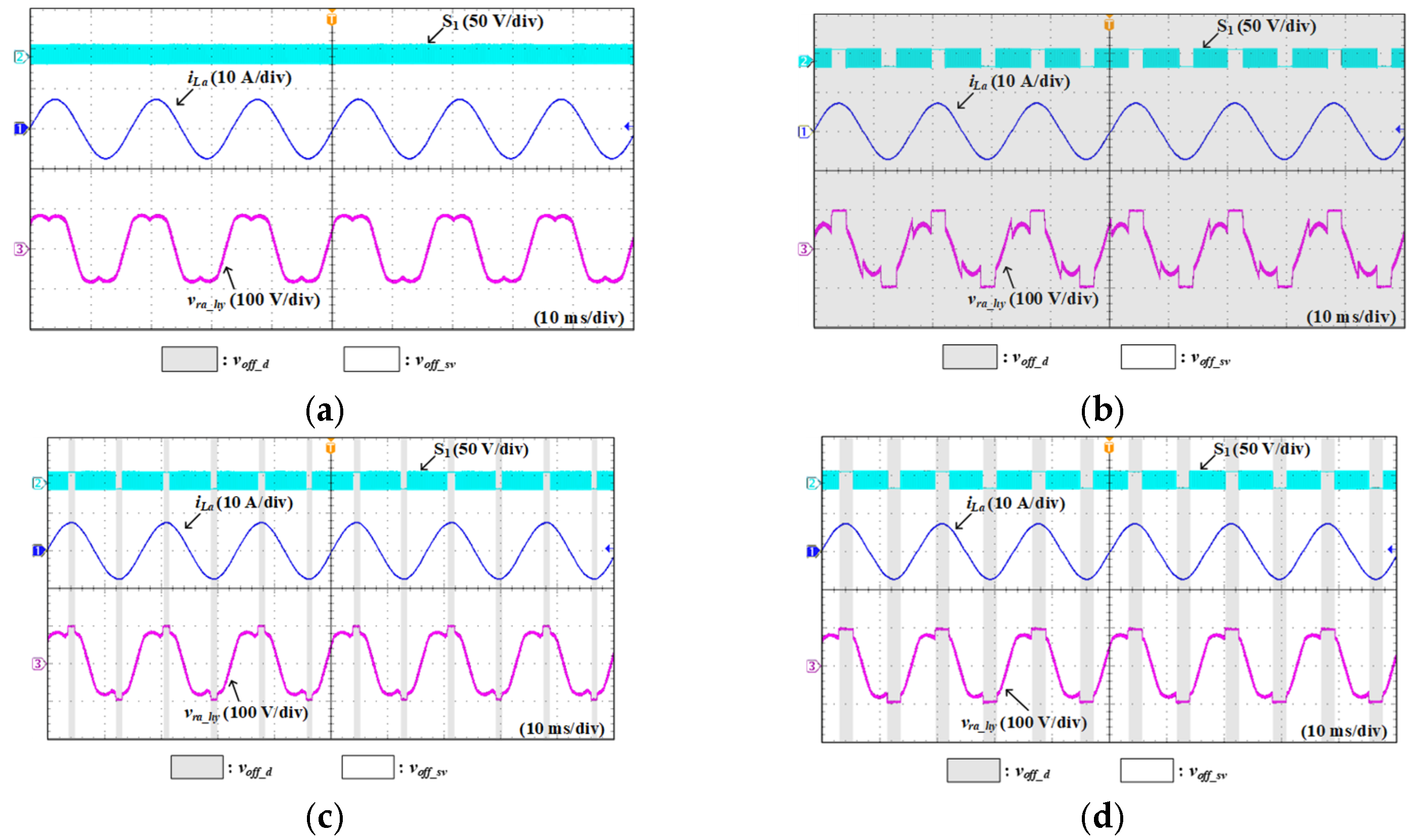
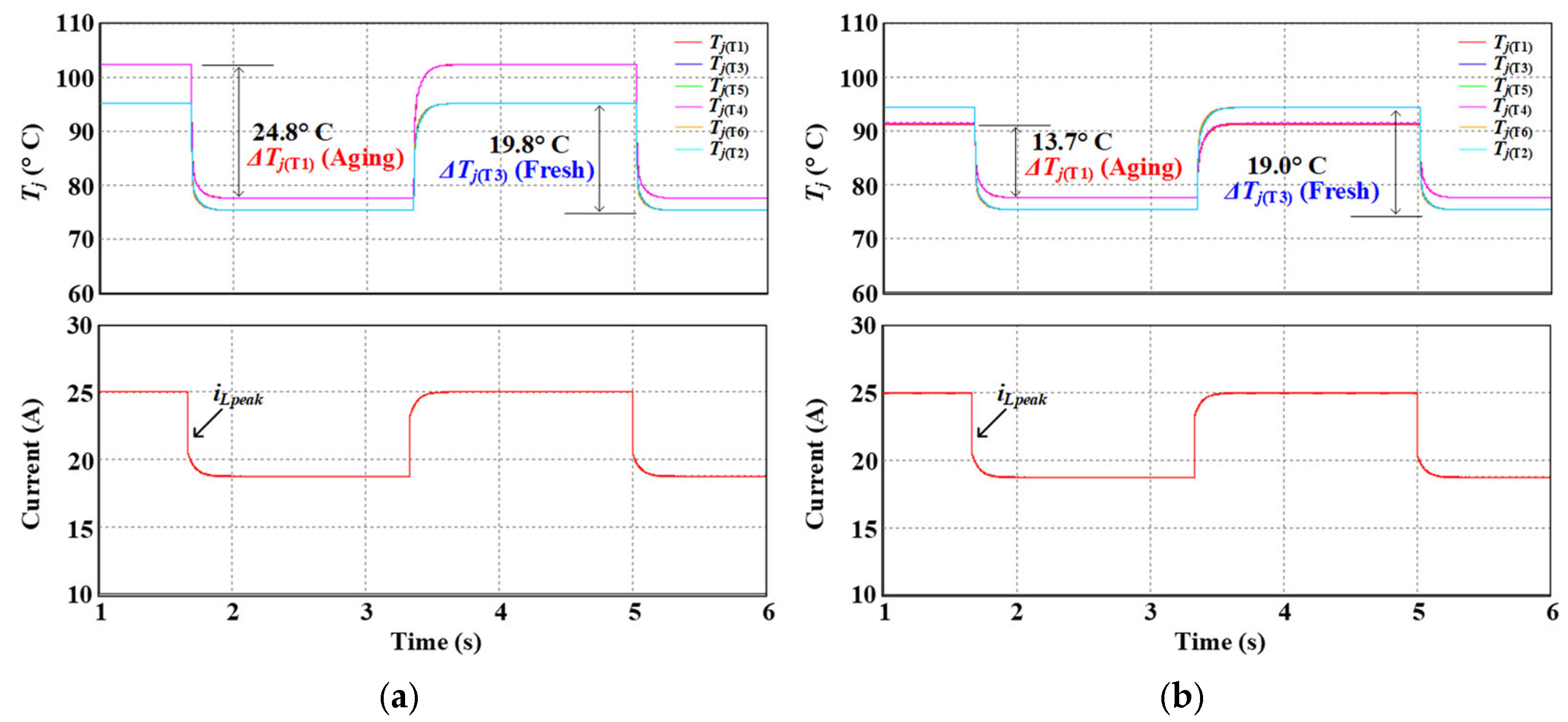
5. Experimental Results
6. Analysis of Lifetime Extension Effect of Proposed Technique
6.1. Foster Thermal Model
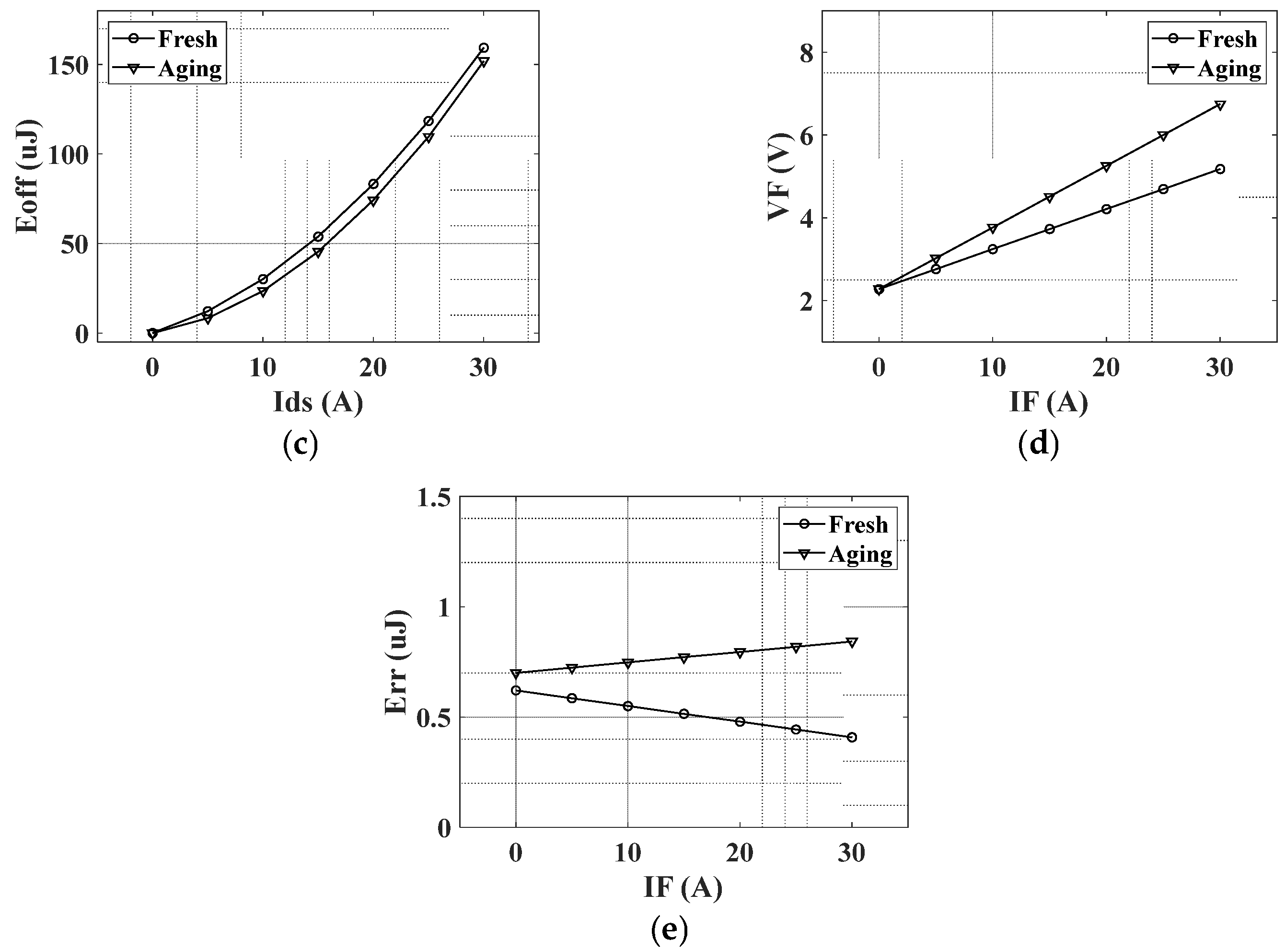
6.2. Loss of Fresh and Aging SiC Mosfet
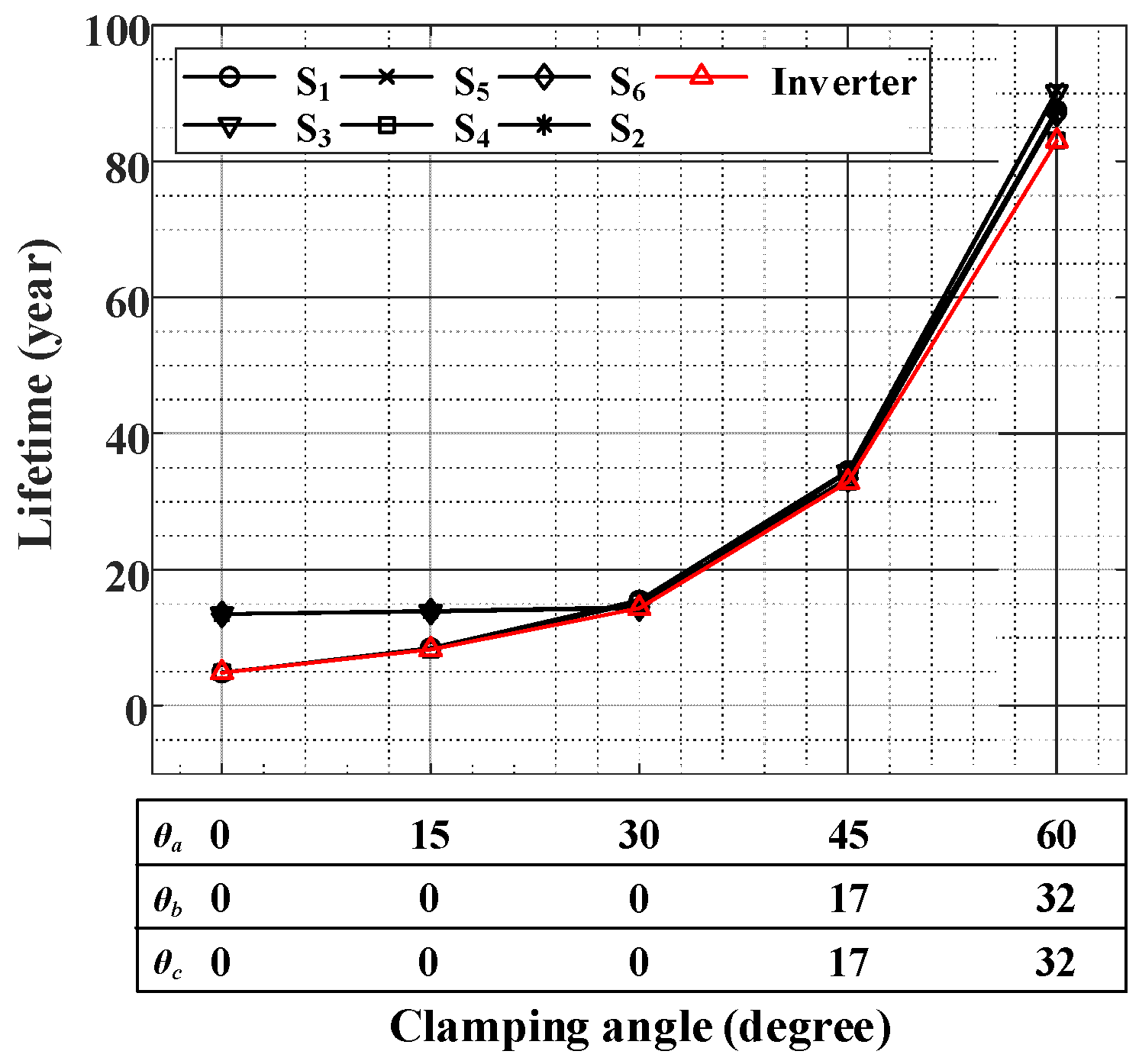
6.3. Lifetime Estimation Using Junction Temperature
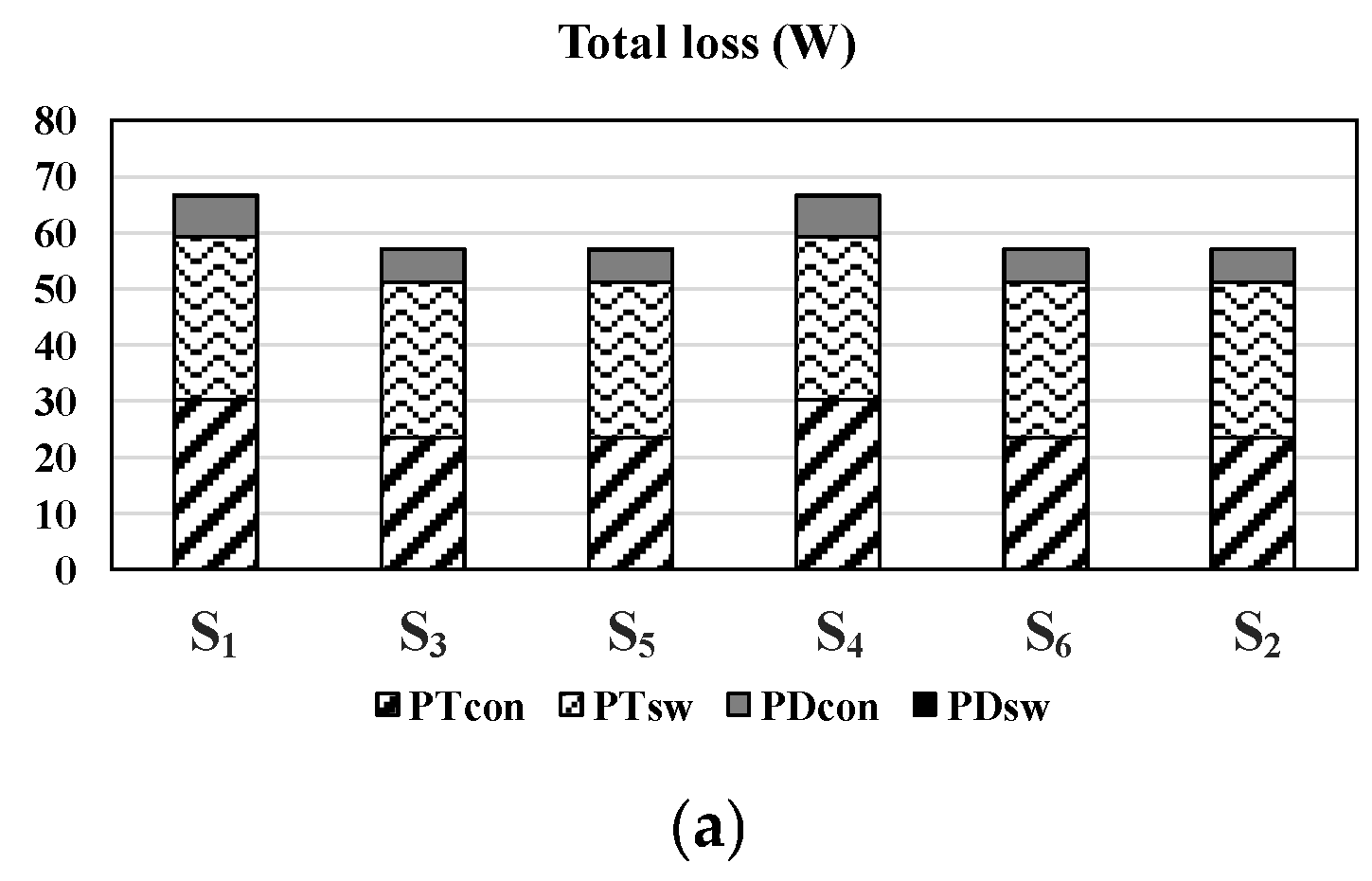
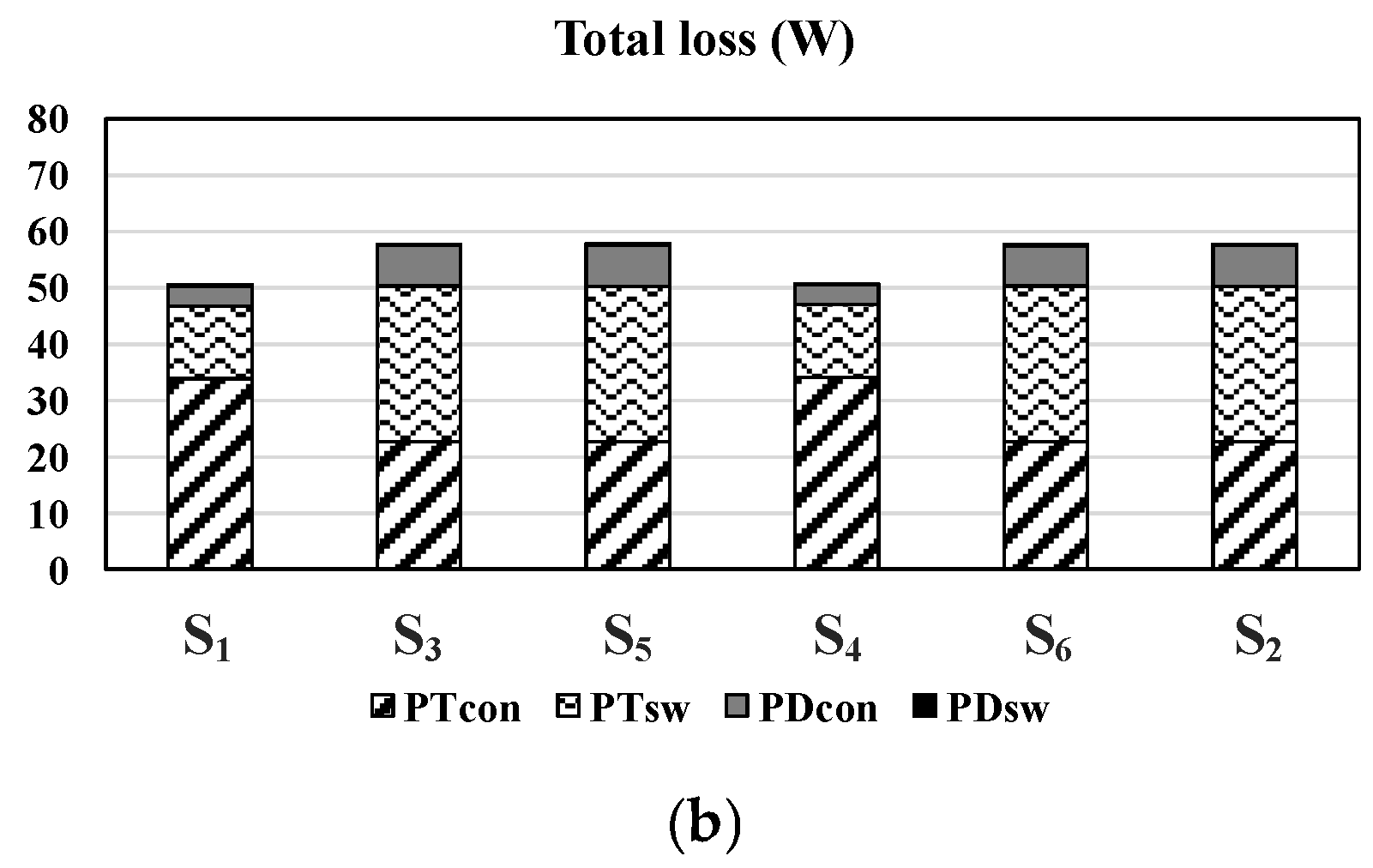
7. Comparison of Loss and Load Current Quality between Conventional and Proposed Techniques
8. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Jahns, T.M.; Sarlioglu, B. The Incredible Shrinking Motor Drive: Accelerating the Transition to Integrated Motor Drives. IEEE Power Electron. Mag. 2020, 7, 18–27. [Google Scholar] [CrossRef]
- Farhadi, M.; Yang, F.; Pu, S.; Vankayalapati, B.T.; Akin, B. Temperature-Independent Gate-Oxide Degradation Monitoring of SiC MOSFETs Based on Junction Capacitances. IEEE Trans. Power Electron. 2021, 36, 8308–8324. [Google Scholar] [CrossRef]
- Pu, S.; Yang, F.; Vankayalapati, B.T.; Akin, B. Aging Mechanisms and Accelerated Lifetime Tests for SiC MOSFETs: An Overview. IEEE J. Emerg. Sel. Top. Power Electron. 2021, 10, 1232–1254. [Google Scholar] [CrossRef]
- Ugur, E.; Xu, C.; Yang, F.; Pu, S.; Alin, B. A New Complete Condition Monitoring Method for SiC Power MOSFETs. IEEE Trans. Ind. Electron. 2020, 68, 1654–2021. [Google Scholar] [CrossRef]
- Yang, S.; Bryant, A.; Mawby, P.; Xiang, D.; Ran, L.; Tavner, P. An industry-based survey of reliability in power electronic converters. IEEE Trans. Ind. Appl. 2011, 47, 1441–1451. [Google Scholar] [CrossRef]
- Ni, Z.; Lyu, X.; Yadav, O.P.; Singh, B.N.; Zheng, S.; Cao, D. Overview of Real-Time Lifetime Prediction and Extension for SiC Power Converters. IEEE Trans. Power Electron. 2019, 35, 7765–7794. [Google Scholar] [CrossRef]
- Wei, L.; McGuire, J.; Lukaszewski, R.A. Analysis of PWM frequency control to improve the lifetime of PWM inverter. IEEE Trans. Ind. Appl. 2011, 47, 922–929. [Google Scholar]
- Lemmens, J.; Vanassche, P.; Driesen, J. Optimal Control of Traction Motor Drives Under Electrothermal Constraints. IEEE J. Emerg. Sel. Top. Power Electron. 2014, 2, 249–263. [Google Scholar] [CrossRef]
- Polom, T.A.; Wang, B.; Lorenz, R.D. Control of Junction Temperature and Its Rate of Change at Thermal Boundaries via Precise Loss Manipulation. IEEE Trans. Ind. Appl. 2017, 53, 4796–4806. [Google Scholar] [CrossRef]
- Ko, Y.; Andresen, M.; Buticchi, G.; Liserre, M. Thermally Compensated Discontinuous Modulation Strategy for Cascaded H-Bridge Converters. IEEE Trans. Power Electron. 2017, 33, 2704–2713. [Google Scholar] [CrossRef]
- Ko, Y.; Andresen, M.; Buticchi, G.; Liserre, M. Discontinuous-Modulation-Based Active Thermal Control of Power Electronic Modules in Wind Farms. IEEE Trans. Power Electron. 2019, 34, 301–310. [Google Scholar] [CrossRef]
- Ko, Y.; Kuprat, J.; Pugliese, S.; Liserre, M. Modulation Strategies for Thermal Stress Control of CHB Inverters. IEEE Trans. Power Electron. 2021, 37, 3515–3527. [Google Scholar] [CrossRef]
- Xu, J.; Soeiro, T.B.; Gao, F.; Tang, H.; Bauer, P. Carrier-Based Generalized Discontinuous PWM Strategy for Single-Phase Three-Legs Active Power Decoupling Converters. IEEE Trans. Ind. Electron. 2020, 68, 11609–11613. [Google Scholar] [CrossRef]
- Ma, K.; Liserre, M.; Blaabjerg, F. Reactive power influence on the thermal cycling of multi-MW wind power inverter. IEEE Trans. Ind. Appl. 2013, 49, 922–930. [Google Scholar] [CrossRef]
- Aly, M.; Ahmed, E.M.; Shoyama, M. Thermal Stresses Relief Carrier-Based PWM Strategy for Single-Phase Multilevel Inverters. IEEE Trans. Power Electron. 2017, 32, 9376–9388. [Google Scholar] [CrossRef]
- Kim, J.-C.; Kwak, S. Per-Phase Switching Frequency Control Method to Extend Lifespan of Three-Phase Voltage Source Inverters. IEEE Access 2022, 10, 115849–115868. [Google Scholar] [CrossRef]
- Murdock, D.; Torres, J.; Connors, J.; Lorenz, R. Active thermal control of power electronic modules. IEEE Trans. Ind. Appl. 2006, 42, 552–558. [Google Scholar] [CrossRef]
- Blasko, V. Analysis of a hybrid PWM based on modified space vector and triangle comparison methods. IEEE Trans. Ind. Appl. 1997, 33, 756–764. [Google Scholar] [CrossRef]
- Nguyen, T.D.; Hobraiche, J.; Patin, N.; Friedrich, G.; Vilain, J.-P. A direct digital technique implementation of general discontinuous pulse width modulation strategy. IEEE Trans. Ind. Electron. 2011, 58, 4445–4454. [Google Scholar] [CrossRef]
- Dusmez, S.; Duran, H.; Akin, B. Remaining Useful Lifetime Estimation for Thermally Stressed Power MOSFETs Based on on-State Resistance Variation. IEEE Trans. Ind. Appl. 2016, 52, 2554–2563. [Google Scholar] [CrossRef]
- Pu, S.; Ugur, E.; Yang, F.; Akin, B. In situ Degradation Monitoring of SiC MOSFET Based on Switching Transient Measurement. IEEE Trans. Ind. Electron. 2019, 67, 5092–5100. [Google Scholar] [CrossRef]
- Du, M.; Xin, J.; Wang, H.; Ouyang, Z. Aging Diagnosis of Bond Wire Using On-State Drain-Source Voltage Separation for SiC MOSFET. IEEE Trans. Device Mater. Reliab. 2020, 21, 41–47. [Google Scholar] [CrossRef]
- Van der Broeck, H.W.; Skudelny, H.-C.; Stanke, G.V. Analysis and realization of a pulsewidth modulator based on voltage space vectors. IEEE Trans. Ind. Appl. 1988, 24, 142–150. [Google Scholar] [CrossRef]
- Bayerer, R.; Herrmann, T.; Licht, T.; Lutz, J.; Feller, M. Model for power cycling lifetime of IGBT modules—Various factors influencing lifetime. In Proceedings of the 5th International Conference on Integrated Power Electronics Systems, Nuremberg, Germany, 11–13 March 2008; pp. 1–6. [Google Scholar]
- Lutz, J. IGBT-modules: Design for reliability. In Proceedings of the 13th European Conference on Power Electronics and Applications, Barcelona, Spain, 8–10 September 2009; pp. 1–3. [Google Scholar]


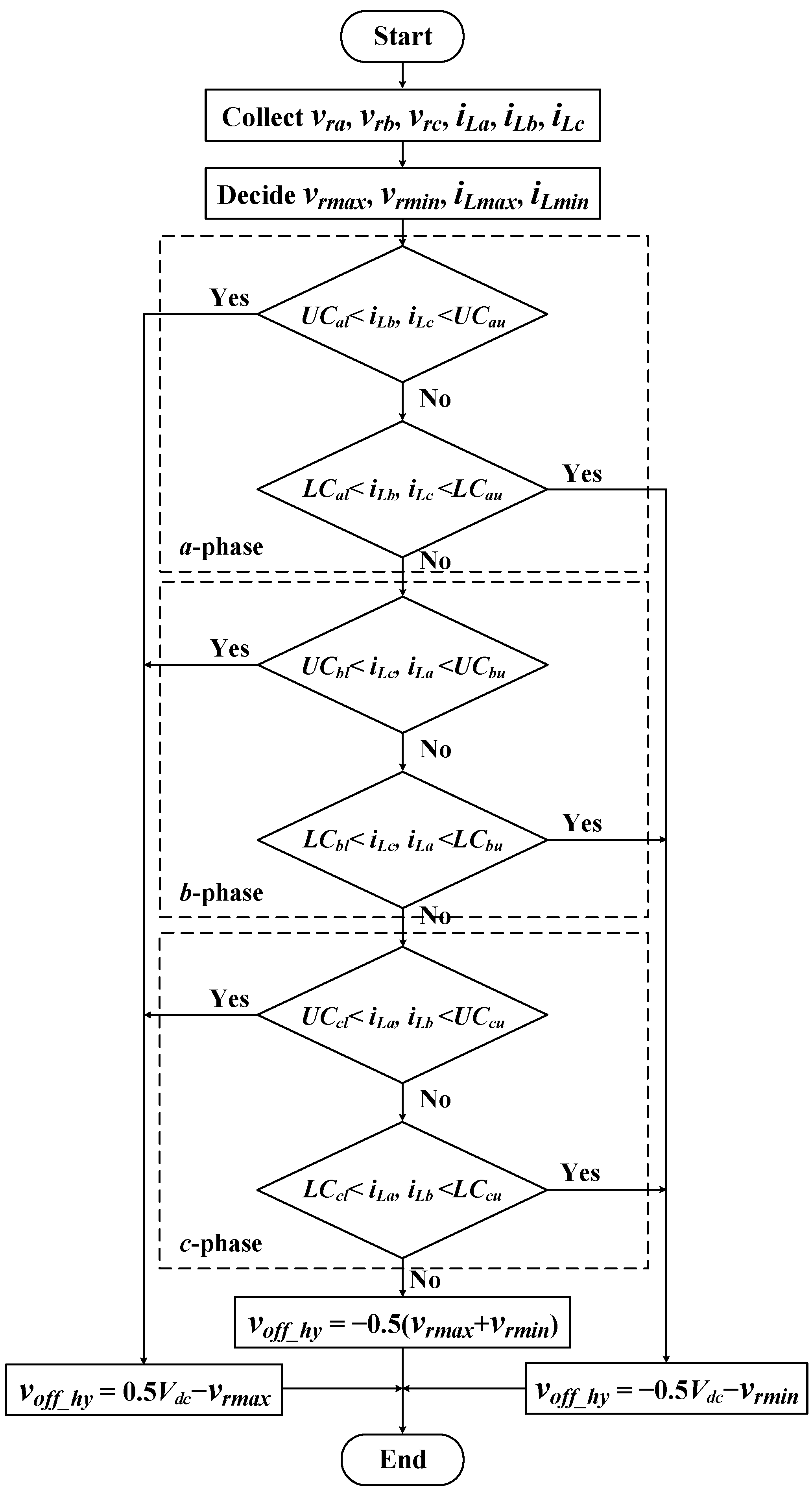

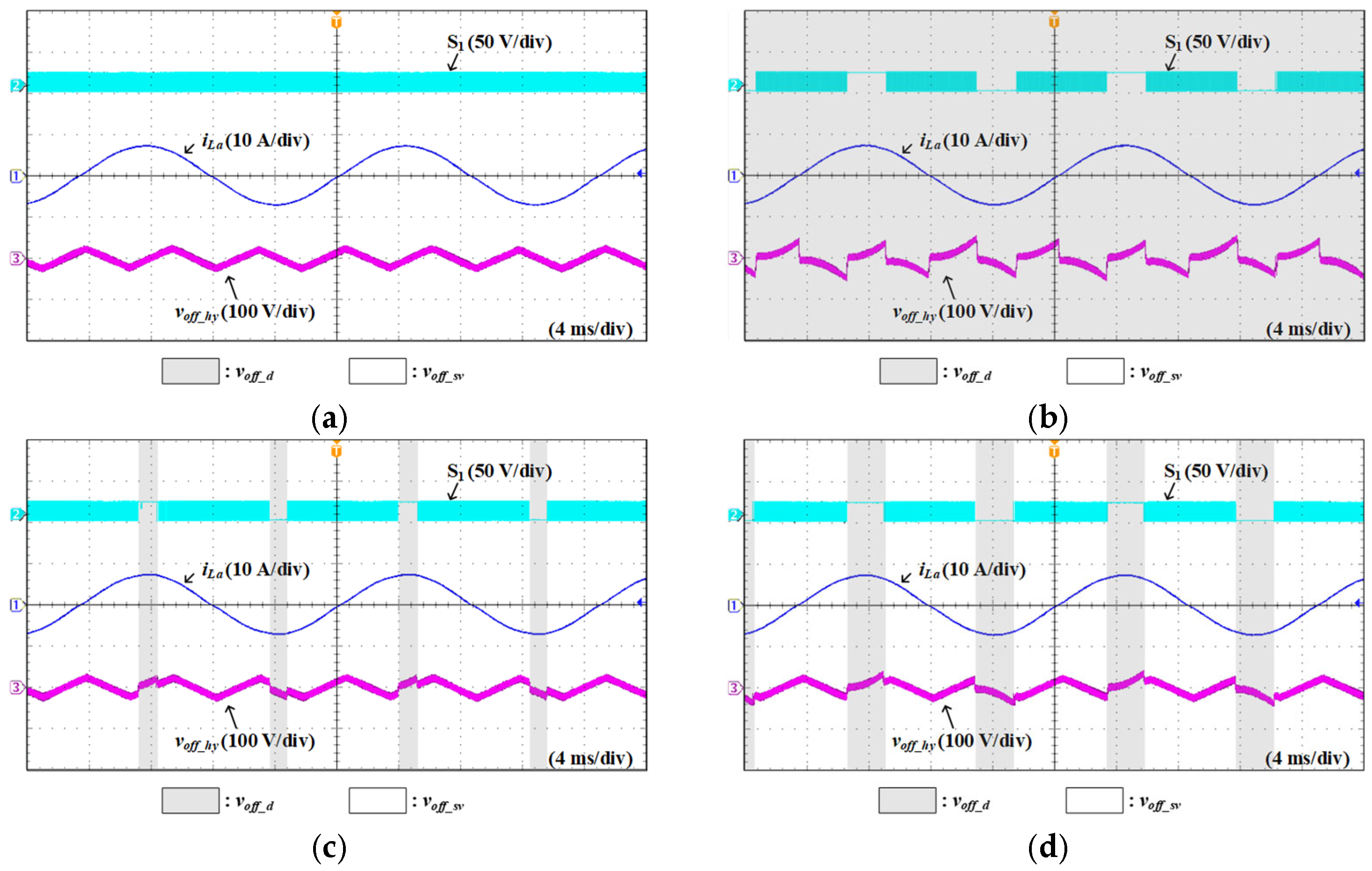







| Phase | Clamping Region | Condition |
|---|---|---|
| a-phase | Upper | UCal < iLb < UCau and UCal < iLc < UCau |
| Lower | LCal < iLb < LCau and LCal < iLc < LCau | |
| b-phase | Upper | UCbl < iLc < UCbu and UCbl < iLa < UCbu |
| Lower | LCbl < iLc < LCbu and LCbl < iLa < LCbu | |
| c-phase | Upper | UCcl < iLa < UCcu and UCcl < iLb < UCcu |
| Lower | LCcl < iLa < LCcu and LCcl < iLb < LCcu |
| Parameters | Value |
|---|---|
| L | 10 mH |
| R | 10 Ω |
| Cdc | 680 μF |
| Vdc | 200 V |
| iLpeak | 8 A |
| Frequency of reference load current | 60 Hz |
| Carrier frequency | 20 kHz |
| Parameters | Value |
|---|---|
| A | 9.3 |
| β1 | |
| β2 | |
| β3 | |
| β4 | |
| β5 | |
| β6 | |
| ton (s) | 1.66 |
| iB (A) | 10 |
| Vc (V) | 6.5 |
| db (μm) | 400 |
| R1 (K/W) | R2 (K/W) | R3 (K/W) | τ1 (s) | τ2 (s) | τ3 (s) |
|---|---|---|---|---|---|
| 0.092 | 0.721 | 0.068 | 0.00008 | 0.00473 | 0.00566 |
| Parameters | Value |
|---|---|
| L | 10 mH |
| R | 15 Ω |
| Cdc | 1100 μF |
| Vdc | 1000 V |
| iLpeak | 25 A |
| Frequency of reference load current | 60 Hz |
| Carrier frequency | 50 kHz |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kim, J.; Nguyen, M.-H.; Kwak, S.; Choi, S. Lifetime Extension Method for Three-Phase Voltage Source Converters Using Discontinuous PWM Scheme with Hybrid Offset Voltage. Machines 2023, 11, 612. https://doi.org/10.3390/machines11060612
Kim J, Nguyen M-H, Kwak S, Choi S. Lifetime Extension Method for Three-Phase Voltage Source Converters Using Discontinuous PWM Scheme with Hybrid Offset Voltage. Machines. 2023; 11(6):612. https://doi.org/10.3390/machines11060612
Chicago/Turabian StyleKim, Jaechang, Minh-Hoang Nguyen, Sangshin Kwak, and Seungdeog Choi. 2023. "Lifetime Extension Method for Three-Phase Voltage Source Converters Using Discontinuous PWM Scheme with Hybrid Offset Voltage" Machines 11, no. 6: 612. https://doi.org/10.3390/machines11060612
APA StyleKim, J., Nguyen, M.-H., Kwak, S., & Choi, S. (2023). Lifetime Extension Method for Three-Phase Voltage Source Converters Using Discontinuous PWM Scheme with Hybrid Offset Voltage. Machines, 11(6), 612. https://doi.org/10.3390/machines11060612






