Modeling of the Variation Propagation for Complex-Shaped Workpieces in Multi-Stage Machining Processes
Abstract
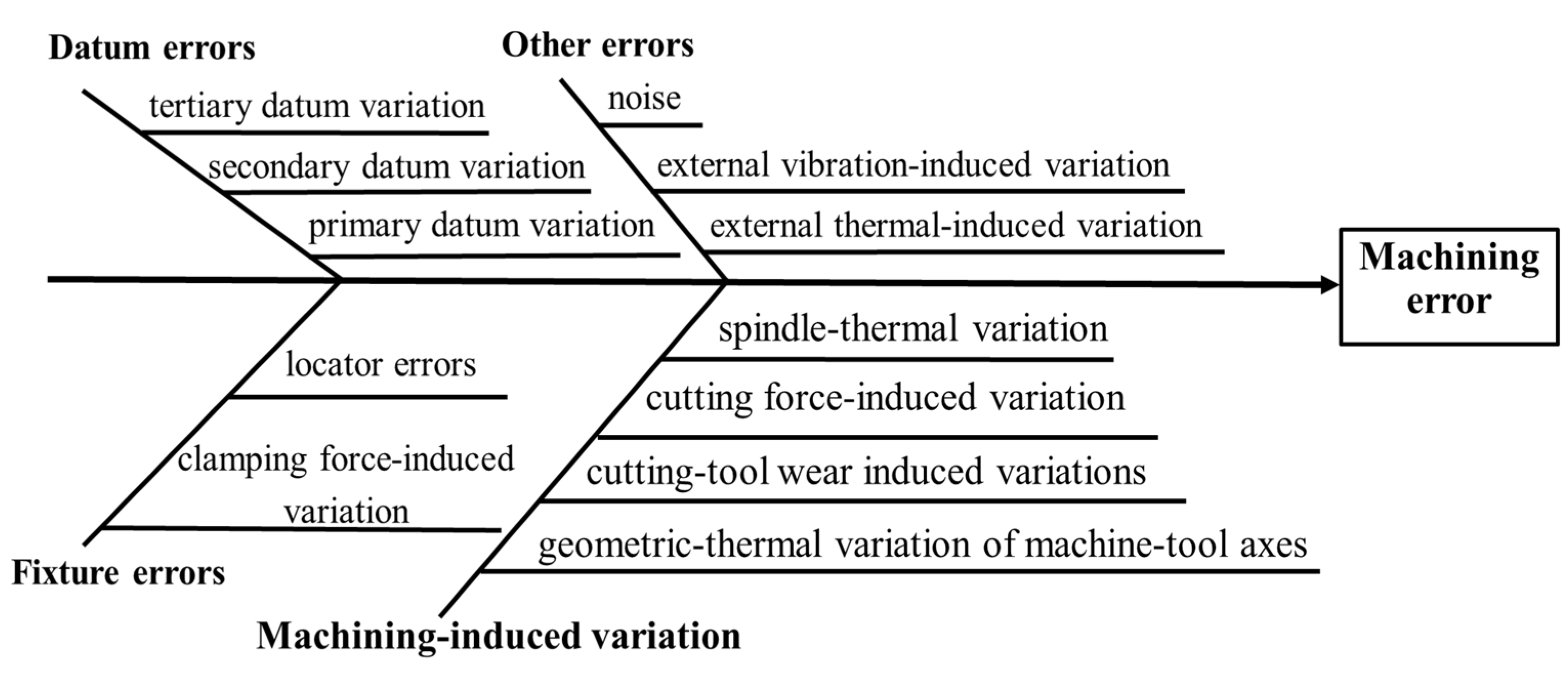
1. Introduction
2. Datum Error Analysis
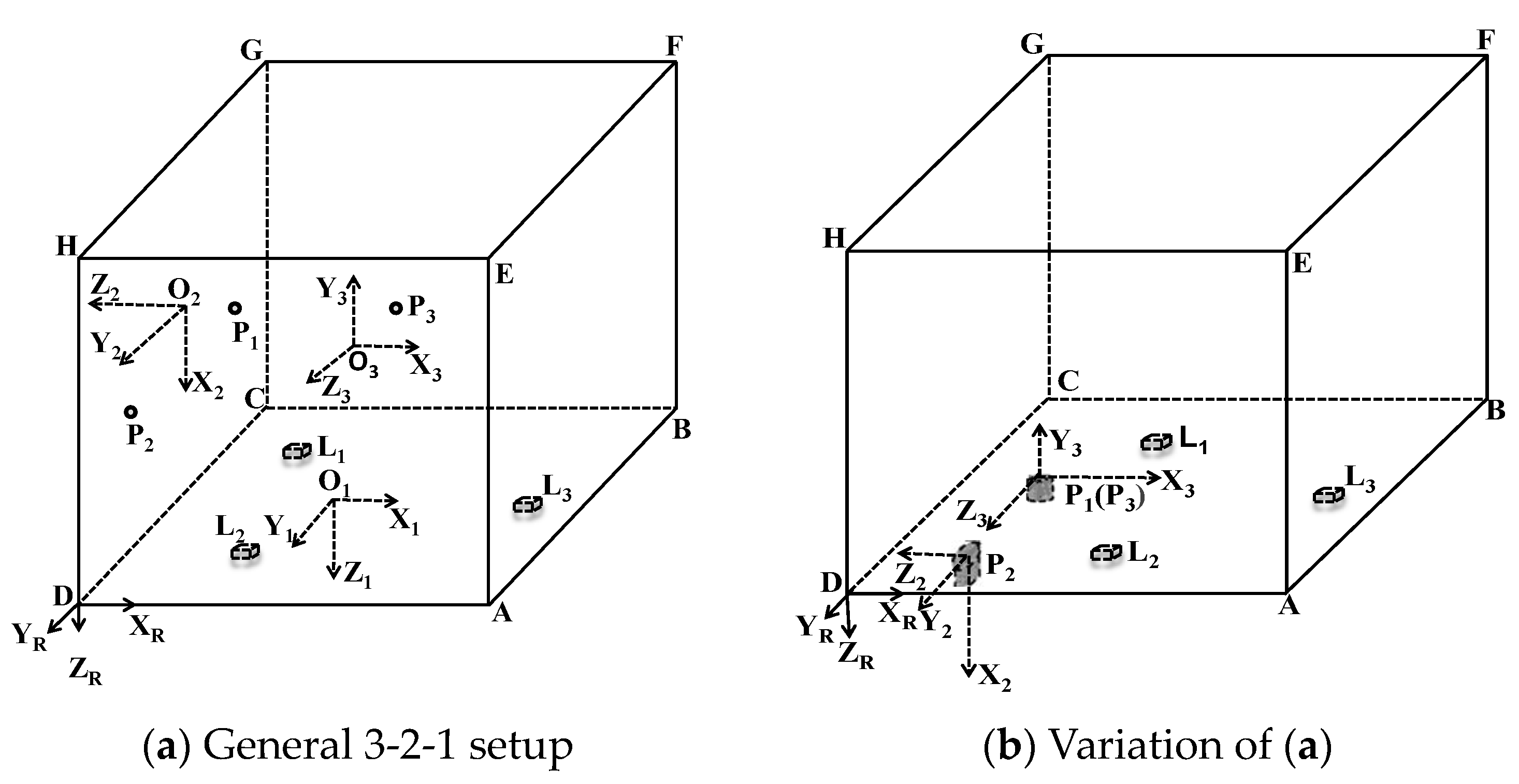
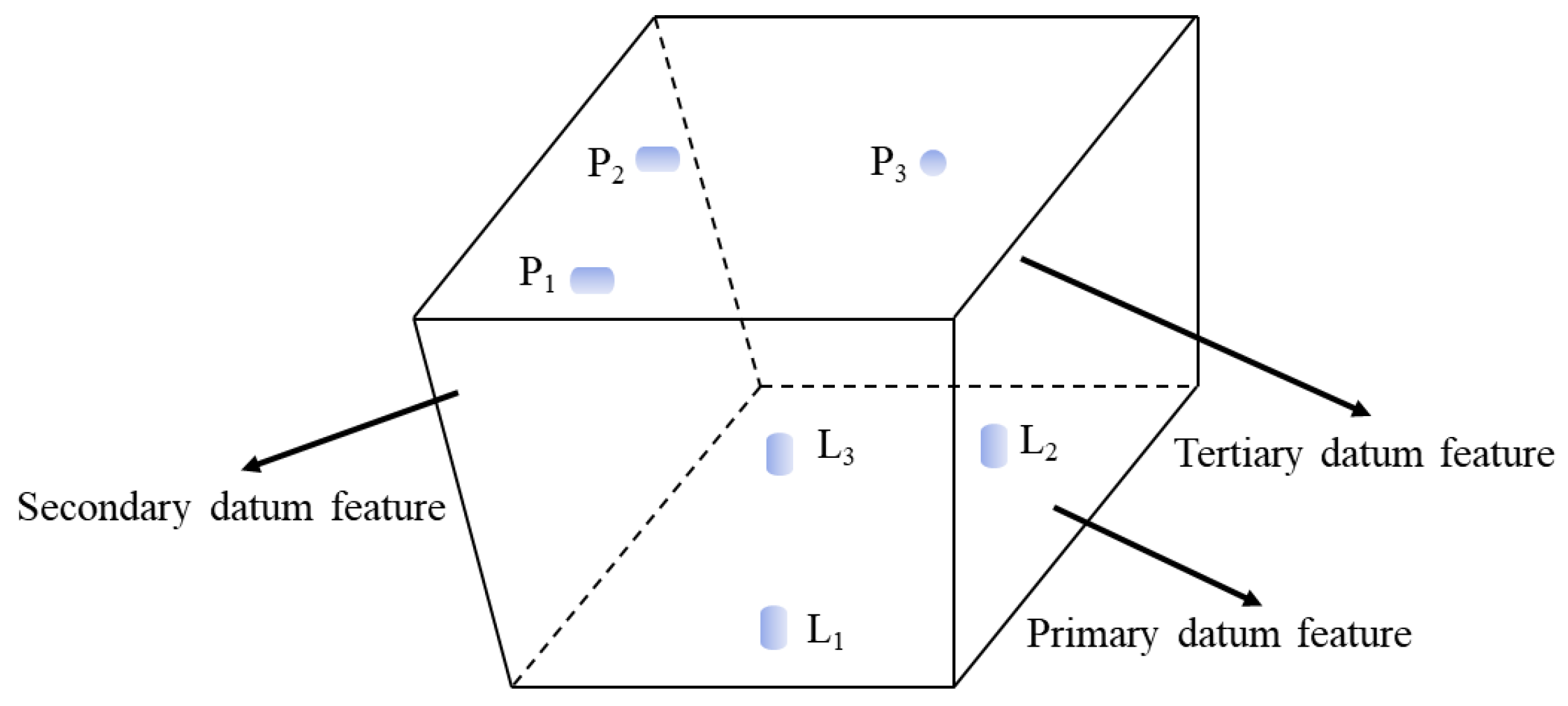
2.1. Fixture Layouts Description
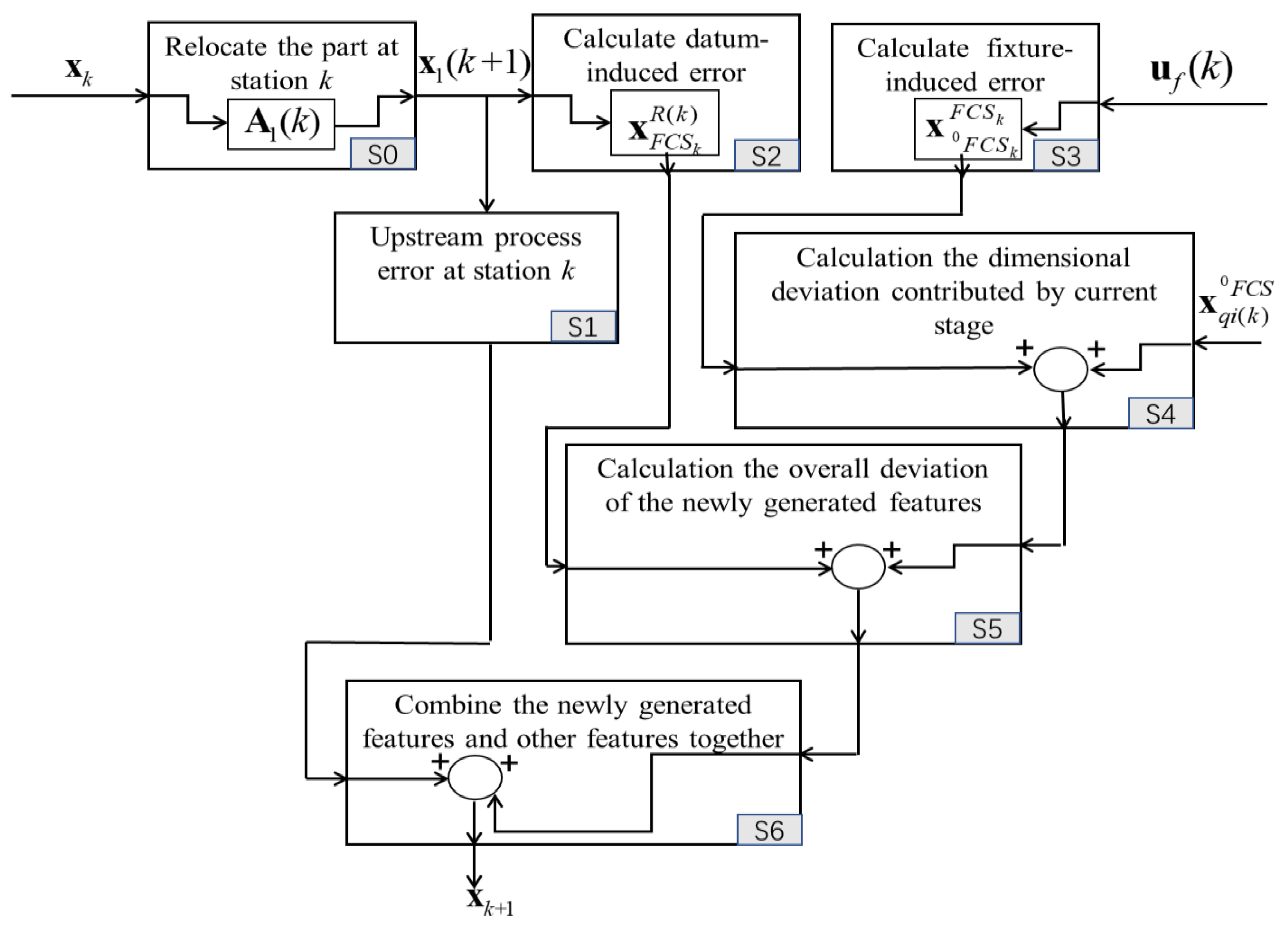
2.2. Datum Error Model
2.3. Time-Dependent Variations
2.4. Percentage Contributions for the Modified Model
3. Case Study
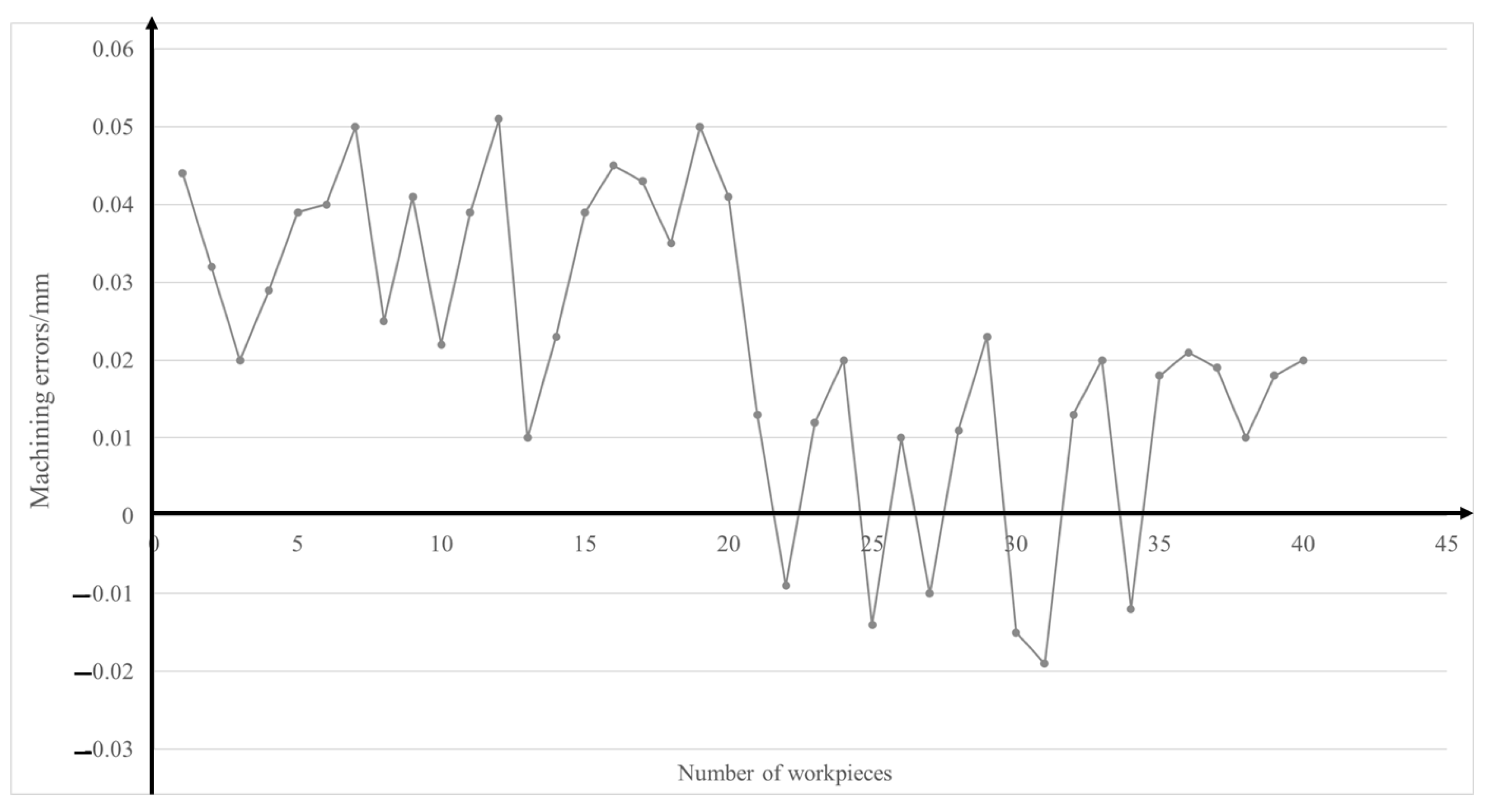
3.1. Simulation Case Study
3.2. Experimental Case Study
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| FCS | Fixture coordinate system. |
| LCSi | ith local coordinate system. |
| RCS | Reference coordinate system. The reference coordinate system is for the features of the workpiece. |
| HTM | Homogeneous transformation matrix. |
| HTM between RCS and LCSi. | |
| Rotational matrix between RCS and LCSi. | |
| , , | Transformation matrices for datum-induced error. |
| Position and orientation deviation of LCSn from nominal positions with regard to RCS. | |
| , , | Three elements of . |
| , , | Three elements of . |
| Differential transformation matrix of . | |
| Vector of the origin of LCSn expressed in RCS. | |
| , , | Three elements of . |
| Euler rotational angles between RCS and LCSn. | |
| , , | Three elements of . |
| Differential motion vector representing the deviation of LCS in RCS. It is a stack of and and is expressed in LCS. |
Appendix A
References
- Ramesh, R.; Mannan, M.A.; Poo, A.N. Error compensation in machine tools-a review part I: Geometric, cutting-force induced and fixture dependent errors. Int. J. Mach. Tools Manuf. 2000, 40, 1235–1256. [Google Scholar] [CrossRef]
- Cai, W.; Hu, S.J.; Yuan, J. A variational method of robust fixture configuration design for 3-Dworkpieces. ASME J. Manuf. Sci. Eng. 1997, 119, 593–602. [Google Scholar] [CrossRef]
- Dong, Z.; Jiao, L.; Wang, X.; Liang, Z.; Liu, Z.; Yi, J. FEA-based prediction of machined surface errors for dynamic fixture-workpiece system during milling process. Int. J. Adv. Manuf. Technol. 2016, 85, 299–315. [Google Scholar] [CrossRef]
- Ramesh, R.; Mannan, M.A.; Poo, A.N. Variation compensation in machine tools—A review part II: Thermal variations. Int. J. Mach. Tools Manuf. 2000, 40, 1235–1256. [Google Scholar] [CrossRef]
- Nguyen, H.T.; Wang, H.; Hu, S.J. Characterization of cutting force induced surface shape variation in face milling using high-definition metrology. J. Manuf. Sci. Eng. Trans. ASME 2013, 135, 41014. [Google Scholar] [CrossRef]
- Liu, S.; Jin, S.; Zhang, X.; Chen, K.; Tian, A.; Xi, L. A coupled model for the prediction of surface variation in face milling large-scale workpiece with complex geometry. J. Manuf. Sci. Eng. Trans. ASME 2019, 141, 31009–31014. [Google Scholar] [CrossRef]
- Wang, K.; Li, G.L.; Du, S.C.; Xi, L.F.; Xia, T.B. State space modelling of variation propagation in multistage machining processes for variable stiffness structure workpieces. Int. J. Prod. Res. 2020, 59, 4033–4052. [Google Scholar] [CrossRef]
- Wang, H.; Huang, Q.; Katz, R. Multi-operational machining processes modeling for sequential root cause identification and measurement reduction. J. Manuf. Sci. Eng. Trans. ASME 2005, 127, 512–521. [Google Scholar] [CrossRef]
- Hu, S.J.; Koren, Y. Stream-of-variation theory for automotive body assembly. CIRP Ann. 1997, 46, 1–6. [Google Scholar] [CrossRef]
- Jin, J.; Shi, J. State space modeling of sheet metal assembly for dimensional control. J. Manuf. Sci. Eng. 1999, 121, 756–762. [Google Scholar] [CrossRef]
- Guo, J.; Li, B.; Liu, Z.; Hong, J.; Wu, X. Integration of geometric variation and part deformation into variation propagation of 3-D assemblies. Int. J. Prod. Res. 2016, 54, 5708–5721. [Google Scholar] [CrossRef]
- Gianfranco, G.; Galetto, M.; Franceschini, F. Product complexity and design of inspection strategies for assembly manufacturing processes. Int. J. Prod. Res. 2018, 56, 4056–4066. [Google Scholar]
- Zhong, W.; Huang, Y.; Hu, S.J. Modeling variation propagation in machining systems with different configurations. ASME Int. Mech. Eng. Congr. Expo. 2002, 671, 97–106. [Google Scholar]
- Huang, Q.; Shi, J.; Yuan, J. Part dimensional error and its propagation modeling in multi-operational machining processes. J. Manuf. Sci. Eng. 2003, 125, 255–262. [Google Scholar] [CrossRef]
- Zhou, S.; Huang, Q.; Shi, J. State space modeling of dimensional variation propagation in multistage machining process using differential motion vectors. IEEE Trans. Robot. Autom. 2003, 19, 296–309. [Google Scholar] [CrossRef]
- Loose, J.P.; Zhou, S.; Ceglarek, D. Kinematic analysis of dimensional variation propagation for multistage machining processes with general fixture layouts. IEEE Trans. Robot. Autom. 2007, 4, 141–152. [Google Scholar] [CrossRef]
- Abellan-Nebot, J.V.; Liu, J.; Subirón, F.R.; Shi, J. State space modeling of variation propagation in multistation machining processes considering machining-induced variations. J. Manuf. Sci. Eng. 2012, 134, 021002. [Google Scholar] [CrossRef]
- Abellan-Nebot, J.V.; Liu, J. Variation propagation modeling for multi-station machining processes with fixtures based on locating surfaces. Int. J. Prod. Res. 2013, 51, 4667–4681. [Google Scholar] [CrossRef]
- Liu, J.; Shi, J.; Hu, S.J. Quality-assured setup planning based on the stream-of-variation model for multi-stage machining processes. IIE Trans. 2009, 41, 323–334. [Google Scholar] [CrossRef]
- Zhang, M.; Djurdjanovic, D.; Ni, J. Diagnosibility and sensitivity analysis for multi-station machining processes. Int. J. Mach. Tools Manuf. 2007, 47, 646–657. [Google Scholar] [CrossRef]
- Yang, F.; Xing, Y.; Li, X. A comprehensive error compensation strategy for machining process with general fixture layouts. Int. J. Adv. Manuf. Technol. 2020, 107, 2707–2717. [Google Scholar] [CrossRef]
- Du, S.; Yao, X.; Huang, D. Engineering model-based Bayesian monitoring of ramp-up phase of multistage manufacturing process. Int. J. Prod. Res. 2015, 53, 4594–4613. [Google Scholar] [CrossRef]
- Djurdjanovic, D.; Ni, J. Stream-of-variation (SoV)-based measurement scheme analysis in multistation machining systems. IEEE Trans. Autom. Sci. Eng. 2006, 3, 407–422. [Google Scholar] [CrossRef]
- Qin, Y.; Zhao, L.; Yao, Y.; Xu, D. Multistage machining processes variation propagation analysis based on machining processes weighted network performance. Int. J. Adv. Manuf. Technol. 2011, 55, 487–499. [Google Scholar] [CrossRef]
- Wang, K.; Du, S.; Xi, L. Three-dimensional tolerance analysis modelling of variation propagation in multistage machining processes for general shape workpieces. Int. J. Precis. Eng. Manuf. 2020, 21, 31–44. [Google Scholar] [CrossRef]
- Abellan-Nebot, J.V.; Liu, J.; Subiron, F.R. Process-oriented tolerancing using the extended stream of variation model. Comput. Ind. 2013, 6, 485–498. [Google Scholar] [CrossRef]
- Loose, J.-P.; Zhou, Q.; Zhou, S.; Ceglarek, D. Integrating GD&T into dimensional variation models for multistage machining processes. Int. J. Prod. Res. 2010, 48, 3129–3149. [Google Scholar]
- Yang, F.; Jin, S.; Li, Z. A comprehensive study of linear variation propagation modeling methods for multistage machining processes. Int. J. Adv. Manuf. Technol. 2017, 90, 2139–2151. [Google Scholar] [CrossRef]
- Yang, F.; Sun Jin, S.; Li, Z. A modification of dmvs based state space model of variation propagation for multistage machining processes. Assem. Autom. 2017, 37, 381–390. [Google Scholar] [CrossRef]
- Kun Wang, K.; Yin, Y.; Du, S.; Xi, L. Variation management of key control characteristics in multistage machining processes based on quality-cost equilibrium. J. Manuf. Syst. 2021, 59, 441–452. [Google Scholar] [CrossRef]
- Zhu, M.; Ge, G.; Feng, X.; Du, Z.; Yang, J. A novel error compensation method for multistage machining processes based on differential motion vector sets of multiple contour points. J. Manuf. Sci. Eng. 2020, 143, 061010. [Google Scholar] [CrossRef]
- Zuo, X.; Zhou, Y.; Xiong, T.; Zhang, C.; Zhang, C.; Zhou, X. A control method with considering error coupling in milling multi-feature thin-walled parts. Int. J. Adv. Manuf. Technol. 2022, 122, 1525–1537. [Google Scholar] [CrossRef]
- Yang, F.; Jin, S.; Li, Z.; Ding, S.; Ma, X. A new error compensation model for machining process based on differential motion vectors. Int. J. Adv. Manuf. Technol. 2017, 93, 2943–2954. [Google Scholar] [CrossRef]
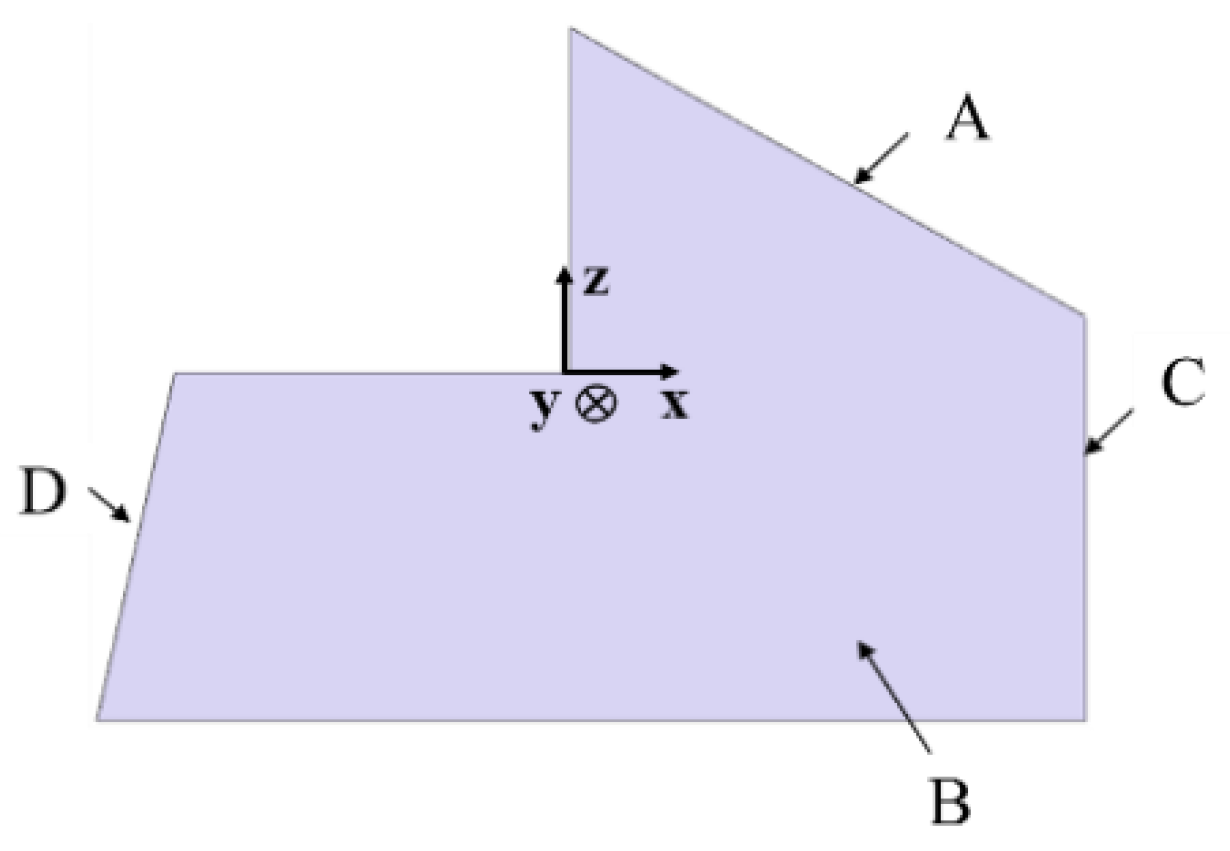
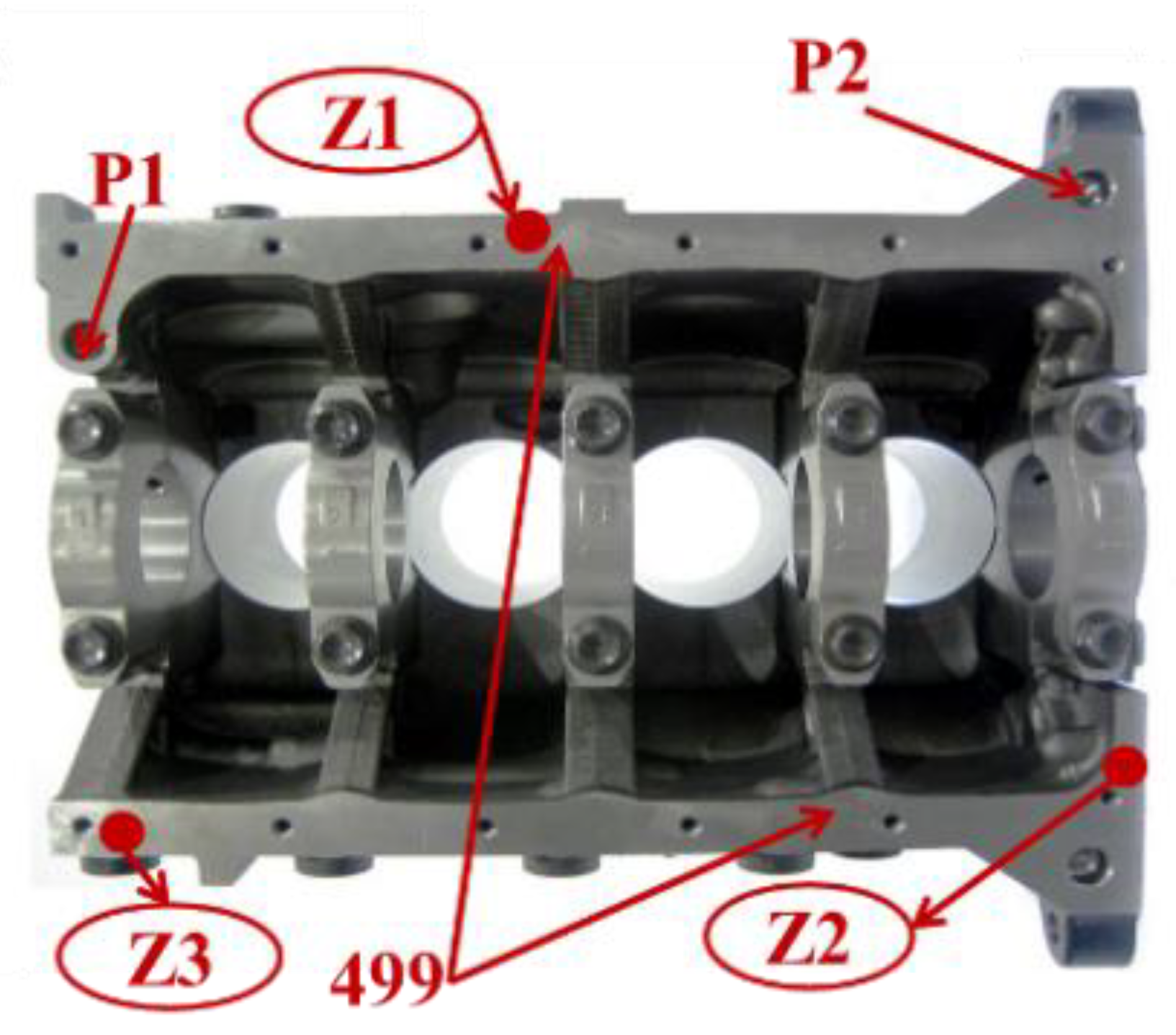
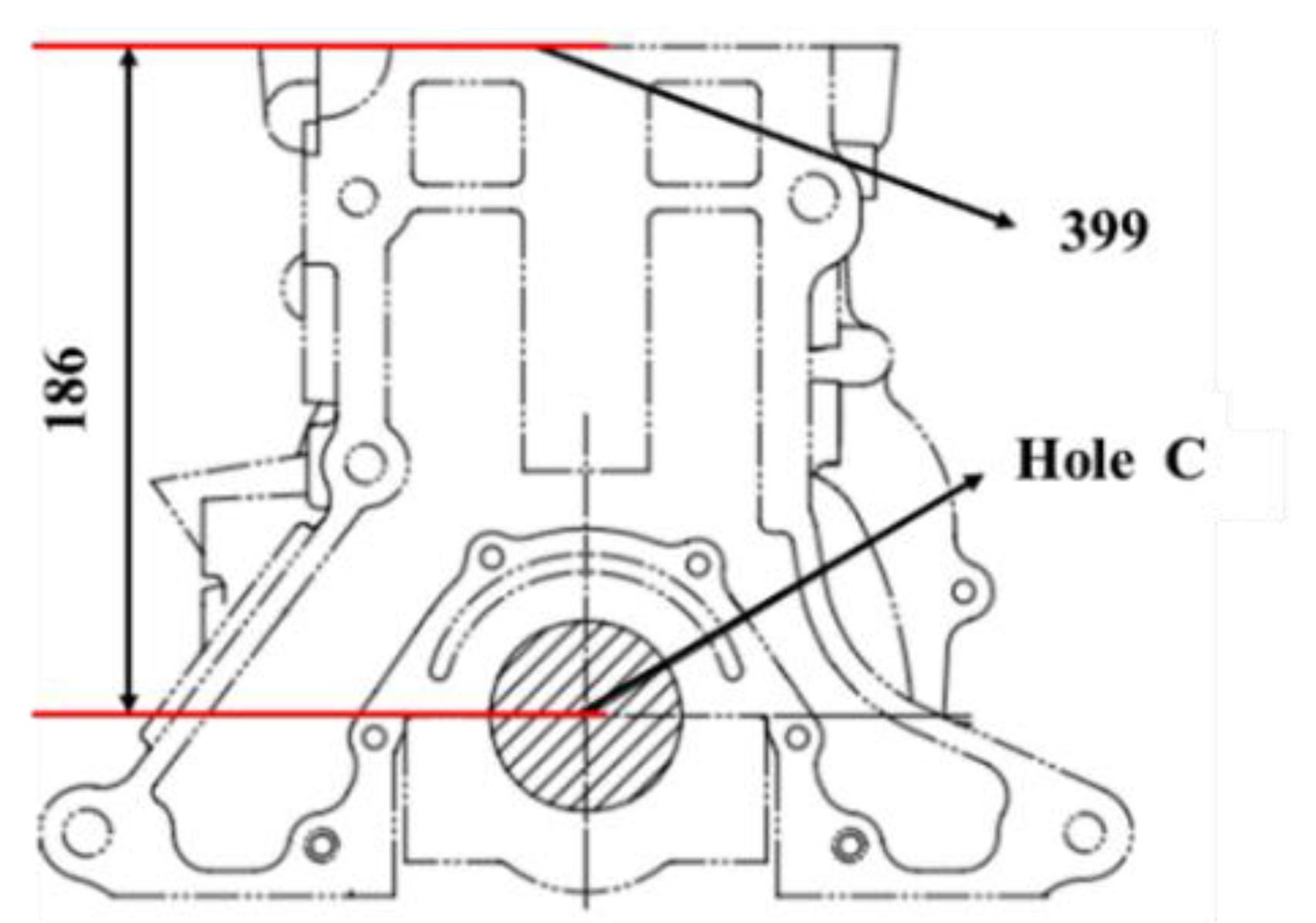
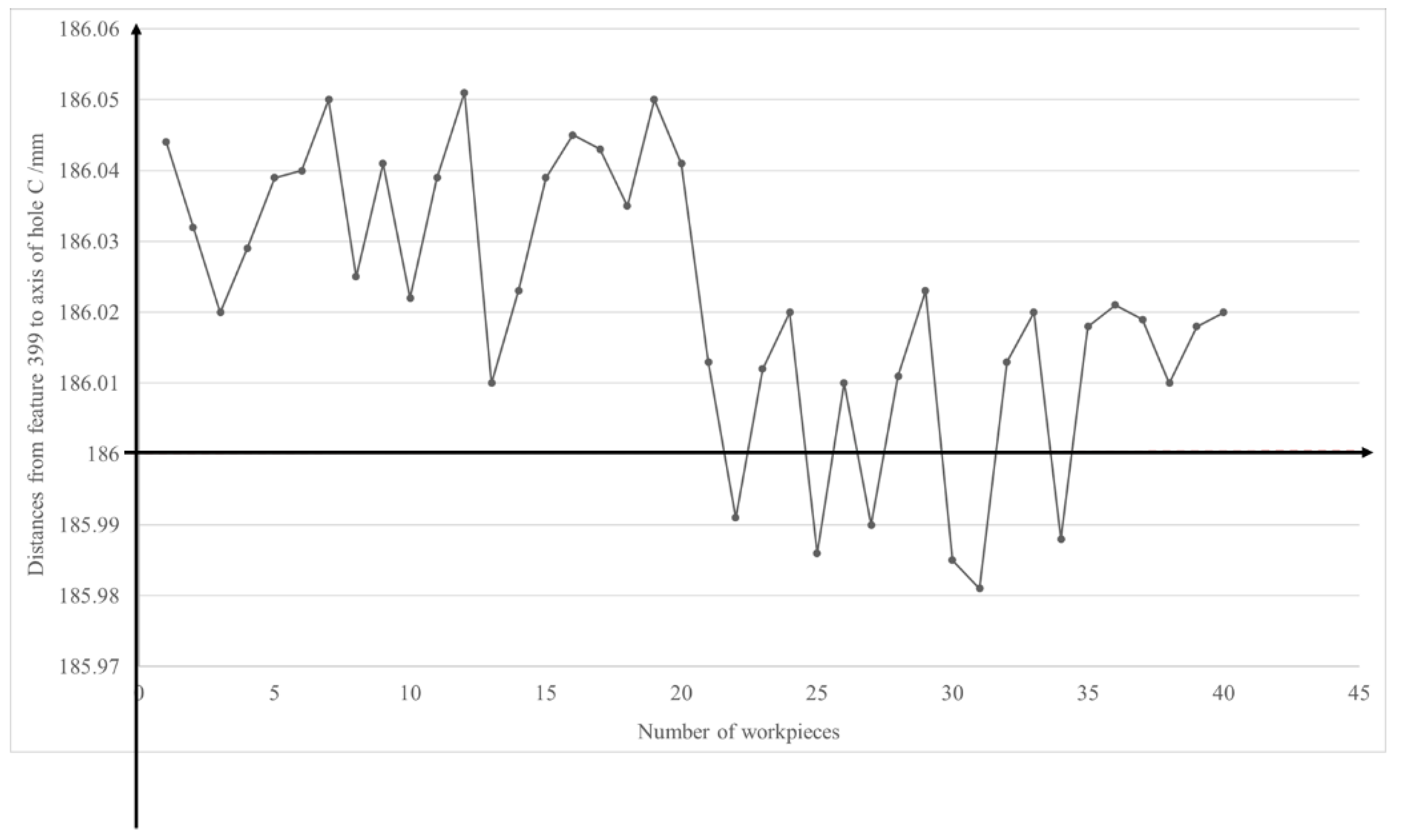
| Locator Index | Datum Feature | Locator Coordinate in FCS | Outgoing Normal Direction |
|---|---|---|---|
| 1 | A | [40, 30, 100] | [0.4997, 0, 0.8662] |
| 2 | A | [70, 70, 80] | [0.4997, 0, 0.8662] |
| 3 | A | [110, 30, 40] | [0.4997, 0, 0.8662] |
| 4 | B | [−90, 0, 80] | [0, −1, 0] |
| 5 | B | [90, 0, 0] | [0, −1, 0] |
| 6 | C | [130, 50, −95] | [1, 0, 0] |
| Feature Number | ||
|---|---|---|
| A | [0, 0, 110] | [0, 0.5233, 0] |
| B | [45, 0, 0] | [π/2, 0, 0] |
| C | [156, 40, −85] | [0, π/2, 0] |
| D | [−130, 0, 20] | [0, −π – 0.8, 0] |
| Number | Variation Values | Sources Description |
|---|---|---|
| 1 | Primary datum error | |
| 2 | Secondary datum error | |
| 3 | Tertiary datum error | |
| 4 | Fixture error | |
| 5 | Machine tool path error |
| Locator Index | Coordinate | Orientations | From Feature |
|---|---|---|---|
| L1 | [−103, −182.75, 50.5] | [0, 0, 0] | Primary datum feature |
| L2 | [81.5, −7.5, 50.5] | [0, 0, 0] | |
| L3 | [97, −309.5, 50.5] | [0, 0, 0] | |
| P1 | [−65, −312.5, 50.5] | [0, −π/2, 0] | Secondary datum feature |
| P2 | [−111, −17, 50.5] | [0, −π/2, 0] | |
| P3 | [−65, −312.5, 50.5] | [π/2, 0, 0] | Tertiary datum feature |
| Number | Variation Values | Sources Description |
|---|---|---|
| 1 | Primary datum error | |
| Fixture error |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, F.; Zhang, P.; Zhang, X.; Cao, J.; Xing, Y. Modeling of the Variation Propagation for Complex-Shaped Workpieces in Multi-Stage Machining Processes. Machines 2023, 11, 603. https://doi.org/10.3390/machines11060603
Yang F, Zhang P, Zhang X, Cao J, Xing Y. Modeling of the Variation Propagation for Complex-Shaped Workpieces in Multi-Stage Machining Processes. Machines. 2023; 11(6):603. https://doi.org/10.3390/machines11060603
Chicago/Turabian StyleYang, Fuyong, Peiyue Zhang, Xiaobing Zhang, Juyong Cao, and Yanfeng Xing. 2023. "Modeling of the Variation Propagation for Complex-Shaped Workpieces in Multi-Stage Machining Processes" Machines 11, no. 6: 603. https://doi.org/10.3390/machines11060603
APA StyleYang, F., Zhang, P., Zhang, X., Cao, J., & Xing, Y. (2023). Modeling of the Variation Propagation for Complex-Shaped Workpieces in Multi-Stage Machining Processes. Machines, 11(6), 603. https://doi.org/10.3390/machines11060603






