A Study on the Improvement of Power Density of Axial Flux Motors for Collaborative Robot Joints through Same-Direction Skew
Abstract
1. Introduction
2. Features of Axial Flux Motors
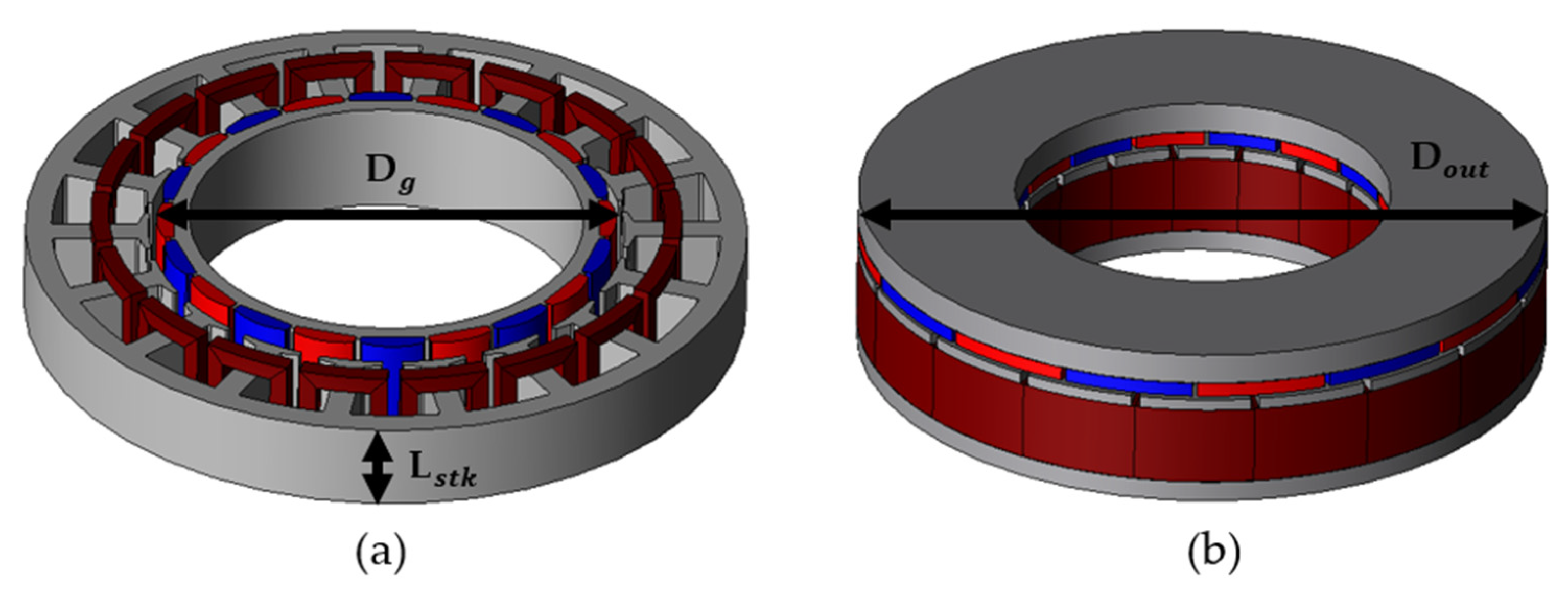
3. The 200 W Collaborative Robot Joint Motor
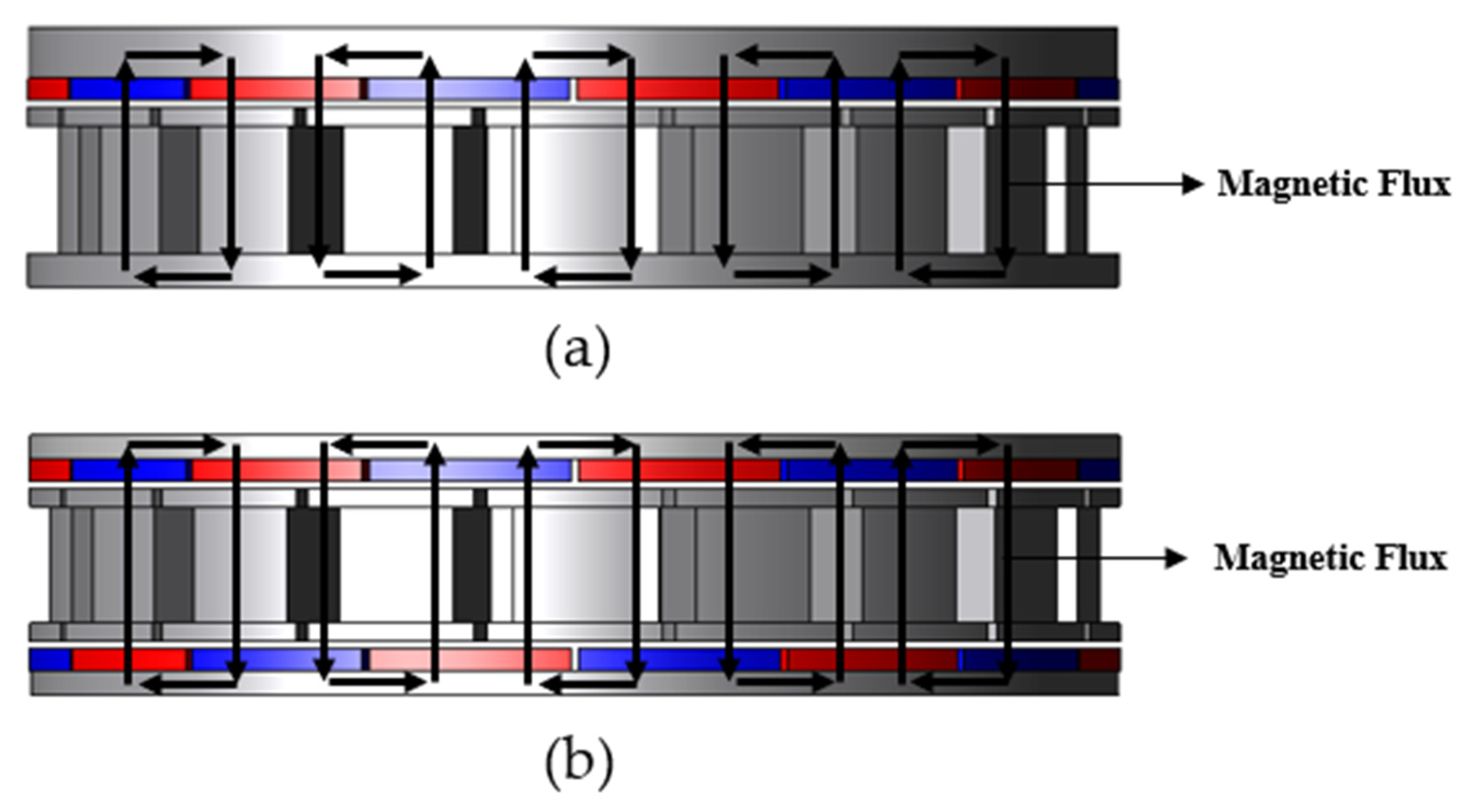
4. Same-Direction Skew
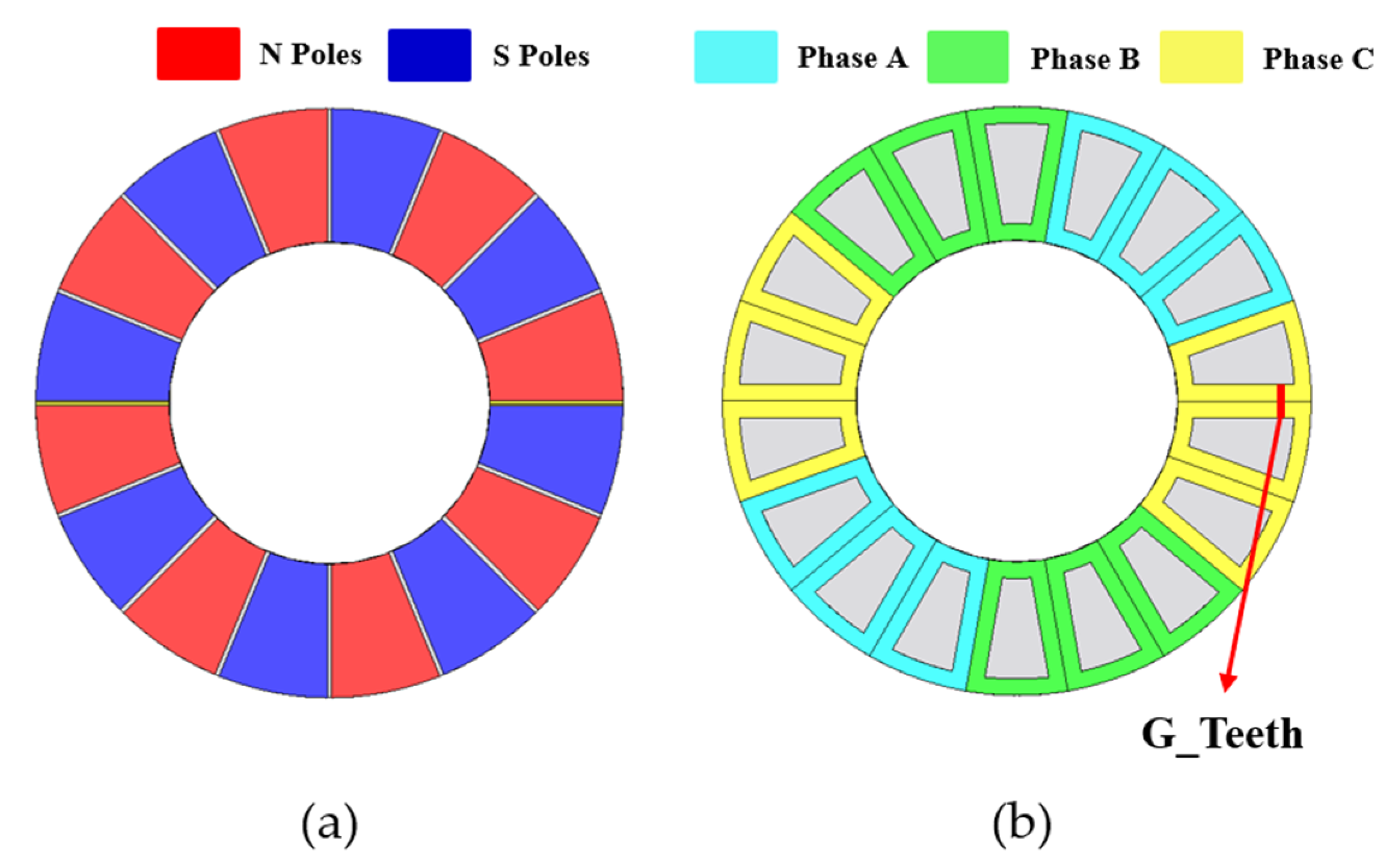
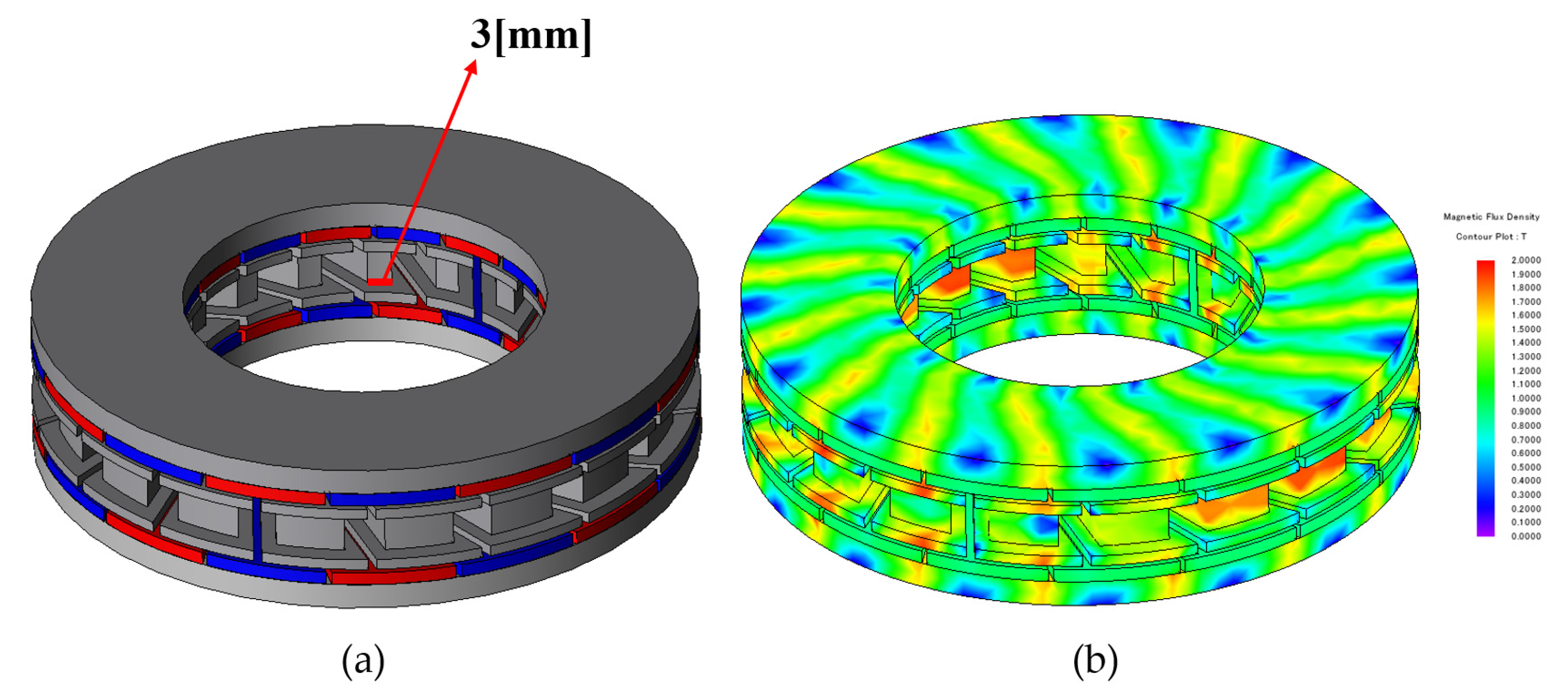
4.1. Principal of Same-Direction Skew
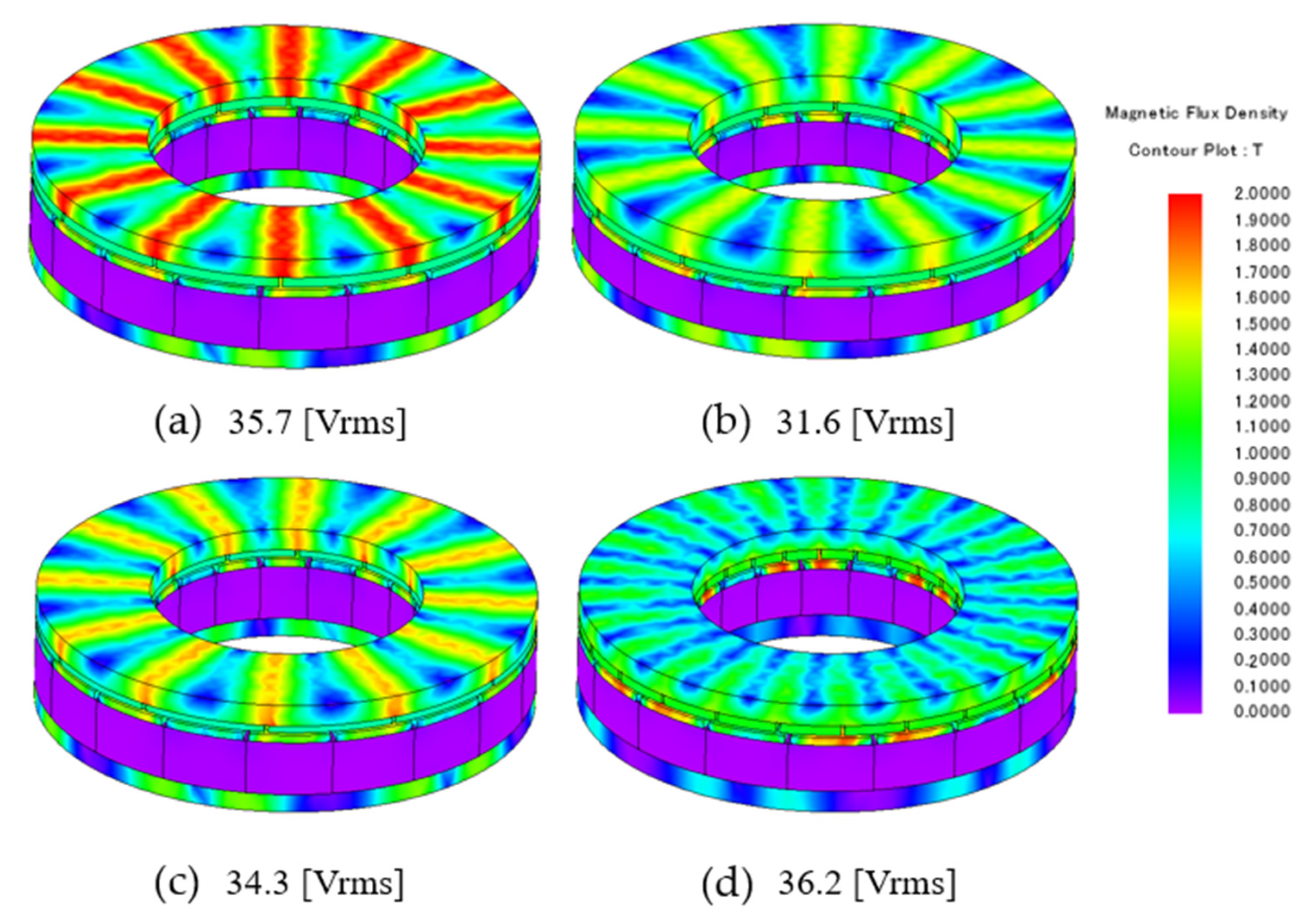
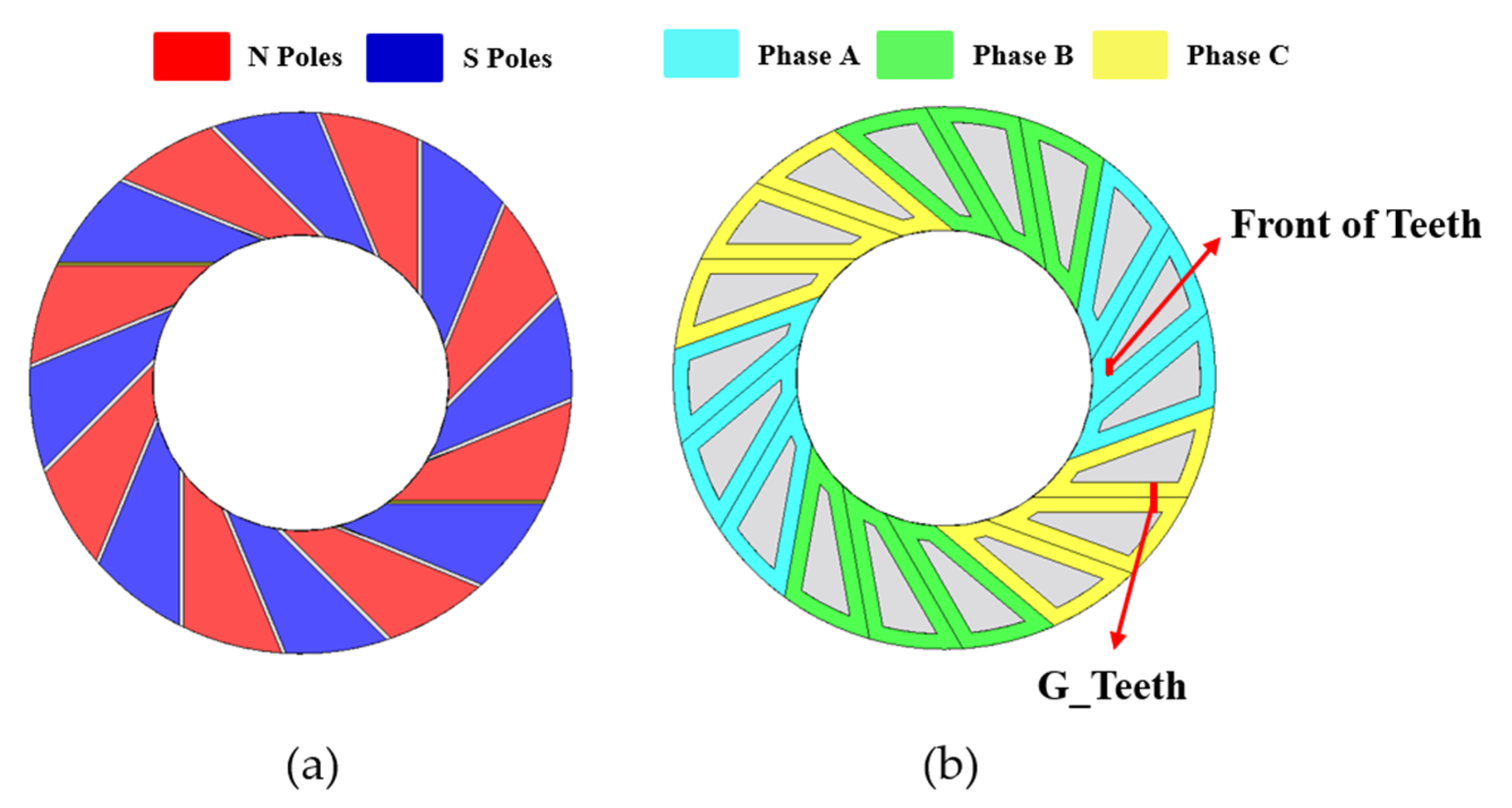
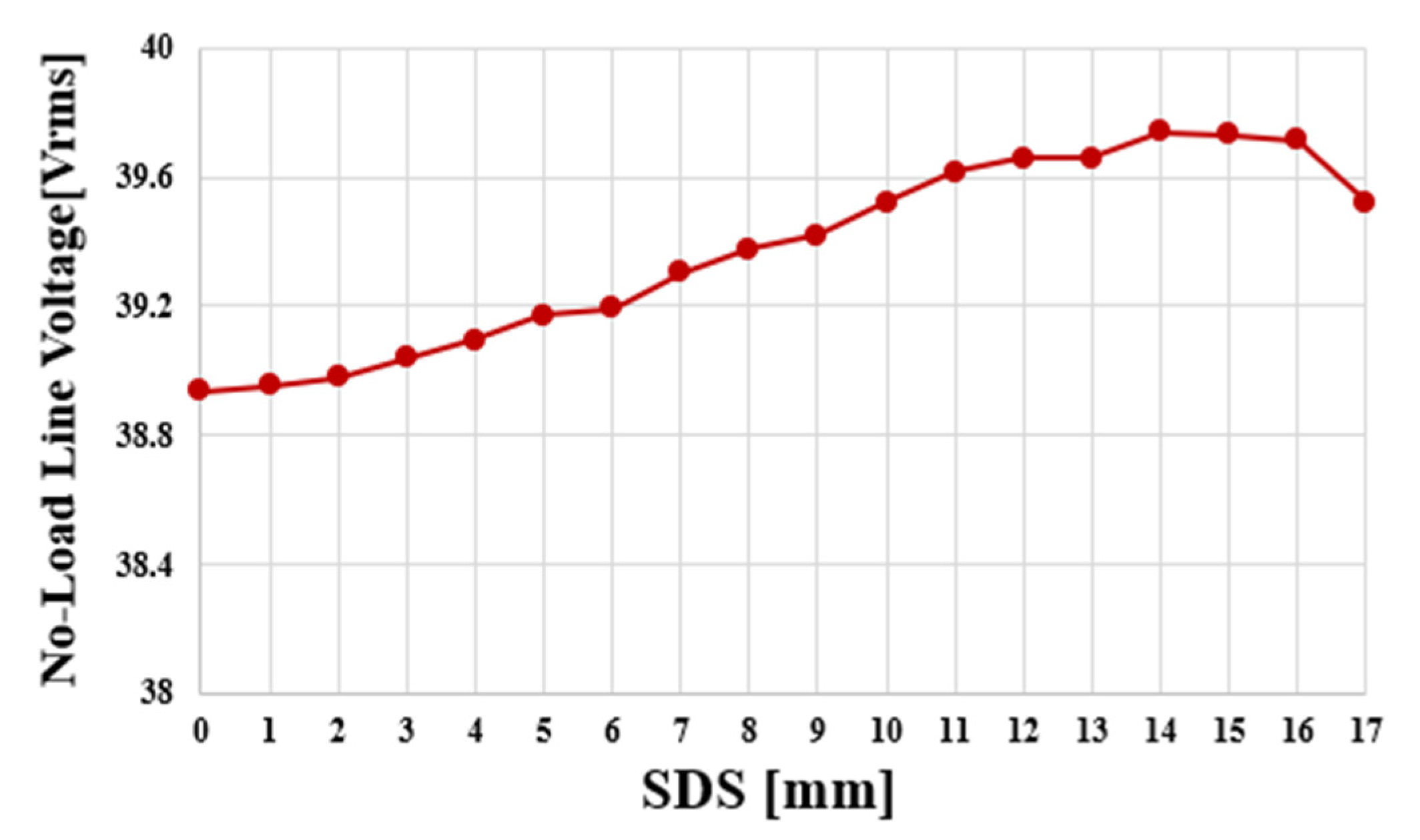
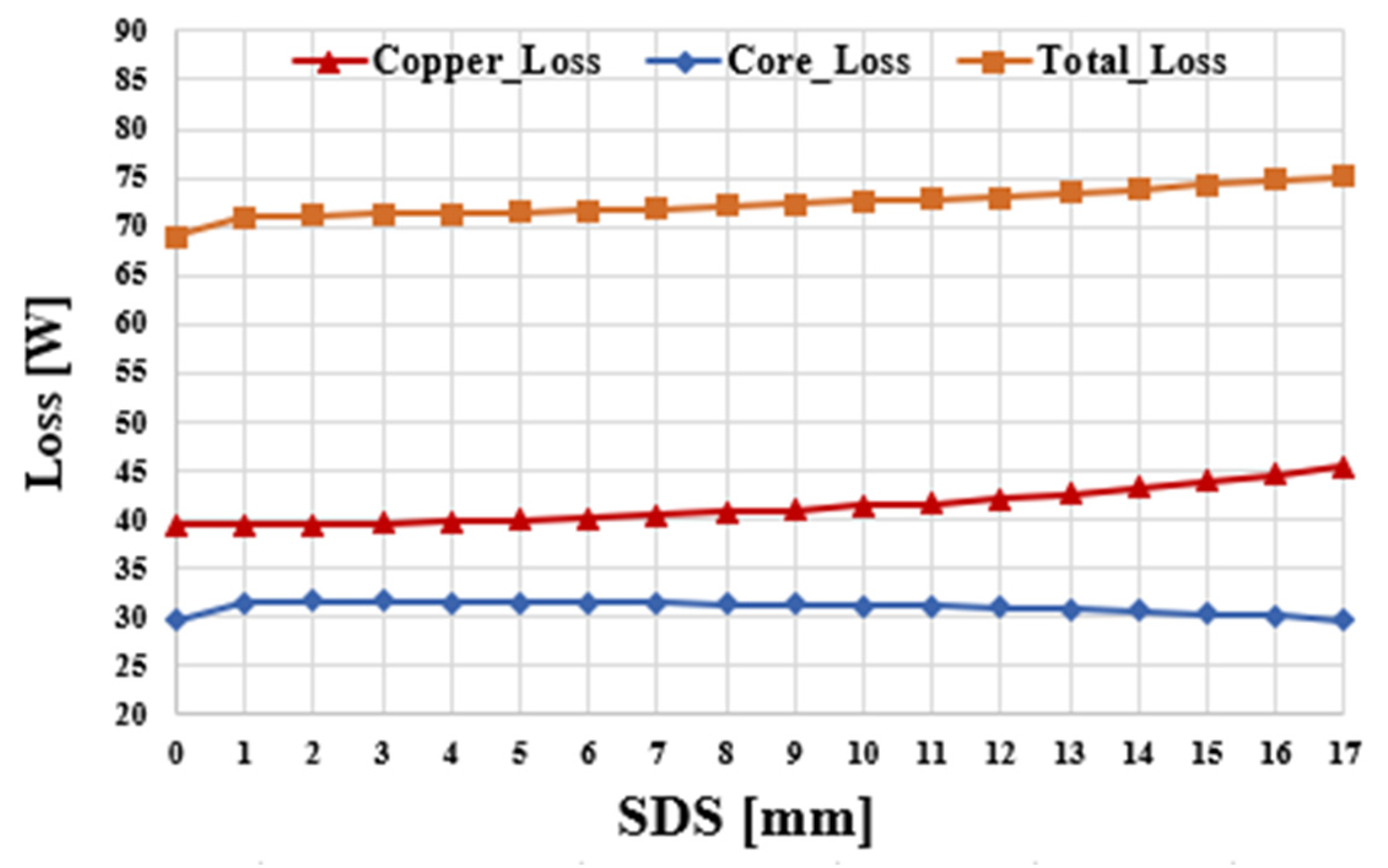
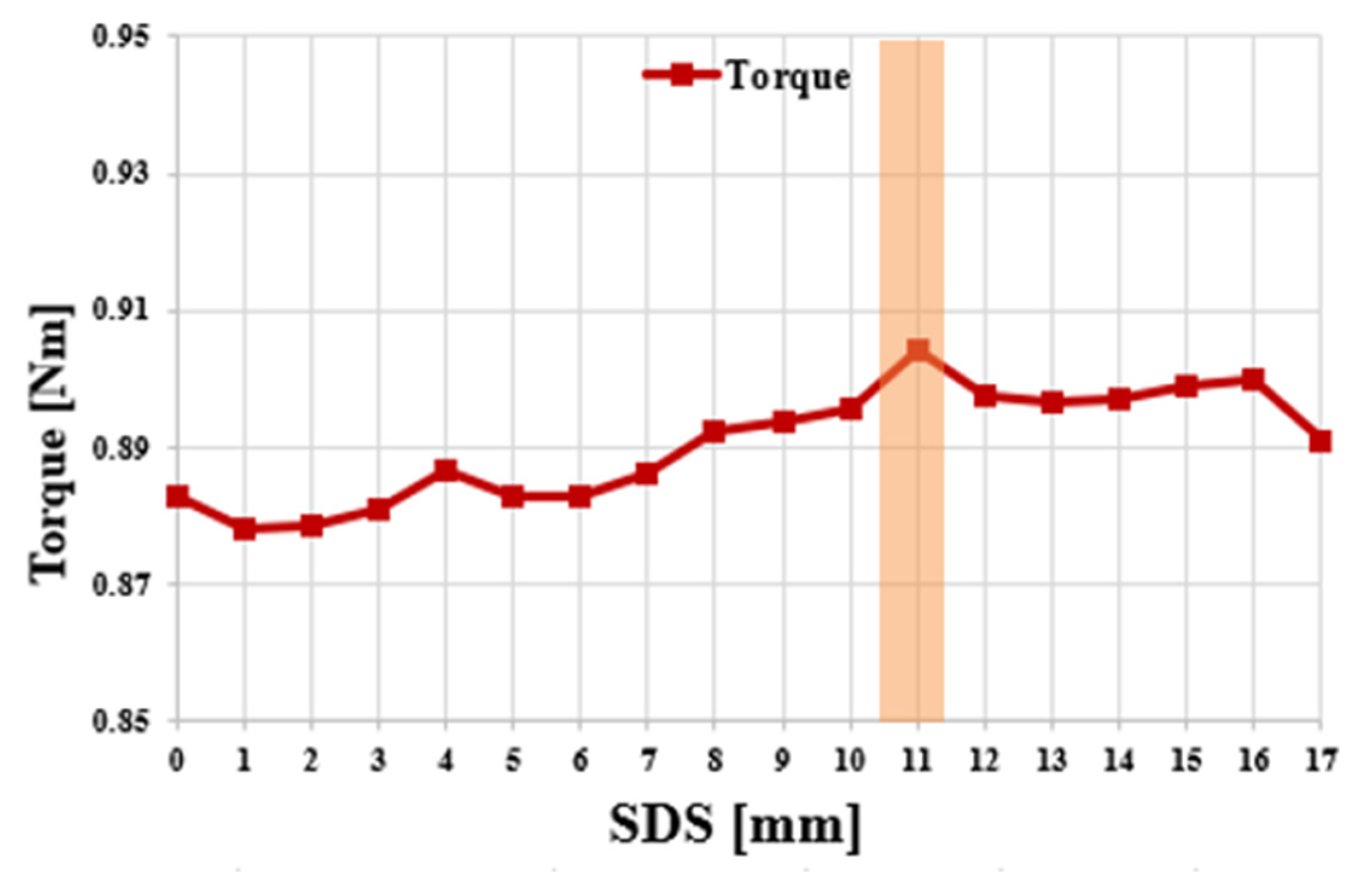
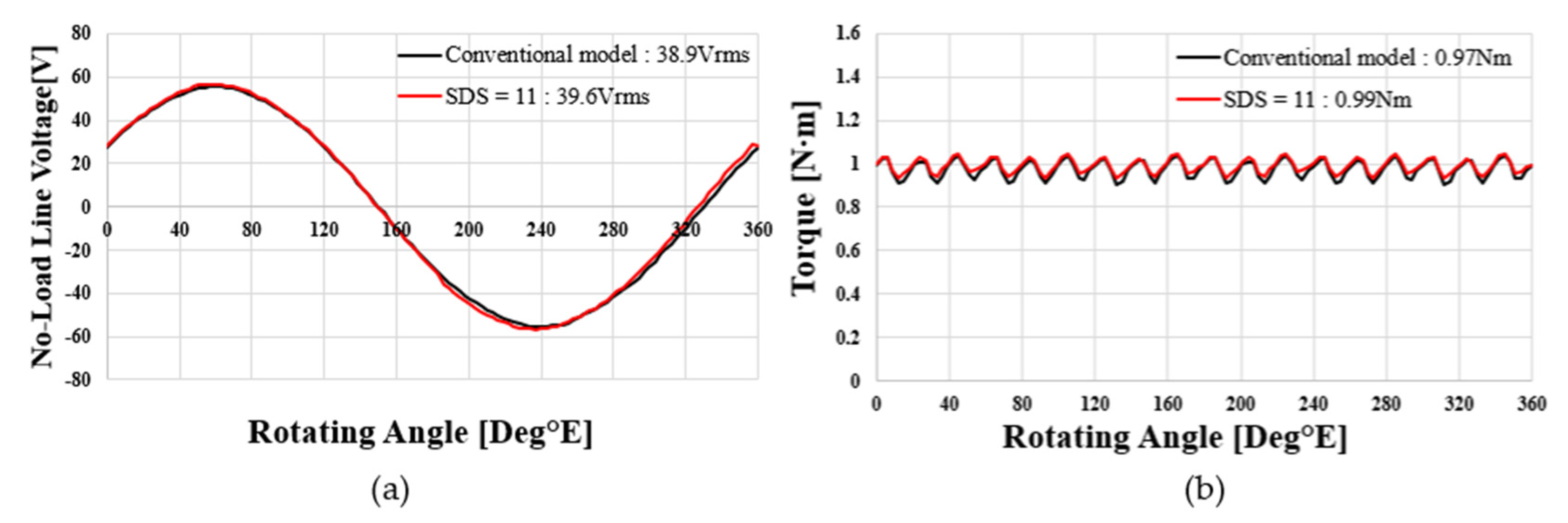
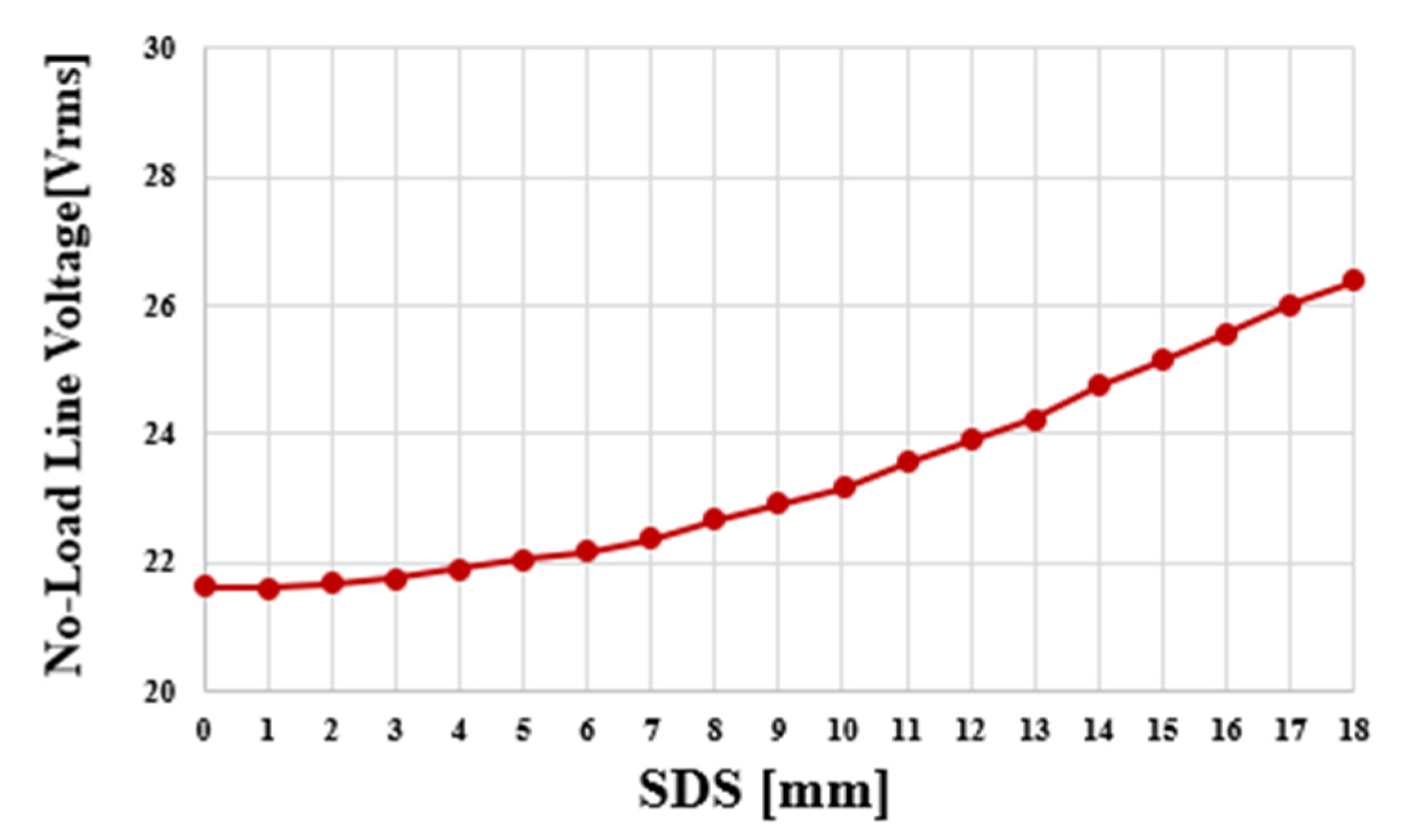
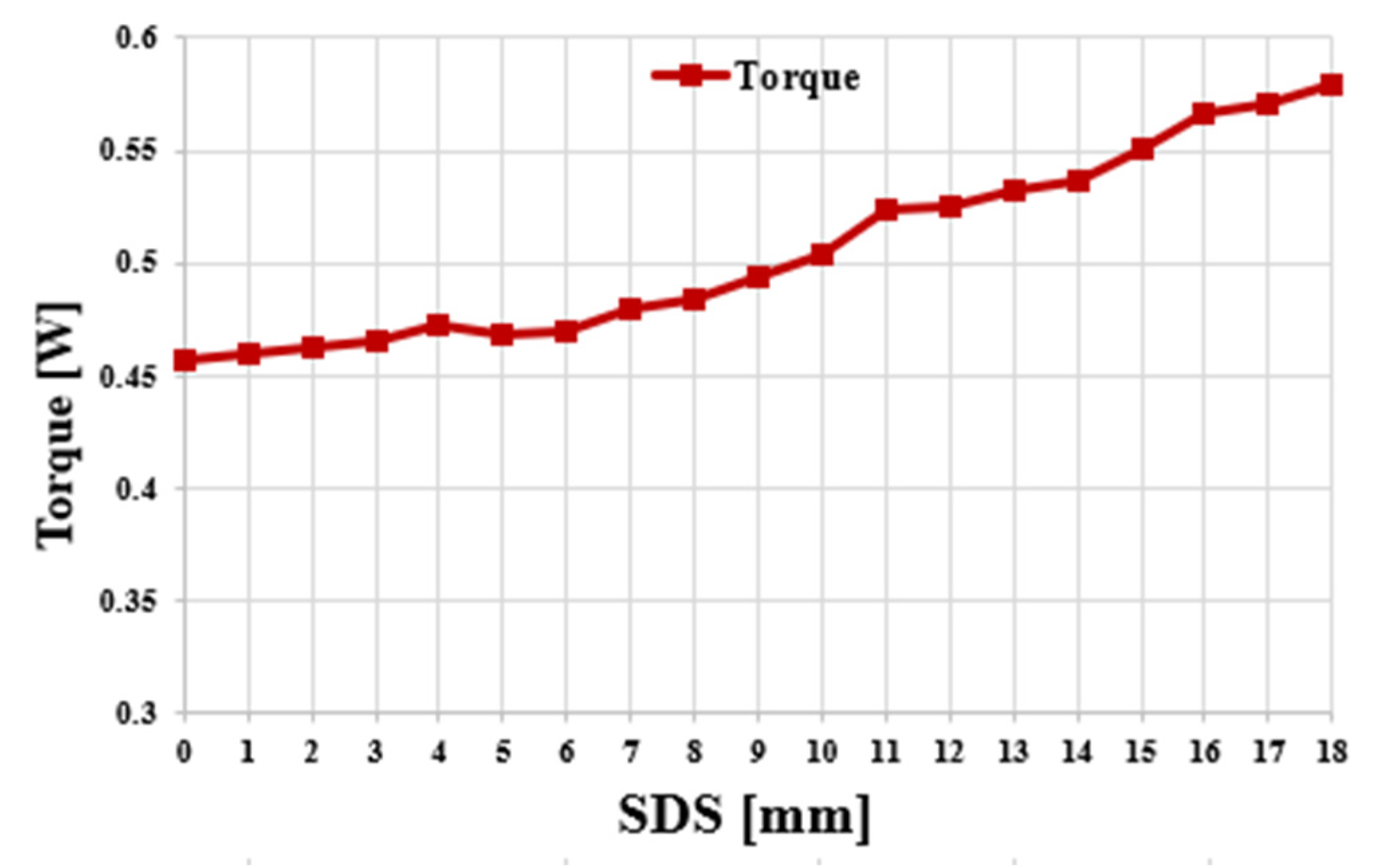
4.2. Numerical Approach to Same-Direction Skew
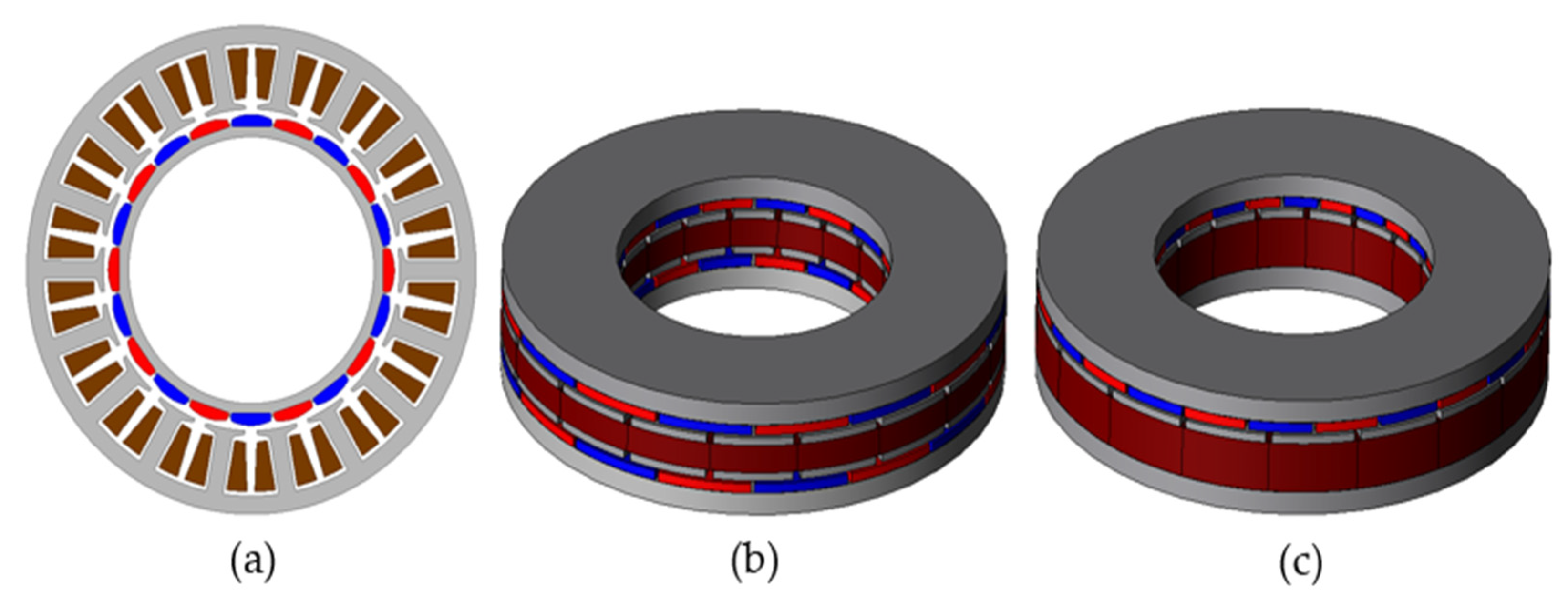
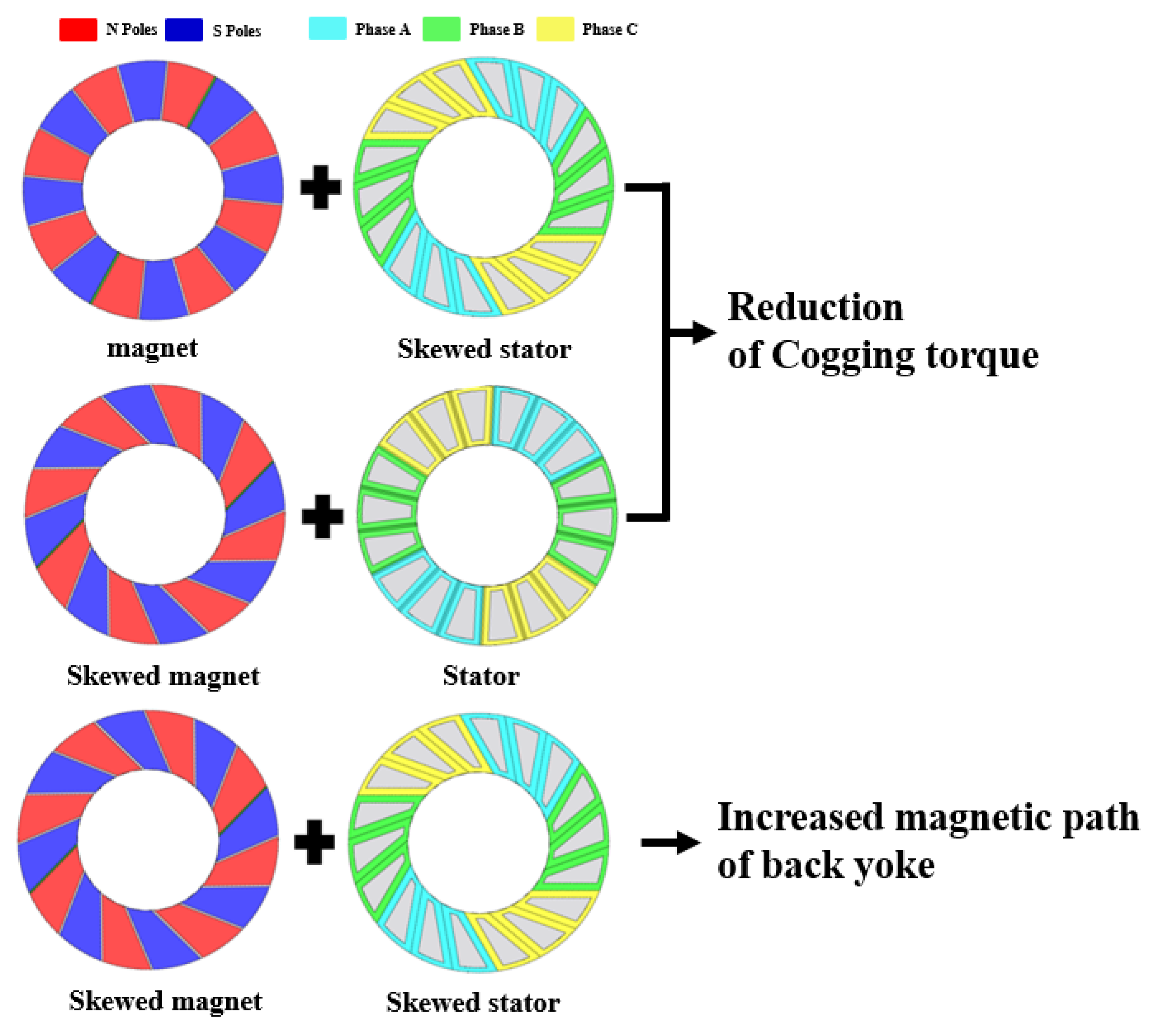
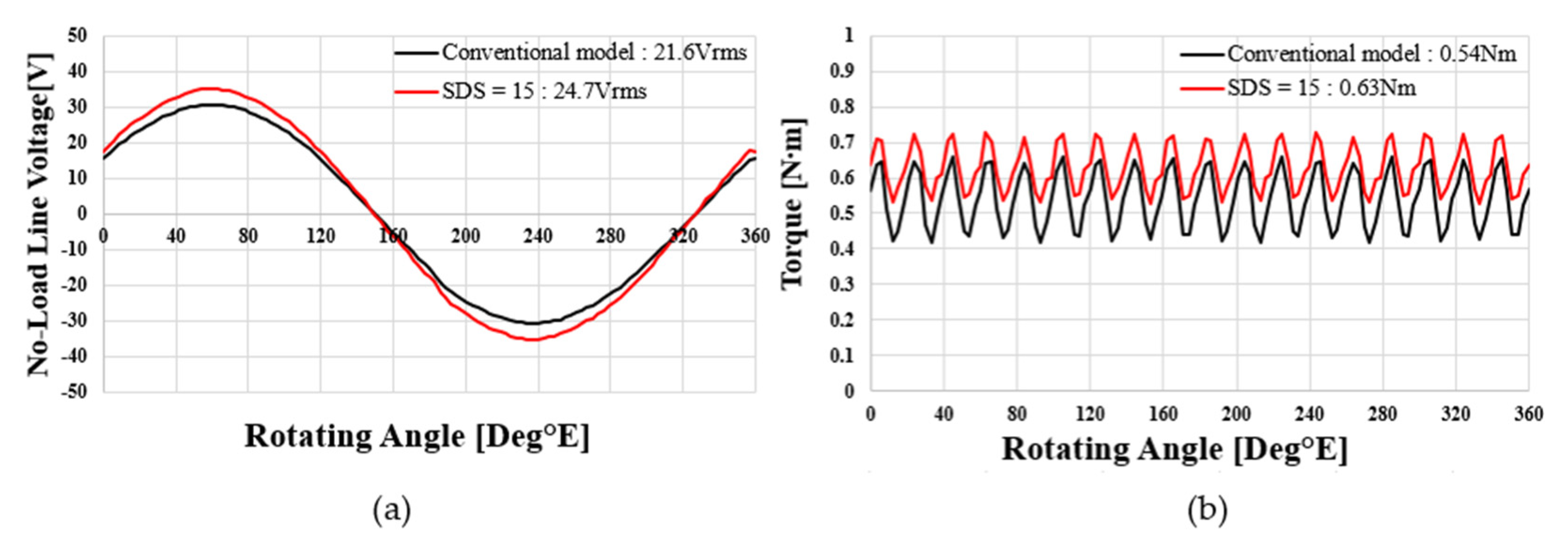
4.3. Same-Direction Skew Effect by Rotor Type
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Jing, L.; Tang, W.; Wang, T.; Ben, T.; Qu, R. Performance Analysis of Magnetically Geared Permanent Magnet Brushless Motor for Hybrid Electric Vehicles. IEEE Trans. Transp. Electrif. 2022, 8, 2874–2883. [Google Scholar] [CrossRef]
- Fang, L.; Li, D.; Qu, R. Torque Improvement of Vernier Permanent Magnet Machine with Larger Rotor Pole Pairs than Stator Teeth Number. IEEE Trans. Ind. Electron. 2023. [Google Scholar] [CrossRef]
- Jing, L.; Liu, W.; Tang, W.; Qu, R. Design and Optimization of Coaxial Magnetic Gear with Double-Layer PMs and Spoke Structure for Tidal Power Generation. IEEE/ASME Trans. Mechatron. 2023. [Google Scholar] [CrossRef]
- Nishanth, F.; Van Verdeghem, J.; Severson, E.L. A Review of Axial Flux Permanent Magnet Machine Technology. IEEE Trans. Ind. Appl. 2023. [Google Scholar] [CrossRef]
- Cavagnino, A.; Lazzari, M.; Profumo, F.; Tenconi, A. A comparison between the axial flux and the radial flux structures for PM synchronous motors. In Proceedings of the Conference Record of the 2001 IEEE Industry Applications Conference: 36th IAS Annual Meeting (Cat. No.01CH37248), Chicago, IL, USA, 30 September–4 October 2001; Volume 3, pp. 1611–1618. [Google Scholar] [CrossRef]
- Bruzinga, G.R.; Filho, A.J.S.; Pelizari, A. Analysis and Design of 3 kW Axial Flux Permanent Magnet Synchronous Motor for Electric Car. IEEE Lat. Am. Trans. 2022, 20, 855–863. [Google Scholar] [CrossRef]
- Ferreira, Â.P.; Costa, A.F. Electromagnetic finite element design of axial flux permanent magnet machines for low speed applications. In Proceedings of the 2014 International Conference on Electrical Machines (ICEM), Berlin, Germany, 2–5 September 2014; pp. 1139–1145. [Google Scholar] [CrossRef]
- Neethu, S.; Nikam, S.P.; Wankhede, A.K.; Pal, S.; Fernandes, B.G. High speed coreless axial flux permanent magnet motor with printed circuit board winding. In Proceedings of the 2017 IEEE Industry Applications Society Annual Meeting, Cincinnati, OH, USA; 2017; pp. 1–6. [Google Scholar] [CrossRef]
- Neethu, S.; Nikam, S.P.; Pal, S.; Wankhede, A.K.; Fernandes, B.G. Performance Comparison Between PCB-Stator and Laminated-Core-Stator-Based Designs of Axial Flux Permanent Magnet Motors for High-Speed Low-Power Applications. IEEE Trans. Ind. Electron. 2020, 67, 5269–5277. [Google Scholar] [CrossRef]
- Chen, Y.; Pillay, P.; Khan, A. PM wind generator topologies. IEEE Trans. Ind. Appl. 2005, 41, 1619–1626. [Google Scholar] [CrossRef]
- Colton, J.; Patterson, D.; Hudgins, J. Design of a low-cost and efficient integrated starter-alternator. In Proceedings of the 2008 4th IET Conference on Power Electronics, Machines and Drives, York, UK, 2–4 April 2008; pp. 357–361. [Google Scholar] [CrossRef]
- Liu, L.; Guo, F.; Zou, Z.; Duffy, V.G. Application, development and future opportunities of collaborative robots (cobots) in manufacturing: A literature review. Int. J. Hum. Comput. Interact. 2022, 1–18. [Google Scholar] [CrossRef]
- Wu, S.-H.; Hong, X.-S. Integrating Computer Vision and Natural Language Instruction for Collaborative Robot Human-Robot Interaction. In Proceedings of the 2020 International Automatic Control Conference (CACS), Hsinchu, Taiwan, 4–7 November 2020; pp. 1–5. [Google Scholar] [CrossRef]
- Hentout, A.; Aouache, M.; Maoudj, A.; Akli, I. Human–robot interaction in industrial collaborative robotics: A literature review of the decade 2008–2017. Adv. Robot. 2019, 33, 764–799. [Google Scholar] [CrossRef]
- Chalmers, B.J.; Spooner, E. An axial-flux permanent-magnet generator for a gearless wind energy system. IEEE Trans. Energy Convers. 1999, 14, 251–257. [Google Scholar] [CrossRef]
- Soderlund, L.; Eriksson, J.-T.; Salonen, J.; Vihriala, H.; Perala, R. A permanent-magnet generator for wind power applications. IEEE Trans. Magn. 1996, 32, 2389–2392. [Google Scholar] [CrossRef]
- Jia, L.; Lin, M.; Le, W.; Li, N.; Kong, Y. Dual-Skew Magnet for Cogging Torque Minimization of Axial Flux PMSM with Segmented Stator. IEEE Trans. Magn. 2020, 56, 7507306. [Google Scholar] [CrossRef]
- Aydin, M.; Zhu, Z.Q.; Lipo, T.A.; Howe, D. Minimization of Cogging Torque in Axial-Flux Permanent-Magnet Machines: Design Concepts. IEEE Trans. Magn. 2007, 43, 3614–3622. [Google Scholar] [CrossRef]
- Kim, D.H.; Choi, J.H.; Son, C.W.; Baek, Y.S. Theoretical analysis and experiments of axial flux pm motors with minimized cogging torque. J. Mech. Sci. Technol. 2009, 23, 335–343. [Google Scholar] [CrossRef]
- Kumar, P.; Srivastava, R.K. Influence of Rotor Magnet Shapes on Performance of Axial Flux Permanent Magnet Machines. Prog. Electromagn. Res. C 2018, 85, 155–165. [Google Scholar] [CrossRef]
- Gieras, J.F.; Wang, R.J.; Kamper, M.J. Axial Flux Permanent Magnet Brushless Machines; Springer Science & Business Media: Dordrecht, The Netherlands, 2008. [Google Scholar]
- Aydin, M.; Huang, S.; Lipo, T.A. Torque quality and comparison of internal and external rotor axial flux surface-magnet disc machines. IEEE Trans. Ind. Electron. 2006, 53, 822–830. [Google Scholar] [CrossRef]




| Dimensions | Conventional Radial Flux Motor | Axial Flux Motor | |
|---|---|---|---|
| Rated output | 200 | 200 | W |
| Rated speed | 3500 | 3500 | rpm |
| Rated current | 5.3 | 5.3 | Arms |
| Pole/slot | 20/18 | 16/18 | - |
| Stator outer/inner diameter | 82/54 | 82/54 | mm |
| Rotor outer/inner diameter | 52.2/44.6 | 82/54 | mm |
| Air gap | 0.6 | 0.6 | mm |
| Stack length | 13 | 19.6 | mm |
| Winding length | 19.6 | 10.6 | mm |
| Permanent magnet | N42SH | N42SH | - |
| Stator core | 35PN230 | Somaloy 700 3P | - |
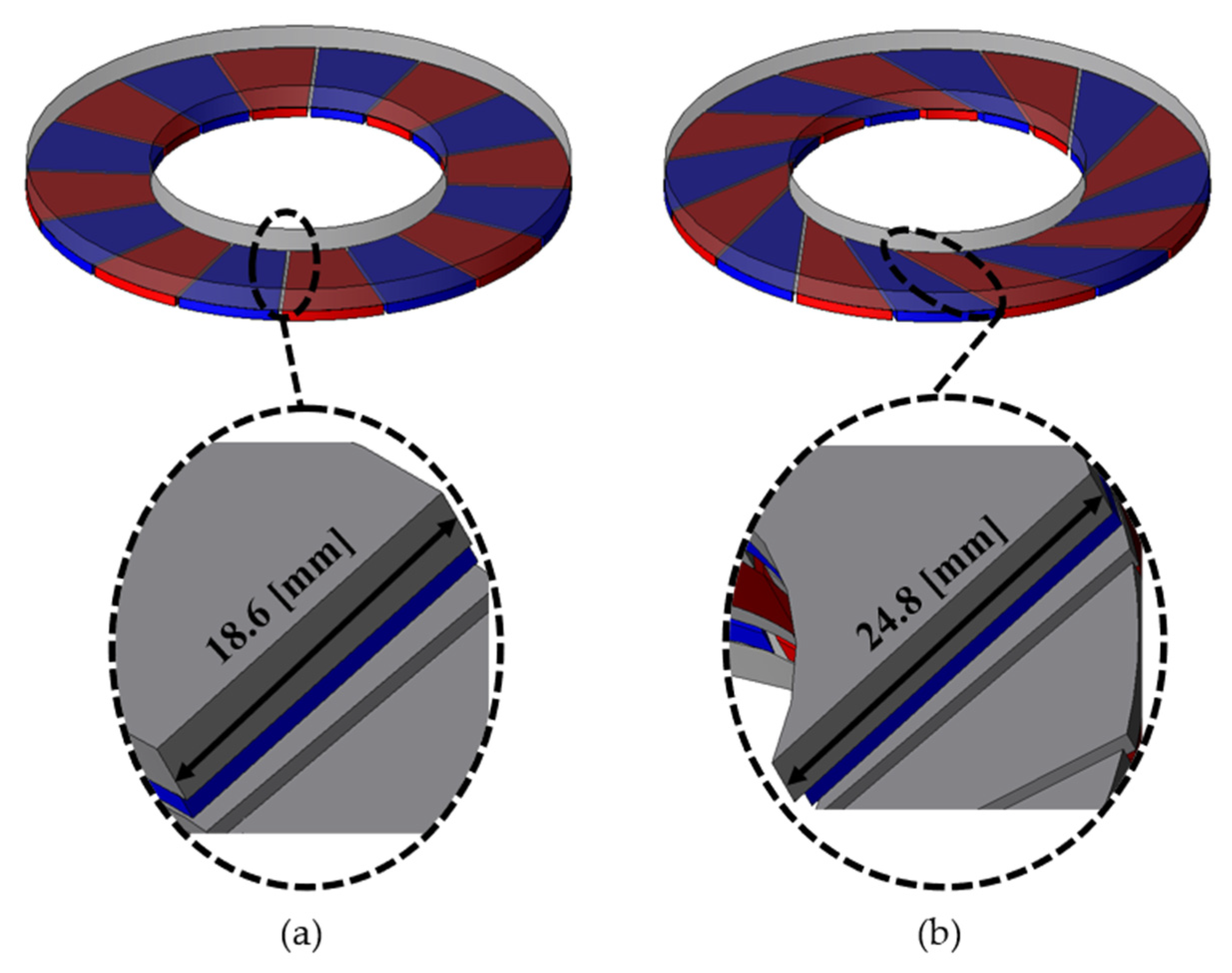
| SDS [mm] | Rotor Back Yoke Cross Section | Double-Type L_Rotor BY [mm] | Single-Type L_Stator BY [mm] | Double-Type L_Teeth [mm] | Single-Type L_Teeth [mm] |
|---|---|---|---|---|---|
| 0 | 81.78 | 3.8 | 2.53 | 4.8 | 9.67 |
| 1 | 3.79 | 2.53 | 4.82 | 9.68 | |
| 2 | 3.78 | 2.52 | 4.84 | 9.7 | |
| 3 | 3.76 | 2.51 | 4.88 | 9.73 | |
| 4 | 3.74 | 2.49 | 4.92 | 9.77 | |
| 5 | 3.72 | 2.48 | 4.96 | 9.8 | |
| 6 | 3.69 | 2.46 | 5.02 | 9.85 | |
| 7 | 3.65 | 2.43 | 5.1 | 9.92 | |
| 8 | 3.61 | 2.41 | 5.18 | 9.98 | |
| 9 | 3.57 | 2.38 | 5.26 | 10.05 | |
| 10 | 3.51 | 2.34 | 5.38 | 10.15 | |
| 11 | 3.45 | 2.3 | 5.5 | 10.25 | |
| 12 | 3.39 | 2.26 | 5.62 | 10.35 | |
| 13 | 3.31 | 2.21 | 5.78 | 10.48 | |
| 14 | 3.23 | 2.15 | 5.94 | 10.62 | |
| 15 | 3.14 | 2.09 | 6.12 | 10.77 | |
| 16 | 3.03 | 2.02 | 6.34 | 10.95 | |
| 17 | 2.91 | 1.94 | 6.58 | 11.15 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hong, M.-K.; Pyo, H.-J.; Song, S.-W.; Jung, D.-H.; Kim, W.-H. A Study on the Improvement of Power Density of Axial Flux Motors for Collaborative Robot Joints through Same-Direction Skew. Machines 2023, 11, 591. https://doi.org/10.3390/machines11060591
Hong M-K, Pyo H-J, Song S-W, Jung D-H, Kim W-H. A Study on the Improvement of Power Density of Axial Flux Motors for Collaborative Robot Joints through Same-Direction Skew. Machines. 2023; 11(6):591. https://doi.org/10.3390/machines11060591
Chicago/Turabian StyleHong, Min-Ki, Hyun-Jo Pyo, Si-Woo Song, Dong-Hoon Jung, and Won-Ho Kim. 2023. "A Study on the Improvement of Power Density of Axial Flux Motors for Collaborative Robot Joints through Same-Direction Skew" Machines 11, no. 6: 591. https://doi.org/10.3390/machines11060591
APA StyleHong, M.-K., Pyo, H.-J., Song, S.-W., Jung, D.-H., & Kim, W.-H. (2023). A Study on the Improvement of Power Density of Axial Flux Motors for Collaborative Robot Joints through Same-Direction Skew. Machines, 11(6), 591. https://doi.org/10.3390/machines11060591







