A Review on the Design and Application of Compliant Mechanism-Based Fast-Tool Servos for Ultraprecision Machining
Abstract
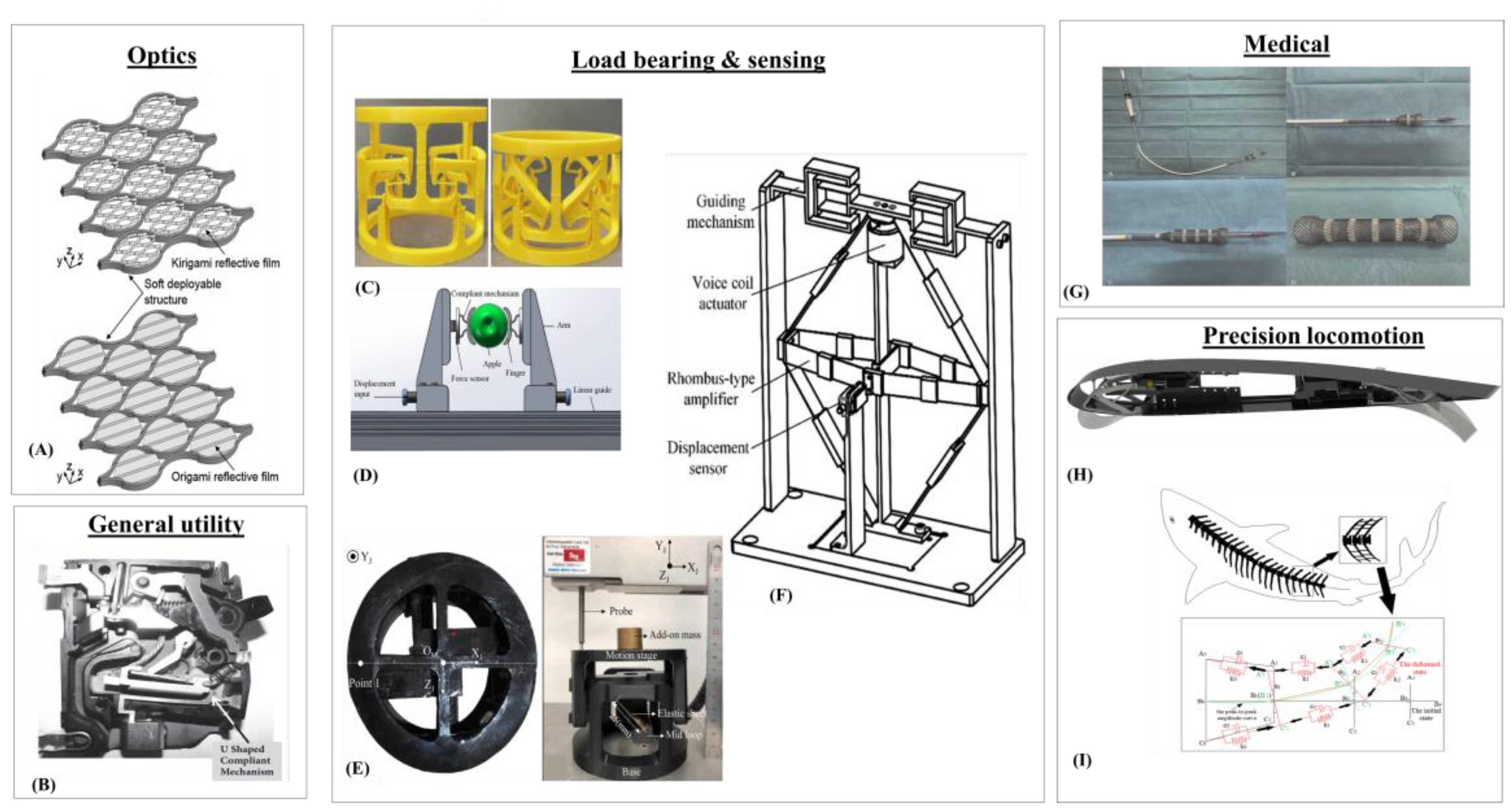
1. Introduction
2. Designing Ultraprecision Capable CMs
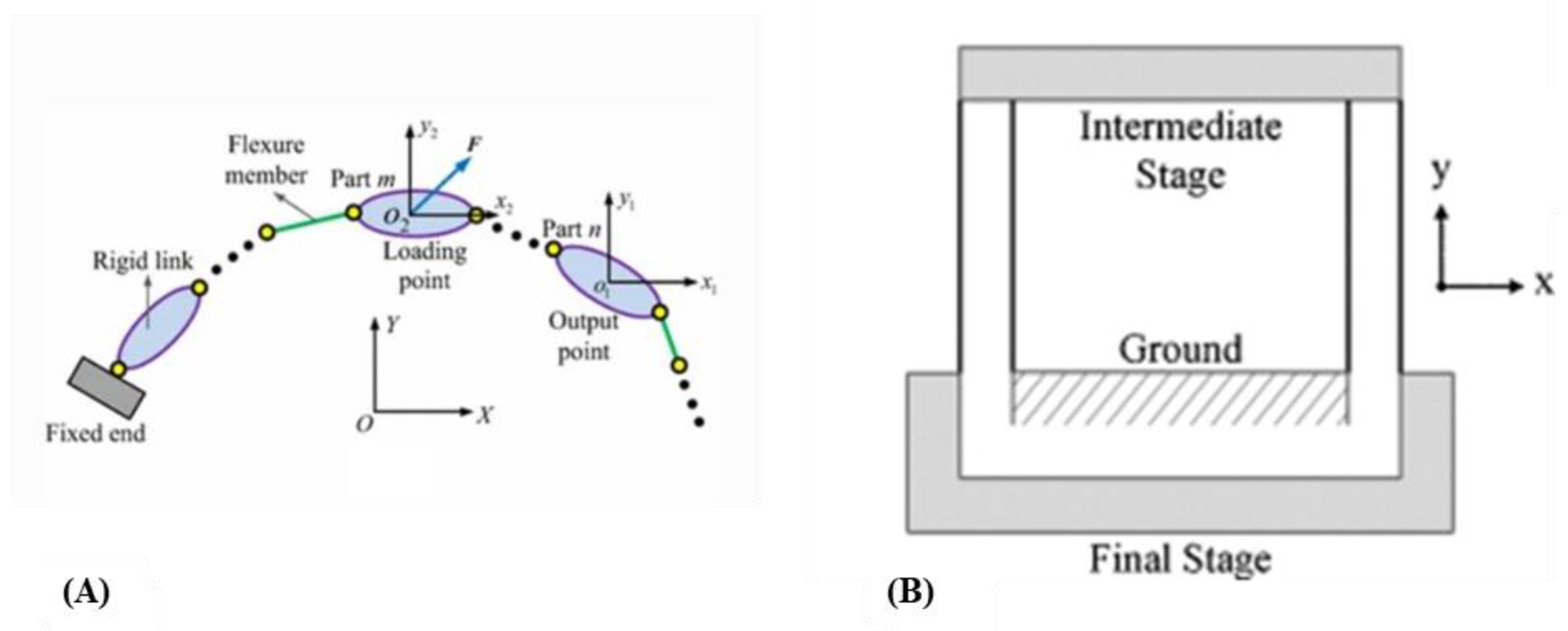
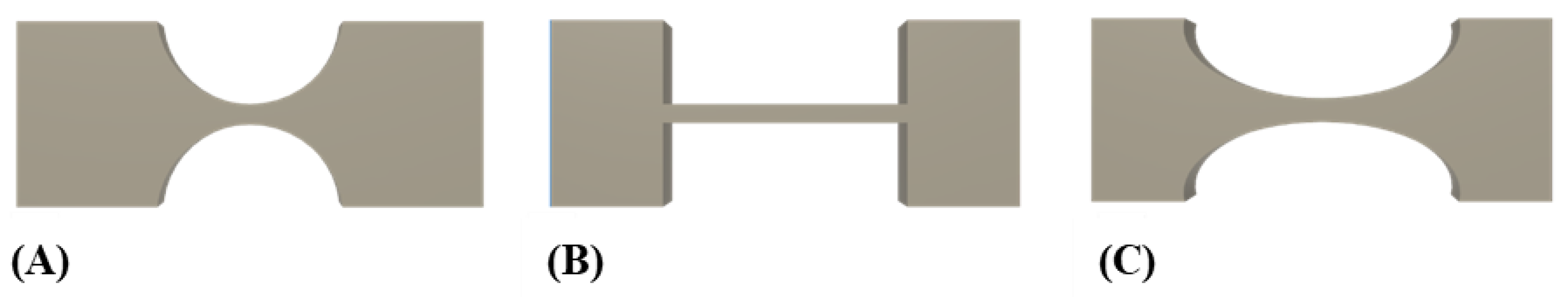
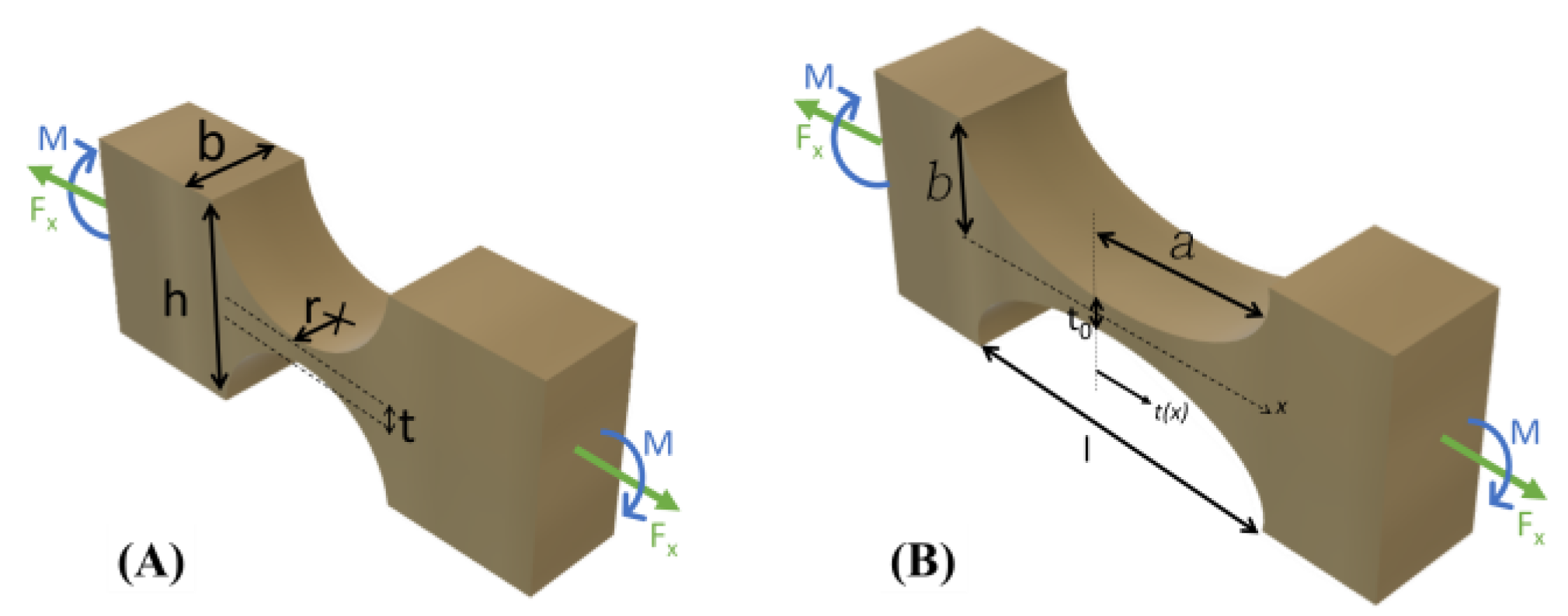
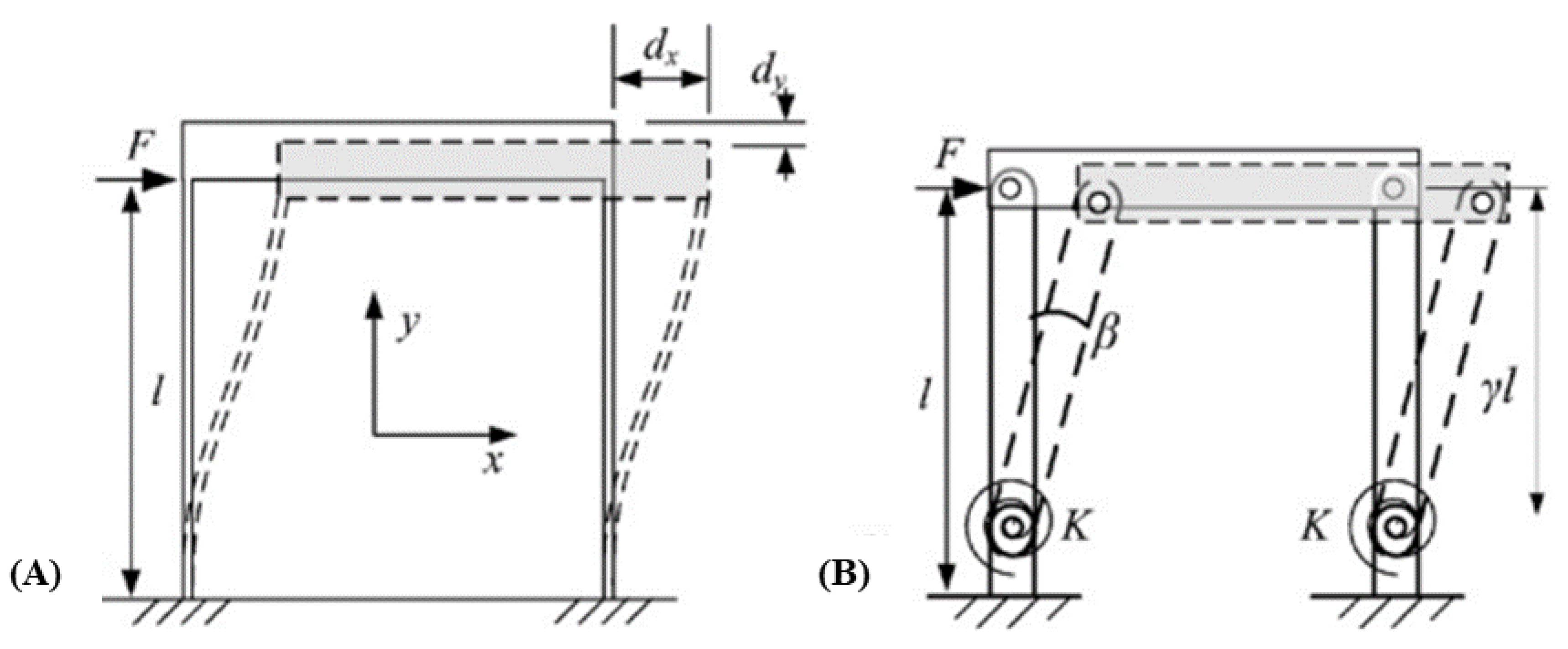
2.1. Fundamental Flexure Hinge Modelling
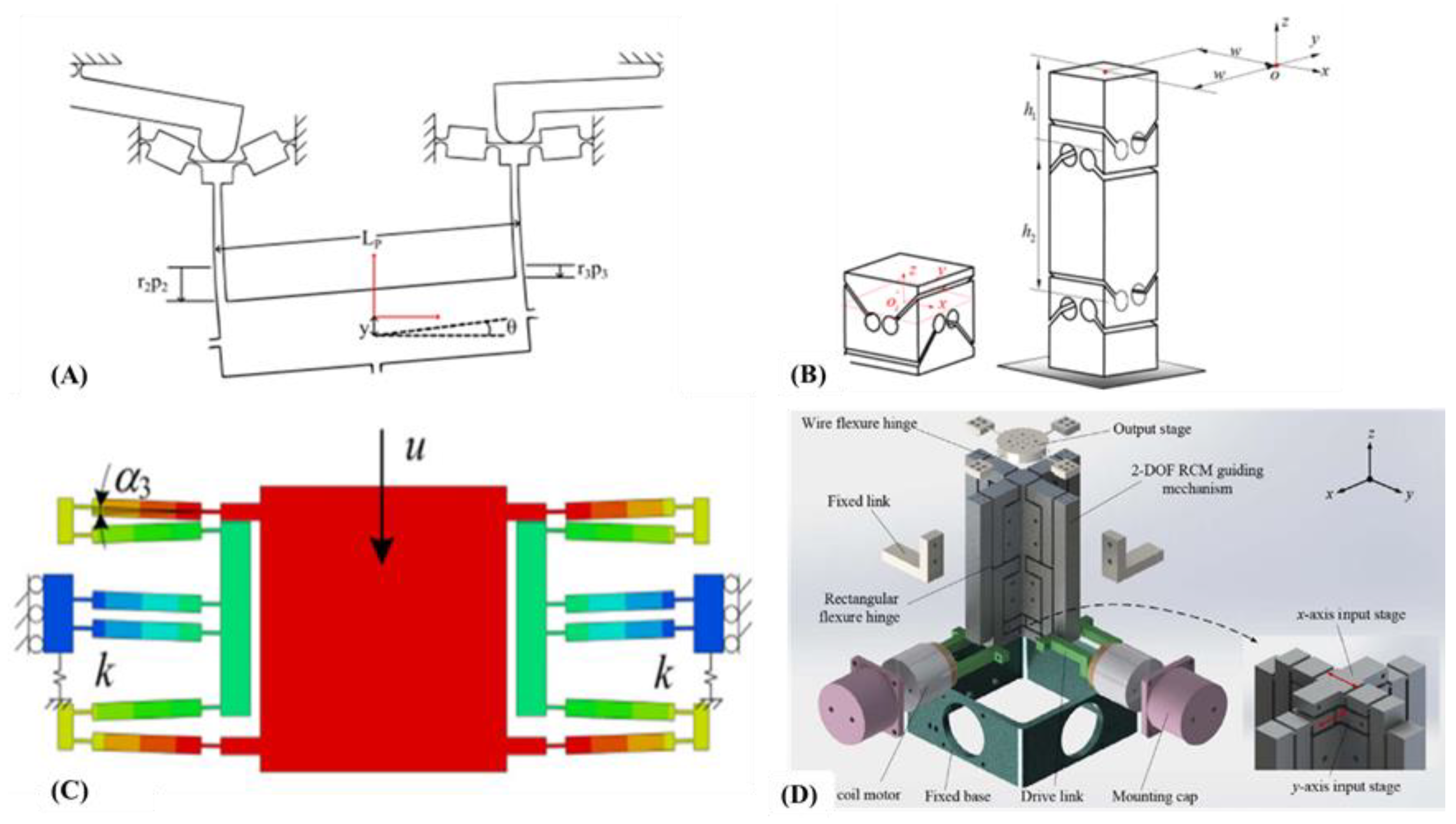
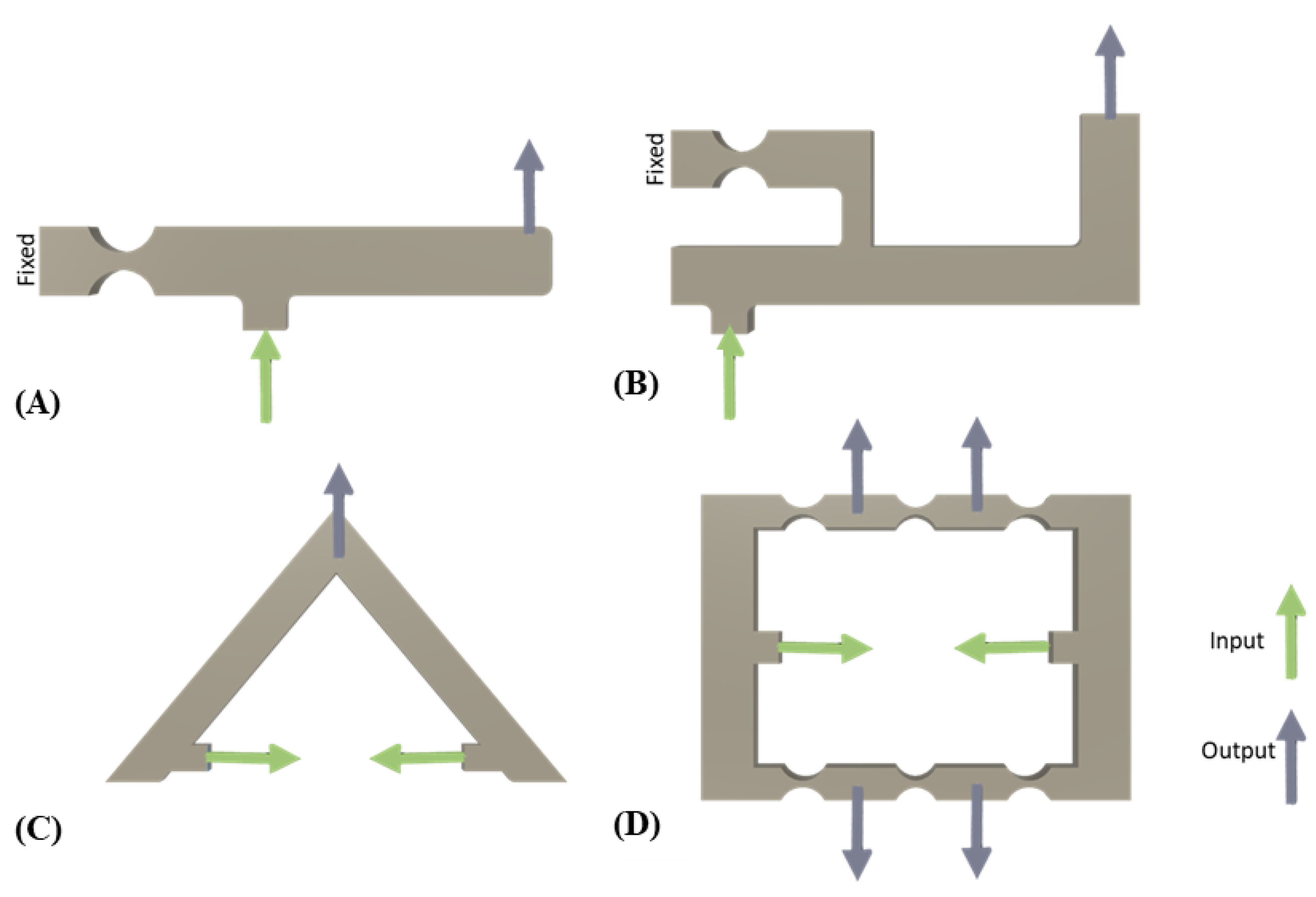
2.2. Motion Decoupling
2.3. Amplification Structures
2.4. Actuators for CM-Based FTSs
2.4.1. Piezoelectric Actuator
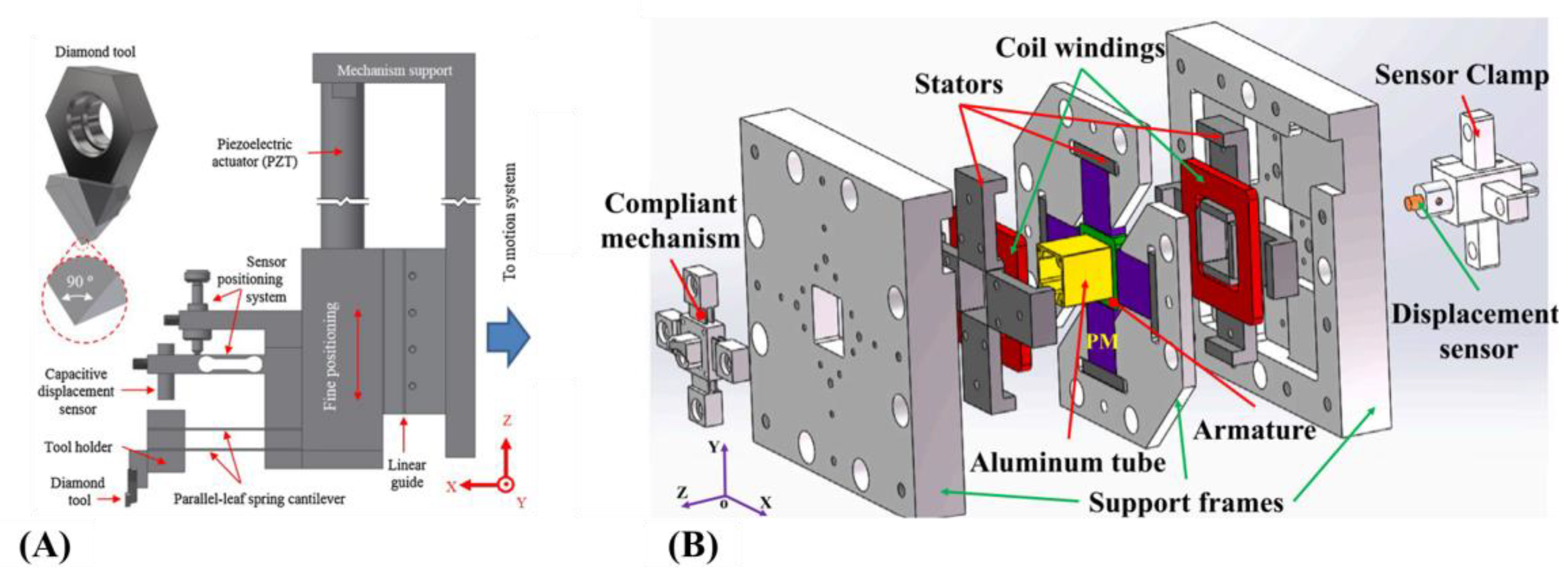
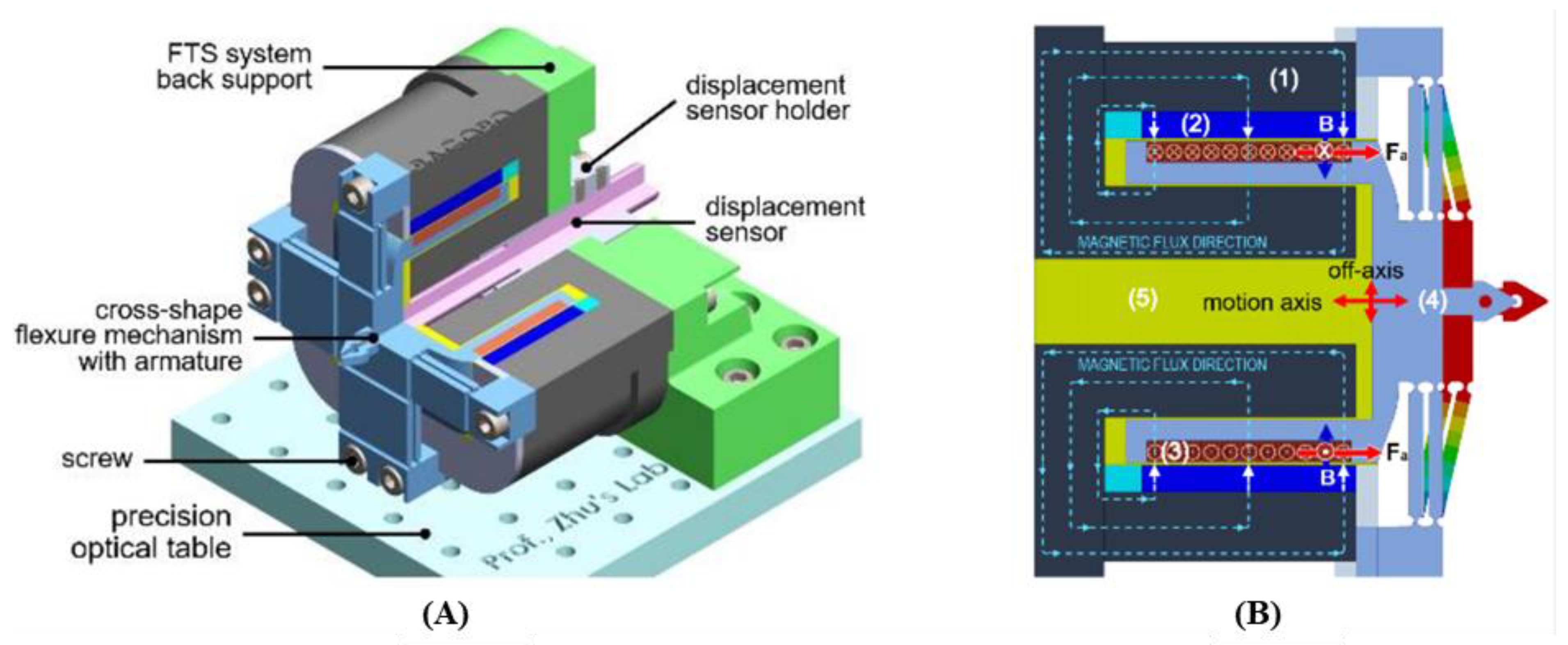
2.4.2. Electromagnetic Types
2.4.3. VCM or Piezoelectric Actuator for Ultraprecision Machining?
2.5. Manufacturing of CM-Based FTSs
2.6. General Design Methodologies
2.6.1. Building Blocks Approach
2.6.2. Freedom and Constraint Topology

2.6.3. Pseudo-Rigid Beam Modelling
2.6.4. Kinematics Approach
2.6.5. Topology Optimization
2.6.6. Data Driven Design Approach
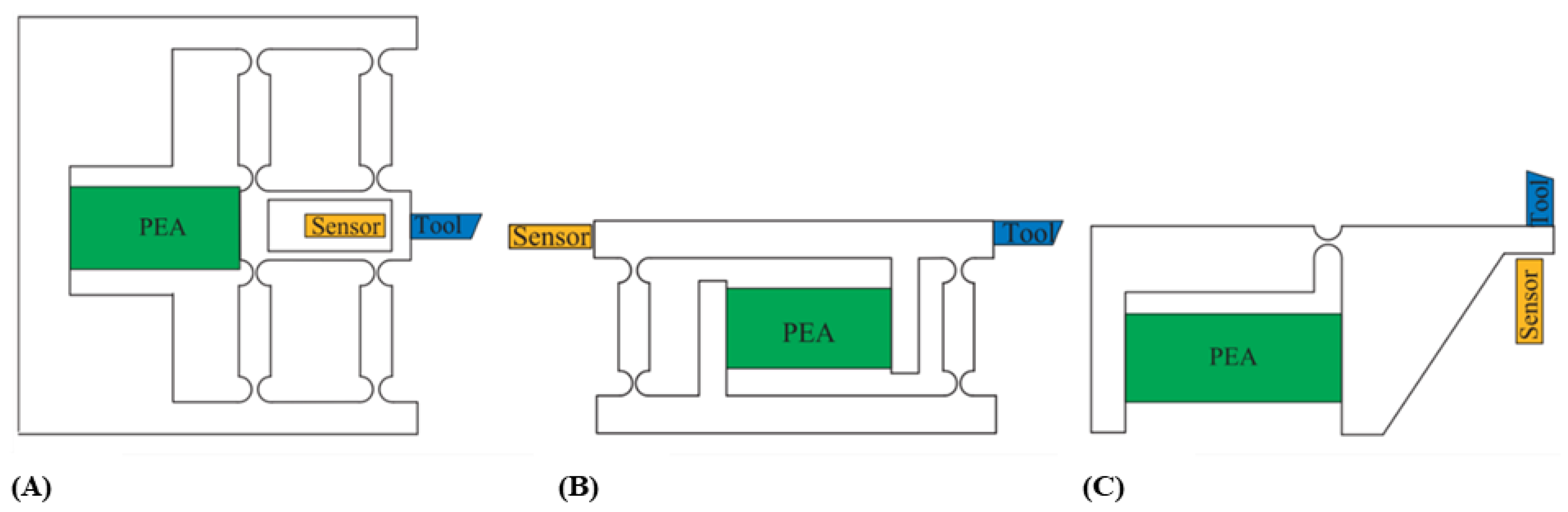
2.7. Actuator Isolation
2.8. Types of CM-Based FTSs
2.8.1. 1DOF FTS
2.8.2. Long Stroke FTSs
2.8.3. Multiple DOF FTS
2.8.4. Swing Output FTS
2.8.5. Self-Sensing FTS
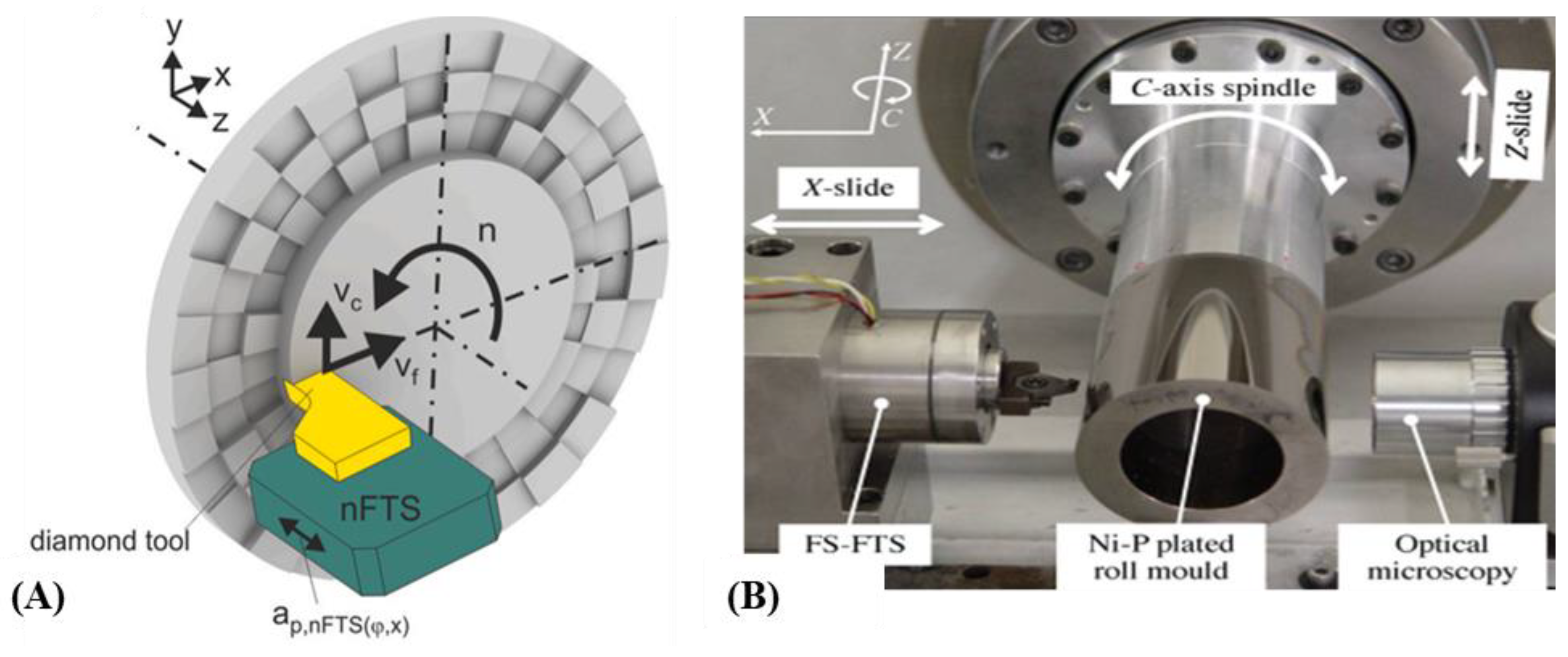
3. FTS Machining Performance Affecting Factors
3.1. Machining Parameters
3.2. CM Structural Stiffness
3.3. Dynamic Characteristics
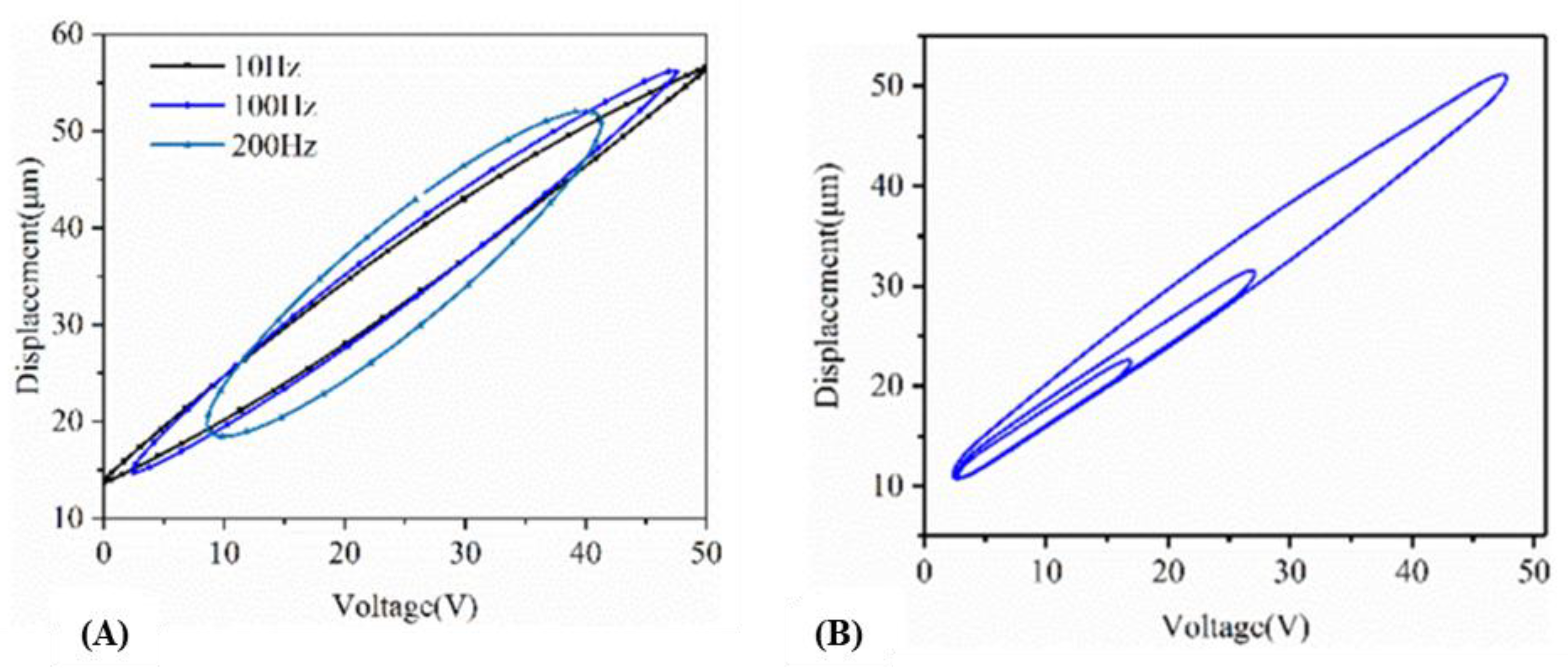
3.4. Hysteresis
3.5. Fatigue
3.6. Thermal
4. Future Research
4.1. Towards Data-Driven Design Approach
4.2. Machining of Hierarchical Structured Surfaces with CM-Based Tools

4.3. Multimaterial FTSs
4.4. Integration of Innovative Flexure Hinges
4.5. CM Design Based on Automated Feature Identification
4.6. CAD/CAM Integration
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Boni, C.; Royer-Carfagni, G. A New Flexural-Tensegrity Bow. Mech. Mach. Theory 2021, 164, 104398. [Google Scholar] [CrossRef]
- Howell, L.L.; Magleby, S.P.; Olsen, B.M. Handbook of Compliant Mechanisms; John Wiley & Sons: Hoboken, NJ, USA, 2013. [Google Scholar] [CrossRef]
- Marathe, P.; Pardeshi, S.S.; Deshmukh, B. Development of Bridge and Lever Type Compact Compliant Mechanism for Micro Positioning Systems. J. Phys. Conf. Ser. 2021, 1969, 12006. [Google Scholar] [CrossRef]
- Kirmse, S.; Campanile, L.F.; Hasse, A. Synthesis of Compliant Mechanisms with Selective Compliance-An Advanced Procedure. Mech. Mach. Theory 2021, 157, 104184. [Google Scholar] [CrossRef]
- Liang, Y.; Sun, K.; Cheng, G. Discrete Variable Topology Optimization for Compliant Mechanism Design via Sequential Approximate Integer Programming with Trust Region (SAIP-TR). Struct. Multidiscip. Optim. 2020, 62, 2851–2879. [Google Scholar] [CrossRef]
- Olesnavage, K.M.; Johnson, W.B.; Professor, M.J.M.; Winter, A.G. Design and Testing of a Prosthetic Foot with Interchangeable Custom Springs for Evaluating Lower Leg Trajectory Error, an Optimization Metric for Prosthetic Feet. J. Mech. Robot. 2018, 10, 021010. [Google Scholar] [CrossRef]
- Miao, Y.; Zheng, J. Optimization Design of Compliant Constant-Force Mechanism for Apple Picking Actuator. Comput. Electron. Agric. 2020, 170, 105232. [Google Scholar] [CrossRef]
- Zhang, Y.; Ge, W.; Zhang, Z.; Mo, X.; Zhang, Y. Design of Compliant Mechanism-Based Variable Camber Morphing Wing with Nonlinear Large Deformation. Int. J. Adv. Robot. Syst. 2019, 16, 1–19. [Google Scholar] [CrossRef]
- Ahuett-Garza, H.; Melecio, J.I.; Orta, P. Modal Analysis of a New Thermosensitive Actuator Design for Circuit Breakers Based on Mesoscale U-Shaped Compliant Mechanisms. Math. Probl. Eng. 2018, 2018, 2850309. [Google Scholar] [CrossRef]
- Hansen, B.J.; Carron, C.J.; Jensen, B.D.; Hawkins, A.R.; Schultz, S.M. Plastic Latching Accelerometer Based on Bistable Compliant Mechanisms. Smart Mater. Struct. 2007, 16, 1967–1972. [Google Scholar] [CrossRef]
- Wang, W.; Li, C.; Rodrigue, H.; Yuan, F.; Han, M.W.; Cho, M.; Ahn, S.H. Kirigami/Origami-Based Soft Deployable Reflector for Optical Beam Steering. Adv. Funct. Mater. 2017, 27, 1604214. [Google Scholar] [CrossRef]
- Cui, Z.; Jiang, H. Design, Analysis, and Simulation of a Planar Serial-Parallel Mechanism for a Compliant Robotic Fish with Variable Stiffness. Res. Artic. Adv. Mech. Eng. 2016, 8, 1–13. [Google Scholar] [CrossRef]
- Liu, T.; Hao, G. Design of Deployable Structures by Using Bistable Compliant Mechanisms. Micromachines 2022, 13, 651. [Google Scholar] [CrossRef] [PubMed]
- Li, S.; Hao, G. Design and Nonlinear Spatial Analysis of Compliant Anti-Buckling Universal Joints. Int. J. Mech. Sci. 2022, 219, 107111. [Google Scholar] [CrossRef]
- Li, Y.; Zhu, X.; Bi, S.; Guo, R.; Sun, J.; Hu, W. Design and Development of Compliant Mechanisms for Electromagnetic Force Balance Sensor. Precis. Eng. 2020, 64, 157–164. [Google Scholar] [CrossRef]
- Wiese, M.S.; Dratsch, T.; Plum, P.S.; Lorenz, F.; Rieck, I.; Pinto Dos Santos, D.; Alakus, H.; Bludau, M.; Kleinert, R.; Goeser, T.; et al. Palliation of Malignant Dysphagia with a Segmented Self-Expanding Metal Stent: A STROBE-Compliant Article. Medicine 2021, 100, e27052. [Google Scholar] [CrossRef]
- Taniguchi, N. Current Status in, and Future Trends of, Ultraprecision Machining and Ultrafine Materials Processing. CIRP Ann. 1983, 32, 573–582. [Google Scholar] [CrossRef]
- Khaghani, A.; Cheng, K. Investigation on an Innovative Approach for Clamping Contact Lens Mould Inserts in Ultraprecision Machining Using an Adaptive Precision Chuck and Its Application Perspectives. Int. J. Adv. Manuf. Technol. 2020, 111, 839–850. [Google Scholar] [CrossRef]
- Chen, S.; Xue, S.; Zhai, D.; Tie, G. Measurement of Freeform Optical Surfaces: Trade-Off between Accuracy and Dynamic Range. Laser Photon. Rev. 2020, 14, 1900365. [Google Scholar] [CrossRef]
- Yang, T.; Jin, G.F.; Zhu, J. Automated Design of Freeform Imaging Systems. Light Sci. Appl. 2017, 6, e17081. [Google Scholar] [CrossRef]
- Brinksmeier, E.; Gläbe, R.; Schönemann, L. Diamond Micro Chiseling of Large-Scale Retroreflective Arrays. Precis. Eng. 2012, 36, 650–657. [Google Scholar] [CrossRef]
- Huang, R.; Zhang, X.; Neo, W.K.; Kumar, A.S.; Liu, K. Ultra-Precision Machining of Grayscale Pixelated Micro Images on Metal Surface. Precis. Eng. 2018, 52, 211–220. [Google Scholar] [CrossRef]
- Zhang, X.; Huang, R.; Liu, K.; Kumar, A.S.; Shan, X. Rotating-Tool Diamond Turning of Fresnel Lenses on a Roller Mold for Manufacturing of Functional Optical Film. Precis. Eng. 2018, 51, 445–457. [Google Scholar] [CrossRef]
- Neo, D.W.K.; Kumar, A.S.; Rahman, M. An Automated Guilloche Machining Technique for the Fabrication of Polygonal Fresnel Lens Array. Precis. Eng. 2015, 41, 55–62. [Google Scholar] [CrossRef]
- Jin, N.T.Y.; Jin, N.T.Y.; Lim, Z.H.; Zhou, G.; Liu, K.; Kumar, A.S. Design and Fabrication of Composite Polygonal Fresnel Lenses. Opt. Express 2021, 29, 36516–36534. [Google Scholar] [CrossRef]
- Wu, B.; Zong, W. A Modified Diamond Micro Chiseling Method for Machining Large Scale Retroreflective Microstructure on Nickel Phosphorus Alloy. J. Mater. Process. Technol. 2022, 307, 117676. [Google Scholar] [CrossRef]
- Zhang, X.Q.; Huang, R.; Kumar, A.S.; Liu, K. High-Efficiency Swinging-Rotating Diamond Shaping of Fresnel Lenses on Roller Molds. CIRP Ann. 2018, 67, 121–124. [Google Scholar] [CrossRef]
- Chen, Z.Z.; Chen, J.N.; Zhang, X.Q.; Zhu, L.M. Fabrication of Polygonal Fresnel Lenses with a Rotating Cutting Tool on Three-Axis Ultraprecision Lathes. Precis. Eng. 2022, 76, 385–396. [Google Scholar] [CrossRef]
- Zhou, H.X.; Tan, K.K. Speed Non-Circular Piston Turning Using Extracted Computer Numerical Control (CNC) Approach High. In Proceedings of the 2004 IEEE Region 10 Conference TENCON, Chiang Mai, Thailand, 24–24 November 2004. [Google Scholar] [CrossRef]
- Wang, H.; Yang, S. Design and Control of a Fast Tool Servo Used in Noncircular Piston Turning Process. Mech. Syst. Signal Process. 2011, 36, 87–94. [Google Scholar] [CrossRef]
- Boinski, A.K.; Riemer, O.; Karpuschewski, B.; Schneider, M.; Guttmann, M.; Worgull, M. Fast Tool Machining and Hot Embossing for the Manufacture of Diffractive Structured Surfaces. Precis. Eng. 2022, 74, 12–19. [Google Scholar] [CrossRef]
- Chen, Y.-L.; Wang, S.; Shimizu, Y.; Ito, S.; Gao, W.; Ju, B.-F. An In-Process Measurement Method for Repair of Defective Microstructures by Using a Fast Tool Servo with a Force Sensor. Precis. Eng. 2015, 39, 134–142. [Google Scholar] [CrossRef]
- Ju, B.F.; Zhu, W.L.; Yang, S.; Yang, K. Scanning Tunneling Microscopy-Based in Situ Measurement of Fast Tool Servo-Assisted Diamond Turning Micro-Structures. Meas. Sci. Technol. 2014, 25, 55004. [Google Scholar] [CrossRef]
- Ping, D.; Gan, S.W.; Wong, Y.S.; Hong, G.S.; Mustafizur, R.; Yao, J. Optimized Tool Path Generation for Fast Tool Servo Diamond Turning of Micro-Structured Surfaces. Int. J. Adv. Manuf. Technol. 2012, 63, 1137–1152. [Google Scholar] [CrossRef]
- Zhang, H.; Dong, G.; Zhou, M.; Song, C.; Huang, Y.; Du, K. A New Variable Structure Sliding Mode Control Strategy for FTS in Diamond-Cutting Microstructured Surfaces. Int. J. Adv. Manuf. Technol. 2012, 65, 1177–1184. [Google Scholar] [CrossRef]
- Ludwick, S.J.; Chargin, D.A.; Calzaretta, J.A.; Trumper, D.L. Design of a Rotary Fast Tool Servo for Ophthalmic Lens Fabrication. Precis. Eng. 1999, 23, 253–259. [Google Scholar] [CrossRef]
- Li, D.; Wang, B.O.; Qiao, Z.; Jiang, X. Ultraprecision Machining of Microlens Arrays with Integrated On-Machine Surface Metrology. Opt. Express 2019, 27, 212–224. [Google Scholar] [CrossRef]
- Keeratihattayakorn, S.; Tangpornprasert, P.; Prasongcharoen, W.; Virulsri, C. Out-of-Roundness Compensation Technique in Machining of Femoral Head Prosthesis Using Conventional CNC Machine. Int. J. Adv. Manuf. Technol. 2020, 107, 2537–2545. [Google Scholar] [CrossRef]
- Wee, D.; Neo, K.; Kumar, A.S.; Rahman, M. A Novel Surface Analytical Model for Cutting Linearization Error in Fast Tool/Slow Slide Servo Diamond Turning. Precis. Eng. 2014, 38, 849–860. [Google Scholar] [CrossRef]
- Zhu, L.; Li, Z.; Fang, F.; Huang, S.; Zhang, X. Review on Fast Tool Servo Machining of Optical Freeform Surfaces. Int. J. Adv. Manuf. Technol. 2018, 95, 2071–2092. [Google Scholar] [CrossRef]
- Gong, Z.; Huo, D.; Niu, Z.; Chen, W.; Cheng, K. Robustness Evaluation of Control Algorithms for a Long-Stroke Fast Tool Servo. J. Manuf. Process. 2022, 80, 458–468. [Google Scholar] [CrossRef]
- Brinksmeier, E.; Karpuschewski, B.; Yan, J.; Schönemann, L. Manufacturing of Multiscale Structured Surfaces. CIRP Ann. 2020, 69, 717–739. [Google Scholar] [CrossRef]
- Brinksmeier, E.; Glä, R.; Schö, L. Review on Diamond-Machining Processes for the Generation of Functional Surface Structures. CIRP J. Manuf. Sci. Technol. 2012, 5, 1–7. [Google Scholar] [CrossRef]
- Zhang, S.; Zhou, Y.; Zhang, H.; Xiong, Z.; To, S. Advances in Ultra-Precision Machining of Micro-Structured Functional Surfaces and Their Typical Applications. Int. J. Mach. Tools Manuf. 2019, 142, 16–41. [Google Scholar] [CrossRef]
- Brigham Young University, Compliant Mechanisms Research. About Compliant Mechanisms|Compliant Mechanisms. Available online: https://www.compliantmechanisms.byu.edu/about-compliant-mechanisms (accessed on 21 March 2023).
- Tian, Y.; Shirinzadeh, B.; Zhang, D.; Zhong, Y. Three Flexure Hinges for Compliant Mechanism Designs Based on Dimensionless Graph Analysis. Precis. Eng. 2010, 34, 92–100. [Google Scholar] [CrossRef]
- Dong, W.; Sun, L.; Du, Z. Stiffness Research on a High-Precision, Large-Workspace Parallel Mechanism with Compliant Joints. Precis. Eng. 2008, 32, 222–231. [Google Scholar] [CrossRef]
- Wang, L.-P.; Jiang, Y.; Li, T.-M. Analytical Compliance Modeling of Serial Flexure-Based Compliant Mechanism Under Arbitrary Applied Load. Chin. J. Mech. Eng. 2017, 30, 951–962. [Google Scholar] [CrossRef]
- Panas, R.M. Large Displacement Behavior of Double Parallelogram Flexure Mechanisms with Underconstraint Eliminators. Precis. Eng. 2016, 46, 399–408. [Google Scholar] [CrossRef]
- Aravind Raghavendra, M.R.; Senthil Kumar, A.; Nikhil Jagdish, B. Design and Analysis of Flexure-Hinge Parameter in Microgripper. Int. J. Adv. Manuf. Technol. 2010, 49, 1185–1193. [Google Scholar] [CrossRef]
- Smith, S.T. Flexures: Elements of Elastic Mechanisms. In Flexures; CRC Press: London, UK, 2000. [Google Scholar] [CrossRef]
- Shao, J.Y.; Ding, Y.C.; Liu, H.Z.; Li, X.M.; Tian, H.M.; Xiao, B.H. Monolithic Design of a Compliant Template Orientation Stage for Step Imprint Lithography. Proc. Inst. Mech. Eng. Part B J. Eng. Manuf. 2011, 226, 221–228. [Google Scholar] [CrossRef]
- Li, Y.; Xu, Q. A Totally Decoupled Piezo-Driven XYZ Flexure Parallel Micropositioning Stage for Micro/Nanomanipulation. IEEE Trans. Autom. Sci. Eng. 2011, 8, 265–279. [Google Scholar] [CrossRef]
- Shang, J.; Tian, Y.; Li, Z. A Novel Voice Coil Motor-Driven Compliant Micropositioning Stage Based on Flexure Mechanism. Rev. Sci. Instrum. 2015, 86, 95001. [Google Scholar] [CrossRef]
- Bhagat, U.; Shirinzadeh, B.; Clark, L.; Chea, P.; Qin, Y.; Tian, Y.; Zhang, D. Design and Analysis of a Novel Flexure-Based 3-DOF Mechanism. Mech. Mach. Theory 2013, 74, 173–187. [Google Scholar] [CrossRef]
- Wang, F.; Zhao, X.; Huo, Z.; Shi, B.; Liang, C.; Tian, Y.; Zhang, D. A 2-DOF Nano-Positioning Scanner with Novel Compound Decoupling-Guiding Mechanism. Mech. Mach. Theory 2021, 155, 104066. [Google Scholar] [CrossRef]
- Chen, W.; Chen, S.; Qu, J.; Chen, W. A Large-Range Compliant Remote Center of Motion Stage with Input/Output Decoupling. Precis. Eng. 2017, 51, 468–480. [Google Scholar] [CrossRef]
- Xu, Q.; Li, Y. Analytical Modeling, Optimization and Testing of a Compound Bridge-Type Compliant Displacement Amplifier. Mech. Mach. Theory 2011, 46, 183–200. [Google Scholar] [CrossRef]
- Chen, W.; Qu, J.; Chen, W.; Zhang, J. A Compliant Dual-Axis Gripper with Integrated Position and Force Sensing. Mechatronics 2017, 47, 105–115. [Google Scholar] [CrossRef]
- Li, Y.; Ge, W.; Zhang, X.; Tong, X. Optimization and Experiment of a Novel Compliant Focusing Mechanism for Space Remote Sensor. Sensors 2020, 20, 6826. [Google Scholar] [CrossRef]
- Ai, W.; Xu, Q. New Structural Design of a Compliant Gripper Based on the Scott-Russell Mechanism Regular Paper. Int. J. Adv. Robot. Syst. 2014, 11, 192. [Google Scholar] [CrossRef]
- Jiang, J.; Mockensturm, E. A Motion Amplifier Using an Axially Driven Buckling Beam: I. Design and Experiments. Nonlinear Dyn. 2006, 43, 391–409. [Google Scholar] [CrossRef]
- Dong, W.; Chen, F.; Gao, F.; Yang, M.; Sun, L.; Du, Z.; Tang, J.; Zhang, D. Development and Analysis of a Bridge-Lever-Type Displacement Amplifier Based on Hybrid Flexure Hinges. Precis. Eng. 2018, 54, 171–181. [Google Scholar] [CrossRef]
- Sosnicki, O.; Pages, A.; Pacheco, C.; Maillard, T. Servo Piezo Tool SPT400MML for the Fast and Precise Machining of Free Forms. Int. J. Adv. Manuf. Technol. 2009, 47, 903–910. [Google Scholar] [CrossRef]
- Liu, H.; Sun, Y.; Zhu, B.; Hu, Y.; Xie, W. Inertial Force Control and Balance Error Analysis of Fast Tool Servo Based on the Voice Coil Motor. In Proceedings of the 2014 20th International Conference on Automation and Computing, Cranfield, UK, 12–13 September 2014; pp. 243–247. [Google Scholar] [CrossRef]
- Gutierrez, H.M.; Ro, P.I. Sliding-Mode Control of a Nonlinear-Input System: Application to a Magnetically Levitated Fast-Tool Servo. IEEE Trans. Ind. Electron. 1998, 45, 921–927. [Google Scholar] [CrossRef]
- Liu, D.; Sutherland, J.W.; Moon, K.S.; Stums, T.J.; Kashani, A.R. Surface Texture Improvement in the Turning Process Via Application of a Magnetostrictively Actuated Tool Holder. J. Dyn. Syst. Meas. Control. 1998, 120, 193–199. [Google Scholar] [CrossRef]
- Trumper, D.L.; Lu, X. Fast Tool Servos: Advances in Precision, Acceleration, and Bandwidth. In Towards Synthesis of Micro-/Nano-Systems; Springer: London, UK, 2007; pp. 11–19. [Google Scholar] [CrossRef]
- Ding, B.; Yang, Z.-X.; Zhang, G.; Xiao, X. Optimum Design and Analysis of Flexure-Based Mechanism for Non-Circular Diamond Turning Operation. Res. Artic. Adv. Mech. Eng. 2017, 9, 1–10. [Google Scholar] [CrossRef]
- Hou, W.; Zhang, X. Dynamic Analysis of Flexible Linkage Mechanisms under Uniform Temperature Change. J. Sound Vib. 2009, 319, 570–592. [Google Scholar] [CrossRef]
- Scheiding, S.; Yi, A.Y.; Gebhardt, A.; Li, L.; Risse, S.; Eberhardt, R.; Shin, G.C.; Jung, I.W.; Malyarchuk, V.; Song, J.Z.; et al. Freeform Manufacturing of a Microoptical Lens Array on a Steep Curved Substrate by Use of a Voice Coil Fast Tool Servo. Opt. Express 2011, 19, 23938–23951. [Google Scholar] [CrossRef]
- Wu, D.; Xie, X.; Zhou, S. Design of a Normal Stress Electromagnetic Fast Linear Actuator. IEEE Trans. Magn. 2010, 46, 1007–1014. [Google Scholar] [CrossRef]
- Zhang, Y.; Peng, Y.; Sun, Z.; Yu, H. A Novel Stick-Slip Piezoelectric Actuator Based on a Triangular Compliant Driving Mechanism. IEEE Trans. Ind. Electron. 2019, 66, 5374–5382. [Google Scholar] [CrossRef]
- Tang, H.; Li, Y. Development and Active Disturbance Rejection Control of a Compliant Micro-/Nanopositioning Piezostage with Dual Mode. IEEE Trans. Ind. Electron. 2014, 61, 1475–1492. [Google Scholar] [CrossRef]
- Jingzhuo, J.; Liu, B. Optimum Efficiency Control of Traveling-Wave Ultrasonic Motor System. IEEE Trans. Ind. Electron. 2011, 58, 4822–4829. [Google Scholar] [CrossRef]
- Wang, R.; Hu, Y.; Shen, D.; Ma, J.; Li, J.; Wen, J. A Novel Piezoelectric Inchworm Actuator Driven by One Channel Direct Current Signal. IEEE Trans. Ind. Electron. 2021, 68, 2015–2023. [Google Scholar] [CrossRef]
- Yang, S.; Li, Y.; Xia, X.; Ning, P.; Ruan, W.; Zheng, R.; Lu, X. A Topology Optimization Method and Experimental Verification of Piezoelectric Stick-Slip Actuator with Flexure Hinge Mechanism. Arch. Appl. Mech. 2022, 92, 271–285. [Google Scholar] [CrossRef]
- Hussain, I.; Xia, W.; Zhao, D.; Huang, P.; Zhu, Z. Multi-Physical Design and Resonant Controller Based Trajectory Tracking of the Electromagnetically Driven Fast Tool Servo. Actuators 2020, 9, 28. [Google Scholar] [CrossRef]
- Wu, Z.; Xu, Q. Survey on Recent Designs of Compliant Micro-/Nano-Positioning Stages. Actuators 2018, 7, 5. [Google Scholar] [CrossRef]
- Lu, X.D.; Trumper, D.L. Ultrafast Tool Servos for Diamond Turning. CIRP Ann. 2005, 54, 383–388. [Google Scholar] [CrossRef]
- Guo, H.; Wang, D.; Xu, J. Research on a High-Frequency Response Direct Drive Valve System Based on Voice Coil Motor. IEEE Trans. Power Electron. 2013, 28, 2483–2492. [Google Scholar] [CrossRef]
- Shokrani, A.; Dhokia, V.; Newman, S.T. Environmentally Conscious Machining of Difficult-to-Machine Materials with Regard to Cutting Fluids. Int. J. Mach. Tools Manuf. 2012, 57, 83–101. [Google Scholar] [CrossRef]
- Tao, Y.; Li, Z.; Hu, P.; Chen, F.-W.; Ju, B.-F.; Chen, Y.-L. High-Accurate Cutting Forces Estimation by Machine Learning with Voice Coil Motor-Driven Fast Tool Servo for Micro/Nano Cutting. Precis. Eng. 2023, 79, 291–299. [Google Scholar] [CrossRef]
- Chen, Y.-L.; Tao, Y.; Hu, P.; Wu, L.; Ju, B.-F. Self-Sensing of Cutting Forces in Diamond Cutting by Utilizing a Voice Coil Motor-Driven Fast Tool Servo. Precis. Eng. 2021, 71, 178–186. [Google Scholar] [CrossRef]
- Li, H.; Tang, H.; Li, J.; Chen, X. Design, Fabrication, and Testing of a 3-DOF Piezo Fast Tool Servo for Microstructure Machining. Precis. Eng. 2021, 72, 756–768. [Google Scholar] [CrossRef]
- Yang, X.; Zhu, W.L. Design, Analysis, and Test of a Novel Self-Sensing Fast Tool Servo. IEEE Trans. Ind. Inform. 2020, 16, 4447–4455. [Google Scholar] [CrossRef]
- Zhu, Z.; Du, H.; Zhou, R.; Huang, P.; Zhu, W.L.; Guo, P. Design and Trajectory Tracking of a Nanometric Ultra-Fast Tool Servo. IEEE Trans. Ind. Electron. 2020, 67, 432–441. [Google Scholar] [CrossRef]
- Kim, C.J.; Kota, S.; Moon, Y.-M. An Instant Center Approach Toward the Conceptual Design of Compliant Mechanisms. J. Mech. Des. 2006, 128, 542–550. [Google Scholar] [CrossRef]
- Hopkins, J.B.; Culpepper, M.L. Synthesis of Multi-Degree of Freedom, Parallel Flexure System Concepts via Freedom and Constraint Topology (FACT)—Part I: Principles. Precis. Eng. 2010, 34, 259–270. [Google Scholar] [CrossRef]
- Howell, L.L.; Midha, A. A Method for the Design of Compliant Mechanisms with Small-Length Flexural Pivots. J. Mech. Des. 1994, 116, 280–290. [Google Scholar] [CrossRef]
- Dong, X.; Pei, X.; Kong, X. Mobility and Singularity Analysis of a Class of Two Degrees of Freedom Rotational Parallel Mechanisms Using a Visual Graphic Approach. J. Mech. Robot. 2012, 4, 041006. [Google Scholar] [CrossRef]
- Sigmund, O. On the Design of Compliant Mechanisms Using Topology Optimization*. Mech. Struct. Mach. 2007, 25, 493–524. [Google Scholar] [CrossRef]
- Awtar, S.; Slocum, A.H. Constraint-Based Design of Parallel Kinematic XY Flexure Mechanisms. J. Mech. Des. 2007, 129, 816–830. [Google Scholar] [CrossRef]
- Dibiasio, C.M.; Culpepper, M.L. A Building Block Synthesis Approach for Precision Flexure Systems with Integrated, Strain-Based Position Sensing. Precis. Eng. 2012, 36, 673–679. [Google Scholar] [CrossRef]
- MacHekposhti, D.F.; Herder, J.L.; Semon, G.; Tolou, N. A Compliant Micro Frequency Quadrupler Transmission Utilizing Singularity. J. Microelectromech. Syst. 2018, 27, 506–512. [Google Scholar] [CrossRef]
- Nijssen, J.P.A.; Radaelli, G.; Kim, C.J.; Herder, J.L. Overview and Kinetostatic Characterization of Compliant Shell Mechanism Building Blocks. J. Mech. Robot. 2020, 12, 061009. [Google Scholar] [CrossRef]
- Berre, J.; Geiskopf, F.; Rubbert, L.; Renaud, P. Toward the Design of Kresling Tower Origami as a Compliant Building Block. J. Mech. Robot. 2022, 14, 045002. [Google Scholar] [CrossRef]
- Hopkins, J.B.; Culpepper, M.L. Synthesis of Multi-Degree of Freedom, Parallel Flexure System Concepts via Freedom and Constraint Topology (FACT). Part II: Practice. Precis. Eng. 2010, 34, 271–278. [Google Scholar] [CrossRef]
- Lin, S.; Kong, X.; Wang, C.; Zhai, Y.; Yang, L. A Novel Three-Dimensional Elliptical Vibration Cutting Device Based on the Freedom and Constraint Topologies Theory. J. Eng. Manuf. 2019, 233, 675–685. [Google Scholar] [CrossRef]
- Lusk, C. Using Pseudo-Rigid Body Models. In Handbook of Compliant Mechanisms; John Wiley & Sons: Hoboken, NJ, USA, 2013; pp. 55–76. [Google Scholar] [CrossRef]
- Pei, X.; Yu, J.; Zong, G.; Bi, S. An Effective Pseudo-Rigid-Body Method for Beam-Based Compliant Mechanisms. Precis. Eng. 2010, 34, 634–639. [Google Scholar] [CrossRef]
- Liu, P.; Yan, P. A Modified Pseudo-Rigid-Body Modeling Approach for Compliant Mechanisms with Fixed-Guided Beam Flexures. Mech. Sci 2017, 8, 359–368. [Google Scholar] [CrossRef]
- Xu, H.; Gan, J.; Zhang, X. A Generalized Pseudo-Rigid-Body PPRR Model for Both Straight and Circular Beams in Compliant Mechanisms. Mech. Mach. Theory 2020, 154, 104054. [Google Scholar] [CrossRef]
- Ruiz, A.; Campa, F.J.; Roldán-Paraponiaris, C.; Altuzarra, O.; Pinto, C. Experimental Validation of the Kinematic Design of 3-PRS Compliant Parallel Mechanisms. Mechatronics 2016, 39, 77–88. [Google Scholar] [CrossRef]
- Wang, N.; Zhang, Z.; Zhang, X.; Cui, C. Optimization of a 2-DOF Micro-Positioning Stage Using Corrugated Flexure Units. Mech. Mach. Theory 2018, 121, 683–696. [Google Scholar] [CrossRef]
- Arredondo-Soto, M.; Cuan-Urquizo, E.; Gómez-Espinosa, A. The Compliance Matrix Method for the Kinetostatic Analysis of Flexure-Based Compliant Parallel Mechanisms: Conventions and General Force–Displacement Cases. Mech. Mach. Theory 2022, 168, 104583. [Google Scholar] [CrossRef]
- Lobontiu, N. Compliant Mechanisms: Design of Flexure Hinges. In Compliant Mechanisms; CRC Press: Boca Raton, FL, USA, 2002. [Google Scholar] [CrossRef]
- Wang, J.; Yang, Y.; Yang, R.; Feng, P.; Guo, P. On the Validity of Compliance-Based Matrix Method in Output Compliance Modeling of Flexure-Hinge Mechanism. Precis. Eng. 2019, 56, 485–495. [Google Scholar] [CrossRef]
- Ling, M.; Cao, J.; Jiang, Z.; Zeng, M.; Li, Q. Optimal Design of a Piezo-Actuated 2-DOF Millimeter-Range Monolithic Flexure Mechanism with a Pseudo-Static Model. Mech. Syst. Signal Process. 2019, 115, 120–131. [Google Scholar] [CrossRef]
- Zhu, B.; Zhang, X.; Zhang, H.; Liang, J.; Zang, H.; Li, H.; Wang, R. Design of Compliant Mechanisms Using Continuum Topology Optimization: A Review. Mech. Mach. Theory 2020, 143, 103622. [Google Scholar] [CrossRef]
- Pedersen, C.B.W.; Buhl, T.; Sigmund, O. Topology Synthesis of Large-Displacement Compliant Mechanisms. Int. J. Numer. Methods Eng. 2001, 50, 2683–2705. [Google Scholar] [CrossRef]
- Yin, L.; Ananthasuresh, G.K. Mechanics Based Design of Structures and Machines An International Journal Design of Distributed Compliant Mechanisms. Mech. Based Des. Struct. Mach. 2007, 31, 151–179. [Google Scholar] [CrossRef]
- Qiao, G.; Ning, P.; Gao, Q.; Shih, C.J.; Lin, C.F. A Two-Stage Topological Optimum Design for Monolithic Compliant Microgripper Integrated with Flexure Hinges. J. Phys. Conf. Ser. 2006, 34, 840–846. [Google Scholar] [CrossRef]
- Le Chau, N.; Tran, N.T.; Dao, T.-P. An Optimal Design Method for Compliant Mechanisms. Math. Probl. Eng. 2021, 2021, 5599624. [Google Scholar] [CrossRef]
- Dao, T.-P.; Huang, S.-C.; Toan Thang, P. Hybrid Taguchi-Cuckoo Search Algorithm for Optimization of a Compliant Focus Positioning Platform. Appl. Soft Comput. 2017, 57, 526–538. [Google Scholar] [CrossRef]
- Tian, Y.; Zhang, D.; Shirinzadeh, B. Dynamic Modelling of a Flexure-Based Mechanism for Ultra-Precision Grinding Operation. Precis. Eng. 2011, 35, 554–565. [Google Scholar] [CrossRef]
- Zhou, X.; Wang, R.; Liu, Q.; Zhu, Z. Development of a 2-Degree-of-Freedom Decoupled Flexural Mechanism for Micro/Nanomachining. J. Eng. Manuf. 2015, 229, 1900–1911. [Google Scholar] [CrossRef]
- Hopkins, J.B.; McCalib, D. Synthesizing Multi-Axis Flexure Systems with Decoupled Actuators. Precis. Eng. 2016, 46, 206–220. [Google Scholar] [CrossRef]
- Hao, G.; Kong, X. A Structure Design Method for Compliant Parallel Manipulators with Actuation Isolation. Mech. Sci. 2016, 7, 247–253. [Google Scholar] [CrossRef]
- Zhao, D.; Zhu, Z.; Huang, P.; Guo, P.; Zhu, L.; Zhu, Z. Development of a Piezoelectrically Actuated Dual-Stage Fast Tool Servo. Mech. Syst. Signal Process. 2020, 144, 106873. [Google Scholar] [CrossRef]
- Zhu, Z.; Zhou, X.; Liu, Q.; Zhao, S. Multi-Objective Optimum Design of Fast Tool Servo Based on Improved Differential Evolution Algorithm. J. Mech. Sci. Technol. 2011, 25, 3141–3149. [Google Scholar] [CrossRef]
- Elfizy, A.T.; Bone, G.M.; Elbestawi, M.A. Design and Control of a Dual-Stage Feed Drive. Int. J. Mach. Tools Manuf. 2005, 45, 153–165. [Google Scholar] [CrossRef]
- Kim, H.S.; Lee, K.-I.; Lee, K.M.; Bang, Y.B. Fabrication of Free-Form Surfaces Using a Long-Stroke Fast Tool Servo and Corrective Figuring with on-Machine Measurement. Int. J. Mach. Tools Manuf. 2009, 49, 991–997. [Google Scholar] [CrossRef]
- Ling, M.; Yuan, L.; Luo, Z.; Huang, T.; Zhang, X. Enhancing Dynamic Bandwidth of Amplified Piezoelectric Actuators by a Hybrid Lever and Bridge-Type Compliant Mechanism. Actuators 2022, 11, 134. [Google Scholar] [CrossRef]
- Gong, Z.; Huo, D.; Niu, Z.; Chen, W.; Cheng, K. A Novel Long-Stroke Fast Tool Servo System with Counterbalance and Its Application to the Ultra-Precision Machining of Microstructured Surfaces. Mech. Syst. Signal Process. 2022, 173, 109063. [Google Scholar] [CrossRef]
- Zhu, Z.; Zhou, X.; Liu, Z.; Wang, R.; Zhu, L. Development of a Piezoelectrically Actuated Two-Degree-of-Freedom Fast Tool Servo with Decoupled Motions for Micro-/Nanomachining. Precis. Eng. 2014, 38, 809–820. [Google Scholar] [CrossRef]
- Tang, H.; Li, H.; To, S.; Yu, K.-M.; He, Y.; Gao, J.; Chen, X.; Li, J. Design and Control of a New 3-PUU Fast Tool Servo for Complex Microstructure Machining. Int. J. Adv. Manuf. Technol. 2017, 94, 3503–3517. [Google Scholar] [CrossRef]
- Liu, Y.; Zheng, Y.; Gu, Y.; Lin, J.; Lu, M.; Xu, Z.; Fu, B. Development of Piezo-Actuated Two-Degree-of-Freedom Fast Tool Servo System. Micromachines 2019, 10, 337. [Google Scholar] [CrossRef]
- Wang, R.; Zhou, X.; Meng, G. Development of A New Type of 2-DOF Piezo-Actuated Pseudo-Decoupled Compliant Mechanism for Elliptical Vibration Machining. Micromachines 2019, 10, 122. [Google Scholar] [CrossRef] [PubMed]
- Zhu, Z.; To, S.; Zhu, W.L.; Li, Y.; Huang, P. Optimum Design of a Piezo-Actuated Triaxial Compliant Mechanism for Nanocutting. IEEE Trans. Ind. Electron. 2018, 65, 6362–6371. [Google Scholar] [CrossRef]
- Zhu, Z.H.; Chen, L.; Niu, Y.; Pu, X.; Huang, P.; To, S.; Zhu, L.; Zhu, Z. Tri-Axial Fast Tool Servo Using Hybrid Electromagnetic-Piezoelectric Actuation for Diamond Turning. IEEE Trans. Ind. Electron. 2021, 69, 1728–1738. [Google Scholar] [CrossRef]
- Kim, M.; Lee, D.W.; Lee, S.; Kim, Y.; Jung, Y. Effects of Hinge Design of Horizontal-Swing Fast Tool Servo (HFTS) for Micro-Patterning on a Roll. Int. J. Adv. Manuf. Technol. 2018, 95, 233–241. [Google Scholar] [CrossRef]
- Chen, Y.L.; Cai, Y.; Shimizu, Y.; Ito, S.; Gao, W.; Ju, B.F. On-Machine Measurement of Microtool Wear and Cutting Edge Chipping by Using a Diamond Edge Artifact. Precis. Eng. 2016, 43, 462–467. [Google Scholar] [CrossRef]
- Zhou, J.H.; Pang, C.K.; Lewis, F.L.; Zhong, Z.W. Intelligent Diagnosis and Prognosis of Tool Wear Using Dominant Feature Identification. IEEE Trans. Ind. Inform. 2009, 5, 454–464. [Google Scholar] [CrossRef]
- Zhu, W.L.; Yang, X.; Zhu, Z. Development of a Highly Flexible Lattice-Structure-Based Force Sensing Mechanism. IEEE Trans. Ind. Inform. 2019, 15, 5943–5953. [Google Scholar] [CrossRef]
- Herrera-Granados, G.; Morita, N.; Hidai, H.; Matsusaka, S.; Chiba, A.; Ashida, K.; Ogura, I.; Okazaki, Y. Development of a Non-Rigid Micro-Scale Cutting Mechanism Applying a Normal Cutting Force Control System. Precis. Eng. 2016, 43, 544–553. [Google Scholar] [CrossRef]
- Huang, W.-W.; Li, L.; Zhu, Z.; Zhu, L.-M. Modeling, Design and Control of Normal-Stressed Electromagnetic Actuated Fast Tool Servos. Mech. Syst. Signal Process. 2022, 178, 888–3270. [Google Scholar] [CrossRef]
- Mishra, V.; Garg, H.; Karar, V.; Khan, G.S. Ultra-Precision Diamond Turning Process. In Micro and Nano Machining of Engineering Materials. Materials Forming, Machining and Tribology; Springer: Cham, Switzerland, 2019. [Google Scholar] [CrossRef]
- Holthusen, A.-K.; Riemer, O.; Brinksmeier, E. Manufacturing and Materials Processing Material Impact on Diamond Machining of Diffractive Optical Structures for UV-Application. J. Manuf. Mater. Process. 2018, 2, 15. [Google Scholar] [CrossRef]
- Yu, D.P.; Wong, Y.S.; Hong, G.S. Ultraprecision Machining of Micro-Structured Functional Surfaces on Brittle Materials. J. Micromech. Microeng. 2011, 21, 95011. [Google Scholar] [CrossRef]
- Cardoso, M.; Carbone, G.; Vogel-Heuser, B.; Zhang, D.; Perrelli, M.; Cosco, F.; Gagliardi, F.; Mundo, D. In-Process Chatter Detection Using Signal Analysis in Frequency and Time-Frequency Domain. Machines 2021, 10, 24. [Google Scholar] [CrossRef]
- Jumare, A.I.; Abou-El-Hossein, K.; Goosen, W.E.; Cheng, Y.-C.; Abdulkadir, L.N.; Odedeyi, P.B.; Liman, M.M. Prediction Model for Single-Point Diamond Tool-Tip Wear during Machining of Optical Grade Silicon. Int. J. Adv. Manuf. Technol. 2018, 98, 2519–2529. [Google Scholar] [CrossRef]
- Cheng, K. (Ed.) Machining Dynamics: Fundamentals, Applications and Practices; Springer Series in Advanced Manufacturing; Springer: London, UK, 2009; ISBN 978-1-84628-367-3. [Google Scholar]
- Zhu, W.L.; Yang, X.; Duan, F.; Zhu, Z.; Ju, B.F. Design and Adaptive Terminal Sliding Mode Control of a Fast Tool Servo System for Diamond Machining of Freeform Surfaces. IEEE Trans. Ind. Electron. 2019, 66, 4912–4922. [Google Scholar] [CrossRef]
- Lv, B.; Lin, B.; Cao, Z.; Li, B.; Wang, G. A Parallel 3-DOF Micro-Nano Motion Stage for Vibration-Assisted Milling. Mech. Mach. Theory 2022, 173, 104854. [Google Scholar] [CrossRef]
- Zhu, Z.; Zhou, X. A Novel Fractional Order Model for the Dynamic Hysteresis of Piezoelectrically Actuated Fast Tool Servo. Materials 2012, 5, 2465–2485. [Google Scholar] [CrossRef]
- Zhou, X.; Zhang, L.; Yang, Z.; Sun, L. Modeling and Inverse Compensation of Cross-Coupling Hysteresis in Piezoceramics under Multi-Input. Micromachines 2021, 12, 86. [Google Scholar] [CrossRef]
- Keong Neo, W.; Senthil Kumar, A.; Rahman, M. A Review on the Current Research Trends in Ductile Regime Machining. Int. J. Adv. Manuf. Technol. 2012, 63, 465–480. [Google Scholar] [CrossRef]
- Tian, Y.; Shirinzadeh, B.; Zhang, D. A Flexure-Based Mechanism and Control Methodology for Ultra-Precision Turning Operation. Precis. Eng. 2009, 33, 160–166. [Google Scholar] [CrossRef]
- Yong, J.; Liu, J.; Guan, C.; Dai, Y.; Li, F. Fabrication and Evaluation of Complicated Microstructures on Cylindrical Surface. Optik 2021, 241, 167074. [Google Scholar] [CrossRef]
- Dirksen, F.; Anselmann, M.; Zohdi, T.I.; Lammering, R. Incorporation of Flexural Hinge Fatigue-Life Cycle Criteria into the Topological Design of Compliant Small-Scale Devices. Precis. Eng. 2013, 37, 531–541. [Google Scholar] [CrossRef]
- Zhang, X.; Hou, W. Dynamic Analysis of the Precision Compliant Mechanisms Considering Thermal Effect. Precis. Eng. 2010, 34, 592–606. [Google Scholar] [CrossRef]
- Manoach, E.; Ribeiro, P. Coupled, Thermoelastic, Large Amplitude Vibrations of Timoshenko Beams. Int. J. Mech. Sci. 2004, 46, 1589–1606. [Google Scholar] [CrossRef]
- Li, H.; Hao, G. Position-Space-Based Design of a Symmetric Spatial Translational Compliant Mechanism for Micro-/Nano-Manipulation. Micromachines 2018, 9, 189. [Google Scholar] [CrossRef] [PubMed]
- Le Chau, N.; Tran, N.T.; Dao, T.-P. Design Optimization for a Compliant Mechanism Based on Computational Intelligence Method. Neural Comput. Appl. 2021, 33, 9565–9587. [Google Scholar] [CrossRef]
- Amine, M.; Abdallah, B.; Khemili, I.; Aifaoui, N. Flexible Slider Crank Mechanism Synthesis Using Meta-Heuristic Optimization Techniques: A New Designer Tool Assistance for a Compliant Mechanism Synthesis. Artif. Intell. Rev. 2020, 53, 2809–2840. [Google Scholar] [CrossRef]
- He, Y.; Zhou, T.; Dong, X.; Liu, P.; Zhao, W.; Wang, X.; Hu, Y.; Yan, J. Generation of High-Saturation Two-Level Iridescent Structures by Vibration-Assisted Fly Cutting. Mater. Des. 2020, 193, 108839. [Google Scholar] [CrossRef]
- Wee, D.; Neo, K.; Kumar, A.S.; Rahman, M. CAx-Technologies for Hybrid Fast Tool/Slow Slide Servo Diamond Turning of Freeform Surface. J. Eng. Manuf. 2016, 230, 1465–1479. [Google Scholar] [CrossRef]
- Sharma, D.K.; Baghel, V.; Kumar, R.; Avasthi, D.K.; Singh Sikarwar, B. Recent Developments in Fabrication of Super-Hydrophobic Surfaces: A Review. In Advances in Industrial and Production Engineering; Lecture Notes in Mechanical Engineering; Springer: Singapore, 2019. [Google Scholar] [CrossRef]
- Yuan, Y.; Zhang, D.; Jing, X.; Zhu, H.; Zhu, W.L.; Cao, J.; Ehmann, K.F. Fabrication of Hierarchical Freeform Surfaces by 2D Compliant Vibration-Assisted Cutting. Int. J. Mech. Sci. 2019, 152, 454–464. [Google Scholar] [CrossRef]
- Guo, P.; Ehmann, K.F. Development of a Tertiary Motion Generator for Elliptical Vibration Texturing. Precis. Eng. 2013, 37, 364–371. [Google Scholar] [CrossRef]
- Gouker, R.M.; Gupta, S.K.; Bruck, H.A.; Holzschuh, T. Manufacturing of Multi-Material Compliant Mechanisms Using Multi-Material Molding. Int. J. Adv. Manuf. Technol. 2006, 30, 1049–1075. [Google Scholar] [CrossRef]
- Bejgerowski, W.; Gerdes, J.W.; Gupta, S.K.; Bruck, H.A. Design and Fabrication of Miniature Compliant Hinges for Multi-Material Compliant Mechanisms. Int. J. Adv. Manuf. Technol. 2011, 57, 437–452. [Google Scholar] [CrossRef]
- Wu, J.; Cai, S.; Cui, J.; Tan, J. A Generalized Analytical Compliance Model for Cartwheel Flexure Hinges. Rev. Sci. Instrum. 2015, 86, 105003. [Google Scholar] [CrossRef]
- Wu, J.; Zhang, Y.; Lu, Y.; Wen, Z.; Bin, D.; Tan, J. Modeling and Design of a Two-Axis Elliptical Notch Flexure Hinge Modeling and Design of a Two-Axis Elliptical Notch Flexure Hinge. Rev. Sci. Instrum. 2018, 89, 45010. [Google Scholar] [CrossRef] [PubMed]
- Moon, J.-H.; Pahk, H.J.; Lee, B.-G. Design, Modeling, and Testing of a Novel 6-DOF Micropositioning Stage with Low Profile and Low Parasitic Motion. Int. J. Adv. Manuf. Technol. 2011, 55, 163–176. [Google Scholar] [CrossRef]
- Qiu, L.; Yue, X.; Xie, Z. Design and Analysis of Multicavity Flexure Hinge (MCFH) Based on Three-Dimensional Continuum Topology Optimization. Mech. Mach. Theory 2019, 139, 21–33. [Google Scholar] [CrossRef]
- Pan, A.J.; Wu, B.J.; Zhang, C.Y.; Wang, D.H.; Jiubin Tan, E. Design and Analyze of Flexure Hinges Based on Triply Periodic Minimal Surface Lattice. Precis. Eng. 2020, 68, 338–350. [Google Scholar] [CrossRef]
- Jensen, B.D.; Howell, L.L. The Modeling of Cross-Axis Flexural Pivots. Mech. Mach. Theory 2002, 37, 461–476. [Google Scholar] [CrossRef]
- Pei, X.; Yu, J.; Zong, G.; Bi, S.; Su, H. The Modeling of Cartwheel Flexural Hinges. Mech. Mach. Theory 2009, 44, 1900–1909. [Google Scholar] [CrossRef]
- Yew, N.; Tan, J.; Wee, D.; Neo, K.; Zhang, X.; Liu, K.; Senthil Kumar, A. Ultra-Precision Direct Diamond Shaping of Functional Micro Features. J. Manuf. Process. 2021, 64, 209–223. [Google Scholar] [CrossRef]
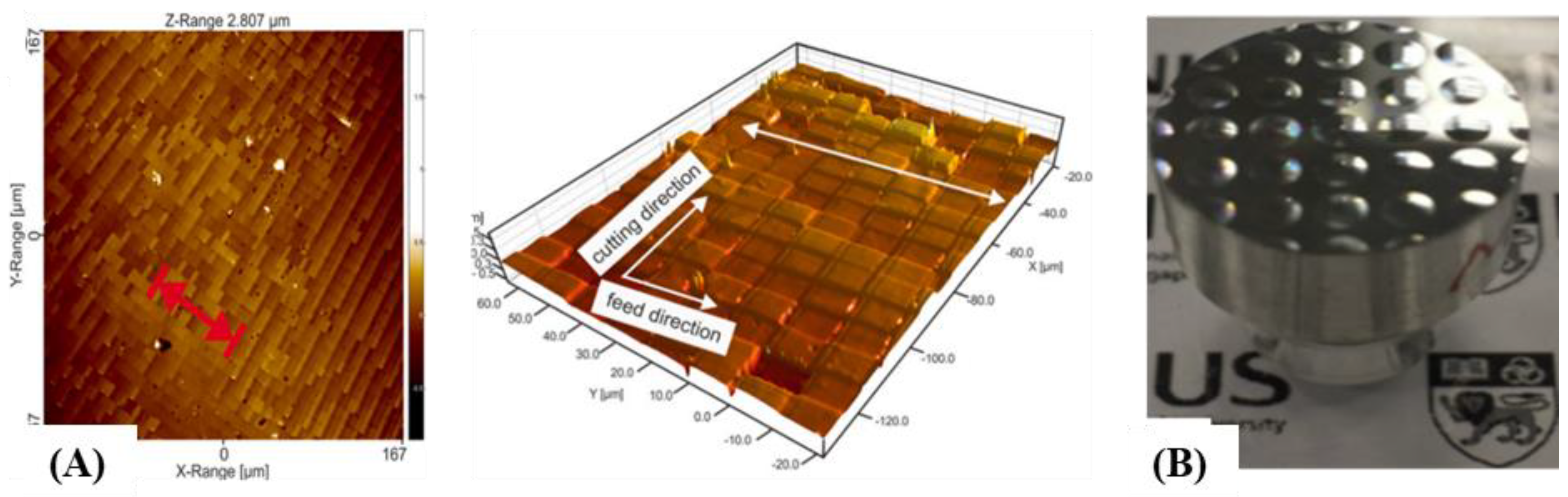





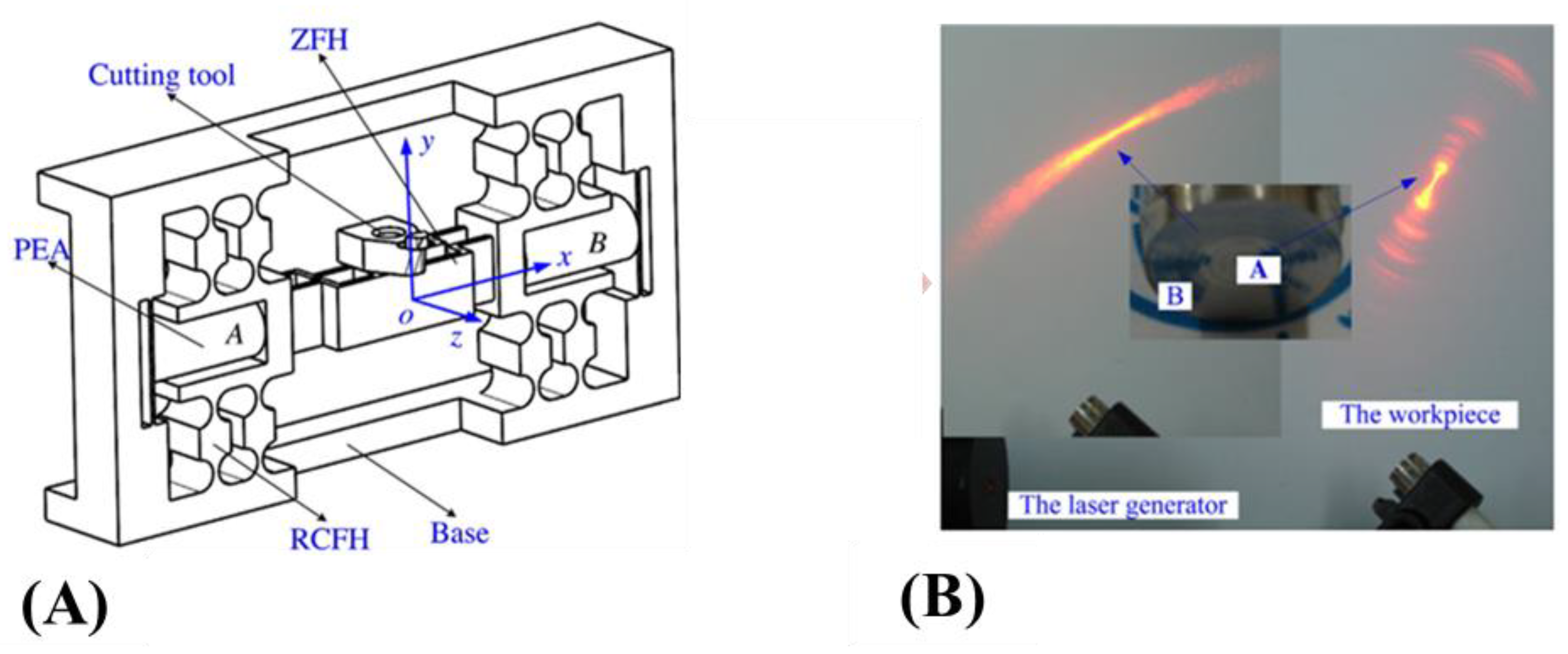
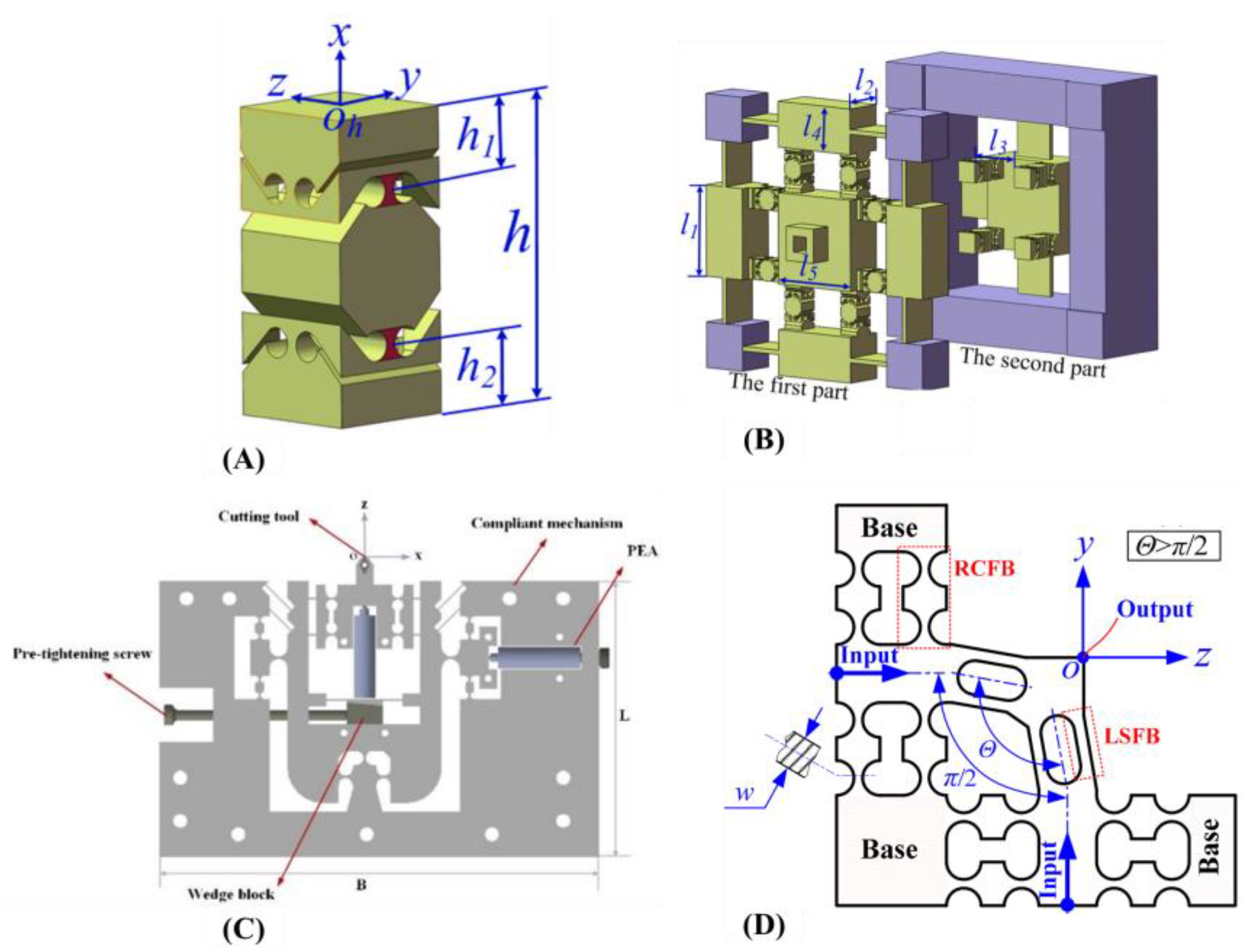
| Piezoelectric Actuator Type | Strengths | Weaknesses |
|---|---|---|
| Direct push |
|
|
| Ultrasonic |
|
|
| Inchworm [76] |
| |
| Stick-slip |
|
| FTS Configuration | Maximum Output Stroke/µm | Static Stiffness/Nµm−1 | 1st Mode Frequency/Hz | Positioning Error | Coupling Error | Limitations | |
|---|---|---|---|---|---|---|---|
| 1DOF | Standard 1DOF FTS | 10.25 | 21.9224 | 7259 | Hysteresis: 2 µm | - | Moderate hysteresis |
| Dual staged FTS [120] | 11.16 | 4.99 | 4700 | ±300 nm | - | Requires advanced controls | |
| Long stroke | MCVEP [30] | 540 | - | 321 | 5 µm | - | High hysteresis, low 1st mode |
| LFTS [123] | - | - | - | Max 40 nm | - | - | |
| Counterbalanced FTS [125] | 1500 | 31.05 | 100 | 0.145 µm | - | Reduced 1st mode | |
| Multiple DOF | Z-shaped flexure hinged 2-DOF FTS [126] | z-axis: Max 27.03 x-axis: −8.739 to 7.544 | 7.2 | 2976.8 | Max 0.8 µm | z-axis: 2.29% x-axis: 4.33% | - |
| 3-PUU FTS [127] | Actuation to any point within a 170 × 160 × 120 workspace | - | 66 | Max 600 nm | - | Very low 1st mode | |
| 4-bar flexure mechanism 2DOF FTS [128] | z-axis: Max 17 x-axis: Max 22.5 | 18.12 in x-axis 15.32 in z-axis | 1200 | - | z-axis: 1.47% x-axis: 2.22% | - | |
| Triaxial FTS [130] | Average 12.48 in all x, y and z directions | 16.93 | 3700 | Max 0.1 µm | z-axis: 2.51% y-axis: 1.37% x-axis: 3.8% | - | |
| Dual axis notch flexure 3DOF FTS [85] | Maximum 40 | x-axis: 16.45 y-axis: 16.37 z-axis: 15.92 | 873 | ±0.7 µm | z-axis: 2% y-axis: 0.5% x-axis: 0.5% | Reduced 1st mode | |
| Hybrid electromagnetic-piezoelectric FTS [131] | Maximum: 20 | z-axis: 6.1 y-axis: 5.1 x-axis: 4.9 | x-axis: 704 y-axis: 786 z-axis: 3607 | Planar: ±0.257 µm Vertical: ±64 nm | z-axis: ±0.77% y-axis: 1.37% x-axis: 1.61% | Advanced controls | |
| Swing output | hFTS [132] | Max swing: 7 | - | Min 5000 | - | - | - |
| Self-sensing | AFM inspired FTS [136] | - | 41.68 × 10−3 | 420 | - | - | Reduced 1st mode |
| Normal-stressed electromagnetic actuated FTS [137] | 35.2 | 10.04 | 2260 | Max 3.66% at 100 Hz | - | - | |
| sFTS [86] | - | x-axis: 760 y-axis: 2.94 z-axis: 760 | 1360 | ±0.4% at 2 Hz ±0.8% at 5 Hz ±1.7% at 10 Hz ±3.1% at 20 Hz | - | Reduced 1st mode | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Paniselvam, V.; Jin Tan, N.Y.; Anantharajan, S.K. A Review on the Design and Application of Compliant Mechanism-Based Fast-Tool Servos for Ultraprecision Machining. Machines 2023, 11, 450. https://doi.org/10.3390/machines11040450
Paniselvam V, Jin Tan NY, Anantharajan SK. A Review on the Design and Application of Compliant Mechanism-Based Fast-Tool Servos for Ultraprecision Machining. Machines. 2023; 11(4):450. https://doi.org/10.3390/machines11040450
Chicago/Turabian StylePaniselvam, Vinodth, Nicholas Yew Jin Tan, and Senthil Kumar Anantharajan. 2023. "A Review on the Design and Application of Compliant Mechanism-Based Fast-Tool Servos for Ultraprecision Machining" Machines 11, no. 4: 450. https://doi.org/10.3390/machines11040450
APA StylePaniselvam, V., Jin Tan, N. Y., & Anantharajan, S. K. (2023). A Review on the Design and Application of Compliant Mechanism-Based Fast-Tool Servos for Ultraprecision Machining. Machines, 11(4), 450. https://doi.org/10.3390/machines11040450