Bounded Positioning Control of Manipulators Subject to Base Oscillation and Payload Uncertainty
Abstract
:1. Introduction
- Compared with other OBMs, the prior information of vehicle induced base oscillation is more difficult to predict or measure in practice, especially when the vehicle embarks on erratic uneven terrains.
- Different from other OBMs, vehicle-mounted telesurgery manipulator operates in the non-inertial frame. This will lead to greater nonlinearity, and in turn bring more challenges to the control design.
- Most of current studies on OBMs do not consider the problems of input boundedness and payload uncertainty.
- This control has a simpler structure and can be implemented more easily. It is actually a continuous varying-gain PD (proportional differential) control. However, control has the problem of high order, and is difficult to solve and implement in practice. High order slide mode control has similar problems, while low order one has chattering problem.
- The robust stability proof of proposed control is easier, due to control gains of which are related to a given implicit Lyapunov function [34].
- The proposed control also has boundary property that other controls do no have.
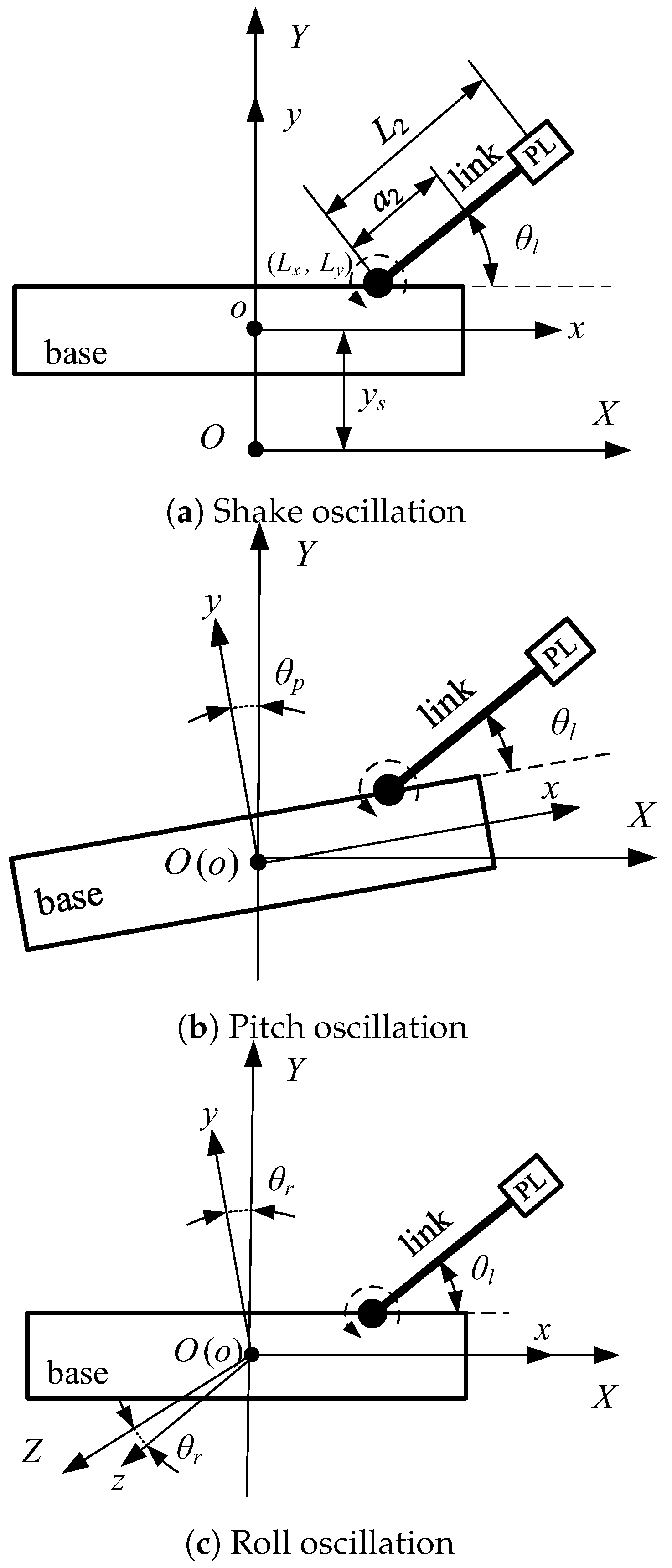
2. Problem Statements
3. Main Development
3.1. Constant PD Control
3.2. Implicit-Lyapunov-Function Based Control
4. Performance Evaluation
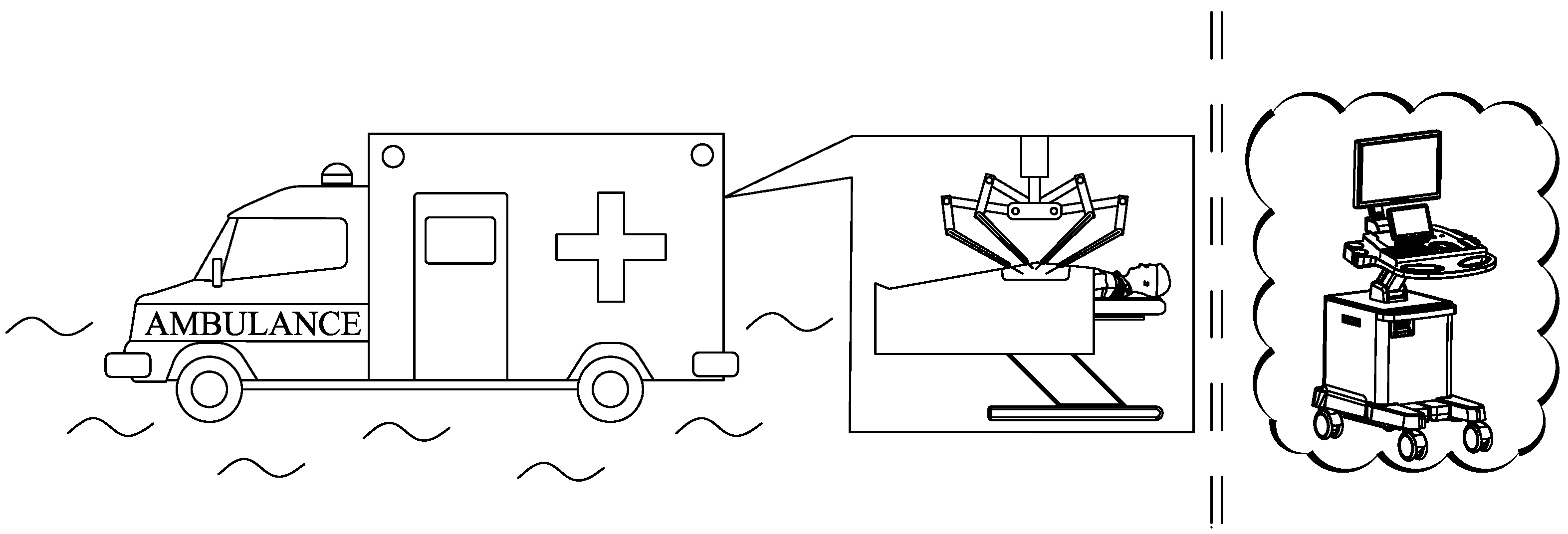
4.1. System Development
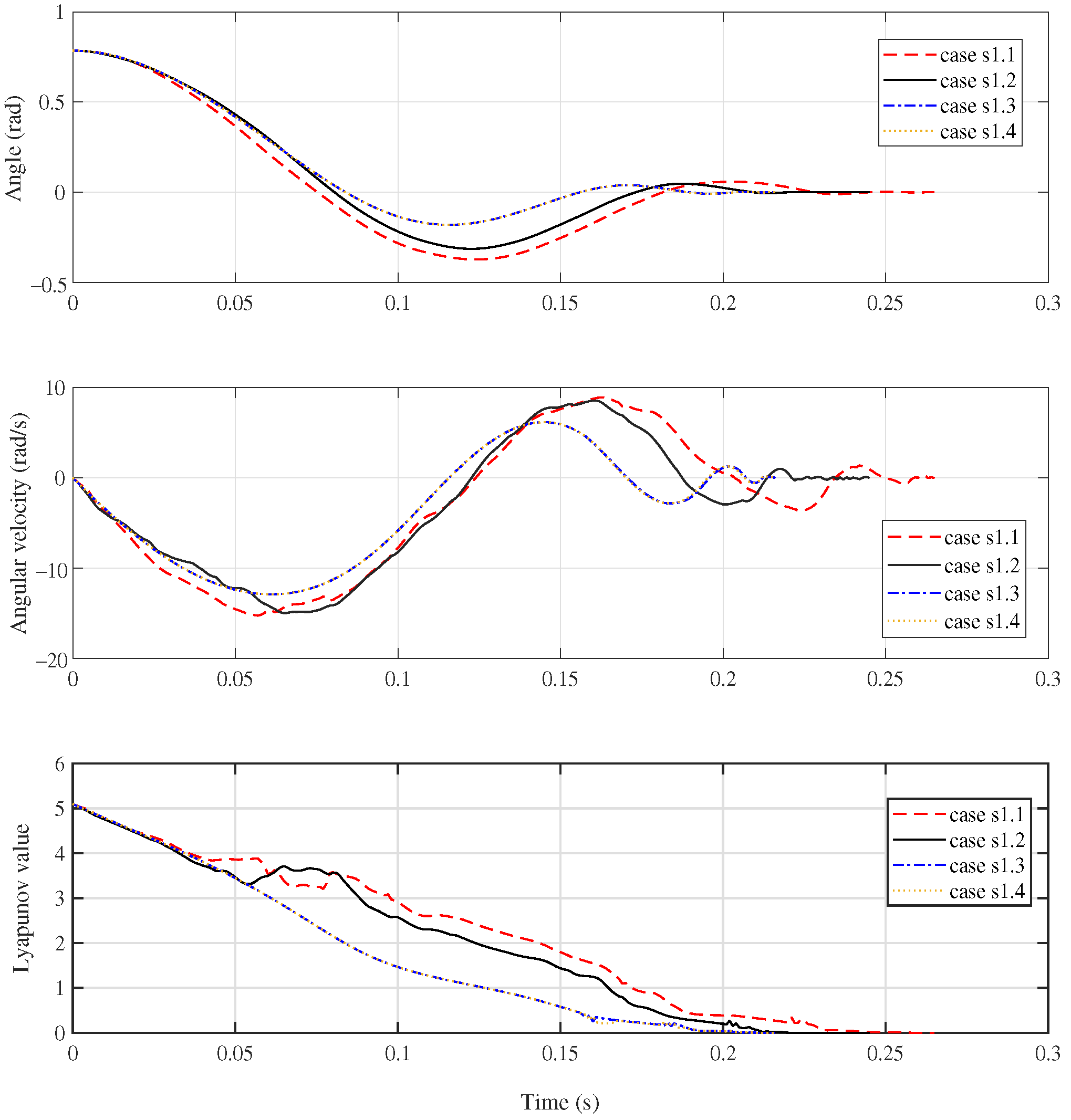
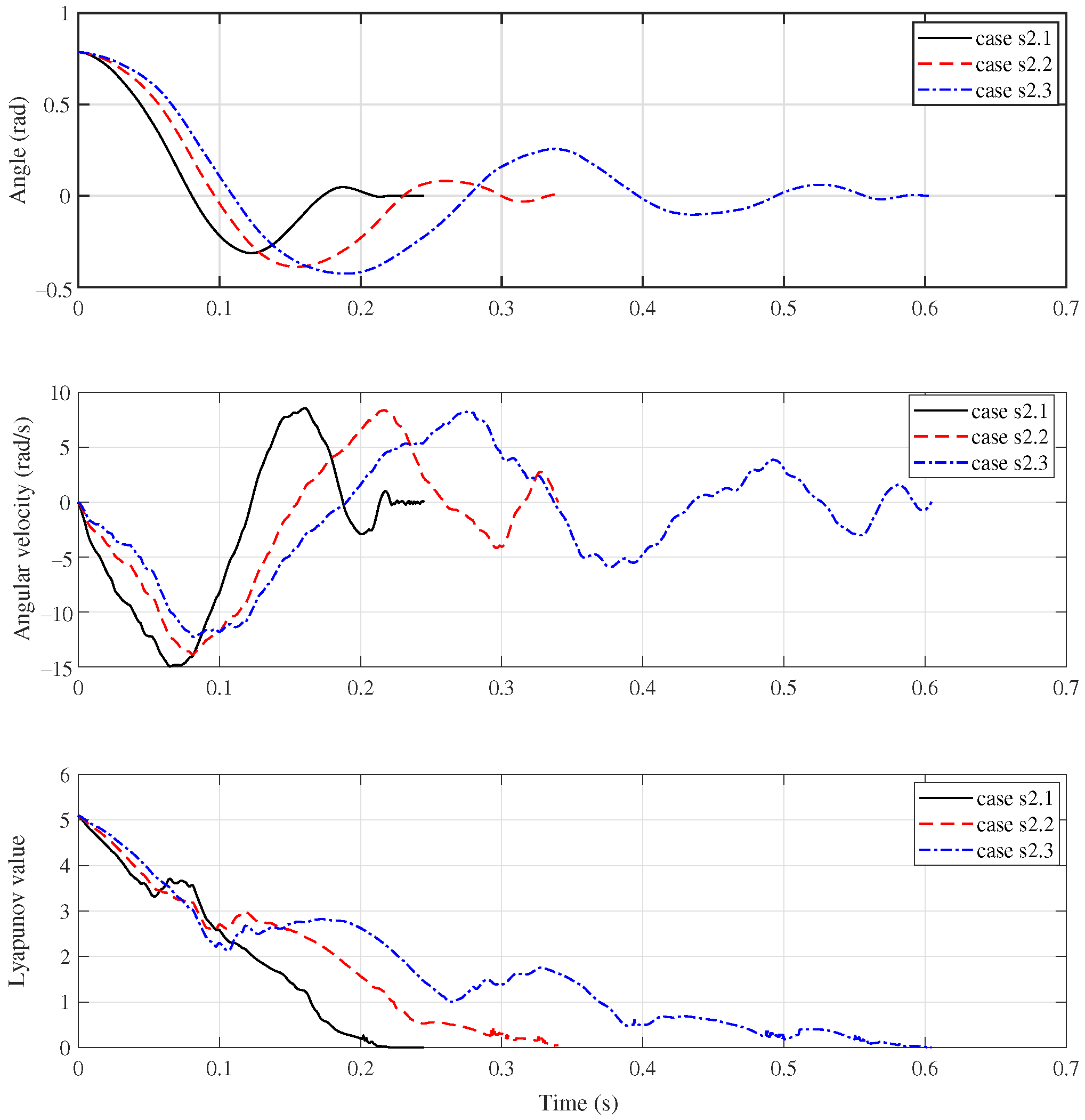
4.2. Simulations and Result Analysis
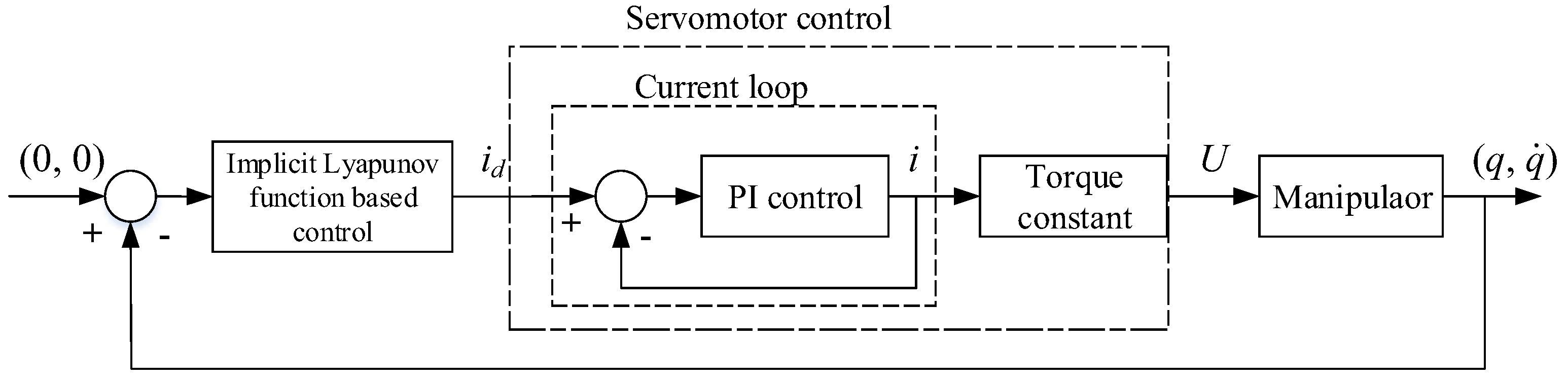
4.3. Hardware Configurations
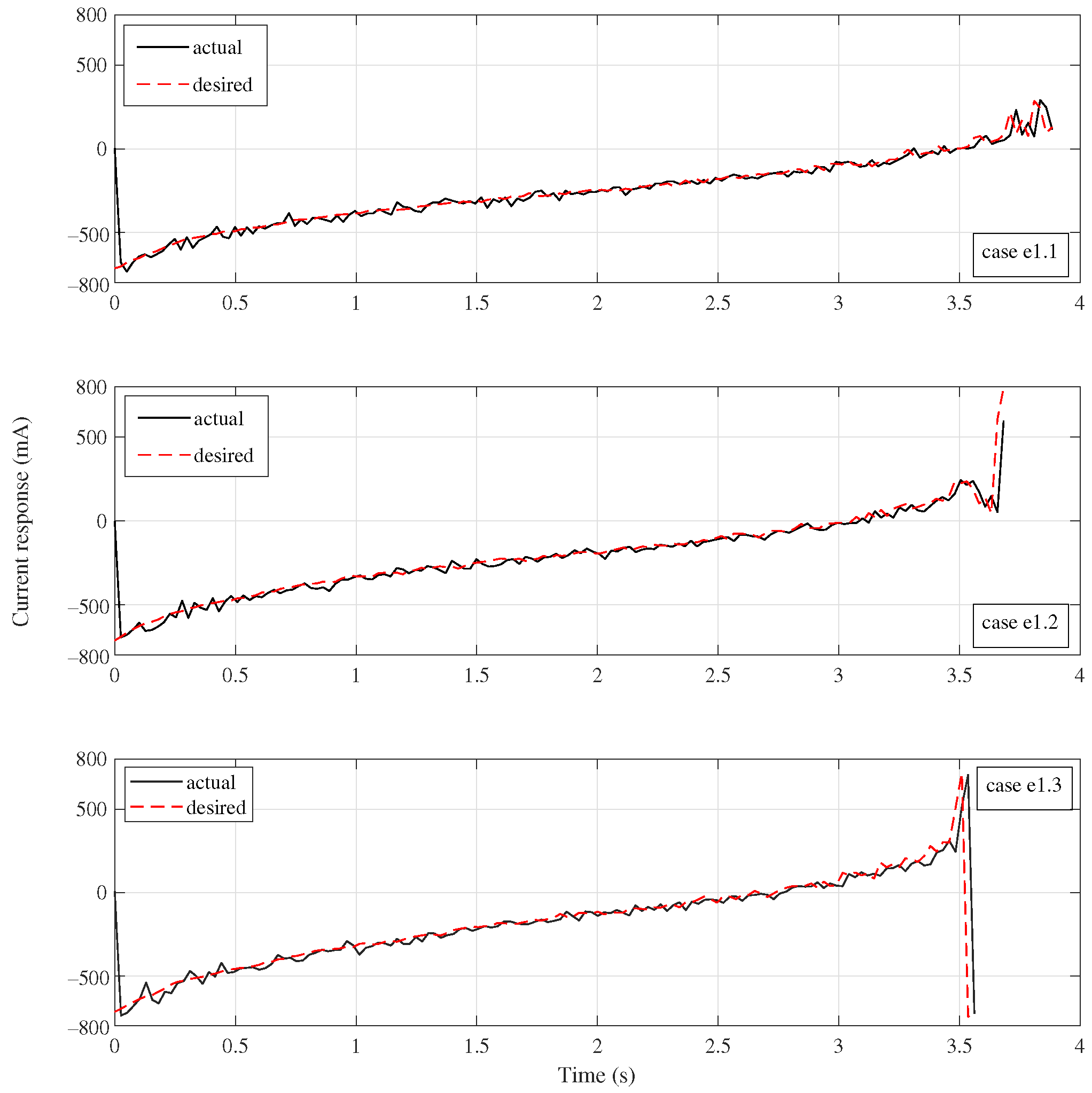
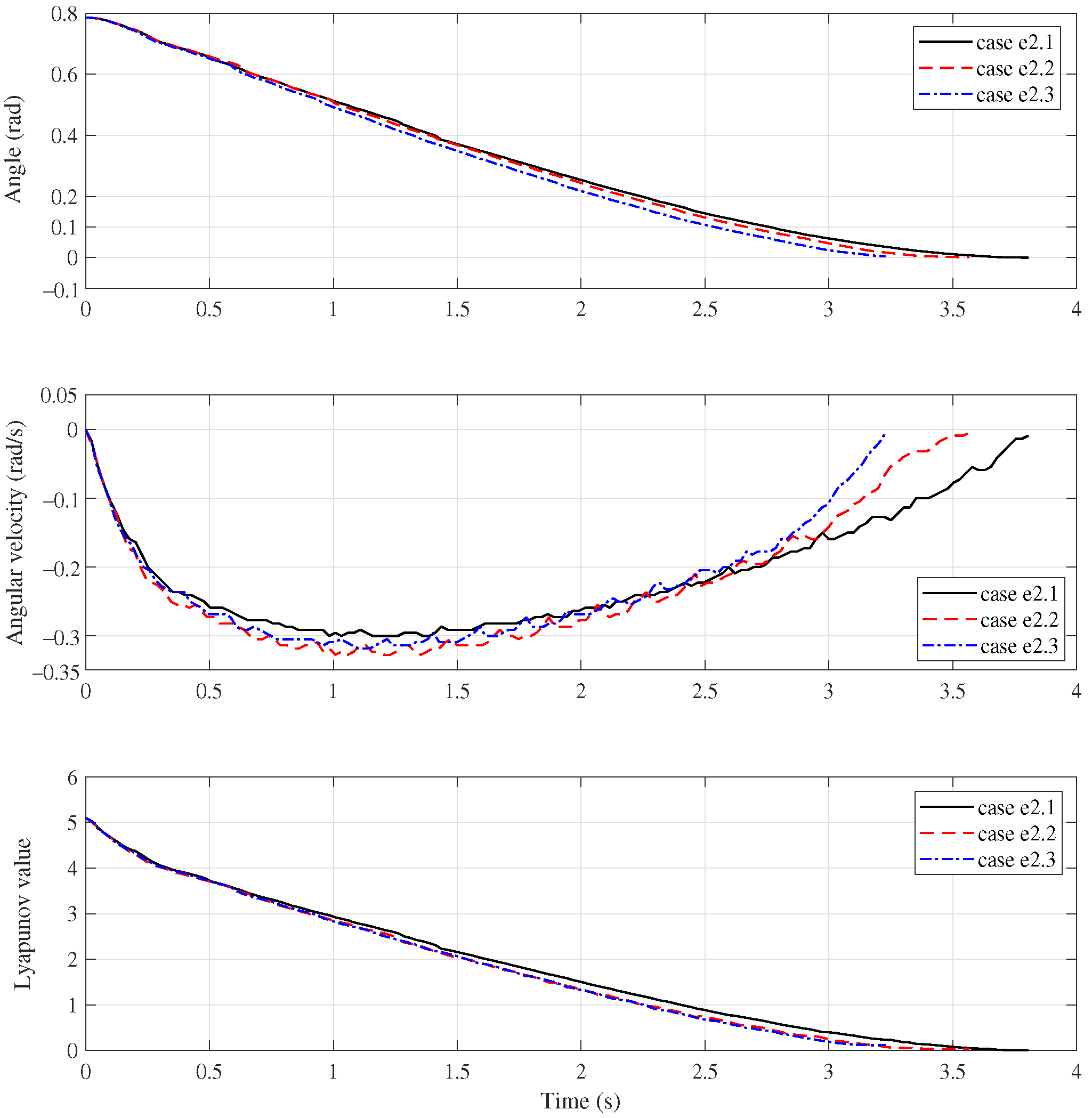
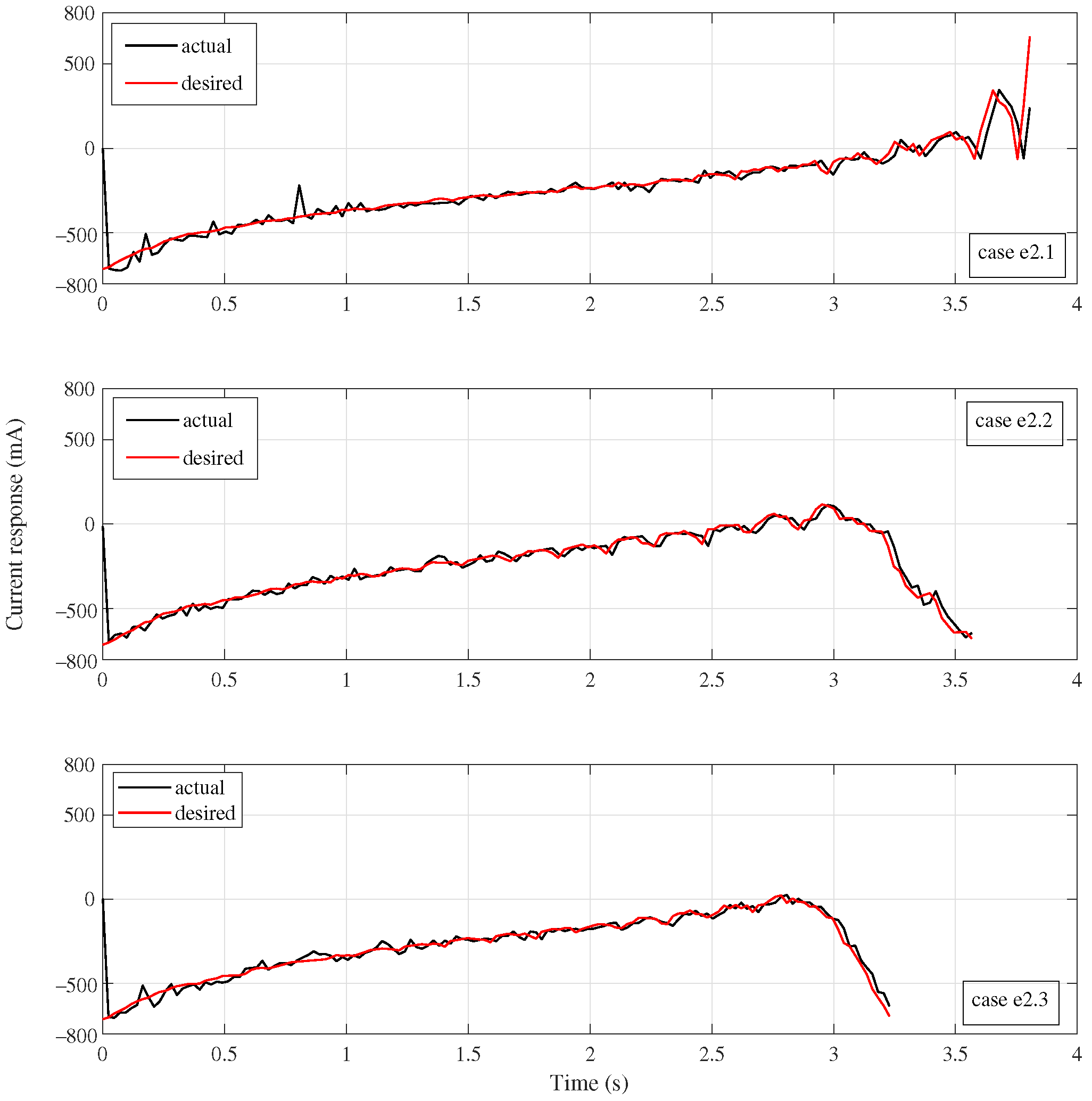
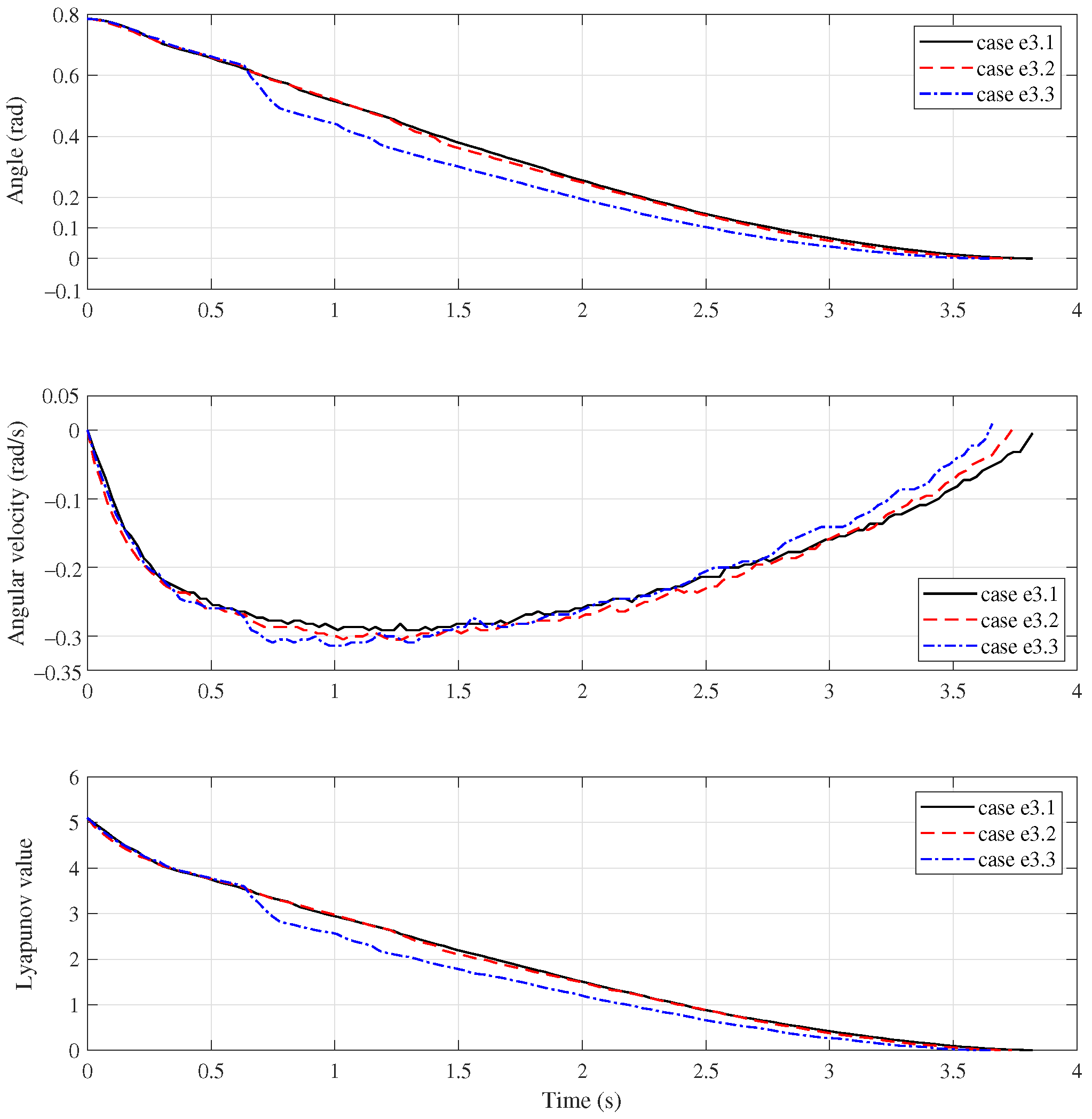
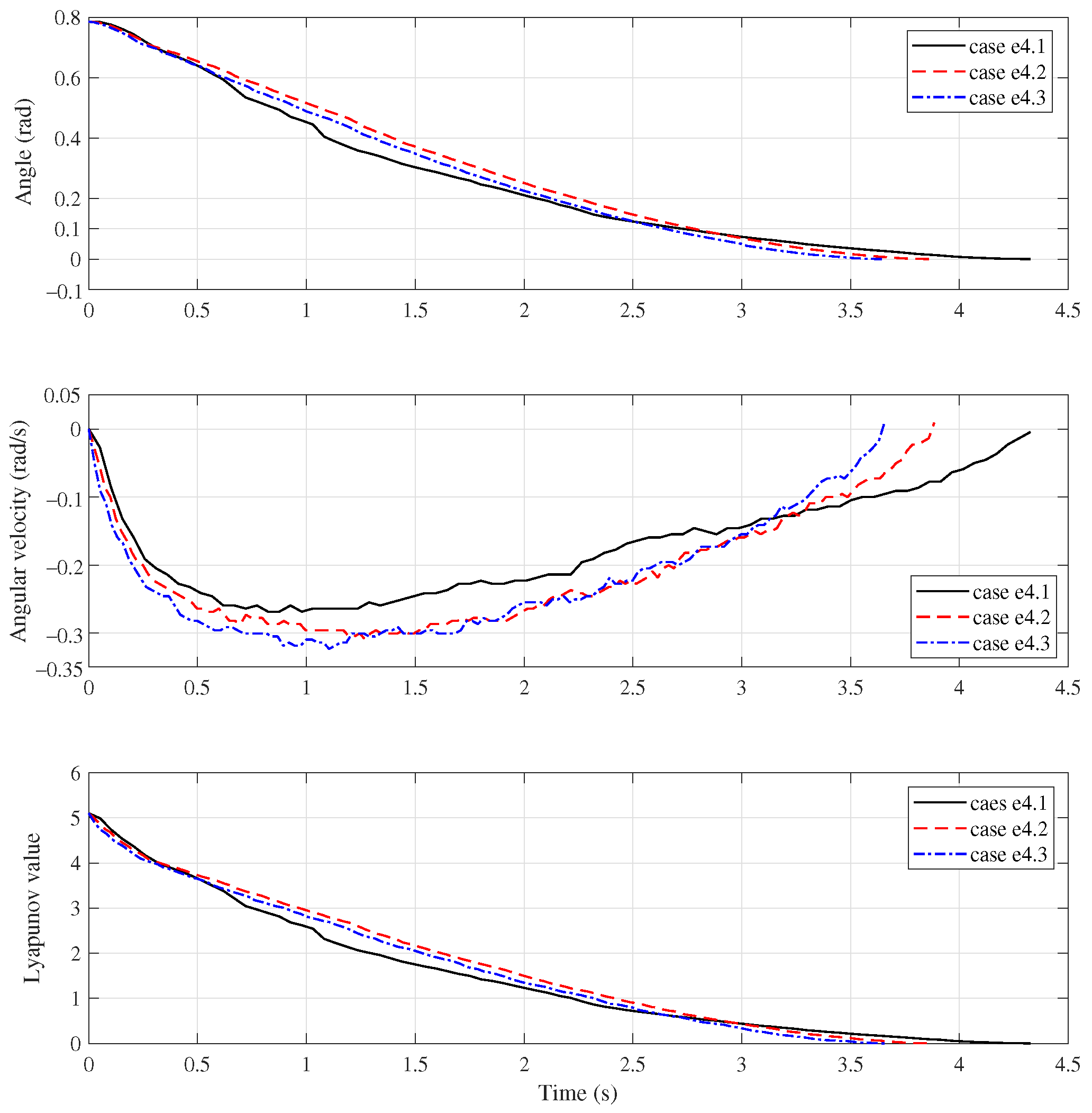
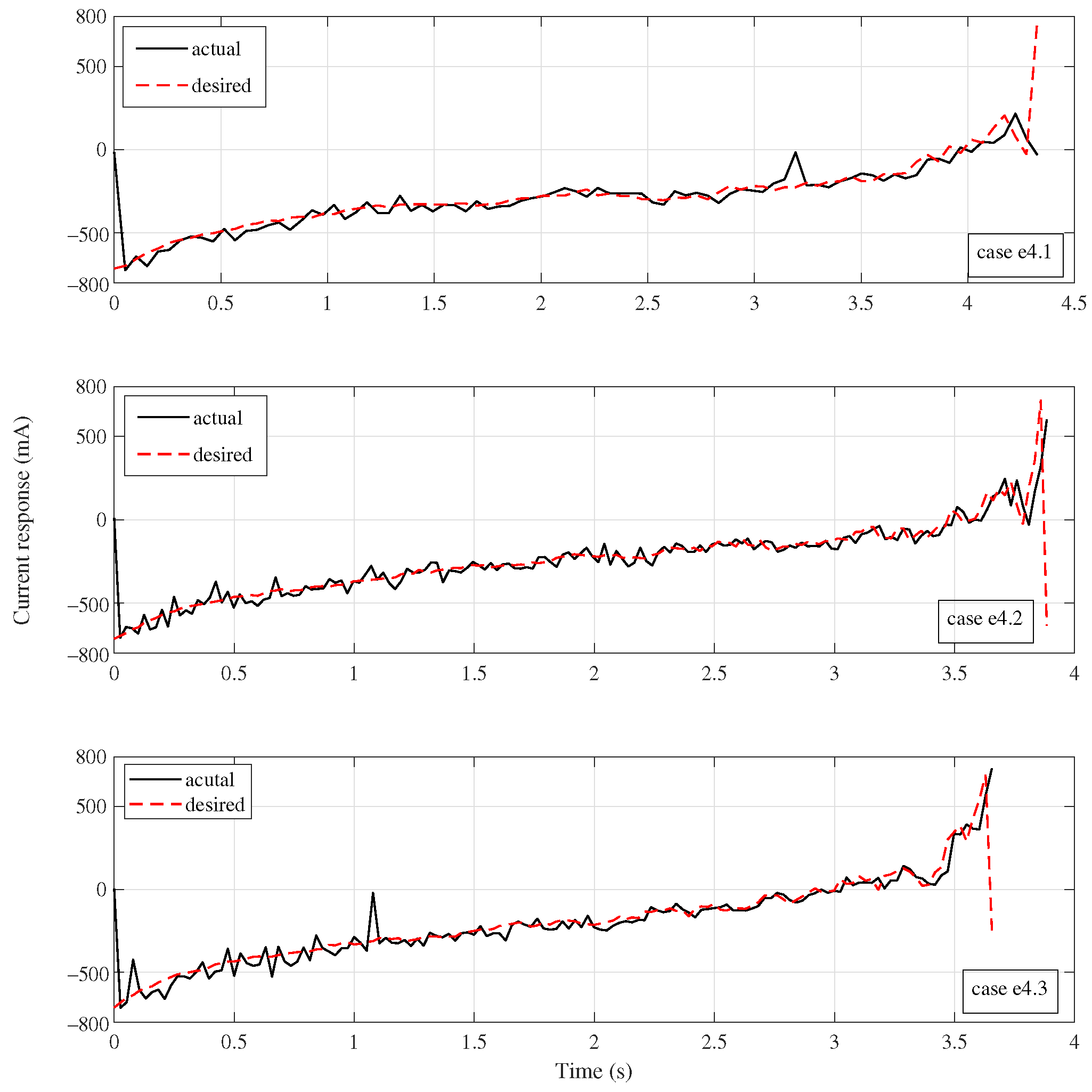
4.4. Experiment Results and Analysis
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Toda, M. Robust Motion Control of Oscillatory-Base Manipulators, 1st ed.; Springer International Publishing: Cham, Switzerland, 2016; pp. 7–9. [Google Scholar]
- Zhang, Y.; Liu, Y.; Xie, Z.; Liu, Y.; Cao, B.; Liu, H. Visual servo control of the macro/micro manipulator with base vibration suppression and backlash compensation. Appl. Sci. 2022, 12, 8386. [Google Scholar] [CrossRef]
- Palma, P.; Seweryn, K.; Rybus, T. Impedance control using selected compliant prismatic joint in a free-floating space manipulator. Aerospace 2022, 9, 406. [Google Scholar] [CrossRef]
- Zhang, S.; Cheng, S.; Jin, Z. A control method of mobile manipulator based on null-space task planning and hybrid control. Machines 2022, 10, 1222. [Google Scholar] [CrossRef]
- González-García, J.; Narcizo-Nuci, N.A.; Gómez-Espinosa, A.; García-Valdovinos, L.G.; Salgado-Jiménez, T. Finite-time controller for coordinated navigation of unmanned underwater vehicles in a collaborative manipulation task. Sensors 2023, 23, 239. [Google Scholar] [CrossRef] [PubMed]
- Martin-Abadal, M.; Oliver-Codina, G.; Gonzalez-Cid, Y. Real-time pipe and valve characterisation and mapping for autonomous underwater intervention tasks. Sensors 2022, 22, 8141. [Google Scholar] [CrossRef]
- Cao, Y.; Li, T.; Hao, L. Nonlinear model predictive control of shipboard boom cranes based on moving horizon state estimation. J. Mar. Sci. Eng. 2023, 11, 4. [Google Scholar] [CrossRef]
- Chen, M.; Yuan, G.; Li, C.B.; Zhang, X.; Li, L. Dynamic analysis and extreme response evaluation of lifting operation of the offshore wind turbine jacket foundation using a floating crane vessel. J. Mar. Sci. Eng. 2022, 10, 2023. [Google Scholar] [CrossRef]
- Guo, Y.; Hou, B. Implicit Lyapunov function-based tracking control of a novel ammunition autoloader with base oscillation and payload uncertainty. Nonlinear Dyn. 2017, 87, 741–753. [Google Scholar]
- Guo, Y.; Xi, B.; Huynh, V.T.; Wang, Z. Robust tracking control of MBT autoloaders with oscillatory chassis and compliant actuators. Nonlinear Dyn. 2019, 99, 2185–2200. [Google Scholar] [CrossRef]
- Wang, S.; Yang, Y.; Li, G.; Du, H.; Wei, Y. Microscopic vibration suppression for a high-speed macro-micro manipulator with parameter perturbation. Mech. Syst. Signal Process. 2022, 179, 109332. [Google Scholar] [CrossRef]
- Yang, Y.; Wei, Y.; Lou, J.; Fu, L.; Fang, S.; Chen, T. Dynamic modeling and adaptive vibration suppression of a high-speed macro-micro manipulator. J. Sound Vib. 2018, 442, 318–342. [Google Scholar] [CrossRef]
- Chen, T.; Lou, J.; Yang, Y.; Ren, Z.; Xu, C. Vibration suppression of a high-speed macro-micro integrated system using computational optimal control. IEEE Trans. Ind. Electron. 2020, 67, 7841–7850. [Google Scholar] [CrossRef]
- Park, H.C.; Chakir, S.; Kim, Y.B.; Lee, D.H. A robust payload control system design for offshore cranes: Experimental study. Electronics 2021, 10, 462. [Google Scholar] [CrossRef]
- Qian, Y.; Fang, Y. Switching logic-based nonlinear feedback control of offshore ship-mounted tower cranes: A disturbance observer-based approach. IEEE Trans. Autom. Sci. Eng. 2019, 16, 1–12. [Google Scholar] [CrossRef]
- Wang, Y.; Wang, S.; Wei, Q.; Tan, M.; Zhou, C.; Yu, J. Development of an underwater manipulator and its free-floating autonomous operation. IEEE ASME Trans. Mechatron. 2016, 21, 815–824. [Google Scholar] [CrossRef]
- Xue, G.; Liu, Y.; Shi, Z.; Guo, L.; Li, Z. Research on trajectory tracking control of underwater vehicle manipulator system based on model-free adaptive control method. J. Mar. Sci. Eng. 2022, 10, 652. [Google Scholar] [CrossRef]
- Toda, M. An H∞ control-based approach to robust control of mechanical systems with oscillatory bases. IEEE Trans. Rob. Autom. 2004, 20, 283–296. [Google Scholar] [CrossRef]
- Londhea, P.S.; Mohanb, S.; Patrea, B.M.; Waghmarea, L.M. Robust task-space control of an autonomous underwater vehicle manipulator system by PID-like fuzzy control scheme with disturbance estimator. Ocean Eng. 2017, 139, 1–13. [Google Scholar] [CrossRef]
- Cai, Y.; Zheng, S.; Liu, W.; Qu, Z.; Zhu, J.; Han, J. Sliding-mode control of ship-mounted Stewart platforms for wave compensation using velocity feedforward. Ocean Eng. 2021, 236, 109477. [Google Scholar] [CrossRef]
- Sato, M.; Toda, M. Robust motion control of an oscillatory-base manipulator in a global coordinate system. IEEE Trans. Ind. Electron. 2015, 62, 1163–1174. [Google Scholar] [CrossRef]
- Leban, F.A.; Diaz-Gonzalez, J.; Parker, G.G.; Zhao, W. Inverse kinematic control of a dual crane system experiencing base motion. IEEE Trans. Control Syst. Technol. 2015, 23, 331–339. [Google Scholar] [CrossRef]
- Sun, N.; Fang, Y.; Chen, H.; Fu, Y.; Lu, B. Nonlinear stabilizing control for ship-mounted cranes with ship roll and heave movements: Design, analysis, and experiments. IEEE Trans. Syst. Man Cybern. 2018, 48, 1781–1793. [Google Scholar] [CrossRef]
- Sun, N.; Yang, T.; Chen, H.; Fang, Y. Dynamic feedback antiswing control of shipboard cranes without velocity measurement: Theory and hardware experiments. IEEE Trans. Industr. Inform. 2019, 15, 2879–2891. [Google Scholar] [CrossRef]
- Wang, H.; Xie, Y. Passivity based adaptive Jacobian tracking for free-floating space manipulators without using spacecraft acceleration. Automatica 2009, 45, 1510–1517. [Google Scholar] [CrossRef]
- Chu, Z.; Cui, J.; Sun, F. Fuzzy adaptive disturbance-observer-based robust tracking control of electrically driven free-floating space manipulator. IEEE Syst. J. 2014, 8, 343–352. [Google Scholar] [CrossRef]
- Guo, Y.; Xi, B.; Mei, R.; Xu, S.; Wang, Z. Singular-perturbed control for a novel SEA-actuated MBT autoloader subject to chassis oscillations. Nonlinear Dyn. 2020, 101, 2263–2281. [Google Scholar] [CrossRef]
- Nie, S.; Qian, F.; Chen, L.; Tian, L.; Zou, Q. Barrier Lyapunov functions-based dynamic surface control with tracking error constraints for ammunition manipulator electro-hydraulic system. Def. Technol. 2021, 17, 836–845. [Google Scholar] [CrossRef]
- Wang, X.; Hou, B. Continuous time-varying feedback control of a robotic manipulator with base vibration and load uncertainty. J. Vib. Control 2021, 27, 392–403. [Google Scholar] [CrossRef]
- Izadbakhsh, A. Robust control design for rigid-link flexible-joint electrically driven robot subjected to constraint: Theory and experimental verification. Nonlinear Dyn. 2016, 85, 751–765. [Google Scholar] [CrossRef]
- Fan, Y.; Huang, H.; Yang, C. Fixed-time incremental neural control for manipulator based on composite learning with input saturation. Actuators 2022, 11, 373. [Google Scholar] [CrossRef]
- Liu, S.; Yang, H.; Liu, Z.; Zhang, Z.; Li, Y. Observer-based independent joint control for a coupled rigid-flexible manipulator with actuator saturation based on distributed parameter model. J. Vib. Control 2022, 10775463221132877. [Google Scholar] [CrossRef]
- Cao, F.; Liu, J. Boundary control for a rigid-flexible manipulator with input constraints based on ordinary differential equations-partial differential equations model. J. Comput. Nonlinear Dyn. 2019, 14, 094501. [Google Scholar] [CrossRef]
- Chernousko, F.L.; Ananievski, I.M.; Reshmin, S.A. Control of Nonlinear Dynamical Systems, 1st ed.; Springer: Berlin, Germany, 2008; pp. 213–227. [Google Scholar]
- Spong, M.W.; Hutchinson, S.; Vidyasagar, M. Robot Modeling and Control, 2nd ed.; John Wiley and Sons Ltd.: Hoboken, NJ, USA, 2020; pp. 19–27. [Google Scholar]
- Ananevskii, I.M. Synthesis of continuous control of a mechanical system with an unknown inertia matrix. J. Comput. Syst. Sci. 2006, 45, 356–367. [Google Scholar] [CrossRef]

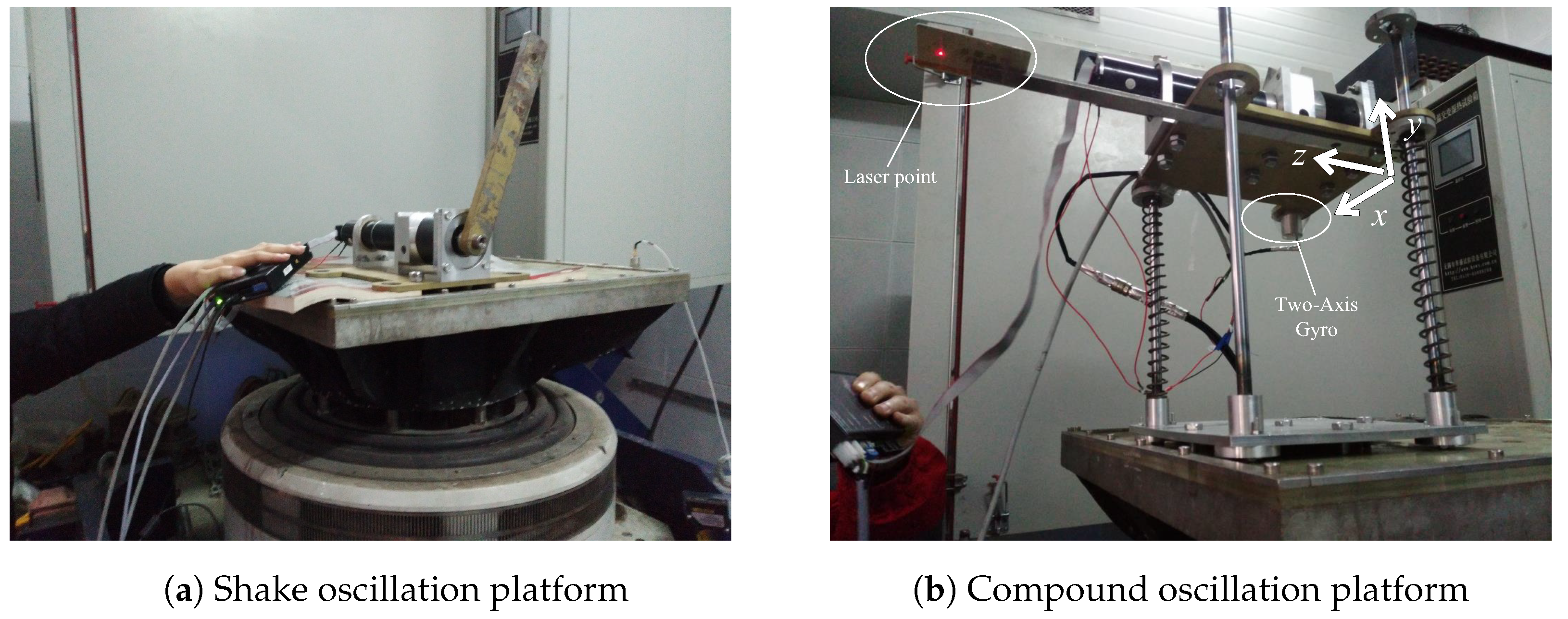


| Group NO. | Case NO. | Control | Oscillation | Payload | |
|---|---|---|---|---|---|
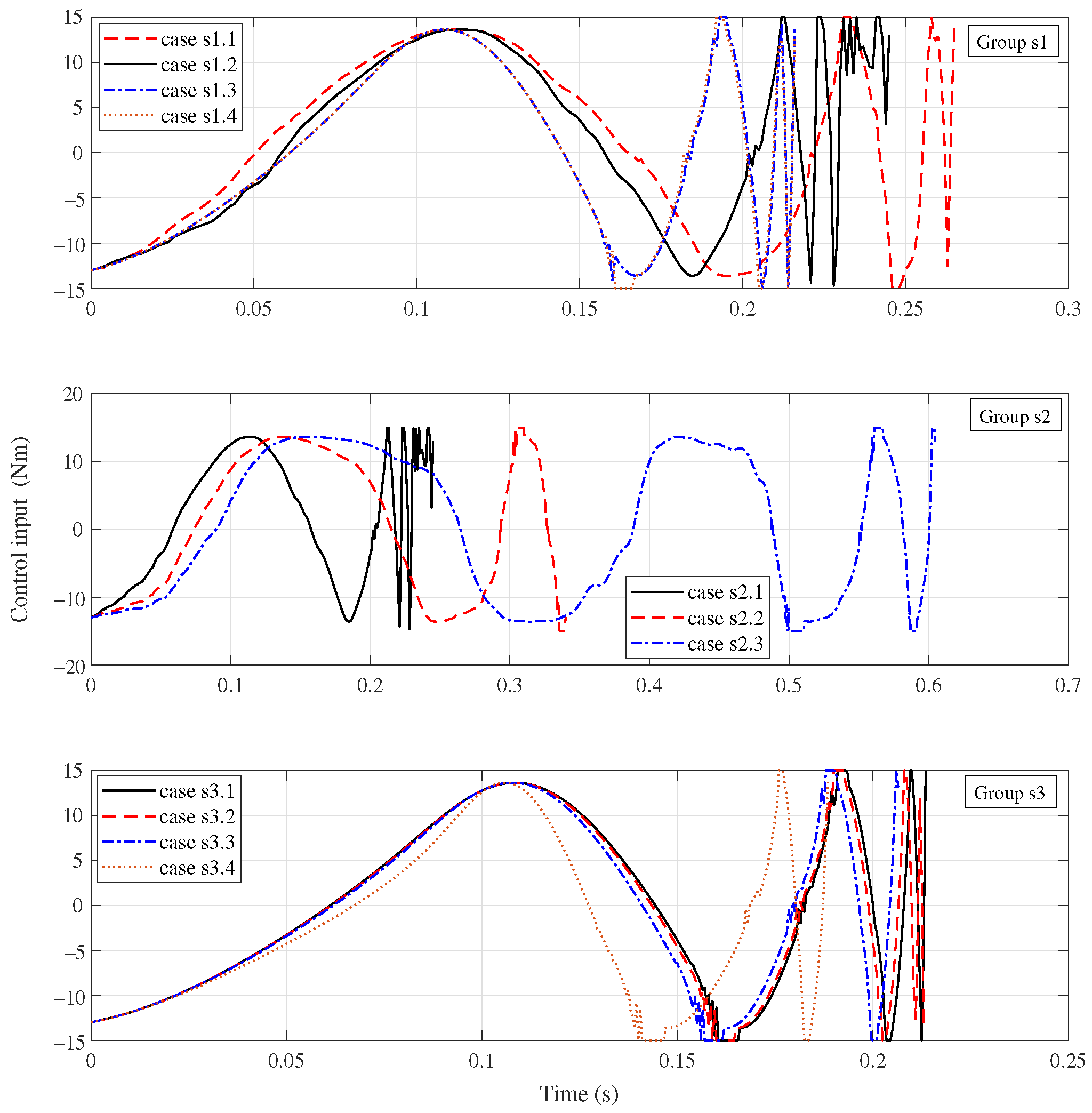
| Group s1 | case s1.1 | IL PD | shake | real | 0 kg |
| case s1.2 | IL PD | pitch | real | 0 kg | |
| case s1.3 | IL PD | roll | real | 0 kg | |
| case s1.4 | IL PD | no | 0 kg | ||
| Group s2 | case s2.1 | IL PD | pitch | real | 0 kg |
| case s2.2 | IL PD | pitch | real | 0.4 kg | |
| case s2.3 | IL PD | pitch | real | 0.8kg | |
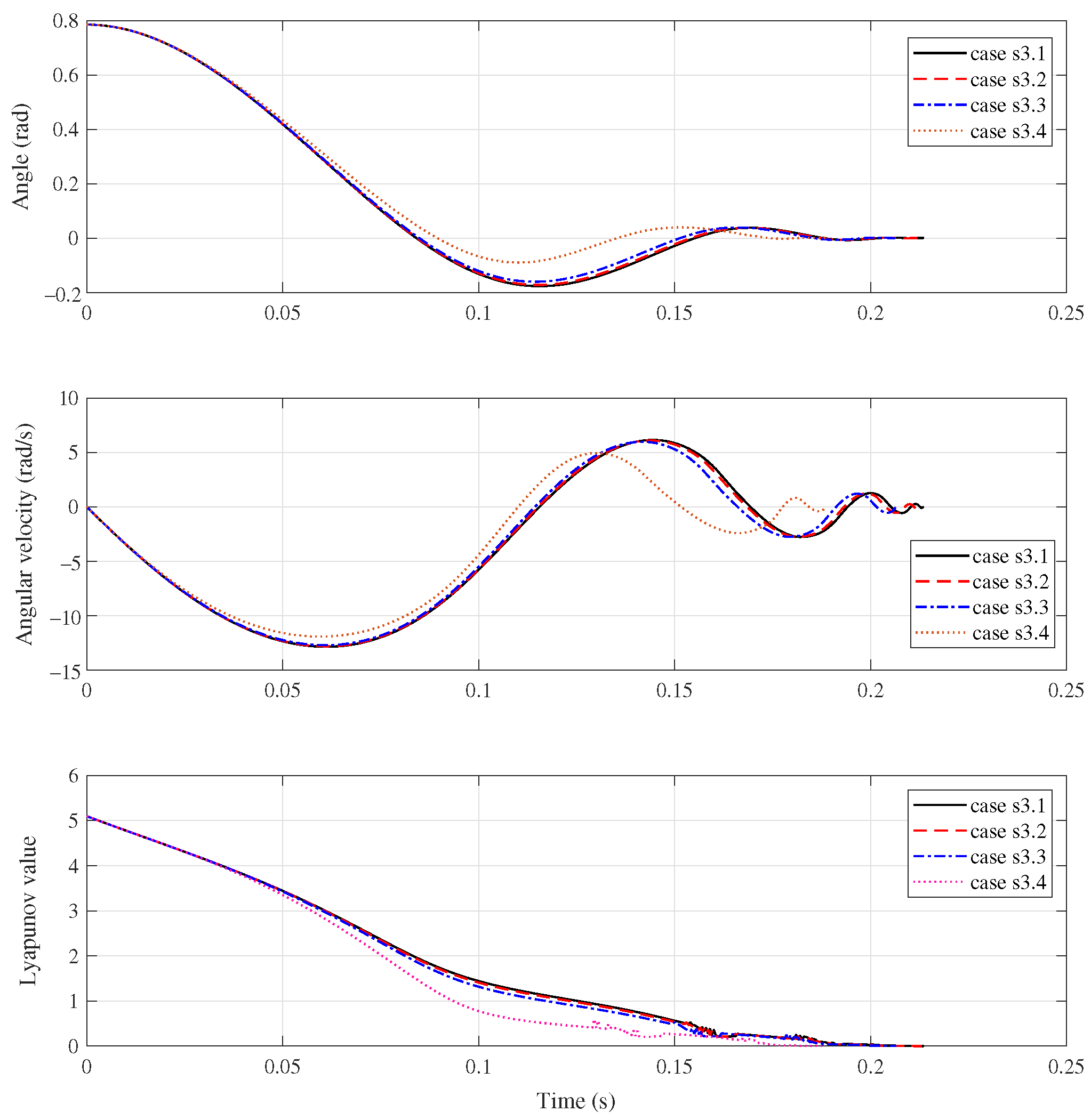
| Group s3 | case s3.1 | IL PD | pitch | sin(t) | 0 kg |
| case s3.2 | IL PD | pitch | sin(t) | 0 kg | |
| case s3.3 | IL PD | pitch | sin(t) | 0 kg | |
| case s3.4 | IL PD | pitch | sin(2t) | 0 kg | |
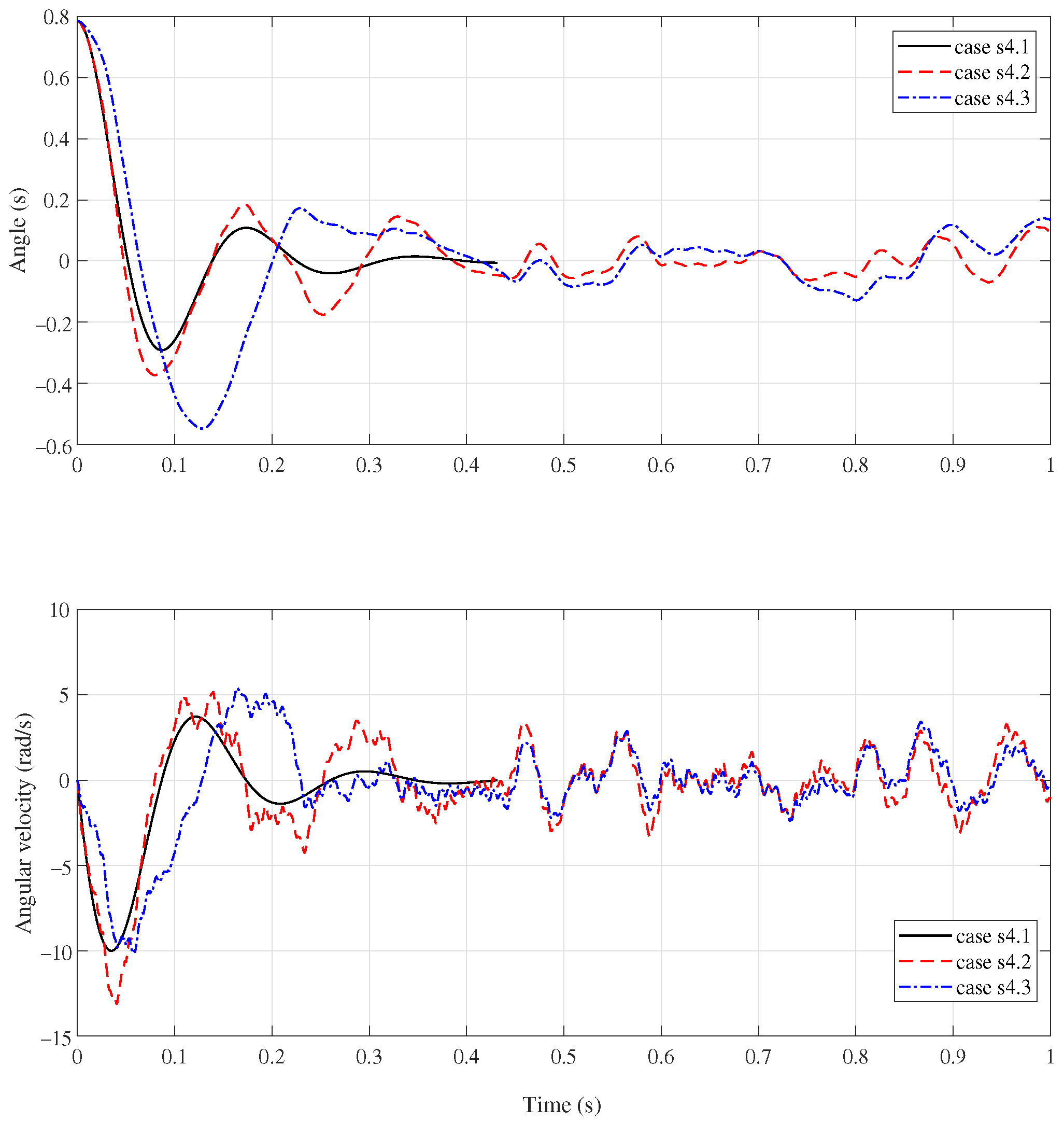
| Group s4 | case s4.1 | PD | no | 0 kg | |
| case s4.2 | PD | pitch | real | 0 kg | |
| case s4.3 | PD | pitch | real | 0.8 kg |
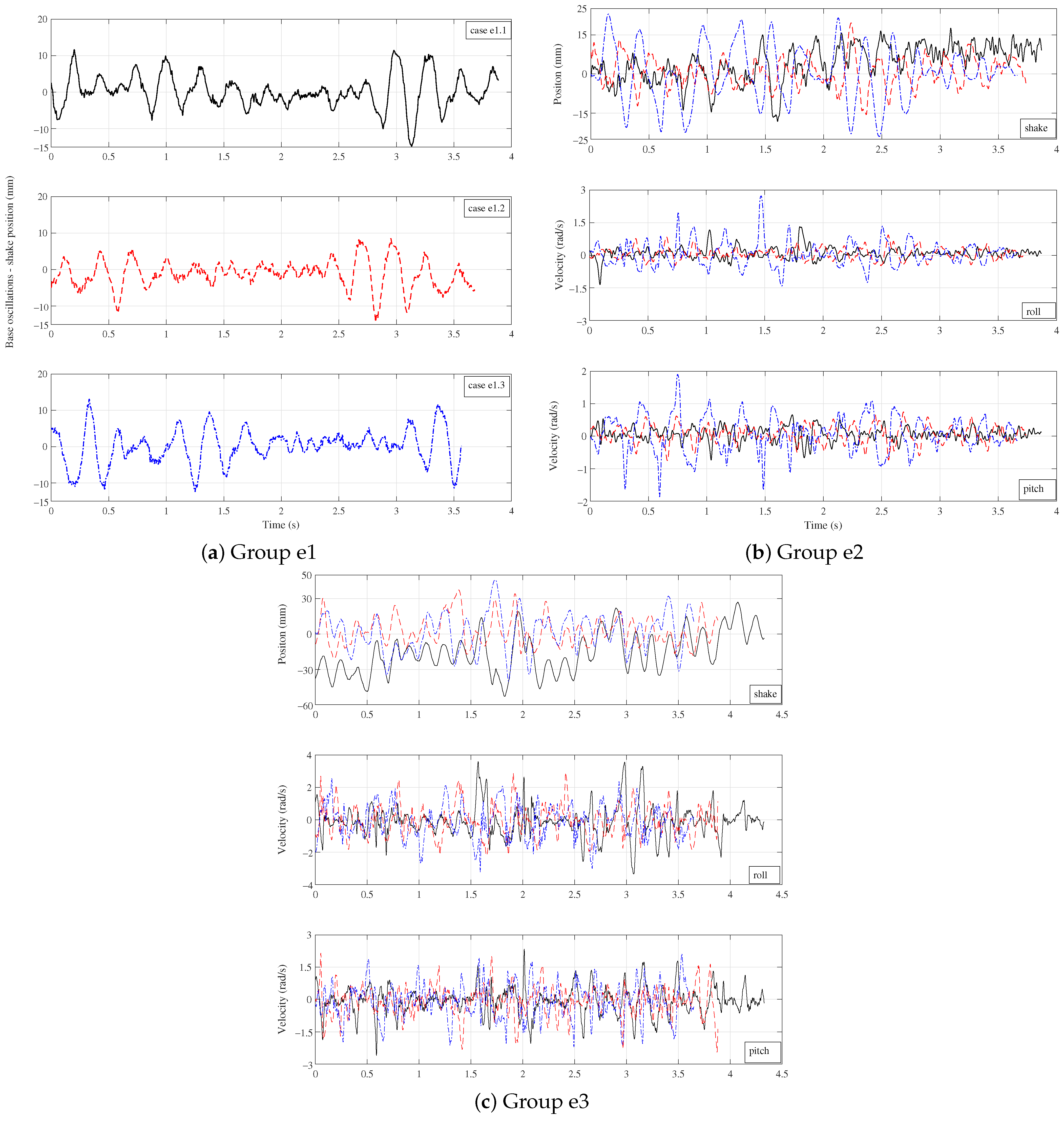
| Group NO. | Case NO. | Oscillation | Payload | |
|---|---|---|---|---|
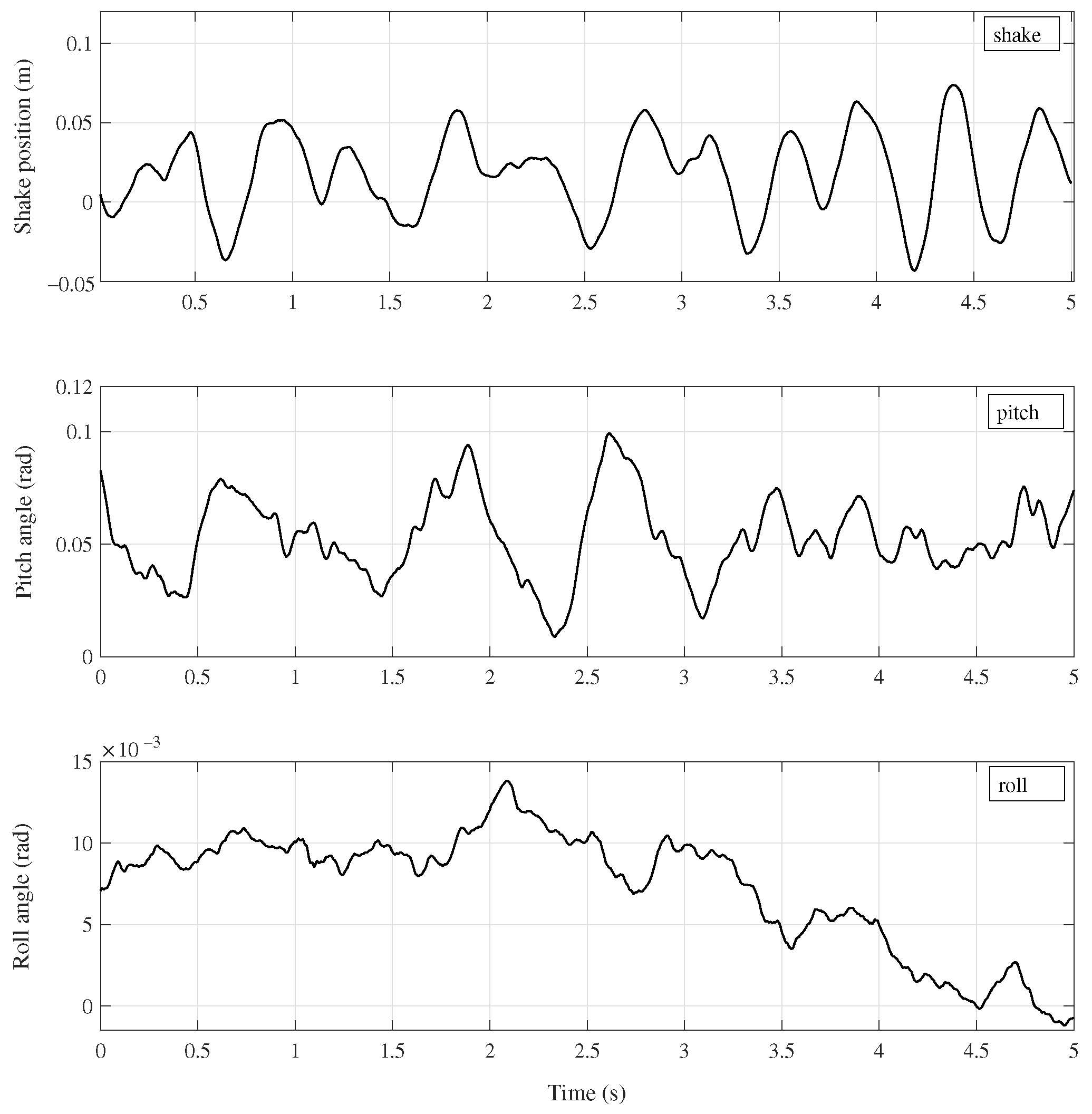
| Group e1 | case e1.1 | shake | real | 0 kg |
| case e1.2 | shake | real | 0.4 kg | |
| case e1.3 | shake | real | 0.8 kg | |
| Group e2 | case e2.1 | shake | sinusoidal | 0 kg |
| case e2.2 | shake | sinusoidal | 0.4 kg | |
| case e2.3 | shake | sinusoidal | 0.8kg | |
| Group e3 | case e3.1 | Compound | real | 0 kg |
| case e3.2 | Compound | real | 0.4 kg | |
| case e3.3 | Compound | real | 0.8 kg | |
| Group e4 | case e4.1 | Compound | sinusoidal | 0 kg |
| case e4.2 | Compound | sinusoidal | 0.4 kg | |
| case e4.3 | Compound | sinusoidal | 0.8 kg |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Guo, Y.; Hou, B.; Hao, Z.; Wang, Z.; Huynh, V.T. Bounded Positioning Control of Manipulators Subject to Base Oscillation and Payload Uncertainty. Machines 2023, 11, 253. https://doi.org/10.3390/machines11020253
Guo Y, Hou B, Hao Z, Wang Z, Huynh VT. Bounded Positioning Control of Manipulators Subject to Base Oscillation and Payload Uncertainty. Machines. 2023; 11(2):253. https://doi.org/10.3390/machines11020253
Chicago/Turabian StyleGuo, Yufei, Baolin Hou, Zhiqiang Hao, Zhigang Wang, and Van Thanh Huynh. 2023. "Bounded Positioning Control of Manipulators Subject to Base Oscillation and Payload Uncertainty" Machines 11, no. 2: 253. https://doi.org/10.3390/machines11020253
APA StyleGuo, Y., Hou, B., Hao, Z., Wang, Z., & Huynh, V. T. (2023). Bounded Positioning Control of Manipulators Subject to Base Oscillation and Payload Uncertainty. Machines, 11(2), 253. https://doi.org/10.3390/machines11020253





