Design and Analysis of an Adaptive Obstacle-Overcoming Tracked Robot with Passive Swing Arms
Abstract
:1. Introduction
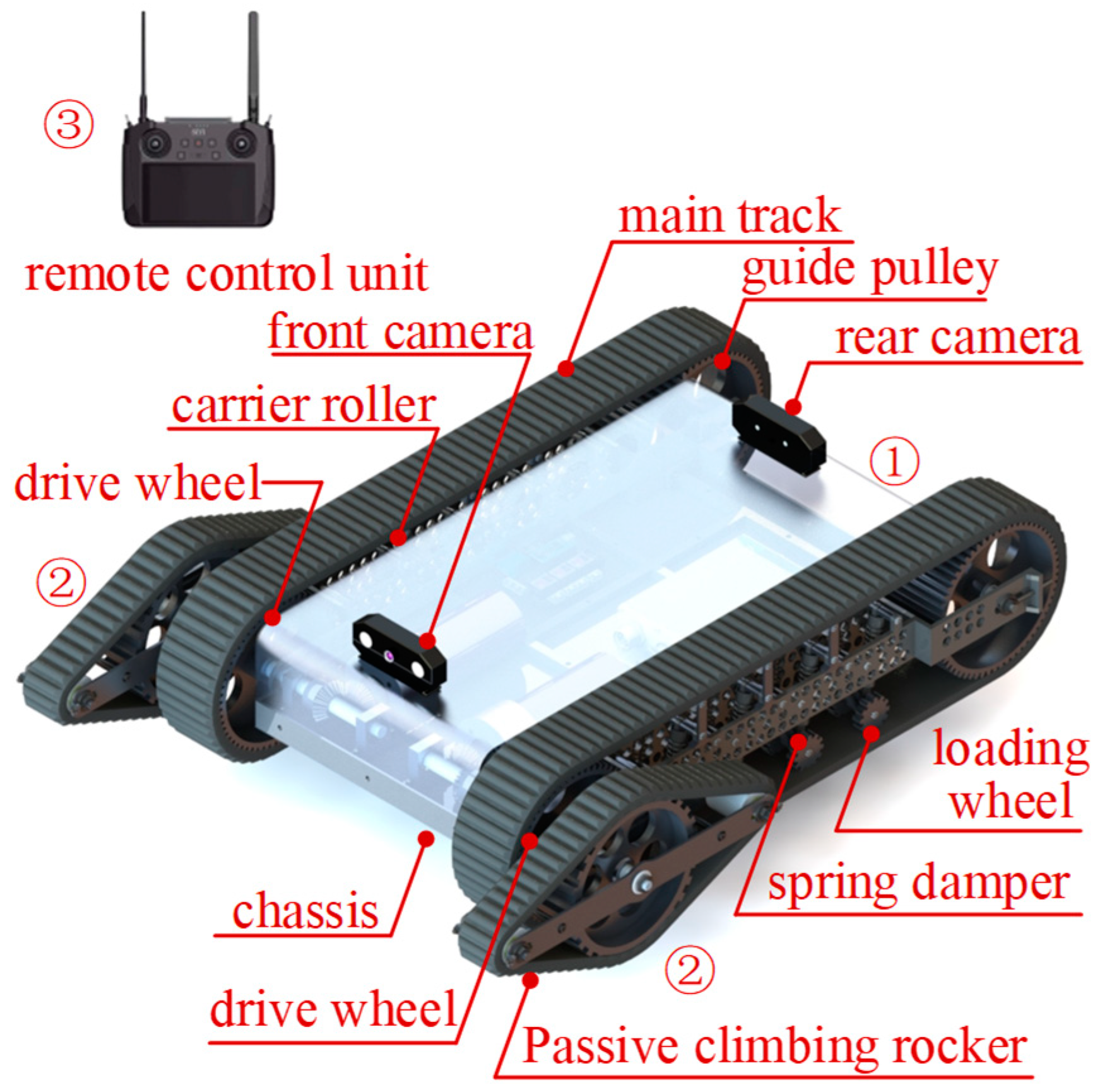
2. Structure Design of the Robot
2.1. The Composition of the Robot
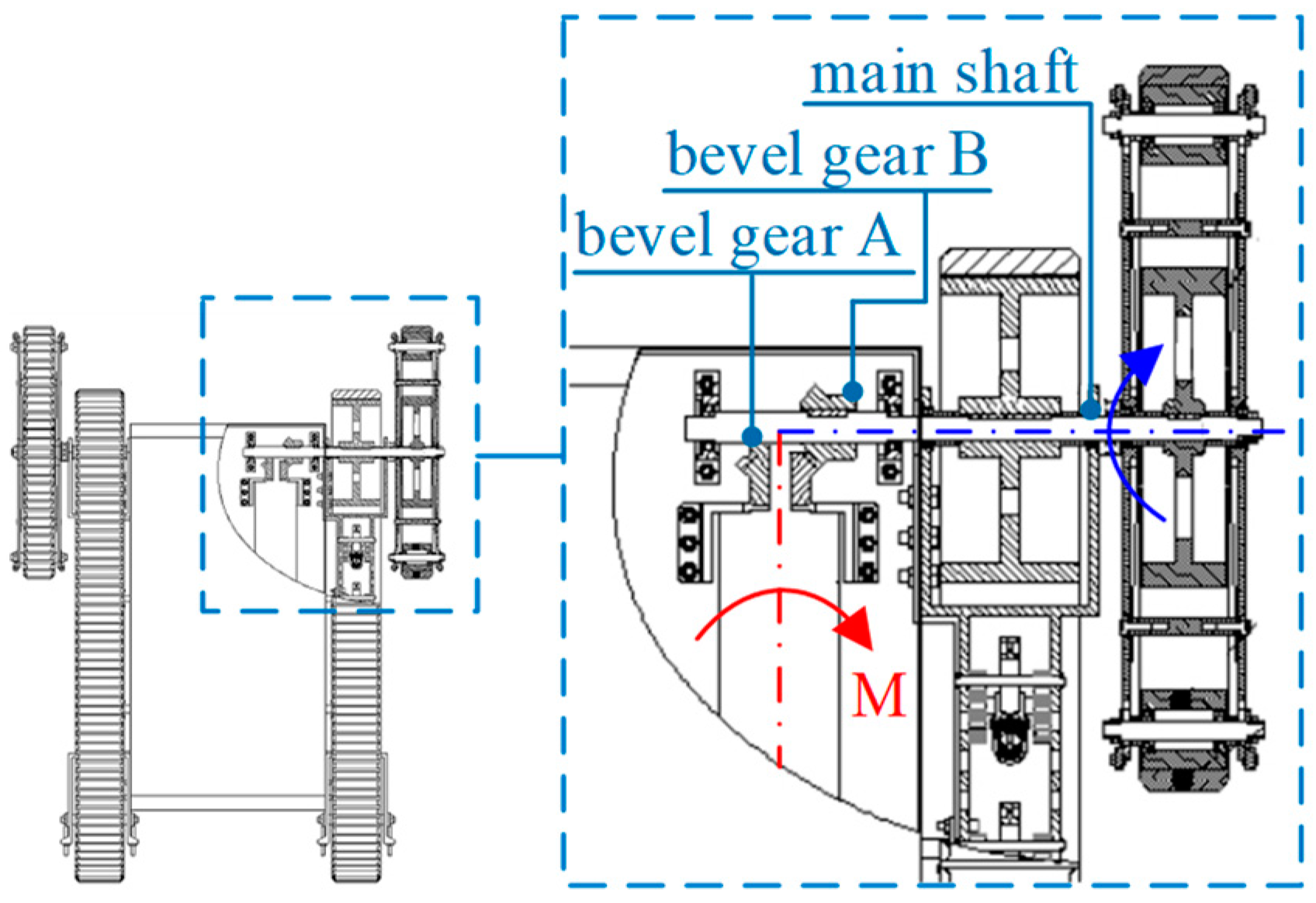
2.2. The Transmission System
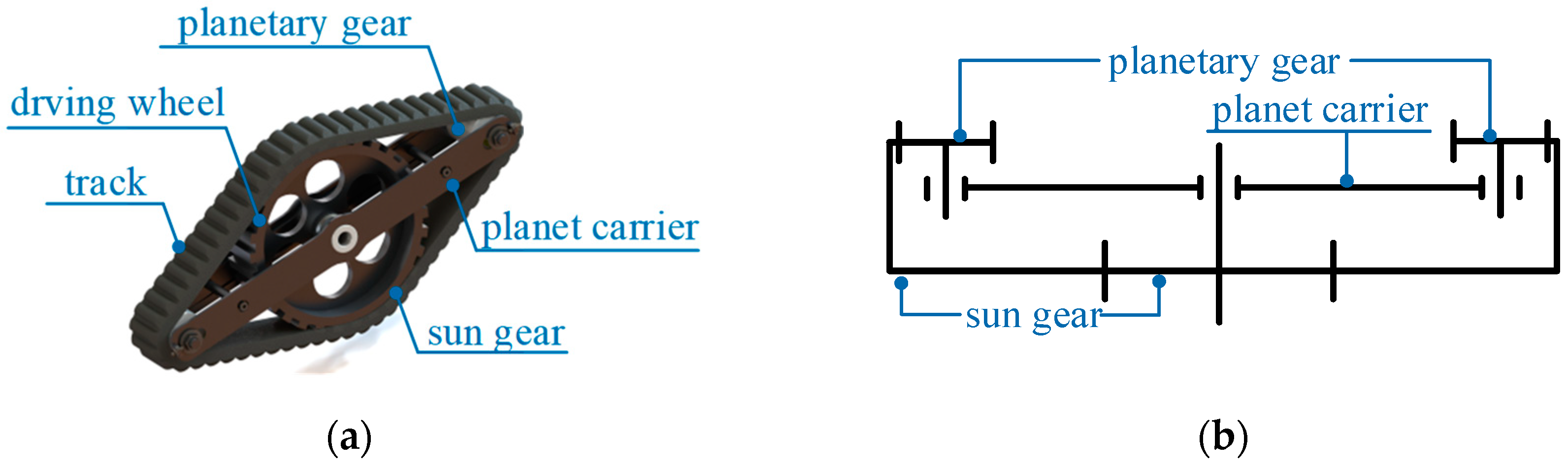
2.3. The Composition and Working Principle of Passive Swing Arms
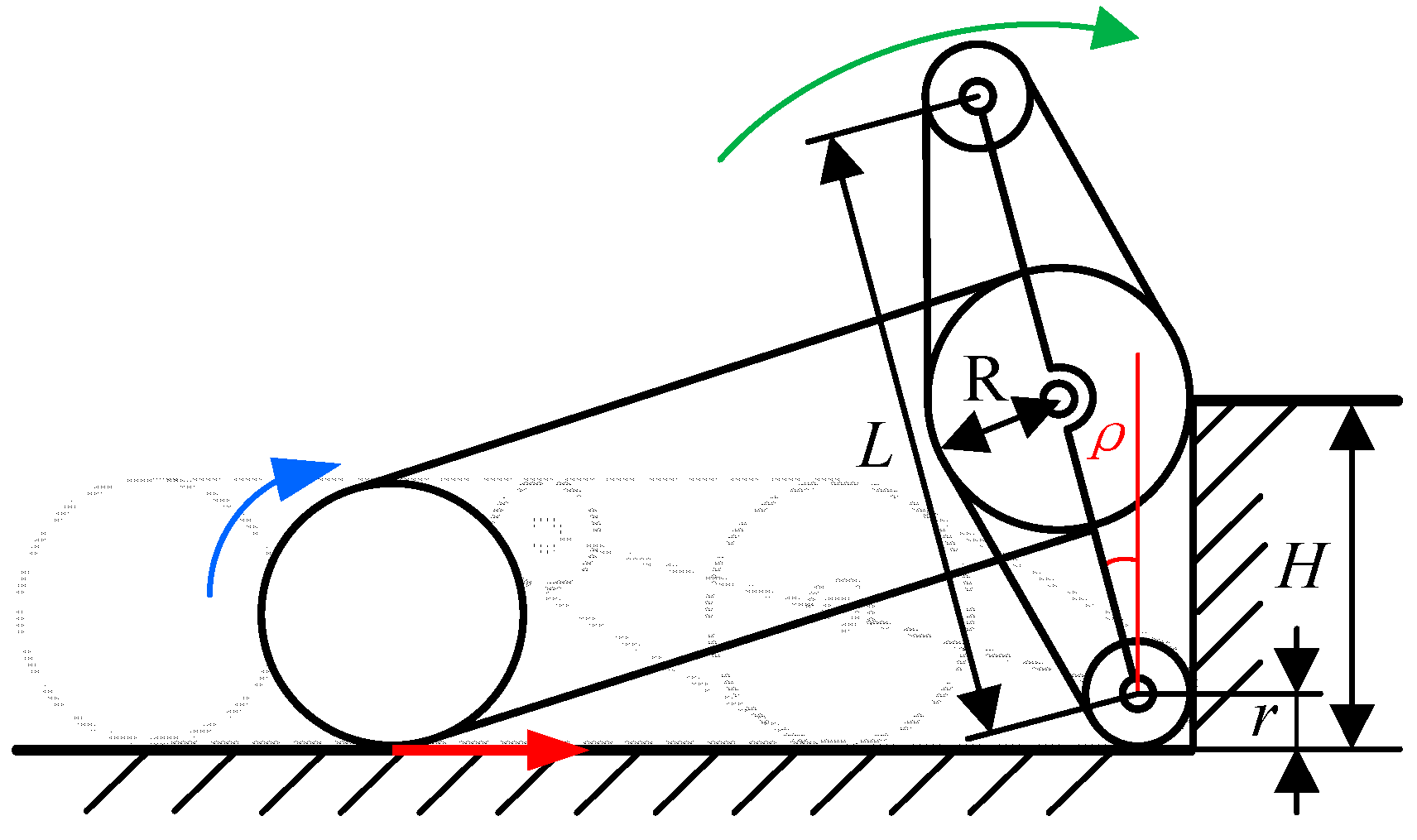
3. Obstacle-Overcoming Analysis
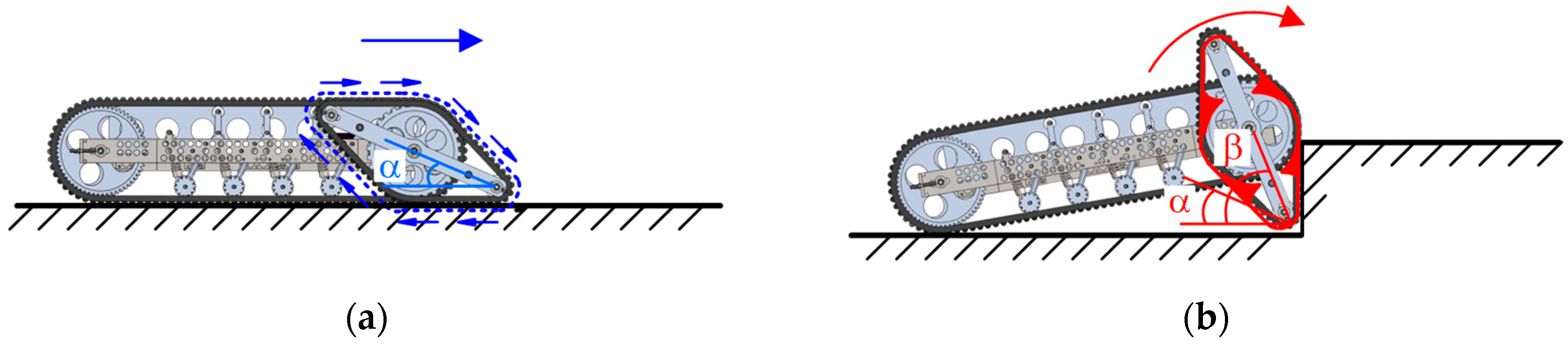
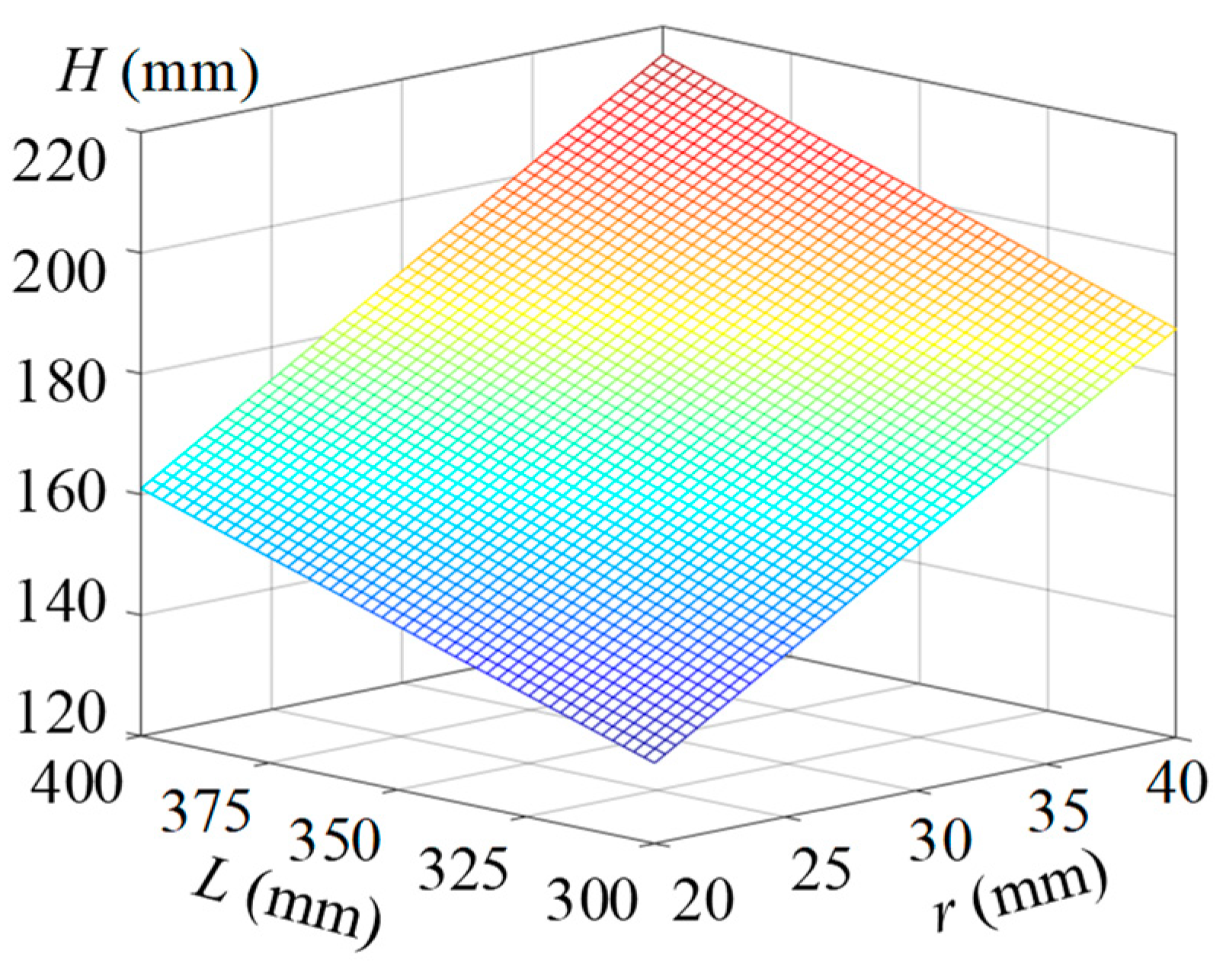
3.1. Kinematic Analysis of Single-Step-Climbing Ability
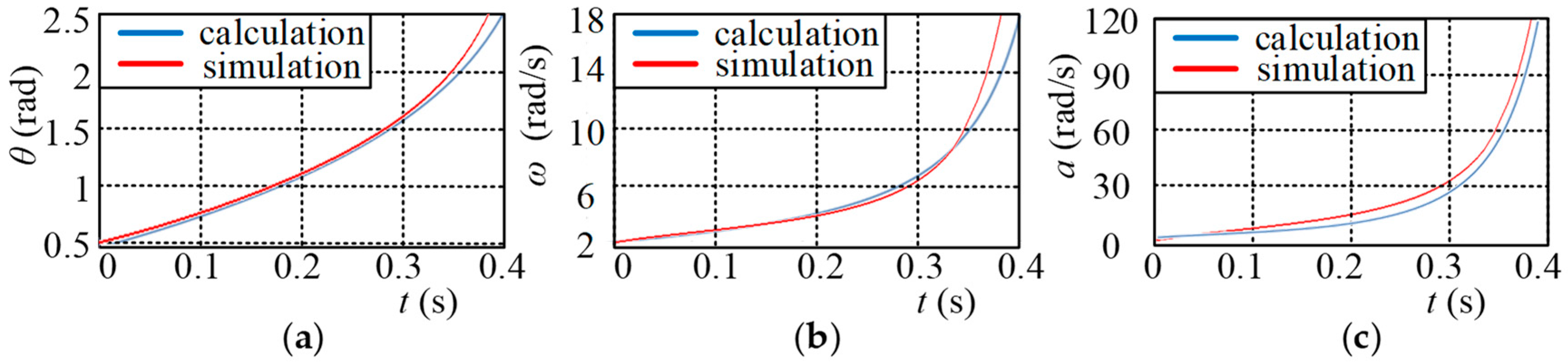
3.2. Dynamic Analysis of Single-Step Climbing
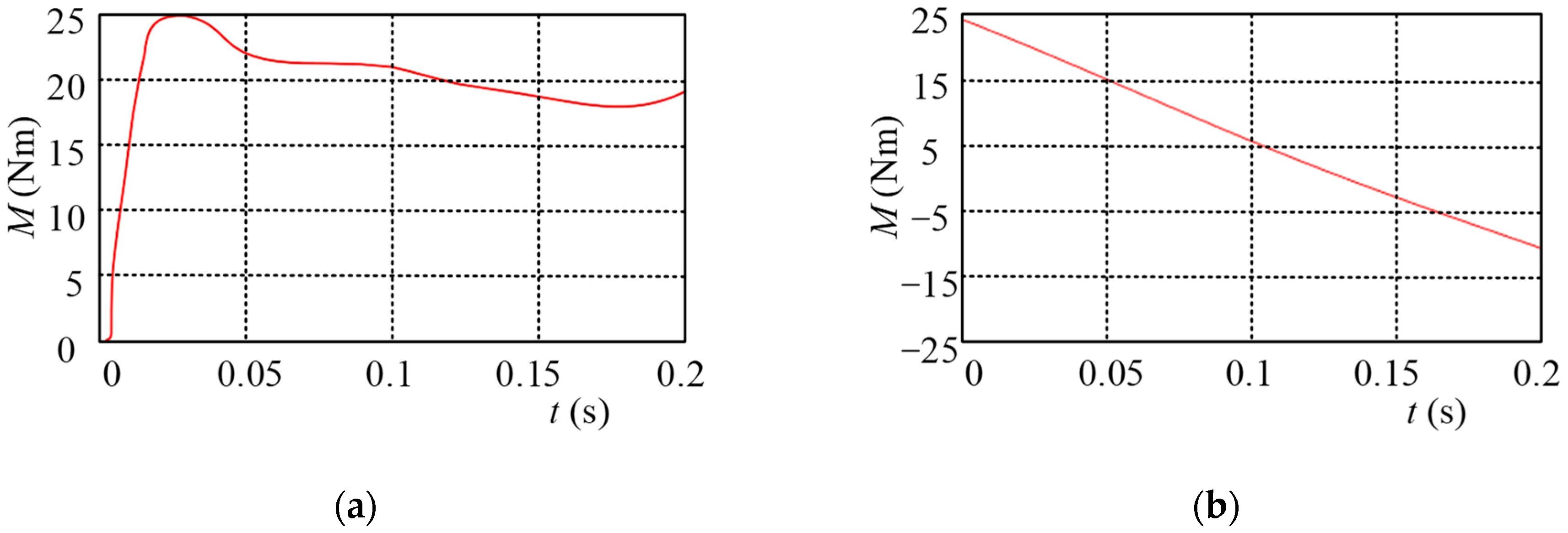
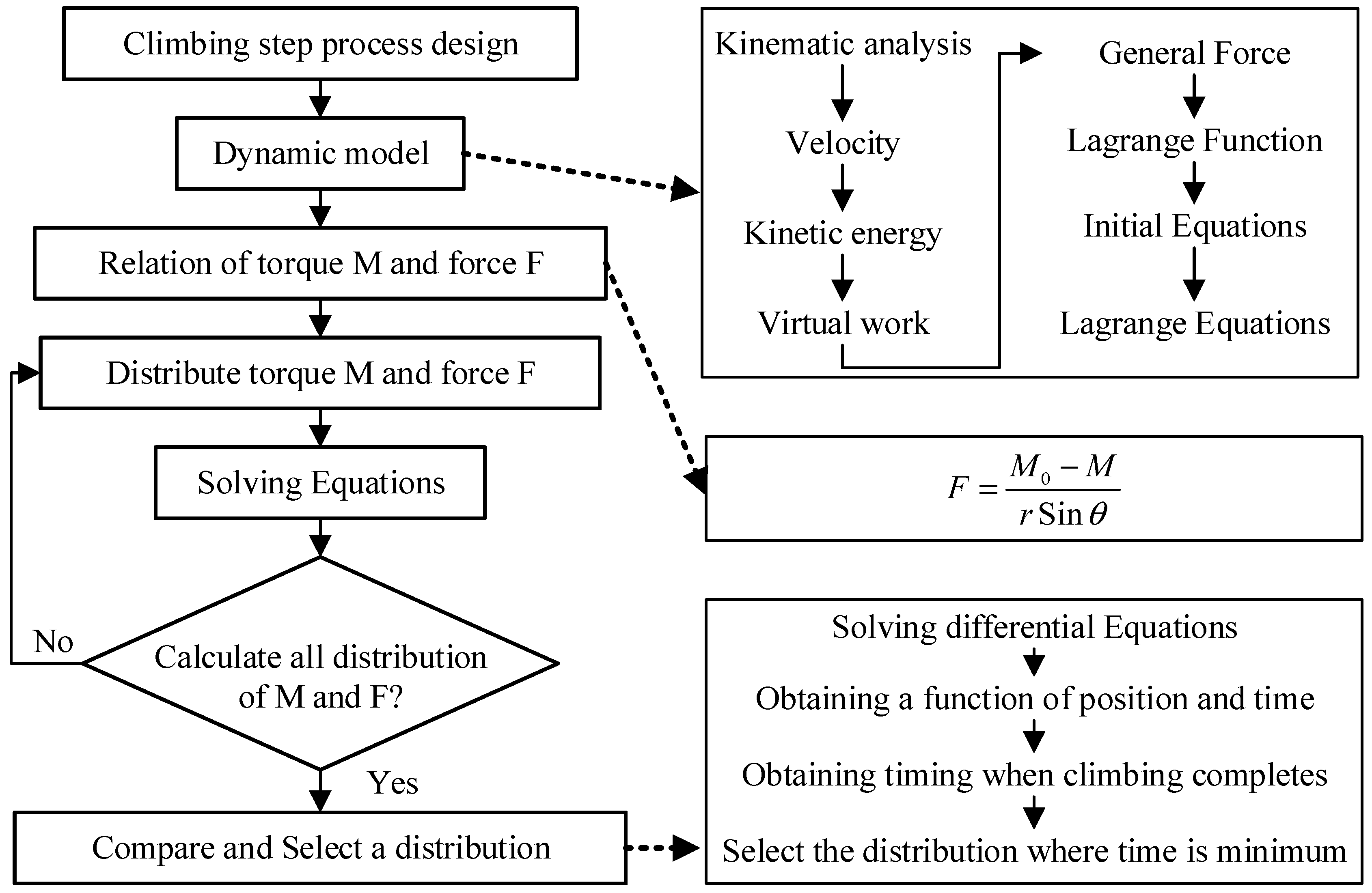
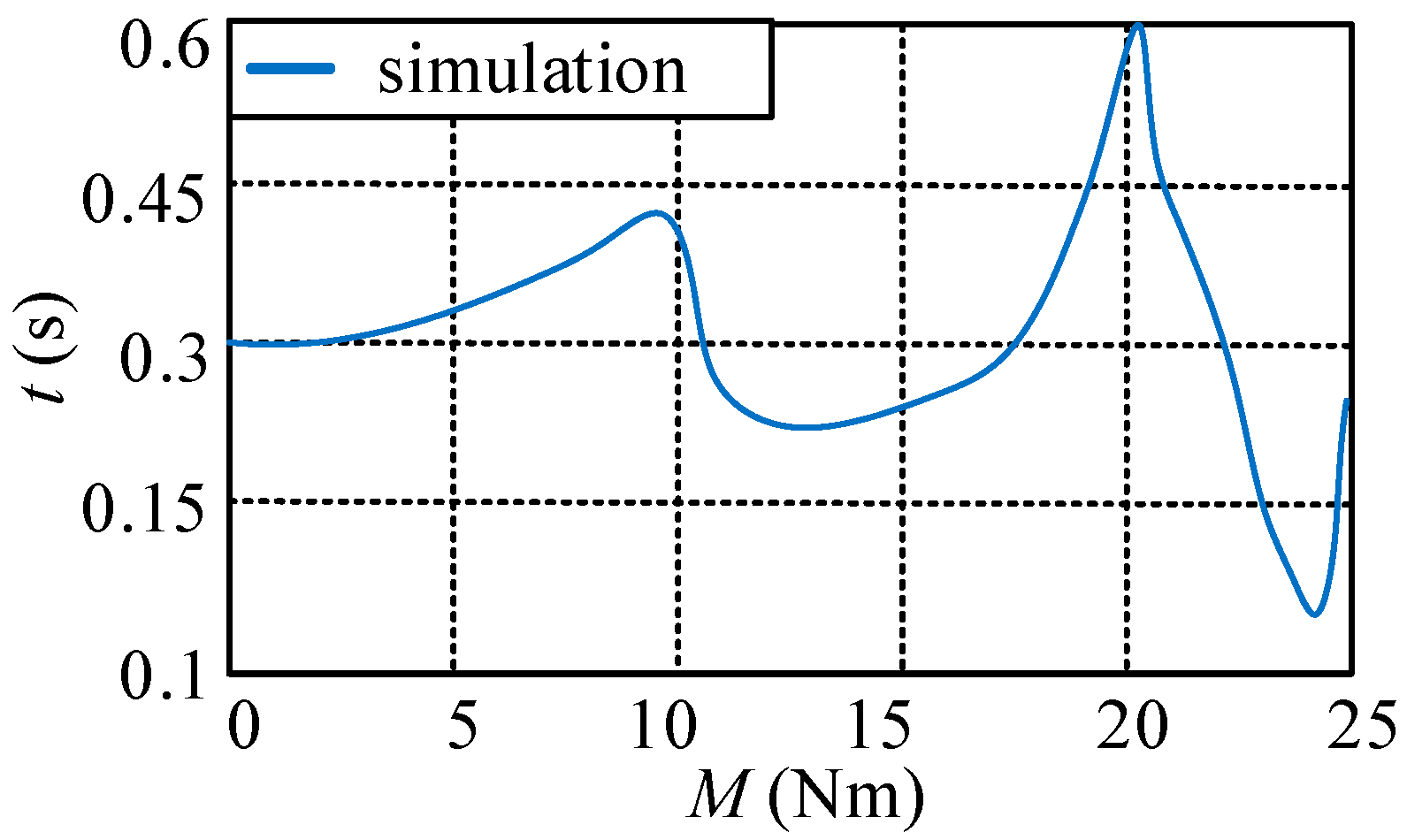
3.3. Driving Force Distribution Analysis
- (1)
- Solving the differential Equations;
- (2)
- Obtaining a function of position and time;
- (3)
- Obtaining the time when climbing completes;
- (4)
- Calculate all the distributions and select the distribution where the time is minimum.
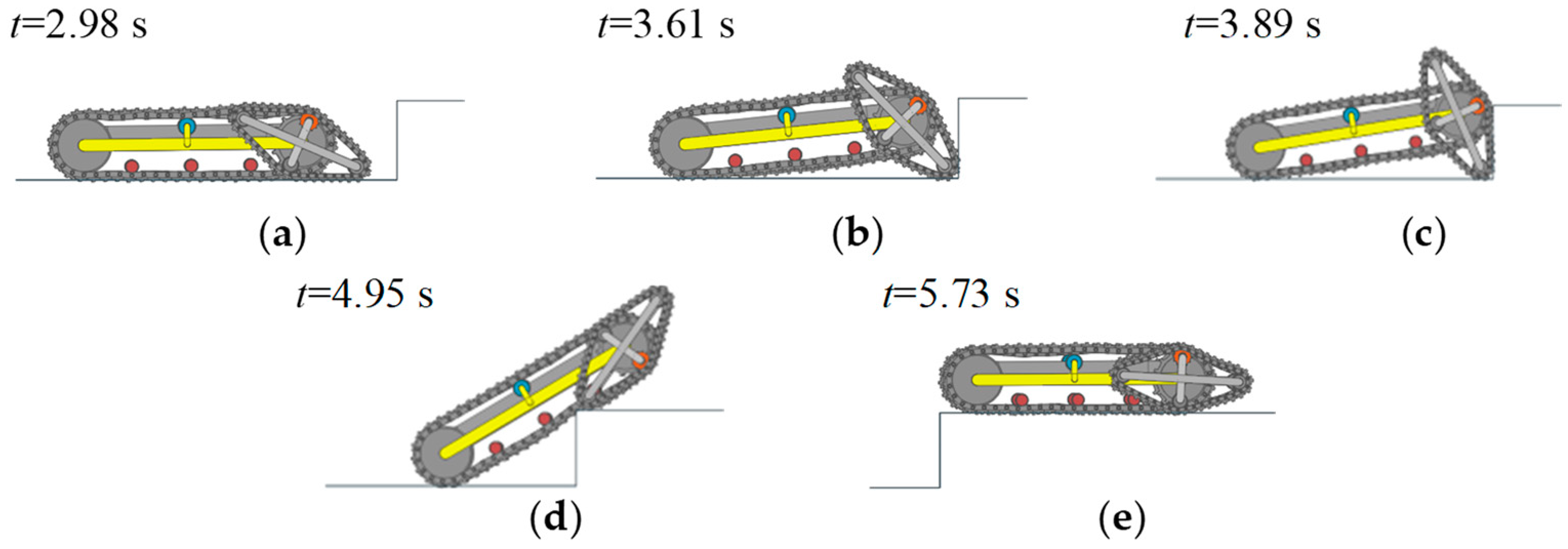
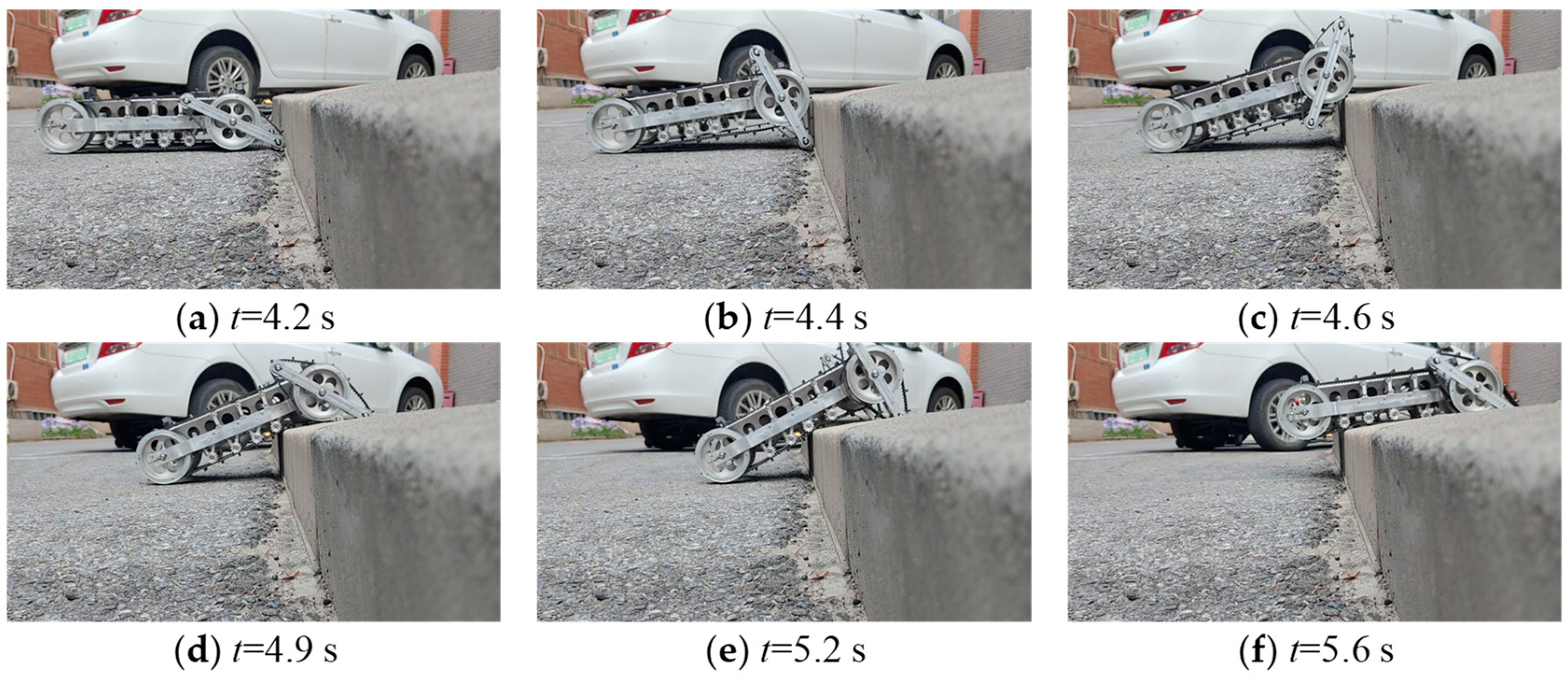
3.4. Simulation of the Process of Obstacle Overcoming
4. Prototype and Experiment
4.1. Design of the Control System
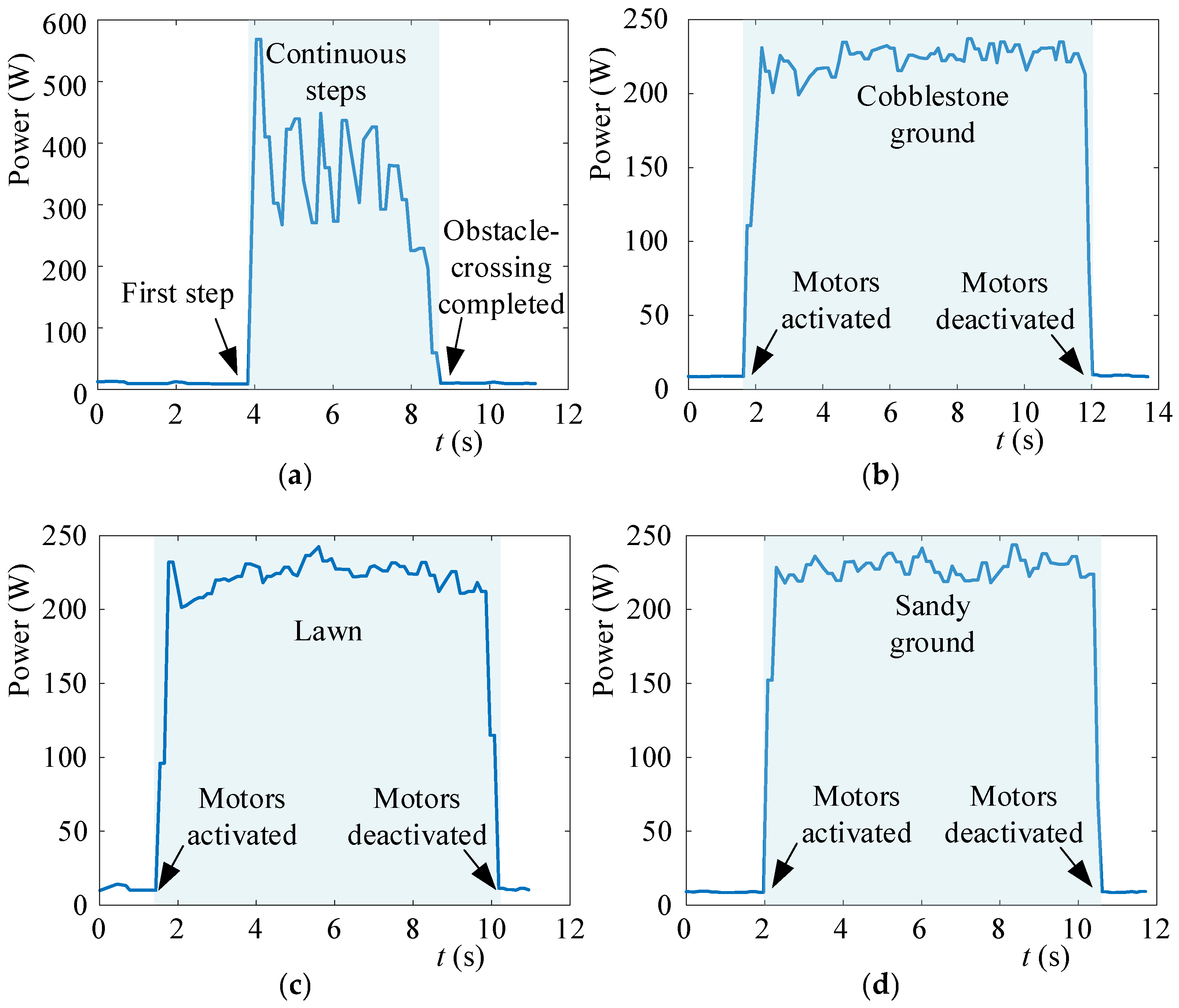
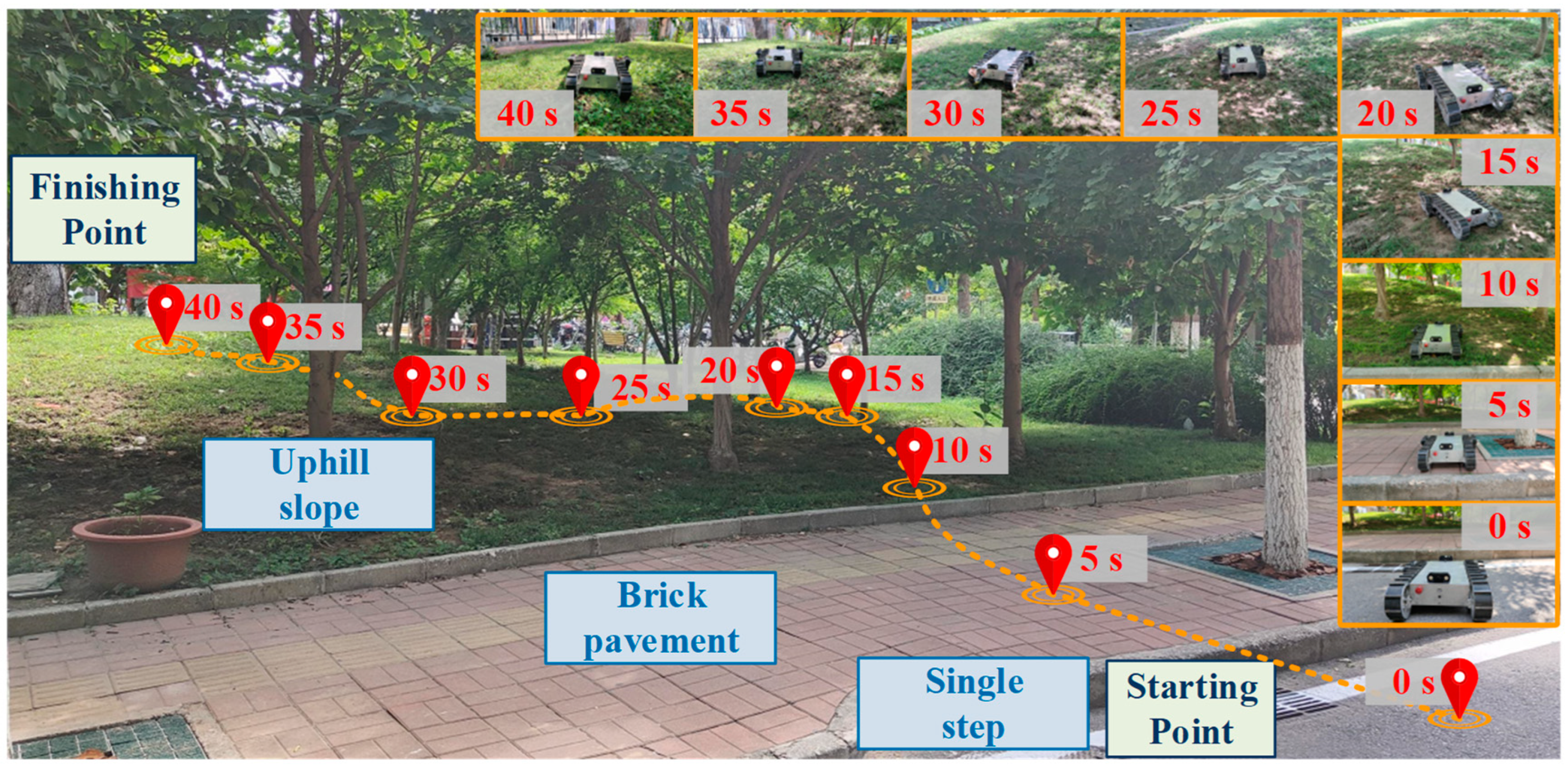
4.2. Experimental Tests
5. Discussion and Future Works
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Delmerico, J.; Mintchev, S.; Giusti, A.; Gromov, B.; Melo, K.; Horvat, T.; Cadena, C.; Hutter, M.; Ijspeert, A.; Floreano, D.; et al. The Current State and Future Outlook of Rescue Robotics. J. Field Robot. 2019, 36, 1171–1191. [Google Scholar] [CrossRef]
- Ugenti, A.; Vulpi, F.; Domínguez, R.; Cordes, F.; Milella, A.; Reina, G. On the Role of Feature and Signal Selection for Terrain Learning in Planetary Exploration Robots. J. Field Robot. 2022, 39, 355–370. [Google Scholar] [CrossRef]
- Milella, A.; Reina, G.; Nielsen, M. A Multi-Sensor Robotic Platform for Ground Mapping and Estimation beyond the Visible Spectrum. Precis. Agric 2019, 20, 423–444. [Google Scholar] [CrossRef]
- Nakajima, S. Concept of a Novel Four-Wheel-Type Mobile Robot for Rough Terrain, RT-Mover. In Proceedings of the 2009 IEEE/RSJ International Conference on Intelligent Robots and Systems, St. Louis, MO, USA, 10–15 October 2009; pp. 3257–3264. [Google Scholar]
- Jiang, H.; Xu, G.; Zeng, W.; Gao, F. Design and Kinematic Modeling of a Passively-Actively Transformable Mobile Robot. Mech. Mach. Theory 2019, 142, 103591. [Google Scholar] [CrossRef]
- Katz, B.; Carlo, J.D.; Kim, S. Mini Cheetah: A Platform for Pushing the Limits of Dynamic Quadruped Control. In Proceedings of the 2019 International Conference on Robotics and Automation (ICRA), Montreal, QC, Canada, 20–24 May 2019; pp. 6295–6301. [Google Scholar]
- Zi, P.; Xu, K.; Tian, Y.; Ding, X. A Mechanical Adhesive Gripper Inspired by Beetle Claw for a Rock Climbing Robot. Mech. Mach. Theory 2023, 181, 105168. [Google Scholar] [CrossRef]
- Tang, Z.; Dai, J.S. Bifurcated Configurations and Their Variations of an 8-Bar Linkage Derived from an 8-Kaleidocycle. Mech. Mach. Theory 2018, 121, 745–754. [Google Scholar] [CrossRef]
- Tang, Z.; Wang, K.; Spyrakos-Papastavridis, E. Origaker: A Novel Multi-Mimicry Quadruped Robot Based on A Metamorphic Mechanism. J. Mech. Robot. 2022, 14, 060907. [Google Scholar] [CrossRef]
- Yao, S.; Yao, Y.; Liu, R.; Liu, C. A Portable Off-Road Crawling Hexapod Robot. J. Field Robot. 2022, 39, 739–762. [Google Scholar] [CrossRef]
- Klemm, V.; Morra, A.; Salzmann, C.; Tschopp, F.; Bodie, K.; Gulich, L.; Küng, N.; Mannhart, D.; Pfister, C.; Vierneisel, M.; et al. Ascento: A Two-Wheeled Jumping Robot. In Proceedings of the 2019 International Conference on Robotics and Automation (ICRA), Montreal, QC, Canada, 20–24 May 2019; pp. 7515–7521. [Google Scholar]
- Bjelonic, M.; Sankar, P.K.; Bellicoso, C.D.; Vallery, H.; Hutter, M. Rolling in the Deep—Hybrid Locomotion for Wheeled-Legged Robots Using Online Trajectory Optimization. IEEE Robot. Autom. Lett. 2020, 5, 3626–3633. [Google Scholar] [CrossRef]
- Zhang, G.; Ma, S.; Liu, J.; Zeng, X.; Kong, L.; Li, Y. Q-Whex: A Simple and Highly Mobile Quasi-Wheeled Hexapod Robot. J. Field Robot. 2023, 40, 1444–1459. [Google Scholar] [CrossRef]
- Chen, H.-Y.; Wang, T.-H.; Ho, K.-C.; Ko, C.-Y.; Lin, P.-C.; Lin, P.-C. Development of a Novel Leg-Wheel Module with Fast Transformation and Leaping Capability. Mech. Mach. Theory 2021, 163, 104348. [Google Scholar] [CrossRef]
- Rubio, F.; Valero, F.; Llopis-Albert, C. A Review of Mobile Robots: Concepts, Methods, Theoretical Framework, and Applications. Int. J. Adv. Robot. Syst. 2019, 16, 2. [Google Scholar] [CrossRef]
- Bruzzone, L.; Nodehi, S.E.; Fanghella, P. Tracked Locomotion Systems for Ground Mobile Robots: A Review. Machines 2022, 10, 648. [Google Scholar] [CrossRef]
- Fukuoka, Y.; Oshino, K.; Ibrahim, A.N. Negotiating Uneven Terrain by a Simple Teleoperated Tracked Vehicle with Internally Movable Center of Gravity. Appl. Sci. 2022, 12, 525. [Google Scholar] [CrossRef]
- Zong, C.; Ji, Z.; Yu, J.; Yu, H. An Angle-Changeable Tracked Robot with Human-Robot Interaction in Unstructured Environments. Assem. Autom. 2020, 40, 565–575. [Google Scholar] [CrossRef]
- Yamauchi, B.M. PackBot: A Versatile Platform for Military Robotics. In Proceedings of the Unmanned Ground Vehicle Technology VI, Orlando, FL, USA, 2 September 2004; SPIE: Bellingham, WA, USA; Volume 5422, pp. 228–237. [Google Scholar]
- Han, X.; Lin, M.; Wu, X.; Yang, J. Design of An Articulated-Tracked Mobile Robot with Two Swing Arms. In Proceedings of the 2019 IEEE 4th International Conference on Advanced Robotics and Mechatronics (ICARM), Toyonaka, Japan, 3–5 July 2019; pp. 684–689. [Google Scholar]
- Wang, W.; Wu, D.; Wang, Q.; Deng, Z.; Du, Z. Stability Analysis of a Tracked Mobile Robot in Climbing Stairs Process. In Proceedings of the 2012 IEEE International Conference on Mechatronics and Automation, Chengdu, China, 5–8 August 2012; pp. 1669–1674. [Google Scholar]
- Guo, W.; Qiu, J.; Xu, X.; Wu, J. TALBOT: A Track-Leg Transformable Robot. Sensors 2022, 22, 1470. [Google Scholar] [CrossRef] [PubMed]
- Bruzzone, L.; Baggetta, M.; Nodehi, S.E.; Bilancia, P.; Fanghella, P. Functional Design of a Hybrid Leg-Wheel-Track Ground Mobile Robot. Machines 2021, 9, 10. [Google Scholar] [CrossRef]
- Nodehi, S.E.; Bruzzone, L.; Fanghella, P. SnakeTrack, A Bio-Inspired, Single Track Mobile Robot with Compliant Vertebral Column for Surveillance and Inspection. In Proceedings of the Advances in Service and Industrial Robotics, Klagenfurt, Austria, 8–10 June 2022; Müller, A., Brandstötter, M., Eds.; Springer International Publishing: Cham, Switzerland, 2022; pp. 513–520. [Google Scholar]
- Kislassi, T.; Zarrouk, D. A Minimally Actuated Reconfigurable Continuous Track Robot. IEEE Robot. Autom. Lett. 2020, 5, 652–659. [Google Scholar] [CrossRef]
- Liu, J.; Wang, Y.; Ma, S.; Li, B. Analysis of Stairs-Climbing Ability for a Tracked Reconfigurable Modular Robot. In Proceedings of the IEEE International Safety, Security and Rescue Rototics, Workshop, Kobe, Japan, 6–9 June 2005; pp. 36–41. [Google Scholar]
- Gong, Z.; Xie, F.; Liu, X.-J.; Shentu, S. Obstacle-Crossing Strategy and Formation Parameters Optimization of a Multi-Tracked-Mobile-Robot System with a Parallel Manipulator. Mech. Mach. Theory 2020, 152, 103919. [Google Scholar] [CrossRef]
- Lan, G.; Ma, S.; Inoue, K.; Hamamatsu, Y. Development of a Novel Crawler Mechanism with Polymorphic Locomotion. Adv. Robot. 2007, 21, 421–440. [Google Scholar] [CrossRef]
- Seo, T.; Sitti, M. Tank-Like Module-Based Climbing Robot Using Passive Compliant Joints. IEEE/ASME Trans. Mechatron. 2013, 18, 397–408. [Google Scholar] [CrossRef]
- Kim, J.; Kim, J.; Lee, D. Mobile Robot with Passively Articulated Driving Tracks for High Terrainability and Maneuverability on Unstructured Rough Terrain: Design, Analysis, and Performance Evaluation. J. Mech. Sci. Technol. 2018, 32, 5389–5400. [Google Scholar] [CrossRef]
- Kim, J.; Jeong, H.; Lee, D. Performance Optimization of a Passively Articulated Mobile Robot by Minimizing Maximum Required Friction Coefficient on Rough Terrain Driving. Mech. Mach. Theory 2021, 164, 104368. [Google Scholar] [CrossRef]
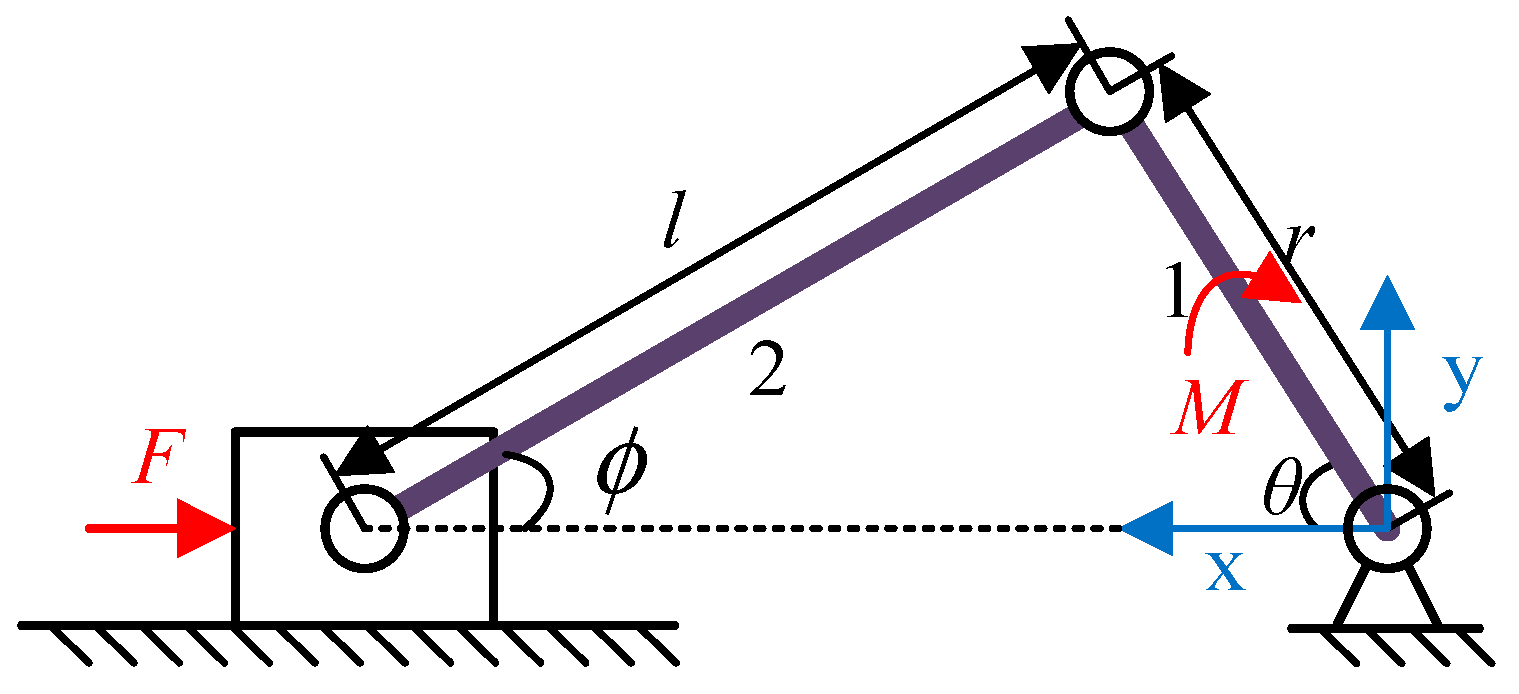




| Parameter | Value |
|---|---|
| (kg) | 3 |
| (kg) | 20 |
| (kg) | 7 |
| l (m) | 0.6 |
| r (m) | 0.2 |
| g (m/s2) | 9.8 |
| Length (mm) | Width (mm) | Height (mm) | Weight (kg) | Number of Motors | Top Speed(m/s) | Surmountable Obstacle Height (mm) |
|---|---|---|---|---|---|---|
| 780 | 600 | 200 | 20 | 2 | 2 | 250 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, R.; Zhang, X.; Hu, S.; Wu, J.; Feng, Y.; Yao, Y.-a. Design and Analysis of an Adaptive Obstacle-Overcoming Tracked Robot with Passive Swing Arms. Machines 2023, 11, 1051. https://doi.org/10.3390/machines11121051
Li R, Zhang X, Hu S, Wu J, Feng Y, Yao Y-a. Design and Analysis of an Adaptive Obstacle-Overcoming Tracked Robot with Passive Swing Arms. Machines. 2023; 11(12):1051. https://doi.org/10.3390/machines11121051
Chicago/Turabian StyleLi, Ruiming, Xianhong Zhang, Shaoheng Hu, Jianxu Wu, Yu Feng, and Yan-an Yao. 2023. "Design and Analysis of an Adaptive Obstacle-Overcoming Tracked Robot with Passive Swing Arms" Machines 11, no. 12: 1051. https://doi.org/10.3390/machines11121051
APA StyleLi, R., Zhang, X., Hu, S., Wu, J., Feng, Y., & Yao, Y.-a. (2023). Design and Analysis of an Adaptive Obstacle-Overcoming Tracked Robot with Passive Swing Arms. Machines, 11(12), 1051. https://doi.org/10.3390/machines11121051




