On the Dynamics of an Enhanced Coaxial Inertial Exciter for Vibratory Machines
Abstract
1. Introduction
2. Design of Vibrator and Methods of Research
2.1. Dynamical Model of the Inertial Vibration Exciter with a Single Asynchronous Electric Motor
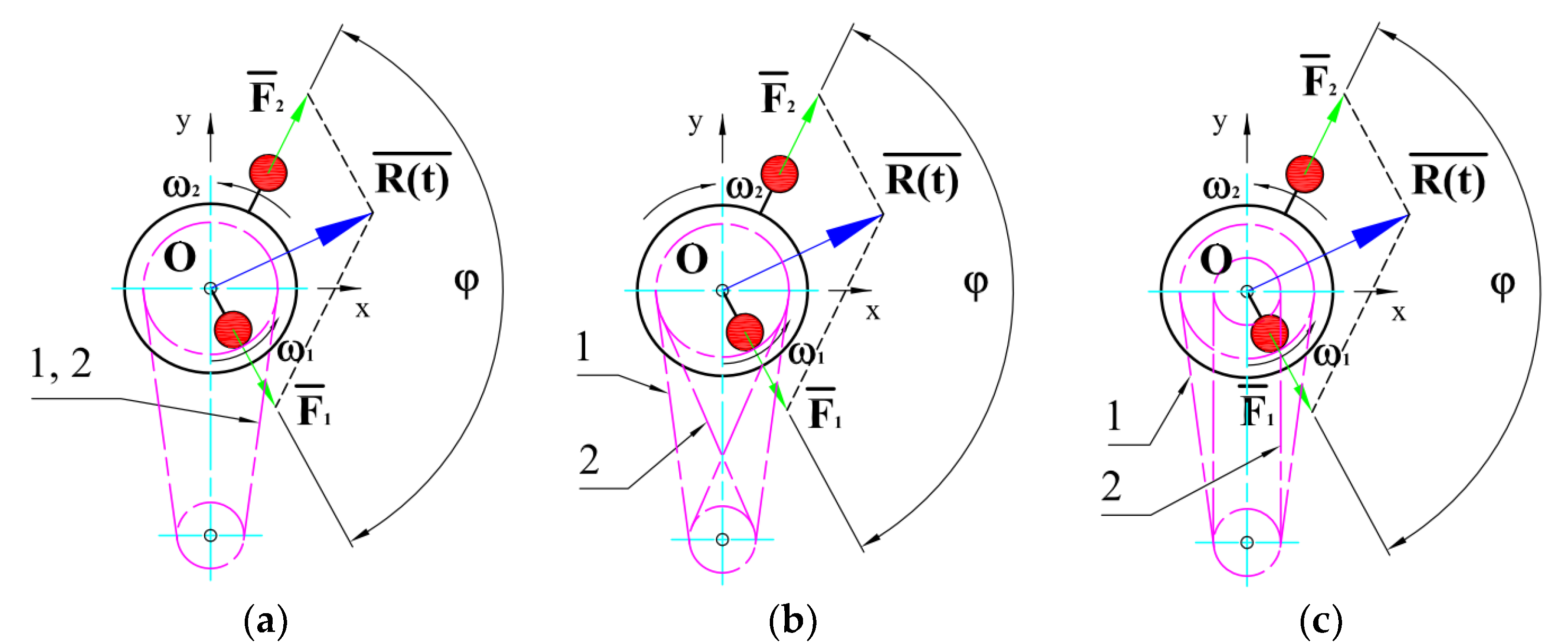
2.2. Dynamical Model of the Vibratory System with Kinematically Synchronized Unbalanced Rotors
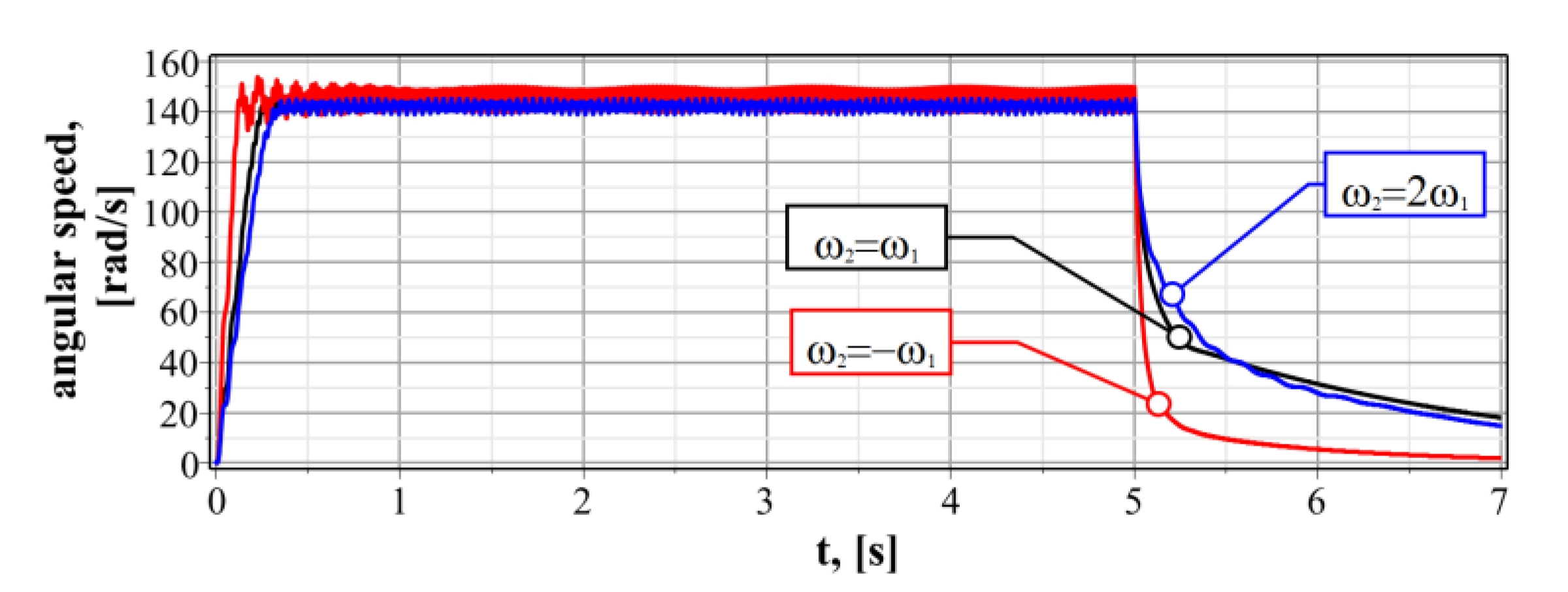
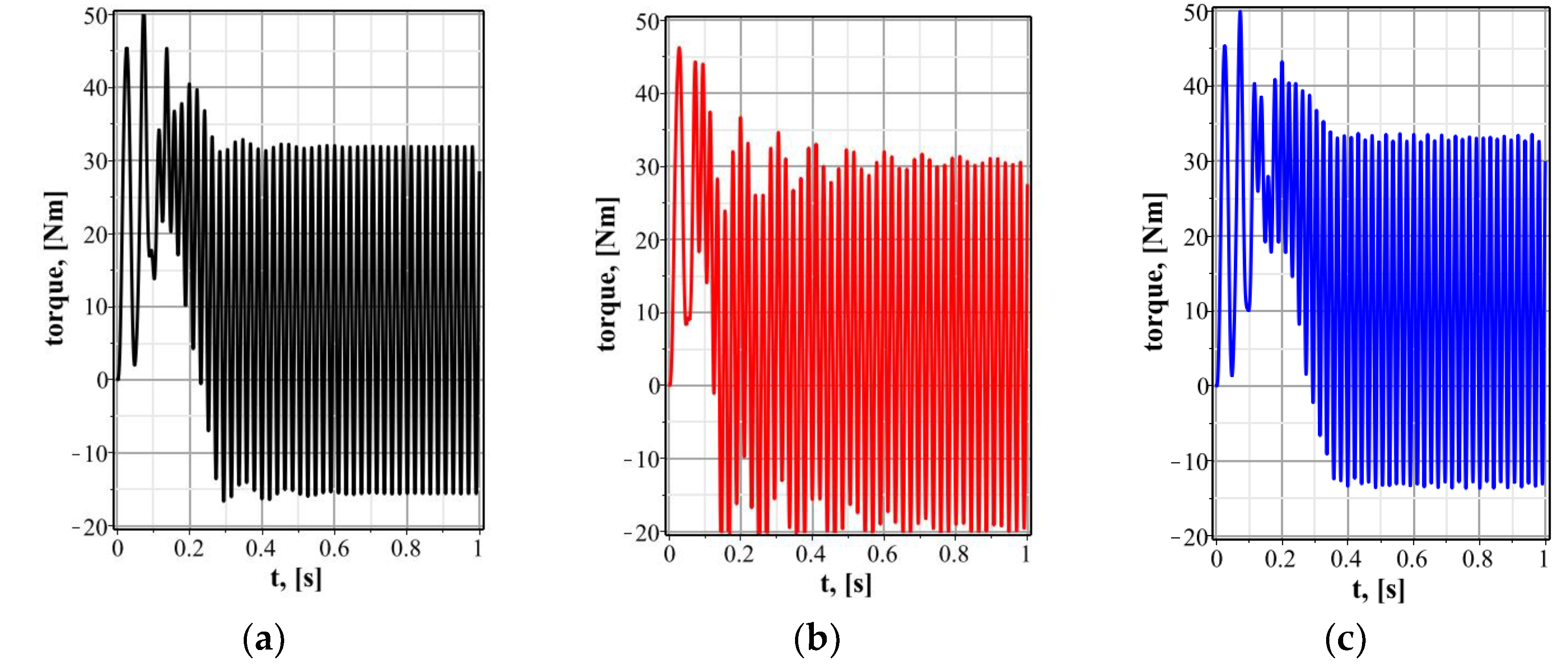
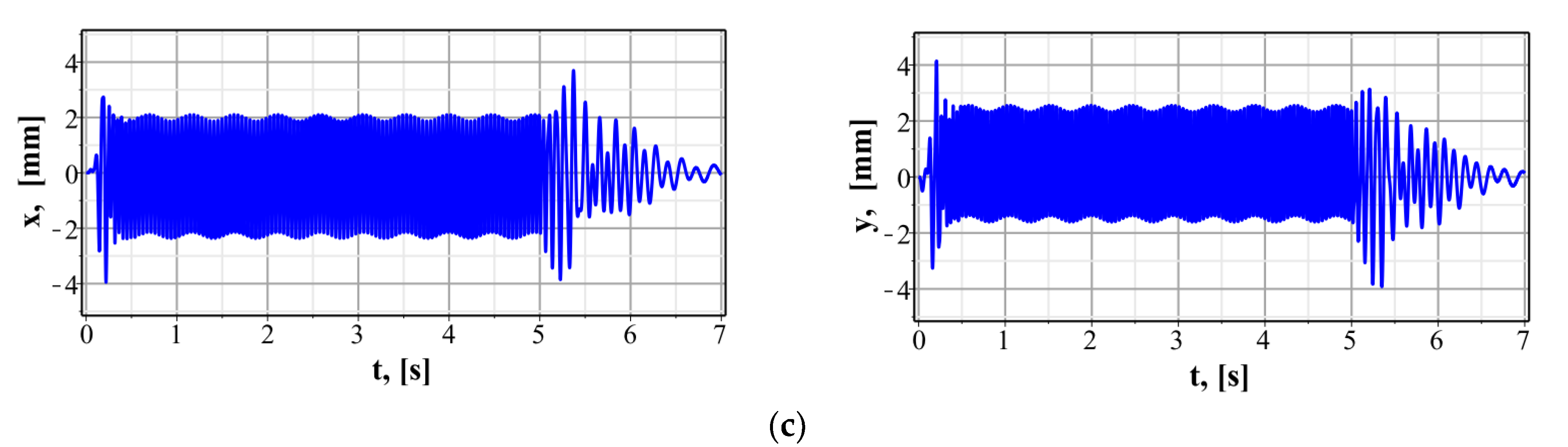
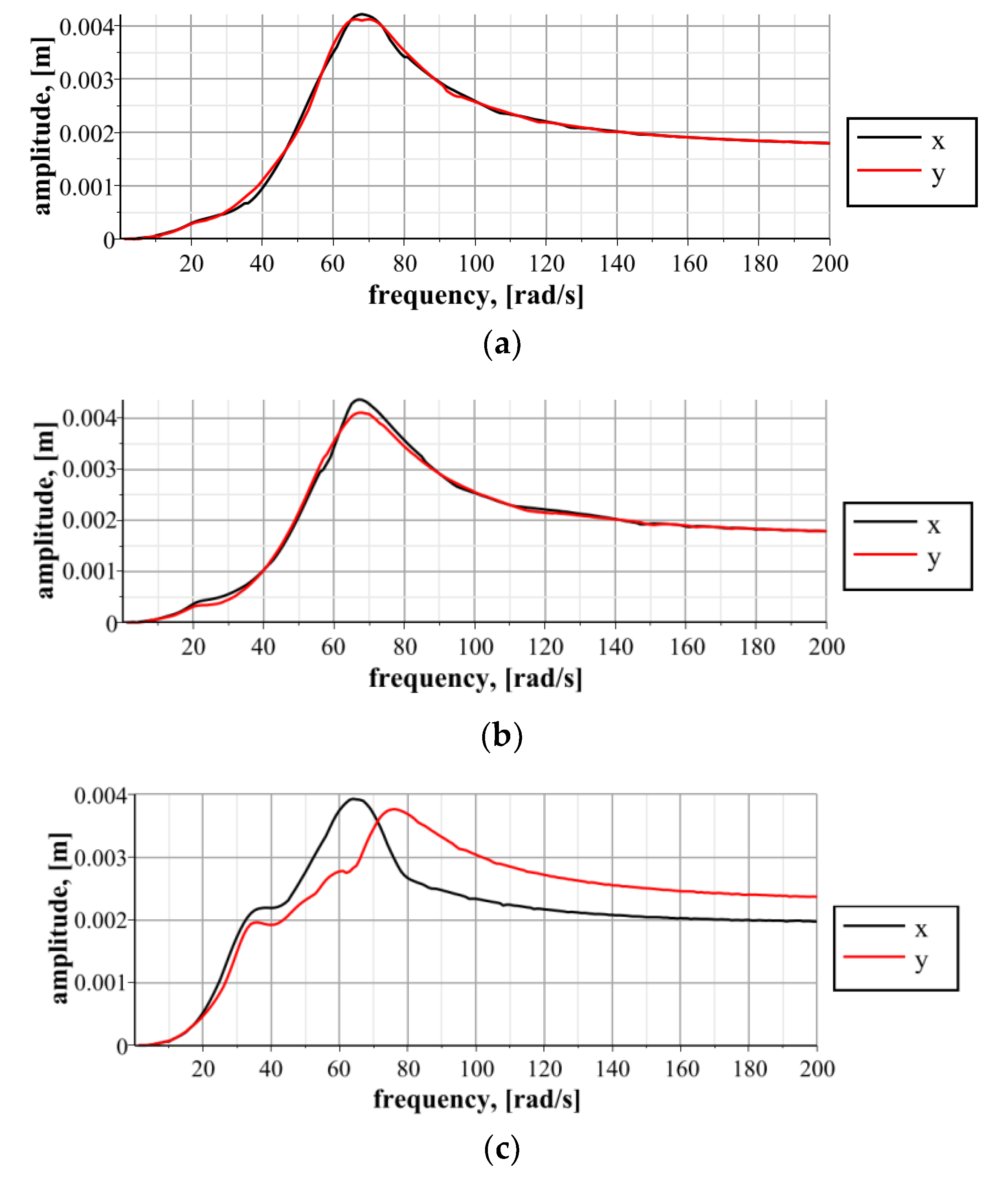
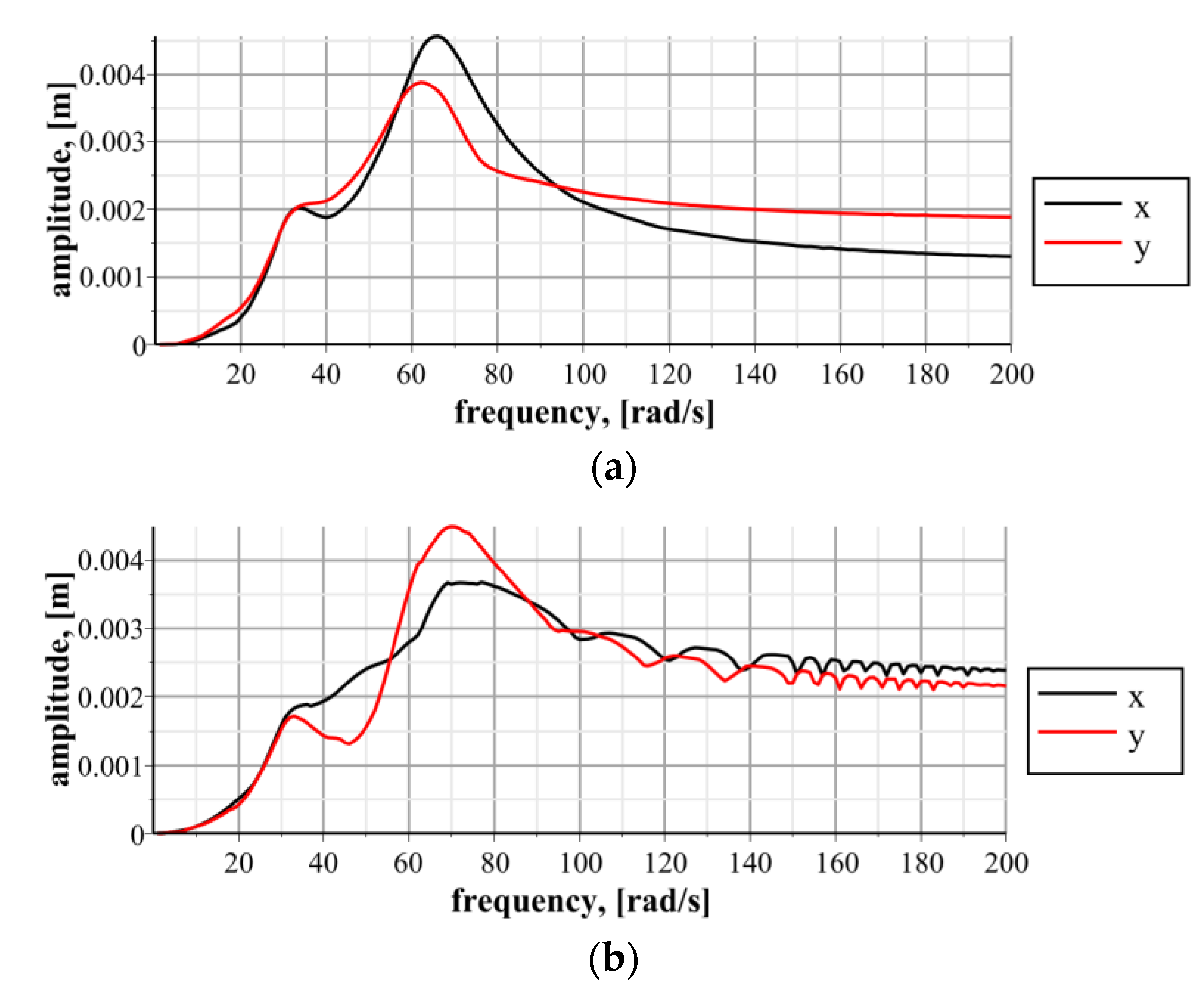
3. Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
References
- Shah, K.P. Construction, Working and Maintenance of Electric Vibrators and Vibrating Screens. Available online: https://practicalmaintenance.net/wp-content/uploads/Construction-Working-and-Maintenance-of-Vibrators-and-Vibrating-Screens.pdf (accessed on 8 December 2022).
- Nguyen, V.X.; Nguyen, K.L.; Dinh, G.N. Study of the Dynamics and Analysis of the Effect of the Position of the Vibration Motor to the Oscillation of Vibrating Screen. J. Phys. Conf. Ser. 2019, 1384, 012035. [Google Scholar] [CrossRef]
- Despotovic, D.Z.; Pavlovic, A.M.; Radakovic, J. Using Regulated Drive of Vibratory Screens with Unbalanced Motors. J. Mechatron. Autom. Identif. Technol. 2017, 1, 20–25. [Google Scholar]
- Despotović, Ž.V.; Pavlović, A.M.; Ivanić, D. Exciting Force Frequency Control of Unbalanced Vibratory Actuators. In Proceedings of the 2019 20th International Symposium on Power Electronics (Ee), Novi Sad, Serbia, 23–26 October 2019; pp. 1–6. [Google Scholar]
- Zhao, G.; Wang, X.; Lin, D.; Xu, N.; Yu, C.; Geng, R. Study of Double-Deck Vibrating Flip-Flow Screen Based on Dynamic Stiffness Characteristics of Shear Springs. Minerals 2021, 11, 928. [Google Scholar] [CrossRef]
- Ogonowski, S.; Krauze, P. Trajectory Control for Vibrating Screen with Magnetorheological Dampers. Sensors 2022, 22, 4225. [Google Scholar] [CrossRef] [PubMed]
- Jia, L.; Zhang, J.; Zhou, L.; Wen, B. Multifrequency-Controlled Synchronization of Three Eccentric Rotors Driven by Induction Motors in the Same Direction. J. Low Freq. Noise Vib. Act. Control 2019, 38, 615–632. [Google Scholar] [CrossRef]
- Zhang, X.; Gu, D.; Yue, H.; Li, M.; Wen, B. Synchronization and Stability of a Far-Resonant Vibrating System with Three Rollers Driven by Two Vibrators. Appl. Math. Model. 2021, 91, 261–279. [Google Scholar] [CrossRef]
- Zhang, X.; Hu, W.; Gao, Z.; Liu, Y.; Wen, B.-C. Composite Synchronization on Two Pairs of Vibrators in a Far Super-Resonant Vibrating System with the Single Rigid Frame. J. Low Freq. Noise Vib. Act. Control 2021, 40, 2064–2076. [Google Scholar] [CrossRef]
- Gursky, V.M.; Kuzio, I.V.; Lanets, O.S.; Kisała, P.; Tolegenova, A.; Syzdykpayeva, A. Implementation of Dual-Frequency Resonant Vibratory Machines with Pulsed Electromagnetic Drive. Prz. Elektrotechniczny 2019, 95, 41–46. [Google Scholar] [CrossRef]
- Filimonikhin, G.; Yatsun, V. Conditions of Replacing a Single-Frequency Vibro-Exciter with a Dual-Frequency One in the Form of Passive Auto-Balancer. Nauk. Visnyk Natsionalnoho Hirnychoho Universytetu 2017, 1, 61–68. [Google Scholar]
- Modrzewski, R.; Obraniak, A.; Rylski, A.; Siczek, K. A Study on the Dynamic Behavior of a Sieve in an Industrial Sifter. Appl. Sci. 2022, 12, 8590. [Google Scholar] [CrossRef]
- Chen, X.; Liu, J.; Li, L. Dynamics of the Vibration System Driven by Three Homodromy Eccentric Rotors Using Control Synchronization. Appl. Sci. 2021, 11, 7691. [Google Scholar] [CrossRef]
- Cieplok, G.; Wójcik, K. Conditions for Self-Synchronization of Inertial Vibrators of Vibratory Conveyors in General Motion. J. Theor. Appl. Mech. 2020, 58, 513–524. [Google Scholar] [CrossRef]
- Zhang, X.; Zhang, W.; Chen, W.; Zhang, X.; Wang, Z.; Wen, B. Theoretical, Numerical and Experimental Studies on Times–Frequency Synchronization of the Three Exciters Based on the Asymptotic Method. J. Vib. Eng. Technol. 2022, 10, 1091–1109. [Google Scholar] [CrossRef]
- Zhang, X.; Zhang, W.; Chen, W.; Hu, W.; Zhang, X.; Wen, B.-C. Synchronization Behaviors of a Vibrating Mechanical System with Adjustable Frequencies and Motion Trajectories. J. Low Freq. Noise Vib. Act. Control 2022, 41, 945–969. [Google Scholar] [CrossRef]
- Fang, P.; Wang, Y.; Hou, Y.; Wu, Y. Synchronous Control of Multi-Motor Coupled with Pendulum in a Vibration System. IEEE Access 2020, 8, 51964–51975. [Google Scholar] [CrossRef]
- Krot, P.V. Dynamical Processes in a Multi-Motor Gear Drive of Heavy Slabbing Mill. J. Vibroengineering 2019, 21, 2064–2081. [Google Scholar] [CrossRef]
- Sedaghati, R.; Suleman, A.; Tabarrok, B. Structural Optimization with Frequency Constraints Using the Finite Element Force Method. AIAA J. 2012, 40, 382–388. [Google Scholar] [CrossRef]
- Zou, M.; Fang, P.; Hou, Y.; Wang, Y.; Hou, D.; Peng, H. Synchronization Analysis of Two Eccentric Rotors with Double-Frequency Excitation Considering Sliding Mode Control. Commun. Nonlinear Sci. Numer. Simul. 2021, 92, 105458. [Google Scholar] [CrossRef]
- Jia, L.; Kong, X.; Zhang, J.; Liu, Y.; Wen, B. Multiple-Frequency Controlled Synchronization of Two Homodromy Eccentric Rotors in a Vibratory System. Shock. Vib. 2018, 2018, 4941357. [Google Scholar] [CrossRef]
- Fang, P.; Shi, S.; Zou, M.; Lu, X.; Wang, D. Self-Synchronization and Control-Synchronization of Dual-Rotor Space Vibration System. Int. J. Non-Linear Mech. 2022, 139, 103869. [Google Scholar] [CrossRef]
- Xiong, G.; Hou, Y.; Fang, P.; Du, M. Stability and Synchronous Characteristics of Dual-Rotors Vibrating System Considering the Material Effects. J. Vib. Eng. Technol. 2022. [Google Scholar] [CrossRef]
- Korendiy, V.; Kuzio, I.; Nikipchuk, S.; Kotsiumbas, O.; Dmyterko, P. On the Dynamic Behavior of an Asymmetric Self-Regulated Planetary-Type Vibration Exciter. Vibroengineering PROCEDIA 2022, 42, 7–13. [Google Scholar] [CrossRef]
- Korendiy, V.; Gurey, V.; Borovets, V.; Kotsiumbas, O.; Lozynskyy, V. Generating Various Motion Paths of Single-Mass Vibratory System Equipped with Symmetric Planetary-Type Vibration Exciter. Vibroengineering PROCEDIA 2022, 43, 7–13. [Google Scholar] [CrossRef]
- Nazarenko, I.; Gaidaichuk, V.; Dedov, O.; Diachenko, O. Investigation of Vibration Machine Movement with a Multimode Oscillation Spectrum. East.-Eur. J. Enterp. Technol. 2017, 6, 28–36. [Google Scholar] [CrossRef]
- Yu, C.; Wang, X.; Pang, K.; Zhao, G.; Sun, W. Dynamic Characteristics of a Vibrating Flip-Flow Screen and Analysis for Screening 3 Mm Iron Ore. Shock. Vib. 2020, 2020, 1031659. [Google Scholar] [CrossRef]
- Moncada, M.M.; Rodríguez, C.G. Dynamic Modeling of a Vibrating Screen Considering the Ore Inertia and Force of the Ore over the Screen Calculated with Discrete Element Method. Shock. Vib. 2018, 2018, 1714738. [Google Scholar] [CrossRef]
- Chen, Z.; Tong, X.; Li, Z. Numerical Investigation on the Sieving Performance of Elliptical Vibrating Screen. Processes 2020, 8, 1151. [Google Scholar] [CrossRef]
- Gursky, V.; Krot, P.; Korendiy, V.; Zimroz, R. Dynamic Analysis of an Enhanced Multi-Frequency Inertial Exciter for Industrial Vibrating Machines. Machines 2022, 10, 130. [Google Scholar] [CrossRef]
- Gursky, V.; Kuzio, I.; Krot, P.; Zimroz, R. Energy-Saving Inertial Drive for Dual-Frequency Excitation of Vibrating Machines. Energies 2021, 14, 71. [Google Scholar] [CrossRef]
- Czubak, P. Vibratory Conveyor of the Controlled Transport Velocity with the Possibility of the Reversal Operations. J. Vibroengineering 2016, 18, 3539–3547. [Google Scholar] [CrossRef]
- Obertyukh, R.; Slabkyi, A.; Petrov, O.; Bakalets, D.; Sukhorukov, S. Substantiation of the Design Calculation Method for the Vibroturning Device. In Advances in Design, Simulation, Manufacturing; Ivanov, V., Trojanowska, J., Pavlenko, I., Rauch, E., Peraković, D., Eds.; Springer International Publishing: Cham, Switzerland, 2022; pp. 185–195. [Google Scholar]
- Yaroshevich, N.; Puts, V.; Yaroshevich, Т.; Herasymchuk, O. Slow Oscillations in Systems with Inertial Vibration Exciters. Vibroengineering PROCEDIA 2020, 32, 20–25. [Google Scholar] [CrossRef]
- Yaroshevich, N.; Yaroshevych, O.; Lyshuk, V. Drive Dynamics of Vibratory Machines with Inertia Excitation. In Vibration Engineering and Technology of Machinery; Balthazar, J.M., Ed.; Springer International Publishing: Cham, Switzerland, 2021; pp. 37–47. [Google Scholar]
- Yatsun, V.; Filimonikhin, G.; Podoprygora, N.; Pirogov, V. Studying the Excitation of Resonance Oscillations in a Rotor on Isotropic Supports by a Pendulum, a Ball, a Roller. East.-Eur. J. Enterp. Technol. 2019, 6, 32–43. [Google Scholar] [CrossRef]
- Filimonikhin, G.; Yatsun, V.; Kyrychenko, A.; Hrechka, A.; Shcherbyna, K. Synthesizing a Resonance Anti-Phase Two-Mass Vibratory Machine Whose Operation Is Based on the Sommerfeld Effect. East.-Eur. J. Enterp. Technol. 2020, 6, 42–50. [Google Scholar] [CrossRef]
- Liu, Y.; Zhang, X.; Gu, D.; Jia, L.; Wen, B. Synchronization of a Dual-Mass Vibrating System with Two Exciters. Shock. Vib. 2020, 2020, e9345652. [Google Scholar] [CrossRef]
- Jannati, M.; Sutikno, T. Modelling of a 3-Phase Induction Motor under Open-Phase Fault Using Matlab/Simulink. Int. J. Power Electron. Drive Syst. IJPEDS 2016, 7, 1146–1152. [Google Scholar] [CrossRef]
- Chaban, A.; Łukasik, Z.; Popenda, A.; Szafraniec, A. Mathematical Modelling of Transient Processes in an Asynchronous Drive with a Long Shaft Including Cardan Joints. Energies 2021, 14, 5692. [Google Scholar] [CrossRef]
- Sudheer, H.; Kodad, S.F.; Sarvesh, B. Improved Sensorless Direct Torque Control of Induction Motor Using Fuzzy Logic and Neural Network Based Duty Ratio Controller. IAES Int. J. Artif. Intell. IJ-AI 2017, 6, 79–90. [Google Scholar] [CrossRef]
- Yaroshevich, M.P.; Zabrodets, I.P.; Yaroshevich, T.S. Dynamics of Vibrating Machines Starting with Unbalanced Drive in Case of Bearing Body Flat Vibrations. Nauk. Visnyk Natsionalnoho Hirnychoho Universytetu 2015, 3, 39–45. [Google Scholar]
- Wu, M.; Chen, F.; Li, A.; Chen, Z.; Sun, N. A Novel Vibration Isolator for Vibrating Screen Based on Magnetorheological Damper. J. Mech. Sci. Technol. 2021, 35, 4343–4352. [Google Scholar] [CrossRef]
- Krot, P.; Zimroz, R.; Michalak, A.; Wodecki, J.; Ogonowski, S.; Drozda, M.; Jach, M. Development and Verification of the Diagnostic Model of the Sieving Screen. Shock. Vib. 2020, 2020, e8015465. [Google Scholar] [CrossRef]
- Chen, B.; Yan, J.; Yin, Z.; Tamma, K.K. A New Study on Dynamic Adjustment of Vibration Direction Angle for Dual-Motor-Driven Vibrating Screen. Proc. Inst. Mech. Eng. Part E J. Process Mech. Eng. 2021, 235, 186–196. [Google Scholar] [CrossRef]






| Parameters | Symbol | Values |
|---|---|---|
| Electric power | P | 1.1 kW |
| Nominal voltage | U0 | 230 V |
| Nominal speed | n | 1420 rpm |
| Number of poles | p | 2 |
| Stator resistance | Rs | 7.6 Ω |
| Rotor resistance | Rr | 3.6 Ω |
| Stator inductance | Ls | 0.6015 H |
| Rotor inductance | Lr | 0.6015 H |
| Mutual inductance | Lm | 0.58 H |
| Moment of inertia | J | 0.005 kg⋅m2 |
| Parameters | Symbol | Values |
|---|---|---|
| Total mass of exciter | m | 100 kg |
| Unbalanced mass 1 | m1 | 5 kg |
| Unbalanced mass 2 | m2 | 5 kg |
| Springs’ stiffness in horizontal and vertical directions | kx, ky | 3.944 × 105 N/m |
| Coefficient of viscous damping in horizontal and vertical directions | cx, cy | 2512 N⋅s/m |
| Coefficient of viscous friction in bearings | γ | 0.01 N m s/rad |
| Static moment of the internal unbalanced rotor | m1r1 | 0.15 kg⋅m |
| Static moment of the external unbalanced rotor | m2r2 | 0.06 kg⋅m |
| Total inertial moment of rotating masses | I | 0.015 kg⋅m2 |
| Bearing inner diameters | d01 d02 | 0.050 m 0.120 m |
| Friction coefficient in bearings | f | 0.004 |
| Parameters | Angular Velocities Ratio ω2/ω1 | ||
|---|---|---|---|
| 1 | −1 | 2 | |
| Static moments of the unbalanced rotors 1/2, kg⋅m | 0.15/0.06 | ||
| Angular speed of the unbalanced rotors 1/2, rad/s | 148.7/148.7 | 148.7/−148.7 | 148.7/297.4 |
| Disturbing force, kN | 3.6 | 2–4.5 | 2–8.2 |
| Total mass, kg | 100 | ||
| Displacement amplitude, mm | 2 | ||
| Acceleration, m/s2 | 40.7 | 53.2 | 89.4 |
| Power, kW | 1.1 | ||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gurskyi, V.; Korendiy, V.; Krot, P.; Zimroz, R.; Kachur, O.; Maherus, N. On the Dynamics of an Enhanced Coaxial Inertial Exciter for Vibratory Machines. Machines 2023, 11, 97. https://doi.org/10.3390/machines11010097
Gurskyi V, Korendiy V, Krot P, Zimroz R, Kachur O, Maherus N. On the Dynamics of an Enhanced Coaxial Inertial Exciter for Vibratory Machines. Machines. 2023; 11(1):97. https://doi.org/10.3390/machines11010097
Chicago/Turabian StyleGurskyi, Volodymyr, Vitaliy Korendiy, Pavlo Krot, Radosław Zimroz, Oleksandr Kachur, and Nadiia Maherus. 2023. "On the Dynamics of an Enhanced Coaxial Inertial Exciter for Vibratory Machines" Machines 11, no. 1: 97. https://doi.org/10.3390/machines11010097
APA StyleGurskyi, V., Korendiy, V., Krot, P., Zimroz, R., Kachur, O., & Maherus, N. (2023). On the Dynamics of an Enhanced Coaxial Inertial Exciter for Vibratory Machines. Machines, 11(1), 97. https://doi.org/10.3390/machines11010097











