Estimations of Compressor Stall and Surge Using Passage Stall Behaviors
Abstract
1. Introduction
2. Stall Inception Theory
3. Developed Model
3.1. Single-Passage Computations
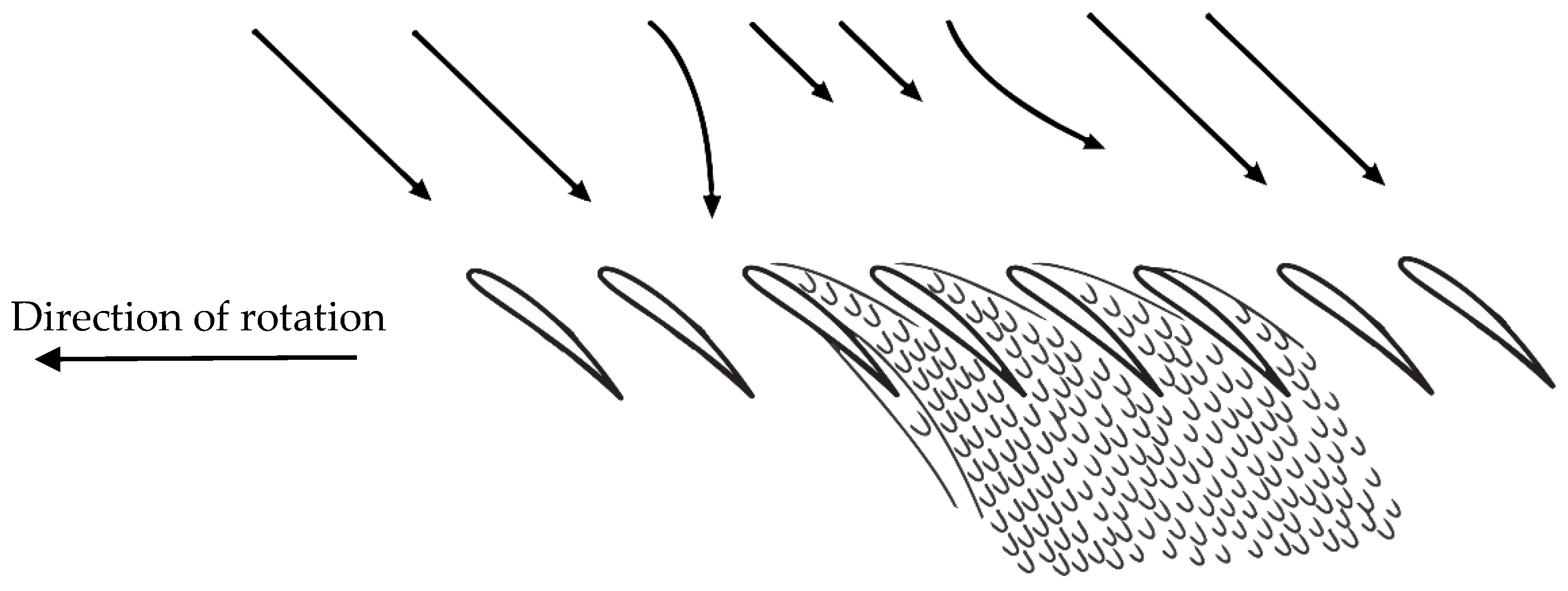
3.2. Reconstruction of Full-Annulus Compressor Conceptually
3.3. Generation of Pressure Signals
3.4. Calculations of Total Energy and Spectral Entropy
3.5. ANOVA Analysis
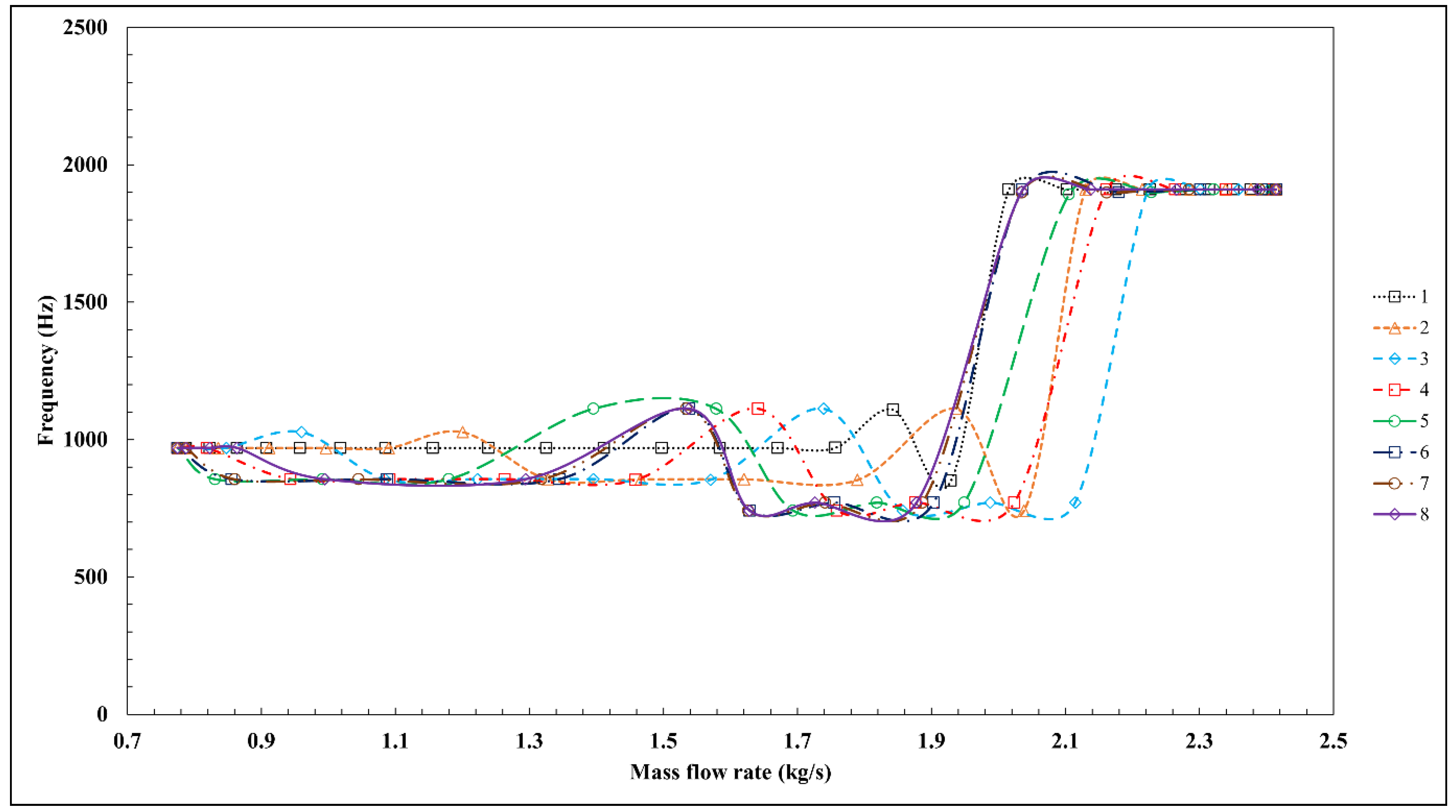
3.6. Stall Cell Frequency
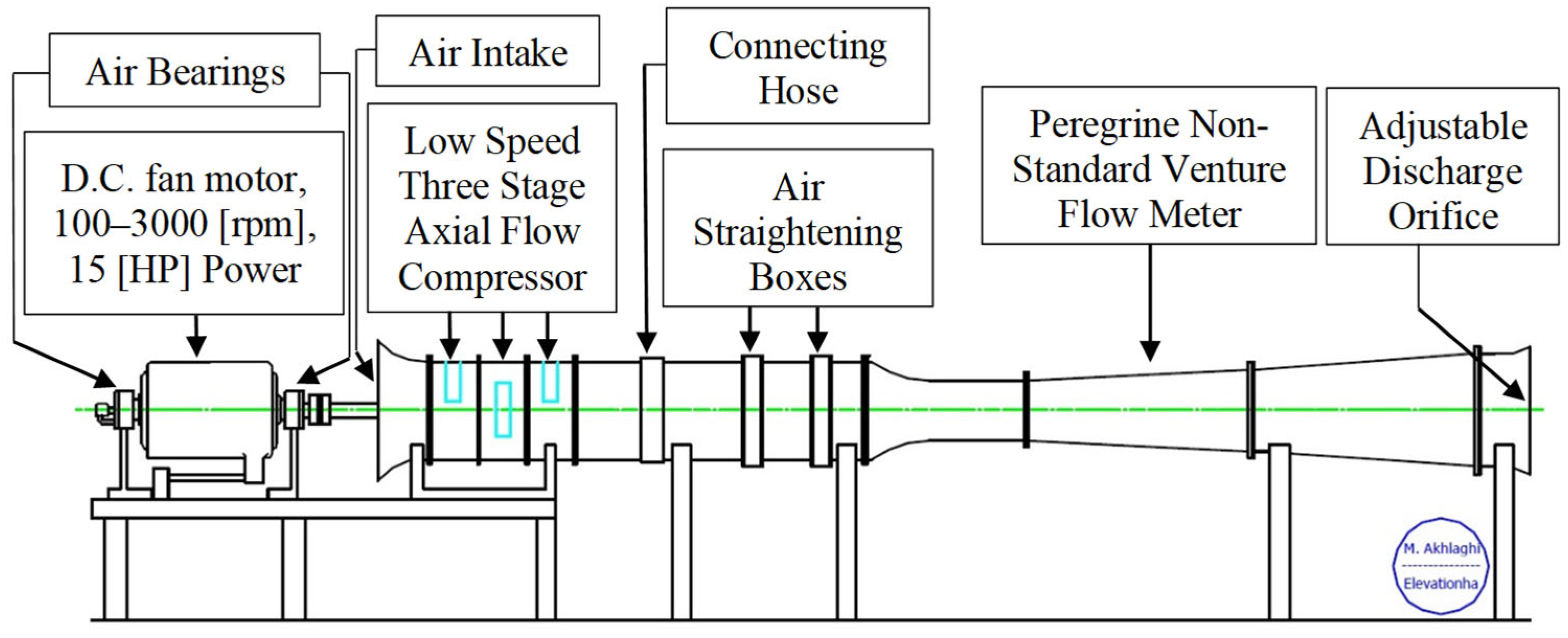
4. Experimental Compressor
5. Numerical Details
5.1. Governing Equations
5.2. Turbulence Model
5.3. Meshing Details and Boundary Conditions
5.4. Grid Independence Study
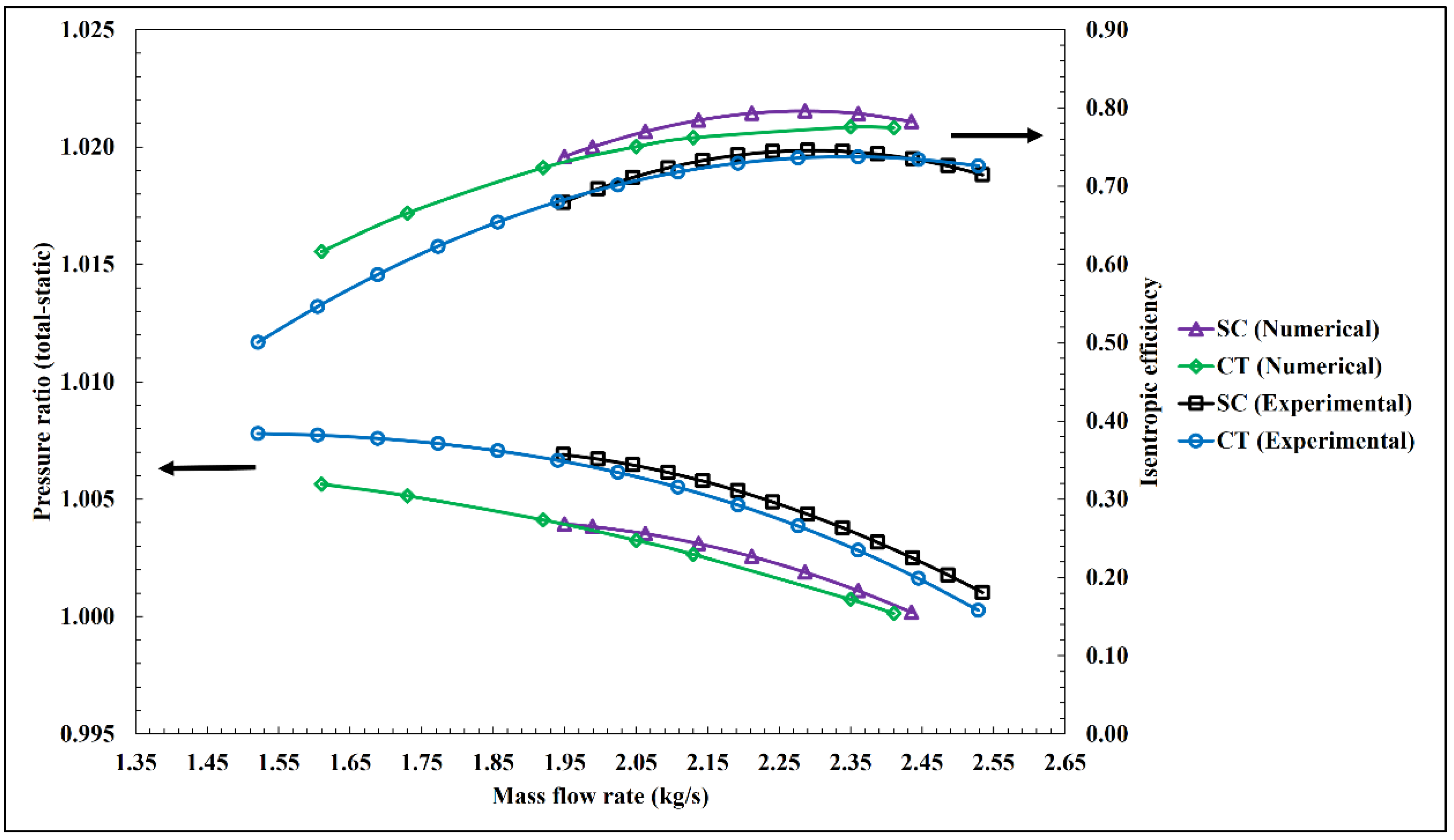
6. Validation of the Developed Model and the Predictions of Stall and Surge
7. Conclusions
- The reconstruction of the full-annulus compressor based on the presence of disturbance including no disturbance, formation, and growth of disturbance and a systematic approach can be implemented successfully to reproduce pre-stall, in-stall, and surge flow regimes.
- The reconstructed compressor coincides with the stalling patterns of actual compressors, including pre-stall, in-stall, surge, and breakdown conditions.
- Spectral entropy and total energy due to secondary flow oscillations are effective indicators of rotating stall and surge instabilities.
- Statistical ANOVA analysis and its sum of squares of total not only validate the results in terms of statistics but also can unite various results of total energy and spectral entropy.
- The united results can show the correlation between total energy and spectral entropy and compressor operating conditions easily.
- The predicted stall inception and surge operating points coincide well with the experimental data with minimal error.
- The developed stability model can detect the multiblade rotating stall and surge instabilities and can be utilized in the preliminary design stage of a compressor.
- The formation and disappearance of new frequencies provide a rough estimation of rotating stall.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| ANOVA | Analysis of variance |
| BPF | Blade passing frequency |
| CT | Casing treatment |
| E | Total energy |
| f | Probability |
| FFT | Fast Fourier transform |
| Pressure signal | |
| Probability distribution | |
| SC | Solid casing |
| SE | Spectral entropy |
| SS | Sum of square |
| Power distribution | |
| Grand mean |
References
- Day, I.J. Stall, surge and 75 years of research. J. Turbomach. 2016, 138, 1–16. [Google Scholar] [CrossRef]
- Cumpsty, N.A. Compressor Aerodynamics, 1st ed.; Longman Scientific & Technical: Essex, UK, 1989; pp. 359–362. [Google Scholar]
- Dixon, S.L.; Hall, C.A. Fluid Mechanics and Thermodynamics of Turbomachinery, 7th ed.; Elsevier: Oxford, UK, 2014; pp. 198–200. [Google Scholar]
- Stenning, A.; Kriebel, A. Stall Propagation in a Cascade of Aerofoils. Trans. ASME 1958, 80, 777–789. [Google Scholar]
- Stenning, A. Rotating Stall and Surge. J. Fluids Eng. 1980, 102, 14–20. [Google Scholar] [CrossRef]
- Greitzer, E.M. Review axial compressor stall phenomena. J. Fluids Eng. 1980, 102, 134–151. [Google Scholar] [CrossRef]
- Greitzer, E. Surge and Rotating Stall in Axial Flow Compressors—Part I: Theoretical Compression System Model. ASME J. Eng. Gas Turbines Power 1976, 98, 190–198. [Google Scholar] [CrossRef]
- Greitzer, E. Surge and Rotating Stall in Axial Flow Compressors—Part II: Experimental Results and Comparison with Theory. ASME J. Eng. Gas Turbines Power 1976, 98, 199–211. [Google Scholar] [CrossRef]
- Cumpsty, N.; Greitzer, E.M. A Simple Model for Compressor Stall Cell Propagation. J. Eng. Power 1982, 104, 170–176. [Google Scholar] [CrossRef]
- Moore, F. A Theory of Rotating Stall of Multistage Axial Compressors: Part I—Small Disturbances. ASME J. Eng. Gas Turbines Power 1984, 106, 313–320. [Google Scholar] [CrossRef]
- Moore, F. A Theory of Rotating Stall of Multistage Axial Compressors: Part II—Finite Disturbances. ASME J. Eng. Gas Turbines Power 1984, 106, 321–326. [Google Scholar] [CrossRef]
- Moore, F. A Theory of Rotating Stall of Multistage Axial Compressors: Part III—Limit Cycles. ASME J. Eng. Gas Turbines Power 1984, 106, 327–334. [Google Scholar] [CrossRef]
- Gogoi, A.; Verma, S.; Sane, S.K. A model for rotating stall and surge in axial flow compressors. In Proceedings of the 38th AIAA/ASME/SAE/ASEE Joint Propulsion Conference & Exhibit, Indianapolis, IN, USA, 7–10 July 2002. [Google Scholar]
- Benavides, E.M. On the Theoretical Calculation of the Stability Line of an Axial-flow Compressor Stage. Int. J. Turbo. Jet-Engines 2011, 28, 285–298. [Google Scholar] [CrossRef]
- Benavides, E.M.; Juste, G.L. Analytical Calculation of Stall-inception and Surge Points for an Axial-flow Compressor Rotor. Int. J. Turbo. JET-Engines 2012, 29, 243–257. [Google Scholar] [CrossRef][Green Version]
- Vo, H.D.; Tan, C.S.; Greitzer, E.M. Criteria for spike initiated rotating stall. J. Turbomach. 2008, 130, 011023-1-9. [Google Scholar] [CrossRef]
- Lu, X.; Chu, C.; Zhu, J.; Wu, Y. Mechanism of the interaction between casing treatment and tip leakage flow in a subsonic axial compressor. In Proceedings of the GT2006 ASME Turbo Expo ASME Paper 2006 Power for Land Sea and Air, Barcelona, Spain, 8–11 May 2006. [Google Scholar]
- Khaleghi, H. Effect of axial skewed slot casing treatment in a transonic fan. Proc. IMechE Part G J. Aerosp. Eng. 2017, 231, 2646–2653. [Google Scholar] [CrossRef]
- Zhou, X.; Zhao, Q. Investigation on Axial Effect of Slot Casing Treatment in a Transonic Compressor. Appl. Therm. Eng. 2017, 126, 53–69. [Google Scholar] [CrossRef]
- Hwang, Y.; Kang, S.H. Numerical study on the effects of casing treatment on unsteadiness of tip leakage flow in an axial compressor. In Proceedings of the ASME Turbo Expo 2012 GT2012, Copenhagen, Denmark, 11–15 June 2012. [Google Scholar]
- Gourdain, N.; Burguburu, S.; Leboeuf, F.; Michon, G.J. Simulation of rotating stall in a whole stage of an axial compressor. Comput. Fluids 2010, 39, 1644–1655. [Google Scholar] [CrossRef]
- Cornelius, C.; Biesinger, T.; Galpin, P.; Braune, A. Experimental and computational analysis of a multistage axial compressor including stall prediction by steady and transient CFD methods. J. Turbomach. 2014, 136, 1–12. [Google Scholar] [CrossRef]
- Day, I.J.; Greitzer, E.M.; Cumpsty, N.A. Prediction of compressor performance in rotating stall. J. Eng. Power 1978, 100, 1–12. [Google Scholar] [CrossRef]
- Tan, C.S.; Day, I.; Morris, S.; Wadia, A. Spike-type compressor stall inception, detection, and control. Annu. Rev. Fluid Mech. 2010, 42, 275–300. [Google Scholar] [CrossRef]
- Akhlaghi, M. Application of a Vane-Recessed Tubular-Passage Casing Treatment to a Multistage Axial-Flow Compressor. Ph.D. Thesis, University of Cranfield, Cranfield, UK, 2001. [Google Scholar]
- Akhlaghi, M.; Elder, R.L.; Ramsden, K.W. Effects of a vane-recessed tubular-passage passive stall control technique on a multistage, low-speed, axial-flow compressor: Results of tests on the first stage with the rear stages removed. In Proceedings of the ASME Turbo Expo 2003 Power for Land Sea and Air, Atlanta, GE, USA, 16–19 June 2003. [Google Scholar]
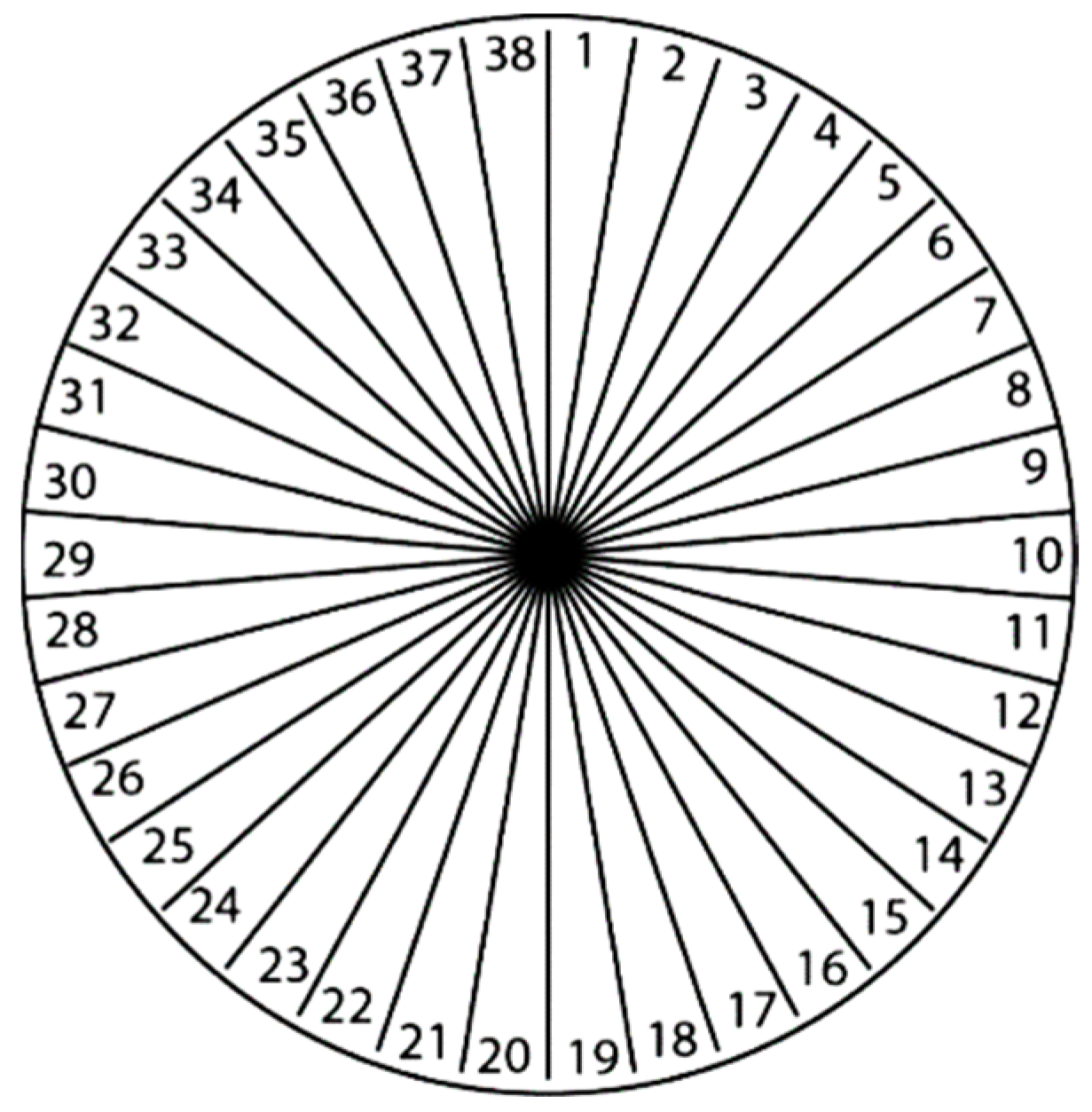


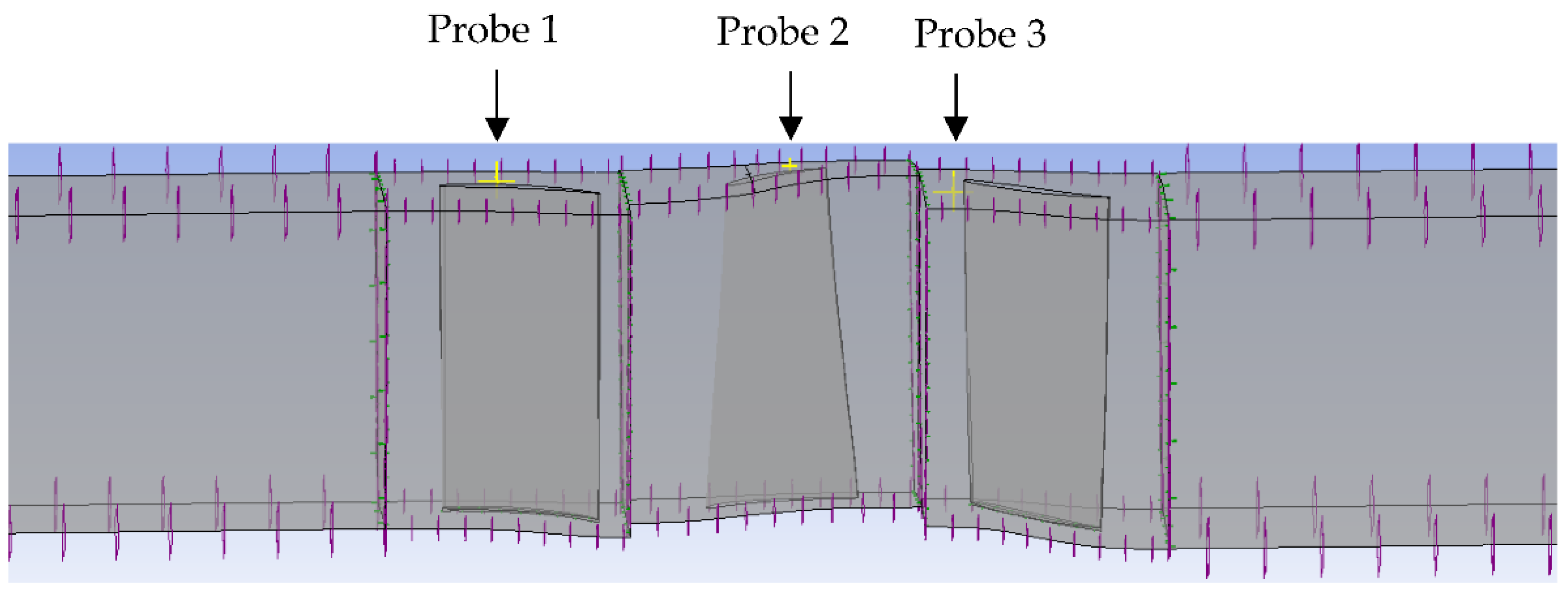

| Code | Mass Flow in One Passage (kg/s) |
|---|---|
| A | 0.0635306 |
| B | 0.0617871 |
| C | 0.0559985 |
| D | 0.0540200 |
| E | 0.0504862 |
| F | 0.0453949 |
| G | 0.0424009 |
| H | 0.0406331 |
| I | 0.0382239 |
| J | 0.0266138 |
| K | 0.0203937 |
| Run | Total Mass Flow Rate (kg/s) | Combination Code |
|---|---|---|
| 1 | 2.4141628 | AAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAA |
| 2 | 2.4106758 | AAAAAAAAAAAAAAAAAABBAAAAAAAAAAAAAAAAAA |
| 3 | 2.3956116 | AAAAAAAAAAAAAAAAABCCBAAAAAAAAAAAAAAAAA |
| 4 | 2.3765904 | AAAAAAAAAAAAAAAABCDDCBAAAAAAAAAAAAAAAA |
| 5 | 2.35050 | AAAAAAAAAAAAAAABCDEEDCBAAAAAAAAAAAAAAA |
| 6 | 2.3142302 | AAAAAAAAAAAAAABCDEFFEDCBAAAAAAAAAAAAAA |
| 7 | 2.2719708 | AAAAAAAAAAAAABCDEFGGFEDCBAAAAAAAAAAAAA |
| 8 | 2.2261758 | AAAAAAAAAAAABCDEFGHHGFEDCBAAAAAAAAAAAA |
| 9 | 2.1755624 | AAAAAAAAAAABCDEFGHIIHGFEDCBAAAAAAAAAAA |
| 10 | 2.1017288 | AAAAAAAAAABCDEFGHIJJIHGFEDCBAAAAAAAAAA |
| 11 | 2.015455 | AAAAAAAAABCDEFGHIJKKJIHGFEDCBAAAAAAAAA |
| 12 | 1.9291812 | AAAAAAAABCDEFGHIJKKKKJIHGFEDCBAAAAAAAA |
| 13 | 1.8429074 | AAAAAAABCDEFGHIJKKKKKKJIHGFEDCBAAAAAAA |
| 14 | 1.7566336 | AAAAAABCDEFGHIJKKKKKKKKJIHGFEDCBAAAAAA |
| 15 | 1.6703598 | AAAAABCDEFGHIJKKKKKKKKKKJIHGFEDCBAAAAA |
| 16 | 1.584086 | AAAABCDEFGHIJKKKKKKKKKKKKJIHGFEDCBAAAA |
| 17 | 1.4978122 | AAABCDEFGHIJKKKKKKKKKKKKKKJIHGFEDCBAAA |
| 18 | 1.4115384 | AABCDEFGHIJKKKKKKKKKKKKKKKKJIHGFEDCBAA |
| 19 | 1.3252646 | ABCDEFGHIJKKKKKKKKKKKKKKKKKKJIHGFEDCBA |
| 20 | 1.2389908 | BCDEFGHIJKKKKKKKKKKKKKKKKKKKKJIHGFEDCB |
| 21 | 1.156204 | CDEFGHIJKKKKKKKKKKKKKKKKKKKKKKJIHGFEDC |
| 22 | 1.0849944 | DEFGHIJKKKKKKKKKKKKKKKKKKKKKKKKJIHGFED |
| 23 | 1.0177418 | EFGHIJKKKKKKKKKKKKKKKKKKKKKKKKKKJIHGFE |
| 24 | 0.9575568 | FGHIJKKKKKKKKKKKKKKKKKKKKKKKKKKKKJIHGF |
| 25 | 0.9075544 | GHIJKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKJIHG |
| 26 | 0.86354 | HIJKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKJIH |
| 27 | 0.8230612 | IJKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKJI |
| 28 | 0.7874008 | JKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKJ |
| 29 | 0.7749606 | KKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKK |
| Run | Total Mass Flow Rate (kg/s) | Combination Code |
|---|---|---|
| 1 | 2.4141628 | AAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAA |
| 2 | 2.4071888 | AAAAAAAABBAAAAAAAAAAAAAAAAAABBAAAAAAAA |
| 3 | 2.3770604 | AAAAAAABCCBAAAAAAAAAAAAAAAABCCBAAAAAAA |
| 4 | 2.339018 | AAAAAABCDDCBAAAAAAAAAAAAAABCDDCBAAAAAA |
| 5 | 2.2868404 | AAAAABCDEEDCBAAAAAAAAAAAABCDEEDCBAAAAA |
| 6 | 2.2142976 | AAAABCDEFFEDCBAAAAAAAAAABCDEFFEDCBAAAA |
| 7 | 2.1297788 | AAABCDEFGGFEDCBAAAAAAAABCDEFGGFEDCBAAA |
| 8 | 2.0381888 | AABCDEFGHHGFEDCBAAAAAABCDEFGHHGFEDCBAA |
| 9 | 1.936962 | ABCDEFGHIIHGFEDCBAAAABCDEFGHIIHGFEDCBA |
| 10 | 1.7892948 | BCDEFGHIJJIHGFEDCBAABCDEFGHIJJIHGFEDCB |
| 11 | 1.6202342 | CDEFGHIJKKJIHGFEDCBBCDEFGHIJKKJIHGFEDC |
| 12 | 1.4662378 | DEFGHIJKKKKJIHGFEDCCDEFGHIJKKKKJIHGFED |
| 13 | 1.3277756 | EFGHIJKKKKKKJIHGFEDDEFGHIJKKKKKKJIHGFE |
| 14 | 1.200338 | FGHIJKKKKKKKKJIHGFEEFGHIJKKKKKKKKJIHGF |
| 15 | 1.0901506 | GHIJKKKKKKKKKKJIHGFFGHIJKKKKKKKKKKJIHG |
| 16 | 0.9961338 | HIJKKKKKKKKKKKKJIHGGHIJKKKKKKKKKKKKJIH |
| 17 | 0.9116406 | IJKKKKKKKKKKKKKKJIHHIJKKKKKKKKKKKKKKJI |
| 18 | 0.8355014 | JKKKKKKKKKKKKKKKKJIIJKKKKKKKKKKKKKKKKJ |
| 19 | 0.7874008 | KKKKKKKKKKKKKKKKKKJJKKKKKKKKKKKKKKKKKK |
| 20 | 0.7749606 | KKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKK |
| Run | Total Mass Flow Rate (kg/s) | Combination Code |
|---|---|---|
| 1 | 2.4141628 | AAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAA |
| 2 | 2.4037018 | AAAAABBAAAAAAAAAAABBAAAAAAAAAAABBAAAAA |
| 3 | 2.3585092 | AAAABCCBAAAAAAAAABCCBAAAAAAAAABCCBAAAA |
| 4 | 2.3014456 | AAABCDDCBAAAAAAABCDDCBAAAAAAABCDDCBAAA |
| 5 | 2.2231792 | AABCDEEDCBAAAAABCDEEDCBAAAAABCDEEDCBAA |
| 6 | 2.114365 | ABCDEFFEDCBAAABCDEFFEDCBAAABCDEFFEDCBA |
| 7 | 1.9875868 | BCDEFGGFEDCBABCDEFGGFEDCBABCDEFGGFEDCB |
| 8 | 1.8571758 | CDEFGHHGFEDCBCDEFGHHGFEDCBCDEFGHHGFEDC |
| 9 | 1.738951 | DEFGHIIHGFEDCDEFGHIIHGFEDCDEFGHIIHGFED |
| 10 | 1.5705568 | EFGHIJJIHGFEDEFGHIJJIHGFEDEFGHIJJIHGFE |
| 11 | 1.3953744 | FGHIJKKJIHGFEFGHIJKKJIHGFEFGHIJJJJIHGF |
| 12 | 1.2227444 | GHIJKKKKJIHGFGHIJKKKKJIHGFGHIJKKKKJIHG |
| 13 | 1.0847132 | HIJKKKKKKJIHGHIJKKKKKKJIHGHIJKKKKKKJIH |
| 14 | 0.9597412 | IJKKKKKKKKJIHIJKKKKKKKKJIHIJKKKKKKKKJI |
| 15 | 0.8479416 | JKKKKKKKKKKJIJKKKKKKKKKKJIJKKKKKKKKKKJ |
| 16 | 0.7874008 | KKKKKKKKKKKKJKKKKKKKKKKKKJKKKKKKKKKKKK |
| 17 | 0.7749606 | KKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKK |
| Run | Total Mass Flow Rate (kg/s) | Combination Code |
|---|---|---|
| 1 | 2.4141628 | AAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAA |
| 2 | 2.4002148 | AAAABBAAAAAAAABBAAAAAAAABBAAAAAAAABBAA |
| 3 | 2.339958 | AAABCCBAAAAAABCCBAAAAAABCCBAAAAAABCCBA |
| 4 | 2.2638732 | AABCDDCBAAAABCDDCBAAAABCDDCBAAAABCDDCB |
| 5 | 2.1612615 | ABCDEEDCBAABCDEEDCBAABCDEEDCBAABCDEEDC |
| 6 | 2.023708 | BCDEFFEDCBBCDEFFEDCBBCDEFFEDCBBCDEFFED |
| 7 | 1.8763855 | CDEFGGFEDCCDEFGGFEDCCDEFGGFEDCCDEFGGFE |
| 8 | 1.7589746 | DEFGHHGFEDDEFGHHGFEDDEFGHHGFEDDEFGHHGF |
| 9 | 1.6412309 | EFGHIIHGFEEFGHIIHGFEEFGHIIHGFEEFGHIIHG |
| 10 | 1.458337 | FGHIJJIHGFFGHIJJIHGFFGHIJJIHGFFGHIJJIH |
| 11 | 1.2630892 | GHIJKKJIHGGHIJKKJIHGGHIJKKJIHGGHIJKKJI |
| 12 | 1.0912086 | HIJKKKKJIHHIJKKKKJIHHIJKKKKJIHHIJKKKKJ |
| 13 | 0.9433127 | IJKKKKKKJIIJKKKKKKJIIJKKKKKKJIIJKKKKKK |
| 14 | 0.8185013 | JKKKKKKKKJJKKKKKKKKJJKKKKKKKKJJKKKKKKK |
| 15 | 0.7749606 | KKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKK |
| Run | Total Mass Flow Rate (kg/s) | Combination Code |
|---|---|---|
| 1 | 2.4141628 | AAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAA |
| 2 | 2.3967278 | AAABBAAAAAABBAAAAAABBAAAAAABBAAAAAABBA |
| 3 | 2.3214068 | AABCCBAAAABCCBAAAABCCBAAAABCCBAAAABCCB |
| 4 | 2.2280443 | ABCDDCBAABCDDCBAABCDDCBAABCDDCBAABCDDC |
| 5 | 2.1051324 | BCDEEDCBBCDEEDCBBCDEEDCBBCDEEDCBBCDEED |
| 6 | 1.9489775 | CDEFFEDCCDEFFEDCCDEFFEDCCDEFFEDCCDEFFE |
| 7 | 1.8185138 | DEFGGFEDDEFGGFEDDEFGGFEDDEFGGFEDDEFGGF |
| 8 | 1.6932699 | EFGHHGFEEFGHHGFEEFGHHGFEEFGHHGFEEFGHHG |
| 9 | 1.5787322 | FGHIIHGFFGHIIHGFFGHIIHGFFGHIIHGFFGHIIH |
| 10 | 1.395683 | GHIJJIHGGHIJJIHGGHIJJIHGGHIJJIHGGHIJJI |
| 11 | 1.179788 | HIJKKJIHHIJKKJIHHIJKKJIHHIJKKJIHHIJKKJ |
| 12 | 0.9914133 | IJKKKKJIIJKKKKJIIJKKKKJIIJKKKKJIIJKKKK |
| 13 | 0.8309415 | JKKKKKKJJKKKKKKJJKKKKKKJJKKKKKKJJKKKKK |
| 14 | 0.7749606 | KKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKK |
| Run | Total Mass Flow Rate (kg/s) | Combination Code |
|---|---|---|
| 1 | 2.4141628 | AAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAA |
| 2 | 2.3932408 | AABBAAAABBAAAABBAAAABBAAAABBAAAABBAAAA |
| 3 | 2.3011121 | ABCCBAABCCBAABCCBAABCCBAABCCBAABCCBAAB |
| 4 | 2.1794528 | BCDDCBBCDDCBBCDDCBBCDDCBBCDDCBBCDDCBBC |
| 5 | 2.0360749 | CDEEDCCDEEDCCDEEDCCDEEDCCDEEDCCDEEDCCD |
| 6 | 1.9033194 | DEFFEDDEFFEDDEFFEDDEFFEDDEFFEDDEFFEDDE |
| 7 | 1.7552651 | EFGGFEEFGGFEEFGGFEEFGGFEEFGGFEEFGGFEEF |
| 8 | 1.6289426 | FGHHGFFGHHGFFGHHGFFGHHGFFGHHGFFGHHGFFG |
| 9 | 1.5381288 | GHIIHGGHIIHGGHIIHGGHIIHGGHIIHGGHIIHGGH |
| 10 | 1.3445066 | HIJJIHHIJJIHHIJJIHHIJJIHHIJJIHHIJJIHHI |
| 11 | 1.0876145 | IJKKJIIJKKJIIJKKJIIJKKJIIJKKJIIJKKJIIJ |
| 12 | 0.8558219 | JKKKKJJKKKKJJKKKKJJKKKKJJKKKKJJKKKKJJK |
| 13 | 0.7749606 | KKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKK |
| Run | Total Mass Flow Rate (kg/s) | Combination Code |
|---|---|---|
| 1 | 2.4141628 | AAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAA |
| 2 | 2.3897538 | AAABBAAABBAAABBAAABBAAABBAAABBAAABBAAA |
| 3 | 2.2843044 | AABCCBABCCBABCCBABCCBABCCBABCCBABCCBAA |
| 4 | 2.161617 | ABCDDCBCDDCBCDDCBCDDCBCDDCBCDDCBCDDCBA |
| 5 | 2.034649 | BCDEEDCDEEDCDEEDCDEEDCDEEDCDEEDCDEEDCB |
| 6 | 1.8864924 | CDEFFEDEFFEDEFFEDEFFEDEFFEDEFFEDEFFEDC |
| 7 | 1.7410708 | DEFGGFEFGGFEFGGFEFGGFEFGGFEFGGFEFGGFED |
| 8 | 1.6266076 | EFGHHGFGHHGFGHHGFGHHGFGHHGFGHHGFGHHGFE |
| 9 | 1.533995 | FGHIIHGHIIHGHIIHGHIIHGHIIHGHIIHGHIIHGF |
| 10 | 1.3175944 | GHIJJIHIJJIHIJJIHIJJIHIJJIHIJJIHIJJIHG |
| 11 | 1.0451624 | HIJKKJIJKKJIJKKJIJKKJIJKKJIJKKJIJKKJIH |
| 12 | 0.8603818 | IJKKKKJKKKKJKKKKJKKKKJKKKKJKKKKJKKKKJI |
| 13 | 0.7874008 | JKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKJ |
| 14 | 0.7749606 | KKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKK |
| Run | Total Mass Flow Rate (kg/s) | Combination Code |
|---|---|---|
| 1 | 2.4141628 | AAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAA |
| 2 | 2.3862668 | AAAABBAABBAABBAABBAABBAABBAABBAABBAAAA |
| 3 | 2.2657532 | AAABCCBBCCBBCCBBCCBBCCBBCCBBCCBBCCBAAA |
| 4 | 2.1379926 | AABCDDCCDDCCDDCCDDCCDDCCDDCCDDCCDDCBAA |
| 5 | 2.03 | ABCDEEDDEEDDEEDDEEDDEEDDEEDDEEDDEEDCBA |
| 6 | 1.8777088 | BCDEFFEEFFEEFFEEFFEEFFEEFFEEFFEEFFEDCB |
| 7 | 1.73 | CDEFGGFFGGFFGGFFGGFFGGFFGGFFGGFFGGFEDC |
| 8 | 1.63 | DEFGHHGGHHGGHHGGHHGGHHGGHHGGHHGGHHGFED |
| 9 | 1.538276 | EFGHIIHHIIHHIIHHIIHHIIHHIIHHIIHHIIHGFE |
| 10 | 1.294261 | FGHIJJIIJJIIJJIIJJIIJJIIJJIIJJIIJJIHGF |
| 11 | 0.9946358 | GHIJKKJJKKJJKKJJKKJJKKJJKKJJKKJJKKJIHG |
| 12 | 0.86354 | HIJKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKJIH |
| 13 | 0.8230612 | IJKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKJI |
| 14 | 0.7874008 | JKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKJ |
| 15 | 0.7749606 | KKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKKK |
| Source of Variation | Sum of Square | Degrees of Freedom | Mean Squares | F | p-Value |
|---|---|---|---|---|---|
| Treatments | 5.22 × 1011 | 34 | 1.53 × 1010 | 38.28 | 0.000 |
| Error | 9.83 × 1010 | 245 | 4.01 × 108 | ||
| Total | 6.20 × 1011 | 279 |
| Source of Variation | Sum of Square | Degrees of Freedom | Mean Squares | F | p-Value |
|---|---|---|---|---|---|
| Treatments | 9.9265 | 34 | 0.29196 | 27.57 | 0.000 |
| Error | 2.5947 | 245 | 0.01059 | ||
| Total | 12.5212 | 279 |
| Parameter | Value |
|---|---|
| Number of IGV blades | 34 |
| Number of rotor blades | 38 |
| Number of stator blades | 37 |
| Rotor blade tip diameter | 405 mm |
| Rotor blade hub diameter | 284.4 mm |
| Tip clearance | 0.7 mm |
| Hub-to-tip ratio | 0.7 |
| Rotor blade chord | 30.5 mm |
| Rotor blade aspect ratio | 2.0 |
| Parameter | Value | |||||
|---|---|---|---|---|---|---|
| Grid Name | Coarse-1 | Coarse-2 | Medium | Fine-1 | Fine-2 | |
| Total number of nodes | 791,713 | 1,101,803 | 2,224,443 | 5,085,835 | 10,565,038 | |
| Time-averaged pressure at monitor point 1 (Pa) | 100,860 | 100,859 | 100,851 | 100,855 | 100,856 | |
| Time-averaged pressure at monitor point 2 (Pa) | 101,444 | 101,453 | 101,459 | 101,471 | 101,472 | |
| Time-averaged pressure at monitor point 3 (Pa) | 101,879 | 101,898 | 101,947 | 101,973 | 102,002 | |
| Time-averaged pressure ratio | 1.003 | 1.003 | 1.004 | 1.004 | 1.004 | |
| Mesh statistics | Min. angle | 23.8 | 23.9 | 21.9 | 20.8 | 19.4 |
| Max. aspect ratio | 679 | 559 | 427 | 346 | 220 | |
| Operating Point | Prediction | Experimental Data | Error |
|---|---|---|---|
| Stall inception | 1.95 | 1.95 | 0 |
| Surge inception | 1.6 | 1.52 | 5.2% |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Akhlaghi, M.; Azizi, Y.; Nouri, N.M. Estimations of Compressor Stall and Surge Using Passage Stall Behaviors. Machines 2022, 10, 706. https://doi.org/10.3390/machines10080706
Akhlaghi M, Azizi Y, Nouri NM. Estimations of Compressor Stall and Surge Using Passage Stall Behaviors. Machines. 2022; 10(8):706. https://doi.org/10.3390/machines10080706
Chicago/Turabian StyleAkhlaghi, Mohammad, Yahya Azizi, and Nourouz Mohammad Nouri. 2022. "Estimations of Compressor Stall and Surge Using Passage Stall Behaviors" Machines 10, no. 8: 706. https://doi.org/10.3390/machines10080706
APA StyleAkhlaghi, M., Azizi, Y., & Nouri, N. M. (2022). Estimations of Compressor Stall and Surge Using Passage Stall Behaviors. Machines, 10(8), 706. https://doi.org/10.3390/machines10080706