Dynamic Modeling, Optimization and Experiment for a High-Speed Spur Gear Set
Abstract
:1. Introduction
2. Static Analysis
2.1. Parameters of Spur Gear Pair
2.2. Unloaded and Loaded Tooth Contact Analysis
3. Optimization of Gear Tooth Tip Relief Modifications
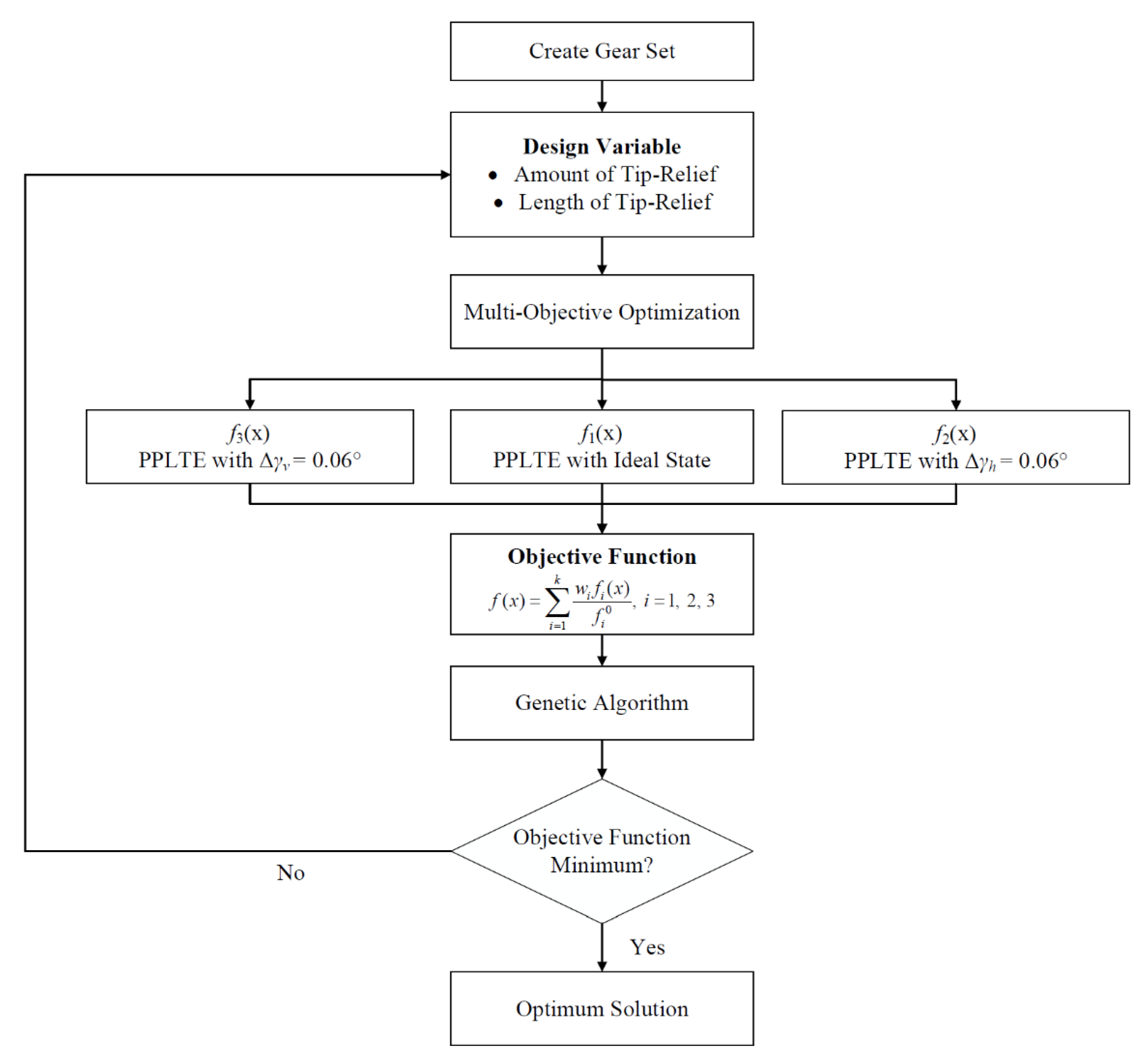
3.1. Formulation of Optimization and DV
3.2. Optimization Results
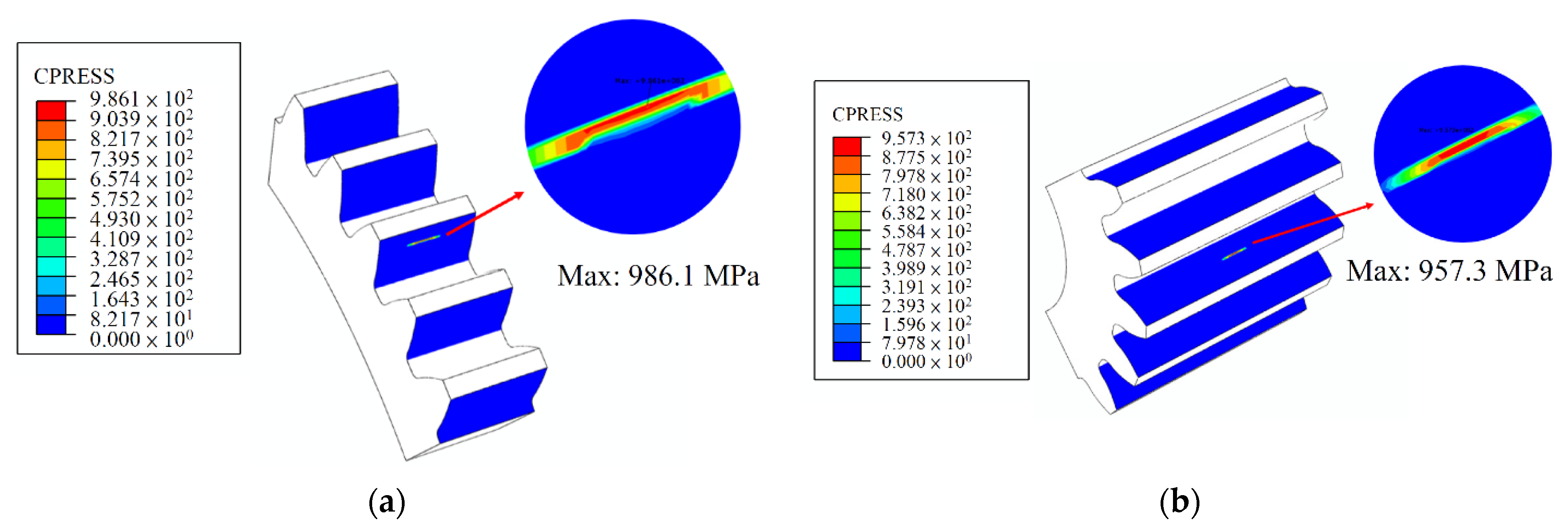
3.3. Contact Stress Analysis of Optimum Design
4. Dynamic Analysis
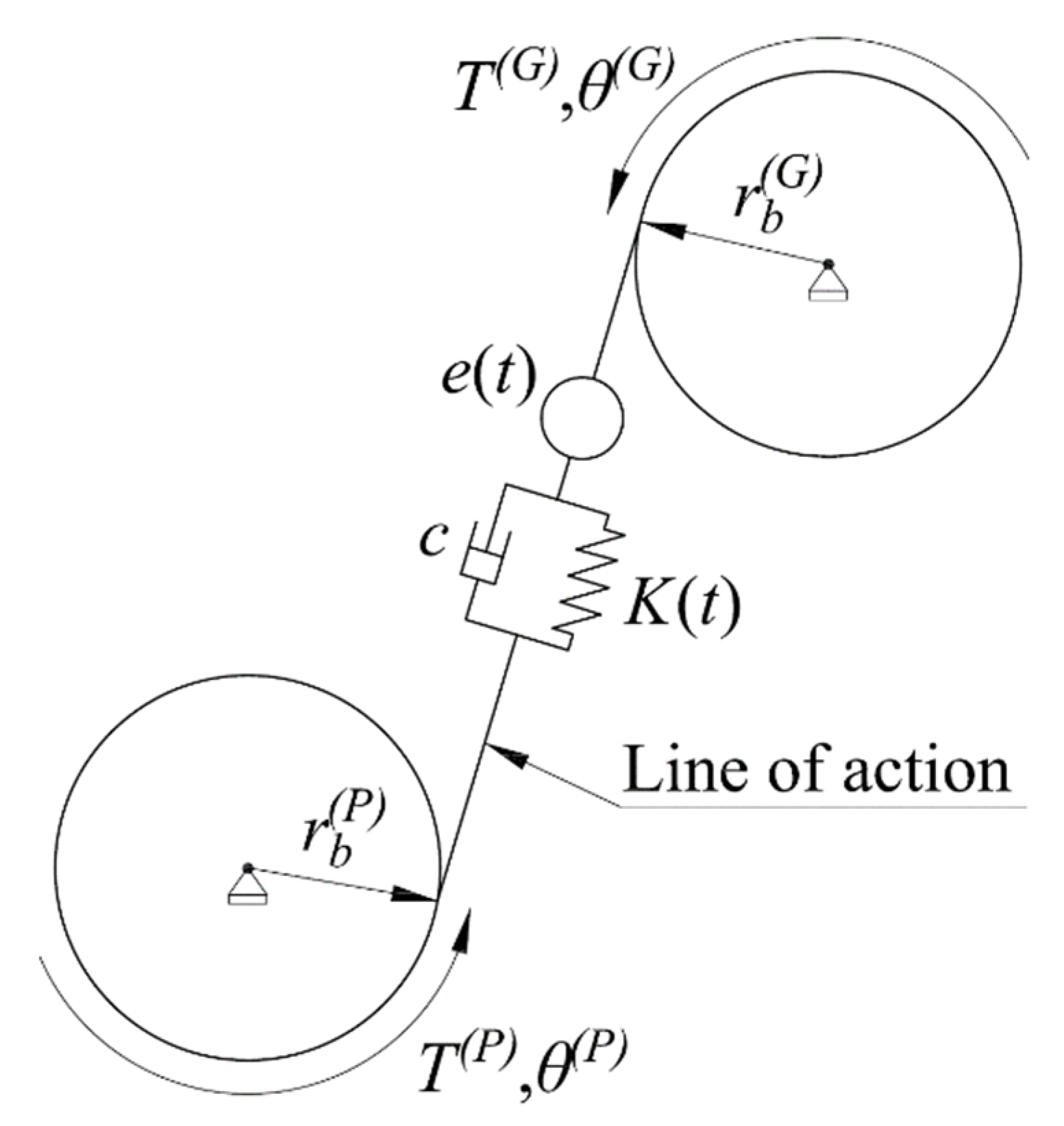
4.1. Dynamic Model
- The gear meshing process was fully lubricated; thus, the influence of friction was ignored.
- Only the mesh stiffness of the gear pair was considered; the compliances of shafts and bearings were disregarded.
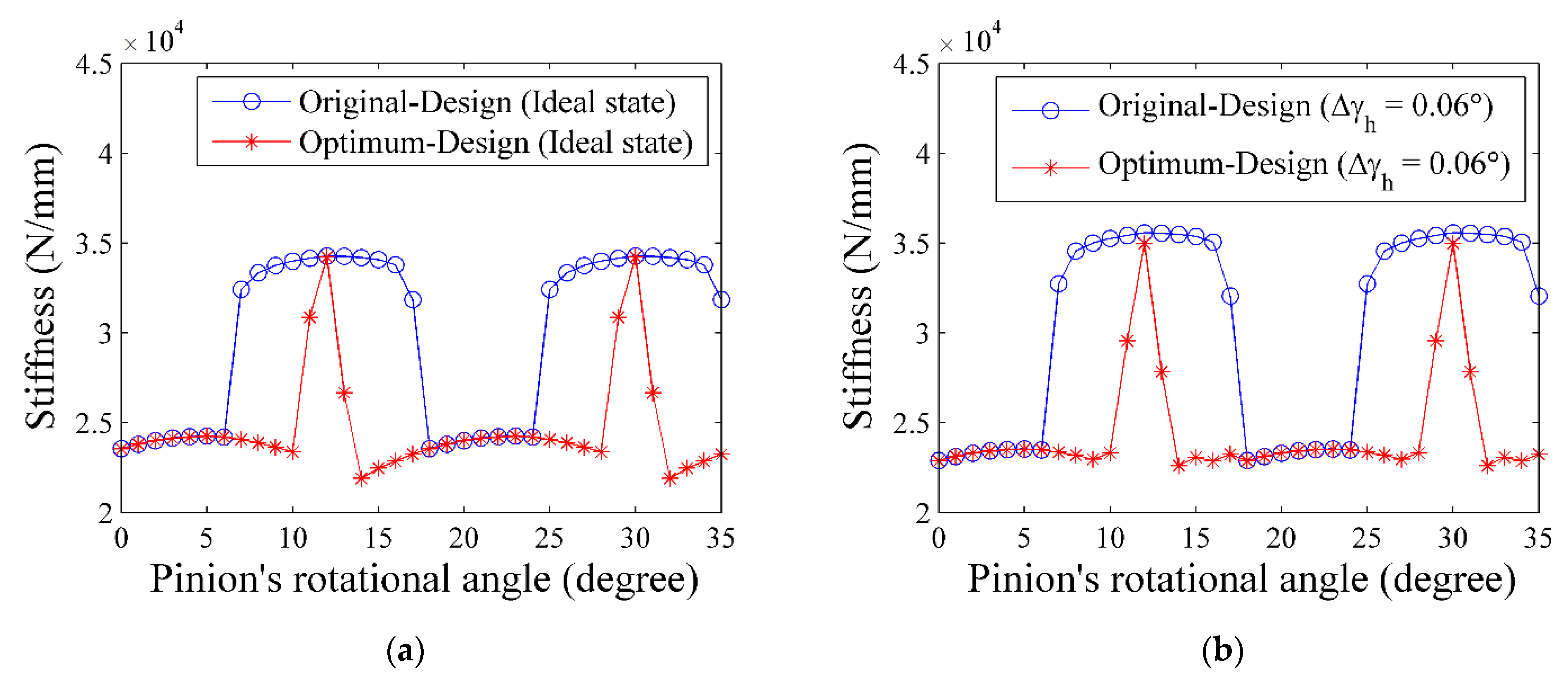
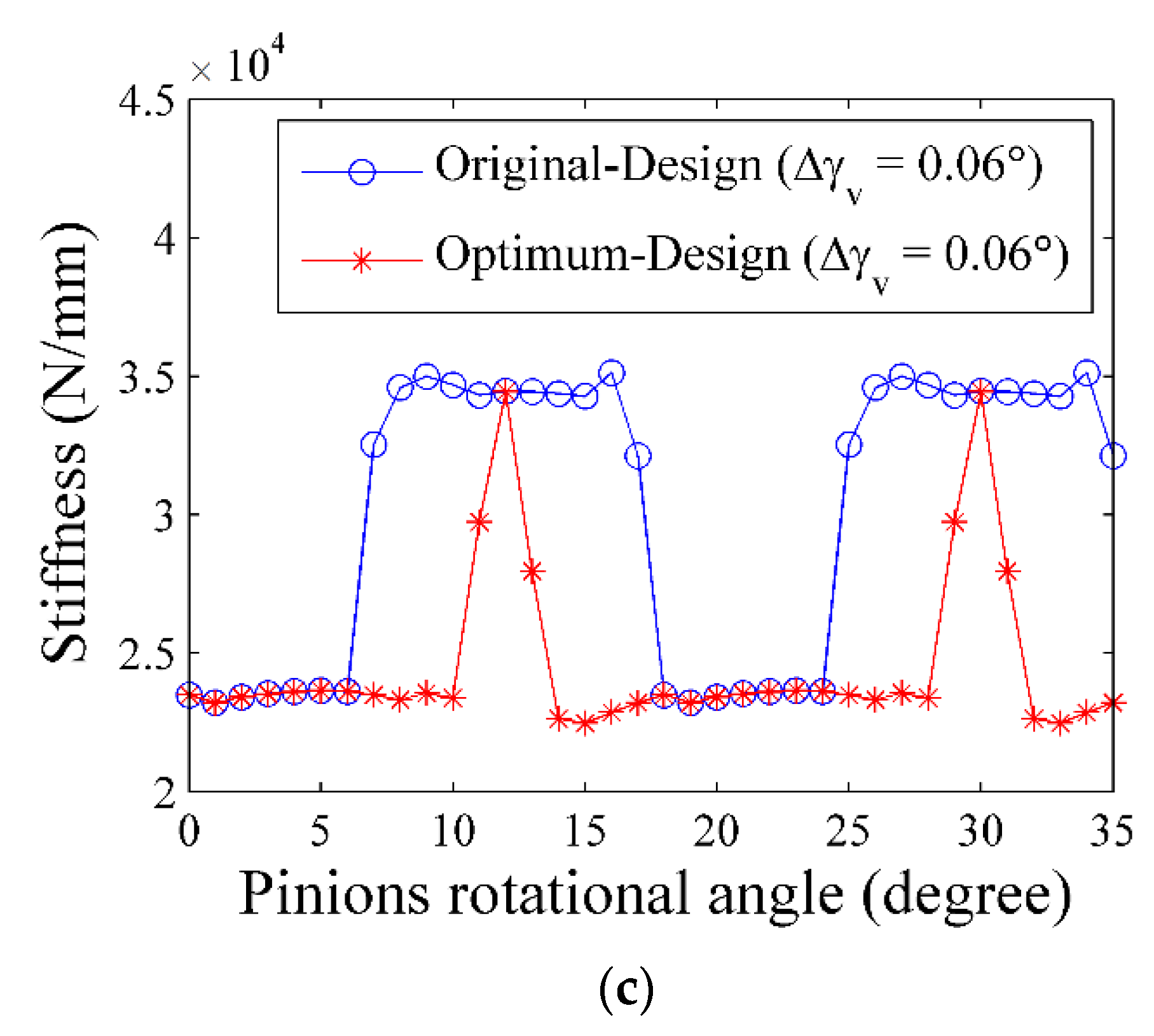
4.2. Mesh Stiffness
4.3. DTE and Acceleration
5. Dynamic Experiments
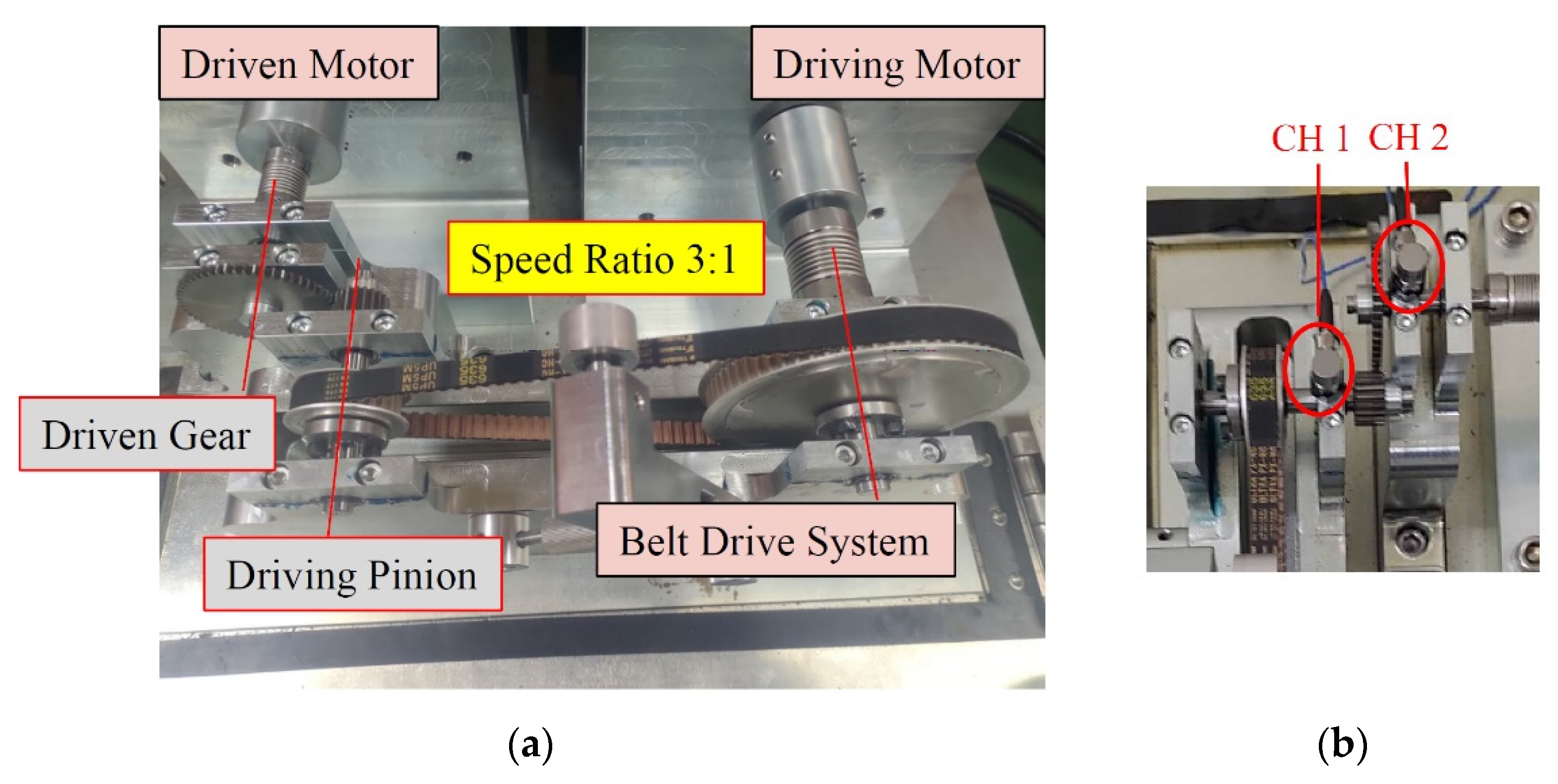
5.1. Experimental Framework
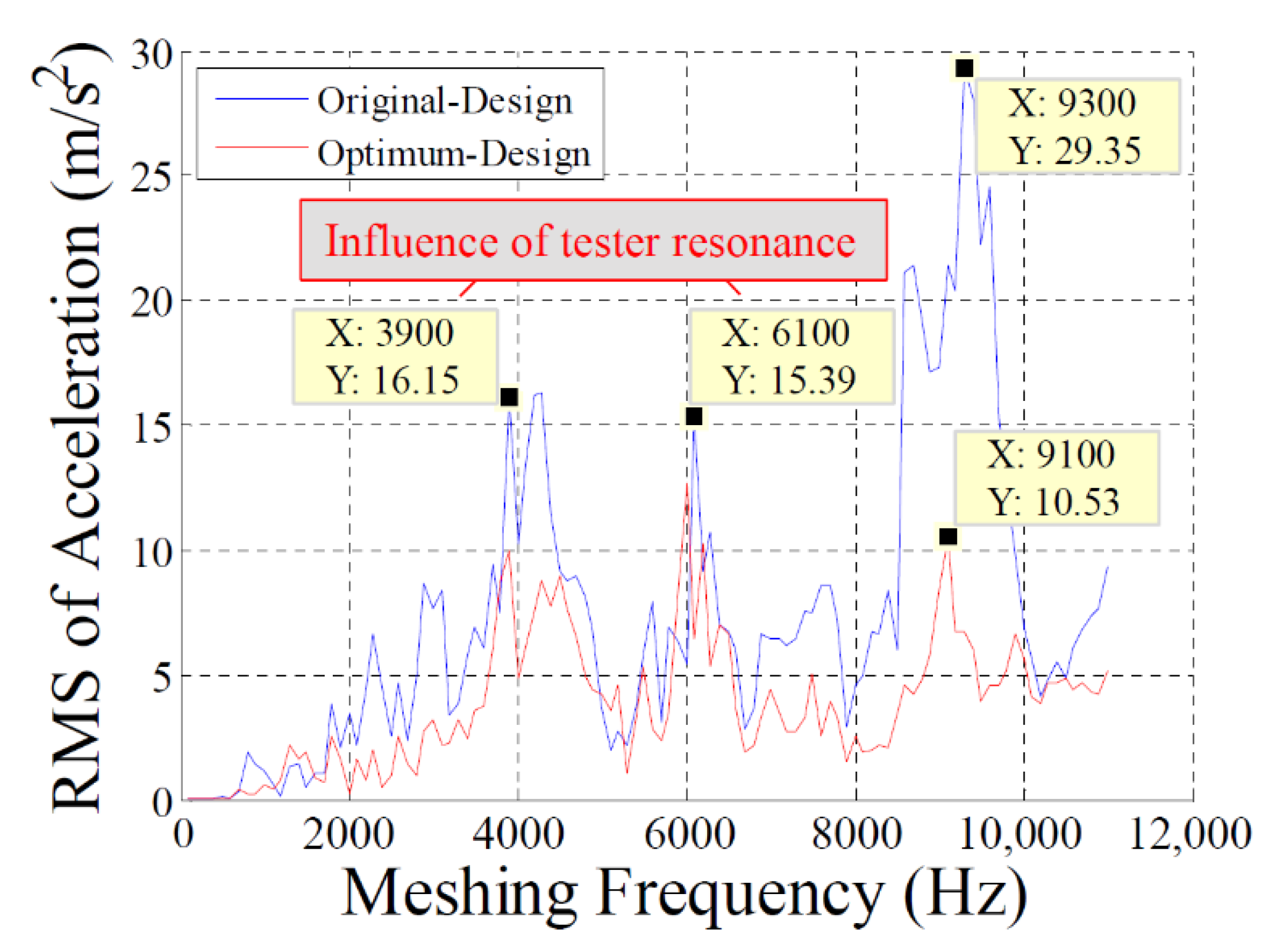
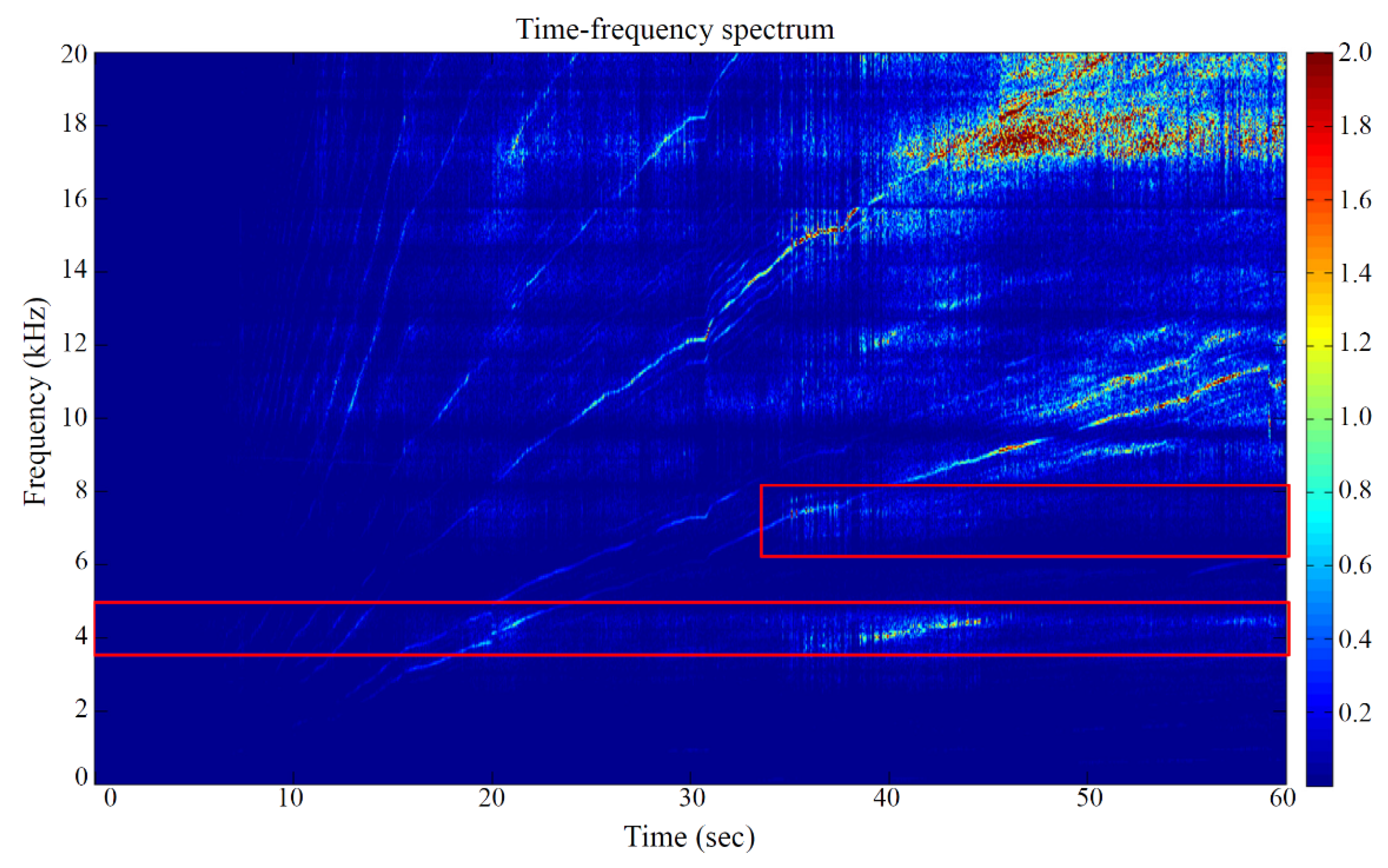
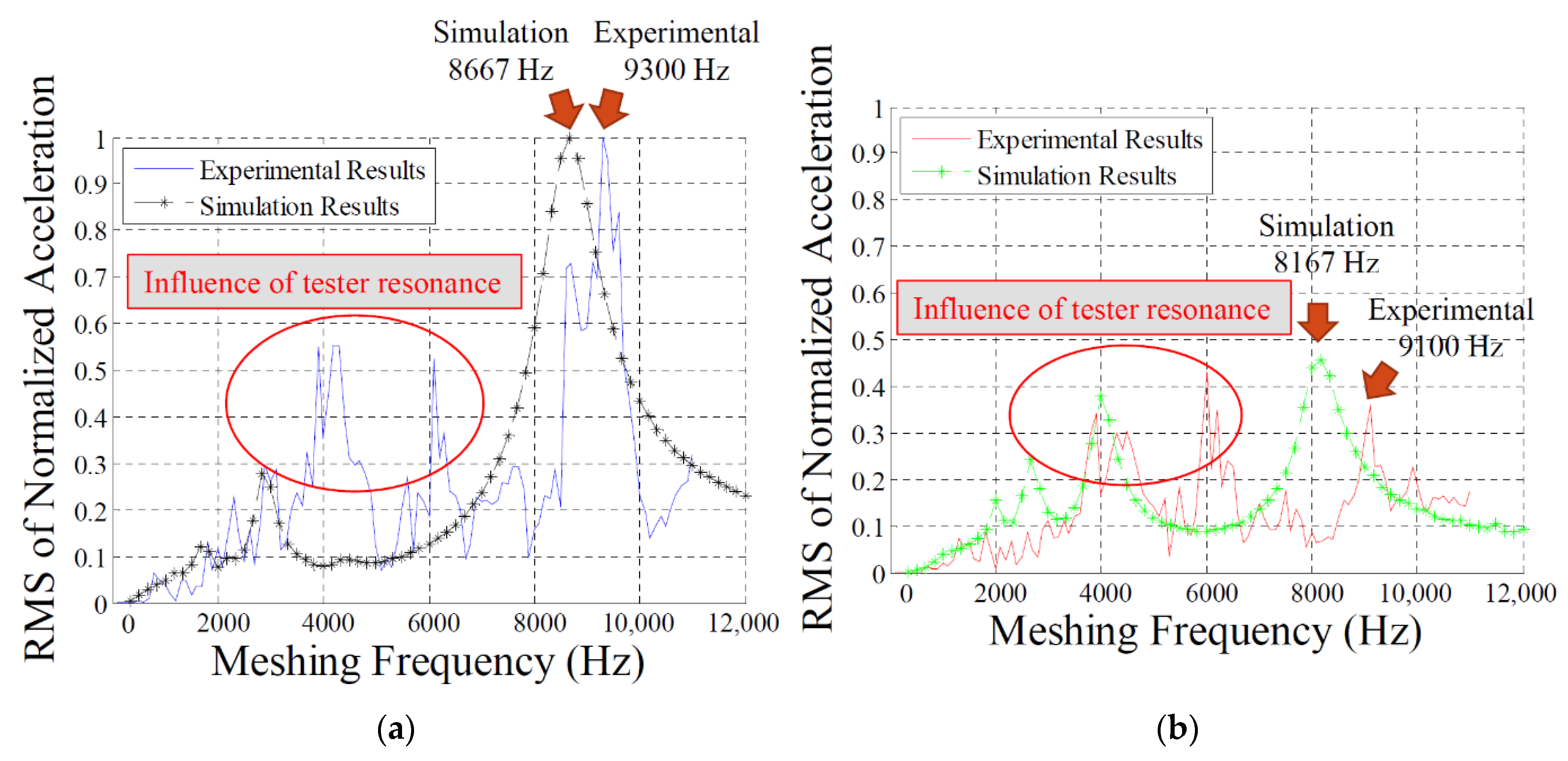
5.2. Results of Dynamic Tests
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Smith, J.D. Gear Noise and Vibration, 2nd ed.; Marcel Dekker: New York, NY, USA, 2003. [Google Scholar]
- Litvin, F.L. Theory of Gearing; NASA Reference Publication 1212: Washington, DC, USA, 1989. [Google Scholar]
- Litvin, F.L.; Fuentes, A. Gear Geometry and Applied Theory, 2nd ed.; Cambridge University Press: New York, NY, USA, 2004. [Google Scholar]
- Chang, S.L.; Tsay, C.B.; Tseng, C.H. Kinematic optimization of a modified helical gear train. J. Mech. Des. 1997, 119, 307–314. [Google Scholar] [CrossRef]
- Chen, Y.C.; Tsay, C.B. Stress analysis of a helical gear set with localized bearing contact. Finite Elem. Anal. Des. 2002, 38, 707–723. [Google Scholar] [CrossRef]
- Bonori, G.; Barbieri, M.; Pellicano, F. Optimum profile modifications of spur gears by means of genetic algorithms. J. Sound Vib. 2008, 313, 603–616. [Google Scholar] [CrossRef]
- Faggioni, M.; Samani, F.S.; Bertacchi, G.; Pellicano, F. Dynamic optimization of spur gears. Mech. Mach. Theory 2011, 46, 544–557. [Google Scholar] [CrossRef]
- Ogawa, Y.; Matsumura, S.; Houjoh, H.; Sato, T. Rotational vibration of a spur gear pair having tooth helix deviation: Effect of lead modifications. In Proceedings of the 2003 ASME Design Engineering Technical Conferences and Computers and Information in Engineering Conference, Chicago, IL, USA, 2–6 September 2003; pp. 433–440. [Google Scholar]
- Houjoh, H.; Ratanasumawong, C.; Matsumura, S. Utilization of synchronous averaging for inspection of tooth surface undulations on gears (Localization of nonmesh harmonic components to individual gear). J. Appl. Mech. 2007, 74, 269–278. [Google Scholar] [CrossRef]
- Ratanasumawong, C.; Matsumura, S.; Houjoh, H. An alternative method for evaluating gear tooth surface geometry based on synchronous average of vibration of a gear pair. In Proceedings of the 2007 ASME Design Engineering Technical Conferences and Computers and Information in Engineering Conference, Las Vegas, NV, USA, 4–7 September 2007; pp. 395–403. [Google Scholar]
- Ratanasumawong, C.; Matsumura, S.; Tatsuno, T.; Houjoh, H. Estimating gear tooth surface geometry by means of the vibration measurement: Distinction of the vibration characteristics of gears with tooth surface form error. J. Mech. Des. 2009, 131, 101003. [Google Scholar] [CrossRef]
- Kahraman, A.; Singh, R. Non-linear dynamics of a spur gear pair. J. Sound Vib. 1990, 142, 49–75. [Google Scholar] [CrossRef]
- Kahraman, A.; Singh, R. Non-linear dynamics of a geared rotor-bearing system with multiple clearances. J. Sound Vib. 1991, 144, 469–506. [Google Scholar] [CrossRef]
- Kahraman, A.; Singh, R. Interactions between time-varying mesh stiffness and clearance non-linearities in a geared system. J. Sound Vib. 1991, 146, 135–156. [Google Scholar] [CrossRef]
- Kahraman, A.; Blankenship, G.W. Experiments on nonlinear dynamic behavior of an oscillator with clearance and periodically time-varying parameters. J. Appl. Mech. 1997, 64, 217–226. [Google Scholar] [CrossRef]
- Kahraman, A.; Blankenship, G.W. Effect of involute tip relief on dynamic response of spur gear pairs. J. Mech. Des. 1999, 121, 313–315. [Google Scholar] [CrossRef]
- Hotait, M.; Kahraman, A. Experiments on the relationship between the dynamic transmission error and the dynamic stress factor of spur gear pairs. Mech. Mach. Theory 2013, 70, 116–128. [Google Scholar] [CrossRef]
- Inalpolat, M.; Handschuh, M.; Kahraman, A. Influence of indexing errors on dynamic response of spur gear pairs. Mech. Syst. Signal Process. 2015, 60, 391–405. [Google Scholar] [CrossRef]
- Anichowski, B.J.; Kahraman, A.; Talbot, D. Dynamic transmission error measurements from spur gear pairs having tooth indexing errors. In Proceedings of the International Design Engineering Technical Conferences and Computers and Information in Engineering Conference, Cleveland, OH, USA, 6–9 August 2017; pp. 1–8. [Google Scholar]
- Wang, Z.G.; Chen, Y.C. Design of a helical gear set with adequate linear tip-relief leading to improved static and dynamic characteristics. Mech. Mach. Theory 2020, 147, 103742. [Google Scholar] [CrossRef]







| Design Parameters | Unit | Pinion | Gear |
|---|---|---|---|
| Number of teeth | - 1 | 20 | 58 |
| Module | mm | 1.0 | 1.0 |
| Pressure angle | ° | 20 | 20 |
| Face width | mm | 12 | 4.07 |
| Rotational speed | rpm | 30,000 | 10,344 |
| Torque | N-mm | 329.35 | 955.11 |
| Longitudinal crowning amount | μm | 0 | 6 |
| Center distance | mm | 39 | |
| DV | Lower Bound | Upper Bound |
|---|---|---|
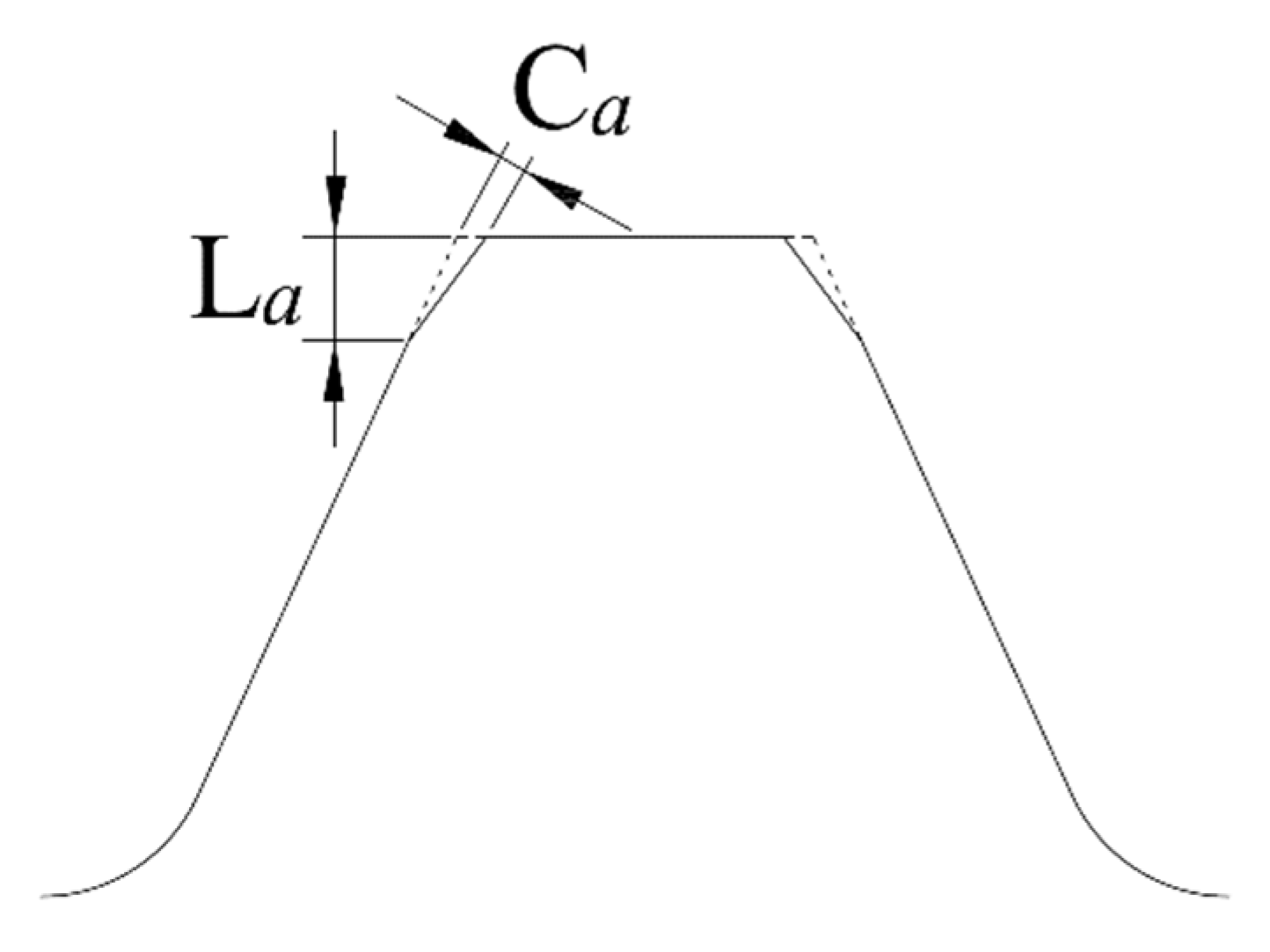
| Ca (μm) | 5 | 30 |
| La (mm) | 0.5 | 2 |
| Case | Assembly Conditions | PPLTE of Original Design (μm) |
|---|---|---|
| (i) | Ideal | 0.721 |
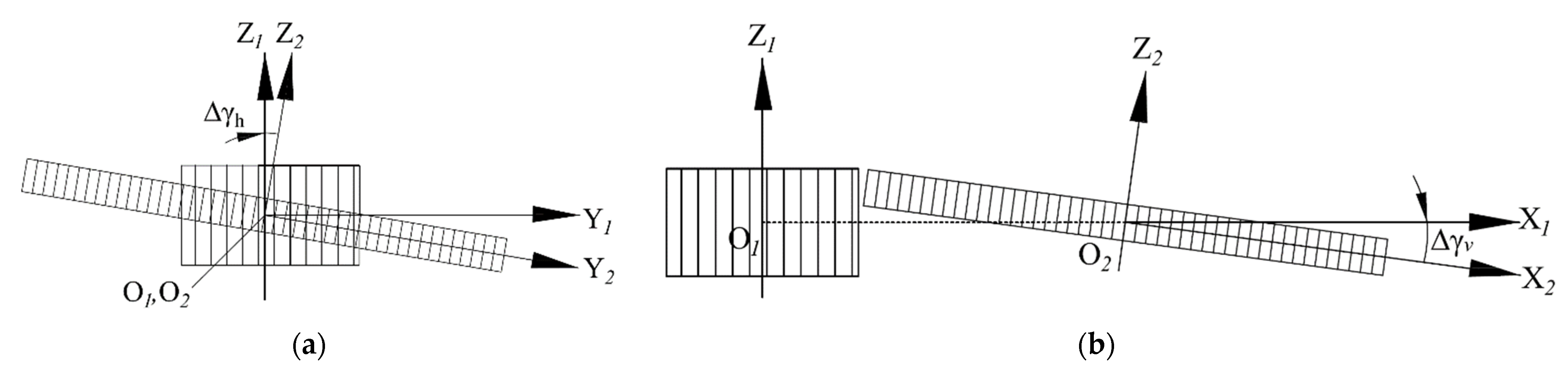
| (ii) | Horizontal axial misalignment = 0.06° | 0.721 |
| (iii) | Vertical axial misalignment = 0.06° | 0.720 |
| Original Design | Optimum Design | |||
|---|---|---|---|---|
| Pinion | Gear | Pinion | Gear | |
| Tip Relief amount Ca(µm) | - | - | 6 | 6 |
| Tip Relief Length La (mm) | - | - | 0.83 | 0.83 |
| Longitudinal crowning (µm) | - | 6 | - | 6 |
| Assembly Conditions | Original Design | Optimum Design | Improvement (%) |
|---|---|---|---|
| Ideal state | 0.721 | 0.191 | 73.5 |
| Horizontal axial misalignment ∆γh = 0.06° | 0.721 | 0.197 | 72.7 |
| Vertical axial misalignment ∆γv = 0.06° | 0.720 | 0.192 | 73.3 |
| Assembly Conditions | Original Design | Optimum Design | Improvement (%) |
|---|---|---|---|
| Ideal state | 77.38 | 36.30 | 53.1 |
| Horizontal axial misalignment = 0.06° | 99.54 | 40.49 | 59.3 |
| Vertical axial misalignment = 0.06° | 96.32 | 39.63 | 58.9 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, Z.-G.; Lo, C.-C.; Chen, Y.-C.; Liu, H.-C. Dynamic Modeling, Optimization and Experiment for a High-Speed Spur Gear Set. Machines 2022, 10, 653. https://doi.org/10.3390/machines10080653
Wang Z-G, Lo C-C, Chen Y-C, Liu H-C. Dynamic Modeling, Optimization and Experiment for a High-Speed Spur Gear Set. Machines. 2022; 10(8):653. https://doi.org/10.3390/machines10080653
Chicago/Turabian StyleWang, Zhi-Gen, Chien-Cheng Lo, Yi-Cheng Chen, and Hung-Chih Liu. 2022. "Dynamic Modeling, Optimization and Experiment for a High-Speed Spur Gear Set" Machines 10, no. 8: 653. https://doi.org/10.3390/machines10080653
APA StyleWang, Z.-G., Lo, C.-C., Chen, Y.-C., & Liu, H.-C. (2022). Dynamic Modeling, Optimization and Experiment for a High-Speed Spur Gear Set. Machines, 10(8), 653. https://doi.org/10.3390/machines10080653






