Design of the Drive Mechanism of a Rotating Feeding Device
Abstract
1. Introduction
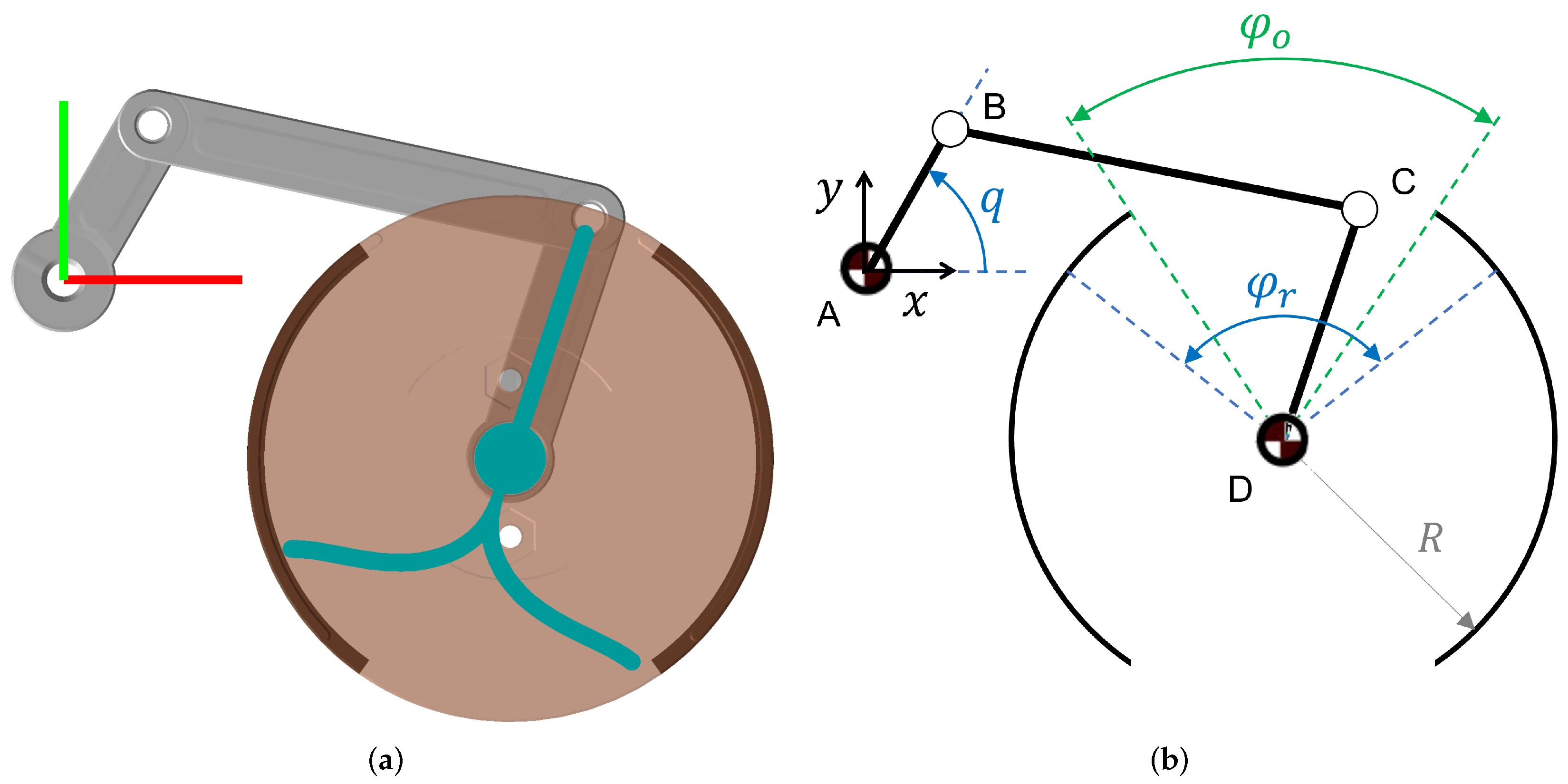
2. Mechanical Design
- A fixed cylindrical structure, in brown in Figure 1a, which acts as a container for the components to be kitted. The cylinder axis is horizontal, and the curved surface has two openings: one at the top to receive new components, and one at the bottom to exit the components.
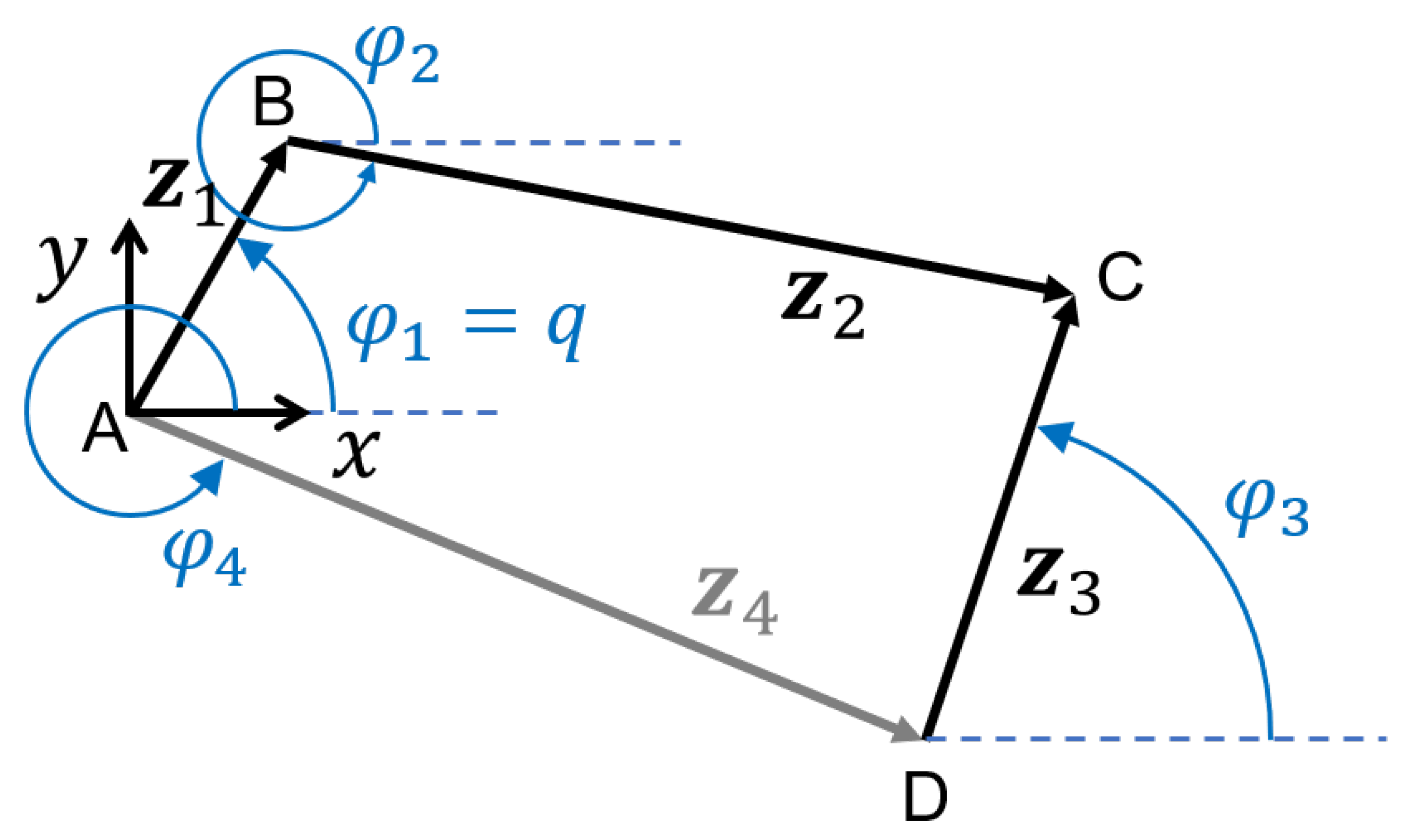
2.1. Design of the Four-Bar Linkage Mechanism
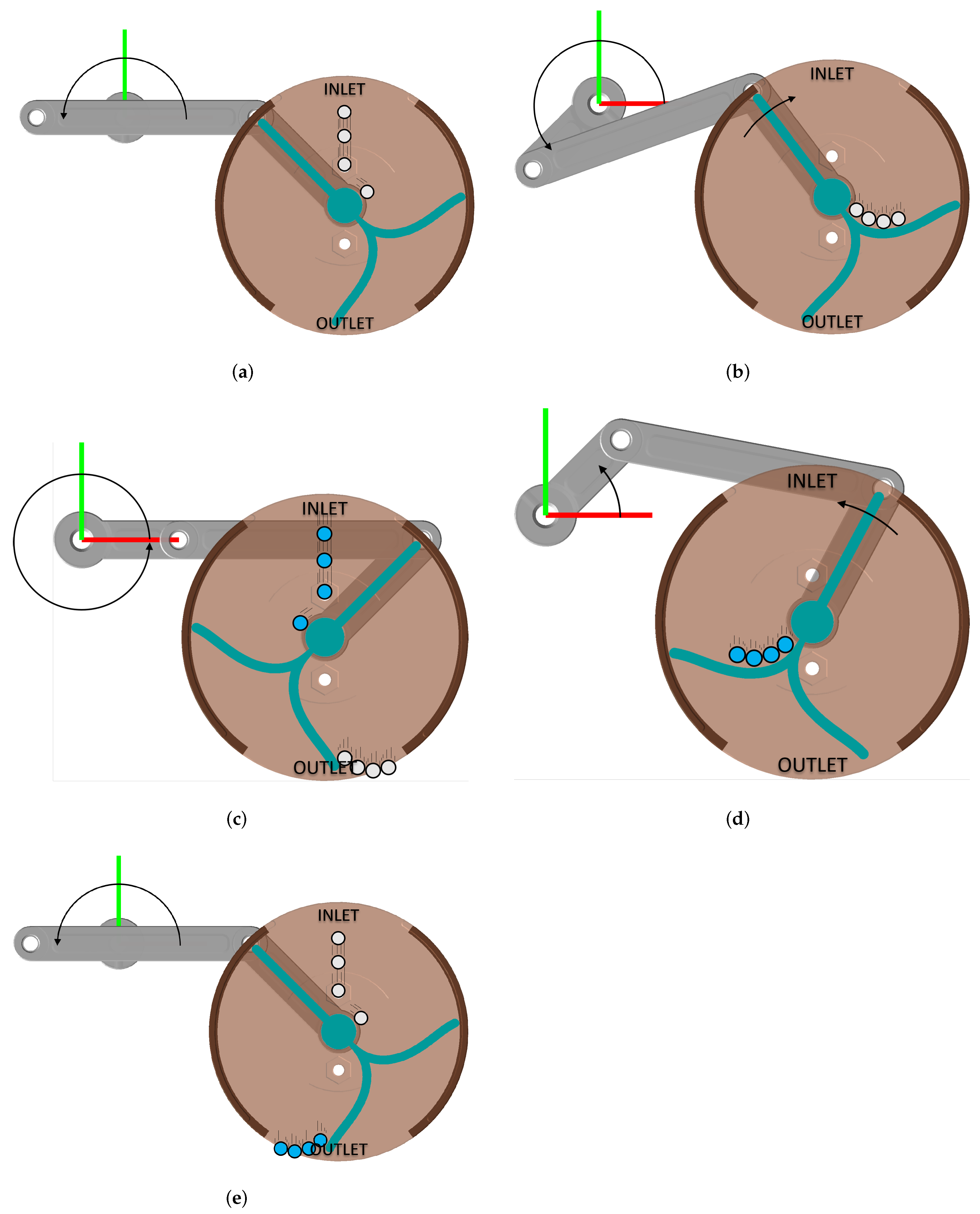
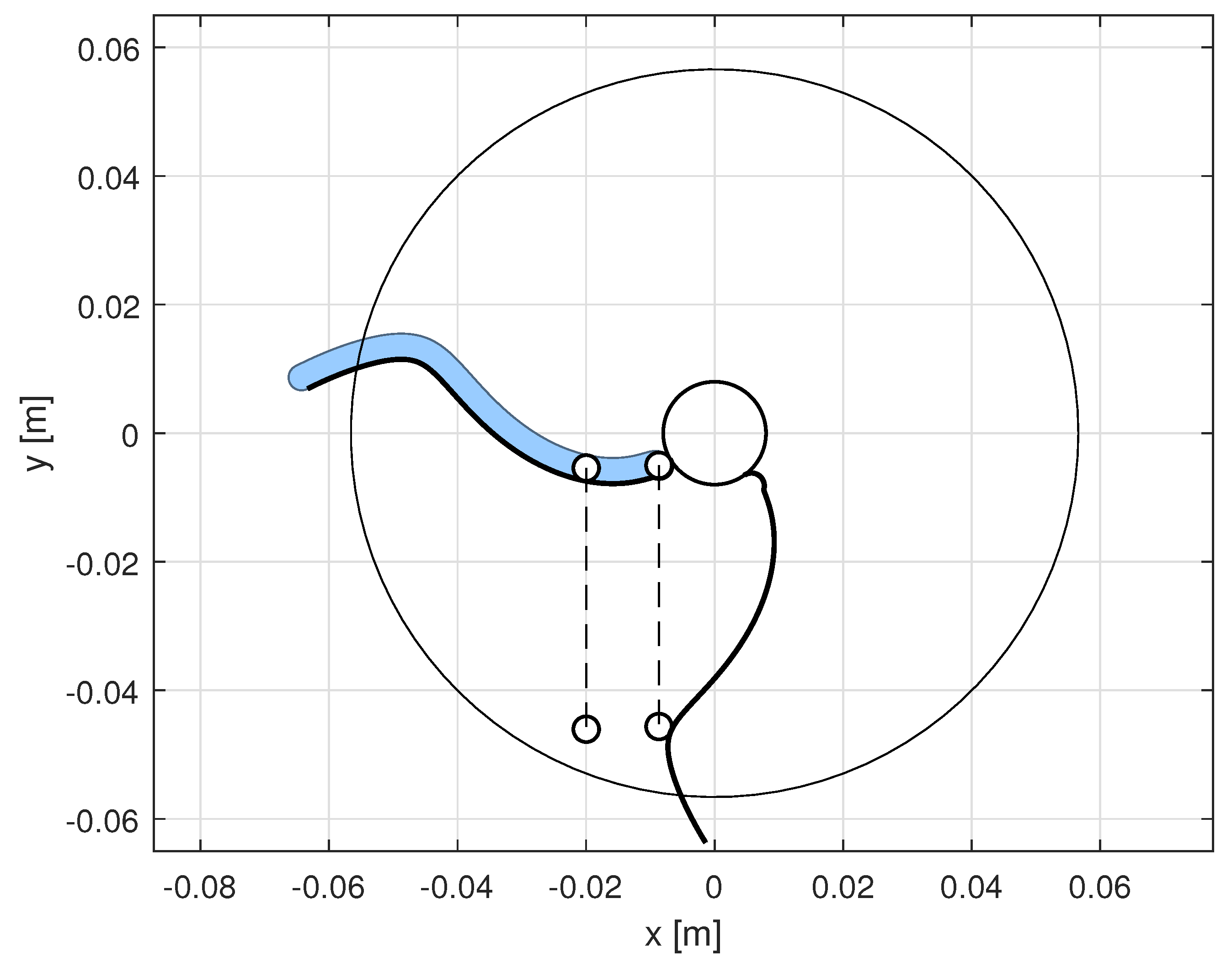
2.2. Design of the Blades
- The starting position of the component falling on the blade is calculated. This position is arbitrary and can be chosen according to specific needs (e.g., the motor shaft dimensions).
- The free falling motion law of the component is calculated to retrieve the movement of the component in the absolute reference frame (0):
- By imposing a specific motion law of the rotary distributor it is possible to calculate the orientation of the reference frame relative to the rotary distributor. In particular, if is the rotation angle of the rotary distributor, the transformation of coordinates between the absolute reference frame (0) and relative reference frame (r) is described by the transformation matrix:
- The position of the center of the component in the relative reference frame can be calculated by means of :
- The blade shape is the lower envelope of the circles centered in in the reference frame. In such a way, the blade will always be very close to the component during its fall.
3. Validation
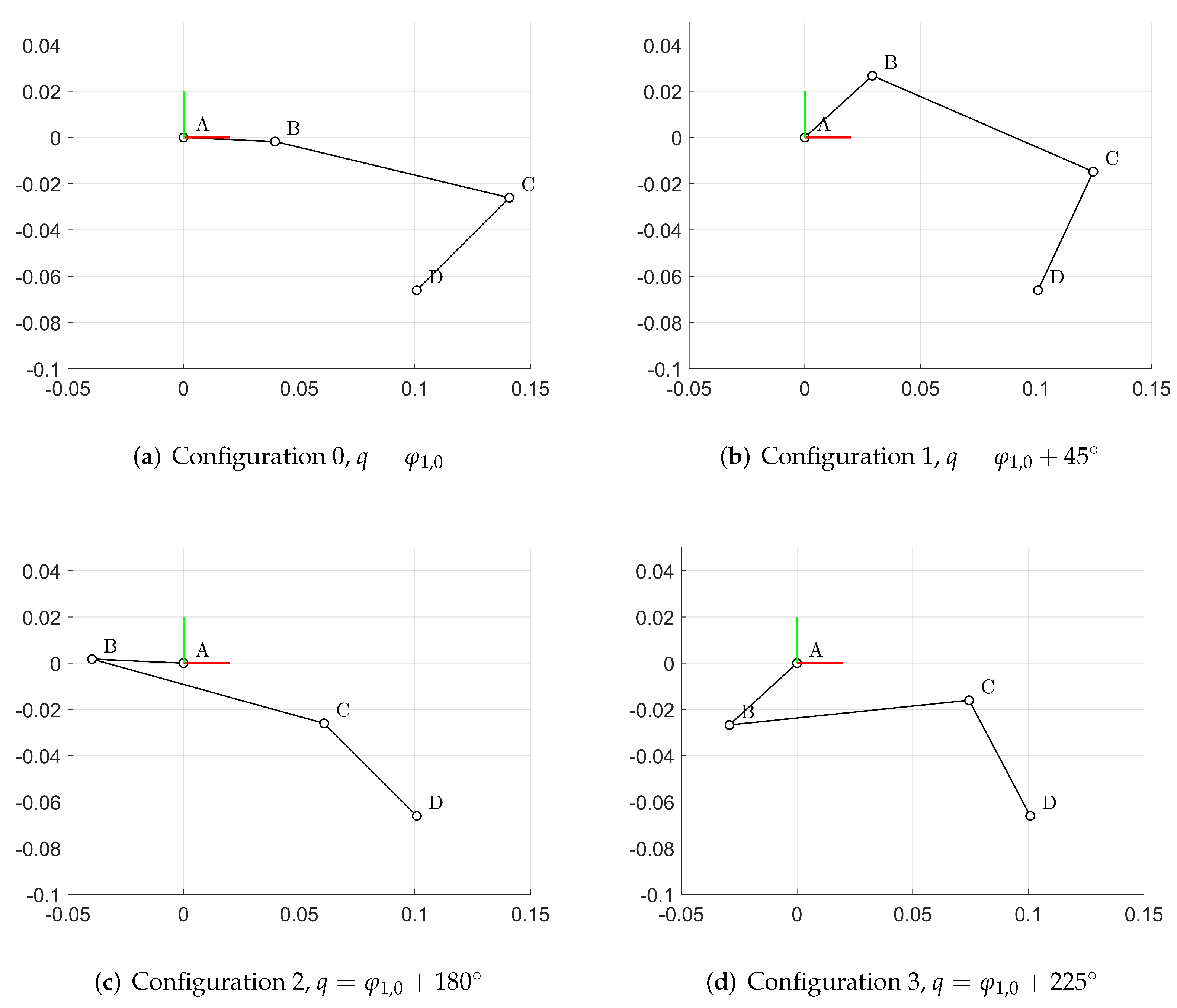
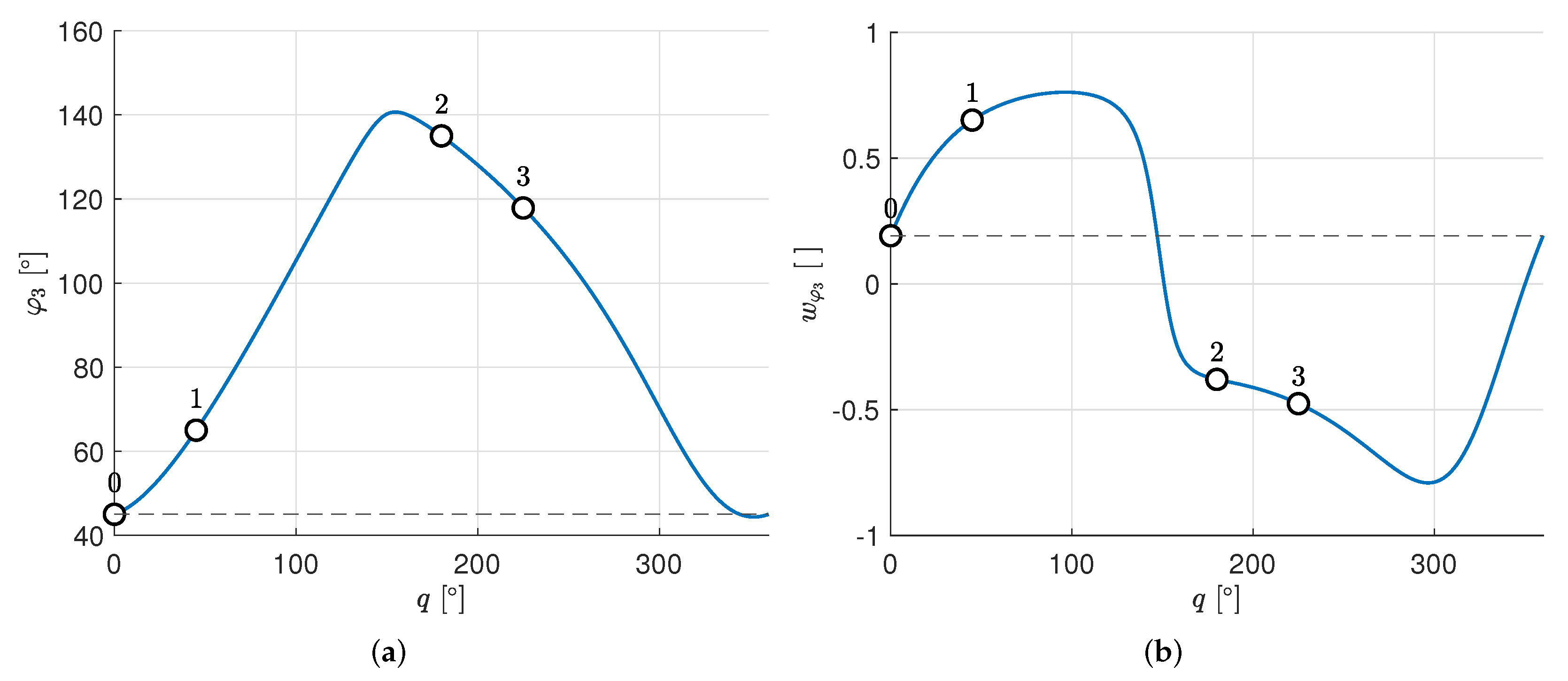
3.1. Mechanism Synthesis
- The angular top opening range is arbitrary but should be wide enough to allow multiple pieces to fall within the device without falling outside of the cylinder. Moreover, it is mandatory that . Indeed, if the top opening range is wider than the blade stroke, the two compartments will always have an open top gap which could allow components to pass through. As a result, the first configuration has been chosen so that . Since configuration 0 must be reached once every crank full rotation, this first displacement, which represents half of the full movement of the rocker, must be performed in half rotation; thus, .
- The other two displacements are chosen so that the rocker moves from the dead points by a certain amount with the same crank displacement. In other words, , and .
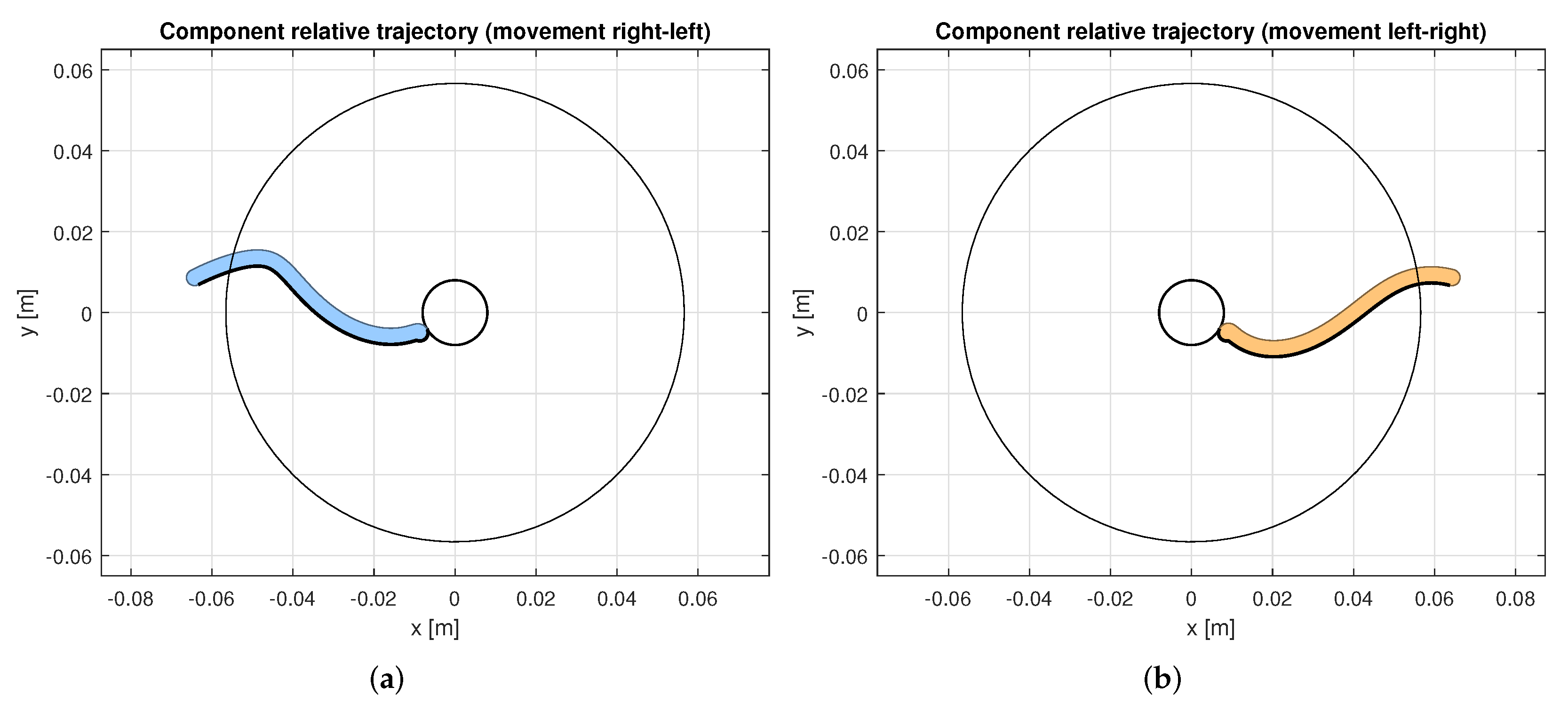
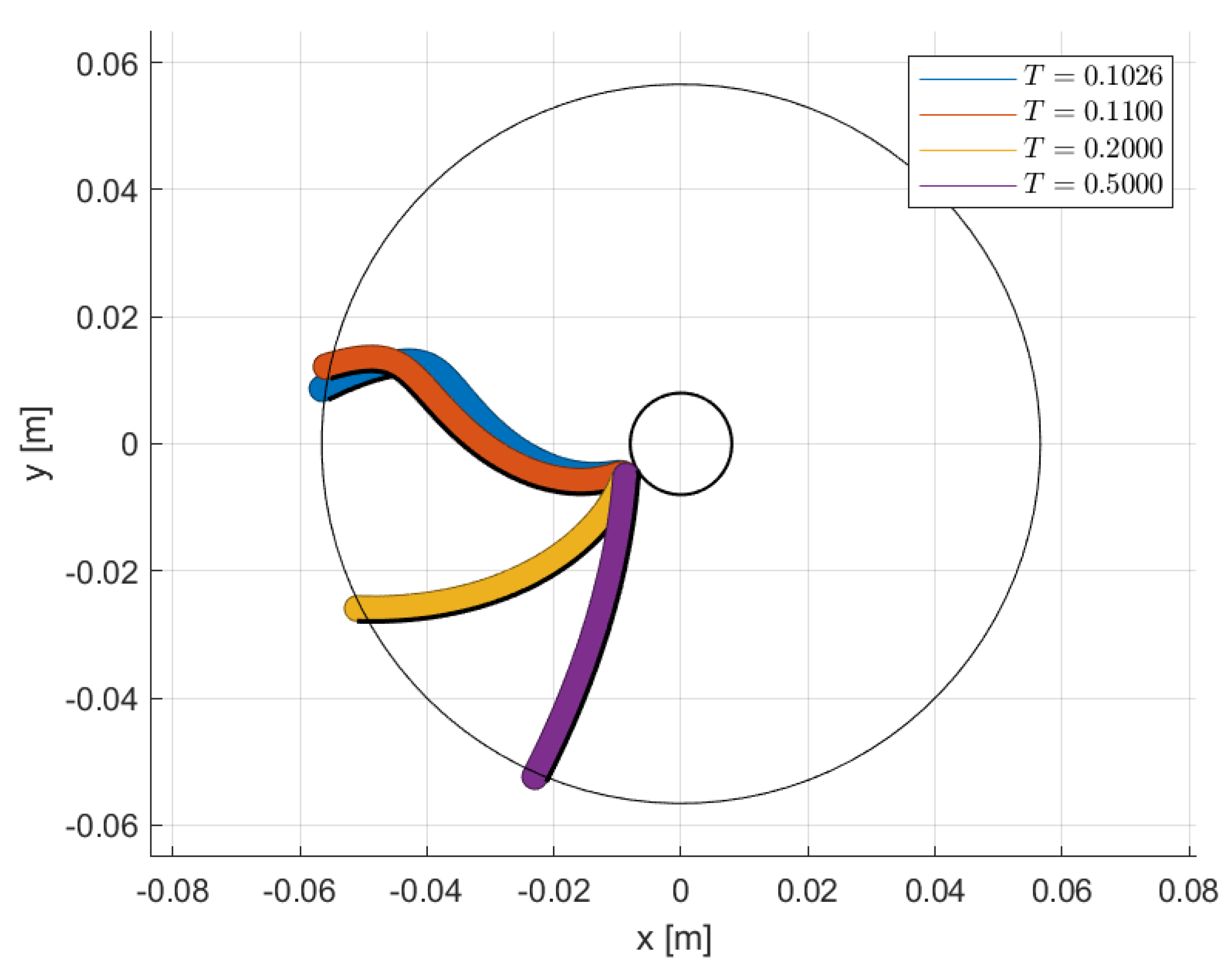
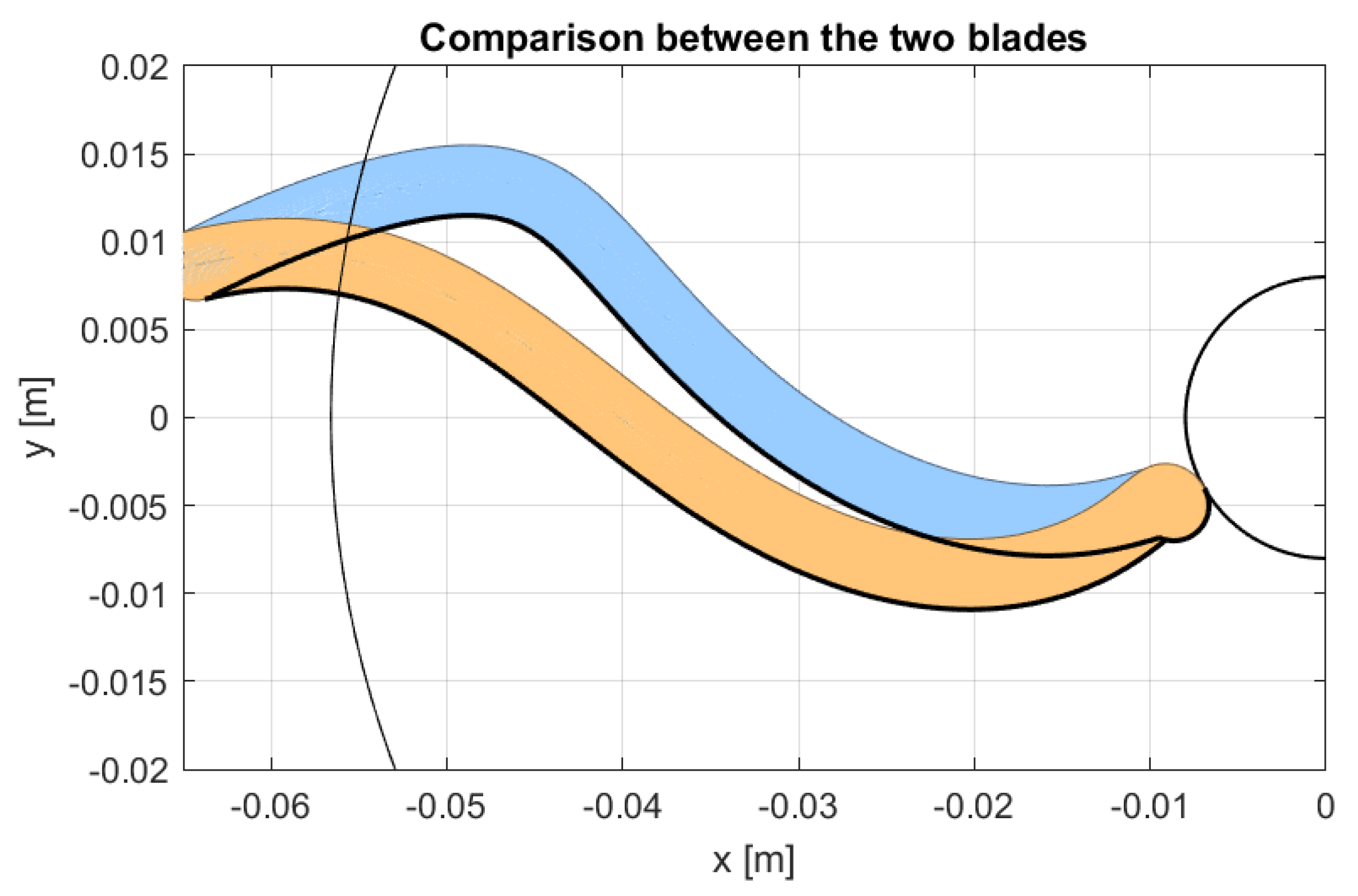
3.2. Blade Design
- for the movement from left to right: for ;
- for the movement from right to left: for .
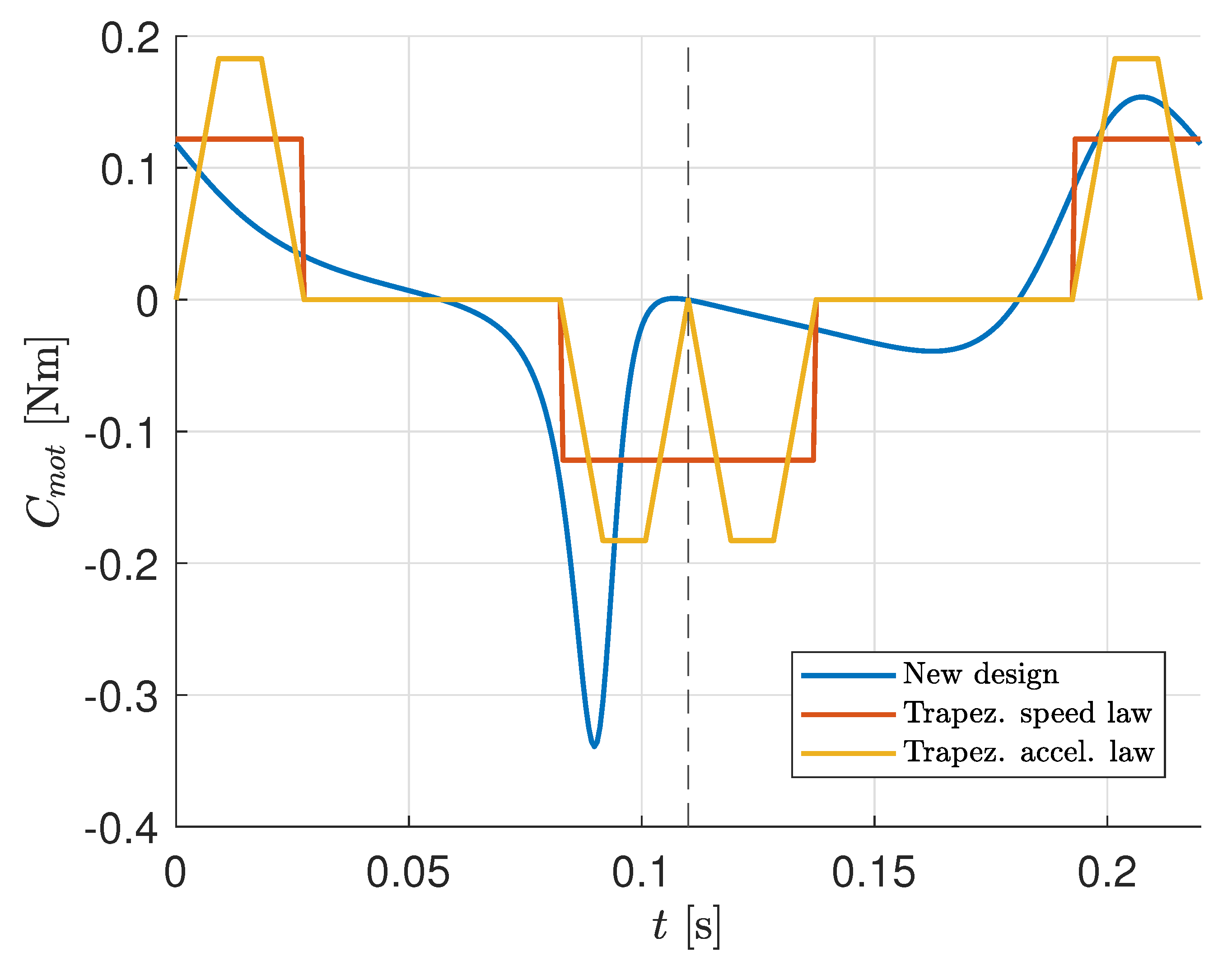
3.3. Motor Torques
4. Experimental Results
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Heilala, J.; Montonen, J.; Väätäinen, O. Life cycle and unit-cost analysis for modular reconfigurable flexible light assembly systems. Proc. Inst. Mech. Eng. Part B J. Eng. Manuf. 2008, 222, 1289–1299. [Google Scholar] [CrossRef]
- Faccio, M.; Bottin, M.; Rosati, G. Collaborative and traditional robotic assembly: A comparison model. Int. J. Adv. Manuf. Technol. 2019, 102, 1355–1372. [Google Scholar] [CrossRef]
- Limère, V.; Landeghem, H.V.; Goetschalckx, M.; Aghezzaf, E.; McGinnis, L.F. Optimising part feeding in the automotive assembly industry: Deciding between kitting and line stocking. Int. J. Prod. Res. 2012, 50, 4046–4060. [Google Scholar] [CrossRef]
- Höhner, D.; Wirtz, S.; Scherer, V. A numerical study on the influence of particle shape on hopper discharge within the polyhedral and multi-sphere discrete element method. Powder Technol. 2012, 226, 16–28. [Google Scholar] [CrossRef]
- Huang, X.; Zheng, Q.; Yu, A.; Yan, W. Optimised curved hoppers with maximum mass discharge rate—An experimental study. Powder Technol. 2021, 377, 350–360. [Google Scholar] [CrossRef]
- Cox, G.; McCue, S.; Thamwattana, N.; Hill, J. Perturbation solutions for flow through symmetrical hoppers with inserts and asymmetrical wedge hoppers. J. Eng. Math. 2005, 52, 63–91. [Google Scholar] [CrossRef]
- Liu, H.; Jia, F.; Xiao, Y.; Han, Y.; Li, G.; Li, A.; Bai, S. Numerical analysis of the effect of the contraction rate of the curved hopper on flow characteristics of the silo discharge. Powder Technol. 2019, 356, 858–870. [Google Scholar] [CrossRef]
- Huang, X.; Zheng, Q.; Liu, D.; Yu, A.; Yan, W. A design method of hopper shape optimization with improved mass flow pattern and reduced particle segregation. Chem. Eng. Sci. 2022, 253, 117579. [Google Scholar] [CrossRef]
- Ketterhagen, W.R.; Hancock, B.C. Optimizing the design of eccentric feed hoppers for tablet presses using DEM. Comput. Chem. Eng. 2010, 34, 1072–1081. [Google Scholar] [CrossRef]
- Timoleanov, K.; Savenkov, D.; Gorgadze, L. Features of designing feeding hoppers of loose materials of low productivity in agriculture. In Proceedings of the International Conference on Modern Trends in Manufacturing Technologies and Equipment (ICMTMTE 2018), Sevastopol, Russia, 10–14 September 2018; Volume 224. [Google Scholar] [CrossRef][Green Version]
- Comand, N.; Minto, R.; Boschetti, G.; Faccio, M.; Rosati, G. Optimization of a Kitting Line: A Case Study. Robotics 2019, 8, 70. [Google Scholar] [CrossRef]
- Roshanbin, A.; Tirmizi, A.; Raeymaekers, S.; Verhees, D.; Afzal, M.R. Towards Easing Automation of Robotic Kitting Operations. In Proceedings of the 2022 IEEE 17th International Conference on Control & Automation (ICCA), Naples, Italy, 27–30 June 2022; Volume 2022, pp. 424–431. [Google Scholar] [CrossRef]
- Fager, P.; Hanson, R.; Fasth-Berglund, A. Dual Robot Kit preparation in batch preparation of component kits for mixed model assembly. IFAC PapersOnLine 2020, 53, 10627–10632. [Google Scholar] [CrossRef]
- Pantyukhina, E.; Preis, V.; Khachaturian, A. Feed rate evaluation of mechanical toothed hopper-feeding device with ring orientator for parts, asymmetric at the ends. IFAC PapersOnLine 2019, 53, 1260. [Google Scholar] [CrossRef]
- Gao, X.; Xie, G.; Xu, Y.; Yu, Y.; Lai, Q. Application of a staggered symmetrical spiral groove wheel on a quantitative feeding device and investigation of particle motion characteristics based on DEM. Powder Technol. 2022, 407, 117650. [Google Scholar] [CrossRef]
- Comand, N.; Bottin, M.; Rosati, G. Improving Components Feeding: A Rotatory Device. Mech. Mach. Sci. 2021, 91, 468–475. [Google Scholar] [CrossRef]
- Comand, N.; Bottin, M.; Rosati, G.; Agrawal, S. A dynamic model for the optimization of rotatory feeding devices. Mech. Mach. Theory 2021, 166, 104479. [Google Scholar] [CrossRef]
- Rosati, G.; Cenci, S.; Boschetti, G.; Zanotto, D.; Masiero, S. Design of a single-dof active hand orthosis for neurorehabilitation. In Proceedings of the 2009 IEEE International Conference on Rehabilitation Robotics, Kyoto, Japan, 23–26 June 2009; pp. 161–166. [Google Scholar] [CrossRef]
- Jianwei, S.; Jinkui, C.; Baoyu, S. A unified model of harmonic characteristic parameter method for dimensional synthesis of linkage mechanism. Appl. Math. Model. 2012, 36, 6001–6010. [Google Scholar] [CrossRef]
- Cabrera, J.; Simon, A.; Prado, M. Optimal synthesis of mechanisms with genetic algorithms. Mech. Mach. Theory 2002, 37, 1165–1177. [Google Scholar] [CrossRef]
- Acharyya, S.; Mandal, M. Performance of EAs for four-bar linkage synthesis. Mech. Mach. Theory 2009, 44, 1784–1794. [Google Scholar] [CrossRef]
- Huang, Q.; Yu, Y.; Zhang, K.; Li, S.; Lu, H.; Li, J.; Zhang, A.; Mei, T. Optimal synthesis of mechanisms using repellency evolutionary algorithm. Knowl.-Based Syst. 2022, 239, 107928. [Google Scholar] [CrossRef]


| N | Unknowns | Equations | Solutions |
|---|---|---|---|
| 1 | 7 (,,,,,,) | 2 | |
| 2 | 8 (,,,,,,,) | 4 | |
| 3 | 9 (,,,,,,,,) | 6 | |
| 4 | 10 (,,,,,,,,,) | 8 | |
| 5 | 11 (,,,,,,,,,,) | 10 | |
| 6 | 12 (,,,,,,,,,,,) | 12 | 1 |
| Parameter | Value | Unit | Unknown | Value | Unit |
|---|---|---|---|---|---|
| 180 | [] | 20 | [] | ||
| 45 | [] | [mm] | |||
| 225 | [] | 45 | [] | ||
| 90 | [] | ||||
| 20 | [] | ||||
| 70 | [] |
| Parameter | Value | Unit | Parameter | Value | Unit |
|---|---|---|---|---|---|
| [mm] | [] | ||||
| [mm] | [] | ||||
| [mm] | [] |
| Parameter | Left Blade | Right Blade |
|---|---|---|
| [mm] | ||
| [mm] | ||
| r [mm] | 4 | 4 |
| T [s] |
| Components | s | s | s |
|---|---|---|---|
| 1 | ✓ | ✓ | ✓ |
| 2 | ≈ | ✓ | ✓ |
| 6 | × | ≈ | ✓ |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bottin, M.; Minto, R.; Rosati, G. Design of the Drive Mechanism of a Rotating Feeding Device. Machines 2022, 10, 1160. https://doi.org/10.3390/machines10121160
Bottin M, Minto R, Rosati G. Design of the Drive Mechanism of a Rotating Feeding Device. Machines. 2022; 10(12):1160. https://doi.org/10.3390/machines10121160
Chicago/Turabian StyleBottin, Matteo, Riccardo Minto, and Giulio Rosati. 2022. "Design of the Drive Mechanism of a Rotating Feeding Device" Machines 10, no. 12: 1160. https://doi.org/10.3390/machines10121160
APA StyleBottin, M., Minto, R., & Rosati, G. (2022). Design of the Drive Mechanism of a Rotating Feeding Device. Machines, 10(12), 1160. https://doi.org/10.3390/machines10121160








