A Study on the Cavitation and Pressure Pulsation Characteristics in the Impeller of an LNG Submerged Pump
Abstract
:1. Introduction
2. Simulation Model
2.1. Computational Model
2.2. Meshing
2.3. Grid Independence Verification
2.4. Boundary Conditions and Turbulence Model
3. External Characteristic Test
4. Analysis of Numerical Results
4.1. External Characteristic Test Verification
4.2. Cavitation Characteristic Curve
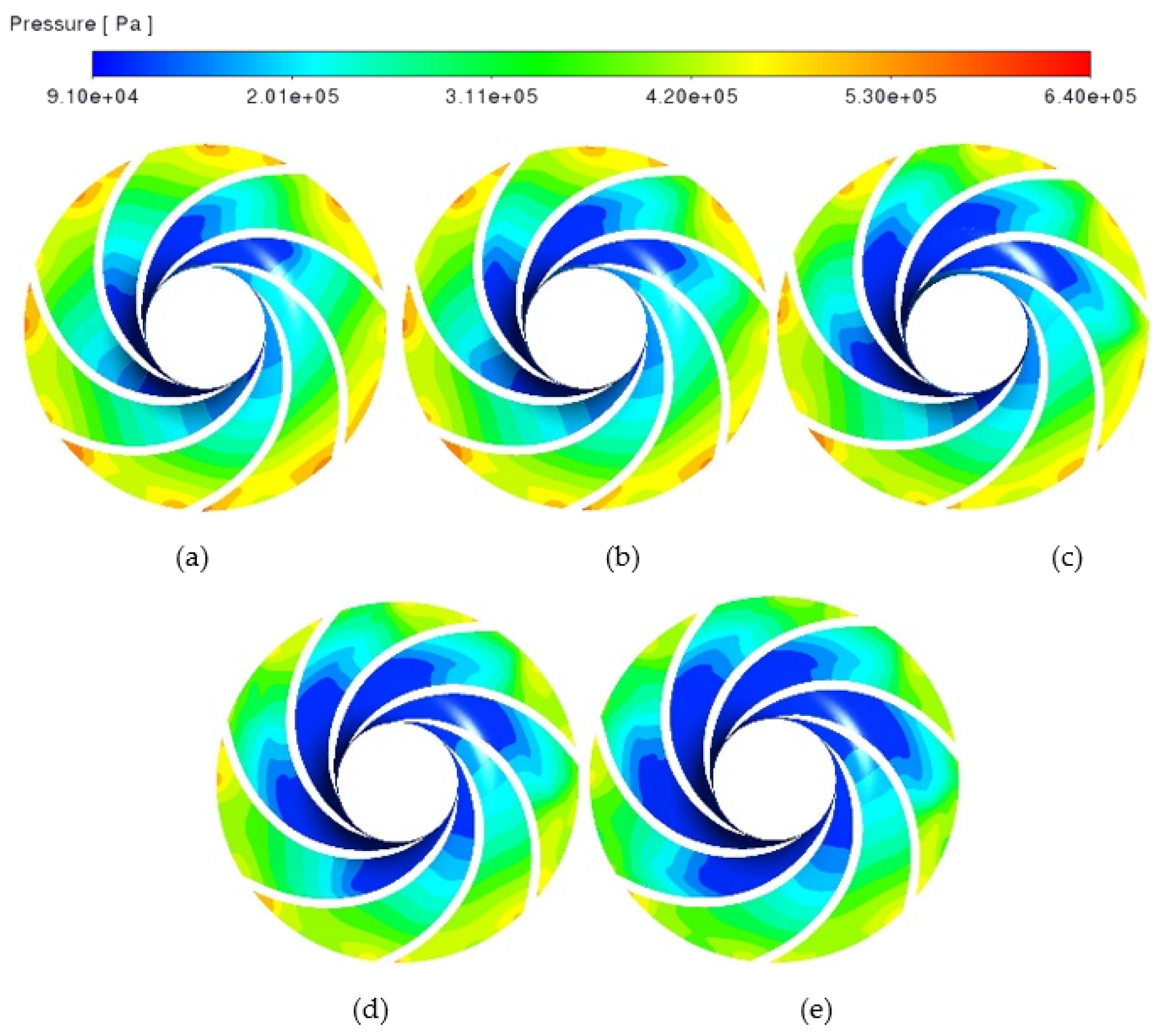
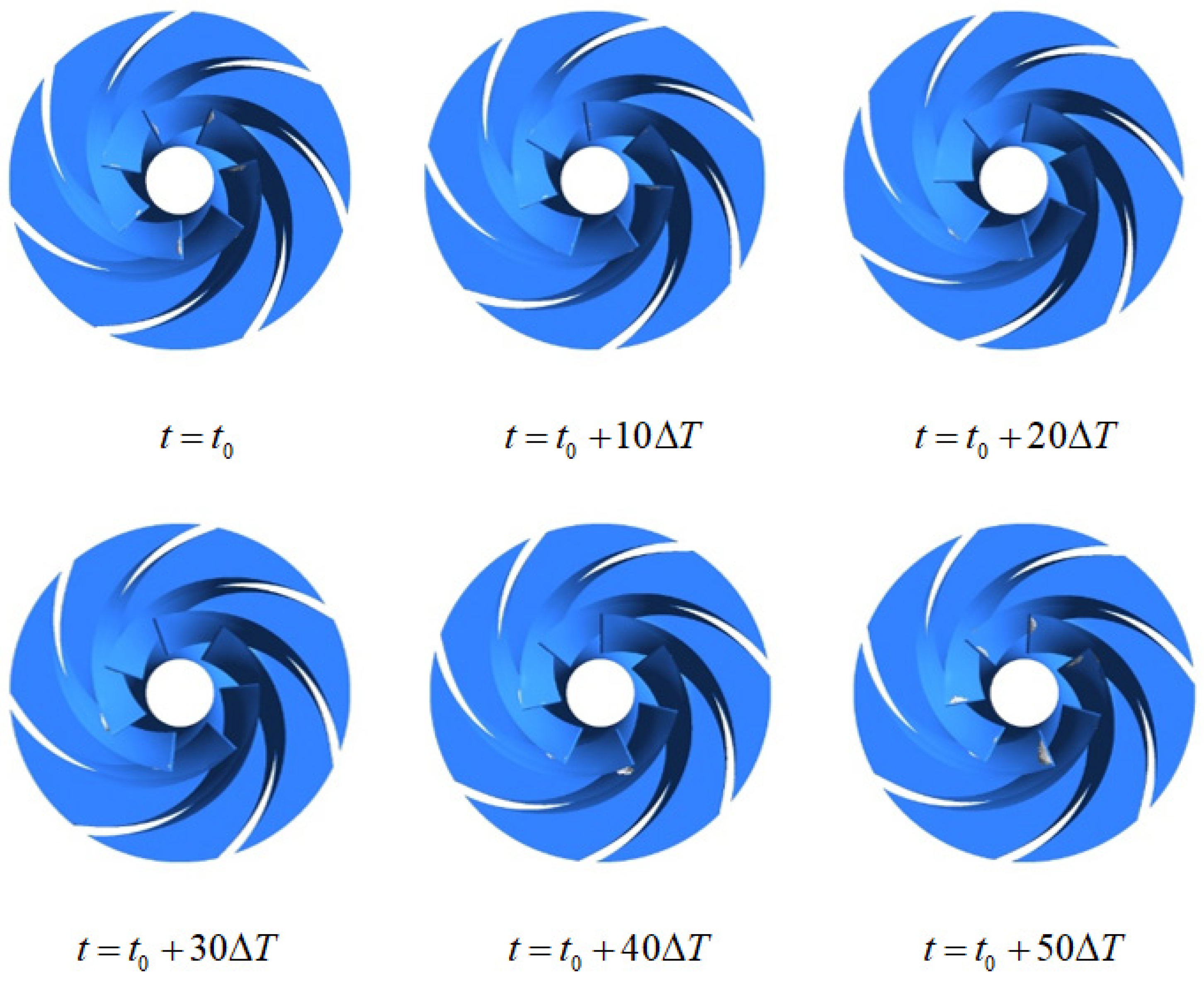
4.3. Analysis of the Cavitation in the Impeller
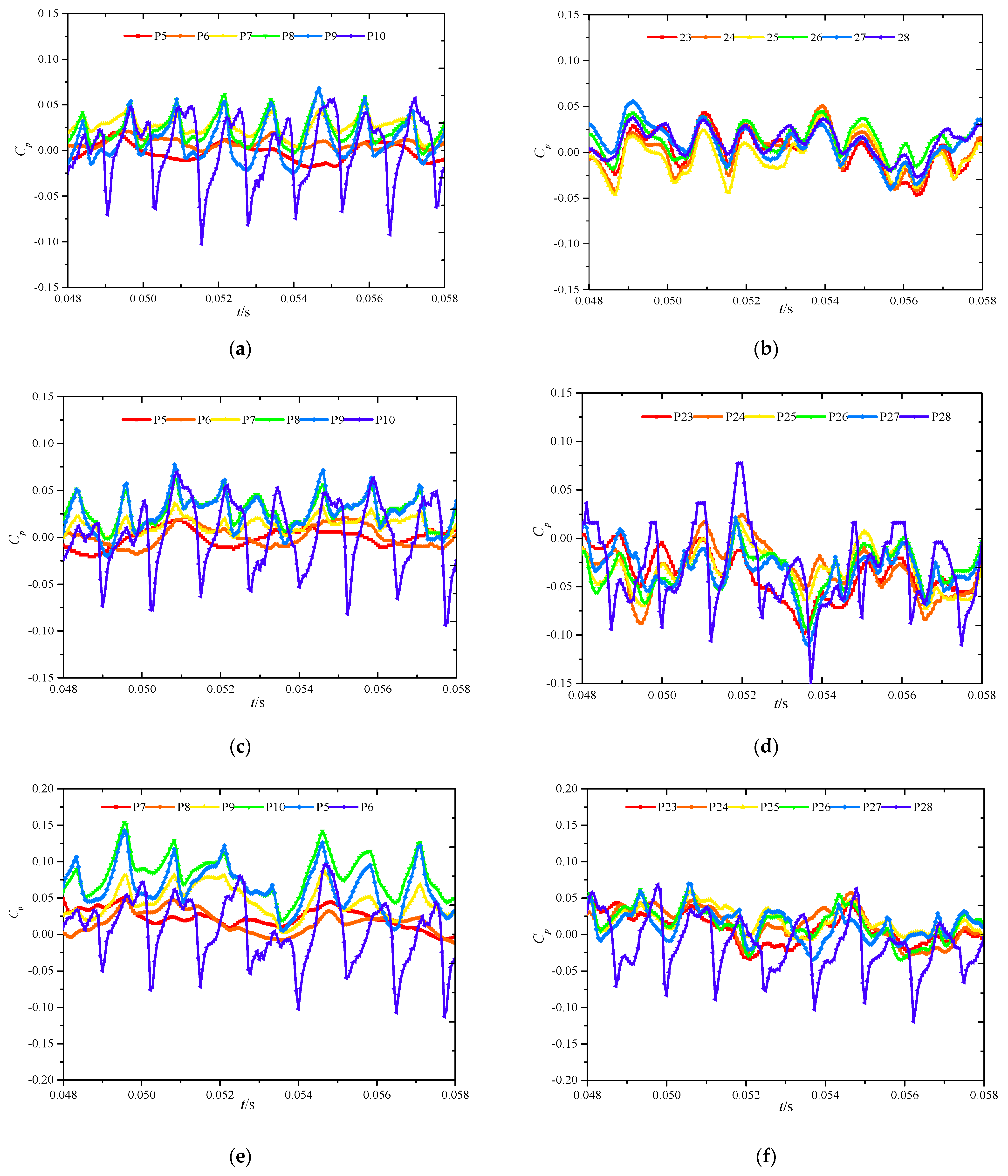
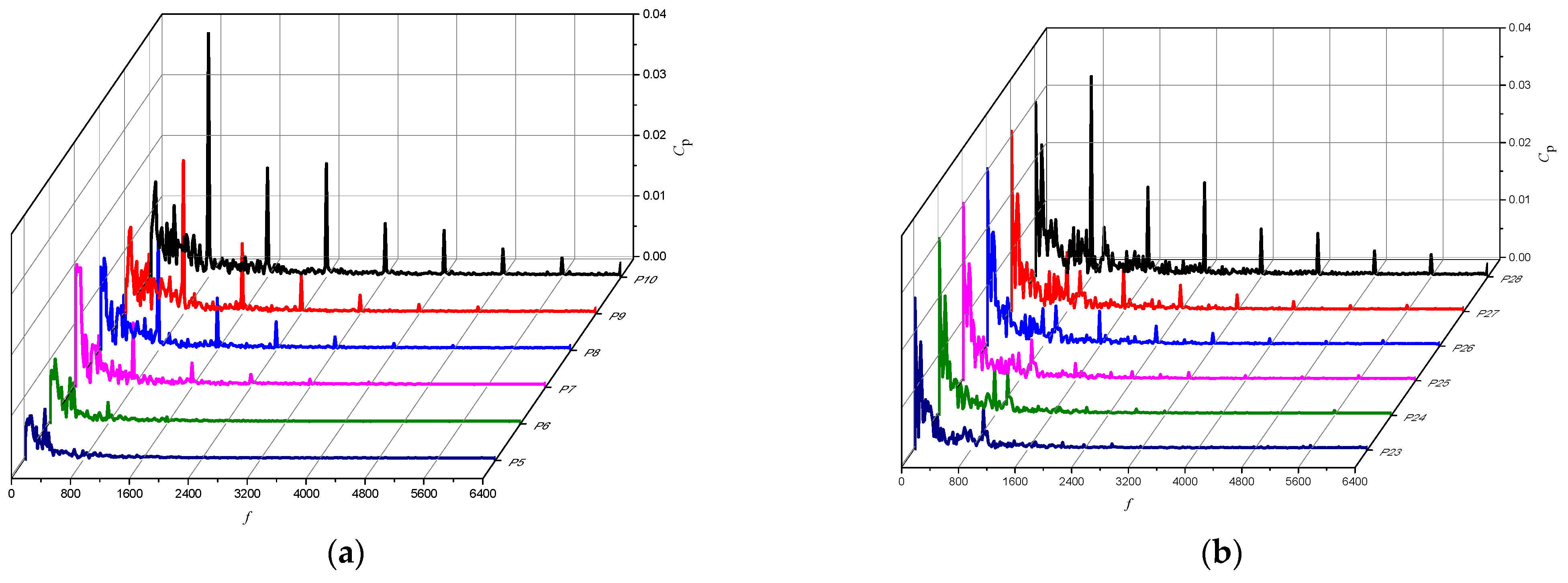
4.4. Analysis of the Pressure Fluctuation Characteristics in the Impeller
4.4.1. Pressure Pulsation under Non-Cavitation Conditions
- (1)
- Time Domain analysis
- (2)
- Frequency Domain Analysis
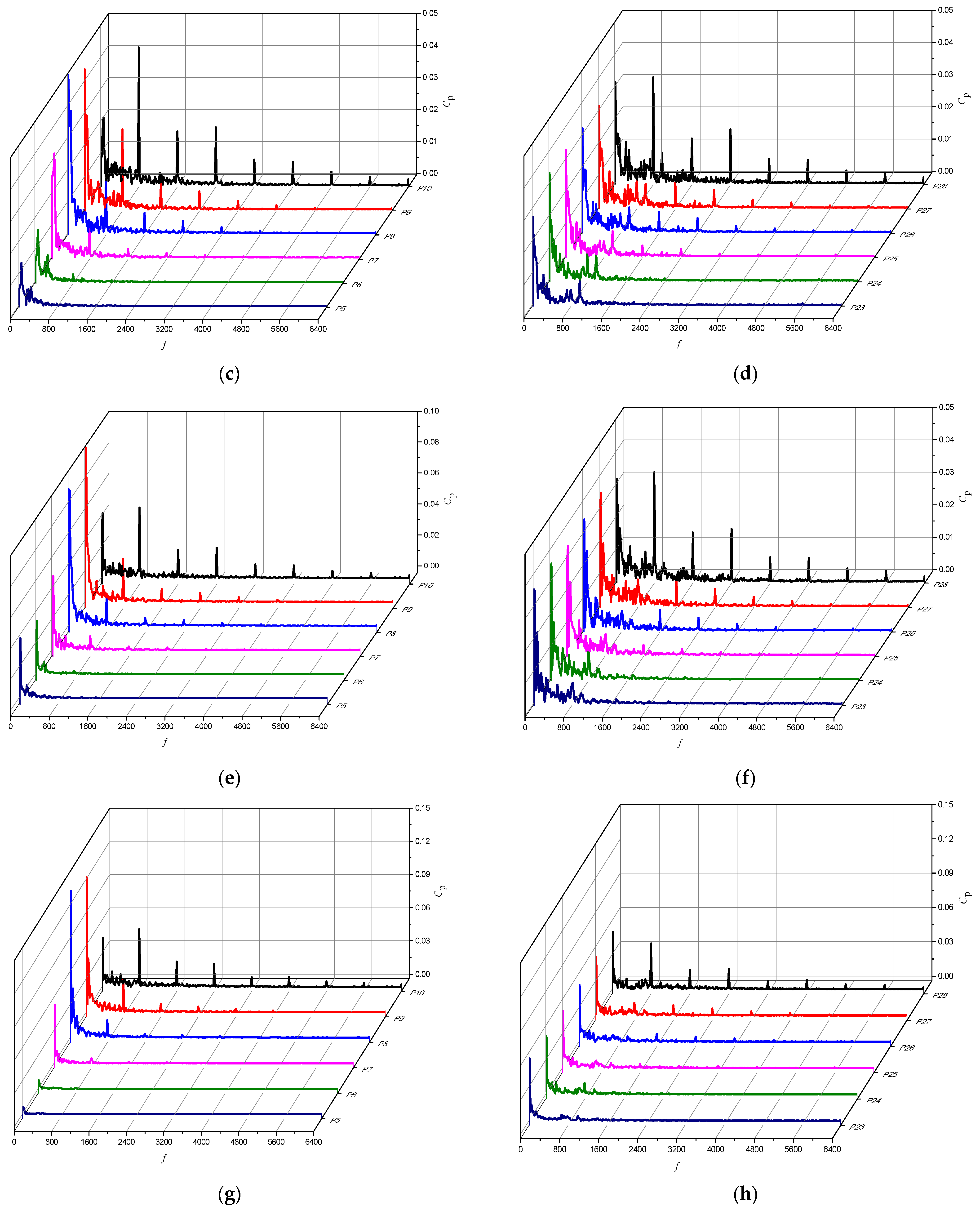
4.4.2. Pressure Pulsation under Cavitation Condition
- (1)
- Time Domain Analysis
- (2)
- Frequency Domain Analysis
5. Conclusions
- (1)
- The cavitation in the impeller is first generated near the inlet edge at the back of the blade. With the decrease in NPSHa, the cavitation diffuses along the back of the blade to the outlet, and with the increase in impeller channel area, the bubble gradually expands to the blade working surface. Due to the dynamic and static interference between the impeller and guide vane, the distribution of cavitation in each impeller channel is asymmetric.
- (2)
- The pressure pulsation in the impeller of the LNG submerged pump is greatly affected by the flow. With the increase in flow, the pressure pulsation in the impeller becomes periodically stronger, the amplitude decreases, and the low-frequency and broadband signals gradually disappear. The pulsation source of the internal pressure of the impeller is in the inlet edge of the positive guide vane. The amplitude of the internal pressure pulsation of the impeller is the largest at the dynamic and static interface, and the pulsation amplitude decreases gradually with the distance away from the pulsation source.
- (3)
- The time domain and frequency domain characteristics of pressure pulsation in the primary impeller and secondary impeller are the same. The channel pressure does not affect the amplitude of pressure pulsation. Due to the uneven inflow of the primary guide vane, the low-frequency component of the pressure fluctuation spectrum in the secondary impeller is greater than that in the primary impeller.
- (4)
- The occurrence of cavitation greatly influences the frequency domain distribution of pressure pulsation in the impeller of the LNG submerged pump. With the decrease in NPSHa, a low-frequency signal appears in the frequency domain of the pressure pulsation in the impeller, and the amplitude of the low-frequency signal continuously increases. The influence of cavitation on the pressure pulsation in the primary impeller is greater than that in the secondary impeller. When critical cavitation occurs in the pump, the amplitude of the low-frequency signal in the frequency domain of the pressure pulsation in the primary impeller increases and exceeds the blade frequency, becoming the main frequency.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Guan, X.F. Modern Pump Theory and Design; China Aerospace Publishing House: Beijing, China, 2011. [Google Scholar]
- Gu, A. LNG Technical Manual; Machinery Industry Press: Beijing, China, 2010. [Google Scholar]
- Lindsay, R.B. On the pressure developed in a liquid during the collapse of a spherical cavity. In Men of Physics Lord Rayleigh—The Man & His Work; Elsevier: Amsterdam, The Netherlands, 1970; pp. 221–226. [Google Scholar]
- Plesset, M.S.; Devine, R.E. Effect of exposure time on cavitation damage. ASME J. Basic Eng. Trans. 1965, 88, 31. [Google Scholar] [CrossRef]
- Plesset, M.S.; Prosperetti, A. Bubble dynamics and cavitation. Annu. Rev. Fluid Mech. 1977, 9, 145–185. [Google Scholar] [CrossRef]
- Huang, B.; Wang, G.; Zhang, B.; Yu, Z. Evaluation of cavitation model for numerical simulation of cloud cavitation flow. J. Beijing Inst. Technol. 2009, 29, 785–789. [Google Scholar]
- Avdeev, A.A. Laws of vapor bubble growth in the superhearted liquid volume (Thermal growth scheme). High Temp. 2014, 52, 588–602. [Google Scholar] [CrossRef]
- Zhang, Y.; Luo, X.; Xu, H.; Wu, Y. Improvement of thermodynamic cavitation model and its numerical application. J. Eng. Thermophys. 2010, 31, 1671–1674. [Google Scholar]
- Ji, B.; Luo, X.; Wu, Y.; Zhang, Y.; Xu, H. Simulation of high temperature water cavitation considering thermodynamic effect. J. Tsinghua Univ. 2010, 50, 262–265. [Google Scholar]
- Wang, W.; Lin, Y.; Wang, X.; Wang, Y. Influence of thermodynamic effect on cavitation at high temperature. J. Drain. Irrig. Mech. Eng. 2014, 32, 835–839. [Google Scholar]
- Shi, S.; Wang, G.; Hu, C.; Gao, D. Experimental study on hydrodynamic pulsation characteristics of cavitation in water at different temperatures. J. Mech. Eng. 2014, 8, 174–181. [Google Scholar] [CrossRef]
- Shi, S.; Wang, G.; Ma, R. Numerical study on cavitation characteristics of low temperature fluid. Eng. Mech. 2012, 5, 61–67. [Google Scholar]
- Shi, S.; Wang, G.; Chen, G. Application evaluation of mass transfer model in cavitation flow calculation considering thermodynamic effects. J. Appl. Mech. 2011, 6, 589–594+672. [Google Scholar]
- Li, J.; Liu, L.; Li, G.; Feng, Z. Numerical prediction of cavitation flow in centrifugal pump impeller. J. Eng. Thermophys. 2007, 28, 948–950. [Google Scholar]
- Wang, Y.; Liu, H.; Liu, D.; Yuan, S.; Wang, J.; Jiang, L. Application of the two-phase three-component computational model to predict cavitating flow in a centrifugal pump and its validation. Comput. Fluids 2016, 131, 142–150. [Google Scholar] [CrossRef]
- Rakibuzzaman, M.; Kim, K.; Suh, S.H. Numerical and experimental investigation of cavitation flows in a multistage centrifugal pump. J. Mech. Sci. Technol. 2018, 32, 1071–1078. [Google Scholar] [CrossRef]
- Wang, J. Numerical Calculation and Experimental Study on Cavitation Erosion of Hydraulic Equipment. Master’s Thesis, Jiangsu University, Zhenjiang, China, 2015. [Google Scholar]
- Jiang, H. Research on assembly height detection method of engine cooling water pump impeller. Intern. Combust. Engine Parts 2018, 8, 40–42. [Google Scholar]
- Liao, N.; Xie, D. Discussion on cavitation of water pump of lj465q series engine. Equip. Manuf. Technol. 2010, 2, 177–179. [Google Scholar]
- Shen, D. CFD Numerical Simulation of Engine Cooling Water Pump. Master’s Thesis, Jilin University, Changchun, China, 2014. [Google Scholar]
- Li, W.; Shi, W.; Pei, B.; Zhang, H.; Lu, W. Numerical simulation and improvement of cavitation characteristics of engine cooling water pump. Trans. Csice 2013, 2, 165–170. [Google Scholar]
- Li, W.; Shi, W.; Zhang, H.; Pei, B.; Lu, W. Prediction of cavitation performance of engine cooling water pump based on CFD. J. Drain. Irrig. Mach. Eng. 2012, 30, 176–180. [Google Scholar]
- Hou, H.; Zhang, Y.; Li, Z.; Jiang, T.; Zhang, J.; Xu, C. Numerical analysis of entropy production on a LNG cryogenic submerged pump. J. Nat. Gas Sci. Eng. 2016, 36, 87–96. [Google Scholar] [CrossRef]
- Cao, H. Numerical Study on Cavitation Flow Characteristics at Low Temperature. Master’s Thesis, Harbin Institute of Technology, Harbin, China, 2010. [Google Scholar]
- Ma, X.; Wei, Y.; Wang, C.; Huang, W. Numerical simulation of low temperature cavitation flow of liquid nitrogen around hydrofoil. J. Ship Mech. 2012, 12, 1345–1352. [Google Scholar]
- Hord, J. Cavitation in Liquid Cryogenics III—Ogive; Technical Report No. NASA-CR-2242; NASA: Washington, DC, USA, 1973; pp. 10–60. [Google Scholar]
- Franc, J.P.; Rebattet, C.; Coulon, A. An experimental investigation of thermal effects in a cavitating inducer. J. Fluids Eng. 2004, 126, 716–723. [Google Scholar] [CrossRef]
- Franc, J.P.; Pellone, C. Analysis of thermal effects in a cavitating inducer using rayleigh equation. J. Fluids Eng. 2007, 129, 974–983. [Google Scholar] [CrossRef]
- Gustavsson, J.P.R.; Denning, K.C.; Segal, C. Hydrofoil cavitation under strong thermodynamic effect. J. Fluids Eng. 2008, 130, 091303. [Google Scholar] [CrossRef]
- Guo, S.; Maruta, Y. Experimental investigations on pressure fluctuations and vibration of the impeller in a centrifugal pump with vaned diffusers. JSME Int. J. 2005, 48, 136–143. [Google Scholar] [CrossRef] [Green Version]
- Fernández, J.; Marcos, A.; Barrio, R.; Blanco, E.; Parrondo, J. Studies of the flow of air in a mixed-flow pump using numerical simulations. Proc. Inst. Mech. Eng. Part A J. Power Energy 2011, 225, 647–654. [Google Scholar] [CrossRef]
- Yuan, J.; Fu, Y.; Liu, Y.; Zhang, J.; Pei, J. Analysis of pressure fluctuation characteristics in volute of centrifugal pump based on large eddy simulation. J. Drain. Irrig. Mech. Eng. 2010, 28, 310–314. [Google Scholar]
- Wang, F.; Zhang, L.; Zhang, Z. Study on pressure fluctuation characteristics of unsteady flow field in axial flow pump. J. Hydraul. Eng. 2007, 38, 1003–1009. [Google Scholar]
- Ni, Y.; Pan, Z.; Li, H.; Yuan, J. Application of outlet pressure fluctuation characteristics in cavitation monitoring of centrifugal pump. J. Drain. Irrig. Mech. Eng. 2006, 24, 40–43. [Google Scholar]
- Zhang, N. Study on Excitation Characteristics of Unsteady Flow in Centrifugal Pump. Master’s Thesis, Jiangsu University, Zhenjiang, China, 2016. [Google Scholar]
- Cheng, H.Y.; Ji, B.; Long, X.P.; Huai, W.; Farhat, M. A review of cavitation in tip-leakage flow and its control. J. Hydrodyn. 2021, 33, 226–242. [Google Scholar] [CrossRef]
- Deng, Z.; Jiang, J.; Liu, X. Analysis of unsteady cavitation in the internal flow field of a centrifugal pump. Water Resour. Power 2021, 39, 166–170. [Google Scholar]
- Li, W.; Ji, L.; Li, E.; Shi, W.; Agarwal, R.; Zhou, L. Numerical investigation of energy loss mechanism of mixed-flow pump under stall condition. Renew. Energy 2020, 167, 740–760. [Google Scholar] [CrossRef]
- Ji, L.; Li, W.; Shi, W.; Chang, H.; Yang, Z. Energy characteristics of mixed-flow pump under different tip clearances based on entropy production analysis. Energy 2020, 199, 1–15. [Google Scholar] [CrossRef]
- Feng, J.; Tang, X.; Wang, W.; Ying, R.; Zhang, T. Verification method of numerical simulation reliability based on grid independence and time independence. J. Shihezi Univ. 2017, 35, 52–56. [Google Scholar]
- Li, W.; Li, E.; Ji, L.; Zhou, L.; Shi, W.; Zhu, Y. Mechanism and propagation characteristics of rotating stall in a mixed-flow pump. Renew. Energy 2020, 153, 74–92. [Google Scholar] [CrossRef]
- Ji, L.; Li, W.; Shi, W.; Tian, F.; Agarwal, R. Effect of blade thickness on rotating stall of mixed-flow pump using entropy generation analysis. Energy 2021, 236, 121–381. [Google Scholar] [CrossRef]
- Kang, S.; Shi, L.; Dai, L.; Fan, F. Error analysis and grid convergence of CFD simulation. J. Eng. Thermophys. 2010, 31, 2009–2013. [Google Scholar]
- Wang, H.; Hu, Q.; Yang, Y.; Wang, C. Performance differences of electrical submersible pump under variable speed schemes. Int. J. Simul. Model. 2021, 20, 76–86. [Google Scholar] [CrossRef]
- Zhou, J.; Zhao, M.; Wang, C.; Gao, Z. Optimal design of diversion piers of lateral intake pumping station based on orthogonal test. Shock. Vib. 2021, 2021, 6616456. [Google Scholar] [CrossRef]
- Zhang, L.; Wang, C.; Zhang, Y.; Xiang, W.; He, Z.; Shi, W. Numerical study of coupled flow in blocking pulsed jet impinging on a rotating wall. J. Braz. Soc. Mech. Sci. Eng. 2021, 43, 508. [Google Scholar] [CrossRef]
- Shi, L.; Zhu, J.; Tang, F.; Wang, C. Multi-Disciplinary optimization design of axial-flow pump impellers based on the approximation model. Energies 2020, 13, 779. [Google Scholar] [CrossRef] [Green Version]
- Wang, H.; Long, B.; Wang, C.; Han, C.; Li, L. Effects of the impeller blade with a slot structure on the centrifugal pump performance. Energies 2020, 13, 1628. [Google Scholar] [CrossRef]
- Zhu, Y.; Li, G.; Wang, R.; Tang, S.; Su, H.; Cao, K. Intelligent fault diagnosis of hydraulic piston pump combining improved lenet-5 and pso hyperparameter optimization. Appl. Acoust. 2021, 183, 108336. [Google Scholar] [CrossRef]
- Li, W. Study on Cavitation Mechanism of Engine Cooling Water Pump under Thermodynamic Effect. Master’s Thesis, Jiangsu University, Zhenjiang, China, 2016. [Google Scholar]
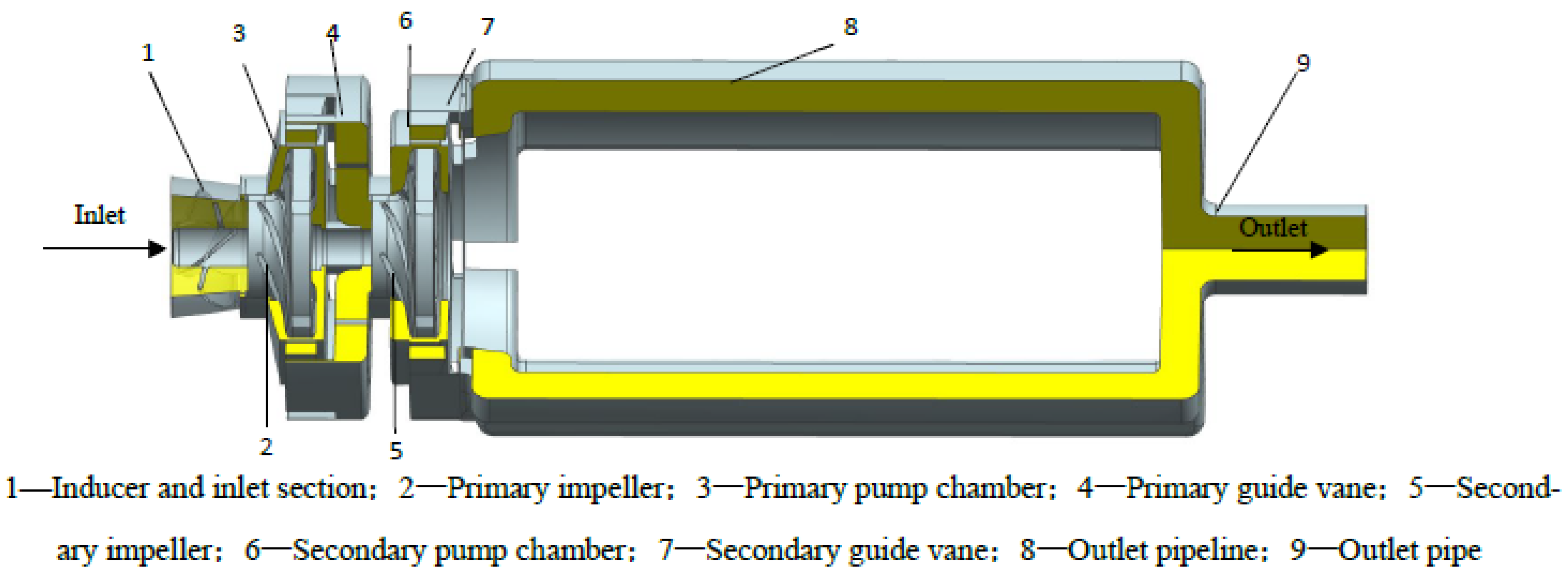


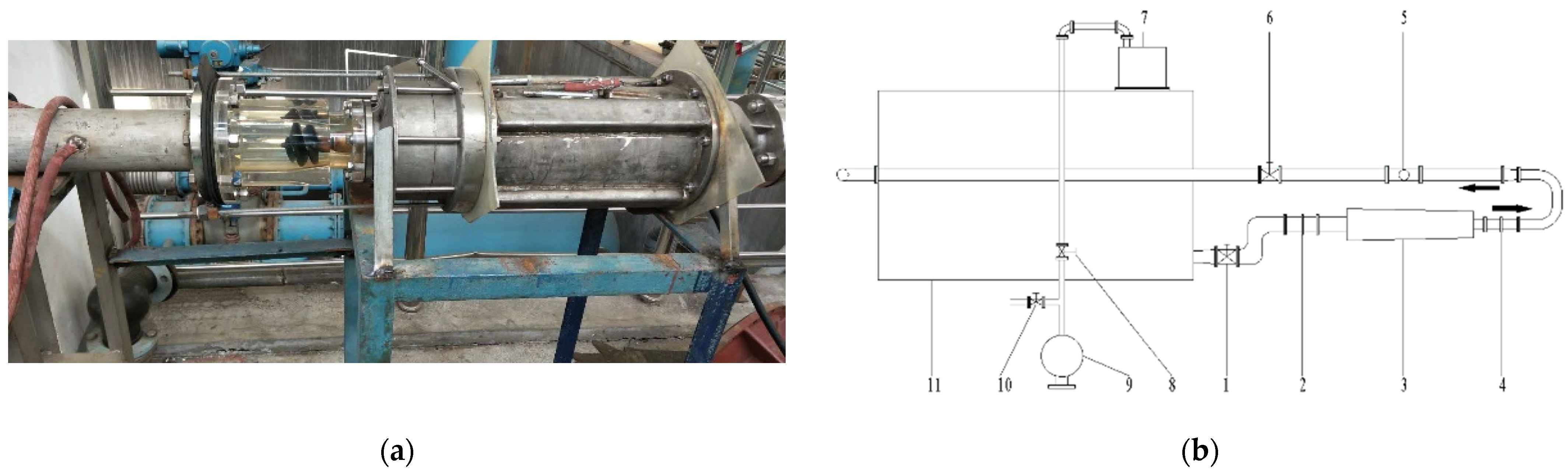
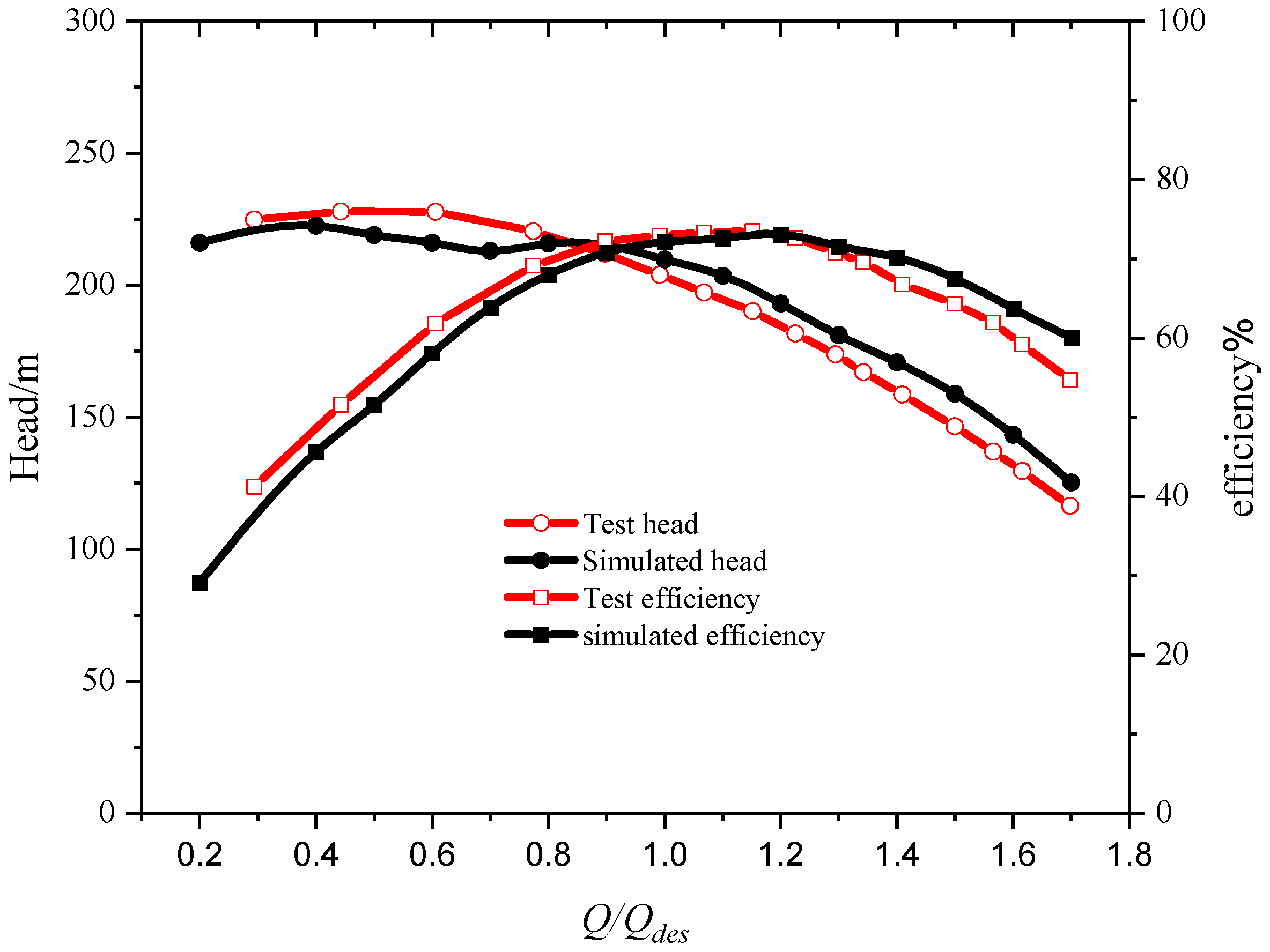
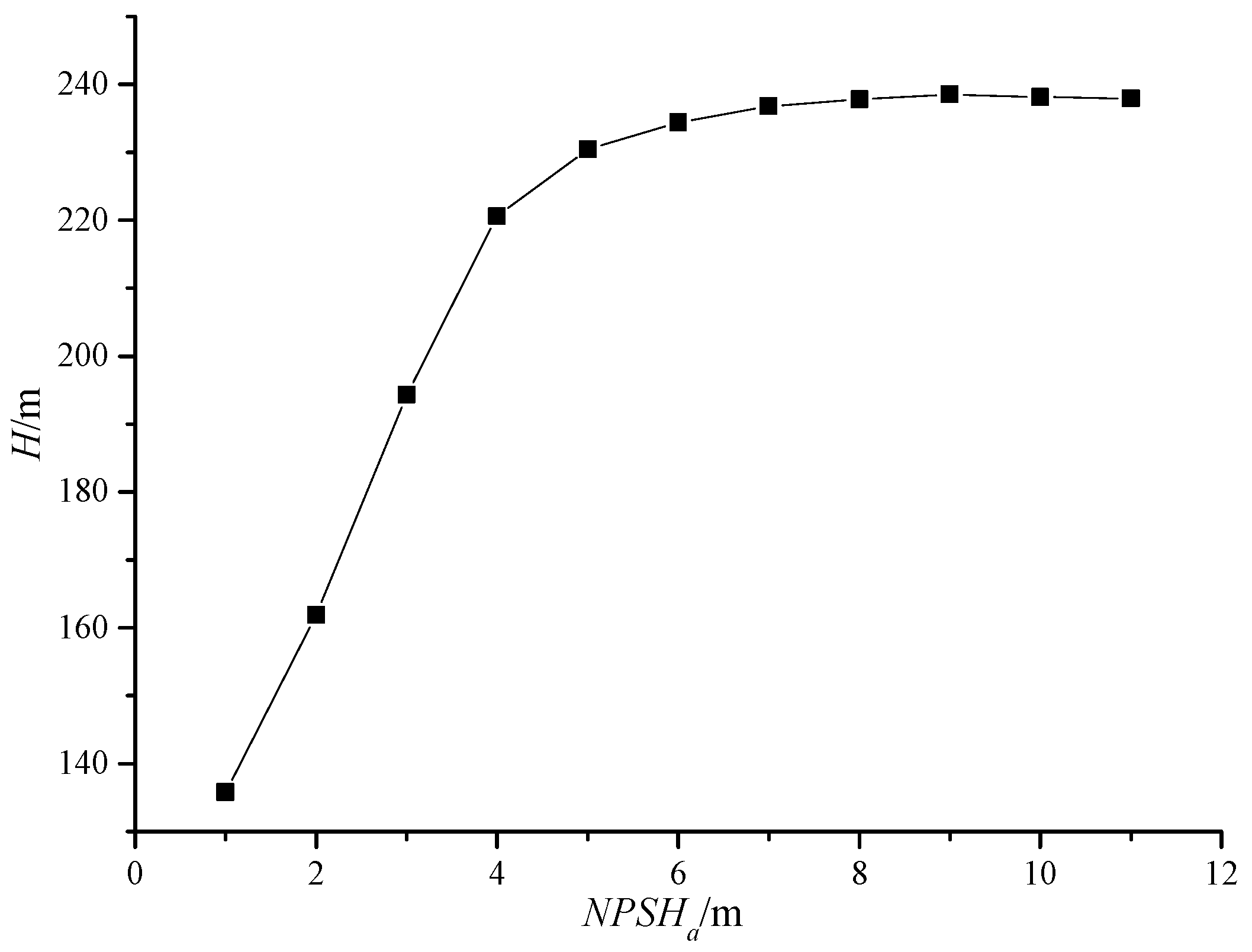



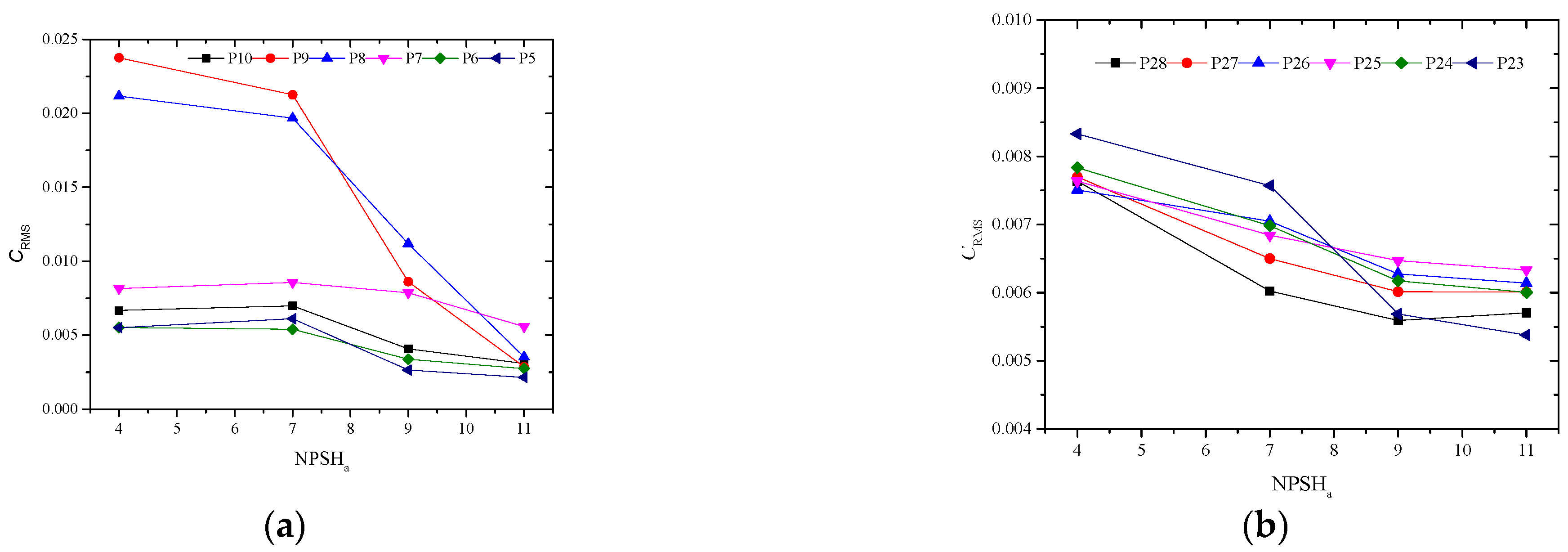
| Content | Parameters |
|---|---|
| Structural | Submerged centrifugal pump |
| Series | Two-stage |
| Design speed n | 6000 r/min |
| Specific speed ns | 89.4 |
| Flow at design operating point Q | 60 m3/h |
| Head at design operating point H | 200 m |
| NPSH at design operating point NPSHa | ≤5.5 m |
| Conveying medium | Liquified natural gas (LNG) |
| Content | Parameters |
|---|---|
| Impeller inlet diameter Ds | 68 mm |
| Hub diameter dh | 30 mm |
| Blade inlet diameter D1 | 68 mm |
| Blade outlet diameter D2 | 150 mm |
| Impeller outlet width b2 | 10 mm |
| Blade inlet angle β1 | 24° |
| Blade outlet angle β2 | 30° |
| Number of blades Z | 7 |
| Blade wrap angle φ | 120° |
| Scheme | Total Number of Grids | Head (m) |
|---|---|---|
| 1 | 2,439,179 | 227.8 |
| 2 | 3,252,239 | 228.2 |
| 3 | 4,065,299 | 236.7 |
| 4 | 4,878,358 | 236.2 |
| NPSHa | S = 0.1 | S = 0.25 | S = 0.5 | S = 0.75 |
|---|---|---|---|---|
| 9 m |  |  |  |  |
| 6 m |  |  |  |  |
| 5 m |  |  |  |  |
| 4 m |  |  |  |  |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, W.; Li, S.; Ji, L.; Zhao, X.; Shi, W.; Agarwal, R.K.; Awais, M.; Yang, Y. A Study on the Cavitation and Pressure Pulsation Characteristics in the Impeller of an LNG Submerged Pump. Machines 2022, 10, 14. https://doi.org/10.3390/machines10010014
Li W, Li S, Ji L, Zhao X, Shi W, Agarwal RK, Awais M, Yang Y. A Study on the Cavitation and Pressure Pulsation Characteristics in the Impeller of an LNG Submerged Pump. Machines. 2022; 10(1):14. https://doi.org/10.3390/machines10010014
Chicago/Turabian StyleLi, Wei, Shuo Li, Leilei Ji, Xiaofan Zhao, Weidong Shi, Ramesh K. Agarwal, Muhammad Awais, and Yang Yang. 2022. "A Study on the Cavitation and Pressure Pulsation Characteristics in the Impeller of an LNG Submerged Pump" Machines 10, no. 1: 14. https://doi.org/10.3390/machines10010014
APA StyleLi, W., Li, S., Ji, L., Zhao, X., Shi, W., Agarwal, R. K., Awais, M., & Yang, Y. (2022). A Study on the Cavitation and Pressure Pulsation Characteristics in the Impeller of an LNG Submerged Pump. Machines, 10(1), 14. https://doi.org/10.3390/machines10010014










