Solutions of Nonlinear Fractional-Order Differential Equation Systems Using a Numerical Technique
Abstract
1. Introduction and Mathematical Preliminaries
2. Essential Definitions
2.1. Fundamental Concepts and Key Properties
2.2. Basic Concepts of ET
2.3. Investigation of MTDM
Principle of the MTDM
3. Basic Idea of MTDEM
3.1. Convergence Analysis
3.2. Error Analysis
3.3. Demonstrative Example
- For each interval
- For
4. Numerical Experiments and Discussion
- So,
4.1. TabularResults
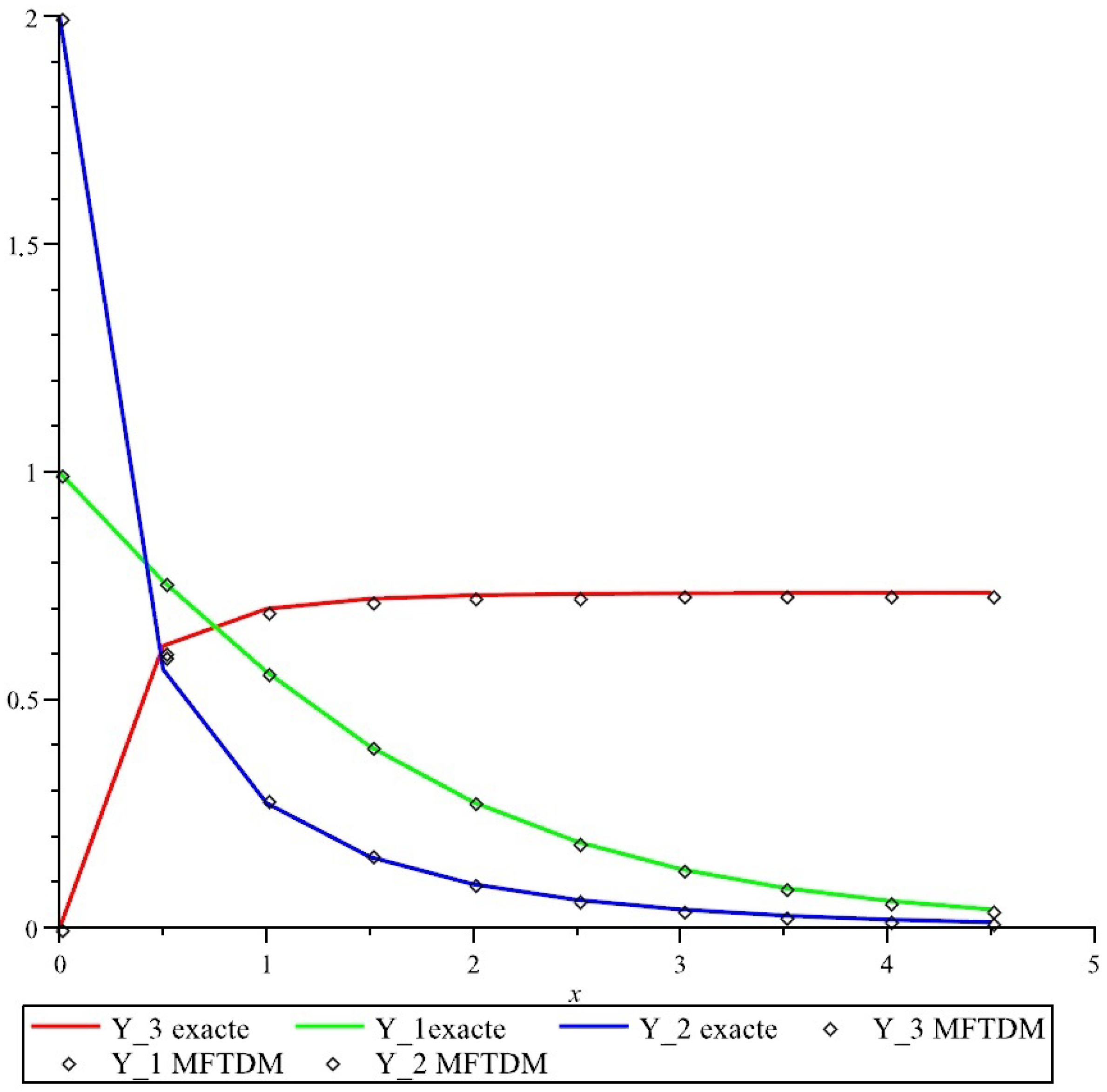
4.2. Graphical Analysis
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Adomian, G. Solution of physical problems by decomposition. Comp. Math. Appl. 1994, 27, 145–154. [Google Scholar] [CrossRef]
- Ziane, D.; Belghaba, K.; Cherif, M.H. Fractional homotopy perturbation transform method for solving the time-fractional KdV, k(2,2) and Burgers equations. Int. J. Open Prob. Comput. Sci. Math. 2015, 8, 63–75. [Google Scholar]
- He, J.H. A coupling method of homotoping technique and perturbation technique for nonlinear problems. Int. J. Nonlinear Mech. 2000, 35, 37–43. [Google Scholar]
- Abel-Rady, A.S.; Rida, S.Z.; Arafa, A.A.M.; Abedi-Rahim, H.R. Variational iteration Sumudu transform method for solving fractional nonlinear gaz dynamics equation. Int. J. Res. Stud. Sci. Eng. Technol. 2014, 1, 82–90. [Google Scholar]
- Hilall, E.; Elzaki, M.T. Solution of nonlinear partial differential equations by new Laplace variational iteration method. J. Funct. Spaces 2014, 2014, 790714. [Google Scholar] [CrossRef]
- Elzaki, T.M.; Elzaki, S.M. On the connections between Laplace and Elzaki transforms. Adv. Theor. Appl. Math. 2014, 6, 1–10. [Google Scholar]
- Afshan, K.; Tauseef, M.S. Coupling of Laplace transform and correction functional for wave equations. Word J. Mod. Simul. 2013, 9, 173–180. [Google Scholar]
- Gul, N.; Noor, S.; Saeed, A.M.; Aldhabani, M.S.; Ullah, R. Analytical solution of the systems of nolinear fractional partial differential equations using conformale Laplace transform iterative method. Fractal Fract. 2025, 10, 1945–1966. [Google Scholar]
- Kumar, D.; Singh, J.; Rathore, S. Sumudu decomposition method for nonlinear equations. Int. Math. Forum 2012, 7, 515–521. [Google Scholar]
- Singh, J.; Kumar, D.; Sushila, D. Homotopy perturbation Sumudu transform method for nonlinear equations. Adv. Theor. Appl. Mach. 2011, 4, 165–175. [Google Scholar]
- Rathore, S.; Kumar, D.; Singh, J.; Gupta, S. Homotopy analysis Sumudu transform method for nonlinear equations. Int. J. Ind. Math. 2012, 4, 301–314. [Google Scholar]
- Elzaki, T.M. The new integral transform Elzaki transform. Glob. J. Pure Appl. Math. 2011, 7, 57–64. [Google Scholar]
- Elzaki, T.M.; Hilal, E.M.A. Homotopy perturbation and Elzaki transform for solving nonlinear partial differential equations. Math. Theor. Mod. 2012, 2, 33–42. [Google Scholar]
- Elzaki, T.M.; Elzaki, S.M.; Elnour, E.A. On the new integral transform “Elzaki Transform” fundamental properties investigations and applications. Glob. J. Math. Sci. 2012, 2, 1–13. [Google Scholar]
- Elzaki, T.M. On the Elzaki transform and ordinary differential equation with variable coefficients. Adv. Theor. Appl. Math. 2011, 6, 13–18. [Google Scholar]
- Elzaki, T.M.; Biazar, J. Homotopy perturbation method and Elzaki transform for solving system of nonlinear partial differential equatios. World Appl. Sci. 2013, 24, 944–948. [Google Scholar]
- Ige, O.E.; Oderinu, R.A.; Elzaki, T.M. Adomian polynomial and Elzaki transform method for solving Klein Gordon equations. Int. J. Appl. Math. 2019, 32, 451–468. [Google Scholar] [CrossRef]
- Riabi, L.; Belghaba, K.; Cherif, M.H.; Ziane, D. Homotopy perturbation method combined with ZZ transform to solve some nonlinear fractional differential equations. Int. J. Anal. Appl. 2019, 17, 406–419. [Google Scholar]
- Al-Refai, M.; Abu-Dalu, M.; Al-Rawashde, A. Telescoping decomposition method for solving first order nonlinear differential equations. In Proceedings of the International Multi-Conference of Engineers and Computer Scientists, (IMECS 2008), Kowloon, Hong Kong, 19–21 March 2008. [Google Scholar]
- Khalid, M.; Sultana, M.; Zaidi, F.; Arshad, U. An Elzaki transform decomposition algorithm applied to a class of non-linear differential equations. J. Nat. Sci. Res. 2015, 5, 48–55. [Google Scholar]
- Nibron, M.; Mishra, A.K.; Kabala, D. Telescoping decomposition method for solving second order nonlinear differential equations. Int. J. Eng. Res. Technol. 2014, 3, 590–595. [Google Scholar]
- Ige, O.E.; Heilio, M.; Oderinu, R.A.; Elzaki, T.M. Adomian polynomial and Elzaki transform method of solving third order Korteweg-De Vries equatios. Glob. J. Pure Appl. Math. 2019, 15, 261–277. [Google Scholar]
- Zafar, Z.U.A. ZZ transform method. Int. J. Adv. Eng. Glob. Technol. 2016, 4, 1605–1611. [Google Scholar]
- Zafar, Z.U.A. Application of ZZ transform method on some fractional differential equation. Int. J. Adv. Eng. Glob. Technol. 2016, 4, 1355–1363. [Google Scholar]
- Ziane, D. The combined of Homotopy analysis method with new transform for nonlinear partial differential equations. Malaya J. Math. 2018, 6, 34–40. [Google Scholar] [CrossRef]
- Saadeh, R.; Qazza, A.; Burqan, A. A new integral transform: ARA transform and its properties and applications. Symmetry 2020, 12, 925. [Google Scholar] [CrossRef]
- Raghavendar, K.; Pavani, K.; Aruna, K.; Okposo, N.I.; Inc, M. Application of Natural Transform Decomposition Method for Solution of Fractional Richards Equation. Contemp. Math. 2024, 5, 5881. [Google Scholar] [CrossRef]
- Kharrat, B.N. New Double Kharrat-Toma Transform and its Application in Partials Differential Equations. World Appl. Sci. J. 2024, 42, 30–36. [Google Scholar]
- Hui, J. Fixed-time fractional-order sliding mode controller with disturbance observer for U-tube steam generator. Renew. Sustain. Energy Rev. 2024, 205, 114829. [Google Scholar] [CrossRef]
- Hui, J.; Lee, Y.; Yuan, J. Load following control of a PWR with load-dependent parameters and perturbations via fixed-time fractional-order sliding mode and disturbance observer techniques. Renew. Sustain. Energy Rev. 2023, 184, 113550. [Google Scholar] [CrossRef]
- Hui, J.; Lee, Y.; Yuan, J. Fractional-order sliding mode load following control via disturbance observer for modular high-temperature gas-cooled reactor system with disturbances. Asian J. Control. 2023, 25, 3513–3523. [Google Scholar] [CrossRef]
- Bouhassoun, A. Multistage telescoping decomposition method for solving fractional differential equations. Int. J. Appl. Math. 2013, 43, 1–7. [Google Scholar]
- Chita, F.; Belghaba, K. Solving systems of nonlinear fractional differential equations using multistage telescoping decomposition method. Asian J. Math. Comput. 2016, 13, 203–214. [Google Scholar]
- Chita, F.; Ayadi, S.; Ege, M.; Ege, O.; Ramaswamy, R. A Novel Approach for Solving Fractional Differential Equations via a Multistage Telescoping Decomposition Method. Fractal Fract. 2025, 9, 65. [Google Scholar] [CrossRef]
- Diethelm, K. The Analysis Fractional Differential Equations; Springer: Berlin/Heidelberg, Germany, 2010. [Google Scholar]

| Numerical Results of | |||
|---|---|---|---|
| Exact Solution | FTDM | MTDEM | |
| 0 | 1 | 1 | 1 |
| 0.5 | 0.6065306555 | 0.606532118 | 0.6065321181 |
| 1 | 0.3678794322 | 0.368055555 | 0.3678812102 |
| 1.5 | 0.2231301511 | 0.225976562 | 0.2231317696 |
| 2 | 0.1353352650 | 0.155555555 | 0.1353365849 |
| 2.5 | 0.0820849592 | 0.173719618 | 0.08227101598 |
| 3 | 0.0497870322 | 0.36250000 | 0.04990001359 |
| 3.5 | 0.0301973534 | 0.90809461 | 0.03026596092 |
| 4 | 0.0183156116 | 2.15555555 | 0.01835727739 |
| 4.5 | 0.0111089688 | 4.6791015 | 0.01113427834 |
| 5 | 0.00673791685 | 9.3680555 | 0.006753297424 |
| Numerical Results of | |||
| Exact Solution | FTDM | MTDEM | |
| 0 | 0 | 0 | 0 |
| 0.5 | 0.3666759377 | 0.366672398 | 0.3666723985 |
| 1 | 0.503346689 | 0.502558020 | 0.5033397218 |
| 1.5 | 0.5151197386 | 0.498914047 | 0.5151123990 |
| 2 | 0.4784218368 | 0.3709221000 | 0.4784160375 |
| 2.5 | 0.428589959 | −0.278 × | 0.4285857901 |
| 3 | 0.3793248078 | −1.711547 × | 0.3793810916 |
| 3.5 | 0.335194617 | −1.1185865 × | 0.3352707834 |
| 4 | 0.297187977 | −9.3550388 × | 0.2972663259 |
| 4.5 | 0.264975565 | −2.0961374 × | 0.2650488343 |
| 5 | 0.2378134754 | −1.8273720 × | 0.2378791642 |
| Numerical Results of | |||
| Exact Solution | FTDM | MTDEM | |
| 0 | 0 | 0 | 0 |
| 0.5 | 0.0267934066 | 0.0267954831 | 0.02679548328 |
| 1 | 0.1287738779 | 0.129386423 | 0.1287790677 |
| 1.5 | 0.2617501102 | 0.275109392 | 0.2617558307 |
| 2 | 0.3862428981 | 0.473522300 | 0.3862473769 |
| 2.5 | 0.4893250815 | 0.85370000000 | 0.4893282235 |
| 3 | 0.5708881599 | 1.711548 × | 0.570939244 |
| 3.5 | 0.6346080284 | 1.1185864 × | 0.634682854 |
| 4 | 0.6844964111 | 9.3550387 × | 0.6845614266 |
| 4.5 | 0.7239154655 | 2.09613743 × | 0.7240019172 |
| 5 | 0.7554486076 | 1.8273720 × | 0.7555525682 |
| Numerical Results of | |||
|---|---|---|---|
| Exact Solution | FTDM | MTDEM | |
| 0 | 1 | 1 | 1 |
| 0.5 | 0.7607628130 | 0.72313384 | 0.7574710997 |
| 1 | 0.5620657341 | −2.366865 | 0.5630034014 |
| 1.5 | 0.3977588680 | −233387.5294 | 0.3999142051 |
| 2 | 0.2756515018 | −5.075903071 × | 0.2778574593 |
| 2.5 | 0.1889960647 | −2.537093652 × | 0.1908877708 |
| 3 | 0.1288690558 | −5.567442828 × | 0.1303783537 |
| 3.5 | 0.0876386351 | −7.171360175 × | 0.08879997703 |
| 4 | 0.0595422272 | −6.351686445 × | 0.06041790370 |
| 4.5 | 0.0111089688 | −4.259538599 × | 0.01113427834 |
| 5 | 0.00673791685 | −2.303969156 × | 0.006753297424 |
| Numerical Results of | |||
| Exact Solution | FTDM | MTDEM | |
| 0 | 2 | 2 | 2 |
| 0.5 | 0.56737111575 | 0.79840051 | 0.5963406996 |
| 1 | 0.2735835848 | −0.097095 | 0.2839292143 |
| 1.5 | 0.1555627924 | −32212.1151 | 0.1607265228 |
| 2 | 0.0956977104 | −7.627578871 × | 0.09863836696 |
| 2.5 | 0.0614374820 | −3.937826779 × | 0.06328816258 |
| 3 | 0.0404791844 | −8.787034972 × | 0.04170595050 |
| 3.5 | 0.02711140440 | −1.143495031 × | 0.02795104991 |
| 4 | 0.0183532975 | −1.019837235 × | 0.01893960688 |
| 4.5 | 0.264975565 | −6.873555629 × | 0.2650488343 |
| 5 | 0.2378134754 | −3.732045475 × | 0.2378791642 |
| Numerical Results of | |||
| Exact Solution | FTDM | MTDEM | |
| 0 | 0 | 0 | 0 |
| 0.5 | 0.6193923396 | 0.52228093 | 0.6063342002 |
| 1 | 0.7007036555 | 3.023075 | 0.6950151510 |
| 1.5 | 0.7227514273 | 27829.6859 | 0.7186485735 |
| 2 | 0.7304239536 | 6.352213879 × | 0.7268158212 |
| 2.5 | 0.7334444988 | 3.237496837 × | 0.7300226878 |
| 3 | 0.7347200720 | 7.177257515 × | 0.7313763410 |
| 3.5 | 0.7352823904 | 9.303162403 × | 0.7319735550 |
| 4 | 0.7355371750 | 8.275031673 × | 0.7322445803 |
| 4.5 | 0.7239154655 | 5.566547764 × | 0.7240019172 |
| 5 | 0.7554486076 | 3.018007469 × | 0.7555525682 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Boukedroun, M.; Ayadi, S.; Chita, F.; Erden Ege, M.; Ege, O.; Ramaswamy, R. Solutions of Nonlinear Fractional-Order Differential Equation Systems Using a Numerical Technique. Axioms 2025, 14, 233. https://doi.org/10.3390/axioms14040233
Boukedroun M, Ayadi S, Chita F, Erden Ege M, Ege O, Ramaswamy R. Solutions of Nonlinear Fractional-Order Differential Equation Systems Using a Numerical Technique. Axioms. 2025; 14(4):233. https://doi.org/10.3390/axioms14040233
Chicago/Turabian StyleBoukedroun, Mohammed, Souad Ayadi, Fouzia Chita, Meltem Erden Ege, Ozgur Ege, and Rajagopalan Ramaswamy. 2025. "Solutions of Nonlinear Fractional-Order Differential Equation Systems Using a Numerical Technique" Axioms 14, no. 4: 233. https://doi.org/10.3390/axioms14040233
APA StyleBoukedroun, M., Ayadi, S., Chita, F., Erden Ege, M., Ege, O., & Ramaswamy, R. (2025). Solutions of Nonlinear Fractional-Order Differential Equation Systems Using a Numerical Technique. Axioms, 14(4), 233. https://doi.org/10.3390/axioms14040233





