Advanced Methods for Conformable Time-Fractional Differential Equations: Logarithmic Non-Polynomial Splines
Abstract
1. Introduction
2. Logarithmic Non-Polynomial Spline (LNPS) Construct
3. Truncation Error
4. Application of Conformable LNPSM to Time-Fractional Coupled TFBH Equation
- for .
- for all .
- if is a constant function.
- .
- .
- if is differentiable.
5. Stability Analysis of Conformable LNPSM
6. Numerical Results and Discussion
7. Conclusions
- High Accuracy: The scheme achieves a truncation error with sixth-order convergence, ensuring high precision in numerical solutions.
- Unconditional Stability: The logarithmic non-polynomial spline method is proven to be unconditionally stable, making it reliable for a wide range of problems.
- Superior Performance: Comparative analysis using and norms demonstrates that the method outperforms both the cubic B-spline and Caputo non-polynomial methods.
- Applicability: The method is well-suited for solving conformable time-fractional equations, showing its versatility and effectiveness in complex scenarios.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| FDEs | Fractional Differential Equations |
| TFBH | Time-Fractional Burgers–Huxley |
| LNPSM | Logarithmic Non-Polynomial Spline Method |
| KdV | Korteweg–De Vries |
References
- Debnath, L. A brief historical introduction to fractional calculus. Int. J. Math. Educ. Sci. Technol. 2004, 35, 487–501. [Google Scholar] [CrossRef]
- Sivalingam, S.M.; Kumar, P.; Govindaraj, V. A Chebyshev neural network-based numerical scheme to solve distributed-order fractional differential equations. Comput. Math. Appl. 2024, 164, 150–165. [Google Scholar] [CrossRef]
- Abu Arqub, O. Numerical solutions for the Robin time-fractional partial differential equations of heat and fluid flows based on the reproducing kernel algorithm. Int. J. Numer. Methods Heat Fluid Flow 2018, 28, 828–856. [Google Scholar] [CrossRef]
- Bagley, R.L.; Torvik, P.J. A Theoretical Basis for the Application of Fractional Calculus to Viscoelasticity. J. Rheol. 1983, 27, 201–210. [Google Scholar] [CrossRef]
- Uchaikin, V. Fractional Derivatives for Physicists and Engineers; Springer: Berlin/Heidelberg, Germany, 2013. [Google Scholar]
- Mohammed, P.O.; Srivastava, H.M.; Baleanu, D.; Al-Sarairah, E.; Yousif, M.A.; Chorfi, N. Analytical and approximate monotone solutions of the mixed order fractional nabla operators subject to bounded conditions. J. Comput. Appl. Math. 2024, 264, 626–639. [Google Scholar] [CrossRef]
- Yousif, M.A.; Hamasalh, F.K.; Zeeshan, A.; Abdelwahed, M. Efficient simulation of Time-Fractional Korteweg-de Vries equation via conformable-Caputo non-Polynomial spline method. PLoS ONE 2024, 19, e0303760. [Google Scholar] [CrossRef] [PubMed]
- Agarwal, P.; Baleanu, D.; Chen, Y.; Momani, S.; Machado, T. Fractional Calculus; Springer: Berlin/Heidelberg, Germany, 2018. [Google Scholar]
- Podlubny, I. Fractional Differential Equations: An Introduction to Fractional Derivatives, Fractional Differential Equations, to Methods of Their Solution and Some of Their Applications; Academic Press: Cambridge, MA, USA, 1999. [Google Scholar]
- Kilbas, A.A.; Srivastava, H.M.; Trujillo, J.J. Heory and Applications of Fractional Differential Equations; Elsevier: Amsterdam, The Netherlands, 2006. [Google Scholar]
- Sivalingam, S.M.; Govindaraj, V. Physics-informed neural network-based scheme and its error analysis for ψ-Caputo type fractional differential equations. Phys. Scr. 2024, 99, 096002. [Google Scholar] [CrossRef]
- Khalil, R.; Al Horani, M.; Yousef, A.; Sababheh, M. A new definition of fractional derivative. J. Comput. Appl. Math. 2014, 264, 65–70. [Google Scholar] [CrossRef]
- Noureen, R.; Naeem, M.N.; Baleanu, D.; Mohammed, P.O.; Almusawa, M.Y. Application of trigonometric B-spline functions for solving Caputo time fractional gas dynamics equation. AIMS Math. 2023, 8, 25343–25370. [Google Scholar] [CrossRef]
- Sadiya, U.; Inc, M.; Arefin, M.A.; Uddin, M.H. Consistent travelling waves solutions to the non-linear time fractional Klein–Gordon and Sine-Gordon equations through extended tanh-function approach. J. Taibah Univ. Sci. 2022, 16, 594–607. [Google Scholar] [CrossRef]
- Li, M.; Ding, X.; Xu, Q. Non-polynomial spline method for the time-fractional nonlinear Schrödinger equation. Adv. Differ. Equ. 2018, 2018, 318. [Google Scholar] [CrossRef]
- Yousif, M.A.; Hamasalh, F.K. The fractional non-polynomial spline method: Precision and modeling improvements. Math. Comput. Simul. 2024, 218, 512–525. [Google Scholar] [CrossRef]
- Srivastava, H.M.; Saad, K.M.; Hamanah, W.M. Certain new models of the multi-space fractal-fractional Kuramoto-Sivashinsky and Korteweg-de Vries equations. Mathematics 2022, 10, 1089. [Google Scholar] [CrossRef]
- Zou, G.-A. Numerical solutions to time-fractional stochastic partial differential equations. Numer. Algorithms 2019, 82, 553–571. [Google Scholar] [CrossRef]
- Mohamed, M.Z.; Hamza, A.E.; Sedeeg, A.K.H. Conformable double Sumudu transformations an efficient approximation solutions to the fractional coupled Burger’s equation. Ain Shams Eng. J. 2023, 14, 101879. [Google Scholar] [CrossRef]
- Yousif, M.A.; Juan, L.G.; Pshtiwan, O.M.; Nejmeddine, C.; Dumitru, B. A computational study of time-fractional gas dynamics models by means of conformable finite difference method. AIMS Math. 2024, 9, 19843–19858. [Google Scholar] [CrossRef]
- Yousif, M.A.; Hamasalh, F.K. A Hybrid Non-Polynomial Spline Method and Conformable Fractional Continuity Equation. Mathematics 2023, 11, 3799. [Google Scholar] [CrossRef]
- Wang, X.Y.; Zhu, Z.S.; Lu, Y.K. Solitary wave solutions of the generalized Burgers–Huxley equation. J. Phys. A Math. Gen. 1990, 23, 271–274. [Google Scholar] [CrossRef]
- Hodgkin, A.L.; Huxley, A.F. A quantitative description of membrane current and its application to conduction and excitation in nerve. J. Physiol. 1952, 117, 500–544. [Google Scholar] [CrossRef]
- Fitzhugh, R. Mathematical models of excitation and propagation in nerve. In Biological Engineering; Schwan, H.P., Ed.; McGraw-Hill: New York, NY, USA, 1969. [Google Scholar]
- Abdul, M.; Mohsin, K.; Noreen, A.; Dumitru, B. Numerical approximation of inhomogeneous time fractional Burgers–Huxley equation with B-spline functions and Caputo derivative. Eng. Comput. 2022, 38, 885–900. [Google Scholar]
- Deng, X. Traveling wave solutions for the generalized Burgers–Huxley equation. Appl. Math. Comput. 2008, 204, 733–737. [Google Scholar]
- Zhou, S.; Cheng, X. A linearly semi-implicit compact scheme for the Burgers–Huxley equation. Int. J. Comput. Math. 2011, 88, 795–804. [Google Scholar] [CrossRef]
- Gupta, V.; Kadalbajoo, M.K. A singular perturbation approach to solve Burgers–Huxley equation via monotone finite difference scheme on layer adaptive mesh. Commun. Nonlinear Sci. Numer. Simul. 2011, 16, 1825–1844. [Google Scholar] [CrossRef]
- Dehghan, M.; Saray, B.N.; Lakestani, M. Three methods based on the interpolation scaling functions and the mixed collocation finite difference schemes for the numerical solution of the nonlinear generalized Burgers–Huxley equation. Math. Comput. Model 2012, 55, 1129–1142. [Google Scholar] [CrossRef]
- Mohanty, R.K.; Dai, W.; Liu, D. Operator compact method of accuracy two in time and four in space for the solution of time dependent Burgers-Huxley equation. Numer. Algorithms 2015, 70, 591–605. [Google Scholar] [CrossRef]
- Zibaei, S.; Zeinadini, M.; Namjoo, M. Numerical solutions of Burgers–Huxley equation by exact finite difference and NSFD schemes. J. Differ. Equ. Appl. 2016, 22, 1098–1113. [Google Scholar] [CrossRef]
- Yusuf, A.; Aliyu, A.I.; Baleanu, D. Lie symmetry analysis and explicit solutions for the time fractional generalized Burgers–Huxley equation. Opt. Quantum Electron 2018, 50, 94. [Google Scholar]
- Alinia, N.; Zarebnia, M. A numerical algorithm based on a new kind of tension B-spline function for solving Burgers–Huxley equation. Numer. Algorithms 2019, 82, 1121–1142. [Google Scholar] [CrossRef]
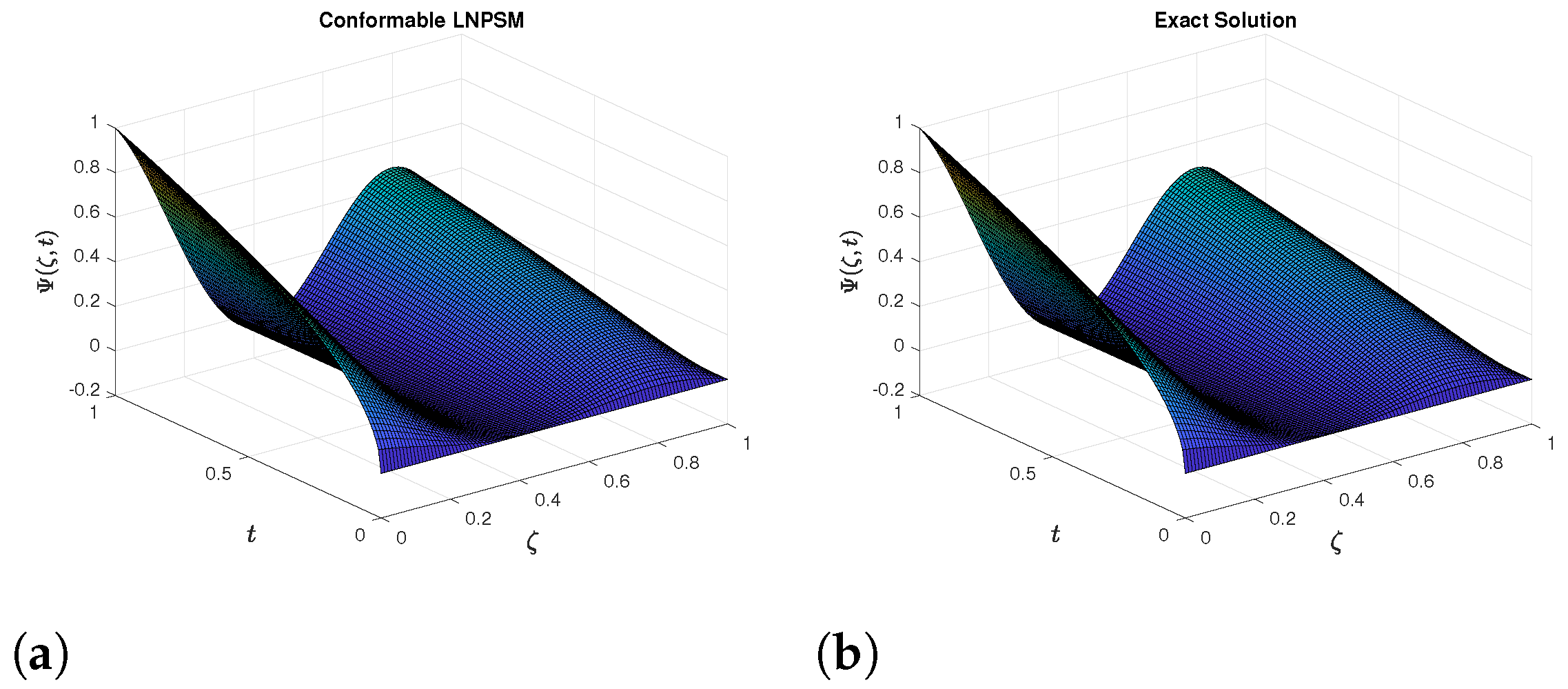

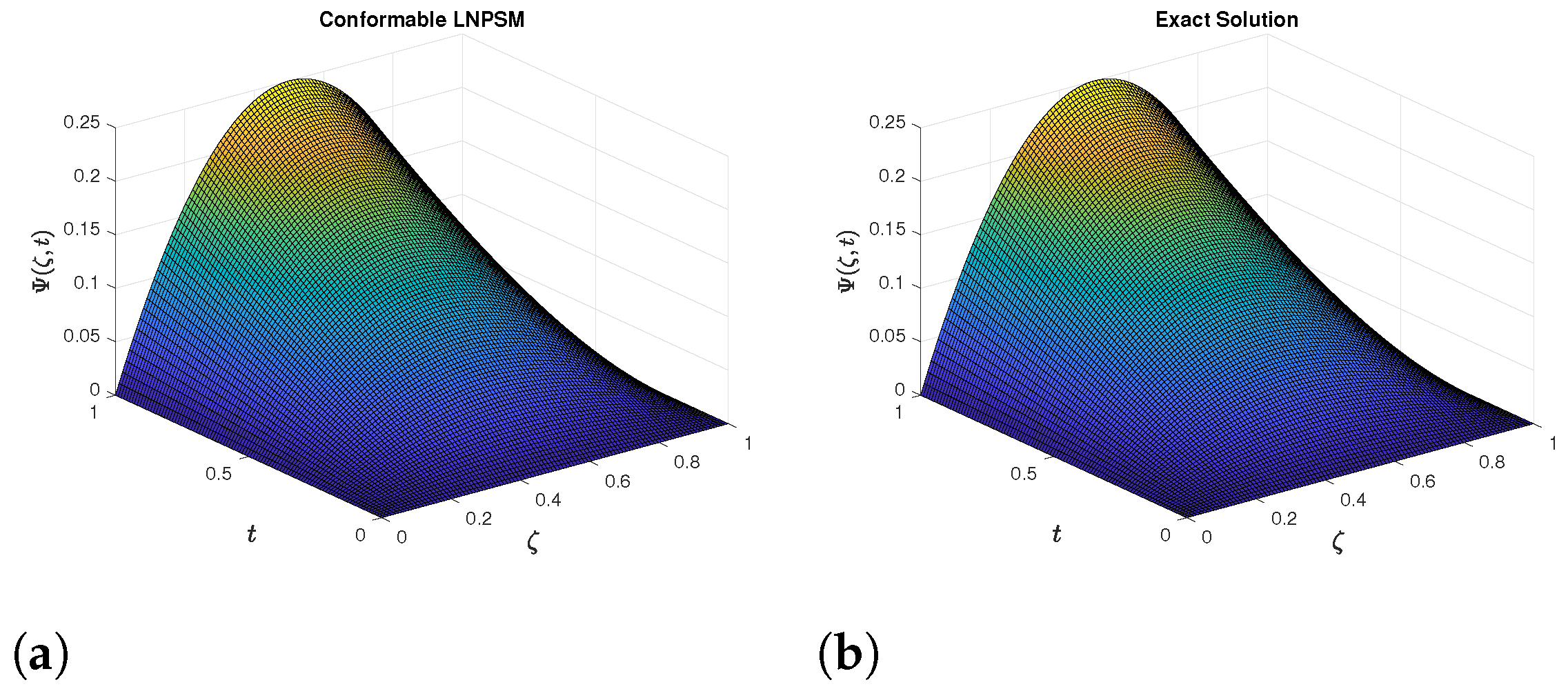
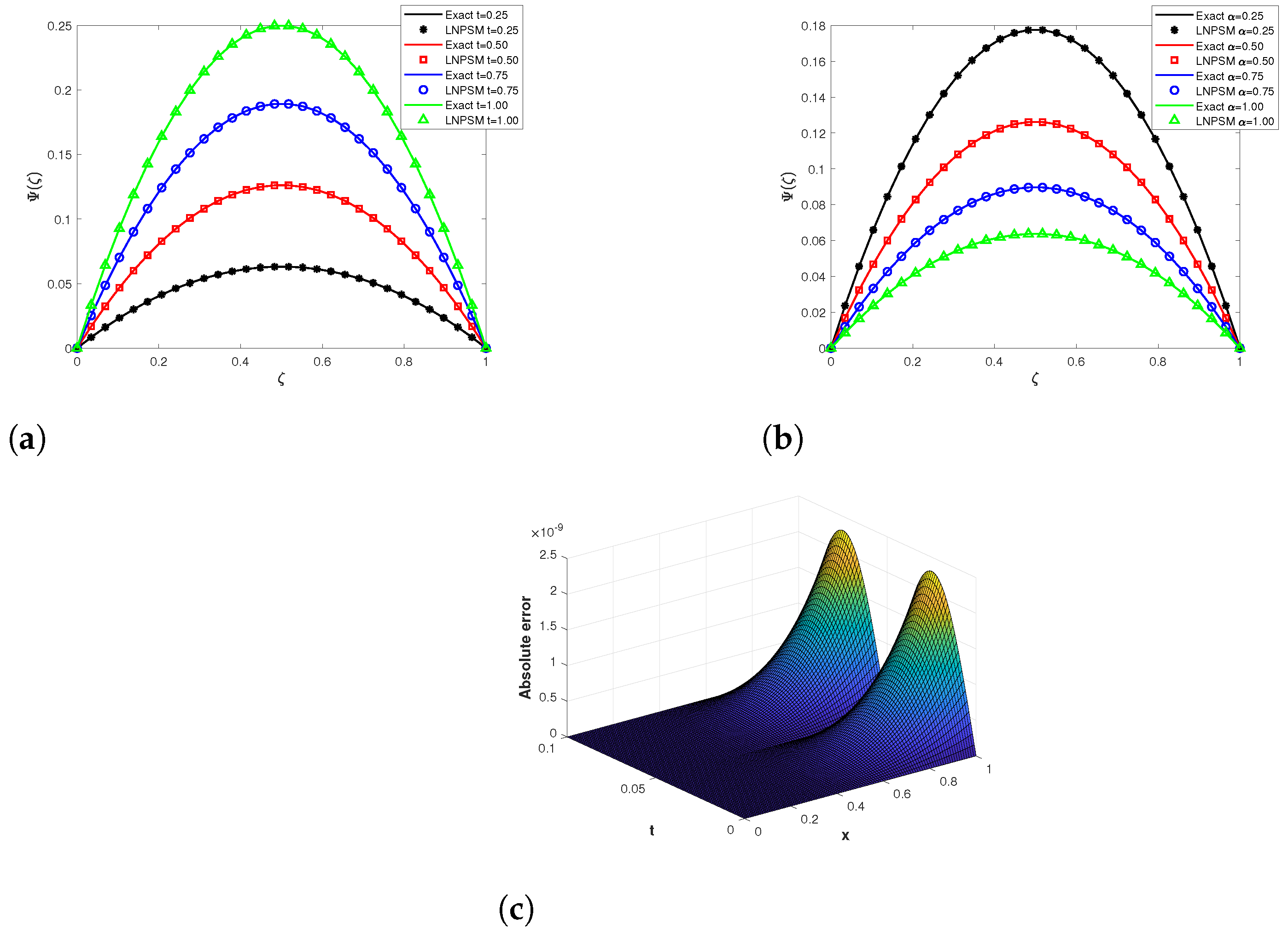
| LNPSM | [25] | ||||
|---|---|---|---|---|---|
| n | ||||
|---|---|---|---|---|
| 50 | ⋯ | ⋯ | ||
| 100 | ||||
| 200 |
| LNPSM | [25] | ||||
|---|---|---|---|---|---|
| n | ||||
|---|---|---|---|---|
| 50 | ⋯ | ⋯ | ||
| 100 | ||||
| 200 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yousif, M.A.; Agarwal, R.P.; Mohammed, P.O.; Lupas, A.A.; Jan, R.; Chorfi, N. Advanced Methods for Conformable Time-Fractional Differential Equations: Logarithmic Non-Polynomial Splines. Axioms 2024, 13, 551. https://doi.org/10.3390/axioms13080551
Yousif MA, Agarwal RP, Mohammed PO, Lupas AA, Jan R, Chorfi N. Advanced Methods for Conformable Time-Fractional Differential Equations: Logarithmic Non-Polynomial Splines. Axioms. 2024; 13(8):551. https://doi.org/10.3390/axioms13080551
Chicago/Turabian StyleYousif, Majeed A., Ravi P. Agarwal, Pshtiwan Othman Mohammed, Alina Alb Lupas, Rashid Jan, and Nejmeddine Chorfi. 2024. "Advanced Methods for Conformable Time-Fractional Differential Equations: Logarithmic Non-Polynomial Splines" Axioms 13, no. 8: 551. https://doi.org/10.3390/axioms13080551
APA StyleYousif, M. A., Agarwal, R. P., Mohammed, P. O., Lupas, A. A., Jan, R., & Chorfi, N. (2024). Advanced Methods for Conformable Time-Fractional Differential Equations: Logarithmic Non-Polynomial Splines. Axioms, 13(8), 551. https://doi.org/10.3390/axioms13080551












