Designing a Secure Mechanism for Image Transferring System Based on Uncertain Fractional Order Chaotic Systems and NLFPID Sliding Mode Controller
Abstract
:1. Introduction
- -
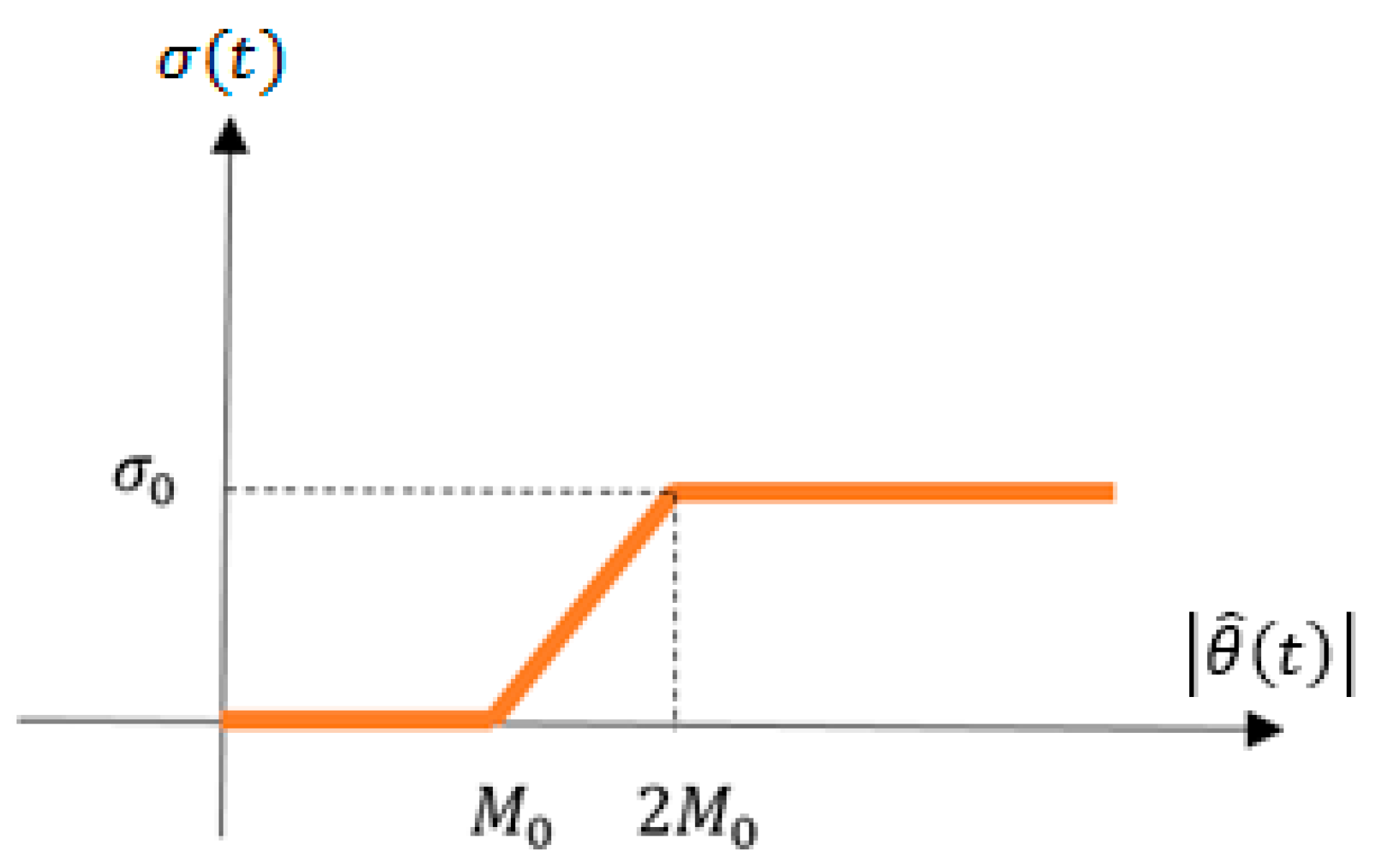
- The use of the nonlinear fractional PID (NLFOPID) sliding surface instead of typical sliding surfaces.
- -
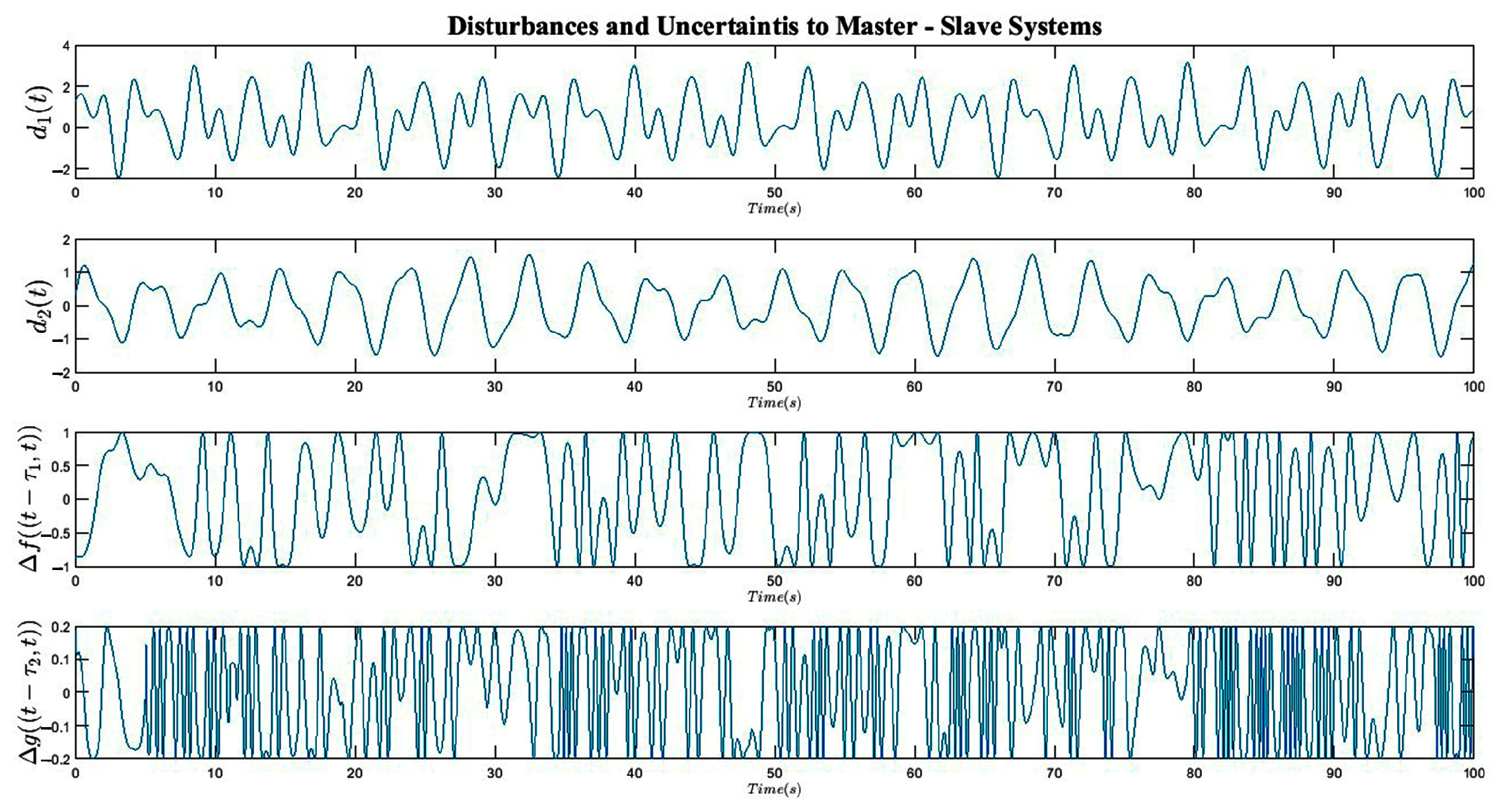
- The presence of unknown time delays
- -
- The presence of uncertainty and disturbance with unknown boundaries. Then, using the suitable Lyapunov function and update laws, a control signal was extracted that could be used to overcome the chattering problem by properly adjusting the controller parameters. This is a critical issue for the suggested controller’s implementation. In [10,18], a controller for the synchronization of chaotic systems in finite time was constructed utilizing a sliding surface, and the synchronization of the integer order chaotic system was investigated in [19].
2. Preliminary Definitions of Fractional Order Differentiation
3. System Descriptor Equations
4. The Sliding Mode Control Approach Based on Fractional Order Nonlinear PID Controllers
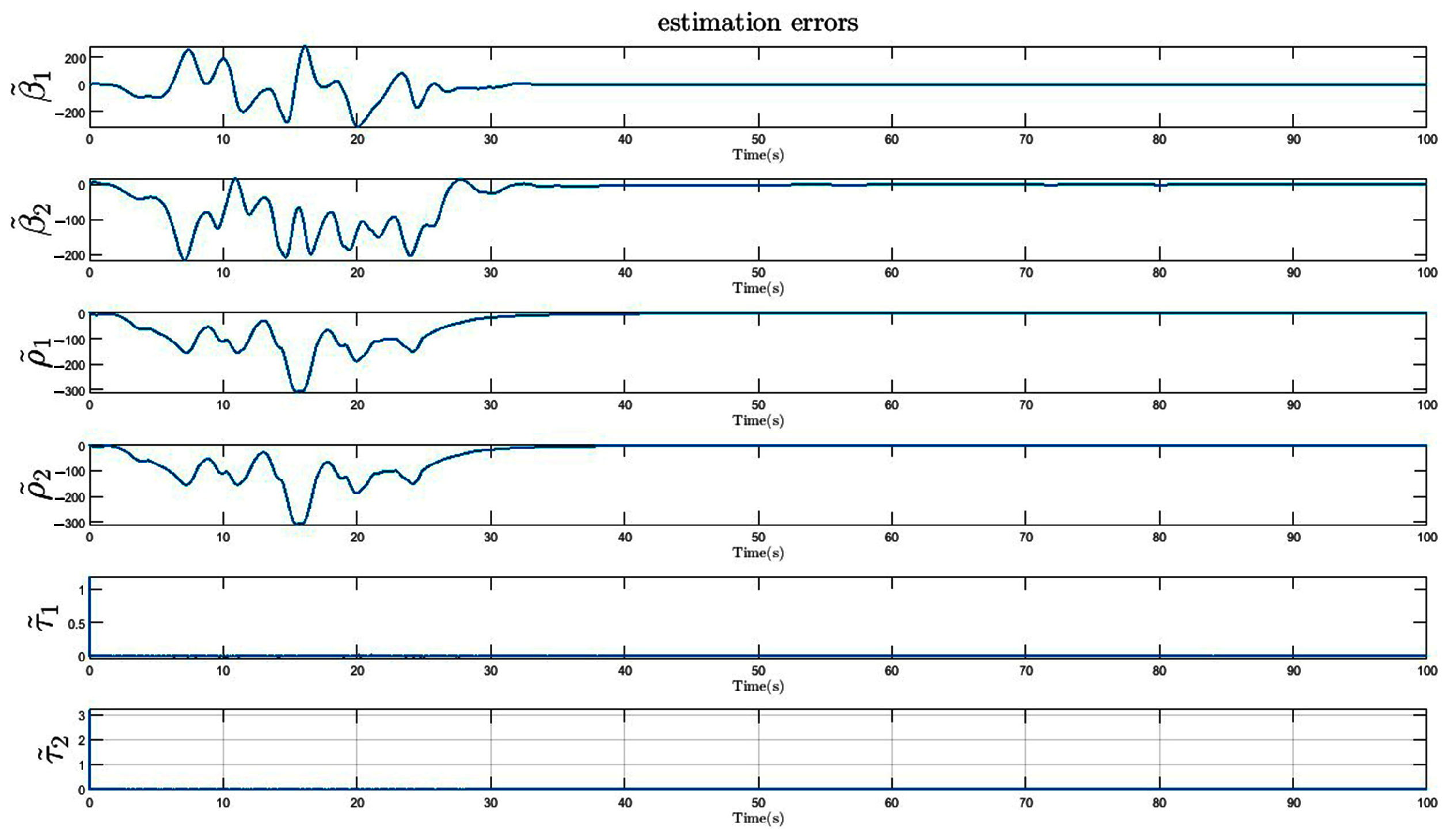
5. Stability Analysis and Determining the Update Laws
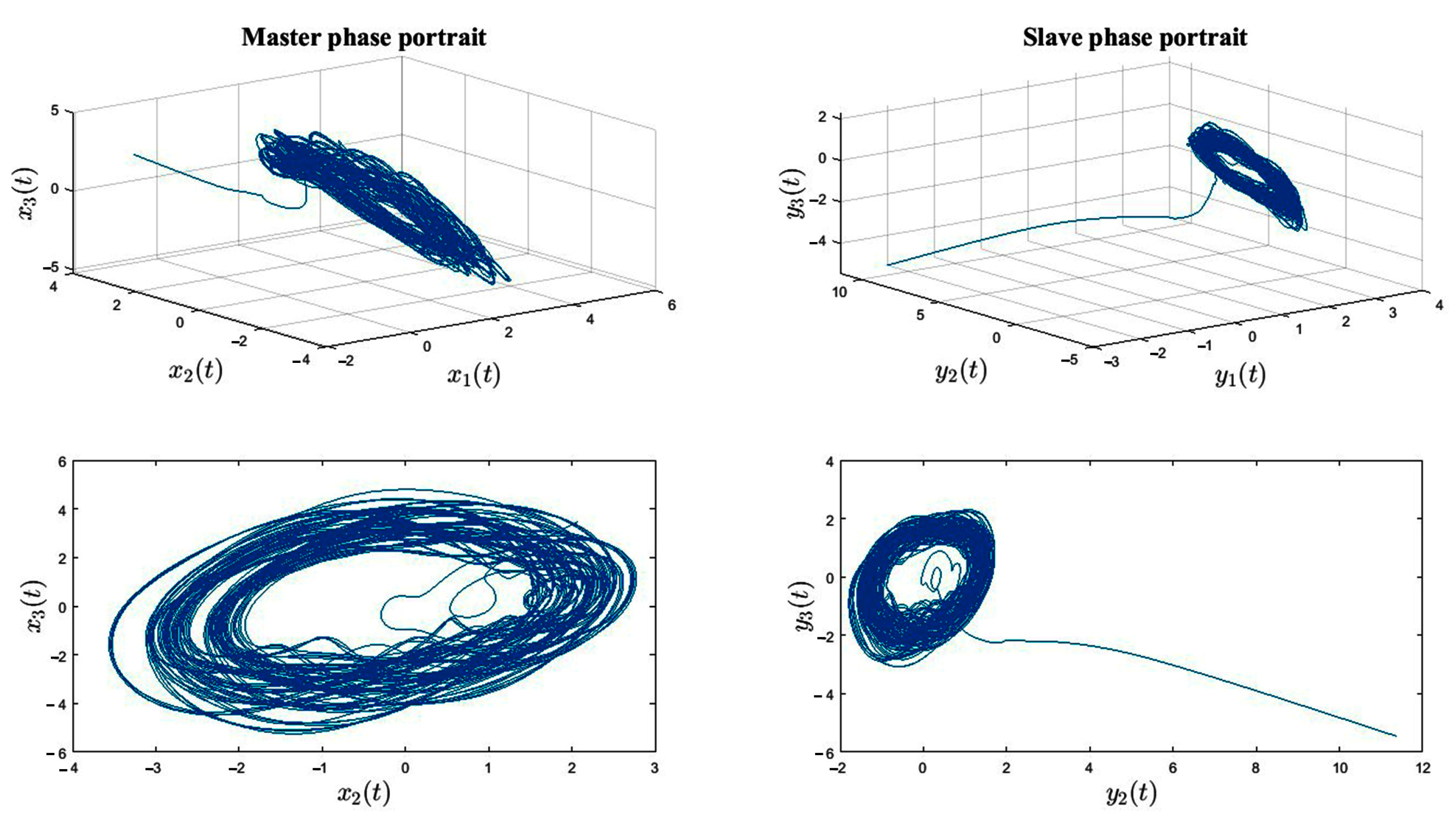
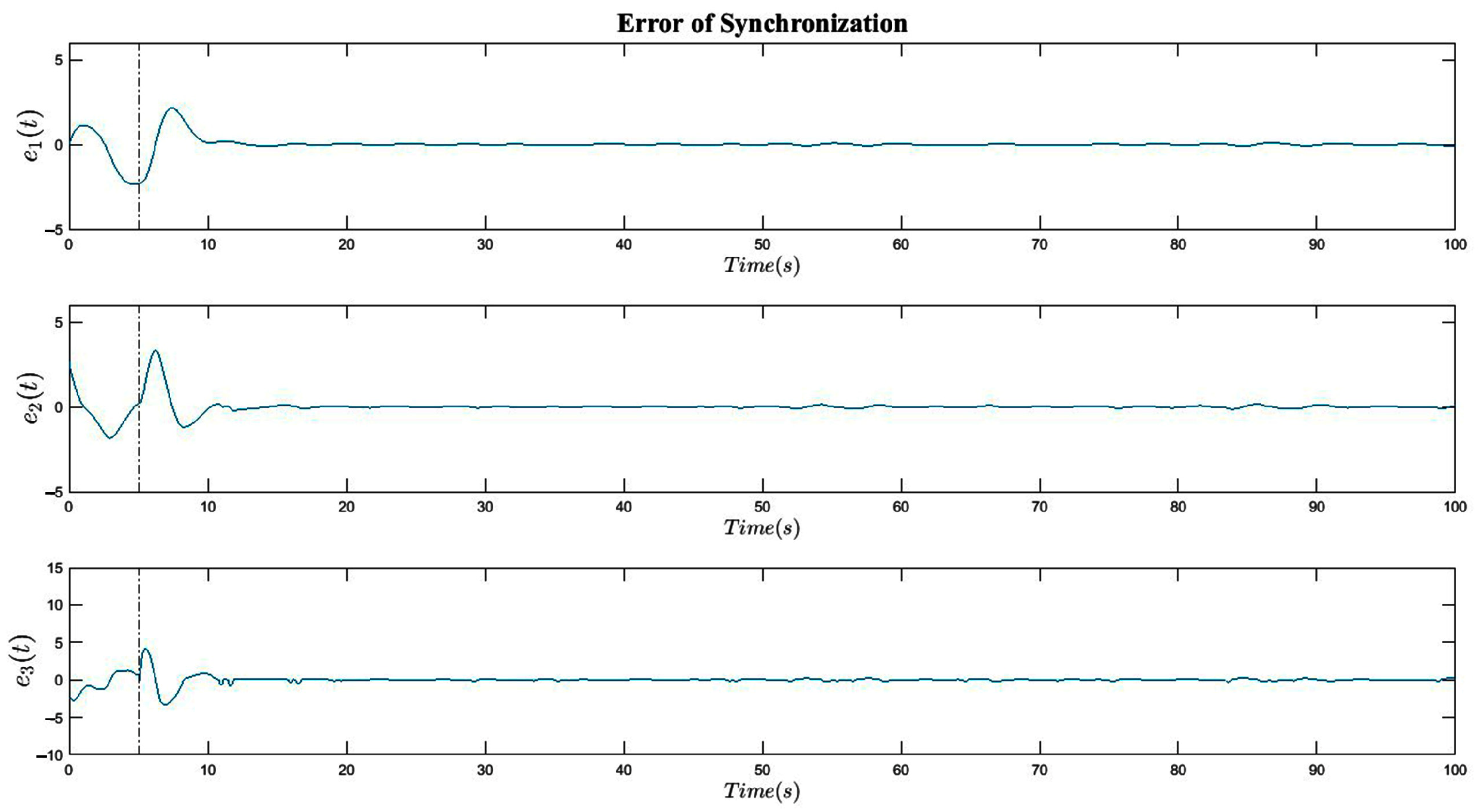
6. Simulation Results
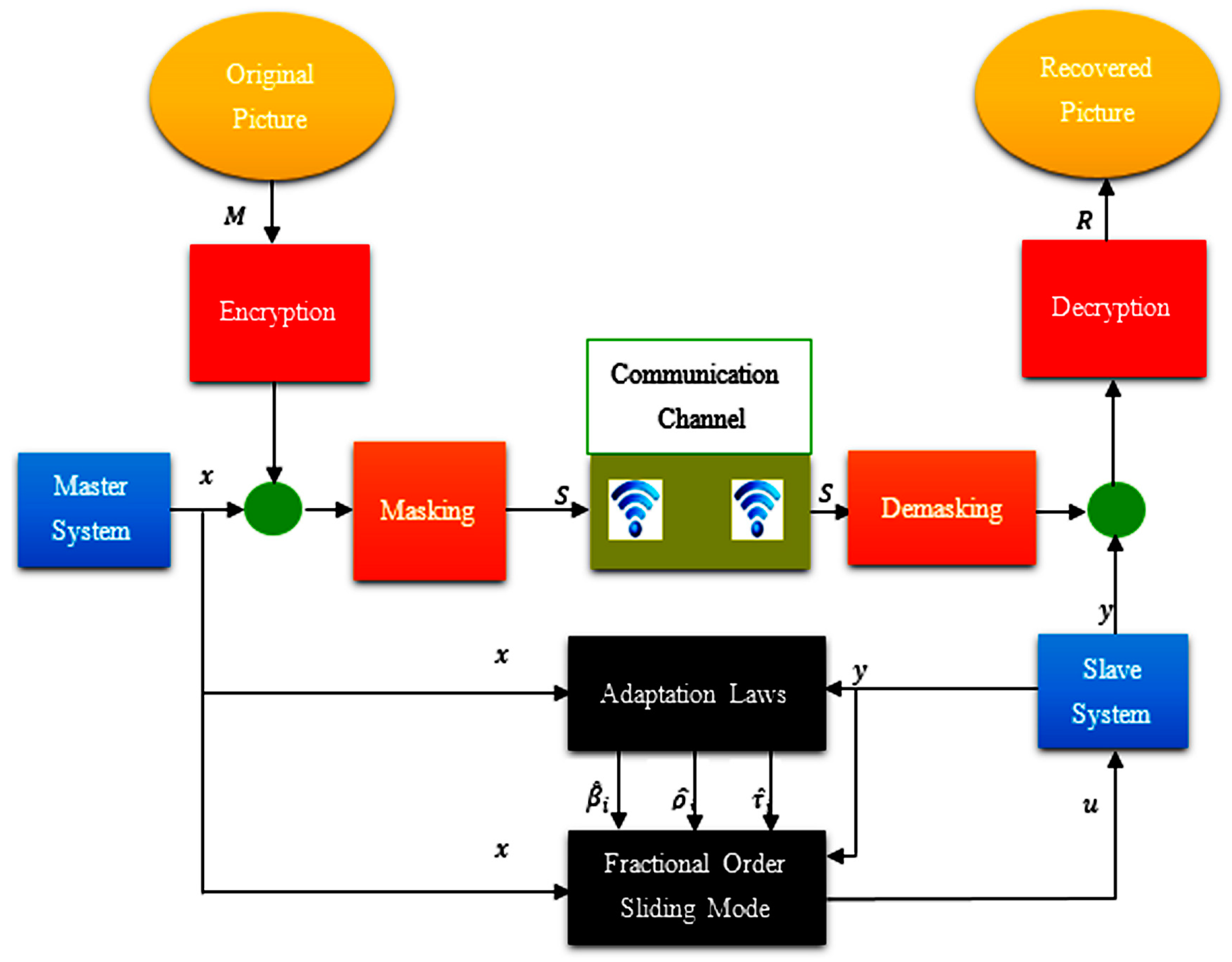
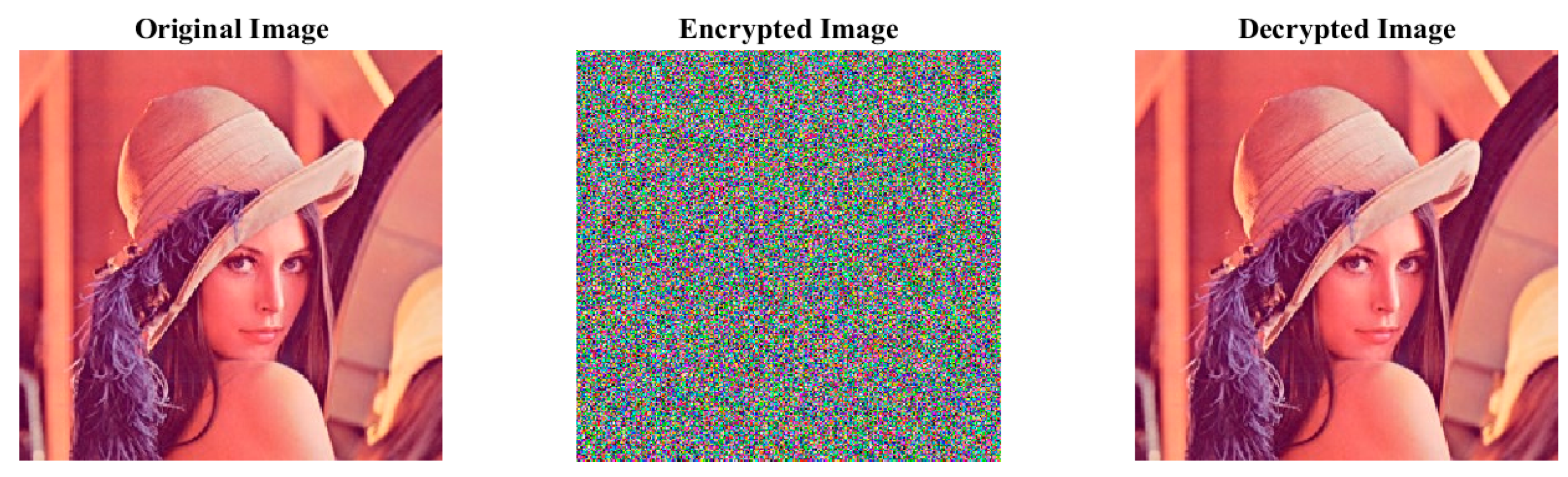
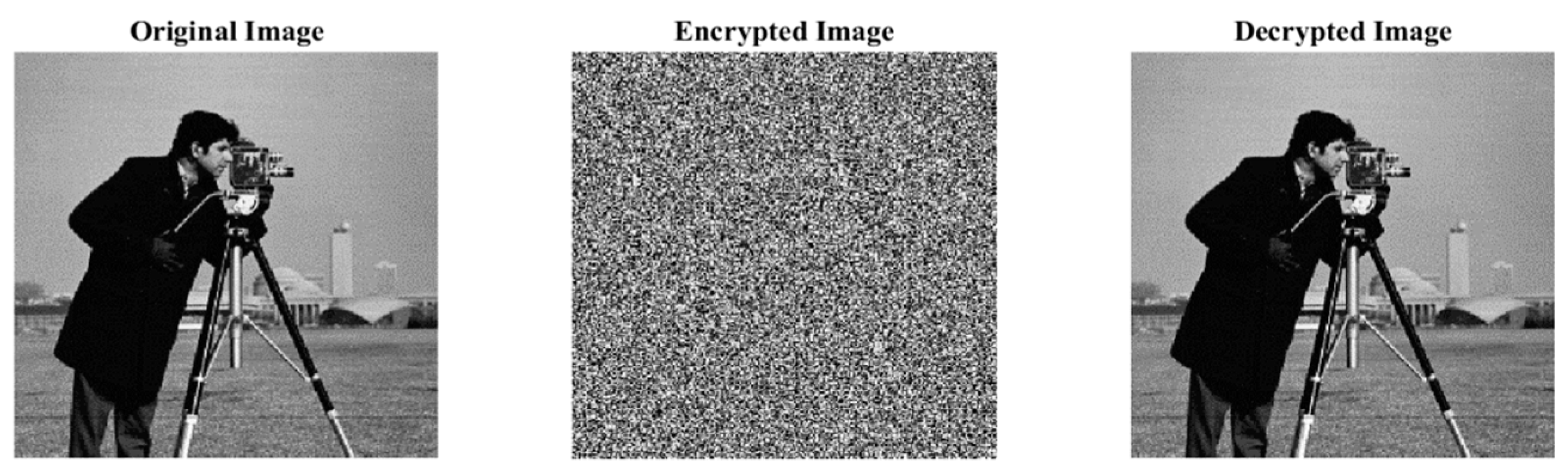
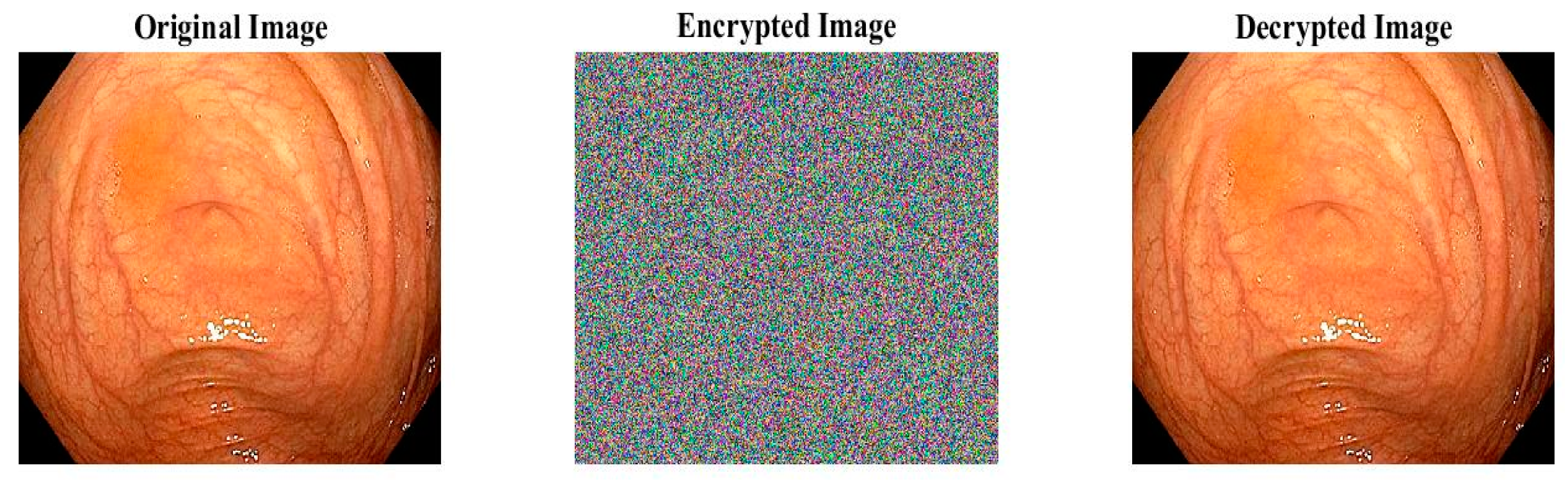
7. Application of Secure Communication in Encryption and Image Retrieval
8. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Lorenz, E.N. Deterministic Non-Periodic Flow. J. Atmos. Sci. 1963, 20, 130–141. [Google Scholar] [CrossRef]
- Chen, G.R.; Ueta, T. Yet Another Chaotic Attractor. Int. J. Bifurc. Chaos Appl. Sci. Eng. 1999, 9, 1465–1466. [Google Scholar] [CrossRef]
- Lü, J.H.; Chen, G.R. A New Chaotic Attractor Coined. Int. J. Bifurc. Chaos Appl. Sci. Eng. 2002, 12, 659–661. [Google Scholar] [CrossRef]
- Liu, C.X.; Liu, T.; Liu, L.; Liu, K. A New Chaotic Attractor. Chaos Solitons Fractals 2004, 22, 1031–1038. [Google Scholar] [CrossRef]
- Fernandez, A.; Baleanu, D.; Srivastava, H. Series representations for fractionalcalculus operators involving generalised Mittag-Leffler functions. Commun. Nonlinear Sci. Numer. Simul. 2019, 67, 517–527. [Google Scholar] [CrossRef]
- Zhang, J.; Gao, F.; Chen, Y.; Zou, Y. Parameter identification of fractional-order chaotic system based on chemical reaction optimization. In Proceedings of the 2018 2nd International Conference on Management Engineering, Software Engineering and Service Sciences, New York, NY, USA, 13–15 January 2018; pp. 217–222. [Google Scholar]
- Ionescu, C.; Lopes, A.; Copot, D.; Machado, J.T.; Bates, J. The role of fractionalcalculus in modeling biological phenomena: A review. Commun. Nonlinear Sci. Numer. Simul. 2017, 51, 141–159. [Google Scholar] [CrossRef]
- Smida, M.B.; Sakly, A.; Vaidyanathan, S.; Azar, A.T. Control-based maximum power point tracking for a grid-connected hybrid renewable energy system optimized by particle swarm optimization. In Advances in System Dynamics and Control; Tomorrow’s Research Today: Rochester, NY, USA, 2018; pp. 58–89. [Google Scholar]
- Vinagre, B.; Feliu, V. Modeling and control of dynamic system using fractional calculus: Application to lectrochemical processes and flexible structures. In Proceedings of the 41st IEEE Conference on Decision and Control, Las Vegas, NV, USA, 10–13 December 2002; Volume 1, pp. 214–239. [Google Scholar]
- Li, R.-G.; Wu, H.-N. Secure communication on fractionalorder chaotic systems via adaptive sliding mode control with teaching–learning–feedback-based optimization. Nonlinear Dyn. 2018, 92, 1221–1243. [Google Scholar]
- Mandelbrot, B.B.; Van Ness, J.W. Fractional Brownian motions, fractional noises and applications. SIAM Rev. 1968, 10, 422–437. [Google Scholar] [CrossRef]
- Duarte, F.B.; Machado, J.T. Chaotic phenomena and fractional-order dynamics in the trajectory control of redundant manipulators. Nonlinear Dyn. 2002, 29, 315–342. [Google Scholar] [CrossRef]
- Petráš, I. Fractional-order nonlinear controllers: Design and implementation notes. In Proceedings of the 2016 17th International Carpathian Control Conference (ICCC), High Tatras, Slovakia, 29 May–1 June 2016; IEEE: Piscataway, NJ, USA, 2016; pp. 579–583. [Google Scholar]
- Mirrezapour, S.Z.; Zare, A. A new fractional sliding mode controller based on nonlinear fractional-order proportional integral derivative controller structure to synchronize fractional-order chaotic systems with uncertainty and disturbances. J. Vib. Control. 2021, 28, 1–13. [Google Scholar] [CrossRef]
- Zare, A.; Mirrezapour, S.Z.; Hallaji, M.; Shoeibi, A.; Jafari, M.; Ghassemi, N.; Alizadehsani, R.; Mosavi, A. Robust Adaptive Synchronization of a Class of Uncertain Chaotic Systems with Unknown Time-Delay. Appl. Sci. 2020, 10, 8875. [Google Scholar] [CrossRef]
- Mohammadpour, S.; Binazadeh, T. Robust Observer-Based Synchronization of Unified Chaotic Systems in the Presence of Dead-Zone Nonlinearity Input. J. Control Iran. Soc. Instrum. Control Eng. (ISICE) 2018, 11, 25–36. [Google Scholar]
- Modiri, A.; Mobayen, S. Adaptive terminal sliding mode control scheme for synchronization of fractional-order uncertain chaotic systems. ISA Trans. 2020, 105, 33–50. [Google Scholar] [CrossRef]
- Mostafaee, J.; Mobayen, S.; Vaseghi, B.; Vahedi, M. Dynamical Analysis and Finite-Time Fast Synchronization of a Novel Autonomous Hyper-Chaotic System. J. Intell. Proced. Electr. Technol. 2021, 12, 47. [Google Scholar]
- Rasouli, M.; Zare, A.; Hallaji, M.; Alizadehsani, R. The Synchronization of a Class of Time-Delayed Chaotic Systems Using Sliding Mode Control Based on a Fractional-Order Nonlinear PID Sliding Surface and Its Application in Secure Communication. Axioms 2022, 11, 738. [Google Scholar] [CrossRef]
- Aghababa, M.P. Finite-time chaos control and synchronization of fractional order nonautonomous chaotic (hyperchaotic) systems using fractional nonsingular terminal sliding mode technique. Nonlinear Dynam. 2012, 69, 247–261. [Google Scholar] [CrossRef]
- Balochian, S.; Sedigh, A.K.; Zare, A. Variable structure control of linear time invariant fractional order systems using a finite number of state feedback law. Commun. Nonlinear Sci. Numer. Simul. 2011, 16, 1433–1442. [Google Scholar] [CrossRef]
- Chen, W.; Dai, H.; Song, Y.; Zhang, Z. Convex Lyapunov functions for stability analysis of fractional order systems. IET Control Theory Appl. 2017, 11, 1070–1074. [Google Scholar] [CrossRef]
- Aguila-Camacho, N.; Duarte-Mermoud, M.A.; Gallegos, J.A. Lyapunov functions for fractional order systems. Commun. Nonlinear Sci. Numer. Simul. 2014, 19, 2951–2957. [Google Scholar] [CrossRef]
- Chen, X.; Park, J.H.; Cao, J.; Qiu, J. Sliding mode synchronization of multiple chaotic systems with uncertainties and disturbances. Appl. Math. Comput. 2017, 308, 161–173. [Google Scholar] [CrossRef]
- Draa, K.C.; Zemouche, A.; Alma, M.; Voos, H.; Darouach, M. New Trends in Observer-Based Control a Practical Guide to Process and Engineering Applications; Elsevier: Amsterdam, The Netherlands, 2019; pp. 99–135. [Google Scholar]
- Petras, I. Fractional-Order Nonlinear Systems: Modeling, Analysis and Simulation; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2011. [Google Scholar]
- Kekha Javan, A.A.; Zare, A.; Alizadehsani, R. Multi-State Synchronization of Chaotic Systems with Distributed Fractional Order Derivatives and Its Application in Secure Communications. Big Data Cogn. Comput. 2022, 6, 82. [Google Scholar] [CrossRef]









| Symbol | Concept | Symbol | Concept |
|---|---|---|---|
| Disturbance bound | Disturbance bound estimate | ||
| Uncertainty bound | Uncertainty bound estimate | ||
| Lipschitz constant | Time delay bound estimate | ||
| Time delay | Disturbance bound estimate error | ||
| Disturbance upper bound | Uncertainty bound estimate error | ||
| Uncertainty upper bound | Time delay estimate error | ||
| Time delay upper bound | Positive constant number | ||
| Time delay lower bound | Small positive constant number |
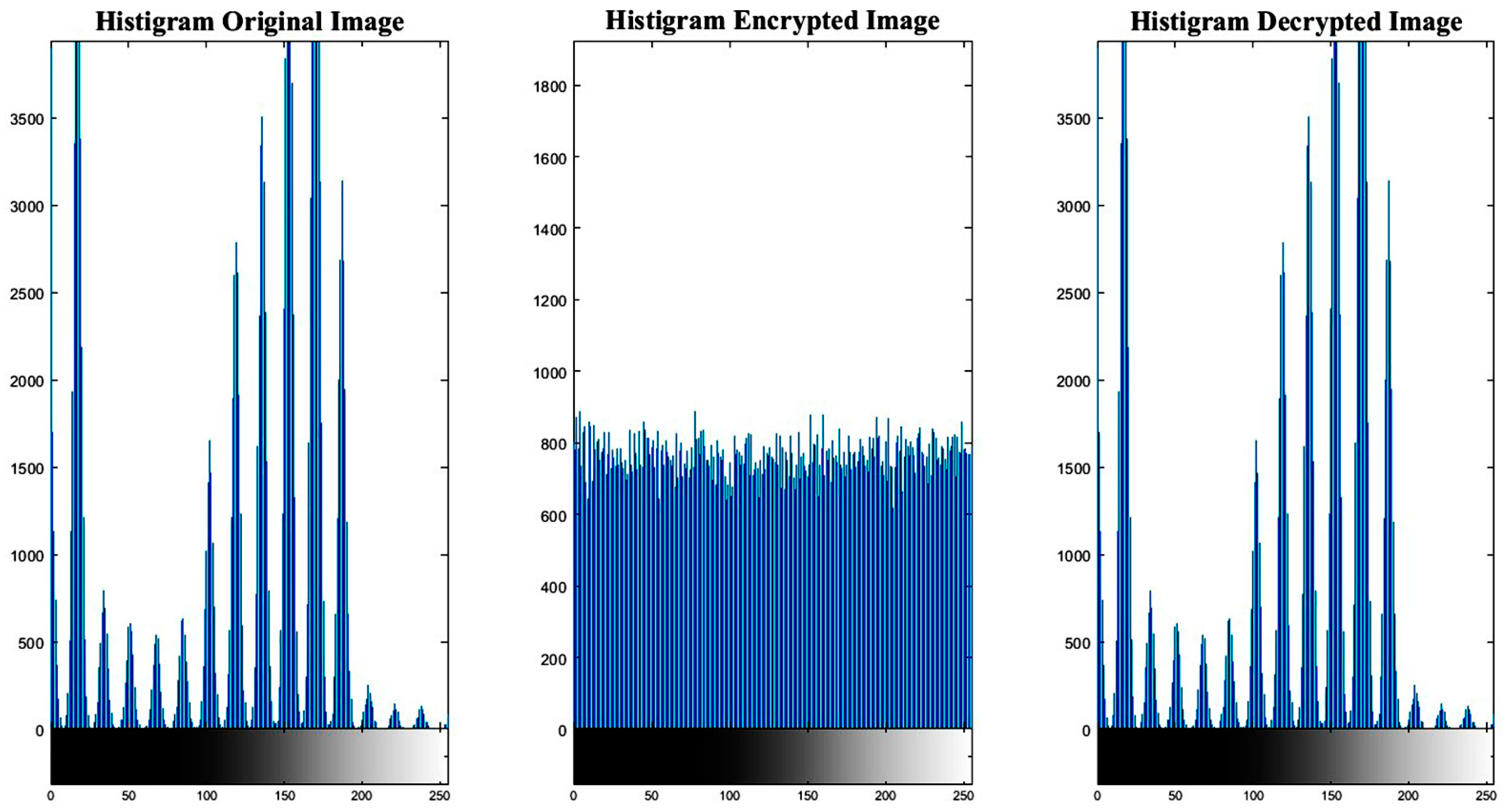
| Images | Histogram | Correlation | Differential Attack | PSNR | Information Entropy | ||
|---|---|---|---|---|---|---|---|
| Standard | Encrypted | NPCR (%) | UACI (%) | ||||
| Images 10 | 21,153.1171 | 21,148.239 | 0.0068 | 99.68 | 33.23 | 8.10 | 7.9690 |
| Images 12 | 18,144.3510 | 18,143.750 | 0.0043 | 99.40 | 33.46 | 8.27 | 7.9700 |
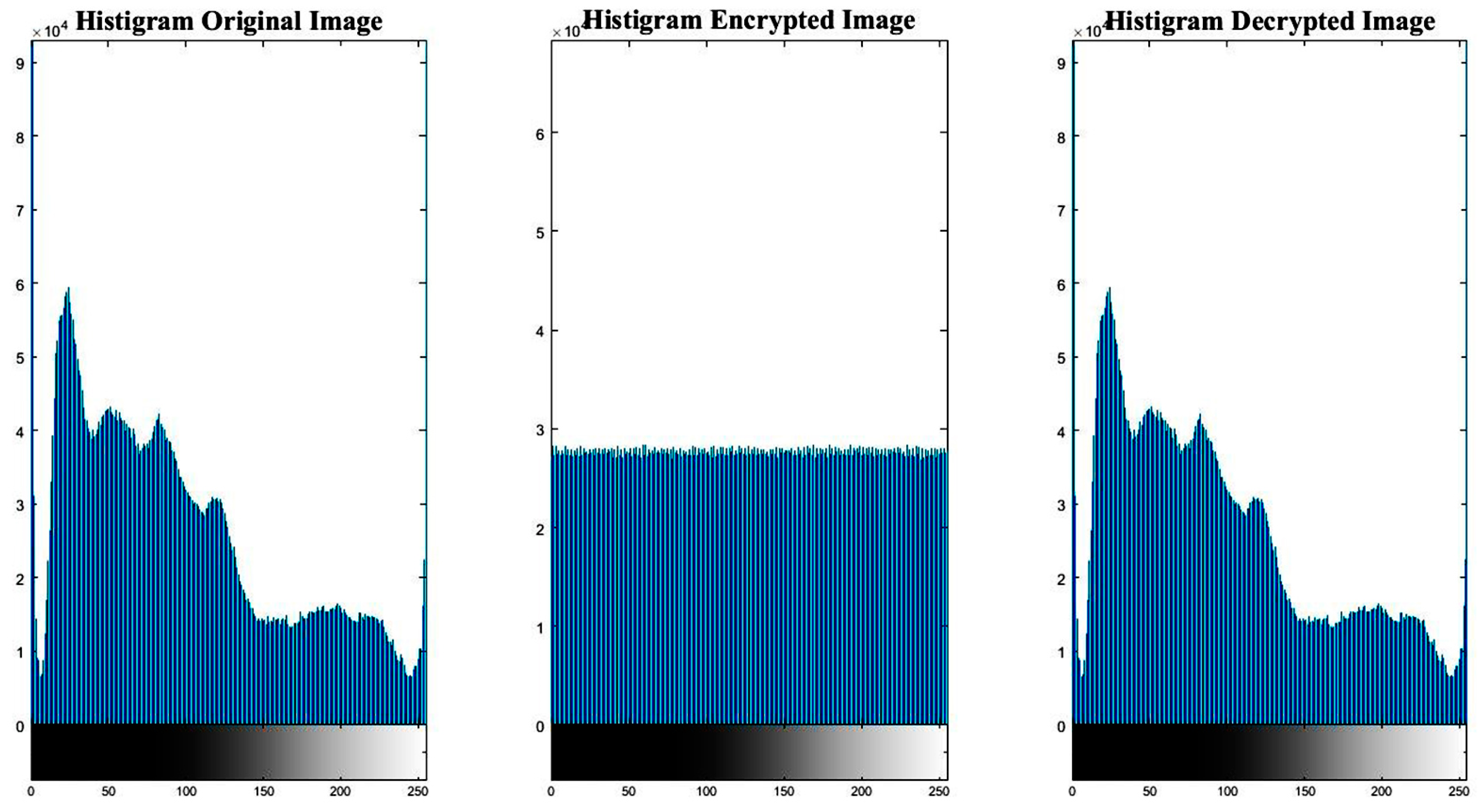
| Images | Histogram | Correlation | Differential Attack | PSNR | Information Entropy | ||
|---|---|---|---|---|---|---|---|
| Main | Decoded | NPCR (%) | UACI (%) | ||||
| Images 14 | 398,232.09375 | 398,201.1053 | 0.9923 | 99.21 | 33.55 | 8.9671 | 7.9783 |
| Images 16 | 24,466.718750 | 24,421.32934 | 0.9953 | 99.48 | 33.21 | 8.0221 | 7.9458 |
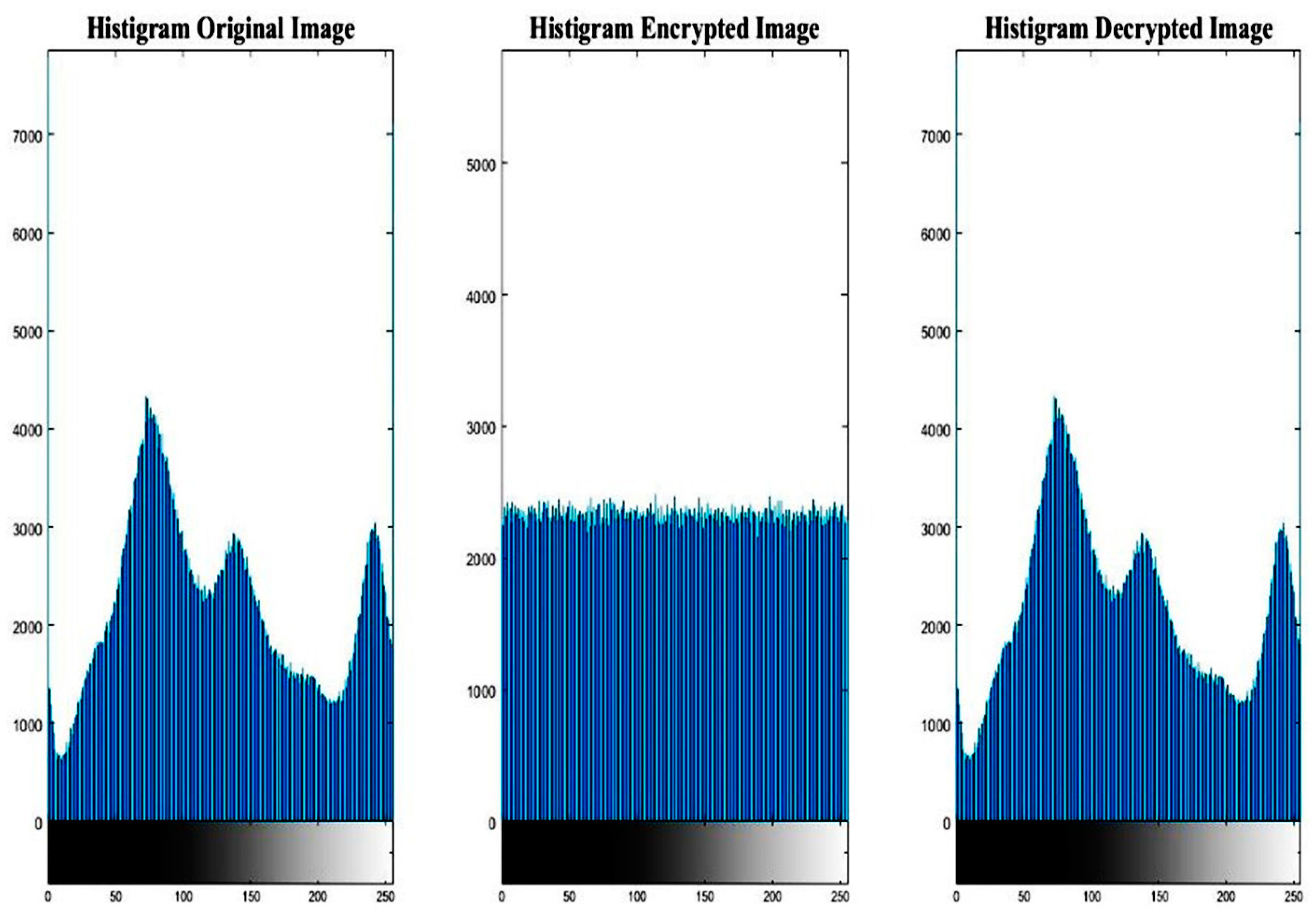
| Images | Histogram | Correlation | Differential Attack | PSNR | Information Entropy | ||
|---|---|---|---|---|---|---|---|
| Standard | Decrypted | NPCR (%) | UACI (%) | ||||
| Images 18 | 65,536 | 65,535 | 0.9986 | 99.96 | 33.46 | 9.23 | 7.9627 |
| Images 20 | 65,536 | 65,534 | 0.9987 | 99.97 | 33.47 | 9.24 | 7.9842 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rasouli, M.; Zare, A.; Yaghoubi, H.; Alizadehsani, R. Designing a Secure Mechanism for Image Transferring System Based on Uncertain Fractional Order Chaotic Systems and NLFPID Sliding Mode Controller. Axioms 2023, 12, 828. https://doi.org/10.3390/axioms12090828
Rasouli M, Zare A, Yaghoubi H, Alizadehsani R. Designing a Secure Mechanism for Image Transferring System Based on Uncertain Fractional Order Chaotic Systems and NLFPID Sliding Mode Controller. Axioms. 2023; 12(9):828. https://doi.org/10.3390/axioms12090828
Chicago/Turabian StyleRasouli, Mohammad, Assef Zare, Hassan Yaghoubi, and Roohallah Alizadehsani. 2023. "Designing a Secure Mechanism for Image Transferring System Based on Uncertain Fractional Order Chaotic Systems and NLFPID Sliding Mode Controller" Axioms 12, no. 9: 828. https://doi.org/10.3390/axioms12090828
APA StyleRasouli, M., Zare, A., Yaghoubi, H., & Alizadehsani, R. (2023). Designing a Secure Mechanism for Image Transferring System Based on Uncertain Fractional Order Chaotic Systems and NLFPID Sliding Mode Controller. Axioms, 12(9), 828. https://doi.org/10.3390/axioms12090828







