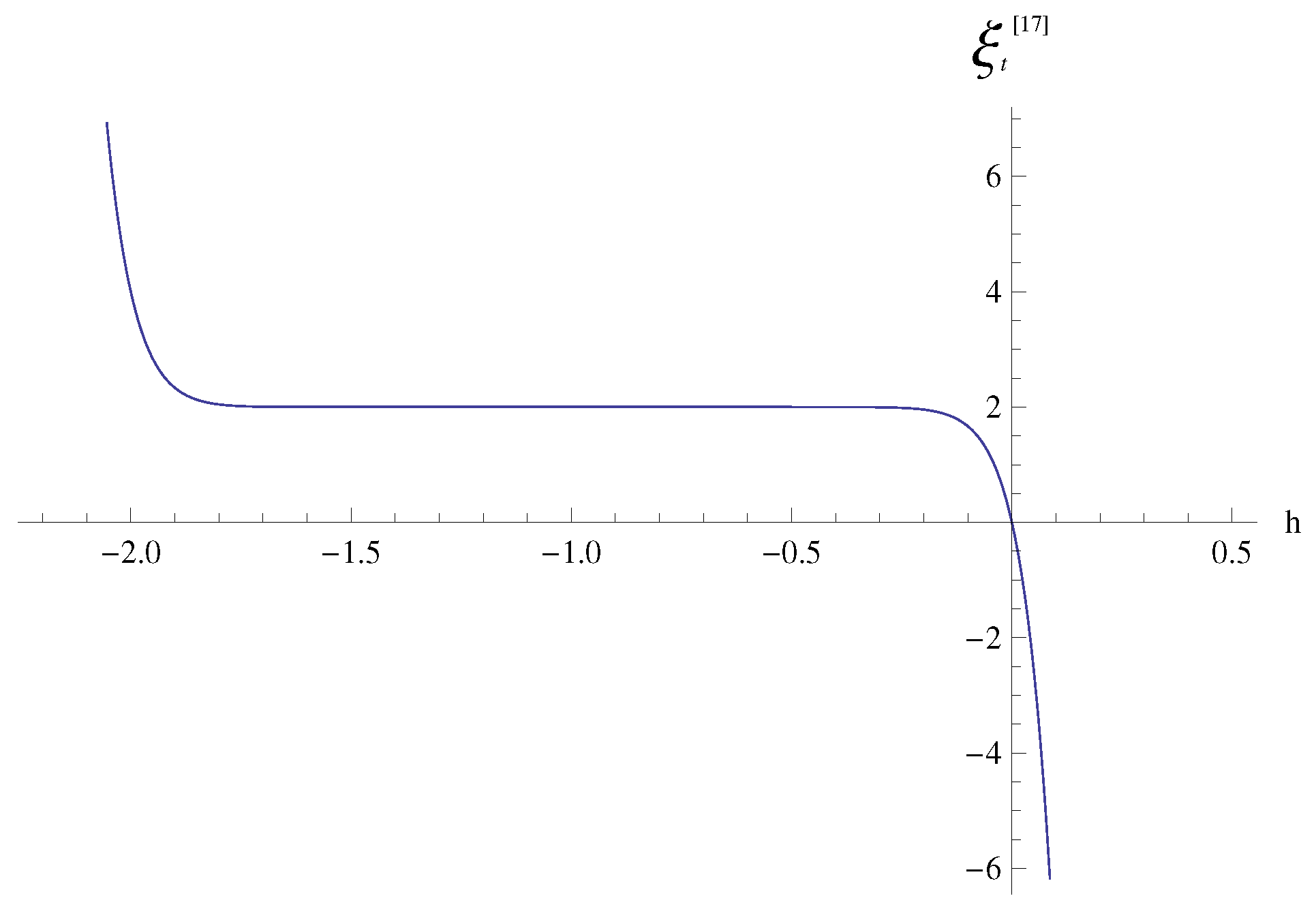1. Introduction
During the last few decades, the topic of fractional calculus, as it provides more realistic mathematical models than those obtained by the classical technique for a great deal of phenomenons in different disciplines, has captured the attention of mathematicians worldwide [
1,
2,
3,
4,
5,
6,
7,
8,
9,
10,
11,
12]. Unfortunately, obtaining the analytical form of the solution for fractional PDEs is more difficult than those obtained in integer PDEs. In the literature, there are several research articles that have been produced by many researchers, whereby different numerical techniques through which to handle the solution of fractional partial differential equation models (which appear in different applications and disciplines), were introduced, such as the Adomian decomposition method (ADM), the Laplace decomposition method (LDM), the homotopy analysis method (HAM), and the homotopy perturbation method (HPM) (see [
13,
14,
15,
16,
17,
18,
19,
20,
21]).
The
q-homotopy analysis method developed by El-Tavil and Huseen [
22,
23], where
and
n are positive integers, is a modified version of the HAM introduced by Liao [
24,
25,
26,
27,
28]. Another powerful numerical technique is the
q-homotopy analysis transform method presented in [
29,
30,
31]. This method combines the HAM and the Laplace transform method. The existence of the factor
in the power series solution that is generated by the
q-HATM leads to a faster convergence than the original version of the homotopy analysis method.
Although these techniques have been widely used recently in handling linear and nonlinear problems, it is observed that the application of the ADM and the LDM both require decomposing the nonlinear terms into an infinite sum of polynomials, called Adomian polynomials, in the problem under consideration. The evaluation of these polynomials mostly requires tedious algebraic work. On the other hand, the HPM is viewed as a particular case of the HAM, which is achieved by setting the parameter . Thus, all results generated by the HPM can also be reproduced by the HAM as a particular case. Moreover, the HPM requires a good enough initial guess to ensure convergence, which is not necessary for the HAM as it involves the parameter h, which enables one to control the convergence region. Also, for some strongly nonlinear problems, it fails to produce a convergent series solution; meanwhile, in HAM, the parameter h can be used to ensure the convergence of the power series solution for such problems. Thus, the HAM is a powerful technique that overcomes all the drawbacks of the abovementioned methods. In fact, the q-HATM is a modification of the HAM, and besides all the advantages inherited from the HAM, it involves the factor in its series solutions, which accelerates the convergence.
Let us mention that, in the theory of the HAM, there is no theoretical technique that can be easily used to obtain the range of the auxiliary parameter h. But, in some limited cases (when a closed form of the series solution is obtained), these values can be determined by testing the convergence of this series. Unfortunately, most closed form series solutions are not obtained in practice, and the solutions are approximated by truncated ones. Therefore, the range of this parameter can be determined graphically using the h-curve, and is based on approximate truncated series solutions. Furthermore, the range on which the h-curve occurs is almost horizontal.
In this article, we will explore the efficiency of the
q-HATM in solving, subject to a Dirichlet non-local condition of an integral type, a singular fractional IBVP. Namely, we will consider the numerical solution of the following fractional-order parabolic equation:
where
and
are known functions, and
is an operator given by
, in which
denotes the fractional derivative in the Caputo sense of order
which is defined by the following [
7,
8]:
where
n is a positive integer and
denotes the Gamma function.
The Laplace transform
of the Caputo fractional derivative
is defined as follows [
6,
32]:
where
denotes the Laplace transform of the function
.
Model (
1) arises in a great deal of applications, such as control theory, aerodynamics, biology, viscoelasticity, quantum mechanics, nuclear physics, etc. (see [
33,
34,
35,
36,
37,
38,
39,
40,
41,
42] and the references therein). For the proof regarding that Problem (
1) is well posed, we refer the reader to [
43,
44].
The rest of this paper is structured in the following way: In
Section 2, we provide the basic ideas of the
q-HATM. In
Section 3, we employ the
q-HATM to numerically solve Problem (
1), as well as provide some numerical examples to test the power and validity of this method in handling the solution of this problem. Finally, we provide some comments and conclusions in
Section 4.
2. Basic Concepts of the q-HATM
Consider a general fractional PDE as follows:
in which
denotes the fractional Caputo derivative of order
,
R is a linear differential operator, while
stands for the nonlinear one, and
is a known function. Performing the Laplace transform for Equation (
3) provides
that is,
Then, according to the HAM method [
24], a nonlinear operator
can be defined as
where
and
are the real valued functions in
, and
q. Thus, we take the zeroth-order deformation equation
where
is a nonzero auxiliary function,
ℏ is a non vanishing parameter (which enables one to adjust the convergence of the required series solution),
is an embedding parameter,
denotes the Laplace transform operator,
is an initial guess for the exact solution
, and
is an unknown function.
It is clear that when we put
and
in Equation (
4), it implies the following:
Thus, as
q deforms continuously from 0 to
, the function
converges from the initial approximation
to the exact solution
.
Now, the expansion of
in a Taylor series with respect to
q leads to
where
As Liao pointed out in [
26], if the parameter
ℏ, the operator
, the initial guess
, and the auxiliary function
are well chosen, then Power series (
5) will converge at
to one of the solutions of the original problem, which is given in a power series form as follows:
In fact, these four components are presented in the zeroth deformation equation on which the success of the method relies. The choice of the operator depends on the equation to be solved, and usually it is chosen out of the operators in a problem that can be simply inverted. While the existence of the convergence control parameter ℏ distinguishes the HAM from other competent methods, it also occurs in the series solution, and it is used to adjust the convergence region. Thus, its permissible range can be predicted graphically by using the ℏ-curves. Moreover, one has a great degree of freedom to choose its value from this range.
The function is chosen in such a way that the zeroth deformation equation produces the analytic solution as q, which deforms from 0 to . Finally, the initial guess can be chosen depending on the operator, as well as by the initial or boundary conditions in the problem.
Now, we differentiate the equation of the zeroth-order deformation Equation (
4)
k-times with respect to
q, by dividing by
, and by then setting
, which produces the
-order deformation equation
where
and
Next, by performing the inverse Laplace transform to Equation (
6), the components
can then be determined recursively by the iterative scheme
where
3. Application of the Method
The existence of the non-local integral condition in Problem (
1) makes computations very complicated. Thus, to overcome this issue and to investigate the applicability and efficiency of the
q-HATM for solving this problem, we transformed the integral condition in (
1) to equivalent classical conditions. To this end, assume that
and
. Then, multiply the equation
by
x, and integrate over the interval
. This implies
or equivalently
which implies
.
Conversely, assume that
and
. Again, multiply Equation (
7) by
x, and integrate over the interval
. Then, in view of Equation (
8), we conclude that
, which means
for all
, where
c is a constant.
In particular we have , since the initial condition satisfies the compatibility condition, which implies
Therefore, the condition
in Problem (
1) is equivalent to the conditions
and
. Hence, Problem (
1) is equivalent to
provided that
, where
, and
are the given functions.
Now, in view of (
2) taking a Laplace transform of both sides of the PDE in (
9), this implies the following:
Thus, we take the operator
as follows:
Hence, choosing the auxiliary function
implies that the
-order deformation equation is given as
where
Therefore, the successive terms of the approximate series solution can be computed recursively through the scheme
and the solution will be given as follows:
Next, we test the efficiency of Scheme (
10) in handling the numerical solutions of fractional problems of Type (
9) by considering the following set of examples:
Example 1. Consider the homogeneous fractional order PDEannexed with the conditions Solution.
Since the equation is homogeneous, it is evident that , as .
Now, let
, and since
in view of (
10), we obtain the following:
If we continue in this manner, we obtain
thus, the numerical solution is as follows:
Now, if the parameter
ℏ satisfies
, then Series (
11) converges, and we obtain the following series solution:
Let us mention that at
, the last series converges to the analytical solution
.
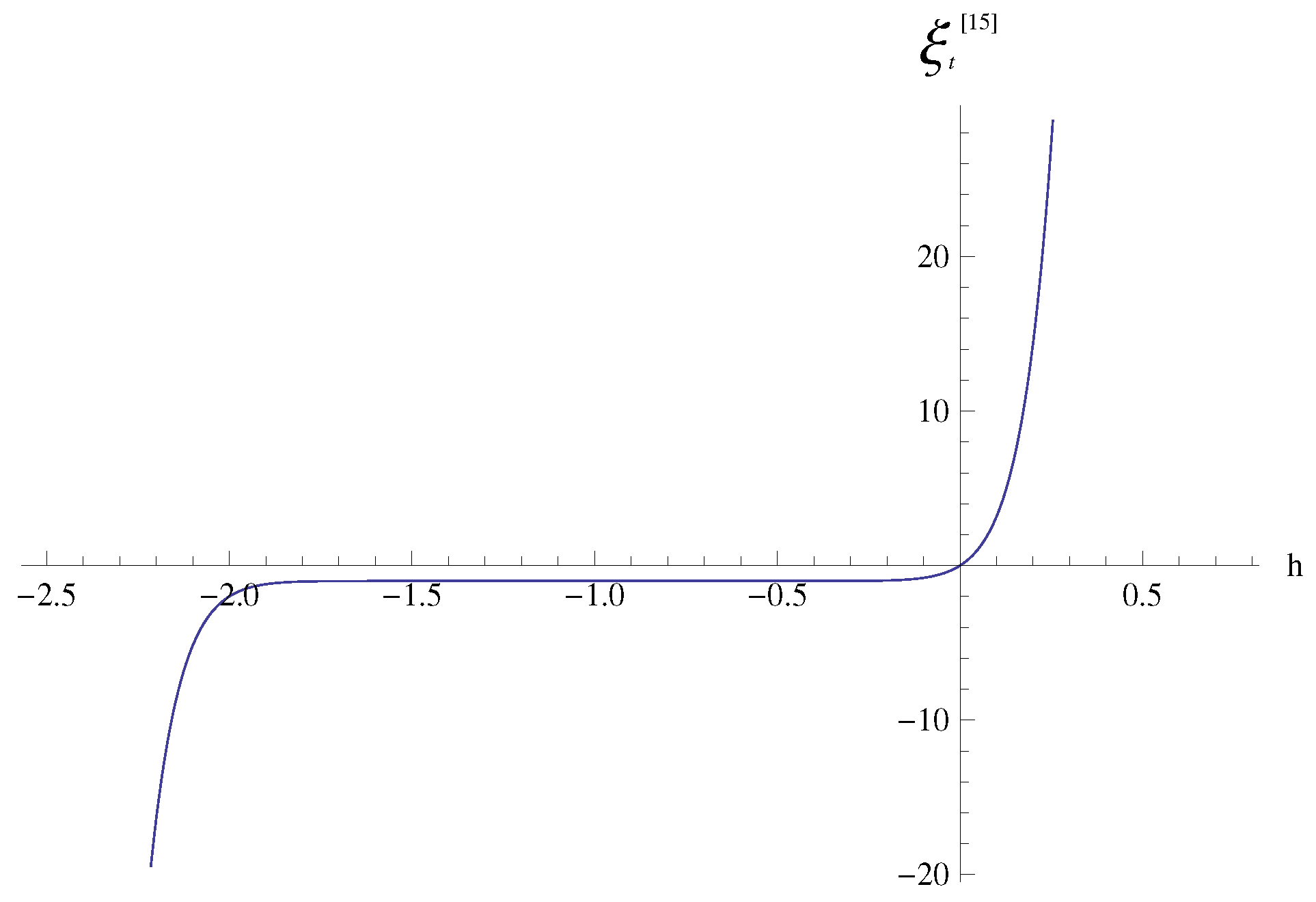
Figure 1 displays the
h-curve that corresponds to the
-order numerical series solution, and this shows that the values of
ℏ that lead to a convergent series solution are located in the interval
.
Figure 2 depicts the graphs of the truncated series solution, which used various number of terms together with the corresponding exact solution of Example 1 at
, and
. This shows that the truncated series solution of order
almost coincides with the analytical solution, which indicates the rapid convergence of this method.
In
Table 1,
Table 2 and
Table 3, we present the numerical solutions of Example 1 that result from the
-order truncated series solution
for the many values of
k, with
at various values of
,
x, and
t. As we can see, the results in these tables illustrate the rapid convergences of the generated series solution, which occur just after a few terms.
Table 4 shows the error resulting from the absolute difference
of the analytical solution and the numerical truncated series solution of Example 1 for various values of
x and
t. It illustrates the rapid convergence of the numerical solution that is obtained by utilizing the
q-HATM.
Example 2. Consider the nonhomogeneous fractional-order partial differential equationannexed with the following conditions: Solution.
Here ; hence, it is easy to check that .
Now, let
. Since
in view of (
10), we obtain the following:
If we continue in this manner, we obtain
hence the following:
Now, if the parameter
ℏ satisfies
, then (
12) converges, and we obtain the following:
It is clear that at
, and the last series converges to the exact solution
.
Figure 3 displays the
h-curve that corresponds to the
-order numerical series solution. In addition, it shows that the values of
ℏ that lead to a convergent series solution are located in the interval
.
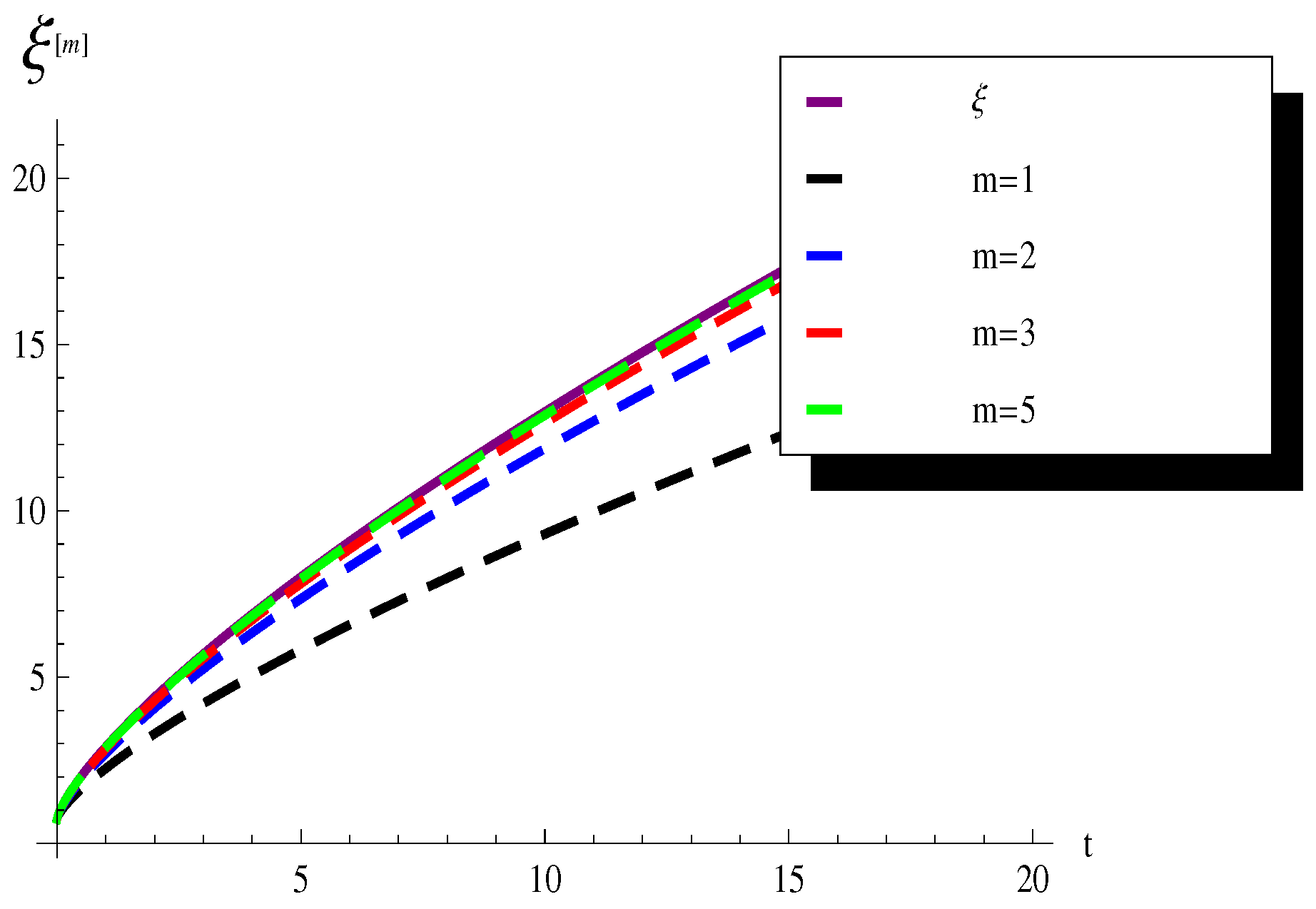
Figure 4 depicts the graphs of the truncated series solution that use various numbers of terms together with the corresponding analytical solution of Example 2 at
, and
. This shows that the truncated series solution of order
almost coincides with the analytical solution, which indicates the rapid convergence of this method.
In
Table 5,
Table 6 and
Table 7, we present the approximate solutions of Example 2 that result from the
-order series solution
for the many values of
k,with
at various values of
,
x, and
t. As we can see, the results in these tables illustrate the rapid convergences of the generated series solutions.
Table 8 shows the error that results from the absolute difference
of the analytical solution, as well as the numerical truncated series solution of Example 1 for the various values of
x and
t. This illustrates the rapid convergence of the numerical solution that results from utilizing the
q-HATM.
Example 3. Consider the nonhomogeneous fractional order partial differential equation:annexed with the conditions: Solution.
Here, ; hence, it is easy to see that .
Next, let
. Since
in view of (
10), we obtain the following:
If we continue in this way we obtain
hence
Now, if the parameter
ℏ satisfies
, then Series (
13) converges, and we obtain the following:
It is clear that at
, the last series converges to the exact solution
.
Figure 5 displays the
h-curve that corresponds to the
-order numerical series solution. Furthermore, it shows that the values of
ℏ that lead to a convergent series solution are located in the interval
.
Figure 6 depicts the graphs of the truncated series solution that use various numbers of terms together with the corresponding exact solution of Example 3 at
, and
. This shows that the truncated series solution of order
almost coincides with the analytical solution, which indicates the rapid convergence of this method.
In
Table 9,
Table 10 and
Table 11, we present the approximate solutions of Example 3 that are produced by an
-order series solution
for many values of
m with
, which is achieved by using various values of
,
x, and
t. As we can see, the results in these tables illustrate the rapid convergences of the generated series solutions.
Table 12 shows the error that results from the absolute difference
of the analytical solution, as well as the numerical truncated series solution of Example 1 for various values of
x and
t. It illustrates the rapid convergence of the numerical solution that results from utilizing the
q-HATM.