Handling a Commensurate, Incommensurate, and Singular Fractional-Order Linear Time-Invariant System
Abstract
1. Introduction
2. Adomain Decomposition Method
3. FoLTI System
3.1. Commensurate FoLTI System
3.2. Incommensurate FoLTI System
3.3. Singular FoLTI System
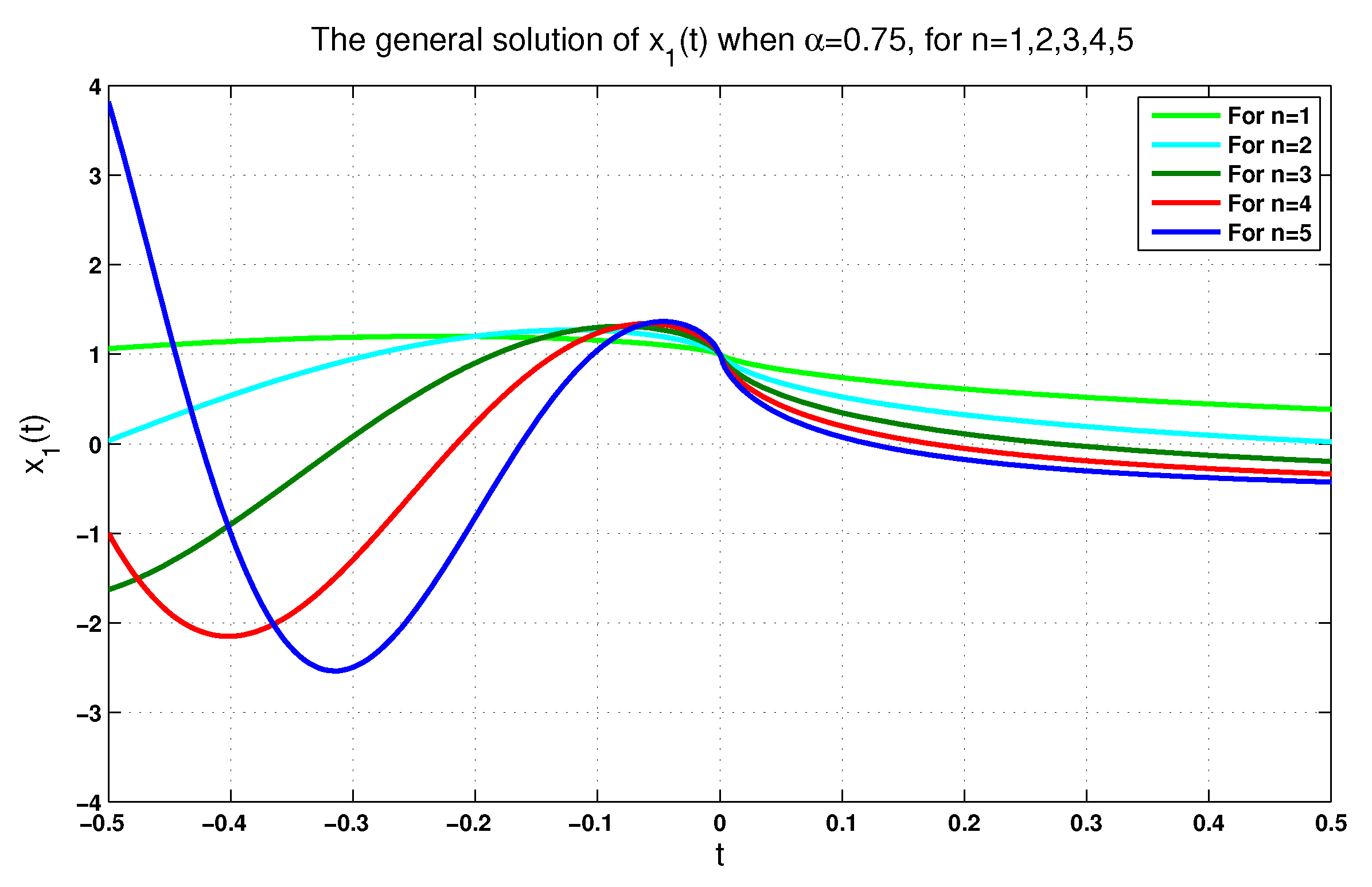
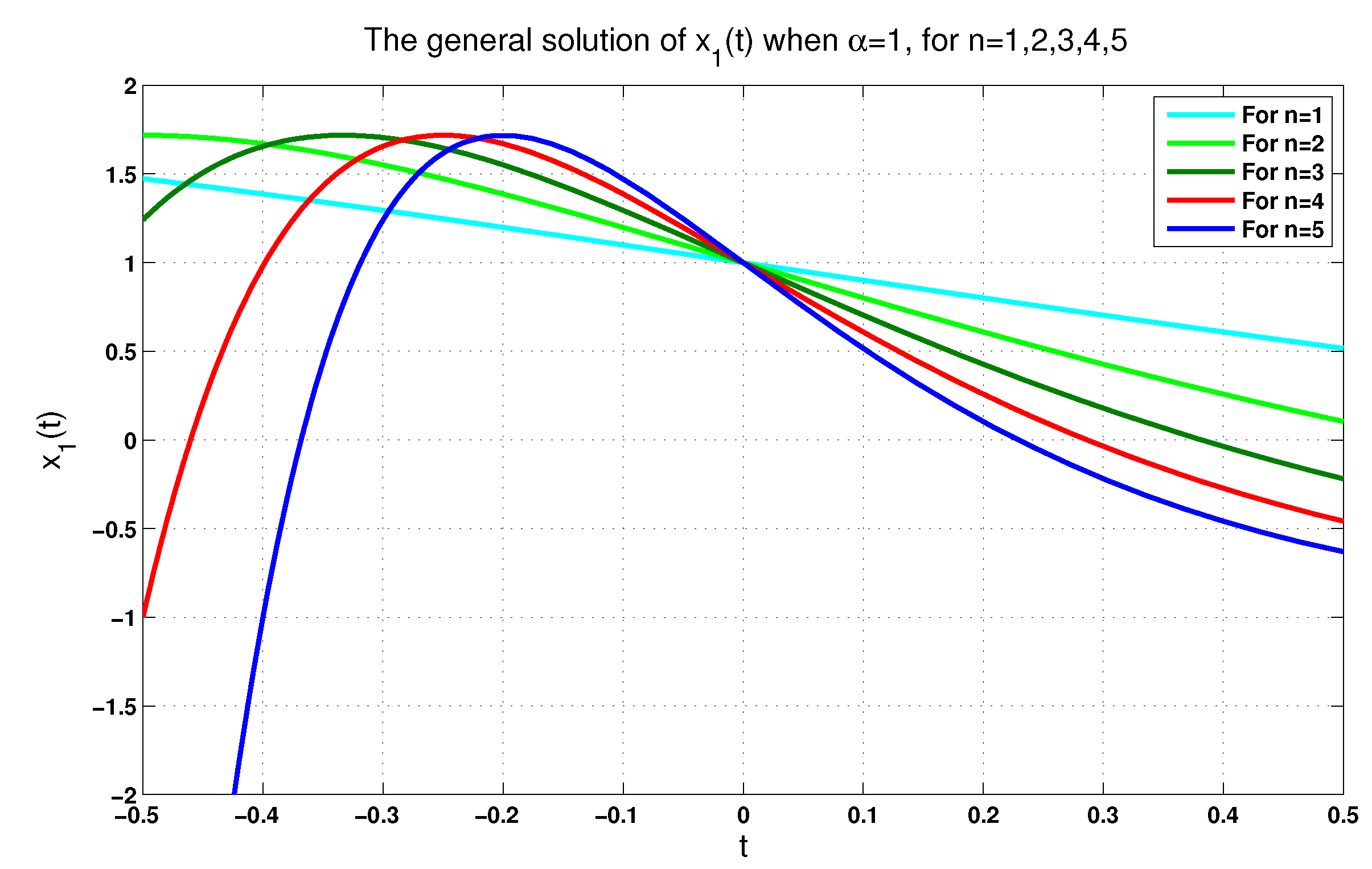
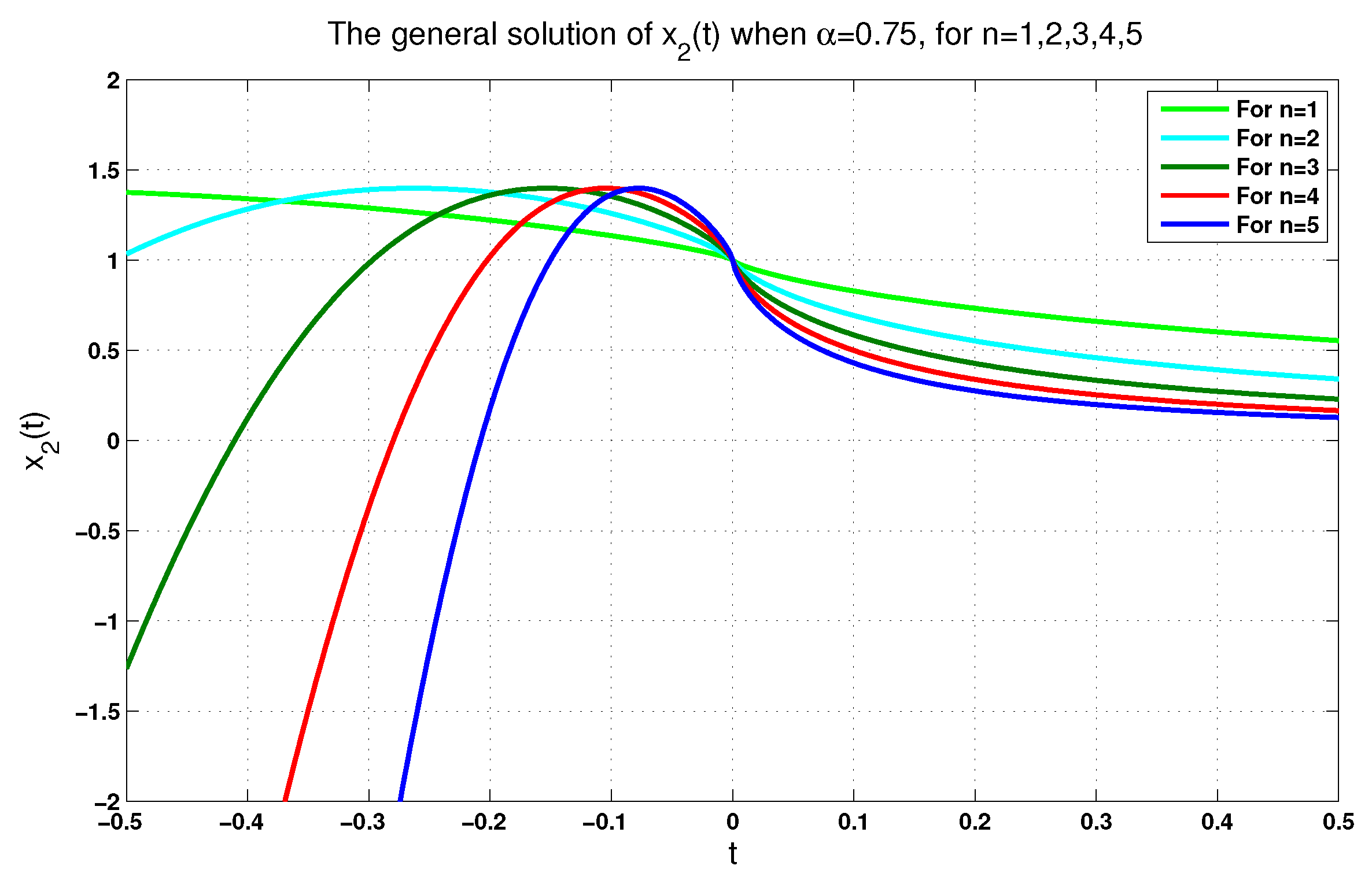
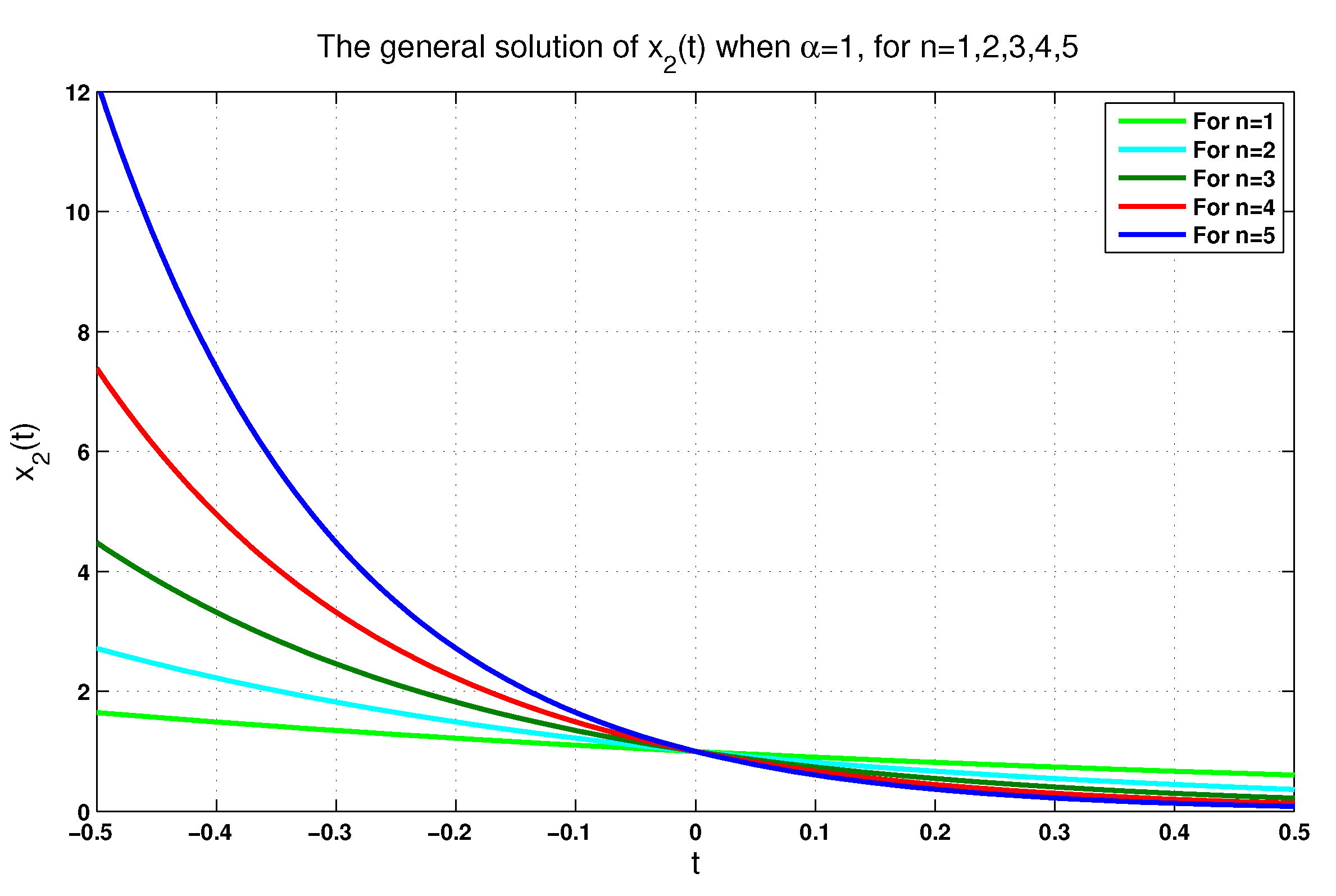
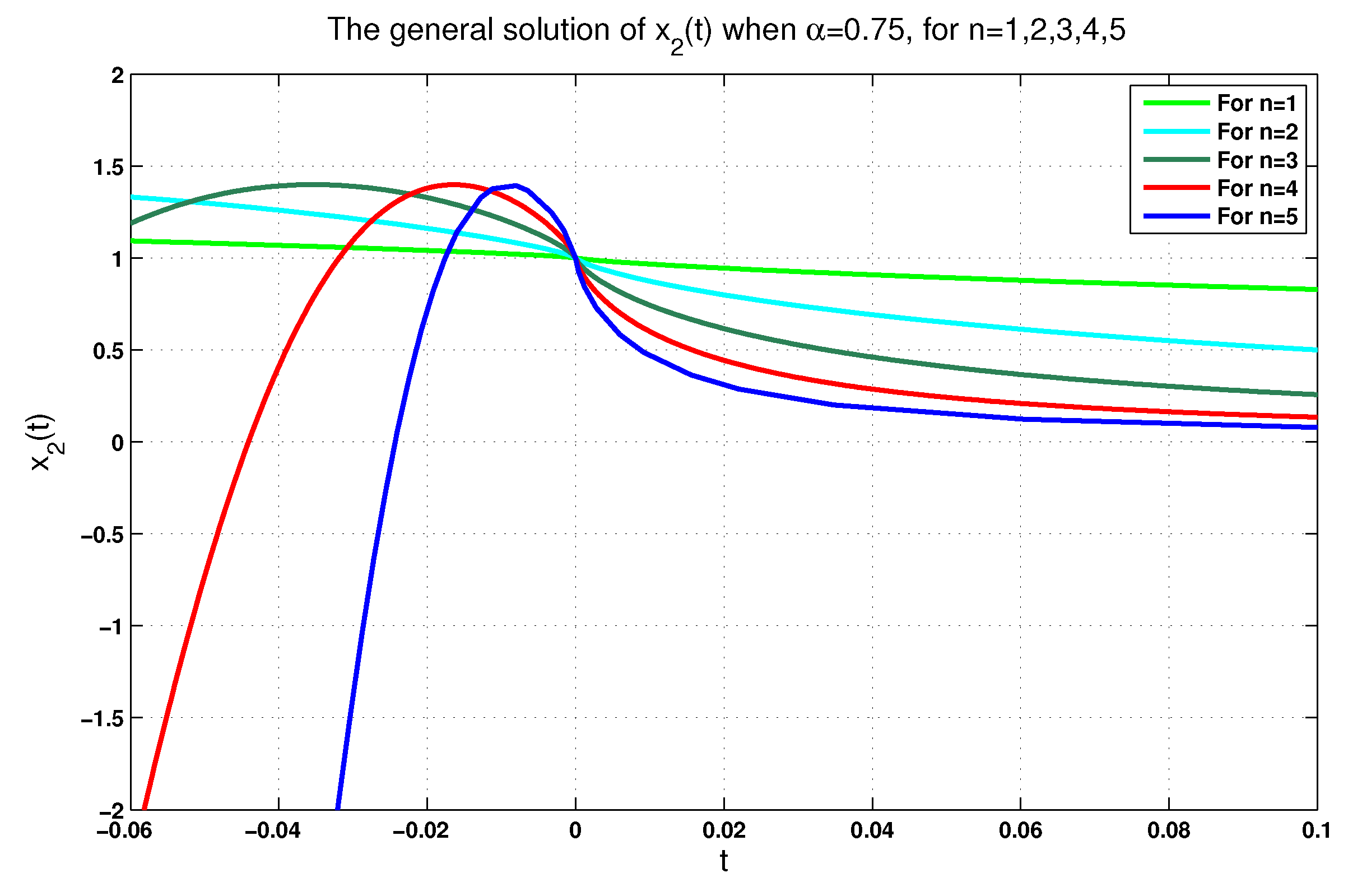
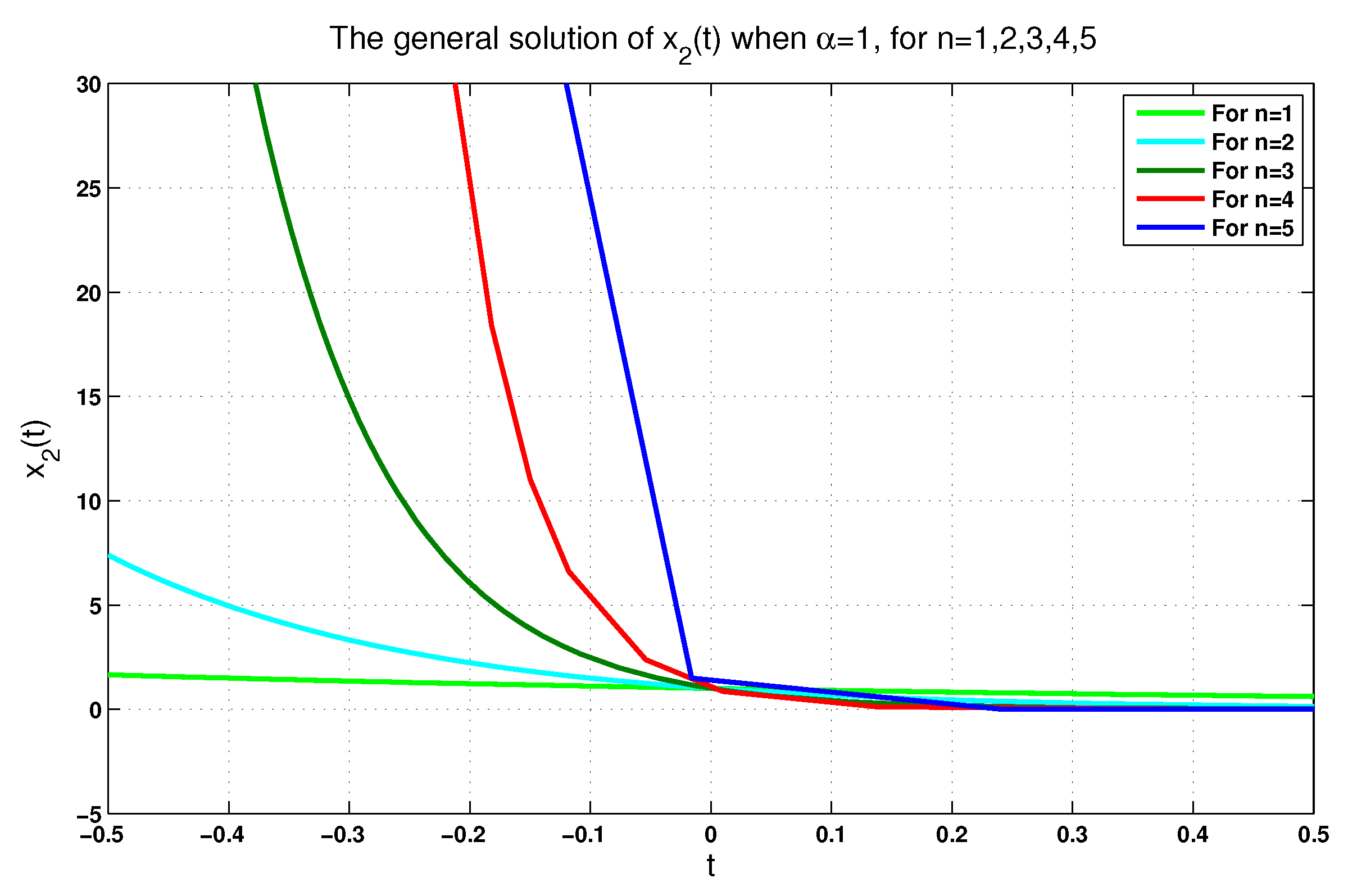
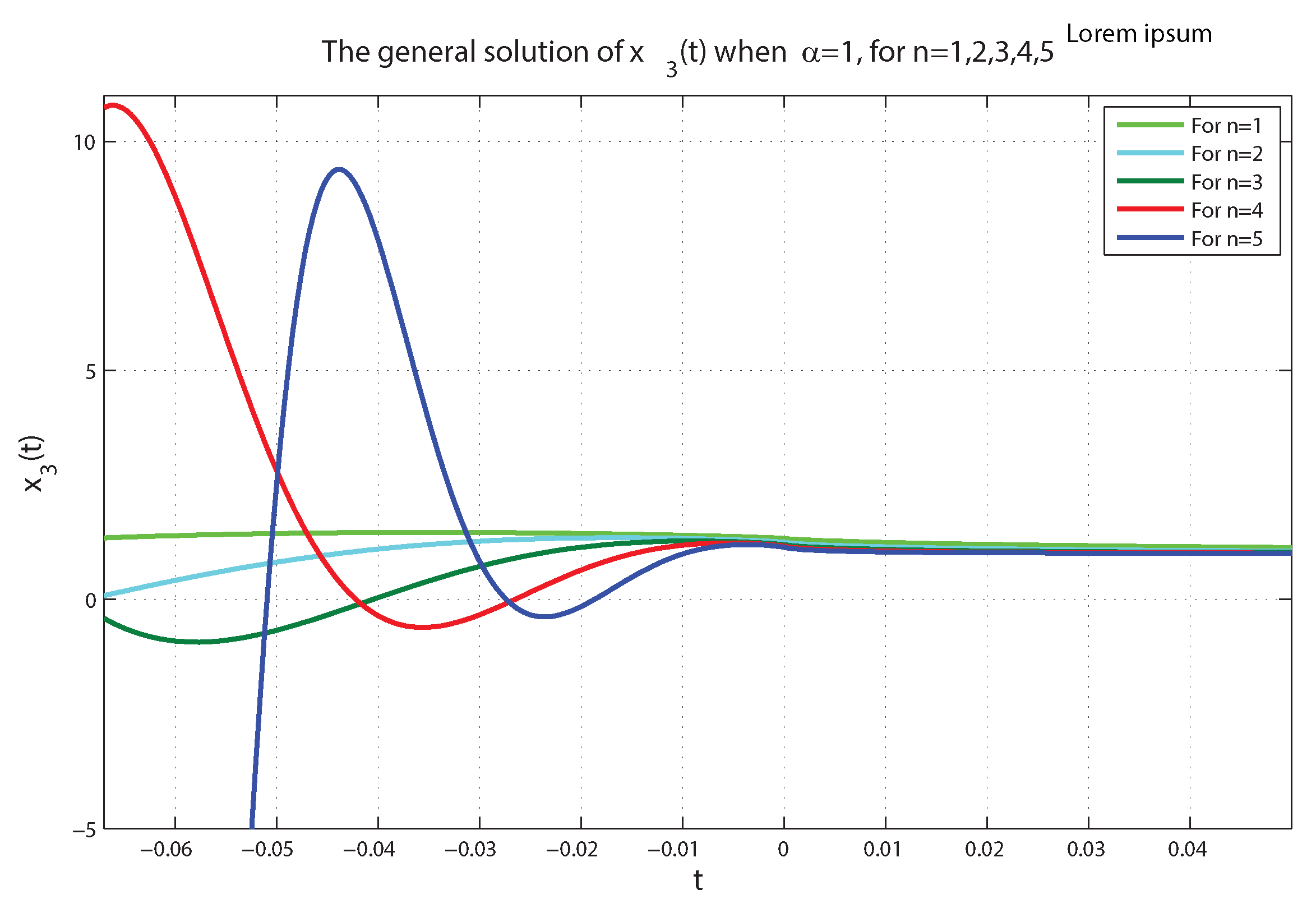
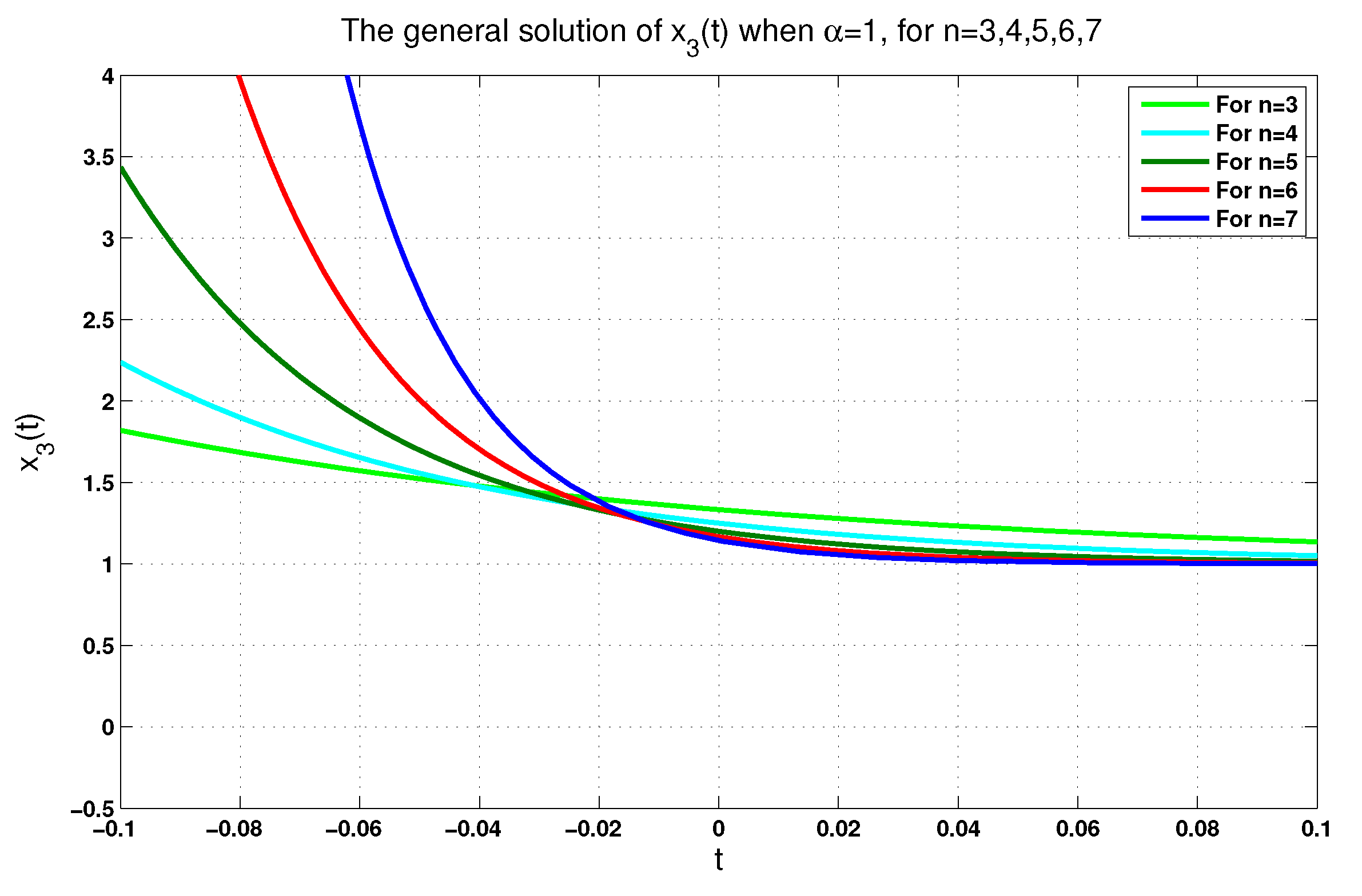
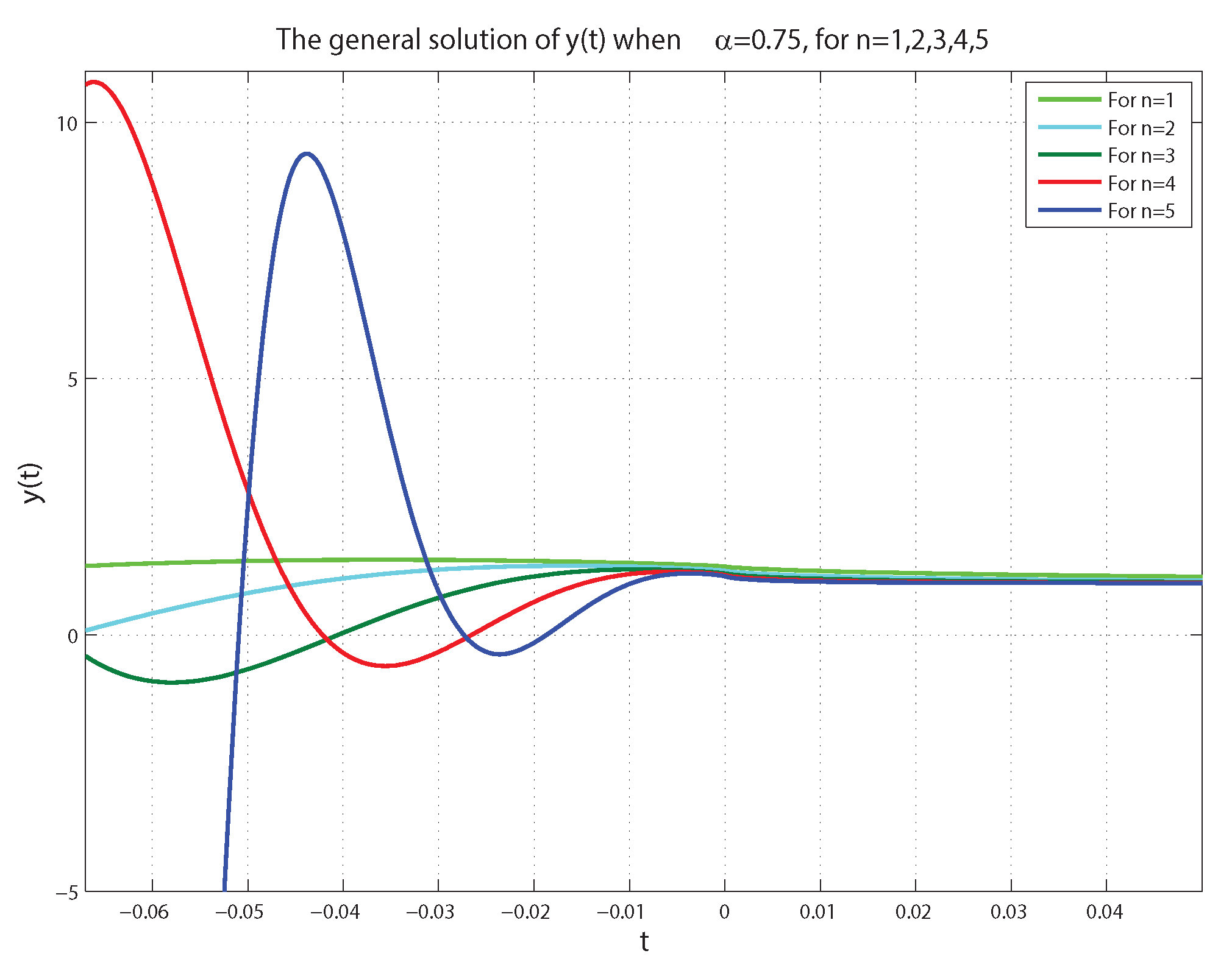
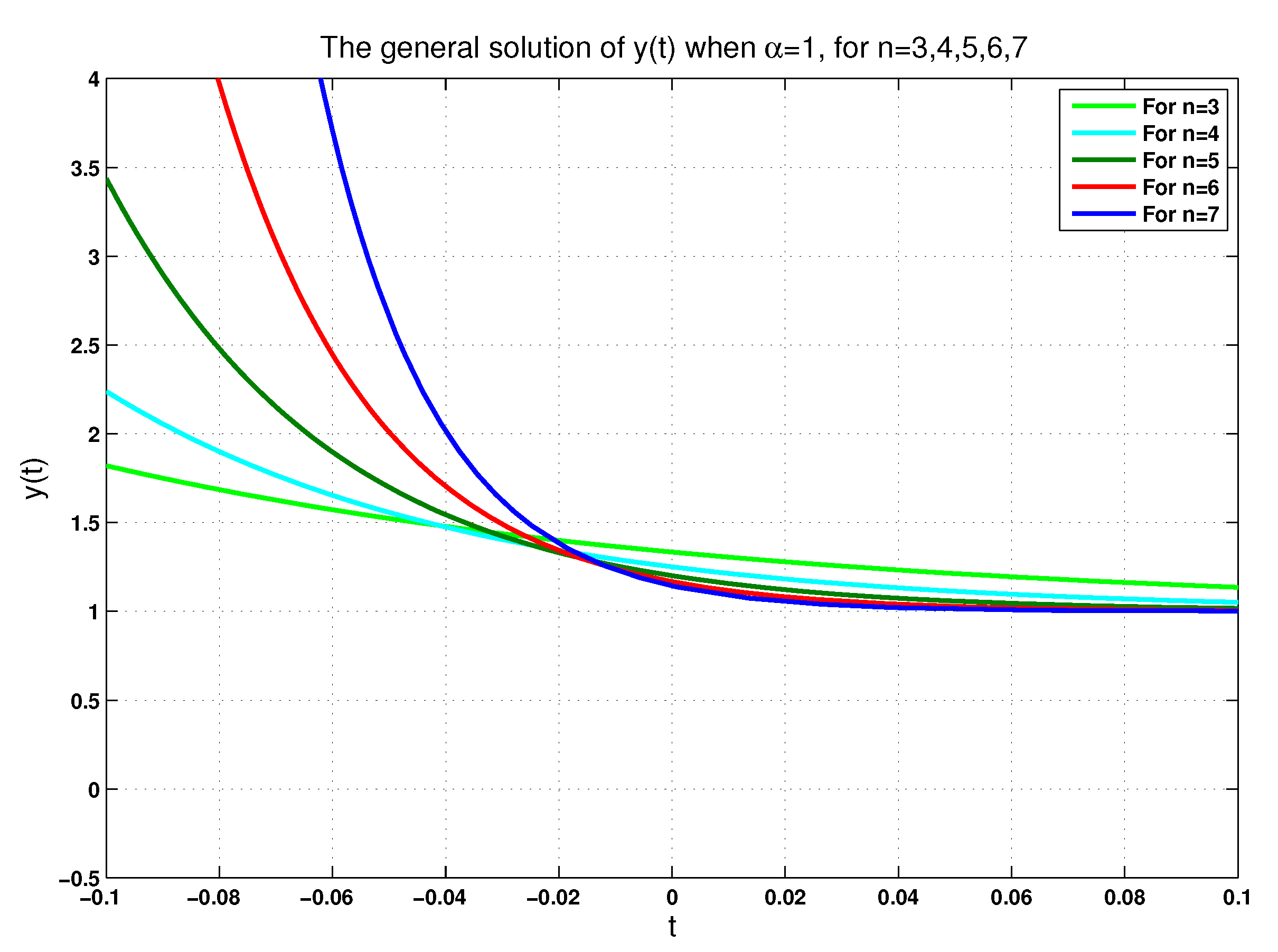
4. Illustrative Examples
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Batiha, I.; Alshorm, S.; Jebril, I.; Hammad, M. A Brief Review about Fractional Calculus. Int. J. Open Probl. Comput. Sci. Math. 2022, 15, 39–56. [Google Scholar]
- Batiha, I.M.; Obeidat, A.; Alshorm, S.; Alotaibi, A.; Alsubaie, H.; Momani, S.; Albdareen, M.; Zouidi, F.; Eldin, S.M.; Jahanshah, H. A Numerical Confirmation of a Fractional-Order COVID-19 Model’s Efficiency. Symmetry 2022, 14, 2583. [Google Scholar] [CrossRef]
- Batiha, I.M.; Ababneh, O.Y.; Al-Nana, A.A.; Alshanti, W.G.; Alshorm, S.; Momani, S. A Numerical Implementation of Fractional-Order PID Controllers for Autonomous Vehicles. Axioms 2023, 12, 306. [Google Scholar] [CrossRef]
- Rania, S.; Qazza, A.; Burqan, A.; Al-Omari, S. On Time Fractional Partial Differential Equations and Their Solution by Certain Formable Transform Decomposition Method. Comput. Model. Eng. Sci. 2023, 136, 3121–3139. [Google Scholar]
- Bezziou, M.; Jebril, I.; Dahmani, Z. A new nonlinear duffing system with sequential fractional derivatives. Chaos Solitons Fractals 2021, 151, 111247. [Google Scholar] [CrossRef]
- Mathieu, B.; Lay, L.L.; Oustaloup, A. Identification of non integer order systems in the time domain. In Proceedings of the Symposium on Control, Optimization and Supervision, Lille, France, 9–12 July 1996; pp. 843–847. [Google Scholar]
- Ahmad, W.M.; El-Khazali, R.; Al-Assaf, Y. Stabilization of generalized fractional order chaotic systems using state feedback control. Chaos Solitons Fractals 2004, 22, 141–150. [Google Scholar] [CrossRef]
- Batiha, I.M.; Alshorm, S.; Ouannas, A.; Momani, S.; Ababneh, O.Y.; Albdareen, M. Modified Three-Point Fractional Formulas with Richardson Extrapolation. Mathematics 2022, 10, 3489. [Google Scholar] [CrossRef]
- Guechi, S.; Guechi, M. Taylor approximation for solving linear and nonlinear Ill-Posed Volterra equations via an iteration method. Gen. Lett. Math. 2022, 11, 18–25. [Google Scholar] [CrossRef]
- Podlubny, I. Fractional Differential Equations; Academic Press: San Diego, CA, USA, 1999. [Google Scholar]
- Shah, N.A.; Ebaid, A.; Oreyeni, T.; Yook, S.-J. MHD and porous effects on free convection flow of viscous fluid between vertical parallel plates: Advance thermal analysis. Waves Random Complex Media 2023, 1–13. [Google Scholar] [CrossRef]
- Shah, N.A.; Khan, I. Heat transfer analysis in a second grade fluid over and oscillating vertical plate using fractional Caputo–Fabrizio derivatives. Eur. Phys. J. C 2016, 75, 362. [Google Scholar] [CrossRef]
- Imran, M.A.; Khan, I.; Ahmad, M.; Shah, N.A.; Nazar, M. Heat and mass transport of differential type fluid with non-integer order time-fractional Caputo derivatives. J. Mol. Liq. 2017, 229, 67–75. [Google Scholar] [CrossRef]
- George, A. Solving Frontier Problems of Physics: The Decomposition Method; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2013; Volume 60. [Google Scholar]
- George, A. Nonlinear Stochastic Operator Equations; Academic Press: Cambridge, MA, USA, 2014. [Google Scholar]
- Rach, R. On the Adomian (decomposition) method and comparisons with Picard’s method. J. Math. Anal. Appl. 1987, 128, 480–483. [Google Scholar] [CrossRef]
- El-Sayed, A.M.A.; Hashem, H.H.G.; Ziada, E.A.A. Picard and Adomian decomposition methods for a quadratic integral equation of fractional order. Comp. Appl. Math. 2014, 33, 95–109. [Google Scholar] [CrossRef]
- Adomian, G.; Rach, R. Inversion of nonlinear stochastic operators. J. Math. Anal. Appl. 1983, 91, 39–46. [Google Scholar] [CrossRef]
- Adomian, G.; Rach, R. Analytic solution of nonlinear boundary-value problems in several dimensions by decomposition. J. Math. Anal. Appl. 1993, 174, 118–137. [Google Scholar] [CrossRef]
- Abdul-Majid, W. A reliable modification of ADM. Appl. Math. Comput. 1999, 102, 77–86. [Google Scholar]
- Abdul-Majid, W.; El-Sayed, S.M. A new modification of the ADM for linear and nonlinear operators. Appl. Math. Comput. 2001, 122, 393–405. [Google Scholar]
- Duan, J.-S. Recurrence triangle for adomian polynomials. Appl. Math. Comput. 2010, 216, 1235–1241. [Google Scholar] [CrossRef]
- Duan, J.-S.; Rach, R. A new modification of the ADM for solving boundary value problems for higher order nonlinear differential equations. Appl. Math. Comput. 2011, 218, 4090–4118. [Google Scholar]
- Sabatier, J.; Farges, C.; Trigeassou, J.-C. Fractional systems state space description: Some wrong ideas and proposed solutions. J. Vib. Control 2014, 20, 1076–1084. [Google Scholar] [CrossRef]
- Lorenzo, C.F.; Hartley, T.T. Initialized fractional calculus. Int. J. Appl. Math. 2000, 3, 249–266. [Google Scholar]
- Maamri, N.; Trigeassou, J.-C. A Plea for the Integration of Fractional Differential Systems: The Initial Value Problem. Fractal Fract. 2022, 6, 550. [Google Scholar] [CrossRef]
- Diethelm, K. The Analysis of Fractional Differential Equations; Springer: Berlin/Heidelberg, Germany, 2004. [Google Scholar]
- Batiha, I.M.; Bataihah, A.; Al-Nana, A.A.; Alshorm, S.; Jebril, I.H.; Zraiqat, A. A numerical scheme for dealing with fractional initial value problem. Int. J. Innov. Comput. Inf. Control 2023, 19, 763–774. [Google Scholar]
- Kaczorek, T. Polynomial Approach to Fractional Descriptor Electrical Circuits; Computational Models for Business and Engineering Domains-ITHEA: Rzeszow, Poland, 2014. [Google Scholar]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Batiha, I.M.; Talafha, O.; Ababneh, O.Y.; Alshorm, S.; Momani, S. Handling a Commensurate, Incommensurate, and Singular Fractional-Order Linear Time-Invariant System. Axioms 2023, 12, 771. https://doi.org/10.3390/axioms12080771
Batiha IM, Talafha O, Ababneh OY, Alshorm S, Momani S. Handling a Commensurate, Incommensurate, and Singular Fractional-Order Linear Time-Invariant System. Axioms. 2023; 12(8):771. https://doi.org/10.3390/axioms12080771
Chicago/Turabian StyleBatiha, Iqbal M., Omar Talafha, Osama Y. Ababneh, Shameseddin Alshorm, and Shaher Momani. 2023. "Handling a Commensurate, Incommensurate, and Singular Fractional-Order Linear Time-Invariant System" Axioms 12, no. 8: 771. https://doi.org/10.3390/axioms12080771
APA StyleBatiha, I. M., Talafha, O., Ababneh, O. Y., Alshorm, S., & Momani, S. (2023). Handling a Commensurate, Incommensurate, and Singular Fractional-Order Linear Time-Invariant System. Axioms, 12(8), 771. https://doi.org/10.3390/axioms12080771






