Complexity and Chaos Analysis for Two-Dimensional Discrete-Time Predator–Prey Leslie–Gower Model with Fractional Orders
Abstract
1. Introduction
2. Model Description of the Fractional Discrete System
3. Nonlinear Dynamics of the Fractional Discrete-Time Predator–Prey Leslie–Gower Model
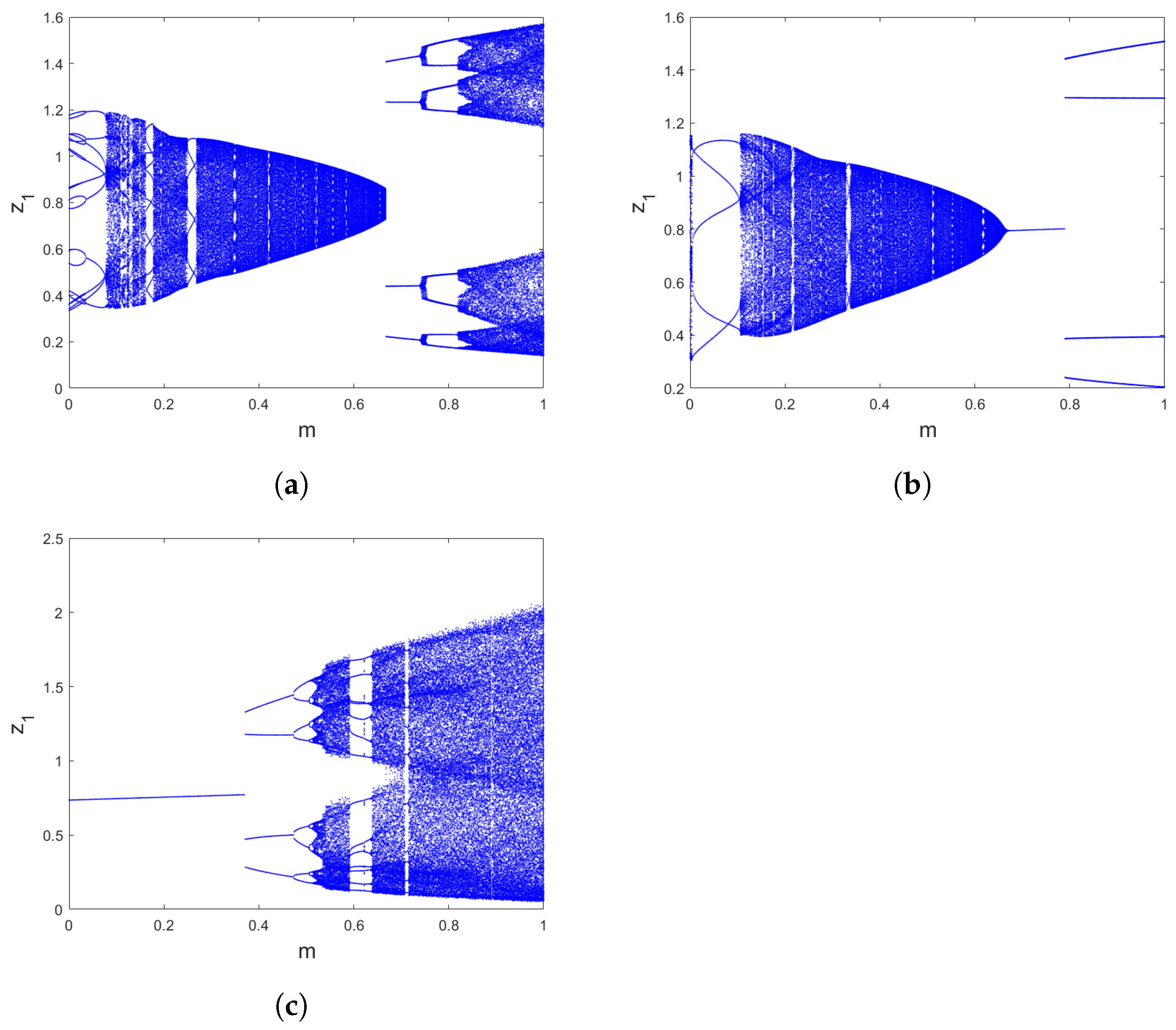
3.1. Commensurate Order FDNN Model
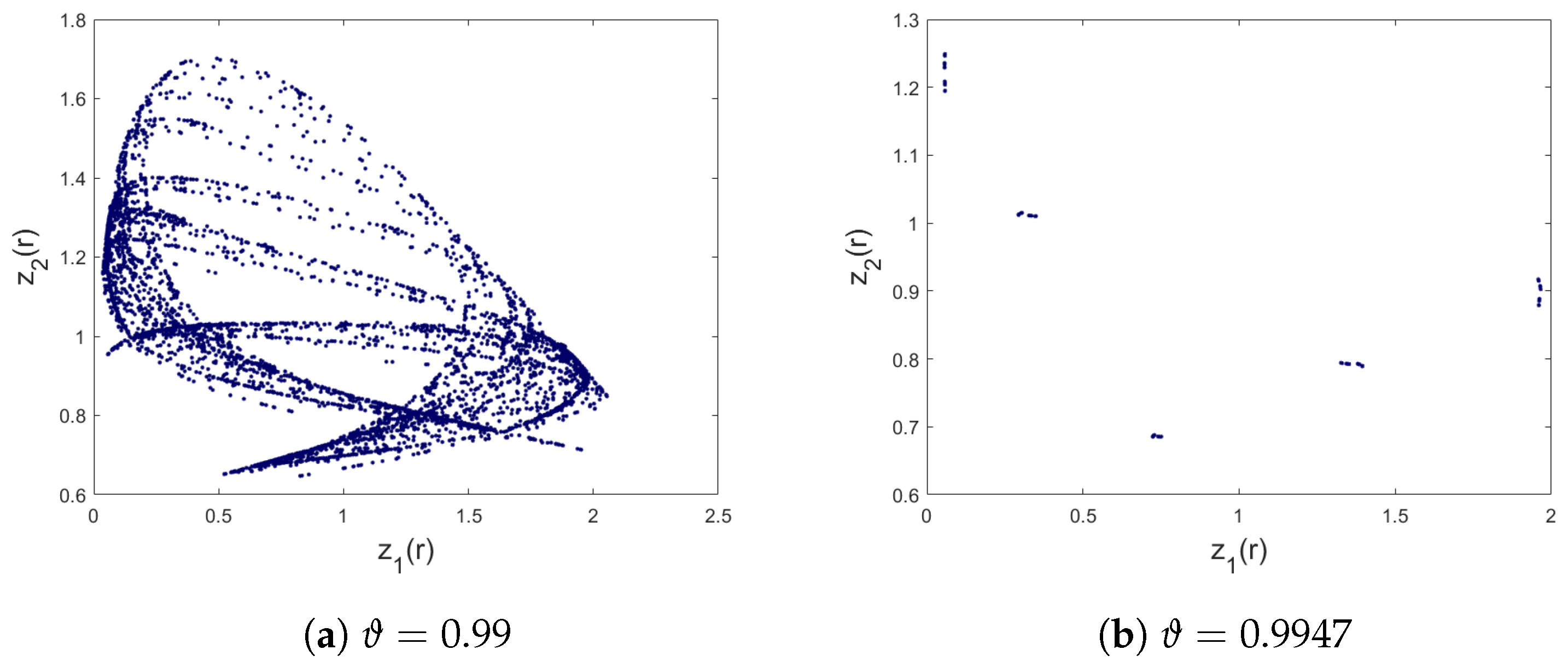
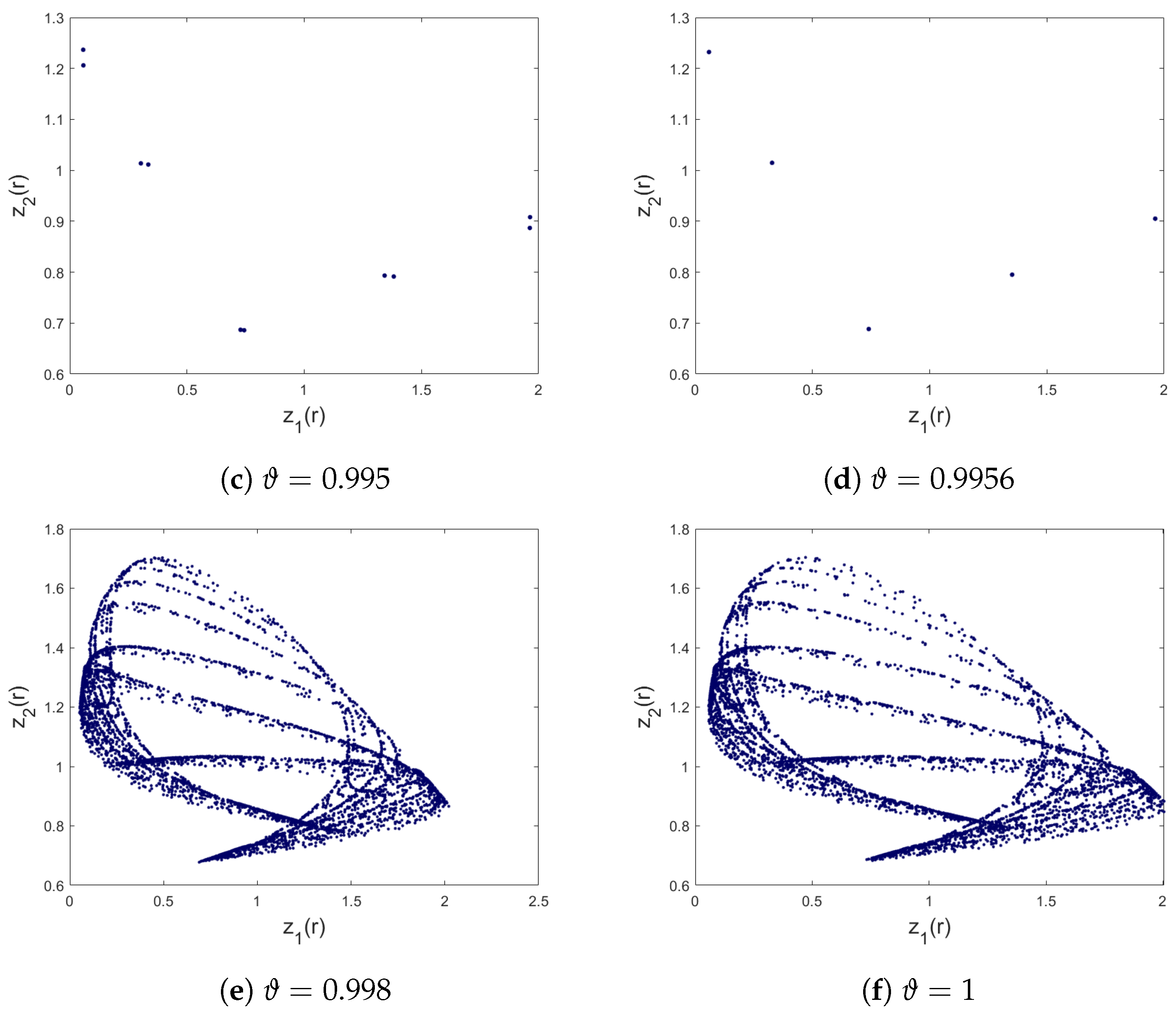
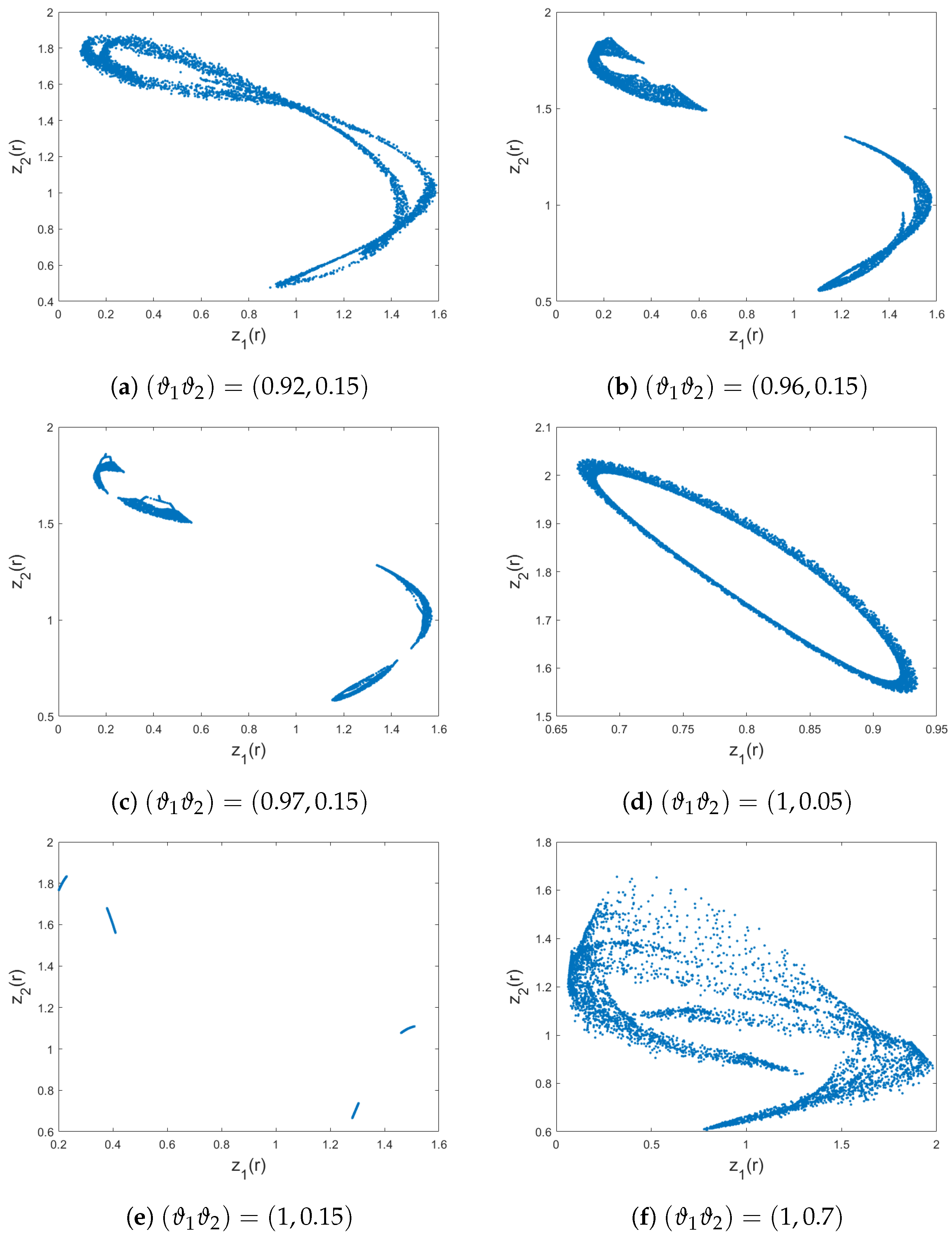
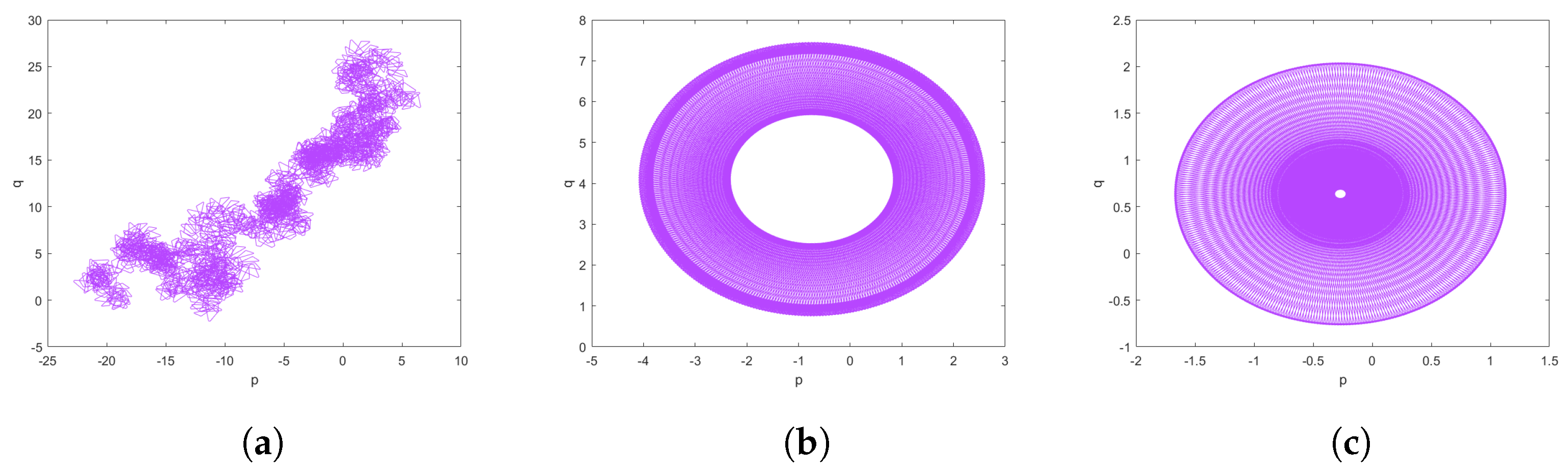
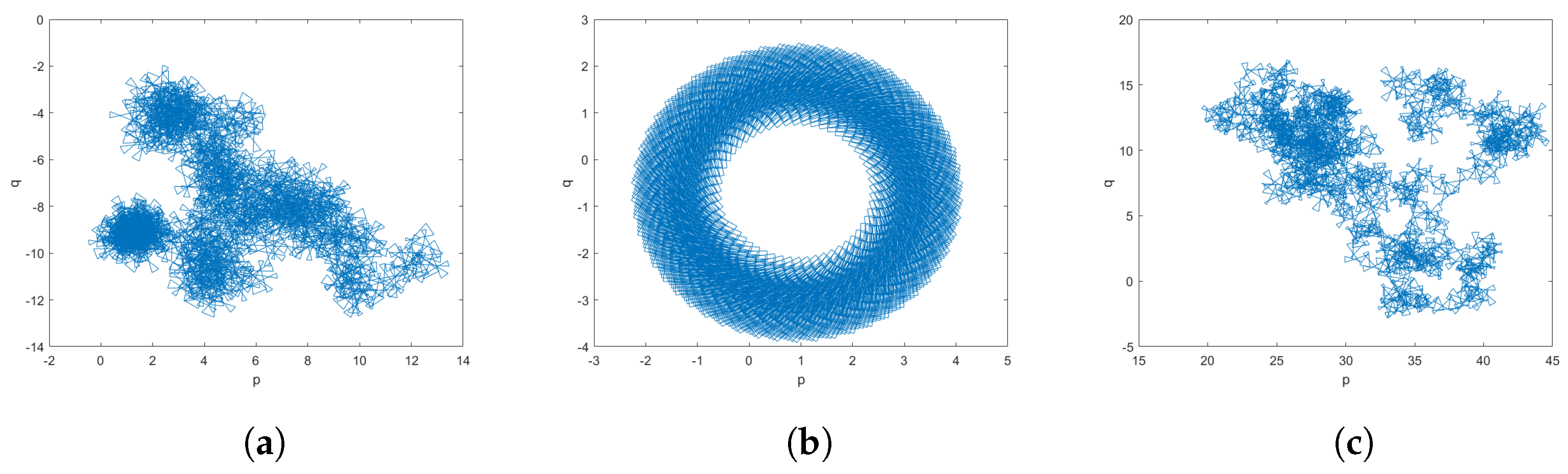
3.2. Incommensurate Fractional Discrete System
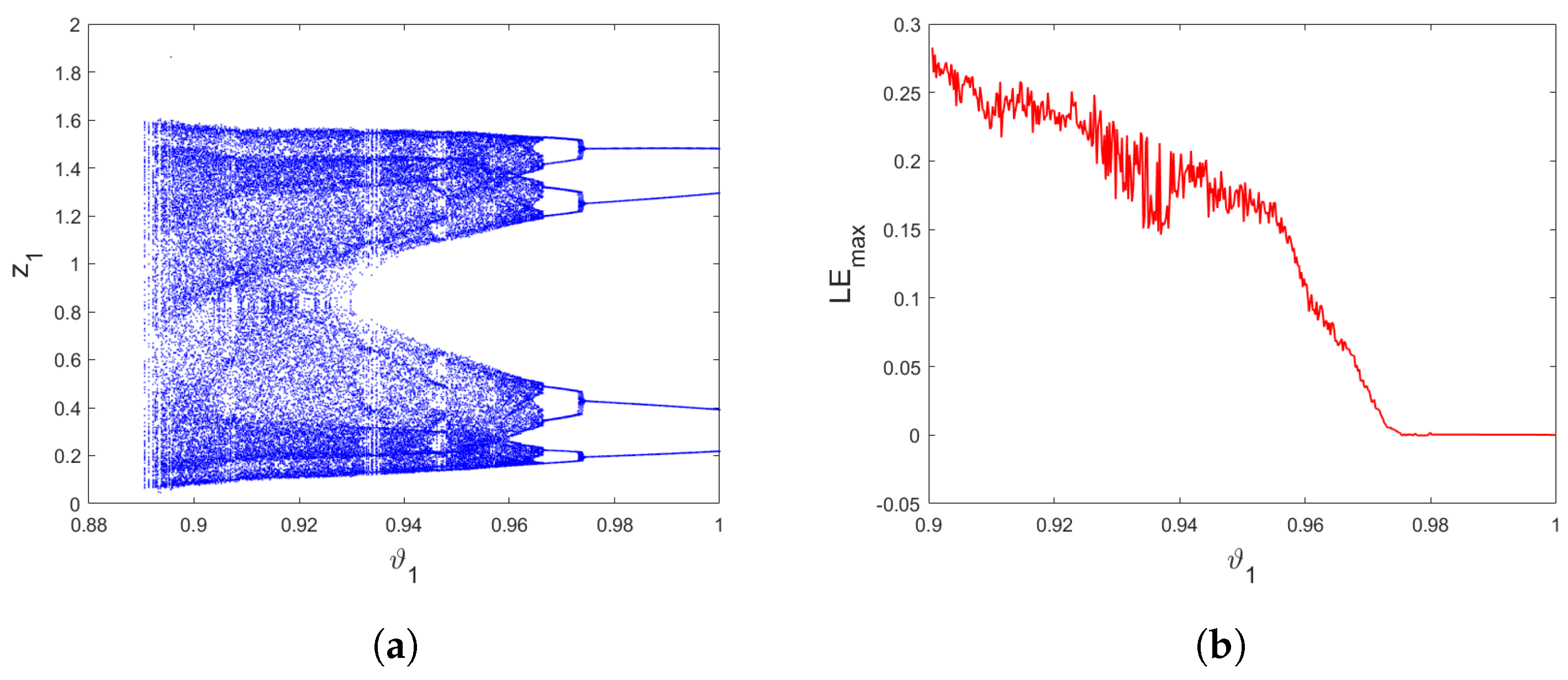
4. The 0–1 Test for Chaos and the Complexity Analysis of the Model
4.1. The 0–1 Test of the Model
4.2. The ApEn of the Model
4.3. The Complexity of the Model
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Ali, I.; Saleem, M.T. Spatiotemporal Dynamics of Reaction–Diffusion System and Its Application to Turing Pattern Formation in a Gray–Scott Model. Mathematics 2023, 11, 1459. [Google Scholar] [CrossRef]
- Atici, F.M.; Eloe, P. Discrete fractional calculus with the nabla operator. Electron. J. Qual. Theory Differ. Equ. 2009, 3, 1–12. [Google Scholar] [CrossRef]
- Anastassiou, G.A. Principles of delta fractional calculus on time scales and inequalities. Math. Comput. Model. 2010, 52, 556–566. [Google Scholar] [CrossRef]
- Abdeljawad, T. On Riemann and Caputo fractional differences. Comput. Math. Appl. 2011, 62, 1602–1611. [Google Scholar] [CrossRef]
- Hanif, A.; Kashif Butt, A.I.; Ahmad, W. Numerical approach to solve Caputo-Fabrizio-fractional model of Corona pandemic with Optimal Control Design and analysis. Math. Methods Appl. Sci. 2023, 46, 9751–9782. [Google Scholar] [CrossRef]
- He, Z.Y.; Abbes, A.; Jahanshahi, H.; Alotaibi, N.D.; Wang, Y. Fractional-order discrete-time SIR epidemic model with vaccination: Chaos and complexity. Mathematics 2022, 10, 165. [Google Scholar] [CrossRef]
- Shatnawi, M.T.; Abbes, A.; Ouannas, A.; Batiha, I.M. A new two-dimensional fractional discrete rational map: Chaos and complexity. Phys. Scr. 2022, 98, 015208. [Google Scholar] [CrossRef]
- Vignesh, D.; Banerjee, S. Dynamical analysis of a fractional discrete-time vocal system. Nonlinear Dyn. 2023, 111, 4501–4515. [Google Scholar] [CrossRef]
- Abbes, A.; Ouannas, A.; Shawagfeh, N.; Khennaoui, A.A. Incommensurate Fractional Discrete Neural Network: Chaos and complexity. Eur. Phys. J. Plus 2022, 137, 235. [Google Scholar] [CrossRef]
- Shatnawi, M.T.; Abbes, A.; Ouannas, A.; Batiha, I.M. Hidden multistability of fractional discrete non-equilibrium point memristor based map. Phys. Scr. 2023, 98, 035213. [Google Scholar] [CrossRef]
- Abbes, A.; Ouannas, A.; Shawagfeh, N.; Jahanshahi, H. The fractional-order discrete COVID-19 pandemic model: Stability and chaos. Nonlinear Dyn. 2023, 111, 965–983. [Google Scholar] [CrossRef] [PubMed]
- Batiha, I.M.; Alshorm, S.; Jebril, I.; Zraiqat, A.; Momani, Z.; Momani, S. Modified 5-point fractional formula with Richardson extrapolation. AIMS Math. 2023, 8, 9520–9534. [Google Scholar] [CrossRef]
- Butt, A.I.K.; Imran, M.; Batool, S.; Nuwairan, M.A. Theoretical Analysis of a COVID-19 CF-Fractional Model to Optimally Control the Spread of Pandemic. Symmetry 2023, 15, 380. [Google Scholar] [CrossRef]
- Abbes, A.; Ouannas, A.; Shawagfeh, N. The incommensurate fractional discrete macroeconomic system: Bifurcation, chaos and complexity. Chin. Phys. B 2023, 32, 030203. [Google Scholar] [CrossRef]
- Khennaoui, A.A.; Ouannas, A.; Bendoukha, S.; Grassi, G.; Lozi, R.P.; Pham, V.T. On fractional–order discrete–time systems: Chaos, stabilization and synchronization. Chaos Solitons Fractals 2019, 119, 150–162. [Google Scholar] [CrossRef]
- Ouannas, A.; Khennaoui, A.A.; Momani, S.; Grassi, G.; Pham, V.T. Chaos and control of a three-dimensional fractional order discrete-time system with no equilibrium and its synchronization. AIP Adv. 2020, 10, 045310. [Google Scholar] [CrossRef]
- Ouannas, A.; Khennaoui, A.A.; Batiha, I.M.; Pham, V.T. Synchronization between fractional chaotic maps with different dimensions. In Fractional-Order Design; Radwan, A.G., Khanday, F.A., Said, L.A., Eds.; Volume 3 in Emerging Methodologies and Applications in Modelling; Academic Press: Cambridge, MA, USA, 2022; pp. 89–121. [Google Scholar]
- Saadeh, R.; Abbes, A.; Al-Husban, A.; Ouannas, A.; Grassi, G. The Fractional Discrete Predator–Prey Model: Chaos, Control and Synchronization. Fractal Fract. 2023, 7, 120. [Google Scholar] [CrossRef]
- Rahmi, E.; Darti, I.; Suryanto, A.; Trisilowati. A modified Leslie–Gower model incorporating Beddington–deangelis functional response, double Allee effect and memory effect. Fractal Fract. 2021, 5, 84. [Google Scholar] [CrossRef]
- Lin, S.; Chen, F.; Li, Z.; Chen, L. Complex dynamic behaviors of a modified discrete Leslie–Gower Predator–prey system with fear effect on prey species. Axioms 2022, 11, 520. [Google Scholar] [CrossRef]
- Mondal, N.; Barman, D.; Roy, J.; Alam, S.; Sajid, M. A modified Leslie–Gower fractional order prey-predator interaction model incorporating the effect of fear on prey. J. Appl. Anal. Comput. 2023, 13, 198–232. [Google Scholar] [CrossRef]
- Yuan, L.G.; Yang, Q.G. Bifurcation, invariant curve and hybrid control in a discrete-time predator-prey system. Appl. Math. Model. 2015, 39, 2345–2362. [Google Scholar] [CrossRef]
- Ajaz, M.B.; Saeed, U.; Din, Q.; Ali, I.; Siddiqui, M.I. Bifurcation analysis and chaos control in discrete-time modified Leslie–Gower prey harvesting model. Adv. Differ. Equ. 2020, 2020, 45. [Google Scholar] [CrossRef]
- Din, Q. Complexity and chaos control in a discrete-time prey-predator model. Commun. Nonlinear Sci. Numer. Simul. 2017, 49, 113–134. [Google Scholar] [CrossRef]
- Khan, A.Q.; Bukhari, S.A.H.; Almatrafi, M.B. Global dynamics, Neimark–Sacker bifurcation and hybrid control in a Leslie’s prey-predator model. Alex. Eng. J. 2022, 61, 11391–11404. [Google Scholar] [CrossRef]
- Vinoth, S.; Sivasamy, R.; Sathiyanathan, K.; Unyong, B.; Vadivel, R.; Gunasekaran, N. A novel discrete-time Leslie–Gower model with the impact of Allee effect in predator population. Complexity 2022, 2022, 6931354. [Google Scholar] [CrossRef]
- Allee, W.C. Animal Aggregations, a Study in General Sociology; The University of Chicago Press: Chicago, IL, USA, 1931. [Google Scholar]
- Aziz-Alaoui, M.A.; Okiye, M.D. Boundedness and global stability for a predator-prey model with modified Leslie–Gower and Holling-type II schemes. Appl. Math. Lett. 2003, 16, 1069–1075. [Google Scholar] [CrossRef]
- Wu, G.C.; Baleanu, D. Discrete fractional logistic map and its chaos. Nonlinear Dyn. 2014, 75, 283–287. [Google Scholar] [CrossRef]
- Wu, G.C.; Baleanu, D. Jacobian matrix algorithm for Lyapunov exponents of the discrete fractional maps. Commun. Nonlinear Sci. Numer. Simul. 2015, 22, 95–100. [Google Scholar] [CrossRef]
- Gottwald, G.; Melbourne, I. The 0–1 test for chaos: A review. In Chaos Detection and Predictability; Springer: Berlin/Heidelberg, Germany, 2016; pp. 221–247. [Google Scholar]
- Pincus, S. Approximate entropy as a measure of system complexity. Proc. Natl. Acad. Sci. USA 1991, 88, 2297–2301. [Google Scholar] [CrossRef]
- Shen, E.; Cai, Z.; Gu, F. Mathematical foundation of a new complexity measure. Appl. Math. Mech. 2005, 26, 1188–1196. [Google Scholar]
- He, S.; Sun, K.; Wang, H. Complexity analysis and DSP implementation of the fractional-order Lorenz hyperchaotic system. Entropy 2015, 17, 8299–8311. [Google Scholar] [CrossRef]






Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hamadneh, T.; Abbes, A.; Falahah, I.A.; AL-Khassawneh, Y.A.; Heilat, A.S.; Al-Husban, A.; Ouannas, A. Complexity and Chaos Analysis for Two-Dimensional Discrete-Time Predator–Prey Leslie–Gower Model with Fractional Orders. Axioms 2023, 12, 561. https://doi.org/10.3390/axioms12060561
Hamadneh T, Abbes A, Falahah IA, AL-Khassawneh YA, Heilat AS, Al-Husban A, Ouannas A. Complexity and Chaos Analysis for Two-Dimensional Discrete-Time Predator–Prey Leslie–Gower Model with Fractional Orders. Axioms. 2023; 12(6):561. https://doi.org/10.3390/axioms12060561
Chicago/Turabian StyleHamadneh, Tareq, Abderrahmane Abbes, Ibraheem Abu Falahah, Yazan Alaya AL-Khassawneh, Ahmed Salem Heilat, Abdallah Al-Husban, and Adel Ouannas. 2023. "Complexity and Chaos Analysis for Two-Dimensional Discrete-Time Predator–Prey Leslie–Gower Model with Fractional Orders" Axioms 12, no. 6: 561. https://doi.org/10.3390/axioms12060561
APA StyleHamadneh, T., Abbes, A., Falahah, I. A., AL-Khassawneh, Y. A., Heilat, A. S., Al-Husban, A., & Ouannas, A. (2023). Complexity and Chaos Analysis for Two-Dimensional Discrete-Time Predator–Prey Leslie–Gower Model with Fractional Orders. Axioms, 12(6), 561. https://doi.org/10.3390/axioms12060561






