Consistency of the Estimator for the Common Mean in Fixed-Effect Meta-Analyses
Abstract
1. Introduction
2. Fixed-Effect Meta-Analysis
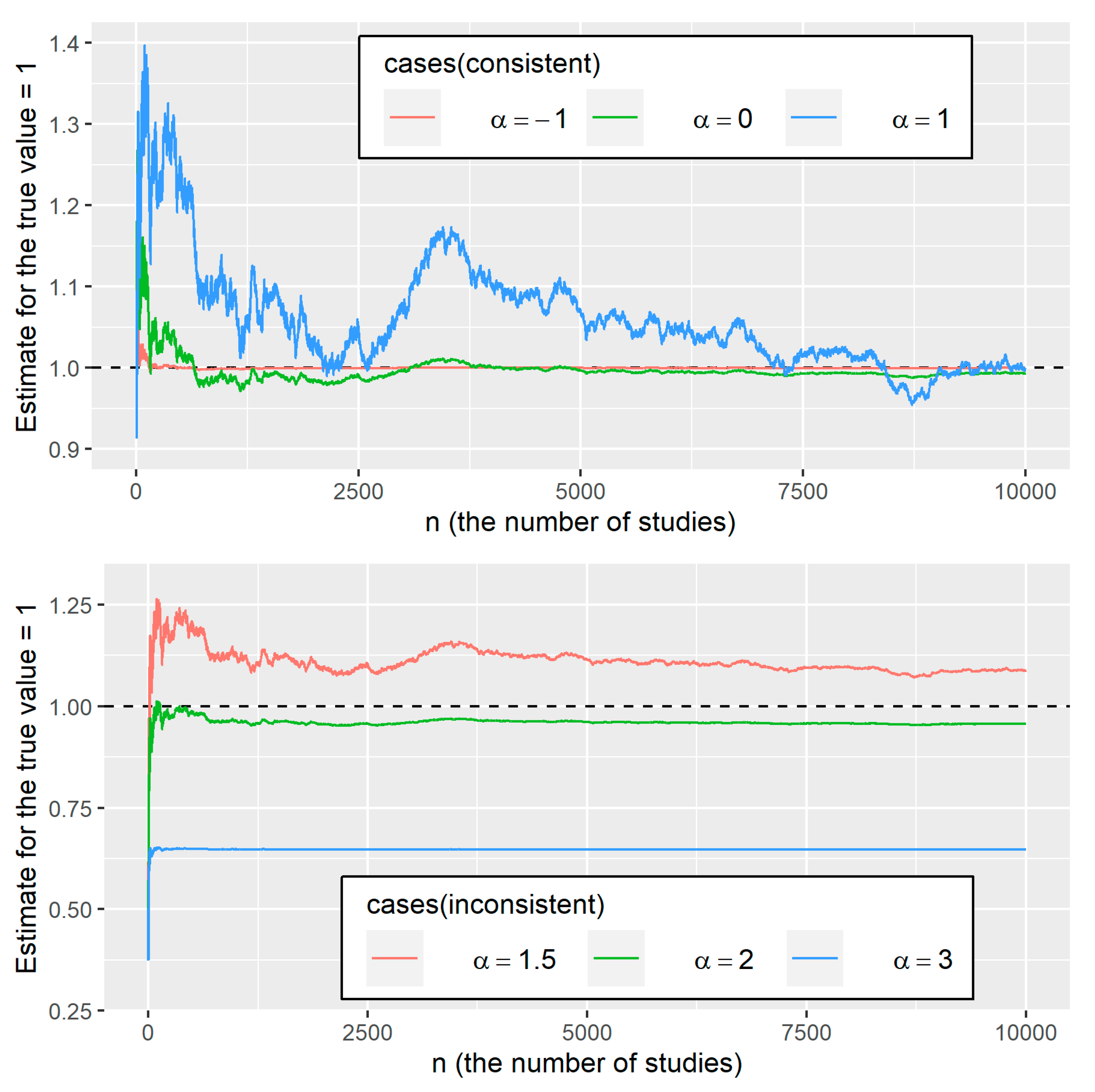
3. Consistency
- giving a consistent estimator by
- giving a consistent estimator by
- giving a consistent estimator by
- giving an inconsistent estimator by
- giving an inconsistent estimator by
- giving an inconsistent estimator by
4. Data Analysis
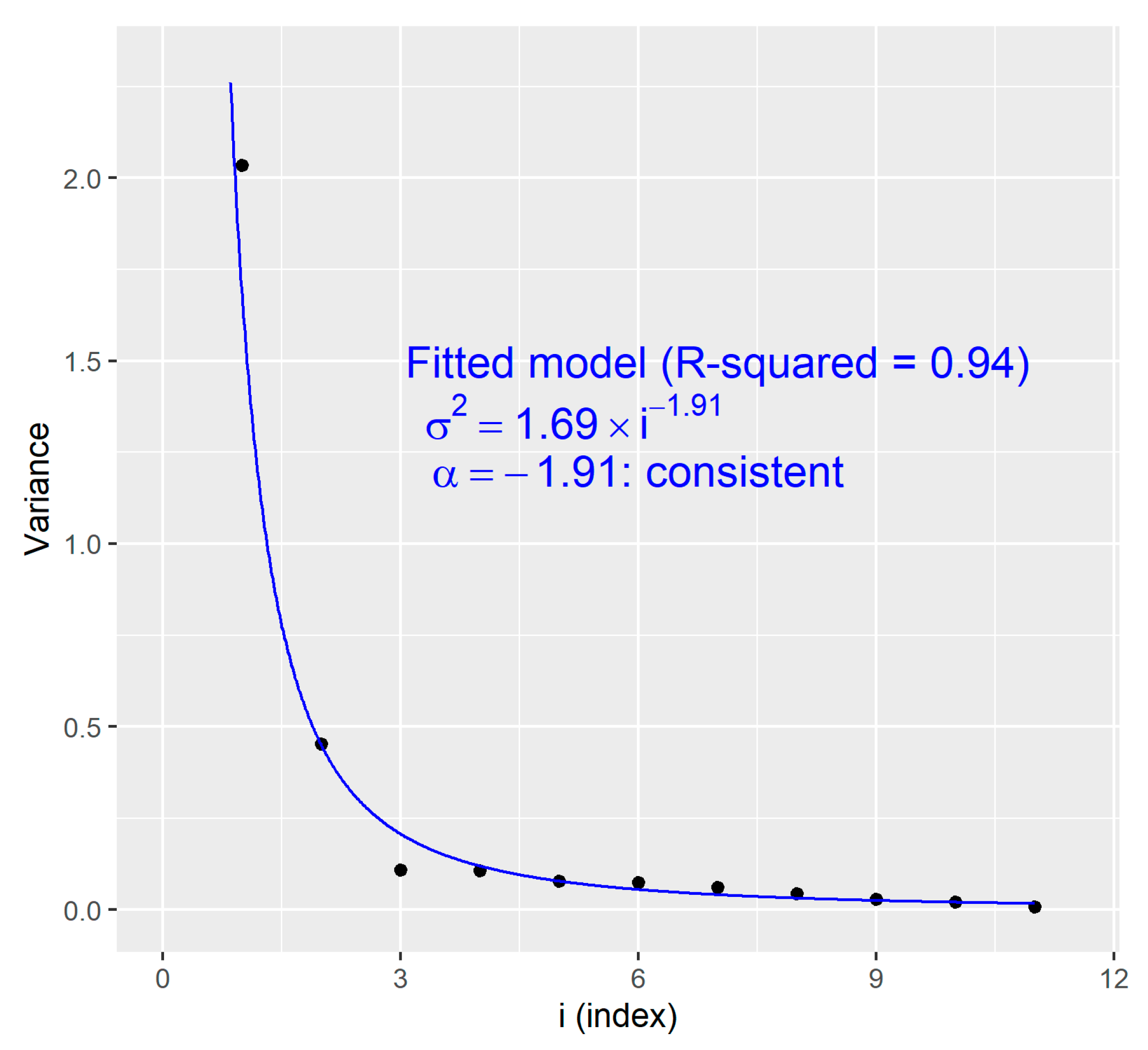
4.1. Allergic Reaction Data
4.2. Diabetes Data
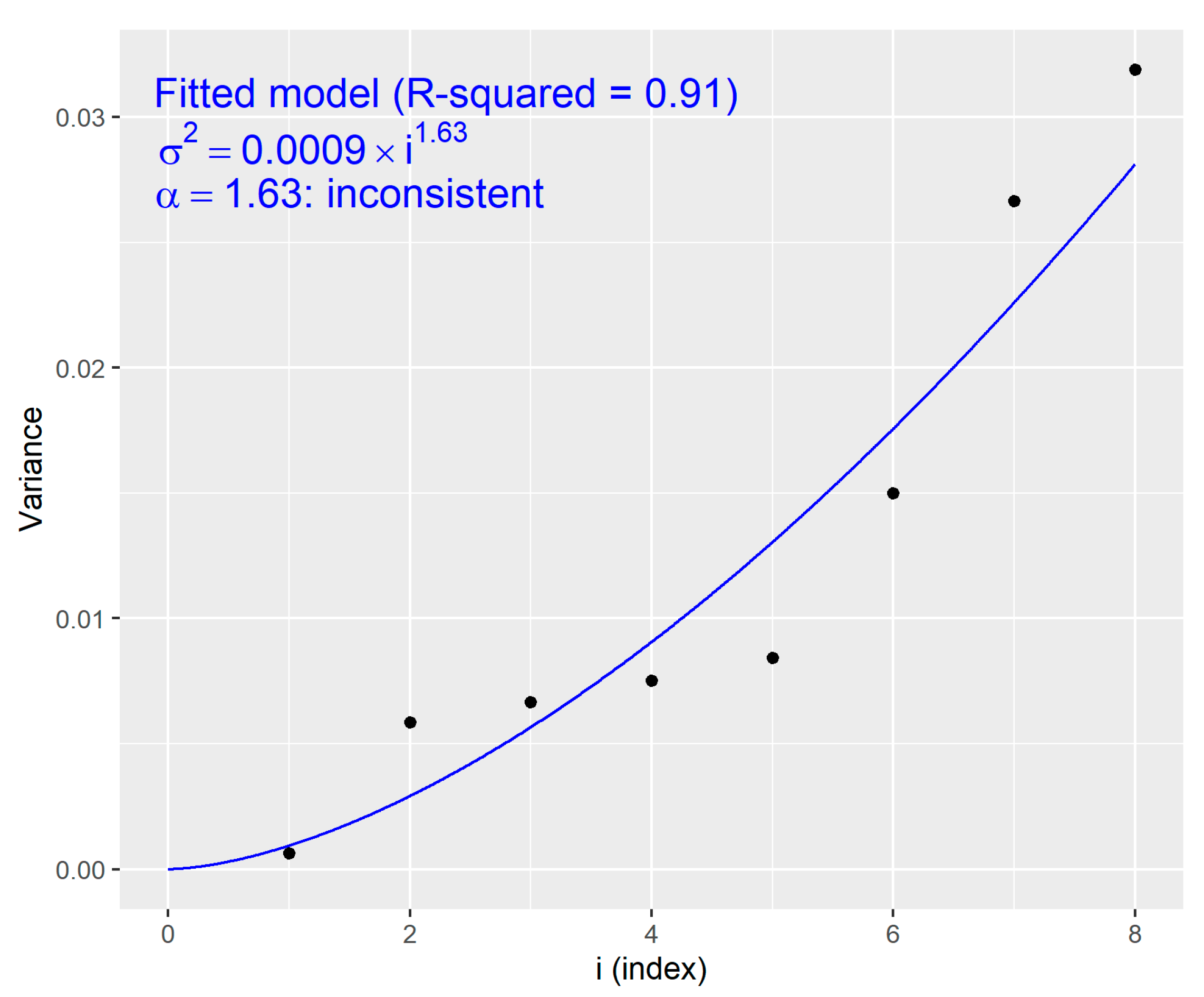
4.3. COVID-19 Data
5. Extension to Unknown Variances
6. Conclusions and Discussion
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A. Pooled Variance Estimator
Appendix B
Appendix C
References
- Borenstein, M.; Hedges, L.V.; Higgins, J.P.; Rothstein, H.R. Introduction to Meta-Analysis; John Wiley & Sons: Hoboken, NJ, USA, 2011. [Google Scholar]
- Kaiser, T.; Menkhoff, L. Financial Education in Schools: A Meta-analysis of Experimental Studies. Econ. Educ. Rev. 2020, 78, 101930. [Google Scholar] [CrossRef]
- Leung, Y.; Oates, J.; Chan, S.P. Voice, Articulation, and Prosody Contribute to Listener Perceptions of Speaker Gender: A Systematic Review and Meta-analysis. J. Speech Lang. Hear. Res. 2018, 61, 266–297. [Google Scholar] [CrossRef]
- DerSimonian, R.; Laird, N. Meta-analysis in Clinical Trials Revisited. Contemp. Clin. Trials 2015, 45, 139–145. [Google Scholar] [CrossRef]
- Fleiss, J.L. The Statistical Basis of Meta-analysis. Stat. Methods Med. Res. 1993, 2, 121–145. [Google Scholar] [CrossRef]
- Reyna-Villasmil, E.; Caponcello, M.G.; Maldonado, N.; Olivares, P.; Caroccia, N.; Bonazzetti, C.; Tazza, B.; Carrara, E.; Giannella, M.; Tacconelli, E.; et al. Association of Patients’ Epidemiological Characteristics and Comorbidities with Severity and Related Mortality Risk of SARS-CoV-2 Infection: Results of an Umbrella Systematic Review and Meta-Analysis. Biomedicines 2022, 10, 2437. [Google Scholar] [CrossRef]
- Pranata, R.; Lim, M.A.; Huang, I.; Raharjo, S.B.; Lukito, A.A. Hypertension is Associated with Increased Mortality and Severity of Disease in COVID-19 Pneumonia: A Systematic Review, Meta-analysis and Meta-regression. J. Renin-Angiotensin-Aldosterone Syst. 2020, 21, 1470320320926899. [Google Scholar] [CrossRef] [PubMed]
- Wang, Y.; Kala, M.P.; Jafar, T.H. Factors Associated with Psychological Distress During the Coronavirus Disease 2019 (COVID-19) Pandemic on the Predominantly General Population: A Systematic Review and Meta-analysis. PLoS ONE 2020, 15, e0244630. [Google Scholar] [CrossRef]
- Rice, K.; Higgins, J.P.; Lumley, T. A Re-evaluation of Fixed Effect (s) Meta-analysis. J. R. Stat. Soc. Ser. A 2018, 181, 205–227. [Google Scholar] [CrossRef]
- Lehmann, E.L. Elements of Large-Sample Theory; Springer Science & Business Media: Berlin, Germany, 2010. [Google Scholar]
- Serfling, R.J. Approximation Theorems of Mathematical Statistics; John Wiley & Sons: Hoboken, NY, USA, 1980. [Google Scholar]
- Shao, J. Mathematical Statistics; Springer Science & Business Media: New York, NY, USA, 2003. [Google Scholar]
- Shih, J.H.; Konno, Y.; Chang, Y.-T.; Emura, T. Estimation of a Common Mean Vector in Bivariate Meta-Analysis under the FGM copula. Statistics 2019, 53, 673–695. [Google Scholar] [CrossRef]
- Shih, J.H.; Konno, Y.; Chang, Y.T.; Emura, T. Copula-based Estimation Methods for a Common Mean Vector for Bivariate Meta-analyses. Symmetry 2002, 14, 186. [Google Scholar] [CrossRef]
- Shinozaki, N.; Chang, Y.T. Minimaxity of Empirical Bayes Estimators Shrinking Toward the Grand Mean when Variances are Unequal. Commun. Stat.-Theor. Methods 1996, 25, 183–199. [Google Scholar] [CrossRef]
- Singh, H.P.; Vishwakarma, G.K. A Family of Estimators of Population Mean Using Auxiliary Information in Stratified Sampling. Commun. Stat.-Theor. Methods 2008, 37, 1038–1050. [Google Scholar] [CrossRef]
- Malekzadeh, A.; Kharrati-Kopaei, M. Inferences on the Common Mean of Several Normal Populations under Hetero-scedasticity. Comput. Stat. 2018, 33, 1367–1384. [Google Scholar] [CrossRef]
- Bradley, R.A.; Gart, J.J. The Asymptotic Properties of ML Estimators when Sampling from Associated Populations. Biometrika 1962, 49, 205–214. [Google Scholar] [CrossRef]
- Emura, T.; Hu, Y.H.; Konno, Y. Asymptotic Inference for Maximum Likelihood Estimators under the Special Exponential Family with Double-truncation. Stat. Pap. 2017, 58, 877–909. [Google Scholar] [CrossRef]
- Kontopantelis, E.; Reeves, D. Performance of Statistical Methods for Meta-analysis when True Study Effects are Non-normally Distributed: A Simulation Study. Stat. Methods Med. Res. 2010, 21, 409–426. [Google Scholar] [CrossRef]
- Taketomi, N.; Konno, Y.; Chang, Y.T.; Emura, T. A Meta-analysis for Simultaneously Estimating Individual Means with Shrinkage, Isotonic Regression and Pretests. Axioms 2021, 10, 267. [Google Scholar] [CrossRef]
- Taketomi, N.; Michimae, H.; Chang, Y.T.; Emura, T. meta. Shrinkage: An R Package for Meta-analyses for Simultaneously Estimating Individual Means. Algorithms 2022, 15, 26. [Google Scholar] [CrossRef]
- Mori, M.; Kakuma, T. Development of a Prediction Model for Subjective Physical Symptoms Induced during Systematic Anatomy Practice among Medical Students. Kurume Med. J. 2023; in press. [Google Scholar]
- Dingena, C.F.; Arofikina, D.; Campbell, M.D.; Holmes, M.J.; Scott, E.M.; Zulyniak, M.A. Nutritional and Exercise-Focused Lifestyle Interventions and Glycemic Control in Women with Diabetes in Pregnancy: A Systematic Review and Meta-Analysis of Randomized Clinical Trials. Nutrients 2023, 15, 323. [Google Scholar] [CrossRef]
- Pal, N.; Lin, J.J.; Chang, C.H.; Kumar, S. A revisit to the common mean problem: Comparing the maximum likelihood estimator with the Graybill–Deal estimator. Comput. Stat. Data Anal. 2007, 51, 5673–5681. [Google Scholar] [CrossRef]
- Sinha, B.K.; Hartung, J.; Knapp, G. Statistical Meta-Analysis with Applications; John Wiley & Sons: Hoboken, NJ, USA, 2011. [Google Scholar]
- Nikoloulopoulos, A.K. A Multinomial Quadrivariate D-vine Copula Mixed Model for Meta-analysis of Diagnostic Studies in the Presence of Non-evaluable Subjects. Stat. Methods Med. Res. 2020, 29, 2988–3005. [Google Scholar] [CrossRef] [PubMed]
- Yamaguchi, Y.; Maruo, K. Bivariate Beta-binomial Model using Gaussian Copula for Bivariate Meta-analysis of Two Binary Outcomes with Low incidence. Jpn. J. Stat. Data. Sci. 2019, 2, 347–373. [Google Scholar] [CrossRef]
- Mavridis, D.; Salanti, G. A Practical Introduction to Multivariate Meta-analysis. Stat. Methods Med. Res. 2013, 22, 133–158. [Google Scholar] [CrossRef] [PubMed]
- Garg, N.; Misra, N. Estimation of Order Restricted Location/scale Parameters of a General Bivariate Distribution under General Loss Function: Some Unified Results. Jpn. J. Stat. Data. Sci. 2022, 5, 553–576. [Google Scholar] [CrossRef]
- Jena, P.; Ranjan Tripathy, M. Estimating Positive Powers of the Scale Parameters under Order Restriction for Two Normal Populations with a Common Mean. Statistics 2022, 56, 598–630. [Google Scholar] [CrossRef]
- Chesneau, C. Theoretical Study of Some Angle Parameter Trigonometric Copulas. Modelling 2022, 3, 140–163. [Google Scholar] [CrossRef]
- Susam, S.O. A multi-parameter Generalized Farlie-Gumbel-Morgenstern Bivariate Copula Family via Bernstein Polynomial. Hacet. J. Math. Stat. 2022, 51, 618–631. [Google Scholar] [CrossRef]
- Chesneau, C. Theoretical Contributions to Three Generalized Versions of the Celebioglu–Cuadras Copula. Analytics 2023, 2, 31–54. [Google Scholar] [CrossRef]
- Cheung, M.W.L.; Chan, W. Meta-analytic Structural Equation Modeling: A Two-stage Approach. Psychol. Methods 2005, 10, 40. [Google Scholar] [CrossRef]
- Standsyah, R.E.; Otok, B.W.; Suharsono, A. Fixed Effect Meta-Analytic Structural Equation Modeling (MASEM) Estimation Using Generalized Method of Moments (GMM). Symmetry 2021, 13, 2273. [Google Scholar] [CrossRef]

| i (Year) | Sample Size (Male) | Mean (Male) | SD (Male) | Sample Size (Female) | Mean (Female) | SD (Female) | MD | SE | SE2 |
|---|---|---|---|---|---|---|---|---|---|
| 1 (2015) | 74 | 5.09 | 1.59 | 42 | 5.26 | 1.65 | −0.17 | 0.3114 | 0.0970 |
| 2 (2016) | 79 | 4.84 | 1.62 | 37 | 5.42 | 1.69 | −0.58 | 0.3272 | 0.1071 |
| 3 (2018) | 79 | 4.81 | 1.54 | 34 | 5.26 | 1.69 | −0.45 | 0.3253 | 0.1058 |
| 4 (2019) | 74 | 4.86 | 1.62 | 33 | 5.61 | 1.58 | −0.75 | 0.3366 | 0.1133 |
| 5 (2022) | 73 | 4.79 | 1.68 | 37 | 5.35 | 1.83 | −0.56 | 0.3494 | 0.1221 |
| Study | Sample Size | (mmol/L) | SE | |
|---|---|---|---|---|
| Aslfalah 2020 | 60 | −0.70 | 0.0256 | 0.0007 |
| Fei 2014 | 97 | −0.47 | 0.1224 | 0.0150 |
| Hajimoosayi 2020 | 70 | −0.20 | 0.0816 | 0.0067 |
| Jamilian 2018 | 40 | −0.40 | 0.1786 | 0.0319 |
| Jamilian 2019 | 60 | −0.10 | 0.0765 | 0.0059 |
| Jamilian 2020 | 51 | −0.33 | 0.0918 | 0.0084 |
| Lindsay 2015 | 100 | 0.01 | 0.0867 | 0.0075 |
| Ostadmohammadi 2019 | 54 | −0.20 | 0.1633 | 0.0267 |
| Study | Sample Size | log (RR) | SE | SE2 |
|---|---|---|---|---|
| Akbari 2020 | 440 | 0.6881 | 0.6732 | 0.4532 |
| Bai 2000 | 127 | 0.5933 | 0.2754 | 0.0758 |
| Cao 2020 | 102 | 1.1756 | 0.2821 | 0.0796 |
| Chen 2020 | 123 | 0.5365 | 0.2493 | 0.0621 |
| Chen T 2020 | 274 | 0.6780 | 0.1713 | 0.0294 |
| Fu 2020 | 200 | 0.5878 | 0.3302 | 0.1090 |
| Grasselli 2020 | 1591 | 0.4637 | 0.0956 | 0.0091 |
| Li 2020 | 102 | 0.5247 | 0.3272 | 0.1071 |
| Luo 2020 | 403 | 1.2326 | 0.1489 | 0.0222 |
| Yuan 2020 | 27 | 2.8904 | 1.4263 | 2.0344 |
| Zhou 2020 | 191 | 1.1378 | 0.2097 | 0.0440 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Taketomi, N.; Emura, T. Consistency of the Estimator for the Common Mean in Fixed-Effect Meta-Analyses. Axioms 2023, 12, 503. https://doi.org/10.3390/axioms12050503
Taketomi N, Emura T. Consistency of the Estimator for the Common Mean in Fixed-Effect Meta-Analyses. Axioms. 2023; 12(5):503. https://doi.org/10.3390/axioms12050503
Chicago/Turabian StyleTaketomi, Nanami, and Takeshi Emura. 2023. "Consistency of the Estimator for the Common Mean in Fixed-Effect Meta-Analyses" Axioms 12, no. 5: 503. https://doi.org/10.3390/axioms12050503
APA StyleTaketomi, N., & Emura, T. (2023). Consistency of the Estimator for the Common Mean in Fixed-Effect Meta-Analyses. Axioms, 12(5), 503. https://doi.org/10.3390/axioms12050503









