Various Solitons and Other Wave Solutions to the (2+1)-Dimensional Heisenberg Ferromagnetic Spin Chain Dynamical Model
Abstract
1. Introduction
2. The Methods
2.1. The SSBM
2.2. The ERSSM
3. Applications
3.1. Application of the Sardar-Subequation Method
3.2. Application of the Extended Rational Sine–Cosine and Sinh–Cosh Methods
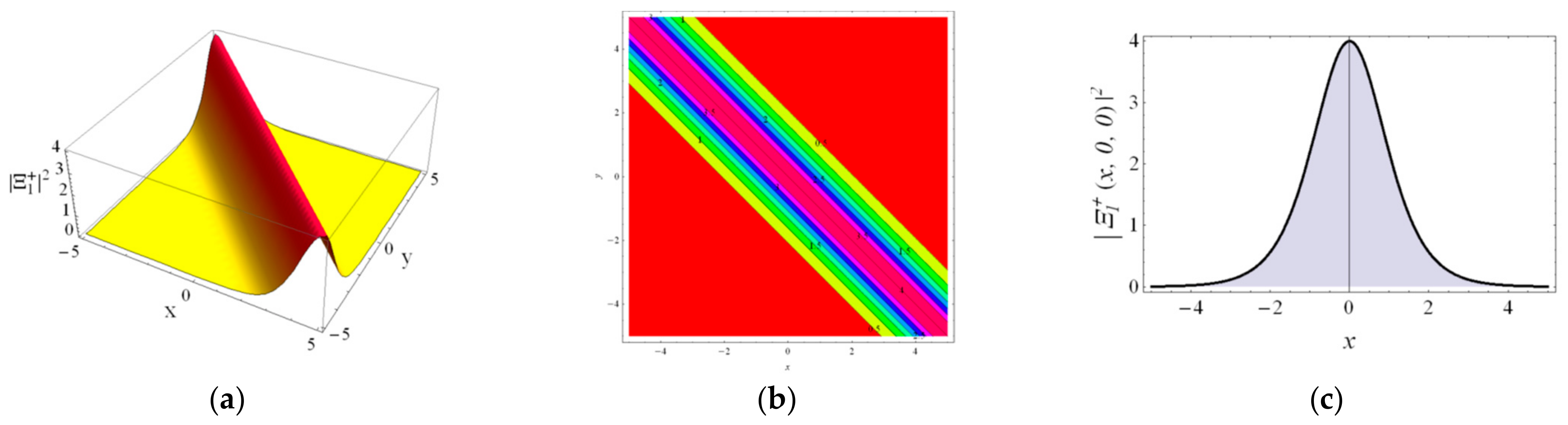
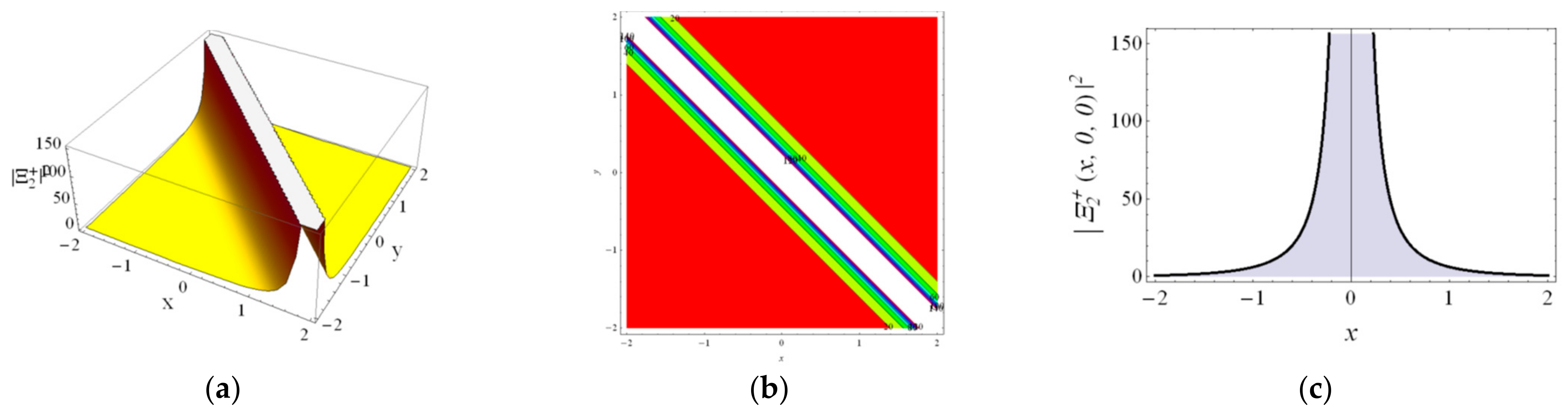
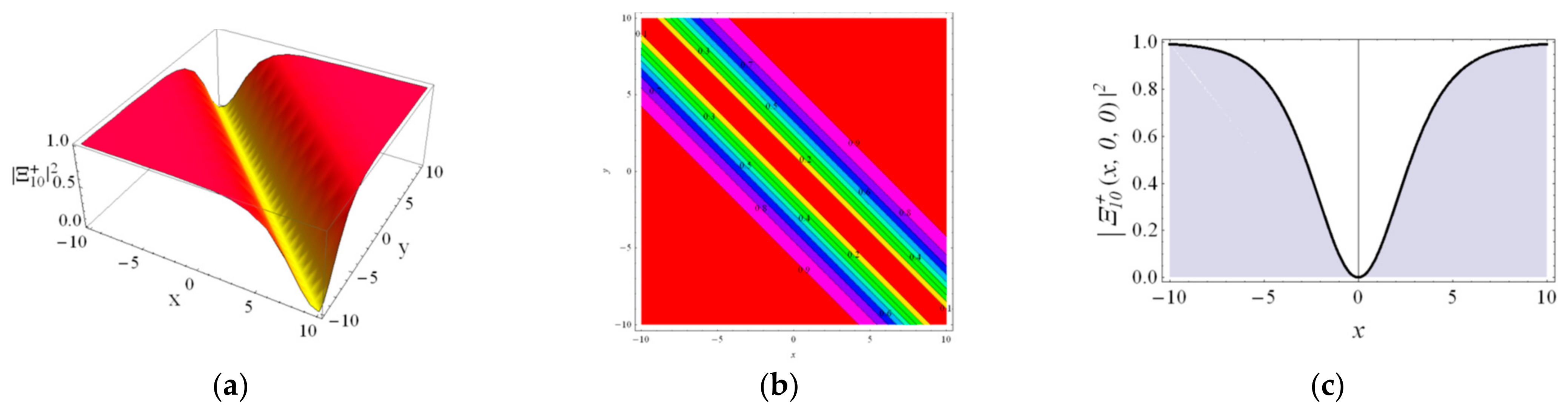
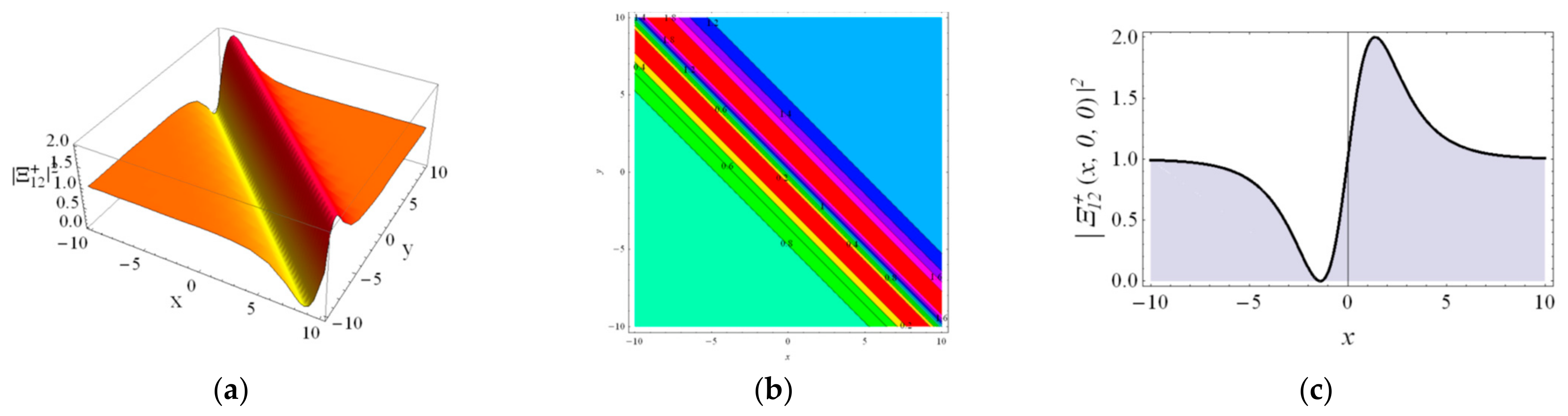
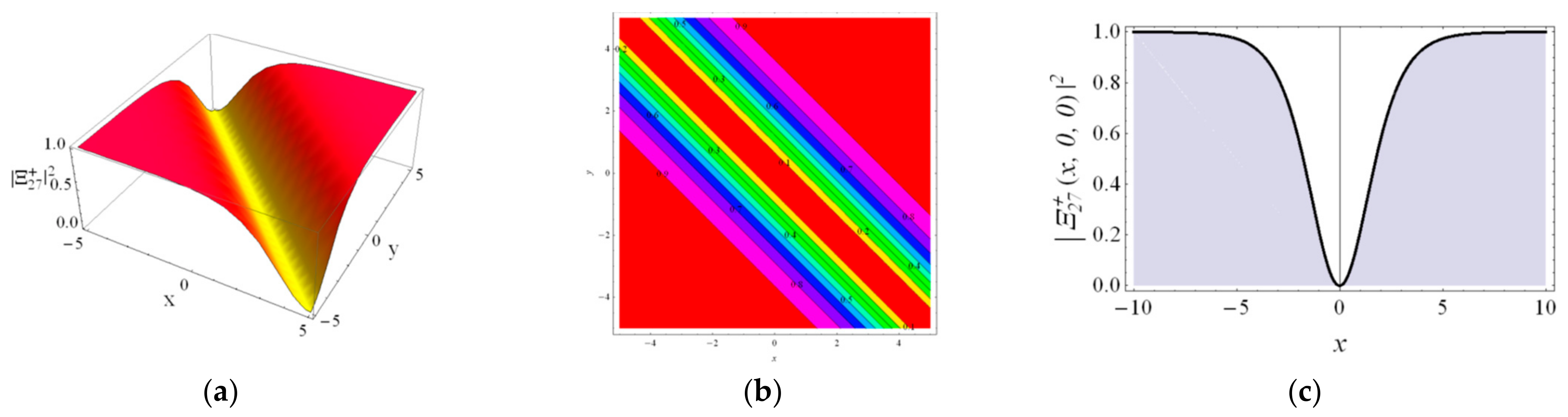
4. Discussion and the Physical Interpretations
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Lü, X.; Chen, S.-J. New general interaction solutions to the KPI equation via an optional decoupling condition approach. Commun. Nonlinear Sci. Numer. Simul. 2021, 103, 105939. [Google Scholar] [CrossRef]
- Alam, M.N.; Tunç, C. Constructions of the optical solitons and other solitons to the conformable fractional Zakharov-Kuznetsov equation with power law nonlinearity. J. Taibah Univ. Sci. 2020, 14, 94–100. [Google Scholar] [CrossRef]
- Hosseini, K.; Mirzazadeh, M.; Rabiei, F.; Baskonus, H.M.; Yel, G. Dark optical solitons to the Biswas-Arshed equation with high order dispersions and absence of the self-phase modulation. Optik 2020, 209, 164576. [Google Scholar] [CrossRef]
- Hosseini, K.; Salahshour, S.; Mirzazadeh, M. Bright and dark solitons of a weakly nonlocal Schrödinger equation involving the parabolic law nonlinearity. Optik 2021, 227, 166042. [Google Scholar] [CrossRef]
- Wang, K.J. Diverse wave structures to the modified Benjamin-Bona-Mahony equation in the optical illusions field. Mod. Phys. Lett. B 2023, 2023, 2350012. [Google Scholar] [CrossRef]
- Ali, K.K.; Yilmazer, R.; Baskonus, H.M.; Bulut, H. New wave behaviors and stability analysis of the Gilson–Pickering equation in plasma physics. Indian J. Phys. 2021, 95, 1003–1008. [Google Scholar] [CrossRef]
- Cheemaa, N.; Seadawy, A.R.; Chen, S. Some new families of solitary wave solutions of the generalized Schamel equation and their applications in plasma physics. Eur. Phys. J. Plus 2019, 134, 117. [Google Scholar] [CrossRef]
- Sohail, M.; Chu, Y.M.; El-zahar, E.R.; Nazir, U.; Naseem, T. Contribution of joule heating and viscous dissipation on three dimensional flow of Casson model comprising temperature dependent conductance utilizing shooting method. Phys. Scr. 2021, 96, 085208. [Google Scholar] [CrossRef]
- He, J.H.; Yang, Q.; He, C.H.; Khan, Y. A simple frequency formulation for the tangent oscillator. Axioms 2021, 10, 320. [Google Scholar] [CrossRef]
- Wang, K.; Si, J. Dynamic properties of the attachment oscillator arising in the nanophysics. Open Phys. 2023, 21, 20220214. [Google Scholar] [CrossRef]
- Taiwo, T.J.; Njah, A.N.; Oghre, E.O. Solution of Schrodinger equation using Tridiagonal representation approach in nonrelativistic quantum mechanics: A pedagogical approach. arXiv 2018, arXiv:1810.05509. [Google Scholar]
- Van Assche, W. Solution of an open problem about two families of orthogonal polynomials. SIGMA. Symmetry Integr. Geom. Methods Appl. 2019, 15, 005. [Google Scholar]
- Gurefe, Y.; Misirli, E.; Sonmezoglu, A.; Ekici, M. Extended trial equation method to generalized nonlinear partial differential equations. Appl. Math. Comput. 2013, 219, 5253–5260. [Google Scholar] [CrossRef]
- Hu, J.Y.; Feng, X.B.; Yang, Y.F. Optical envelope patterns perturbation with full nonlinearity for Gerdjikov–Ivanov equation by trial equation method. Optik 2021, 240, 166877. [Google Scholar] [CrossRef]
- Baskonus, H.M.; Bulut, H.; Sulaiman, T.A. New complex hyperbolic structures to the lonngren-wave equation by using sine-gordon expansion method. Appl. Math. Nonlinear Sci. 2019, 4, 129–138. [Google Scholar] [CrossRef]
- Yel, G.; Baskonus, H.M.; Bulut, H. Novel archetypes of new coupled Konno-Oono equation by using sine-Gordon expansion method. Opt. Quantum Electron. 2017, 49, 285. [Google Scholar] [CrossRef]
- Alam, M.N.; Akbar, M.A. Exact traveling wave solutions of the KP-BBM equation by using the new approach of generalized (G′/G)-expansion method. SpringerPlus 2013, 2, 617. [Google Scholar] [CrossRef]
- Teymuri Sindi, C.; Manafian, J. Wave solutions for variants of the KdV-Burger and the K (n, n)-Burger equations by the generalized G′/G-expansion method. Math. Methods Appl. Sci. 2017, 40, 4350–4363. [Google Scholar] [CrossRef]
- Mahak, N.; Akram, G. Exact solitary wave solutions by extended rational sine-cosine and extended rational sinh-cosh techniques. Phys. Scr. 2019, 94, 115212. [Google Scholar] [CrossRef]
- Mahak, N.; Akram, G. Extension of rational sine-cosine and rational sinh-cosh techniques to extract solutions for the perturbed NLSE with Kerr law nonlinearity. Eur. Phys. J. Plus 2019, 134, 159. [Google Scholar] [CrossRef]
- Wang, K.J.; Liu, J.H. Diverse optical solitons to the nonlinear Schrödinger equation via two novel techniques. Eur. Phys. J. Plus 2023, 138, 74. [Google Scholar] [CrossRef]
- El-Wakil, S.A.; Abdou, M.A. The extended Fan sub-equation method and its applications for a class of nonlinear evolution equations. Chaos Solitons Fractals 2008, 36, 343–353. [Google Scholar] [CrossRef]
- Liu, J.-G.; Ye, Q. Stripe solitons and lump solutions for a generalized Kadomtsev-Petviashvili equation with variable coefficients in fluid mechanics. Nonlinear Dyn 2019, 96, 23–29. [Google Scholar] [CrossRef]
- Darwish, A.; Ahmed, H.M.; Arnous, A.H.; Shehab, M.F. Optical solitons of Biswas–Arshed equation in birefringent fibers using improved modified extended tanh-function method. Optik 2021, 227, 165385. [Google Scholar] [CrossRef]
- Liu, J.-G.; Wazwaz, A.M.; Zhu, W.H. Solitary and lump waves interaction in variable-coefficient nonlinear evolution equation by a modified Ansatz with variable coefficients. J. Appl. Anal. Comput. 2022, 12, 517–532. [Google Scholar] [CrossRef]
- Wang, K.J.; Liu, J.H.; Si, J.; Shi, F.; Wang, G.-D. N-soliton, breather, lump solutions and diverse travelling wave solutions of the fractional (2+1)-dimensional Boussinesq equation. Fractals 2023, 31, 2350023. [Google Scholar] [CrossRef]
- Zulfiqar, A.; Ahmad, J. Soliton solutions of fractional modified unstable Schrödinger equation using Exp-function method. Results Phys. 2020, 19, 103476. [Google Scholar] [CrossRef]
- Barman, H.K.; Roy, R.; Mahmud, F.; Akbar, M.A.; Osman, M.S. Harmonizing wave solutions to the Fokas-Lenells model through the generalized Kudryashov method. Optik 2021, 229, 166294. [Google Scholar] [CrossRef]
- Ji-Guang, R.; Li-Hong, W.; Yu, Z.; He, J. Rational solutions for the Fokas system. Commun. Theor. Phys. 2015, 64, 605. [Google Scholar]
- Seadawy, A.R.; Nasreen, N.; Lu, D.; Arshad, M. Arising wave propagation in nonlinear media for the (2+1)-dimensional Heisenberg ferromagnetic spin chain dynamical model. Phys. A Stat. Mech. Its Appl. 2020, 538, 122846. [Google Scholar] [CrossRef]
- Hosseini, K.; Salahshour, S.; Mirzazadeh, M.; Ahmadian, A.; Baleanu, D.; Khoshrang, A. The (2+1)-dimensional Heisenberg ferromagnetic spin chain equation: Its solitons and Jacobi elliptic function solutions. Eur. Phys. J. Plus 2021, 136, 1–9. [Google Scholar] [CrossRef]
- Yang, D. Traveling waves and bifurcations for the (2+1)-dimensional Heisenberg ferromagnetic spin chain equation. Optik 2021, 248, 168058. [Google Scholar] [CrossRef]
- Sahoo, S.; Tripathy, A. New exact solitary solutions of the (2+1)-dimensional Heisenberg ferromagnetic spin chain equation. Eur. Phys. J. Plus 2022, 137, 390. [Google Scholar] [CrossRef]
- Inc, M.; Aliyu, A.I.; Yusuf, A.; Baleanu, D. Optical solitons and modulation instability analysis of an integrable model of (2+1)-dimensional Heisenberg ferromagnetic spin chain equation. Superlattices Microstruct. 2017, 112, 628–638. [Google Scholar] [CrossRef]
- Aliyu, A.I.; Li, Y.; İnç, M.; Wang, G. Solitons and complexitons to the (2+1)-dimensional Heisenberg ferromagnetic spin chain model. Int. J. Mod. Phys. B 2019, 33, 1950368. [Google Scholar] [CrossRef]
- Osman, M.S.; Tariq, K.U.; Bekir, A.; Elmoasry, A. Investigation of soliton solutions with different wave structures to the (2+1)-dimensional Heisenberg ferromagnetic spin chain equation. Commun. Theor. Phys. 2020, 72, 035002. [Google Scholar] [CrossRef]
- Wang, K.-J.; Shi, F.; Wang, G.-D. Abundant soliton structures to the (2+1)-dimensional Heisenberg ferromagnetic spin chain dynamical model. Adv. Math. Phys. 2023, 2023, 4348758. [Google Scholar] [CrossRef]
- Rehman, H.U.; Seadawy, A.R.; Younis, M.; Yasin, S.; Raza, S.T.R.; Althobaiti, S. Monochromatic optical beam propagation of paraxial dynamical model in Kerr media. Results Phys. 2021, 31, 105015. [Google Scholar] [CrossRef]
- Wang, K.J.; Si, J. Diverse optical solitons to the complex Ginzburg-Landau equation with Kerr law nonlinearity in the nonlinear optical fiber. Eur. Phys. J. Plus 2023, 138, 187. [Google Scholar] [CrossRef]
- Esen, H.; Ozdemir, N.; Secer, A.; Bayram, M. On solitary wave solutions for the perturbed Chen-Lee-Liu equation via an analytical approach. Optik 2021, 245, 167641. [Google Scholar] [CrossRef]
- Cinar, M.; Onder, I.; Secer, A.; Yusuf, A.; Sulaiman, T.A.; Bayram, M.; Aydin, H. The analytical solutions of Zoomeron equation via extended rational sin-cos and sinh-cosh methods. Phys. Scr. 2021, 96, 094002. [Google Scholar] [CrossRef]
- Wang, K.-J.; Shi, F.; Si, J.; Liu, J.-H.; Wang, G.-D. Non-differentiable exact solutions of the local fractional Zakharov-Kuznetsov equation on the Cantor sets. Fractals 2023, 31, 2350028. [Google Scholar] [CrossRef]
- Seadawy, A.R.; Bilal, M.; Younis, M.; Rizvi, S.T.R. Resonant optical solitons with conformable time-fractional nonlinear Schrödinger equation. Int. J. Mod. Phys. B 2021, 35, 2150044. [Google Scholar] [CrossRef]
- Wang, K.L. Novel scheme for the fractal-fractional short water wave model with unsmooth boundaries. Fractals 2022, 30, 2250193. [Google Scholar] [CrossRef]
- Wang, K.L. Novel travelling wave solutions for the fractal Zakharov-Kuznetsov-Benjamin-Bona-Mahony model. Fractals 2022, 30, 2250170. [Google Scholar] [CrossRef]
- Zhang, S.; Zhang, H.Q. Fractional sub-equation method and its applications to nonlinear fractional PDEs. Phys. Lett. A 2011, 375, 1069–1073. [Google Scholar] [CrossRef]
| Parameter | Description |
|---|---|
| The lattice parameter | |
| the coefficient of bilinear exchange interactions along the -direction | |
| the coefficient of bilinear exchange interactions along the -direction | |
| the neighboring interaction along the diagonal | |
| the uniaxial crystal field anisotropy paramete |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shi, F.; Wang, K.-J. Various Solitons and Other Wave Solutions to the (2+1)-Dimensional Heisenberg Ferromagnetic Spin Chain Dynamical Model. Axioms 2023, 12, 354. https://doi.org/10.3390/axioms12040354
Shi F, Wang K-J. Various Solitons and Other Wave Solutions to the (2+1)-Dimensional Heisenberg Ferromagnetic Spin Chain Dynamical Model. Axioms. 2023; 12(4):354. https://doi.org/10.3390/axioms12040354
Chicago/Turabian StyleShi, Feng, and Kang-Jia Wang. 2023. "Various Solitons and Other Wave Solutions to the (2+1)-Dimensional Heisenberg Ferromagnetic Spin Chain Dynamical Model" Axioms 12, no. 4: 354. https://doi.org/10.3390/axioms12040354
APA StyleShi, F., & Wang, K.-J. (2023). Various Solitons and Other Wave Solutions to the (2+1)-Dimensional Heisenberg Ferromagnetic Spin Chain Dynamical Model. Axioms, 12(4), 354. https://doi.org/10.3390/axioms12040354






