A Study of the Monotonic Properties of Solutions of Neutral Differential Equations and Their Applications
Abstract
1. Introduction
- (C1)
- and are continuous on and satisfy the conditions: and does not vanish identically on any half-line for .
- (C2)
- and are continuous delay functions on and fulfil the conditions: and .
2. Monotonic Properties
- (D1)
- for
- (D2)
- for
- (C)
- there is a such that .
- (a)
- ,
- (b)
- ,
3. Oscillation Results
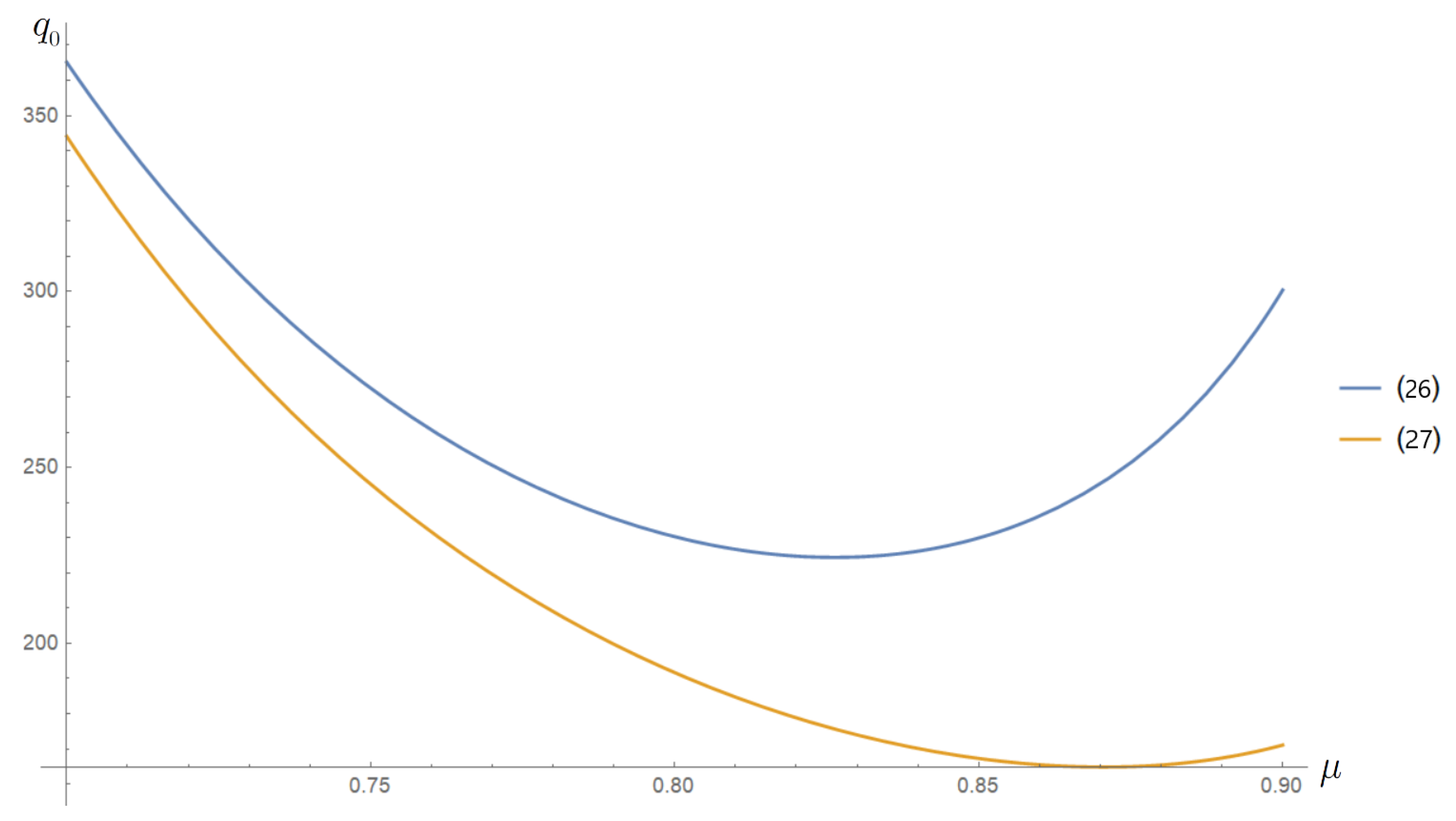
4. Application and Discussion
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Hale, J.K. Functional differential equations. In Analytic Theory of Differential Equations; Springer: Berlin/Heidelberg, Germany, 1971; pp. 9–22. [Google Scholar]
- Goldbeter, A. Dissipative structures in biological systems: Bistability, oscillations, spatial patterns and waves. Philos. Trans. R. Soc. A 2018, 376, 20170376. [Google Scholar] [CrossRef]
- Gopalsamy, K. Stability and Oscillations in Delay Differential Equations of Population Dynamics; Kluwer: Dordrecht, The Netherlands, 1992. [Google Scholar]
- Mackey, M.C.; Glass, L. Oscillations and chaos in physiological control systems. Science 1977, 197, 287–289. [Google Scholar] [CrossRef] [PubMed]
- Rihan, F.A. Delay Differential Equations and Applications to Biology; Springer Nature: Singapore, 2021. [Google Scholar]
- Gyori, I.; Ladas, G. Oscillation Theory of Delay Differential Equations with Applications; Clarendon Press: Oxford, UK, 1991. [Google Scholar]
- Erbe, L.H.; Kong, Q.; Zhong, B.G. Oscillation Theory for Functional Differential Equations; Marcel Dekker: New York, NY, USA, 1995. [Google Scholar]
- Agarwal, R.P.; Grace, S.R.; O’Regan, D. Oscillation Theory for Second Order Dynamic Equations; Series in Mathematical Analysis and Applications; Taylor & Francis Ltd.: London, UK, 2003; Volume 5. [Google Scholar]
- Agarwal, R.P.; Bohner, M.; Li, W.-T. Nonoscillation and oscillation: Theory for functional differential equations. In Monographs and Textbooks in Pure and Applied Mathematics; Marcel Dekker, Inc.: New York, NY, USA, 2004; Volume 267. [Google Scholar]
- Saker, S. Oscillation Theory of Delay Differential and Difference Equations: Second and Third Orders; LAP Lambert Academic Publishing: Saarbruecken, Germany, 2010. [Google Scholar]
- Bohner, M.; Grace, S.R.; Jadlovská, I. Oscillation criteria for second-order neutral delay differential equations. Electron. J. Qual. Theory Differ. Equ. 2017, 60, 2017. [Google Scholar] [CrossRef]
- Džurina, J.; Grace, S.R.; Jadlovska, I.; Li, T. Oscillation criteria for second-order Emden–Fowler delay differential equations with a sublinear neutral term. Math. Nachr. 2020, 293, 910–922. [Google Scholar] [CrossRef]
- Grace, S.R.; Džurina, J.; Jadlovská, I.; Li, T. An improved approach for studying oscillation of second-order neutral delay differential equations. J. Inequalities Appl. 2018, 193, 2018. [Google Scholar] [CrossRef] [PubMed]
- Moaaz, O.; Ramos, H.; Awrejcewicz, J. Second-order Emden–Fowler neutral differential equations: A new precise criterion for oscillation. Appl. Math. Lett. 2021, 118, 107172. [Google Scholar] [CrossRef]
- Bohner, M.; Grace, S.R.; Jadlovská, I. Sharp oscillation criteria for second-order neutral delay differential equations. Math. Method. Appl. Sci. 2020, 43, 10041–10053. [Google Scholar] [CrossRef]
- Jadlovská, I. New criteria for sharp oscillation of second-order neutral delay differential equations. Mathematics 2021, 9, 2089. [Google Scholar] [CrossRef]
- Agarwal, R.P.; Bohner, M.; Li, T.; Zhang, C. A new approach in the study of oscillatory behavior of even-order neutral delay differential equations. Appl. Math. Comput. 2013, 225, 787–794. [Google Scholar] [CrossRef]
- Li, T.; Rogovchenko, Y.V. Oscillation criteria for even-order neutral differential equations. Appl. Math. Lett. 2016, 61, 35–41. [Google Scholar] [CrossRef]
- Baculikova, B.; Dzurina, J.; Graef, J.R. On the oscillation of higher order delay differential equations. J. Math. Sci. 2012, 187, 13–24. [Google Scholar] [CrossRef]
- Zhang, C.; Agarwal, R.P.; Bohner, M.; Li, T. New results for oscillatory behavior of even-order half-linear delay differential equations. Appl. Math. Lett. 2013, 26, 179–183. [Google Scholar] [CrossRef]
- Li, T.; Rogovchenko, Y.V. On asymptotic behavior of solutions to higher-order sublinear Emden–Fowler delay differential equations. Appl. Math. Lett. 2017, 67, 53–59. [Google Scholar] [CrossRef]
- Moaaz, O.; Muhib, A. New oscillation criteria for nonlinear delay differential equations of fourth-order. Appl. Math. Comput. 2020, 377, 125192. [Google Scholar] [CrossRef]
- Moaaz, O.; Muhib, A.; Baleanu, D.; Alharbi, W.; Mahmoud, E.E. Simplified and improved criteria for oscillation of delay differential equations of fourth order. Adv. Differ. Equ. 2021, 2021, 295. [Google Scholar] [CrossRef]
- Zhang, Q.; Yan, J.; Gao, L. Oscillation behavior of even order nonlinear neutral differential equations with variable coefficients. Comput. Math. Appl. 2010, 59, 426–430. [Google Scholar] [CrossRef]
- Baculíková, B.; Dzurina, J.; Li, T. Oscillation results for even-order quasilinear neutral functional differential equations. Electron. J. Differ. Equ. 2011, 2011, 1–9. [Google Scholar]
- Baculíková, B.; Dzurina, J. Oscillation theorems for higher order neutral differential equations. Appl. Math. Comput. 2012, 219, 3769–3778. [Google Scholar] [CrossRef]
- Salah, H.; Moaaz, O.; Cesarano, C.; Elabbasy, E.M. Oscillation of higher-order canonical delay differential equations: Comparison theorems. Phys. Scr. 2023, 98, 024003. [Google Scholar] [CrossRef]
- Agarwal, R.P.; Grace, S.R.; O’Regan, D. Oscillation Theory for Difference and Functional Differential Equations; Kluwer Academic: Dordrecht, The Netherlands, 2000. [Google Scholar]
- Chatzarakis, G.E.; Grace, S.R.; Jadlovská, I.; Li, T.; Tunç, E. Oscillation criteria for third-order Emden–Fowler differential equations with unbounded neutral coefficients. Complexity 2019, 2019, 5691758. [Google Scholar] [CrossRef]
- Philos, C.G. On the existence of nonoscillatory solutions tending to zero at ∞ for dif ferential equations with positive delays. Arch. Math. 1981, 36, 168–178. [Google Scholar] [CrossRef]
- Kitamura, Y.; Kusano, T. Oscillation of first-order nonlinear differential equations with deviating arguments. Proc. Amer. Math. Soc. 1980, 78, 64–68. [Google Scholar] [CrossRef]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Moaaz, O.; Alhgilan, A.E. A Study of the Monotonic Properties of Solutions of Neutral Differential Equations and Their Applications. Axioms 2023, 12, 346. https://doi.org/10.3390/axioms12040346
Moaaz O, Alhgilan AE. A Study of the Monotonic Properties of Solutions of Neutral Differential Equations and Their Applications. Axioms. 2023; 12(4):346. https://doi.org/10.3390/axioms12040346
Chicago/Turabian StyleMoaaz, Osama, and Abtehal E. Alhgilan. 2023. "A Study of the Monotonic Properties of Solutions of Neutral Differential Equations and Their Applications" Axioms 12, no. 4: 346. https://doi.org/10.3390/axioms12040346
APA StyleMoaaz, O., & Alhgilan, A. E. (2023). A Study of the Monotonic Properties of Solutions of Neutral Differential Equations and Their Applications. Axioms, 12(4), 346. https://doi.org/10.3390/axioms12040346