On Some Branched Continued Fraction Expansions for Horn’s Hypergeometric Function H4(a,b;c,d;z1,z2) Ratios
Abstract
1. Introduction
- (i)
- To construct the BCFE;
- (ii)
- To prove the convergence of the constructed expansion;
- (iii)
- To prove the convergence of the BCF to the function of which it is an expansion.
2. Expansions
3. Convergence of BCFE for
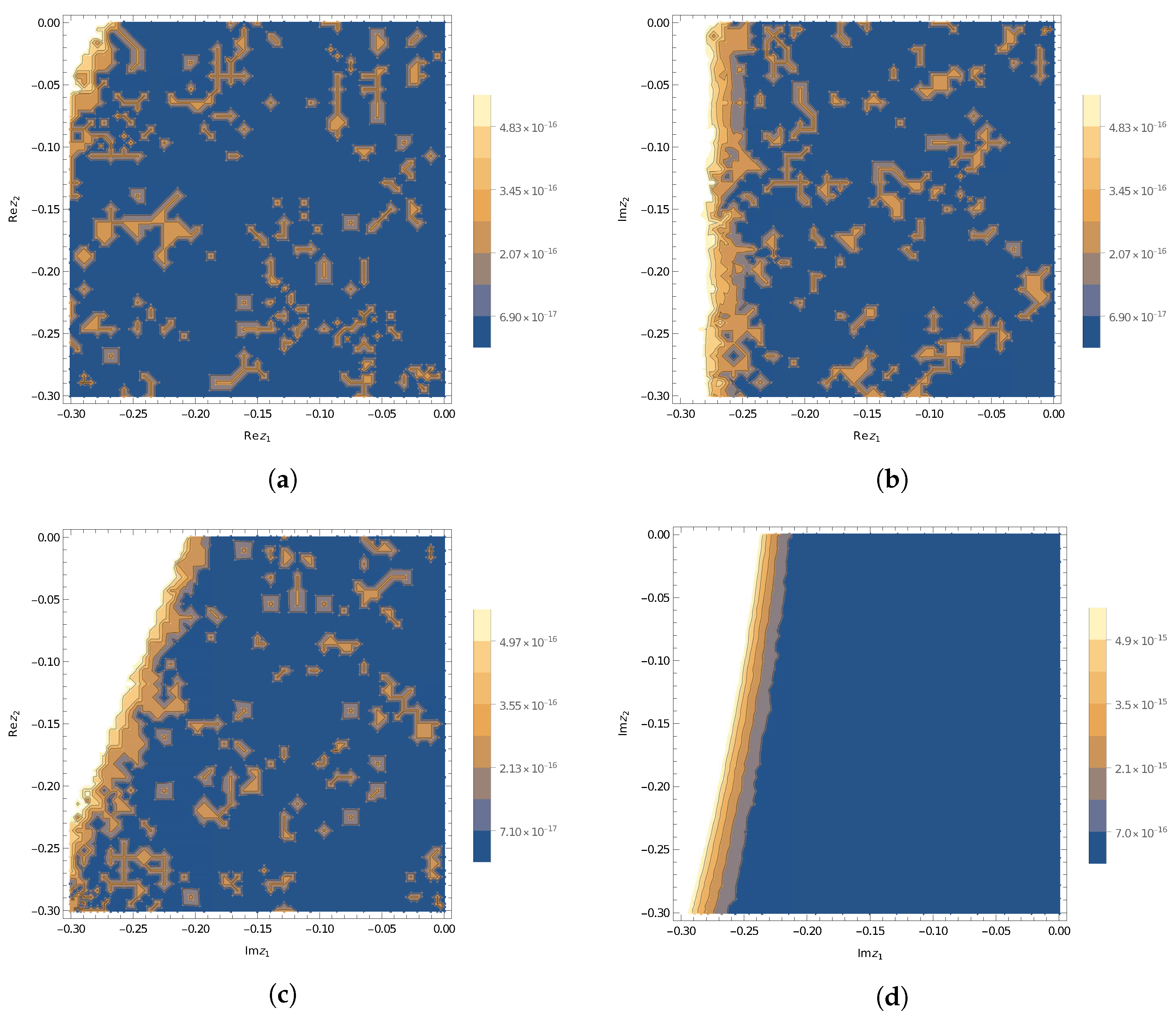
4. Numerical Experiments
5. Discussion
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| BCF | Branched continued fraction |
| BCFE | Branched continued fraction expansion |
| FBCFE | Formal branched continued fraction expansion |
| DPS | Double power series |
| FDPS | Formal double power series |
References
- Bodnarchuk, P.I.; Skorobogatko, V.Y. Branched Continued Fractions and Their Applications; Naukova Dumka: Kyiv, Ukraine, 1974. (In Ukrainian) [Google Scholar]
- Scorobogatko, V.Y. Theory of Branched Continued Fractions and Its Applications in Computational Mathematics; Nauka: Moscow, Russia, 1983. (In Russian) [Google Scholar]
- Komatsu, T. Asymmetric circular graph with Hosoya index and negative continued fractions. Carpathian Math. Publ. 2021, 13, 608–618. [Google Scholar] [CrossRef]
- Komatsu, T. Branched continued fractions associated with Hosoya index of the tree graph. MATCH Commun. Math. Comput. Chem. 2020, 84, 399–428. [Google Scholar]
- Cuyt, A. A review of multivariate Padé approximation theory. J. Comput. Appl. Math. 1985, 12–13, 221–232. [Google Scholar] [CrossRef]
- Cuyt, A.; Verdonk, B. A review of branched continued fraction theory for the construction of multivariate rational approximants. Appl. Numer. Math. 1988, 4, 263–271. [Google Scholar] [CrossRef]
- Petreolle, M.; Sokal, A.D. Lattice paths and branched continued fractions II. Multivariate Lah polynomials and Lah symmetric functions. Eur. J. Combin. 2021, 92, 103235. [Google Scholar] [CrossRef]
- Petreolle, M.; Sokal, A.D.; Zhu, B.X. Lattice paths and branched continued fractions: An infinite sequence of generalizations of the Stieltjes-Rogers and Thron-Rogers polynomials, with coefficientwise Hankel-total positivity. arXiv 2020, arXiv:1807.03271v2. [Google Scholar]
- Kuchminskaya, K.; Siemaszko, W. Rational Approximation and Interpolation of Functions by Branched Continued Fractions; Lecture Notes in Mathematics; Springer: Berlin/Heidelberg, Germany, 1987; Volume 1237, pp. 24–40. [Google Scholar] [CrossRef]
- Antonova, T.; Dmytryshyn, R.; Kravtsiv, V. Branched continued fraction expansions of Horn’s hypergeometric function H3 ratios. Mathematics 2021, 9, 148. [Google Scholar] [CrossRef]
- Hoyenko, N.P. Correspondence principle and convergence of sequences of analytic functions of several variables. Mat. Visn. Nauk. Tov. Im. Shevchenka. 2007, 4, 42–48. (In Ukrainian) [Google Scholar]
- Cuyt, A.A.M.; Petersen, V.; Verdonk, B.; Waadeland, H.; Jones, W.B. Handbook of Continued Fractions for Special Functions; Springer: Dordrecht, The Netherlands, 2008. [Google Scholar]
- Hoyenko, N.P.; Manzij, O.S. Expansion of Appel F1 and Lauricella hypergeometric functions into branched continued fractions. Visnyk Lviv. Univ. Ser. Mech.-Math. 1997, 48, 17–26. (In Ukrainian) [Google Scholar]
- Hoyenko, N.; Hladun, V.; Manzij, O. On the infinite remains of the Nörlund branched continued fraction for Appell hypergeometric functions. Carpathian Math. Publ. 2014, 6, 11–25. (In Ukrainian) [Google Scholar] [CrossRef]
- Bodnar, D.I. Expansion of a ratio of hypergeometric functions of two variables in branching continued fractions. J. Math. Sci. 1993, 64, 1155–1158. [Google Scholar] [CrossRef]
- Manzii, O.S. On the approximation of an Appell hypergeometric function by a branched continued fraction. J. Math. Sci. 1998, 90, 2376–2380. [Google Scholar] [CrossRef]
- Bodnar, D.I. Multidimensional C-fractions. J. Math. Sci. 1998, 90, 2352–2359. [Google Scholar] [CrossRef]
- Hladun, V.R.; Hoyenko, N.P.; Manzij, O.S.; Ventyk, L. On convergence of function F4(1, 2; 2, 2; z1, z2) expansion into a branched continued fraction. Math. Model. Comput. 2022, 9, 767–778. [Google Scholar] [CrossRef]
- Bodnar, D.I.; Manzii, O.S. Expansion of the ratio of Appel hypergeometric functions F3 into a branching continued fraction and its limit behavior. J. Math. Sci. 2001, 107, 3550–3554. [Google Scholar] [CrossRef]
- Manzii, O.S. Investigation of expansion of the ratio of Appel hypergeometric functions F3 into a branching continued fraction. Approx. Theor. Its Appl. Pr. Inst. Math. NAS Ukr. 2000, 31, 344–353. (In Ukrainian) [Google Scholar]
- Antonova, T.M. On convergence of branched continued fraction expansions of Horn’s hypergeometric function H3 ratios. Carpathian Math. Publ. 2021, 13, 642–650. [Google Scholar] [CrossRef]
- Antonova, T.; Dmytryshyn, R.; Sharyn, S. Branched continued fraction representations of ratios of Horn’s confluent function H6. Constr. Math. Anal. 2023, 6, 22–37. [Google Scholar] [CrossRef]
- Horn, J. Hypergeometrische Funktionen zweier Veränderlichen. Math. Ann. 1931, 105, 381–407. [Google Scholar] [CrossRef]
- Horn, J. Hypergeometrische Funktionen zweier Veränderlichen. Math. Ann. 1935, 111, 638–677. [Google Scholar] [CrossRef]
- Horn, J. Hypergeometrische Funktionen zweier Veränderlichen. Math. Ann. 1937, 113, 242–291. [Google Scholar] [CrossRef]
- Bailey, W.N. Generalised Hypergeometric Series; Cambridge University Press: Cambridge, UK, 1935. [Google Scholar]
- Erdélyi, A.; Magnus, W.; Oberhettinger, F.; Tricomi, F.G. Higher Transcendental Functions; McGraw-Hill Book Co.: New York, NY, USA, 1953; Volume 1. [Google Scholar]
- Srivastava, H.M.; Karlsson, P.W. Multiple Gaussian Hypergeometric Series; Horwood, E., Ed.; Halsted Press: Chichester, UK, 1985. [Google Scholar]
- Antonova, T.; Dmytryshyn, R.; Sharyn, S. Generalized hypergeometric function 3F2 ratios and branched continued fraction expansions. Axioms 2021, 10, 310. [Google Scholar] [CrossRef]
- Dmytryshyn, R.I.; Lutsiv, I.-A.V. Three- and four-term recurrence relations for Horn’s hypergeometric function H4. Res. Math. 2022, 30, 21–29. [Google Scholar] [CrossRef]
- Jones, W.B.; Thron, W.J. Continued Fractions: Analytic Theory and Applications; Addison-Wesley Pub. Co.: Reading, MA, USA, 1980. [Google Scholar]
- Bodnar, D.I. Branched Continued Fractions; Naukova Dumka: Kyiv, Ukraine, 1986. (In Russian) [Google Scholar]
- Cuyt, A.; Verdonk, B. Evaluation of branched continued fractions using block-tridiagonal linear systems. IMA J. Numer. Anal. 1988, 8, 209–217. [Google Scholar] [CrossRef]
- Murphy, J.A.; O’Donohoe, M.R. A two-variable generalization of the Stieltjes-type continued fraction. J. Comput. Appl. Math. 1978, 4, 181–190. [Google Scholar] [CrossRef]
- Bilanyk, I.B. A truncation error bound for some branched continued fractions of the special form. Mat. Stud. 2019, 52, 115–123. [Google Scholar] [CrossRef]
- Bodnar, D.I.; Bilanyk, I.B. On the convergence of branched continued fractions of a special form in angular domains. J. Math. Sci. 2020, 246, 188–200. [Google Scholar] [CrossRef]
- Bodnar, D.I.; Bilanyk, I.B. Two-dimensional generalization of the Thron-Jones theorem on the parabolic domains of convergence of continued fractions. Ukr. Mat. Zhurn. 2022, 74, 1155–1169. [Google Scholar] [CrossRef]
- Bodnar, D.I.; Bilanyk, I.B. Estimation of the rates of pointwise and uniform convergence of branched continued fractions with inequivalent variables. J. Math. Sci. 2022, 265, 423–437. [Google Scholar] [CrossRef]
- Siemaszko, W. Branched continued fractions for double power series. J. Comput. Appl. Math. 1980, 6, 121–125. [Google Scholar] [CrossRef]
- Bilanyk, I.B.; Bodnar, D.I.; Buyak, L.M. Representation of a quotient of solutions of a four-term linear recurrence relation in the form of a branched continued fraction. Carpathian Math. Publ. 2019, 11, 33–41. [Google Scholar] [CrossRef]
- Lorentzen, L.; Waadeland, H. Continued Fractions—Volume 1: Convergence Theory, 2nd ed.; Atlantis Press: Amsterdam, The Netherlands, 2008. [Google Scholar]
- Bodnar, O.S.; Dmytryshyn, R.I. On the convergence of multidimensional S-fractions with independent variables. Carpathian Math. Publ. 2018, 9, 58–64. [Google Scholar] [CrossRef]
- Shabat, B.V. Introduce in the Complex Analysis; Nauka: Moskow, Russia, 1969. (In Russian) [Google Scholar]
- Vladimirov, V.S. Methods of the Theory of Functions of Several Complex Variables; Nauka: Moscow, Russia, 1964. (In Russian) [Google Scholar]
- Bodnar, D.I.; Bilanyk, I.B. Parabolic convergence regions of branched continued fractions of the special form. Carpathian Math. Publ. 2021, 13, 619–630. [Google Scholar] [CrossRef]
- Bodnar, O.S.; Dmytryshyn, R.I.; Sharyn, S.V. On the convergence of multidimensional S-fractions with independent variables. Carpathian Math. Publ. 2020, 12, 353–359. [Google Scholar] [CrossRef]
- Dmytryshyn, R.I. On some of convergence domains of multidimensional S-fractions with independent variables. Carpathian Math. Publ. 2019, 11, 54–58. [Google Scholar] [CrossRef]
- Antonova, T.M.; Dmytryshyn, R.I. Truncation error bounds for branched continued fraction . Ukr. Math. J. 2020, 72, 1018–1029. [Google Scholar] [CrossRef]
- Antonova, T.M.; Dmytryshyn, R.I. Truncation error bounds for branched continued fraction whose partial denominators are equal to unity. Mat. Stud. 2020, 54, 3–14. [Google Scholar] [CrossRef]
- Brychkov, Y.A.; Savischenko, N.V. On some formulas for the Horn functions H4(a, b; c, c′; w, z) and (a; c, c′; w, z). Integral Transforms Spec. Funct. 2021, 32, 969–987. [Google Scholar] [CrossRef]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Antonova, T.; Dmytryshyn, R.; Lutsiv, I.-A.; Sharyn, S. On Some Branched Continued Fraction Expansions for Horn’s Hypergeometric Function H4(a,b;c,d;z1,z2) Ratios. Axioms 2023, 12, 299. https://doi.org/10.3390/axioms12030299
Antonova T, Dmytryshyn R, Lutsiv I-A, Sharyn S. On Some Branched Continued Fraction Expansions for Horn’s Hypergeometric Function H4(a,b;c,d;z1,z2) Ratios. Axioms. 2023; 12(3):299. https://doi.org/10.3390/axioms12030299
Chicago/Turabian StyleAntonova, Tamara, Roman Dmytryshyn, Ilona-Anna Lutsiv, and Serhii Sharyn. 2023. "On Some Branched Continued Fraction Expansions for Horn’s Hypergeometric Function H4(a,b;c,d;z1,z2) Ratios" Axioms 12, no. 3: 299. https://doi.org/10.3390/axioms12030299
APA StyleAntonova, T., Dmytryshyn, R., Lutsiv, I.-A., & Sharyn, S. (2023). On Some Branched Continued Fraction Expansions for Horn’s Hypergeometric Function H4(a,b;c,d;z1,z2) Ratios. Axioms, 12(3), 299. https://doi.org/10.3390/axioms12030299








