A Non-Equilibrium Interpolation Scheme for IB-LBM Optimized by Approximate Force
Abstract
1. Introduction
2. Related Work
- Immersed boundary method and lattice Boltzmann method
- The conventional immersed boundary–lattice Boltzmann method (IB-LBM)
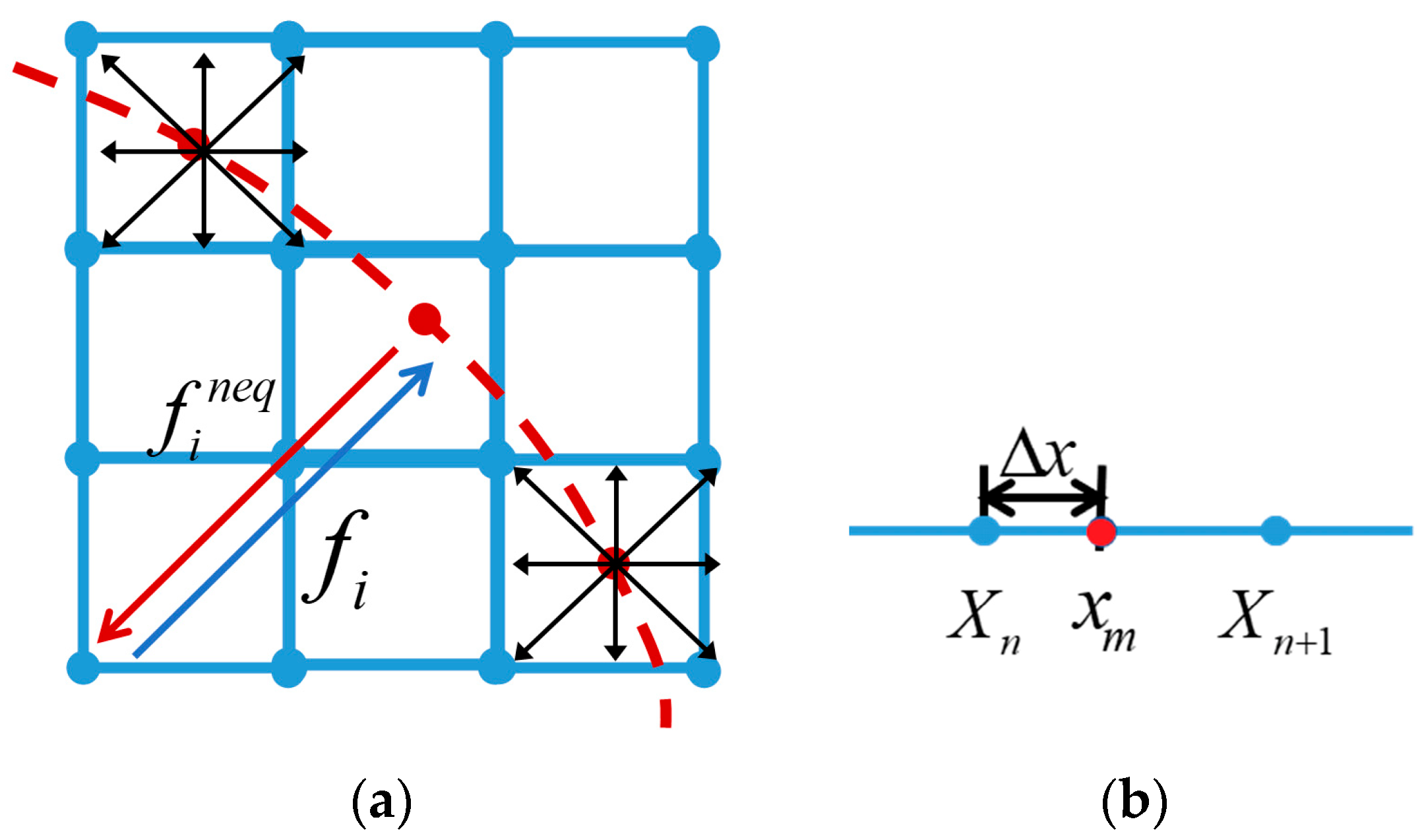
3. Present IB-LBM: A Non-Equilibrium Scheme and an Optimized Approximate Force
- Spread operator and interpolation operator in the non-equilibrium Scheme
- Optimization of the proposed IB-LBM with approximate force on the IB
- The whole process of the present IB-LBM
4. Results
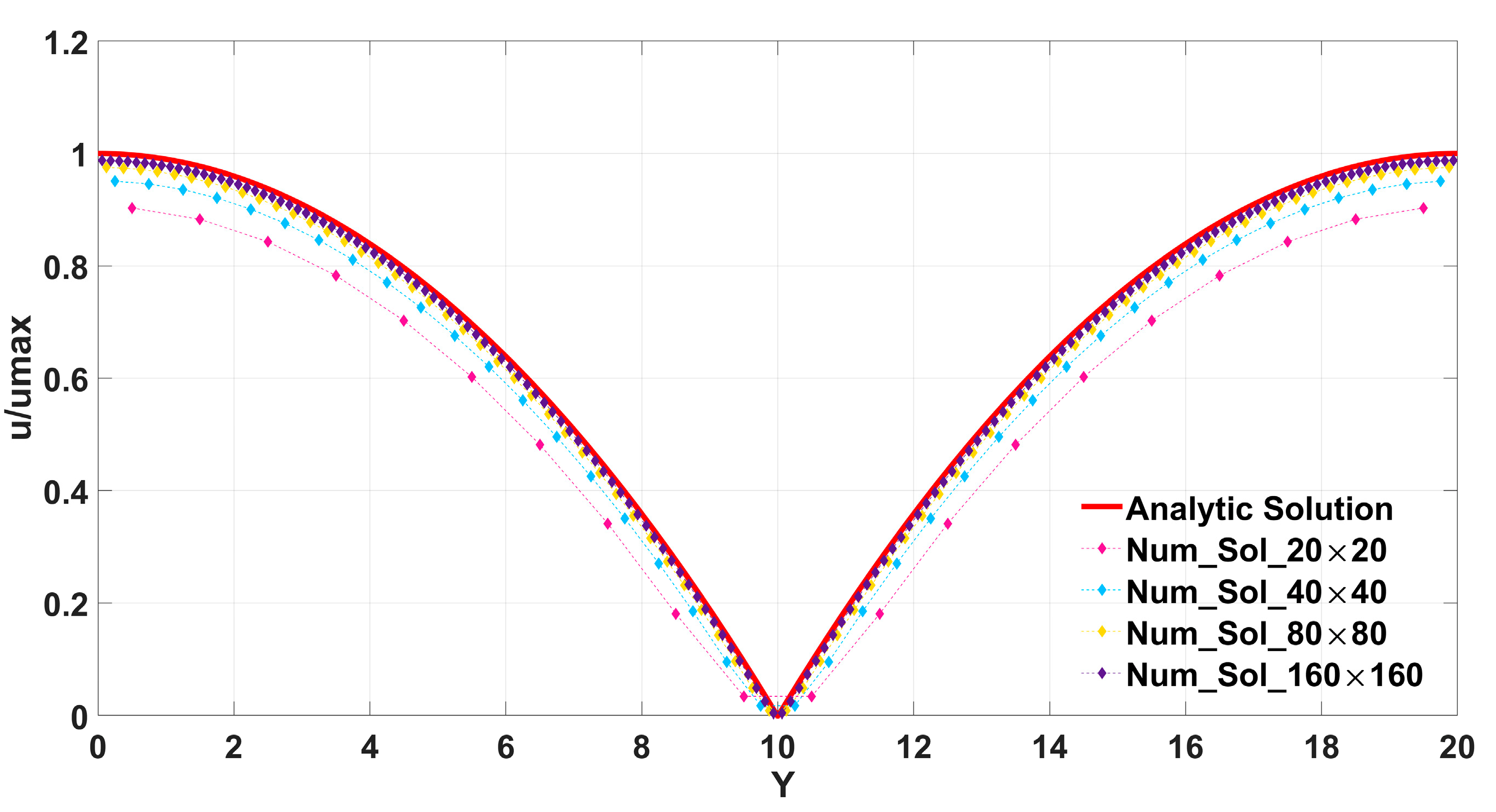
4.1. Symmetrical Poiseuille Flow with an Immersed Boundary
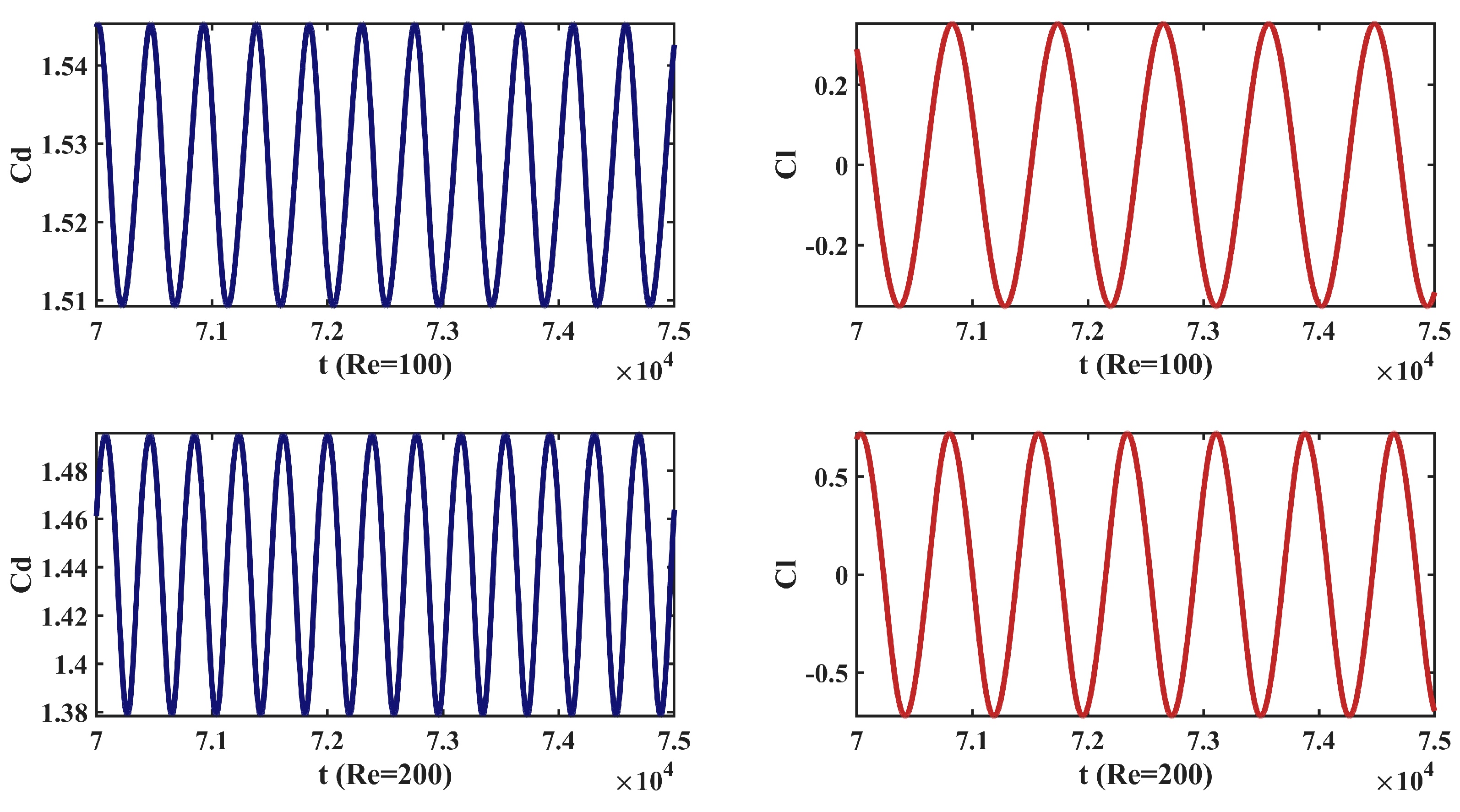
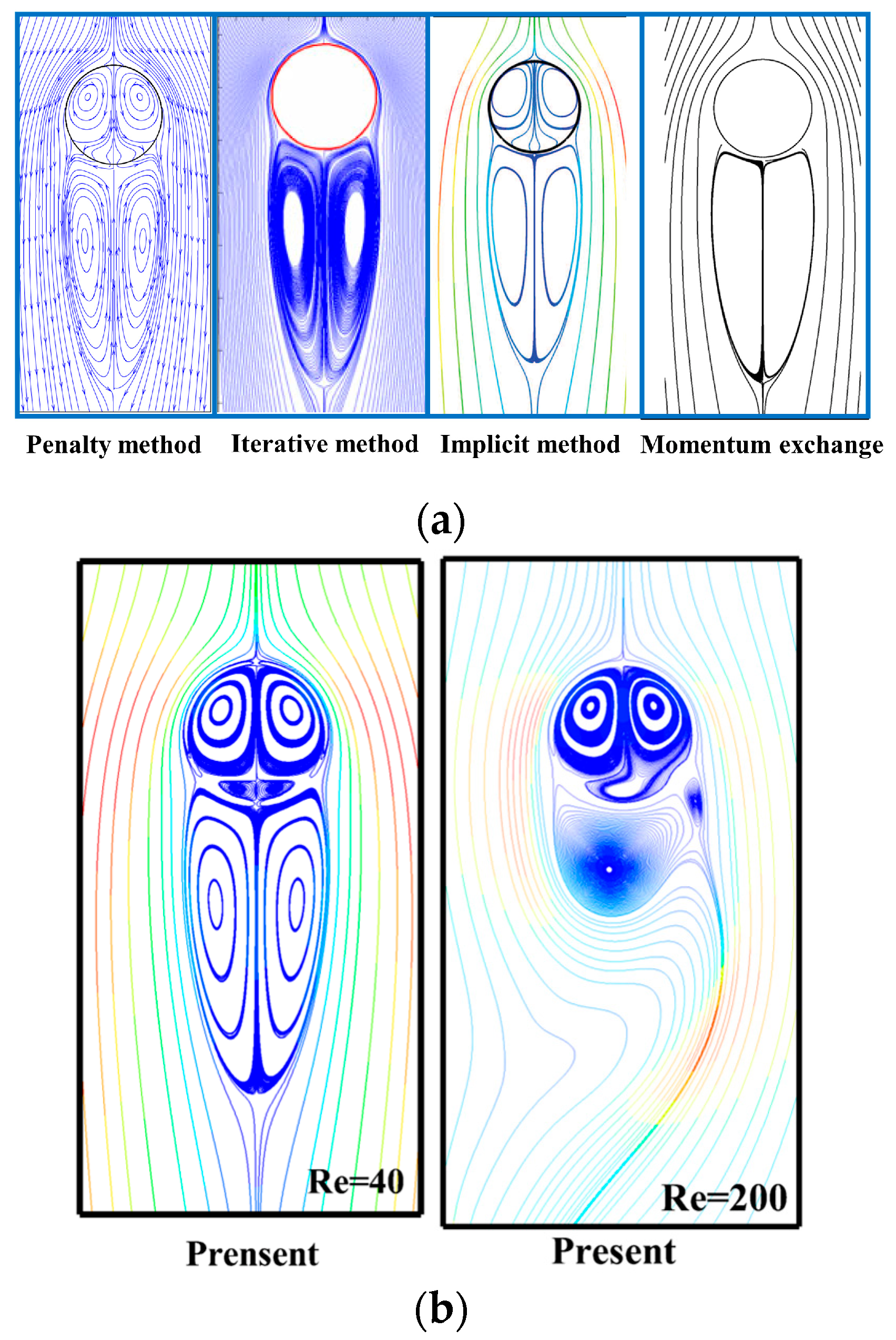
4.2. Flow Past a Fixed Circular Cylinder
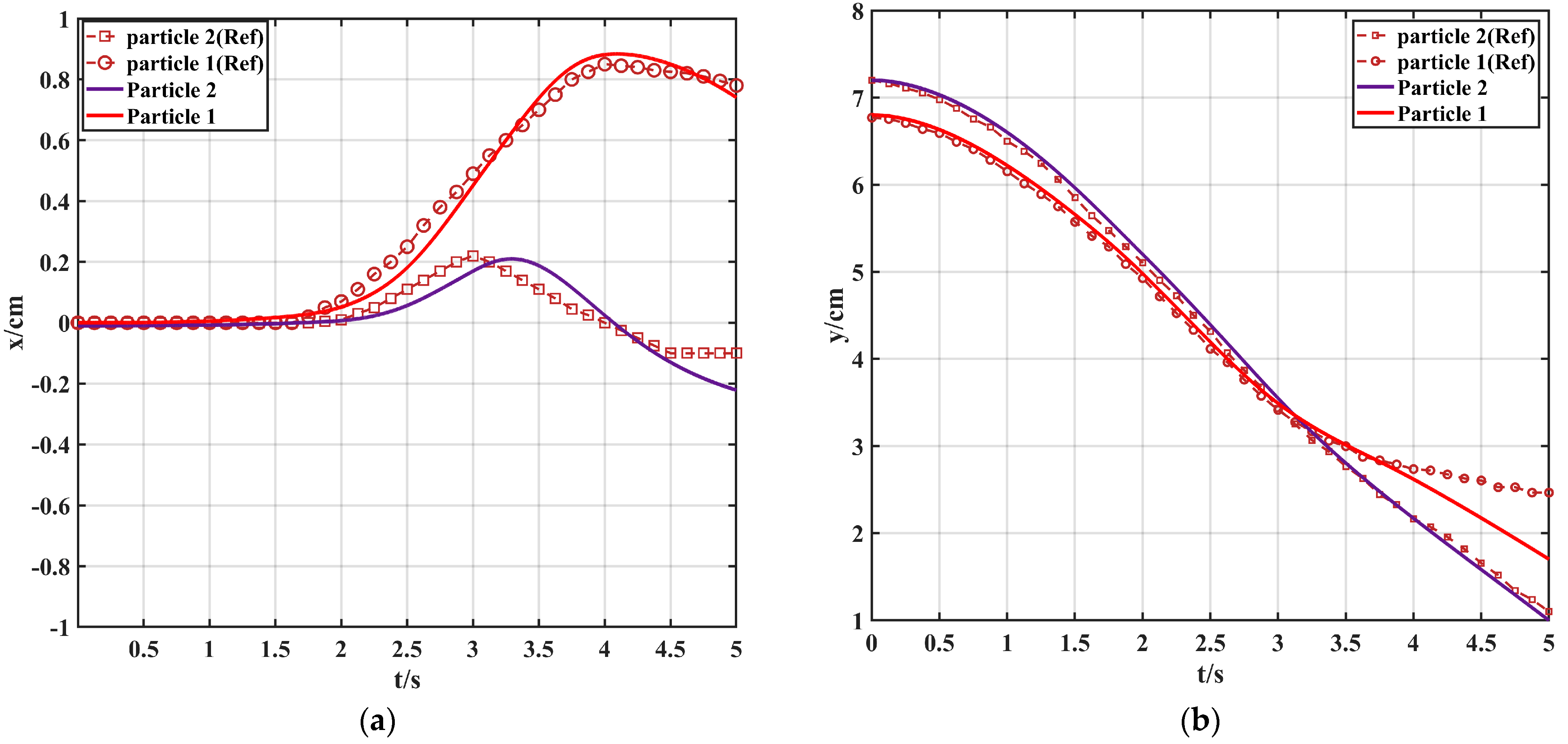
4.3. Sedimentation and Collision of Moving Particles
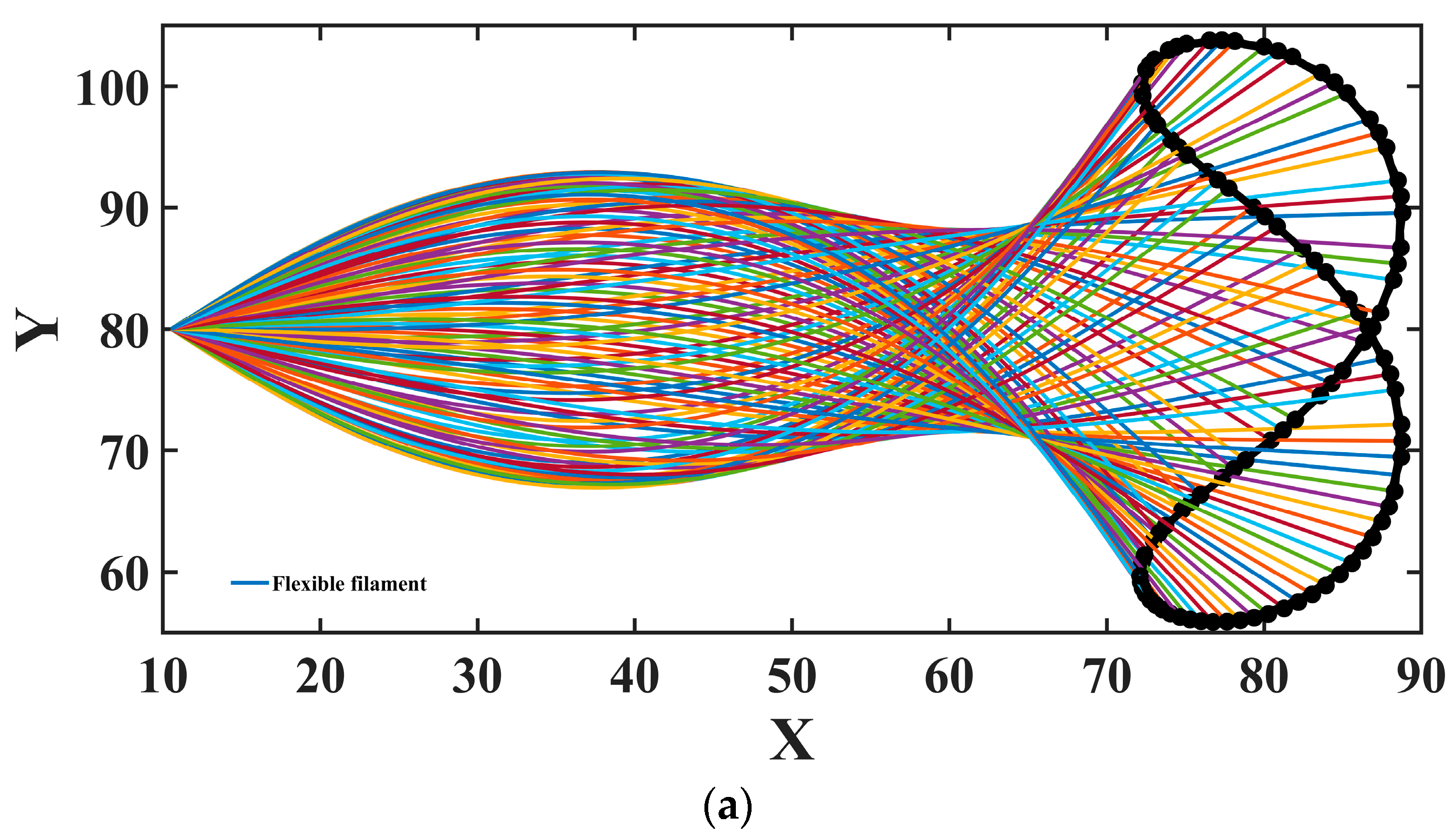
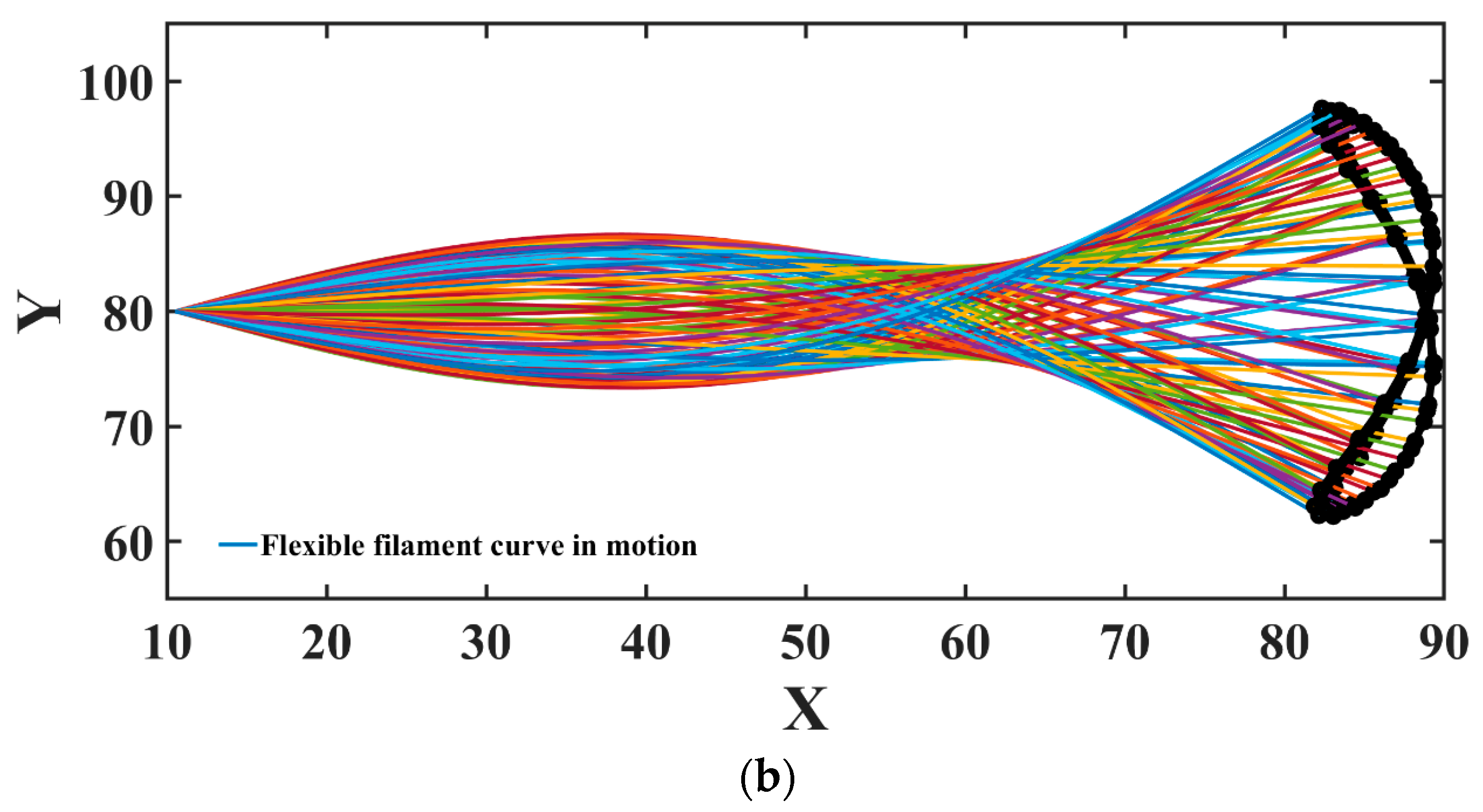
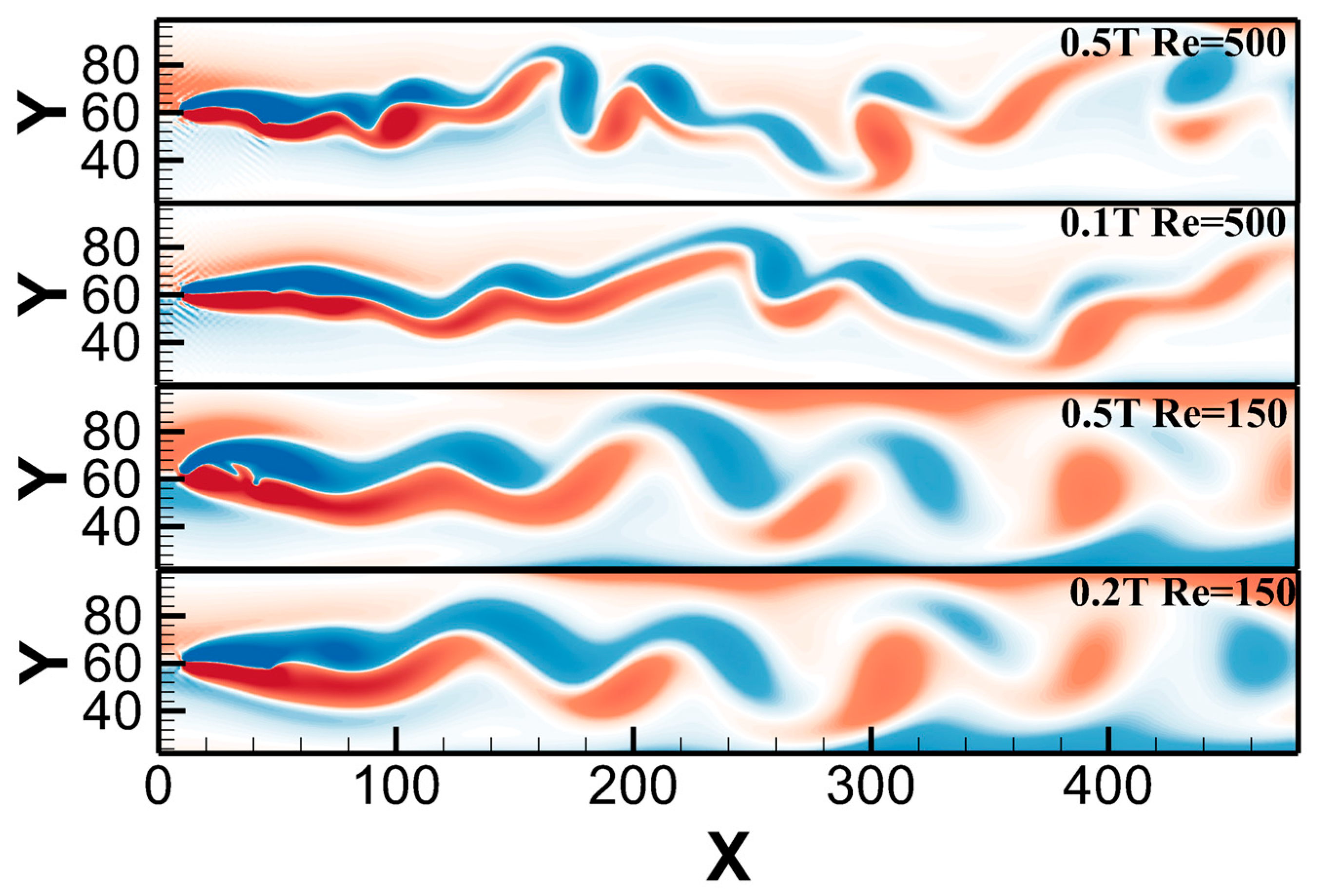
4.4. A Flexible Filament Fixed at One End
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Hou, G.; Wang, J.; Layton, A. Numerical methods for fluid-structure interaction—A review. Commun. Comput. Phys. 2012, 12, 337–377. [Google Scholar] [CrossRef]
- Anderson, R.W.; Elliott, N.S.; Pember, R.B. An arbitrary Lagrangian–Eulerian method with adaptive mesh refinement for the solution of the Euler equations. J. Comput. Phys. 2004, 199, 598–617. [Google Scholar] [CrossRef]
- Longatte, E.; Bendjeddou, Z.; Souli, M. Application of arbitrary Lagrange Euler equationtions to flow-induced vibration problems. J. Pressure Vessel Technol. 2003, 125, 411–417. [Google Scholar] [CrossRef]
- Liu, M.B.; Liu, G. Smoothed particle hydrodynamics (SPH): An overview and recent developments. Arch. Comput. Methods Eng. 2010, 17, 25–76. [Google Scholar] [CrossRef]
- Antoci, C.; Gallati, M.; Sibilla, S. Numerical simulation of fluid interaction by SPH. Comput. Struct. 2007, 85, 879–890. [Google Scholar] [CrossRef]
- Peskin, C.S. Flow patterns around heart valves: A numerical method. J. Comput. Phys. 1972, 10, 252–271. [Google Scholar] [CrossRef]
- Griffith, B.E.; Patankar, N.A. Immersed methods for fluid–structure interaction. Annu. Rev. Fluid Mech. 2020, 52, 421–448. [Google Scholar] [CrossRef]
- Ou, Z.; Chi, C.; Guo, L.; Thévenin, D. A directional ghost-cell immersed boundary method for low Mach number reacting flows with interphase heat and mass transfer. J. Comput. Phys. 2022, 468, 111447. [Google Scholar] [CrossRef]
- Yousefzadeh, M.; Battiato, I. High order ghost-cell immersed boundary method for generalized boundary conditions. Int. J. Heat Mass Transf. 2019, 137, 585–598. [Google Scholar] [CrossRef]
- McNamara, G.R.; Zanetti, G. Use of the Boltzmann equation to simulate lattice-gas automata. Phys. Rev. Lett. 1988, 61, 2332. [Google Scholar] [CrossRef]
- Duda, B.; Fares, E. Application of a Lattice-Boltzmann method to the separated flow over the NASA hump. In Proceedings of the 54th AIAA Aerospace Sciences Meeting, San Diego, CA, USA, 4–8 January 2016; p. 1836. [Google Scholar]
- Krüger, T.; Kusumaatmaja, H.; Kuzmin, A.; Shardt, O. The Lattice Boltzmann Method: Principles and Practice, 1st ed.; Springer International Publishing: Cham, Switzerland, 2017; pp. 22–670. ISBN 978-3-319-44649-3. [Google Scholar]
- Gallivan, M.A.; Noble, D.R.; Georgiadis, J.G.; Buckius, R.O. An evaluation of the bounce-back boundary condition for lattice Boltzmann simulations. Int. J. Numer. Methods Fluids 1997, 25, 249–263. [Google Scholar] [CrossRef]
- Yin, X.; Zhang, J. An improved bounce-back scheme for complex boundary conditions in lattice Boltzmann method. J. Comput. Phys. 2012, 231, 4295–4303. [Google Scholar] [CrossRef]
- Saurabh, K.; Solovchuk, M.; Sheu, T.W.H. Investigating ion transport inside the pentameric ion channel encoded in COVID-19 E protein. Phys. Rev. E 2020, 102, 052408. [Google Scholar] [CrossRef] [PubMed]
- Feng, Z.G.; Michaelides, E.E. The immersed boundary-lattice Boltzmann method for solving fluid–particles interaction problems. J. Comput. Phys. 2004, 195, 602–628. [Google Scholar] [CrossRef]
- Feng, Z.G.; Michaelides, E.E. Proteus: A direct forcing method in the simulations of particulate flows. J. Comput. Phys. 2005, 202, 20–51. [Google Scholar] [CrossRef]
- Niu, X.D.; Shu, C.; Chew, Y.T. A momentum exchange-based immersed boundary-lattice Boltzmann method for simulating incompressible viscous flows. Phys. Lett. A 2006, 354, 173–182. [Google Scholar] [CrossRef]
- Wu, J.; Shu, C. Implicit velocity correction-based immersed boundary-lattice Boltzmann method and its applications. J. Comput. Phys. 2009, 228, 1963–1979. [Google Scholar] [CrossRef]
- Wu, J.; Zhan, J.; Zhao, N.; Wang, T. A robust immersed boundary-lattice Boltzmann method for simulation of fluid-structure interaction problems. Commun. Comput. Phys. 2016, 20, 156–178. [Google Scholar] [CrossRef]
- Kang, S.K.; Hassan, Y.A. A comparative study of direct-forcing immersed boundary-lattice Boltzmann methods for stationary complex boundaries. Int. J. Numer. Methods Fluids 2011, 66, 1132–1158. [Google Scholar] [CrossRef]
- Seta, T.; Rojas, R.; Hayashi, K.; Tomiyama, A. Implicit-correction-based immersed boundary–lattice Boltzmann method with two relaxation times. Phys. Rev. E 2014, 89, 023307. [Google Scholar] [CrossRef]
- Hu, Y.; Yuan, H.; Shu, S.; Niu, X.; Li, M. An improved momentum exchanged-based immersed boundary–lattice Boltzmann method by using an iterative technique. Comput. Math. Appl. 2014, 68, 140–155. [Google Scholar] [CrossRef]
- Yuan, H.Z.; Niu, X.D.; Shu, S.; Li, M.; Yamaguchi, H. A momentum exchange-based immersed boundary-lattice Boltzmann method for simulating a flexible filament in an incompressible flow. Comput. Math. Appl. 2014, 67, 1039–1056. [Google Scholar] [CrossRef]
- Zhou, Q.; Fan, L.S. A second-order accurate immersed boundary-lattice Boltzmann method for particle-laden flows. J. Comput. Phys. 2012, 268, 269–301. [Google Scholar] [CrossRef]
- Wang, W.Q.; Yan, Y.; Liu, G.R. An IB-LBM implementation for fluid-solid interactions with an MLS approximation for implicit coupling. Appl. Math. Model. 2018, 62, 638–653. [Google Scholar] [CrossRef]
- Afra, B.; Nazari, M.; Kayhani, M.H.; Delouei, A.A.; Ahmadi, G. An immersed boundary-lattice Boltzmann method combined with a robust lattice spring model for solving flow–structure interaction problems. Appl. Math. Model. 2018, 55, 502–521. [Google Scholar] [CrossRef]
- Tao, S.; He, Q.; Chen, J.; Chen, B.; Yang, G.; Wu, Z. A non-iterative immersed boundary-lattice Boltzmann method with boundary condition enforced for fluid–solid flows. Appl. Math. Model. 2019, 76, 362–379. [Google Scholar] [CrossRef]
- Wang, Z.; Wei, Y.; Qian, Y. A bounce back-immersed boundary-lattice Boltzmann model for curved boundary. Appl. Math. Model. 2020, 81, 428–440. [Google Scholar] [CrossRef]
- Qin, J.; Kolahdouz, E.M.; Griffith, B.E. An immersed interface-lattice Boltzmann method for fluid-structure interaction. J. Comput. Phys. 2012, 428, 109807. [Google Scholar] [CrossRef]
- Yang, F.; Gu, X.; Xia, X.; Zhang, Q. A peridynamics-immersed boundary-lattice Boltzmann method for fluid-structure interaction analysis. Ocean Eng. 2012, 264, 112528. [Google Scholar] [CrossRef]
- Qin, S.; Jiang, M.; Ma, K.; Su, J.; Liu, Z. Fully resolved simulations of viscoelastic suspensions by an efficient immersed boundary-lattice Boltzmann method. Particuology 2023, 75, 26–49. [Google Scholar] [CrossRef]
- Peskin, C.S. The immersed boundary method. Acta Numer. 2002, 11, 479–517. [Google Scholar] [CrossRef]
- Bhatnagar, P.L.; Gross, E.P.; Krook, M. Title of the article. A model for collision processes in gases. I. Small amplitude processes in charged and neutral one-component systems. Phys. Rev. 1954, 94, 511. [Google Scholar] [CrossRef]
- He, X.; Luo, L.S. Theory of the lattice Boltzmann method: From the Boltzmann equation to the lattice Boltzmann equation. Phys. Rev. E 1997, 56, 6811. [Google Scholar] [CrossRef]
- Guo, Z.; Zheng, C. Discrete lattice effects on the forcing term in the lattice Boltzmann method. Phys. Rev. E 2002, 65, 046308. [Google Scholar] [CrossRef] [PubMed]
- Qian, Y.H.; d’Humières, D.; Lallemand, P. Lattice BGK models for Navier-Stokes equation. Europhys. Lett. 1992, 17, 479. [Google Scholar] [CrossRef]
- Zhao-Li, G.; Chu-Guang, Z.; Bao-Chang, S. Non-equilibrium extrapolation method for velocity and pressure boundary conditions in the lattice Boltzmann method. Chin. Phys. 2002, 11, 366. [Google Scholar] [CrossRef]
- Shan, X.; Chen, H. Lattice Boltzmann model for simulating flows with multiple phases and components. Phys. Rev. E 1993, 47, 1815. [Google Scholar] [CrossRef]
- Carlson, D.R.; Widnall, S.E.; Peeters, M.F. A flow-visualization study of transition in plane Poiseuille flow. J. Fluid Mech. 1982, 121, 487–505. [Google Scholar] [CrossRef]
- Su, S.W.; Lai, M.C.; Lin, C.A. An immersed boundary technique for simulating complex flows with rigid boundary. Comput. Fluids 2007, 36, 313–324. [Google Scholar] [CrossRef]
- Tritton, D.J. Experiments on the flow past a circular cylinder at low Reynolds numbers. J. Fluid Mech. 1959, 9, 547–567. [Google Scholar] [CrossRef]
- Calhoun, D. A Cartesian grid method for solving the two-dimensional stream function-vorticity equations in irregular regions. J. Comput. Phys. 2002, 176, 231–275. [Google Scholar] [CrossRef]
- Zhu, L.; Peskin, C.S. Simulation of a flapping flexible filament in a flowing soap film by the immersed boundary method. J. Comput. Phys. 2002, 179, 452–468. [Google Scholar] [CrossRef]
- Huang, W.X.; Shin, S.J.; Sung, H.J. Simulation of flexible filaments in a uniform flow by the immersed boundary method. J. Comput. Phys. 2007, 226, 2206–2383. [Google Scholar] [CrossRef]
- Xu, Y.Q.; Wang, M.Y.; Liu, Q.Y.; Tang, X.Y.; Tian, F.B. External force-induced focus pattern of a flexible filament in a viscous fluid. Appl. Math. Model. 2018, 53, 369–383. [Google Scholar] [CrossRef]




| Mesh | Rate | Rate | ||
|---|---|---|---|---|
| 0.0955329 | 0.0635722 | |||
| 0.0480705 | 0.99085 | 0.0322156 | 0.98064 | |
| 0.0245173 | 0.97135 | 0.0162343 | 0.98871 | |
| 0.0123796 | 0.98584 | 0.0081475 | 0.99462 |
| Grid | Grid Ratio | ||
|---|---|---|---|
| 2 | 0.0651623 | ||
| 1.11 | 0.0635722 | 0.02440 | |
| 1 | 0.0642422 | 0.01053 | |
| 0.67 | 0.0644133 | 0.00266 |
| Reference | Tritton [42] | Calhoun [43] | Wu [19] | Qin [30] | Hu [23] | Present | |
|---|---|---|---|---|---|---|---|
| Re = 20 | 2.22 | 2.19 | 2.091 | 2.230 | 2.213 | 2.298 | |
| Re = 40 | 1.48 | 1.62 | 1.565 | 1.689 | 1.660 | 1.693 | |
| Re = 100 | 1.33 | 13.364 | 1.510 | 1.418 | 1.527 | ||
| 0.298 | 0.353 | 0.367 | 0.355 | ||||
| 0.175 | 0.163 | 0.169 | 0.166 | 0.176 | |||
| Re = 200 | 1.77 | 1.349 | 1.493 | 1.394 | 1.495 | ||
| 0.67 | 0.718 | 0.712 | 0.723 | ||||
| 0.202 | 0.193 | 0.199 | 0.195 | 0.208 | |||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, B.; Shi, W. A Non-Equilibrium Interpolation Scheme for IB-LBM Optimized by Approximate Force. Axioms 2023, 12, 298. https://doi.org/10.3390/axioms12030298
Liu B, Shi W. A Non-Equilibrium Interpolation Scheme for IB-LBM Optimized by Approximate Force. Axioms. 2023; 12(3):298. https://doi.org/10.3390/axioms12030298
Chicago/Turabian StyleLiu, Bowen, and Weiping Shi. 2023. "A Non-Equilibrium Interpolation Scheme for IB-LBM Optimized by Approximate Force" Axioms 12, no. 3: 298. https://doi.org/10.3390/axioms12030298
APA StyleLiu, B., & Shi, W. (2023). A Non-Equilibrium Interpolation Scheme for IB-LBM Optimized by Approximate Force. Axioms, 12(3), 298. https://doi.org/10.3390/axioms12030298





