Study of Time-Fractional Nonlinear Model Governing Unsteady Flow of Polytropic Gas

Abstract

The present study is concerned with studying the dynamical behavior of two space-dimensional nonlinear time-fractional models governing the unsteady-flow of polytropic-gas (in brief, pGas) that occurred in cosmology and astronomy. For this purpose, two efficient hybrid methods so-called optimal homotopy analysis $\mathbb{J}$-transform method (${}_O$HA$\mathbb{J}$TM) and $\mathbb{J}$-variational iteration transform method ($\mathbb{J}$-VITM) have been adopted. The _OHA$\mathbb{J}$TM is the hybrid method, where optimal-homotopy analysis method (${}_O$HAM) is utilized after implementing the properties of $\mathbb{J}$-transform ($\mathbb{J}$T), and in $\mathbb{J}$-VITM is the $\mathbb{J}$-transform-based variational iteration method. Banach’s fixed point approach is adopted to analyze the convergence of these methods. It is demonstrated that $\mathbb{J}$-VITM is $\mathrm{T}$-stable, and the evaluated dynamics of pGas are described in terms of Mittag–Leffler functions. The proposed evaluation confirms that the implemented methods perform better for the referred model equation of pGas. In addition, for a given iteration, the proposed behavior via ${}_O$HA$\mathbb{J}$TM performs better in producing more accurate behavior in comparison to $\mathbb{J}$-VITM and the methods introduced recently.

1. Introduction

Fractional calculus (FC) is one of the growing/youthful branches of applied mathematics that is a generalized concept of differential equations from an integer order to positive fractional order. It is a preferred selection in modeling complicated physical realistic situations marked by hereditary/memory behaviors. It is because of the nonlocal nature of these operators [1,2]. FC is a useful tool for showcasing the transition of highly complicated nonlinear dynamics with long-term memory effects. In contrast to ordinary derivatives, identifying fractional order derivatives of a function requires its entire history [3]. This nonlocal property, referred as the memory consequence, allows it even more convenient to characterize real-world physical systems using differential equations with fractional derivatives. In recent decades, investigating the evolution of fractional order systems, such as chaos, complexity, stability, bifurcation, and synchronization has emerged as an exciting area of research in areas of research and development [4,5,6,7], and the fractional partial differential equations (FPDEs) are more appropriate for modeling numerous realistic situations such as in optics, earthquake propagation, population growth, volcanic eruption, signal processing, the process of reaction/diffusion, in electrical networking, control theory, hydrology, astrophysics, and in biological systems [8,9,10,11,12,13].

To know about the behavior of a model, one must know about its solution behaviors, and many physical phenomena can be represented by a suitable model in terms of the nonlinear fractional partial differential equations (NFPDEs), and the evaluation of the solution behaviors of such type of model is quite difficult, and so the study of these NFPDEs is of vital importance. In the last three decades, various rigorous new techniques are investigated to elucidate a system of NFPDEs. In consequence, Liao [14] developed a rigorous technique so-called homotopy analysis method for studying many types of nonlinear partial differential equations (NPDEs) like differential-integral/algebraic equations/partial differential equations/ordinary differential equations and associated coupled systems or the fractional models of the above-mentioned types equations. Differ from all the perturbation/nonperturbation approaches for nonlinear differential equations (NDEs), HAM generates an effective/easy technique to assure the convergent solutions by suitable selection of different base functions (see [9,13,15] and inside articles for more details).

In this article, the fractional order model of gas-dynamic equations administering the development of the two-space dimensional unsteady flow of an ideal gas has been studied. Write $P=K{\rho }^{1+(1/\kappa )}$, where $\rho =U/V\to$ energy density, $U\to$ total energy of the gas, $V\to$ container volume, $\kappa \to$ polytropic index, and $K\to$ a constant. In the sequel, degenerate electron gas and adiabatic gas are two instances of such types of gases. The investigation of polytropic gases identified an essential job in cosmology and astronomy [16], and its behavior is found dark energy-like [11], and the special case of the pGas model has been utilized in astrophysics in stellar wind and accretion problems. In recent years, the researchers generalized the model of gas-dynamic equations governing the advancement of unsteady progression of an ideal-gas of fraction order [17,18]. We need both evolution equations for $\rho$ and P due to the energy density and $\rho$ and polytropic index. The value of $P=K{\rho }^{1+(1/k)}$ in the below system (1) is due to the dynamics of a strongly nonlocal reaction–diffusion population model [19]. The fractional model of equations of a pGas [15,20] given below in (1) via two hybrid techniques, namely - ${}_O$HA$\mathbb{J}$TM and $\mathbb{J}$-VITM

$$\begin{array}{cc}\hfill & {}_{\tau }{\mathcal{D}}_C^{\alpha }{\wp }_1+{\wp }_1\frac{\partial {\wp }_1}{\partial z_1}+{\wp }_2\frac{\partial {\wp }_1}{\partial z_2}+\frac{1}{\rho }\frac{\partial P}{\partial z_1}=0\hfill \\ \hfill & {}_{\tau }{\mathcal{D}}_C^{\alpha }{\wp }_2+{\wp }_1\frac{\partial {\wp }_2}{\partial z_1}+{\wp }_2\frac{\partial {\wp }_2}{\partial z_2}+\frac{1}{\rho }\frac{\partial P}{\partial z_2}=0\hfill \\ \hfill & {}_{\tau }{\mathcal{D}}_C^{\alpha }\rho +{\wp }_1\frac{\partial \rho }{\partial z_1}+{\wp }_2\frac{\partial \rho }{\partial z_2}+\rho \frac{\partial {\wp }_1}{\partial z_1}+\rho \frac{\partial {\wp }_2}{\partial z_2}=0\hfill \\ \hfill & {}_{\tau }{\mathcal{D}}_C^{\alpha }P+{\wp }_1\frac{\partial P}{\partial z_1}+{\wp }_2\frac{\partial P}{\partial z_2}+\Omega P\frac{\partial {\wp }_1}{\partial z_1}+\Omega P\frac{\partial {\wp }_2}{\partial z_2}=0\hfill \end{array}$$

(1)

with initial condition

$${\wp }_1(z_{1,2},0)=f_1\left(z_{1,2}\right), {\wp }_2(z_{1,2},0)=f_2\left(z_{1,2}\right), \rho (z_{1,2},0)=f_3\left(z_{1,2}\right), P(z_{1,2},0)=f_4\left(z_{1,2}\right), z_{1,2}=(z_1,z_2),$$

where ${\wp }_1(z_{1,2},\tau )$ and ${\wp }_2(z_{1,2},\tau )\to$velocity components, $\rho (z_{1,2},\tau )$ is the density, $P(z_{1,2},\tau )\to$ pressure and $\Omega \to$ratio of specific heat and refers adiabatic index. ${}_{\tau }{\mathcal{D}}_C^{\alpha }(·)$ is the Caputo-fractional differential operator (C-FDO) as defined below:

Definition 1

([1,2]). The C-FDO  ${}_{\tau }{\mathcal{D}}_C^{\alpha }\phi (z_{1,2},\tau )$ of order  $\kappa -1<\alpha \le \kappa$ of a function $\phi \in C_{\mu },$ $\mu \ge -1$  is defined by  ${}_{\tau }{\mathcal{D}}_C^{\kappa }\phi (z_{1,2},\tau ):=\frac{{\partial }^{\kappa }\phi (z_{1,2},\tau )}{\partial {\tau }^{\kappa }}$ and

• ${}_{\tau }{\mathcal{D}}_C^{\alpha }\phi (z_{1,2},\tau )={}_{\tau }{\mathcal{D}}_C^{-(\kappa -\alpha )}{}_{\tau }{\mathcal{D}}_C^{\kappa }\phi (z_{1,2},\tau )=\frac{1}{\Gamma (\kappa -\alpha )}{\int }_0^{\tau }{(\tau -ϵ)}^{\kappa -(\alpha +1)}\frac{{\partial }^{\kappa }\phi (z_{1,2},ϵ)}{\partial {ϵ}^{\kappa }}dϵ,$
• $whenever\kappa -1<\alpha <\kappa$

In addition, let${}_{\tau }{\mathcal{D}}_C^{-\alpha }\phi (z_{1,2},\tau )$is the αth order Riemann–Liouville fractional integral operator (RLFIO) on φ. Then

• ${}_{\tau }{\mathcal{D}}_C^0\phi (z_{1,2},\tau ):=\phi (z_{1,2},\tau )$
• and
• ${}_{\tau }{\mathcal{D}}_C^{-\alpha }\phi (z_{1,2},\tau )=\frac{1}{\Gamma \left(\alpha \right)}{\int }_0^{\tau }{(\tau -ϵ)}^{\alpha -1}\phi (z_{1,2},ϵ)dϵ, \alpha >0, \tau >0.$

The readers are referred to [1,2,21,22] for more details on fractional calculus. The researchers have beendeveloped/ implemented various rigorous methods for studying behaviors of various models occurred in terms of NFPDEs (see [22,23,24,25,26,27,28,29,30,31,32,33,34,35,36,37]). The behavior of the integer order system of the referred model equations was analyzed using distinct techniques like Adomian decomposition method (ADM) [38], variational iteration technique (VIT) [17], and HAM [18]. Recently, numerical simulation and behavior of fractional order system have been investigated by fractional natural decomposition method (NDM) [20] and q-homotopy analysis transform method (q-HATM) [15]. In the present work, the novel integral transform called $\mathbb{J}$-transform is implemented in combination with two efficient techniques, namely oHAM and VIM to investigate the nonlinear time-fractional model governing unsteady flow of polytropic. The main strategy of our work in considering the $\mathbb{J}$-transform is that it is the generalized form of the Laplace transform and the Elzaki transform. Also, in case of the $\mathbb{J}$-transform, we will get the two-dimensional frequency domain, which will give us more degree of freedom to analyze the respective solutions. The proposed fractional model interprets the most realistic behavior for considered fractional orders and which states the originality of the paper. The relative error solutions are presented in terms of logarithmic plots for different fractional orders. We have achieved the faster rate of convergence of the obtained series solution to the exact solution with the help of optimal value of the convergence control parameter.

The rest part of the work is structured as follows: Section 2 reports some basic literature to complete understanding of the work. In Section 3, we report the basic procedure for $\mathbb{J}$-VITM and its stability/convergence analysis. In Section 4, we report the basic procedure for ${}_O$HA$\mathbb{J}$TM and its convergence analysis. Validity/effectiveness/efficiency of the aforesaid methods is tested in Section 5 by considering test examples of the fractional model equation of pGas. At last, concluding remark is reported in Section 6.

2. Basic Concepts

Banach’s fixed point approach and $\mathbb{J}$T-based basics are revisited to understand the rest part of the study. Let us denote $\Pi =(\Pi ,d)$ as a metric space.

Definition 2

([39]). Let $\mathrm{T}$ be a contraction on metric space Π; this is a map that satisfies the following condition

$$d(\mathrm{T}y,\mathrm{T}{y}_1)\le \gamma d(y,y_1)y,y_1\in \Pi ,$$

(2)

for some positive real $\gamma \in {\mathbb{R}}^{+}$ less than unity, i.e., $0<\gamma <1$, where ${\mathbb{R}}^{+}$ be set of positive reals.

Theorem 1

(Banach’s Fixed-Point Theorem [39]). A contraction $\mathrm{T}$ over complete space Π always has a unique fixed point.

In the sequel, if ${\left\{y_{\lambda }\right\}}_{\lambda =1}^{\infty }$ is a iterative sequence formulated via the iterative procedure $y_{\lambda +1}=\mathrm{T}{y}_{\lambda }$ with $y_0\in \Pi$ (arbitrary) such that $y_{\lambda }$ approaches the unique fixed point y as $\lambda \to \infty$, the error estimates are evaluated as follows

• $d(y_{\lambda },y)\le \frac{{\gamma }^{\lambda }}{1-\gamma }d(y_0,y_1),$ (prior-etimate), and
• and
• $d(y_{\lambda },y)\le \frac{\gamma }{1-\gamma }d(y_{\lambda -1},y_{\lambda }).$ (posterior-estimate).

Theorem 2

([40,41]). A self-map $\mathrm{T}$ defined over Π (Banach space) is termed as Picard T-stable if the condition $d(\mathrm{T}y,\mathrm{T}{y}_1)\le \kappa d(y,\mathrm{T}y)+\gamma d(y,y_1), \forall y,y_1\in \Pi$ holds true for some non-negative real κ, and γ with $0\le \gamma <1$.

$\mathbb{J}$-Transform and Its Properties

The transformation

$$\mathbb{J}\left[\psi \left(\tau \right)\right](s,\vartheta )=\Psi (s,\vartheta ):=\vartheta {\int }_0^{\infty }{e}^{\left(\frac{-s\tau }{\vartheta }\right)}\psi \left(\tau \right)d\tau ,$$

(3)

is referred to as $\mathbb{J}$-transform of $\psi \in \mathcal{F}$ (provided it exists), $s,\vartheta$ are transformed variables, and $\mathcal{F}$ is the set functions of exponential order satisfying the following conditions

$\mathcal{F}=\left\{\psi :\exists m_1,m_2>0, 0<\Gamma <\infty \mathrm{such} \mathrm{that} \left|\psi \left(\tau \right)\right|\le \Gamma exp\left(\frac{\left|\tau \right|}{r_j}\right), \mathrm{if} \tau \in {(-1)}^j\times [0,\infty )\right\},$

The properties of $\mathbb{J}$T are listed is the following

Lemma 1

(Properties of $\mathbb{J}$T, [42]).Let $\mathcal{G}(z,s,\vartheta )$ and $\Psi (z,s,\vartheta )$ are $\mathbb{J}$T of $g(z,\tau ),\psi (z,\tau )\in \mathcal{F}$, respectively. Then

(a)

$\mathbb{J}\left[\frac{{\tau }^{\kappa \alpha +\lambda }}{\Gamma (1+\lambda +\kappa \alpha )}\right](s,\vartheta )=\frac{{\vartheta }^{\kappa \alpha +\lambda +2}}{s^{\kappa \alpha +\lambda +1}}, \lambda ,\kappa =0,1,2,\dots$

(b)

$\mathbb{J}\left[\frac{{\partial }^{\kappa }g(z,\tau )}{\partial {\tau }^{\kappa }}\right](s,\vartheta )=\frac{s^{\kappa }}{{\vartheta }^{\kappa }}\mathcal{G}(z,s,\vartheta )-{\sum }_{\ell =1}^{\kappa }\frac{s^{\kappa -\ell }}{{\vartheta }^{\kappa -(\ell +1)}}\frac{{\partial }^{\ell -1}g(z,0^{+})}{\partial {\tau }^{\ell -1}}, \kappa \ge 1;$

(c)

$\mathbb{J}\left[A_{1}g(z,\tau )+A_2\psi (z,\tau )\right](s,\vartheta )=A_1\mathcal{G}(z,s,\vartheta )+A_2\Psi (z,s,\vartheta );$

(d)

$\mathbb{J}\left[(g\ast \psi )(z,\tau )\right](s,\vartheta )=\frac{1}{\vartheta }\mathcal{G}(z,s,\vartheta )\Psi (z,s,\vartheta )$, where$g\ast \psi$is the convolution of g and ψ.

The properties of $\mathbb{J}$T for fractional calculus [31] that we use to study the behavior of referred model equation is mentioned below

Lemma 2.

If $\ominus (z_{1,2},s,\vartheta )$ is the $\mathbb{J}$-transform of$\psi (z_{1,2},\tau )\in \mathcal{F}$, then

(i)

$\mathbb{J}\left[{}_{\tau }{\mathcal{D}}_C^{-\alpha }\psi (z_{1,2},\tau )\right](s,\vartheta )=\frac{{\vartheta }^{\alpha }}{s^{\alpha }}\Psi (z_{1,2},s,\vartheta ),$

(ii)

$\mathbb{J}\left[{}_{\tau }{\mathcal{D}}_C^{\alpha }\psi (z_{1,2},\tau )\right](s,\vartheta )={\left(\frac{s}{\vartheta }\right)}^{\alpha }\Psi (z_{1,2},s,\vartheta )-{\sum }_{\ell =1}^{\kappa }\frac{s^{\alpha -\ell }}{{\vartheta }^{\alpha -(\ell +1)}}\frac{{\partial }^{\ell -1}\psi (z,0^{+})}{\partial {\tau }^{\ell -1}},\kappa -1<\alpha \le \kappa \in \mathbb{N}.$

where ${}_{\tau }{\mathcal{D}}_C^{\alpha }\psi (z_{1,2},\tau )$, ${}_{\tau }{\mathcal{D}}_C^{-\alpha }\psi (z_{1,2},\tau )$ denote C-FDO and Riemann–Liouville FIO of ψ of order α.

Proof. 

The proof is reported in [31]. □

3. Procedure of Variational Iteration Technique (VIT)

The variational theory-based technique so-called VIT is an efficient technique introduced by He [43] for the study of various models that occurred in terms of classical differential equations. After He’s seminal work, VIT and its modified forms has been introduced for studying various types of nonlinear problems of integer orders [44,45,46,47] and fractional order [47,48,49,50,51].

Consider time-fractional nonlinear partial differential equation (TF-NPDE).

$${}_{\tau }{\mathcal{D}}_C^{\alpha }\psi (z_{1,2},\tau )+\mathcal{T}\psi (z_{1,2},\tau )=0,\kappa -1<\alpha \le \kappa ,$$

(4)

where $z_{1,2}=(z_1,z_2)$ be space-variable of 2-dimensions, ${}_{\tau }{\mathcal{D}}_C^{\alpha }(·)$ is Caputo FDO [23,24,25], $\mathcal{T}(·)$ nonlinear differential operator involving linear operators and nonhomogeneous/source term as well, and $\kappa \in \mathbb{N}$.

The basic procedure of VIT for TF-NPDE, the correction functional of (4) in mVIT [47], is given via

$${\psi }_{\lambda +1}\left(z_{1,2},\tau \right)={\psi }_{\lambda }\left(z_{1,2},\tau \right)+{\int }_0^{\tau }\theta (\tau ,ϵ)\left[{}_{\tau }{\mathcal{D}}_C^{\alpha }\psi (z_{1,2},ϵ)+\mathcal{T}{\tilde{\psi }}_{\lambda }\left(z_{1,2},ϵ\right)\right]dϵ,$$

(5)

where $\theta (\tau ,ϵ)$ refers to Lagrange multiplier to be determine, ${\psi }_{\lambda }$ is $\lambda$th-iteration solution, and ${\tilde{\psi }}_{\lambda }$ is the restricted variation [52]. The evaluation of the Lagrange multiplier is a tedious task in studying the behavior of TF-NPDE. On imposing optimality criteria to the functional as in (5), we have

$$\delta {\psi }_{\lambda +1}\left(z_{1,2},\tau \right)=\delta {\psi }_{\lambda }\left(z_{1,2},\tau \right)+\delta {\int }_0^t\theta (\tau ,ϵ){}_{\tau }{\mathcal{D}}_C^{\alpha }{\psi }_{\lambda }\left(z_{1,2},ϵ\right)dϵ=0,$$

The evaluation of the Lagrange multiplier $\theta (\tau ,ϵ)$ in the above equation is tough for fraction case $(\alpha \ne \kappa )$ [51]. The implementation of the properties of an integral transform with variational theory [53,54] makes the evaluation procedure for finding the optimal value of the Lagrange multiplier easily.

3.1. Procedure of $\mathbb{J}$-VITM for NFPDEs

The $\mathbb{J}$-VITM is a hybrid method that is based on properties of $\mathbb{J}$T and variational theory (see [31]) that we implemented for studying nonlinear fractional partial differential equations (NFPDEs).

Impose $\mathbb{J}$T to NFPDEs (4) and adopt the property ${}_{\tau }{\mathcal{D}}_C^{\alpha }\psi (z_{1,2},\tau )$ of $\mathbb{J}$T from Theorem 2(ii), we have

$${\left(\frac{s}{\vartheta }\right)}^{\alpha }\psi (z_{1,2},s,\vartheta )-\sum _{\ell =1}^{\kappa }\frac{s^{\alpha -\ell }}{{\vartheta }^{\alpha -(\ell +1)}}{\left.\frac{{\partial }^{\ell -1}\psi }{\partial {\tau }^{\ell -1}}\right|}_{\tau =0}+\mathbb{J}\left[\mathcal{T}{\tilde{\psi }}_{\lambda }\left(z_{1,2},ϵ\right)\right]\theta (s,\vartheta )=0.$$

(6)

In sequel to modified variational iteration technique, correction functional for (6) constructed as

$$\begin{array}{cc}\hfill {\psi }_{\lambda +1}(z_{1,2},s,\vartheta )& ={\psi }_{\lambda }(z_{1,2},s,\vartheta )+\theta (s,\vartheta )\left({\left(\frac{s}{\vartheta }\right)}^{\alpha }{\psi }_{\lambda }(z_{1,2},s,\vartheta )-\sum _{\ell =1}^{\kappa }\frac{s^{\alpha -\ell }}{{\vartheta }^{\alpha -(\ell +1)}}{\left.\frac{{\partial }^{\ell -1}{\psi }_{\lambda }}{\partial {\tau }^{\ell -1}}\right|}_{\tau =0}\right)\hfill \\ \hfill & -\theta (s,\vartheta )\mathbb{J}\left[\tilde{\mathcal{T}}{\psi }_{\lambda }\left(z_{1,2},\tau \right)\right](s,\vartheta ).\hfill \end{array}$$

(7)

where ${\tilde{\psi }}_{\lambda }$ and $\tilde{\mathcal{T}}$ restricted variations, i.e., $\delta {\tilde{\varphi }}_{\lambda }=0$ and $\delta \tilde{\mathcal{T}}=0$.

The variational operator $\delta$ to (7) with the above-mentioned property leads to

$$\delta {\psi }_{\lambda +1}(z_{1,2},s,\vartheta )=\delta {\psi }_{\lambda }(z_{1,2},s,\vartheta ) \left(1+\theta (s,\vartheta ){\left(\frac{s}{\vartheta }\right)}^{\alpha }\right),$$

(8)

The optimality condition: $\delta {\psi }_{\lambda +1}(z_{1,2},s,\vartheta )=0$ for (7) in (8) leads to the optimal value of the Lagrange multiplier $\theta (s,\vartheta )=-{\left(\frac{\vartheta }{s}\right)}^{\alpha }$. Thus, (7) reduces to

$$\begin{array}{cc}\hfill {\psi }_{\lambda +1}(z_{1,2},s,\vartheta )& =\sum _{\ell =1}^{\kappa }\left(\frac{{\vartheta }^{\ell +1}}{s^{\ell }}\right){\left.\frac{{\partial }^{\ell -1}{\psi }_{\lambda }}{\partial {\tau }^{\ell -1}}\right|}_{\tau =0}-{\left(\frac{\vartheta }{s}\right)}^{\alpha }\mathbb{J}\left[\mathcal{T}{\psi }_{\lambda }\left(z_{1,2},\tau \right)\right](s,\vartheta ).\hfill \end{array}$$

(9)

The inverse $\mathbb{J}$T operator with (9) leads to

$$\begin{array}{c}\hfill {\psi }_{\lambda +1}(z_{1,2},\tau )=\mathrm{T}{\psi }_{\lambda }(z_{1,2},\tau ),\lambda =0,1,2,\dots \end{array}$$

(10)

where

• $\mathrm{T}{\psi }_{\lambda }(z_{1,2},\tau )={\psi }_{\lambda }^0(z_{1,2},\tau )-{\mathbb{J}}^{-1}\left[{\left(\frac{\vartheta }{s}\right)}^{\alpha }\mathbb{J}\left[\mathcal{T}{\psi }_{\lambda }\left(z_{1,2},\tau \right)\right](s,\vartheta )\right],$ and
• ${\psi }_{\lambda }^0(z_{1,2},\tau )={\sum }_{\ell =1}^{\kappa }\left(\frac{{\tau }^{\ell -1}}{\Gamma (\ell )}\right){\left.\frac{{\partial }^{\ell -1}{\psi }_{\lambda }}{\partial {\tau }^{\ell -1}}\right|}_{\tau =0}.$

it is the desired $(\lambda +1)$th iterative solution of NFPDEs (4), and when $\kappa =1$, the solution at $(\lambda +1)$th iteration read from (10) as

$${\psi }_{\lambda +1}(z_{1,2},\tau )=\mathrm{T}{\psi }_{\lambda }(z_{1,2},\tau )={\psi }_{\lambda }(z_{1,2},0)-{\mathbb{J}}^{-1}\left[{\left(\frac{\vartheta }{s}\right)}^{\alpha }\mathbb{J}\left[\mathcal{T}{\psi }_{\lambda }\left(z_{1,2},\tau \right)\right](s,\vartheta )\right].$$

(11)

3.2. Convergence and Stability Analysis of $\mathbb{J}$-VITM

The analysis of convergence and stability for the aforesaid $\mathbb{J}$-VITM is provided in the following theorem. For sake of convenience, we read ${\psi }_n$ in place of ${\psi }_n(z_{1,2},\tau )$ throughout this section

Theorem 3

(Stability analysis). Let a self-map $\mathrm{T}:\mathbf{B}\to \mathbf{B}$, where $(\mathbf{B},\parallel ·\parallel )$ is the Banach space; then, the iterative results via iteration formula (10) are: ${\psi }_{\lambda +1}(X,\tau )=\mathrm{T}{\psi }_{\lambda }(X,\tau )$ is Picard $\mathrm{T}$ stable if $\exists {\eta }_0 >0$ for which the following axioms hold true for every τ.

(a)

$∥\mathcal{T}\left({\psi }_p\right)-\mathcal{T}\left({\psi }_n\right)∥\le ∥\mathcal{T}\left({\psi }_p-{\psi }_n\right)∥\le {\eta }_0\parallel {\psi }_p-{\psi }_n\parallel$

(b)

$\theta ={\eta }_0∥\frac{{\tau }^{\alpha }}{\Gamma (\alpha +1)}∥<1$.

Proof. 

Let $p,n\in \mathbb{N}$.Then,

$$\begin{array}{cc}\hfill \mathrm{T}{\psi }_p-\mathrm{T}{\psi }_n& ={\psi }_p^0-{\psi }_n^0+{\mathbb{J}}^{-1}\left[{\left(\frac{\vartheta }{s}\right)}^{\alpha }\mathbb{J}\left[\mathcal{T}{\psi }_p\right](s,\vartheta )\right]-{\mathbb{J}}^{-1}\left[{\left(\frac{\vartheta }{s}\right)}^{\alpha }\mathbb{J}\left[\mathcal{T}{\psi }_n\right](s,\vartheta )\right],\hfill \\ \hfill & ={\psi }_p^0-{\psi }_n^0+{\mathbb{J}}^{-1}\left[{\left(\frac{\vartheta }{s}\right)}^{\alpha }\mathbb{J}\left[\mathcal{T}{\psi }_p-\mathcal{T}{\psi }_n\right](s,\vartheta )\right],\hfill \end{array}$$

(12)

as ${\psi }_p^0={\psi }_n^0$ at each iteration holds from the initial condition. Imposing norm to both sides of (12) with condition (a) leads to

$$\begin{array}{cc}\hfill ∥\mathrm{T}{\psi }_p-\mathrm{T}{\psi }_n∥& \le {\mathbb{J}}^{-1}\left[{\left(\frac{\vartheta }{s}\right)}^{\alpha }\mathbb{J}\left[∥\mathcal{T}{\psi }_p-\mathcal{T}{\psi }_n∥\right](s,\vartheta )\right]\hfill \\ \hfill & \le {\eta }_0∥{\psi }_p-{\psi }_n∥\left({\mathbb{J}}^{-1}\left[\frac{{\vartheta }^{2+\alpha }}{s^{\alpha }}\right]\right)\le \theta ∥{\psi }_p-{\psi }_n∥,\hfill \end{array}$$

and this can be expressed in the following form

$$\begin{array}{c}\hfill ∥\mathrm{T}{\psi }_p-\mathrm{T}{\psi }_n∥\le \beta \parallel {\psi }_p-\mathrm{T}{\psi }_p\parallel +\theta \parallel {\psi }_p-{\psi }_n\parallel , \mathrm{for} \beta \ge 0\end{array}$$

(13)

which confirms that the proposed $\mathbb{J}$-VITM is Picard $\mathrm{T}$ stable whever $\theta <1$ (see Theorem 2). □

Theorem 4

(Convergence analysis). In a Banach space $\mathbf{B}=\left(C[\Omega \times (0,T\left)\right],\parallel ·\parallel \right)$, let ${\left\{{\psi }_n\right\}}_1^{\infty }$ be a sequence from the iteration procedure (10): ${\psi }_{\lambda +1}=\mathrm{T}{\psi }_{\lambda }$, where $\mathrm{T}$ be associated self-map on $\mathbf{B}$. Then

(a)

${\left\{{\psi }_n\right\}}_1^{\infty }$with${\psi }_0\in \mathbf{B}$ (initial value) is convergent.

(b)

A unique fixed point exist for  $\mathrm{T}$in $\mathbf{B}$.

(c)

In κth order iterative results, the error bounds as derived as

• $∥\psi -{\psi }_{\kappa }∥\le \frac{{\theta }^{\kappa }}{1-\theta }∥{\psi }_1-{\psi }_0∥$   (Prior-estimate of error),
• and
• $∥\psi -{\psi }_{\kappa }∥\le \frac{1}{1-\theta }∥{\psi }_1-{\psi }_0∥, 0\le \theta <1$ (posterior-error estimate)

Proof. 

For the complete proof please visit [31]. □

4. Basic Procedure of ${}_O$HA$\mathbb{J}$TM

The basic solution procedure of ${}_O$HA$\mathbb{J}$TM for NFPDEs (4) is derived in [31], is reported in the following. On operating $\mathbb{J}$T to NFPDEs (4) with the help of the property of $\mathbb{J}$T to get (6) that can be expressed as

$$\mathbb{J}\left[\psi (z_{1,2},\tau )\right](s,\vartheta )-\sum _{\ell =1}^{\kappa }\frac{{\vartheta }^{\ell +1}}{s^{\ell }}{\left.\frac{{\partial }^{\ell -1}\psi }{\partial {\tau }^{\ell -1}}\right|}_{\tau =0}+{\left(\frac{\vartheta }{s}\right)}^{\alpha }\mathbb{J}\left[\mathcal{T}{\tilde{\psi }}_{\lambda }\left(z_{1,2},\tau \right)\right](s,\vartheta )=0.$$

Set nonlinear operator as

$$\beth \left[\phi (z_{1,2},\tau ;\aleph )\right]=\mathbb{J}\left[\phi (z_{1,2},\tau ;\aleph )\right](s,\vartheta )-\sum _{\ell =1}^{\kappa }\frac{{\vartheta }^{\ell +1}}{s^{\ell }}{\left.\frac{{\partial }^{\ell -1}\psi }{\partial {\tau }^{\ell -1}}\right|}_{\tau =0}+{\left(\frac{\vartheta }{s}\right)}^{\alpha }\mathbb{J}\left[\mathcal{T}\phi (z_{1,2},\tau ;\aleph )\right](s,\vartheta ).$$

where $\phi (z_{1,2},\tau ;\aleph )$ is the real-valued map of $\aleph ,z_{1,2},\tau$; $\aleph \in [0,1]\to$ standard embedded-parameter.

The following zeroth-order deformation equation as in [12,14] is

$$(1-\aleph )\mathbb{J}\left[\phi (z_{1,2},\tau ;\aleph )-{\psi }_0(z_{1,2},\tau )\right](s,\vartheta )=\aleph \hslash H(z_{1,2},\tau )\beth \left[\phi (z_{1,2},\tau ;\aleph )\right],$$

(14)

where $\hslash \ne 0, H(z_{1,2},\tau )\to$ is the auxiliary function/parameter, and ${\psi }_0(z_{1,2},\tau )\to$ is the initial guess of $\psi (z_{1,2},\tau )$. Remark that ${}_O$HA$\mathbb{J}$TM have a merit in selecting auxiliary things in procedure.

• For $\aleph =01$,
• $\phi (z_{1,2},\tau ;0)=\psi (z_{1,2},0)$ and $\phi (z_{1,2},\tau ;1)=\psi (z_{1,2},\tau )$,

and it signifies that when ℵ moving from 0 to 1, the solution $\phi (z_{1,2},\tau ;\aleph )$ moves simultaneously from initial approximation: ${\psi }_0(z_{1,2},\tau )$ to the exact solution behavior: $\psi (z_{1,2},\tau )$.

Expand $\phi (z_{1,2},\tau ;\aleph )$ via Taylor’s formula in the powers of ℵ as:

$$\phi (z_{1,2},\tau ;\aleph )={\psi }_0(z_{1,2},\tau )+\sum _{\lambda =1}^{\infty }{\psi }_{\lambda }(z_{1,2},\tau ){\aleph }^{\lambda },$$

(15)

where ${\psi }_{\lambda }(z_{1,2},\tau )={\left.\frac{1}{\lambda !}\frac{{\partial }^{\lambda }\phi }{\partial {\aleph }^{\lambda }}\right|}_{\aleph =0},$ on selecting suitable value of ℏ improves convergence region to the solution as in (15). Convergence of result (15) at $\aleph =1$ can be secured via selecting appropriate values of $\hslash ,H(z_{1,2},\tau )\ne 0$ and the initial guess, and so

$$\psi (z_{1,2},\tau )={\psi }_0(z_{1,2},\tau )+\sum _{\lambda =1}^{\infty }{\psi }_{\lambda }(z_{1,2},\tau ).$$

(16)

Set

$${\overrightarrow{\psi }}_{\lambda }(z_{1,2},\tau )=\left({\psi }_0(z_{1,2},\tau ), {\psi }_1(z_{1,2},\tau ), {\psi }_2(z_{1,2},\tau ), \dots , {\psi }_{\lambda }(z_{1,2},\tau )\right).$$

In the squel, $\lambda$th order deformation equation is evaluated as

$$\mathbb{J}\left[{\psi }_{\lambda }(z_{1,2},\tau )-{\chi }_{\lambda }{\psi }_{\lambda -1}(z_{1,2},\tau )\right](s,\vartheta )=\hslash \aleph H(z_{1,2},\tau ){\mathcal{P}}_{\lambda }\left({\overrightarrow{\psi }}_{\lambda -1}(z_{1,2},\tau )\right),$$

(17)

where ${\chi }_{\lambda }=0$ if $\lambda \le 1$ and 1 otherwise.

On imposing inverse operator of $\mathbb{J}$T to (17) with $\aleph =1,H(z_{1,2},\tau )=1$, we have

$${\psi }_{\lambda }(z_{1,2},\tau )={\chi }_{\lambda }{\psi }_{\lambda -1}(z_{1,2},\tau )+\hslash {\mathbb{J}}^{-1}\left[{\mathcal{P}}_{\lambda }({\overrightarrow{\psi }}_{\lambda -1}(z_{1,2},\tau ))\right]$$

(18)

where

$${\mathcal{P}}_{\lambda }\left({\overrightarrow{\psi }}_{\lambda -1}(z_{1,2},\tau )\right)=\frac{1}{(\lambda -1)!}{\left.\frac{{\partial }^{\lambda -1}\mathcal{T}\left[\phi (z_{1,2},\tau ,\aleph )\right]}{\partial {\aleph }^{\lambda -1}}\right|}_{\aleph =0}.$$

On evaluation ${\psi }_{\lambda }(z_{1,2},\tau )$, $\lambda \ge 1$. We can calculate Mth-order series behavior of (4) is evaluated as:

$$S_M(z_{1,2},\tau )=\sum _{\lambda =0}^M{\psi }_{\lambda }(z_{1,2},\tau ),$$

(19)

which converges to $\psi (z_{1,2},\tau )$, the exact behavior of the Equation (4) accurately for sufficiently large M (see the following).

Theorem 5

(Convergence & Error Estimates in ${}_O$HA$\mathbb{J}$TM). If ∃ θ with $0<\theta <1$, for which the condition $\parallel {\psi }_{\ell +1}(z_{1,2},\tau )\parallel \le \theta \parallel {\psi }_{\ell }(z_{1,2},\tau )\parallel , \ell \ge 1$ holds true, then

(a)

The approximate Mth order ${}_O$HA$\mathbb{J}$TM result $S_M(z_{1,2},\tau )$ in (19) for NFPDEs (4) evaluated from converges (16) as $M\to \infty$.

(b)

the maximum absolute error in $S_M(z_{1,2},\tau )$ is

$$∥\psi (z_{1,2},\tau )-S_M(z_{1,2},\tau )∥\le \frac{{\theta }^{M+1}}{1-\theta }∥{\psi }_0(z_{1,2},\tau )∥.$$

(20)

(c)

In addition, as for as the result in (16) convergent, where ${\psi }_{\lambda }(z_{1,2},\tau )$’s are evaluated by (18). Then, the result recorded from (16) is the exact solution behavior of NFPDEs (4).

Proof. 

The assumption leads to

$$∥{\psi }_1(z_{1,2},\tau )∥\le \theta ∥{\psi }_0(z_{1,2},\tau )∥, ∥{\psi }_2(z_{1,2},\tau )∥\le \theta ∥{\psi }_1(z_{1,2},\tau )∥\le {\theta }^2∥{\psi }_0(z_{1,2},\tau )∥, \dots ∥{\psi }_{\ell }(z_{1,2},\tau )∥\le {\theta }^{\ell }∥{\psi }_0(z_{1,2},\tau )∥.$$

In consequence, and so, for $M,N\in \mathbb{N}$ with $N>M$, we get

$$∥S_M(z_{1,2},\tau )-S_N(z_{1,2},\tau )∥=∥\sum _{j=M+1}^N{\psi }_j(z_{1,2},\tau )∥\le ∥{\psi }_0(z_{1,2},\tau )∥\sum _{j=M+1}^N{\theta }^j=∥{\psi }_0(z_{1,2},\tau )∥\frac{(1-{\theta }^{N-M}){\theta }^{M+1}}{1-\theta }.$$

Moreover, $1-{\lambda }^{N-M}<1$ as $0<\lambda <1$, and so, the above inequality reduces to

$$∥S_M(z_{1,2},\tau )-S_N(z_{1,2},\tau )∥\le ∥{\psi }_0(z_{1,2},\tau )∥\frac{{\theta }^{M+1}}{1-\theta }\to 0\mathrm{as}M\to \infty .$$

(21)

implying that ${\left\{S_M(z_{1,2},\tau )\right\}}_{M=1}^{\infty }$ is Cauchy sequence, and so, it is convergent.

Part (b) is obtained direct by taking $N\to \infty$ in (21) as follows

$$∥S_M(z_{1,2},\tau )-\psi (z_{1,2},\tau )∥\le ∥{\psi }_0(z_{1,2},\tau )∥\frac{{\theta }^{M+1}}{1-\theta }.$$

(22)

(c) In special case, when $N-1=M=\kappa .$ Then, from Equation (21), we get

$(\ast )$${lim}_{\kappa \to \infty }{\psi }_{\kappa }(z_{1,2},\tau )=0.$

Since

$(\ast \ast )$ ${\psi }_{\kappa }(z_{1,2},\tau )={\sum }_{\lambda =1}^{\kappa }\left[{\psi }_{\lambda }(z_{1,2},\tau )-{\chi }_{\lambda }{\psi }_{\lambda -1}(z_{1,2},\tau )\right].$

Use condition $(\ast )$ and $(\ast \ast )$ in (18) with property $\aleph \ne 0$ to get

${lim}_{\kappa \to \infty }{\sum }_{\lambda =1}^{\kappa }{\mathcal{P}}_{\lambda }\left({\overrightarrow{\psi }}_{\lambda -1}(z_{1,2},\tau )\right)={\sum }_{\lambda =1}^{\infty }{\mathcal{P}}_{\lambda }\left({\overrightarrow{\psi }}_{\lambda -1}(z_{1,2},\tau )\right)=0.$

and so

$$\begin{gathered} {\sum }_{\lambda =1}^{\infty }{\mathcal{P}}_{\lambda }\left({\overrightarrow{\psi }}_{\lambda -1}(z_{1,2},\tau )\right)={\sum }_{\lambda =1}^{\infty }\left[\mathbb{J}\left[{\psi }_{\lambda -1}(z_{1,2},\tau )\right](s,\vartheta )-(1-{\chi }_{\lambda }){\displaystyle {\sum }_{\ell =1}^{\lambda }}\frac{{\vartheta }^{\ell +1}}{s^{\ell }}{\left.\frac{{\partial }^{\ell -1}\psi }{\partial {\tau }^{\ell -1}}\right|}_{\tau =0}+{\left(\frac{\vartheta }{s}\right)}^{\alpha }\mathbb{J}\left[\mathcal{T}{\tilde{\psi }}_{\lambda -1}\left(z_{1,2},\tau \right)\right](s,\vartheta )\right] \\ ={\left(\frac{\vartheta }{s}\right)}^{\alpha }\mathbb{J}\left[{}_{\tau }{\mathcal{D}}_{\alpha }^C\left(\psi (z_{1,2},\tau )\right)+\mathcal{T}\left[\psi (z_{1,2},\tau )\right]\right]=0{⟹}_{\tau }{\mathcal{D}}_{\alpha }^C\left(\psi (z_{1,2},\tau )\right)+\mathcal{T}\left[\varphi (z_{1,2},\tau )\right]=0, \end{gathered}$$

which confirms that the behavior $\psi (z_{1,2},\tau )$ in (16) is the exact exact behavior to NFPDEs (4). □

Evaluation of Optimal Value of the Convergence Control Parameter (ℏ)

The efficiency/validity of ${}_O$HA$\mathbb{J}$TM is confirmed by measuring the $L_2$ or residual-errors. The square residual error [12,14] in the Mth-order solution behavior $S_M(z_{1,2},\tau )$ as in (19)

$${\Delta }_M(\hslash )={\int }_{a_1}^{b_1}{\int }_{a_2}^{b_2}{\int }_{a_3}^{b_3}{\left({\mathcal{R}}^{\oslash }\left[S_M(z_1,z_2,\tau )\right]\right)}^{2}d{z}_{1}d{z}_{2}d\tau ,$$

(23)

where ${\mathcal{R}}^{\oslash }\left[S_M(z_{1,2},\tau )\right]$ is refer to residual error in the solution behavior of order M as $S_M(z_{1,2},\tau )$, and controlling parameter ℏ appeared in solution (19) have a significant role and that receive faster convergence rate on suitable adjustment of ℏ. Precisely, the optimal value for ℏ is values of ℏ within the ℏ-region correspond to that ${\Delta }_M(\hslash )$ is minimized, and so ℏ correspond for which $\frac{d{\Delta }_M(\hslash )}{d\hslash }=0.$ To CPU time under consideration, following formula in place of (23) is preferred.

$${\Delta }_M(\hslash )=\frac{1}{c_1 c_2 c_3}\sum _{j=0}^{c_1}\sum _{k=0}^{c_2}\sum _{l=0}^{c_3}\left({\mathcal{R}}^{\oslash }{\left[S_M(j\delta z_1,k\delta z_2,l\delta t)\right]}^2\right).$$

(24)

where $\delta z_1=\frac{b_1-a_1}{c_1}$, $\delta z_2=\frac{b_2-a_2}{c_2}$ and $\delta \tau =\frac{b_3-a_3}{c_3}$. We set $c_1=c_2=c_3=10.$

5. Validation of Technique

To validate the efficiency and accuracy of the proposed techniques, we consider the fractional order system of equations of governing unsteady flow of a polytropic gas.

Take the fractional order system as described in Equation (1) subject to the initial conditions:

$${\psi }_1(z_{1,2},0)=e^{z_1+z_2}, {\psi }_2(z_{1,2},0)=-1-e^{z_1+z_2}, \rho (z_{1,2},0)=e^{z_1+z_2}, P(z_{1,2},0)=\eta$$

(25)

where $\eta$ is a real constant.

5.1. Validation of $\mathbb{J}$-VITM

By implementing the iteration formula Equation (10) of $\mathbb{J}$-VITM on the system of Equation (1) with ICs (25), we obtain the following recurrence relation

$$\begin{array}{cc}\hfill & {\wp }_{1,\lambda +1}={\wp }_{1,\lambda }(z_{1,2},0)-{\mathbb{J}}^{-1}\left[{\left(\frac{\vartheta }{s}\right)}^{\alpha }\mathbb{J}\left[{\wp }_{1,\lambda }\frac{\partial {\wp }_{1,\lambda }}{\partial z_1}+{\wp }_{2,\lambda }\frac{\partial {\wp }_{1,\lambda }}{\partial z_2}+\frac{1}{{\rho }_{\lambda }}\frac{\partial P_{\lambda }}{\partial z_1}\right](s,\vartheta )\right]\hfill \\ \hfill & {\wp }_{2,\lambda +1}={\wp }_{2,\lambda }(z_{1,2},0)-{\mathbb{J}}^{-1}\left[{\left(\frac{\vartheta }{s}\right)}^{\alpha }\mathbb{J}\left[{\wp }_{1,\lambda }\frac{\partial {\wp }_{2,\lambda }}{\partial z_1}+{\wp }_{2,\lambda }\frac{\partial {\wp }_{2,\lambda }}{\partial z_2}+\frac{1}{{\rho }_{\lambda }}\frac{\partial P_{\lambda }}{\partial z_2}\right](s,\vartheta )\right]\hfill \\ \hfill & {\rho }_{\lambda +1}={\rho }_{\lambda }(z_{1,2},0)-{\mathbb{J}}^{-1}\left[{\left(\frac{\vartheta }{s}\right)}^{\alpha }\mathbb{J}\left[{\wp }_{1,\lambda }\frac{\partial {\rho }_{\lambda }}{\partial z_1}+{\wp }_{2,\lambda }\frac{\partial {\rho }_{\lambda }}{\partial z_2}+{\rho }_{\lambda }\frac{\partial {\wp }_{1,\lambda }}{\partial z_1}+{\rho }_{\lambda }\frac{\partial {\wp }_{2,\lambda }}{\partial z_2}\right](s,\vartheta )\right]\hfill \\ \hfill & P_{\lambda +1}=P_{\lambda }(z_{1,2},0)-{\mathbb{J}}^{-1}\left[{\left(\frac{\vartheta }{s}\right)}^{\alpha }\mathbb{J}\left[{\wp }_{1,\lambda }\frac{\partial P_{\lambda }}{\partial z_1}+{\wp }_{2,\lambda }\frac{\partial P_{\lambda }}{\partial z_2}+\Omega P_{\lambda }\frac{\partial {\wp }_{1,\lambda }}{\partial z_1}+\Omega P\frac{\partial {\wp }_{2,\lambda }}{\partial z_2}\right](s,\vartheta )\right]\hfill \end{array}$$

(26)

On solving the recurrence (26), we get

At first iteration:

$$\begin{array}{cc}\hfill & {\wp }_{1,1}=e^{z_1+z_2}\left(1+\frac{{\tau }^{\alpha }}{\Gamma (\alpha +1)}\right); {\wp }_{2,1}=-1-e^{z_1+z_2}\left(1+\frac{{\tau }^{\alpha }}{\Gamma (\alpha +1)}\right)\hfill \\ \hfill & {\rho }_1=e^{z_1+z_2}\left(1+\frac{{\tau }^{\alpha }}{\Gamma (\alpha +1)}\right); P_1=\eta \hfill \end{array}$$

At second iteration:

$$\begin{array}{cc}\hfill & {\wp }_{1,2}=e^{z_1+z_2}\left(1+\frac{{\tau }^{\alpha }}{\Gamma (\alpha +1)}+\frac{{\tau }^{2\alpha }}{\Gamma (2\alpha +1)}\right); {\wp }_{2,2}=-1-e^{z_1+z_2}\left(1+\frac{{\tau }^{\alpha }}{\Gamma (\alpha +1)}+\frac{{\tau }^{2\alpha }}{\Gamma (2\alpha +1)}\right)\hfill \\ \hfill & {\rho }_2=e^{z_1+z_2}\left(1+\frac{{\tau }^{\alpha }}{\Gamma (\alpha +1)}+\frac{{\tau }^{2\alpha }}{\Gamma (2\alpha +1)}\right); P_2=\eta \hfill \end{array}$$

At third iteration:

$$\begin{array}{cc}\hfill & {\wp }_{1,3}=e^{z_1+z_2}\sum _{j=0}^3\frac{{\tau }^{j\alpha }}{\Gamma (j\alpha +1)},{\wp }_{2,3}=-1-e^{z_1+z_2}\sum _{j=0}^3\frac{{\tau }^{j\alpha }}{\Gamma (j\alpha +1)},\hfill \\ \hfill & {\rho }_3=e^{z_1+z_2}\sum _{j=0}^3\frac{{\tau }^{j\alpha }}{\Gamma (j\alpha +1)},P_3=\eta \hfill \end{array}$$

In similar fashion, $\kappa$th order iterative results for $\kappa =6,10$ are given computed as

$$\begin{array}{cc}\hfill & {\wp }_{1,6}=e^{z_1+z_2}\sum _{j=0}^6\frac{{\tau }^{j\alpha }}{\Gamma (j\alpha +1)},{\wp }_{2,6}=-1-e^{z_1+z_2}\sum _{j=0}^6\frac{{\tau }^{j\alpha }}{\Gamma (j\alpha +1)}\hfill \\ \hfill & {\rho }_6=e^{z_1+z_2}\sum _{j=0}^6\frac{{\tau }^{j\alpha }}{\Gamma (j\alpha +1)},P_6=\eta ,\hfill \end{array}$$

and

$$\begin{array}{cc}\hfill & {\wp }_{1,10}=e^{z_1+z_2}\sum _{j=0}^{10}\frac{{\tau }^{j\alpha }}{\Gamma (j\alpha +1)},{\wp }_{2,10}=-1-e^{z_1+z_2}\sum _{j=0}^{10}\frac{{\tau }^{j\alpha }}{\Gamma (j\alpha +1)}\hfill \\ \hfill & {\rho }_{10}=e^{z_1+z_2}\sum _{j=0}^{10}\frac{{\tau }^{j\alpha }}{\Gamma (j\alpha +1)},P_{10}=\eta .\hfill \end{array}$$

This concludes that general $\kappa$th order iterative solutions is of the form

$$\begin{array}{cc}\hfill & {\wp }_{1,\kappa }=e^{z_1+z_2}\sum _{j=0}^{\kappa }\frac{{\tau }^{j\alpha }}{\Gamma (j\alpha +1)}, {\wp }_{2,\kappa }=-1-e^{z_1+z_2}\sum _{j=0}^{\kappa }\frac{{\tau }^{j\alpha }}{\Gamma (j\alpha +1)}\hfill \\ \hfill & {\rho }_{\kappa }=e^{z_1+z_2}\sum _{j=0}^{\kappa }\frac{{\tau }^{j\alpha }}{\Gamma (j\alpha +1)}, P_{\kappa }=\eta .\hfill \end{array}$$

In consequence, $\kappa$th order iterative solutions converges to the exact solutions as $\kappa \to \infty$:

$$\begin{array}{cc}\hfill & {\wp }_1=e^{z_1+z_2}\sum _{j=0}^{\infty }\frac{{\tau }^{j\alpha }}{\Gamma (j\alpha +1)}=e^{z_1+z_2}{E}_{\alpha ,1}\left({\tau }^{\alpha }\right)\hfill \\ \hfill & {\wp }_2=-1-e^{z_1+z_2}\sum _{j=0}^{\infty }\frac{{\tau }^{j\alpha }}{\Gamma (j\alpha +1)}=-1-e^{z_1+z_2}{E}_{\alpha ,1}\left({\tau }^{\alpha }\right)\hfill \\ \hfill & \rho =e^{z_1+z_2}\sum _{j=0}^{\infty }\frac{{\tau }^{j\alpha }}{\Gamma (j\alpha +1)}=e^{z_1+z_2}{E}_{\alpha ,1}\left({\tau }^{\alpha }\right)\hfill \\ \hfill & P=\eta .\hfill \end{array}$$

In special case, when $\alpha =1$ the above results converges to the exact solutions:

$${\wp }_1(z_{1,2},\tau )=e^{z_1+z_2+\tau },{\wp }_2(z_{1,2},\tau )=-1-e^{z_1+z_2+\tau },\rho (z_{1,2},\tau )=e^{z_1+z_2+\tau },P(z_{1,2},\tau )=\eta .$$

(27)

5.2. Validation of ${}_O$HA$\mathbb{J}$TM

Imposing $\mathbb{J}$-transform on system of Equation (1) with ICs (25), we get

$$\begin{array}{cc}\hfill & \mathbb{J}\left[{\wp }_1(z_{1,2},\tau )\right](s,\vartheta )-\frac{{\vartheta }^2}{s}{e}^{z_1+z_2}+{\left(\frac{\vartheta }{s}\right)}^{\alpha }\mathbb{J}\left[{\wp }_1\frac{\partial {\wp }_1}{\partial z_1}+{\wp }_2\frac{\partial {\wp }_1}{\partial z_2}+\frac{1}{\rho }\frac{\partial P}{\partial z_1}\right](s,\vartheta )=0.\hfill \\ \hfill & \mathbb{J}\left[{\wp }_2(z_{1,2},\tau )\right](s,\vartheta )-\frac{{\vartheta }^2}{s}\left(-1-e^{z_1+z_2}\right)+{\left(\frac{\vartheta }{s}\right)}^{\alpha }\mathbb{J}\left[{\wp }_1\frac{\partial {\wp }_2}{\partial z_1}+{\wp }_2\frac{\partial {\wp }_2}{\partial z_2}+\frac{1}{\rho }\frac{\partial P}{\partial z_2}\right](s,\vartheta )=0.\hfill \\ \hfill & \mathbb{J}\left[\rho (z_{1,2},\tau )\right](s,\vartheta )-\frac{{\vartheta }^2}{s}{e}^{z_1+z_2}+{\left(\frac{\vartheta }{s}\right)}^{\alpha }\mathbb{J}\left[{\wp }_1\frac{\partial \rho }{\partial z_1}+{\wp }_2\frac{\partial \rho }{\partial z_2}+\rho \frac{\partial {\wp }_1}{\partial z_1}+\rho \frac{\partial {\wp }_2}{\partial z_2}\right](s,\vartheta )=0.\hfill \\ \hfill & \mathbb{J}\left[P(z_{1,2},\tau )\right](s,\vartheta )-\frac{{\vartheta }^2}{s}\eta +{\left(\frac{\vartheta }{s}\right)}^{\alpha }\mathbb{J}\left[{\wp }_1\frac{\partial P}{\partial z_1}+{\wp }_2\frac{\partial P}{\partial z_2}+\Omega P\frac{\partial {\wp }_1}{\partial z_1}+\Omega P\frac{\partial {\wp }_2}{\partial z_2}\right](s,\vartheta )=0.\hfill \end{array}$$

(28)

Formulate nonlinear operator as follows:

$$\begin{array}{cc}\hfill & {\beth }_1[{\phi }_1(z_{1,2},\tau ;\aleph ),{\phi }_2(z_{1,2},\tau ;\aleph ),{\phi }_3(z_{1,2},\tau ;\aleph ),{\phi }_4(z_{1,2},\tau ;\aleph )]=\mathbb{J}\left[{\phi }_1(z_{1,2},\tau ;\aleph )\right](s,\vartheta )-\frac{{\vartheta }^2}{s}{e}^{z_1+z_2}\hfill \\ & +{\left(\frac{\vartheta }{s}\right)}^{\alpha }\mathbb{J}\left[{\phi }_1(z_{1,2},\tau ;\aleph )\frac{\partial }{\partial z_1}{\phi }_1(z_{1,2},\tau ;\aleph )+{\phi }_2(z_{1,2},\tau ;\aleph )\frac{\partial }{\partial z_2}{\phi }_1(z_{1,2},\tau ;\aleph )+\frac{1}{{\phi }_3(z_{1,2},\tau ;\aleph )}\frac{\partial }{\partial z_1}{\phi }_4(z_{1,2},\tau ;\aleph )\right](s,\vartheta )\hfill \\ \hfill & {\beth }_2[{\phi }_1(z_{1,2},\tau ;\aleph ),{\phi }_2(z_{1,2},\tau ;\aleph ),{\phi }_3(z_{1,2},\tau ;\aleph ),{\phi }_4(z_{1,2},\tau ;\aleph )]=\mathbb{J}\left[{\phi }_2(z_{1,2},\tau ;\aleph )\right](s,\vartheta )-\frac{{\vartheta }^2}{s}\left(-1-e^{z_1+z_2}\right)+{\left(\frac{\vartheta }{s}\right)}^{\alpha }\hfill \\ \hfill & \mathbb{J}\left[{\phi }_1(z_{1,2},\tau ;\aleph )\frac{\partial }{\partial z_1}{\phi }_2(z_{1,2},\tau ;\aleph )+{\phi }_2(z_{1,2},\tau ;\aleph )\frac{\partial }{\partial z_2}{\phi }_2(z_{1,2},\tau ;\aleph )+\frac{1}{{\phi }_3(z_{1,2},\tau ;\aleph )}\frac{\partial }{\partial z_2}{\phi }_4(z_{1,2},\tau ;\aleph )\right](s,\vartheta )\hfill \\ \hfill & {\beth }_3[{\phi }_1(z_{1,2},\tau ;\aleph ),{\phi }_2(z_{1,2},\tau ;\aleph ),{\phi }_3(z_{1,2},\tau ;\aleph ),{\phi }_4(z_{1,2},\tau ;\aleph )]=\mathbb{J}\left[{\phi }_2(z_{1,2},\tau ;\aleph )\right](s,\vartheta )\hfill \\ \hfill & -\frac{{\vartheta }^2}{s}{e}^{z_1+z_2}+{\left(\frac{\vartheta }{s}\right)}^{\alpha }\mathbb{J}\left[{\phi }_1(z_{1,2},\tau ;\aleph )\frac{\partial }{\partial z_1}{\phi }_3(z_{1,2},\tau ;\aleph )+{\phi }_2(z_{1,2},\tau ;\aleph )\frac{\partial }{\partial z_2}{\phi }_3(z_{1,2},\tau ;\aleph )\right.\hfill \\ \hfill & \left.+{\phi }_3(z_{1,2},\tau ;\aleph )\frac{\partial }{\partial z_1}{\phi }_1(z_{1,2},\tau ;\aleph )+{\phi }_3(z_{1,2},\tau ;\aleph )\frac{\partial }{\partial z_2}{\phi }_2(z_{1,2},\tau ;\aleph )\right](s,\vartheta )\hfill \\ \hfill & {\beth }_4[{\phi }_1(z_{1,2},\tau ;\aleph ),{\phi }_2(z_{1,2},\tau ;\aleph ),{\phi }_3(z_{1,2},\tau ;\aleph ),{\phi }_4(z_{1,2},\tau ;\aleph )]=\mathbb{J}\left[{\phi }_1(z_{1,2},\tau ;\aleph )\right](s,\vartheta )-\frac{{\vartheta }^2}{s}\eta \hfill \\ \hfill & +{\left(\frac{\vartheta }{s}\right)}^{\alpha }\mathbb{J}\left[{\phi }_1(z_{1,2},\tau ;\aleph )\frac{\partial }{\partial z_1}{\phi }_4(z_{1,2},\tau ;\aleph )+{\phi }_2(z_{1,2},\tau ;\aleph )\frac{\partial }{\partial z_2}{\phi }_4(z_{1,2},\tau ;\aleph )\right.\hfill \\ \hfill & \left.+\Omega {\phi }_4(z_{1,2},\tau ;\aleph )\frac{\partial }{\partial z_1}{\phi }_1(z_{1,2},\tau ;\aleph )+\Omega {\phi }_4(z_{1,2},\tau ;\aleph )\frac{\partial }{\partial z_2}{\phi }_2(z_{1,2},\tau ;\aleph )\right](s,\vartheta ).\hfill \end{array}$$

(29)

Utilizing (31) in (18) to obtain the recursive formula

$$\begin{array}{cc}\hfill & {\wp }_{1,\lambda }(z_{1,2},\tau )={\chi }_{\lambda }{\wp }_{1,\lambda -1}(z_{1,2},\tau )+\hslash {\mathbb{J}}^{-1}\left[{\mathcal{P}}_{\lambda }^1\left[{\overrightarrow{\wp }}_{1,\lambda -1},{\overrightarrow{\wp }}_{2,\lambda -1},{\overrightarrow{\rho }}_{\lambda -1},{\overrightarrow{\mathit{P}}}_{\lambda -1}\right]\right]\hfill \\ \hfill & {\wp }_{2,\lambda }(z_{1,2},\tau )={\chi }_{\lambda }{\wp }_{2,\lambda -1}(z_{1,2},\tau )+\hslash {\mathbb{J}}^{-1}\left[{\mathcal{P}}_{\lambda }^2\left[{\overrightarrow{\wp }}_{1,\lambda -1},{\overrightarrow{\wp }}_{2,\lambda -1},{\overrightarrow{\rho }}_{\lambda -1},{\overrightarrow{\mathit{P}}}_{\lambda -1}\right]\right]\hfill \\ \hfill & {\rho }_{\lambda }(z_{1,2},\tau )={\chi }_{\lambda }{\rho }_{\lambda -1}(z_{1,2},\tau )+\hslash {\mathbb{J}}^{-1}\left[{\mathcal{P}}_{\lambda }^3\left[{\overrightarrow{\wp }}_{1,\lambda -1},{\overrightarrow{\wp }}_{2,\lambda -1},{\overrightarrow{\rho }}_{\lambda -1},{\overrightarrow{\mathit{P}}}_{\lambda -1}\right]\right]\hfill \\ \hfill & P_{\lambda }(z_{1,2},\tau )={\chi }_{\lambda }{P}_{\lambda -1}(z_{1,2},\tau )+\hslash {\mathbb{J}}^{-1}\left[{\mathcal{P}}_{\lambda }^4\left[{\overrightarrow{\wp }}_{1,\lambda -1},{\overrightarrow{\wp }}_{2,\lambda -1},{\overrightarrow{\rho }}_{\lambda -1},{\overrightarrow{\mathit{P}}}_{\lambda -1}\right]\right]\hfill \end{array}$$

(30)

where

$$\begin{array}{cc}\hfill & {\mathcal{P}}_{\lambda }^1\left[{\overrightarrow{\wp }}_{1,\lambda -1},{\overrightarrow{\wp }}_{2,\lambda -1},{\overrightarrow{\rho }}_{\lambda -1},{\overrightarrow{P}}_{\lambda -1}\right]=\mathbb{J}\left[{\wp }_{1,\lambda -1}(z_{1,2},\tau )\right](s,\vartheta )-\frac{(1-{\chi }_{\lambda }){\vartheta }^2}{s}{e}^{z_1+z_2}\hfill \\ \hfill & +{\left(\frac{\vartheta }{s}\right)}^{\alpha }\mathbb{J}\left[\sum _{j=0}^{\lambda -1}\left[{\wp }_{1,\lambda -1-j}\frac{\partial {\wp }_{1,j}}{\partial z_1}+{\wp }_{2,\lambda -1-j}\frac{\partial {\wp }_{1,j}}{\partial z_2}+\frac{1}{{\rho }_j}\frac{\partial }{\partial z_1}{P}_{\lambda -1-j}\right]\right](s,\vartheta )\hfill \\ \hfill & {\mathcal{P}}_{\lambda }^2({\overrightarrow{\wp }}_{1,\lambda -1},{\overrightarrow{\wp }}_{2,\lambda -1},{\overrightarrow{\rho }}_{\lambda -1},{\overrightarrow{P}}_{\lambda -1})=\mathbb{J}\left[{\wp }_{2,\lambda -1}(z_{1,2},\tau )\right](s,\vartheta )-\frac{(1-{\chi }_{\lambda }){\vartheta }^2}{s}\left(-1-e^{z_1+z_2}\right)\hfill \\ \hfill & +{\left(\frac{\vartheta }{s}\right)}^{\alpha }\mathbb{J}\left[\sum _{j=0}^{\lambda -1}\left[{\wp }_{1,\lambda -1-j}\frac{\partial {\wp }_{2,j}}{\partial z_1}+{\wp }_{2,\lambda -1-j}\frac{\partial {\wp }_{2,j}}{\partial z_2}+\frac{1}{{\rho }_j}\frac{\partial }{\partial z_2}{P}_{\lambda -1-j}\right]\right](s,\vartheta )\hfill \\ \hfill & {\mathcal{P}}_{\lambda }^3({\overrightarrow{\wp }}_{1,\lambda -1},{\overrightarrow{\wp }}_{2,\lambda -1},{\overrightarrow{\rho }}_{\lambda -1},{\overrightarrow{P}}_{\lambda -1})=\mathbb{J}\left[{\rho }_{\lambda -1}(z_{1,2},\tau )\right](s,\vartheta )-\frac{(1-{\chi }_{\lambda }){\vartheta }^2}{s}{e}^{z_1+z_2}\hfill \\ \hfill & +{\left(\frac{\vartheta }{s}\right)}^{\alpha }\mathbb{J}\left[\sum _{j=0}^{\lambda -1}\left[{\wp }_{1,\lambda -1-j}\frac{\partial {\rho }_j}{\partial z_1}+{\wp }_{2,\lambda -1-j}\frac{\partial {\rho }_j}{\partial z_2}+{\rho }_{\lambda -1-j}\frac{\partial {\wp }_{1,j}}{\partial z_1}+{\rho }_{\lambda -1-j}\frac{\partial {\wp }_{2,j}}{\partial z_2}\right]\right](s,\vartheta )\hfill \\ \hfill & {\mathcal{P}}_{\lambda }^4({\overrightarrow{\wp }}_{1,\lambda -1},{\overrightarrow{\wp }}_{2,\lambda -1},{\overrightarrow{\rho }}_{\lambda -1},{\overrightarrow{P}}_{\lambda -1})=\mathbb{J}\left[P_{\lambda -1}(z_{1,2},\tau )\right](s,\vartheta )-\frac{(1-{\chi }_{\lambda }){\vartheta }^2}{s}\eta \hfill \\ \hfill & +{\left(\frac{\vartheta }{s}\right)}^{\alpha }\mathbb{J}\left[\sum _{j=0}^{\lambda -1}\left[{\wp }_{1,\lambda -j-1}\frac{\partial P_j}{\partial z_1}+{\wp }_{2,\lambda -j-1}\frac{\partial P_j}{\partial z_2}+\Omega P_{\lambda -j-1}\frac{\partial {\wp }_{1,j}}{\partial z_1}+\Omega P_{\lambda -j-1}\frac{\partial {\wp }_{2,j}}{\partial z_2}\right]\right](s,\vartheta ).\hfill \end{array}$$

(31)

with the aid of Mathematica software, solve recurrence relation (30).

At first iteration:

$${\wp }_{1,1}(z_{1,2},\tau )=-\frac{\hslash {\tau }^{\alpha }{e}^{z_1+z_2}}{\Gamma (\alpha +1)};{\wp }_{2,1}(z_{1,2},\tau )=\frac{\hslash {\tau }^{\alpha }{e}^{z_1+z_2}}{\Gamma (\alpha +1)};{\rho }_1(z_{1,2},\tau )=-\frac{\hslash {\tau }^{\alpha }{e}^{z_1+z_2}}{\Gamma (\alpha +1)};P_1(z_{1,2},\tau )=0.$$

At second iteration:

$$\begin{array}{cc}\hfill & {\wp }_{1,2}(z_{1,2},\tau )=e^{z_1+z_2}\left(\frac{{\hslash }^2{\tau }^{2\alpha }}{\Gamma (2\alpha +1)}-\frac{(\hslash +{\hslash }^2){\tau }^{\alpha }}{\Gamma (\alpha +1)}\right);{\wp }_{2,2}(z_{1,2},\tau )=e^{z_1+z_2}\left(-\frac{{\hslash }^2{\tau }^{2\alpha }}{\Gamma (2\alpha +1)}+\frac{(\hslash +{\hslash }^2){\tau }^{\alpha }}{\Gamma (\alpha +1)}\right)\hfill \\ \hfill & {\rho }_2(z_{1,2},\tau )=e^{z_1+z_2}\left(\frac{{\hslash }^2{\tau }^{2\alpha }}{\Gamma (2\alpha +1)}-\frac{(\hslash +{\hslash }^2){\tau }^{\alpha }}{\Gamma (\alpha +1)}\right);P_2(z_{1,2},\tau )=0.\hfill \end{array}$$

At third iteration:

$$\begin{array}{cc}\hfill & {\wp }_{1,3}(z_{1,2},\tau )=e^{z_1+z_2}\left(-\frac{{\hslash }^3{\tau }^{3\alpha }}{\Gamma (3\alpha +1)}\frac{2({\hslash }^2+{\hslash }^3){\tau }^{2\alpha }}{\Gamma (2\alpha +1)}-\frac{(\hslash +{\hslash }^2+{\hslash }^3){\tau }^{\alpha }}{\Gamma (\alpha +1)}\right)\hfill \\ \hfill & {\wp }_{2,3}(z_{1,2},\tau )=e^{z_1+z_2}\left(\frac{{\hslash }^3{\tau }^{3\alpha }}{\Gamma (3\alpha +1)}-\frac{2({\hslash }^2+{\hslash }^3){\tau }^{2\alpha }}{\Gamma (2\alpha +1)}+\frac{(\hslash +{\hslash }^2+{\hslash }^3){\tau }^{\alpha }}{\Gamma (\alpha +1)}\right)\hfill \\ \hfill & {\rho }_3(z_{1,2},\tau )=e^{z_1+z_2}\left(-\frac{{\hslash }^3{\tau }^{3\alpha }}{\Gamma (3\alpha +1)}\frac{2({\hslash }^2+{\hslash }^3){\tau }^{2\alpha }}{\Gamma (2\alpha +1)}-\frac{(\hslash +{\hslash }^2+{\hslash }^3){\tau }^{\alpha }}{\Gamma (\alpha +1)}\right)\hfill \\ \hfill & P_3(z_{1,2},\tau )=0.\hfill \end{array}$$

In sequel, the terms corresponding to $\lambda \ge 4$ for the system of equation can be computed from (30). The 6th order approximate results for the system is

$$\begin{array}{cc}\hfill & S_6{\wp }_1(z_{1,2},\tau )=\sum _{\lambda =0}^6{\wp }_{1,\lambda }(z_{1,2},\tau );S_6{\wp }_2(z_{1,2},\tau )=\sum _{\lambda =0}^6{\wp }_{2,\lambda }(z_{1,2},\tau ),\hfill \\ \hfill & S_6\rho (z_{1,2},\tau )=\sum _{\lambda =0}^6{\rho }_{\lambda }(z_{1,2},\tau );S_{6}P(z_{1,2},\tau )=\sum _{\lambda =0}^6{P}_{\lambda }(z_{1,2},\tau ).\hfill \end{array}$$

(32)

This series solution (32) with $\hslash =-1$ reduced to

$$\begin{array}{cc}\hfill & S_6{\wp }_1(z_{1,2},\tau )=e^{z_1+z_2}\sum _{\lambda =0}^6\frac{{\tau }^{\lambda \alpha }}{\Gamma (\lambda \alpha +1)},S_6{\wp }_2(z_{1,2},\tau )=-1-e^{z_1+z_2}\sum _{\lambda =0}^6\frac{{\tau }^{\lambda \alpha }}{\Gamma (\lambda \alpha +1)}\hfill \\ \hfill & S_6\rho (z_{1,2},\tau )=e^{z_1+z_2}\sum _{\lambda =0}^6\frac{{\tau }^{\lambda \alpha }}{\Gamma (\lambda \alpha +1)},S_{6}P(z_{1,2},\tau )=\eta .\hfill \end{array}$$

which is the adjacent form of the exact solution (27) obtained via $\mathbb{J}$-VITM. In addition for $\alpha =1$, it is an adjacent form of the exact solution (27).

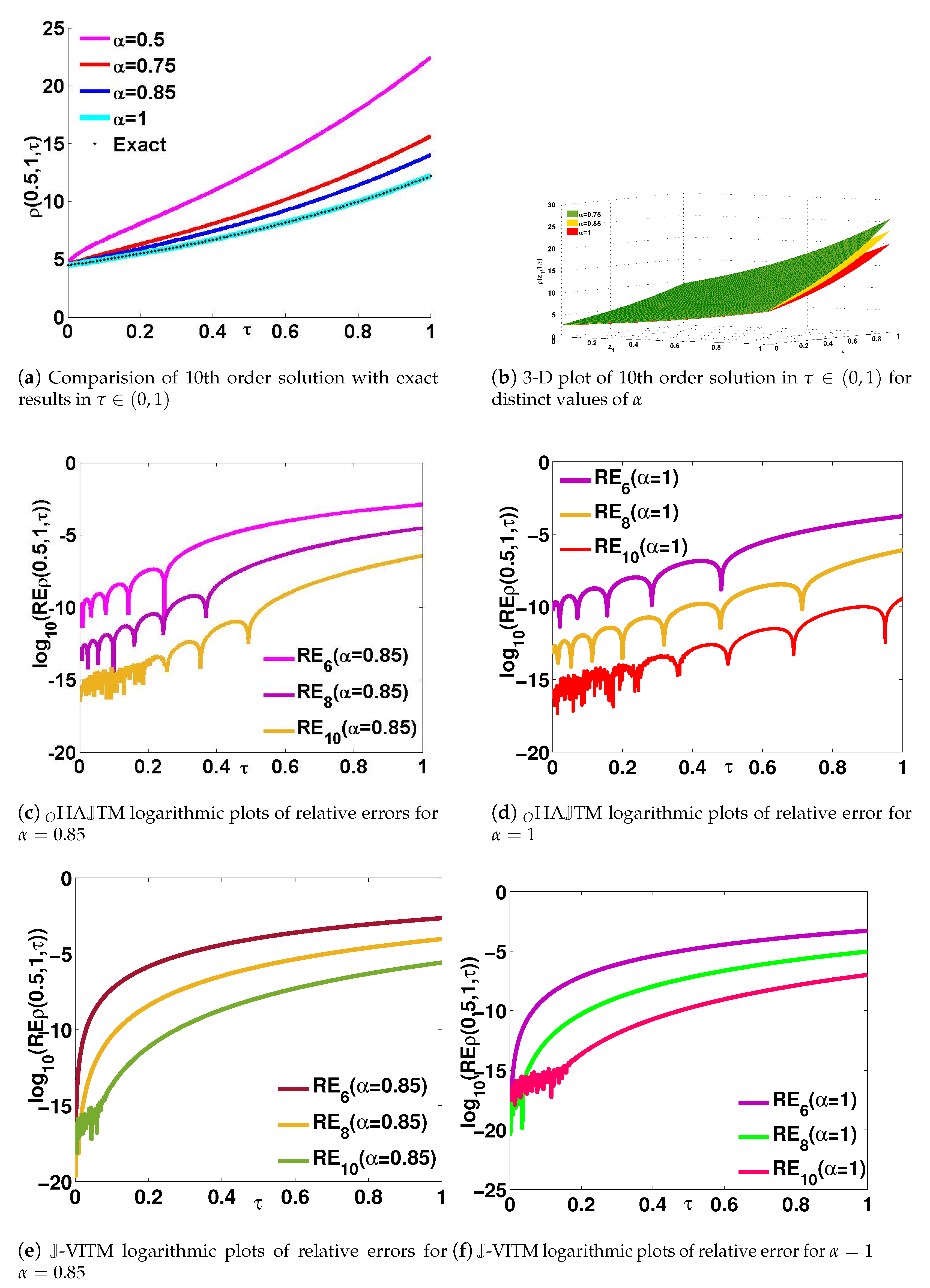
5.3. Result and Discussion

Throughout computation fixed $z_2=1$. The comparison in absolute errors $\kappa$th order results $(\kappa =6,10)$ for ${\wp }_1$/$\rho$ and ${\wp }_2$ via $\mathbb{J}$-VITM and ${}_O$HA$\mathbb{J}$TM in $0<\tau ,z_1<1$ are reported in

Table 1. Comparison of absolute errors $\kappa$th order results $(\kappa =6,10)$ for ${\wp }_1$/$\rho$ via $\mathbb{J}$-VITM and ${}_O$HA$\mathbb{J}$TM for $0<z_1<1,z_2=1$ at different time levels $0<\tau \le 1$.

		${\wp }_{1}6\left(\rho 6\right)$				${\wp }_{1}10\left(\rho 10\right)$
$(z_1,z_2,\tau )$		$\hslash =-1$	$\hslash =-\mathbf{1}.\mathbf{0692}$			$\hslash =-1$	$\hslash =-1.0349$
	$\mathbb{J}$-VITM	HA $\mathbb{J}$TM	${}_O$HA $\mathbb{J}$TM		$\mathbb{J}$-VITM	HA$\mathbb{J}$TM	${}_O$HA $\mathbb{J}$TM
(0.25,1,0.25)	4.3627 $\times {10}^{-8}$	4.3627 $\times {10}^{-8}$	2.0887 $\times {10}^{-9}$		2.1316 $\times {10}^{-14}$	3.9968 $\times {10}^{-14}$	2.5757 $\times {10}^{-14}$
(0.25,1,0.5)	5.7683 $\times {10}^{-6}$	5.7683 $\times {10}^{-6}$	1.6874 $\times {10}^{-7}$		4.4546 $\times {10}^{-11}$	4.4606 $\times {10}^{-11}$	1.1191 $\times {10}^{-13}$
(0.25,1,0.75)	1.0189 $\times {10}^{-4}$	1.0189 $\times {10}^{-4}$	3.4828 $\times {10}^{-7}$		3.9379 $\times {10}^{-9}$	3.9380 $\times {10}^{-9}$	3.3333 $\times {10}^{-12}$
(0.25,1,1)	7.8977 $\times {10}^{-5}$	7.8977 $\times {10}^{-4}$	1.5869 $\times {10}^{-5}$		9.5331 $\times {10}^{-8}$	9.5331 $\times {10}^{-8}$	1.7106 $\times {10}^{-10}$
(0.5,1,0.25)	5.6018 $\times {10}^{-8}$	5.6018 $\times {10}^{-8}$	2.6820 $\times {10}^{-9}$		2.7534 $\times {10}^{-14}$	6.0396 $\times {10}^{-14}$	1.7764 $\times {10}^{-15}$
(0.5,1,0.5)	7.4066 $\times {10}^{-6}$	7.4066 $\times {10}^{-6}$	2.1667 $\times {10}^{-7}$		5.7198 $\times {10}^{-11}$	5.7214 $\times {10}^{-11}$	4.0856 $\times {10}^{-14}$
(0.5,1,075)	1.3083 $\times {10}^{-4}$	1.3083 $\times {10}^{-4}$	4.4720 $\times {10}^{-7}$		5.0564 $\times {10}^{-9}$	5.0564 $\times {10}^{-9}$	4.6523 $\times {10}^{-12}$
(0.5,1,1)	1.0141 $\times {10}^{-3}$	1.0141 $\times {10}^{-3}$	2.0376 $\times {10}^{-5}$		1.2241 $\times {10}^{-7}$	1.2241 $\times {10}^{-7}$	2.1923 $\times {10}^{-10}$
(0.75,1,0.25)	7.1929 $\times {10}^{-8}$	7.1929 $\times {10}^{-8}$	3.4437 $\times {10}^{-9}$		3.4639 $\times {10}^{-14}$	4.1744 $\times {10}^{-14}$	6.3949 $\times {10}^{-14}$
(0.75,1,0.5)	9.5103 $\times {10}^{-6}$	9.5103 $\times {10}^{-6}$	2.7821 $\times {10}^{-7}$		7.3443 $\times {10}^{-11}$	7.3399 $\times {10}^{-11}$	6.5725 $\times {10}^{-14}$
(0.75,1,0.75)	1.6799 $\times {10}^{-4}$	1.6799 $\times {10}^{-4}$	5.7422 $\times {10}^{-7}$		6.4926 $\times {10}^{-9}$	6.4929 $\times {10}^{-9}$	5.4818 $\times {10}^{-12}$
(0.75,1,1)	1.3021 $\times {10}^{-3}$	1.3021 $\times {10}^{-3}$	2.6164 $\times {10}^{-5}$		1.5717 $\times {10}^{-7}$	1.5717 $\times {10}^{-7}$	2.8172 $\times {10}^{-10}$
(1,1,0.25)	9.2359 $\times {10}^{-8}$	9.2359 $\times {10}^{-8}$	4.4219 $\times {10}^{-9}$		4.4409 $\times {10}^{-14}$	2.6645 $\times {10}^{-14}$	4.7962 $\times {10}^{-14}$
(1,1,0.5)	1.2211 $\times {10}^{-5}$	1.2211 $\times {10}^{-5}$	3.5723 $\times {10}^{-7}$		9.4301 $\times {10}^{-11}$	9.4241 $\times {10}^{-11}$	3.8547 $\times {10}^{-13}$
(1,1,0.75)	2.1570 $\times {10}^{-4}$	2.1570 $\times {10}^{-4}$	7.3731 $\times {10}^{-7}$		8.3366 $\times {10}^{-9}$	8.3368 $\times {10}^{-9}$	6.9846 $\times {10}^{-12}$
(1,1,1)	1.6719 $\times {10}^{-3}$	1.6719 $\times {10}^{-3}$	3.3595 $\times {10}^{-5}$		2.0181 $\times {10}^{-7}$	2.0181 $\times {10}^{-7}$	3.6275 $\times {10}^{-10}$
CPU Time	1.8290	4.0150			3.2340	12.0300

and

Table 2. Comparison of absolute errors $\kappa$th order results $(\kappa =6,10)$ for ${\wp }_2$ via $\mathbb{J}$-VITM and ${}_O$HA$\mathbb{J}$TM for $0<z_1<1,z_2=1$ at different time levels $0<\tau \le 1$.

		${\mathit{\wp }}_{2}6$				${\mathit{\wp }}_{2}10$
$(z_1,z_2,\tau )$		$\hslash =-1$	$\hslash =-1.0692$			$\hslash =-1$	$\hslash =-1.0349$
	$\mathbb{J}$-VITM	HA $\mathbb{J}$TM	${}_O$HA $\mathbb{J}$TM		$\mathbb{J}$-VITM	HA $\mathbb{J}$TM	${}_O$HA $\mathbb{J}$TM
(0.25,1,0.25)	4.3627 $\times {10}^{-8}$	4.3627 $\times {10}^{-8}$	9.3490 $\times {10}^{-12}$		2.0428 $\times {10}^{-14}$	2.5757 $\times {10}^{-14}$	2.5757 $\times {10}^{-14}$
(0.25,1,0.5)	5.7683 $\times {10}^{-6}$	5.7683 $\times {10}^{-6}$	1.7979 $\times {10}^{-7}$		4.4546 $\times {10}^{-11}$	4.4578 $\times {10}^{-11}$	1.1191 $\times {10}^{-13}$
(0.25,1,0.75)	1.0189 $\times {10}^{-4}$	1.0189 $\times {10}^{-4}$	5.4706 $\times {10}^{-7}$		3.9379 $\times {10}^{-9}$	3.9380 $\times {10}^{-9}$	3.3342 $\times {10}^{-12}$
(0.25,1,1)	7.8977 $\times {10}^{-4}$	7.8977 $\times {10}^{-4}$	1.5031 $\times {10}^{-5}$		9.5331 $\times {10}^{-8}$	9.5331 $\times {10}^{-8}$	1.7106 $\times {10}^{-10}$
(0.5,1,0.25)	5.6018 $\times {10}^{-8}$	5.6018 $\times {10}^{-8}$	1.2006 $\times {10}^{-11}$		2.8422 $\times {10}^{-14}$	6.0396 $\times {10}^{-14}$	1.7764 $\times {10}^{-15}$
(0.5,1,0.5)	7.4066 $\times {10}^{-6}$	7.4066 $\times {10}^{-6}$	2.3086 $\times {10}^{-7}$		5.7197 $\times {10}^{-11}$	5.7213 $\times {10}^{-11}$	4.0856 $\times {10}^{-14}$
(0.5,1,075)	1.3083 $\times {10}^{-4}$	1.3083 $\times {10}^{-4}$	7.0244 $\times {10}^{-7}$		5.0564 $\times {10}^{-9}$	5.0564 $\times {10}^{-9}$	4.6523 $\times {10}^{-12}$
(0.5,1,1)	1.0141 $\times {10}^{-3}$	1.0141 $\times {10}^{-3}$	1.9301 $\times {10}^{-5}$		1.2241 $\times {10}^{-7}$	1.2241 $\times {10}^{-7}$	2.1923 $\times {10}^{-10}$
(0.75,1,0.25)	7.1929 $\times {10}^{-8}$	7.1929 $\times {10}^{-8}$	1.5415 $\times {10}^{-11}$		3.5527 $\times {10}^{-14}$	4.2633 $\times {10}^{-14}$	6.3949 $\times {10}^{-14}$
(0.75,1,0.5)	9.5103 $\times {10}^{-6}$	9.5103 $\times {10}^{-6}$	2.9643 $\times {10}^{-7}$		7.3443 $\times {10}^{-11}$	7.3399 $\times {10}^{-11}$	6.5725 $\times {10}^{-14}$
(0.75,1,0.75)	1.6799 $\times {10}^{-4}$	1.6799 $\times {10}^{-4}$	9.0195 $\times {10}^{-7}$		6.4926 $\times {10}^{-9}$	6.4929 $\times {10}^{-9}$	5.3682 $\times {10}^{-12}$
(0.75,1,1)	1.3021 $\times {10}^{-3}$	1.3021 $\times {10}^{-3}$	2.4783 $\times {10}^{-5}$		1.5717 $\times {10}^{-7}$	1.5717 $\times {10}^{-7}$	2.8173 $\times {10}^{-10}$
(1,1,0.25)	9.2359 $\times {10}^{-8}$	9.2359 $\times {10}^{-8}$	1.9796 $\times {10}^{-11}$		4.4409 $\times {10}^{-14}$	5.1514 $\times {10}^{-14}$	9.5923 $\times {10}^{-14}$
(1,1,0.5)	1.2211 $\times {10}^{-5}$	1.2211 $\times {10}^{-5}$	3.8062 $\times {10}^{-7}$		9.4301 $\times {10}^{-11}$	9.4298 $\times {10}^{-11}$	3.6415 $\times {10}^{-13}$
(1,1,0.75)	2.1570 $\times {10}^{-4}$	2.1570 $\times {10}^{-4}$	1.1581 $\times {10}^{-6}$		8.3366 $\times {10}^{-9}$	8.3369 $\times {10}^{-9}$	7.0415 $\times {10}^{-12}$
(1,1,1)	1.6719 $\times {10}^{-3}$	1.6719 $\times {10}^{-3}$	3.1822 $\times {10}^{-5}$		2.0181 $\times {10}^{-7}$	2.0181 $\times {10}^{-7}$	3.6265 $\times {10}^{-10}$

, respectively. In consequence,

Table 3. Comparison of 10th order results for ${\wp }_1$ and ${\wp }_2$ via ${}_O$HA$\mathbb{J}$TM with optimal value of ℏ for $0<z_1<1,z_2=1$ at different time levels $0<\tau \le 1$ with exact results.

	${\wp }_1$				${\wp }_2$
$(z_1,z_2,\tau )$	$\alpha =0.85$	$\mathit{\alpha }=1$	Exact		$\mathit{\alpha }=0.85$	$\alpha =1$	Exact
(0.25,1,0.25)	4.8728202329	4.4816890703	4.4816890703		5.8728202329	5.4816890703	5.4816890703
(0.25,1,0.5)	6.4418579146	5.7546026760	5.7546026760		7.4418579146	6.7546026760	6.7546026760
(0.25,1,0.75)	8.4088209398	7.3890560989	7.3890560989		9.4088209398	8.3890560989	8.3890560989
(0.25,1,1)	10.9090468543	9.4877358365	9.4877358364		11.9090468543	10.4877358365	10.4877358364
(0.5,1,0.25)	6.2568250301	5.7546026760	5.7546026760		7.2568250301	6.7546026760	6.7546026760
(0.5,1,0.5)	8.2715092930	7.3890560989	7.3890560989		9.2715092930	8.3890560989	8.3890560989
(0.5,1,075)	10.7971398111	9.4877358364	9.4877358364		11.7971398111	10.4877358364	10.4877358364
(0.5,1,1)	14.0074934328	12.1824939609	12.1824939607		15.0074934328	13.1824939609	13.1824939607
(0.75,1,0.25)	8.0339223664	7.3890560989	7.3890560989		9.0339223664	8.3890560989	8.3890560989
(0.75,1,0.5)	10.6208281666	9.4877358364	9.4877358364		11.6208281666	10.4877358364	10.4877358364
(0.75,1,0.75)	13.8638019450	12.1824939607	12.1824939607		14.8638019450	13.1824939607	13.1824939607
(0.75,1,1)	17.9859775918	15.6426318845	15.6426318842		18.9859775918	16.6426318845	16.6426318842
(1,1,0.25)	10.3157605141	9.4877358364	9.4877358364		11.3157605141	10.4877358364	10.4877358364
(1,1,0.5)	13.6374133121	12.1824939607	12.1824939607		14.6374133121	13.1824939607	13.1824939607
(1,1,0.75)	17.8014740693	15.6426318842	15.6426318842		18.8014740693	16.6426318842	16.6426318842
(1,1,1)	23.0944523718	20.0855369235	20.0855369232		24.0944523718	21.0855369236	21.0855369232

reports the comparison of exact results with 10th order results for ${\wp }_1$ and ${\wp }_2$ computed via ${}_O$HA$\mathbb{J}$TM with optimal value of ℏ for $0<z_1<1,z_2=1$ at different time levels $0<\tau \le 1$. The computation is carried out by taking $\hslash =-1$ and $\hslash =-1.0692$ (optimal value). One can see that, we can achieve faster convergence rate with the help of optimal value of the convergence control parameter (ℏ). The obtained error solutions witness the efficacy of the projected schemes.

For ${\wp }_1$: Figure 1a,b depict 2D and 3D behavior of 10th-order computed results for different $\alpha$. There is a significant variation in the obtained solutions for different fractional order $\alpha$. For the accuracy of the projected schemes, we can consider plots for $\alpha =1$ where the secured solutions are in best match with the exact solutions of the problem under consideration. Figure 1c,d depicts logarithmic plots of relative errors in $\kappa$th iterative results $(\kappa =6,8,10)$ via ${}_O$HA$\mathbb{J}$TM for $\alpha =0.85,1$, respectively, while Figure 1e,f depicts logarithmic plots of relative errors in $\kappa$th iterative results $(\kappa =6,8,10)$ via $\mathbb{J}$-VITM for $\alpha =0.85,1$, respectively. The value of the relative error for the obtained solution is decreases as we increase the iterations. In the 10th-order iteration, we have achieved the better solutions as compare to the previous iterations.

For ${\wp }_2$: Figure 2a,b, depict 2D and 3D behavior of 10th-order computed results for different $\alpha$. The velocity ${\wp }_2$ decreases with increase in time variable $\tau$. $\alpha =1$ curve matches exactly with the exact solution of the considered problem. The velocity ${\wp }_2$ drops faster for the decreasing fractional order $\alpha$. The 3D view of variation of the solution ${\wp }_2$ for different fractional order is presented to analyze the influence of fractional parameter $\alpha$. Figure 2c,d depicts logarithmic plots of relative errors in $\kappa$th iterative results $(\kappa =6,8,10)$ via ${}_O$HA$\mathbb{J}$TM for $\alpha =0.85,1$, respectively while Figure 2e,f depicts logarithmic plots of relative errors in $\kappa$th iterative results $(\kappa =6,8,10)$ via $\mathbb{J}$-VITM for $\alpha =0.85,1$ respectively. As we increase the number of iterations, we are getting the better approximate solution for both projected algorithms. These plots gives an explaination about how large the absolute error is in comparision with the exact numerical value of the solution.

For $\rho$: Figure 3a,b depict 2D and 3D behavior of 10th-order computed results for different $\alpha$. We can see that the density $\rho$ increases with increase in time $\tau$. The density distribution over the space with coordinates $(z_1,z_2,\tau )$ is presented in Figure 3b. Figure 3c,d depicts logarithmic plots of relative errors in $\kappa$th iterative results $(\kappa =6,8,10)$ via ${}_O$HA$\mathbb{J}$TM for $\alpha =0.85,1$, respectively, while Figure 3e,f depicts logarithmic plots of relative errors in $\kappa$th iterative results $(\kappa =6,8,10)$ via $\mathbb{J}$-VITM for $\alpha =0.85,1$, respectively. Table 3 cites that we have achieved the solution which is in best match with the exact solution of the considered problem. We can observe the same in Figure 3.

It is easy to demonstrate numerically from Figure 1c–f Figure 2c–f and Figure 3c–f and Table 1 and Table 2 that for a given order of approximation, ${}_O$HA$\mathbb{J}$TM with optimal ℏ are of high accuracy but requires larger CPU time as compared to $\mathbb{J}$-VITM. In addition both of the proposed hybrid methods converges, that is, ${}_O$HA$\mathbb{J}$TM with optimal ℏ converges faster than $\mathbb{J}$-VITM. For $\hslash =-1$, the rate of convergence of ${}_O$HA$\mathbb{J}$TM is the same as that of $\mathbb{J}$-VITM while $\mathbb{J}$-VITM requires less computational timethan ${}_O$HA$\mathbb{J}$TM.

6. Conclusions

In that present work studied, two space-dimensional time-fractional models governing the unsteady flow of pGas via two new efficient techniques so-called ${}_O$HA$\mathbb{J}$TM and $\mathbb{J}$-VITM. Both techniques are shown convergent with help of the Banach’s fixed point approach, and $\mathbb{J}$-VITM is shown $\mathrm{T}$-stable.

For an arbitrary fractional order $\alpha$, the evaluated solution behavior of the referred model equation is expressed in the form of well known Mittag–Leffler function. The effectiveness/validity of the evaluated new approximations is demonstrated via a numerical test example of a two space-dimensional time-fractional model governing the unsteady flow of a pGas by computing the absolute-errors/relative-error.

The numerical evaluation demonstrates that both of the developed techniques are convergent and perform better for the considered time-fractional model governing the unsteady flow of pGas. In addition, for given iteration new results by ${}_O$HA$\mathbb{J}$TM with optimal convergence control parameter (ℏ) are of high accuracy but require larger CPU time as compared to $\mathbb{J}$-VITM, that is, ${}_O$HA$\mathbb{J}$TM with optimal ℏ converges faster than $\mathbb{J}$-VITM. It is remarkably mentioned that for $\hslash =-1$, both methods converge to the exact results with the same rate of convergence while $\mathbb{J}$-VITM requires less computational time than ${}_O$HA$\mathbb{J}$TM. The motivation of this work is to explore the fractional behaviour of the considered model. We have observed the significant variations in the solutions for different fractional orders, which may lead to various physical consequences for the future work.