Aristotelian Diagrams for the Proportional Quantifier ‘Most’
Abstract
1. Introduction
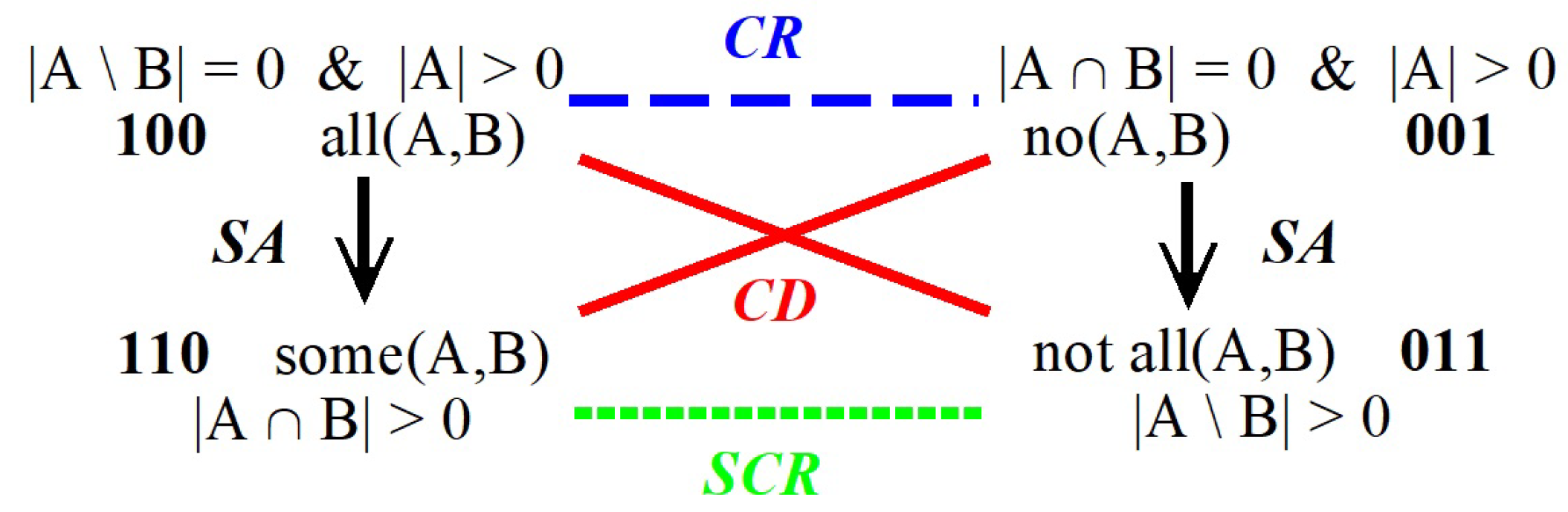
| a. | contradictory | , | iff | and cannot be true together and |
| and cannot be false together, | ||||
| b. | contrary | , | iff | and cannot be true together but |
| and can be false together, | ||||
| c. | subcontrary | , | iff | and can be true together but |
| and cannot be false together, | ||||
| d. | in subalternation | , | iff | entails but does not entail . |
| { | most(A,B), | ||||
| ¬most(A,B), | |||||
| most(A,¬B), | |||||
| ¬most(A,¬B) | } |
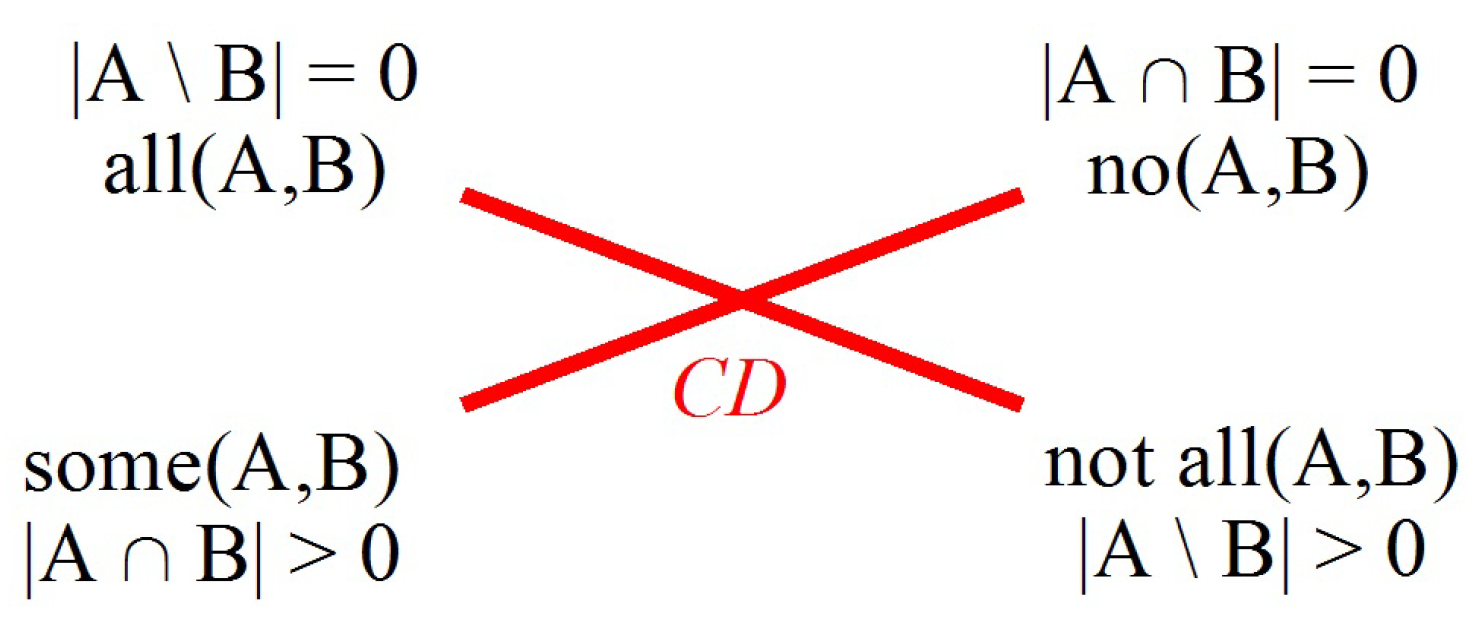
| { | all(A,B), | all(A,B) | |||||
| not all(A,B), | ¬all(A,B) | ||||||
| no(A,B), | all(A,¬B) | ||||||
| some(A,B), | } | ¬all(A,¬B) |
2. Aristotelian Squares for ‘Most’ versus ‘All’
2.1. Aristotelian Relations for ‘Most’ versus ‘All’
2.2. Bitstring Semantics for ‘Most’ versus ‘All’
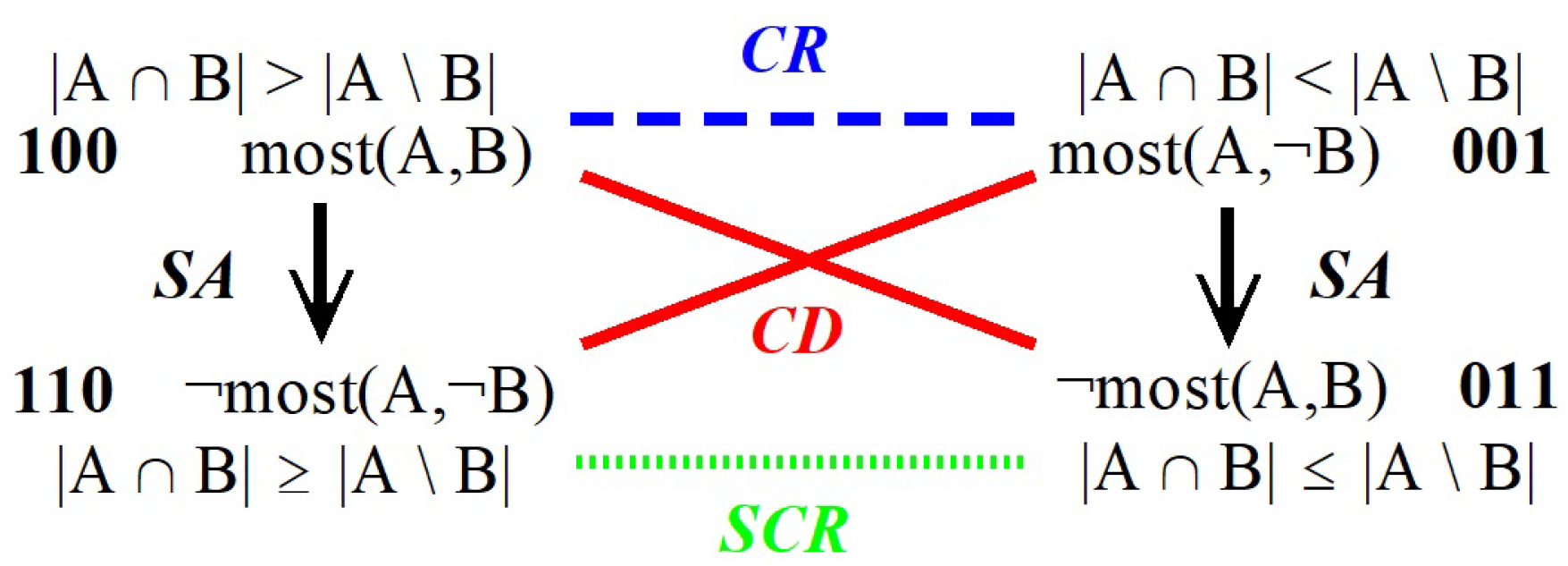
| = | { | : more than half (A,B), | |||
| : exactly half (A,B), | |||||
| : less than half (A,B) | } |
| = | 100 | |||
| = | 011 | |||
| = | 001 | |||
| = | 110 |
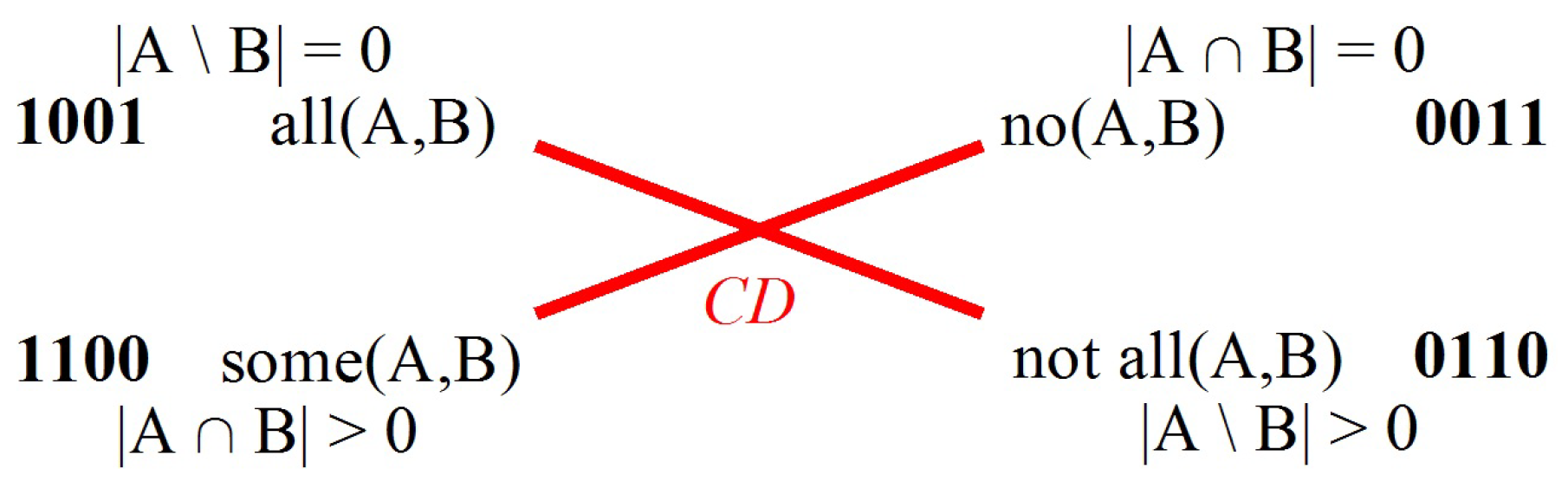
| = | { | : all A are B & there are A’s, | |||
| : some but not all A are B, | |||||
| : no A are B & there are A’s, | |||||
| : there are no A’s | } |
| = | 1001 | |||
| = | 0110 | |||
| = | 0011 | |||
| = | 1100 |
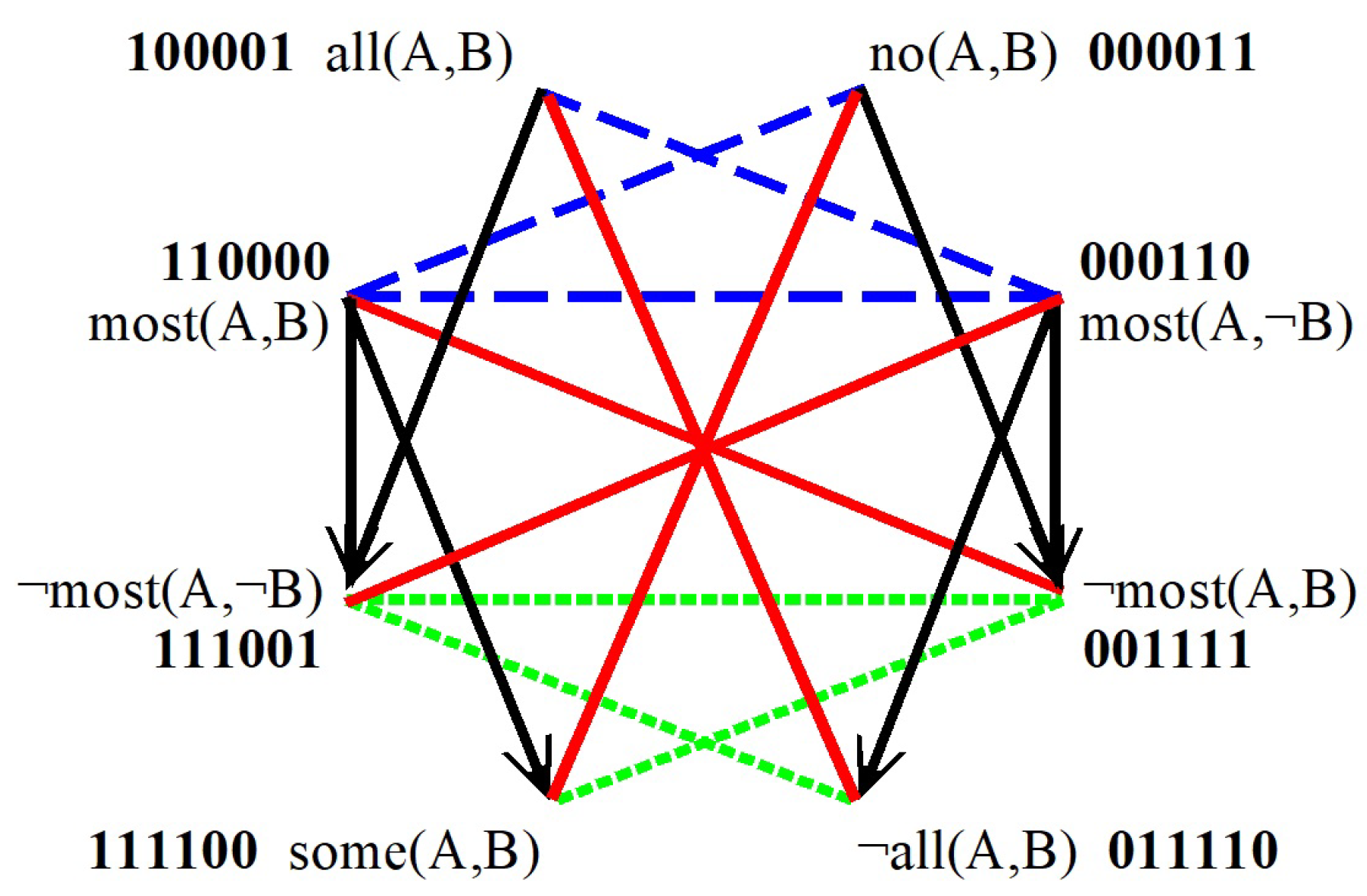
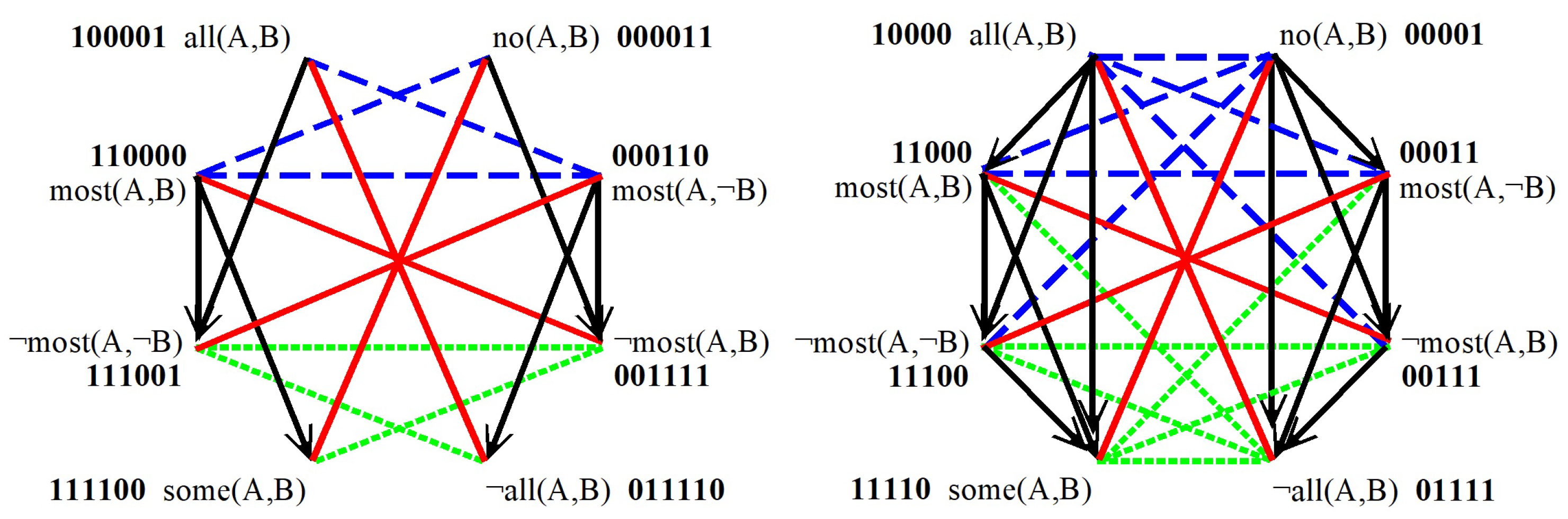
3. A First Aristotelian Octagon for ‘Most’ and ‘All’
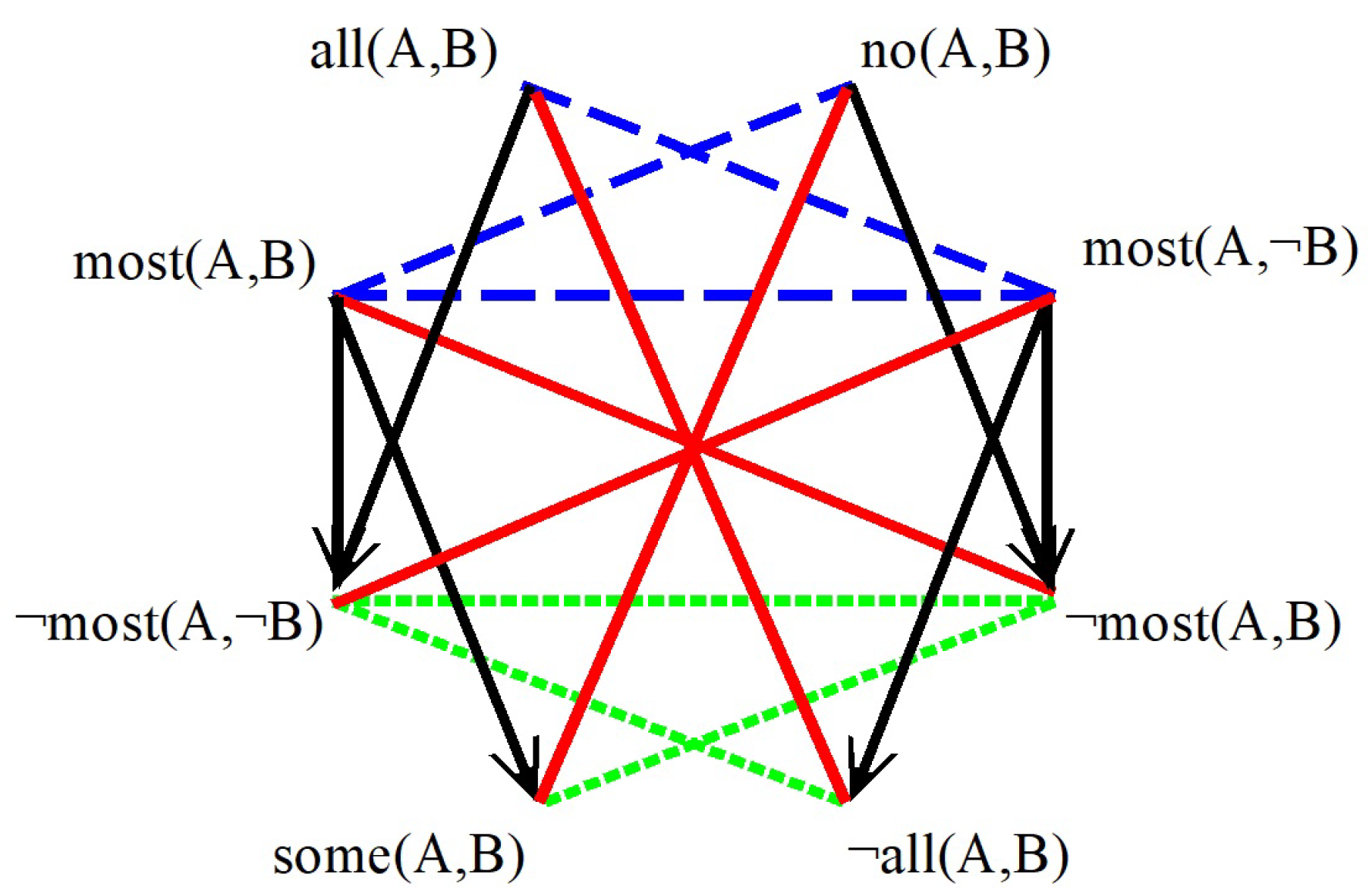
| = | { | all(A,B), | |||
| no(A,B), | |||||
| most(A,B), | |||||
| most(A,¬B), | |||||
| ¬most(A,¬B), | |||||
| ¬most(A,B), | |||||
| some(A,B), | |||||
| ¬all(A,B) | } |
3.1. Aristotelian Relations in the First Octagon
| SA[ | most(A,B), | some(A,B) | ] | if | then | |
| SA[ | most(A,¬B), | ¬all(A,B) | ] | if | then | |
| SA[ | all(A,B), | ¬most(A,¬B) | ] | if | then | |
| SA[ | no(A,B), | ¬most(A,B) | ] | if | then |
| CR[ | all(A,B), | most(A,¬B) | ] | CR[ | most(A,B), | no(A,B) | ] |
| SCR[ | ¬most(A,¬B), | ¬all(A,B) | ] | SCR[ | some(A,B), | ¬most(A,B) | ] |
| { | all(A,B), | most(A,¬B), | ¬most(A,¬B), | ¬all(A,B) | } | ||
| { | most(A,B), | no(A,B), | some(A,B), | ¬most(A,B) | } |
| { | most(A,B), | all(A,B), | ¬all(A,B), | ¬most(A,B) | } | ||
| { | no(A,B), | most(A,¬B), | ¬most(A,¬B), | some(A,B) | } |
3.2. Bitstring Semantics for the First Octagon
| = | { | : more than half (A,B), | |||
| : exactly half (A,B), | |||||
| : less than half (A,B) | } | ||||
| = | { | : all A are B & there are A’s, | |||
| : some but not all A are B, | |||||
| : no A are B & there are A’s, | |||||
| : there are no A’s | } |
| { | : all A are B and there are A’s, | |||
| : most but not all A’s are B, | ||||
| : exactly half the A’s are B, | ||||
| : most but not all A’s are not B, | ||||
| : no A’s are B, but there are A’s, | ||||
| : there are no A’s | } |
| = | 100001 | |||
| = | 000011 | |||
| = | 110000 | |||
| = | 000110 | |||
| = | 111001 | |||
| = | 001111 | |||
| = | 111100 | |||
| = | 011110 |
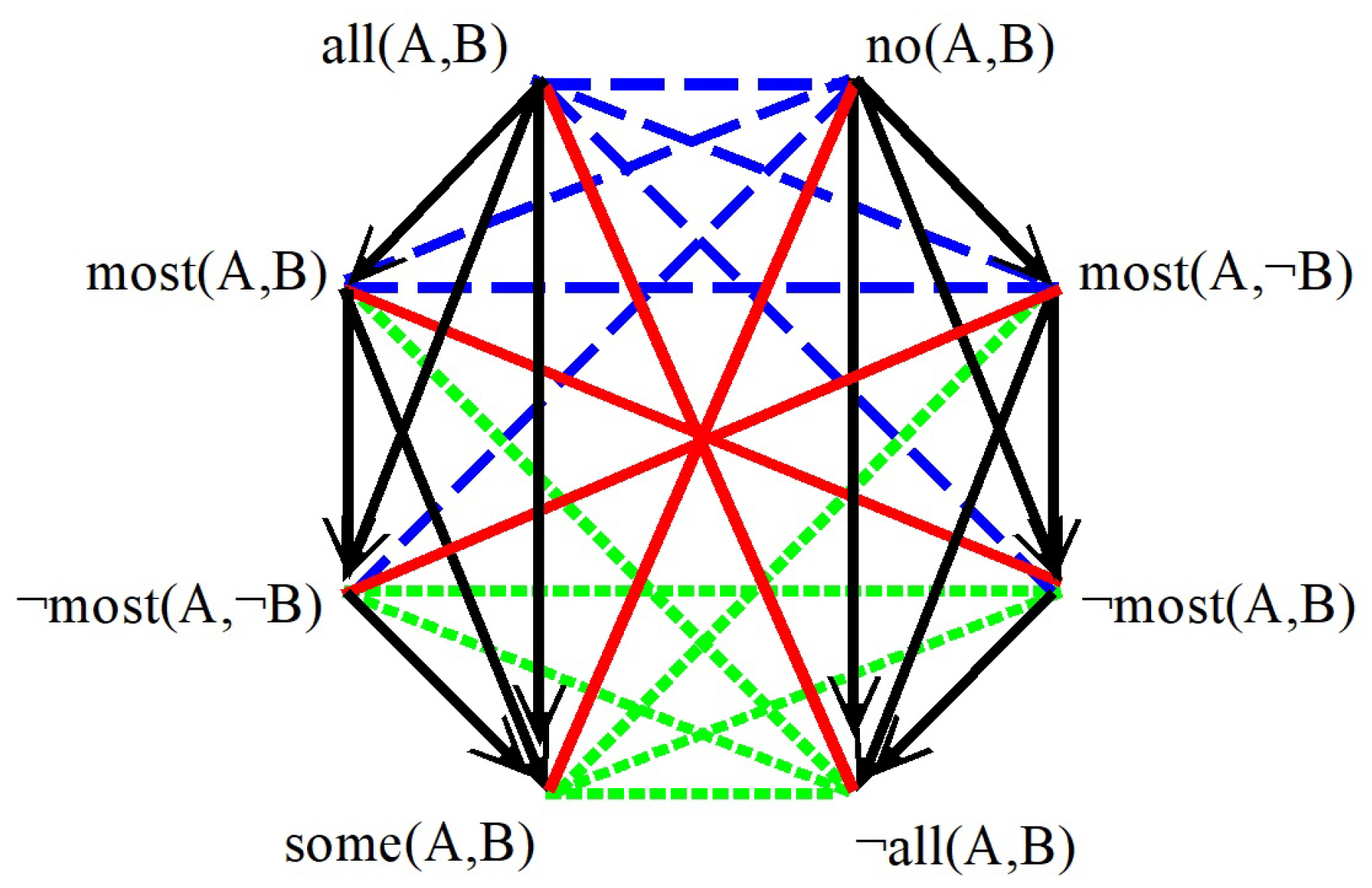
4. A Second Aristotelian Octagon for ‘Most’ and ‘All’
| = | { | all(A,B), | |||
| ¬all(A,B), | |||||
| no(A,B), | |||||
| some(A,B) | } |
| = | { | : all A are B & there are A’s, | |||
| : some but not all A are B, | |||||
| : no A are B & there are A’s | } |
| = | 100 | |||
| = | 011 | |||
| = | 001 | |||
| = | 110 |
| = | { | all(A,B), | |||
| no(A,B), | |||||
| most(A,B), | |||||
| most(A,¬B), | |||||
| ¬most(A,¬B), | |||||
| ¬most(A,B), | |||||
| some(A,B), | |||||
| ¬all(A,B) | } |
4.1. Aristotelian Relations in the Second Octagon
| SA[ | all(A,B), | most(A,B) | ] | SA[ | ¬most(A,B), | ¬all(A,B) | ] |
| SA[ | no(A,B), | most(A,¬B) | ] | SA[ | ¬most(A,¬B), | some(A,B) | ] |
| CR[ | all(A,B), | ¬most(A,B) | ] | CR[ | ¬most(A,¬B), | no(A,B) | ] |
| SCR[ | most(A,B), | ¬all(A,B) | ] | SCR[ | some(A,B), | most(A,¬B) | ] |
| relations in | ...relative to | ...relative to |
| contradiction | 4 | 4 |
| contrariety | 3 | 6 |
| subcontrariety | 3 | 6 |
| subalternation | 6 | 12 |
| unconnectedness | 12 | 0 |
| total () | 28 | 28 |
| = | |||||||
| = | { | all(A,B), | most(A,¬B), | ¬most(A,¬B), | ¬all(A,B) | } | |
| = | { | most(A,B), | no(A,B), | some(A,B), | ¬most(A,B) | } | |
| = | |||||||
| = | { | most(A,B), | all(A,B), | ¬all(A,B), | ¬most(A,B) | } | |
| = | { | no(A,B), | most(A,¬B), | ¬most(A,¬B), | some(A,B) | } |
4.2. Bitstring Semantics for the Second Octagon
| = | { | : more than half (A,B), | |||
| : exactly half (A,B), | |||||
| : less than half (A,B) | } | ||||
| = | { | : all A are B & there are A’s, | |||
| : some but not all A are B, | |||||
| : no A are B & there are A’s, | } |
| { | : All A are B and there are A’s, | |||
| : Most but not all A’s are B, | ||||
| : Exactly half the A’s are B, | ||||
| : Most but not all A’s are not B, | ||||
| : No A’s are B, but there are A’s, | } |
| = | 10000 | |||
| = | 00001 | |||
| = | 11000 | |||
| = | 00011 | |||
| = | 11100 | |||
| = | 00111 | |||
| = | 11110 | |||
| = | 01111 |
5. Conclusions
Author Contributions
Funding

Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Demey, L.; Smessaert, H. Combinatorial Bitstring Semantics for Arbitrary Logical Fragments. J. Philos. Log. 2018, 47, 325–363. [Google Scholar] [CrossRef]
- Smessaert, H.; Demey, L. Logical Geometries and Information in the Square of Opposition. J. Log. Lang. Inf. 2014, 23, 527–565. [Google Scholar] [CrossRef]
- Barwise, J.; Cooper, R. Generalized Quantifiers and Natural Language. Linguist. Philos. 1981, 4, 159–219. [Google Scholar] [CrossRef]
- Keenan, E.L. The Semantics of Determiners. In The Handbook of Contemporary Semantic Theory; Lappin, S., Ed.; Blackwell: Hoboken, NJ, USA, 1996; pp. 41–64. [Google Scholar]
- Peters, S.; Westerståhl, D. Quantifiers in Language and Logic; Oxford University Press: Oxford, UK, 2006. [Google Scholar]
- Peterson, P.L. On the Logic of “Few”, “Many”, and “Most”. Notre Dame J. Form. Log. 1979, 20, 155–179. [Google Scholar] [CrossRef]
- Peterson, P.L. Intermediate Quantities. Logic, Linguistics, and Aristotelian Semantics; Ashgate: Farnham, UK, 2000. [Google Scholar]
- Brown, M. Generalized Quantifiers and the Square of Opposition. Notre Dame J. Form. Log. 1984, 25, 303–322. [Google Scholar] [CrossRef]
- Veloso, S.R.M.; Veloso, P.A.S. On Modulated Logics for ‘Generally’: Some Metamathematical Issues. In Aspects of Universal Logic; Béziau, J.Y., Costa-Leite, A., Facchini, A., Eds.; Centre de Recherches Sémiologiques—Université de Neuchâtel: Neuchâtel, Switzerland, 2004; pp. 146–168. [Google Scholar]
- Murinová, P.; Novák, V. Analysis of Generalized Square of Opposition with Intermediate Quantifiers. Fuzzy Sets Syst. 2014, 242, 89–113. [Google Scholar] [CrossRef]
- Murinová, P.; Novák, V. Syllogisms and 5-Square of Opposition with Intermediate Quantifiers in Fuzzy Natural Logic. Log. Univers. 2016, 10, 339–357. [Google Scholar] [CrossRef]
- Béziau, J.Y.; Payette, G. Preface. In The Square of Opposition. A General Framework for Cognition; Béziau, J.Y., Payette, G., Eds.; Peter Lang: Bern, Switzerland, 2012; pp. 9–22. [Google Scholar]
- Demey, L.; Smessaert, H. Duality in Logic and Language. In Internet Encyclopedia of Philosophy; Fieser, J., Dowden, B., Eds.; University of Tennessee: Martin, TN, USA, 2016. [Google Scholar]
- Demey, L.; Smessaert, H. Aristotelian and Duality Relations Beyond the Square of Opposition. In Diagrammatic Representation and Inference; Chapman, P., Stapleton, G., Moktefi, A., Perez-Kriz, S., Bellucci, F., Eds.; LNCS 10871; Springer: Berlin/Heidelberg, Germany, 2018; pp. 640–656. [Google Scholar]
- Demey, L.; Smessaert, H. Using Multigraphs to Study the Interaction Between Opposition, Implication and Duality Relations in Logical Squares. In Diagrammatic Representation and Inference; Pietarinen, A.V., Chapman, P., Bosveld-de Smet, L., Giardino, V., Corter, J., Linker, S., Eds.; LNCS 12169; Springer: Berlin/Heidelberg, Germany, 2020; pp. 385–393. [Google Scholar]
- Smessaert, H.; Demey, L. The Unreasonable Effectiveness of Bitstrings in Logical Geometry. In The Square of Opposition: A Cornerstone of Thought; Béziau, J.Y., Basti, G., Eds.; Springer: Berlin/Heidelberg, Germany, 2017; pp. 197–214. [Google Scholar]
- Demey, L. Computing the Maximal Boolean Complexity of Families of Aristotelian Diagrams. J. Log. Comput. 2018, 28, 1323–1339. [Google Scholar] [CrossRef]
- Demey, L. Metalogic, Metalanguage and Logical Geometry. Log. Anal. 2019, 248, 453–478. [Google Scholar]
- Frijters, S. Aristotelian Diagrams for the Modal Logic of All and Only. Presented at the SQUARE 2022 Congress, Leuven, Belgium, 9–13 September 2022.
- Lenzen, W. How to Square Knowledge and Belief. In Around and Beyond the Square of Opposition; Béziau, J.Y., Jacquette, D., Eds.; Springer: Berlin/Heidelberg, Germany, 2012; pp. 305–311. [Google Scholar]
- Moretti, A. The Geometry of Logical Opposition. Ph.D. Thesis, University of Neuchâtel, Neuchâtel, Switzerland, 2009. [Google Scholar]
- Beziau, J.Y. The New Rising of the Square of Opposition. In Around and Beyond the Square of Opposition; Beziau, J.Y., Jacquette, D., Eds.; Springer: Berlin/Heidelberg, Germany, 2012; pp. 3–19. [Google Scholar]
- Klima, G. John Buridan, Summulae de Dialectica; Yale University Press: New Haven, CT, USA, 2001. [Google Scholar]
- Demey, L. Boolean Considerations on John Buridan’s Octagons of Opposition. Hist. Philos. Log. 2019, 40, 116–134. [Google Scholar] [CrossRef]
- Johnson, W. Logic. Part I; Cambridge University Press: Cambridge, UK, 1921. [Google Scholar]
- Keynes, J.N. Studies and Exercises in Formal Logic; MacMillan: New York, NY, USA, 1884. [Google Scholar]
- Demey, L. Logic-Sensitivity of Aristotelian Diagrams in Non-Normal Modal Logics. Axioms 2021, 10, 128. [Google Scholar] [CrossRef]
- Demey, L. Interactively Illustrating the Context-Sensitivity of Aristotelian Diagrams. In Modeling and Using Context; Christiansen, H., Stojanovic, I., Papadopoulos, G., Eds.; LNCS 9405; Springer: Berlin/Heidelberg, Germany, 2015; pp. 331–345. [Google Scholar]
- Hackl, M. On the Grammar and Processing of Proportional Quantifiers: Most versus More than Half. Nat. Lang. Semant. 2009, 17, 63–98. [Google Scholar] [CrossRef]
- Solt, S. On Measurement and Quantification: The Case of “Most” and “More than Half”. Language 2016, 92, 65–100. [Google Scholar] [CrossRef]


| Aristotelian Diagram | ||||
|---|---|---|---|---|
| 4 | 3 | classical square | ||
| 4 | 3 | classical square | ||
| 4 | 4 | degenerate square | ||
| 4 | 3 | classical square | ||
| 8 | 6 | new type of octagon | ||
| 8 | 5 | Lenzen octagon |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Smessaert, H.; Demey, L. Aristotelian Diagrams for the Proportional Quantifier ‘Most’. Axioms 2023, 12, 236. https://doi.org/10.3390/axioms12030236
Smessaert H, Demey L. Aristotelian Diagrams for the Proportional Quantifier ‘Most’. Axioms. 2023; 12(3):236. https://doi.org/10.3390/axioms12030236
Chicago/Turabian StyleSmessaert, Hans, and Lorenz Demey. 2023. "Aristotelian Diagrams for the Proportional Quantifier ‘Most’" Axioms 12, no. 3: 236. https://doi.org/10.3390/axioms12030236
APA StyleSmessaert, H., & Demey, L. (2023). Aristotelian Diagrams for the Proportional Quantifier ‘Most’. Axioms, 12(3), 236. https://doi.org/10.3390/axioms12030236






