Analysis of Novel Oscillations of Quantized Mechanical Energy in Mass-Accreting Nano-Oscillator Systems
Abstract
:1. Introduction
2. Preliminary Analysis in Classical Domain
3. Quantum Analysis
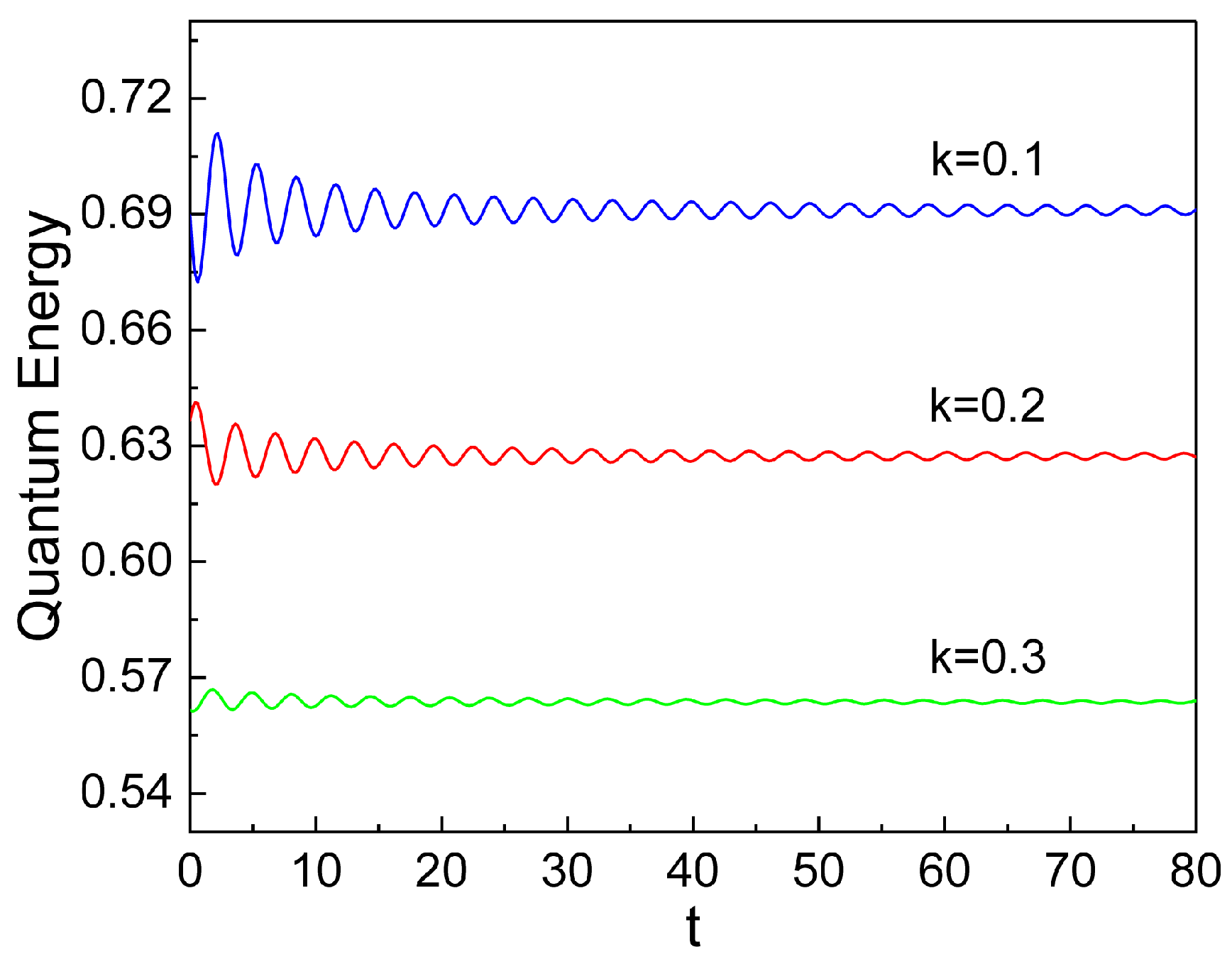
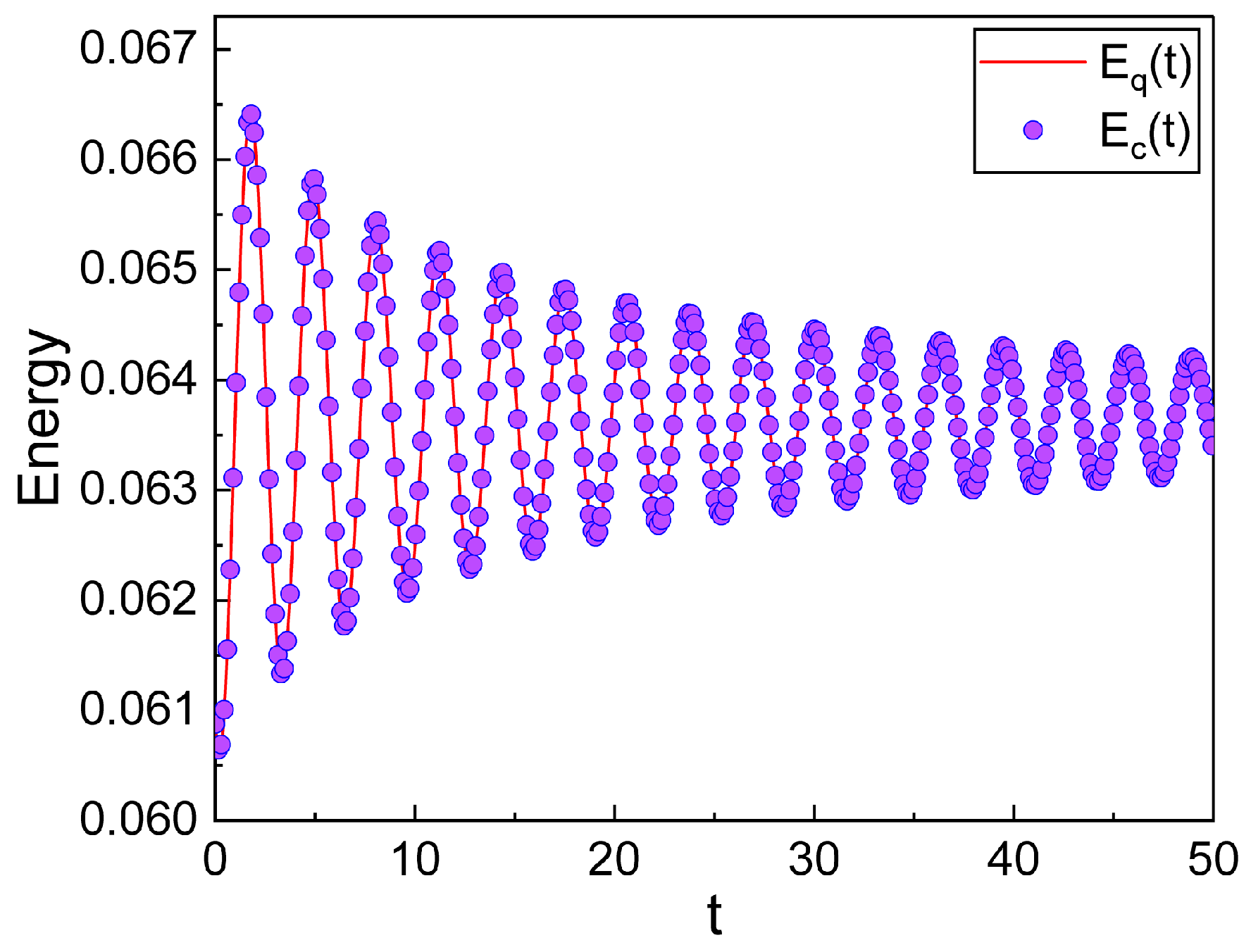
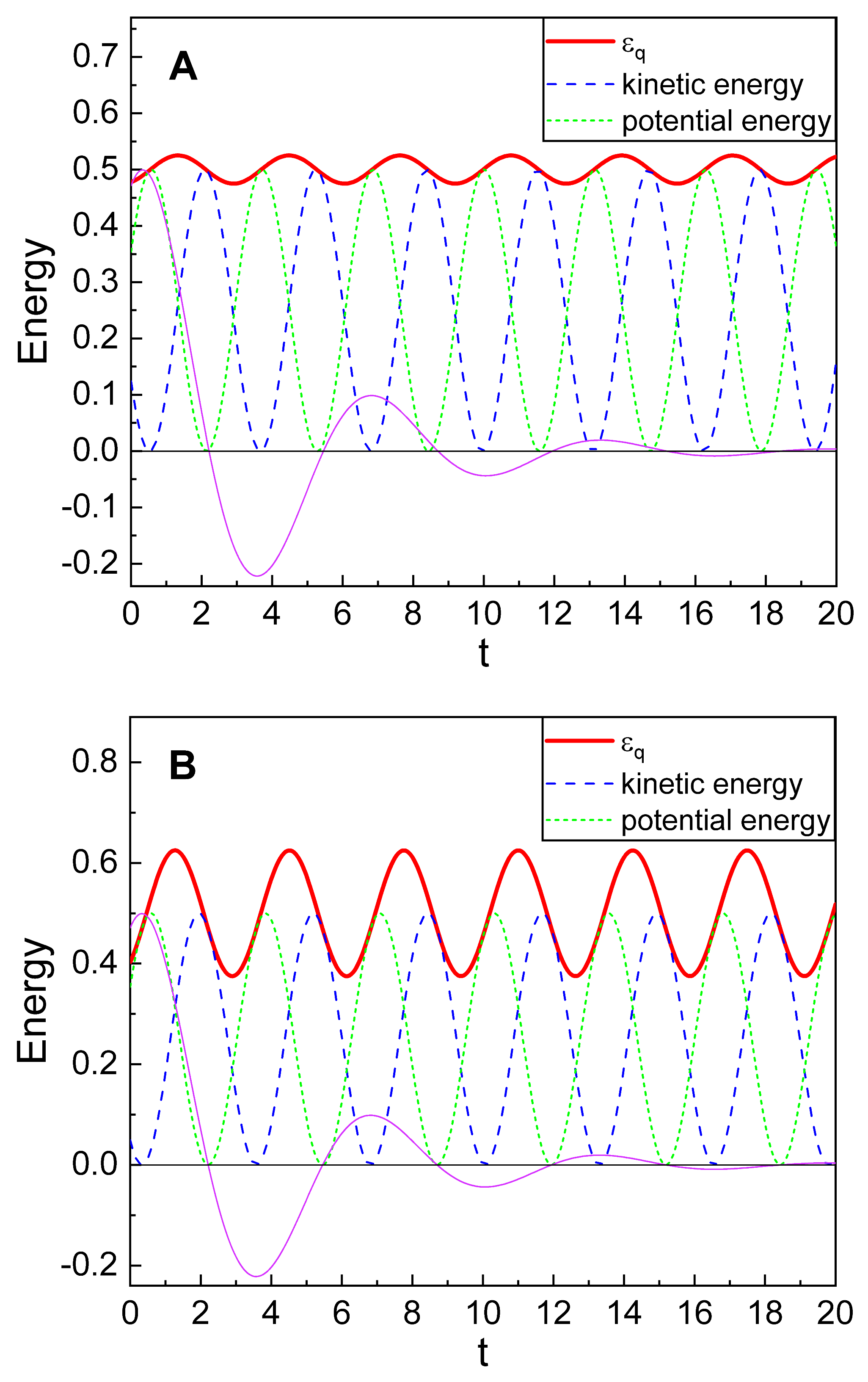
3.1. Linearly Mass-Accreting Oscillator
3.2. Comparison with the Exponentially Mass-Accreting Oscillator
4. Conclusions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Choi, J.R. Unitary transformation approach for the phase of the damped driven harmonic oscillator. Mod. Phys. Lett. B 2003, 17, 1365–1376. [Google Scholar] [CrossRef]
- Pétri, J. Forced oscillations in a hydrodynamical accretion disk and QPOs. Astrophys. Space Sci. 2006, 302, 117–139. [Google Scholar] [CrossRef] [Green Version]
- Zhang, S.-H.; Yan, Z.-Y. Frequency dependence of the entanglement entropy production in a system of coupled driven nonlinear oscillators. Entropy 2019, 21, 889. [Google Scholar] [CrossRef] [Green Version]
- Golovinski, P.A. Dynamics of driven Brownian inverted oscillator. Phys. Lett. A 2020, 384, 126203. [Google Scholar] [CrossRef]
- Kenfack, S.C.; Nguimeya, G.P.; Talla, P.K.; Fotue, A.J.; Fobasso, M.F.C.; Tiotsop, M.; Djomou, J.R.D.; Ekosso, C.M.; Fai, L.C. Decoherence of driven coupled harmonic oscillator. J. Nanosci. Curr. Res. 2016, 1, 1000104. [Google Scholar]
- Marchiolli, M.A.; Mizrahi, S.S. Dissipative mass-accreting quantum oscillator. J. Phys. A Math. Gen. 1997, 30, 2619–2635. [Google Scholar] [CrossRef]
- Ray, J.R. Dissipation and quantum theory. Lett. Al Nuovo Cimento 1979, 25, 47–50. [Google Scholar] [CrossRef]
- Choi, J.R.; Song, J.N.; Hong, S.J. Wigner distribution function of superposed quantum states for a time-dependent oscillator-like Hamiltonian system. J. Theor. Appl. Phys. 2012, 6, 26. [Google Scholar] [CrossRef] [Green Version]
- Choi, J.R. Analysis of quantum energy for Caldirola-Kanai Hamiltonian systems in coherent states. Results Phys. 2013, 3, 115–121. [Google Scholar] [CrossRef] [Green Version]
- González-Acosta, E.; Corona-Galindo, M.G. Noether’s theorem and the invariants for dissipative and driven dissipative like systems. Rev. Mex. Fis. 1992, 38, 511–517. [Google Scholar]
- Dekker, H. Classical and quantum mechanics of the damped harmonic oscillator. Phys. Rep. 1981, 80, 1–110. [Google Scholar] [CrossRef]
- Rosenau da Costa, M.; Caldeira, A.O.; Dutra, S.M.; Westfahl, H., Jr. Exact diagonalization of two quantum models for the damped harmonic oscillator. Phys. Rev. A 2000, 61, 022107. [Google Scholar] [CrossRef] [Green Version]
- Schuch, D. Some remarks on analytical solutions for a damped quantum parametric oscillator. J. Phys. Conf. Ser. 2019, 1275, 012033. [Google Scholar] [CrossRef]
- Aguiar, V.; Guedes, I. Fisher information of quantum damped harmonic oscillators. Phys. Scr. 2015, 90, 045207. [Google Scholar] [CrossRef]
- Lawson, L.M.; Avossevou, G.Y.H.; Gouba, L. Lewis-Riesenfeld quantization and SU(1,1) coherent states for 2D damped harmonic oscillator. J. Math. Phys. 2018, 59, 112101. [Google Scholar] [CrossRef] [Green Version]
- Nowak, M.A.; Wagoner, R.V. Diskoseismology: Probing accretion disks. I. Trapped adiabatic oscillations. Astrophys. J. 1991, 378, 656–664. [Google Scholar] [CrossRef]
- Lubow, S.H.; Prinole, J.E. Wave propagation in accretion disks: Axisymmetric case. Astrophys. J. 1993, 409, 360–371. [Google Scholar] [CrossRef]
- Wagoner, R.V. Relativistic and Newtonian diskoseismology. New Astron. Rev. 2008, 51, 828–834. [Google Scholar] [CrossRef]
- Cox, J.P. Adiabatic oscillations of accretion disks. Astrophys. J. 1981, 247, 1070–1077. [Google Scholar] [CrossRef]
- Li, L.-X.; Goodman, J.; Narayan, R. Nonaxisymmetric g-mode and p-mode instability in a hydrodynamic thin accretion disk. Astrophys. J. 2003, 593, 593–980. [Google Scholar] [CrossRef] [Green Version]
- California Institute of Technology CalTech. Multiple Crystal Oscillator Measuring Apparatus. U.S. Patent 3879992 A, 29 April 1975. [Google Scholar]
- Caldirola, P. Porze non conservative nella meccanica quantistica. Nuovo Cimento 1941, 18, 393–400. [Google Scholar] [CrossRef]
- Kanai, E. On the quantization of dissipative systems. Prog. Theor. Phys. 1948, 3, 440–442. [Google Scholar] [CrossRef]
- Milburn, G.J.; Holmes, C.A. Quantum coherence and classical chaos in a pulsed parametric oscillator with a Kerr nonlinearity. Phys. Rev. A 1991, 44, 4704–4711. [Google Scholar] [CrossRef] [Green Version]
- Wielinga, B.; Milburn, G.J. Chaos and coherence in an optical system subject to photon nondemolition measurement. Phys. Rev. A 1992, 46, 762–770. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Leoński, W. Quantum and classical dynamics for a pulsed nonlinear oscillator. Physica A 1996, 233, 365–378. [Google Scholar] [CrossRef]
- Kowalewska-Kudłaszyk, A.; Kalaga, J.K.; Leoński, W. Wigner-function nonclassicality as indicator of quantum chaos. Phys. Rev. E 2008, 78, 066219. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Lewis, H.R., Jr. Classical and quantum systems with time-dependent harmonic-oscillator-type Hamiltonians. Phys. Rev. Lett. 1967, 18, 510–512. [Google Scholar] [CrossRef]
- Lewis, H.R., Jr.; Riesenfeld, W.B. An exact quantum theory of the time-dependent harmonic oscillator and of a charged particle in a time-dependent electromagnetic field. J. Math. Phys. 1969, 10, 1458–1473. [Google Scholar] [CrossRef]
- Abdalla, M.S.; Choi, J.R. Propagator for the time-dependent charged oscillator via linear and quadratic invariants. Ann. Phys. 2007, 322, 2795–2810. [Google Scholar] [CrossRef]
- Choi, J.R. Exact quantum theory of noninteracting electrons with time-dependent effective mass in a time-dependent magnetic field. J. Phys. Condens. Matter 2003, 15, 823–832. [Google Scholar] [CrossRef]
- Choi, J.R. An approach to dark energy problem through linear invariants. Chin. Phys. C 2011, 35, 233–242. [Google Scholar] [CrossRef]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Choi, J.R. Analysis of Novel Oscillations of Quantized Mechanical Energy in Mass-Accreting Nano-Oscillator Systems. Axioms 2021, 10, 153. https://doi.org/10.3390/axioms10030153
Choi JR. Analysis of Novel Oscillations of Quantized Mechanical Energy in Mass-Accreting Nano-Oscillator Systems. Axioms. 2021; 10(3):153. https://doi.org/10.3390/axioms10030153
Chicago/Turabian StyleChoi, Jeong Ryeol. 2021. "Analysis of Novel Oscillations of Quantized Mechanical Energy in Mass-Accreting Nano-Oscillator Systems" Axioms 10, no. 3: 153. https://doi.org/10.3390/axioms10030153
APA StyleChoi, J. R. (2021). Analysis of Novel Oscillations of Quantized Mechanical Energy in Mass-Accreting Nano-Oscillator Systems. Axioms, 10(3), 153. https://doi.org/10.3390/axioms10030153




