1. Introduction
Reinjection is crucial for all types of geothermal reservoirs in production and can be the key factor in the success or failure of geothermal power development [
1]. The injection of heat-depleted brines into clastic sedimentary reservoirs with alternating clay, sand, and sandstone sequences has long been considered a delicate subject among petroleum and geothermal operators. Without thorough and careful planning, the injection can turn into a disaster, with scenarios including the reinjection of incompatible waters into the formation, particle entrainment, capture, and release, or unsuccessful well completion, which often leads to irreparable damage to the well and formation [
1,
2,
3,
4,
5,
6,
7,
8,
9,
10,
11].
Sandstones often have poor reservoir quality [
12,
13]. This is due to the early development of secondary mineral phases and mechanical compaction, which reduce permeability and porosity [
14,
15,
16]. The two most important cementing mineral phases for sandstones are carbonate minerals (calcite, dolomite, ankerite) and quartz. The factors determining the solubility of these mineral phases (e.g., temperature, pressure, solute content, and pH) often have opposite effects on the solubility of the two phases. The solubility of CaCO
3 (e.g., calcite) is primarily controlled by the amount of dissolved CO
2 and pH and increases with decreasing temperature. On the other hand, the solubility of SiO
2 (e.g., quartz, amorphous SiO
2) decreases with decreasing temperature. Similarly, a change in pH has the opposite effect on solubility, with the solubility of CaCO
3 being highest at low pH (pH < 7, acidic medium) and that of SiO
2 being highest at high pH (pH > 9–10, alkaline medium). In natural systems, the precipitation of carbonate minerals is typically promoted by the exsolution of dissolved CO
2—driven by a decline in pore pressure or an increase in temperature or salinity (i.e., “salting out”)—which can lead to a significant increase in pH. The solubility of both SiO
2 and CaCO
3 increases with pressure and, to a certain extent, with an increase in dissolved ion concentrations [
17,
18,
19].
This investigation focuses on water–rock interactions in a sandstone geothermal reservoir during recharge conditions, specifically in the Szentes area of Hungary. Previous studies, such as Markó et al. [
6], describe injection-related issues in a Hungarian sandstone doublet system, emphasizing formation damage and pressure drawdown. Their research addresses similar reservoir conditions in the Pannonian Basin and highlights the risk of fines migration. Ungemach [
3] provides a comprehensive operational perspective on reinjecting cooled geothermal brines into clastic formations and emphasizes potential plugging hazards. Sætre et al. [
12] employed reactive transport modeling for intra-basaltic sandstones in the North Atlantic. Although they conducted 1D simulations to examine diagenetic alteration, the geological context—basalt–sandstone interfaces—differs from the unconsolidated sandstones of the Pannonian Basin. Koroncz et al. [
13] investigated the Upper Miocene–Pliocene (Upper Pannonian) reservoir from a petrophysical standpoint, focusing on permeability and lithology. While this study considers scaling and diagenesis, it does not model short-term versus long-term geochemical reactions at the same experimental depth, meaning that it does not provide actual lab-measured reaction rates under controlled temperature and pressure conditions.
In this study, we design a series of laboratory experiments to evaluate geochemical interactions under specific conditions. Geochemical simulations of these laboratory experiments with two different thermodynamic databases align with the kinetic data obtained. Thermodynamic and kinetic models were developed to estimate water–rock interactions induced by the reinjection of heat-depleted water at the Szentes Geothermal Area. The findings of this research underline the importance of laboratory testing and site-specific analysis of geochemical reactions based on local conditions and provide a solid theoretical foundation and valuable technical guidance for understanding water–rock interactions, as well as addressing potential chemical plugging issues that may arise during geothermal development in sandstone reservoirs in Hungary.
2. Geological Setting
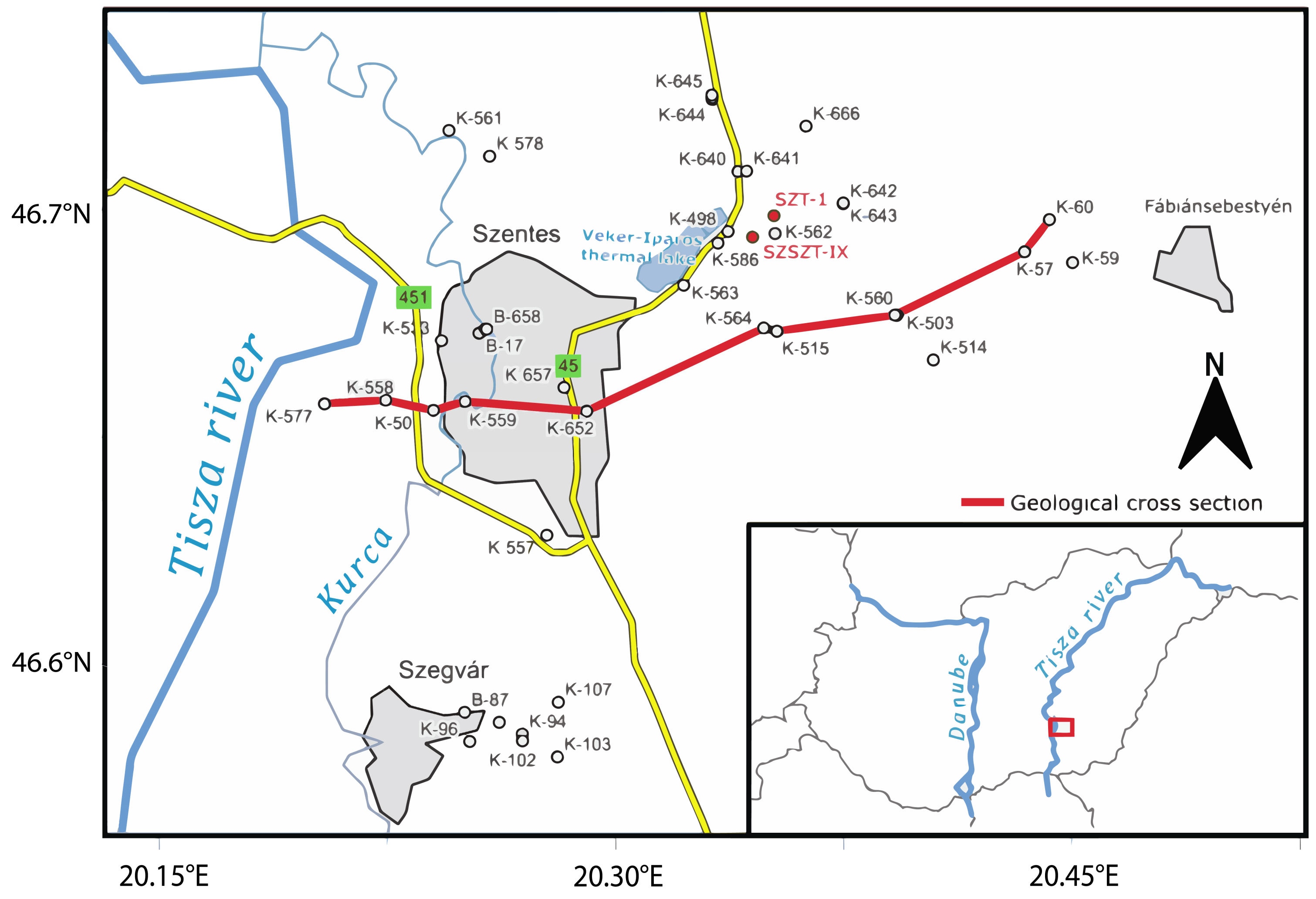
This study was conducted as part of a research and development project in the Szentes area (see
Figure 1). During the project, two wells, SZT-1 and SZSZT-IX, were drilled and sampled continuously, but using different methods (rock chips, drill core) at various depth intervals, with a focus on sandy formations, in line with the project’s objectives. The Szentes area is located in the northeastern section of the Makó–Hódmezővásárhely Trough, where the pre-Neogene basement lies at depths of 4500–5000 m [
20,
21,
22]. During the Miocene, the trough was filled with gravel and conglomerate as a result of erosion from the Algyő and Pusztaföldvár Highs, leading to the deposition of porous Pannonian sediments [
23,
24]. In this study area, the pre-Neogene basement has been reached by only two boreholes, FÁB-4 and Szentes ÉK-1, which have exposed Upper Cretaceous sandstone and siltstone, as well as Lower Triassic sandstone and clay layers [
25]. During the Pannonian period, the sedimentary environments transitioned vertically from a deep basin to a delta slope, then to a delta front, and ultimately to a delta plain [
26]. The total thickness of the Pannonian sediments can exceed 3000 m [
26]. The thermal wells in Szentes revealed the Upper Pannonian (Upper Miocene–Pliocene) Újfalu Formation, characterized by delta front and delta plain facies, overlain by a delta top and alluvial plain facies known as the Zagyva Formation [
26,
27]. The Újfalu Formation consists of sandstone, siltstone, and clay marl, with sandstone layers being predominant. It is primarily composed of channel and mouth bar sediments, which possess good reservoir properties, but have limited lateral extension [
27]. However, due to multiple erosion and superposition events, these layers are hydrodynamically interconnected. The overlying Zagyva Formation represents a depositional environment characterized by an alluvial plain with a highly heterogeneous composition. In this formation, the alternating layers of sandstone, silty sand, silty clay, and clay are quite common. In the alluvial plain, sedimentary cycles are dominated by channel and point-bar patterns [
26,
27]. The upper boundary of the Zagyva Formation is indicated by a decrease in the sandstone content, which is typically easy to identify. The Quaternary layers deposited over the Upper Pliocene sediments have an average thickness of 600–700 m and contribute to the water supply of Szentes.
Geothermal Reservoir Characterization
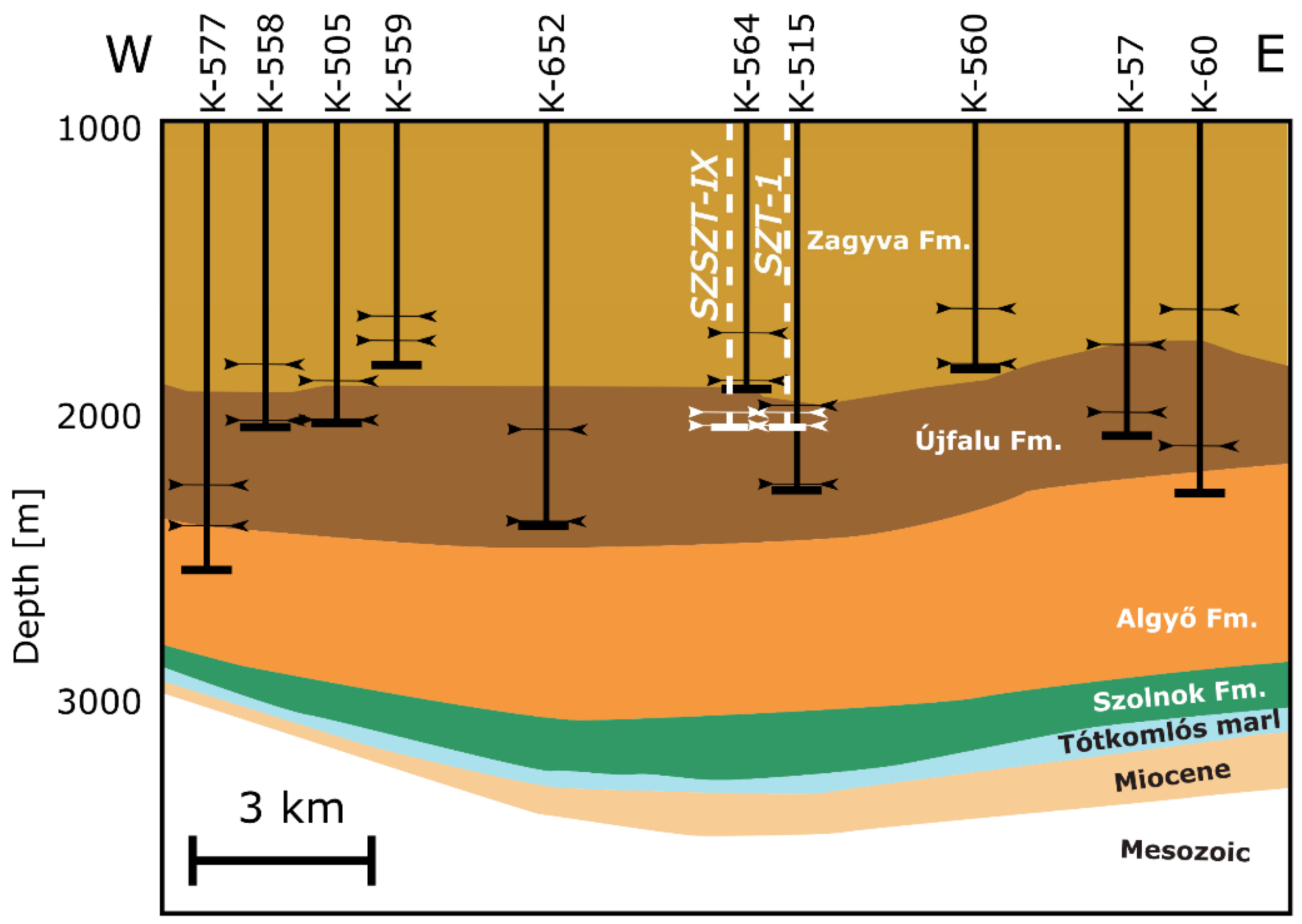
Based on the production history and well-test analysis of the 40 active wells in the Szentes Geothermal Field, three groups of aquifer layers can be identified. Most of the wells have production intervals completed in the Újfalu Formation. A stratigraphic cross-section of the study area, illustrating the wells and their production intervals, is presented in
Figure 2. The upper aquifer layer group, referred to as Level A, consists of wells with production intervals located in the Újfalu and Zagyva Formations, at depths ranging from 1500 to 1800 m, with an average permeability of 1500 millidarcy (mD) [
13,
27]. The middle aquifer layer group, Level B, comprises wells with production intervals between depths of 1800 and 2000 m, primarily in the Újfalu Formation and partially in the Zagyva Formation, exhibiting an average permeability of 500 mD [
13]. The lower aquifer layer group, Level C, comprises wells drilled below 2000 m, all of which are entirely within the Újfalu Formation, with an average permeability ranging from 1000 to 2000 mD. Thermal water production is primarily dominated by wells screened in Level B.
4. Results and Discussion
The geothermal area of Szentes is one of Hungary’s oldest and most intensively produced areas, where long-term thermal water extraction has resulted in a significant pressure drop [
30]. For this reason, it is essential to investigate the possibilities and impacts of reinjecting used thermal water. The mineral composition of the sandstones in the area includes calcite, dolomite, quartz, feldspar (albite and microcline), kaolinite, Ca-montmorillonite, and chlorite. If the cement phase of the sandstone is composed mostly of carbonate minerals (as in the Szolnok Sandstone Formation), an incorrectly chosen acid well treatment or dissolution due to the inflow of heat-depleted water can cause irreversible damage to the unconsolidated sandstone, leading to sand inflow into the well.
4.1. Mineralogical Characterization of the Geothermal Reservoir
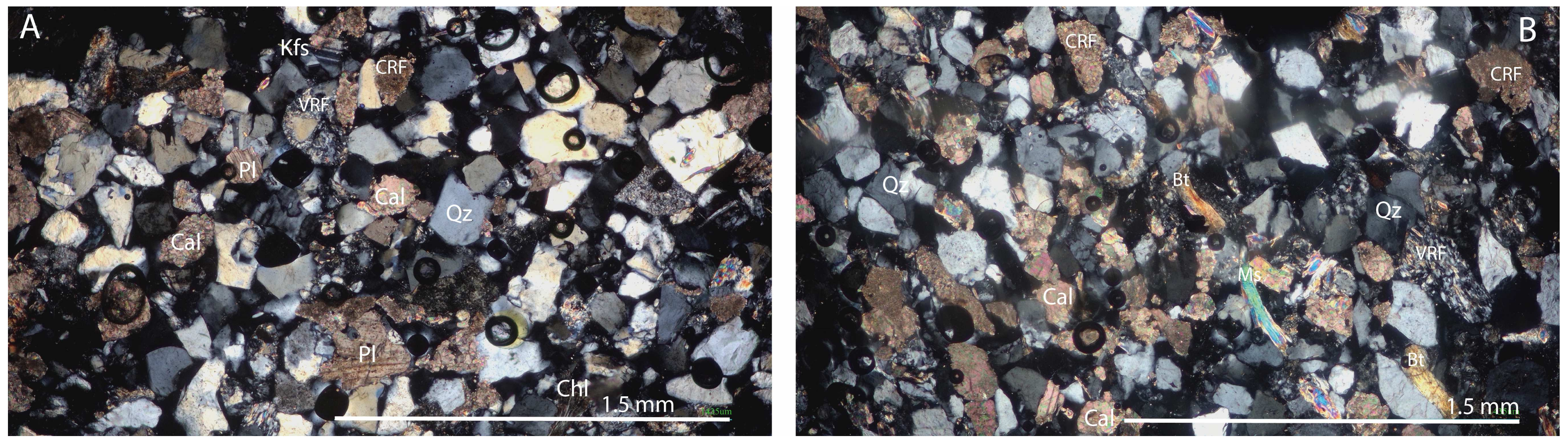
The thin section analysis shows that the gray to light gray sandstones are characterized by well- to very-well-sorted grains (
Figure 3). The grain size ranges from very fine to medium, with a predominantly fine distribution, and the grains are subangular to very angular, exhibiting low sphericity in their shape. The main composition includes quartz, feldspar (both K-feldspar and plagioclase), and mica (muscovite and chloritized biotite), along with carbonates, and minor components like granules, opaque minerals (coalified plant fragments, hematite), as well as accessory minerals such as zircon, apatite, staurolite, and tourmaline (see
Figure 3). The sandstones are primarily poorly cemented. Cement phases are composed of calcite and dolomite carbonates, as well as clay minerals such as sericite, montmorillonite, kaolinite, and illite. Micritic calcite cement is found only in patches and narrow bands.
A weak textural orientation is indicated by the presence of oriented mica plates. The dark gray argillaceous marl and siltstone appear as massive units or as alternating layers of marl and siltstone laminae. The fine-grained marls and siltstones contain a significant amount of mica. The dark coloration is attributed to an increased presence of coaly plant fragments and clay minerals.
4.2. Calorimetry Experiments and Models
The experiments showed the dissolution of the mineral phases present under the experimental conditions, as indicated by elevated Na
+, Si
4+, Ca
2+, K
+, Mg
2+, Fe
2+, Al
3+, and Mn
2+ ions in solutions in the experiment using a model NaHCO
3 solution and elevated Na
+, Cl, and SO
42− ions in the experiments using natural formation water (
Table 2). As expected, the experiment using natural formation water and the bigger grain size fraction (>250 µm) showed more limited reactions than experiments with the <63 µm grain size fraction within the same timeframe, emphasizing the influence of surface area on the reaction rate. Moreover, the calorimetry experiments were conducted at an elevated temperature (120 °C) compared to the formation temperature (~90 °C). Hence, the experimental reaction rates are expected to overestimate reaction rates in the field scale.
The results of the PHREEQC-3 geochemical models (
Figure 4) are consistent with the results of laboratory experiments and are similar in that they show increased ion concentrations in the solution after 3.5 days in the following order, HCO
3− > Na
+ > Si
4+ > K
+ > (Cl
−) > Al
3+ > (SO
42−) > Ca
2+ > Mg
2+ > (Fe
2+), with the ions in parentheses appearing only in the models using natural water. The main difference between the two models using the two databases is that the model using the
Thermoddem database indicates a higher dissolved HCO
3− concentration than the models run with the
PHREEQC database, while for other ions such as Na
+, Ca
2+, and Mg
2+, the
PHREEQC database predicts higher concentrations. The Na
+ ion concentrations predicted by the
PHREEQC database match better with the experimental data, while concentrations of Ca
2+ and Mg
2+ are predicted better by the
Thermoddem database. The two models are consistent in predicting the dissolution of K-feldspar, albite, dolomite, and montmorillonite, as well as the precipitation of calcite and chlorite, and the initial dissolution and subsequent precipitation of quartz in both the model NaHCO
3 solution and natural water experiments. The precipitation of secondary silicate minerals, such as chlorite, can be quite sluggish; therefore, the modeling results should be interpreted with caution, particularly given the short time frame considered in this study. Kaolinite only shows dissolution in models run with the
Thermoddem database, but later, precipitation was observed in models run with the
PHREEQC database. The dissolution of feldspars and the precipitation of secondary minerals, including kaolinite, illite, and quartz, are significant diagenetic processes in arkosic sandstones [
35]. Yuan et al. [
35] conducted laboratory dissolution experiments, analyzed petrologic data, and measured in situ fluid chemistry to investigate feldspar dissolution and the precipitation of secondary minerals in sandstone. By iteratively adjusting their models and comparing the simulated outputs to observed fluid compositions, they demonstrated that effective calibration significantly enhances the reliability of predictive simulations.
Additionally, in models run using natural water composition, the precipitation of goethite and hematite was observed for both datasets. The latter reaction suggests that redox processes, such as oxidation, can also contribute to mineral precipitation in the system. Therefore, it is important to consider the contact of the recycled water with the atmosphere at the surface.
4.3. Modeling Water–Rock Interactions Induced by the Reinjection of Heat-Depleted Water at the Szentes Geothermal Area
The reinjected thermal water is at a lower temperature than the reservoir. The chemical effect of this temperature difference was investigated using an equilibrium and kinetic batch, as well as kinetic 1D reactive transport models at 180 bar pressure and 90 °C and 40 °C. The effect of temperature decrease is estimated by comparing the results obtained at the two different temperatures.
Equilibrium models show a minimal increase in pH (by 1.3–1.4 units) and a decrease in the concentration of several dissolved ions (HCO
3− > Na
+ > Si
4+ > Al
3+ > K
+ > Cl
− > SO
42− > Fe
2+) in both models due to the injection of the lower temperature solution into the reservoir that has been equilibrated with the atmosphere. In the case of Ca
2+ and Mg
2+ ions, the models estimate different directions of change: decreasing concentrations when using the
PHREEQC database and increasing concentrations when using
Thermoddem. The two models are consistent in predicting the dissolution of calcite and precipitation of quartz, kaolinite, and dolomite, but for some mineral phases, the predictions are less clear, e.g., using the
PHREEQC database, in addition to the above-mentioned minerals, chlorite dissolution and minor precipitation of feldspars (albite and K feldspar) and hematite are observed, while using the
Thermoddem database, montmorillonite dissolution and chlorite precipitation are observed. Differences between the models may stem from variations in how mineral chemistry and dissolution reactions are represented across different databases, especially for more complex minerals, even when reaction kinetics are defined separately. For example, the dissolution of montmorillonite is defined as Ca
0.165Al
2.33Si
3.67O
10(OH)
2 + 12H
2O = 0.165Ca
2+ + 2.33Al(OH)
4− + 3.67H
4SiO
4 + 2H
+ in the
PHREEQC database [Ca-montmorillonite] and as Ca
0.17Mg
0.34Al
1.66Si
4O
10(OH)
2 + 6H
+ + 4H
2O = 1.66Al
3+ + 0.170Ca
2+ + 0.340Mg
2+ + 4H
4SiO
4 in the
Thermoddem database [Montmorillonite (MgCa)]. Such slight differences may cause the model to favor certain mineral reactions over others when simulating complex systems. In our case, these mineral reactions, however, occur at rates six to eight orders of magnitude lower than the dissolution of calcite and precipitation of quartz, kaolinite, and dolomite. Thus, we conclude that both databases are in general agreement regarding the direction of the dominant long-term geochemical reactions expected in the reservoir. Minor differences in the extent of quartz and carbonate mineral reactions between the two models lead to opposing predictions of porosity change. The
PHREEQC-based model predicts a porosity decrease of 0.014%, whereas the
Thermoddem-based model predicts a porosity increase of 0.011%. These changes, however, are negligible in comparison to the estimated initial porosity of 20%. It should be noted that equilibrium models for the reinjection of cooled geothermal water in the Szentes Area deviate from those of the laboratory experiments. For example, while calcite precipitation was modeled in the laboratory experiments, the models predict calcite dissolution under real-life conditions. This discrepancy can be attributed to temperature differences between the two scenarios: the laboratory experiments were conducted at higher temperatures (120 °C), promoting calcite precipitation, whereas in the reservoir, cooling from the reinjection of colder water—capable of holding more dissolved CO
2—increases calcite solubility [
36]. Dissolution of even small amounts of calcite cement can positively impact reservoir performance by enhancing permeability, enlarging pore spaces, and improving fluid flow and injectivity. However, it may also pose risks by triggering rock matrix instability and fines migration, which can be particularly significant in poorly consolidated sandstone reservoirs, leading to pore throat blockage and reduced flow capacity.
Equilibrium models provide insights into the long-term behavior of the reservoir; however, geochemical reactions in a reinjection scenario exhibit both spatial and temporal variability. Consequently, it is essential to understand how these reactions may occur in the vicinity of the reinjection well, as they can influence injectivity and reservoir stability. Kinetic models can complement this understanding by capturing localized and short-term reaction dynamics.
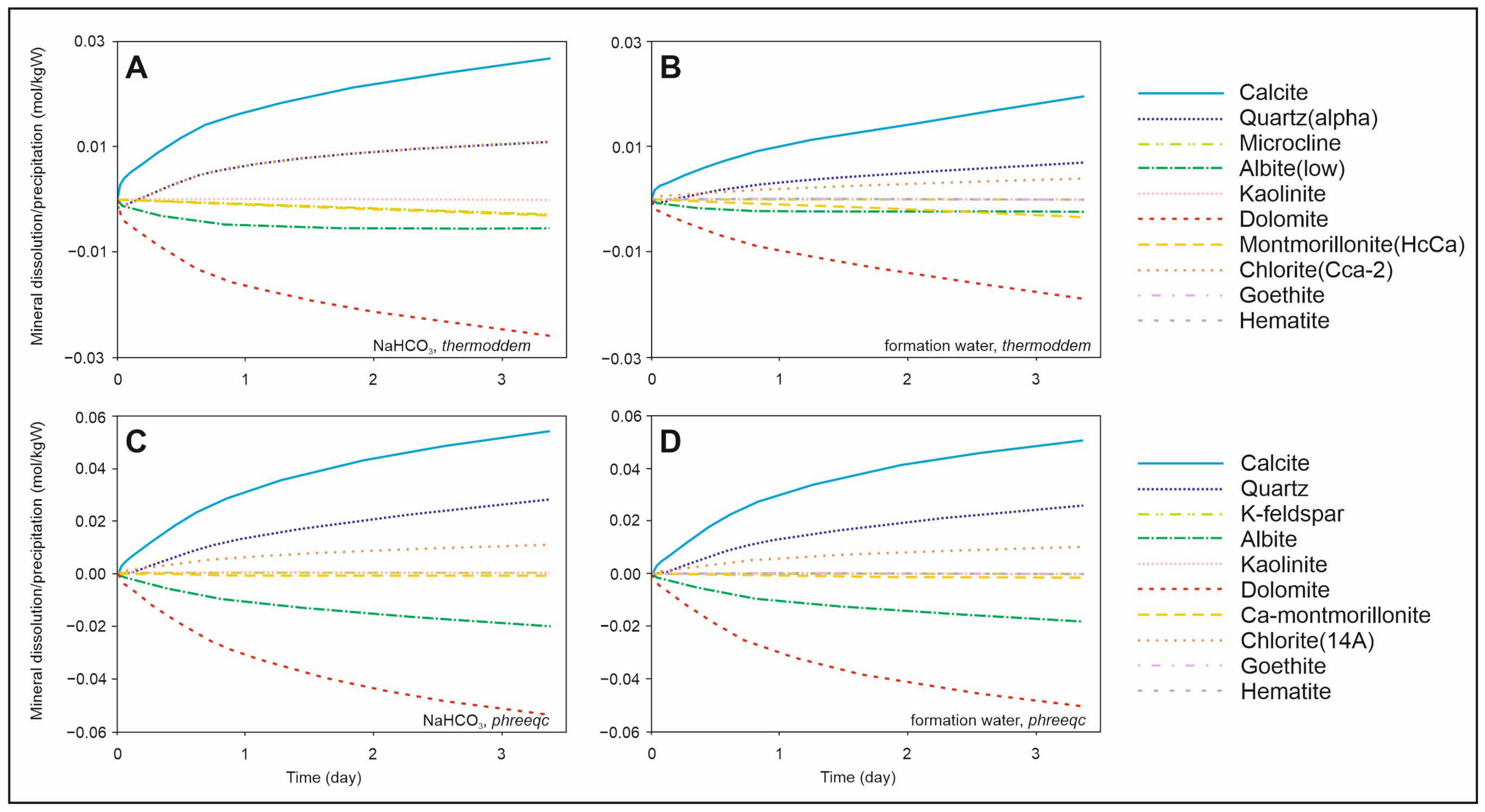
Figure 5 presents the results of kinetic batch models, revealing significant discrepancies between the databases, as detailed below. According to the results obtained using the
Thermoddem database, a decrease in temperature leads to a slight increase in pH, variations in dissolved ion concentrations, and the dissolution of calcite and montmorillonite, while quartz, kaolinite, and dolomite precipitate. These findings are in good agreement with the results of thermodynamic batch models. In contrast, simulations using the
PHREEQC database indicate a continued increase in pH, a predominant decrease in dissolved ion concentrations, and the dissolution of quartz, kaolinite, calcite, and chlorite, accompanied by the simultaneous precipitation of dolomite and montmorillonite.
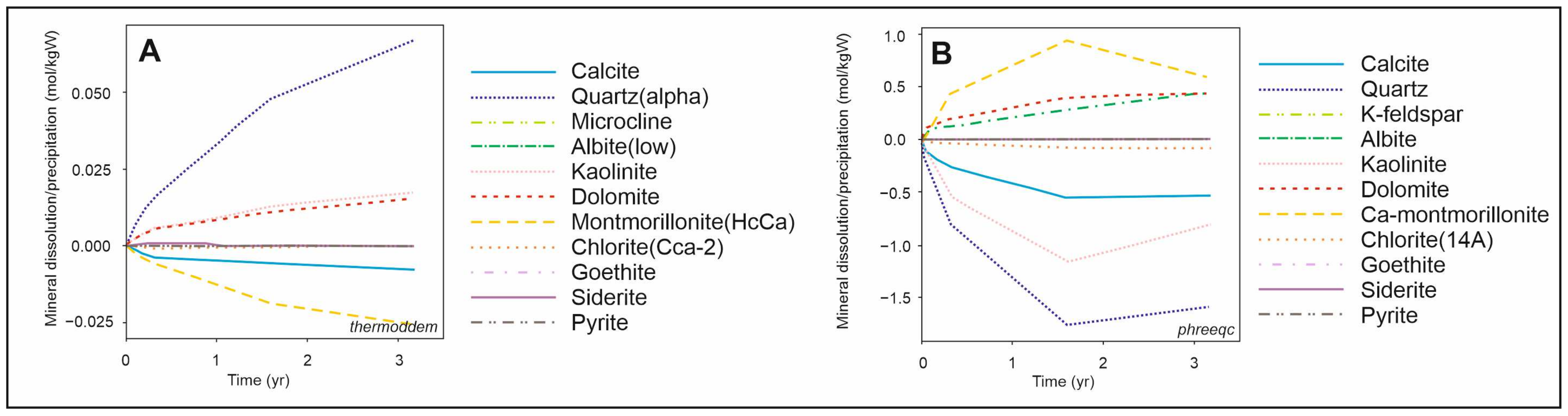
The kinetic 1D reactive transport models (
Figure 6) indicate that montmorillonite and calcite will dissolve in the rock as the temperature decreases, with parallel precipitation of quartz and dolomite using the
Thermoddem database. Models from the
PHREEQC database suggest that the lower temperature in the rock will significantly reduce the intensity of reactions compared to the reference case.
The significant discrepancies between the outcomes of kinetic models employing different thermodynamic databases underscore the need for further investigation. The recent study of Gelencsér et al. [
37] demonstrated that some reactions predicted by geochemical models using unmodified thermodynamic databases may yield unreliable results when compared to laboratory experiments, emphasizing the importance of validating modeling outcomes through experimental work. Strategies to determine which model predictions more closely reflect reality may include the design of additional laboratory experiments featuring the continuous monitoring of ion concentration changes, extended experimental durations, and the simulation of temperature variations. A flow-through reactor setup may be particularly well-suited for such experiments. Furthermore, short-term field injection tests could provide valuable insights into changes in injectivity and permeability. Multidimensional reactive transport models (e.g., with TOUGHREACT) may further refine our understanding of the spatial distribution of mineral–water reactions and changes in porosity and permeability.
4.4. Comparison with Other Sandstone Reservoirs
A detailed analysis conducted by Markó et al. [
6], utilizing hydrogeochemical modeling and laboratory analysis, examined the types of precipitating minerals at the Mezőberény study site in the South-Eastern Great Plain of Hungary. The model predicted the precipitation of goethite and calcite, which was subsequently confirmed using a sample analysis. This confirms the potential for the precipitation of carbonates, iron, and manganese minerals in the area. The primary reason for this precipitation is linked to the fluid composition (higher HCO
3−, Na
+, Ca
2+, and Fe
2+ concentrations), higher reservoir temperature (112 °C), and slight differences in mineralogy (e.g., barite, gypsum) in the case of the Mezőberény wells compared to our data. The formation of predominantly carbonate scaling has been observed at several geothermal sites in the Great Hungarian Plain [
17]. This increased potential for scaling is likely due to fluid–rock interactions in the shallow-water Pannonian aquifers, which contain a significant amount of carbonate grains [
18]. The presence of dissolved calcium and bicarbonate ions contributes to precipitation, particularly during pressure drops that occur during production in the area of Mezőberény. In contrast, at Szentes, calcite dissolution is predicted during the reinjection of cooled thermal water, accompanied by the precipitation of quartz, kaolinite, and dolomite.
Sætre et al. [
12] investigated reactive transport processes in intra-basaltic sandstone reservoirs located on the North Atlantic Margin. They utilized laboratory-derived data and numerical modeling to analyze dissolution–precipitation reactions during fluid injection. They discovered that the evolution of primary minerals and the initial formation water supplied the essential elements needed for the precipitation of secondary minerals. Additionally, changes in aqueous silica concentration indicated that the silica released from the dissolution of plagioclase exceeded the silica consumed by the precipitation of quartz, K-feldspar, and illite. Modeled mineral precipitates (quartz and clay minerals) in the reservoir at Szentes may form through such a process.
5. Conclusions
Geochemical and reactive transport models can provide insight into the potential chemical effects of reinjecting cooled thermal water. However, the primary challenge at present is the significant discrepancy in the results provided by thermodynamic databases, which cannot yet be resolved based on the available information. It is, therefore, important to compare the models with laboratory experiments and field tests. The periodic extraction of recovery wells and long-term monitoring of the chemical composition of the water brought to the surface can help to better understand site-specific responses. It is also important to note that the reaction, largely dissolution, of carbonate minerals may affect the area around the injection wells in the short term. On the one hand, this is advantageous, as it can increase the porosity/permeability of the country rocks near the well. On the other hand, it also poses a risk in case these minerals are present as a cementing phase, as the reaction can significantly weaken the physical properties of the rock and cause fines migration (e.g., clay minerals) in the reservoir, potentially leading to formation damage.
Contrary to popular belief, geochemical models that predict the distribution of species in aqueous fluids do not always yield unique results [
38]. In such scenarios, there can be multiple geochemical systems that adequately meet the conditions established by the modeler. The modeling software may identify any of the potential solutions to the governing equations, depending on the starting point of the iteration process [
39].
In this study, we emphasize the significance of laboratory testing and the interaction between various potential problem sources. In conclusion, this concept enhances risk analysis during the exploration and site-development phases, helping to predict and prevent injectivity issues. We recommend using this approach as part of a checklist for reinjection sites that are experiencing challenges with reinserting water into a sandstone aquifer. A site-specific analysis can further enhance understanding based on local conditions. The most important takeaway is that damage prognosis and the optimal design of water injection strategies are typically empirical and tailored to specific sites. Therefore, it is crucial to conduct carefully designed and implemented field tests and laboratory experiments on formation cores to ensure reliable water injection programs.
Our reactive transport study results indicate that calcite dissolution occurs alongside the precipitation of quartz, kaolinite, and dolomite. However, predictions for some mineral phases are less clear. For instance, using the PHREEQC database, we observe chlorite dissolution and minor precipitation of feldspars (such as albite and K-feldspar) and hematite. In contrast, the Thermoddem database reveals the dissolution of montmorillonite and the precipitation of chlorite. In subsurface environments, silicate mineral reaction rates may be inhibited by the slow formation of secondary silicate phases; therefore, the modeling results should be interpreted with caution, as they may not fully capture these kinetic limitations.
Our models predict and quantify changes in porosity, which affects permeability and injection efficiency. Here are the short- and long-term problems and solutions.
Short term: The dissolution of calcite can enhance permeability, particularly in zones with high calcite content and favorable reservoir conditions. This process enlarges pore spaces and improves fluid flow, leading to better near-wellbore performance and increased productivity or injectivity.
Long term: However, the risk of permeability impairment grows over time if the reservoir water chemistry promotes the re-precipitation of dissolved minerals or if rock matrix instability triggers the migration of fine particles, which can block pore throats and reduce flow capacity.
Solution: To optimize the benefits while minimizing the risks, it is crucial to integrate key reservoir parameters—including porosity, permeability, mineralogical composition, formation water chemistry, and mechanical stability—into a comprehensive, balanced risk–benefit framework that supports effective decision-making and long-term reservoir management.
The PHREEQC geochemical and reactive transport modeling method described in this study offers a multi-step approach to understanding and predicting the chemical effects of reinjecting cooled thermal water. However, a significant challenge currently lies in the discrepancies in results from thermodynamic databases, which cannot be resolved with the available information. Therefore, additional work is necessary to clarify the expected reactions by implementing the following measures:
Conducting further long-term laboratory experiments and sensitivity analyses on thermodynamic databases.
Gathering more information on the pore solution present at depth, including the concentrations of dissolved ions and gases, as well as the chemistry of the cooled thermal water intended for reinjection.
Refining the reactive mineral composition based on petrographic studies, with particular focus on the mineral phases in contact with the pore space.
Future investigations should also include the following:
Assessing dissolved oxygen content and its chemical effects, since cooled thermal water may equilibrate with the atmosphere prior to reinjection.
Estimating porosity and permeability and their spatial inhomogeneity in the reservoir.
Employing multiphase, multidimensional models (e.g., with TOUGHREACT).
Comparing models with experimental data and field tests.