A Reduced-Order Fluid Flow Model for Gas Injection into Porous Media: For Application in Carbon Sequestration in Mine Tailings
Abstract
:1. Introduction
2. Methodology
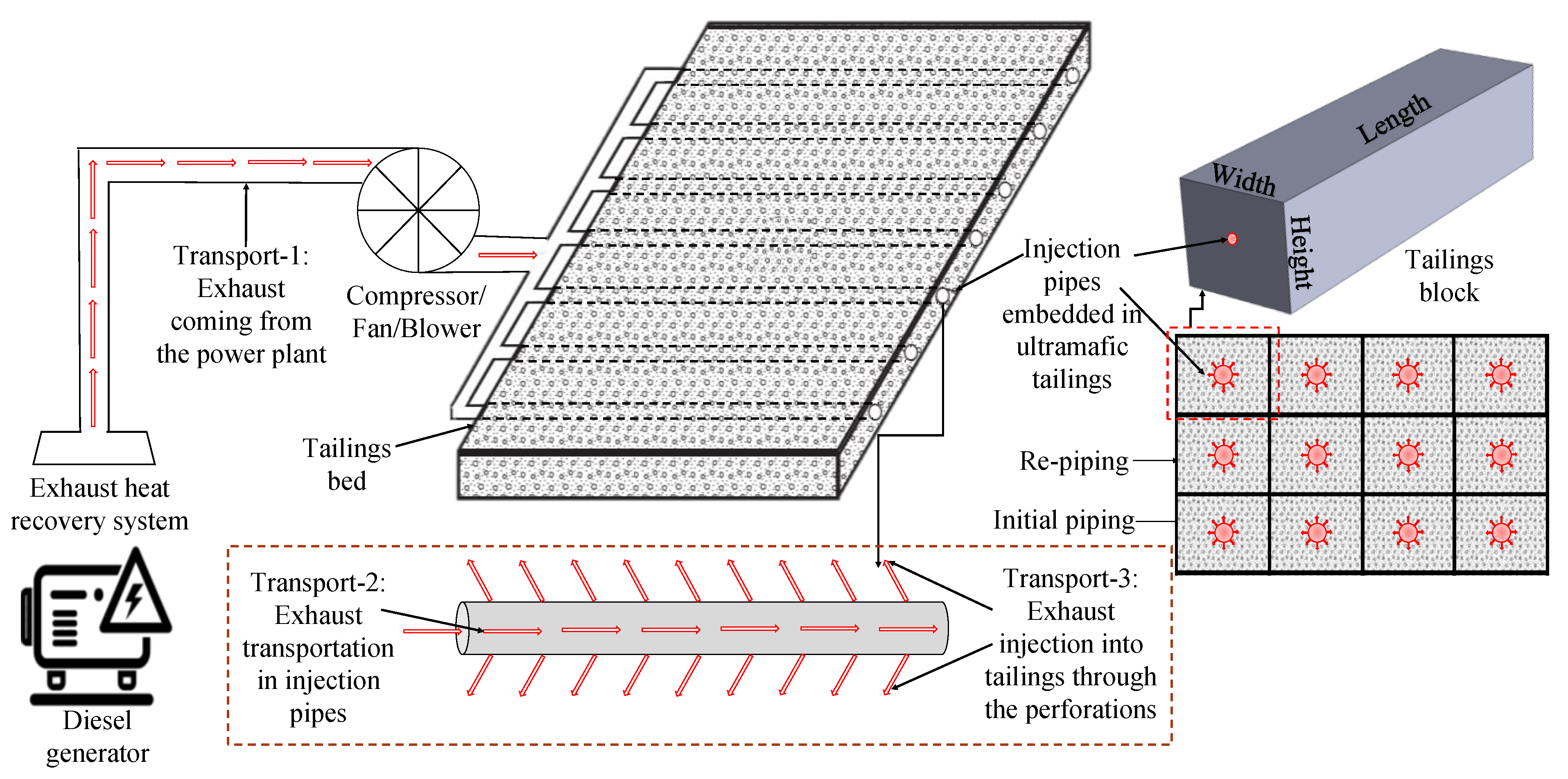
2.1. Conceptual Design
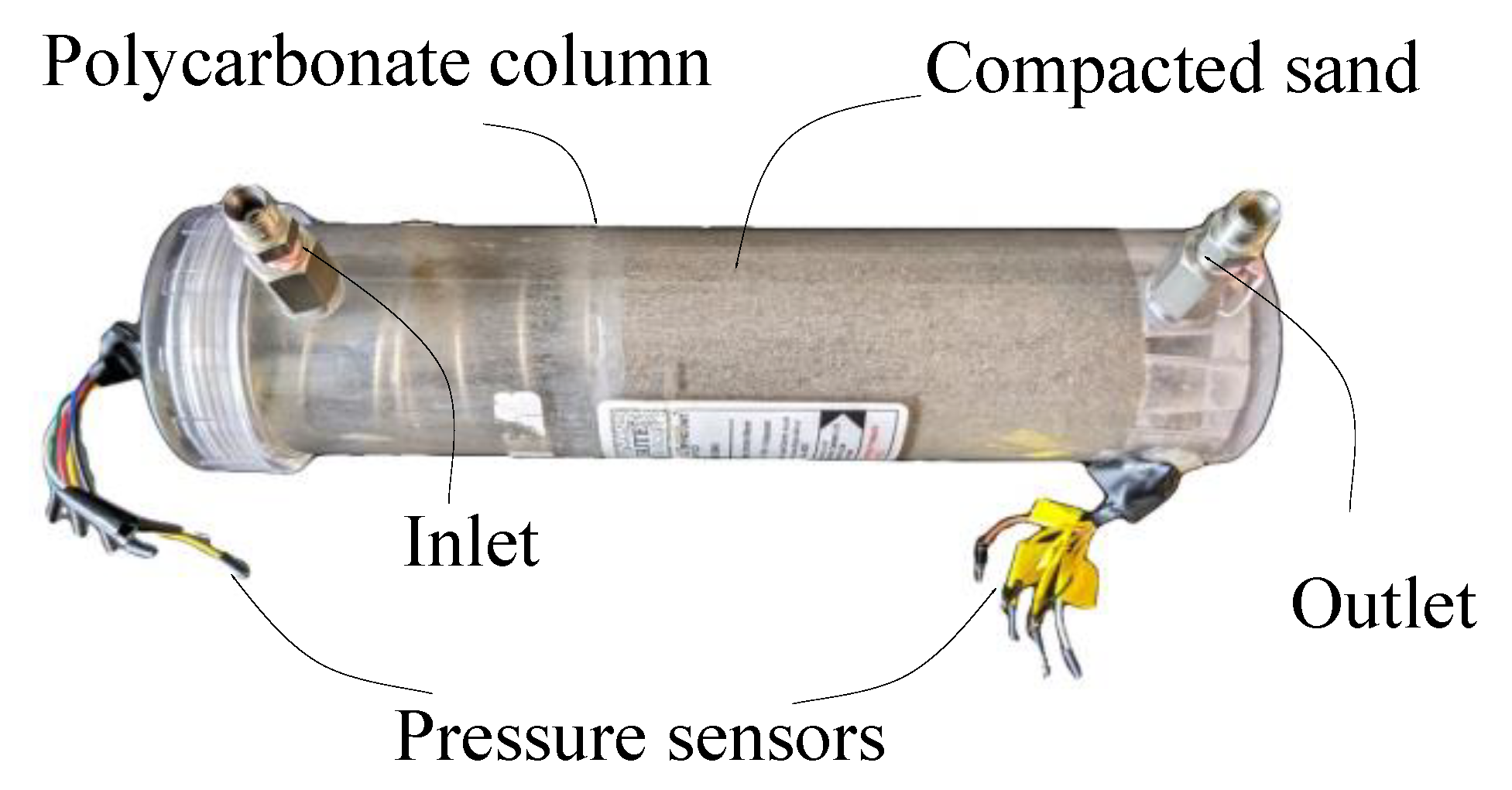
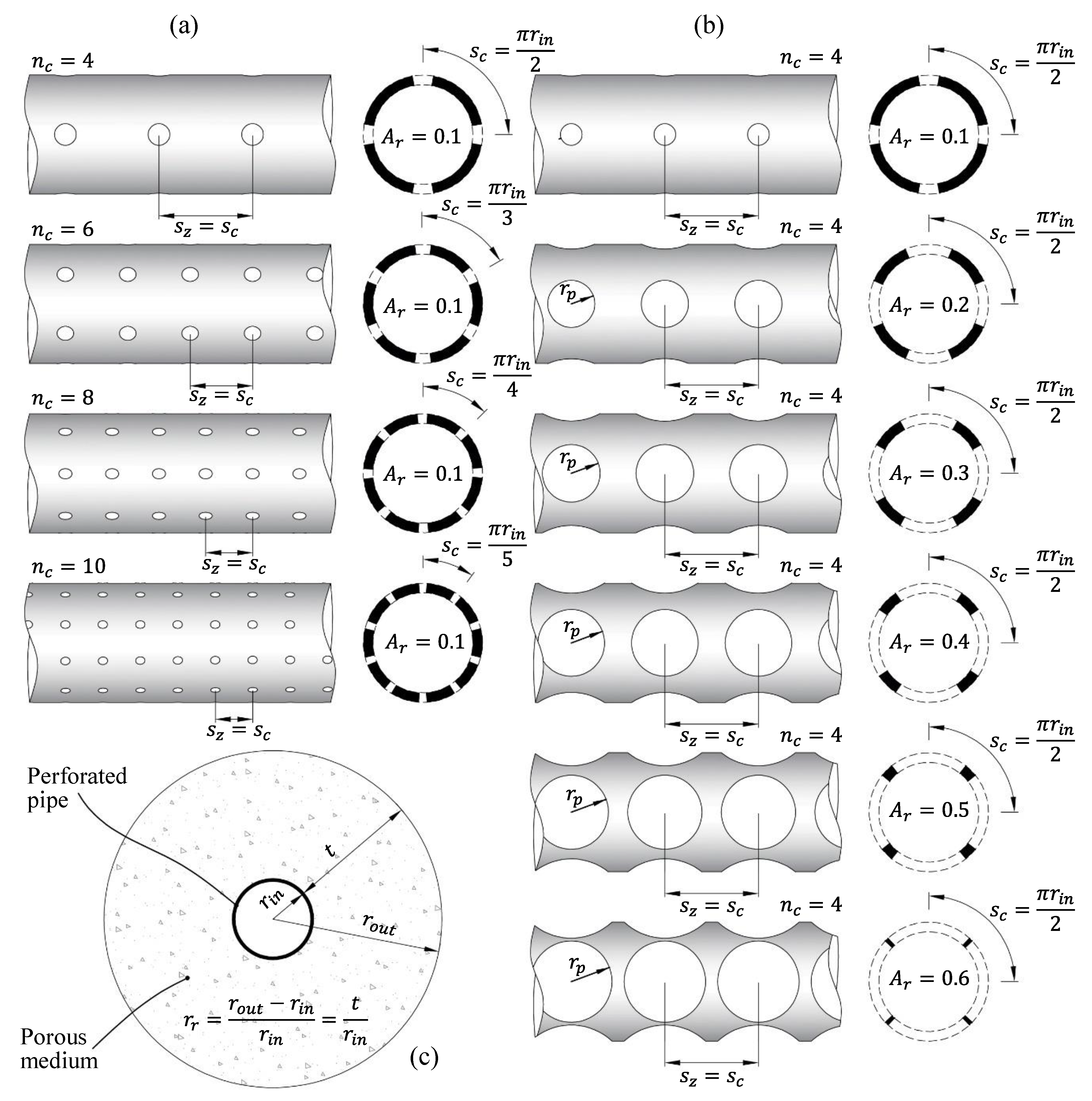
2.2. Perforated Injection Experimental Design
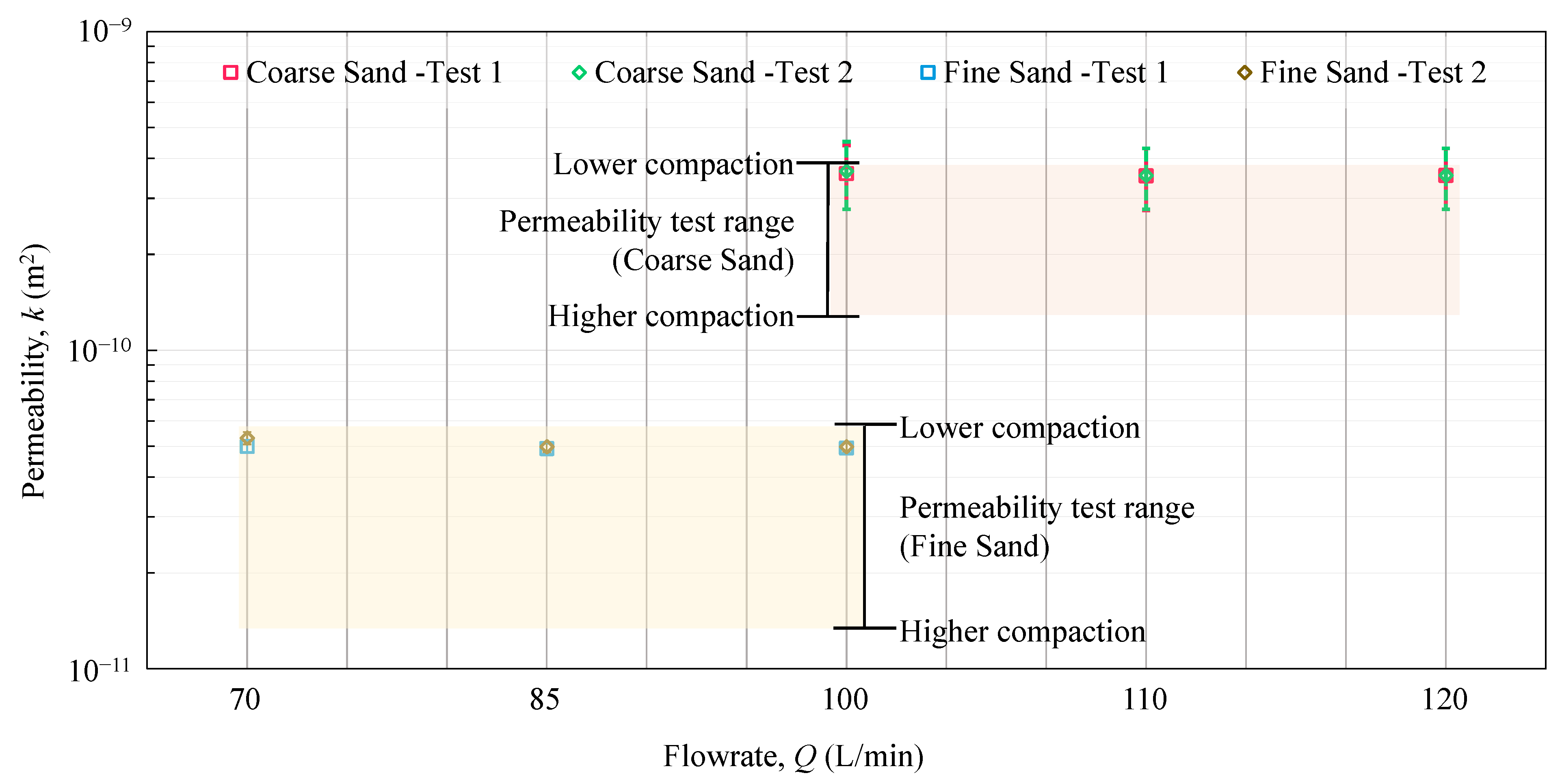
Permeability Measurement
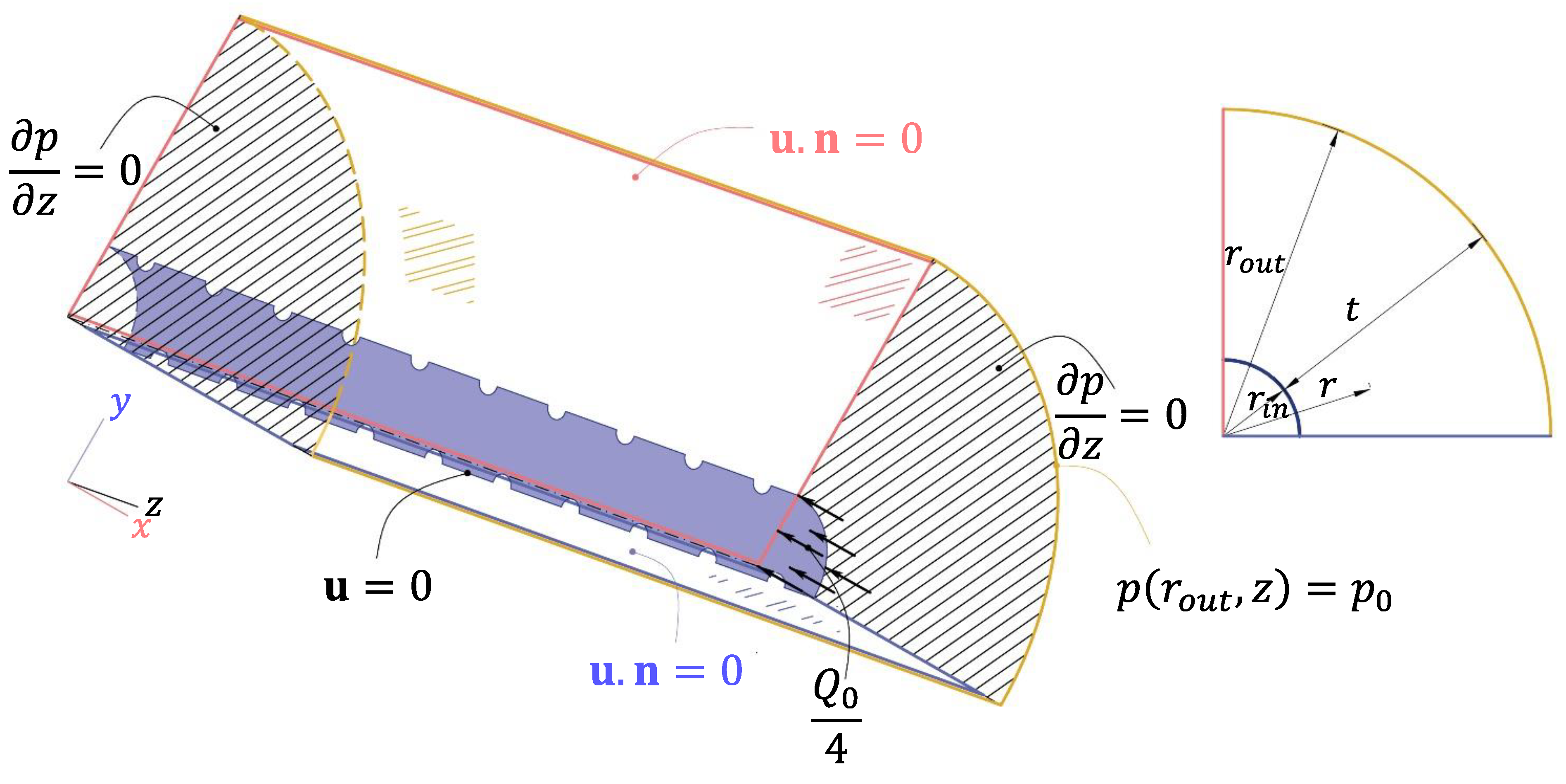
2.3. 3D Model Development
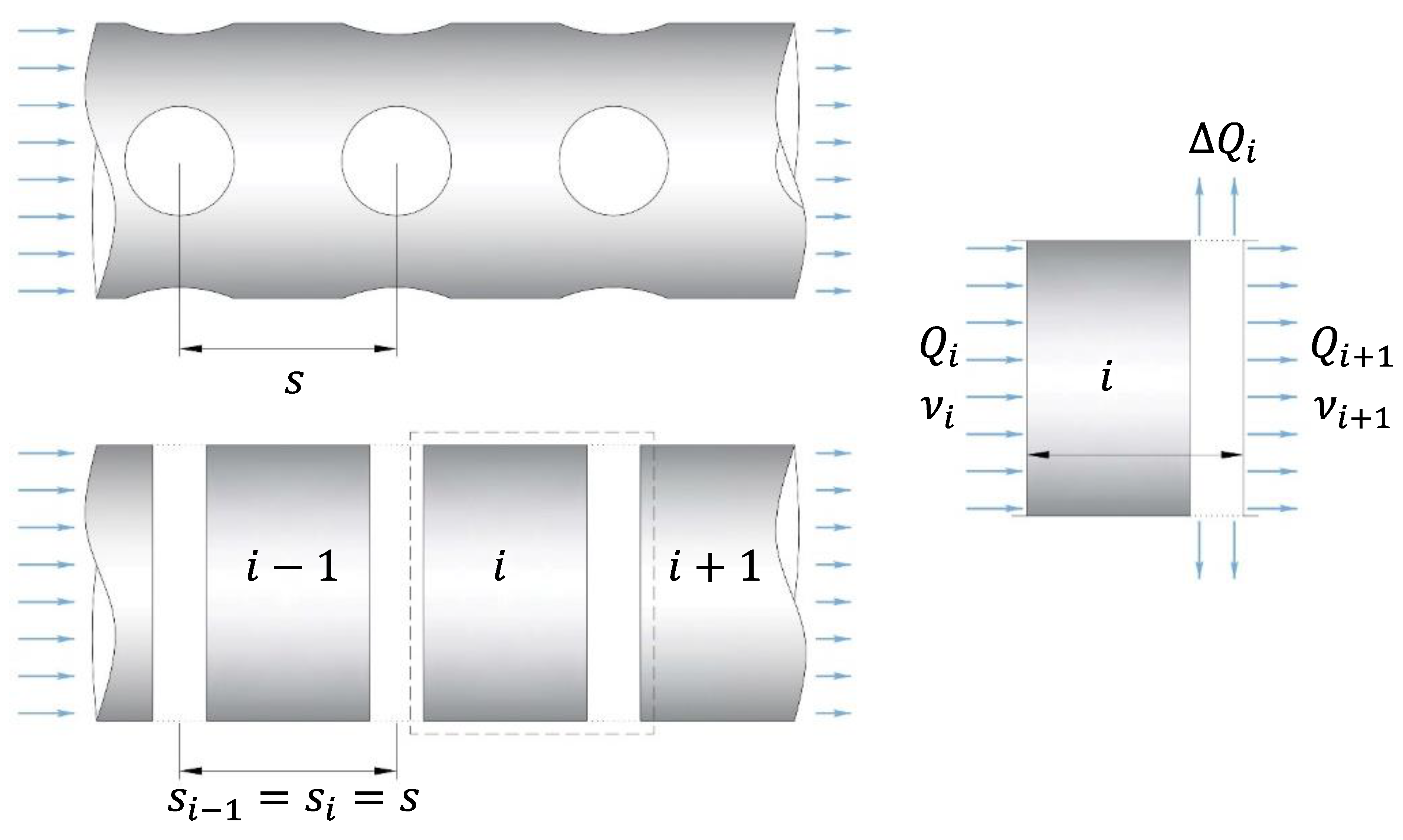
2.4. (1 + 1)D Model Development
3. Results and Discussion
3.1. Validation of the Numerical Models
3.1.1. Experimental Results
3.1.2. Comparison of the Experimental and Numerical Results
3.2. Progression of the (1 + 1)D Reduced-Order Model
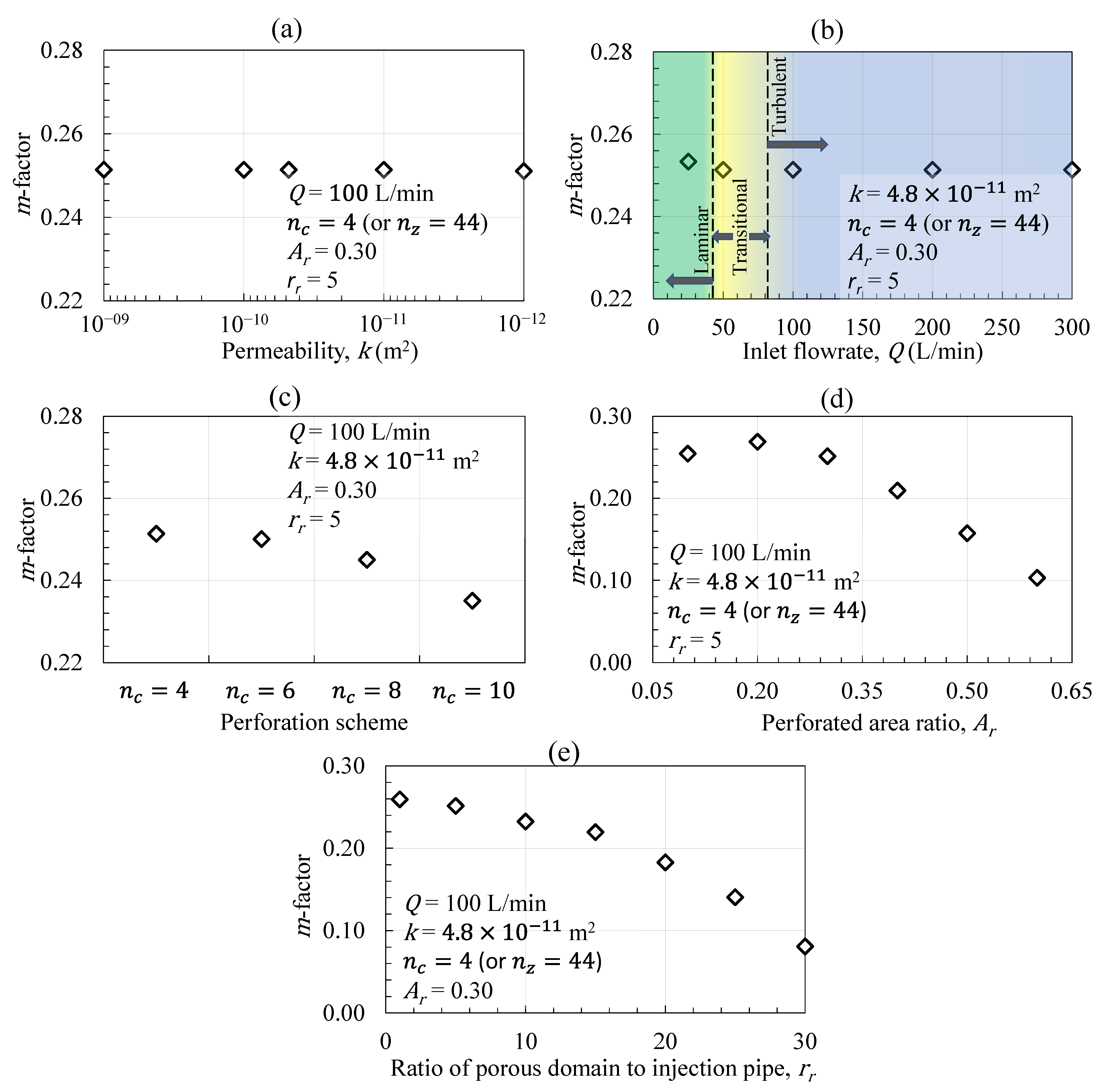
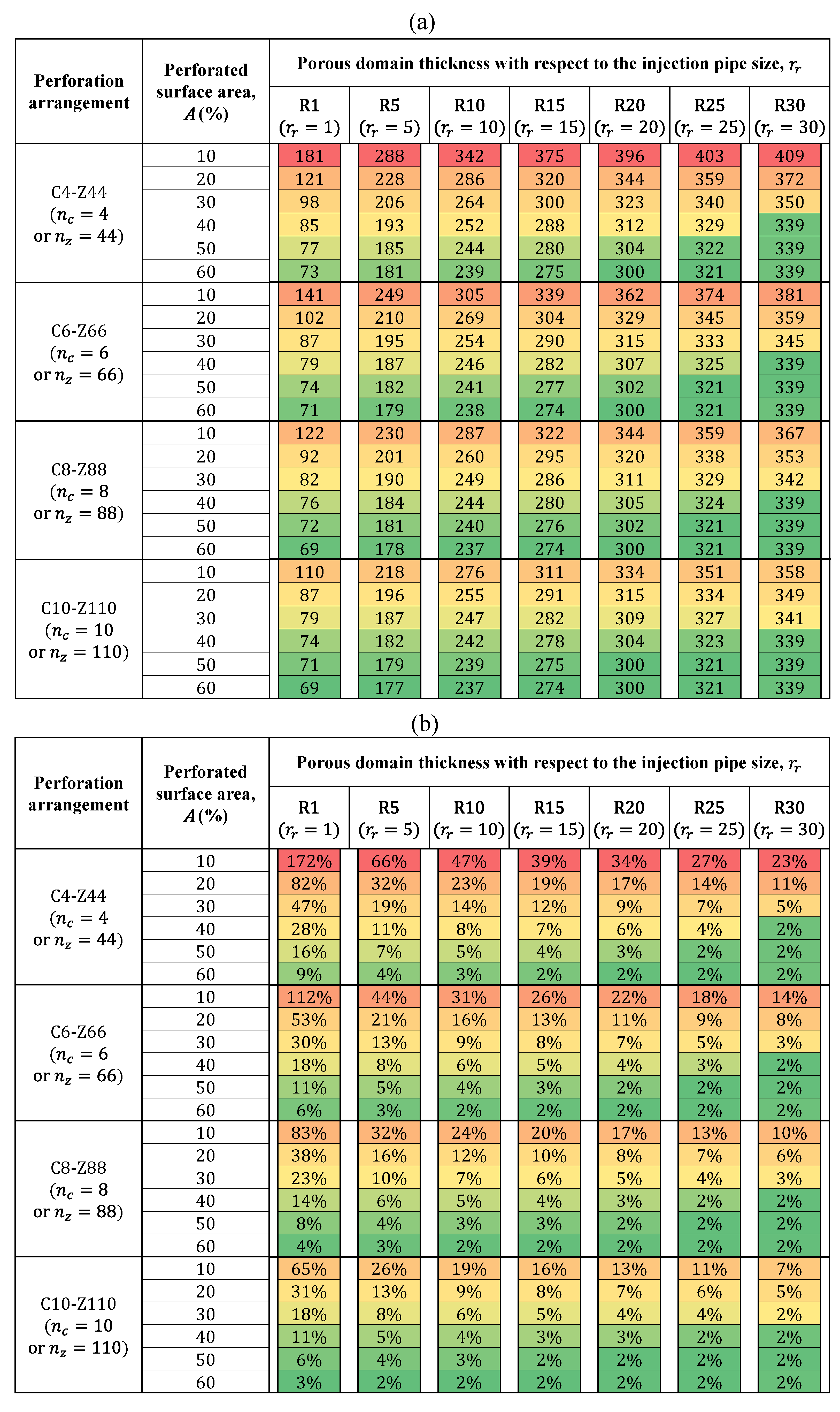
3.2.1. Impact of Design Parameters on the m-Factor
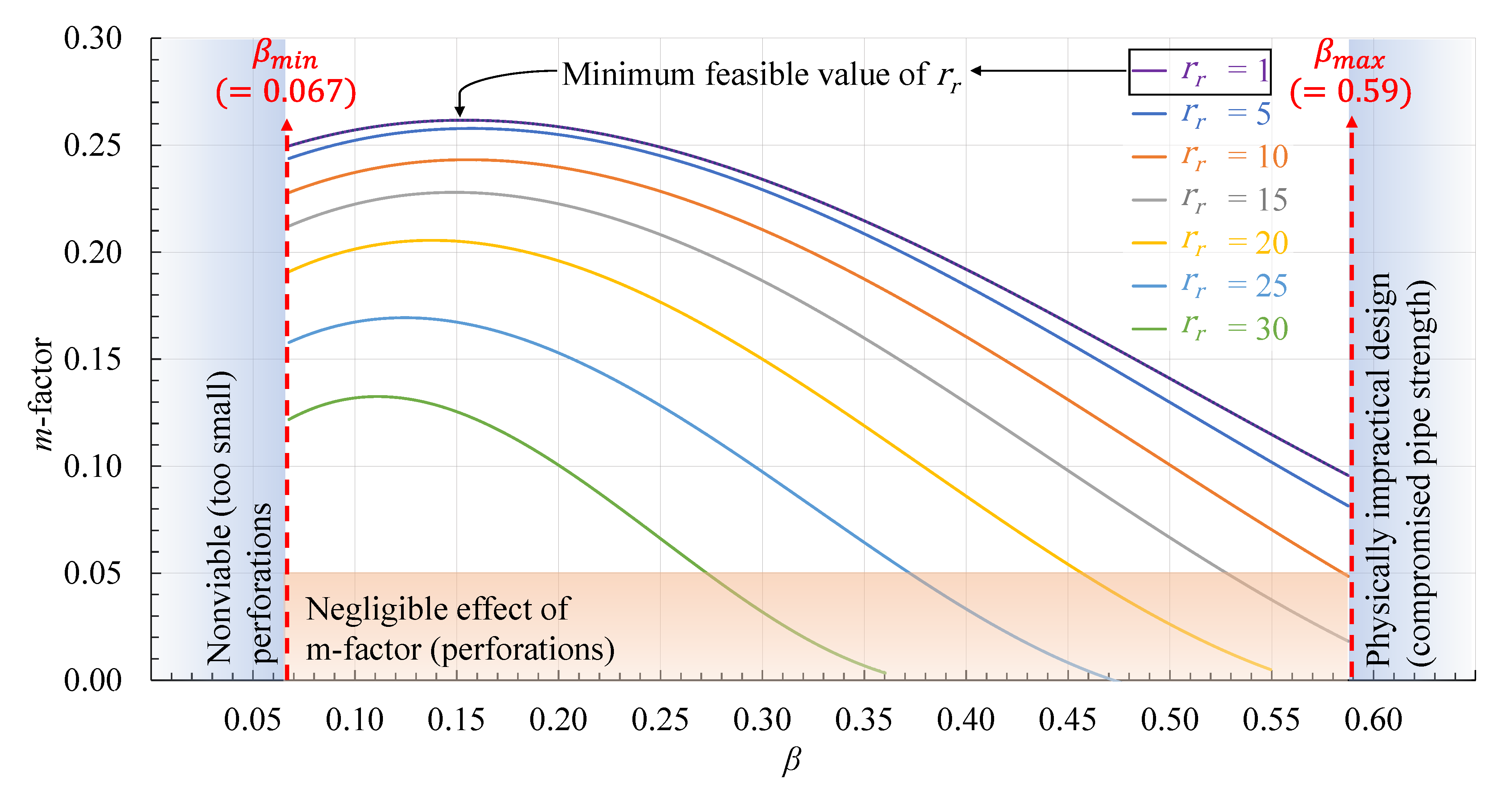
3.2.2. Establishing Dimensionless Correlations between Design Parameters and m-Factor
3.3. Evaluation of the (1 + 1)D Model’s Performance
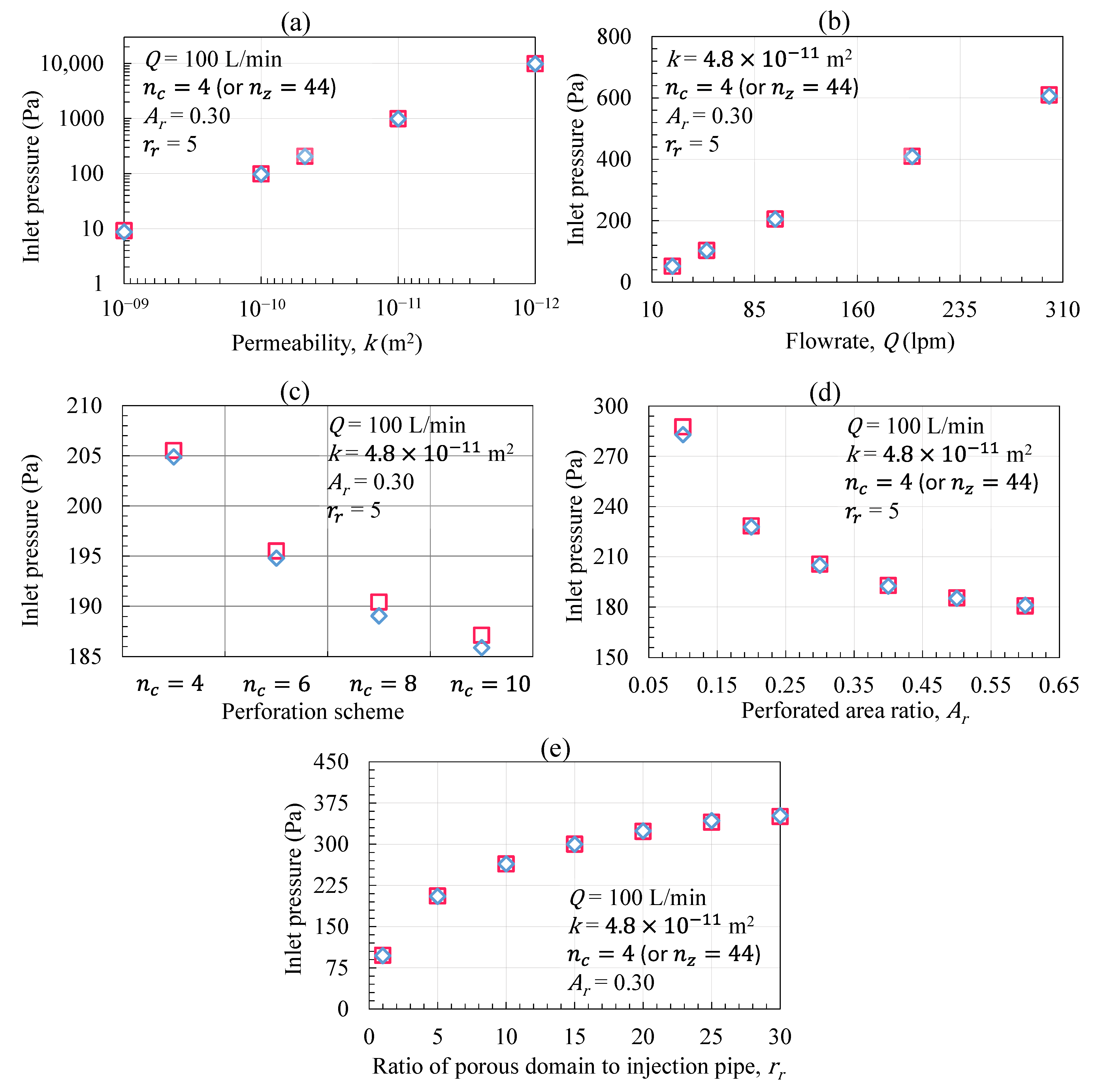
3.3.1. Inlet Pressure
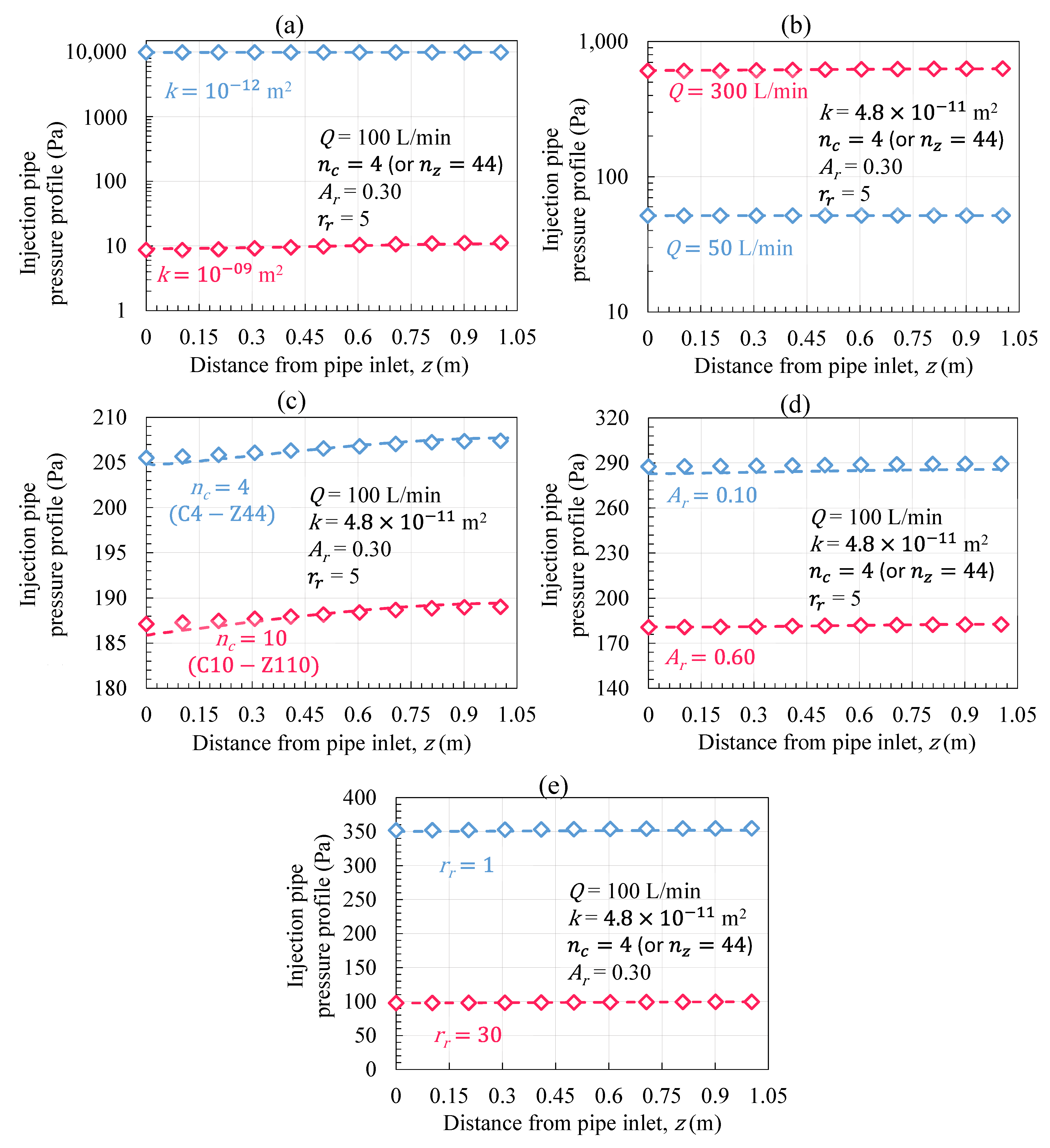
3.3.2. Pressure Profile throughout the Injection Pipe
3.3.3. Outflow Distribution
3.4. Application of the (1 + 1)D to an Optimal Design
4. Conclusions
- The large-scale implementation of CO2 injection into mine tailings for carbon sequestration requires an accurate understanding of the fluid flow to design the operation economically.
- A 3D FE model and a (1 + 1)D ROM were developed in this study to assess injection pressures and gas outflow. An experimental investigation was conducted, and the experimental and analytical results were compared to establish the validity of the models. Upon this validation, the effort was made to develop the (1 + 1)D model as it required significantly less computational resources and time than the 3D FE model.
- A variable called the m-factor was introduced, and its values were calculated for a wide range of feasible operating and design parameters to correlate the impact of the perforation arrangement on the pressure profile.
- The (1 + 1)D model was compared with the 3D FE model and was found to perform consistently well in estimating the behavior of the gauge pressure through the perforated pipe, the pressure at the pipe inlet, and the outflow through the perforations into the surrounding porous tailings.
- Validation of the model with the experimental and FE results established its viability for use in large-scale injection designs. Finally, the developed (1 + 1)D ROM was employed to establish a framework for constructing optimum design cases from an energy and pressure perspective.
- Future work will focus on developing a cost model for optimal design scenarios selected from the (1 + 1)D model results. Optimal designs require the injection pressures and necessary power requirements to be minimized. The authors are also assessing other operating parameters that will impact the practical and economic feasibility of the proposed system.
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| CFD | Computational fluid dynamics |
| CS | Coarse Sand |
| D | Dimensional |
| FE | Finite element |
| FS | Fine Sand |
| IPL | Injection pressure loss |
| PSD | Particle size distribution |
| ROM | Reduced-order model |
| S | Sensor |
| TPL | Transportation pressure loss |
| TSF | Tailings storage facility |
| Area (m2) | |
| Perforated area ratio of the pipe | |
| Forchheimer coefficient | |
| Permeability (m2) | |
| Length of the injection pipe (m) | |
| Distance from pipe inlet (m) | |
| Pressure (Pa) | |
| Ambient pressure (Pa) | |
| Volumetric flow rate (m3/s) | |
| Radius (m) | |
| Reynolds number | |
| Pipe inlet radius (m) | |
| Perforation radius (m) | |
| The ratio of the porous domain thickness to the injection pipe diameter | |
| Pitch (m) | |
| Pipe roughness (m) | |
| Pressure drops or change (Pa) | |
| Change of volumetric flow rate (m3/s) | |
| Density (kg/m3) | |
| Darcy–Weisbach friction coefficient | |
| Dynamic viscosity (Pa·s) | |
| Mid-perforation pressure (Pa) | |
| measured | |
| Error | |
| Gauge | |
| Hydraulic | |
| Index | |
| Recovery | |
| Drop | |
| Circumference | |
| Axial direction | |
| Inlet |
References
- Royer, J. Status of Remote/Off-Grid Communities in Canada; Natural Resources Canada: Ottawa, ON, Canada, 2013.
- Baidya, D.; de Brito, M.A.R.; Sasmito, A.P.; Scoble, M.; Ghoreishi-Madiseh, S.A. Recovering waste heat from diesel generator exhaust; an opportunity for combined heat and power generation in remote Canadian mines. J. Clean. Prod. 2019, 225, 785–805. [Google Scholar] [CrossRef]
- de Brito, M.A.R.; Baidya, D.; Ghoreishi-Madiseh, S.A. Techno-Economic Feasibility Assessment of a Diesel Exhaust Heat Recovery System to Preheat Mine Intake Air in Remote Cold Climate Regions. Int. J. Min. Sci. Technol. 2020, 30, 517–523. [Google Scholar] [CrossRef]
- Ruddiman, W.F. The Anthropogenic Greenhouse Era Began Thousands of Years Ago. Clim. Chang. 2003, 61, 261–293. [Google Scholar] [CrossRef]
- Giammar, D.E.; Bruant, R.G.; Peters, C.A. Forsterite dissolution and magnesite precipitation at conditions relevant for deep saline aquifer storage and sequestration of carbon dioxide. Chem. Geol. 2005, 217, 257–276. [Google Scholar] [CrossRef]
- Larachi, F.; Daldoul, I.; Beaudoin, G. Fixation of CO2 by chrysotile in low-pressure dry and moist carbonation: Ex-situ and in-situ characterizations. Geochim. Cosmochim. Acta 2010, 74, 3051–3075. [Google Scholar] [CrossRef]
- O’connor, W.K.; Dahlin, D.C.; Rush, G.E.; Dahlin, C.L.; Collins, W.K. Carbon dioxide sequestration by direct mineral carbonation: Process mineralogy of feed and products. Min. Met. Explor. 2002, 19, 95–101. [Google Scholar] [CrossRef] [Green Version]
- Oelkers, E.H.; Gislason, S.R.; Matter, J. Mineral Carbonation of CO2. Elements 2008, 4, 333–337. [Google Scholar] [CrossRef]
- Assima, G.P.; Larachi, F.; Beaudoin, G.; Molson, J. Dynamics of carbon dioxide uptake in chrysotile mining residues—Effect of mineralogy and liquid saturation. Int. J. Greenh. Gas Control 2013, 12, 124–135. [Google Scholar] [CrossRef]
- Wilson, S.; Dipple, G.M.; Power, I.; Thom, J.M.; Anderson, R.G.; Raudsepp, M.; Gabites, J.E.; Southam, G. Carbon Dioxide Fixation within Mine Wastes of Ultramafic-Hosted Ore Deposits: Examples from the Clinton Creek and Cassiar Chrysotile Deposits, Canada. Econ. Geol. 2009, 104, 95–112. [Google Scholar] [CrossRef]
- Wilson, S.A.; Raudsepp, M.; Dipple, G.M. Verifying and quantifying carbon fixation in minerals from serpentine-rich mine tailings using the Rietveld method with X-ray powder diffraction data. Am. Miner. 2006, 91, 1331–1341. [Google Scholar] [CrossRef]
- Mervine, E.M.; Wilson, S.; Power, I.M.; Dipple, G.M.; Turvey, C.C.; Hamilton, J.; Vanderzee, S.; Raudsepp, M.; Southam, C.; Matter, J.M.; et al. Potential for offsetting diamond mine carbon emissions through mineral carbonation of processed kimberlite: An assessment of De Beers mine sites in South Africa and Canada. Miner. Pet. 2018, 112, 755–765. [Google Scholar] [CrossRef]
- Power, I.M.; McCutcheon, J.; Harrison, A.L.; Wilson, S.A.; Dipple, G.M.; Kelly, S.; Southam, C.; Southam, G. Strategizing Carbon-Neutral Mines: A Case for Pilot Projects. Minerals 2014, 4, 399–436. [Google Scholar] [CrossRef] [Green Version]
- Power, I.M.; Wilson, S.A.; Dipple, G.M. Serpentinite Carbonation for CO2 Sequestration. Elements 2013, 9, 115–121. [Google Scholar] [CrossRef]
- Woodall, C.M.; Lu, X.; Dipple, G.; Wilcox, J. Carbon Mineralization with North American PGM Mine Tailings—Characterization and Reactivity Analysis. Minerals 2021, 11, 844. [Google Scholar] [CrossRef]
- Wilson, S.A.; Barker, S.L.L.; Dipple, G.M.; Atudorei, V. Isotopic Disequilibrium during Uptake of Atmospheric CO2 into Mine Process Waters: Implications for CO2 Sequestration. Environ. Sci. Technol. 2010, 44, 9522–9529. [Google Scholar] [CrossRef] [PubMed]
- Wilson, S.A.; Dipple, G.M.; Power, I.M.; Barker, S.L.L.; Fallon, S.J.; Southam, G. Subarctic Weathering of Mineral Wastes Provides a Sink for Atmospheric CO2. Environ. Sci. Technol. 2011, 45, 7727–7736. [Google Scholar] [CrossRef]
- Harrison, A.L.; Power, I.M.; Dipple, G.M. Accelerated Carbonation of Brucite in Mine Tailings for Carbon Sequestration. Environ. Sci. Technol. 2013, 47, 126–134. [Google Scholar] [CrossRef]
- Assima, G.P.; Larachi, F.; Molson, J.; Beaudoin, G. New tools for stimulating dissolution and carbonation of ultramafic mining residues. Can. J. Chem. Eng. 2014, 92, 2029–2038. [Google Scholar] [CrossRef]
- Harrison, A.L.; Power, I.M.; Dipple, G.M.; Mayer, K.U.; Wilson, S.A. Controls on Carbon Mineralization in Ultramafic Mine Tailings. In Proceedings of the American Geophysical Union Fall Meeting, San Francisco, CA, USA, 9 December 2013. [Google Scholar]
- Kelemen, P.B.; McQueen, N.; Wilcox, J.; Renforth, P.; Dipple, G.; Vankeuren, A.P. Engineered carbon mineralization in ultramafic rocks for CO2 removal from air: Review and new insights. Chem. Geol. 2020, 550, 119628. [Google Scholar] [CrossRef]
- Harrison, A.L.; Dipple, G.M.; Power, I.M.; Ulrich Mayer, K. Influence of surface passivation and water content on mineral reactions in unsaturated porous media: Implications for brucite carbonation and CO2 sequestration. Geochim. Cosmochim. Acta 2015, 148, 477–495. [Google Scholar] [CrossRef]
- Harrison, A.L.; Dipple, G.M.; Power, I.M.; Mayer, K.U. The Impact of Evolving Mineral–Water–Gas Interfacial Areas on Mineral–Fluid Reaction Rates in Unsaturated Porous Media. Chem. Geol. 2016, 421, 65–80. [Google Scholar] [CrossRef]
- Wynands, E. Carbon Mineralization in Ultramafic Mine Tailings Via CO2 Injection. Master’s Thesis, University of British Columbia, Vancouver, BC, Canada, 2021. [Google Scholar]
- Azadi, M.; Aminossadati, S.M.; Chen, Z. Development of an integrated reservoir-wellbore model to examine the hydrodynamic behaviour of perforated pipes. J. Pet. Sci. Eng. 2017, 156, 269–281. [Google Scholar] [CrossRef] [Green Version]
- Oyarce, P.; Gurovich, L.; Duarte, V. Experimental Evaluation of Agricultural Drains. J. Irrig. Drain. Eng. 2016, 143, 1–13. [Google Scholar] [CrossRef]
- Wang, J. Theory of flow distribution in manifolds. Chem. Eng. J. 2011, 168, 1331–1345. [Google Scholar] [CrossRef]
- Ravikumar, V.; Ranganathan, C.R.; Bosu, S.S. Analytical Equation for Variation of Discharge in Drip Irrigation Laterals. J. Irrig. Drain. Eng. 2003, 129, 295–298. [Google Scholar] [CrossRef]
- Liu, H.; Zong, Q.; Lv, H.; Jin, J. Analytical equation for outflow along the flow in a perforated fluid distribution pipe. PLoS ONE 2017, 12, e0185842. [Google Scholar] [CrossRef] [Green Version]
- Acrivos, A.; Babcock, B.; Pigford, R. Flow distributions in manifolds. Chem. Eng. Sci. 1959, 10, 112–124. [Google Scholar] [CrossRef]
- Greskovich, E.J.; O’Bara, J.T. Perforated-Pipe Distributors. Ind. Eng. Chem. Proc. Des. Dev. 1968, 7, 593–595. [Google Scholar] [CrossRef]
- Kulkarni, A.V.; Roy, S.S.; Joshi, J.B. Pressure and Flow Distribution in Pipe and Ring Spargers: Experimental Measurements and Cfd Simulation—Sciencedirect. Chem. Eng. J. 2007, 133, 173–186. [Google Scholar] [CrossRef]
- Lu, G.-M.; Li, Y.-H.; Hassani, F.; Zhang, X. The influence of microwave irradiation on thermal properties of main rock-forming minerals. Appl. Therm. Eng. 2017, 112, 1523–1532. [Google Scholar] [CrossRef]
- Vallesquino, P.; Luque-Escamilla, P.L. New Algorithm for Hydraulic Calculation in Irrigation Laterals. J. Irrig. Drain. Eng. 2001, 127, 254–260. [Google Scholar] [CrossRef]
- Maynes, D.; Holt, G.J.; Blotter, J. Cavitation Inception and Head Loss Due to Liquid Flow Through Perforated Plates of Varying Thickness. J. Fluids Eng. 2013, 135, 031302. [Google Scholar] [CrossRef]
- Kapadia, S.; Anderson, W. Sensitivity analysis for solid oxide fuel cells using a three-dimensional numerical model. J. Power Sources 2009, 189, 1074–1082. [Google Scholar] [CrossRef]
- Afrin, T.; Kaye, N.B.; Khan, A.A.; Testik, F.Y. Parametric Study of Perforated Pipe Underdrains Surrounded by Loose Aggregate. J. Hydraul. Eng. 2016, 142, 04016066. [Google Scholar] [CrossRef]
- Afrin, T.; Khan, A.A.; Kaye, N.B.; Testik, F.Y. Numerical Model for the Hydraulic Performance of Perforated Pipe Underdrains Surrounded by Loose Aggregate. J. Hydraul. Eng. 2016, 142, 04016018. [Google Scholar] [CrossRef]
- Minocha, N.; Joshi, J.B. 3D CFD simulation of turbulent flow distribution and pressure drop in a dividing manifold system using openfoam. Int. J. Heat Mass Transf. 2020, 151, 119420. [Google Scholar] [CrossRef]
- Braun, J.; Sousa, J.; Paniagua, G. Numerical Assessment of the Convective Heat Transfer in Rotating Detonation Combustors Using a Reduced-Order Model. Appl. Sci. 2018, 8, 893. [Google Scholar] [CrossRef] [Green Version]
- Amiri, L.; De Brito, M.A.R.; Baidya, D.; Kuyuk, A.F.; Ghoreishi-Madiseh, S.A.; Sasmito, A.P.; Hassani, F.P. Numerical investigation of rock-pile based waste heat storage for remote communities in cold climates. Appl. Energy 2019, 252, 113475. [Google Scholar] [CrossRef]
- Baidya, D.; de Brito, M.A.R.; Ghoreishi-Madiseh, S.A. Techno-economic feasibility investigation of incorporating an energy storage with an exhaust heat recovery system for underground mines in cold climatic regions. Appl. Energy 2020, 273, 115289. [Google Scholar] [CrossRef]
- Baidya, D.; de Brito, M.A.R.; Sasmito, A.P.; Ghoreishi-Madiseh, S.A. Diesel generator exhaust heat recovery fully-coupled with intake air heating for off-grid mining operations: An experimental, numerical, and analytical evaluation. Int. J. Min. Sci. Technol. 2021, 32, 155–169. [Google Scholar] [CrossRef]
- Robert, H.R. Perry’s Chemical Engineering Handbook, 8th ed.; Mcgraw-Hill: New York, NY, USA, 2007. [Google Scholar]
- Zhong, W.; Xu, K.; Li, X.; Liao, Y.; Tao, G.; Kagawa, T. Determination of pressure drop for air flow through sintered metal porous media using a modified Ergun equation. Adv. Powder Technol. 2016, 27, 1134–1140. [Google Scholar] [CrossRef]
- Kaviany, M. Principles of Heat Transfer in Porous Media; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2012. [Google Scholar]
- Nield, D.A.; Bejan, A. Convection in Porous Media; Springer: New York, NY, USA, 2006; Volume 3. [Google Scholar]
- Ho, C.K.; Webb, S.W. Gas Transport in Porous Media; Springer: New York, NY, USA, 2006; Volume 20. [Google Scholar]
- Brown, G.O. The History of the Darcy-Weisbach Equation for Pipe Flow Resistance. In Environmental and Water Resources History; American Society of Civil Engineers: Reston, VA, USA, 2002; pp. 34–43. [Google Scholar] [CrossRef] [Green Version]
- Economides, M.J.; Hill, A.D. Christine Ehlig-Economides Ding Zhu. In Petroleum Production Systems, 2nd ed.; Pearson Education: Westford, MA, USA, 2013; ISBN -13: 978-0-13-703158-0. [Google Scholar]
- Wang, J.; Gao, Z.; Gan, G.; Wu, D. Analytical solution of flow coefficients for a uniformly distributed porous channel. Chem. Eng. J. 2001, 84, 1–6. [Google Scholar] [CrossRef]




Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Baidya, D.; Wynands, E.; Samea, P.; Ghoreishi-Madiseh, S.A.; Dipple, G. A Reduced-Order Fluid Flow Model for Gas Injection into Porous Media: For Application in Carbon Sequestration in Mine Tailings. Minerals 2023, 13, 855. https://doi.org/10.3390/min13070855
Baidya D, Wynands E, Samea P, Ghoreishi-Madiseh SA, Dipple G. A Reduced-Order Fluid Flow Model for Gas Injection into Porous Media: For Application in Carbon Sequestration in Mine Tailings. Minerals. 2023; 13(7):855. https://doi.org/10.3390/min13070855
Chicago/Turabian StyleBaidya, Durjoy, Eric Wynands, Parham Samea, Seyed Ali Ghoreishi-Madiseh, and Gregory Dipple. 2023. "A Reduced-Order Fluid Flow Model for Gas Injection into Porous Media: For Application in Carbon Sequestration in Mine Tailings" Minerals 13, no. 7: 855. https://doi.org/10.3390/min13070855
APA StyleBaidya, D., Wynands, E., Samea, P., Ghoreishi-Madiseh, S. A., & Dipple, G. (2023). A Reduced-Order Fluid Flow Model for Gas Injection into Porous Media: For Application in Carbon Sequestration in Mine Tailings. Minerals, 13(7), 855. https://doi.org/10.3390/min13070855






