Modeling of Sand Triaxial Specimens under Compression: Introducing an Elasto-Plastic Finite Element Model to Capture the Impact of Specimens’ Heterogeneity
Abstract
1. Introduction
2. Application of 3D-DIC on Triaxial Tests
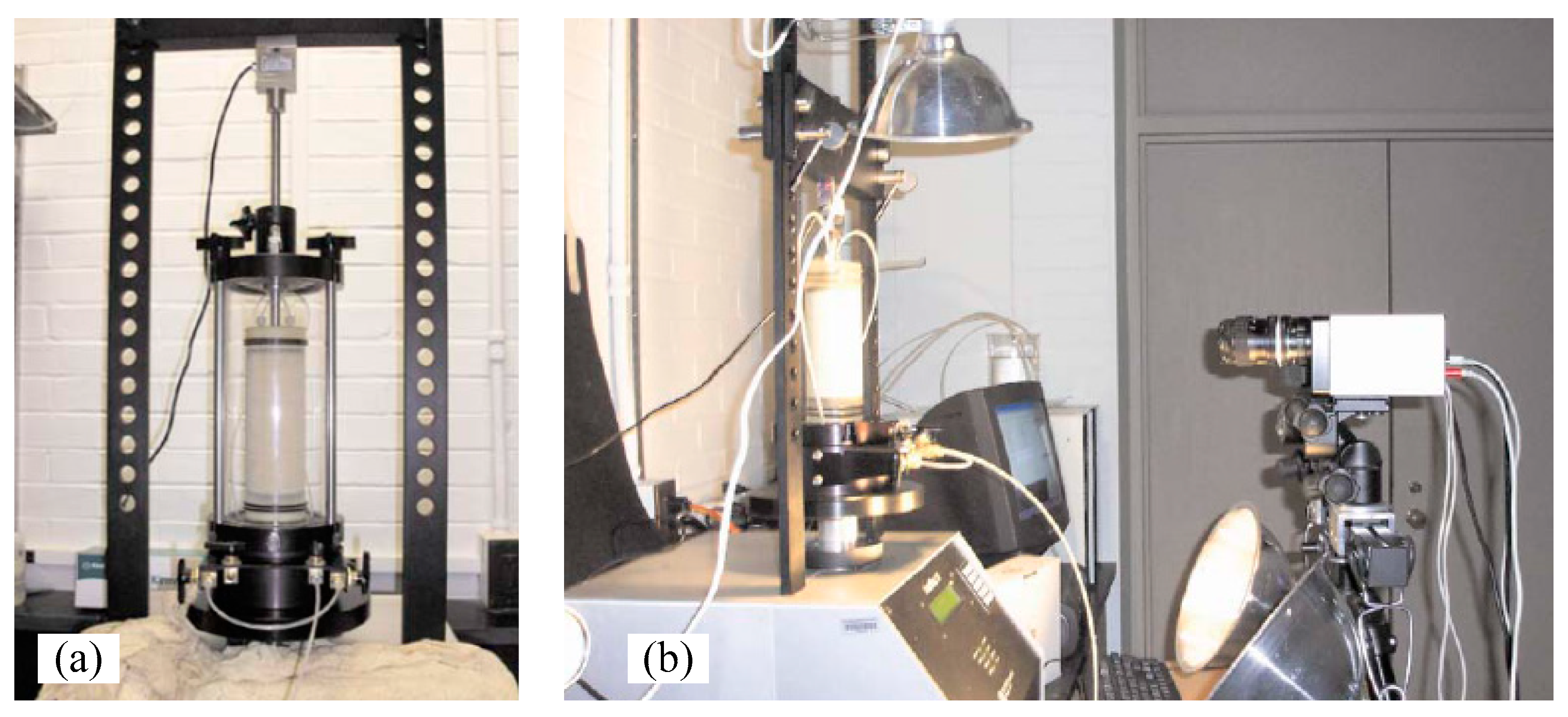
2.1. Triaxial Test
2.2. Digital Image Correlation (3D-DIC)
3. Finite Element Modeling of Triaxial Tests
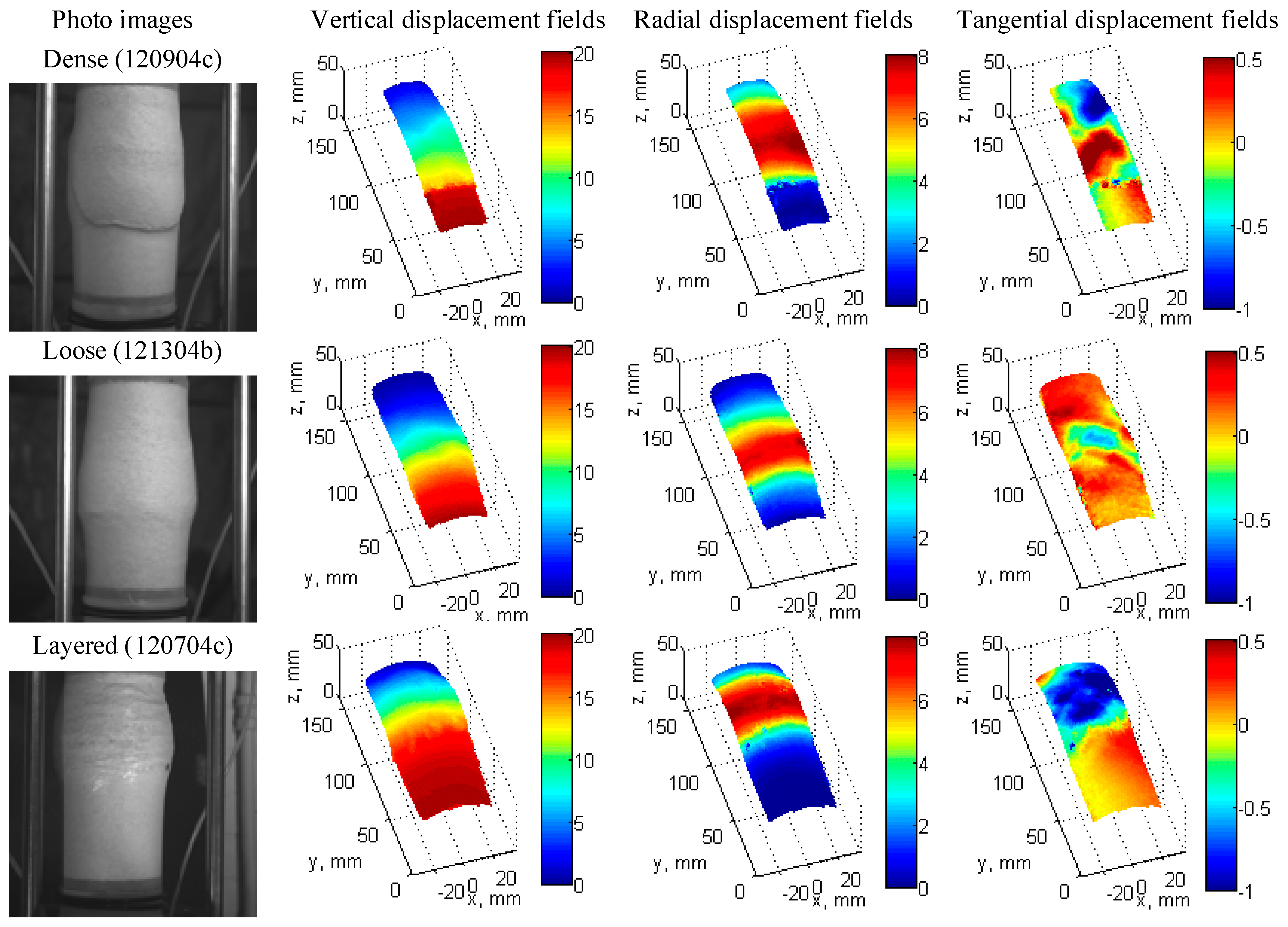
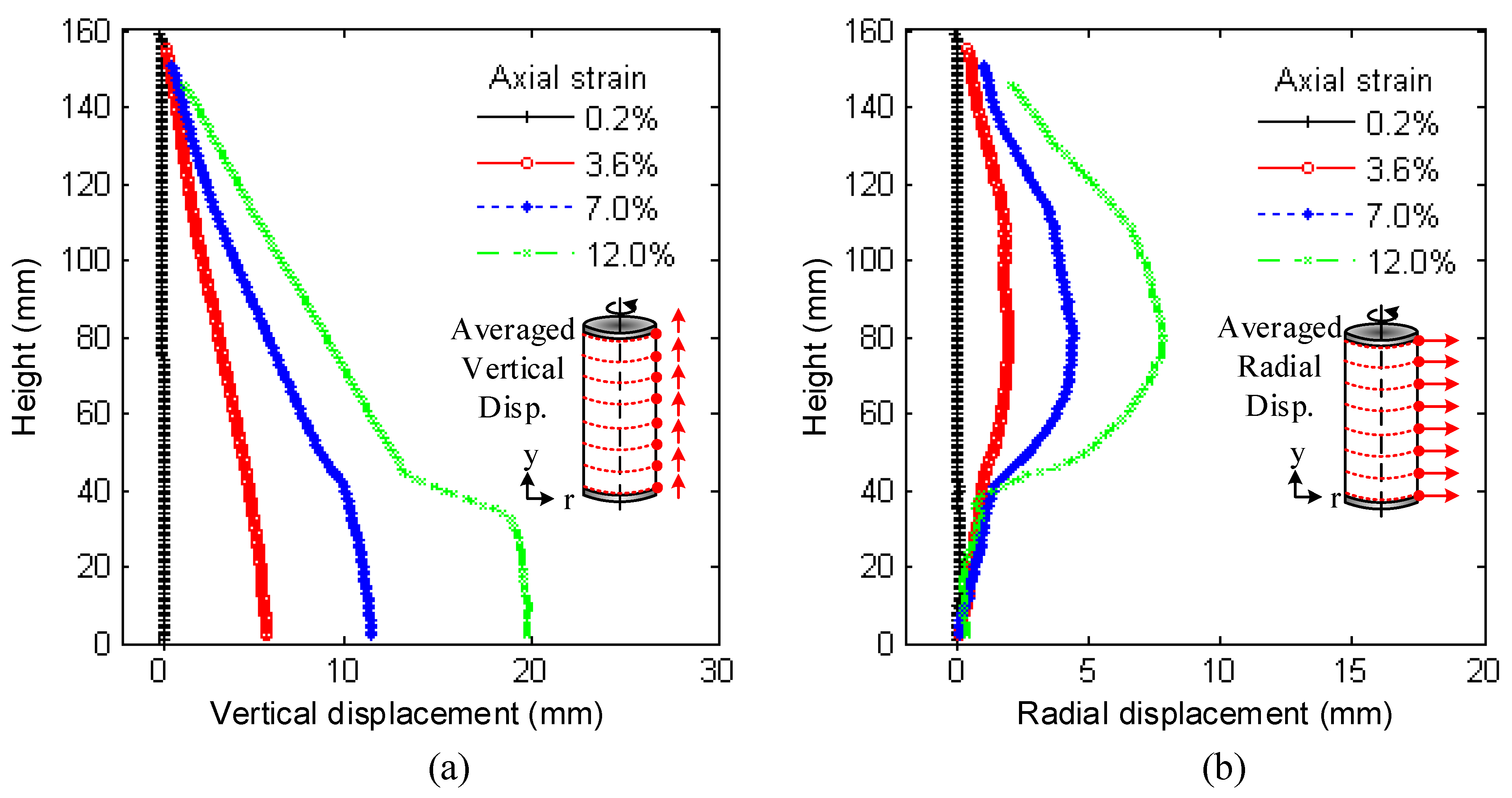
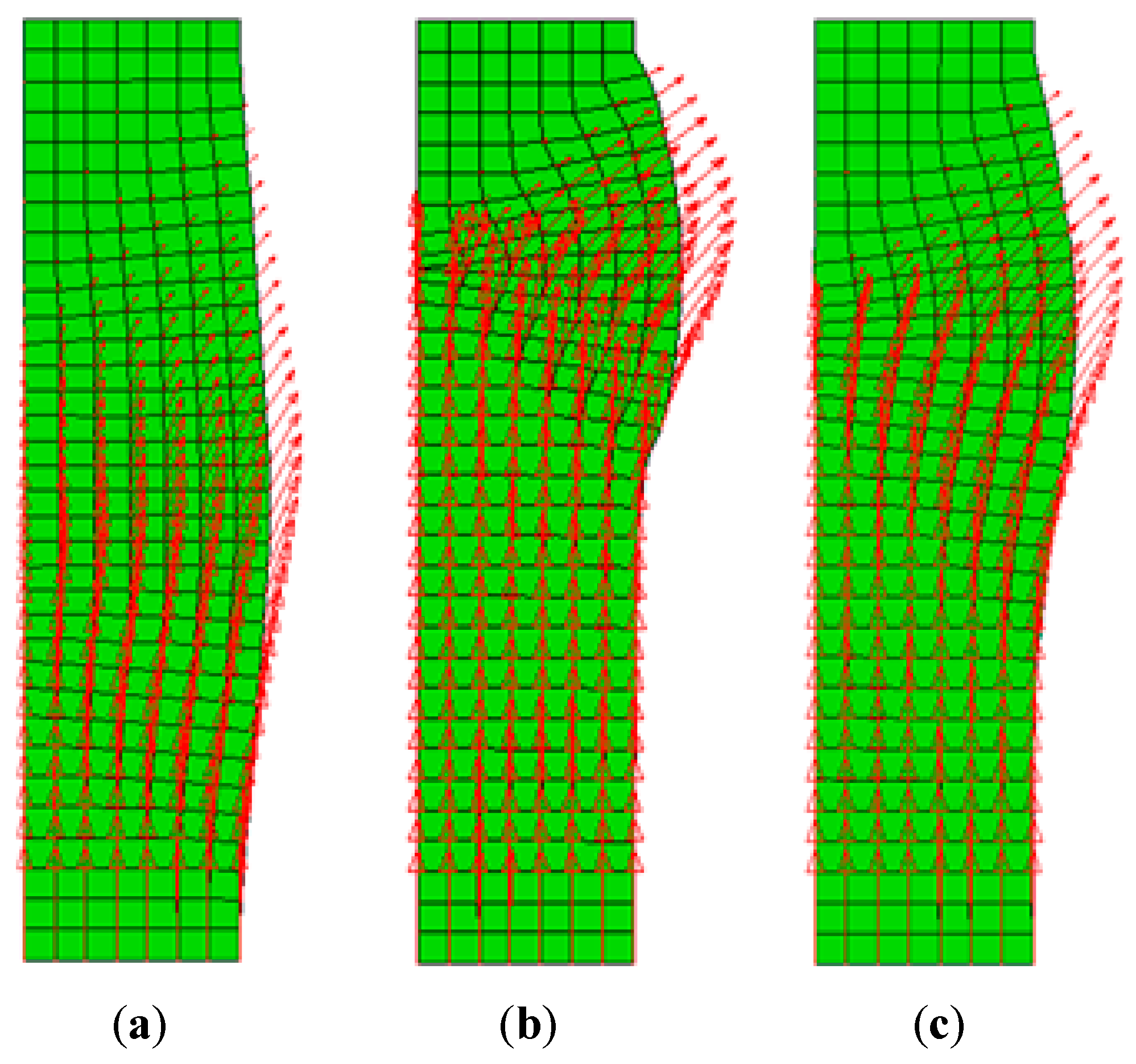
3.1. Experimental Behavior of Dense, Loose, and Half-Dense Half-Loose Specimens
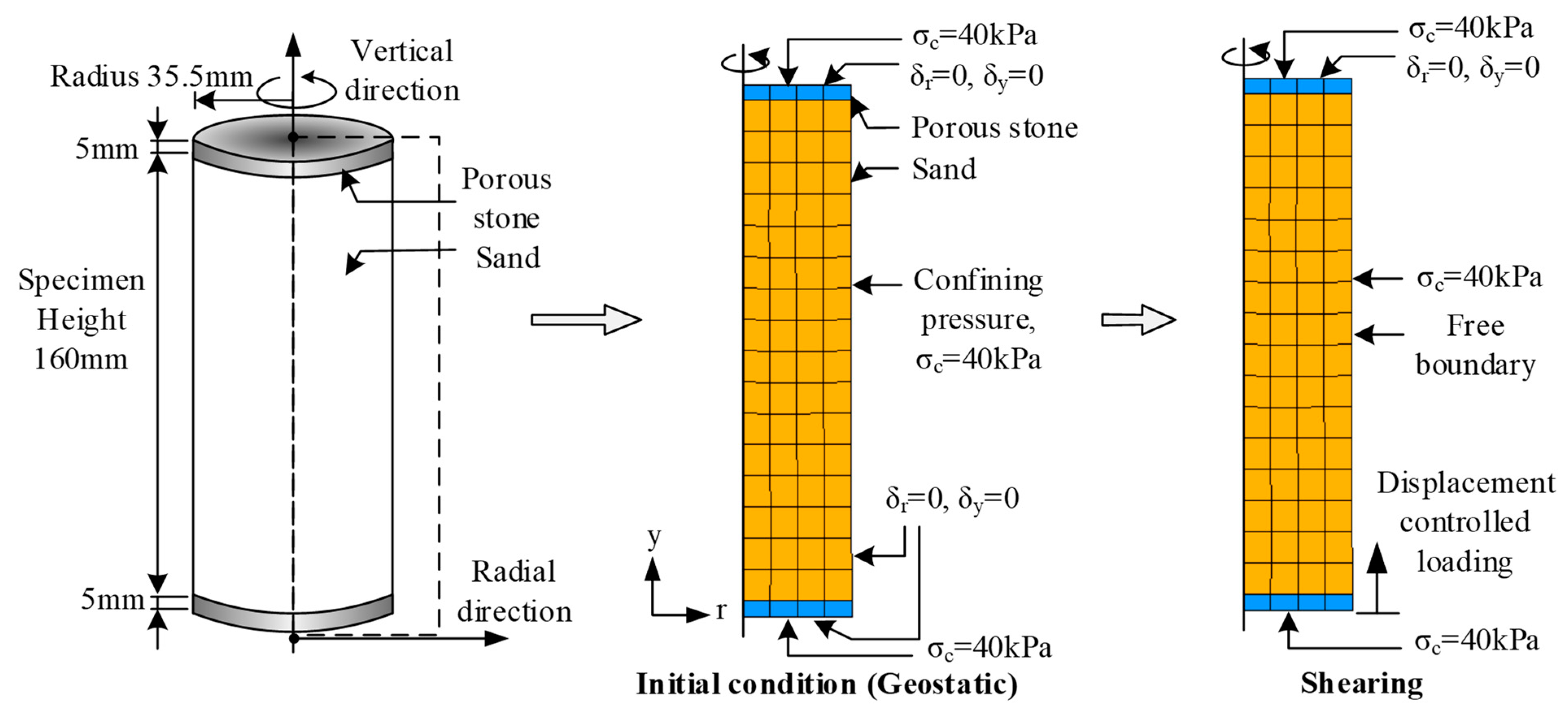
3.2. Finite Element Model
3.3. Proposed Elasto-Plastic Constitutive Model and Parameters
3.4. Analysis Cases of Homogeneous and Heterogeneous Specimens
4. Results and Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Medina-Cetina, Z.; Song, A.; Zhu, Y.; Pineda-Contreras, A.R.; Rechenmacher, A. Global and Local Deformation Effects of Dry Vacuum-Consolidated Triaxial Compression Tests on Sand Specimens: Making a Database Available for the Calibration and Development of Forward Models. Materials 2022, 15, 1528. [Google Scholar] [CrossRef] [PubMed]
- Zhu, Y.; Medina-Cetina, Z.; Pineda-Contreras, A.R. Spatio-Temporal Statistical Characterization of Boundary Kinematic Phenomena of Triaxial Sand Specimens. Materials 2022, 15, 2189. [Google Scholar] [CrossRef] [PubMed]
- Zhu, Y.; Medina-Cetina, Z. Assessment of Spatio-Temporal Kinematic Phenomena Observed along the Boundary of Triaxial Sand Specimens. Appl. Sci. 2022, 12, 8091. [Google Scholar] [CrossRef]
- Zhu, Y.; Medina-Cetina, Z. Statistical Characterization of Boundary Kinematics Observed on a Series of Triaxial Sand Specimens. Appl. Sci. 2022, 12, 11413. [Google Scholar] [CrossRef]
- Duncan, J.M. The role of advanced constitutive relations in practical applications. In Proceedings of the 13th International Conference Soil Mechanics and Foundation Engineering, New Delhi, India, 5–10 January 1994; Volume 5, pp. 31–48. [Google Scholar]
- Potts, D.M. Numerical analysis: A virtual dream or practical reality? Géotechnique 2003, 53, 535–573. [Google Scholar] [CrossRef]
- Brinkgreve, R.B.J. Selection of Soil Models and Parameters for Geotechnical Engineering Application: Soil Constitutive Models: Evaluation, Selection, and Calibration. Geotech. Spec. Publ. 2005, 128, 69–98. [Google Scholar] [CrossRef]
- Lade, P.; Kim, M. Single hardening constitutive model for frictional materials III. Comparisons with experimental data. Comput. Geotech. 1988, 6, 31–47. [Google Scholar] [CrossRef]
- Boldyrev, G.G.; Idrisov, I.K.; Valeev, D.N. Determination of parameters for soil models. Soil Mech. Found. Eng. 2006, 43, 101–108. [Google Scholar] [CrossRef]
- Lade, P.V. Overview of constitutive models for soils. In Soil Constitutive Models: Evaluation, Selection, and Calibration; Yamamuro, J.A., Kaliakin, V.N., Eds.; ASCE Geotechnical Special Publication No. 128; ASCE: Reston, VA, USA, 2005; pp. 1–34. [Google Scholar] [CrossRef]
- Sutton, M.; Wolters, W.; Peters, W.; Ranson, W.; McNeill, S. Determination of displacements using an improved digital correlation method. Image Vis. Comput. 1983, 1, 133–139. [Google Scholar] [CrossRef]
- Sutton, M.A.; McNeill, S.R.; Helm, J.D.; Chao, Y.J. Advances in Two-Dimensional and Three-Dimensional Computer Vision. Photomech. Top. Appl. Phys. 2000, 77, 323–372. [Google Scholar] [CrossRef]
- Sutton, M.; Yan, J.; Tiwari, V.; Schreier, H.; Orteu, J. The effect of out-of-plane motion on 2D and 3D digital image correlation measurements. Opt. Lasers Eng. 2008, 46, 746–757. [Google Scholar] [CrossRef]
- Schreier, H.; Orteu, J.-J.; Sutton, M.A. Image Correlation for Shape, Motion and Deformation Measurements; Springer Science & Business Media: Boston, MA, USA, 2009. [Google Scholar] [CrossRef]
- Hall, S.; Bornert, M.; Desrues, J.; Pannier, Y.; Lenoir, N.; Viggiani, G.; Bésuelle, P. Discrete and continuum analysis of localised deformation in sand using X-ray μCT and volumetric digital image correlation. Géotechnique 2010, 60, 315–322. [Google Scholar] [CrossRef]
- Higo, Y.; Oka, F.; Sato, T.; Matsushima, Y.; Kimoto, S. Investigation of localized deformation in partially saturated sand under triaxial compression using microfocus X-ray CT with digital image correlation. Soils Found. 2013, 53, 181–198. [Google Scholar] [CrossRef]
- Chaney, R.; Demars, K.; Macari, E.; Parker, J.; Costes, N. Measurement of Volume Changes in Triaxial Tests Using Digital Imaging Techniques. Geotech. Test. J. 1997, 20, 103. [Google Scholar] [CrossRef]
- Suits, L.D.; Sheahan, T.; Rechenmacher, A.; Finno, R. Digital Image Correlation to Evaluate Shear Banding in Dilative Sands. Geotech. Test. J. 2004, 27, 10864. [Google Scholar] [CrossRef]
- Rechenmacher, A.L.; Abedi, S.; Chupin, O.; Orlando, A.D. Characterization of mesoscale instabilities in localized granular shear using digital image correlation. Acta Geotech. 2011, 6, 205–217. [Google Scholar] [CrossRef]
- Wang, P.; Sang, Y.; Shao, L.; Guo, X. Measurement of the deformation of sand in a plane strain compression experiment using incremental digital image correlation. Acta Geotech. 2018, 14, 547–557. [Google Scholar] [CrossRef]
- Belheine, N.; Plassiard, J.-P.; Donzé, F.-V.; Darve, F.; Seridi, A. Numerical simulation of drained triaxial test using 3D discrete element modeling. Comput. Geotech. 2009, 36, 320–331. [Google Scholar] [CrossRef]
- Cil, M.B.; Alshibli, K.A. 3D analysis of kinematic behavior of granular materials in triaxial testing using DEM with flexible membrane boundary. Acta Geotech. 2013, 9, 287–298. [Google Scholar] [CrossRef]
- Lee, S.J.; Hashash, Y.M.; Nezami, E.G. Simulation of triaxial compression tests with polyhedral discrete elements. Comput. Geotech. 2012, 43, 92–100. [Google Scholar] [CrossRef]
- Lu, Y.; Frost, D. Three-Dimensional DEM Modeling of Triaxial Compression of Sands. In Proceedings of the GeoShanghai International Conference 2010, Shanghai, China, 3–5 June 2010; pp. 220–226. [Google Scholar] [CrossRef]
- Kawamoto, R.; Andò, E.; Viggiani, G.; Andrade, J.E. Level set discrete element method for three-dimensional computations with triaxial case study. J. Mech. Phys. Solids 2016, 91, 1–13. [Google Scholar] [CrossRef]
- Kawamoto, R.; Andò, E.; Viggiani, G.; Andrade, J.E. All you need is shape: Predicting shear banding in sand with LS-DEM. J. Mech. Phys. Solids 2018, 111, 375–392. [Google Scholar] [CrossRef]
- Kozicki, J.; Tejchman, J. Numerical simulations of triaxial test with sand using DEM. Arch. Hydro-Eng. Environ. Mech. 2009, 56, 149–172. [Google Scholar]
- Huang, W.; Sun, D.; Sloan, S. Analysis of the failure mode and softening behaviour of sands in true triaxial tests. Int. J. Solids Struct. 2007, 44, 1423–1437. [Google Scholar] [CrossRef]
- Mozaffari, M.; Liu, W.; Ghafghazi, M. Influence of specimen nonuniformity and end restraint conditions on drained triaxial compression test results in sand. Can. Geotech. J. 2022, 99, 1–13. [Google Scholar] [CrossRef]
- Medina-Cetina, Z.; Rechenmacher, A. Influence of boundary conditions, specimen geometry and material heterogeneity on model calibration from triaxial tests. Int. J. Numer. Anal. Methods Géoméch. 2009, 34, 627–643. [Google Scholar] [CrossRef]
- ABAQUS Inc. ABAQUS User’s Manual, version 6.14.; ABAQUS Inc.: Palo Alto, CA, USA, 2022. [Google Scholar]
- Song, A.; Medina-Cetina, Z.; Rechenmacher, A.L. Local deformation analysis of a sand specimen using 3D digital image correlation for the calibration of a simple elasto-plastic model. In Proceedings of the GeoCongress 2012: State of the Art and Practice in Geotechnical Engineering, Oakland, CA, USA, 25–29 March 2012; pp. 2292–2301. [Google Scholar] [CrossRef]
- Geocomp Corporation. Geocomp Triaxial Automated System; Geocomp Corporation: Acton, MA, USA, 2002. [Google Scholar]
- Medina-Cetina, Z. Probabilistic Calibration of a Soil Model. Ph.D. Thesis, The John Hopkins University, Baltimore, MD, USA, 2006. [Google Scholar]
- Correlated Solutions, Inc. VIC-3D; Correlated Solutions, Inc.: Irmo, SC, USA, 2010. [Google Scholar]
- Das, B.M. Principles of Geotechnical Engineering, 5th ed.; Thomson Learning: Pacific Grove, CA, USA, 2001. [Google Scholar]
- Finno, R.J.; Rechenmacher, A.L. Effects of Consolidation History on Critical State of Sand. J. Geotech. Geoenviron. Eng. 2003, 129, 350–360. [Google Scholar] [CrossRef]
- Song, A. Deformation Analysis of Sand Specimens Using 3D Digital Image Correlation for the Calibration of an Elasto-Plastic Model. Ph.D. Thesis, Texas A & M University, College Station, TX, USA, 2012. [Google Scholar]
- Gay, O.; Boutonnier, L.; Foray, P.; Flavigny, E. Laboratory characterization of Hostun RF sand at very low confining stresses. In Deformation Characteristics of Geomaterials; Di Benedetto, H., Doanh, T., Geoffroy, H., Sauzeat, C., Eds.; A. A. Balkema: Lisse, The Netherlands, 2003; pp. 423–430. [Google Scholar] [CrossRef]
- Potts, D.M.; Zdravkovic, L. Finite Element Analysis in Geotechnical Engineering; Thomas Telford Publishing: London, UK, 1999; Volume 1-Theory. [Google Scholar]



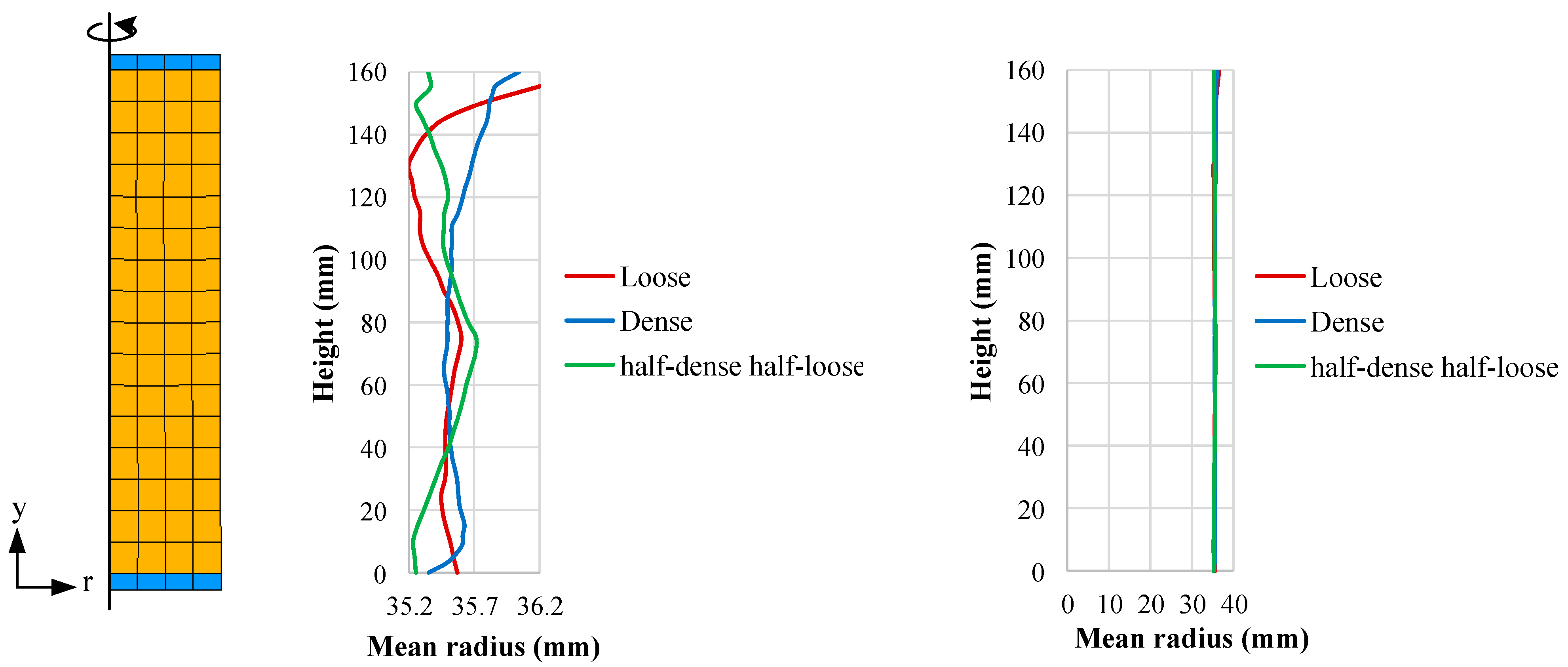
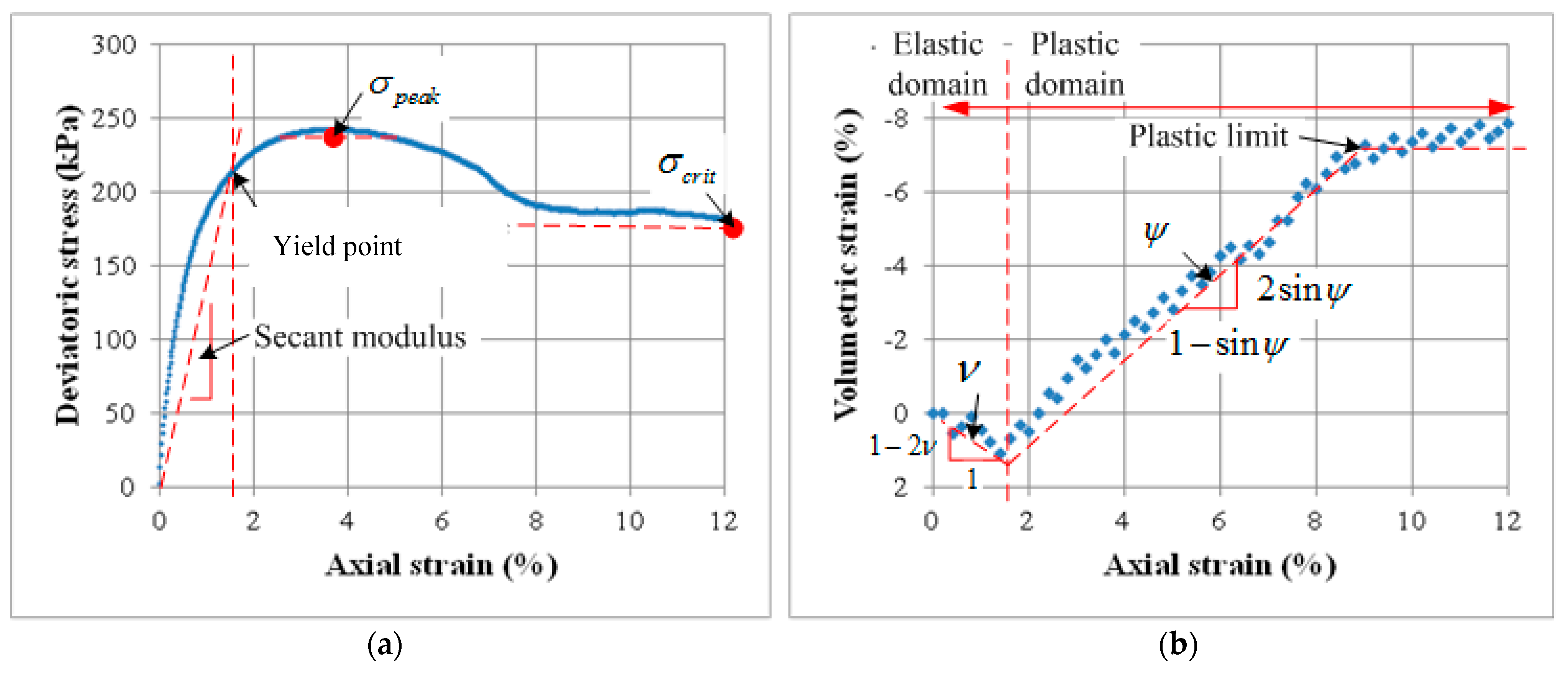

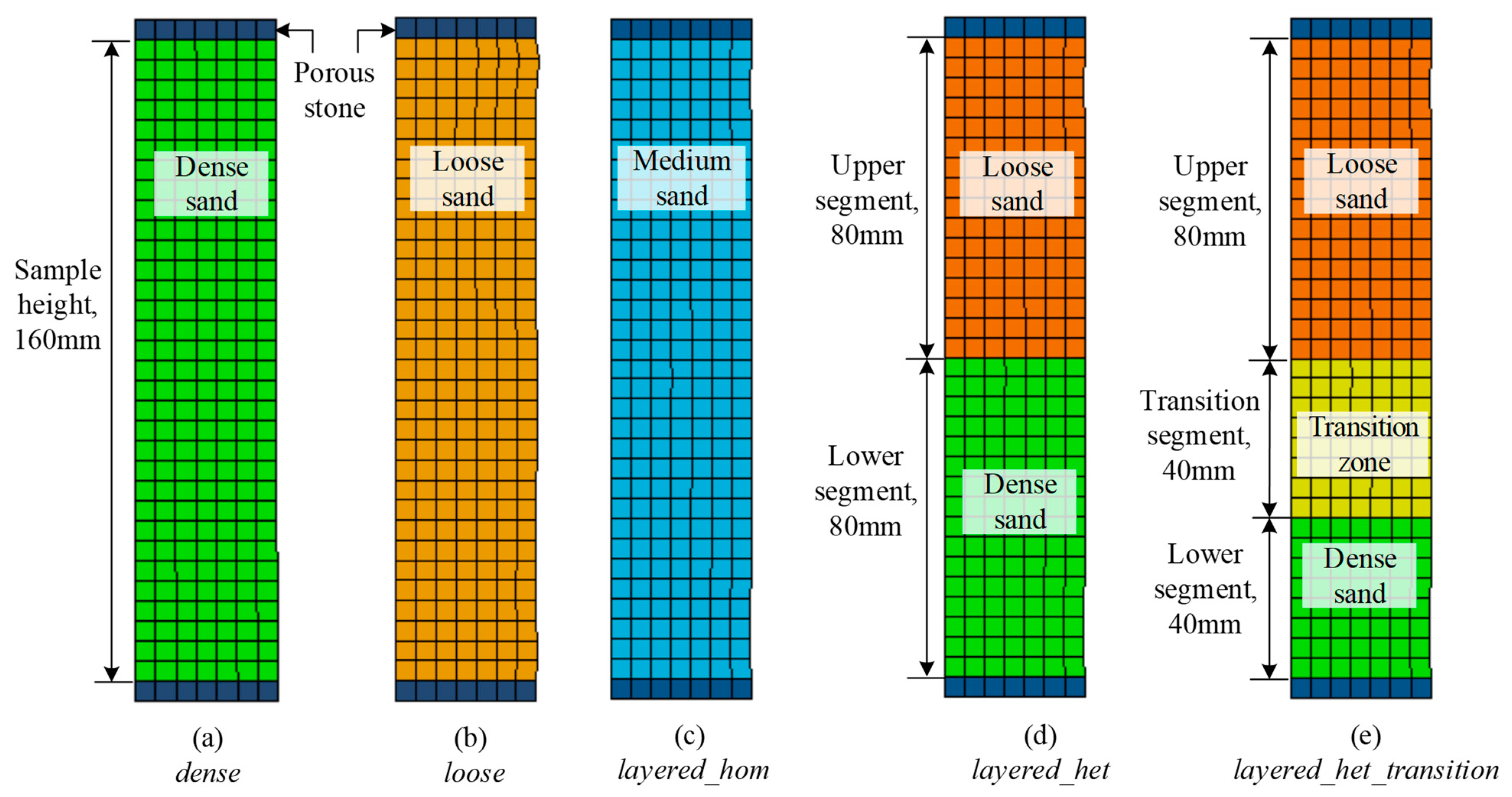
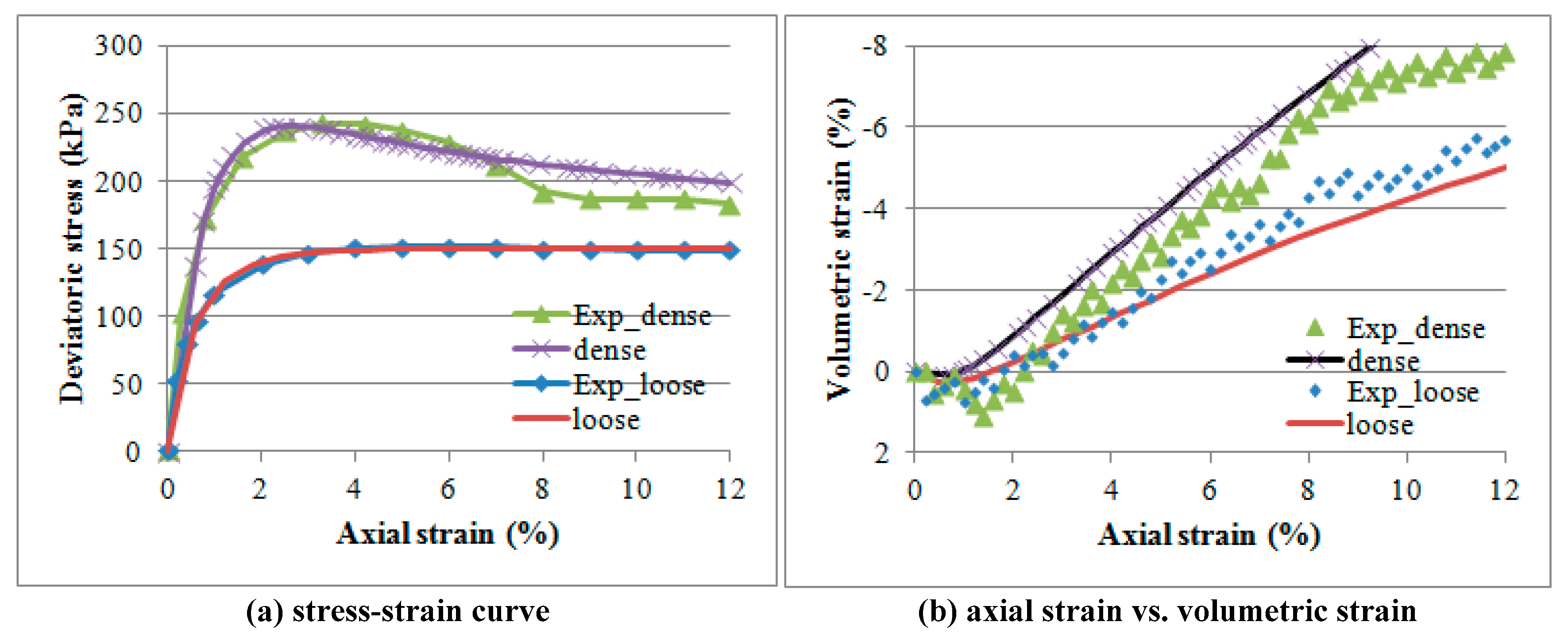
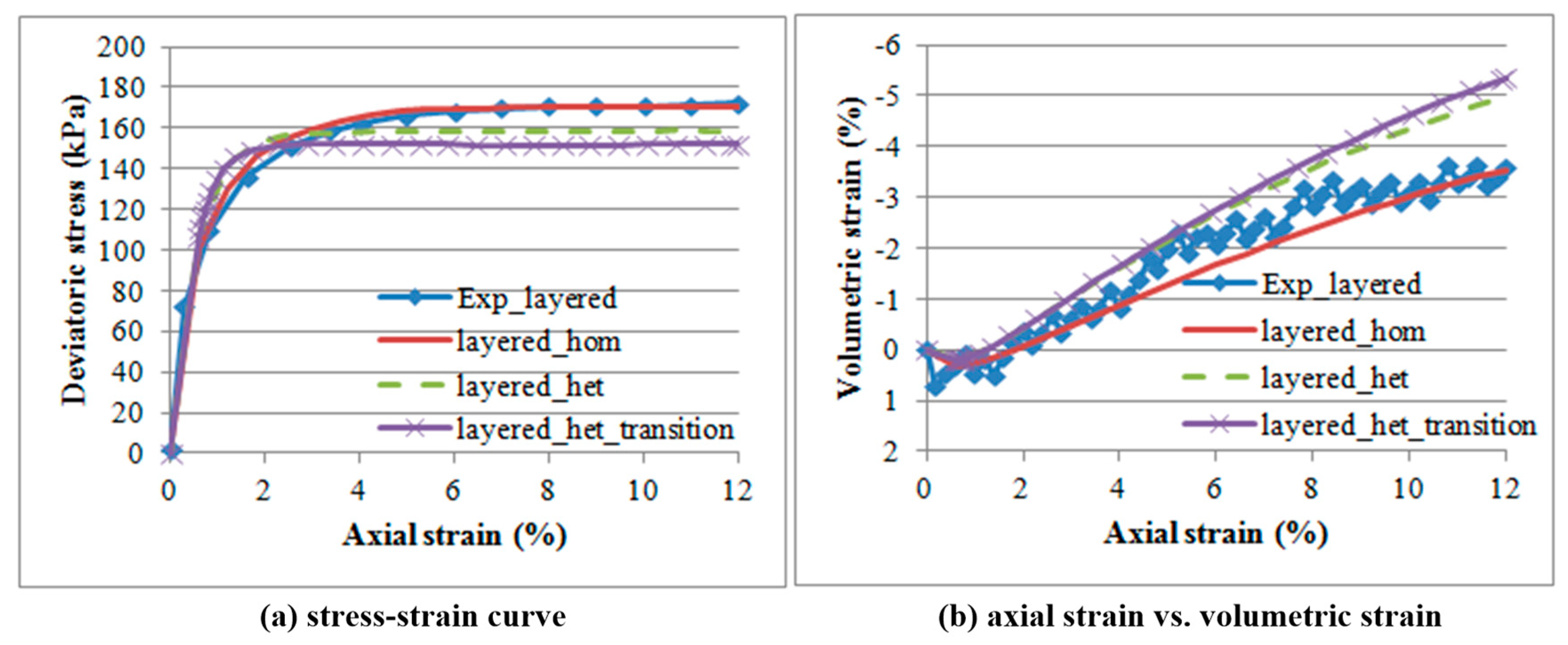
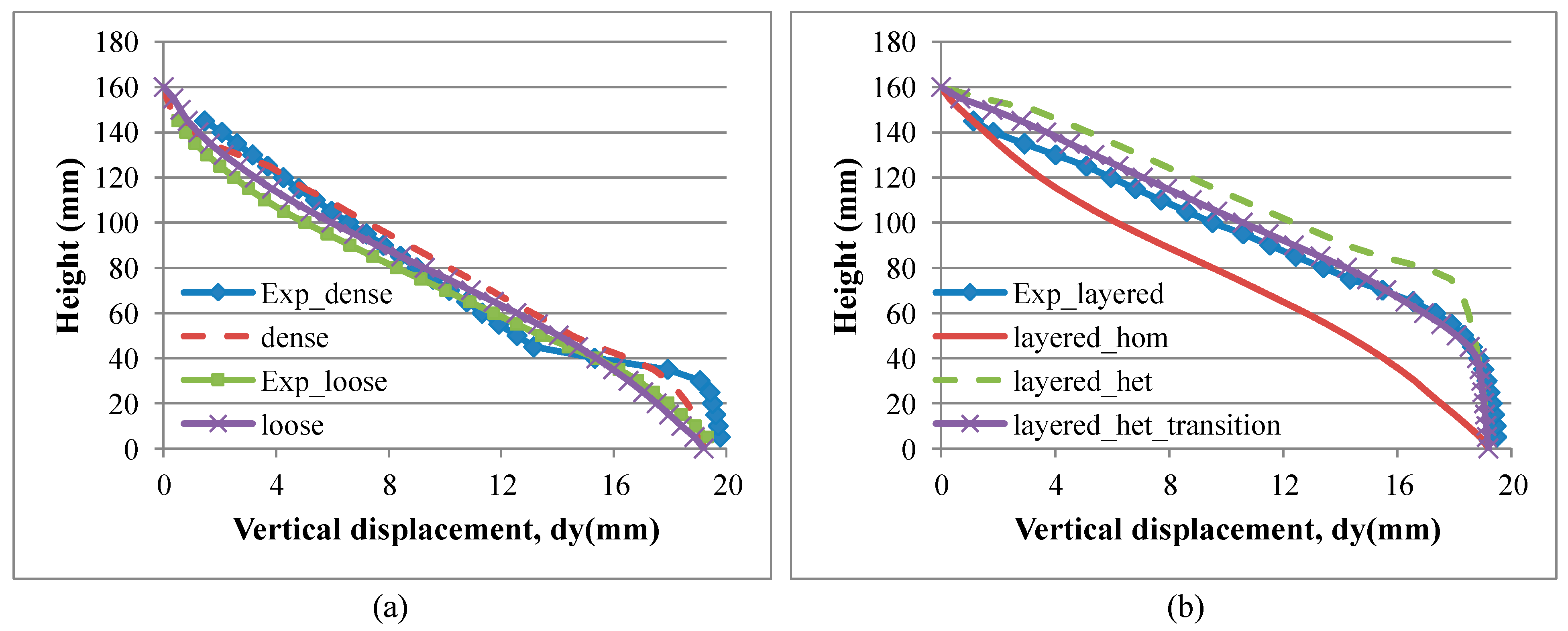
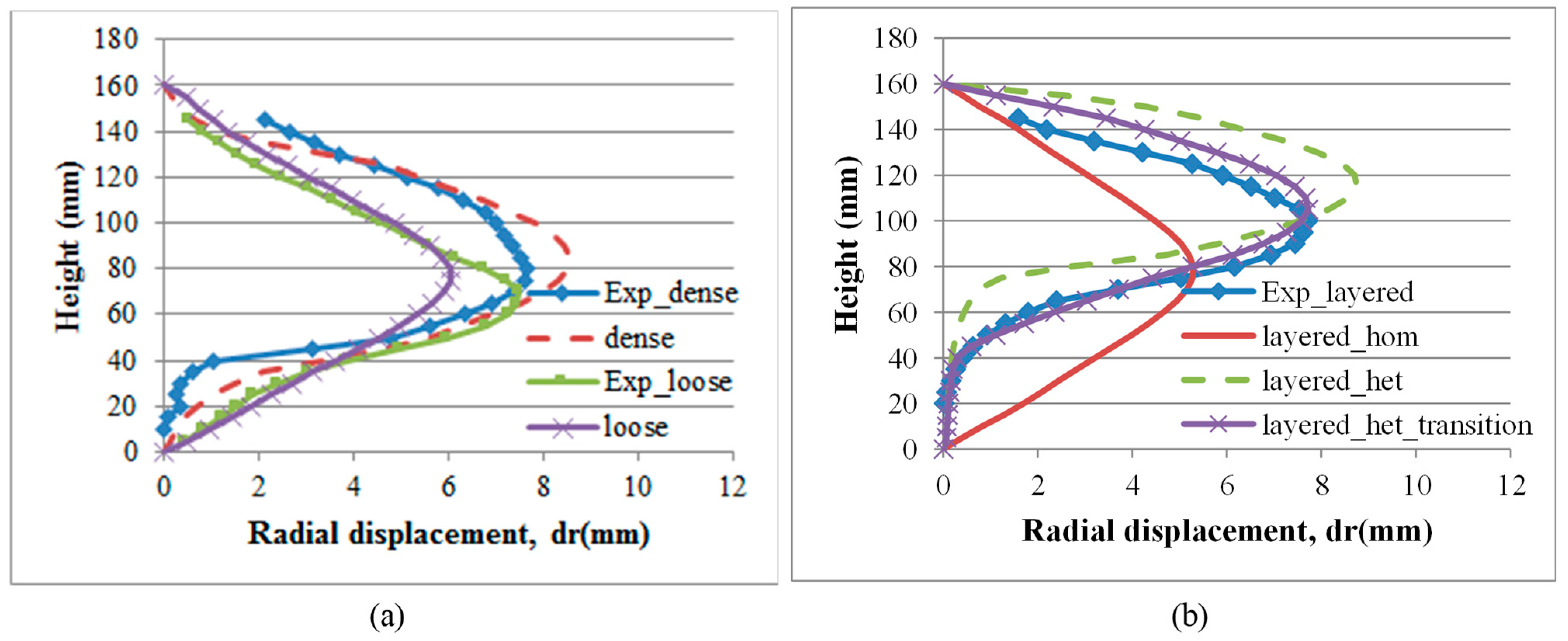
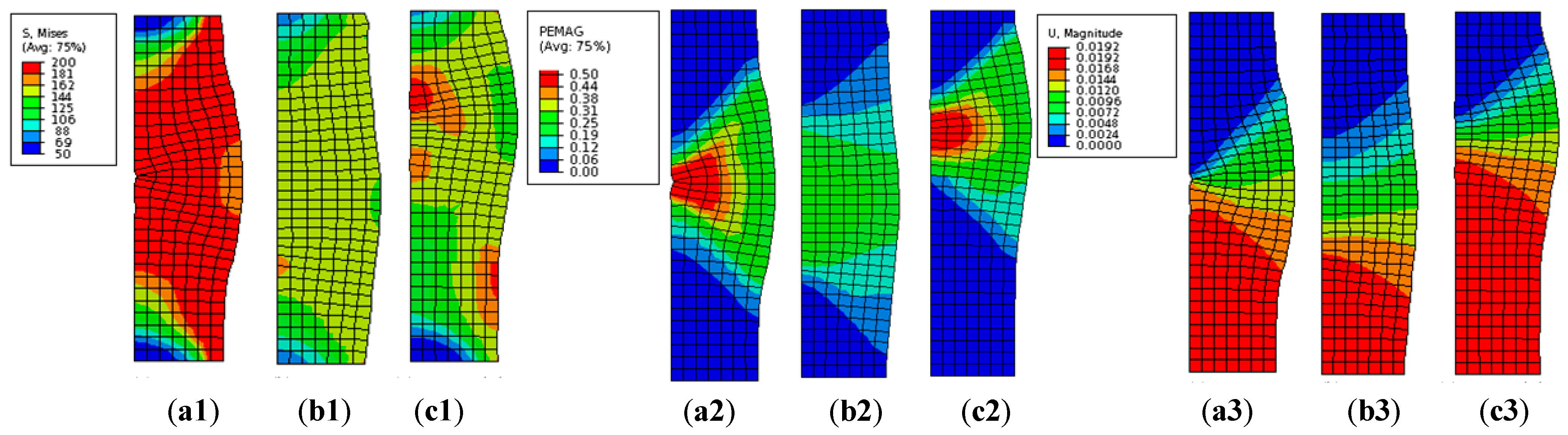
| Case | Test Name | Height (mm) | Diameter (mm) | Initial Density (kg/m3) | Relative Density (%) | Confinement (kPa) | Sample Preparation |
|---|---|---|---|---|---|---|---|
| Dense | 120904c | 159.67 | 71.11 | 1713.13 | 91.83 | 40 | Vibratory compaction |
| Loose | 121304b | 158.17 | 70.86 | 1588.84 | 46.39 | 40 | Dry pluviation |
| Half-densehalf-loose layered | 120704c | 157.67 | 70.88 | 1648.06 (avg.) | 68.90 (avg.) | 40 | Vibratory compaction (two layers) |
| Upper | 78.17 | 70.68 | 1549.61 | 30.54 | 40 | ||
| Lower | 79.50 | 71.27 | 1764.17 | 98.87 | 40 |
| Case | Configuration | Unit Weight (kN/m3) | Young’s Modulus (kPa) | Poisson’s Ratio | Friction Angle (deg) | Dilation Angle (deg) |
|---|---|---|---|---|---|---|
| Dense | Dense | 20 | 21,559 | 0.44 | 43.09 | 22.78 |
| Loose | Loose | 20 | 15,818 | 0.25 | 32.86 | 14.48 |
| Layered_hom | Medium | 20 | 18,164 | 0.20 | 32.12 | 11.97 |
| Layered_het | Upper loose | 20 | 15,818 | 0.25 | 32.86 | 14.48 |
| Lower dense | 20 | 21,559 | 0.44 | 43.09 | 22.78 | |
| Layered_het_transition | Upper loose | 20 | 15,818 | 0.25 | 32.86 | 14.48 |
| Transition zone | 20 | 20,361 | 0.37 | 36.86 | 18.19 | |
| Lower dense | 20 | 21,559 | 0.44 | 43.09 | 22.78 | |
| Porous stone | - | 20 | 1,000,000 | 0.20 | - | - |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Song, A.; Pineda-Contreras, A.R.; Medina-Cetina, Z. Modeling of Sand Triaxial Specimens under Compression: Introducing an Elasto-Plastic Finite Element Model to Capture the Impact of Specimens’ Heterogeneity. Minerals 2023, 13, 498. https://doi.org/10.3390/min13040498
Song A, Pineda-Contreras AR, Medina-Cetina Z. Modeling of Sand Triaxial Specimens under Compression: Introducing an Elasto-Plastic Finite Element Model to Capture the Impact of Specimens’ Heterogeneity. Minerals. 2023; 13(4):498. https://doi.org/10.3390/min13040498
Chicago/Turabian StyleSong, Ahran, Alma Rosa Pineda-Contreras, and Zenon Medina-Cetina. 2023. "Modeling of Sand Triaxial Specimens under Compression: Introducing an Elasto-Plastic Finite Element Model to Capture the Impact of Specimens’ Heterogeneity" Minerals 13, no. 4: 498. https://doi.org/10.3390/min13040498
APA StyleSong, A., Pineda-Contreras, A. R., & Medina-Cetina, Z. (2023). Modeling of Sand Triaxial Specimens under Compression: Introducing an Elasto-Plastic Finite Element Model to Capture the Impact of Specimens’ Heterogeneity. Minerals, 13(4), 498. https://doi.org/10.3390/min13040498







