Effect of Agitation on the Dissolution of APAM with Different Molecular Weights and the Equivalent Diameter of Coal Slime Settling Floc with Different Particle Sizes
Abstract
:1. Introduction
2. Test
2.1. Test Materials and Instruments
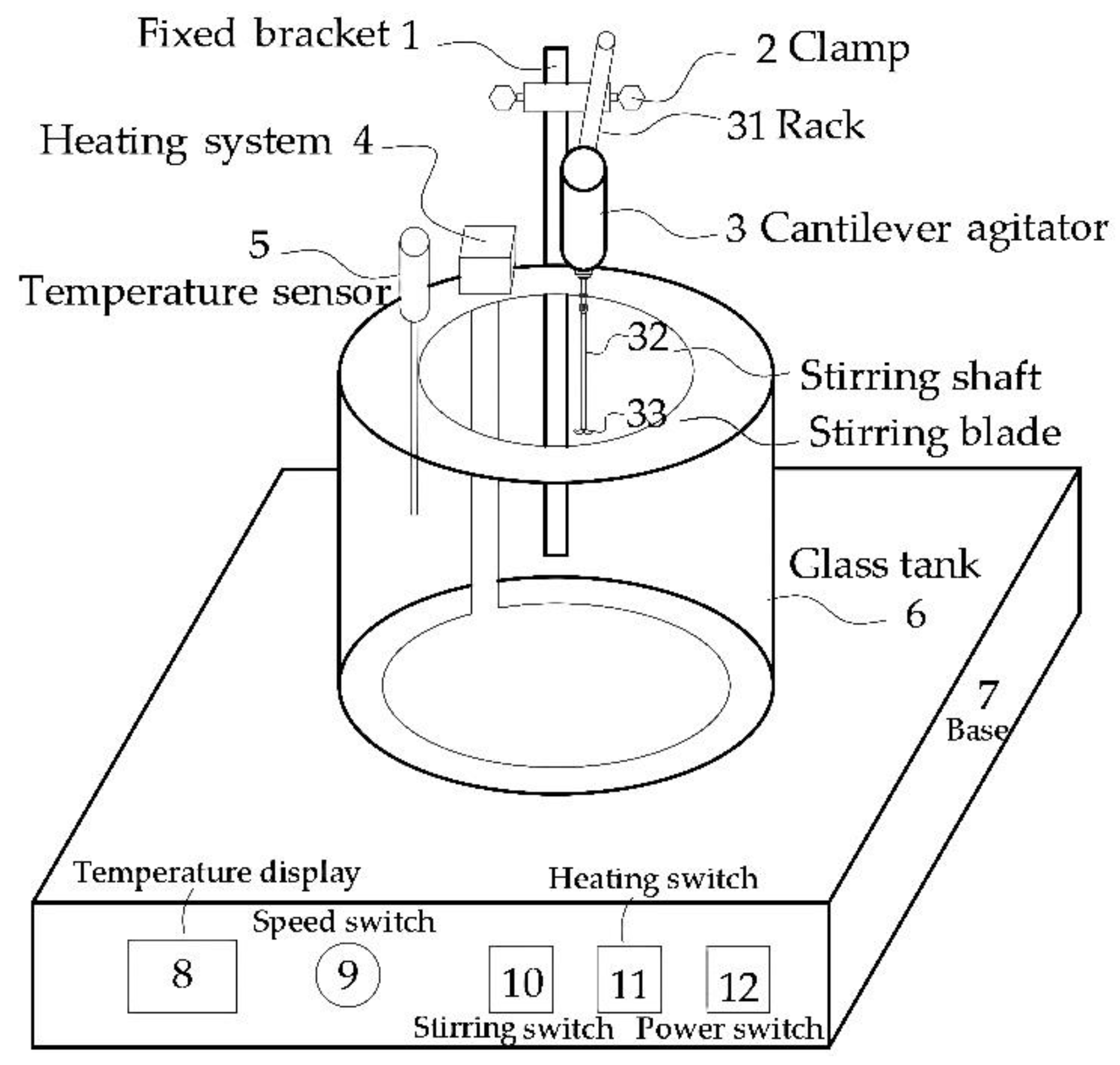
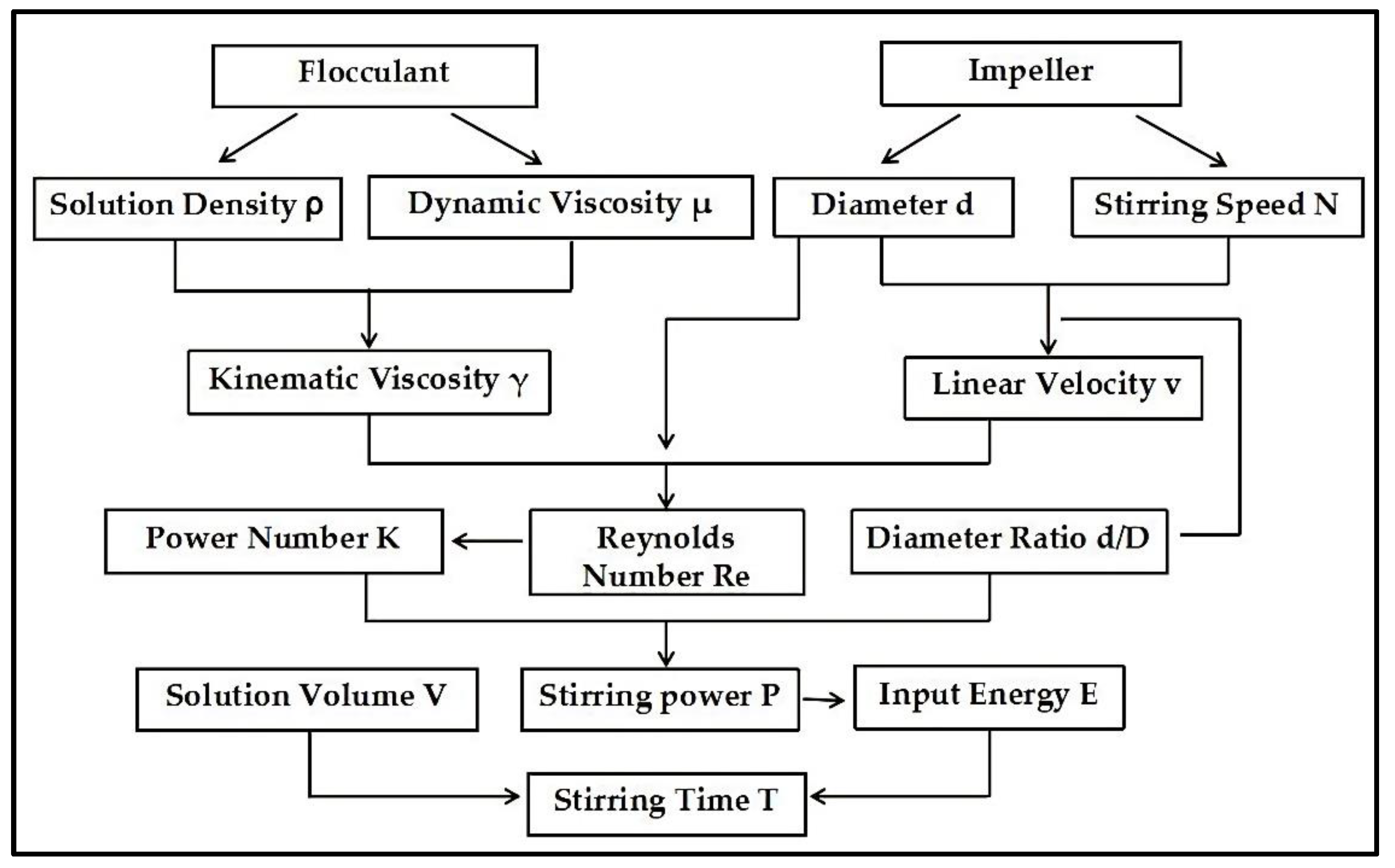
2.2. Test Methods and Significance
2.3. Data Calculation
3. Results and Analysis
- (1)
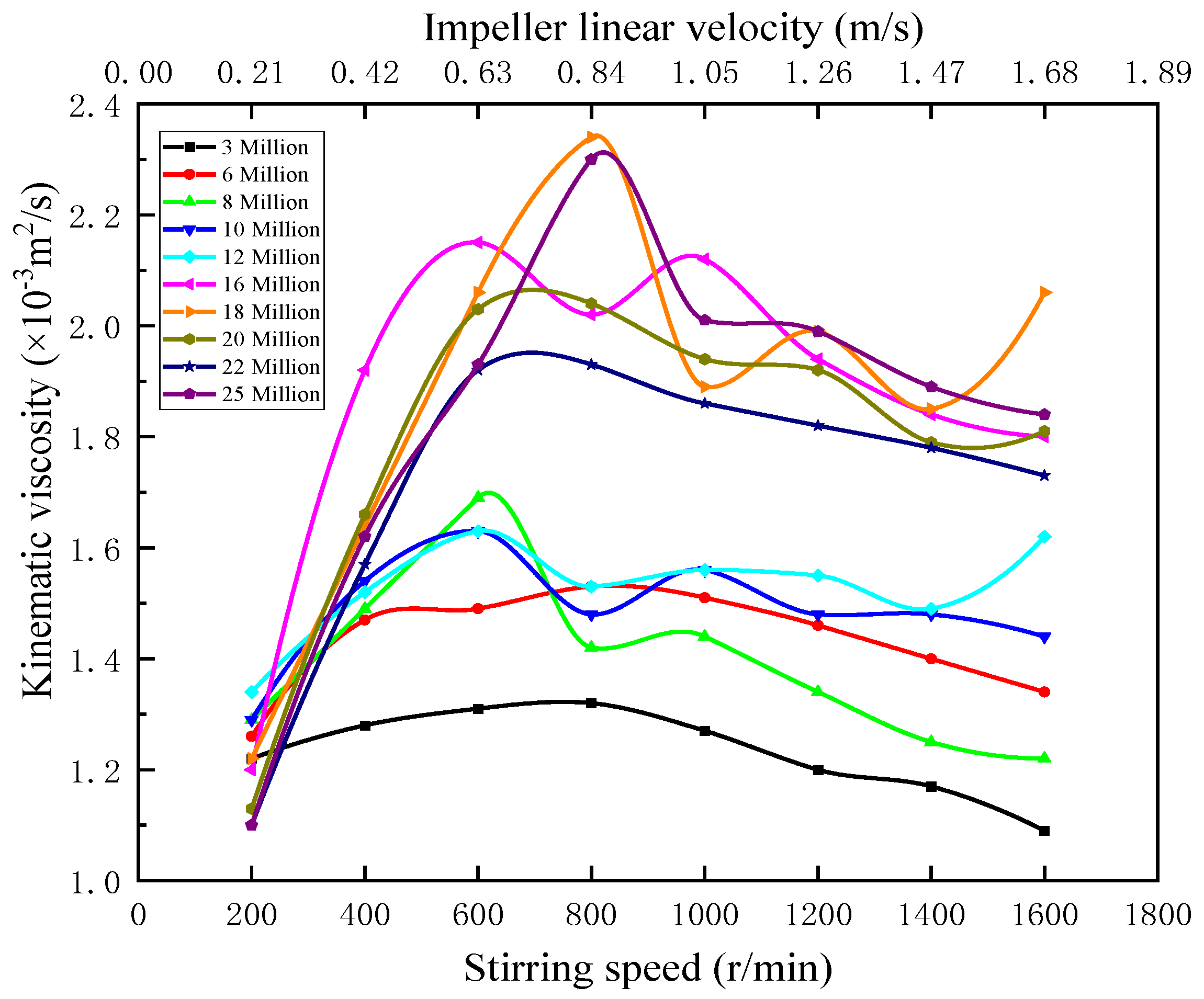
- Table 1 and Table 2 indicate that the optimum stirring speed N = 800 r/min for 3–6 million molecular weight APAM corresponds to an impeller linear velocity v=0.84 m/s; The optimum stirring speed N = 600 r/min for 8–16 million molecular weight APAM corresponds to an impeller linear velocity v = 0.63 m/s; The optimum stirring speed N = 800 r/min for 18–25 million molecular weight APAM corresponds to an impeller linear velocity v = 0.84 m/s.
- (2)
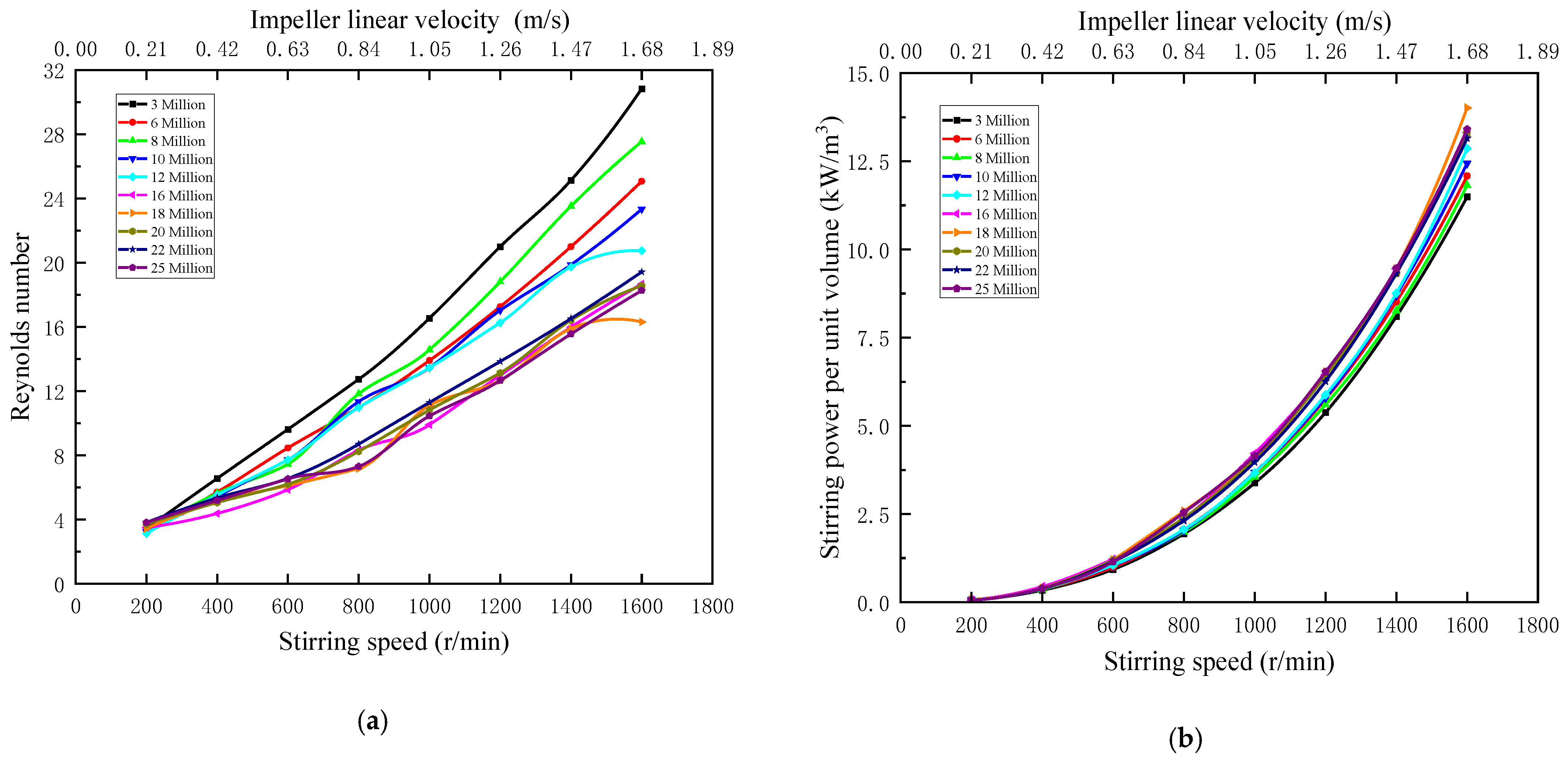
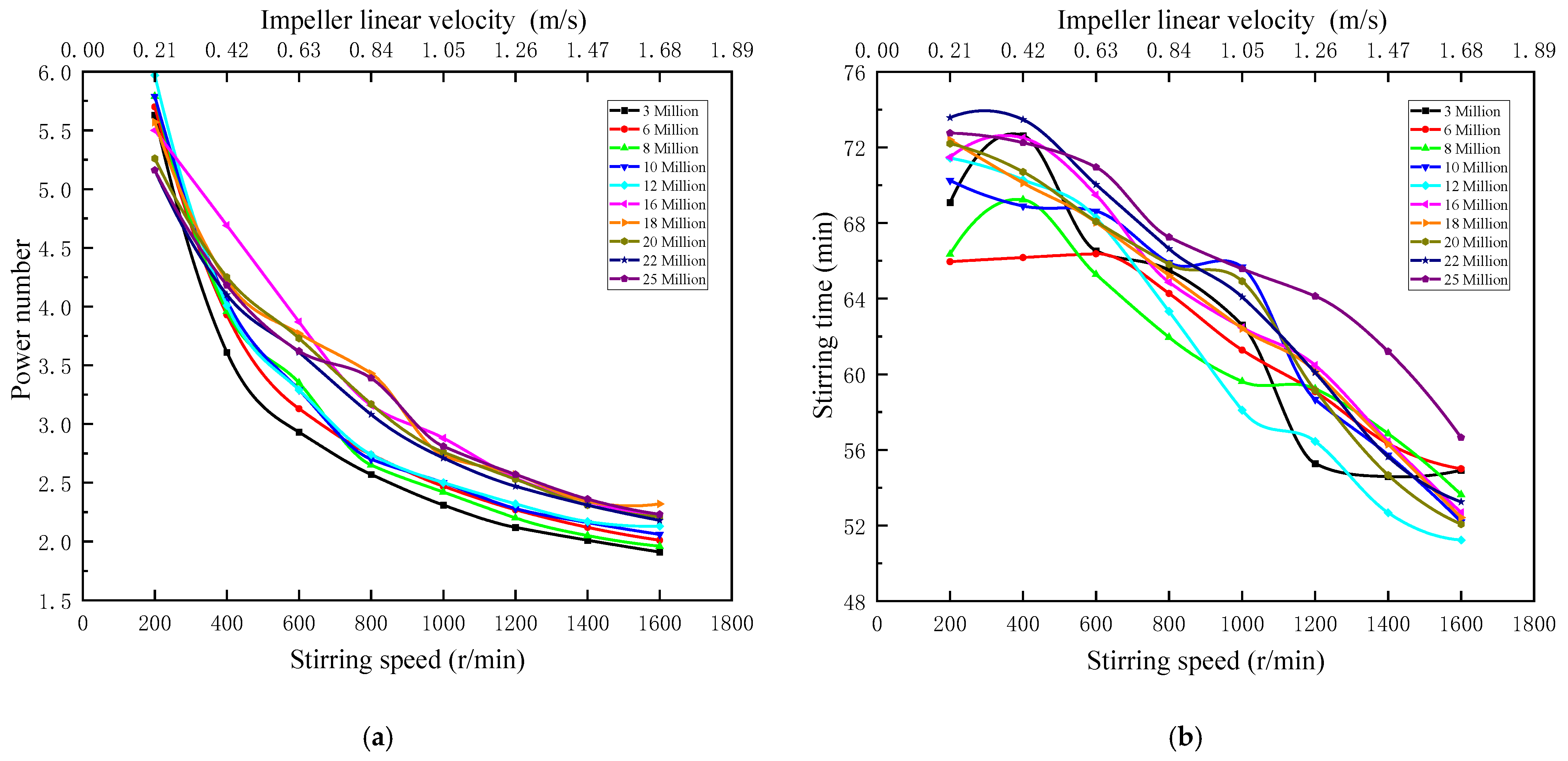
- Figure 2, Figure 3 and Figure 4 indicate that when the stirring speed ranges from 200 to 1600 r/min, the corresponding impeller linear velocity ranges from 0.21 to 1.68 m/s, the dissolution parameters are positively correlated: kinematic viscosity and molecular weight, Reynolds number and linear velocity, power number and molecular weight, stirring power and linear velocity, stirring power and molecular weight, stirring time and molecular weight; There are negative correlations between Reynolds number and molecular weight, power number and linear velocity, stirring time and linear velocity.
- (3)
- Based on the original stirring power formula, the single factor d of impeller diameter is replaced by the two factors D of stirring bucket diameter and the ratio of the impeller to stirring bucket diameter d/D as the influencing parameters of stirring power. Through data fitting, the improved stirring power formula applicable to the dissolution of APAM is , which is obtained by fitting all the data from the stirring dissolution test of 3–25 million APAM.
3.1. Kinematic Viscosity versus Stirring Speed
3.2. Reynolds Number & Stirring Power per Unit Volume versus Stirring Speed
3.3. Power Number & Stirring Time versus Stirring Speed
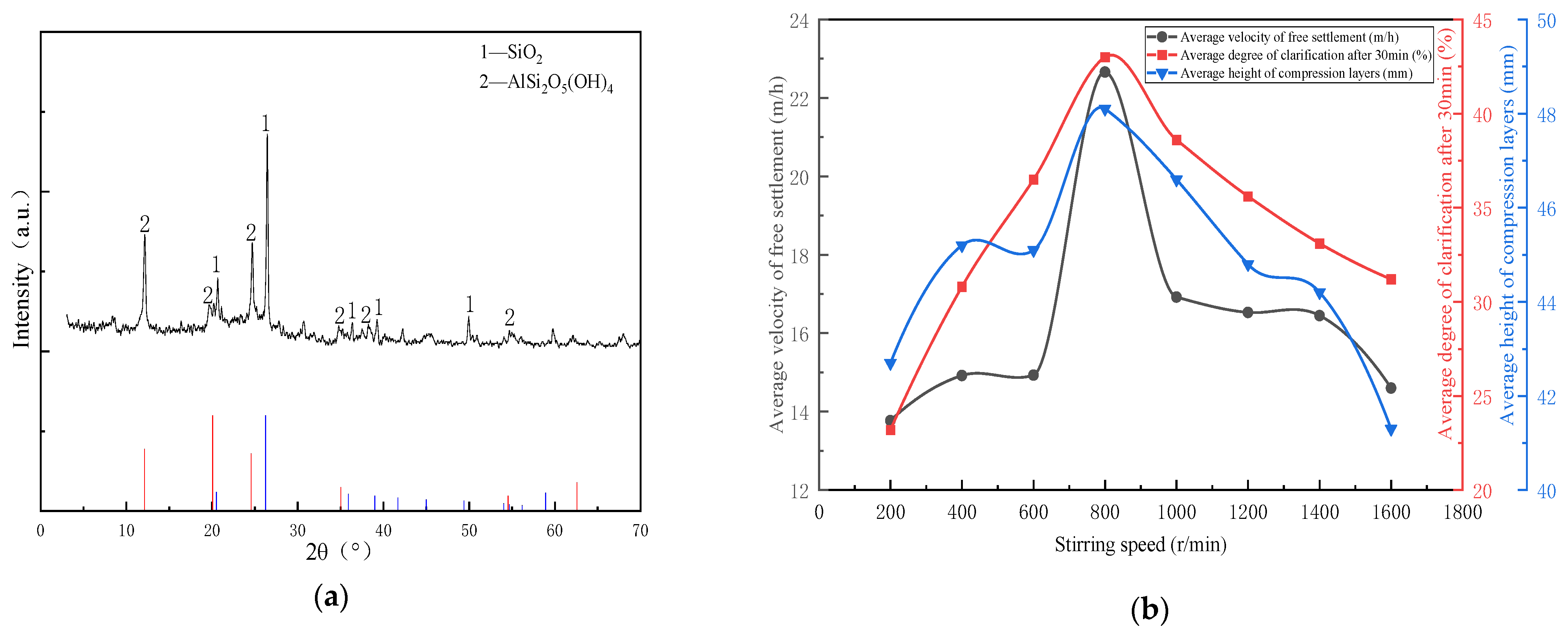
3.4. APAM Flocculation Performance Determination at Different Stirring Speeds
4. Equivalent Diameter of Settling Floc with Different Particle Sizes of Slime
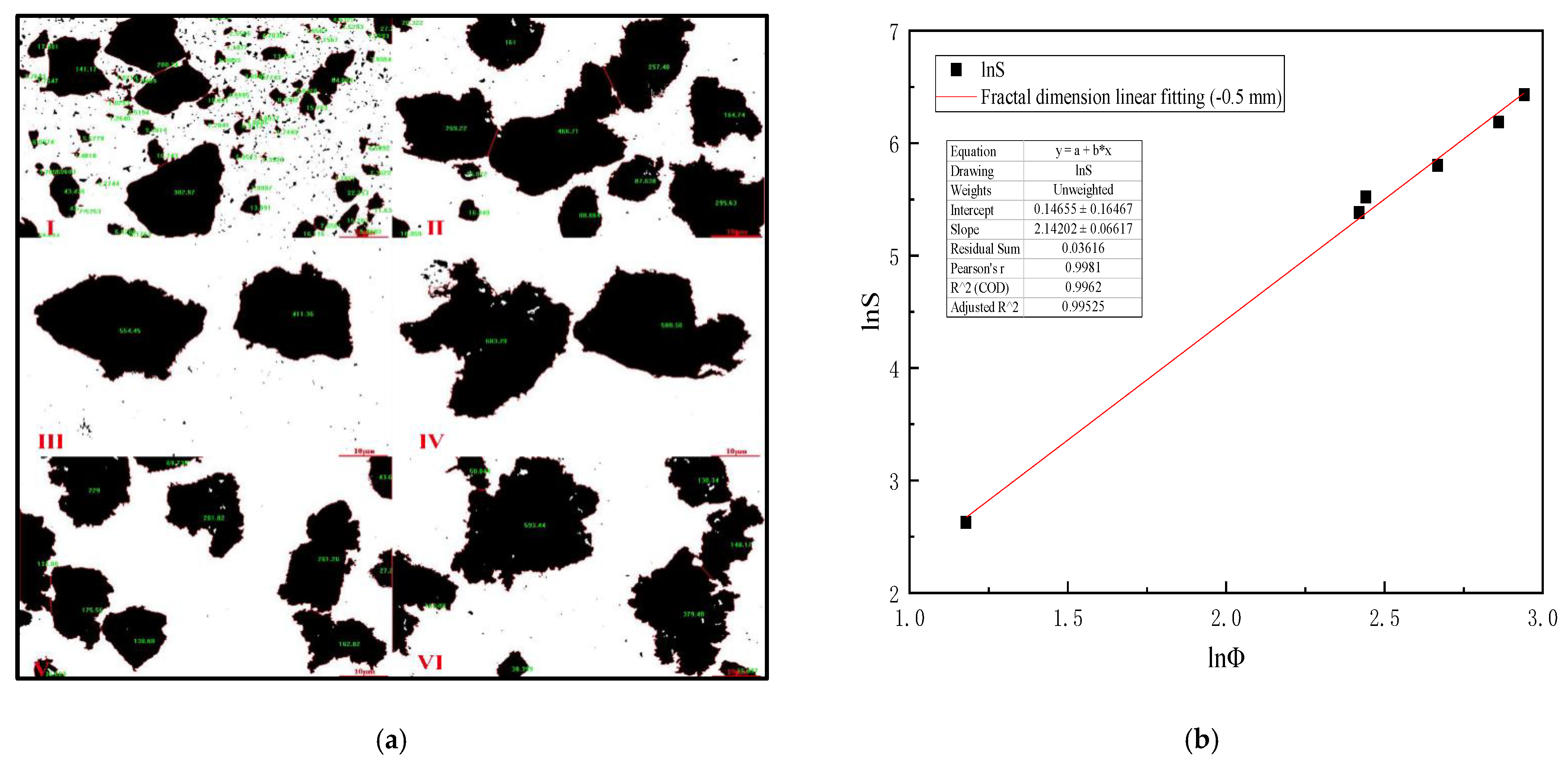
4.1. Mixing Speed versus Equivalent Diameter of Settling Floc (−0.5 mm Slime)
4.2. Mixing Speed versus Equivalent Diameter of Settling Floc (0.5–0.25 mm Slime)
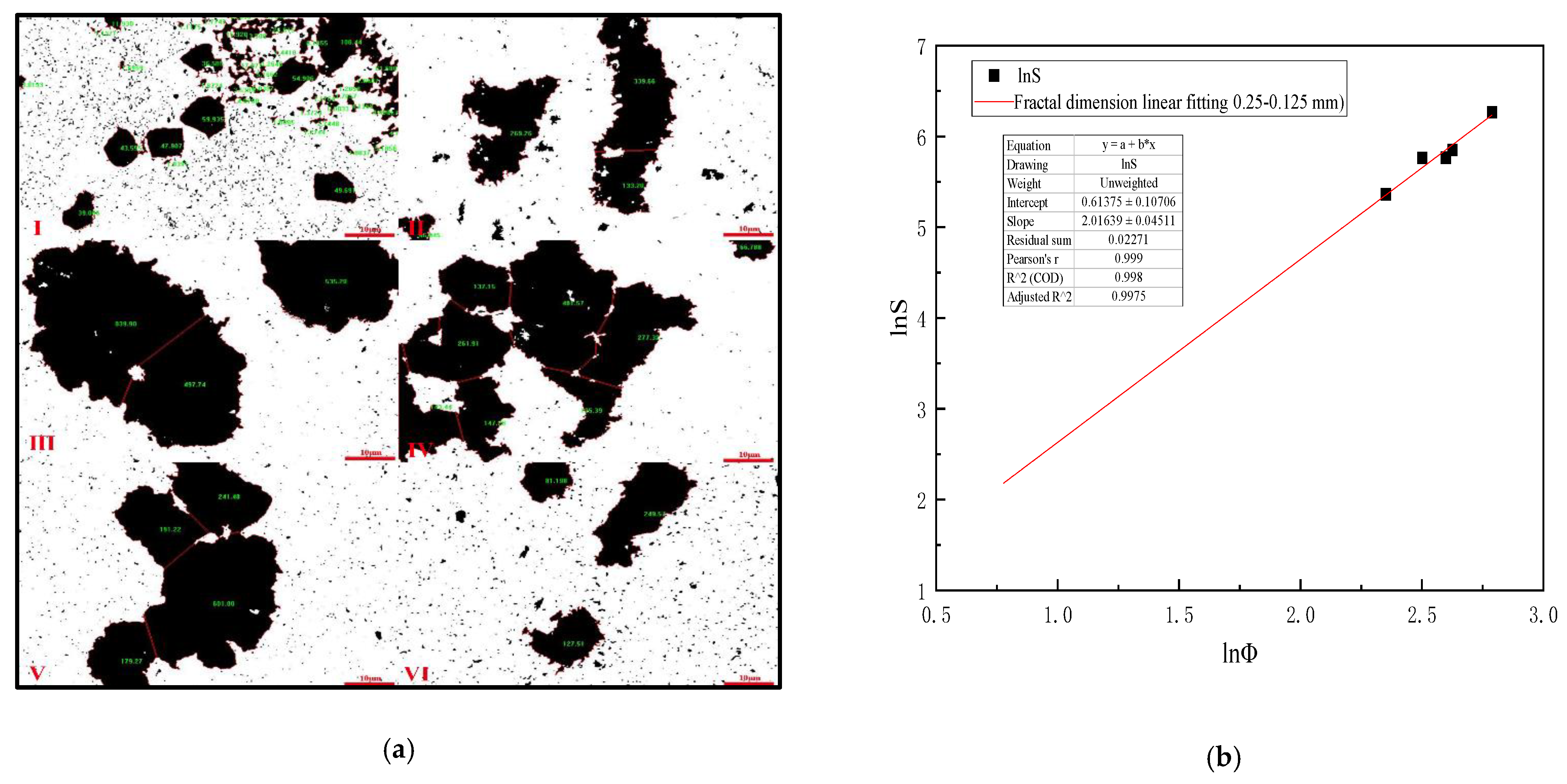
4.3. Mixing Speed versus Equivalent Diameter of Settling Floc (0.25–0.125 mm Slime)
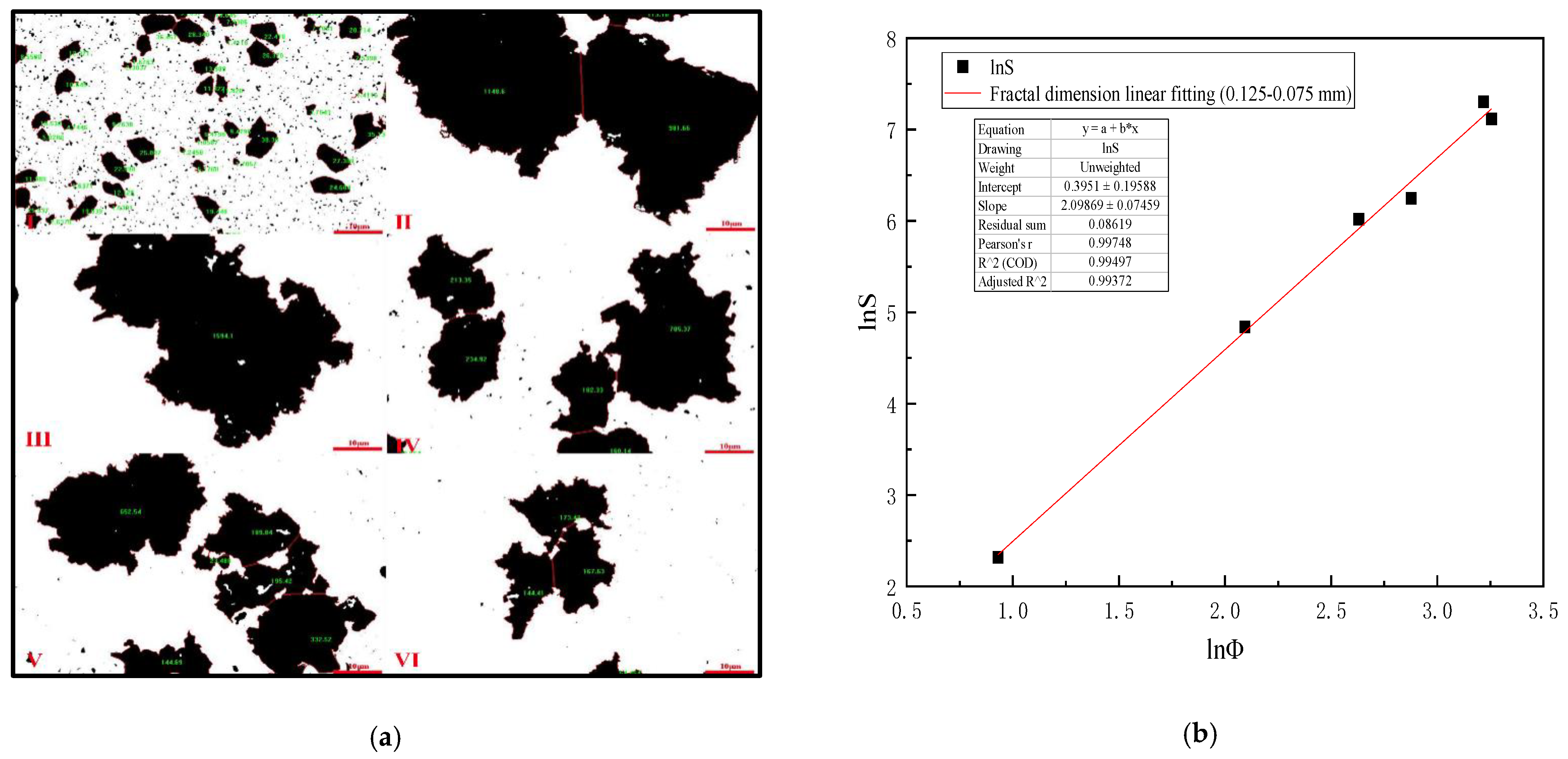
4.4. Mixing Speed versus Equivalent Diameter of Settling Floc (0.125–0.075 mm Slime)
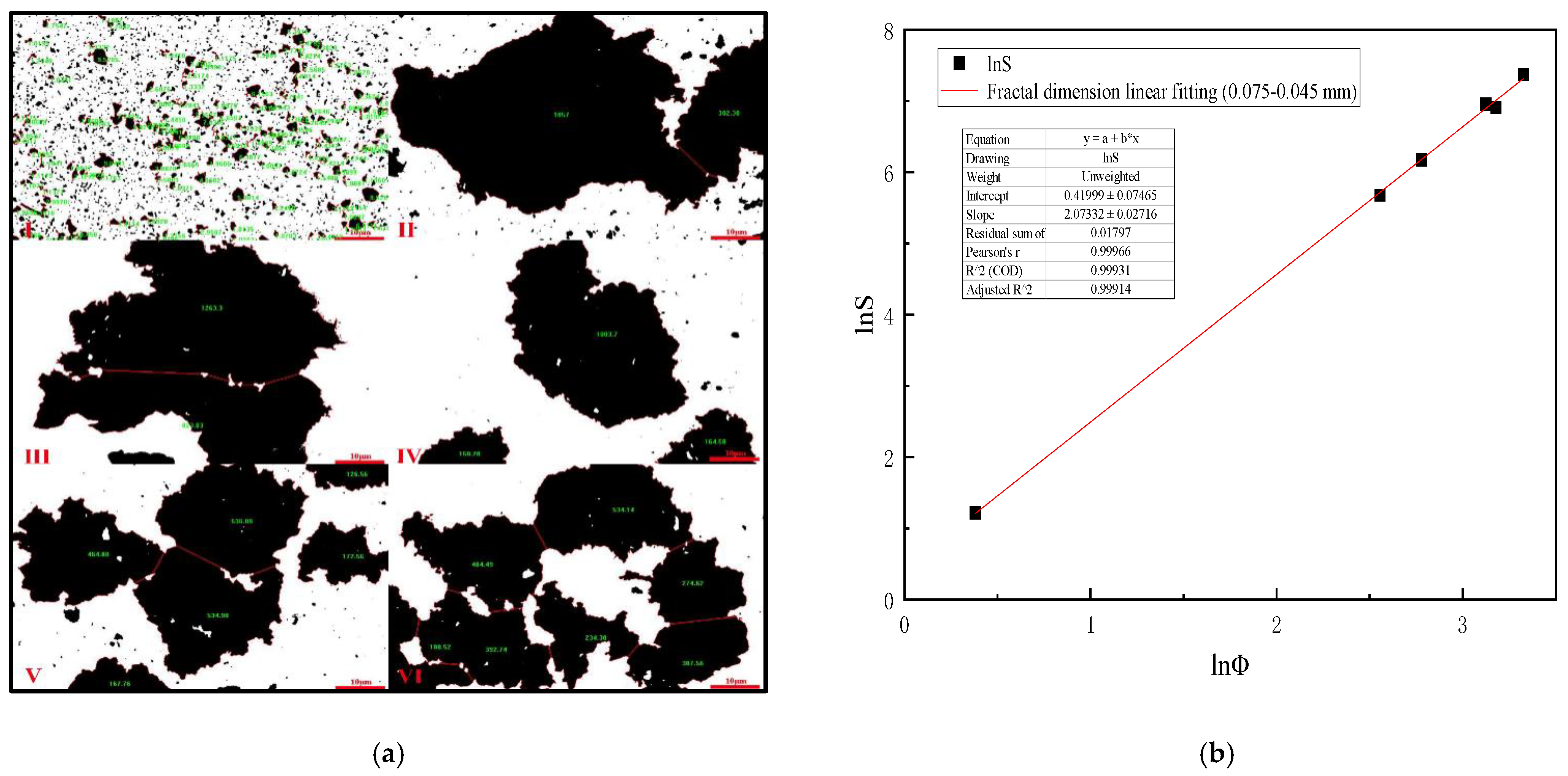
4.5. Mixing Speed versus Equivalent Diameter of Settling Floc (0.075–0.045 mm Slime)
4.6. Mixing Speed versus Equivalent Diameter of Settling Floc (−0.045 mm Slime)
4.7. Results and Discussion
- (1)
- When the mixing speed N* = 600 r/min (impeller linear velocity v* = 0.63 m/s), the equivalent diameter of settling floc of −0.5 mm slime is the largest; When N* = 200 r/min or 400 r/min (v* = 0.21 m/s or 0.42 m/s), the equivalent diameters of 0.5–0.25 mm, 0.25–0.125 mm, 0.125–0.075 mm, 0.75–0.045 mm and −0.045 mm slime settling floc are the largest.
- (2)
- The equivalent diameter is positively correlated with the size of slime, and the equivalent diameter of −0.045 mm slime settling floc is the largest; The size of slime is the dominant factor of fractal dimension change, and the fractal dimension of −0.5 mm slime settling floc is the largest.
- (3)
- When the mixing speed is 0–1000 r/min, the characteristic parameters are positively correlated with: statistical diameter and floc area; The negative correlations are as follows: particle size and floc area, floc perimeter, and statistical diameter.
5. Flocculant Dissolution—Stirring Time Derivation Method
5.1. Derivation Method and Purpose
5.2. Application Formula of Stirring Time Method
5.3. Contents of Derivation
5.4. Industrial Application of Stirring Time Derivation Method
6. Conclusions
- (1)
- The optimum stirring speed is 800 r/min for 3–6 million molecular weight APAM corresponds to an impeller linear velocity is 0.84 m/s; The optimum stirring speed is 600 r/min for 8–16 million molecular weight APAM corresponds to an impeller linear velocity is 0.63 m/s; The optimum stirring speed is 800 r/min for 18–25 million molecular weight APAM corresponds to an impeller linear velocity is 0.84 m/s. Through data fitting, the improved stirring power formula for APAM dissolution is .
- (2)
- When the stirring speed is 200 to 1600 r/min, the corresponding impeller linear velocity is 0.21 to 1.68 m/s, the positively correlated dissolution parameters are kinematic viscosity and molecular weight, Reynolds number and linear velocity, power number and molecular weight, stirring power and linear velocity, stirring power and molecular weight, stirring time and molecular weight; There are negative correlations between Reynolds number and molecular weight, power number and linear velocity, stirring time and linear velocity.
- (3)
- When the mixing speed is 600 r/min, the equivalent diameter of −0.5 mm slime settling floc is the largest. When the mixing speed is 200 r/min or 400 r/min, the equivalent diameters of 0.5–0.25 mm, 0.25–0.125 mm, 0.125–0.075 mm, 0.075–0.045 mm and −0.045 mm slime settling floc are the largest. The equivalent diameter is positively correlated with the size of slime, and the equivalent diameter of −0.045 mm slime settling floc is the largest. The size of slime is the dominant factor in the change of fractal dimension. The fractal dimension of −0.5 mm slime settling floc is the largest, and the fractal dimension is little affected by mixing speed.
- (4)
- The general formula of the relation between Reynolds number Re and power number K is: K = 10Re−0.5. The general formula of the relation between stirring power per unit volume Pv and stirring time T is: T = −1.4Pv + 69.4, and a flocculant dissolution—stirring time derivation method for different geometric parameters of agitator drum model or industrial agitator drum is proposed.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Wang, S.; Wei, M.; Tao, T.; Song, X.; Liu, W.; Yu, H. Internal structure evolution characteristics of high concentration unclassified tailings mortar containing APAM. Chin. J. Nonferrous Met. 2022, 32, 11. [Google Scholar] [CrossRef]
- Min, F.; Wang, T.; Ren, B.; Chen, J.; Zhu, J.; Zhang, M. Experimental and molecular simulation study of the adsorption behavior of APAM at water/kaolinite innterface. J. China Univ. Min. Technol. 2022, 51, 572–580. [Google Scholar] [CrossRef]
- Xia, W.; Li, Y.; Wu, F.; Niu, C. Enhanced flotation selectivity of fine coal from kaolinite by anionic polyacrylamide pre-conditioning. J. Mol. Liq. 2021, 334, 116083. [Google Scholar] [CrossRef]
- Blanco, Á.; Negro, C.; Fuente, E.; Tijero, J. Effect of Shearing Forces and Flocculant Overdose on Filler Flocculation Mechanisms and Floc Properties. Ind. Eng. Chem. Res. 2005, 44, 9105–9112. [Google Scholar] [CrossRef]
- Yoon, S.; Deng, Y. Flocculation and reflocculation of clay suspension by different polymer systems under turbulent conditions. J. Colloid Interface Sci. 2004, 278, 139–145. [Google Scholar] [CrossRef] [PubMed]
- Garmsiri, M.R.; Haji, H. The effect of grain size on flocculant preparation. Miner. Eng. 2014, 65, 51–53. [Google Scholar] [CrossRef]
- Owen, A.T.; Fawell, P.D.; Swift, J.D. The preparation and ageing of acrylamide/acrylate copolymer flocculant solutions. Int. J. Miner. Process. 2007, 84, 3–14. [Google Scholar] [CrossRef]
- Owen, A.T.; Fawell, P.D.; Swift, J.D.; Farrow, J.B. The impact of polyacrylamide flocculant solution age on flocculation performance. Int. J. Miner. Process. 2002, 67, 123–144. [Google Scholar] [CrossRef]
- Waters, A.G. The Importance of Mixing in the Sedimentation of Flocculated Coal Washery Slurries. Coal Prep. 1985, 2, 35–49. [Google Scholar] [CrossRef]
- Nagata, S. Mixing Principle and Application; Ma, J., Translator; Chemical Industry Press: Beijing, China, 1991. [Google Scholar]


| Molecular Weight /(Million) | Stirring Speed N/(r/min) | Linear Velocity v/(m/s) | Stirring Time T/(min) | Kinematic Viscosity γ/(×10−3m2/s) | Reynolds Number Re | Power Number K | Stirring Power per Unit Volume Pv/(kW/m3) | Energy Input per Unit Volume Ev/(×103kJ/m3) |
|---|---|---|---|---|---|---|---|---|
| 3 | 800 | 0.84 | 65.50 | 1.32 | 12.73 | 2.57 | 1.94 | 7.62 |
| 6 | 800 | 0.84 | 64.27 | 1.53 | 10.98 | 2.74 | 2.06 | 7.94 |
| 8 | 600 | 0.63 | 65.28 | 1.69 | 7.46 | 3.35 | 1.06 | 4.15 |
| 10 | 600 | 0.63 | 68.63 | 1.63 | 7.73 | 3.29 | 1.04 | 4.28 |
| 12 | 600 | 0.63 | 68.27 | 1.63 | 7.73 | 3.29 | 1.05 | 4.30 |
| 16 | 600 | 0.63 | 69.48 | 2.15 | 5.86 | 3.87 | 1.22 | 5.09 |
| 18 | 800 | 0.84 | 65.23 | 2.34 | 7.18 | 3.43 | 2.58 | 10.10 |
| 20 | 800 | 0.84 | 65.82 | 2.04 | 8.24 | 3.17 | 2.39 | 9.44 |
| 22 | 800 | 0.84 | 66.63 | 1.93 | 8.70 | 3.08 | 2.31 | 9.23 |
| 25 | 800 | 0.84 | 67.25 | 2.30 | 7.30 | 3.39 | 2.54 | 10.25 |
| APAM Molecular Weight /(Million) | Optimal Stirring Speed /(r/min) | Optimal Linear Velocity /(m/s) | Optimal Stirring Time /(min) | Optimal Kinematic Viscosity /(×10−3m2/s) | Stirring Power per Unit Volume /(kW/m3) | Energy Input per Unit Volume /(×103kJ/m3) |
|---|---|---|---|---|---|---|
| 3–6 | 800 | 0.84 | 64–66 | 1.3–1.6 | 1.9–2.1 | 7.6–8.0 |
| 8–16 | 600 | 0.63 | 65–70 | 1.6–2.2 | 1.0–1.3 | 4.1–5.1 |
| 18–25 | 800 | 0.84 | 65–68 | 1.9–2.4 | 2.3–2.6 | 9.2–10.3 |
| APAM Stirring Speed /(r/min) | APAM Stirring Time /(min) | Time of Interface Settlement /s | Velocity of Free Settlement /(m/h) | Degree of Clarification after 30 min /% | Height of Compression Layers /mm | APAM Volume Dosage /(mL/L) | APAM Solid Dosage /(g/t) |
|---|---|---|---|---|---|---|---|
| 200 | 72.40 | 32.77 | 13.77 | 23.2 | 42.7 | 3.2–3.6 | 16–18 |
| 400 | 70.12 | 30.02 | 14.92 | 30.8 | 45.2 | 3.2–3.6 | 16–18 |
| 600 | 68.03 | 29.98 | 14.93 | 36.5 | 45.1 | 3.2–4.0 | 16–20 |
| 800 | 65.23 | 19.82 | 22.66 | 43.0 | 48.1 | 3.2–3.6 | 16–18 |
| 1000 | 62.42 | 25.88 | 16.92 | 38.6 | 46.6 | 3.2–4.0 | 16–20 |
| 1200 | 60.15 | 27.49 | 16.53 | 35.6 | 44.8 | 2.8–3.2 | 14–16 |
| 1400 | 56.30 | 28.10 | 16.45 | 33.1 | 44.2 | 2.4–2.8 | 12–14 |
| 1600 | 52.42 | 30.67 | 14.60 | 31.2 | 41.3 | 3.2–3.6 | 16–18 |
| Slime Size/mm | Percentage of This Level/% | Ash/% |
|---|---|---|
| −0.5 | 100 | 44.30 |
| 0.5–0.25 | 52.17 | 39.20 |
| 0.25–0.125 | 20.03 | 45.26 |
| 0.125–0.075 | 21.45 | 46.93 |
| 0.075–0.045 | 6.29 | 49.60 |
| −0.045 | 0.06 | 40.50 |
| Mixing Speed N*/(r/min) | Floc Area S/μm2 | Floc Perimeter C/μm | Floc Aspect Ratio | Mesopore Area S0/μm2 | Equivalent Diameter Φ/μm | Statistical Diameter D*/μm | Floc Fractal Dimension |
|---|---|---|---|---|---|---|---|
| 0 | 13.844 | 13.517 | 1.764 | 0.020 | 3.247 | 2.952 | 2.106 |
| 200 | 332.100 | 100.191 | 1.418 | 0.614 | 14.405 | 19.691 | 2.121 |
| 400 | 487.159 | 117.184 | 1.465 | 0.900 | 17.455 | 23.625 | 2.113 |
| 600 | 621.536 | 147.912 | 1.453 | 1.919 | 18.938 | 27.140 | 2.137 |
| 800 | 250.463 | 97.007 | 1.573 | 1.709 | 11.487 | 16.916 | 2.202 |
| 1000 | 217.873 | 82.418 | 1.523 | 1.219 | 11.246 | 15.504 | 2.164 |
| Mixing Speed N*/(r/min) | Floc Area S/μm2 | Floc Perimeter C/μm | Floc Aspect Ratio | Mesopore Area S0/μm2 | Equivalent Diameter Φ/μm | Statistical Diameter D*/μm | Floc Fractal Dimension |
|---|---|---|---|---|---|---|---|
| 0 | 8.796 | 15.154 | 1.911 | 0.155 | 2.140 | 2.515 | 1.893 |
| 200 | 210.463 | 71.005 | 1.472 | 0.643 | 11.832 | 15.733 | 1.868 |
| 400 | 232.714 | 90.271 | 1.454 | 1.049 | 11.588 | 16.437 | 1.925 |
| 600 | 161.343 | 71.092 | 1.418 | 0.924 | 9.952 | 13.533 | 1.893 |
| 800 | 147.104 | 70.947 | 1.528 | 1.287 | 9.051 | 12.741 | 1.933 |
| 1000 | 133.455 | 57.871 | 1.596 | 0.744 | 9.026 | 11.729 | 1.891 |
| Mixing Speed N*/(r/min) | Floc Area S/μm2 | Floc Perimeter C/μm | Floc Aspect Ratio | Mesopore Area S0/μm2 | Equivalent Diameter Φ/μm | Statistical Diameter D*/μm | Floc Fractal Dimension |
|---|---|---|---|---|---|---|---|
| 0 | 8.809 | 15.046 | 1.855 | 0.124 | 2.174 | 2.603 | 2.011 |
| 200 | 318.213 | 117.278 | 1.731 | 1.450 | 12.192 | 18.481 | 2.059 |
| 400 | 526.273 | 134.425 | 1.765 | 1.172 | 16.253 | 24.452 | 2.027 |
| 600 | 347.649 | 104.718 | 1.638 | 0.835 | 13.801 | 19.316 | 1.995 |
| 800 | 319.651 | 97.070 | 1.630 | 0.480 | 13.441 | 18.969 | 1.983 |
| 1000 | 213.312 | 87.115 | 1.663 | 0.698 | 10.501 | 15.223 | 2.019 |
| Mixing Speed N*/(r/min) | Floc Area S/μm2 | Floc Perimeter C/μm | Floc Aspect Ratio | Mesopore Area S0/μm2 | Equivalent Diameter Φ/μm | Statistical Diameter D*/μm | Floc Fractal Dimension |
|---|---|---|---|---|---|---|---|
| 0 | 10.155 | 13.978 | 1.784 | 0.019 | 2.534 | 3.151 | 2.068 |
| 200 | 1234.052 | 209.218 | 1.559 | 3.533 | 25.911 | 38.391 | 2.066 |
| 400 | 1488.903 | 294.515 | 1.756 | 9.195 | 24.941 | 41.258 | 2.149 |
| 600 | 516.473 | 129.028 | 1.410 | 3.016 | 17.747 | 24.637 | 2.035 |
| 800 | 412.032 | 127.182 | 1.811 | 2.416 | 13.876 | 21.151 | 2.139 |
| 1000 | 126.904 | 64.890 | 1.710 | 0.788 | 8.110 | 11.986 | 2.125 |
| Mixing Speed N*/(r/min) | Floc Area S/μm2 | Floc Perimeter C/μm | Floc Aspect Ratio | Mesopore Area S0/μm2 | Equivalent Diameter Φ/μm | Statistical Diameter D*/μm | Floc Fractal Dimension |
|---|---|---|---|---|---|---|---|
| 0 | 3.379 | 8.300 | 1.708 | 0.021 | 1.463 | 1.930 | 2.096 |
| 200 | 1602.686 | 261.753 | 1.660 | 1.841 | 27.892 | 43.195 | 2.091 |
| 400 | 1054.716 | 194.764 | 1.782 | 2.550 | 22.729 | 33.940 | 2.094 |
| 600 | 1008.134 | 187.500 | 1.474 | 2.127 | 24.032 | 34.498 | 2.043 |
| 800 | 481.434 | 133.881 | 1.542 | 2.811 | 16.085 | 23.397 | 2.072 |
| 1000 | 293.505 | 93.803 | 1.612 | 1.128 | 12.876 | 18.047 | 2.059 |
| Mixing Speed N*/(r/min) | Floc Area S/μm2 | Floc Perimeter C/μm | Floc Aspect Ratio | Mesopore Area S0/μm2 | Equivalent Diameter Φ/μm | Statistical Diameter D*/μm | Floc Fractal Dimension |
|---|---|---|---|---|---|---|---|
| 0 | 2.354 | 8.692 | 1.909 | 0.027 | 1.066 | 1.637 | 1.958 |
| 200 | 1358.856 | 197.174 | 1.670 | 4.624 | 27.597 | 39.726 | 1.954 |
| 400 | 2143.444 | 285.286 | 1.436 | 4.370 | 34.865 | 51.044 | 1.954 |
| 600 | 702.405 | 162.639 | 1.740 | 1.173 | 18.558 | 28.231 | 1.994 |
| 800 | 521.996 | 100.775 | 1.317 | 1.514 | 20.422 | 24.848 | 1.832 |
| 1000 | 450.453 | 132.859 | 1.550 | 2.575 | 15.384 | 23.013 | 1.968 |
| Molecular Weight /(Million) | Re versus K Power Fitting Relationship | Pv versus T Linear Fitting Relationship |
|---|---|---|
| 3 | K = 9.3797Re−0.487 | T = −1.5359Pv + 68.71 |
| 6 | K = 9.8157Re−0.512 | T = −1.0376Pv + 66.148 |
| 8 | K = 9.6223Re−0.5 | T = −1.1622Pv + 66.272 |
| 10 | K = 10.018Re−0.521 | T = −1.5059Pv + 69.669 |
| 12 | K = 10.3Re−0.535 | T = −1.6264Pv + 68.53 |
| 16 | K = 10.397Re−0.544 | T = −1.4681Pv + 70.688 |
| 18 | K = 10.486Re−0.55 | T = −1.3668Pv + 69.917 |
| 20 | K = 10.122Re−0.534 | T = −1.5372Pv + 70.586 |
| 22 | K = 9.952Re−0.526 | T = −1.6101Pv + 71.961 |
| 25 | K = 10.048Re−0.532 | T = −1.1669Pv + 71.84 |
| Average Fitting General Formula | K = 10Re−0.5 | T = −1.4Pv + 69.4 |
| Core Components | Diameter/cm | Length, Width, Height/cm | Note |
|---|---|---|---|
| Impeller | 16 | 10 × 8 × 6 | Number 3, Angle 36° |
| Mixing drum | 68 | 68 × 68 × 68 | Treatment 0.2 m3 |
| Stirring rod | 10 | 10 × 6 × 64 | Connecting impeller |
| Motor | 26 | High 30 | Fixed speed 1440 r/min |
| Reduction gear | 26 | High 23 | Increase output torque |
| Coupling | Upper 26, Lower 30 | High 25 | Cushioning and damping |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, J.; Zhou, W.; Cai, C.; Wang, S.; Zhu, J. Effect of Agitation on the Dissolution of APAM with Different Molecular Weights and the Equivalent Diameter of Coal Slime Settling Floc with Different Particle Sizes. Minerals 2023, 13, 204. https://doi.org/10.3390/min13020204
Li J, Zhou W, Cai C, Wang S, Zhu J. Effect of Agitation on the Dissolution of APAM with Different Molecular Weights and the Equivalent Diameter of Coal Slime Settling Floc with Different Particle Sizes. Minerals. 2023; 13(2):204. https://doi.org/10.3390/min13020204
Chicago/Turabian StyleLi, Jianbo, Wei Zhou, Chuanchuan Cai, Shujie Wang, and Jinbo Zhu. 2023. "Effect of Agitation on the Dissolution of APAM with Different Molecular Weights and the Equivalent Diameter of Coal Slime Settling Floc with Different Particle Sizes" Minerals 13, no. 2: 204. https://doi.org/10.3390/min13020204
APA StyleLi, J., Zhou, W., Cai, C., Wang, S., & Zhu, J. (2023). Effect of Agitation on the Dissolution of APAM with Different Molecular Weights and the Equivalent Diameter of Coal Slime Settling Floc with Different Particle Sizes. Minerals, 13(2), 204. https://doi.org/10.3390/min13020204




