Decoupling Method for the Convective-Dominated Leaching Process of Ion-Adsorption-Type Rare-Earth Ores
Abstract
1. Introduction
2. Experimental
2.1. Ore Samples
2.2. Grade Experiment of Ore Samples
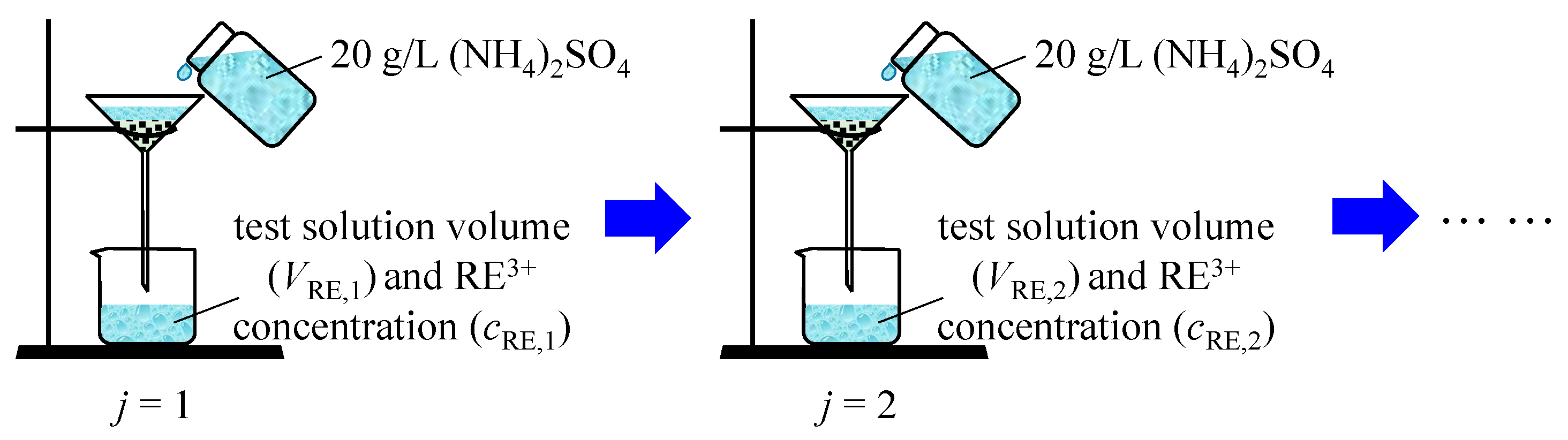
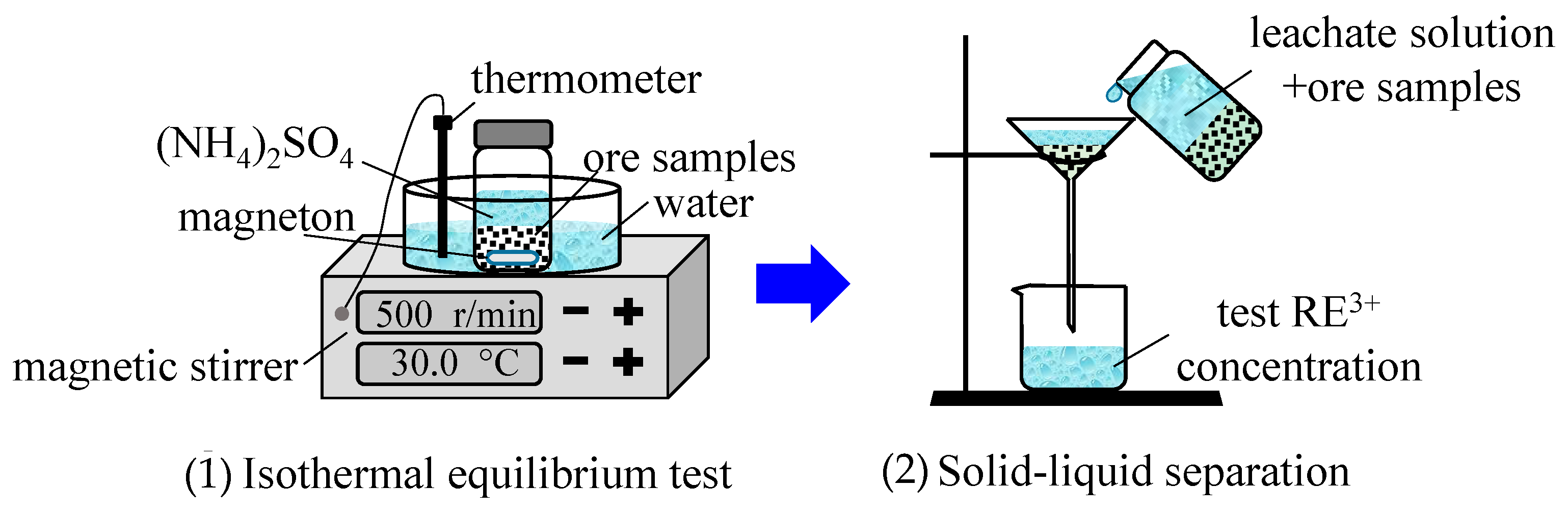
2.3. Ion Exchange Equilibrium Experiment
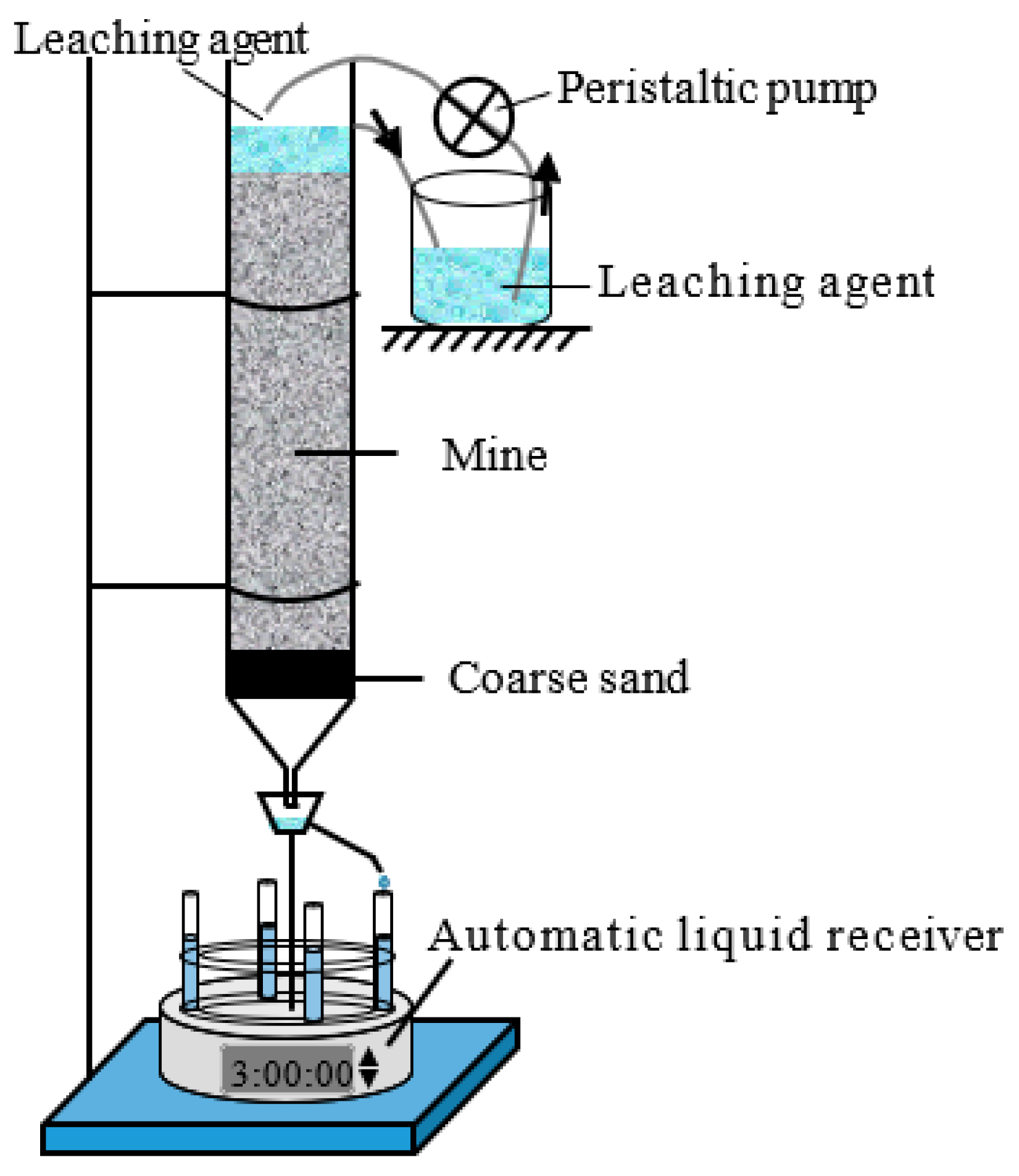
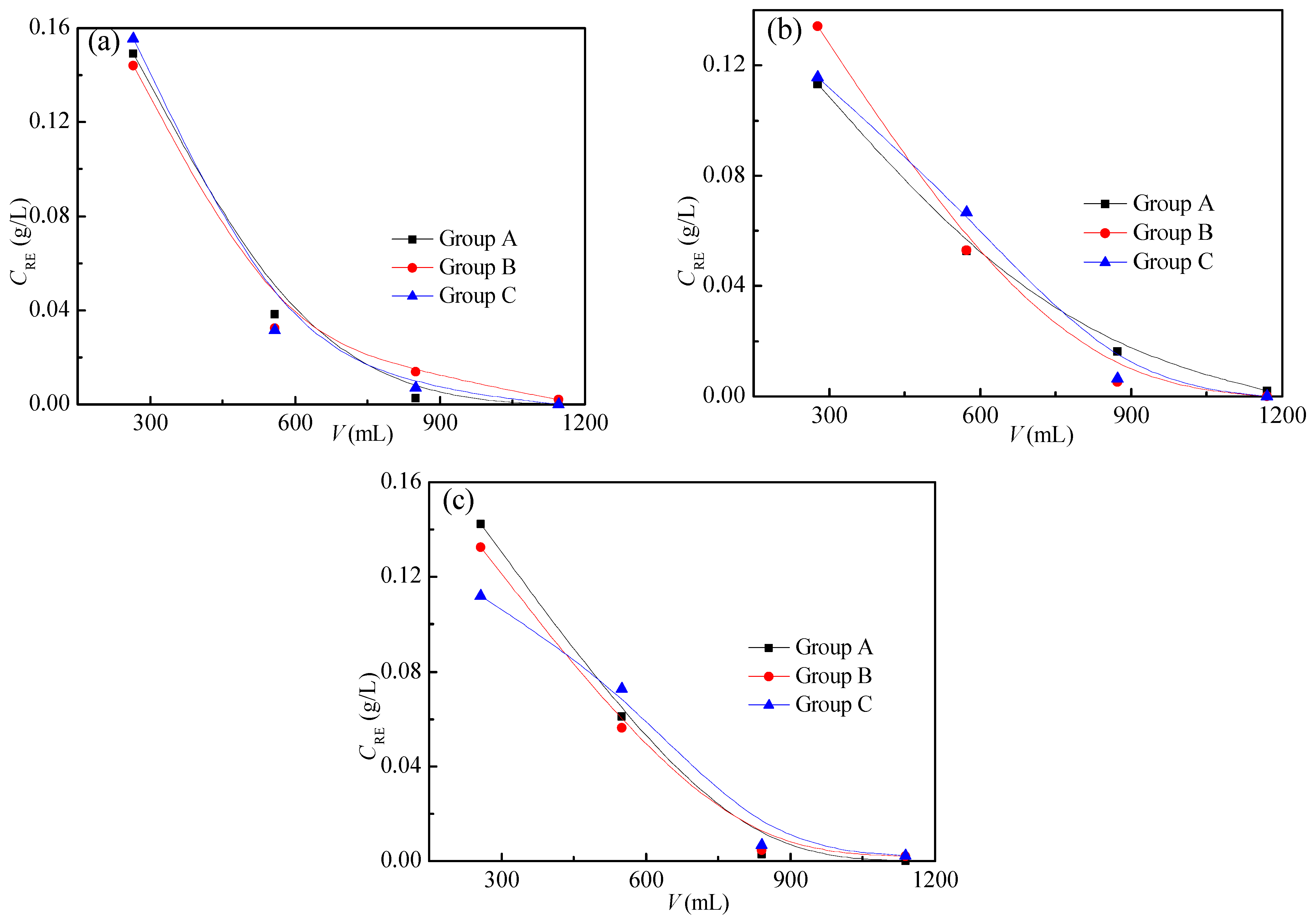
2.4. Column Leaching Experiment
3. Modelling
3.1. Ion-Exchange Equilibrium Model
3.2. Solute Transport Parameters Determination
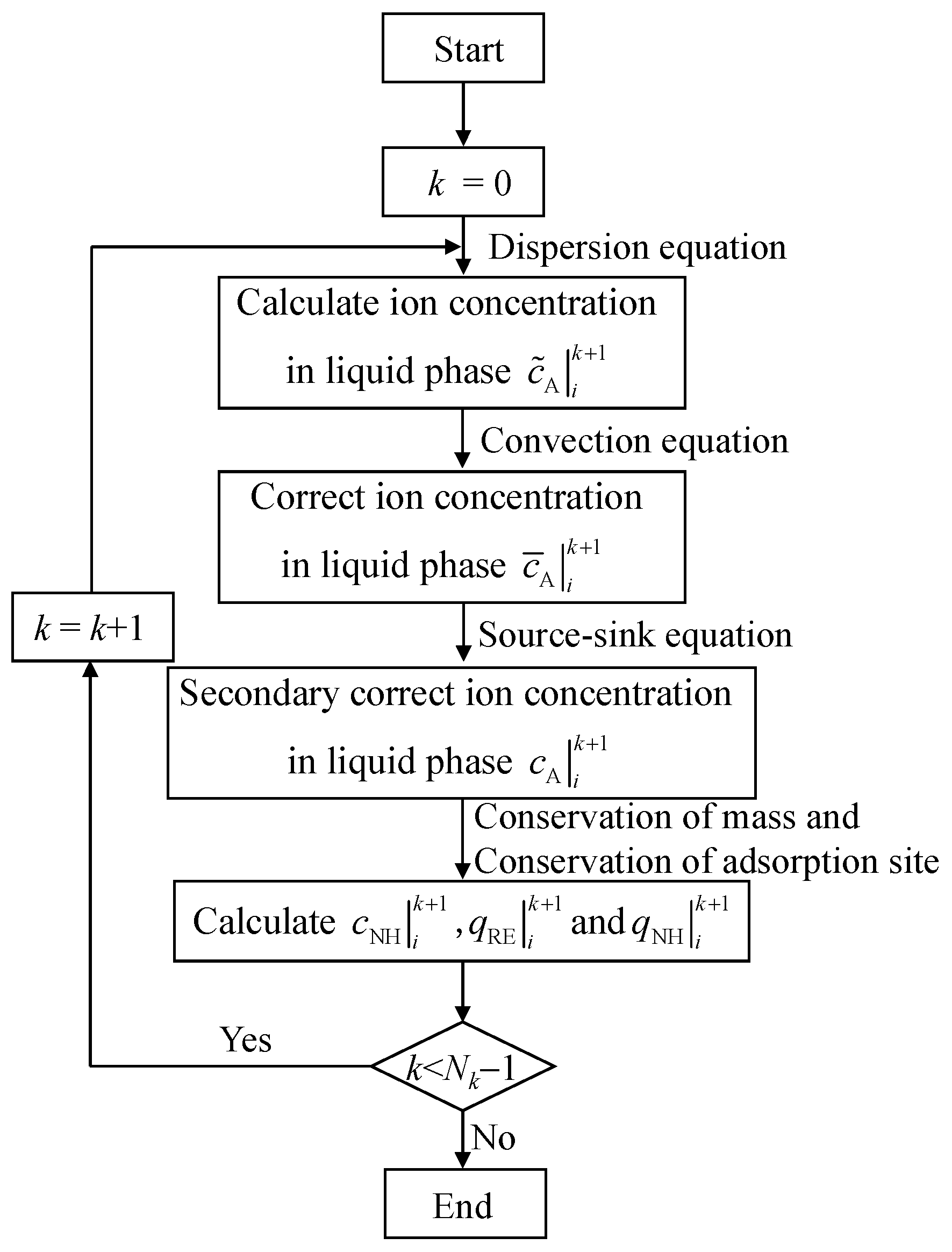
3.3. Decoupling Method
4. Results and Discussion
4.1. Relative Molecular Weight and Valence of the Rare-Earth Ions
4.2. Grades of Ore Samples
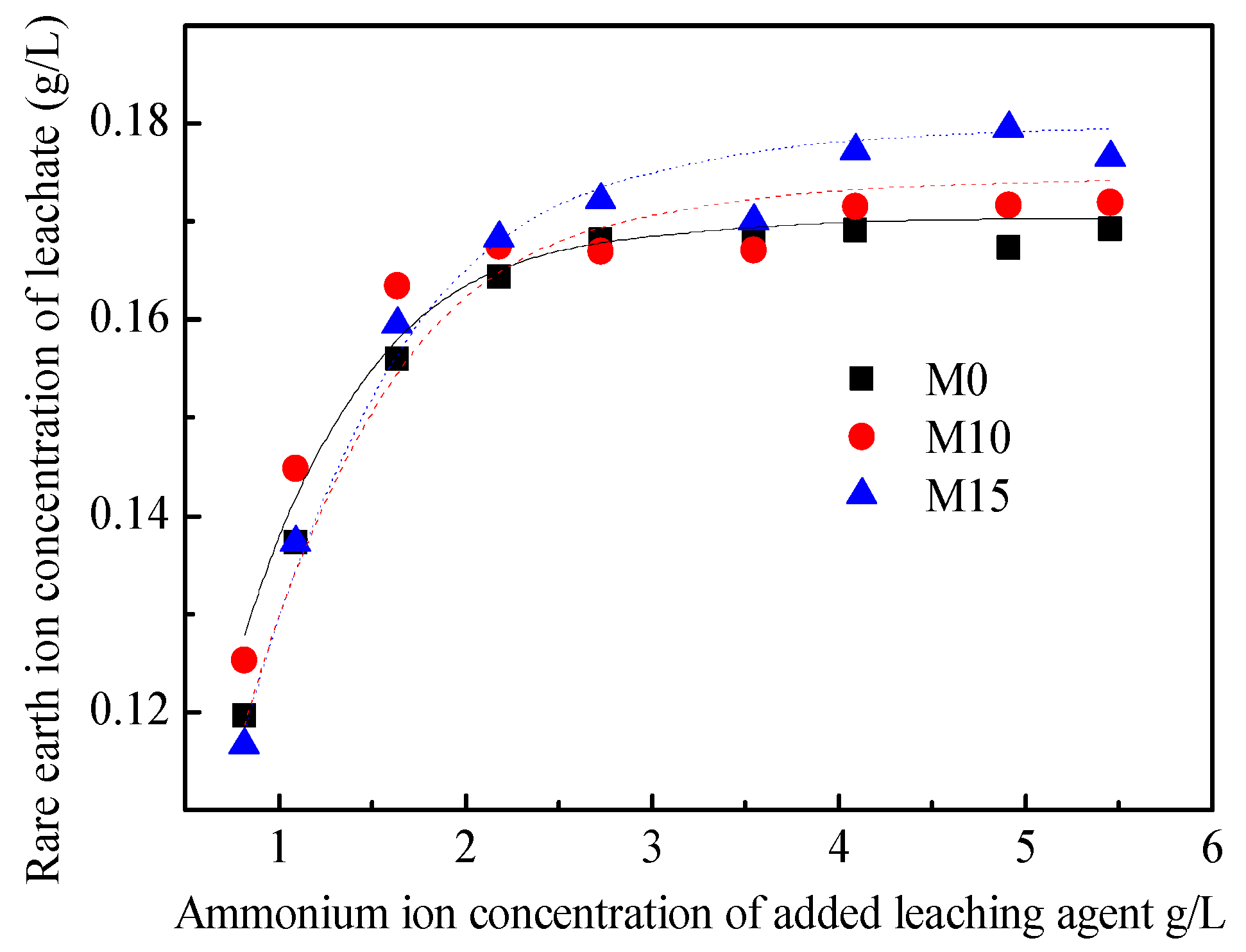
4.3. Ion Exchange Selectivity Coefficient
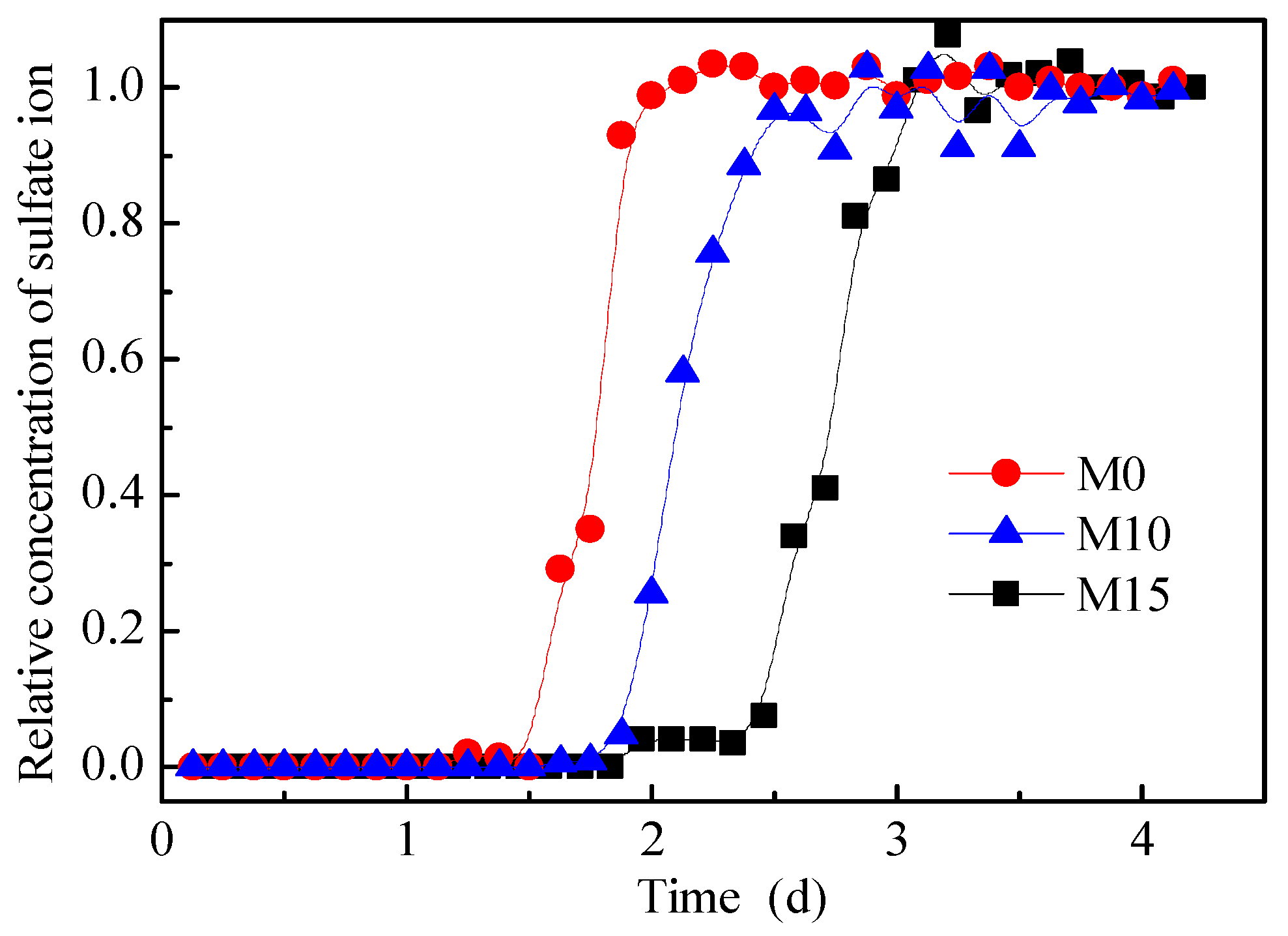
4.4. Determining u and D
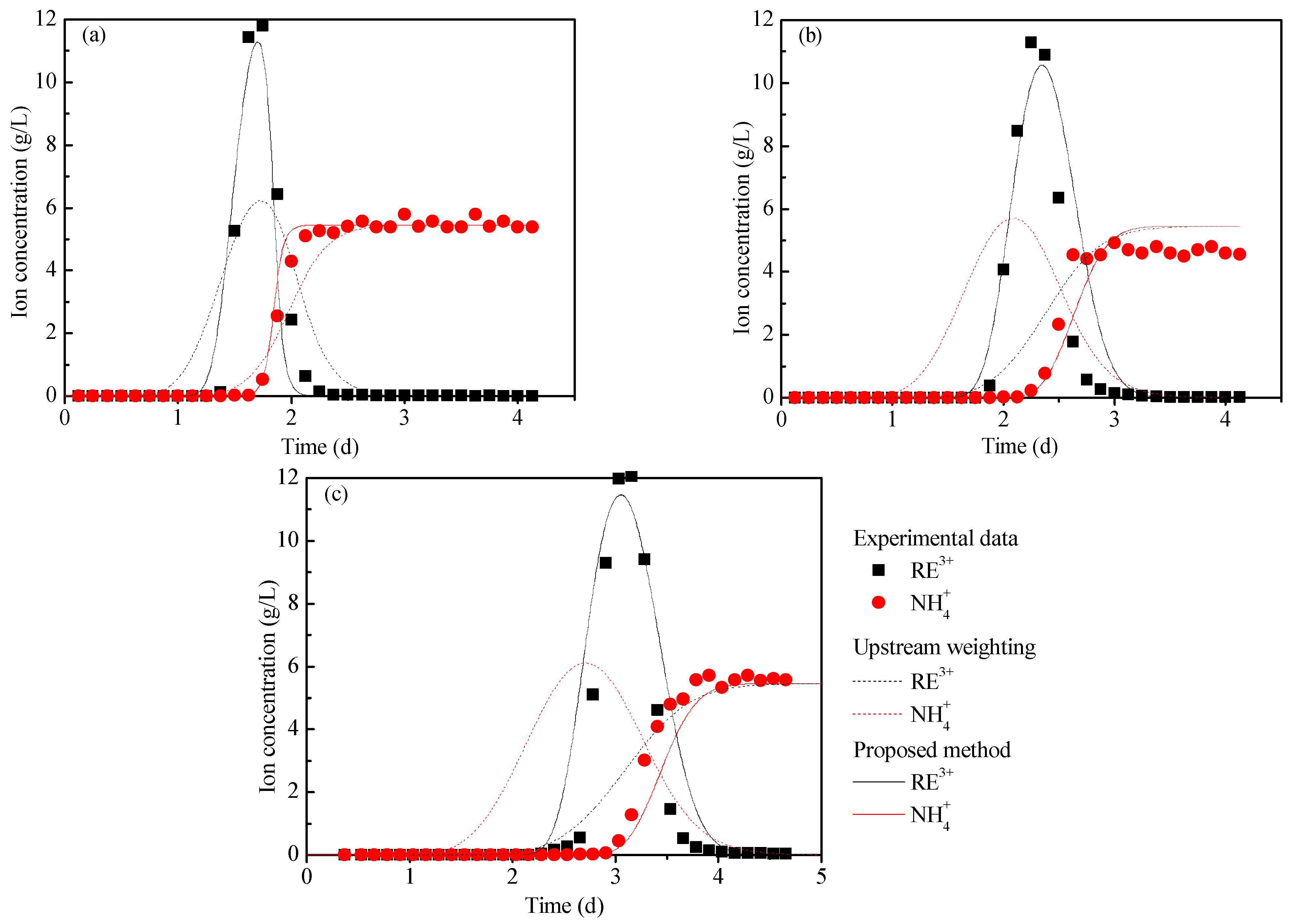
4.5. Model Verification
4.5.1. Theoretical Verification
4.5.2. Experimental Verification
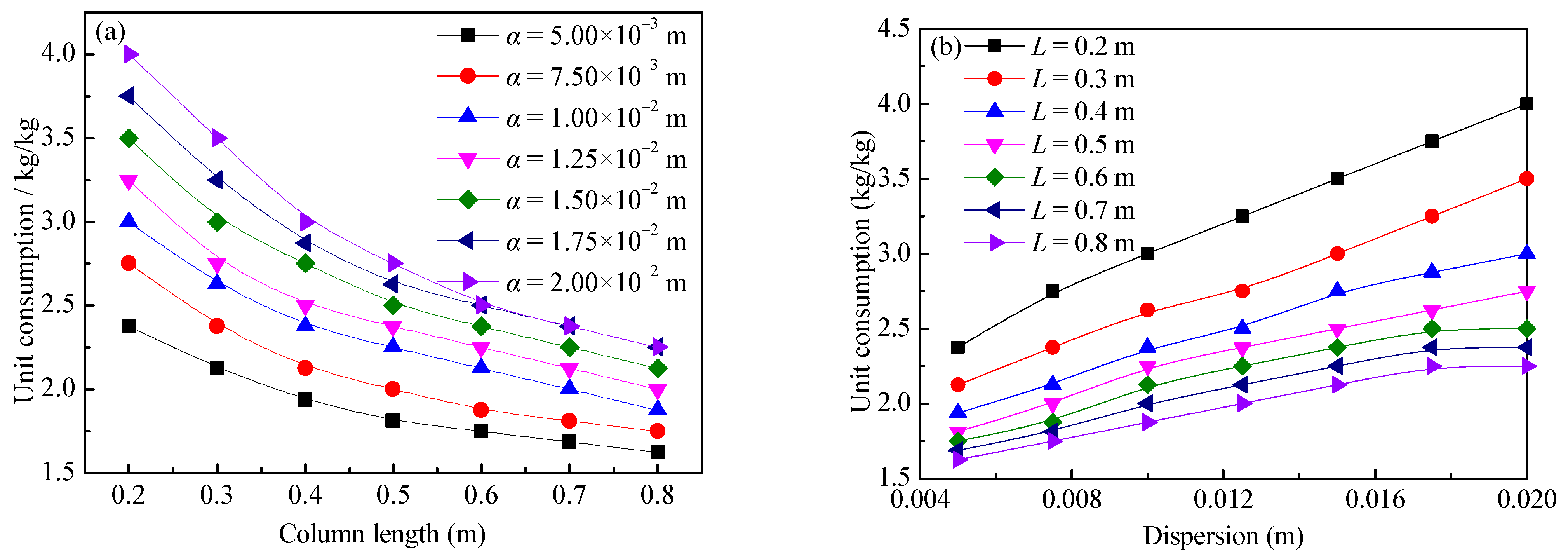
4.6. Optimization of Leaching Agent Consumption
- (1)
- When η0 (the rare-earth leaching rate) ∈ [ηaim − σ, ηaim + σ], the calculation was completed if the result of the preset leaching agent consumption was calculated to be . When the rare-earth leaching rate η < ηaim − σ, the preset leaching agent consumption was updated to . When the rare-earth leaching rate η > ηaim + σ, the preset leaching agent consumption was updated to be ; by using as the amount of leaching agent, the rare-earth leaching rate η1 was then recalculated.
- (2)
- When the rare-earth leaching rate (η0 − ηaim)(η1 − ηaim) < 0, the dichotomy method was used to update the preset leaching agent consumption, otherwise, step (1) was repeated. The abovementioned process was repeated until the rare-earth leaching rate η ∈ [ηaim − σ, ηaim + σ]; the leaching agent consumption was then determined.
5. Conclusions
- (1)
- In this paper, a decoupling method is proposed to solve the solute transport equation. In detail, the solute transport is decoupled into the dispersion, convection, and source-sink equations. The dispersion equation is used to calculate the distribution of the ion concentration, and the convection equation and the source-sink equation are then used to correct the ion concentration.
- (2)
- In comparison to the analytical solutions of the two convection-dominated problems, the upstream weighting method can solve the oscillation problem, but this still incurs a large dispersion error. The method proposed in this paper is in good agreement with the analytical solution, which shows that the proposed method can solve both the oscillation and dispersion problems.
- (3)
- By comparing the simulation results of the leaching process through the proposed method with the experimental data, it can be found that the coefficients of determination of the breakthrough curves of rare-earth ions and ammonium ions are greater than 0.850 and 0.900, respectively, and the peak concentration error of rare-earth ions is less than 7.00%, indicating that the proposed method can simulate the leaching process of IATREOs well.
- (4)
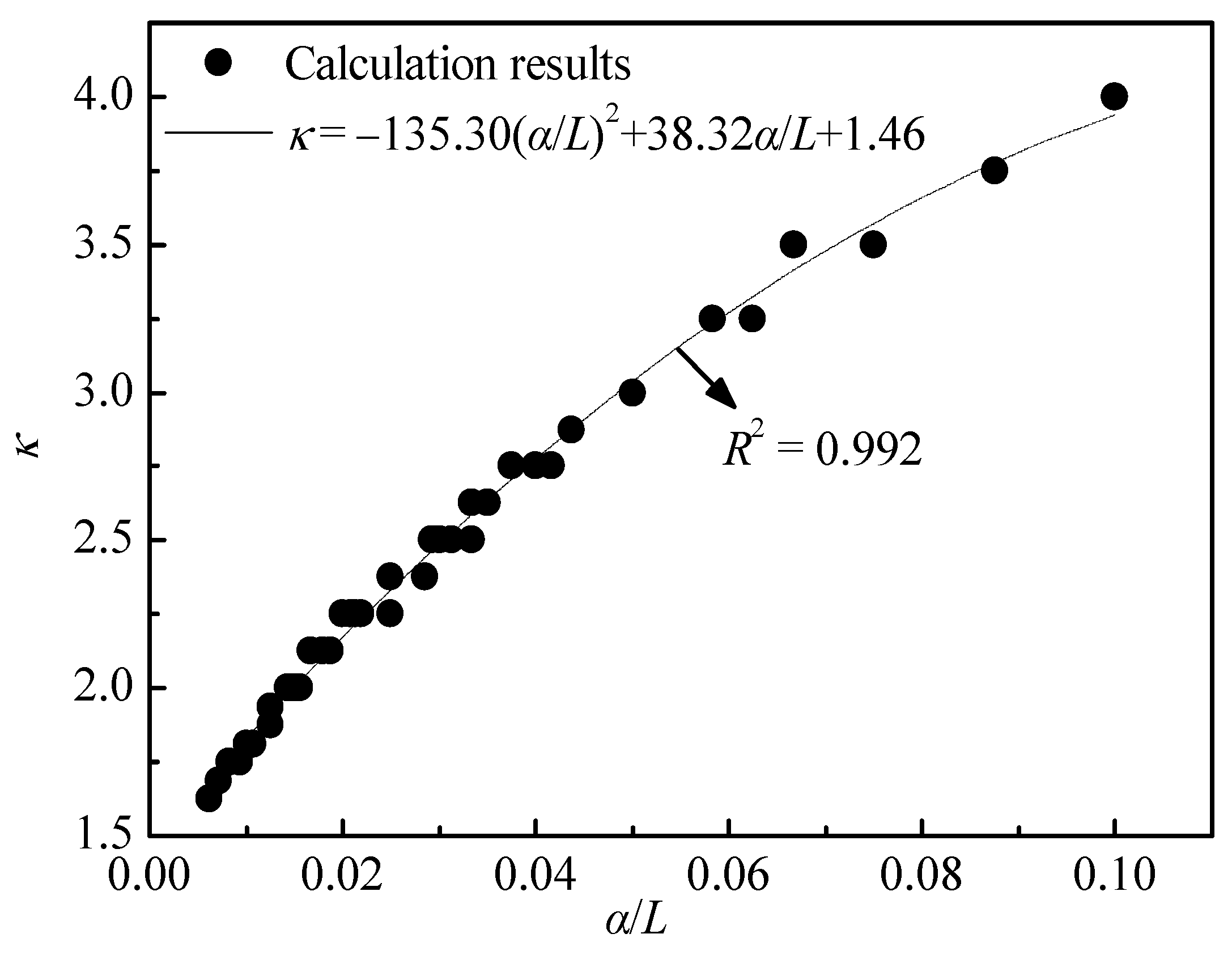
- The leaching agent unit consumption κ is inversely proportional to the column length L, and it has a positive correlation with the dispersion α. The mathematical relationship between the three is κ = −135.30(α/L)2 + 38.32α/L + 1.46.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Guo, Z.Q.; Zhou, J.R.; Zhou, K.F.; Jin, J.F.; Wang, X.J.; Zhao, K. Soil−water characteristics of weathered crust elution-deposited rare earth ores. Trans. Nonferrous Met. Soc. 2021, 31, 1452–1464. [Google Scholar] [CrossRef]
- Xiao, Y.F.; Huang, X.W.; Feng, Z.Y.; Dong, J.S.; Huang, L.; Long, Z.Q. Progress in the green extraction technology for rare earth from ion-adsorption type rare earths ore. Chin. Rare Earths 2015, 36, 109–115. [Google Scholar]
- Chai, X.W.; Li, G.Q.; Zhang, Z.Y.; Chi, R.A.; Chen, Z. Leaching Kinetics of Weathered Crust Elution-Deposited Rare Earth Ore with Compound Ammonium Carboxylate. Minerals 2020, 10, 516. [Google Scholar] [CrossRef]
- Moldoveanu, G.A.; Papangelakis, V.G. An overview of rare-earth recovery by ion-exchange leaching from ion-adsorption clays of various origins. Mineral. Mag. 2016, 80, 63–76. [Google Scholar] [CrossRef]
- Qiu, S.; Yan, H.S.; Hong, B.G.; Long, Q.B.; Xiao, J.; Li, F.J.; Tong, L.C.; Zhou, X.W.; Qiu, T.S. Desorption of REEs from Halloysite and Illite: A Link to the Exploitation of Ion-Adsorption RE Ore Based on Clay Species. Minerals 2022, 12, 1003. [Google Scholar] [CrossRef]
- Qiu, T.S.; Zhu, D.M.; Wu, C.Y.; Wang, L.M. Lattice Boltzmann model for simulation on leaching process of weathered elution-deposited rare earth ore. J. Rare Earths 2017, 35, 1014–1021. [Google Scholar] [CrossRef]
- Li, Y.X. Ion Adsorption Rare Earth Resources and Their Green Extraction; Chemical Industry Press: Beijing, China, 2014. [Google Scholar]
- Chi, R.A.; Tian, J. Chemical and Metallury Process of Weathered Crust Rare Earth Ore; Chemical Industry Press: Beijing, China, 2006. [Google Scholar]
- Wu, A.X.; Yin, S.H.; Wang, H.J.; Su, Y.D. Solute transport mechanism and model of dump leaching. J. Cent. South Univ. (Sci. Technol.) 2006, 37, 385–389. [Google Scholar]
- Guo, Z.Q.; Zhao, K.; Jin, J.F.; Zhu, Z.C.; Li, G. Solute transport mechanism of ion-adsorption type rare earth in-situ leaching mining. J. Chin. Soc. Rare Earths 2019, 37, 121–128. [Google Scholar]
- Long, P.; Wang, G.S.; Tian, J.; Hu, S.L.; Luo, S.H. Simulation of one-dimensional column leaching of weathered crust elution-deposited rare earth ore. Trans. Nonferrous Met. Soc. 2019, 29, 625–633. [Google Scholar] [CrossRef]
- Liu, Q.S.; Xiao, H.; Tan, C.L.; Yu, X.Y.; Wang, D.L.; Qiu, T.S. Simulation research on multi-field coupling in leaching process of ionic rare earth ore based on COMSOL. J. Chin. Soc. Rare Earths 2022, 40, 880–892. [Google Scholar]
- Wang, H.T. Dynamics of Fluid Flow and Contaminant Transport in Porous Media; Higher Education Press: Beijing, China, 2008. [Google Scholar]
- Navnit, J.; Shikha, V. Method of approximations for the convection-dominated anomalous diffusion equation in a rectangular plate using high-resolution compact discretization. MethodsX 2022, 9, 101853. [Google Scholar]
- Hossain, M.A.; Yonge, D.R. Simulating advective-dispersive transport in groundwater: An accurate finite difference model. Appl. Math. Comput. 1999, 105, 221–230. [Google Scholar] [CrossRef]
- Moldrup, P.; Yamaguchi, T.; Rolston, D.E.; Vestergaard, K.; Hansen, J.A. Removing numerically induced dispersion from finite difference models for solute and water transport in unsaturated soils. Soil Sci. 1994, 157, 153–161. [Google Scholar] [CrossRef]
- Chen, Y.; Falconer, R.A. Advection-diffusion modelling using the modified QUICK scheme. Int. J. Numer. Meth. Fluids 1992, 15, 1171–1196. [Google Scholar] [CrossRef]
- Chen, J.M.; Hu, J.W. Reviews on numerical method for solving solute transport problems in the saturated porous media. Hydrog. Eng. Geol. 2003, 2, 99–106. [Google Scholar]
- Wang, Q.M.; Zhou, Z.J. SUPG-Stabilized Virtual Element Method for Optimal Control Problem Governed by a Convection Dominated Diffusion Equation. Entropy 2021, 23, 723. [Google Scholar] [CrossRef] [PubMed]
- Zhang, J.J.; Lin, G.; Li, W.X.; Wu, L.S.; Zeng, L.Z. An iterative local updating ensemble smoother for high-dimensional inverse modeling with multimodal distributions. Water Resour. Res. 2018, 54, 1716–1733. [Google Scholar] [CrossRef]
- Tabata, M.; Uchiumi, S. An exactly computable Lagrange–Galerkin scheme for the Navier–Stokes equations and its error estimates. Math. Comput. 2017, 87, 39–67. [Google Scholar] [CrossRef]
- Douglas, J.; Russell, T.F. Numerical Methods for Convection-Dominated Diffusion Problems Based on Combining the Method of Characteristics with Finite Element or Finite Difference Procedures. SIAM J. Numer. Anal. 1982, 19, 871–885. [Google Scholar] [CrossRef]
- Leonard, B.P.; Lock, A.P.; MacVean, M.K. Conservative Explicit Unrestricted-Time-Step Multidimensional Constancy-Preserving Advection Schemes. J. Atmos. Sci. 1996, 124, 2588–2606. [Google Scholar] [CrossRef]
- Zhou, F.; Hang, S.H.; Feng, J.; Deng, D.H.; Wang, Z.W.; Liu, Q. Study on distribution law of rare earth ions and aluminum ions in ammonium salt leaching process of weathered crust elution-deposited rare earth ores. Chin. J. Nonferr. Met. 2022, 32, 195–205. [Google Scholar]
- Long, P.; Wang, G.S.; Zhang, C.; Huang, Y.; Luo, S.H. A two-parameter model for ion exchange process of ion-adsorption type rare earth ores. J. Rare Earths 2020, 38, 1251–1256. [Google Scholar] [CrossRef]
- Ministry of Environmental Protection of the People’s Republic of China. Water Quality-Determination of Ammonia Nitrogen-Nessler’s Reagent Spectrophotometry; HJ 535; Ministry of Environmental Protection of the People’s Republic of China: Beijing, China, 2009.
- Aviation Industry Corporation of China. Methods for Chemical Analysis of Metal Heat Treatment Salt Bath; HB 7064.3; Aviation Industry Corporation of China: Beijing, China, 1994. [Google Scholar]
- Fan, B.; Zhao, L.S.; Feng, Z.Y.; Liu, D.P.; Yin, W.Q.; Long, Z.Q.; Huang, X.W. Leaching behaviors of calcium and magnesium in ion-adsorption rare earth tailings with magnesium sulfate. Trans. Nonferrous Met. Soc. 2021, 31, 288–296. [Google Scholar] [CrossRef]
- Hu, S.L.; Cao, X.J.; Wang, G.S.; Long, P.; Zhou, X.Y. An ion exchange model for leaching process of weathered crust elution-deposited rare earth. Min. Metall. Eng. 2018, 38, 1–5. [Google Scholar]
- Chi, R.A.; Li, L.F.; Wang, D.Z. Studies of ion exchange equilibrium in clay minerals of adsorbed rare earth. J. Zhongnan Inst. Min. Metall. 1991, 22, 142–148. [Google Scholar]
- Long, P.; Wang, G.S.; Zhang, C.; Yang, Y.J.; Cao, X.J.; Shi, Z.B. Kinetics model for leaching of ion-adsorption type rare earth ores. J. Rare Earths 2020, 38, 1354–1360. [Google Scholar] [CrossRef]
- Li, F.H. Physical Chemistry of Soil; Chemical Industry Press: Beijing, China, 2006. [Google Scholar]
- Mei, Y.; Wu, J.C. New method for reducing the numerical error in solving the problem of contaminant transport in ground water. Adv. Water Sci. 2009, 20, 639–645. [Google Scholar]
- Swami, D.; Sharma, P.K.; Ojha, C.S.P. Simulation of experimental breakthrough curves using multiprocess non-equilibrium model for reactive solute transport in stratified porous media. Sadhana 2014, 39, 1425–1446. [Google Scholar] [CrossRef]
- Swami, D.; Sharma, A.; Sharma, P.K.; Shukla, D.P. Predicting suitability of different scale-dependent dispersivities for reactive solute transport through stratified porous media. J. Rock Mech. Geo-Tech. Eng. 2016, 8, 921–927. [Google Scholar] [CrossRef]

| Component | La2O3 | CeO2 | Pr6O11 | Nd2O3 | Sm2O3 |
| Cotent | 22.73 | 15.54 | 5.08 | 20.71 | 4.9 |
| Component | Eu2O3 | Gd2O3 | Tb4O7 | Dy2O3 | Ho2O3 |
| Cotent | 0.93 | 3.96 | 0.65 | 3.18 | 0.67 |
| Component | Er2O3 | Tm2O3 | Yb2O3 | Lu2O3 | Y2O3 |
| Cotent | 1.86 | 0.33 | 1.55 | 0.29 | 17.63 |
| Parameters | M0 | M10 | M15 |
|---|---|---|---|
| t0.16 (d) | 1.34 | 1.94 | 2.48 |
| t0.5 (d) | 1.51 | 2.10 | 2.72 |
| t0.84 (d) | 1.63 | 2.34 | 2.90 |
| u (m/d) | 1.32 × 10−1 | 9.52 × 10−1 | 7.35 × 10−1 |
| D (m2/d) | 1.27 × 10−4 | 0.84 × 10−4 | 0.45 × 10−4 |
| Ore Samples | Method | Peak Concentration | Coefficient of Determination | ||
|---|---|---|---|---|---|
| Value (g/L) | Error (%) | Rare-Earth Ion | Ammonium Ion | ||
| M0 | Experimental data | 11.81 | - | - | - |
| Upstream weighting | 6.25 | 47.08 | 0.726 | 0.977 | |
| Proposed method | 11.32 | 4.15 | 0.930 | 0.989 | |
| M10 | Experimental data | 11.28 | - | - | - |
| Upstream weighting | 5.72 | 49.29 | 0.525 | 0.947 | |
| Proposed method | 10.58 | 6.21 | 0.859 | 0.951 | |
| M15 | Experimental data | 12.06 | - | - | - |
| Upstream weighting | 6.15 | 45.48 | 0.314 | 0.935 | |
| Proposed method | 11.49 | 1.86 | 0.881 | 0.969 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Huang, Y.; Long, P.; Wang, G.; Luo, S. Decoupling Method for the Convective-Dominated Leaching Process of Ion-Adsorption-Type Rare-Earth Ores. Minerals 2023, 13, 89. https://doi.org/10.3390/min13010089
Huang Y, Long P, Wang G, Luo S. Decoupling Method for the Convective-Dominated Leaching Process of Ion-Adsorption-Type Rare-Earth Ores. Minerals. 2023; 13(1):89. https://doi.org/10.3390/min13010089
Chicago/Turabian StyleHuang, Ying, Ping Long, Guanshi Wang, and Sihai Luo. 2023. "Decoupling Method for the Convective-Dominated Leaching Process of Ion-Adsorption-Type Rare-Earth Ores" Minerals 13, no. 1: 89. https://doi.org/10.3390/min13010089
APA StyleHuang, Y., Long, P., Wang, G., & Luo, S. (2023). Decoupling Method for the Convective-Dominated Leaching Process of Ion-Adsorption-Type Rare-Earth Ores. Minerals, 13(1), 89. https://doi.org/10.3390/min13010089





