Laboratory Studies of Small Strain Stiffness and Modulus Degradation of Warsaw Mineral Cohesive Soils
Abstract
1. Introduction
2. Extended Empirical Formulas for Cohesive Soils
3. Tested Materials, Test Device and Testing Procedure
3.1. Soils Characterization
3.2. Mineralogical Identification
- X-ray structural analysis;
- Thermal analysis;
- Microstructural analysis in scanning electron microscopy (SEM) [45].
3.3. Test Device and Dynamic Testing Procedure
- Selection of the output amplitude, which determined the amount of shear strain applied to the specimen. For this purpose, a step-by-step approach was used [51].
- Drawing of the frequency response curve and observation of resonant frequency from torsional vibrations.
- Stiffness analysis and shear modulus calculation based on the determined resonant frequency.
- Calculation of torsional strain from the angle of twist of the test specimen.
- Repeating of 1-4 steps with an increasing applied voltage, maximum up to 1.0 V.
- Next consolidation stage with an increased effective stress.
- Repeating steps 1–6.
4. Results and Discussion
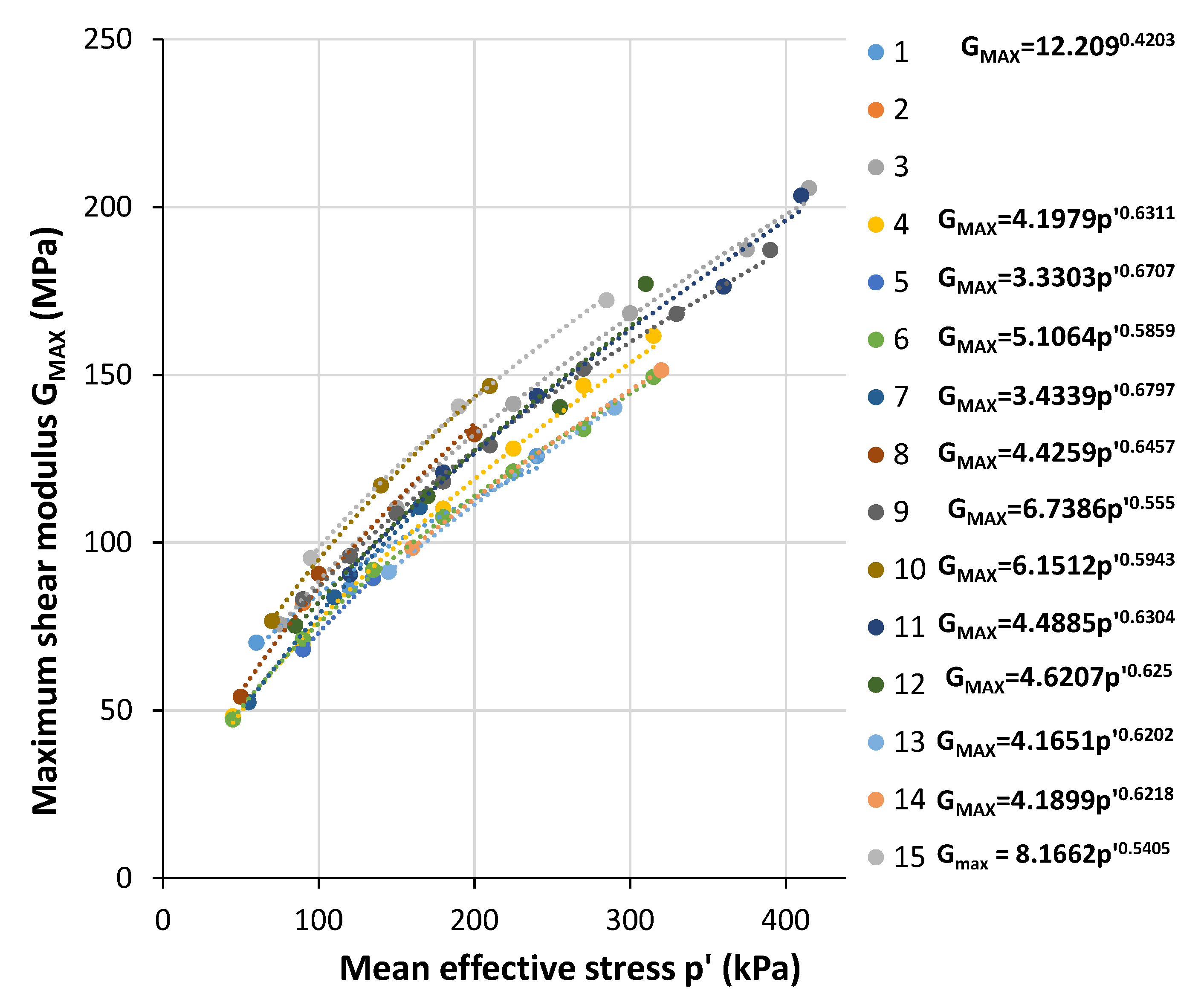
4.1. Small Strain Shear Modulus G0
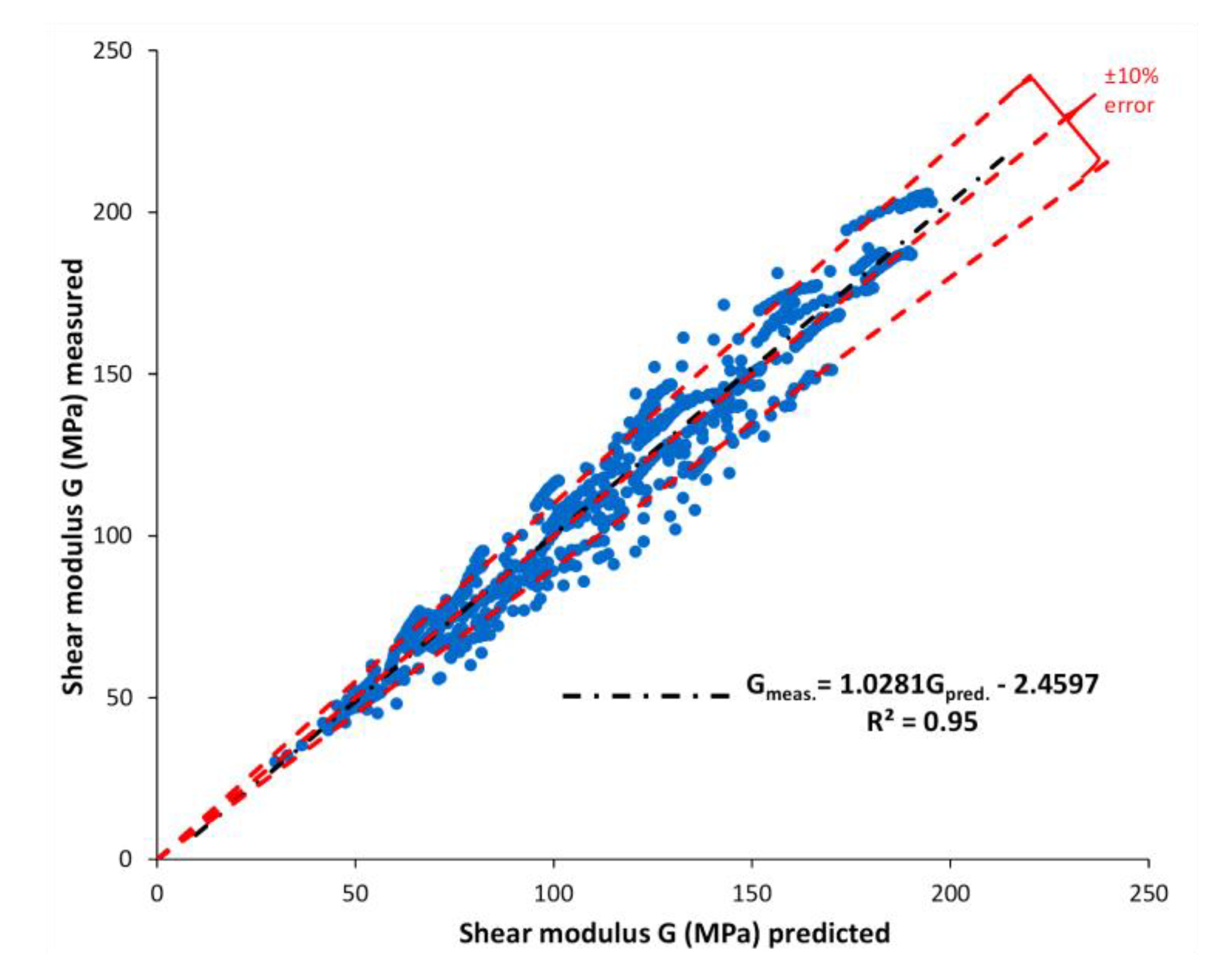
4.2. Empirical Equations for GMAX
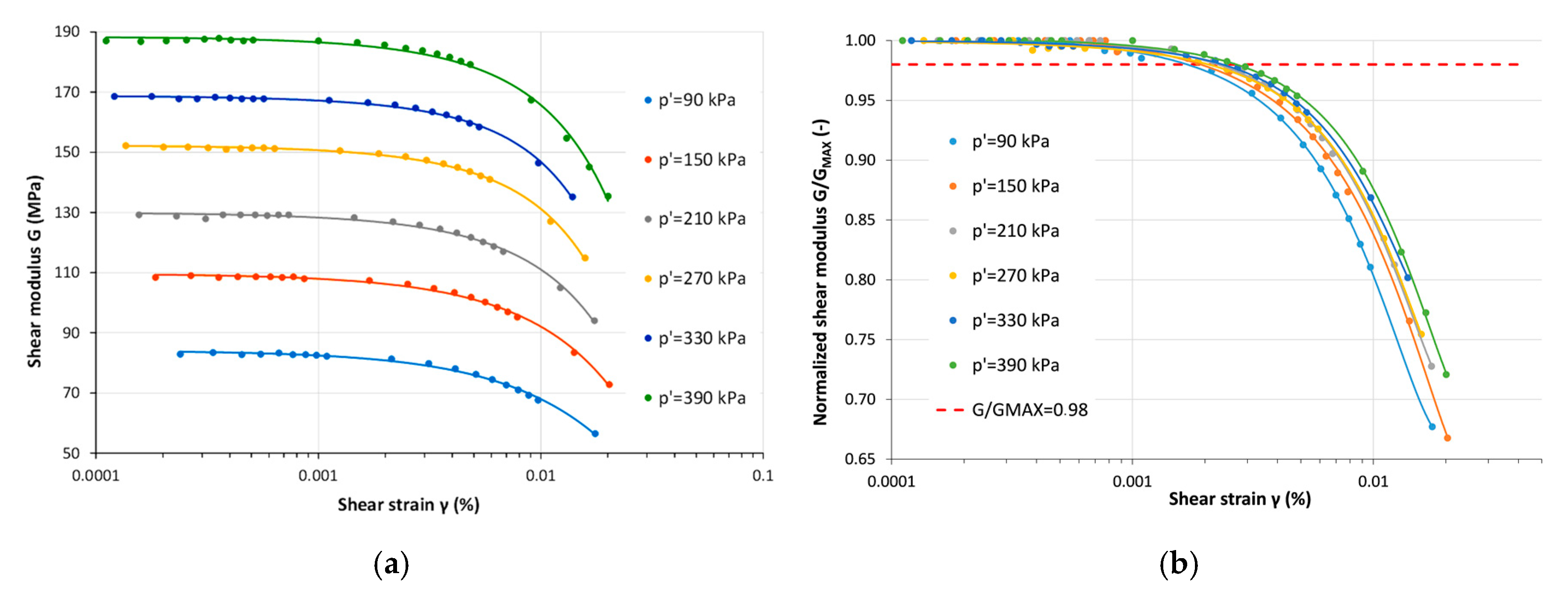
4.3. Modulus DegradationC G(γ)/G0
4.4. Empirical Formulas for G(γ)/GMAX
5. Summary and Conclusions
- The maximum shear modulus increased with an increase in mean effective stress.
- Change of GMAX with increasing effective stress was described with the highest determination coefficient R2 by the power function.
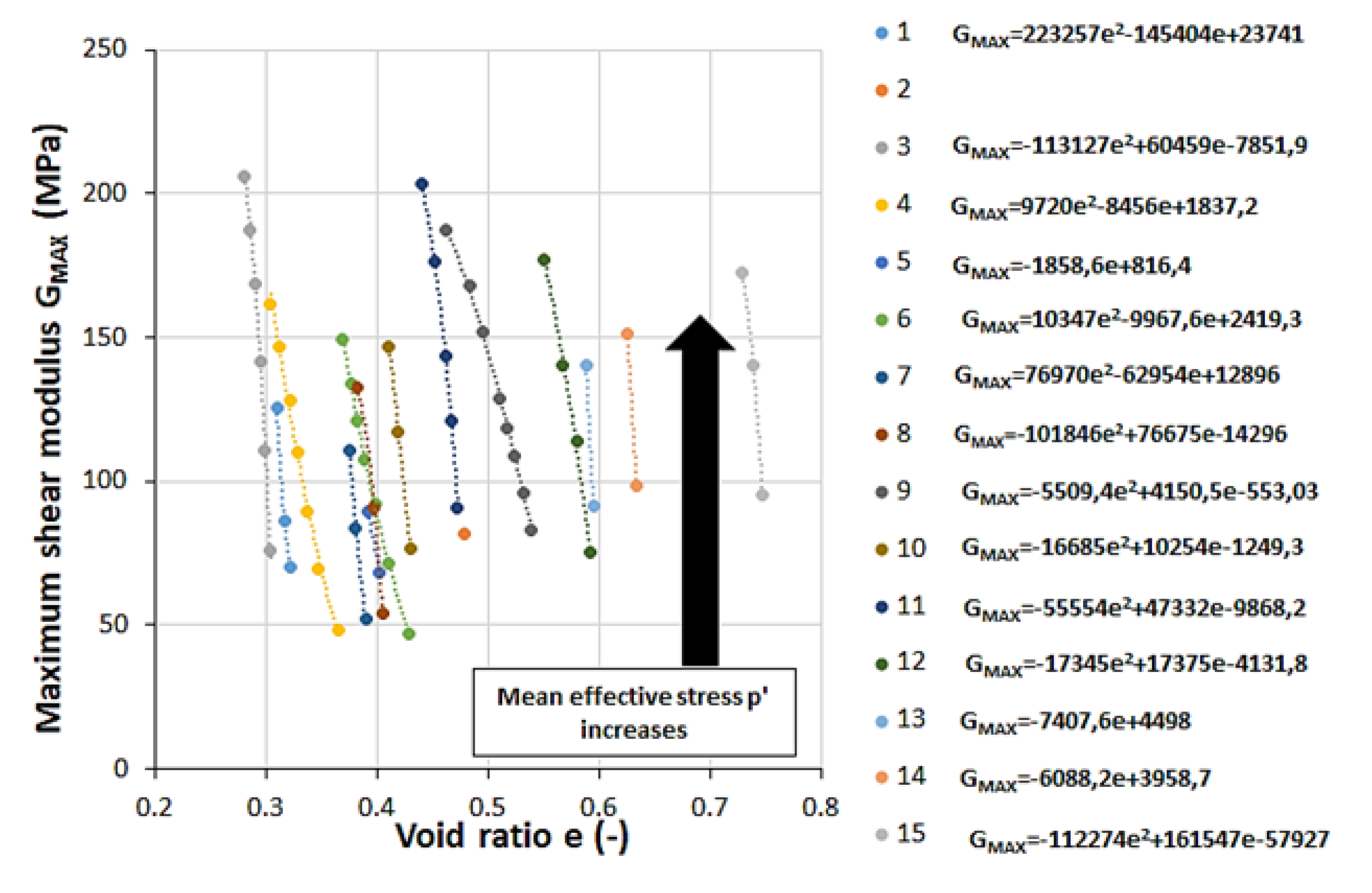
- The significant GMAX empirical model parameter occurred as a void ratio e.
- Change of e with GMAX was described with the highest R2 by quadratic functions.
- Plasticity index (PI) occurred to have a marginal impact on the GMAX value.
- The crated GMAX empirical model consisted of the power function of effective stress p’ and the quadratic function of the void ratio e.
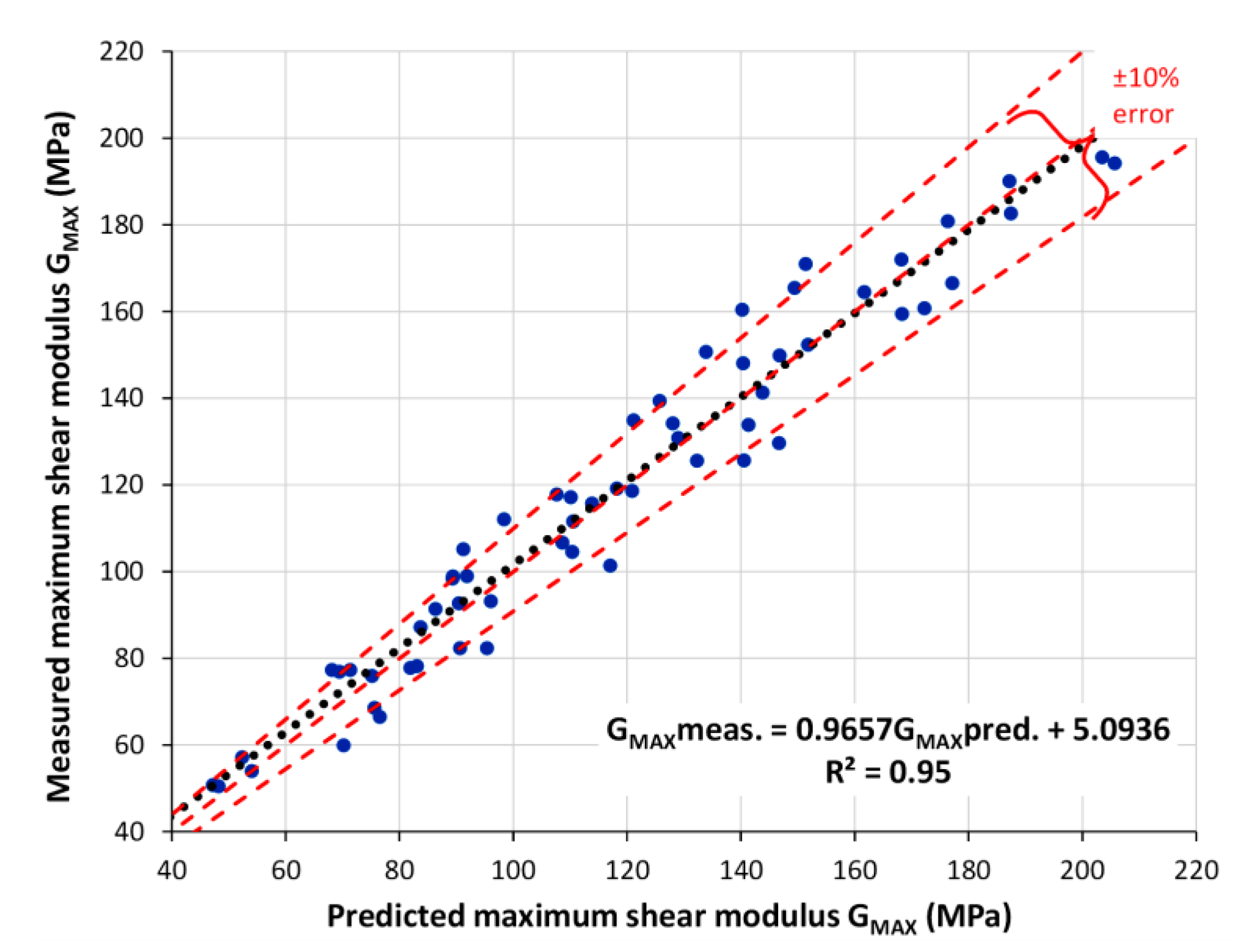
- Proposed GMAX equation calculated the values of the maximum shear modulus of Warsaw cohesive soils with the lowest relative error, of about 6.8%.
- The shear strain had the highest impact on the shear modulus and normalized shear modulus degradation curves.
- With increasing both plasticity and effective stress, nonlinear threshold strain moved to higher shear deformation. However, mean effective stress (p’) had a noticeably smaller impact on the elastic range of shear deformation than plasticity index (PI).
- Proposed by the authors, the empirical model for the normalized shear modulus degradation curve consists of equation estimated by Amir-Faryar et al. [35] for all soils and power functions of the plasticity index (PI).
- The presented equation had the lowest absolute and relative error in comparison with other models, 0.019 and 2.197%, respectively.
- Combined GMAX and G/GMAX equations allowed us to estimate the shear modulus value of Warsaw cohesive soils in a wide shear deformation range with a low relative error of about 6.7%.
Author Contributions
Funding
Conflicts of Interest
References
- Kallioglou, P.; Tika, T.H.; Pitilakis, K. Shear Modulus and Damping Ration of Cohesive Soils. J. Earthq. Eng. 2008, 12, 879–913. [Google Scholar] [CrossRef]
- Atkinson, J. The Mechanics of Soils and Foundations, 2nd ed.; Routledge Taylor & Francis Group: London, UK; New York, NY, USA, 2007. [Google Scholar]
- Gu, X.; Yang, J.; Huang, M. Laboratory measurements of small strain properties of dry sands by bender elements. Soils Found. 2013, 53, 735–745. [Google Scholar] [CrossRef]
- Bai, L. Preloading Effects on Dynamic Sand Behavior by Resonant Column Tests. Ph.D. Thesis, Technischen Universität Berlin, Berlin, Germany, 2011. [Google Scholar]
- Darendeli, M.B. Development of a new family of normalized modulus reduction and material damping curves. Ph.D. Thesis, The University of Texas at Austin, Austin, TX, USA, 2001. [Google Scholar]
- Clayton, C.R.I. Stiffness at small strain: Research and practice. Géotechnique 2011, 61, 5–37. [Google Scholar] [CrossRef]
- Goto, S.; Tatsuoka, F.; Shibuya, S.; Kim, Y.S.; Sato, T. A simple gauge for local small strain measurements in the laboratory. Soils Found. 1991, 31, 169–180. [Google Scholar] [CrossRef]
- Scholey, G.K.; Frost, J.D.; Lo Presti, D.C.F.; Jamiolkowski, M. A review of instrumentaion for measuring small strains during triaxial testing of soil specimens. Astm. Geotech. Test. J. 1995, 18, 137–156. [Google Scholar]
- Jardine, R.J.; Symes, M.J.; Burland, J.B. The measurements of soil stiffness in the triaxial apparatus. Géotechnique 1984, 34, 323–340. [Google Scholar] [CrossRef]
- Hird, C.C.; Yung, P.C.Y. The use of proximity transducer for local strain measurements in triaxial tests. Astm Geotech. Test. J. 1989, 12, 292–296. [Google Scholar]
- Likitlersuang, S.; Teachavorasinskun, S.; Surarak, C.; Oh, E.; Balasubramaniam, A. Small strain stiffness and stiffness degradation curve of Bangkok Clays. Soils Found. 2013, 53, 498–509. [Google Scholar] [CrossRef]
- Kramer, S.L. Geotechnical Earthquake Engineering, 1st ed.; Prentice Hall: New York, NY, USA, 1996. [Google Scholar]
- Hardin, B.O.; Black, W.L. Vibration modulus of normally consolidated clays. ASCE J. Soil. Mech. Found. Div. 1968, 94, 353–369. [Google Scholar]
- Hardin, B.O.; Black, W.L. Vibration modulus of normally consolidated clay–closure. ASCE J. Soil Mech. Found. Div. 1969, 95, 1531–1537. [Google Scholar]
- Lefebvre, G.; Leboeuf, D. Laboratory and field determinations of small-strain shear modulus for a structured Champlain clay. Can. Geotech. J. 1994, 31, 61–70. [Google Scholar] [CrossRef]
- Jardine, R.J. Some observation on the kinematic nature of soil stiffness. Soils Found. 1992, 32, 111–124. [Google Scholar] [CrossRef]
- Gazetas, G. Vibrational characteristics of soil deposits with variable wave velocity. Int. J. Numer. Anal. Methods Geomech. 1982, 6, 1–10. [Google Scholar] [CrossRef]
- Carlton, B.D.; Pestana, J.M. A unified model for estimating the in-situ small strain shear modulus of clays, silts, sands, and gravels. Soil Dyn. Earthq. Eng. 2016, 88, 345–355. [Google Scholar] [CrossRef]
- Gabryś, K.; Sas, W.; Soból, E. Dynamic and cyclic static loading behavior of silty-sandy clay at small and moderate strains. Acta Sci. Pol. Ser. Archit. 2016, 15, 43–55. [Google Scholar]
- Marcuson, W.; Wahls, H. Time effects on dynamic shear modulus of clays. ASCE J. Soil Mech. Found. Div. 1972, 98, 1359–1373. [Google Scholar]
- Kim, T.; Novac, M. Dynamic properties of some cohesive soils of Ontario. Can. Geotech. J. 1981, 18, 371–389. [Google Scholar] [CrossRef]
- Kokusho, T.; Yoshida, Y.; Esashi, Y. Dynamic properties of soft clay for wide strain range. Soils Found. 1982, 22, 1–18. [Google Scholar] [CrossRef]
- Stokoe II, K.H.; Hwang, S.K.; Lee, J.K.; Andrus, R.D. Effects of various parameters on the stiffness and damping of soils at small to medium strains. In Proceedings of the International Symposium, Sapporo, Japan, 12–14 September 1994. [Google Scholar]
- Jamiolkowski, M.; Lancellotta, R.; Lo Presti, D.C.F. Remarks on the stiffness at small strains of six Italian clays. Pre-Fail. Deform. Geomaterials 1995, 2, 817–836. [Google Scholar]
- Shibuya, S.; Tanaka, H. Estimate of elastic shear modulus in Holocene soil deposits. Soils Found. 1996, 36, 45–55. [Google Scholar] [CrossRef]
- D’Elia, B.; Lanzo, G. Laboratory and field determinations of small-strain shear modulus of natural soil deposits. In Proceedings of the 11th World Conference on Earthquake Engineering, Acapulco, Mexico, 23–28 June 1996. [Google Scholar]
- Vrettos, C.; Savidis, S. Shear modulus and damping for Mediterranean sea clays of medium plasticity. In Earthquakes Geotechnical Engineering; e Pinto, P.S., Balkema, A.A., Eds.; CRC Press: Lisboa, Portugal, 1999; pp. 71–76. [Google Scholar]
- Hassanipour, A.; Shafiee, A.; Jafari, M.K. Low-amplitude dynamic properties for compacted sand-clay mixtures. Int. J. Civ. Eng. 2011, 9, 255–264. [Google Scholar]
- Vardanega, P.J.; Bolton, M.D. Stiffness of clays and silts: Normalizing shear modulus and shear strain. J. Geotech. Geoenvironmental Eng. 2013, 139, 1575–1589. [Google Scholar] [CrossRef]
- Sas, W.; Gabryś, K.; Szymański, A. Experimental studies of dynamic properties of Quaternary clayey soils. Soil Dyn. Earthq. Eng. 2017, 95, 29–39. [Google Scholar] [CrossRef]
- Iwasaki, T.; Tatsuoka, F. Shear moduli of sands under cyclic torsional shear loading. Soils Found. 1977, 17, 19–35. [Google Scholar] [CrossRef]
- Vucetic, M.; Dobry, M. Effect of Soil Plasticity on Cyclic Response. ASCE J. Geotech. Eng. 1991, 117, 89–107. [Google Scholar] [CrossRef]
- Ishibashi, I.; Zhang, X. Unified dynamic shear moduli and damping ratios of sand and clay. Soils Found. 1993, 33, 182–191. [Google Scholar] [CrossRef]
- Zhang, J.; Andrus, R.D.; Juang, C.H. Normalized shear modulus and material damping ratio relationships. J. Geotech. Geoenvironmental Eng. 2005, 131, 453–464. [Google Scholar] [CrossRef]
- Amir-Faryar, B.; Aggour, M.S.; McCuen, R.H. Universal model forms for predicting the shear modulus and material damping of soils. Geomech. Geoengin. 2017, 12, 60–71. [Google Scholar] [CrossRef]
- Soból, E.; Głuchowski, A.; Szymański, A.; Sas, W. The New Empirical Equation Describing Damping Phenomenon in Dynamically Loaded Subgrade Cohesive Soils. Appl. Sci. 2019, 9, 4518. [Google Scholar] [CrossRef]
- PN-B-04481:1988. Grunty Budowlane–Badania Próbek Gruntu; PKN: Plock, Poland, 1988. (In Polish) [Google Scholar]
- ISO 17892-4:2016 Geotechnical Investigation and Testing–Laboratory Testing of Soil–Part 4: Determination of Particle Size Distribution. Available online: http:/sklep.pkn.pl/pn-en-iso-17892-4-2017-01e.html (accessed on 18 January 2017).
- ISO. 17892-1:2014 Geotechnical Investigation and Testing–Laboratory Testing of Soil–Part. 1: Determination of Water Content; ISO: Geneva, Switzerland, 2007. [Google Scholar]
- ISO. 17892-12:2018 Geotechnical Investigation and Testing–Laboratory Testing of Soil–Part. 12: Determination of Liquid and Plastic Limits; ISO: Geneva, Switzerland, 2018. [Google Scholar]
- ISO. 17892-5:2017 Geotechnical Investigation and Testing–Laboratory Testing of Soil–Part. 5: Incremental Loading Oedometer Test; ISO: Geneva, Switzerland, 2017. [Google Scholar]
- Wiłun, Z. Zarys Geotechniki, 3rd ed.; Wydawnictwa Komunikacji i Łączności: Warsaw, Poland, 1987. (In Polish) [Google Scholar]
- Khan, M.I. Hydraulic Conductivity of Moderate and Highly Dense Expansive Clay. Ph.D. Thesis, University of Bochum, Bochum, Germany, 2012. [Google Scholar]
- Grim, R.E. Clay Mineralogy; MC Graw-Hill Book Co.: New York, NY, USA, 1960. [Google Scholar]
- Tazaki, K.; Fukuyama, A.; Tazaki, F.; Takehara, T.; Nakamura, K.; Okuno, M.; Hashida, Y.; Hashida, S. Electron Microscopy Observation of Biomineralization within Wood Tissues of Kurogaki. Minerals 2017, 7, 123. [Google Scholar] [CrossRef]
- Niedźwiedzka, K. Skład Mineralny Jako Główny Czynnik Wpływający na Pęcznienie Gruntów Ilastych. MSc Thesis, Warsaw University of Life Sciences, Warsaw, Poland, 2012. (In Polish). [Google Scholar]
- Bekkouche, A.; Djedid, A.; Aissa Mamoune, S. Identification et prevision du gonflement de quelques sols de la region de Tlemeen. Bull. Lab. Ponts Chaussees 2001, 233, 69–77. [Google Scholar]
- Meehan, R.; Karp, L. California Housing Damage Related to Expansive Soils. J. Perform. Constr. Fac. 1994, 8, 139–157. [Google Scholar] [CrossRef]
- Chen, F. Foundations on Expansive Soils; Elsevier Scientific Pub. Co.: Amsterdam, The Netherlands, 1988. [Google Scholar]
- Gabryś, K.; Sas, W.; Soból, E.; Głuchowski, A. Torsional shear device for testing the dynamic properties of recycled material. Studia Geotech. Mech. 2016, 38, 15–24. [Google Scholar] [CrossRef]
- Gabryś, K. Charakterystyki odkształceniowe wybranych gruntów spoistych. Ph.D. Thesis, Warsaw University of Life Sciences, Warsaw, Poland, 2014. (In Polish). [Google Scholar]
- ASTM Standard D4015-92. Test. Methods for Modulus and Damping of Soils by the Resonant Column Method; Annual Book of ASTM Standards; ASTM International: West Conshohocken, PA, USA, 2003; pp. 473–494. [Google Scholar]
- Gabrys, K.; Sobol, E.; Markowska-Lech, K.; Szymański, A. Shear Modulus of Compacted Sandy Clay from Various Laboratory Methods. In IOP Conference Series: Materials Science and Engineering; IOP Publishing: Bristol, UK, 2019. [Google Scholar]
- Li, H.; Senetakis, K.; Coop, M.R. Medium-strain dynamic behavior of fiber-reinforced sand subjected to stress anisotropy. Soil Dyn. Earthq. Eng. 2019, 126, 105764. [Google Scholar] [CrossRef]
- Stokoe, K.H.; Darendeli, M.B.; Andrus, R.D.; Brown, L.T. Dynamic soil properties: Laboratory, field and correlation studies. Earthq. Geotech. Eng. 1999, 811–845. [Google Scholar]
- Vucetic, M. Cyclic threshold shear strains in soils. J. Geotech. Eng. 1994, 120, 2208–2228. [Google Scholar] [CrossRef]
- Matsui, T.; Ohara, H.; Ito, T. Cyclic stress-strain history and shear characteristics of clay. ASCE J. Geotech. Eng. 1980, 106, 1101–1120. [Google Scholar]
- Santos, J.A.; Correia, A.G. Reference threshold shear strain of soil, its application to obtain a unique strain-dependent shear modulus curve for soil. In Proceedings of the 15th International Conference on Soil Mechanics and Geotechnical Engineering, Istanbul, Turkey, 27–31 August 2001; pp. 267–270. [Google Scholar]
- Sun, J.; Golesorkhi, R.; See, H. Dynamic Moduli and Damping Ratio for Cohesive Soils; Report No. UCB/EERC-88/15; University of California Berkeley-Earthquake Engineering Research Center: Berkeley, CA, USA, 1988. [Google Scholar]




| Equations | Soil Type | Reference |
|---|---|---|
| Remolded Edgar Plastic Kaolin and Boston blue clay | Hardin and Black 1968 [13] | |
| Remolded specimens of kaolinite and calcium bentonite | Marcuson and Wahls 1972 [20] | |
| Various clays; e = 0.48-1.36 and PI=12−30.3% | Kim and Novac 1981 [21] | |
| 1. Kaolinite PI = 25% 2. Alluvial clays; e = 1.5−4.0 and PI = 40−100% | Kokusho et al. 1982 [22] | |
| Silty clays | Stokoe et al. 1995 [23] | |
| Italian clays; e = 0.6−3.0 and PI = 10−75% | Jamiolkowski et al. 1995 [24] | |
| Seven clays; e = 1.47−3.29 and PI = 19−116% | Shibuya and Tanaka 1996 [25] | |
| 1. Sandy silt, silty sand 2. Clayey silts; e = 0.48−1.07 and PI = 10−38% | D’Elia and Lanzo 1996 [26] | |
| Clays; e = 0.583–1.068 andPI = 9−27% | Vrettos andSavidis 1999 [27] | |
| Undisturbed cohesive soils; e = 0.365−1.118 and PI = 5−46% | Kallioglou et al. 2008 [1] | |
| Compacted clays and sand-clay mixtures; PI = 12−50% | Hassanipour et al. 2011 [28] | |
| Various clays and silts; e = 0.48−6.15 and PI = 10−150% | Vardanega and Bolton 2013 [29] | |
| Various cohesive soils from Warsaw; e = 0.34−0.6 and PI = 14.8−27.5% | Sas et al. 2017 [30] |
| Equations | Soil Type | Reference |
|---|---|---|
| Various cohesive soils | Ishibashi, I. and Zhang 1993 [33] | |
| Various cohesive soils | Darendeli 2001 [5] | |
| Various cohesive soils | Zhang et al. 2005 [34] | |
| Undisturbed cohesive soils; e = 0.365−1.118 and PI = 5−46% | Kallioglou et al. 2008 [1] | |
| Various clays and silts; e = 0.48−6.15 and PI = 10−150% | Vardanega and Bolton 2013 [29] | |
| 1. Clays 2. Universal model for all soils | Amir-Faryar et al. 2017 [35] |
| Test Site | Test Name | Soil Type | d50 (mm) | WC (%) | LL (%) | PL (%) | PI (%) | p’ (kPa) | e0 (-) | OCR (-) |
|---|---|---|---|---|---|---|---|---|---|---|
| No 1 | 1 | clSa | 0.11 | 11.06 | 17.70 | 11.10 | 6.60 | 60–240 | 0.322 | 3.33–1 |
| 2 | sasiCl | 0.06 | 17.41 | 36.50 | 14.10 | 22.40 | 90 | 0.478 | 1.00 | |
| 3 | sasiCl | 0.1 | 10.76 | 24.50 | 12.47 | 12.03 | 75–415 | 0.304 | 2.13–1 | |
| 4 | saCl | 0.08 | 12.23 | 37.00 | 11.45 | 25.60 | 45–315 | 0.365 | 1.00 | |
| 5 | clSa | 0.095 | 15.57 | 41.70 | 14.26 | 27.40 | 90–315 | 0.403 | 1.00 | |
| 6 | clSa | 0.1 | 10.5 | 18.20 | 9.10 | 9.10 | 45–315 | 0.429 | 1.00 | |
| 7 | sasiCl | 0.09 | 12.68 | 27.10 | 12.32 | 14.80 | 55–165 | 0.389 | 1.00 | |
| No 2 | 8 | saCl | 0.098 | 11.75 | 22.10 | 11.50 | 10.60 | 50–200 | 0.405 | 2.8–1 |
| 9 | sasiCl | 0.078 | 18.85 | 32.75 | 15.96 | 16.80 | 90–390 | 0.562 | 1.00 | |
| 10 | clSa | 0.11 | 14.43 | 21.20 | 12.24 | 8.96 | 70–210 | 0.436 | 1.00 | |
| No 3 | 11 | siCl | 0.018 | 17.53 | 37.25 | 17.14 | 20.10 | 120–410 | 0.473 | 1.00 |
| 12 | siCl | 0.0077 | 19.84 | 44.60 | 19.49 | 25.10 | 85–310 | 0.591 | 2–1 | |
| 13 | siCl | 0.01 | 21.98 | 51.27 | 23.65 | 27.60 | 145–290 | 0.595 | 1.38–1 | |
| 14 | siCl | 0.0091 | 22.95 | 63.50 | 26.82 | 36.70 | 160–320 | 0.634 | 1.25–1 | |
| 15 | Cl | 0.002 | 26.04 | 70.95 | 33.11 | 37.80 | 95–285 | 0.747 | 6.32–2.11 |
| Test Name | Soil Type | Total Clay Minerals (%) | Content of Expansive Clay Minerals (%) | Montmorillonite (%) | Illite (%) | Kaolinite (%) | σcs (kPa) |
|---|---|---|---|---|---|---|---|
| 13 | siCl | 41.30 | 33.60 | 33.60 | trace content | 7.70 | 105 |
| 14 | siCl | 41.30 | 29.20 | 29.20 | 0 | 12.10 | 120 |
| 15 | Cl | 43.70 | 34.50 | 34.50 | trace content | 9.20 | 90 |
| Cl (%) | Si (%) | Sa+Gr (%) | PI (%) | p’ (kPa) | e (-) | OCR (-) | GMAX (MPa) | |
|---|---|---|---|---|---|---|---|---|
| Cl (%) | 1.000 | 0.510 | −0.782 | 0.823 | 0.097 | 0.724 | 0.158 | 0.174 |
| Si (%) | 0.510 | 1.000 | −0.935 | 0.542 | 0.236 | 0.620 | −0.100 | 0.241 |
| Sa+Gr (%) | −0.782 | −0.935 | 1.000 | −0.732 | −0.211 | −0.747 | 0.008 | −0.246 |
| PI (%) | 0.823 | 0.542 | −0.732 | 1.000 | 0.089 | 0.653 | −0.034 | 0.096 |
| p’ (kPa) | 0.097 | 0.236 | −0.211 | 0.089 | 1.000 | −0.016 | −0.167 | 0.971 |
| e (-) | 0.724 | 0.620 | −0.747 | 0.653 | −0.016 | 1.000 | −0.085 | 0.045 |
| OCR (-) | 0.158 | −0.100 | 0.008 | −0.034 | −0.167 | −0.085 | 1.000 | −0.098 |
| GMAX (MPa) | 0.174 | 0.241 | −0.246 | 0.096 | 0.971 | 0.045 | −0.098 | 1.000 |
| Cl (%) | Si (%) | Sa+Gr (%) | PI (%) | p’ (kPa) | e (-) | OCR (-) | γ (%) | G/GMAX (-) | |
|---|---|---|---|---|---|---|---|---|---|
| Cl (%) | 1.000 | 0.538 | −0.775 | 0.830 | 0.093 | 0.726 | 0.387 | 0.045 | 0.062 |
| Si (%) | 0.538 | 1.000 | −0.950 | 0.555 | 0.247 | 0.607 | −0.050 | 0.048 | 0.137 |
| Sa+Gr (%) | −0.775 | −0.950 | 1.000 | −0.725 | −0.219 | −0.725 | −0.106 | −0.053 | −0.126 |
| PI (%) | 0.830 | 0.555 | −0.725 | 1.000 | 0.090 | 0.683 | 0.122 | 0.166 | −0.032 |
| p’ (kPa) | 0.093 | 0.247 | −0.219 | 0.090 | 1.000 | −0.016 | −0.343 | −0.025 | 0.084 |
| e (-) | 0.726 | 0.607 | −0.725 | 0.683 | −0.016 | 1.000 | 0.170 | 0.023 | 0.148 |
| OCR (-) | 0.387 | −0.050 | −0.106 | 0.122 | −0.343 | 0.170 | 1.000 | −0.102 | 0.040 |
| γ (%) | 0.045 | 0.048 | −0.053 | 0.166 | −0.025 | 0.023 | −0.102 | 1.000 | −0.905 |
| G/GMAX (-) | 0.062 | 0.137 | −0.126 | −0.032 | 0.084 | 0.148 | 0.040 | −0.905 | 1.000 |
| Reference | Linear Equation Described a Trend Line G/GMAXmeas.–G/GMAXpred. * | Determination Coefficient R2 | Error type | |
|---|---|---|---|---|
| Absolute (-) | Relative (%) | |||
| Authors Equation (4) | y = 1.0115x−0.0085 | 0.92 | 0.019 | 2.197 |
| Darendeli 2001 [5] | Y = 1.1295x−0.1112 | 0.87 | 0.028 | 3.290 |
| Zhang et al. 2005 [34] | y = 1.5295x−0.51 | 0.87 | 0.029 | 3.732 |
| Kallioglou et al. 2008 [1] | y = 0.5081x+0.5015 | 0.85 | 0.087 | 10.473 |
| Vardanega and Bolton 2013 [29] | y = 1.0781x−0.0618 | 0.86 | 0.028 | 3.283 |
| Amir-Faryar et al. 2017 [35] | y = 1.7552x−0.7458 | 0.83 | 0.034 | 4.391 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Soból, E.; Gabryś, K.; Zabłocka, K.; Šadzevičius, R.; Skominas, R.; Sas, W. Laboratory Studies of Small Strain Stiffness and Modulus Degradation of Warsaw Mineral Cohesive Soils. Minerals 2020, 10, 1127. https://doi.org/10.3390/min10121127
Soból E, Gabryś K, Zabłocka K, Šadzevičius R, Skominas R, Sas W. Laboratory Studies of Small Strain Stiffness and Modulus Degradation of Warsaw Mineral Cohesive Soils. Minerals. 2020; 10(12):1127. https://doi.org/10.3390/min10121127
Chicago/Turabian StyleSoból, Emil, Katarzyna Gabryś, Karina Zabłocka, Raimondas Šadzevičius, Rytis Skominas, and Wojciech Sas. 2020. "Laboratory Studies of Small Strain Stiffness and Modulus Degradation of Warsaw Mineral Cohesive Soils" Minerals 10, no. 12: 1127. https://doi.org/10.3390/min10121127
APA StyleSoból, E., Gabryś, K., Zabłocka, K., Šadzevičius, R., Skominas, R., & Sas, W. (2020). Laboratory Studies of Small Strain Stiffness and Modulus Degradation of Warsaw Mineral Cohesive Soils. Minerals, 10(12), 1127. https://doi.org/10.3390/min10121127







