Some Computational Aspects of Boron Triangular Nanotubes
Abstract
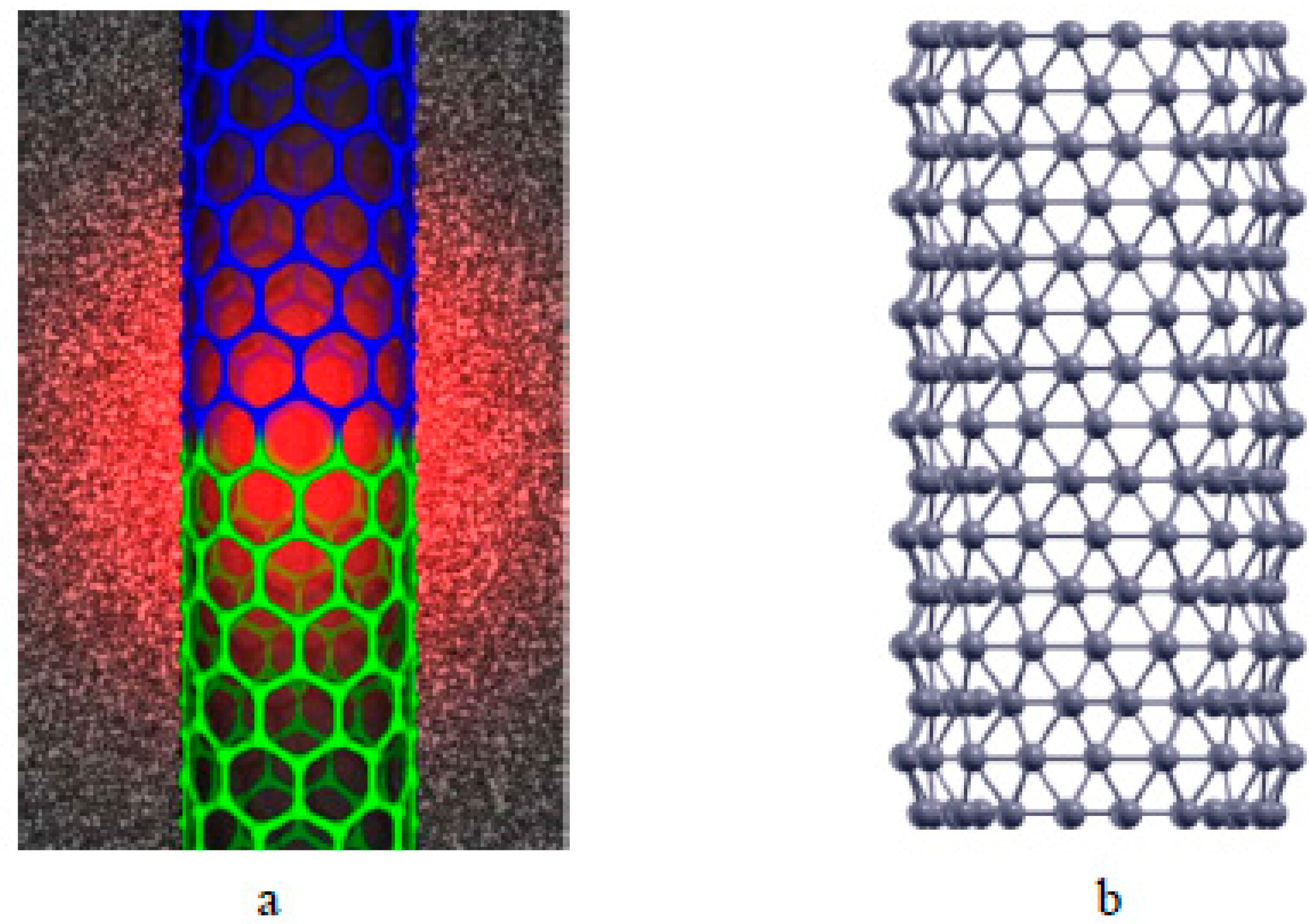
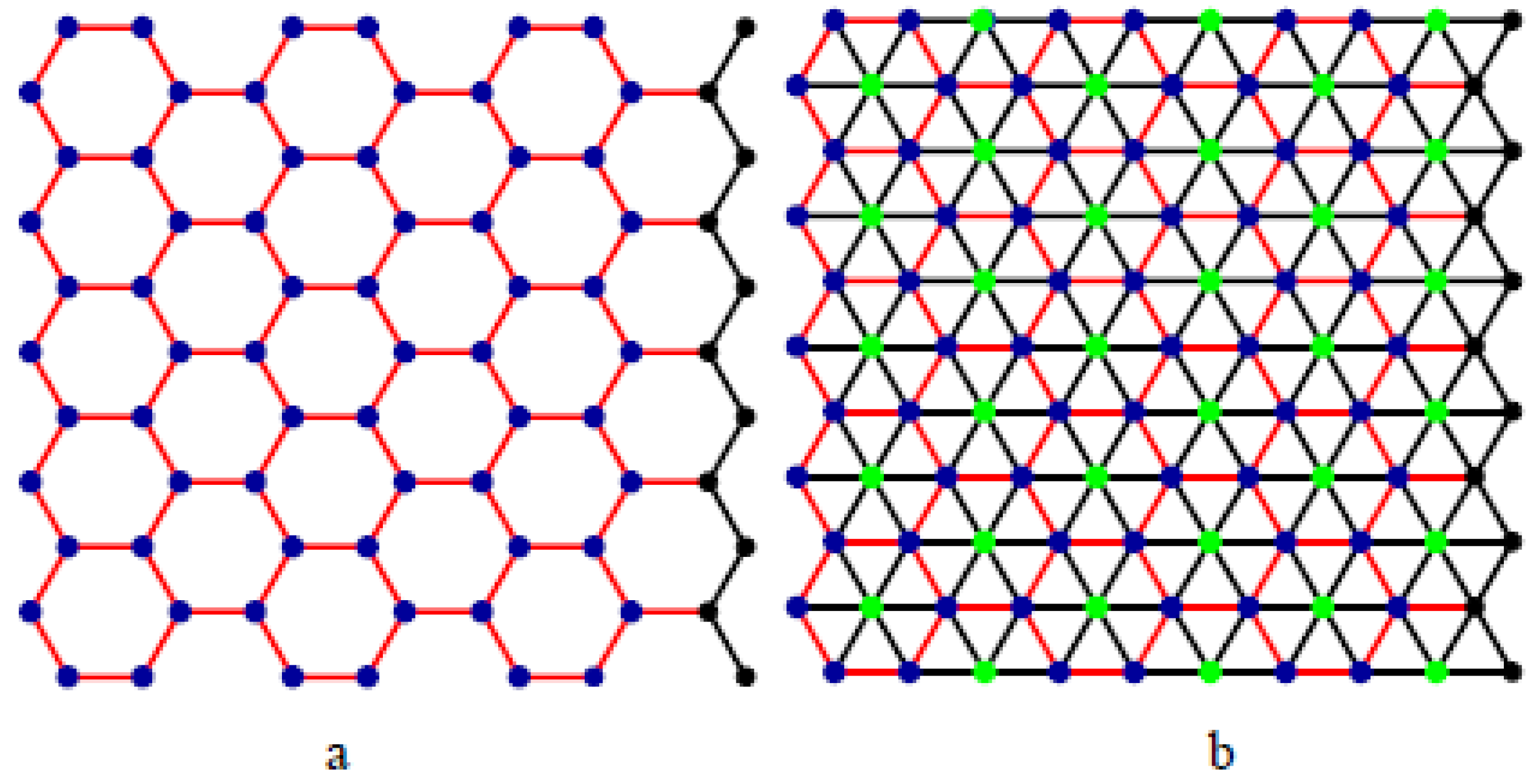
:1. Introduction
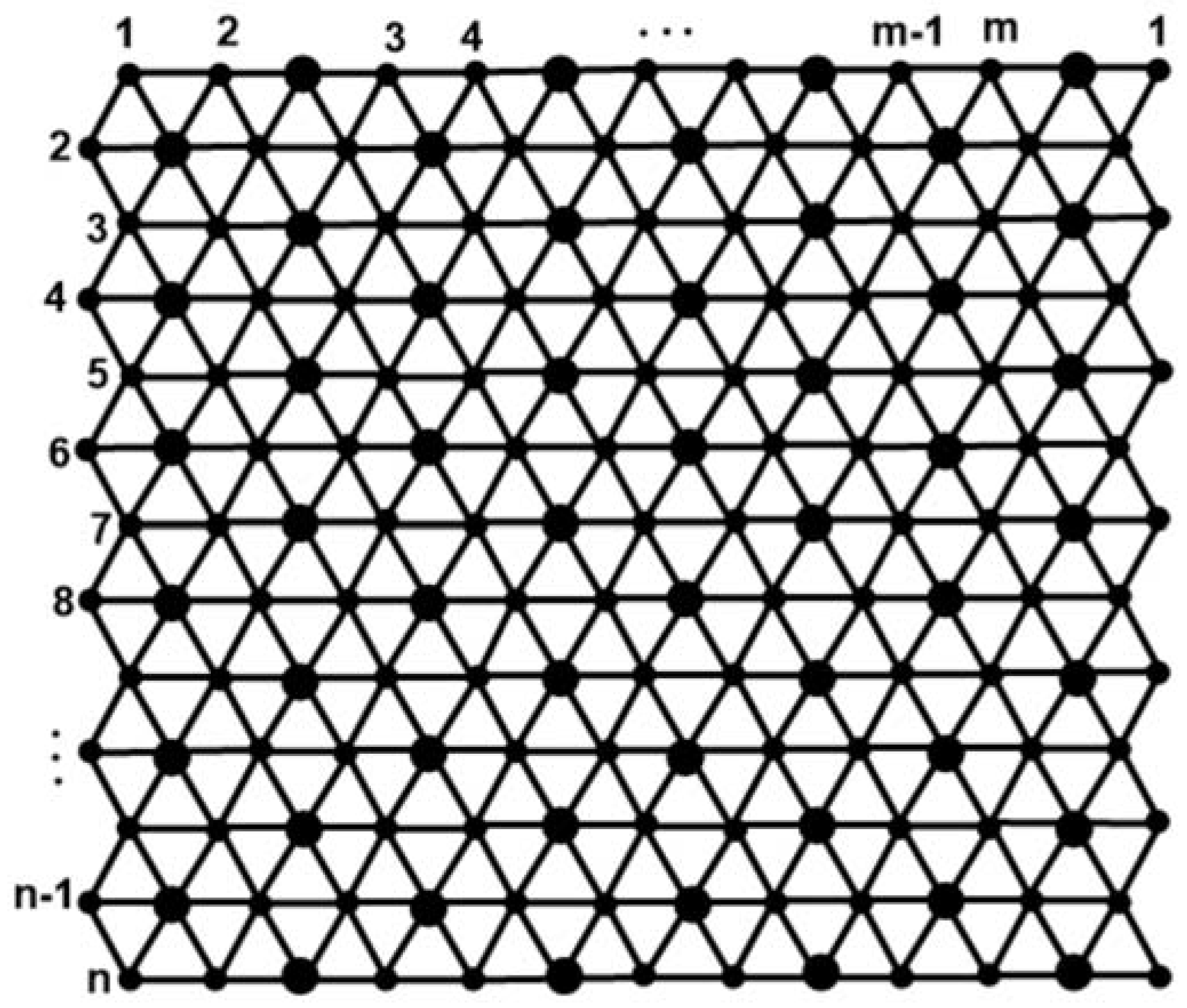
2. Results
2.1. M-Polynomials
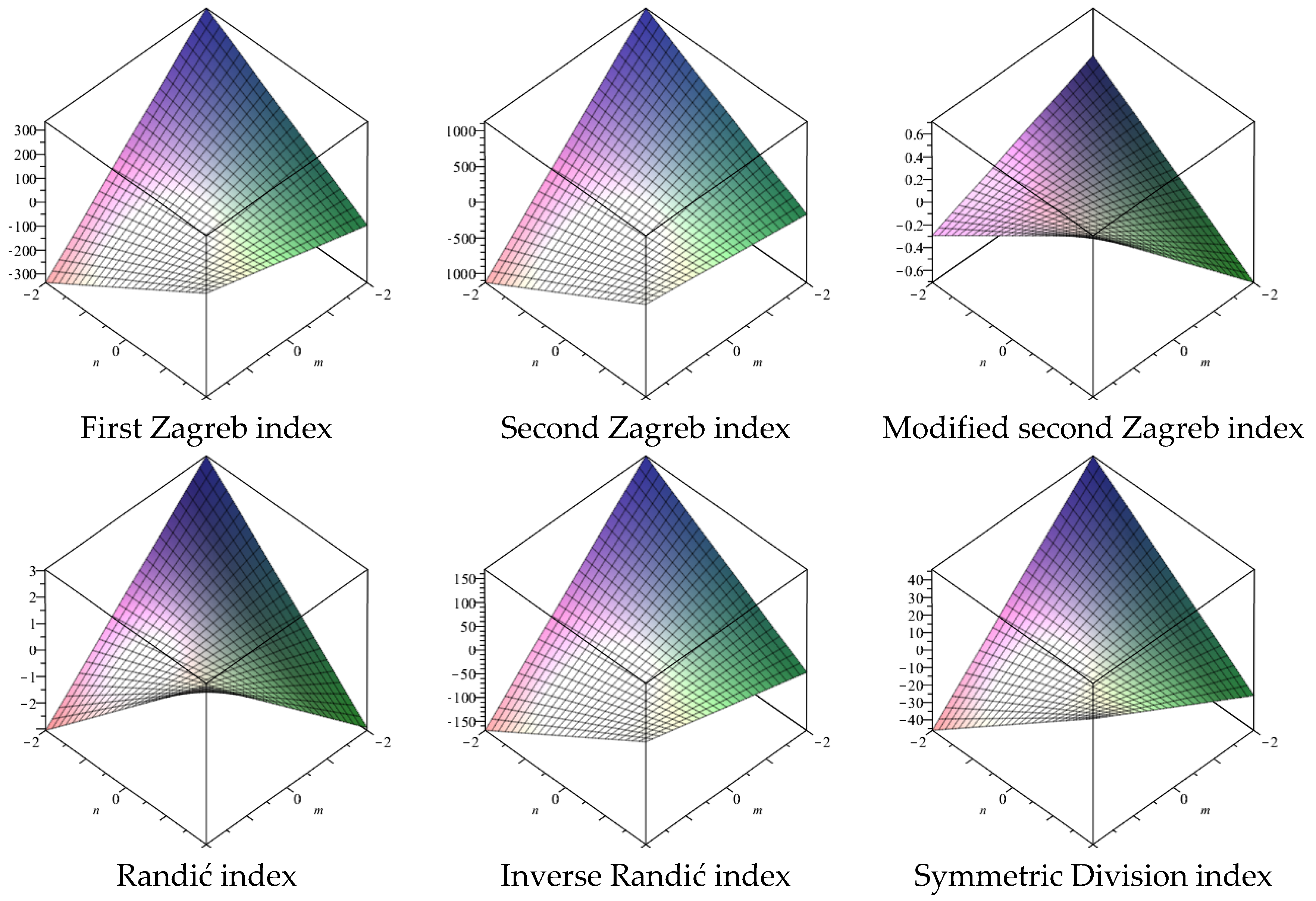
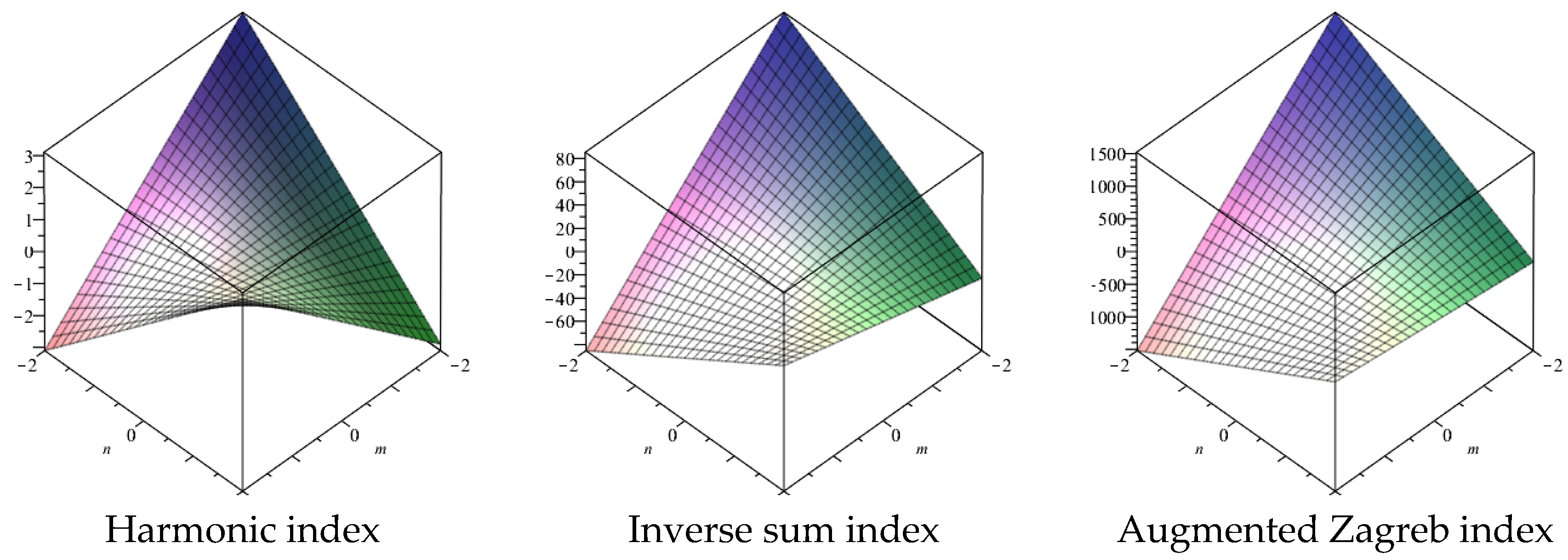
2.2. Topological Indices
3. Conclusions and Discussion
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Bezugly, V.; Kunstmann, J.; Grundkötter-Stock, B.; Frauenheim, T.; Niehaus, T.; Cuniberti, G. Highly conductive boron nanotubes: Transport properties, work functions, and structural stabilities. ACS Nano 2011, 5, 4997–5005. [Google Scholar] [CrossRef] [PubMed]
- West, D.B. An Introduction to Graph Theory; Prentice-Hall: Upper Saddle River, NJ, USA, 1996. [Google Scholar]
- Rucker, G.; Rucker, C. On topological indices, boiling points, and cycloalkanes. J. Chem. Inf. Comput. Sci. 1999, 39, 788–802. [Google Scholar] [CrossRef]
- Klavžar, S.; Gutman, I. A Comparison of the Schultz molecular topological index with the Wiener index. J. Chem. Inf. Comput. Sci. 1996, 36, 1001–1003. [Google Scholar] [CrossRef]
- Brückler, F.M.; Došlić, T.; Graovac, A.; Gutman, I. On a class of distance-based molecular structure descriptors. Chem. Phys. Lett. 2011, 503, 336–338. [Google Scholar] [CrossRef]
- Deng, H.; Yang, J.; Xia, F. A general modeling of some vertex-degree based topological indices in benzenoid systems and phenylenes. Comp. Math. Appl. 2011, 61, 3017–3023. [Google Scholar] [CrossRef]
- Zhang, H.; Zhang, F. The Clar covering polynomial of hexagonal systems. Discret. Appl. Math. 1996, 69, 147–167. [Google Scholar] [CrossRef]
- Gutman, I. Some properties of the Wiener polynomials. Graph Theory Notes N. Y. 1993, 125, 13–18. [Google Scholar]
- Deutsch, E.; Klavzar, S. M-Polynomial, and degree-based topological indices. Iran. J. Math. Chem. 2015, 6, 93–102. [Google Scholar]
- Munir, M.; Nazeer, W.; Rafique, S.; Kang, S.M. M-polynomial and related topological indices of Nanostar dendrimers. Symmetry 2016, 8, 97. [Google Scholar] [CrossRef]
- Munir, M.; Nazeer, W.; Rafique, S.; Nizami, A.R.; Kang, S.M. M-polynomial and degree-based topological indices of titania nanotubes. Symmetry 2016, 8, 117. [Google Scholar] [CrossRef]
- Munir, M.; Nazeer, W.; Rafique, S.; Kang, S.M. M-Polynomial and Degree-Based Topological Indices of Polyhex Nanotubes. Symmetry 2016, 8, 149. [Google Scholar] [CrossRef]
- Ajmal, M.; Nazeer, W.; Munir, M.; Kang, S.M.; Kwun, Y.C. M-polynomials and topological indices of generalized prism and toroidal polyhex networks. Symmetry. Under Review.
- Munir, M.; Nazeer, W.; Shahzadi, S.; Kang, S.M. Some invariants of circulant graphs. Symmetry 2016, 8, 134. [Google Scholar] [CrossRef]
- Wiener, H. Structural determination of paraffin boiling points. J. Am. Chem. Soc. 1947, 69, 17–20. [Google Scholar] [CrossRef] [PubMed]
- Dobrynin, A.A.; Entringer, R.; Gutman, I. Wiener index of trees: Theory and applications. Acta Appl. Math. 2001, 66, 211–249. [Google Scholar] [CrossRef]
- Gutman, I.; Polansky, O.E. Mathematical Concepts in Organic Chemistry; Springer: New York, NY, USA, 1986. [Google Scholar]
- Randic, M. On the characterization of molecular branching. J. Am. Chem. Soc. 1975, 97, 6609–6615. [Google Scholar] [CrossRef]
- Bollobas, B.; Erdos, P. Graphs of extremal weights. Ars Combin. 1998, 50, 225–233. [Google Scholar] [CrossRef]
- Amic, D.; Beslo, D.; Lucic, B.; Nikolic, S.; Trinajstić, N. The Vertex-Connectivity Index Revisited. J. Chem. Inf. Comput. Sci. 1998, 38, 819–822. [Google Scholar] [CrossRef]
- Hu, Y.; Li, X.; Shi, Y.; Xu, T.; Gutman, I. On molecular graphs with smallest and greatest zeroth-Corder general randic index. MATCH Commun. Math. Comput. Chem. 2005, 54, 425–434. [Google Scholar]
- Caporossi, G.; Gutman, I.; Hansen, P.; Pavlovic, L. Graphs with maximum connectivity index. Comput. Biol. Chem. 2003, 27, 85–90. [Google Scholar] [CrossRef]
- Li, X.; Gutman, I. Mathematical aspects of Randic-Type molecular structure descriptors. In Mathematical Chemistry Monographs; University of Kragujevac and Faculty of Science Kragujevac: Kragujevac, Serbia, 2006. [Google Scholar]
- Kier, L.B.; Hall, L.H. Molecular Connectivity in Chemistry and Drug Research; Academic Press: New York, NY, USA, 1976. [Google Scholar]
- Kier, L.B.; Hall, L.H. Molecular Connectivity in Structure-Activity Analysis; Wiley: New York, NY, USA, 1986. [Google Scholar]
- Randić, M. On History of the Randić Index and Emerging Hostility toward Chemical Graph Theory. MATCH Commun. Math. Comput. Chem. 2008, 59, 5–124. [Google Scholar]
- Randić, M. The Connectivity Index 25 Years After. J. Mol. Graphics Modell. 2001, 20, 19–35. [Google Scholar] [CrossRef]
- Gutman, I.; Furtula, B. Recent Results in the Theory of Randić Index; University of Kragujevac: Kragujevac, Serbia, 2008. [Google Scholar]
- Li, X.; Shi, Y. A survey on the Randic index. MATCH Commun. Math. Comput. Chem. 2008, 59, 127–156. [Google Scholar]
- Li, X.; Shi, Y.; Wang, L. An updated survey on the Randić index. Mathematical Chemistry Monographs 2008, 6, 9–47. [Google Scholar]
- Nikolić, S.; Kovačević, G.; Miličević, A.; Trinajstić, N. The Zagreb indices 30 years after. Croat. Chem. Acta 2003, 76, 113–124. [Google Scholar]
- Gutman, I.; Das, K.C. The first Zagreb indices 30 years after. MATCH Commun. Math. Comput. Chem. 2004, 50, 83–92. [Google Scholar]
- Das, K.; Gutman, I. Some properties of the second Zagreb Index. MATCH Commun. Math. Comput. Chem. 2004, 52, 103–112. [Google Scholar]
- Trinajstic, N.; Nikolic, S.; Milicevic, A.; Gutman, I. On Zagreb indices. Kem. Ind. 2010, 59, 577–589. [Google Scholar]
- Vukičević, D.; Graovac, A. Valence connectivities versus Randić, Zagreb and modified Zagreb index: A linear algorithm to check discriminative properties of indices in acyclic molecular graphs. Croat. Chem. Acta 2004, 77, 501–508. [Google Scholar]
- Milicevic, A.; Nikolic, S.; Trinajstic, N. On reformulated Zagreb indices. Mol. Divers. 2004, 8, 393–399. [Google Scholar] [CrossRef] [PubMed]
- Gupta, C.K.; Lokesha, V.; Shwetha, S.B.; Ranjini, P.S. On the symmetric division DEG index of graph. Southeast Asian Bull. Math. 2016, 40, 59–80. [Google Scholar]
- Fajtlowicz, S. On conjectures of Graffiti—II. Congr. Numer. 1987, 60, 187–197. [Google Scholar]
- Favaron, O.; Mahéo, M.; Saclé, J.F. Some eigenvalue properties in graphs (conjectures of Graffiti—II). Discrete Math. 1993, 111, 197–220. [Google Scholar] [CrossRef]
- Balaban, A.T. Highly discriminating distance based numerical descriptor. Chem. Phys. Lett. 1982, 89, 399–404. [Google Scholar] [CrossRef]
- Furtula, B.; Graovac, A.; Vukičević, D. Augmented Zagreb index. J. Math. Chem. 2010, 48, 370–380. [Google Scholar] [CrossRef]
- Das, K.C. Atom–bond connectivity index of graphs. Discr. Appl. Math. 2010, 158, 1181–1188. [Google Scholar] [CrossRef]
- Estrada, E.; Torres, L.; Rodríguez, L.; Gutman, I. An atom–bond connectivity index: Modeling the enthalpy of formation of alkanes. Indian J. Chem. 1998, 37A, 849–855. [Google Scholar]
- Estrada, E. Atom-bond connectivity and the energetic of branched alkanes. Chem. Phys. Lett. 2008, 463, 422–425. [Google Scholar] [CrossRef]

| Topological Index | Derivation from |
|---|---|
| First Zagreb index | |
| Second Zagreb index | |
| Modified Second Zagreb index | |
| Randić index | |
| Inverse Randić index | |
| Symmetric Division Index | |
| Harmonic Index | |
| Inverse sum Index | |
| Augmented Zagreb Index |
| Number of edges |
© 2017 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC-BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Munir, M.; Nazeer, W.; Rafique, S.; Nizami, A.R.; Kang, S.M. Some Computational Aspects of Boron Triangular Nanotubes. Symmetry 2017, 9, 6. https://doi.org/10.3390/sym9010006
Munir M, Nazeer W, Rafique S, Nizami AR, Kang SM. Some Computational Aspects of Boron Triangular Nanotubes. Symmetry. 2017; 9(1):6. https://doi.org/10.3390/sym9010006
Chicago/Turabian StyleMunir, Mobeen, Waqas Nazeer, Shazia Rafique, Abdul Rauf Nizami, and Shin Min Kang. 2017. "Some Computational Aspects of Boron Triangular Nanotubes" Symmetry 9, no. 1: 6. https://doi.org/10.3390/sym9010006
APA StyleMunir, M., Nazeer, W., Rafique, S., Nizami, A. R., & Kang, S. M. (2017). Some Computational Aspects of Boron Triangular Nanotubes. Symmetry, 9(1), 6. https://doi.org/10.3390/sym9010006





