N = (4,4) Supersymmetry and T-Duality
Abstract
:1. Introduction
2. Preface
3. Field Equations and Bianchi Identities
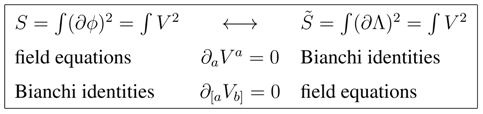
4. The N=(4,4) “Paradox”
5. T-duality between Chiral/Twisted Chiral and Semichiral Fields
5.1. Duality Transformation
5.2. Killing Vectors Preserving N=(4,4)
6. N=(4,4) Supersymmetry in the Two Dual Models
6.1. Supersymmetry in Original Model
6.2. Field Equations and Bianchi Identities
6.3. Supersymmetry Transformations in the Dual Model
6.4. Invariance of Action
6.5. Algebra Closure in the Dual Model
7. Reduction to (1,1) Superspace
8. Examples
8.1. Flat Space
8.2. Non-Quadratic Potential
9. Summary and Conclusions
Acknowledgements
Appendix
A. The Project in a Nutshell
| Original model: | Dual model: |
| where | where |
| Bianchi identities: | Bianchi identities: |
| Field equations: | Field equations: |
| Supersymmetry: | Supersymmetry: |
| constant matrices | not constant |
| susy algebra closes off-shell | susy algebra closes on-shell |
| (using Bianchi identities) | (using field equations) |
| Fourth coordinate: | Fourth coordinate: |
| Invariance of action: | Invariance of action: |
| ⇔ PDEs for | |
| Reduced to : | Reduced to : |
| . |
References and Notes
- Zumino, B. Supersymmetry and Kahler manifolds. Phys. Lett. B 1979, 87, 203–206. [Google Scholar] [CrossRef]
- Alvarez-Gaume, L.; Freedman, D.Z. Geometrical structure and ultraviolet finiteness in the supersymmetric sigma model. Commun. Math. Phys. 1981, 80, 443–451. [Google Scholar] [CrossRef]
- Lindstrom, U.; Rocek, M.; von Unge, R.; Zabzine, M. Generalized Kahler manifolds and linebreak off-shell supersymmetry. Commun. Math. Phys. 2007, 269, 833–849. [Google Scholar] [CrossRef]
- Lindstrom, U.; Rocek, M. Scalar tensor duality and N = 1, N = 2 nonlinear sigma models. Nucl. Phys. B 1983, 222, 285–308. [Google Scholar] [CrossRef]
- Grisaru, M.T.; Massar, M.; Sevrin, A.; Troost, J. Some aspects of N = (2,2), D = 2 supersymmetry. Fortsch. Phys. 1999, 47, 301–307. [Google Scholar] [CrossRef]
- Gates, S.J.; Hull, C.; Rocek, M. Twisted multiplets and new supersymmetric nonlinear sigma models. Nucl. Phys. B 1984, 248, 157–186. [Google Scholar] [CrossRef]
- Bogaerts, J.; Sevrin, A.; van der Loo, S.; van Gils, S. Properties of semichiral superfields. Nucl. Phys. 1999, 562, 277–290. [Google Scholar] [CrossRef]
- Goteman, M.; Lindstrom, U. Pseudo-hyperkahler geometry and generalized Kahler geometry. Lett. Math. Phys. 2011, 95, 211–222. [Google Scholar] [CrossRef]
- Goteman, M.; Lindstrom, U.; Rocek, M.; Ryb, I. Sigma models with off-shell N = (4,4) supersymmetry and noncommuting complex structures. JHEP 2010, 9, 1–21. [Google Scholar]
- Goteman, M.; Lindstrom, U.; Rocek, M. Semichiral sigma models with 4D Hyperkaehler geometry. Available online: http://arxiv.org/abs/1207.4753 (accessed on 19 October 2012).
- Alvarez, E.; Alvarez-Gaume, L.; Bakas, I. Supersymmetry and dualities. Nucl. Phys. Proc. Suppl. 1996, 46, 16–29. [Google Scholar] [CrossRef] [Green Version]
- Bakas, I.; Sfetsos, K. T-duality and world sheet supersymmetry. Phys. Lett. B 1995, 349, 448–457. [Google Scholar] [CrossRef] [Green Version]
- Crichigno, P.M. The semi-chiral quotient, Hyperkahler manifolds and T-duality. 2011. [Google Scholar] [CrossRef]
- Gualtieri, M. Generalized complex geometry. Ann. Math. 2011, 174, 75–123. [Google Scholar] [CrossRef]
- Ivanov, I.T.; Kim, B.B.; Rocek, M. Complex structures, duality and WZW models in extended superspace. Phys. Lett. B 1995, 343, 133–143. [Google Scholar] [CrossRef]
- Buscher, T.; Lindstrom, U.; Rocek, M. New supersymmetric sigma models with Wess-Zumino terms. Phys. Lett. B 1988, 202, 94–98. [Google Scholar] [CrossRef]
- Sevrin, A.; Troost, J. Off-shell formulation of N = 2 nonlinear sigma models. Nucl. Phys. B 1997, 492, 623–646. [Google Scholar] [CrossRef]
- Lindstrom, U.; Ivanov, I.; Rocek, M. New N = 4 superfields and sigma models. Phys. Lett. B 1994, 328, 49–54. [Google Scholar] [CrossRef]
- Dyckmanns, M. A twistor sphere of generalized Kahler potentials on hyperkahler manifolds. Available online: http://arxiv.org/abs/1111.3893 (accessed on 19 October 2012).
- Hjelmeland, S.E.; Lindstrom, U. Duality for the nonspecialist. Available online: http://arxiv.org/abs/hep-th/9705122 (accessed on 19 October 2012).
- At least for an ansatz without central charges.
- Lindstrom, U.; Rocek, M.; Ryb, I.; von Unge, R.; Zabzine, M. New N = (2,2) vector multiplets. JHEP 2007. [Google Scholar] [CrossRef]
- Goteman, M.; Lindstrom, U.; Rocek, M.; Ryb, I. Off-shell N=(4,4) supersymmetry for new (2,2) vector multiplets. JHEP 2011. [Google Scholar] [CrossRef]
- The author wishes to thank Marcos Crichigno and Martin Roček for developing this idea.
- In the literature, the term translational Killing vectors have been used for Killing vectors that are triholomorphic. Since both Killing vectors in Equations (50) and (51) satisfy holomorphicity (46), they are translational in this sense.
© 2012 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution license (http://creativecommons.org/licenses/by/3.0/).
Share and Cite
Göteman, M. N = (4,4) Supersymmetry and T-Duality. Symmetry 2012, 4, 603-625. https://doi.org/10.3390/sym4040603
Göteman M. N = (4,4) Supersymmetry and T-Duality. Symmetry. 2012; 4(4):603-625. https://doi.org/10.3390/sym4040603
Chicago/Turabian StyleGöteman, Malin. 2012. "N = (4,4) Supersymmetry and T-Duality" Symmetry 4, no. 4: 603-625. https://doi.org/10.3390/sym4040603
APA StyleGöteman, M. (2012). N = (4,4) Supersymmetry and T-Duality. Symmetry, 4(4), 603-625. https://doi.org/10.3390/sym4040603



