The Second-Order Approximation of Superpotentials Based on SUSYQM
Abstract
1. Introduction
2. Approximate Expansion of Superpotential
3. The Equation Satisfied by the Zeroth-Order Approximation of the Superpotential
4. The First-Order Approximation of the Superpotential
4.1. The Equation Satisfied by the First-Order Approximation of the Superpotential
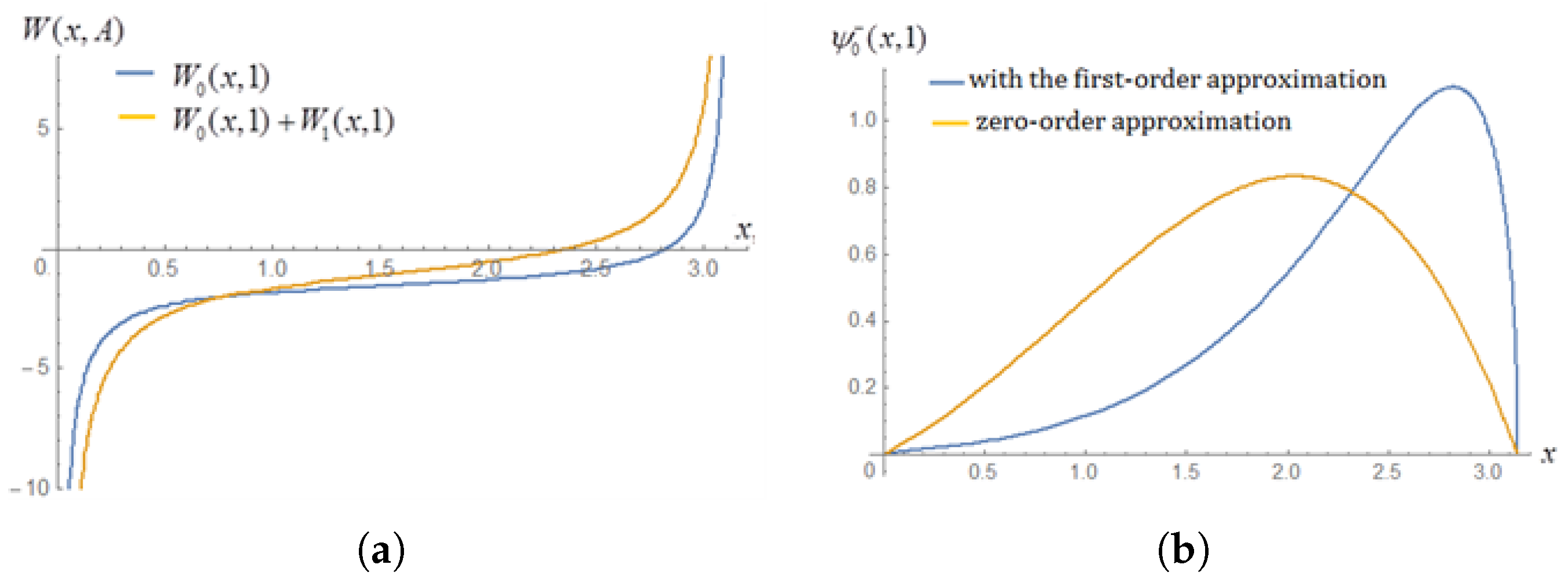
4.2. Example—The First-Order Approximation of the Rosen–Morse (Trigonometric) Superpotential
5. The Second-Order Approximation of the Superpotential
5.1. The Equation Satisfied by the Second-Order Approximation of the Superpotential
5.2. The Choice of the Specific Solution of
5.3. Example—the Second-Order Approximation of the Harmonic Oscillator Superpotential
6. Discussions of
6.1. of the First-Order Approximation
6.2. of the Second-Order Approximation
7. Conclusions and Prospects
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Schleich, W.P.; Greenberger, D.M.; Kobe, D.H.; Scully, M.O. Schrödinger equation revisited. Proc. Natl. Acad. Sci. USA 2013, 110, 5374–5379. [Google Scholar] [PubMed]
- Bhatta, V.S. Plurality of wave-particle duality. Curr. Sci. 2020, 118, 1365–1374. [Google Scholar]
- George, C.; Prigogine, I.; Rosenfeld, L. The macroscopic level of quantum mechanics. Nature 1972, 240, 25–27. [Google Scholar]
- Cooper, F.; Khare, A.; Sukhatme, U. Supersymmetry and quantum mechanics. Phys. Rep. 1995, 251, 267–385. [Google Scholar]
- Junker, G. Supersymmetric Methods in Quantum and Statistical Physics; Springer Science & Business Media: Berlin/Heidelberg, Germany, 1996. [Google Scholar]
- Bagchi, B.K. Supersymmetry in Quantum and Classical Mechanics; CRC Press: Boca Raton, FL, USA, 2000. [Google Scholar]
- Gangopadhyaya, A.; Mallow, J.; Rasinariu, C. Supersymmetric Quantum Mechanics: An Introduction; World Scientific Publishing: Singapore, 2017. [Google Scholar]
- Junker, G. Supersymmetric Methods in Quantum, Statistical and Solid State Physics: Enlarged and Revised Edition; IOP Publishing: London, UK, 2019. [Google Scholar]
- Bougie, J.; Gangopadhyaya, A.; Mallow, J.V. Generation of a complete set of additive shape-invariant potentials from an Euler equation. Phys. Rev. Lett. 2010, 105, 210402. [Google Scholar] [CrossRef] [PubMed]
- Zhong, S.K.; Xie, T.Y.; Dong, L.; Yang, C.X.; Xiong, L.L.; Li, M.; Luo, G. Shape invariance of solvable Schrödinger equations with a generalized hyperbolic tangent superpotential. Results Phys. 2022, 35, 105369. [Google Scholar] [CrossRef]
- Gendenshteîn, L.É. Derivation of exact spectra of the Schrodinger equation by means of supersymmetry. Jetp. Lett. 1983, 38, 356–359. [Google Scholar]
- Natanzon, G.A. General properties of potentials for which the Schrodinger equation can be solved by means of hypergeometric functions. Teor. Mat. Fiz. 1979, 38, 219–229. [Google Scholar]
- Schrödinger, E. Quantisierung als eigenwertproblem. Ann. Phys. 1926, 386, 109–139. [Google Scholar]
- Cooper, F.; Ginocchio, J.N.; Khare, A. Relationship between supersymmetry and solvable potentials. Phys. Rev. D 1987, 36, 2458. [Google Scholar]
- Dutt, R.; Khare, A.; Sukhatme, U.P. Supersymmetry, shape invariance, and exactly solvable potentials. Am. J. Phys. 1988, 56, 163–168. [Google Scholar]
- Dutt, R.; Gangopadhyaya, A.; Sukhatme, U.P. Noncentral potentials and spherical harmonics using supersymmetry and shape invariance. Am. J. Phys. 1997, 65, 400–403. [Google Scholar]
- Xiong, L.; Tan, X.; Zhong, S.; Cheng, W.; Luo, G. A New Solvable Generalized Trigonometric Tangent Potential Based on SUSYQM. Symmetry 2022, 14, 1593. [Google Scholar] [CrossRef]
- Li, M.; Zhong, S.K.; Dong, L.; Xiong, L.L.; Luo, G. Shape Invariance of Solvable Schrödinger Equations with the Generalized Hyperbolic Pöschl-Teller Potential. Adv. Math. Phys. 2022, 2022, 4345342. [Google Scholar] [CrossRef]
- Mallow, J.V.; Gangopadhyaya, A.; Bougie, J.; Rasinariu, C. Inter-relations between additive shape invariant superpotentials. Phys. Lett. 2020, 384, 126129. [Google Scholar]
- Bagchi, B.; Grandati, Y.; Quesne, C. Rational extensions of the trigonometric Darboux-Pöschl-Teller potential based on para-Jacobi polynomials. J. Math. Phys. 2015, 56, 062103. [Google Scholar] [CrossRef]
- Bougie, J.; Gangopadhyaya, A.; Rasinariu, C. The supersymmetric WKB formalism is not exact for all additive shape invariant potentials. J. Phys. A Math. Theor. 2018, 51, 375202. [Google Scholar]
- Quesne, C. Deformed shape invariant superpotentials in quantum mechanics and expansions in powers of ℏ. Symmetry 2020, 12, 1853. [Google Scholar] [CrossRef]
- Fernández, D.J.; Reyes, R. Spectral manipulation of the trigonometric Rosen-Morse potential through supersymmetry. Phys. Scr. 2021, 96, 125250. [Google Scholar] [CrossRef]
- Koohrokhi, T.; Izadpanah, A.; Gerayloo, M. A unified scheme of central symmetric shape-invariant potentials. Pramana 2024, 98, 140. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, Y.; Yin, Y.; Qiu, W.; Cheng, W.; Lu, H.; Luo, G. The Second-Order Approximation of Superpotentials Based on SUSYQM. Symmetry 2025, 17, 493. https://doi.org/10.3390/sym17040493
Liu Y, Yin Y, Qiu W, Cheng W, Lu H, Luo G. The Second-Order Approximation of Superpotentials Based on SUSYQM. Symmetry. 2025; 17(4):493. https://doi.org/10.3390/sym17040493
Chicago/Turabian StyleLiu, Yao, Yin Yin, Wenxin Qiu, Wei Cheng, Huan Lu, and Guang Luo. 2025. "The Second-Order Approximation of Superpotentials Based on SUSYQM" Symmetry 17, no. 4: 493. https://doi.org/10.3390/sym17040493
APA StyleLiu, Y., Yin, Y., Qiu, W., Cheng, W., Lu, H., & Luo, G. (2025). The Second-Order Approximation of Superpotentials Based on SUSYQM. Symmetry, 17(4), 493. https://doi.org/10.3390/sym17040493





