Influence of Bore Parameters and Effective Mass Ratio on the Launching Accuracy of Electromagnetic Launchers
Abstract
1. Introduction
2. Definition and Calculation Method of Launching Accuracy of Electromagnetic Launchers
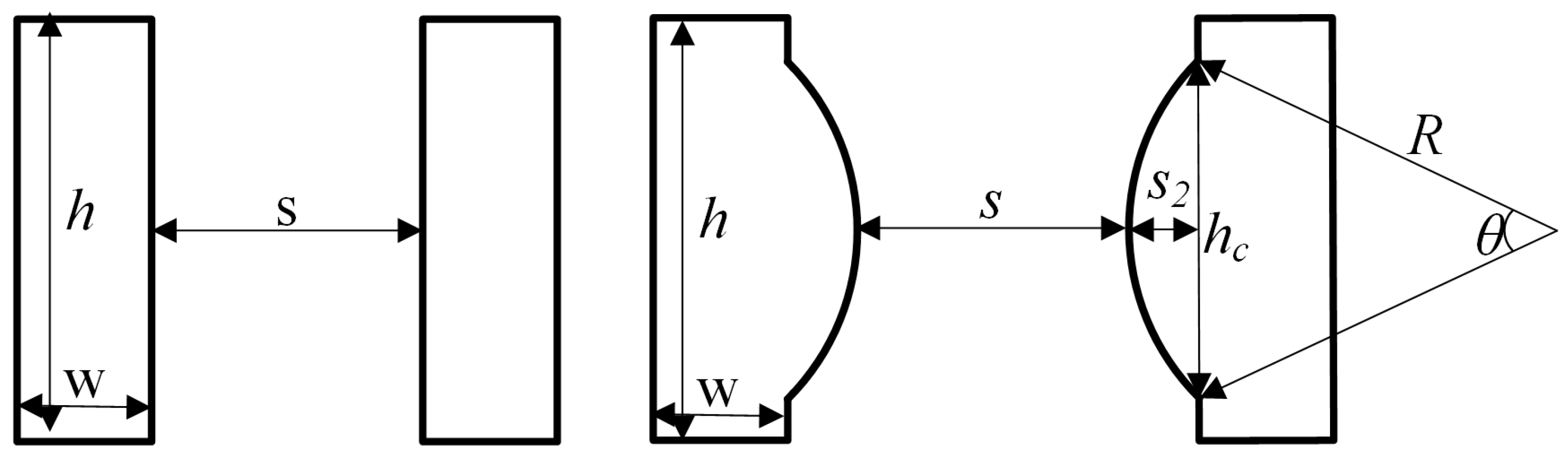
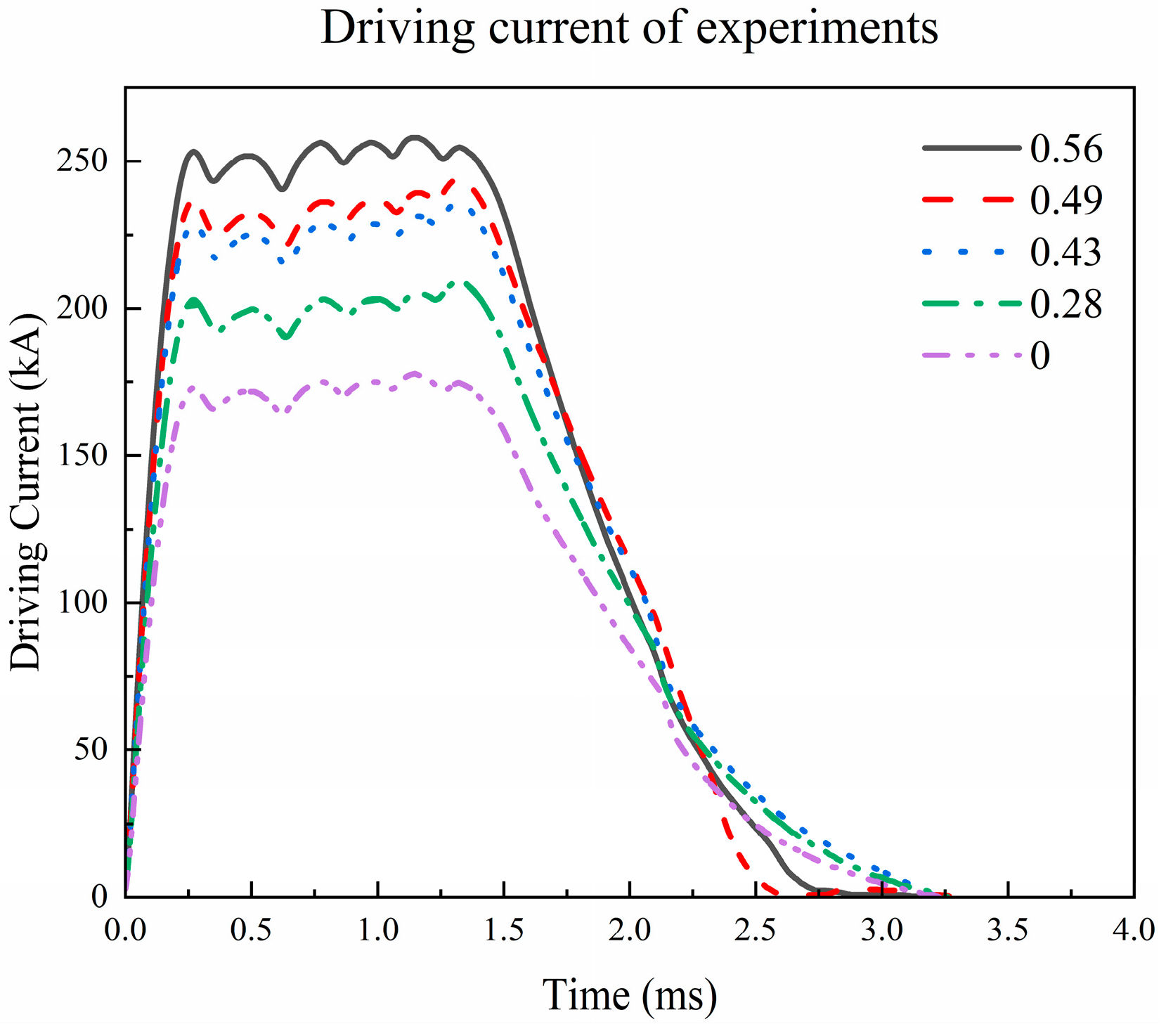
3. Experiment Setup
4. Results and Discussion of Experiments
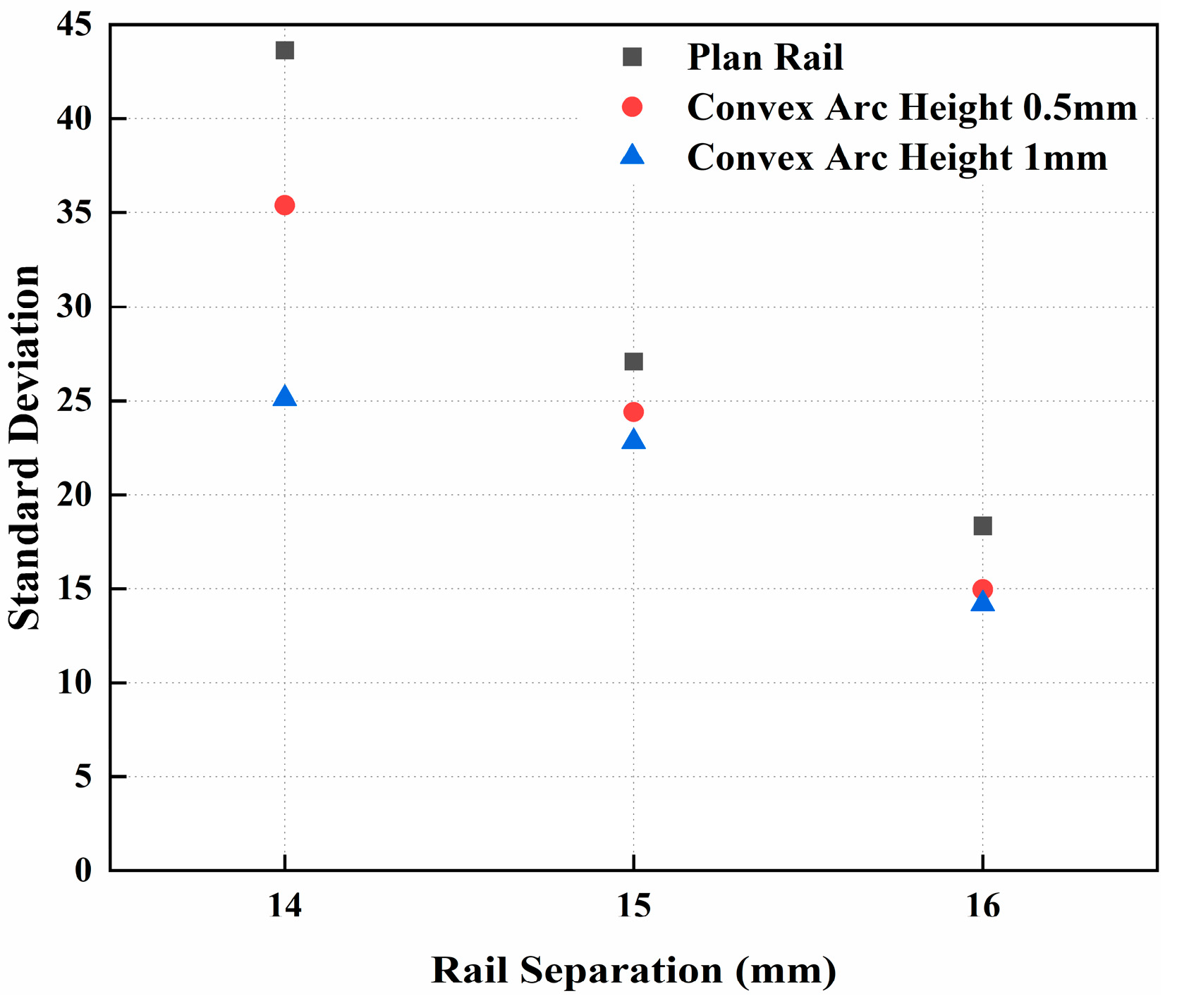
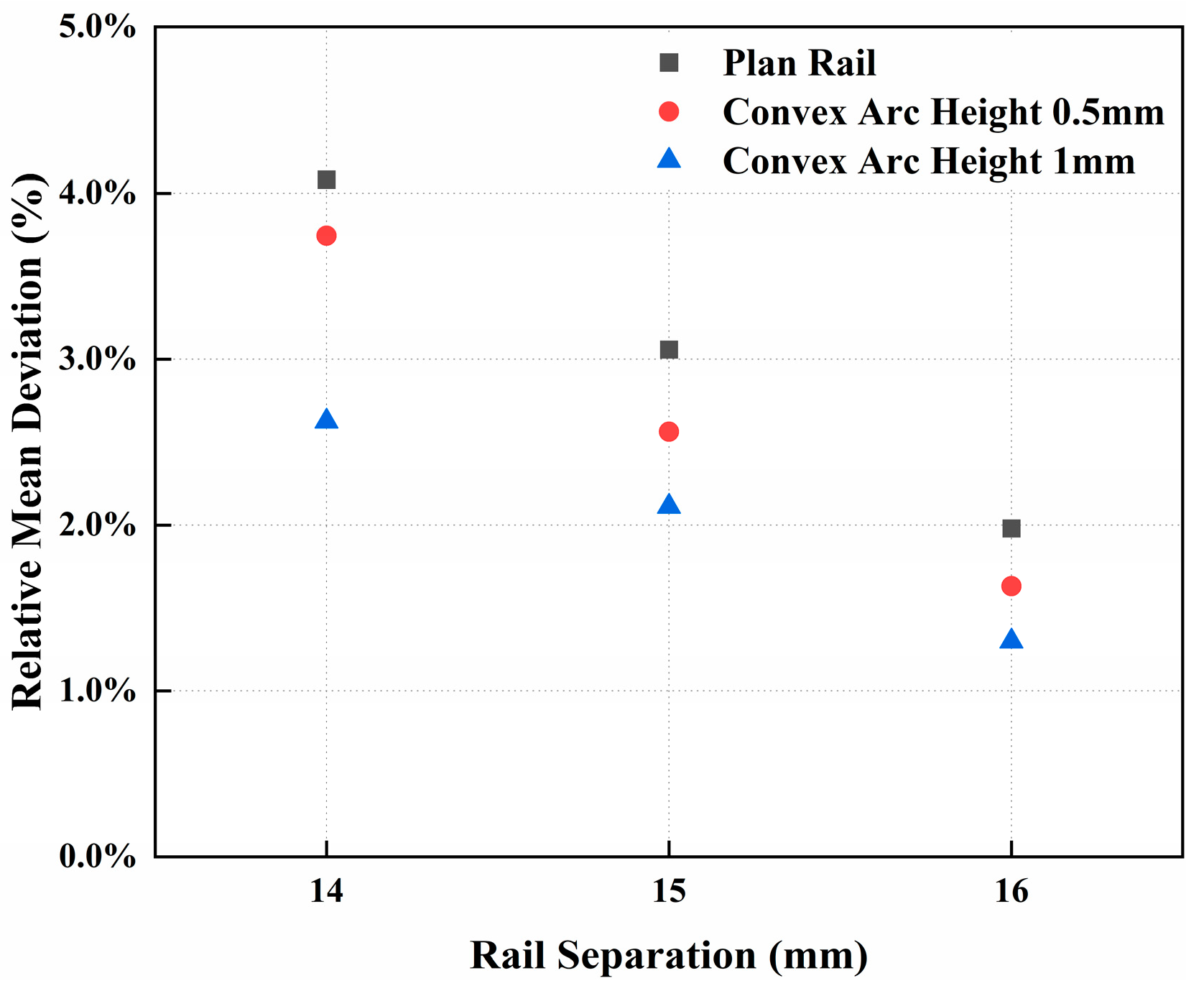
4.1. Influence of Bore Parameters on Consistency of Muzzle Velocity
4.2. Influence of Bore Parameters on Firing Accuracy and Firing Intensity
4.3. Influence of Bore Parameters and Effective Mass Ratio on Muzzle Attitude
4.3.1. Effect of Rail Separation on the Muzzle Attitude
4.3.2. Effect of Convex Arc Height on Muzzle Attitude
4.3.3. Effect of Effective Mass Ratio on the Muzzle Attitude
5. Conclusions and Future Work
- (1)
- Consistency of muzzle velocity:
- (2)
- Firing accuracy and firing intensity:
- (3)
- Muzzle attitude:
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Fair, H.D. Guest Editorial The Past, Present, and Future of Electromagnetic Launch Technology and the IEEE International EML Symposia. IEEE Trans. Plasma Sci. 2013, 41, 1024–1027. [Google Scholar] [CrossRef]
- Li, J.; Yan, P.; Yuan, W. Electromagnetic Gun Technology and Its Development. High Volt. Eng. 2014, 40, 1052–1064. [Google Scholar]
- Li, J.; Jin, L. A Novel Field Scaling Method in Electromagnetic Railguns. IEEE Trans. Plasma Sci. 2017, 45, 1673–1679. [Google Scholar] [CrossRef]
- Zhu, R.; Zhang, Q.; Li, Z.; Jin, L.; Wang, R. Impact physics model and influencing factors of gouging for electromagnetic rail launcher. In Proceedings of the 2014 17th International Symposium on Electromagnetic Launch Technology, San Diego, CA, USA, 7–11 July 2014; pp. 1–6. [Google Scholar]
- Ellis, R.L.; Poynor, J.C.; McGlasson, B.T.; Smith, A.N. Influence of bore and rail geometry on an electromagnetic naval railgun system. IEEE Trans. Magn. 2005, 41, 182–187. [Google Scholar] [CrossRef]
- Li, S.; Cao, R.; Zhou, Y.; Li, J. Performance Analysis of Electromagnetic Railgun Launch System Based on Multiple Experimental Data. IEEE Trans. Plasma Sci. 2019, 47, 524–534. [Google Scholar] [CrossRef]
- Zhang, Q.; Li, J.; Li, S.; Liu, P.; Cao, R.; Cheng, L.; Yuan, W. Simulation and test study on different structure bore of electromagnetic launch. In Proceedings of the 2014 17th International Symposium on Electromagnetic Launch Technology, San Diego, CA, USA, 7–11 July 2014; pp. 1–5. [Google Scholar]
- Hsieh, K.T.; Kim, B.K. International railgun modelling effort. IEEE Trans. Magn. 1997, 33, 245–248. [Google Scholar] [CrossRef]
- Meger, R.A.; Cooper, K.; Jones, H.; Neri, J.; Qadri, S.; Singer, I.L.; Sprague, J.; Wahl, K.J. Analysis of rail surfaces from a multishot railgun. IEEE Trans. Magn. 2005, 41, 211–213. [Google Scholar] [CrossRef]
- Marshall, R.A. Railgun bore geometry, round or square? IEEE Trans. Magn. 1999, 35, 427–431. [Google Scholar] [CrossRef]
- Wang, Z.S.; Liu, Z.Q.; Yang, B.Y.; Liu, Q.W. Research on firing accuracy influenced by each factor. J. Proj. Rocket. Missiles Guid. 2005, 6, 198–200. [Google Scholar]
- Dong, Z.; Guo, B.Q.; Wu, X.; Zhang, J.L. Whe analysis of artillery launch precision optimization. Comput. Simul. 2015, 32, 26–30. [Google Scholar]
- Wang, T.X.; Bo, Y.M.; Zhao, G.P. Simulation research on hit presicion of firing high-speed-on-the-move under influence of barrel-speed. Fire Control Command Control 2015, 40, 8–11. [Google Scholar]
- Allred, L.G. A proposed standard for accuracy computations. In Proceeding of the IEEE Aerospace and Electronics Conference, Dayton, OH, USA, 21–25 May 1990; pp. 351–358. [Google Scholar]
- Peterson, K.A. Numerical Simulation Investigations in Weapon Delivery Probabilities. Ph.D. Thesis, Naval Postgraduate Schoool, Monterey, CA, USA, 2008. [Google Scholar]
- Massoumnia, M.A. Optimal test procedures for evaluating circular probable error. J. Guid. Control Dyn. 1991, 14, 1326–1328. [Google Scholar] [CrossRef]
- Gallant, J.; Vanderbeke, E.; Alouahabi, F.; Schneider, M. Design considerations for an electromagnetic railgun to be used against antiship missiles. IEEE Trans. Plasma Sci. 2013, 41, 2800–2804. [Google Scholar] [CrossRef]
- Shang, X.B. Research on Firing Accuracy Analysis and Evaluation Techniques of Electromagnetic Railgun Exterior Ballistic. Ph.D. Thesis, Harbin Institute of Technology, Harbin, China, 2015. [Google Scholar]
- Ma, P.; Zhou, Y.; Shang, X.; Yang, M. Firing Accuracy Evaluation of Electromagnetic Railgun Based on Multicriteria Optimal Latin Hypercube Design. IEEE Trans. Plasma Sci. 2017, 45, 1503–1511. [Google Scholar] [CrossRef]
- Chen, L.Y.; Yang, F.; Wang, D. Error analysis and index assignment of electromagnetic railgun muzzle velocity. Technol. Innov. Appl. 2018, 1, 19–22. [Google Scholar]
- Ma, P.; Shang, X.; Chao, T.; Yang, M. Simulation-Based Firing Accuracy Analysis for Electromagnetic Railgun with Uncertainty. IEEE Trans. Plasma Sci. 2019, 47, 3358–3365. [Google Scholar] [CrossRef]
- Tang, X.Y.; Lian, H.G.; Yu, J.S.; Du, X. Analysis of calculating method of electromagnetic launch strike accuracy. Technol. Innov. Appl. 2021, 21, 20–22. [Google Scholar]
- Zhang, Q.; Li, H.; Li, J.; Liu, K.; Jin, L.; Xu, W.; Wang, Z. Optimization Analysis of the Bore Cross-Section in Launchers Based on Shooting Accuracy. In Proceedings of the 2024 10th Euro-Asian Pulsed Power Conference, 25th International Conference on High-Power Particle Beams and 20th International Symposium on Electromagnetic Launch Technology (EAPPC/BEAMS/EML), Amsterdam, The Netherlands, 22–26 September 2024; pp. 1–2. [Google Scholar]
- Cao, N. Evaluation Method on Firing Accuracy of Wheeled Artillery. Ph.D. Thesis, Nanjing University of Science & Technology, Nanjing, China, 2012. [Google Scholar]





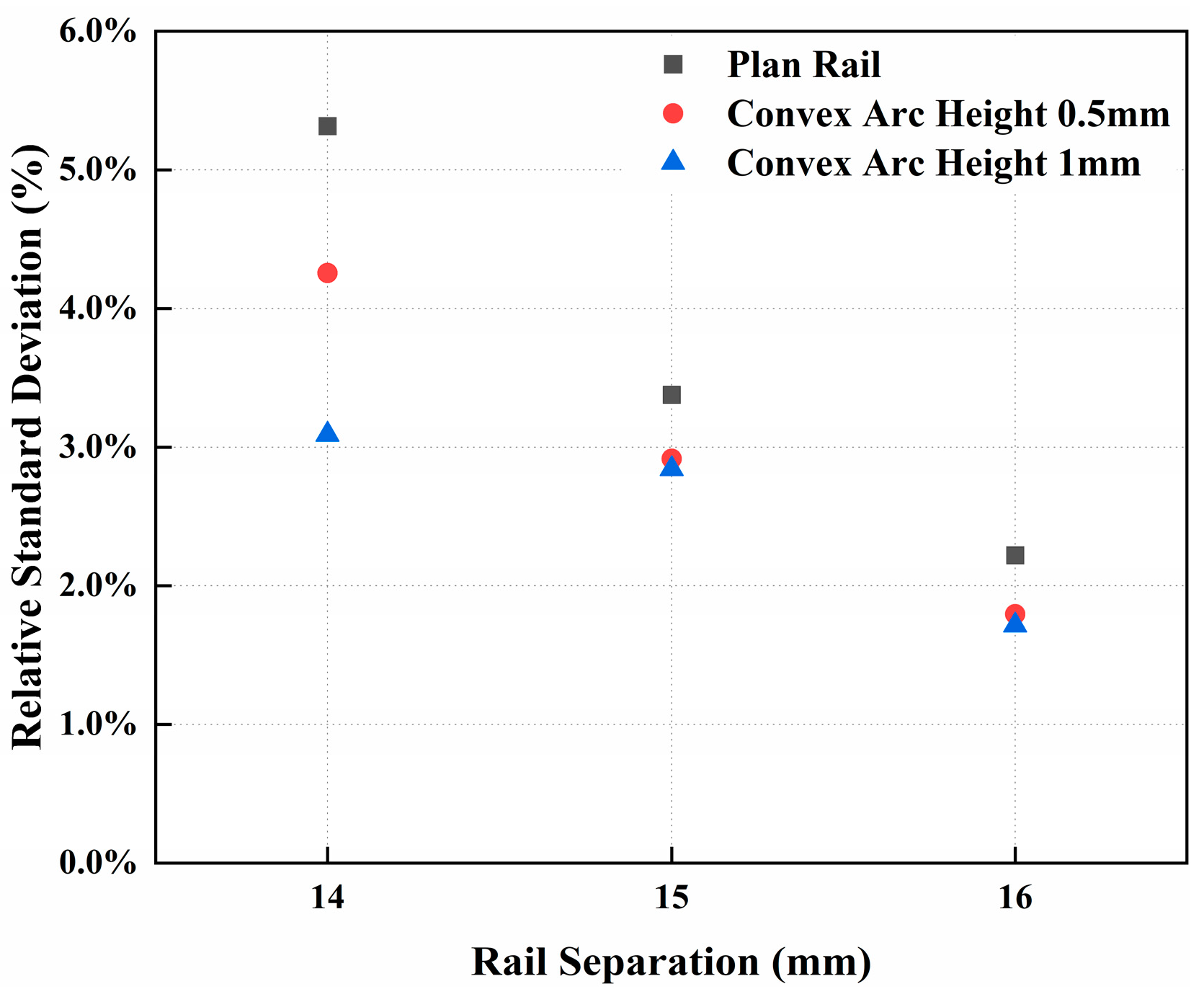


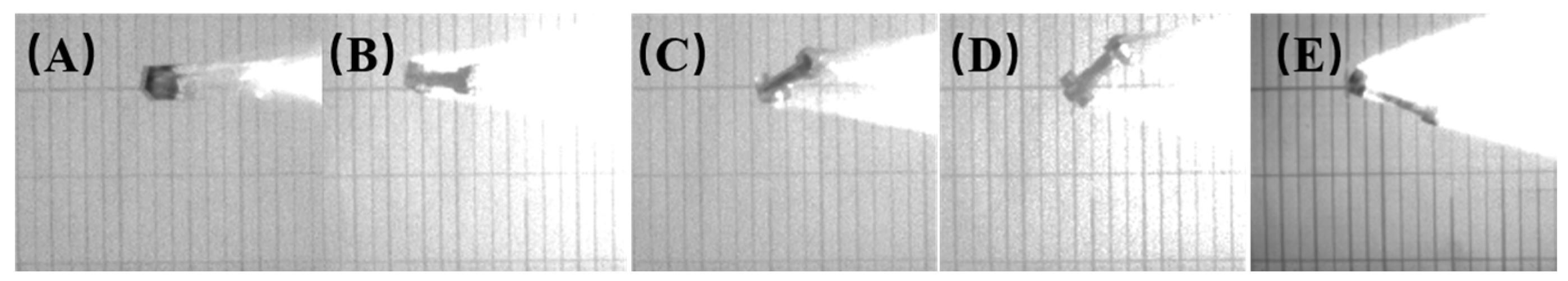



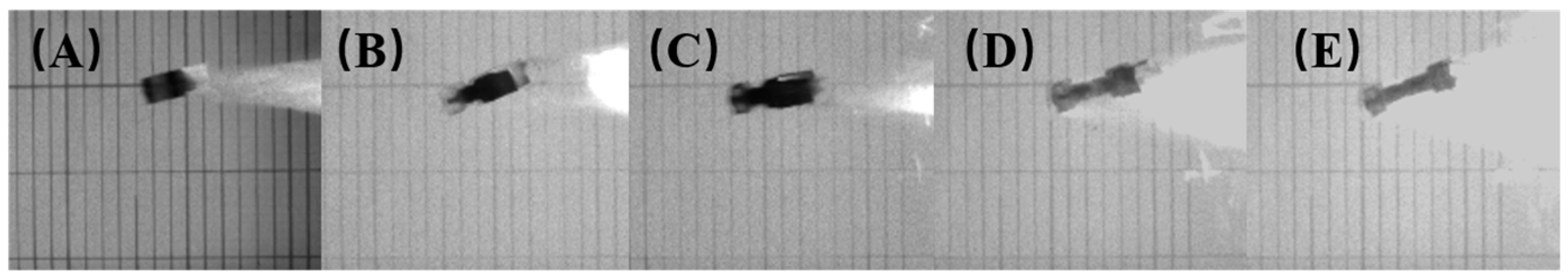



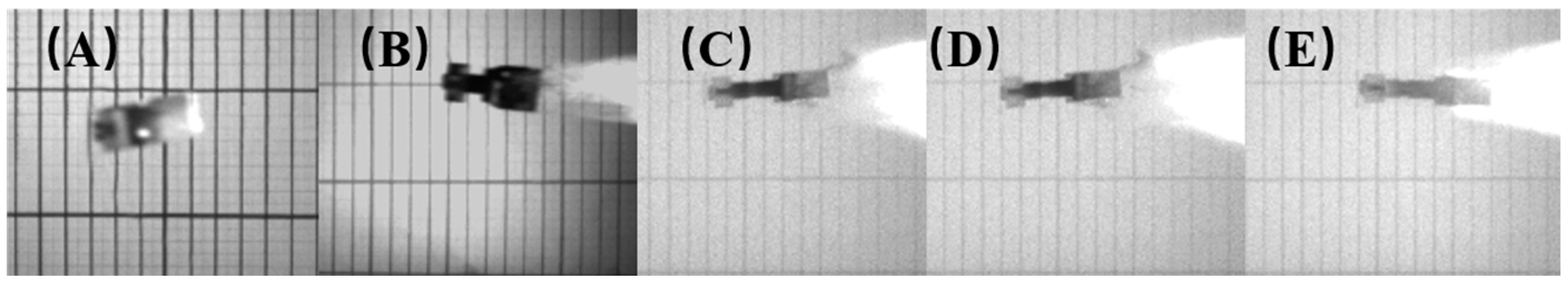
| Experiment Number | Rail Separation (mm) | Convex Arc Height (mm) | σ1 | σ2 |
|---|---|---|---|---|
| 1 | 14 | 0 | 9.60 | 10.34 |
| 2 | 14 | 0 | 14.94 | 9.03 |
| 3 | 14 | 0 | 13.23 | 10.01 |
| 4 | 15 | 0.5 | 5.98 | 6.60 |
| 5 | 15 | 0.5 | 5.84 | 7.97 |
| 6 | 15 | 0.5 | 9.36 | 7.88 |
| 7 | 16 | 1 | 4.41 | 5.79 |
| 8 | 16 | 1 | 5.41 | 6.95 |
| 9 | 16 | 1 | 5.27 | 6.88 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xiao, N.; Yan, P. Influence of Bore Parameters and Effective Mass Ratio on the Launching Accuracy of Electromagnetic Launchers. Symmetry 2025, 17, 404. https://doi.org/10.3390/sym17030404
Xiao N, Yan P. Influence of Bore Parameters and Effective Mass Ratio on the Launching Accuracy of Electromagnetic Launchers. Symmetry. 2025; 17(3):404. https://doi.org/10.3390/sym17030404
Chicago/Turabian StyleXiao, Nan, and Ping Yan. 2025. "Influence of Bore Parameters and Effective Mass Ratio on the Launching Accuracy of Electromagnetic Launchers" Symmetry 17, no. 3: 404. https://doi.org/10.3390/sym17030404
APA StyleXiao, N., & Yan, P. (2025). Influence of Bore Parameters and Effective Mass Ratio on the Launching Accuracy of Electromagnetic Launchers. Symmetry, 17(3), 404. https://doi.org/10.3390/sym17030404






