Perrin Numbers That Are Concatenations of a Perrin Number and a Padovan Number in Base b
Abstract
1. Introduction
2. Preliminaries
3. Results
- (i)
- and
- (ii)
- (iii)
- (i)
- As d is the number of digits of in base b, we have . Then, we findandThis completes the proof.
- (ii)
- Asandwe obtain
- (iii)
- As we get , we get
- (i)
- and
- (ii)
- (iii)
4. Proof of Theorem 1
5. Proof of Theorem 2
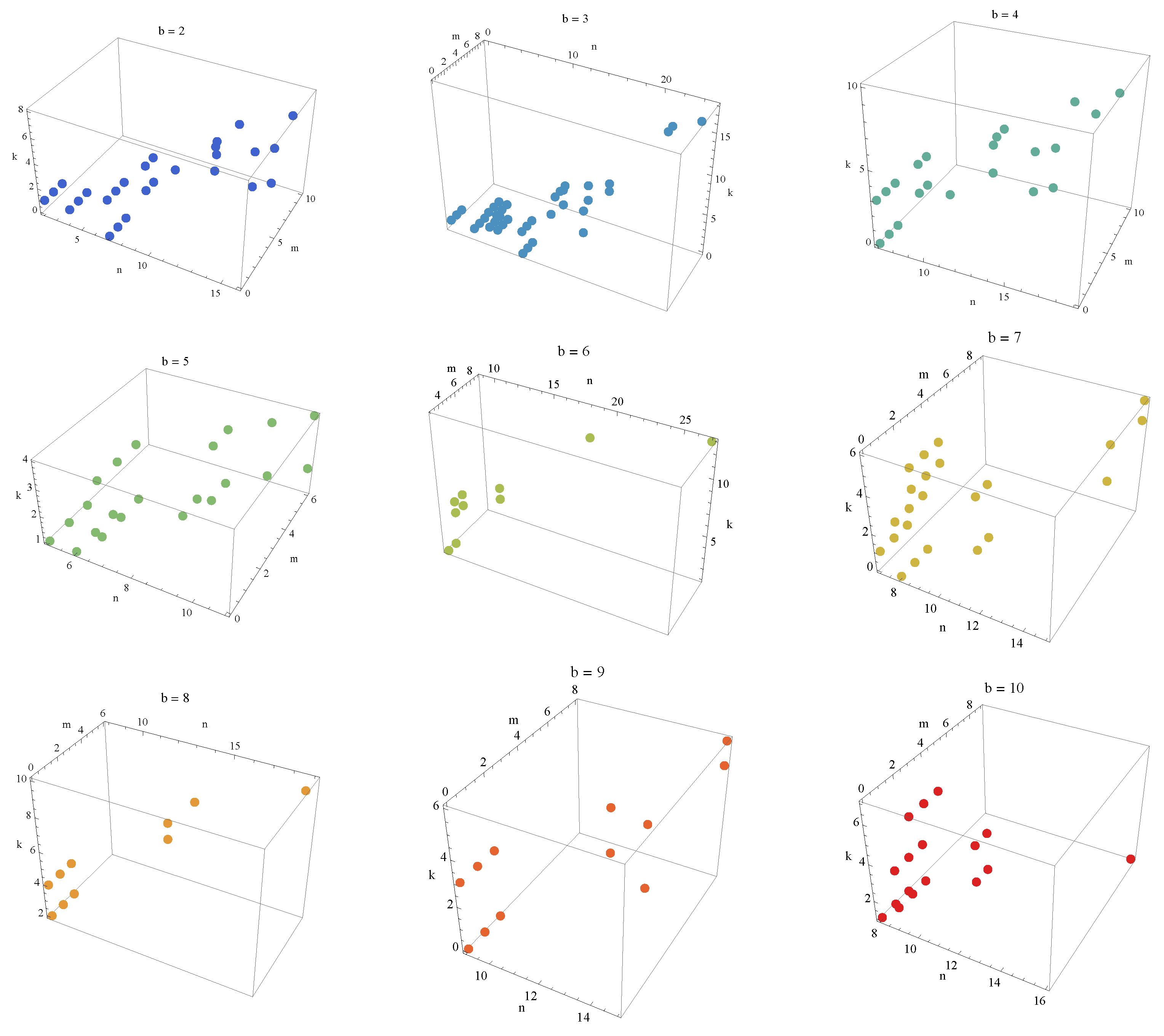
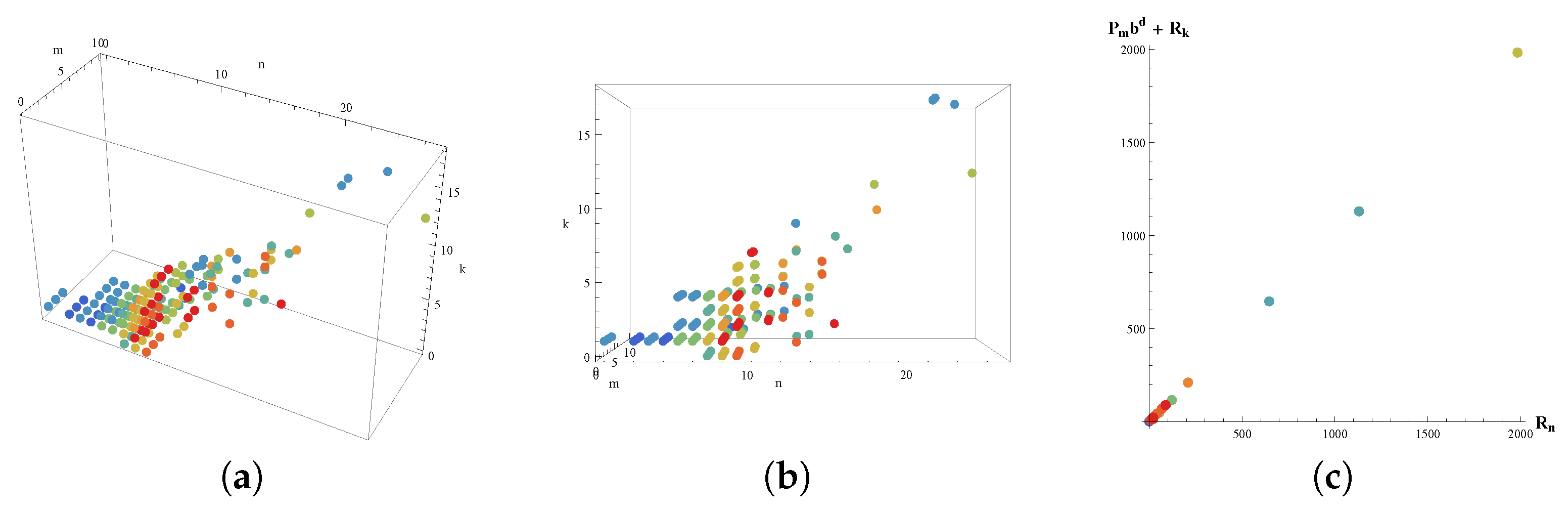
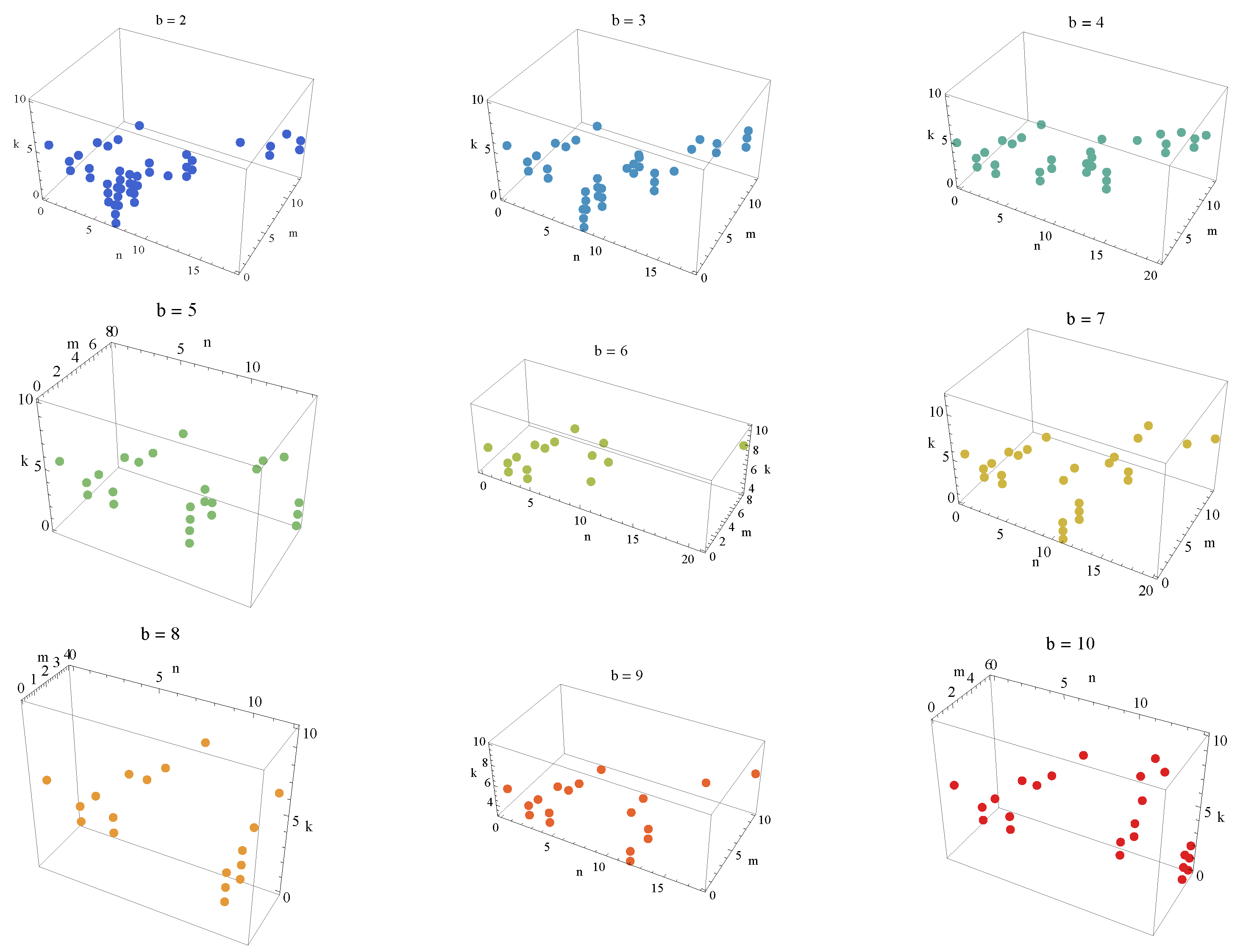
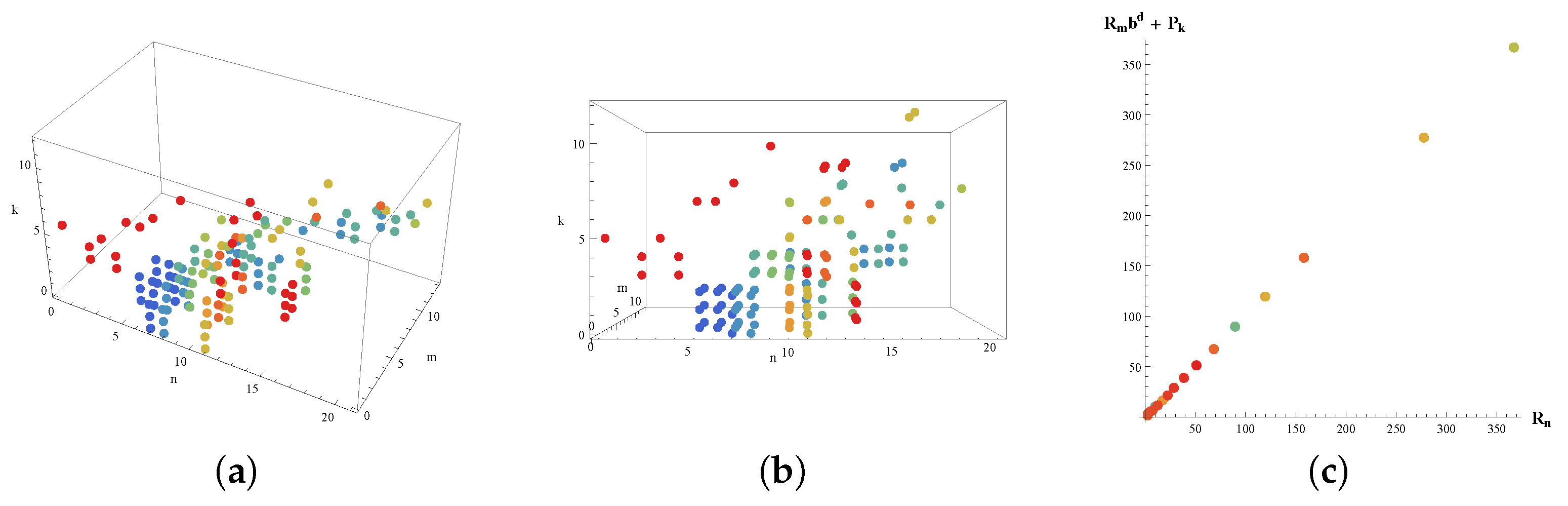
6. Conclusions
Funding
Data Availability Statement
Conflicts of Interest
References
- Sloane, N.J.A. The On-Line Encyclopedia of Integer Sequences (OEIS). 2024. Available online: https://oeis.org (accessed on 1 January 2025).
- Banks, W.D.; Luca, F. Concatenations with binary reccurent sequences. J. Integer Seq. 2005, 8, 3. [Google Scholar]
- Alan, M. On Concatenations of Fibonacci and Lucas Numbers. Bull. Iran. Math. Soc. 2022, 48, 2725–2741. [Google Scholar] [CrossRef]
- Bravo, E.F. On concatenations of Padovan and Perrin numbers. Math. Commun. 2023, 28, 105–119. [Google Scholar]
- Altassan, A.; Alan, M. On Mixed Concatenations of Fibonacci and Lucas Numbers Which are Fibonacci Numbers. arXiv 2022, arXiv:2206.13625. [Google Scholar]
- Erduvan, F. On Concatenations of Two Padovan and Perrin Numbers. Bull. Iran. Math. Soc. 2023, 49, 62. [Google Scholar] [CrossRef]
- Chalebgwa, T.P.; Ddamulira, M. Padovan numbers which are palindromic concatenations of two distinct repdigits. Rev. Real Acad. Cienc. Exactas Fis. Nat. Ser. A Mat. 2021, 115, 108. [Google Scholar] [CrossRef] [PubMed]
- Ddamulira, M. Padovan numbers that are concatenations of two distinct repdigits. Math. Slovaca 2021, 71, 275–284. [Google Scholar] [CrossRef]
- Dujella, A.; Pethò, A. A generalization of a theorem of Baker and Davenport. Q. J. Math. Oxf. 1998, 49, 291–306. [Google Scholar] [CrossRef]
- Baker, A.; Davenport, H. The equations 3x2-2=y2 and 8x2-7=z2. Q. J. Math. Oxford Ser. 1969, 20, 129–137. [Google Scholar] [CrossRef]
- Batte, H.; Chalebgwa, T.P.; Ddamulira, M. Perrin numbers that are concatenations of two distinct repdigits. arXiv 2021, arXiv:2105.08515. [Google Scholar]
- Adédji, K.N.; Dossou-yovo, V.; Rihane, S.E.; Togbé, A. Padovan or Perrin numbers that are concatenations of two distinct base b repdigits. Math. Slovaca 2023, 73, 49–64. [Google Scholar] [CrossRef]
- Bugeaud, Y. Linear Forms in Logarithms and Applications; IRMA Lectures in Mathematics and Theoretical Physics; European Mathematical Society: Zurich, Switzerland, 2008; Volume 28. [Google Scholar]
- Matveev, E.-M. An Explicit lower bound for a homogeneous rational linear form in the logarithms of algebraic numbers II. Izv. Nauk. Ser. Math. 2000, 64, 125–180. (In Russian) [Google Scholar] [CrossRef]
- Bugeaud, Y.; Mignotte, M.; Siksek, S. Classical and modular approaches to exponential Diophantine equations I. Fibonacci and Lucas perfect powers. Ann. Math. 2006, 163, 969–1018. [Google Scholar] [CrossRef]
- Bravo, J.J.; Gomez, C.A.; Luca, F. Powers of two as sums of two k-Fibonacci numbers. Miskolc Math. Notes 2016, 17, 85–100. [Google Scholar] [CrossRef]
- De Weger, B.M.M. Algorithms for Diophantine Equations; CWI Tracts 65; Stichting Mathematisch Centrum: Amsterdam, The Netherlands, 1989. [Google Scholar]
- Legendre, A.M. Essai sur la Th’Eorie des Nombres; Duprat: Paris, France, 1798. [Google Scholar]
- Güney Duman, M. On perfect powers that are difference of two Perrin numbers or two Padovan numbers. Proc. Indian Natl. Sci. Acad. 2024, 90, 124–131. [Google Scholar] [CrossRef]
- Güney Duman, M. The equations Rn = d·Pm and Pn = d·Pm in Padovan and Perrin numbers. In Proceedings of the 11th International Vocational Schools Symposium, Düzce, Türkiye, 5–7 October 2023. [Google Scholar]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Güney Duman, M. Perrin Numbers That Are Concatenations of a Perrin Number and a Padovan Number in Base b. Symmetry 2025, 17, 364. https://doi.org/10.3390/sym17030364
Güney Duman M. Perrin Numbers That Are Concatenations of a Perrin Number and a Padovan Number in Base b. Symmetry. 2025; 17(3):364. https://doi.org/10.3390/sym17030364
Chicago/Turabian StyleGüney Duman, Merve. 2025. "Perrin Numbers That Are Concatenations of a Perrin Number and a Padovan Number in Base b" Symmetry 17, no. 3: 364. https://doi.org/10.3390/sym17030364
APA StyleGüney Duman, M. (2025). Perrin Numbers That Are Concatenations of a Perrin Number and a Padovan Number in Base b. Symmetry, 17(3), 364. https://doi.org/10.3390/sym17030364





